A Self-Operating Time Crystal Model of the Human Brain: Can We Replace Entire Brain Hardware with a 3D Fractal Architecture of Clocks Alone?
Abstract
1. Introduction
2. The Inventions of a Universal Time Crystal (UTC), Time Polycrystal and Space-Time-Topology-Symmetry (STts) Metric: Background Works on Brain Model
2.1. Resonance and Time Crystal
2.1.1. Noise Driven Clocks: Harvesting the Geometry of Cavity and Dielectric Resonators
2.1.2. A Paradigm Shift in the Half-A-Century Old Concept of a Time Crystal
2.1.3. Representing Resonance-Based UTC, Time Polycrystals Using Circles
2.1.4. Twelve Classes of Rhythms Proposed or Reported in the Brain That Could Produce Vortices or Clocks to Build Time Crystal
2.2. Background Human Brain Model Studies as a Precursor to the Proposed Time Crystal Brain Model
2.2.1. Triplet of Triplet Resonance Chain Model of the Human Brain
2.2.2. Rebuilding Real Brain Components in the Simulator and Solving Maxwell’s Equations to Find Resonance Frequencies and Clock-Like Oscillation of Fields
2.2.3. Identification of Primes in the Ratio of Resonance Frequencies: Experiment and Theory
2.3. A Space-Time-Topology-Prime Metric for Analyzing the Human Thought and Conscious Experiences
2.3.1. Quaternion, Octonion and Dodecanion Tensors: Stereographic Projection to Infinity and Feedback from Infinity (PF Network)
2.3.2. Construction and Decomposition of Space-Time-Topology-Prime or Space-Time-Topology-Symmetry (sTtS) Metric
2.4. Tensor Analysis of the Human Brain and the Thought Process
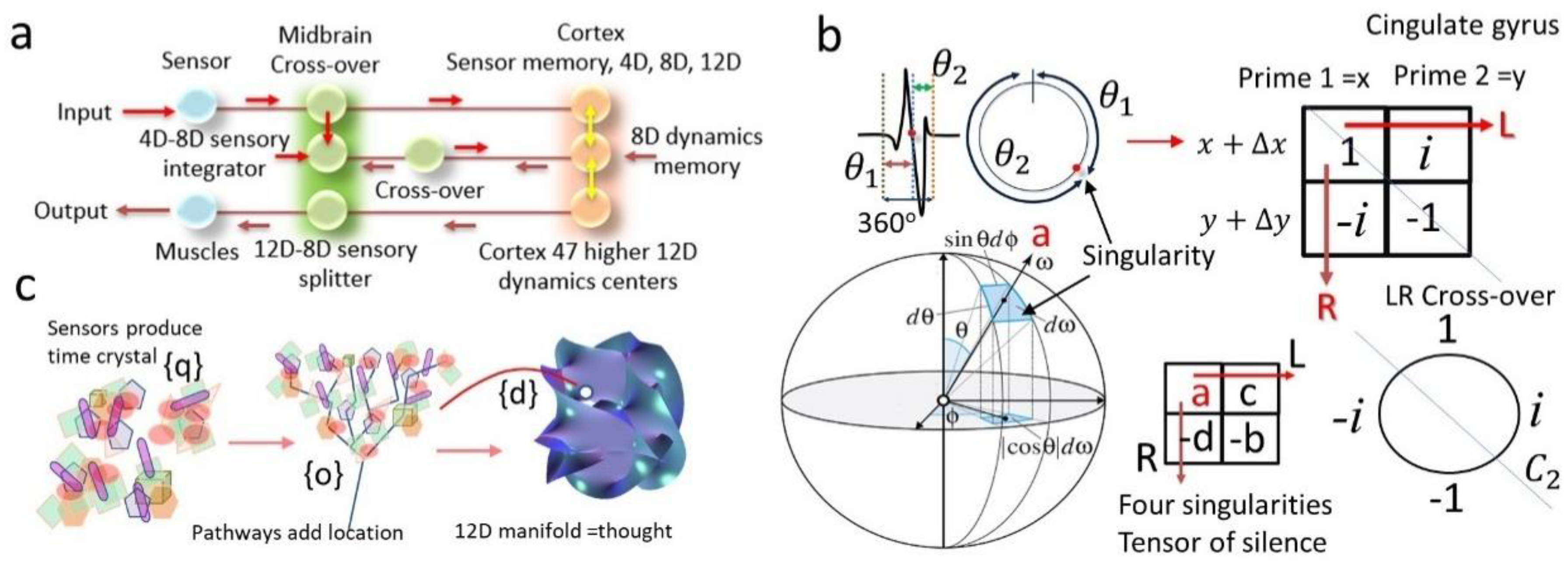
2.4.1. How to Bind Different Clocks Using the Singularity Domains
2.4.2. Tensors of Different Dimensions in Different Parts of the Brain: Data Format
2.4.3. How Tensor Elements are Filled during Interaction of Vortices or Particle-like Field Structure
2.4.4. A Nerve Spike and Time Crystal in a Neuron
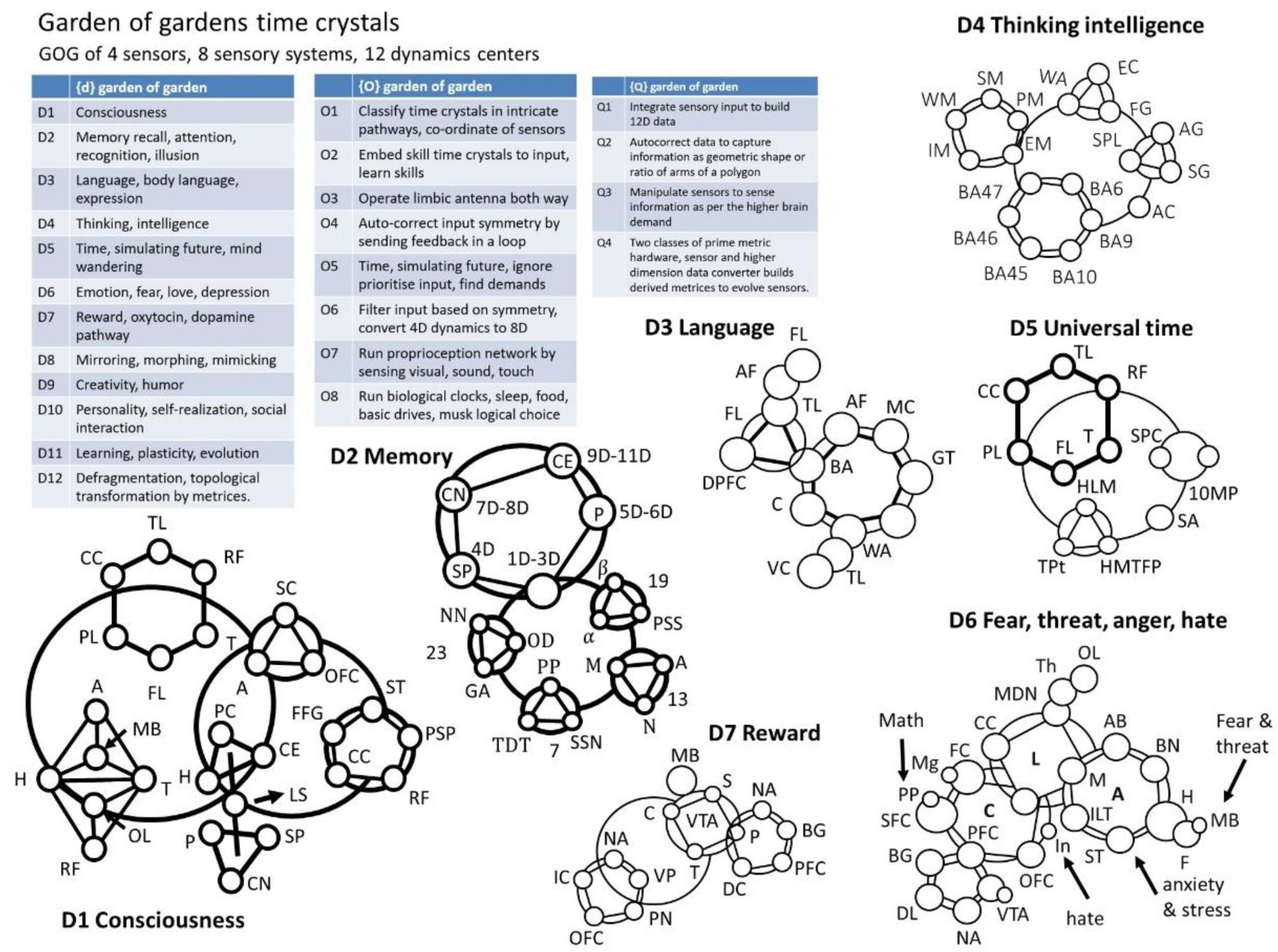
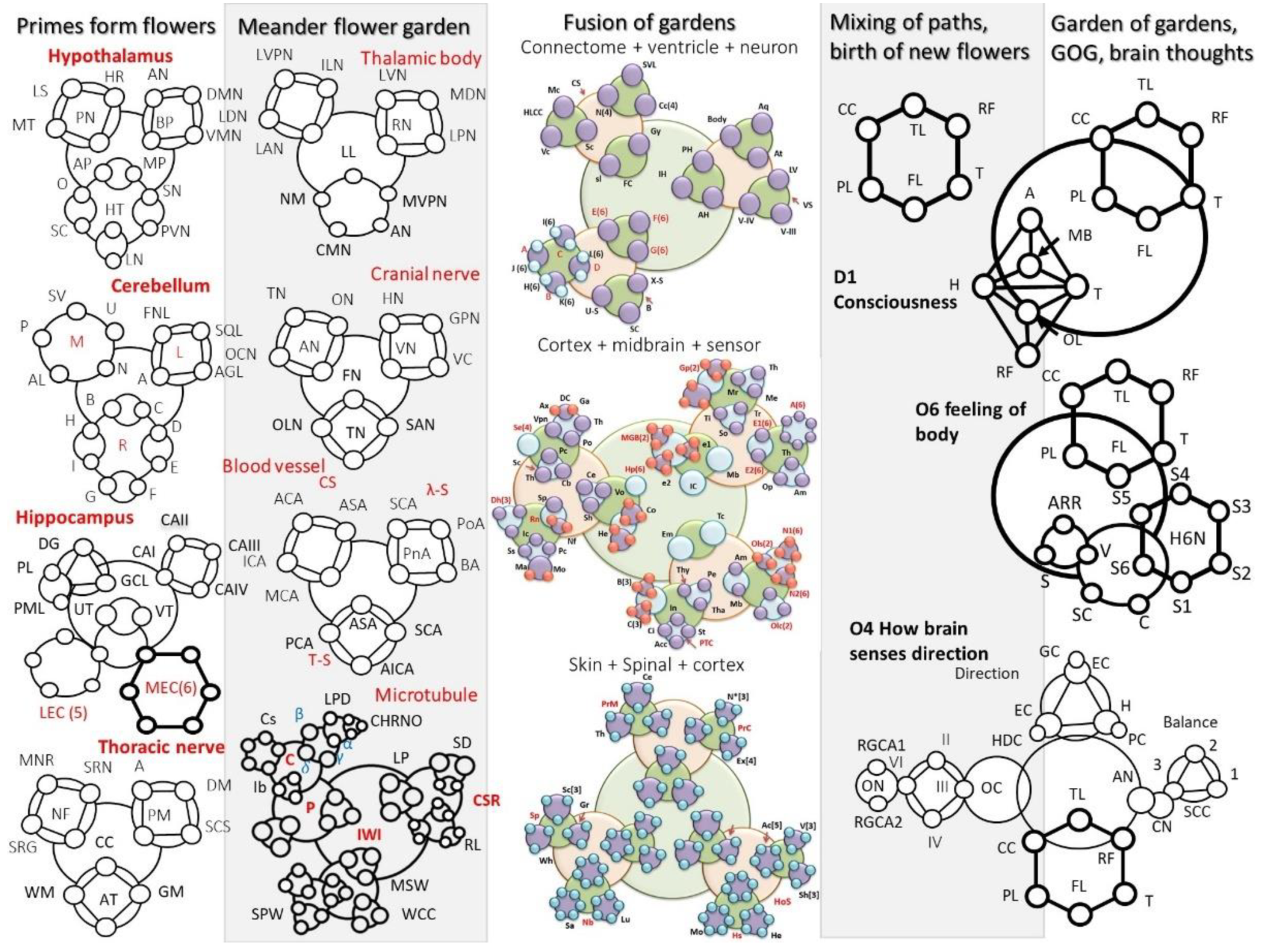
3. Garden of Gardens (GOG): Meander Flower Garden
3.1. Definition of a Meander Flower Garden
3.2. Hopf Bifurcation and Isochrones to Classify Time Crystals Built in a Meander Flower Garden
3.3. Constructing Garden of Garden (GOG) from the Flower Petals of a Meander Flower Garden
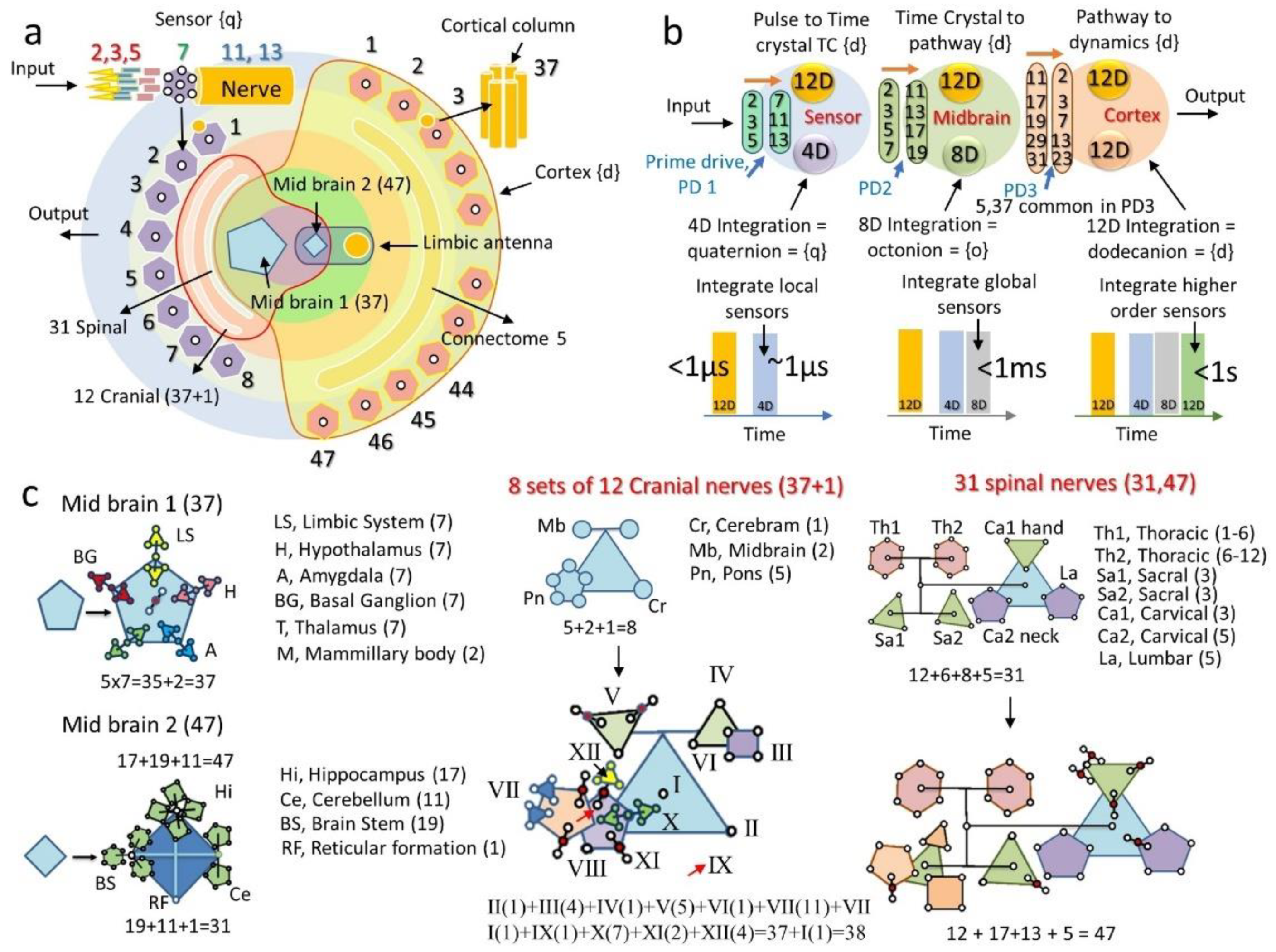
3.4. Selection of 12 Brain Components as Meander Flower Garden for Developing a Human Brain Model
4. Fourth Circuit Element: Hinductor
4.1. Looking beyond Memristor: Inventing a New Kind of Fourth Circuit Element Inspired by Microtubule, DNA and Several Basic Information Processing Devices in Biology
4.2. Synthesis of Time Crystals and Multi-Dimensional Tensors Using Fourth Circuit Element, Hinductor
5. Key Principles for the Development of the Human Brain: Assumptions and Mathematical Criterion for Consciousness
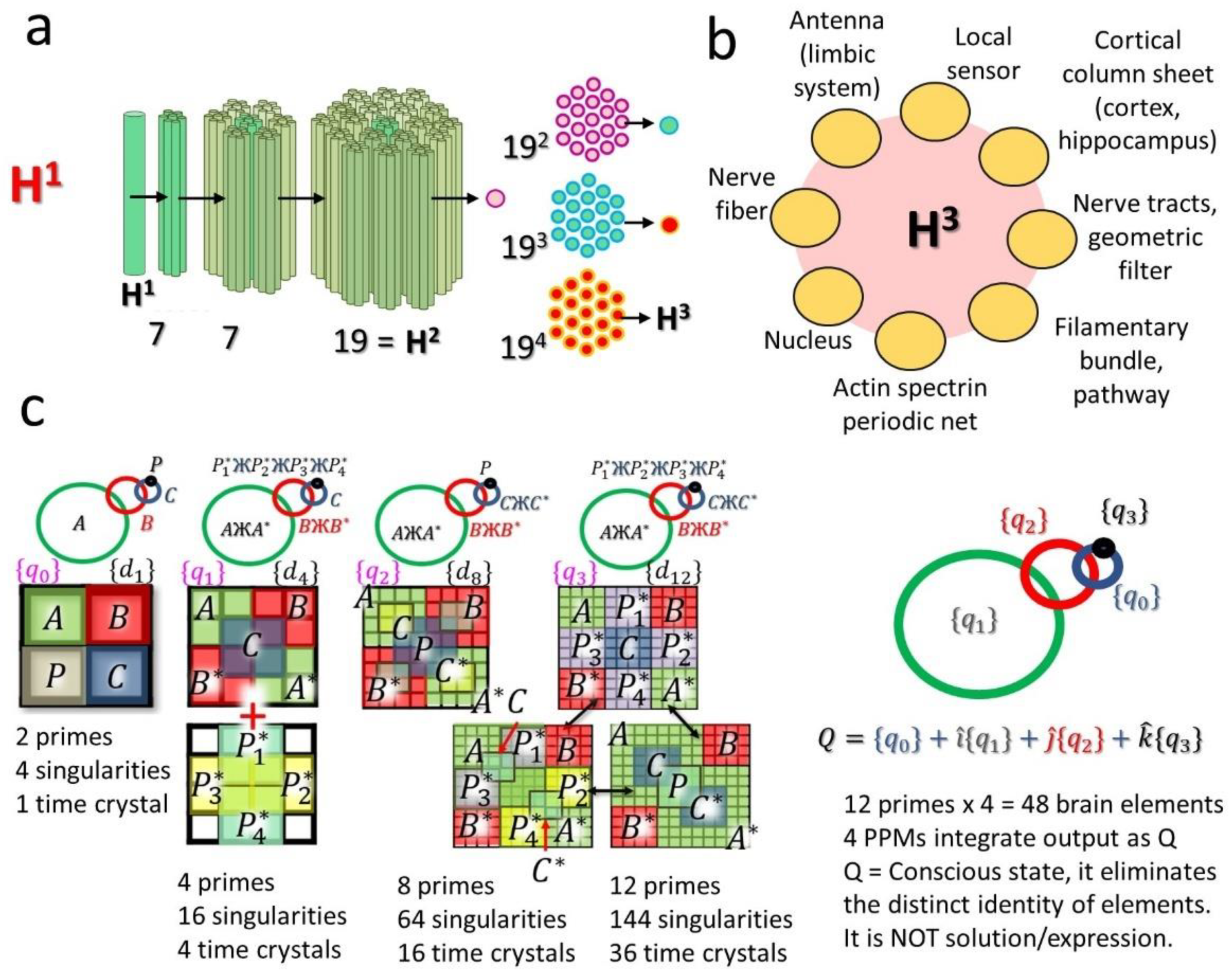
5.1. From Tensors to Primes in the Global Design of the Brain
5.1.1. Integration of 12 Brain Components and Their Parts: The Role of Primes
5.1.2. Tensors in the Brain: Hierarchy of Tensor Dimensions for Integrating the Time Crystals
5.1.3. Midbrain as the Interface between Sensors and the Higher-Level Cortex
5.2. Assumptions and Key Principles of Our Human Brain Model
- Operational language: Periodic clocks prepared by brain cavities at 109 order spatial scale to build an architecture of clocks spread over 1018 orders of time scale, regulate brain operation using a geometric language (geometric musical language, GML). Dielectric and cavity resonators self-assemble within and above to build the spatial architecture. Undefined phase regions of a clock allow other clocks to activate and run. Thus, clocks self-assemble within and above to cover 1018 orders of time scale. GML is the machine language, and it regulates brain operation.
- Unit of information & fundamental information processing device: Clock architecture breaking time symmetry is a unit of information of the brain. A spiral of three concentric cylinders, two water, and one material layer, build the fundamental information processing device H of the brain. H self-assembles to build another H, it’s the first generation H or H1. H1 builds H2, and hexagonal close packing of H2 in the form of a 2D sheet is the fundamental decision-making unit of the brain.
- Decision making: All input information is converted into the architecture of clocks, which changes its 11D dynamics following the StTs metric. The metric incorporates a pattern of primes (Phase prime metric, PPM; JP-2017-150173; World patent WO 2019/026984) to let the clock architecture transform its shape as a function of time. The transformation of clock architecture is the solution to the problem. It is the brain’s simulation of the future. Projection of clock architecture to infinity by creating an infinite series of structures using StTs metric and transforming the clock architecture by receiving feedback from infinity is the thought process of the brain.
- Memory, learning, and principle of circuit evolution: Clocks made of 12 types of carriers building 12 types of vortices (ring of fields act as particles). These vortices act as clocks or memories that transmits through dielectric and cavity resonator-based structures. As the brain learns, the cavity and dielectric resonators change arrangement following a resonance frequency identity that remains constant during brain evolution. The ratio of electrical resonance frequencies is (2.73…), the ratio of magnetic resonance frequencies is (golden ratio, 1.61….), the ratio of mechanical resonance frequencies is , the relation of linking the resonances is .
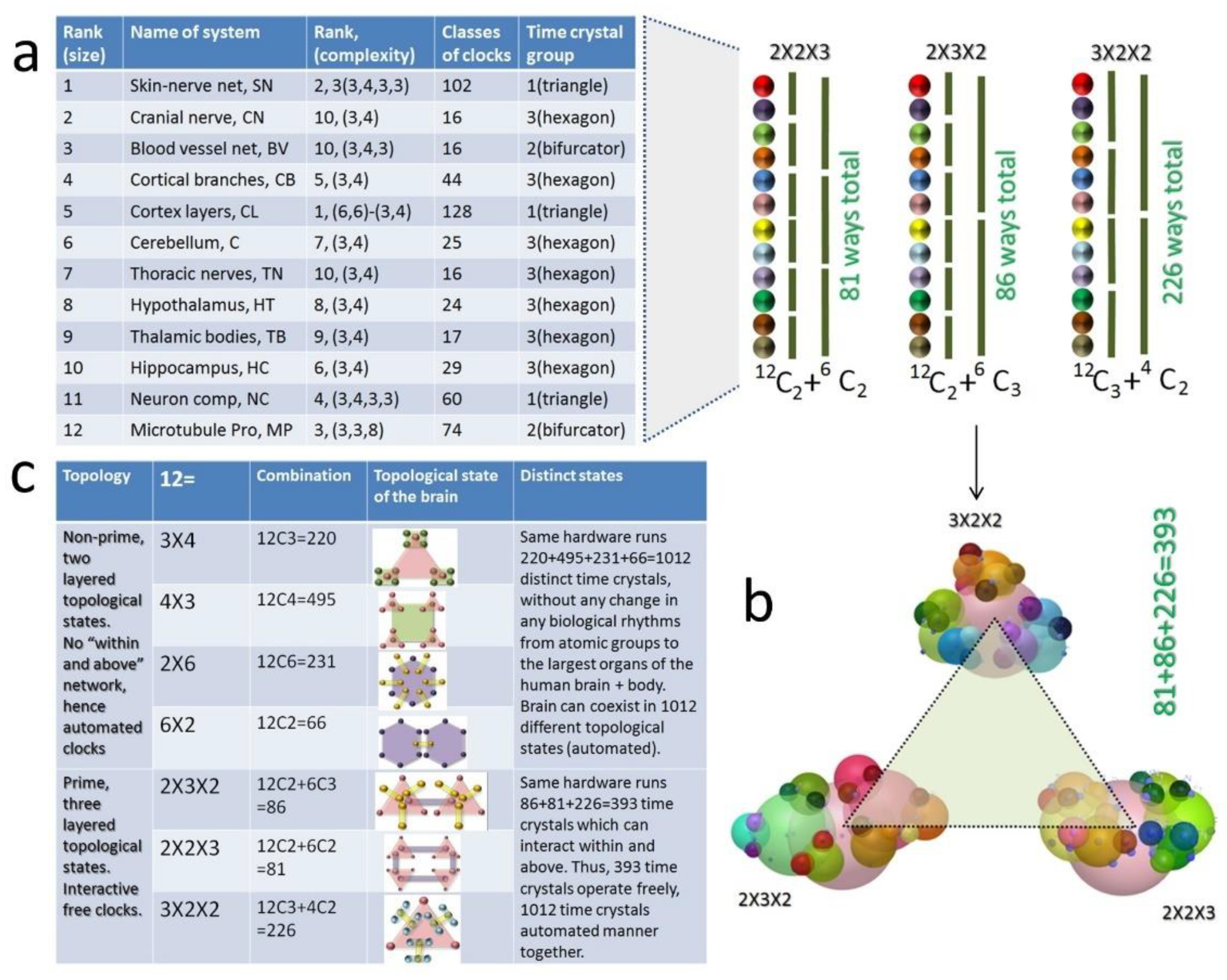
- Origin of consciousness: Each of the 12 major brain organs assembled one inside another, has twelve, time crystal-based information architecture. Since one could rearrange 12 clock architectures in 2 × 2 × 3, 2 × 3 × 2, and 3 × 2 × 2 format, the hardware could operate using three information architectures simultaneously. In this model of the human brain, the ability of the hardware to create, evolve, and operate three distinct, complete information structures simultaneously would be considered as one of the primary requirements of self-awareness.
6. Organs and Statistically Dominating Primes
6.1. Primes in Our Sensory System
6.1.1. Eyes
6.1.2. Ears
6.1.3. Nose
6.1.4. Touch
6.1.5. Tongue
7. Twelve Major Brain Components, 12 Key Dynamic Centers, and Primes in Their Structure Design
7.1. Why Did We Choose 12 Brain Components and Left Others?
7.2. Scale Free Universal Integration of Space, Time, Topology and Prime
7.3. Different Brain Components and How Do They Process Different Primes
7.3.1. Microtubule and Tubulin Protein, DNA, RNA (Molecular Spirals)
7.3.2. Neuron
7.3.3. Cortical Column and Cortex
7.3.4. Cerebellum
7.3.5. Hippocampus
7.3.6. Hypothalamus
7.3.7. Thalamus
7.3.8. Thoracic Nerve System
7.3.9. Cranial Nerve System (4 Sensors)
7.3.10. Blood Vessel Network in the Brain (Mechanical Rhythm Generator, Flow of Magnetic Element)
7.3.11. Cortical Branches or Connectome Geometry
7.3.12. Skin & Sensory Nerve Network (One Sensor)
7.4. A Summary, How the Brain Has Used 15 Prime Symmetries in Building Its Hardware
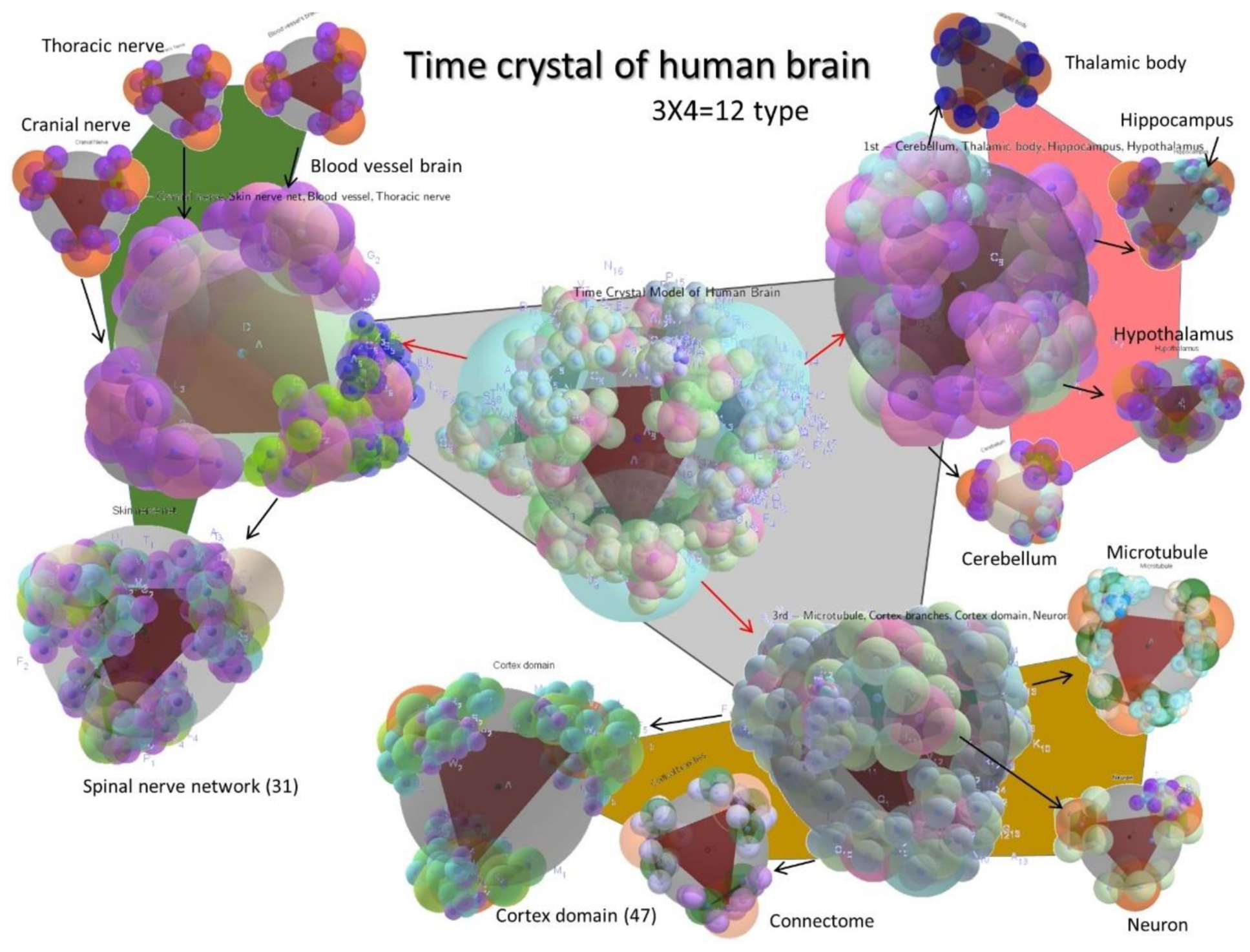
8. Time Crystal Map of the Whole Brain
8.1. Time Crystal Architecture of 12 Key Brain Components: Each Forms a Meander Flower Garden
8.1.1. Skin & Sensory Nerve Network (Largest Organ of the Human Body)
8.1.2. Cortical Branches and the Connectome: Connecting Organs
8.1.3. Functional Loops and Cavity Regions Are Put Together into One, Time Crystal
- D1 Consciousness: Central Executive, CE; Olfactory lobe, OL; Mamillary body, MB; Parietal lobe, PL; Corpus Calosum, CC; Temporal lobe, TL; Fusi form gyrus, FFG; Corpus calosum, CC; Primary sensory pad, PSP; Superior temporal, ST; Sensory pads, SP; Caudate nucleus, CN; Putamen, P; Amygdala, A; Hippocampus, H; Reticular formation, RF; Thalamus, T; Limbic system, LS; Superior caliculus, SC; Orbito-frontal cortex, OFC; Frontal lobe, FL; Parietal cortex, PC.
- D2. Memory: Protein secondary structure, PSS; - helix, ; - sheet, ; Microtubule, M; Actin, A; Neurofilament, N; Neuron, NN; Glia-Astrocyte, GA; Oligo-dendrocyte, OD; Pyramidal & polar, PP; Tear-drop transform, TDT; Super structures of neuron, SSN
- D3, Language and conversation: Arcuate fasciculus, AF; Temporal lobe, TL; Frontal lobe, FL; Dorsal Pre frontal cortex, DPFC; Motor cortex, MC; Broca’s area, BA; Gesdiwind territory, GT; Cerebellum, C; Wernicke’s area, WA; Visual cortex, VC
- D4. Thinking and intelligence: Extrastriate cortex, EC; Fusiform gyrus, FG; Wernicke’s area, WA; angular gyrus, AG; supramarginal gyrus, SG; superior parietal lobule, SPL; Anterior Cingulate, AC; Working memory, WM; Procedural memory, PM; Episodic memory, EM; Semantic memory, SM; Implicit memory, IM
- D5 Sense of universal time, symmetry
- 10 Metrices of prime, 10MP; Statistical prime contribution, SPC; Striatum amygdala, SA; Temporal lobe, TL; Thalamus, T; Parietal lobe, PL; Hippocampus long memory, HLM; Topological projected time, TPt; Frontal lobe, FL; Corpus calosum, CC; Reticular formation, RF; Hippocampus min time filtered pattern, HMTFP
- D6. Fear, threat, anger, hate
- Medical dorsal nucleus, MDN; Limbic system, LS; Thalamus, Th; Posterior portion, PP; Accessory basal, AB; Middle gyrus, Mg; Basal nucleus, BN; Cingulate cortex, CC; Cortex, C; Medical, M; Amygdala, A; Hypothalamus, H; Input lateral terminal, ILT; Olfactory lobe, OL; Fornix, F; Mammillary body, MB; Basal ganglia, BG; Frontal cortex, FC; Superior- Frontal cortex, SFC; Pre-frontal cortex, PFC; Ventral tangential array, VTA; Dopamine level, DL; Styria terminalis, ST; Nucleus acumens, NA; Orbitofrontal cortex, OC; Insula, In.
- D7. Reward
- Basal ganglia, BG; Nucleus accumbens, NA; Ventral tegmental area, VTA; Mid brain, MB; Pre-frontal cortex, PFC; Dopamine cycle, DC; Striatum, S; Pallidum, P; Thalamus, T; Cortex, C; Ventral pallidum, VP; parabrachial nucleus, PN; orbitofrontal cortex OFC; and insular cortex, IC
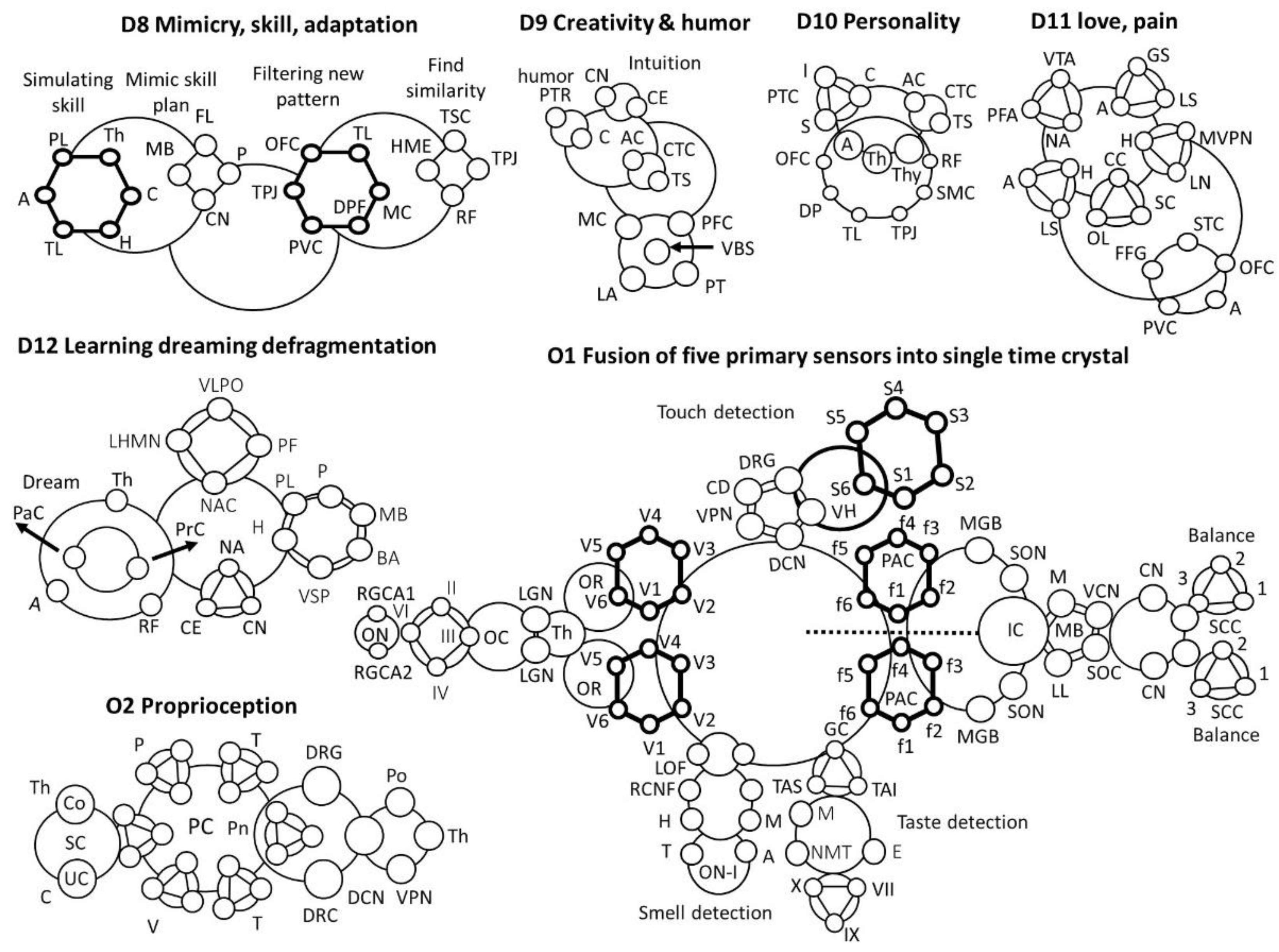
- D8 Mimicry, skill, adaptation
- Parietal lobe, PL; Thalamus, Th; Cerebellum, C; Hippocampus, H; Amygdala, A; Temporal lobe, TL; Caudate nucleus, CN; Mammillary body, MB; Putamen, P; Frontal lobe, FL; Primary visual cortex, PVC; Temporal lobe, TL; Dorsolateral Pre-frontal, DPF; Orbito frontal cortex, OFC; Motor cortex, MC; Reticular formation, RF; Supplementary Motor cortex, SMC; Thalamus sensor cross-over, TSC; Tempo-parietal junction, TPJ; Hippocampal memory encoding, HME
- D9. Creativity & Humor
- ACC Anterior Cingulate, AC; Temporal sulcus, TS; Caudate nucleus, CN; Central executive, CE; Left Amygdala, LA; Pre-frontal cortex, PFC; Motor cortex, MC; Posterior Temporal region, PTR; Motor cortex, MC; Ventral brain stem, VBS; Cerebellum, C; Pyramidal tract, PT
- D10. Personality
- Supplementary motor cortex, SMC; Creativity time crystal, CTC; Personality time crystal, PTC; Insula, I; Cingulate, C; Striatum, S; ACC Anterior Cingulate, AC; Temporal sulcus, TS; Thalamus, Th; Amygdala, A; Reticular formation, RF; Temporo-parietal junction, TPJ; Temporal lobe, TL; Desolation prefrontal, DP; Orbitofrontal cortex, OFC; Thyroid, Thy
- D11 Love and pain
- Lateral nucleus, LN; Medial ventral posterior nucleus, MVPN; Cingulate cortex, CC; Spindle cells, SC; Olfactory lobe, OL; limbic system, LS; Superior temporal cortex, STC; orbito-frontal cortex, OFC; Amygdala, A; Primary visual cortex, PVC; Fusiform gyrus, FFG; ventral tegmental area, VTA; nucleus accumbens, NA; Prefrontal cortex, PFC
- D12 Learning dreaming defragmentation
- Nucleus Accumbens, NA; Thalamus, Th; Caudate nucleus, CN; Reticular formation, RF; Central executive. CE; Prefrontal cortex, PrC; Putamen, P; Parietal cortex, PaC; Hippocampus, H; Broca’s area, BA; Visual scratch pad, VSP; Mamillary body, MB; Amygdala, A; Parietal lobe, PL; ventrolateral preoptic nucleus, VLPO; Parafacial zone, PF; Nucleus accumbens core, NAC; Lateral hypothalamic MCH neurons, LHMN
- O1 Fusion of elementary sensor into single time crystal
- Optic cranial nerve, II; trochlear nerve (IV); abducens nerve (VI); oculomotor nerve (III); Optic Chiasm, OC; Thalamus, Th; Optic Chiasm, OC; Optical nerve, ON; Retinal ganglion cell axon, RGCA; Lateral geniculate nucleus, LGN; Optical radiation, OR
- Cortex domain, CD; Ventral posterior nucleus, VPN; Dorsal column nuclei, DCN; Ventral horn, VH; Dorsal root ganglion, DRG; Touch, Pressure, Vibration, heat/cold, pain, proprioception (muscle), S1-S6
- Receptor cell nerve fiber, RCNF; Medial orbitofrontal, MOF; Tract, T; Amygdala, A; Lateral orbitofrontal, LOF; Hippocampus, H; Midbrain, M; Olfactory nerve, I
- Facial nerve (cranial nerve VII), the lingual branch of the glossopharyngeal nerve (cranial nerve IX), and the superior laryngeal branch of the vagus nerve (Cranial nerve X); Nucleus of medullary tract, NMT; Taste area of somatosensory, TAS; Taste area of insula, TAI; Medulla, M; Gustatory Cortex, GC; Thalamus, Th; Epiglottis, E
- Superior olivary nucleus, SON; Primary auditory cortex, PAC; Superior olive complex, SOC; Mid brain, MB; Lateral lemniscus, LL; Medulla, M; Inferior Colliculus, IC; Ventral Cochlear nucleus, VCN; Semicircular canals, SCC; Auditory nerve, AN; Medial geniculate body, MGB; Inferior colliculus, IC; Cochlear nucleus, CN; Auditory nerve, AN
- O2: Proprioception: Proprioception cells, PC; Pressure, P; Spinal cord, SC; Conscious, Co; Un-conscious, UC; Temperature, T; Cerebellum, C; Thalamus, Th; Vibration, V; Pons, Po; Pain, Pn; Touch, T; Dorsal root axon, DRA; Ventral Posterior nuclei, VPN; Dorsal root ganglion, DRG; Dorsal Column nuclei, DCN.
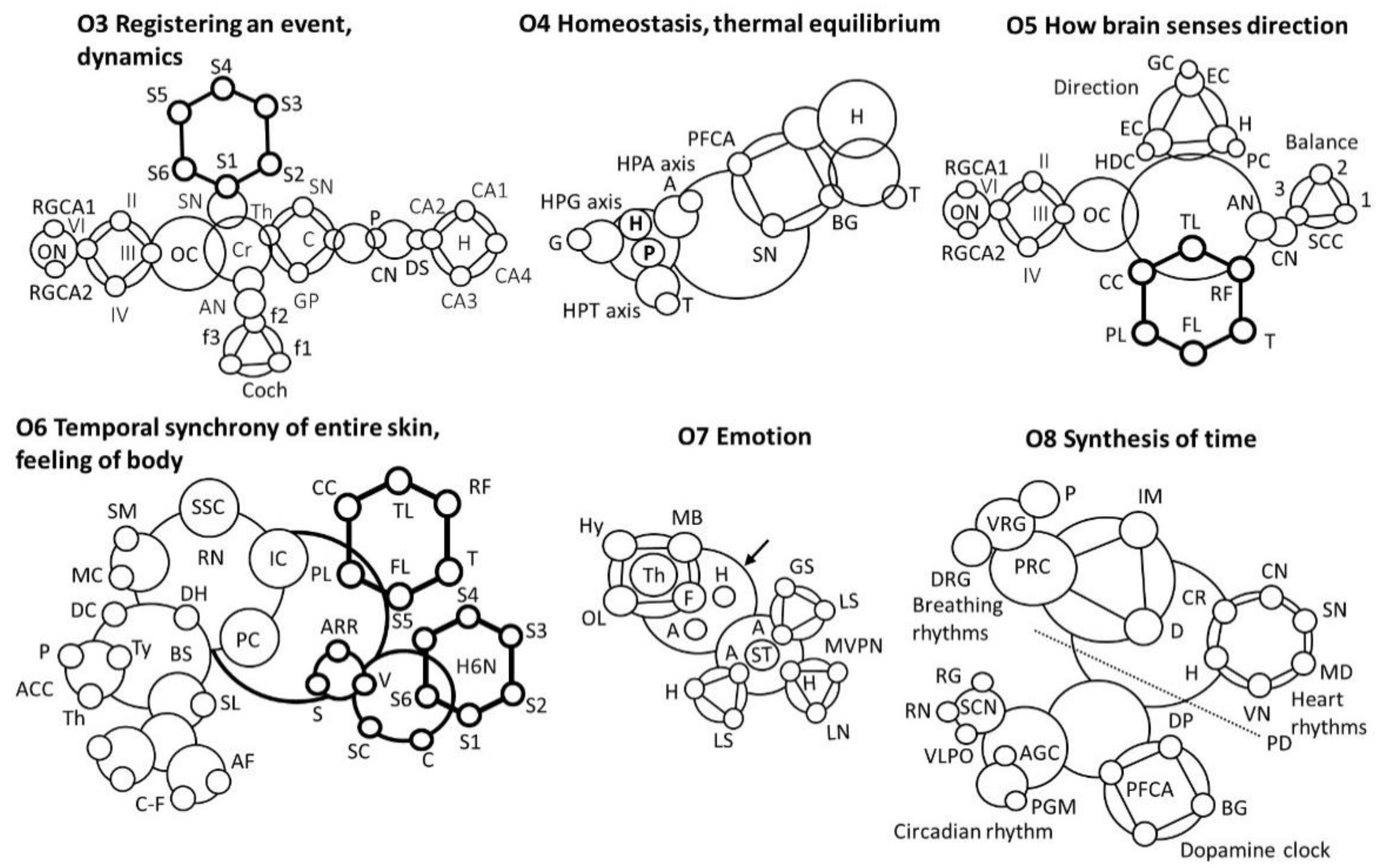
- O3 Motion or movement, audio+visual + time
- Registering an event
- Putamen, P; Caudate nucleus, CN; dorsal stratium, DS; Substantia Nigra, SN; Globus pallidus, GP; Claustrum, C; thalamus; Optical nerve, ON; Cochlea, Coch; Auditory nerve, AN; Retinal ganglion cell axon, RGCA; Spinal nerve, SN; Cerebellum, Cr.
- O4 Homeostasis, thermal equilibrium
- Pituitary, P; Gonad, G; Adrenal, A; Thyroid, T; Retina, R; Basal Ganglia, BG; Hypothalamus, H; Pituitary gland, PG; Melatomic level, ML; Superchiasmatic nucleus, SCN
- O5 How brain senses direction
- Semicircular canals, SCC; Cochlear nucleus, CN; Auditory nerve, AN; Optic cranial nerve, II; trochlear nerve (IV); abducens nerve (VI); oculomotor nerve (III), Optic Chiasm, OC; Retinal ganglion cell axon, RGCA; Temporal lobe, TL; Thalamus, T; Parietal lobe, PL; Frontal lobe, FL; Corpus calosum, CC; Reticular formation, RF; Place cells, PC; Hippocampus, H; Grid cells, GC; Entorhinal cortex, EC; Head direction cell, EDC
- O6 Temporal synchrony of entire skin cover, feeling of body
- Somato sensory Cortex, SSC; Supple mentary motor, SM; Raphe Nucleus, RN; Motor cortex, MC; Spinal loop, SL; Insular Cortex, IC; Descending connection, DC; Analgesic chemical cycles, ACC; Parietal Cortex, PC; Pituitary, P; C- fibre, C-F; Thyroid, Ty; Brain stain, BS; Nerve fibre, NF; Dorsal horn, DH; A-delta fiber, AF; Thalamus, Th; Activate automated response, AAR; Spinal cord, SC; Hippocampus, (6 nucleus), H6N; Shiver, S; Voluntary, V; Cerebral, C; Temporal lobe, TL; Thalamus, T; Parietal lobe, PL; Frontal lobe, FL; Corpus calosum, CC; Reticular formation, RF
- O7 Emotion
- Medial ventral posterior nucleus, MVPN; Amygdala, A; limbic system, LS; GABA secretion, GS; Hippocampus, H; Hypothalamus, H; Mamillary body, MB; Olfactory lobe, OL; Lateral nucleus, LN; Fornix, F; Thalamus, Th; Stria terminalis, ST
- O8 Time
- Medulla, MD; Pons, P; Internal muscle, IM; Internal muscle, IM; Vagus nerve, VN; Diaphragm, D; Ventral Respiratory Group, VRG; Pontine Respiratory Center, PRC; Dorsal Respiratory Group, DRG; Sinoatrial node, SN; Cardiac nerve, CN; Cardio Regulator, CR; Hypothalamus, H; Basal Ganglia, BG; Dopamin Path, DP; Pre-frontal cortex, anterior, PFCA; Substantia Nigra, SN; Pyramidal decussation, PD; Raphe nuclei, RN; Superchiasmatic nuclei, SCN; Adrenal gland cortisol, AGC; Pineal gland melatonin, PGM; Ventro-lateral preoptic nucleus, VLPO; Retina ganglion, RG; Tuberomammillary nucleus, TMN.
8.1.4. Time Crystal of a Neuron
8.1.5. Time Crystal of a Single Microtubule
8.1.6. Time Crystal of the Cerebellum, Thalamic Body, Hippocampus, and the Hypothalamus
8.1.7. The Time Crystal of the Complete Brain
8.2. The Coexistence of Biological Clocks of Various Kinds with the Materials Key Resonances: Could Both Be Linked?
9. Garden of Gardens of Meander Flowers: 20 Conscious Experiences and Their Time Crystal as Reported in the Literature
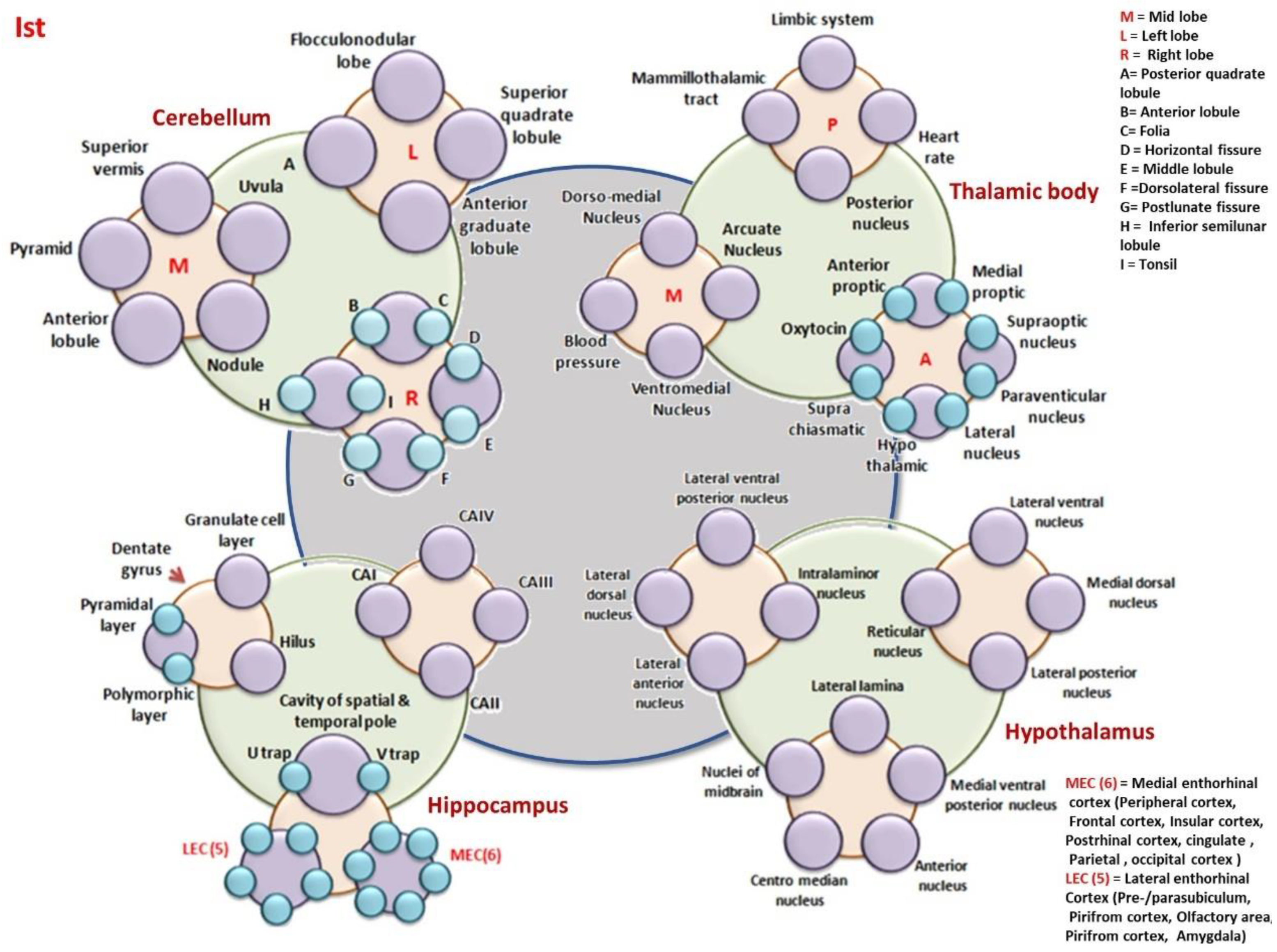
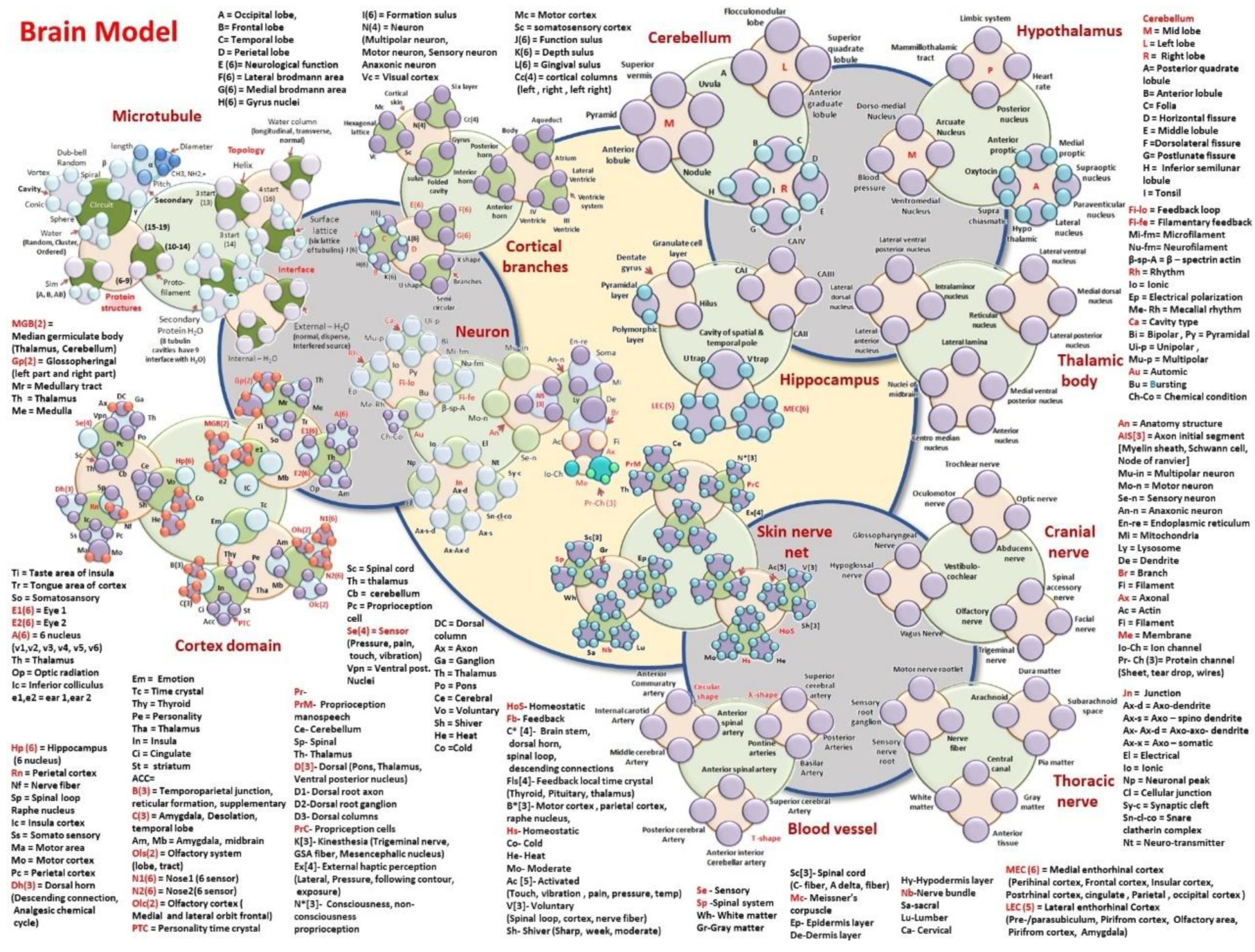
- Hypothalamus: MT-Mammilothalamic tract, LS-Limbic system, HR-Heart rate, PN-Posterior nucleus, DMN-Dorso medial nucleus, AN-Arcuate nucleus, VN-Ventromedial nucleus, BP-Blood pressure, AP-Anterior Proptic, MP-Medial proptic, SN-Supraoptic nucleus, PVN-Paraventricular nucleus, LN-Lateral nucleus, HT-Hypothalamic nucleus, SC-Suprachiasmatic, O-Oxytocin,
- Cerebellum: SV-Superior vermis, P-Pyramid, AL-Anterior lobule, N-Nodule, U-Uvula, AGL- Anterior Graduate Lobule, A-Posterior quadrate lobule, SQL-Superior quadrate lobule, FL-Flocculonodular Lobe, B-Folia, D-Horizontal Fissure, E-Middle lobule, F-Dorsolateral fissure, G-Postlunate Fissure, H-Inferior semilunar lobule, I-Tonsil
- Hippocampus: DG-Dentate Gyrus, GCL-Granulate Cell Layer, H-Hilus, PL-Pyramidal Layer, PML-Polymorphic layer, Cavity of spatial and temporal pole is made of UT and VT, UT-U Trap, VT-V Trap, LEC(5)-Lateral Enthorhinal Cortex, (Pre/Parasubiculum, Pirifrom Cortex, Olfactory area, Amygdala). MEC(6)-Medial Enthorhinal cortex, (Perihinal cortex, frontal cortex, insular cortex, postrhinal cortex, cingulate gyrus, Parietal, Occipital cortex)
- Thoracic nerve: MNR-Motor nerve rootlet, SRN-Sensory root Ganglion, SNR-Sensory nerve root, NF-Nerve Fiber, A-Arachnoid matter, DM-Dura matter, PM-Pia matter, SCS-Suprachnoid space, CC-Central canal, WM-White matter, GM-Gray matter, AT-Anterior Tissue.
- Thalamic body: LVPN-Lateral ventral posterior nucleus, LDN-Lateral dorsal nucleus, LAN-Lateral anterior nucleus, IN-Intralaminor nucleus, LL-Lateral lamina, NM-Nuclei of midbrain, CMN-Centro median nucleus, AN-Anterior nucleus, MVPN-Medial ventral posterior nucleus. RN-Reticular nucleus, LPN-Lateral posterior nucleus, MDN-Medial dorsal nucleus, LVN-Lateral ventral nucleus.
- Cranial nerve: TN: Trochlear nerve, ON-Oculomotor nerve, AN-Abducens nerve, ON-Optic nerve, HN-Hypoglossal nerve, GN-Glossopharyngeal nerve, VC-Vestibulo-cochlear, VN-Vagus nerve, FN-Facial nerve, ON-Olfactory nerve, TN-Trigeminal nerve, SAN-Spinal accessory nerve.
- Blood Vessel’s branches: Circular shape (ACA-Anterior commutatory artery, ICA-Internal carotid artery, MCA-Middle cerebral artery, ASA-Anterior spinal artery), -shape(PA-Pontine Arteries, BA-Basilar artery, PA-Posterior Artery, SCA-Superior cerebral artery), T-shape (ICA-Interior cerebral artery, ASA-Anterior spinal artery, PCA-Posterior cerebral artery, AICA-Anterior Interior cerebral artery)
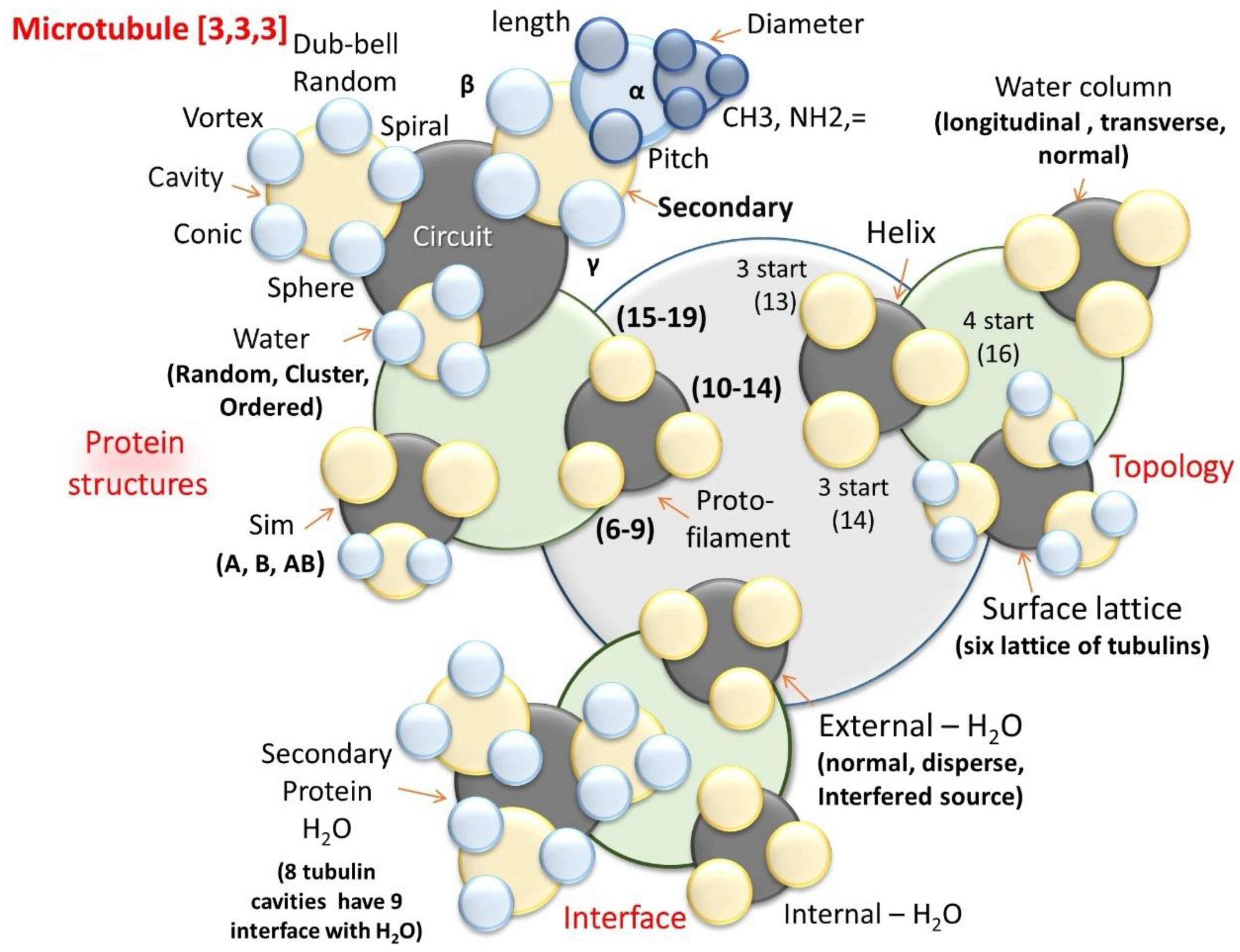
- Microtubule: Tubulin protein structure
- Secondary protein [-helices (LPD-Length pitch and diameter; CHRNO-alkyl, amine), ]. Cavity[Conic, vortex, dumb-bell or tear drop, spiral]. Water layer [Random arrangement, cluster, ordered] Sim on the lattice surface, S(lattice type A, B and AB) and Protofilaments (numbers, 6–9, 10–14, 15–19).
- Topological morphogenesis of microtubule surface
- Helical transport path (periodicity helical gap 2,3, (returns on 13); gap 4 (returns on 16)); Central water channel (Longitudinal, transverse and normal); Surface tubulin lattice (six distinct classes of lattices observed in the tunnelling images)
- Interface between sub-helices & surfaces
- Secondary protein water channel (triplet of triplet domains, 8 tubulin cavities have 9 interfaces with H2O); external surface water layer (1-2molecular layer of water in three modes, normal, disperse, interfered source); Inner core water channel (longitudinal, transverse and normal modes of water).
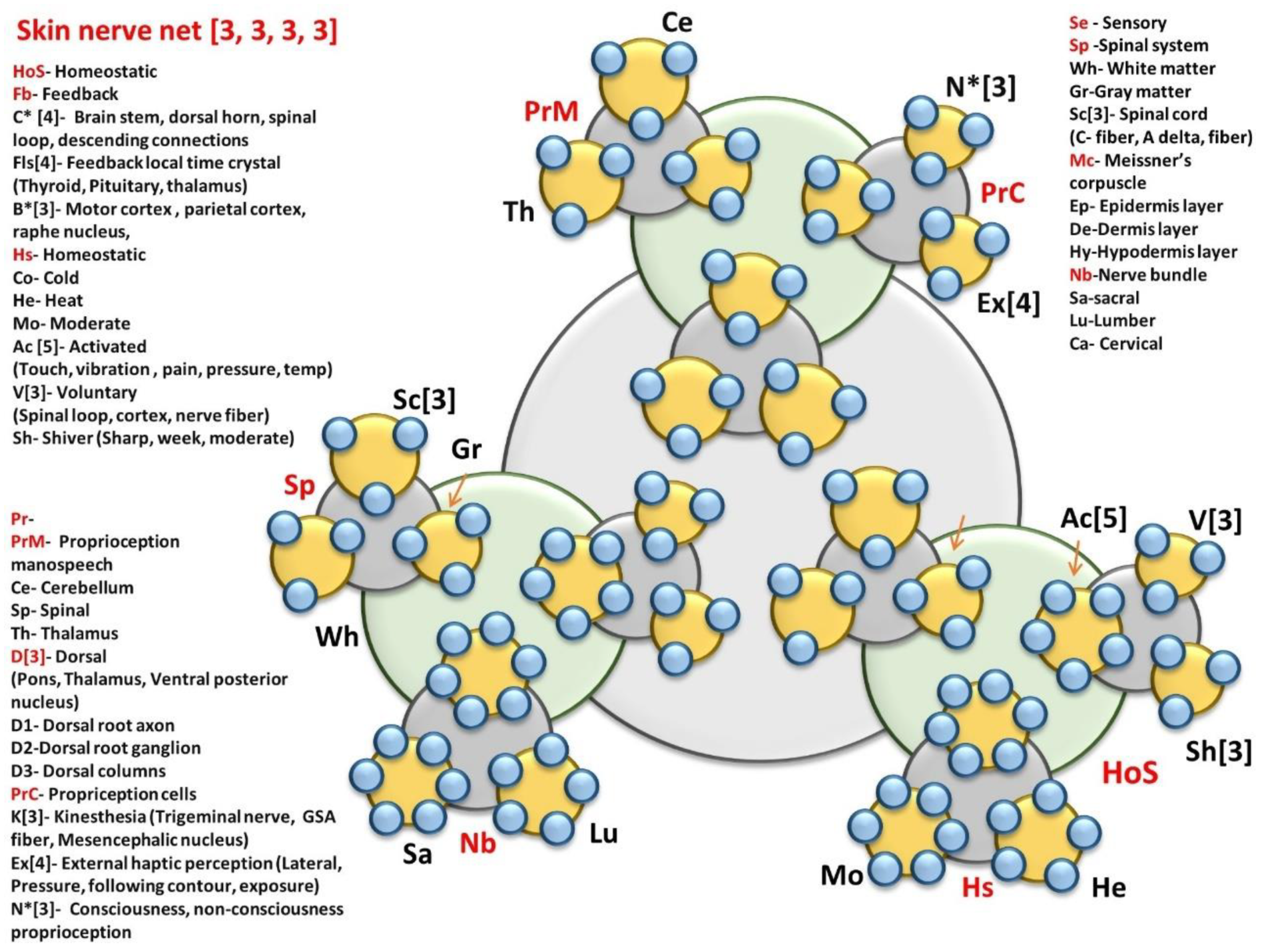
- Skin nerve network: Homeostatic primary clock network
- HoS- Homeostatic; Fb- Feedback; C* [4]- Brain stem, dorsal horn, spinal loop, descending connections; Fls[4]- Feedback local time crystal (Thyroid, Pituitary, thalamus); B*[3]- Motor cortex, parietal cortex, raphe nucleus, Hs- Homeostatic; Co- Cold; He- Heat; Mo- Moderate; Ac [5]- Activated; (Touch, vibration, pain, pressure, temp); V[3]- Voluntary, (Spinal loop, cortex, nerve fiber); Sh- Shiver (Sharp, week, moderate)
- Brain controlled higher level network connected to skin
- PrM- Proprioception manospeech; Ce- Cerebellum; Sp- Spinal; Th- Thalamus; D[3]- Dorsal, (Pons, Thalamus, Ventral posterior nucleus); D1- Dorsal root axon; D2-Dorsal root ganglion; D3- Dorsal columns; PrC- Propriception cells; K[3]- Kinesthesia (Trigeminal nerve, GSA fiber, Mesencephalic nucleus); Ex[4]- External haptic perception (Lateral, Pressure, following contour, exposure); N*[3]- Consciousness, non-consciousness
- Proprioception
- Sensory pathway via spinal cord
- Se – Sensory; Sp -Spinal system; Wh- White matter; Gr-Gray matter; Sc[3]- Spinal cord, (C- fiber, A delta, fiber) Mc- Meissner’s corpuscle; Ep- Epidermis layer; De-Dermis layer; Hy-Hypodermis layer; Nb-Nerve bundle; Sa-sacral; Lu-Lumber; Ca- Cervical
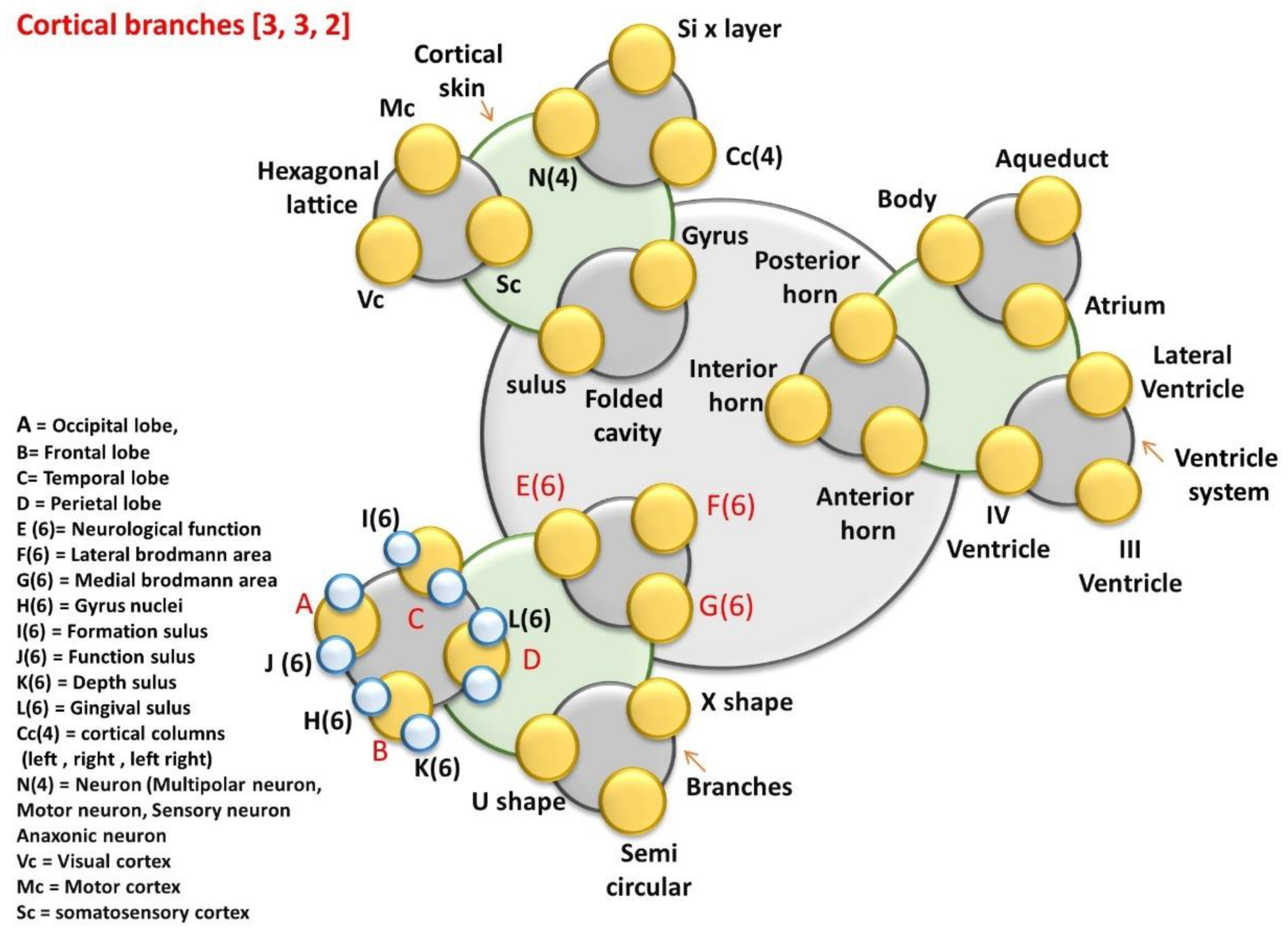
- Cortical branches: Ventricular and geometric cavities
- Aq-Aqueduct, Body, At-Atrium, LV-Lateral Ventricle, VS-Ventricular system, IV-V = IV Ventricle, III-V = III Ventricle, PH-Posterior Horn, IH-Interior Horn, AH-Anterior Horn,
- Top folded hexagonal lattice domains (47 Brodman’s regions)
- CS-Cortical system; SVL-Seven vertical layers of cortical column, N(4), Hexagonal lattice, Mc, Vc, Sc. Folded cavity, Sl-Sulus, Gy-Gyrus, Cc(4).
- Nerve bundle for different functional region.
- E(6), F(6), G(6), L(6), I(6), J(6), H(6), K(6), Three types of branches B, X-S=X-shape, Semi-circular-SC, and U-S-U shape.
- A = Occipital lobe, B = Frontal lobe, C = Temporal lobe, D = Perietal lobe, E (6) = Neurological function, F(6) = Lateral brodmann area, G(6) = Medial brodmann area, H(6) = Gyrus nuclei, I(6) = Formation sulus, J(6) = Function sulus, K(6) = Depth sulus, L(6) = Gingival sulus, Cc(4) = cortical columns (left, right, left right), N(4) = Neuron (Multipolar neuron, Motor neuron, Sensory neuron, Anaxonic neuron, 23 types of neural branches are studied), Vc = Visual cortex, Mc = Motor cortex, Sc = somatosensory cortex
- Cortex domain: MGB(2) = Median germiculate body (Thalamus, Cerebellum); Gp(2) = Glossopheringal (left part and right part); Mr = Medullary tract; Th = Thalamus; Me = Medulla; Ti = Taste area of insula; Tr = Tongue area of cortex; So = Somatosansory; E1(6) = Eye 1; E2(6) = Eye 2; A(6) = 6 nucleus; (v1,v2, v3, v4, v5, v6); Th = Thalamus; Op = Optic radiation; Ic = Inferior colliculus; e1,e2 = ear 1,ear 2.
- Em = Emotional pathways (details in functional time crystals); Tc = Time crystal clusters of hippocampal output, Thy = Thyroid, Pe = Personality time crystal, Tha = Thalamus, In = Insula, Ci = Cingulate gyrus learning pathway, St = striatum, ACC=, B(3) = Temporoparietal junction, reticular formation, supplementary; C(3) = Amygdala, Desolation, temporal lobe; Am, Mb = Amygdala, midbrain; Ols(2) = Olfactory system (lobe, tract); N1(6) = Nose1 (6 sensor, +one controller 6 + 1 = 7); N2(6) = Nose2(6 sensors +one controller, 6 + 1 = 7); Olc(2) = Olfactory cortex (Medial and lateral orbit frontal); PTC = Personality time crystal.
- Sc = 31 Spinal cord cavity’s time crystal; Th = thalamus, Cb = cerebellum, Pc = Proprioception cell based network, Se(4) = Sensor (Pressure, pain, touch, vibration); Vpn = Ventral post. Nuclei, DC = Dorsal column
- Ax = Axonal branches connecting cortical columns, Ga = Ganglion, Po = Pons brain stem, Ce = Cerebral
- Vo = Voluntary, Three sensations of homeostasis trigger large scale information processing, Sh = Shiver; He = Heat; Co = Cold
- Hp (6) = Hippocampus; (6 nucleus, +one controller 6 + 1 = 7); Rn = raphe nucleus, Nf = Nerve fiber singular time crystal, Sp = Spinal loop time crystal built by cross sectional components; Ic = Insula cortex; Ss = Somato sensory; Ma = Motor area, Mo = Motor cortex; Pc = Perietal cortex; Dh(3) = Dorsal horn, (Descending connection, Analgesic chemical cycle)
- All components of the column 4 and column 5 are described in the Figures following this presentation.
- Somatosensory, sensitive vibration, proprioception and fine touch, temperature, vibration, and pain. Deep proprioception. Intermediate postcentral.
- Somatosensory, sensitive vibration, proprioception and fine touch, temperature, vibration, and pain. Deep proprioception. Rostral postcentral.
- Somatosensory, sensitive vibration, proprioception and fine touch, temperature, vibration, and pain. Deep proprioception. Caudal postcentral.
- The motor fibers originate from the precentral gyrus. Searches for periodic events and joins multiple clocking events into a single clocking event. Proprioception.
- Somatosensory coordinating region; Perception of personal space. Processing chaotic pattern, Self-reflection during decision making, temporal context recognition.
- The initiation of movement arises here. Selective attention to rhythmic responses. Generating melodic phrases. Language processing and speech perception. Topographic memory.
- Attention, geometry-based deductive reasoning. Mental rotation, temporal context detection. The recollection of the experience, pains.
- Activates for coherent movement of the visual field, working memory. Still, since this region is responsible for planning and perceptual priming, it is affected by several diseases, executive dysfunction, Schizophrenia, and Alzheimer’s. Proprioception.
- Executive control of behavior. Detect error, identify the difference between conflict and reward. Social judgment, executive memory, abstract thinking, and intentionality.
- The executive function of event and time-based prospective memory. Multiple attentions at a time, expressing intention and response to the thermal stimuli. Forget intentionally.
- It is involved in emotional decision making and processing rewards, planning, encoding new information into long-term memory, and reasoning. Listening without talking.
- Orbitofrontal area, taste, Social Cognition, stabilize mood to a fixed state, and Mental Time. This region is indirectly connected to the global Palladius as well as the substantia nigra, due to efferent to the striatum.
- Insular cortex, behavioral inhibition, calculation, response to fear, feeling pain. Compassion and empathy, perception, motor control, self-awareness, cognitive functioning, and interpersonal experience. It is involved in psychopathology.
- Insular cortex, behavioral inhibition, calculation, response to fear, feeling pain. Compassion and empathy, perception, motor control, self-awareness, cognitive functioning, and interpersonal experience. It is involved in psychopathology.
- Anterior temporal lobe, behavioral inhibition, calculation, response to fear, feeling pain.
- Insular cortex, behavioral inhibition, calculation, response to fear, feeling pain; Compassion and empathy, perception, motor control, self-awareness, cognitive functioning, and interpersonal experience. It is involved in psychopathology.
- V1, primary, calcarine, or striate cortex) is the end organ of the afferent visual system, pattern, and orientation of objects.
- V2, Visual, confronting color and shape, correlating form and name, motion (shape + time).
- V3, Visual mental imagery, connecting words or sentences, and build an associative structure.
- Comprehending metaphor, abstract events, objects; visual to the perceptual whole, thus, processing the task of visual memory processing.
- Complex composition of sounds processing, correlating texts to words, build sentences for language processing.
- Part of the superior temporal gyrus included in the language processing region known as Wernicke’s area. Prosody comprehension. Detecting key tone features of multiple languages.
- Ventral posterior cingulate cortex, comprehension of the concept, emotional language; dynamics of geometric shapes (topokinetics).
- Ventral anterior cingulate cortex, expression; behavioral & motor inhibition, mental timekeeping, feeling pain.
- Subgenual area (part of the Ventromedial prefrontal cortex).
- Ectosplenial portion of the retrosplenial region of the cerebral cortex; dynamics of geometric shapes (topokinetics), comprehension of feelings in the words.
- Piriform cortex, experiencing and realization of emotion.
- Ventral entorhinal cortex, experiencing and realization of emotion.
- Retrosplenial cingulate cortex, comprehension by retrieving memory; dynamics of geometric shapes (topokinetics).
- Part of cingulate cortex, comprehension by retrieving memory; dynamics of geometric shapes (topokinetics).
- Dorsal Posterior cingulate cortex, comprehension by retrieving memory; dynamics of geometric shapes (topokinetics).
- Dorsal anterior cingulate cortex, smell related differentiation, comprehension by retrieving memory; behavioral & motor inhibition, various emotional stimuli, mental timekeeping, feeling pain.
- Part of anterior cingulate cortex, comprehension by retrieving memory; behavioral & motor inhibition, various emotional stimuli, feeling pain, mental timekeeping.
- Dorsal entorhinal cortex (on the Parahippocampal gyrus), comprehension by retrieving memory; experiencing and realization of emotion; Novelty discrimination.
- Perirhinal cortex (in the rhinal sulcus), comprehension by retrieving memory; experiencing and realization of emotion; Novelty discrimination.
- Ectorhinal area, now part of the perirhinal cortex (in the rhinal sulcus); working memory; experiencing and realization of emotion; Novelty discrimination.
- Recognizing true and false memories, episodic encoding. Judging minute changes of known events, objects, face recognition. Semantic relationship extraction.
- The junction that links hearing, vision, memory, emotional awareness, and reactions. Diverse language functions. (11, 38, 47 emotions). Comprehending humor, inferential reasoning to fear, threat, emotional attachment. Comprehending narrative, the composition of voice tone, music.
- Mathematics, abstract reasoning. Executive control of systematic behavior, the sequence of words, and the sequence of tasks organized.
- Region of creativity. Comparing past, present events, memory, images, music, etc. Detecting conflict between intention and sensory drive; often compare tactile and proprioceptive response. Performing music.
- The critical technical parameters of a complex sound are analyzed. Rapid bursts of sounds are decomposed, and all essential parameters are retrieved.
- Retrieving and simulating missing auditory patterns. Segregating isolated parts of the sound, repetition priming effect.
- Digit or abstract numbers, integers, primes stimulate this region. Understanding codes.
- Executive function on identifying grammar rules, retrieving unintelligible speech, expressing emotion, compose music, triggering expressive movement.
- Broca’s area, aesthetic appreciation, editing, or modulating the emotional response. Inhibiting or hiding response, build a filtered sequence of facts, events, data for the rapid expression.
- Executive control on behavior, calculating in mind, estimating self-reflection, detecting conflict and changing strategy and exhibiting empathy.
- Meticulous sensing of stimuli, inhibiting emotional response. Sensitive temporal coherence among various signals, like language, music, etc.
10. The Operational Mechanism of the Brain: Frequently Asked Questions
10.1. How Brain Structures Evolve, How Learning or Thinking Changes the Brain Circuits? What Is Wrong with the Current Neuroscience Model and How Our Model Helps in Bridging Those Missing Links?
10.2. Do All Humans Have Same Time Crystal Architecture? Same PPM Architecture for the Brain? How Do Two Humans Differ?
10.3. How Does a Change in the Arrangement of Clocks Affect Brain Hardware? The Foundation of Neurogenesis in Our Brain Model
10.4. Digital Reconstruction of the Time Crystal Reconstruction of the Human Brain Is Possible? Could Our Brain Model Be Integrated with Modern Neuroscience?
10.5. In Conventional Time Crystal Known for 50 Years, the Unit Cell Is Made of Only One Kind of Atoms or Clocks, Now Many Atoms Participate. How Polycrystals Are Born, Why Are They Necessary?
10.6. Two Different Concepts of Dimensions Are Applied Together in the Brain Model, Was Introducing 12 Dimensions Necessary?
10.7. Introduction of New Mechanics to Explain the Brain, Was It Essential? Does Time Crystal Model Propose the Brain as a Quantum Device or a Classical Device?
10.8. Introduction of Non-Computability in Brain Operation, Was It Essential?
10.9. How Many Different Kinds of Brain Maps Do We Create? Which Brain Map Is Absolute?
10.10. If Some Components Get Destroyed, Does the Brain Loses Memory or the Processing Ability?
10.11. Would There Be Any Other Applications of the STts Metric Built Exclusively for Modeling the Human Brain?
10.12. Is There Any Other Human Brain Model That Argues for the 11D Information Processing? What Are the Differences with the Proposed Time Crystal Model of the Human Brain?
11. Conclusion: A Global Effort Required to Perfect the time Crystal Map of the Whole Brain
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
Appendix A
References
- Winfree, A.T. Exploratory data analysis: Published records of uncued human sleep and waking. In Mathematical Modeling of Circadian Systems; Moore-Ede, M., Czeisler, C.A., Eds.; Raven Press: New York, NY, USA, 1984; pp. 187–200. [Google Scholar]
- Winfree, A. Biological Rhythm Research. In The Geometry of Biological Time, 2nd ed.; Springer: New York, NY, USA, 2001. [Google Scholar]
- Else, D.V.; Bauer, B.; Nayak, C. Floquet time crystals. Phys. Rev. Lett. 2016, 117, 090402. [Google Scholar] [CrossRef] [PubMed]
- Best, E.N. Null Space and Phrase Resetting Curves for the Hodgkin-Huxley Equations. Ph.D. Thesis, Purdue University, West Lafayette, IN, USA, 1976. [Google Scholar]
- Bruce, V.G.; Wright, F.; Pittendrigh, C.S. Resetting the sporulation rhythm in Pilobolus with short light flashes of high intensity. Science 1960, 131, 728–730. [Google Scholar] [CrossRef] [PubMed]
- Yao, N.Y.; Potter, A.C.; Potirniche, I.D.; Vishwanath, A. Discrete time crystals: Rigidity, criticality, and realizations. Phys. Rev. Lett. 2017, 118, 030401. [Google Scholar] [CrossRef] [PubMed]
- Zhang, J.; Hess, P.W.; Kyprianidis, A.; Becker, P.; Lee, A.; Smith, J.; Pagano, G.; Potirniche, I.D.; Potter, A.C.; Vishwanath, A.; et al. Observation of a discrete time crystal. Nature 2017, 543, 217–220. [Google Scholar] [CrossRef] [PubMed]
- Winfree, A.T.; Strogatz, S. Singular filaments organize chemical waves in three dimensions. 4: Wave taxonomy. Physica D 1984, 13, 221–233. [Google Scholar] [CrossRef]
- Betz, A.; Becker, J.U. Phase dependent phase shifts induced by pyruvate and acetaldehyde in oscillating NADH of yeast cells. J. Interdiscipl. Cycle Res. 1984, 6, 167–173. [Google Scholar] [CrossRef]
- Reddy, S.; Sonker, S.; Singh, P.; Saxena, K.; Singh, S.; Chhajed, R.; Tiwari, S.; Karthik, K.V.; Ghosh, S.; Ray, K.; et al. A Brain-like Computer Made of Time Crystal: Could a Metric of Prime Alone Replace a User and Alleviate Programming Forever? Stud. Comput. Intell. 2018, 761, 1–44. [Google Scholar]
- Zykov, V.S. Cycloidal circulation of spiral waves in an excitable medium. Biofizika 1986, 31, 862–865. [Google Scholar]
- Zykov, V.S.; Morozova, O.L. Computer simulation and investigation of spiral autowave stability. J. Nonlin. Biol. 1990, 1, 127–159. [Google Scholar]
- Girelli, F.; Liberati, S.; Sindoni, L. Is the notion of time really fundamental? Symmetry 2011, 3, 89–401. [Google Scholar] [CrossRef]
- Chandrashekaran, M.K.; Engelmann, W. Early and late subjective night phases of the Drosophila pseudoobscura circadian rhythm require different energies of blue light for phase shifting. Z. Naturforsch. 1973, 28, 750–753. [Google Scholar] [CrossRef] [PubMed]
- Chandrashekaran, M.K.; Loher, W. The relationship between the intensity of light pulses and the extent of phase shifts of the circadian rhythm in the exclusion rate of Drosophila pseudoobscura. J. Exp. Zool. 1969, 172, 147–152. [Google Scholar] [CrossRef] [PubMed]
- Kantelhardt, J.W. Fractal and multi-fractal time series. In Mathematics of Complexity and Dynamical Systems; Mayer, R.A., Ed.; Springer: New York, NY, USA, 2011; pp. 463–487. [Google Scholar]
- Shlesinger, M.F. Fractal time in condensed matter. Ann. Rev. Phys. Chem. 1988, 39, 269–290. [Google Scholar] [CrossRef]
- Agrawal, L.; Ghosh, S.; Ghosh, B.; Ray, K.; Sahu, S.; Fujita, D.; Bandyopadhyay, A. Replacing Turing tape with a Fractal tape: A new information theory, associated mechanics and decision making without computing. In Consciousness, 1st ed.; Integrating Indian and Western Perspective, New Age Books: Delhi, India, 2016; pp. 87–159. [Google Scholar]
- Edmu nds, L.N., Jr.; Adams, K.J. Clocked cell cycle clocks. Science 1981, 211, 1002–1013. [Google Scholar] [CrossRef]
- Ghosh, S.; Sahu, S.; Fujita, D.; Bandyopadhyay, A. Design and operation of a brain like computer: A new class of frequency-fractal computing using wireless communication in a supramolecular organic, inorganic systems. Information 2014, 5, 28–99. [Google Scholar] [CrossRef]
- Ghosh, S.; Dutta, M.; Sahu, S.; Fujita, D.; Bandyopadhyay, A. Nano Molecular-platform: A protocol to write energy transmission program inside a molecule for bio-inspired supramolecular engineering. Adv. Func. Mater. 2014, 24, 1364–1371. [Google Scholar] [CrossRef]
- Joshi, A.; Xiao, M. Cavity-QED based unconventional geometric phase dc gates with bichromatic field modes. Phys. Lett. A 2006, 359, 390–395. [Google Scholar] [CrossRef]
- Lauber, H.M.; Weidenhammer, P.; Dubbers, D. Geometric phases and hidden symmetries in simple resonators. Phys. Rev. Lett. 1994, 72, 1004–1007. [Google Scholar] [CrossRef]
- Lee, T.; Broderick, N.G.R.; Brambilla, G. Berry phase magnification in optical microcoil resonators. Opt. Lett. 2011, 36, 2839–2841. [Google Scholar] [CrossRef]
- Pistolesi, F.; Manini, N. Geometric Phases and Multiple Degeneracies in Harmonic Resonators. Phys. Rev. Lett. 2000, 85, 1585. [Google Scholar] [CrossRef]
- Komal, S.; Pushpendra, S.; Pathik, S.; Satyajit, S.; Subrata, G.; Kanad, R.; Daisuke, F.; Anirban, B. Fractal, scale free electromagnetic resonance of a single brain extracted microtubule nanowire, a single tubulin protein and a single neuron. Fractal Fract. 2020, 4, 11. [Google Scholar]
- Brinkmann, K. Metabolic control of temperature compensation in the circadian rhythm of Euglena gracilis. In Biochronometry; Menaker, M., Ed.; National Academy of Sciences: Washington, DC, USA, 1971; pp. 567–593. [Google Scholar]
- Singh, P.; Ray, K.; Fujita, D.; Bandyopadhyay, A. Complete Dielectric Resonator Model of Human Brain from MRI Data: A Journey from Connectome Neural Branching to Single Protein. In Engineering Vibration, Communication and Information Processing, 1st ed.; Ray, K., Sharan, S., Rawat, S., Jain, S., Srivastava, S., Bandyopadhyay, A., Eds.; Springer: Singapore, 2019; Volume 478, pp. 717–733. [Google Scholar]
- Shapere, A.; Wilczek, F. Classical time crystals. Phys. Rev. Lett. 2012, 109, 160402. [Google Scholar] [CrossRef] [PubMed]
- Agrawal, L.; Sahu, S.; Ghosh, S.; Shiga, T.; Fujita, D.; Bandyopadhyay, A. Inventing atomic resolution scanning dielectric microscopy to see a single protein complex operation live at resonance in a neuron without touching or adulterating the cell. J. Integr. Neurosci. 2016, 15, 435–462. [Google Scholar] [CrossRef] [PubMed]
- Ghosh, S.; Sahu, S.; Agrawal, L.; Shiga, T.; Bandyopadhyay, A. Inventing a co-axial atomic resolution patch clamp to study a single resonating protein complex and ultra-low power communication deep inside a living neuron cell. J. Integr. Neurosci. 2016, 15, 403–433. [Google Scholar] [CrossRef]
- Singh, P.; Sahu, P.; Sahu, S.; Ghosh, S.; Fujita, D.; Bandyopadhyay, A. A space-time-topology-prime, stTS metric for a self-operating mathematical universe uses Dodecanion geometric algebra of 2-20 D complex vectors. LNNS Springer 2020, in press. [Google Scholar]
- Singh, P.; Sahu, P.; Sahu, S.; Ghosh, S.; Fujita, D.; Bandyopadhyay, A. Quaternion, octonion to dodecanion manifold: Stereographic projections from infinity lead to a self-operating mathematical universe. AISC Springer 2020, in press. [Google Scholar]
- Wiltshire, M.C.K.; Hajnal, J.V.; Pendry, J.B.; Edwards, D.J.; Stevens, C.J. Metamaterial endoscope for magnetic field transfer: Near field imaging with magnetic wires. Opt. Express 2003, 11, 709–715. [Google Scholar] [CrossRef]
- Milosevic, M. On the Nature of the Evanescent Wave. Appl. Spectrosc. 2013, 26, 126–131. [Google Scholar] [CrossRef]
- Nye, J.F. Polarization effects in the diffraction of electromagnetic waves: The role of disclinations. Proc. R. Soc. Lond. 1983, 387, 105–132. [Google Scholar]
- Rozenfel’d, A.B. A History of Non-Euclidean Geometry: Evolution of the Concept of a Geometric Space; Springer: New York, NY, USA, 1988; p. 373. [Google Scholar]
- Dixon, G. On Quaternions and Octonions: Their Geometry, Arithmetic, and Symmetry. Math. Intell. 2004, 26, 75–77. [Google Scholar] [CrossRef]
- Brodmann, K. Vergleichende Lokalisationslehre der Grosshirnrinde. Leipzig: Johann Ambrosius Barth, 1909. In Wikipedia, the Free Encyclopedia. Available online: https://en.wikipedia.org/w/index.php?title=10%E2%80%9320_system_(EEG)&oldid=922279483 (accessed on 28 February 2020).
- Garey, L.J. Brodmann’s: Localisation in the Cerebral Cortex; Springer: New York, NY, USA, 2006. [Google Scholar]
- Bandyopadhyay, A. Fractal mechanics is not quantum but original: Geometric algebra for a dodecanion. In Nanobrain. The Making of an Artificial Brain from a Time Crystal, 1st ed.; Taylor & Francis Inc.: Bosa Roca, FL, USA, 2020; p. 372. [Google Scholar]
- Lugosi, E. Analysis of meandering in Zykov-kinetics. Physica D 1989, 40, 331–337. [Google Scholar] [CrossRef]
- Gerisch, G.; Hulser, D.; Malchow, D.; Wick, U. Cell communication by periodic cyclic-AMP pulses. Philos. Trans. R. Soc. Lond. 1975, 272, 181–192. [Google Scholar]
- Carter, R. The Human Brain Book: An Illustrated Guide to its Structure, Function, and Disorders; DK: London, UK, 2014; Available online: https://www.dk.com.
- Buzsáki, G. The Rythms of the Brain; Oxford University Press: London, UK, 2006. [Google Scholar] [CrossRef]
- Ghosh, S.; Fujita, D.; Bandyopadhyay, A. Universal Geometric-Musical Language for Big Data Processing in an Assembly of Clocking Resonators. International Patent WO 2019/026983A1, 7 Ferbruary 2019. [Google Scholar]
- Aschoff, J.; Wever, R. Human circadian rhythms: A multioscillatory system. Fed. Proc. 1976, 35, 2326–2332. [Google Scholar]
- Pippinger, N.; Fischer, M.J. Relations among Complexity Measures. J. ACM 1979, 26, 361–381. [Google Scholar] [CrossRef]
- Gurevich, Y.; Shelah, S. Nearly linear time. Lect. Notes Comput. Sci. 1989, 363, 108–118. [Google Scholar]
- Malinowski, J.R.; Laval-Martin, D.L.; Edmunds, L.N., Jr. Circadian oscillators, cell cycles, and singularities: Light perturbations of the free-running rhythm of cell division in Euglena. J. Comp. Physiol. 1985, 155, 257–276. [Google Scholar] [CrossRef]
- Winfree, A.T. Biological rhythrns and the behavior of populations of coupled oscillators. J. Theor. Biol. 1967, 16, 15–42. [Google Scholar] [CrossRef]
- Collins, B. Visualization: From Biology to Culture. In Bridges: Mathematical Connections in Art, Music, and Science; Sarhangi, R., Ed.; Brent Collins: Gower, MO, USA, 2000; pp. 307–314. [Google Scholar]
- Leopold, L.B.; Langbein, W.B. River Meanders. Sci. Am. 1966, 214, 60–73. Available online: https://eps.berkeley.edu/people/lunaleopold/(098)%20River%20Meanders%20-%20Sci%20Amer.pdf. [CrossRef]
- Frank, K.D.; Zimmerman, W.F. Action spectra for phase shifts of a circadian rhythm in Drosophila. Science 1969, 163, 688–689. [Google Scholar] [CrossRef]
- Guckenheimer, J. Isochrons and phaseless sets. J. Math. Biol. 1975, 1, 259–273. [Google Scholar] [CrossRef]
- Sahu, S.; Fujita, D.; Bandyopadhyay, A. An Inductor Made of Arrayed Capacitors. U.S. Patent No. 9019685B2, 28 April 2015. Available online: https://patents.google.com/patent/EP2562776A1/de (accessed on 31 July 2019).
- Michalareas, G.; Vezoli, J.; Pelt, S.v.; Schoffelen, J.M.; Kennedy, H.; Fries, P. Alpha-Beta and Gamma Rhythms Subserve Feedback and Feedforward Influences among Human Visual Cortical Areas. Neuron 2016, 89, 384–397. [Google Scholar] [CrossRef] [PubMed]
- Victor, J.D. Temporal aspects of neuronal coding in the retina and lateral geniculate. Netw. Comput. Neural Syst. 1999, 10, 1–66. [Google Scholar] [CrossRef]
- Moller, A. Auditory Neurophysiology. J. Clin. Neurophysiol. 1994, 11, 284–308. [Google Scholar] [CrossRef] [PubMed]
- MacLeod, K.; Laurent, G. Distinct mechanisms for synchronization and temporal patterning of odor-encoding neural assemblies. Science 1996, 274, 976–979. [Google Scholar] [CrossRef] [PubMed]
- Rose, J.E.; Mountcastle, V. Touch and kinesis. In Hand-Book of Physiology: Neurophysiology; American Physiological Society: Washington, DC, USA, 1959; pp. 387–429. [Google Scholar]
- Khamis, H.; Birznieks, I.; Redmond, S.J. Decoding tactile afferent activity to obtain an estimate of instantaneous force and torque applied to the fingerpad. J. Neurophysiol. 2015, 114, 474–484. [Google Scholar] [CrossRef] [PubMed]
- Keidel, W. The sensory detection of vibrations. In Foundations of Sensory Science; Dawson, W.W., Enoch, J.M., Eds.; Springer: Berlin, Germany, 1984; pp. 465–512. [Google Scholar]
- Arvanitaki, A.; Chalazonitis, N. Electrical properties and temporal organization in oscillatory neurons. In Neurobiology of Invertebrates; Salanki, J., Ed.; Plenum: New York, NY, USA, 1968; pp. 169–174. [Google Scholar]
- Berliner, M.D.; Neurath, P.W. The rhythms of three clock mutants of Ascobolusimmersus. Mycologia 1965, 57, 809–817. [Google Scholar] [CrossRef]
- Sahu, S.; Ghosh, S.; Hirata, K.; Fujita, D.; Bandyopadhyay, A. Multi-level memory-switching properties of a single brain microtubule. Appl. Phys. Lett. 2013, 102, 123701. [Google Scholar] [CrossRef]
- Lam, C.W. Prime Indel: Four-prime-number genetic code for indel decryption and sequence read alignment. Clin. Chim. Acta 2014, 436, 1–4. [Google Scholar] [CrossRef]
- Yan, J.F.; Yan, A.K.; Yan, B.C. Prime numbers and the amino acid code: Analogy in coding properties. J. Theor. Biol. 1991, 151, 333–341. [Google Scholar] [CrossRef]
- Volgyi, B.; Chheda, S.; Bloomfield, S.A. Tracer coupling patterns of the ganglion cell subtypes in the mouse retina. J. Comp. Neurol. 2009, 512, 664–687. [Google Scholar] [CrossRef]
- Kolb, H.; Nelson, R. Amacrine cells of the cat retina. Vis. Res. 1981, 21, 1625–1633. [Google Scholar] [CrossRef]
- Kolb, H.; Nelson, R. Three amacrine cells of the cat retina: Morphology and intraeellular responses. Investig. Ophthal. Vis. Sci. 1981, 20, 184. [Google Scholar]
- Kolb, H.; Linberg, K.A.; Fisher, S.K. The neurons of the human retina: A Golgi study. J. Comp. Neurol. 1992, 318, 147–187. [Google Scholar] [CrossRef] [PubMed]
- Markram, H.; Muller, E.; Ramaswamy, S.; Reimann, M.W.; Abdellah, M.; Sanchez, C.A.; Ailamaki, A.; Alonso-Nanclares, L.; Antille, N.; Arsever, S.; et al. Reconstruction and Simulation of Neocortical Microcircuitry. Cell 2015, 163, 456–492. [Google Scholar] [CrossRef] [PubMed]
- Horton, J.C.; Adams, D.L. The cortical column: A structure without a function. Philos. Trans. R. Soc. Lond. B Biol. Sci. 2005, 360, 837–862. [Google Scholar] [CrossRef] [PubMed]
- Buzsáki, G.; Bragin, A.; Chrobak, J.J.; Nádasdy, Z.; Sík, A.; Ylinen, A. Oscillatory and intermittent synchrony in the hippocampus: Relevance for memory trace formation. In Temporal Coding in the Brain; Buzsàki, G., Llinás, R.R., Singer, W., Berthoz, A., Christen, Y., Eds.; Springer: Berlin, Germany, 1994; pp. 145–172. [Google Scholar]
- Stanley, S.A.; Latcha, K.N.; Schmidt, S.F.; Yu, X.; Nectow, A.R.; Sauer, J.; Dyke, J.P.; Dordick, J.S.; Friedman, J.M. Bidirectional electromagnetic control of the hypothalamus regulates feeding and metabolism. Nature 2016, 531, 647–650. [Google Scholar] [CrossRef] [PubMed]
- Steriade, M.; Deschenes, M. The thalamus as a neuronal oscillator. Brain Res. Rev. 1984, 8, 1–63. [Google Scholar] [CrossRef]
- Jalife, J.; Antzelevitch, C. Phase resetting and annihilation of pacemaker activity in cardiac tissues. Science 1979, 206, 695–697. [Google Scholar] [CrossRef]
- Zeki, S.; Shipp, S. The functional logic of cortical connections. Nature 1988, 335, 311–317. [Google Scholar] [CrossRef]
- Schahmann, J.D.; Pandya, D.N. Fiber Pathways of the Brain; Oxford University Press: Oxford, UK, 2006. [Google Scholar]
- Mackinnon, S.E.; Yee, A.; Ray, W.Z. Nerve transfers for the restoration of hand function after spinal cord injury. J. Neurosurg. 2012, 117, 176–185. [Google Scholar] [CrossRef]
- Nieuwland, M.S.; Ahles, S.P.; Heyselaar, E.; Segaert, K.; Darley, E.; Kazanina, N.; Wolfsthurn, S.V.G.H.; Bartolozzi, F.; Kogan, V.; Ito, A.; et al. Large-scale replication study reveals a limit on probabilistic prediction in language comprehension. eLife 2018, 7, e33468. [Google Scholar] [CrossRef] [PubMed]
- Sporns, O.; Tononi, G.; Kotter, R. The human connectome: A structural description of the human brain. PLoS Comput. Biol. 2005, 1, 245–251. [Google Scholar] [CrossRef]
- Hagmann, P. From Diffusion MRI to Brain Connectomeics. Ph.D. Thesis, Ecole Polytechnique Federale de Lausanne, Lausanne, Switzerland, 2005. [Google Scholar]
- Abbott, L.F. Lapique’s introduction of the integrate-and-fire model neuron. Brain Res. Bull. 1999, 50, 303–304. [Google Scholar] [CrossRef]
- Reynolds, J.H.; Desimone, R. The role of neural mechanisms of attention in solving the binding problem. Neuron 1999, 24, 19–29. [Google Scholar] [CrossRef]
- Xu, K.; Zhong, G.; Zhuang, X. Actin, spectrin and associated proteins form a periodic cytoskeleton structure in axons. Science 2013, 339, 452–456. [Google Scholar] [CrossRef]
- Sahu, S.; Ghosh, S.; Ghosh, B.; Aswani, K.; Hirata, K.; Fujita, D.; Bandyopadhyay, A. Atomic water channel controlling remarkable properties of a single brain microtubule: Correlating single protein to its supramolecular assembly. Biosens. Bioelectron. 2013, 47, 141–148. [Google Scholar] [CrossRef]
- Sahu, S.; Ghosh, S.; Fujita, D.; Bandyopadhyay, A. Live visualizations of single isolated tubulin protein self-assembly via tunneling current: Effect of elctromagnetic pumping during spontaneous growth of microtubule. Sci. Rep. 2014, 4, 7303. [Google Scholar] [CrossRef]
- Glass, L. Synchronization and rhythmic processes in physiology. Nature 2001, 410, 277–284. [Google Scholar] [CrossRef]
- 10–20 System (EEG). Available online: https://en.wikipedia.org/wiki/10%E2%80%9320_system_(EEG) (accessed on 21 October 2019).
- Electroencephalography. Available online: https://en.wikipedia.org/wiki/Electroencephalography (accessed on 14 April 2020).
- Bogdan, P.A.; Rowley, A.G.D.; Rhodes, O.; Furber, S.B. Structural Plasticity on the SpiNNaker Many-Core Neuromorphic System. Front. Neurosci. 2018, 12, 434. [Google Scholar] [CrossRef]
- Yamins, D.L.K.; DiCarlo, J.J. Using goal-driven deep learning models to understand sensory cortex. Nat. Neurosci. 2016, 19, 356–365. [Google Scholar] [CrossRef]
- Leron, B.; Duminda, D.; Duff, M.J.; Hajar, E.; Williams, R. Black holes, qubits and octonions. Phys. Rep. 2009, 471, 113–219. [Google Scholar]
- Buzsáki, G.; Chrobak, J.J. Temporal structure in spatially organized neuronal ensembles: A role for interneuronal networks. Curr. Opin. Neurobiol. 1995, 5, 504–510. [Google Scholar] [CrossRef]
- Hamber, H.W. Quantum Gravitation—The Feynman Path Integral Approach; Springer: New York, NY, USA, 2009; ISBN 978-3-540-85292-6. [Google Scholar]
- Penrose, R. Angular momentum: An approach to combinatorial spacetime. In Quantum Theory and Beyond; Bastin, T., Ed.; Cambridge University Press: Cambridge, UK, 1971. [Google Scholar]
- Penrose, R. Applications of negative dimensional tensors. In Combinatorial Mathematics and Its Application; Welsh, D.J.A., Ed.; Proceeding Conference: Oxford, UK, 1969; pp. 221–244. [Google Scholar]
- Oeckl, R. Generalized lattice gauge theory, spin foams and state sum invariants. J. Geom. Phys. 2003, 46, 308–354. [Google Scholar] [CrossRef]
- Reimann, M.W.; Nolte, M.; Scolamiero, M.; Turner, K.; Perin, R.; Chindemi, G.; Dlotko, P.; Levi, R.; Hess, K.; Markram, H. Cliques of Neurons Bound into Cavities Provide a Missing Link between Structure and Function. Front. Comput. Neurosci. 2017, 11, 48. [Google Scholar] [CrossRef] [PubMed]





© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Singh, P.; Saxena, K.; Singhania, A.; Sahoo, P.; Ghosh, S.; Chhajed, R.; Ray, K.; Fujita, D.; Bandyopadhyay, A. A Self-Operating Time Crystal Model of the Human Brain: Can We Replace Entire Brain Hardware with a 3D Fractal Architecture of Clocks Alone? Information 2020, 11, 238. https://doi.org/10.3390/info11050238
Singh P, Saxena K, Singhania A, Sahoo P, Ghosh S, Chhajed R, Ray K, Fujita D, Bandyopadhyay A. A Self-Operating Time Crystal Model of the Human Brain: Can We Replace Entire Brain Hardware with a 3D Fractal Architecture of Clocks Alone? Information. 2020; 11(5):238. https://doi.org/10.3390/info11050238
Chicago/Turabian StyleSingh, Pushpendra, Komal Saxena, Anup Singhania, Pathik Sahoo, Subrata Ghosh, Rutuja Chhajed, Kanad Ray, Daisuke Fujita, and Anirban Bandyopadhyay. 2020. "A Self-Operating Time Crystal Model of the Human Brain: Can We Replace Entire Brain Hardware with a 3D Fractal Architecture of Clocks Alone?" Information 11, no. 5: 238. https://doi.org/10.3390/info11050238
APA StyleSingh, P., Saxena, K., Singhania, A., Sahoo, P., Ghosh, S., Chhajed, R., Ray, K., Fujita, D., & Bandyopadhyay, A. (2020). A Self-Operating Time Crystal Model of the Human Brain: Can We Replace Entire Brain Hardware with a 3D Fractal Architecture of Clocks Alone? Information, 11(5), 238. https://doi.org/10.3390/info11050238









