Coupled Response of Flexible Multi-Buoy Offshore Floating Photovoltaic Array Under Waves and Currents
Abstract
1. Introduction
2. Geometry and Environment
2.1. Geometrics of Offshore FPV Buoy
2.1.1. Main Dimensions
2.1.2. Connector
2.1.3. Mooring of Offshore FPV
2.2. Environment Parameters
2.2.1. Wave Parameters
2.2.2. Current Parameters
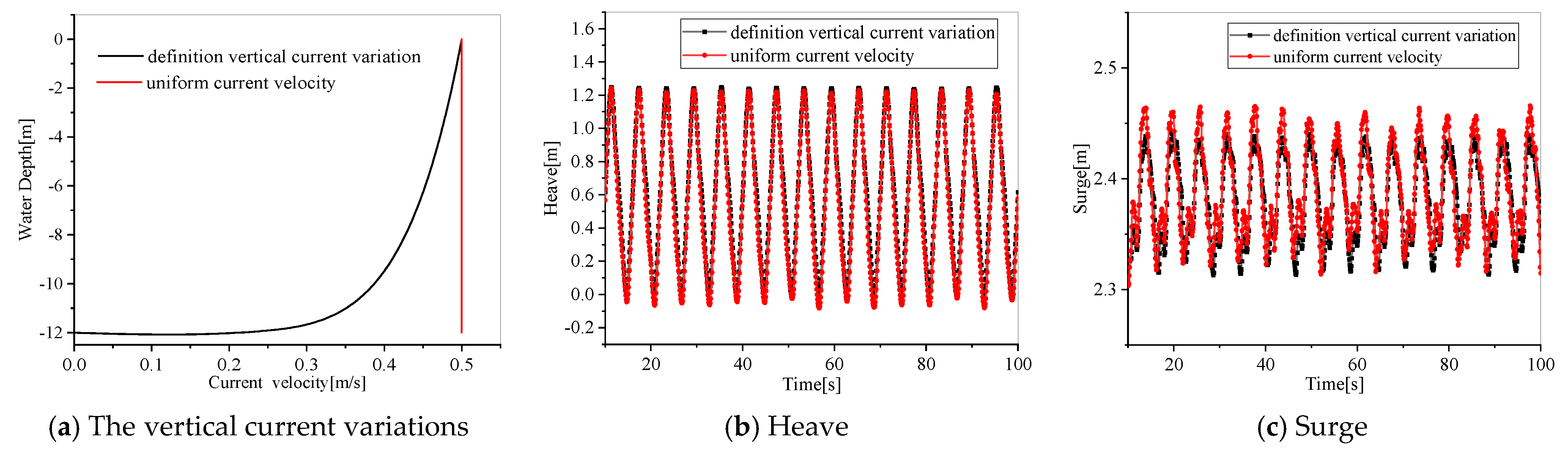
2.2.3. Wave–Current Coupling
2.2.4. Wind Parameters
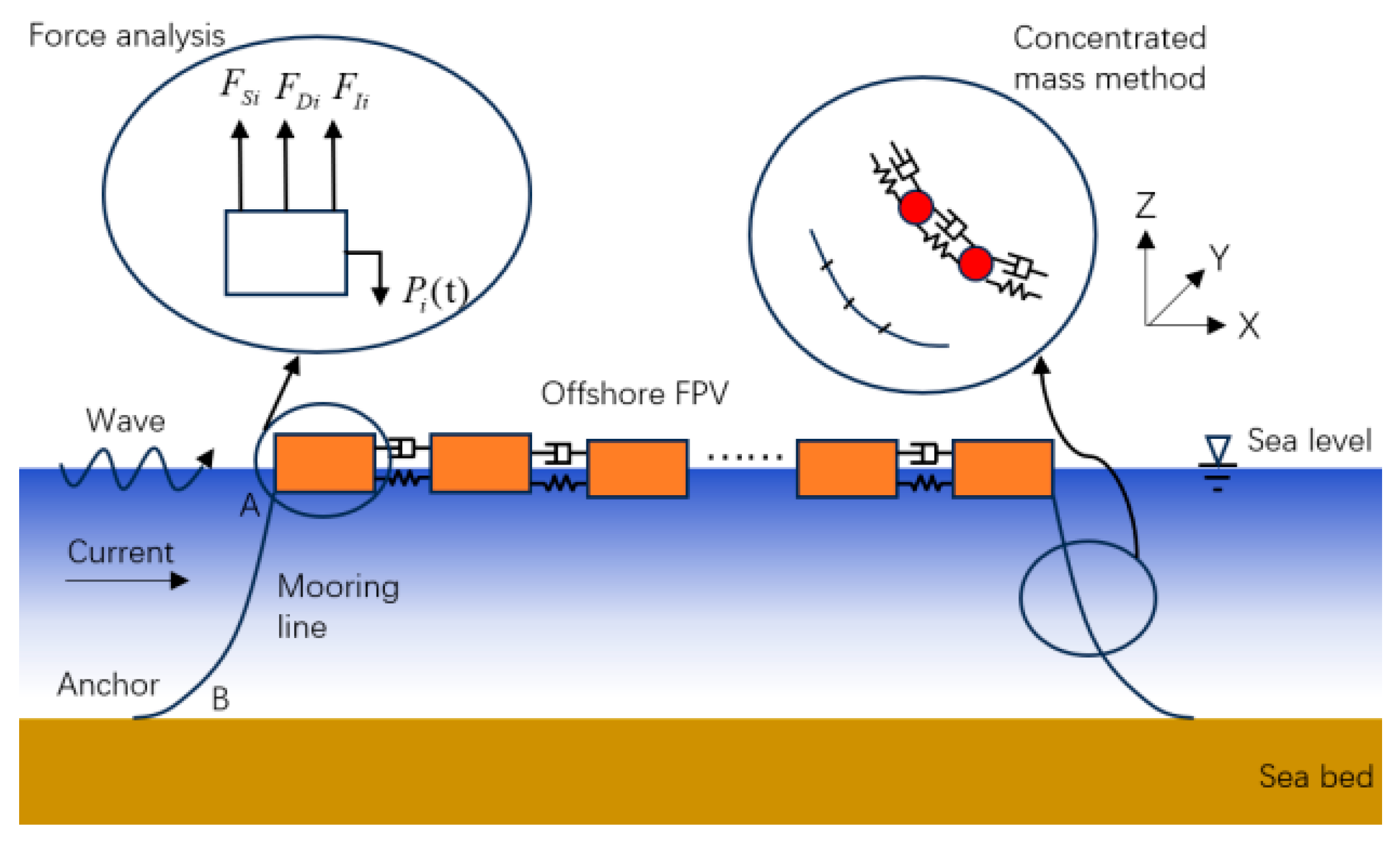
3. Computation and Simulation
3.1. Model Establishment
3.2. Theory of Hydrodynamic Loads in Orcaflex
3.3. Mechanical Analysis of Multi-Buoy Array Based on Multi-Body Dynamics
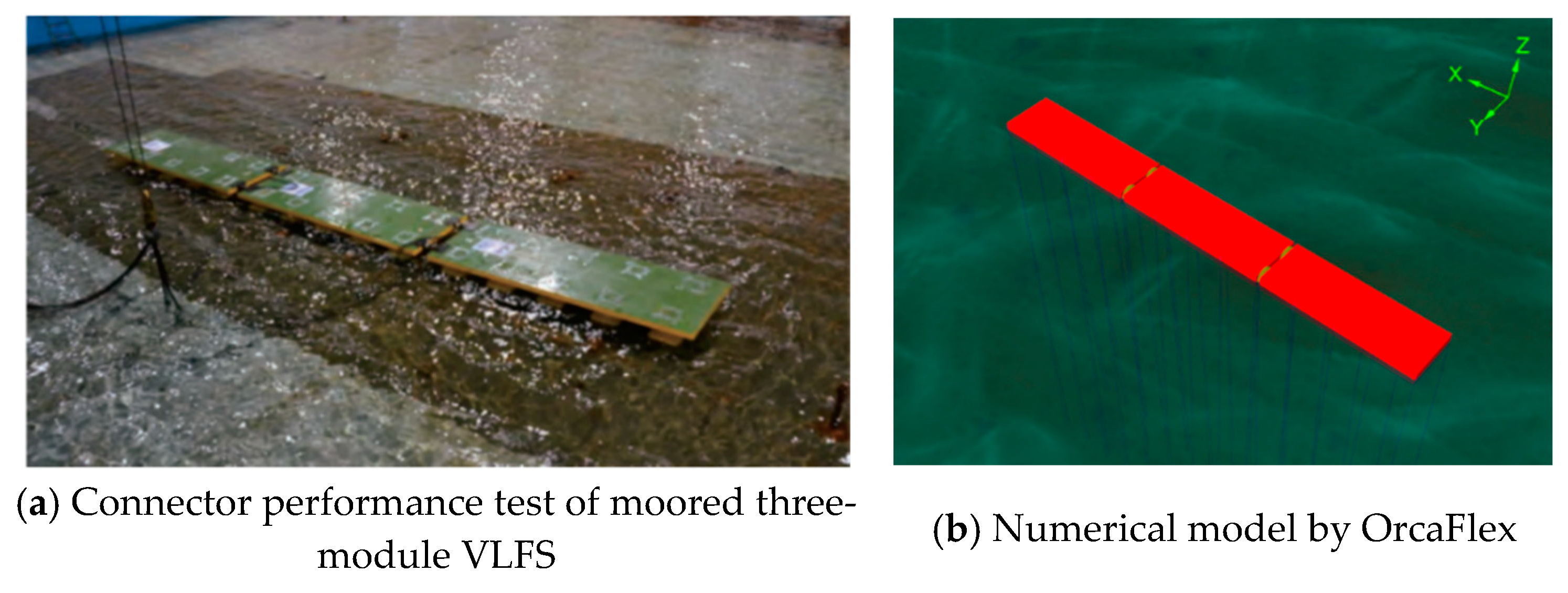
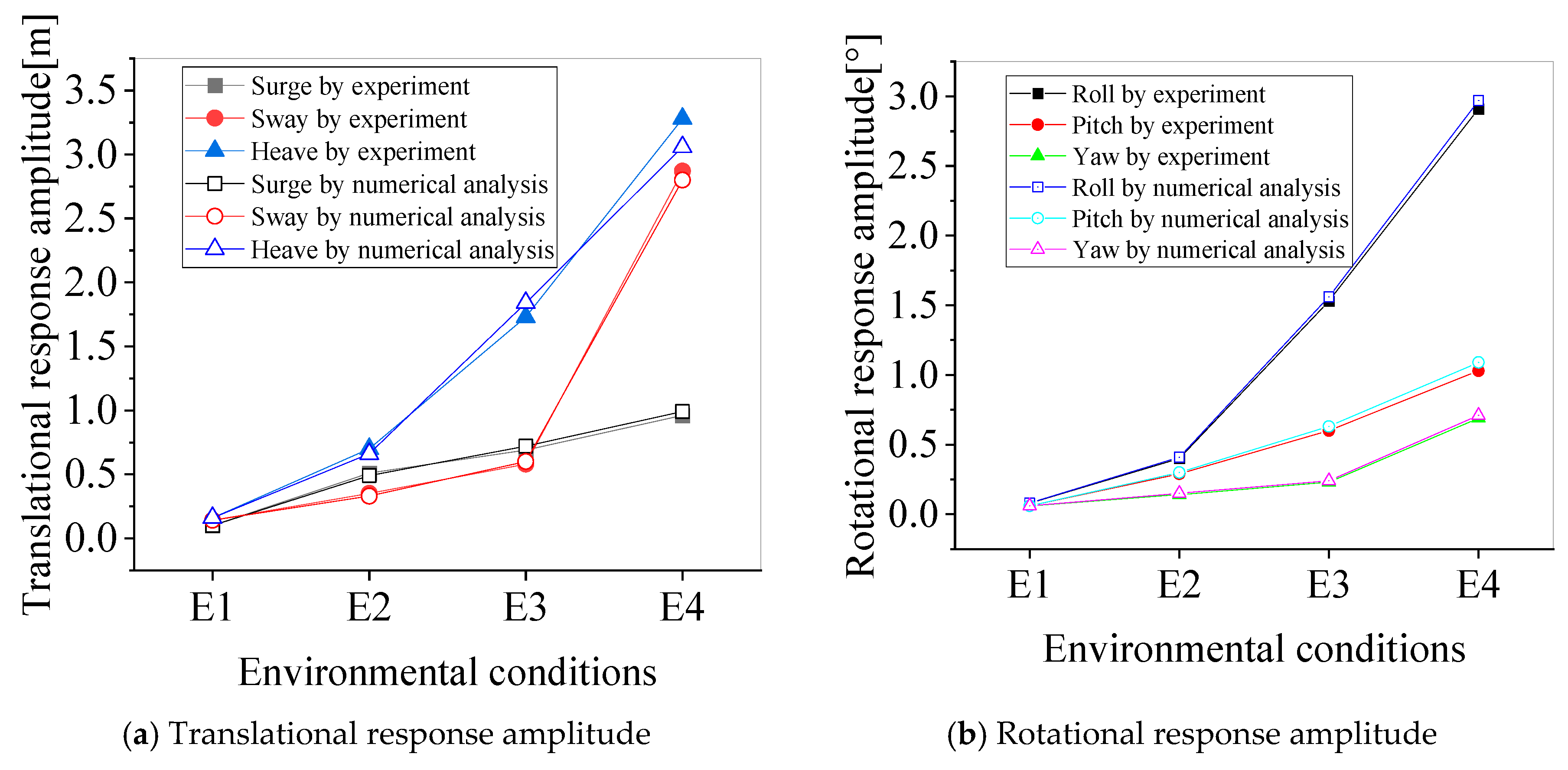
3.4. Validation of Numerical Model
4. Result Analysis
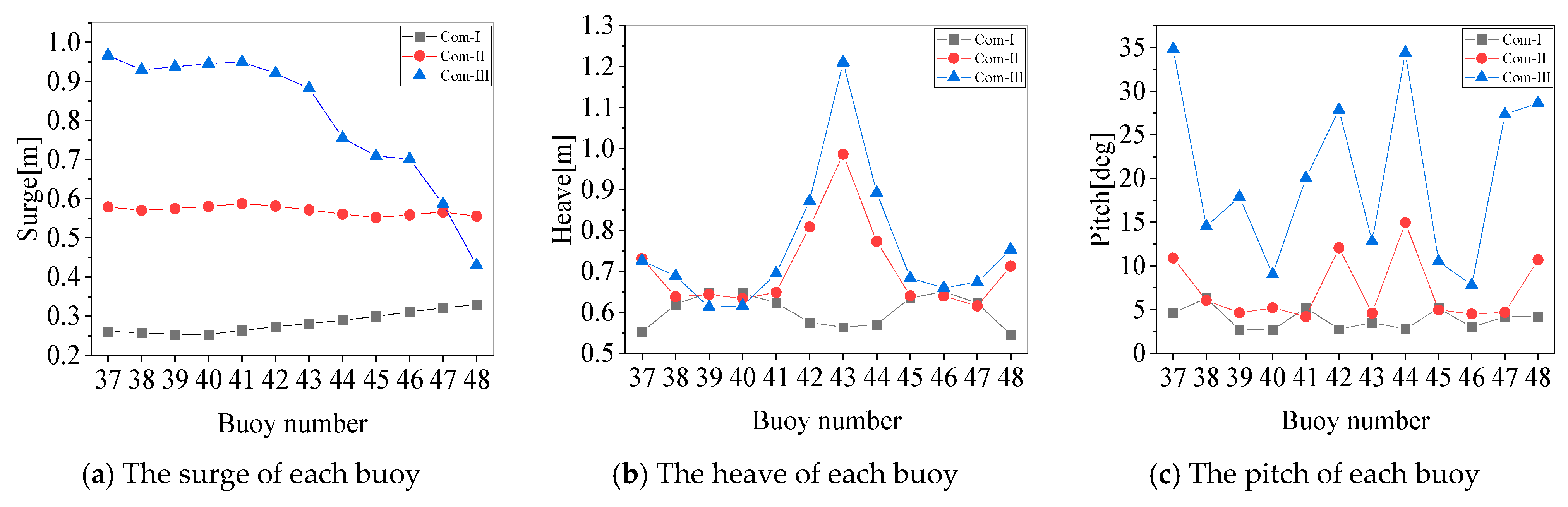
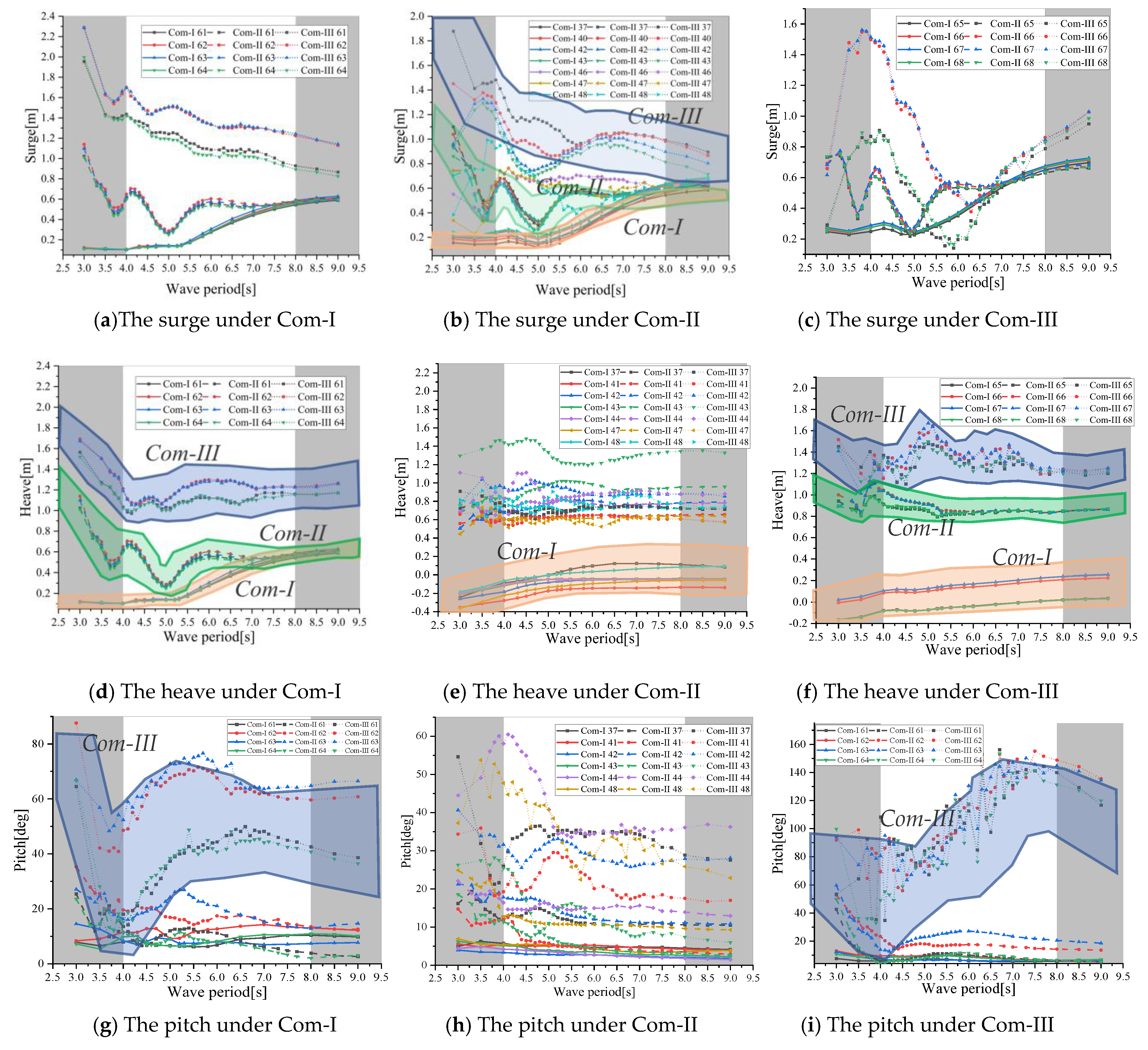
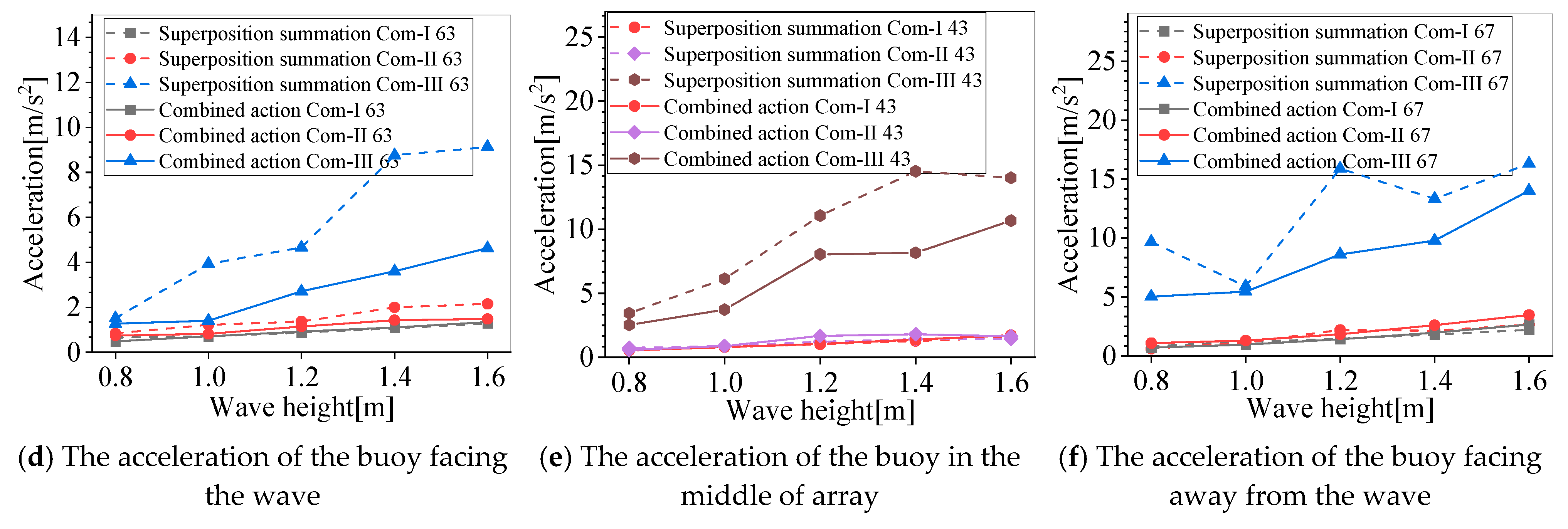
4.1. Buoy’s Motion and Acceleration
4.1.1. Wave-Induced Response
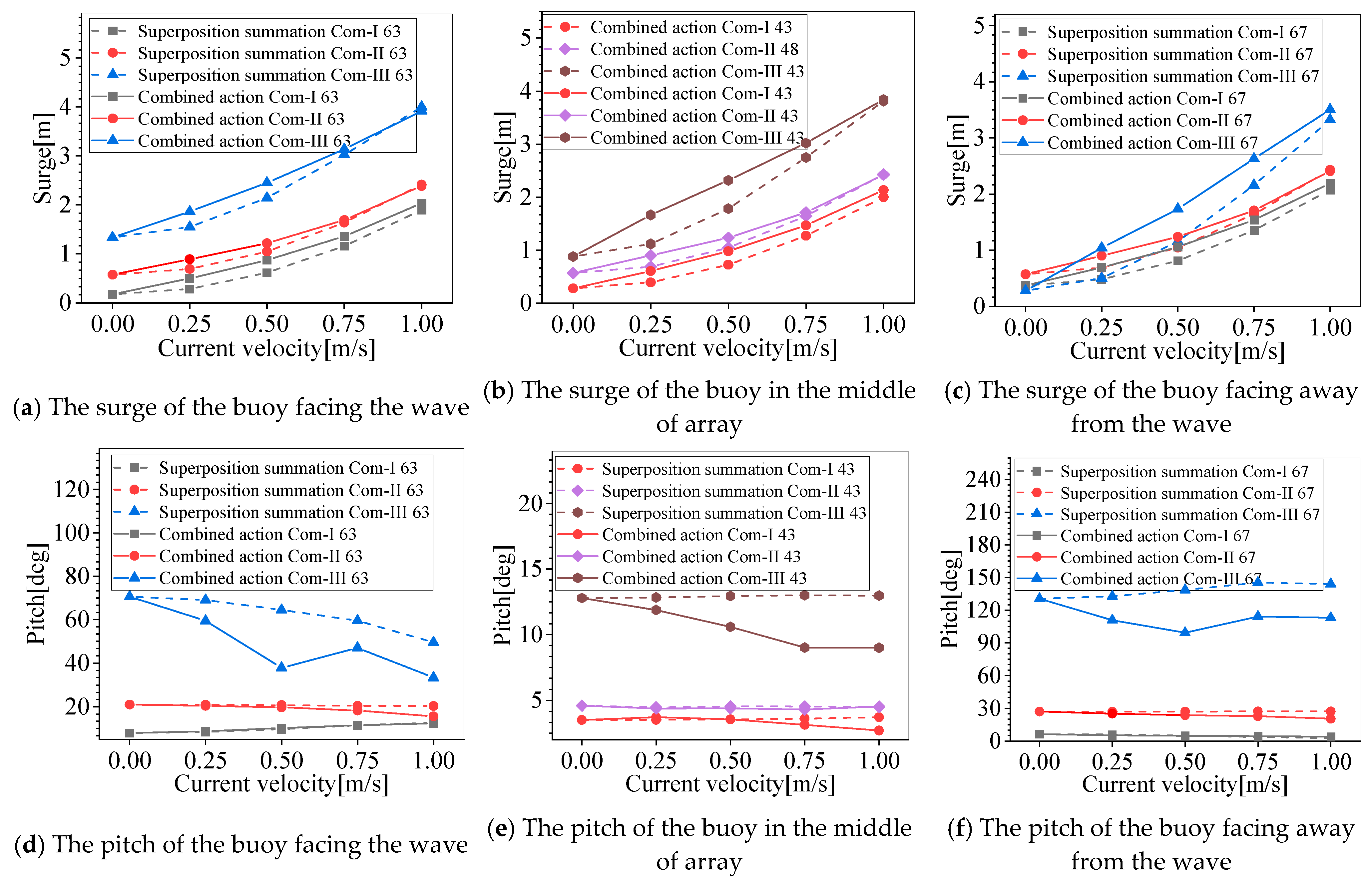
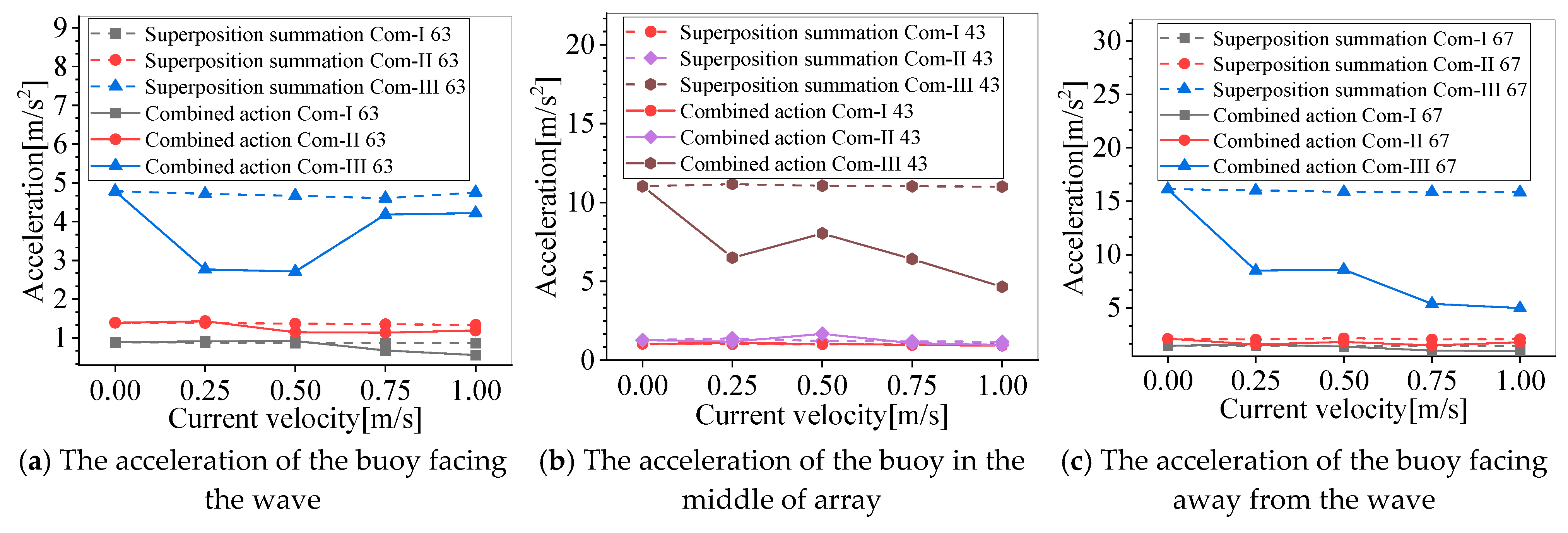
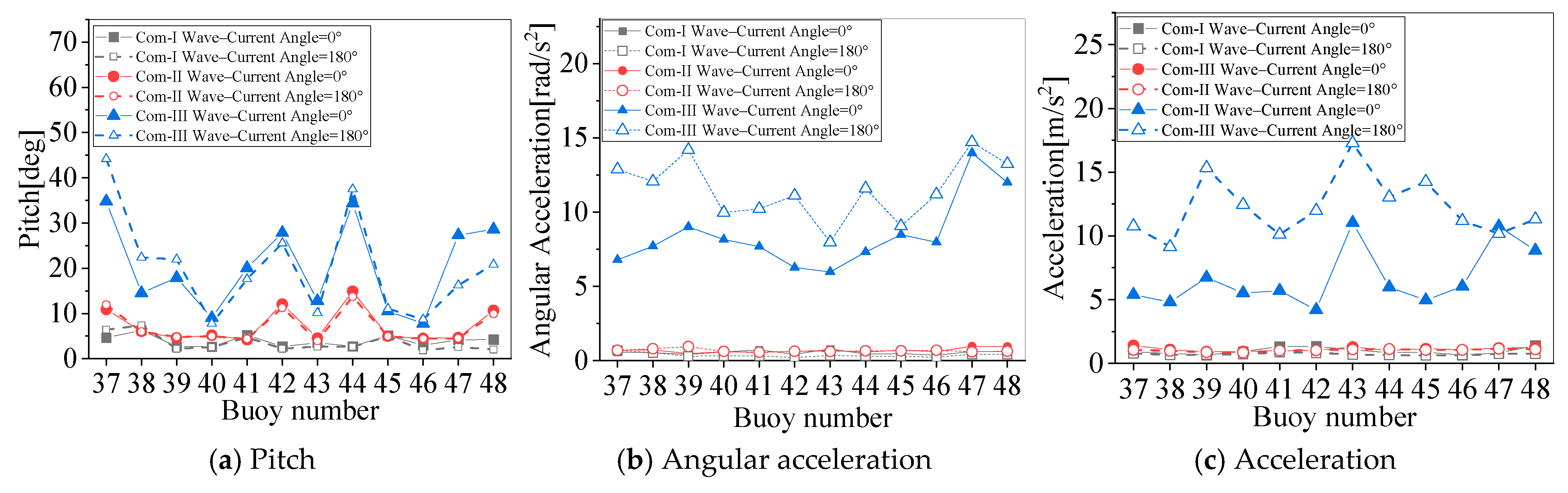
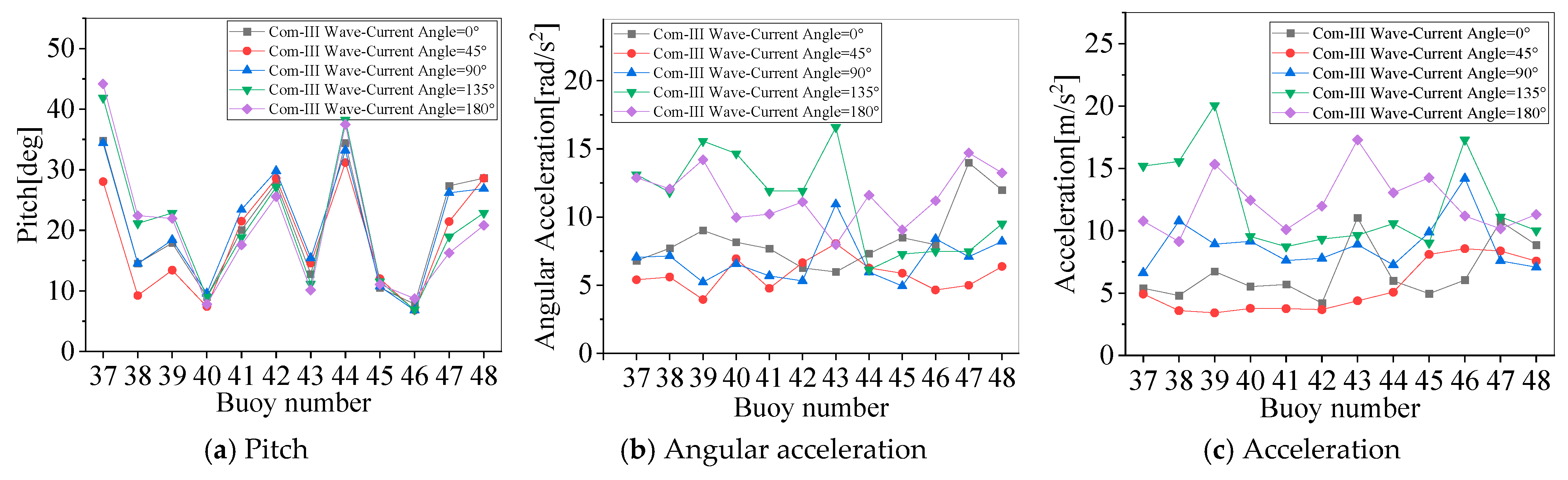
4.1.2. Coupled Response of Buoys Under Wave–Current
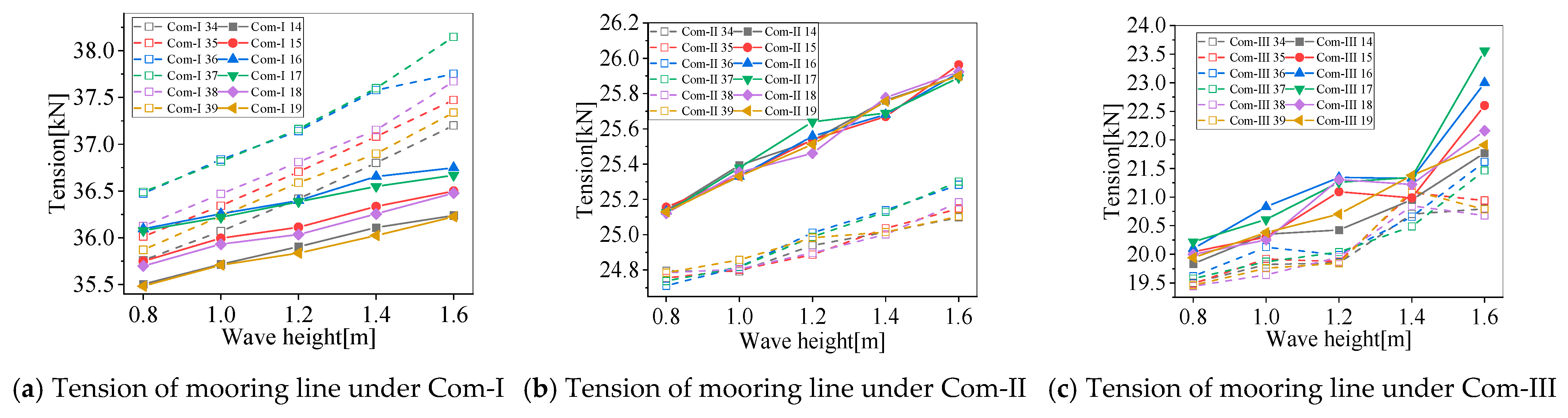
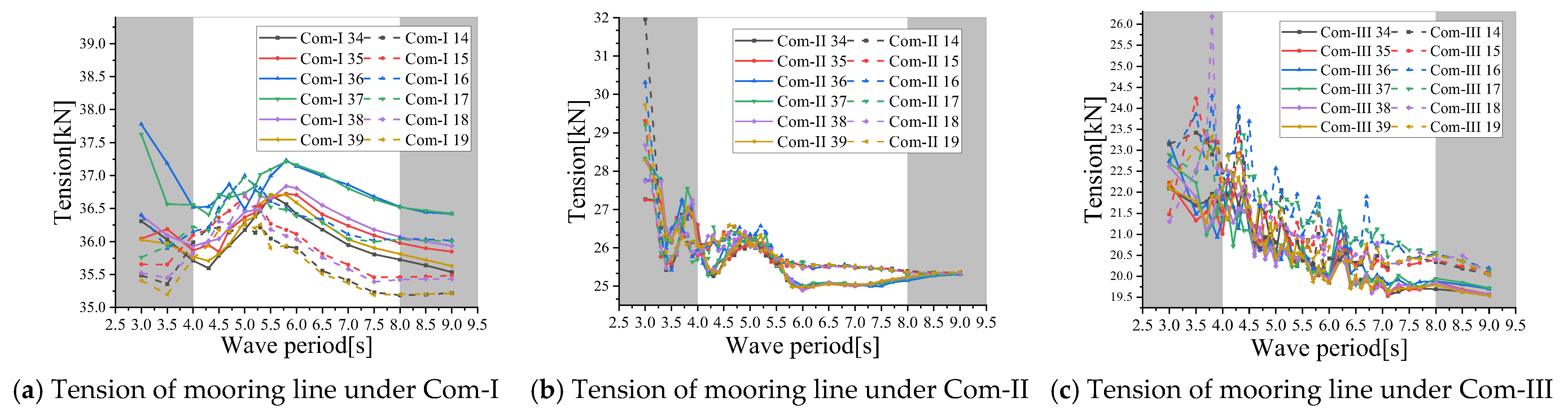
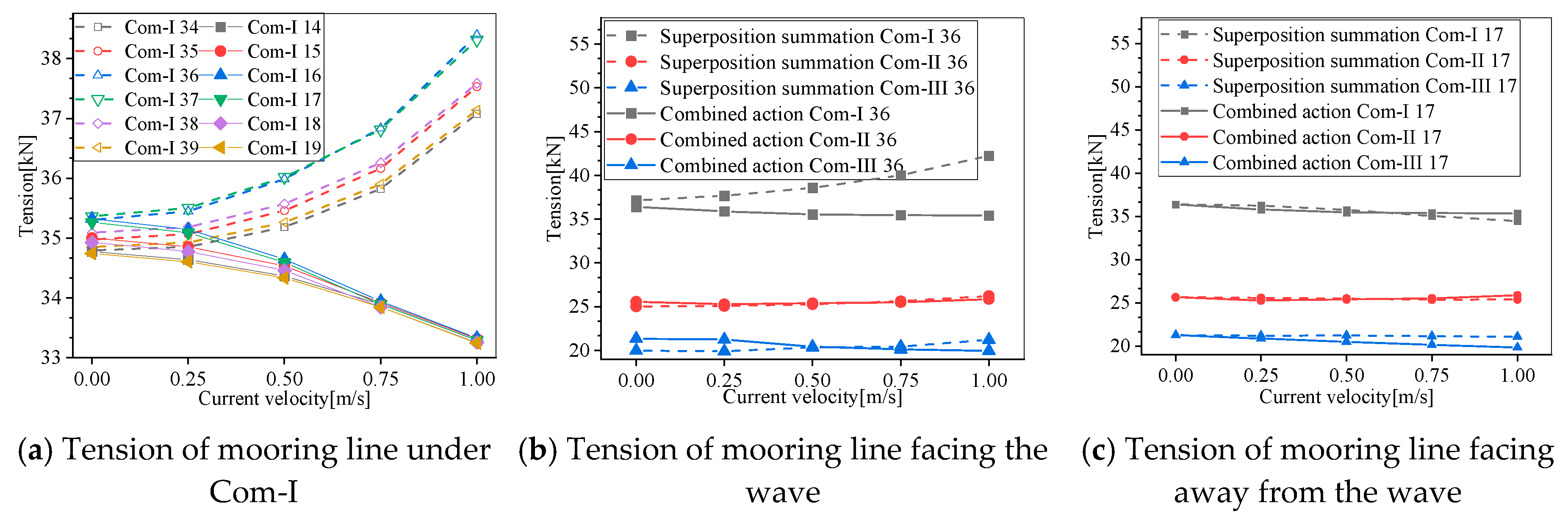
4.2. Coupled Mooring Tension
4.3. Connector Tension
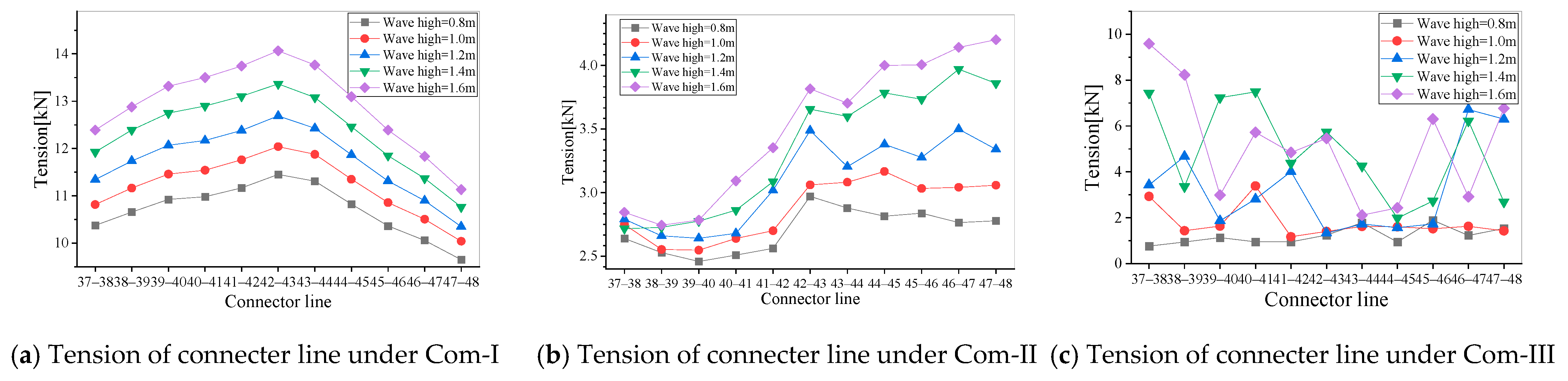
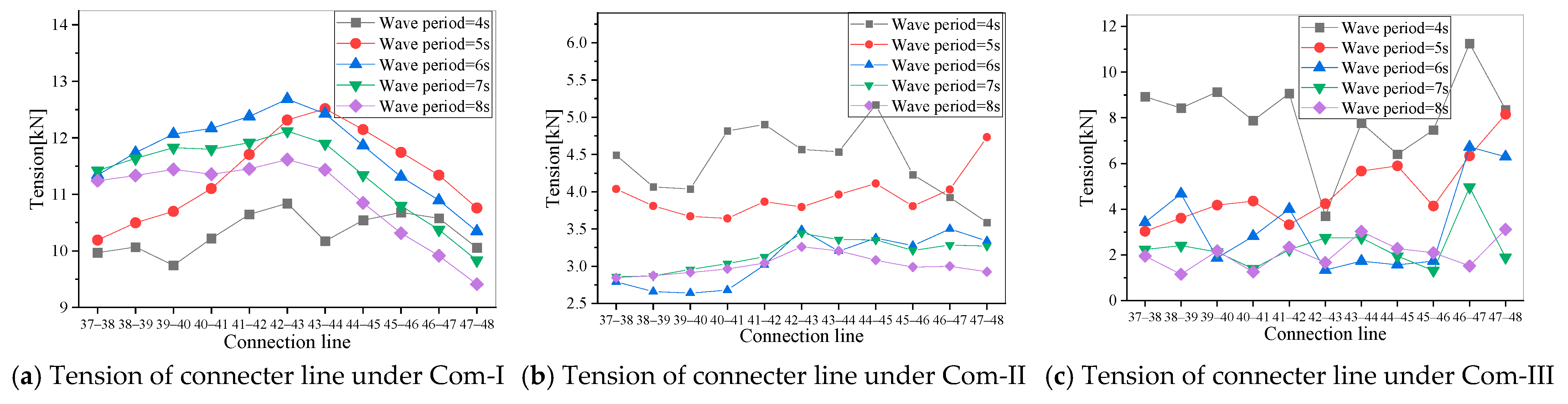
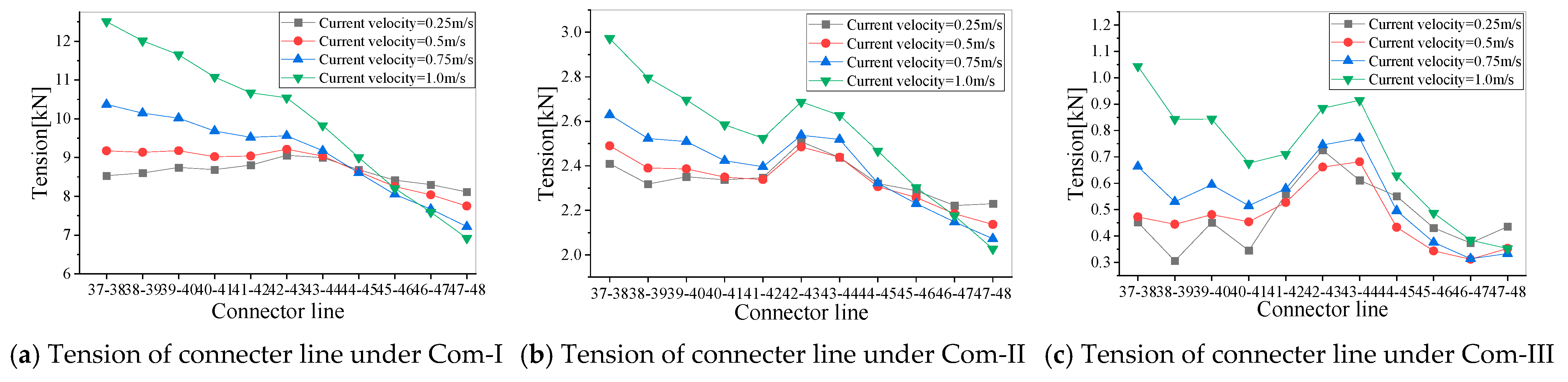
4.3.1. The Effect of Waves on the Longitudinal Connectors’ Tension
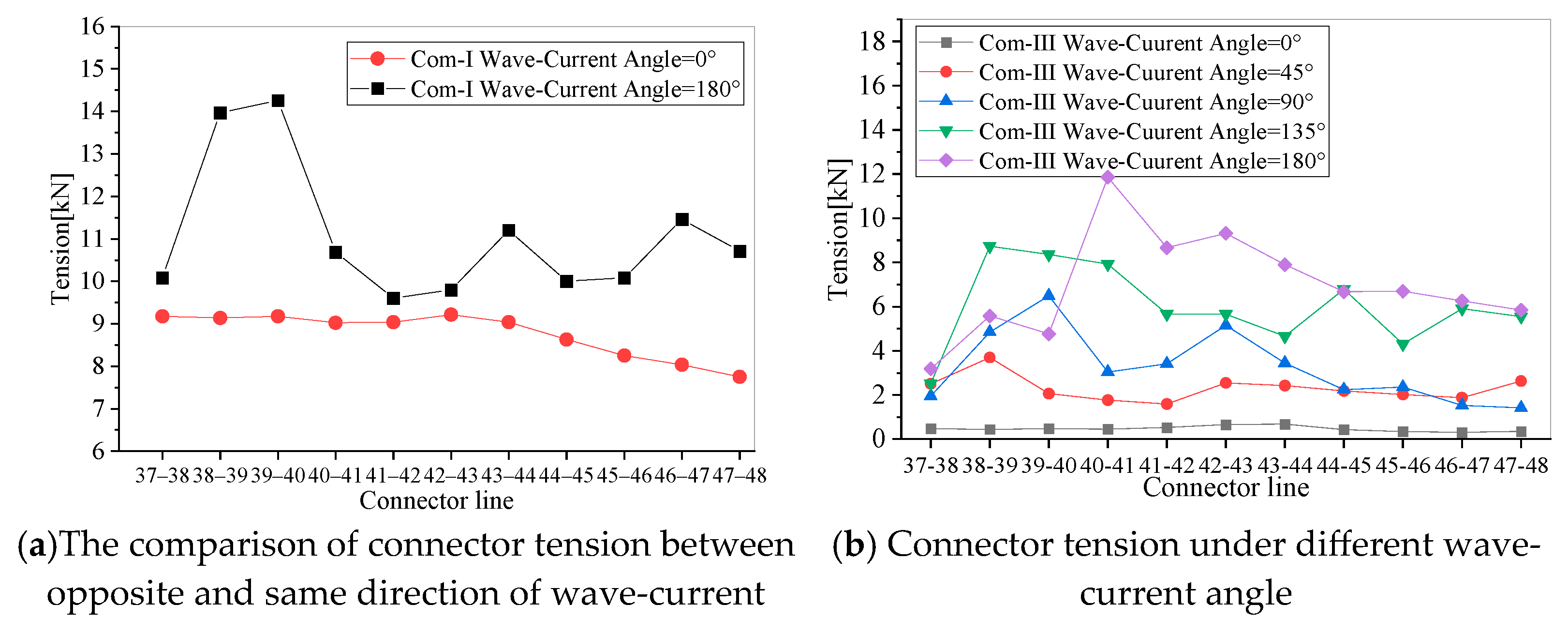
4.3.2. Coupled Effect
5. Conclusions
- (1)
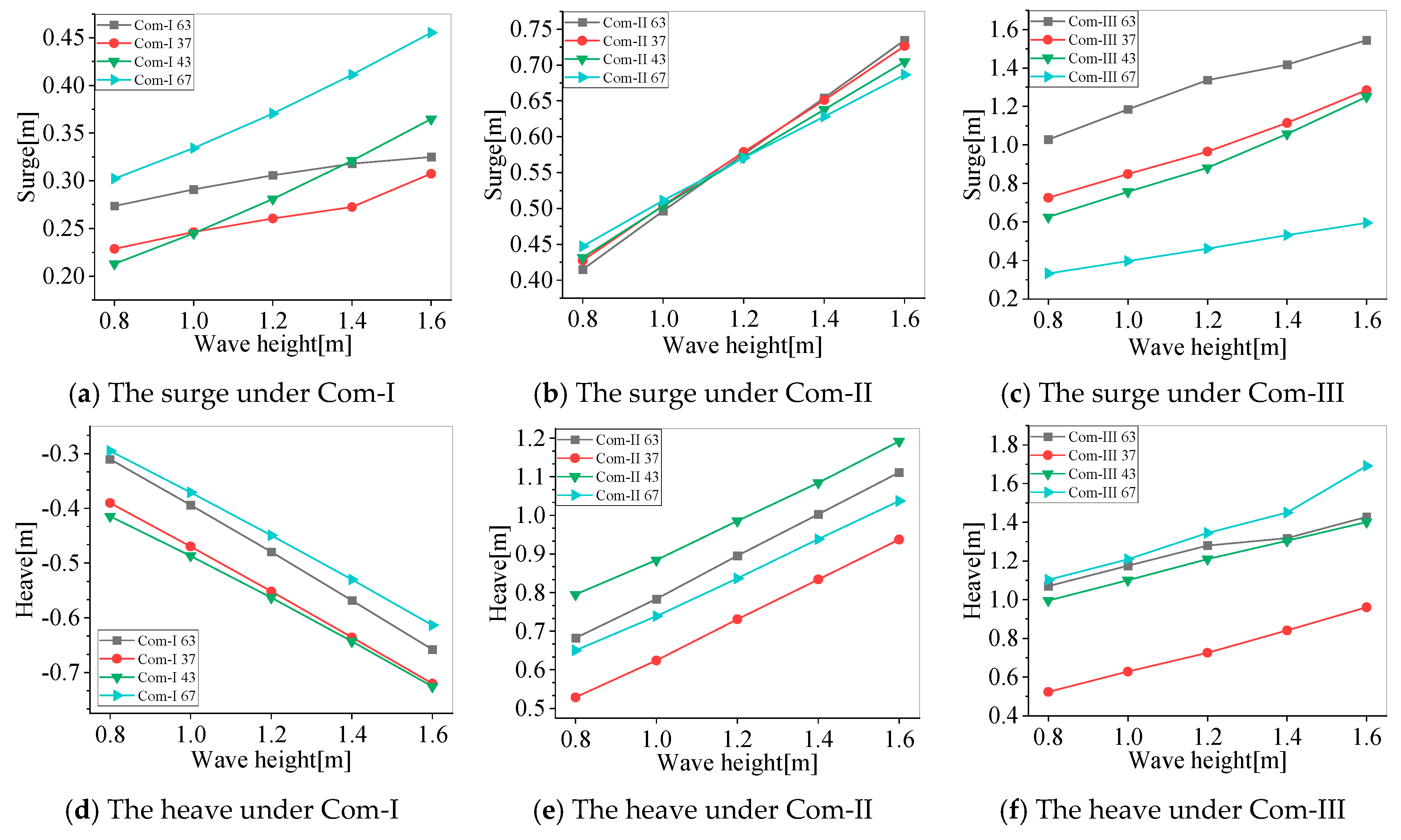
- When responding only to wave excitation, the motion response, including the surge, heave, and pitch, of the buoys in the FPV oscillates severely and increases with the wave height. The effects of the mooring static tension on the hydrodynamic characteristics are obvious for the multiple buoys connected to each other, especially for the buoys facing away from the wave. The maximum mooring tension occurs on moorings facing the wave, as in the array under Com-I. Under Com-III, the tension of the moorings behind the wave is larger than the ones facing the wave. This is the difference between different mooring tensions, which causes the difference in the response of the buoys facing away from the wave under different mooring tensions.
- (2)
- The coupled effect of the wave–current is significant for the floating multi-buoy array. Under the combination action, the motions and accelerations of the buoys facing the actions are decreased as the moorings facing the wave and current are tightened, as the wave and current are in the same direction. Meanwhile, the motions of the buoys facing away from the wave will be violent as they are relaxed.
- (3)
- With the increase in the wave–current angle, the constrained effect of the current on the moorings and connectors is weakened. This results in the increase in the motions, acceleration, and connection force of the buoys facing away from the current and facing the wave, as the pitch response increased by 9.36°, the acceleration by 5.39 m/s2, and the connection by 11.40 kN under the wave and current in the opposite direction (wave–current angle = 180°).
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Baolei, G.; Xu, T.; Ruijia, J.I. Research prospects for offshore floating photovoltaic structures and their hydrodynamic problems. Ocean. Eng. 2024, 42, 190–208. [Google Scholar] [CrossRef]
- Temiz, M.; Dincer, I. Techno-economic analysis of green hydrogen ferries with a floating photovoltaic based marine fueling station. Energy Convers. Manag. 2021, 247, 114760. [Google Scholar] [CrossRef]
- Golroodbari, S.Z.M.; Vaartjes, D.F.; Meit, J.B.L.; van Hoeken, A.P.; Eberveld, M.; Jonker, H.; van Sark, W.G.J.H.M. Pooling the cable: A techno-economic feasibility study of integrating offshore floating photovoltaic solar technology within an offshore wind park. Sol. Energy 2021, 219, 65–74. [Google Scholar] [CrossRef]
- Ocean of Energy. A World’s First: Offshore Floating Solar Farm Installed at the Dutch North Sea. 2019. Available online: https://oceansofenergy.blue/ (accessed on 15 March 2025).
- Onsrud, M. An Experimental Study on the Wave-Induced Vertical Response of an Articulated Multi-Module Floating Solar Island. Master’s Thesis, Norwegian University of Science and Technology, Trondheim, Norway, 2019. [Google Scholar]
- Ocean Sun. Gallery | Ocean Sun. Available online: https://oceansun.no/gallery/ (accessed on 12 March 2025).
- Lee, G.Y.; Joo, H.J.; Yoon, S.J. Design and installation of floating type photovoltaic energy generation system using FRP members. Sol. Energy 2014, 108, 13–27. [Google Scholar] [CrossRef]
- Offshore Energy. SolarDuck Taking Floating Solar Further Offshore. 2021. Available online: https://www.offshore-energy.biz/solarduck-taking-floating-solar-further-offshore/ (accessed on 15 March 2025).
- CIMC. The First Semi-Submersible Offshore Photovoltaic Power Generation Platform in China Was Delivered By Cimc Raffles. 2023. Available online: https://www.offshore-mag.com/renewable-energy/article/14292287/cimc-raffles-delivers-chinas-first-semisub-floating-solar-power-platform (accessed on 7 March 2025).
- Newman, J.N. Wave effects on deformable bodies. Appl. Ocean. Res. 1994, 16, 47–59. [Google Scholar] [CrossRef]
- Hong, S.Y.; Kim, J.H.; Cho, S.K.; Choi, Y.R.; Kim, Y.S. Numerical and experimental study on hydrodynamic interaction of side-by-side moored multiple vessels. Ocean Eng. 2005, 32, 783–801. [Google Scholar] [CrossRef]
- Lee, C.H.; Newman, J.N. An assessment of hydroelasticity for very large hinged vessels. J. Fluids Struct. 2000, 14, 957–970. [Google Scholar] [CrossRef]
- Tajali, Z.; Shafieefar, M. Hydrodynamic analysis of multi-body floating piers under wave action. Ocean Eng. 2011, 38, 1925–1933. [Google Scholar] [CrossRef]
- Qian, J.; Sun, L.; Song, L. Material selection for hawsers for a side-by-side offloading system. J. Mar. Sci. Appl. 2014, 13, 449–454. [Google Scholar] [CrossRef]
- Pessoa, J.; Fonseca, N. Guedes Soares. Side-by-side FLNG and shuttle tanker linear and second order low frequency wave induced dynamics. Ocean Eng. 2016, 111, 234–253. [Google Scholar] [CrossRef]
- Bispo, I.B.S.; Mohapatra, S.C.; Soares, C.G. Numerical analysis of a moored very large floating structure composed by a set of hinged plates. Ocean Eng. 2022, 253, 110785. [Google Scholar] [CrossRef]
- Ding, J.; Wu, Y.-S.; Zhou, Y.; Ma, X.-Z.; Ling, H.J.; Xie, Z. Investigation of connector loads of a 3-module VLFS using experimental and numerical methods. Ocean Eng. 2020, 195, 106684. [Google Scholar] [CrossRef]
- Xu, S.; Wang, S.; Soares, C.G. Review of mooring design for floating wave energy converters. Renew. Sustain. Energy Rev. 2019, 111, 595–621. [Google Scholar] [CrossRef]
- Marco, R.-C.; Tina, G.M. Floating PV Plants; Academic Press: New York, NY, USA, 2020. [Google Scholar]
- Ormberg, H.; Larsen, K. Coupled analysis of floater motion and mooring dynamics for a turret-moored ship. Appl. Ocean. Res. 1998, 20, 55–67. [Google Scholar] [CrossRef]
- Kim, Y.S.; Choi, E.; Yoon, S.J.; Lee, S.O. Three dimensional hydrodynamic-structural analysis of floating type photovoltaic composite structure. In Proceedings of the Advances in Interaction & Multiscale Mechanics (AIMM’10), Jeju, Republic of Korea, 30 May 2010. [Google Scholar]
- Trapani, K.; Millar, D. Hydrodynamic Overview of Flexible Floating Thin Film PV Arrays. In Proceedings of the 3rd Offshore Energy and Storage Symposium, Valletta, Malta, 13–15 July 2016. [Google Scholar]
- Borvik, P.P. Experimental and Numerical Investigation of Floating Solar Islands. Master’s Thesis, NTNU, Taiwan, China, 2017. [Google Scholar]
- Winsvold, J. An Experimental Study on the Wave-Induced Hydroelastic Response of a Floating Solar Island. Master’s Thesis, NTNU, Taiwan, China, 2018. [Google Scholar]
- Kong, Y. Mooring Research of New-Type Floating Structure. Master’s Thesis, Shanghai Jiao Tong University, Shanghai, China, 2019. [Google Scholar]
- Liang, M.; Xu, S.; Wang, X.; Ding, A. Simplification of mooring line number for model testing based on equivalent of vessel/mooring coupled dynamics. J. Mar. Sci. Technol. 2020, 25, 573–588. [Google Scholar] [CrossRef]
- Liang, M.; Xu, S.; Wang, X.; Ding, A. Experimental evaluation of a mooring system simplification methodology for reducing mooring lines in a VLFS model testing at a moderate water depth. Ocean Eng. 2021, 219, 107912. [Google Scholar] [CrossRef]
- Sree, D.K.; Law, A.W.-K.; Pang, D.S.C.; Tan, S.T.; Wang, C.L.; Kew, J.H.; Seow, W.K.; Lim, V.H. Fluid-structural analysis of modular floating solar farms under wave motion. Sol. Energy 2022, 233, 161–181. [Google Scholar] [CrossRef]
- Mohapatra, S.C.; Soares, G.C.; Belibassakis, K. Current Loads on a Horizontal Floating Flexible Membrane in a 3D Channel. J. Mar. Sci. Eng. 2024, 12, 1583. [Google Scholar] [CrossRef]
- Clemente, G.; Ramos-Marin, S.; Soares, G.C. Advances in Maritime Technology and Engineering; Taylor & Francis: London, UK, 2024; pp. 417–425. [Google Scholar]
- Faltinsen, O.M. Sea Loads on Ships and Offshore Structures; Cambridge university press: Cambridge, UK, 1993. [Google Scholar]
- Tcheou, G. Non-Linear Dynamics of Mooring Lines. Doctor’s Thesis, Massachusetts Institute of Technology Department of Ocean Engineering, Cambridge, MA, USA, 1997. [Google Scholar]
- Karadeniz, H.; Saka, M.P.; Togan, V. Water Wave Theories and Wave Loads. In Stochastic Analysis of Offshore Steel Structures: An Analytical Appraisal; Karadeniz, H., Ed.; Springer: London, UK, 2013; pp. 177–252. [Google Scholar]
- Fenton, J.D. A Fifth-Order Stokes Theory for Steady Waves. J. Waterw. Port Coast. Ocean. Eng. 1985, 111, 216–234. [Google Scholar] [CrossRef]
- Young, I.R. Chapter 2—Wave Theory. In Elsevier Ocean Engineering Series; Young, I.R., Ed.; Elsevier: New York, NY, USA, 1999; pp. 3–23. [Google Scholar]
- Brevik, I.; Bjørn, A. Flume experiment on waves and currents. I. Rippled bed. Coast. Eng. 1979, 3, 149–177. [Google Scholar] [CrossRef]
- Morison, J.R.; Johnson, J.W.; Schaaf, S.A. The Force Exerted by Surface Waves on Piles. J. Pet. Technol. 1950, 2, 149–154. [Google Scholar] [CrossRef]
- Orcina. Orca Flex User Manual, Version 11.0; Orcina: Cumbria, UK, 2011.
- Yang, Y.; Bashir, M.; Li, C.; Wang, J. Investigation on Mooring Breakage Effects of a 5 MW Barge-Type Floating Offshore Wind Turbine Using F2A. Ocean Eng. 2021, 233, 108887. [Google Scholar] [CrossRef]
- Wang, Y.; Wang, X.; Xu, S.; Ding, A. Effects of stiffness parameters of flexible connectors of multi-module very large floating structure. Pet. Eng. Constr. 2020, 46, 145–151. [Google Scholar]
- Wang, Y.; Wang, X.; Xu, S.; Ding, A. Influence of multi-module VLFS connector stiffness on dynamic response performance. Ocean Eng. 2018, 36, 11–18. [Google Scholar]



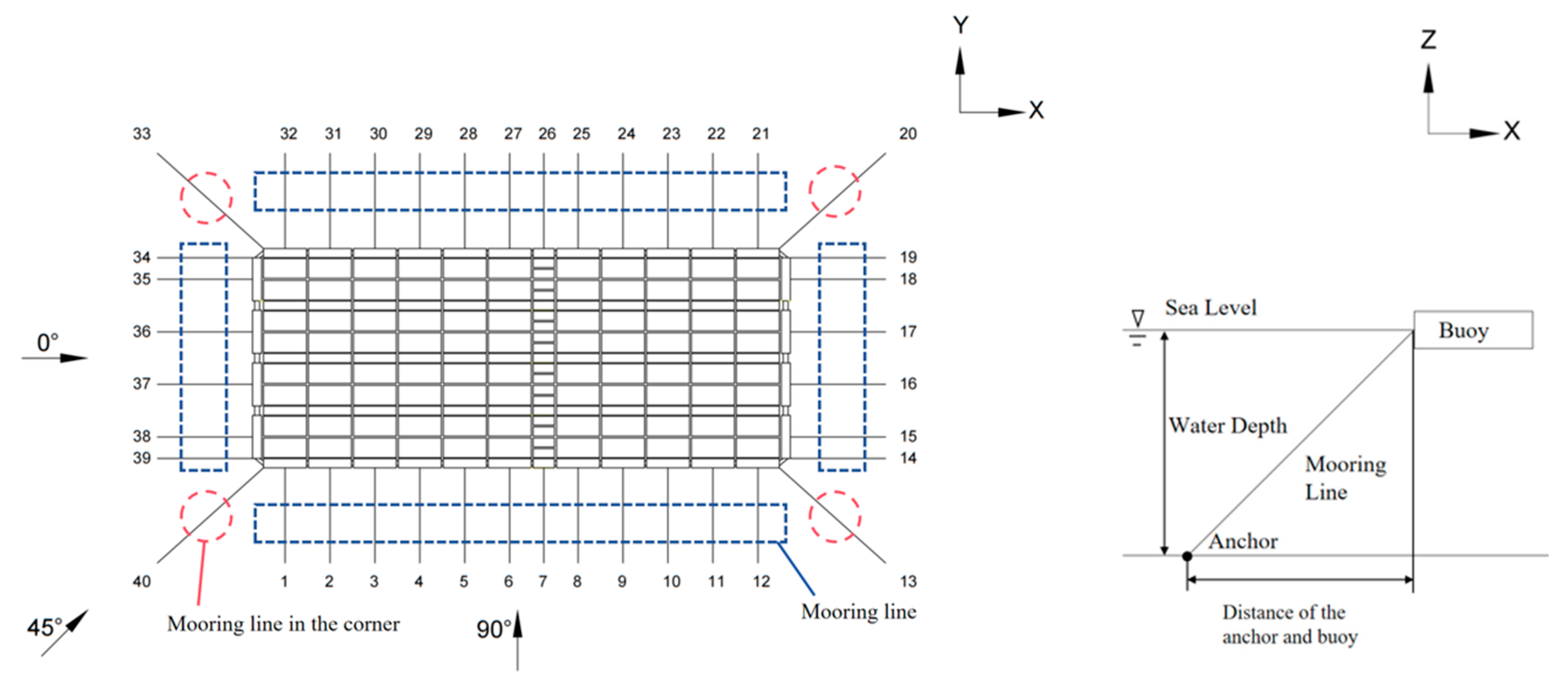
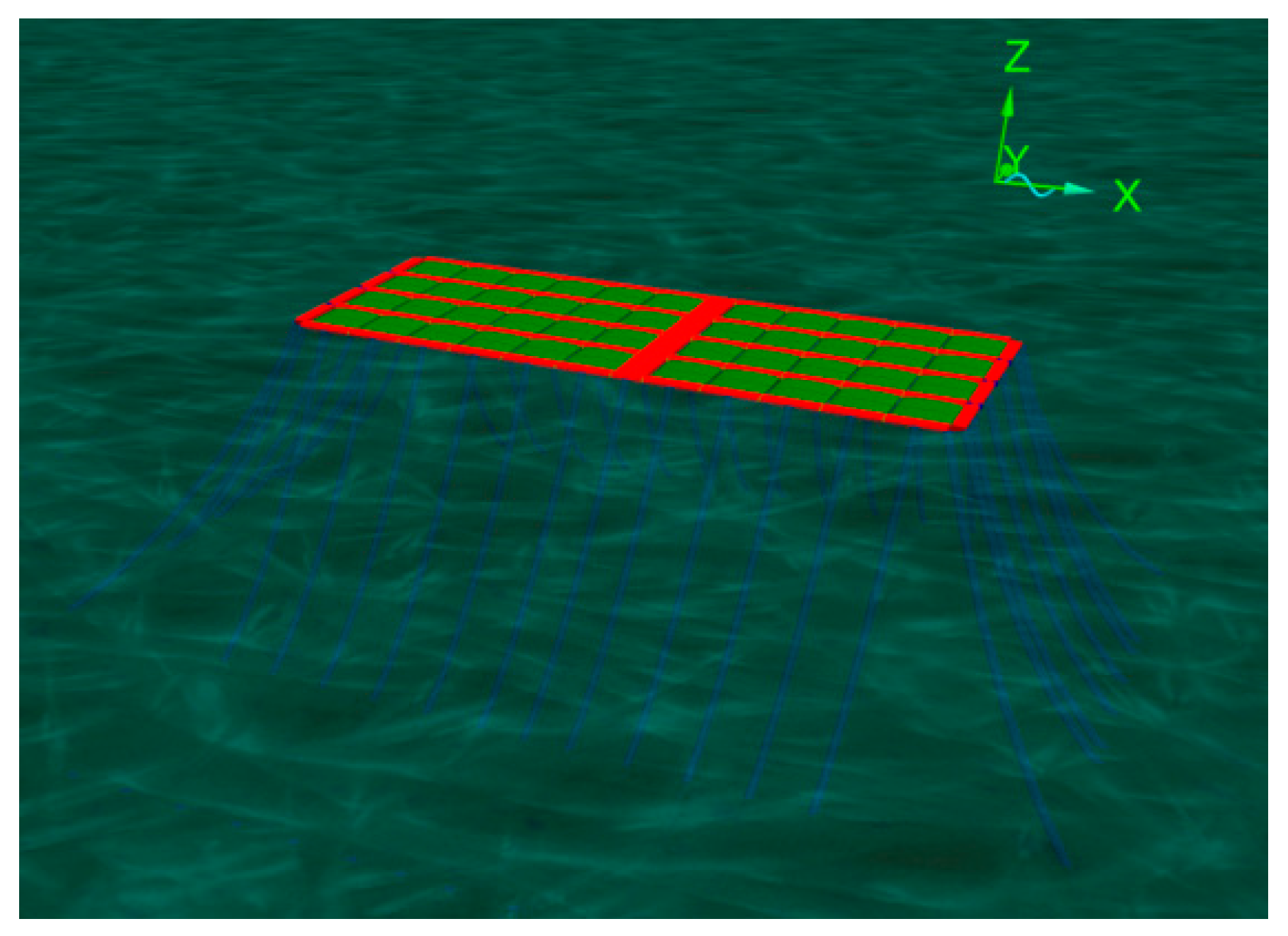







| Main Buoy Size | Unit | Value | Aisle Buoy Size | Unit | Value |
|---|---|---|---|---|---|
| Length | m | 2.260 | Length | m | 1.240 |
| Width | m | 0.480 | Width | m | 0.480 |
| Height | m | 0.265 | Height | m | 0.265 |
| Draught | m | 0.110 | Draught | m | 0.110 |
| Connector | Bending Stiffness [kN/m2] | Axial Stiffness [kN/m] | Torsional Stiffness [kN·m/°] |
|---|---|---|---|
| Between the longitudinal buoys | 0.170 | 100.000 | 0.150 |
| Between the transverse buoys | 259 × 103 | 54.800 × 103 | 1.440 × 106 |
| On the corner of array | 228 × 103 | 48.400 × 103 | 1.330 × 106 |
| Stiffness | Unit | Value |
|---|---|---|
| Young’s modulus | kPa | 212 × 106 |
| Axial stiffness | (kN/m) | 22 × 104 |
| Allowable tension | kN | 100 |
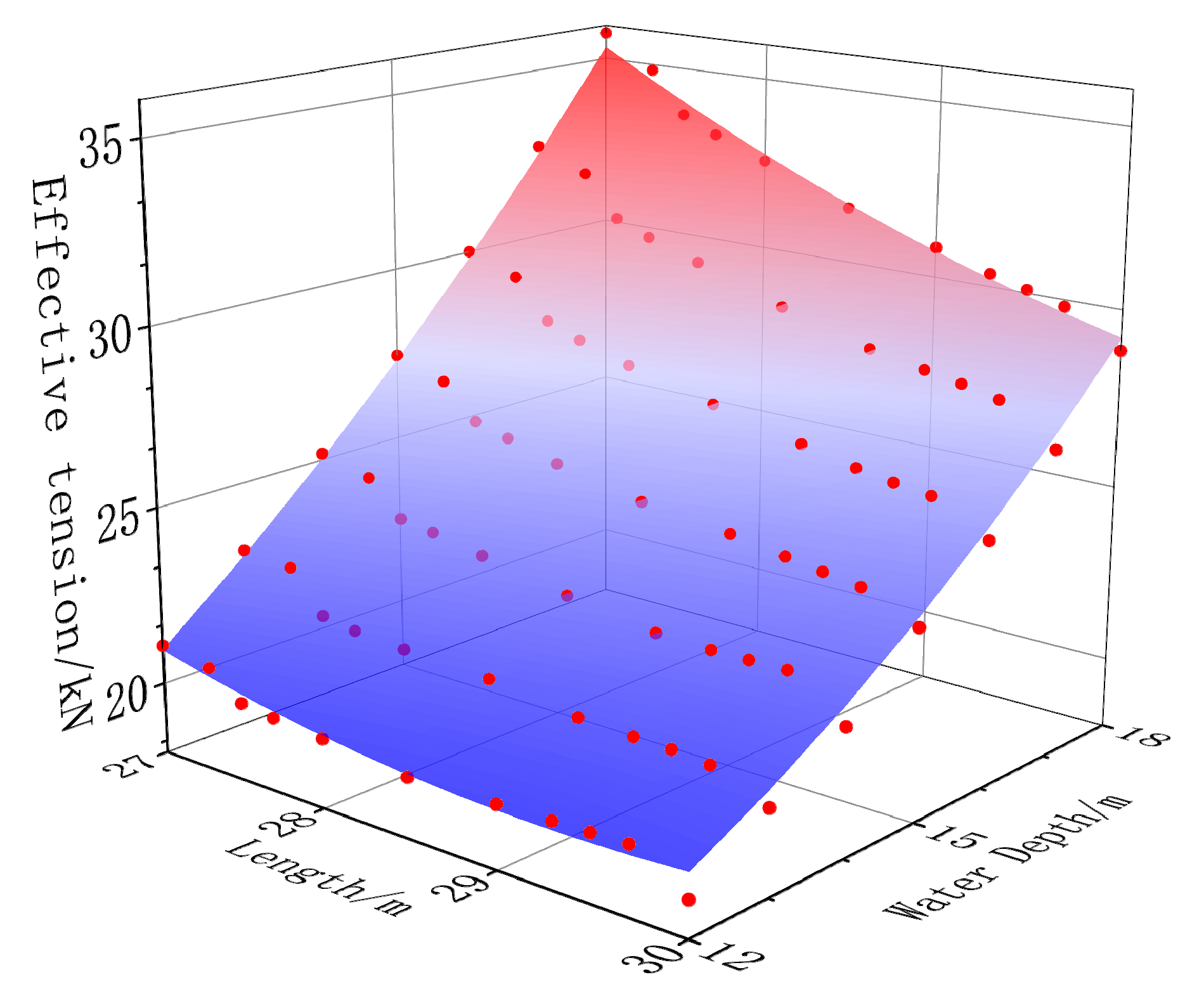
| Method | Function | Correlation Coefficient | Estimated Error Variance |
|---|---|---|---|
| Linear | Te = 30.621 − 1.2245 lm + 1.9876 d | 0.971 | 0.530 |
| Quadratic | Te = 261.9455 − 16.0607 lm − 0.7508 d + 0.2603 lm2 + 0.0913 d2 | 0.979 | 0.399 |
| Quadratic with cross terms | Te = 146.0226 − 11.9933 lm + 6.9774 d + 0.2603 lm2 + 0.0913 d2 − 0.2712 lm d | 0.996 | 0.081 |
| Cubic | Te = 183.2886 − 8.5593 lm + 0.6057 d + 0.003 lm3 + 0.002 d3 | 0.979 | 0.400 |
| Combination | Water Depth [m] | Length of Positive Line [m] | Length of Sideling Line [m] | Effective Tension [kN] |
|---|---|---|---|---|
| Com-I | 18 | 27 | 34 | 35.772 |
| Com-II | 15 | 29 | 36 | 24.584 |
| Com-III | 12 | 30 | 37 | 19.025 |
| Com-IV | 12 | 29 | 36 | 19.776 |
| Com-V | 18 | 29 | 36 | 30.854 |
| Condition | Wave Height [m] | Period [s] | Direction [°] | Wind Velocity [m/s] |
|---|---|---|---|---|
| E1 | 1 | 6.39 | 260 | 10 |
| E2 | 3 | 7.48 | 260 | 10 |
| E3 | 5 | 9.66 | 260 | 36 |
| E4 | 8 | 11.35 | 260 | 56 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Shi, X.-H.; Wang, Y.; Zhang, J.; Guedes Soares, C.; Li, H.; Yu, J. Coupled Response of Flexible Multi-Buoy Offshore Floating Photovoltaic Array Under Waves and Currents. J. Mar. Sci. Eng. 2025, 13, 930. https://doi.org/10.3390/jmse13050930
Shi X-H, Wang Y, Zhang J, Guedes Soares C, Li H, Yu J. Coupled Response of Flexible Multi-Buoy Offshore Floating Photovoltaic Array Under Waves and Currents. Journal of Marine Science and Engineering. 2025; 13(5):930. https://doi.org/10.3390/jmse13050930
Chicago/Turabian StyleShi, Xing-Hua, Yiming Wang, Jing Zhang, C. Guedes Soares, Honglong Li, and Jia Yu. 2025. "Coupled Response of Flexible Multi-Buoy Offshore Floating Photovoltaic Array Under Waves and Currents" Journal of Marine Science and Engineering 13, no. 5: 930. https://doi.org/10.3390/jmse13050930
APA StyleShi, X.-H., Wang, Y., Zhang, J., Guedes Soares, C., Li, H., & Yu, J. (2025). Coupled Response of Flexible Multi-Buoy Offshore Floating Photovoltaic Array Under Waves and Currents. Journal of Marine Science and Engineering, 13(5), 930. https://doi.org/10.3390/jmse13050930









