Key Parameters for Design Analysis and Optimization of Dynamic Inter-Array Power Cable Configurations in Floating Offshore Wind Farms
Abstract
1. Introduction
2. The State of the Art of Dynamic Power Cable Configuration Design Processes
3. Rules and Regulations
- -
- ISO 19900 “Petroleum and natural gas industries—General requirements for offshore structures” [17]
- -
- ISO 19901-1 “Petroleum and natural gas industries—Specific requirements for offshore structures—Part 1: Metocean design and operating considerations” [18]
- -
- ISO 13628-5 “Petroleum and natural gas industries—Design and operation of subsea production systems—Part 5: Subsea umbilicals” [19]
- -
- ISO 13628-11 “Petroleum and natural gas industries—Design and operation of subsea production systems—Part 11: Flexible pipe systems for subsea and marine applications” [20]
- -
- DNV-ST-0119 “Floating wind turbine structures” [16]
- -
- DNV-ST-0359 “Subsea power cables for wind power plants” [21]
- -
- DNV-OS-E301 “Position mooring” [22]
- -
- DNV-RP-0360 “Subsea power cables in shallow water” [23]
- -
- DNV-RP-F401 “Electrical power cables in subsea applications” [24]
- -
- DNV-RP-C205 “Environmental conditions and environmental loads” [25]
- -
- NORSOK-N003 “Action and action effects” [26]
- -
- API RP 2A-LRFD “Recommended practice for planning, designing and constructing fixed offshore platforms—load and resistance factor design” [27]
- -
- API RP 2A-WSD “Recommended practice for planning, designing and constructing fixed offshore platforms—working stress design” [28]
- -
- API SPEC 17E “Specifications for subsea umbilicals” [29]
4. Design Objectives
4.1. Economic Indicators
- -
- Levelized Cost of Energy (LCOE): It is a widely used metric to assess cost-effectiveness by considering total lifetime costs divided by total lifetime energy output, with lower values indicating efficiency [30]. The LCOE of floating offshore wind projects is about five times as high as for bottom-fixed offshore wind projects [1]. Predictions for the future vary, including higher, equal, and lower LCOE values by 2050 for floating compared to bottom-fixed offshore wind projects [1]. Nevertheless, they all predict a decrease in the LCOE for floating offshore wind projects due to technological advancement [31].
- -
- Capital Expenditure (CapEx): This metric refers to the initial investment required to design, manufacture, and install the floating wind turbine and its associated power cables. For offshore wind projects, most lifetime costs occur for the development and construction of the project as CapEx [31].
- -
- Installation and decommissioning costs are considered parts of capital expenditure: A design can have low costs considering the price of the components involved, but it might be costly during transport, installation, and decommissioning. Improper consideration of these costs has been a major problem in past renewable energy projects, such as when removing wave energy converters (WECs). It can quickly lead to bankruptcy [32]. Further, the available equipment for installation and removal must be considered in the design process to avoid using unusual or hardly available equipment, which can be very costly. Also, the choice of ancillaries should be made considering the need for potentially new molding and their installation time, which can increase and raise costs.
- -
- Operating Expenditures (OpEx): These are the day-to-day costs, covering expenses like rent, utilities, salaries, maintenance, and other essential operational needs. Maintenance costs include regular inspections and repairs throughout the lifetime of the power cable configuration. These costs can vary based on cable design, materials used, and accessibility for maintenance crews. Critical components, such as the hang-off section and the touch-down point (TDP), are crucial for the integrity of the system. Addressing these early in the design process can prevent future repair work. Regularly removing marine growth from the power cables and ancillaries can significantly raise maintenance costs. If considered correctly, the need for removals or buoyancy additions throughout the lifetime might be limited. Rinaldi et al. [30], Garcia-Teruel et al. [33], and Yang et al. [34] present analyses including operation and maintenance costs for FOWTs.
- -
- Regulations and permits: Compliance with regulations and permitting requirements can influence design choices and costs. For example, factors such as seabed intervention (e.g., trenching or similar activities), marine life entanglement, trawling entanglement, and ghost net entanglement must be carefully assessed, as these can be regulated and require specific permits. Further, it may be necessary to obtain an initial technical qualification for a new power cable system.
- -
- Risk assessment: Economic indicators should also incorporate risk analysis at every design stage. Identifying potential failure points, downtime risks, and their financial consequences is crucial for estimating overall project costs accurately. It helps to account for unforeseen events such as supply chain disruptions or regulatory changes.
- -
- Future expansions and upgrades: Designing for future expansion and upgrades helps minimize project costs by avoiding costly modifications later. Anticipating potential expansions and technology improvements in FOWT farms, leading to increased power generation, is crucial for long-term viability.
- -
- Minimize cable lengths: Shorter cable lengths reduce both electrical losses and material costs associated with cable manufacturing and installation. Power transmission efficiency can be improved by strategically planning cable configurations and routes and minimizing unnecessary detours, ensuring the electricity reaches its destination with minimal loss. However, a difference in cable length by a few percentage points may not significantly affect capital and operational costs, depending on the project.
- -
- Minimize the use of ancillaries: Ancillaries represent an additional cost to the power cable. Using as few ancillaries as possible can reduce costs as well as installation and maintenance times.
4.2. Performance Indicators
5. Design Variables
5.1. Power Cable Selection
5.2. Configuration Design
5.3. Special Components and Ancillaries
6. Design Constraints
6.1. Environmental Constraints
6.1.1. Wind and Waves
6.1.2. Currents
6.1.3. Internal Waves
6.1.4. Water Depth
6.1.5. Marine Growth
6.1.6. Temperature
6.1.7. Cold Climate
6.1.8. Bathymetry and Geotechnical Conditions
6.1.9. Climate Change Impact
6.2. Response Constraints
6.2.1. Tension and Compression Limits
6.2.2. Curvature Limits
6.2.3. Fatigue
6.2.4. Torsion Limits
6.2.5. Snap Loading
6.2.6. Resonance
6.2.7. Vortex-Induced Vibrations (VIVs)
6.3. Geometrical Constraints
6.3.1. Clearance to the Seabed and Sea Surface
6.3.2. Placement to Other Infrastructure
6.3.3. Inherent Constraints of Ancillaries
6.4. Lifecycle Constraints
6.4.1. Installation
6.4.2. Operation and Maintenance
6.4.3. Decommissioning
6.5. Modeling and Analysis Limitations
6.6. Environmental Impacts
6.7. Socio-Economic Aspects
7. Optimization
7.1. Determining the Most Relevant Optimization Parameters
7.2. Power Cable Configuration Optimization for FOWTs
7.3. Umbilical and Riser Optimization in Further Industries
| Reference | Wang et al., 2011 [189], Yang et al., 2012 [190] | Yang et al., 2021 [191] | Yan et al., 2023 [192] |
|---|---|---|---|
| Optimization technique | Non-dominated Sorting Genetic Algorithm II (NSGA-II) | Non-dominated Sorting Genetic Algorithm II (NSGA-II) | Non-Linear Programming by Quadratic Lagrangian (NLPQL) algorithm |
| Objective function | Minimize the buoyancy per unit length, length of the buoyancy section | Minimize umbilical max. tension strain, and bending moment | Minimize the umbilical length, cumulative fatigue damage |
| Design variables | Buoyancy per unit length, end positions of the buoyancy section | Umbilical thickness, helical angle, length, hang-off angle, buoyancy section length, and position | Angles at the hang-off and buoyancy section ends |
| Umbilical max. top tension, min. bending radius, max. equivalent stress | Umbilical max. tension, max. curvature, min. fatigue life | Min. fatigue life |
| Min. and max. buoyancy per unit length, min. and max. position of the buoyancy section ends | Umbilical min. and max. thickness, min. and max. helical angle, min. and max. length, buoyancy section min. and max. length, min., and max. position; min. and max. hang-off angle | Min. and max. angles at hang-off and buoyancy section ends, umbilical min. and max. length |
| Unidirectional load cases with regular waves, constant current, and current profile | Multidirectional extreme load cases with regular waves, constant current (no current profile provided) | Multidirectional load cases with wave spectrum, current (no current profile provided) |
| Modeling and analysis method | Response Surface Model (RSM) and Radial Basis Function (RBF) approximation models | Approximation through a neural network based on Radial Basis Function (RBF) | Approximation through a surrogate model with Radial Basis Function (RBF) |
| Umbilical configuration type | Lazy wave | Lazy wave | Lazy wave |
| Other components included? | No | Yes: Cross-section | No |
| Reference | Riggs 1989 [193] | Riggs and Leraand 1991 [194] | Larsen and Hanson 1999 [195] | Vieira et al., 2003 [196] | Cunliffe et al., 2004 [197] |
|---|---|---|---|---|---|
| Optimization technique | Sequential Quadratic Programming (SQP) | Adaptive Discretization algorithm | Sequential Quadratic Programming (SQP) | Genetic algorithm (GA) | Genetic algorithm (GA) |
| Objective function | Minimize riser length | Minimize the riser’s overall length | Minimize riser wall thickness linked to material cost | Minimize the acquisition cost of riser sections | Minimize the bill of materials |
| Design variables | Riser section lengths, hang-off height, and buoyancy section weight | Riser section lengths, weight of the second riser section, and the mean location of the vessel | Riser section lengths, wall thickness, and horizontal distance from the lower to upper end (far position) | Riser section lengths, buoyancy section length | Riser section lengths |
| Response constraints | Riser max. top tension, max. top tension variation, min. service life | Riser min. bend radius, max. top tension variation | Riser sections’ max. equivalent stress, critical bending strain, pressure, buckling threshold | Riser max. von Mises stress, max. operating stress | Riser max. wall stress, collapse pressure, collapse propagation |
| Geometrical constraints | Riser min. bend radius, max. angle at hang-off, min. seabed clearance, max. span, buoyancy max. elevation | Riser min. seabed and sea surface clearance, max. buoyancy elevation, min. first section length, min. and max. bottom angle, max. bottom angle variation, max. top angle variation | Riser min. overall length, min. length on the seafloor in the far position | Riser max. top angle, min. and max. section lengths, buoyancy section min. and max. length | Riser far end is horizontal on the seabed for tie-in, material 30 m from TDP on the seabed is changed from titanium to duplex |
| Environmental constraints | Varying hang-off positions | Uniform current, hang-off location position | Hang-off location position, current (no profile provided) | None | Near and far ultimate limit states (ULS) (applied parameters not provided) |
| Modeling and analysis method | Analytic equations; static | Catenary equations, cable elements, static, steady state | Finite Element Analysis (FEA); static | Modeling method not provided; static | Finite Element Analysis (FEA); static, dynamic |
| Riser configuration type | Steep-wave, lazy-wave, lazy-S | Steep-wave | Catenary | Lazy wave | Catenary |
| Other components included? | No | No | No | No | No |
| Reference | Rodrigues 2004 [198] | Roveri et al., 2005 [199] | Lima et al., 2005 [200] | Tanaka and Martins 2006 [201] | Tanaka et al., 2006 [202] |
| Optimization technique | Genetic algorithm (GA) | Parametric analysis | Hybrid fuzzy/Genetic algorithm (GA) | Genetic algorithm (GA) | Simulated Annealing (SA) |
| Objective function | Minimize the acquisition costs of riser components | Find feasible configurations | Minimize riser section lengths and buoyancy section lengths | Minimize acquisition cost and launch cost | Minimize pipe and buoy material volume by price |
| Design variables | Riser section lengths, tendon length, and buoy net buoyancy | Riser section lengths | Riser section lengths, buoyancy section length | Riser section lengths, outer diameters, and buoyancy section outer diameters | Riser overall length, external diameter, and buoy thickness |
| Riser top max. tension, max. traction force, tendon top max. traction force | Riser min. fatigue life | Riser max. von Mises stress | Riser min. curvature radius, propagating buckling collapse criteria, max. stress, max. top tension | Riser propagating buckling collapse criteria, max. stress |
| Tendon vertical max. and min. inclination angles | Riser max. top angle, min. curvature radius, min., and max. section lengths | Riser min. and max. section lengths, max. top angle, buoyancy section min. and max. lengths | Riser min. and max. section lengths, min., and max. outer diameters, buoyancy modules outer min. and max. diameters, max. top angle | Riser max. top angle, min. and max. lengths, min. and max. external diameters, buoy min. and max. thicknesses |
| Variable current velocities, three-dimensional current profile | Current (no profile provided), waves (no specifications provided) | None | Load cases with current at the surface from different directions (no profile provided) | Load cases with different current directions (no profile provided) |
| Modeling and analysis method | Catenary equations integration, steady-state (static) | Differential ordinary equations with the Runge–Kutta method, static, dynamic | Finite Element Analysis (FEA), static | Modeling method not provided, static | Solving differential equations with the Runge–Kutta method, static |
| Riser configuration type | Lazy-S | Lazy-wave | Catenary, lazy wave | Catenary, lazy wave | Catenary, lazy wave |
| Other components included? | No | No | No | No | No |
| Reference | Tanaka and Martins 2007 [203] | Vieira et al., 2008 [204] | Yang et al., 2009 [205] | Tanaka 2009 [206] | Vieira et al., 2009 [188] |
| Optimization technique | Genetic algorithm (GA) | Artificial Immune System (AIS), Genetic algorithm (GA) | Island-based Genetic algorithm (IGA) | Simulated Annealing (SA), Genetic algorithm (GA), Sequential Linear Programming (SLP), Linear Programming, Powell’s Conjugate Directions Method, Quasi-Newton, Interval Division, Fletcher-Reeves, Hessian matrix approximation | Artificial Immune System (AIS), Genetic algorithm (GA), Particle Swarm Optimization (PSO) |
| Objective function | Minimize max. stress amplitude | Minimize riser length and buoy volume by price | Minimize riser material | Minimize volume per price, max. static stress, max. dynamic stress amplitude | Minimize riser length and buoy volume by price |
| Design variables | Riser section lengths, buoyancy floater diameter | Riser section lengths, buoy diameter, length, spacing | Riser wall thickness, coating density | Riser section material, length; buoyancy module number, material, inner and outer diameter | Riser section lengths, buoy diameter, length, spacing |
| Response constraints | Riser min. tension, min. curvature radius, max. stress | Riser max. von Mises stress, max. top stress, min. stress | Riser max. von Mises stress, collapse criteria | Riser buckling threshold, max. top traction, min. and max. tension, max. equivalent tension | Riser max. von Mises stress, max. top stress, min. stress |
| Geometrical constraints | Riser min. and max. section lengths; buoyancy min. and max. floater diameter | Riser min. and max. section lengths, min. and max. top angles, max. top angle variation, buoy min. and max. diameters, min. and max. lengths, min. and max. spacing | Not specified | Riser external diameter > internal diameter, max. length, max. top angle, min. curvature | Riser min. and max. section lengths, min. and max. top angles, max. top angle variation; buoy min. and max. diameters, min. and max. lengths, min. and max. spacing |
| Environmental constraints | One load case with steady current profile, hang-off offset, and motion | Not specified | Not specified | Multidirectional steady current profiles | Not specified |
| Modeling and analysis method | Finite Element Analysis (FEA), frequency domain, static, dynamic | Analytic catenary, static | Finite Element Analysis (FEA), dynamic | Finite Element Analysis (FEA), static, dynamic | Analytic catenary, static |
| Riser configuration type | Catenary and lazy-wave | Lazy wave | Catenary | Lazy wave | Lazy wave |
| Reference | Andrade et al., 2010 [207] | Pina et al., 2008, 2011 [208,209] | Zhen and Yang 2010 [210], Yang and Zheng 2011 [211] | Tanaka and Martins 2011 [212] | Vieira et al., 2012 [187] |
| Optimization technique | Non-dominated Sorting Genetic Algorithm II (NSGA-II) | Particle Swarm Optimization (PSO) | Single-loop reliability-based design optimization (RBDO) | Genetic algorithm (GA); Simulated Annealing (SA) | Genetic algorithm (GA); Particle Swarm Optimization (PSO); Artificial Immune System (AIS) |
| Objective function | Minimize the buoyancy module volume, riser top tension | Minimize the cost of material | Minimize the wall thicknesses of riser sections | Minimize max. dynamic stress amplitude along the riser | Minimize the cost and length of each riser section, and the cost and volume of the buoyancy module type applied |
| Design variables | Riser section lengths, buoyancy section length, buoyancy modules diameters, lengths, and spacing | Riser section lengths, buoyancy section length, buoyancy module length, diameter, spacing | Riser sections’ wall thicknesses | Riser section lengths, buoyancy thickness | Riser section lengths, buoyancy modules diameter, length |
| Response constraints | Installation riser max. tension, stresses, compression, max. VIV fatigue life | Riser max. von Mises stress, max. top tension, min. tension at the bottom | Riser max. dynamic equivalent stress, min. required probability value | Riser propagating buckling collapse criteria, min. curvature, min. tension, max. stress | Riser sections max. von mises Stress, min. tension at seabed sections, max. top tension |
| Geometrical constraints | Sag and hog bend min. and max. vertical positions; TDP min. and max. horizontal position | Riser top min. and max. angle, max. angle variation, min., and max. riser section lengths; buoyancy section min. and max. length; buoyancy module min. and max. diameter, length, spacing | Riser sections min. and max. wall thicknesses | None | Riser min. and max. top angle, max. ‘built-in’ angle variation, min., and max. section lengths; buoyancy modules min. and max. diameter, length, spacing |
| Environ-mental constraints | Multi-directional steady current profiles (profiles not provided) | None | Cosine motion imposed at hang-off | Multidirectional extreme load cases with sea states, steady current profiles | None |
| Modelling and analysis method | Finite Element Analysis (FEA); static, dynamic | Analytical catenary; static | Design of Experiment (DoE) with Finite Element Analysis (FEA), during optimization, a Kriging metamodel constructed by Latin hypercube sampling (LHS); static, dynamic | Linear model using frame finite elements solved iteratively; frequency domain | Analytical catenary; static |
| Riser configuration type | Lazy wave | Lazy wave | Catenary | Catenary, lazy-wave | Lazy-wave |
| Other components included? | No | No | No | No | No |
| Other components included? | No | No | No | No | |
| Reference | Arruda et al., 2012 [213] | Martins 2011 [214], Martins et al., 2013 [215] | Voie and Sødahl 2013 [216] | Nariño et al., 2014 [217] | Chen et al., 2016 [218] |
| Optimization technique | Genetic algorithm (GA) | Non-dominated Sorting Genetic Algorithm II (NSGA-II) | Sequential Quadratic Programming (SQP) | Non-dominated Sorting Genetic Algorithm II (NSGA-II) | Surrogate model: Kriging, Radial Basis Function (RBF); multi-island genetic algorithm (MIGA); Non-linear Programming by Quadratic Lagrangian (NLPQL) algorithm |
| Objective function | Minimize the total volume of floaters | Minimize total riser volume, stress utilization factor | Find a design to meet the target fatigue life | Minimize the amplitude variations in the von Mises stresses and the total length of the riser sections with hydrodynamic dampers | Minimize riser curvature |
| Design variables | The floater section starts and ends on the riser | Riser section lengths, outer diameter, heavy coating wall thickness, overlength fraction, buoyancy module diameter, and well horizontal offset | Weight section length, distance to hang-off, distance to buoyancy section, buoyancy key factor, buoyancy section length, buoyancy key factor | Number, position, and length of the hydrodynamic dampers | Riser section length, buoyancy section length, hang-off to TDP distance |
| Response constraints | Riser max. service tension, max. traction tension at the floaters | Riser max. von Mises stress, min. effective tension, min. radius of curvature | Riser min. fatigue life | Riser max. stress | Riser max. dynamic tension, max. curvature |
| Geometrical constraints | Riser min. seabed clearance, floater section min. distance to hang-off, max. length | None | None | Max total length of the hydrodynamic damper sections | Riser min. and max. section lengths, max. overall length, max. hang-off angle, min. and max. buoyancy section length, min., and max. hang-off to TDP distance, seabed clearance to sag bend |
| Environmental constraints | Load case with current and current profile | Two directions of collinear waves with a spectrum, current | Several unidirectional ultimate and accident limit states with regular waves, hang-off offsets | Wave and current (specifications not provided) | One condition with a regular wave, constant current (no current profile provided) |
| Modeling and analysis method | Finite Element Analysis (FEA), static | Finite Element Analysis (FEA), static, dynamic | Finite Element Analysis (FEA), dynamic | Finite Element Analysis (FEA), static, dynamic | Finite Element Analysis (FEA), dynamic |
| Riser configuration type | Lazy-wave | Vertical access with weight between the hang-off and buoyancy section | Lazy-wave with weights between the buoyancy section and the hang-off | Catenary with hydrodynamic dampers | Steep-wave |
| Other components included? | No | No | No | No | |
| Reference | Yang et al., 2018 [219] | Diakonova et al., 2019 [220] | Cardoso 2019 [186,221] | Ai et al., 2019 [222] | Bhowmik et al., 2019, 2020 [223,224] |
| Optimization technique | Particle Swarm Optimization (PSO) | Brute force with limited load case selection | Globalized Bounded Nelder–Mead method (GBNM) | Genetic algorithm (GA) | Random Forest (RF) metamodel coupled with Evolutionary Algorithm (RF-EA), Radial Basis Function (RBF) metamodel coupled with Evolutionary Algorithm (RBF-EA) |
| Objective function | Maximize the utilization rate of tension, curvature | Minimize the cost of procurement and installation | Minimize total riser length | Minimize the max. stress, fatigue damage | Minimize material cost, fatigue damage |
| Design variables | Riser section length, buoyancy section length, riser wall thickness | Riser top angle, section lengths, buoyancy section length, buoyancy factor | Riser section lengths, buoyancy module diameter, section length, spacing | Riser section length, buoyancy section length, buoyancy module diameter | Riser top angle, sag bend elevation, hog bend elevation, buoyancy section length, buoyancy module diameter |
| Response constraints | Riser max. tension, max. curvature, min. fatigue life, min., and max. yield stress | Riser min. and max. interface loads (topside, seabed), min. fatigue life | Riser combined loading criterion (max. bending moment and effective tension) | None | Riser max. tension at hang-off, min. tension at TDP, max. von Mises stress, min. fatigue life |
| Geometrical constraints | Riser min. and max. section lengths, min. and max. hang-off angles, min. and max. spiral angles at buoyancy section ends, min. and max. buoyancy section lengths; min. and max. wall thicknesses | Riser max. offset | Riser min. and max. section lengths, buoyancy module min. and max. diameters, min. and max. section lengths, min. and max. spacing | Riser min. and max. section lengths, min. and max. vertical offset, max. hang-off angles, buoyancy section min. and max. lengths, buoyancy module min. and max. diameters | Riser max. hang-off angle, min. sag bend seabed clearance |
| Environmental constraints | Unidirectional extreme load cases with waves (wave type not provided), constant current (no current profile provided) | Extreme load case (no specifications provided) | One imposed riser top-end motion case | Unidirectional extreme load case with irregular waves, steady current profile | One extreme condition with a regular wave, constant current, and current profile |
| Modeling and analysis method | Bi-scale response surface | Finite Element Analysis (FEA), static, dynamic | Finite Element Analysis (FEA), dynamic | Finite Element Analysis (FEA), dynamic | Finite Element Analysis (FEA), dynamic |
| Riser configuration type | Lazy wave | Lazy wave | Lazy wave, catenary with hydrodynamic damper | wave | Lazy wave |
| Other components included? | No | No | No | No | No |
| Reference | Lal et al., 2020 [225] | Ogbeifun et al., 2021 [226] | Lal et al., 2021 [227] | Elsas et al., 2021 [228] | |
| Optimization technique | Genetic algorithm (GA) | 2D tabular optimization method | Genetic algorithm (GA) | Bayesian Optimization (BO) algorithm, derivative-free evolutionary hybrid algorithm MIDACO, Globalized Bounded Nelder–Mead (GBNM) method, Genetic algorithm (GA) | |
| Objective function | Minimize bending stress, fatigue | Minimize riser hanging length, hang-off tension, stress utilization, fatigue damage around the bends, smeared buoyancy section length, and thickness | Maximize strength and fatigue response, and minimize the cost of the buoyancy section | Minimize total riser length | |
| Design variables | Riser section lengths, hang-off angle, buoyancy section length, buoyancy factor | Riser hanging length, smeared buoyancy section length, and thickness | Riser length between hang-off and buoyancy section, buoyancy section length | Riser section lengths, buoyancy modules spacing, length, diameter, and hydrodynamic damper section lengths | |
| Response constraints | None | Minimum apparent mass ratio of the buoyant sections | Riser max. von Mises stress | Riser max. effective load | |
| Geometrical constraints | Minimum seabed clearance, sag bend always lower than hog bend | Minimum sag height elevation from the seabed, min. arc height | Riser min. and max. lengths between hang-off and buoyancy section, min. seabed clearance, buoyancy section min. and max. lengths, minimum seabed clearance must be higher than the sag bend | Riser min. and max. section lengths, min. and max. top angles, buoyancy modules’ min. and max. spacing, min. and max. lengths, min. and max. diameters, hydrodynamic dampers’ min. and max. section lengths, min. seabed clearance, max. total riser length | |
| Environmental constraints | Regular heave motion at hang-off | Two unidirectional regular wave load cases | Several load cases (no specifications provided) | One load case with steady current profile, prescribed hang-off motion | |
| Modeling and analysis method | Finite Element Analysis (FEA), dynamic | Finite Element Analysis (FEA), steady-state (static) | Finite Element Analysis (FEA) and neural network, dynamic | Finite Element Analysis (FEA), steady-state (static) | |
| Riser configuration type | Lazy-wave | Catenary, lazy-wave | Lazy-wave | Lazy-wave, catenary with hydrodynamic dampers | |
| Other components included? | No | No | No | No | |
| Reference | Abam et al., 2022 [229] | Abam et al., 2022 [230] | Lal et al., 2023 [231] | ||
| Optimization technique | Combined Genetic Algorithm (GA) and Artificial Neural Network (ANN) | Genetic algorithm (GA) | Particle Swarm Optimization (PSO) | ||
| Objective function | Minimize riser steel weight | Minimize total structural weight | minimize bending stress, fatigue, and material cost | ||
| Design variables | Riser wall thickness, declination angle, and length | Riser wall thickness, length, declination angle at hang-off | Riser hang-off angle, section lengths, buoyancy section length, buoyancy factor | ||
| Bursting criteria, buckling (collapse) criteria, buckling propagation criteria, yielding criteria, and fatigue limit state (FLS) criteria | Bursting criteria, buckling (collapse) criteria, buckling propagation criteria, yielding criteria, fatigue limit state (FLS) criteria | Riser max. von Mises stress | ||
| Riser min. and max. wall thickness, min. and max. declination angle, min. and max. length | Riser min. and max. wall thickness, min. and max. declination angle, min. and max. length | Minimum seabed clearance, Sag bend always lower than hog bend | ||
| One unidirectional load case with irregular waves, wind spectrum, and steady current profile | One unidirectional load case with irregular waves, wind spectrum, and steady current profile | Harmonic motion at the hang-off | ||
| Modelling and analysis method | Finite Element Analysis (FEA), dynamic | Finite Element Analysis (FEA), dynamic | Finite Element Analysis (FEA), dynamic | ||
| Riser configuration type | Catenary | Catenary | Lazy-wave | ||
| Other components included? | No | No | No | ||
8. Conclusions
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
Appendix A. Application Case: Northern North Sea
References
- DNV. Floating Wind: Turning Ambition into Action; Det Norske Veritas: Oslo, Norway, 2023. [Google Scholar]
- Cozzi, L.; Wanner, B. Offshore Wind Outlook 2019: World Energy Outlook Special Report; International Energy Agency (IEA): Paris, France, 2019. [Google Scholar]
- WPED. GCube Releases Decade-Long Research Report on Offshore Wind Project Insurance Claims. Windpower Engineering & Development (WPED). 2021. Available online: https://www.windpowerengineering.com/gcube-releases-decade-long-research-report-on-offshore-wind-project-insurance-claims/ (accessed on 9 October 2023).
- Rentschler, M.U.T.; Adam, F.; Chainho, P.; Krügel, K.; Vicente, P.C. Parametric study of dynamic inter-array cable systems for floating offshore wind turbines. Mar. Syst. Ocean Technol. 2020, 15, 16–25. [Google Scholar] [CrossRef]
- Eldøy, S.; Vold, O.; Graven, H.; Guttormsen, T.; Delp, L. Hywind Scotland Pilot Park Project Plan for Construction Activities 2017; Statoil: Stavanger, Norway, 2017. [Google Scholar]
- Bowie, M.; Parry, A.; Giles, J.; West, A. Development Specification and Layout Plan Kincardine Offshore Windfarm Project; Kincardine Offshore Wind Farm Ltd. (KOWL): Edinburgh, UK, 2018. [Google Scholar]
- Equinor. Hywind Tampen, PL050-PL057-PL089, PUD del II-Konsekvensutredning; Equinor ASA: Stavanger, Norway, 2019. [Google Scholar]
- Duarte, T.; Price, S.; Peiffer, A.; Pinheiro, J.M. WindFloat Atlantic Project: Technology Development Towards Commercial Wind Farms. In Proceedings of the Offshore Technology Conference, Houston, TX, USA, 2–5 May 2022; p. 11. [Google Scholar] [CrossRef]
- Metcentre. Test Areas—Metcentre 2023. Available online: https://www.norwegianoffshorewind.no/about/initiatives/met-centre (accessed on 19 November 2023).
- Yoshimoto, H.; Awashima, Y.; Kitakoji, Y.; Suzuki, H. Development of floating offshore substation and wind turbine for Fukushima FORWARD. In Proceedings of the International Symposium on Marine and Offshore Renewable Energy, Tokyo, Japan, 27–29 October 2013. [Google Scholar]
- Gudmestad, O.T.; Schnepf, A. Design Basis Considerations for the Design of Floating Offshore Wind Turbines. Sustain. Mar. Struct. 2023, 5, 26–34. [Google Scholar] [CrossRef]
- DNV-ST-0145; Offshore Substations. Det Norske Veritas (DNV): Oslo, Norway, 2021.
- Do Prado, V.G.; Monteiro, B.D.F.; De Lima, B.S.L.P.; Jacob, B.P. Optimal design of mooring systems for floating production units based on metamodel-assisted Differential Evolution. Ocean Eng. 2022, 266, 112752. [Google Scholar] [CrossRef]
- Ja’e, I.A.; Osman Ahmed Ali, M.; Yenduri, A.; Nizamani, Z.; Nakayama, A. Optimisation of mooring line parameters for offshore floating structures: A review paper. Ocean Eng. 2022, 247, 110644. [Google Scholar] [CrossRef]
- Griffiths, T.; Draper, S.; Cheng, L.; An, H.; Schläppy, M.-L.; Fogliani, A.; White, D.; Noble, S.; Coles, D.; Johnson, F.; et al. The offshore renewables industry may be better served by new bespoke design guidelines than by automatic adoption of recommended practices developed for oil and gas infrastructure: A recommendation illustrated by subsea cable design. Front. Mar. Sci. 2023, 10, 1030665. [Google Scholar] [CrossRef]
- DNV-ST-0119; Floating Wind Turbine Structures. Det Norske Veritas (DNV): Oslo, Norway, 2021.
- ISO 19900:2019; Petroleum and Natural Gas Industries. General Requirements for Offshore Structures. International Organization for Standardization: Geneva, Switzerland, 2019.
- ISO 19901-1:2015; Petroleum and Natural Gas Industries. Specific Requirements for Offshore Structures. Part 1: Metocean design and operating considerations (EN ISO 19901-1:2015). Standard Norge: Lysaker, Norway, 2015.
- European Standard 13628-5; Petroleum and Natural Gas Industries. Design and Operation of Subsea Production Systems. Part 5: Subsea Umbilicals. International Organization for Standardization: Geneva, Switzerland, 2009.
- European Standard 13628-11; Petroleum and Natural Gas Industries-Design and Operation of Subsea Production Systems-Part 11: Flexible Pipe Systems for Subsea and Marine Applications. International Organization for Standardization: Geneva, Switzerland, 2007.
- DNV-ST-0359; Subsea Power Cables for Wind Power Plants. Det Norske Veritas: Oslo, Norway, 2021.
- DNV-OS-E301; Position Mooring, Offshore Standard. Det Norske Veritas: Oslo, Norway, 2021.
- DNV-RP-0360; Subsea Power Cables in Shallow Water, Recommended Practice. Det Norske Veritas: Oslo, Norway, 2021.
- DNV-RP-F401; Electrical Power Cables in Subsea Applications, Recommended Practice. Det Norske Veritas: Oslo, Norway, 2021.
- DNV-RP-C205; Environmental Conditions and Environmental Loads, Recommended Practice. Det Norske Veritas: Oslo, Norway, 2021.
- NORSOK. N003: Actions and Action Effects. NORSOK: Oslo, Norway, 2018. [Google Scholar]
- API. Recommended Practice for Planning, Designing, and Constructing Fixed Offshore Platforms-Load and Resistance Factor Design, 2A-LRFD; Supplemented 1997; American Petroleum Institute (API): Washington, DC, USA, 1993. [Google Scholar]
- API. Recommended Practice for Planning, Designing, and Constructing Fixed Offshore Platforms-Working Stress Design 2A-WSD; American Petroleum Institute (API): Washington, DC, USA, 2014. [Google Scholar]
- API. Specification for Subsea Umbilicals, API SPEC 17E; American Petroleum Institute: Washington, DC, USA, 2017. [Google Scholar]
- Rinaldi, G.; Garcia-Teruel, A.; Jeffrey, H.; Thies, P.R.; Johanning, L. Incorporating stochastic operation and maintenance models into the techno-economic analysis of floating offshore wind farms. Appl. Energy 2021, 301, 117420. [Google Scholar] [CrossRef]
- Musial, W.; Spitsen, P.; Duffy, P.; Beiter, P.; Shields, M.; Mulas Hernando, D.; Hammond, R.; Marquis, M.; King, J.; Sathish, S. Offshore Wind Market Report: 2022 Edition; U.S Department of Energy, Office of Energy Efficiency and Renewable Energy: Washington, DC, USA, 2022.
- BBC. Jobs Lost as Wave Energy Firm Aquamarine Power Folds. BBC News 2015. Available online: https://www.bbc.com/news/uk-scotland-scotland-business-34901133 (accessed on 18 September 2023).
- Garcia-Teruel, A.; Rinaldi, G.; Thies, P.R.; Johanning, L.; Jeffrey, H. Life cycle assessment of floating offshore wind farms: An evaluation of operation and maintenance. Appl. Energy 2022, 307, 118067. [Google Scholar] [CrossRef]
- Yang, C.; Jia, J.; He, K.; Xue, L.; Jiang, C.; Liu, S.; Zhao, B.; Wu, M.; Cui, H. Comprehensive Analysis and Evaluation of the Operation and Maintenance of Offshore Wind Power Systems: A Survey. Energies 2023, 16, 5562. [Google Scholar] [CrossRef]
- Gudmestad, O.T.; Zolotukhin, A.B.; Jarlsby, E.T. Petroleum Resources with Emphasis on Offshore Fields, 1st ed.; WIT Press: Southampton, UK, 2010. [Google Scholar]
- Kausche, M.; Adam, F.; Dahlhaus, F.; Großmann, J. Floating offshore wind. Economic and ecological challenges of a TLP solution. Renew. Energy 2018, 126, 270–280. [Google Scholar] [CrossRef]
- Ishii, M.; Ueda, E.; Utsunomiya, T.; Kiyomiya, L.; Harada, B. Floating Offshore Wind Power Generation Systems off the Coast of Fukushima-Demonstration and Research Project-General Committee Report; Ministry of Economy, Trade, and Industry; Agency for Natural Resources and Energy: Tokyo, Japan, 2018.
- Dobson, A. Challenges Associated with High Voltage Dynamic Inter-Array Cables. Volume 3: Materials Technology; Pipelines, Risers, and Subsea Systems; American Society of Mechanical Engineers: Melbourne, Australia, 2023; p. V003T04A024. [Google Scholar] [CrossRef]
- Equinor. Hywind Tampen: The World’s First Renewable Power for Offshore Oil and Gas. Hywind Tampen 2022. Available online: https://www.equinor.com/energy/hywind-tampen (accessed on 9 August 2023).
- Fujii, S.; Tanaka, H. Power transmission system for “Fukushima FORWARD Project”—Power cable system for offshore floating-type wind farm pilot plant. In Proceedings of the Fall ICC Meeting, Washington, DC, USA, 9–10 December 2013. [Google Scholar]
- Edwards, E.C.; Holcombe, A.; Brown, S.; Ransley, E.; Hann, M.; Greaves, D. Evolution of floating offshore wind platforms: A review of at-sea devices. Renew. Sustain. Energy Rev. 2023, 183, 113416. [Google Scholar] [CrossRef]
- Hartman, L. Wind Turbines: The Bigger, the Better. Office of Energy Efficiency & Renewable Energy, USA. 2022. Available online: https://www.energy.gov/eere/articles/wind-turbines-bigger-better (accessed on 9 August 2023).
- The Carbon Trust. 132 kV Array Cable Requirements and The Need for Improved Testing Standards; The Carbon Trust: London, UK, 2023. [Google Scholar]
- Johanson, A.; Gjølmesli, E. Dynamic High Voltage Subsea Cables and Fatigue of their Components. In Proceedings of the Offshore Technology Conference, Houston, TX, USA, 1–4 May 2023; OTC: Houston, TX, USA, 2023. [Google Scholar] [CrossRef]
- Ikhennicheu, M.; Lynch, M.; Doole, S.; Borisade, F.; Wendt, F.; Schwarzkopf, M.A.; Matha, D.; Vicente, R.D.; Tim, H.; Ramirez, L.; et al. Review of the State of the Art of Dynamic Cable System Design; Corewind: Copenhagen, Denmark, 2020. [Google Scholar]
- Srinil, N. Cabling to connect offshore wind turbines to onshore facilities. In Offshore Wind Farms; Ng, C., Ran, L., Eds.; Woodhead Publishing: Sawston, UK, 2016; pp. 419–440. [Google Scholar] [CrossRef]
- Antunes, T.A.; Castro, R.; Santos, P.J.; Pires, A.J. Power-from-Shore Optioneering for Integration of Offshore Renewable Energy in Oil and Gas Production. Energies 2023, 17, 151. [Google Scholar] [CrossRef]
- Ringsberg, J.W.; Dieng, L.; Li, Z.; Hagman, I. Characterization of the Mechanical Properties of Low Stiffness Marine Power Cables through Tension, Bending, Torsion, and Fatigue Testing. J. Mar. Sci. Eng. 2023, 11, 1791. [Google Scholar] [CrossRef]
- Thies, P.R.; Harrold, M.; Johanning, L.; Grivas, K.; Georgallis, G. Performance Evaluation of Dynamic HV Cables with Al Conductors for Floating Offshore Wind Turbines. In Proceedings of the ASME 2019 2nd International Offshore Wind Technical Conference, St. Julian’s, Malta, 3–6 November 2019; American Society of Mechanical Engineers: St. Julian’s, Malta, 2019; p. V001T01A039. [Google Scholar] [CrossRef]
- Schnepf, A.; Lopez-Pavon, C.; Ong, M.C.; Yin, G.; Johnsen, Ø. Feasibility study on suspended inter-array power cables between two spar-type offshore wind turbines. Ocean Eng. 2023, 277, 114215. [Google Scholar] [CrossRef]
- Ahmad, I.B.; Schnepf, A.; Ong, M.C. An optimisation methodology for suspended inter-array power cable configurations between two floating offshore wind turbines. Ocean Eng. 2023, 278, 114406. [Google Scholar] [CrossRef]
- UNITECH. TetraSpar Floating Wind Turbine Pilot Installed. GCE Ocean Technology 2021. Available online: https://www.gceocean.no/news/posts/2021/august/tetraspar-floating-wind-turbine-pilot-installed/ (accessed on 15 August 2023).
- Worzyk, T. Submarine Power Cables: Design, Installation, Repair, Environmental Aspects; Springer: Berlin/Heidelberg, Germany, 2009. [Google Scholar] [CrossRef]
- Clausen, T.; D’ Souza, R. DEEPWATER E&P: Dynamic Risers’ Key Component for Deepwater Drilling, Floating Production. Offshore 2001. Available online: https://www.offshore-mag.com/deepwater/article/16759046/deepwater-ep-dynamic-risers-key-component-for-deepwater-drilling-floating-production (accessed on 10 August 2023).
- Rentschler, M.U.T.; Adam, F.; Chainho, P. Design optimization of dynamic inter-array cable systems for floating offshore wind turbines. Renew. Sustain. Energy Rev. 2019, 111, 622–635. [Google Scholar] [CrossRef]
- Thies, P.R.; Harrold, M.; Johanning, L.; Grivas, K.; Georgallis, G. Load, and fatigue evaluation for 66 kV floating offshore wind submarine dynamic power cable. In Proceedings of the Jicable’19-10th International Conference on Insulated Power Cables, Paris-Versailles, France, 23–27 June 2019. [Google Scholar]
- Zhao, S.; Cheng, Y.; Chen, P.; Nie, Y.; Fan, K. A comparison of two dynamic power cable configurations for a floating offshore wind turbine in shallow water. AIP Adv. 2021, 11, 035302. [Google Scholar] [CrossRef]
- Hung, Y.-C.; Yang, R.-Y. Experimental and Numerical Study on Suitable Configuration of Dynamical Power Cable Connected to Floating Substation in Shallow Water. SSRN 2023. Available online: https://dx.doi.org/10.2139/ssrn.4349135 (accessed on 23 April 2025).
- Zhao, S.; Wang, Y.; Chen, P. Dynamic Analysis of Multi-Wave Configurations of a Dynamic Export Cable for a Floating Wind Turbine in Shallow Water. In Proceedings of the 32nd International Ocean and Polar Engineering Conference, Shanghai, China, 5–10 June 2022; p. ISOPE-I-22-061. [Google Scholar]
- Durakovic, A. First Floating-to-Fixed Wind Turbine Connection Completed Offshore China. Offshore Wind 2021. Available online: https://www.offshorewind.biz/2021/09/02/first-floating-to-fixed-wind-turbine-connection-completed-offshore-china/ (accessed on 14 August 2023).
- Durakovic, A. Floating-to-Fixed Wind Turbine Connection Comes Online Offshore China-VIDEO. Offshore Wind 2021. Available online: https://www.offshorewind.biz/2021/12/10/floating-to-fixed-wind-turbine-connection-comes-online-offshore-china-video/ (accessed on 14 August 2023).
- Calvert, S.J.; Balash, C.; Chai, S.; Izarn, C.; Morand, H. Effects of Buoyancy Modules Geometry on Hydro-Elastic and Hydrodynamic Performance of a Steep Wave Riser in Steady Current. Volume 5A: Pipeline and Riser Technology; American Society of Mechanical Engineers: St. John’s, NL, Canada, 2015; p. V05AT04A037. [Google Scholar] [CrossRef]
- Amaechi, C.V.; Chesterton, C.; Butler, H.O.; Wang, F.; Ye, J. Review on the design and mechanics of bonded marine hoses for Catenary Anchor Leg Mooring (CALM) buoys. Ocean Eng. 2021, 242, 110062. [Google Scholar] [CrossRef]
- Taninoki, R.; Abe, K.; Sukegawa, T.; Azuma, D.; Nishikawa, M. Dynamic Cable System for Floating Offshore Wind Power Generation. SEI Tech. Rev. 2017, 190, 45–50. [Google Scholar]
- Thomson, D.; Thomson, A.; Muoniovaara, M.; Long, C.; Scuse, J. Decommissioning Penguin WEC 1 and Mooring Components-Method Statement; Orcades Marine Management Consultants Ltd.: Scotland, UK, 2020. [Google Scholar]
- Araújo, J.B.; Fernandes, A.C.; Sales, J.S., Jr.; Thurler, A.C.; Vilela, A.M. Innovative Oil Offloading System for Deep Water. In Proceedings of the Offshore Technology Conference, Houston, TX, USA, 6–9 May 2019; OTC: Houston, TX, USA, 2019; p. D022S057R006. [Google Scholar] [CrossRef]
- He, N.; Zhang, C.; Kang, Z. Analysis of Coupling Characteristics of the Offloading Buoy System in West Africa Seas. In Proceedings of the Twenty-Eighth (2018) International Ocean and Polar Engineering Conference, Sapporo, Japan, 10–15 June 2018. [Google Scholar]
- Kang, Y.; Sun, L.; Kang, Z.; Chai, S. Coupled Analysis of FPSO and CALM Buoy Offloading System in West Africa. In Proceedings of the ASME 2014 33rd International Conference on Ocean, Offshore and Arctic Engineering, San Francisco, CA, USA, 8–13 June 2014; American Society of Mechanical Engineers: New York, NY, USA, 2014. [Google Scholar] [CrossRef]
- Beier, D.; Schnepf, A.; Van Steel, S.; Ye, N.; Ong, M.C. Fatigue Analysis of Inter-Array Power Cables between Two Floating Offshore Wind Turbines Including a Simplified Method to Estimate Stress Factors. J. Mar. Sci. Eng. 2023, 11, 1254. [Google Scholar] [CrossRef]
- Beier, D. Dynamic and Fatigue Analyses of Suspended Power Cables for Multiple Floating Offshore Wind Turbines. Master’s Thesis, University of Stavanger, Stavanger, Norway, 2023. [Google Scholar]
- Schnepf, A.; Devulder, A.; Johnson, Ø.; Ong, M.C.; Lopez-Pavon, C. Numerical Investigations on Suspended Power Cable Configurations for Floating Offshore Wind Turbines in Deep Water Powering an FPSO. J. Offshore Mech. Arctic. Eng. 2023, 145, 030904. [Google Scholar] [CrossRef]
- Li, D.-R.; Su, Y.-S.; Yang, R.-Y. Experimental and Numerical Study of Suspended Inter-Array Cable Configurations for Floating Offshore Wind Farm. J. Mar. Sci. Eng. 2024, 12, 853. [Google Scholar] [CrossRef]
- Bech, A.; Storvoll, E. Marine Flexible Elongate Element and Method of Installation. US10370905B2, 6 August 2019. [Google Scholar]
- Amaechi, C.V.; Chesterton, C.; Butler, H.O.; Wang, F.; Ye, J. An Overview on Bonded Marine Hoses for Sustainable Fluid Transfer and (Un)Loading Operations via Floating Offshore Structures (FOS). J. Mar. Sci. Eng. 2021, 9, 1236. [Google Scholar] [CrossRef]
- Tan, Z.; Zhang, J.; Sheldrake, T.H.; Hou, Y.; Hill, T. Weight Added Wave (WAW) System in Production Brazil Offshore Application. In Proceedings of the Offshore Technology Conference (OTC), Houston, TX, USA, 6–9 May 2013; OnePetro: Richardson, TX, USA, 2013. [Google Scholar] [CrossRef]
- Hanonge, D.; Luppi, A. Challenges of Flexible Riser Systems in Shallow Waters. In Proceedings of the Offshore Technology Conference (OTC), Houston, TX, USA, 3–6 May 2010; OnePetro: Richardson, TX, USA, 2010; p. 14. [Google Scholar]
- Tess. Large Bore Flexible Umbilical for Aquaculture 2021. Available online: https://techtransfer.no/en/fishing-and-aquaculture/tess/ (accessed on 29 November 2023).
- Martinelli, L.; Lamberti, A.; Ruol, P.; Ricci, P.; Kirrane, P.; Fenton, C.; Johanning, L. Power Umbilical for Ocean Renewable Energy Systems-Feasibility and Dynamic Response Analysis. In Proceedings of the 3rd International Conference on Ocean Energy (ICOE), Bilbao, Spain, 6–9 October 2010; p. 8. [Google Scholar]
- Dai, T.; Sævik, S.; Ye, N. Experimental and numerical studies on dynamic stress and curvature in steel tube umbilicals. Mar. Struct. 2020, 72, 102724. [Google Scholar] [CrossRef]
- Svensson, G.; Sævik, S.; Ringsberg, J.W. Fatigue damage analysis of dynamic power cables by laboratory testing and FE analysis. In Developments in the Analysis and Design of Marine Structures, 1st ed.; CRC Press: London, UK, 2021; pp. 477–484. [Google Scholar] [CrossRef]
- Ovun. Offshore & Subsea-Buoyancy Systems and Cable Protection Solutions; Ovun AS: Åndalsnes, Norway, 2024. [Google Scholar]
- Shaukat, U.; Schnepf, A.; Giljarhus, K.E.T. Flow over a step cylinder using partially averaged Navier-Stokes equations with application towards dynamic subsea power cables. IOP Conf. Ser. Mater. Sci. Eng. 2023, 1294, 012002. [Google Scholar] [CrossRef]
- Li, L.; Gao, Z.; Moan, T. Joint Distribution of Environmental Conditions at Five European Offshore Sites for Design of Combined Wind and Wave Energy Devices. J. Offshore Mech. Arctic. Eng. 2015, 137, 031901. [Google Scholar] [CrossRef]
- Chakrabarti, S.K. (Ed.) Handbook of Offshore Engineering; Elsevier: London, UK, 2005. [Google Scholar] [CrossRef]
- Obhrai, C.; Kalvig, S.; Gudmestad, O.T. A Review of Current Guidelines and Research on Wind Modelling for the Design of Offshore Wind Turbines. In Proceedings of the Twenty-Second International Offshore and Polar Engineering Conference, Rhodes, Greece, 17–22 June 2012; OnePetro: Richardson, TX, USA, 2012. [Google Scholar]
- Bitner-Gregersen, E.M.; Toffoli, A. Occurrence of rogue sea states and consequences for marine structures. Ocean Dyn. 2014, 64, 1457–1468. [Google Scholar] [CrossRef]
- Ponce De León, S.; Guedes Soares, C. Numerical study of the effect of current on waves in the Agulhas Current Retroflection. Ocean Eng. 2022, 264, 112333. [Google Scholar] [CrossRef]
- Marechal, G.; Ardhuin, F. Surface Currents and Significant Wave Height Gradients: Matching Numerical Models and High-Resolution Altimeter Wave Heights in the Agulhas Current Region. J. Geophys. Res. Oceans 2021, 126, 1–16. [Google Scholar] [CrossRef]
- DTU Wind Energy, VORTEX, The World Bank. Offshore Wind Resource Map-Mean Wind Speed 2019. Available online: https://globalwindatlas.info (accessed on 22 November 2023).
- DTU Wind Energy, VORTEX, The World Bank. Offshore Wind Resource Map-Wind Power Density Potential 2019. Available online: https://globalwindatlas.info (accessed on 22 November 2023).
- Olagnon, M.; Quiniou-Ramus, V. Specification of Metocean Design Conditions: Recent Progress and Remaining Gaps. In Proceedings of the Thirteenth International Offshore and Polar Engineering Conference, Honolulu, HI, USA, 25–30 May 2003; OnePetro: Richardson, TX, USA, 2003. [Google Scholar]
- Yamaguchi, A.; Taki, S.; Ishihara, T. Metocean Measurement at Fukushima Offshore Site. In Proceedings of the Grand Renewable Energy 2018 Proceedings, Yokohama, Japan, 20–22 June 2018. [Google Scholar]
- Kim, S.W.; Sævik, S.; Wu, J.; Leira, B.J. Time domain simulation of marine riser vortex-induced vibrations in three-dimensional currents. Appl. Ocean Res. 2022, 120, 103057. [Google Scholar] [CrossRef]
- Santala, M. Examining Variations in West of Shetlands Current Profile Shapes in Light of Regional Oceanography. In Proceedings of the Offshore Technology Conference, Houston, TX, USA, 30 April–3 May 2012; OnePetro: Richardson, TX, USA, 2012. [Google Scholar] [CrossRef]
- Yu, S.; Wu, W.; Naess, A. Current Profile Extreme Prediction in the South China Sea Based on the EOF-ACER Method, by Considering Current Directions. IJOPE 2021, 31, 275–282. [Google Scholar] [CrossRef]
- Saetre, H.J. Acquisition of Critical Metocean Data on the Norwegian Deepwater Frontier. In Proceedings of the Offshore Technology Conference, Houston, TX, USA, 3–6 May 1999; OnePetro: Richardson, TX, USA, 1999. [Google Scholar] [CrossRef]
- McClimans, T.A.; Lønseth, L. Oscillations of frontal currents. Cont. Shelf Res. 1985, 4, 699–707. [Google Scholar] [CrossRef]
- Lima, J.A.M.; Scofano, A.M. New Oceanographic Challenges in Brazilian Deepwater Oil Fields. In Proceedings of the Offshore Technology Conference, Houston, TX, USA, 3–6 May 1999; OnePetro: Richardson, TX, USA, 1999. [Google Scholar] [CrossRef]
- Hyder, P.; Jeans, D.R.G.; Cauquil, E.; Nerzic, R. Observations and predictability of internal solitons in the northern Andaman Sea. Appl. Ocean Res. 2005, 27, 1–11. [Google Scholar] [CrossRef]
- Umehara, M.; Matsuki, S. Measurements of velocity and trajectory of water particle for internal waves in two density layers: Internal Wave Trajectory. Geophys. Res. Lett. 2011, 38, 1–7. [Google Scholar] [CrossRef]
- Felisita, A. On the Application of Steel Lazy Wave Riser for Deepwater Locations with Harsh Environments (no. 330). Ph.D. Thesis, University of Stavanger, Stavanger, Norway, 2016. [Google Scholar]
- GOA-Global Ocean Associates. An Atlas of Internal Solitary-Like Waves and Their Properties. 2004. Available online: http://internalwaveatlas.com/ (accessed on 3 January 2024).
- Marty, A.; Schoefs, F.; Soulard, T.; Berhault, C.; Facq, J.V.; Gaurier, B.; Germain, G. Effect of Roughness of Mussels on Cylinder Forces from a Realistic Shape Modelling. J. Mar. Sci. Eng. 2021, 9, 598. [Google Scholar] [CrossRef]
- Ameryoun, H. Probabilistic Modeling of Wave Actions on Jacket-Type Offshore Wind Turbines in the Presence of Marine Growth. Ph.D. Thesis, Université de Nantes, Nantes, France, 2015. [Google Scholar]
- Okubo, S.; Mitani, S.; Azumaya, O.; Yuto, H.; Niizato, H.; Kosasih, K.M.A.; Takeuchi, K.; Suzuki, H. Survey of Marine Growth on the Dynamic Cable for Floating Offshore Wind Turbine-Comparison of Survey Results and Design Values. In Proceedings of Lectures of the Japan Society of Shipbuilding and Marine Engineering No. 33; Japanese Shipbuilding and Marine Engineering Society: Tokyo, Japan, 2021. [Google Scholar]
- Tian, L.; Yin, Y.; Bing, W.; Jin, E. Antifouling Technology Trends in Marine Environmental Protection. J. Bionic Eng. 2021, 18, 239–263. [Google Scholar] [CrossRef] [PubMed]
- Gulski, E.; Anders, G.J.; Jongen, R.A.; Parciak, J.; Siemiński, J.; Piesowicz, E.; Paszkiewicz, S.; Irska, I. Discussion of the electrical and thermal aspects of offshore wind farms’ power cables’ reliability. Renew. Sustain. Energy Rev. 2021, 151, 111580. [Google Scholar] [CrossRef]
- Maksassi, Z.; Garnier, B.; Moctar, A.O.E.; Schoefs, F.; Schaeffer, E. Thermal Characterization and Thermal Effect Assessment of Biofouling around a Dynamic Submarine Electrical Cable. Energies 2022, 15, 3087. [Google Scholar] [CrossRef]
- Peyton, H.R. Arctic engineering. J. Pet. Technol. 1970, 22, 1076–1082. [Google Scholar] [CrossRef]
- Winther, S.N.; Gudmestad, O.T. Impact of and solutions to the effects of climate change for Longyearbyen, Svalbard, Norway. IOP Conf. Ser. Material Sci. Eng. 2023, 1294, 012036. [Google Scholar] [CrossRef]
- Jorgensen, J.; Griffiths, T.; McGrath, N.; Görmüş, D.; Severs, G.; Thurstan, B.; Pistani, F.; Ledoux, B. Application of STABLEpipe Method C Assessment to Determine On-Bottom Stability of a Cable Crossing in North Sea Mobile Seabed Conditions. In Proceedings of the ASME 2023 42nd International Conference on Ocean, Offshore and Arctic Engineering, vol. 8, Melbourne, Australia, 11–16 June 2023; The American Society of Mechanical Engineers (ASME): New York, NY, USA, 2023. [Google Scholar] [CrossRef]
- McGrath, N.; Griffiths, T.; Jorgensen, J.; Pistani, F.; Draper, S.; Cheng, L. Improved Reliability Assessment Methods for Subsea Cables on Rocky Seabed Using NPV Calculation. In Proceedings of the ASME 2023 42nd International Conference on Ocean, Offshore and Arctic Engineering, vol. 8, Melbourne, Australia, 11–16 June 2023; The American Society of Mechanical Engineers (ASME): New York, NY, USA, 2023. [Google Scholar] [CrossRef]
- Allianz. A Turning Point for Offshore Wind-Global Opportunities and Risk Trends; Allianz: Munich, Germany, 2023. [Google Scholar]
- Bitner-Gregersen, E.M.; Waseda, T.; Parunov, J.; Yim, S.; Hirdaris, S.; Ma, N.; Soares, C.G. Uncertainties in long-term wave modelling. Mar. Struct. 2022, 84, 103217. [Google Scholar] [CrossRef]
- Feng, X.; Tsimplis, M.N.; Yelland, M.J.; Quartly, G.D. Changes in significant and maximum wave heights in the Norwegian Sea. Glob. Planet. Chang. 2014, 113, 68–76. [Google Scholar] [CrossRef]
- Jensen, S. Taiwan May Face Fewer Typhoons—But Harsher Droughts-as the Planet Warms. Reuters 2020. Available online: https://www.reuters.com/article/us-climate-change-taiwan-typhoon-feature-idUSKBN28W00D (accessed on 24 October 2023).
- Lee, H.-Y. One-Third of Taiwan’s Corals Are Dying Due to Bleaching: Researchers. Focus Taiwan-CNA English News 2021. Available online: https://focustaiwan.tw/society/202101120010 (accessed on 23 November 2023).
- Ran, X.; Yu, Z.; Lijun, Z.; Zhou, Z.; Jiangyan, G. Reliability analysis of an umbilical under ultimate tensile load based on response surface approach. Underw. Technol. 2020, 37, 87–93. [Google Scholar] [CrossRef]
- Young, D.; Ng, C.; Oterkus, S.; Li, Q.; Johanning, L. Predicting failure of dynamic cables for floating offshore wind. In Proceedings of the RENEW 2018, Lisboa, Portugal, 8–10 October 2018. [Google Scholar]
- Rabelo, M.A.; Pesce, C.P.; Santos, C.C.P.; Ramos, R.; Franzini, G.R.; Gay Neto, A. An investigation on flexible pipes bird caging triggering. Mar. Struct. 2015, 40, 159–182. [Google Scholar] [CrossRef]
- Grivas, K.; Moraiti, A.; Georgallis, G.; Bareiro, O. D3.4-Final 72.5 kV Dynamic Cable Sample; FLOTANT: Patras, Greece, 2021. [Google Scholar]
- Nasution, F.P.; Sævik, S.; Berge, S. Experimental and finite element analysis of fatigue strength for 300 mm2 copper power conductor. Mar. Struct. 2014, 39, 225–254. [Google Scholar] [CrossRef]
- Yan, Q.; Hong, G.; Lei, Z.; Zhenqin, Y.; Juyue, L. Extreme and Fatigue Analysis of a Dynamic Subsea Power Umbilical. In Volume 5A: Pipelines, Risers, and Subsea Systems; American Society of Mechanical Engineers: Glasgow, UK, 2019; p. V05AT04A004. [Google Scholar] [CrossRef]
- Balena, R.; Williams, P. Risk-Based Structural Analysis of Frade Umbilical Cables. In Proceedings of the 20th International Congress of Mechanical Engineering COBEM 2009, Gramado, Rio Grande Do Sul, Brazil, 15–20 November 2009; Brazilian Society of Mechanical Sciences and Engineering, ABCM: Parana, Brazil, 2009. [Google Scholar]
- Land, E.; Hu, Z.; Haley, N.; Brindley, W.; Ng, C. The effects of surge underprediction on fatigue damage of floating offshore wind turbine dynamic cables. In Advances in the Analysis and Design of Marine Structures, 1st ed.; CRC Press: London, UK, 2023; pp. 503–510. [Google Scholar] [CrossRef]
- Karlsen, S.; Slora, R.; Heide, K.; Lund, S.; Eggertsen, F.; Osborg, P.A. Dynamic Deep-Water Power Cables. In Proceedings of the RAO/CIS Offshore Conference and Exhibition 2009, St. Petersburg, Russia, 15–18 September 2009; p. 10. [Google Scholar]
- Resner, L.; Paszkiewicz, S. Radial Water Barrier in Submarine Cables, Current Solutions, and Innovative Development Directions. Energies 2021, 14, 2761. [Google Scholar] [CrossRef]
- Holcombe, A.; Edwards, E.; Tosdevin, T.; Greaves, D.; Hann, M. A Comparative Study of Potential-Flow-Based Numerical Models to Experimental Tests of a Semi-Submersible Floating Wind Turbine Platform. In Proceedings of the 33rd International Ocean and Polar Engineering Conference, Ottawa, ON, Canada, 19–23 June 2023; International Society of Offshore and Polar Engineers (ISOPE): Cupertino, CA, USA, 2023. [Google Scholar]
- Robertson, A.N.; Gueydon, S.; Bachynski, E.; Wang, L.; Jonkman, J.; Alarcon, D.; Amet, E.; Beardsell, A.; Bonnet, P.; Boudet, B.; et al. OC6 Phase I: Investigating the underprediction of low-frequency hydrodynamic loads and responses of a floating wind turbine. J. Phys. Conf. Ser. 2020, 1618, 032033. [Google Scholar] [CrossRef]
- Robertson, A.N.; Wendt, F.; Jonkman, J.M.; Popko, W.; Dagher, H.; Gueydon, S.; Qvist, J.; Vittori, F.; Azcona, J.; Uzunoglu, E.; et al. OC5 Project Phase II: Validation of Global Loads of the DeepCwind Floating Semisubmersible Wind Turbine. Energy Procedia 2017, 137, 38–57. [Google Scholar] [CrossRef]
- Pinkster, J.A. Low-Frequency Phenomena Associated with Vessels Moored at Sea. Soc. Pet. Eng. J. 1975, 15, 487–494. [Google Scholar] [CrossRef]
- Bryant, M.J.; Hickok, D.D. Umbilical Failure-Mechanisms and Avoidance. In Proceedings of the Subsea Control and Data Acquisition: Proceedings of an International Conference, London, UK, 4–5 April 1990; OnePetro: Richardson, TX, USA, 1990. [Google Scholar]
- Simonsen, A. Inspection and Monitoring Techniques for Unbonded Flexible Risers and Pipelines. Master’s Thesis, University of Stavanger, Stavanger, Norway, 2014. [Google Scholar]
- Saneian, M.; Gao, Y.; Bai, Y.; Liu, T. Analysis of Subsea Umbilical Mechanical Behavior Under Simultaneous Bending, Tension and Torsion. In Proceedings of the 38th International Conference on Ocean, Offshore and Arctic Engineering; Volume 5A: Pipelines, Risers, and Subsea Systems, Glasgow, Scotland, UK, 9–14 June 2019; American Society of Mechanical Engineers (ASME): New York, NY, USA, 2019; p. V05AT04A032. [Google Scholar] [CrossRef]
- Börü, M.E. VIV Fatigue of Dynamic Power Cables Applied in Offshore Wind Turbines. Master’s Thesis, Norwegian University of Science and Technology, Torgarden, Norway, 2021. [Google Scholar]
- Elrick, P.; Venugopal, V. Vortex-Induced Vibrations of Dynamic Power Cable for Floating Wind Turbines. In Proceedings of the 42nd International Conference on Ocean, Offshore and Arctic Engineering; Volume 7: CFD & FSI, Melbourne, Australia, 11–16 June 2023; American Society of Mechanical Engineers (ASME): New York, NY, USA, 2023; p. V007T08A026. [Google Scholar] [CrossRef]
- Fuglsang, A.; Kusangaya, A.J.; Dillon-Gibbons, C.; Bauer, P.; Marcollo, H. Comparison of Vessel Motion Induced-VIV Response of Lazy-Wave and W-Shaped FOWT Power Cable Configurations. In Proceedings of the OMAE Conference, Melbourne, Australia, 12–15 June 2023; ASME: New York, NY, USA, 2023. [Google Scholar] [CrossRef]
- Lal, M.; Sebastian, A.; Rana, Y. Application of Artificial Intelligence for VIV Strake Optimization of Steel Lazy Wave Risers. In Volume 3: Materials Technology; Pipelines, Risers, and Subsea Systems; American Society of Mechanical Engineers: Hamburg, Germany, 2022; p. V003T04A035. [Google Scholar] [CrossRef]
- Yin, D.; Wu, J.; Lie, H.; Jin, J.; Passano, E.; Saevik, S.; Riemer-Sorenson, S.; Rustad, A.M.; Tognarelli, M.A.; Grytyr, G.; et al. Optimization of Hydrodynamic Coefficients for VIV Prediction. Volume 8: CFD and FSI. Virtual, online. Am. Soc. Mech. Eng. 2021, 85185, V008T08A025. [Google Scholar] [CrossRef]
- Liu, D.; Ahmad, I.; Janocha, M.J.; Nystrøm, P.; Ong, M.C. Optimization of bend stiffeners of a suspended inter-array power cable between two floating offshore wind turbines. IOP Conf. Ser. Mater. Sci. Eng. 2023, 1294, 012018. [Google Scholar] [CrossRef]
- Tang, M.; Yan, J.; Chen, J.; Yang, Z.; Yue, Q. Nonlinear analysis and multi-objective optimization for bend stiffeners of flexible riser. J. Mar. Sci. Technol. 2015, 20, 591–603. [Google Scholar] [CrossRef]
- Mainçon, P.; Longva, V. Torsion Failures in Handling Operations for Power Cables, Umbilicals and Flexible Pipes. In Proceedings of the 40th International Conference on Ocean, Offshore and Arctic Engineering; Volume 4: Pipelines, Risers, and Subsea Systems, Virtual online, 21–30 June 2021; American Society of Mechanical Engineers (ASME): New York, NY, USA, 2021; p. V004T04A003. [Google Scholar] [CrossRef]
- Okkerstrøm, L. Sensitivity Analysis of Peak Tension Loads on Subsea Power Cables during Laying Operations. Master’s Thesis, Western Norway University of Applied Sciences, Bergen, Norway, 2021. [Google Scholar]
- Okkerstrøm, L.; Gudmestad, O.T.; Pedersen, E. Simulation of peak tension loads in Subsea power cables during installation. IOP Conf. Ser. Mater. Sci. Eng. 2021, 1201, 012010. [Google Scholar] [CrossRef]
- Buljan, A. World’s First Floating Wind Farm to Undergo First Major Maintenance Campaign, Turbines to Be Towed to Norwegian Port. Offshore Wind 2024. Available online: https://www.offshorewind.biz/2024/01/15/worlds-first-floating-wind-farm-to-undergo-first-major-maintenance-campaign-turbines-to-be-towed-to-norwegian-port/ (accessed on 19 January 2024).
- Al-Sallami, O. Cables Decommissioning in Offshore Wind Farms: Environmental and Economical Perspective. Master’s Thesis, Uppsala Universitet, Uppsala, Sweden, 2021. [Google Scholar]
- ARUP, Marine Scotland. Review of Approaches and Costs of Decommissioning Offshore Wind Installations; ARUP: Scotland, UK, 2018. [Google Scholar]
- Haid, L.; Stewart, G.; Jonkman, J.; Robertson, A.; Lackner, M.; Matha, D. Simulation-Length Requirements in the Loads Analysis of Offshore Floating Wind Turbines. In Proceedings of the 32nd International Conference on Ocean, Offshore and Arctic Engineering; Volume 8: Ocean Renewable Energy, Nantes, France, 9–14 June 2013; American Society of Mechanical Engineers (ASME): New York, NY, USA, 2013; p. V008T09A091. [Google Scholar] [CrossRef]
- Pillai, A.C.; Thies, P.R.; Johanning, L. Mooring system design optimization using a surrogate-assisted multi-objective genetic algorithm. Eng. Optim. 2019, 51, 1370–1392. [Google Scholar] [CrossRef]
- Orcina Ltd. OrcaFlex 2024. Available online: https://www.orcina.com/webhelp/OrcaFlex/ (accessed on 29 February 2024).
- Taormina, B.; Bald, J.; Want, A.; Thouzeau, G.; Lejart, M.; Desroy, N.; Carlier, A. A review of potential impacts of submarine power cables on the marine environment: Knowledge gaps, recommendations, and future directions. Renew. Sustain. Energy Rev. 2018, 96, 380–391. [Google Scholar] [CrossRef]
- Merck, T.; Wasserthal, R. Assessment of the Environmental Impacts of Cables; OSPAR Commission: London, UK, 2009. [Google Scholar]
- Farr, H.; Ruttenberg, B.; Walter, R.K.; Wang, Y.-H.; White, C. Potential environmental effects of deepwater floating offshore wind energy facilities. Ocean Coast. Manag. 2021, 207, 105611. [Google Scholar] [CrossRef]
- Jakubowska-Lehrmann, M.; Białowąs, M.; Otremba, Z.; Hallmann, A.; Śliwińska-Wilczewska, S.; Urban-Malinga, B. Do magnetic fields related to submarine power cables affect the functioning of a common bivalve? Mar. Environ. Res. 2022, 179, 105700. [Google Scholar] [CrossRef]
- Scott, K.; Harsanyi, P.; Easton, B.A.A.; Piper, A.J.R.; Rochas, C.M.V.; Lyndon, A.R. Exposure to Electromagnetic Fields (EMF) from Submarine Power Cables Can Trigger Strength-Dependent Behavioral and Physiological Responses in Edible Crab, Cancer pagurus (L.). J. Mar. Sci. Eng. 2021, 9, 776. [Google Scholar] [CrossRef]
- Dunham, A.; Pegg, J.R.; Carolsfeld, W.; Davies, S.; Murfitt, I.; Boutillier, J. Effects of submarine power transmission cables on a glass sponge reef and associated megafaunal community. Mar. Environ. Res. 2015, 107, 50–60. [Google Scholar] [CrossRef]
- Westerberg, H.; Lagenfelt, I. Sub-Sea power cables and the migration behavior of the European eel. Fish. Manag. Ecol. 2008, 15, 369–375. [Google Scholar] [CrossRef]
- Garavelli, L. 2020 State of the Science Report. In Chapter 8: Encounters of Marine Animals with Marine Renewable Energy Device Mooring Systems and Subsea Cables; A report prepared by Pacific Northwest National Laboratory on behalf of the U.S. Department of Energy; U.S. Department of Energy: Washington, DC, USA, 2020. [Google Scholar] [CrossRef]
- Lin, T.-H.; Yang, R.-Y. Stability Analysis and Environmental Influence Evaluation on a Hybrid Mooring System for a Floating Offshore Wind Turbine. J. Mar. Sci. Eng. 2023, 11, 2236. [Google Scholar] [CrossRef]
- Hooper, T.; Austen, M. The co-location of offshore windfarms and decapod fisheries in the UK: Constraints and opportunities. Mar. Policy 2014, 43, 295–300. [Google Scholar] [CrossRef]
- Krone, R.; Dederer, G.; Kanstinger, P.; Krämer, P.; Schneider, C.; Schmalenbach, I. Mobile demersal megafauna at common offshore wind turbine foundations in the German Bight (North Sea) two years after deployment-increased production rate of Cancer pagurus. Mar. Environ. Res. 2017, 123, 53–61. [Google Scholar] [CrossRef] [PubMed]
- Hutchison, Z.L.; Gill, A.B.; Sigray, P.; He, H.; King, J.W. A modelling evaluation of electromagnetic fields emitted by buried subsea power cables and encountered by marine animals: Considerations for marine renewable energy development. Renew. Energy 2021, 177, 72–81. [Google Scholar] [CrossRef]
- Maxwell, S.M.; Kershaw, F.; Locke, C.C.; Conners, M.G.; Dawson, C.; Aylesworth, S.; Loomis, R.; Johnson, A.F. Potential impacts of floating wind turbine technology on marine species and habitats. J. Environ. Manag. 2022, 307, 114577. [Google Scholar] [CrossRef]
- Hirokawa, E.; Suzuki, H.; Hirabayashi, S.; Muratake, M. Estimation of Risk of Progressive Drifts in a Wind Farm Caused by Collision of Drift Ship. In Proceedings of the 34th International Conference on Ocean, Offshore and Arctic Engineering; Volume 6: Ocean Space Utilization, St. John’s, NL, Canada, 31 May–5 June 2015; American Society of Mechanical Engineers (ASME): New York, NY, USA, 2015; p. V006T05A011. [Google Scholar] [CrossRef]
- Holmås, H.E.; Dale, M.S.; Gjerde, S.S.; Holmås, H.E.; Jacobsen, A.K.; Magnus, E.; Mekki, A.; Notkevich, L.; Persen, G.; Vevatne, T.I. Hywind Tampen-Samfunnsmessige Ringvirkninger; Equinor: Stavanger, Norway, 2019. (In Norwegian) [Google Scholar]
- Kallinger, M.D.; Rapha, J.I.; Trubat Casal, P.; Domínguez-García, J.L. Offshore Electrical Grid Layout Optimization for Floating Wind; A Review. Clean. Technol. 2023, 5, 791–827. [Google Scholar] [CrossRef]
- Song, D.; Yan, J.; Gao, Y.; Wang, L.; Du, X.; Xu, Z.; Zhang, Z.; Yang, J.; Dong, M.; Chen, Y. Optimization of floating wind farm power collection system using a novel two-layer hybrid method. Appl. Energy 2023, 348, 121546. [Google Scholar] [CrossRef]
- Lerch, M.; De-Prada-Gil, M.; Molins, C. A metaheuristic optimization model for the inter-array layout planning of floating offshore wind farms. Int. J. Electr. Power Energy Syst. 2021, 131, 107128. [Google Scholar] [CrossRef]
- Lerch, M.; De-Prada-Gil, M.; Molins, C. Collection Grid Optimization of a Floating Offshore Wind Farm Using Particle Swarm Theory. J. Phys. Conf. Series. 2019, 1356, 012012. [Google Scholar] [CrossRef]
- Giassi, M.; Castellucci, V.; Göteman, M. Economic layout optimization of wave energy parks clustered in electrical subsystems. Appl. Ocean Res. 2020, 101, 102274. [Google Scholar] [CrossRef]
- Patryniak, K.; Collu, M.; Coraddu, A. Multidisciplinary design analysis and optimisation frameworks for floating offshore wind turbines: State of the art. Ocean Eng. 2022, 251, 111002. [Google Scholar] [CrossRef]
- Faraggiana, E.; Giorgi, G.; Sirigu, M.; Ghigo, A.; Bracco, G.; Mattiazzo, G. A review of numerical modelling and optimisation of the floating support structure for offshore wind turbines. J. Ocean Eng. Mar. Energy 2022, 8, 433–456. [Google Scholar] [CrossRef]
- Fylling, I.; Berthelsen, P.A. WINDOPT: An Optimization Tool for Floating Support Structures for Deep Water Wind Turbines. In Proceedings of the 30th International Conference on Ocean, Offshore and Arctic Engineering; Volume 5: Ocean Space Utilization; Ocean Renewable Energy, Rotterdam, The Netherlands, 19–24 June 2011; The American Society of Mechanical Engineers Digital Collection (ASMEDC): New York, NY, USA, 2011; pp. 767–776. [Google Scholar] [CrossRef]
- Brommundt, M.; Krause, L.; Merz, K.; Muskulus, M. Mooring System Optimization for Floating Wind Turbines using Frequency Domain Analysis. Energy Procedia 2012, 24, 289–296. [Google Scholar] [CrossRef]
- Hegseth, J.M.; Bachynski, E.E.; Martins, J.R.R.A. Integrated design optimization of spar floating wind turbines. Mar. Struct. 2020, 72, 102771. [Google Scholar] [CrossRef]
- Leimeister, M.; Kolios, A.; Collu, M.; Thomas, P. Design optimization of the OC3 phase IV floating spar-buoy, based on global limit states. Ocean Eng. 2020, 202, 107186. [Google Scholar] [CrossRef]
- Poirette, Y.; Guiton, M.; Huwart, G.; Sinoquet, D.; Leroy, J.M. An Optimization Method for the Configuration of Inter-Array Cables for Floating Offshore Wind Farm. In Proceedings of the 36th International Conference on Ocean, Offshore and Arctic Engineering; Volume 10: Ocean Renewable Energy, Trondheim, Norway, 25–30 June 2017; American Society of Mechanical Engineers: New York, NY, USA, 2017; p. 10. [Google Scholar] [CrossRef]
- Schnepf, A.; Giljarhus, K.E.T.; Johnsen, Ø.; Lopez-Pavon, C. Dynamic Power Cable Configuration Design for Floating Offshore Wind Turbines Using Gradient-Based Optimization. In Proceedings of the 42nd International Conference on Ocean, Offshore and Arctic Engineering (OMAE); Volume 8: Ocean Renewable Energy, Melbourne, Australia, 11–16 June 2023; American Society of Mechanical Engineers (ASME): New York, NY, USA, 2023; p. 11. [Google Scholar] [CrossRef]
- Schnepf, A. Design Optimisation of Power Cable Configurations for Floating Offshore Wind Turbines. Ph.D. Thesis, University of Stavanger, Stavanger, Norway, 2024. [Google Scholar]
- Schnepf, A.; Giljarhus, K.E.T. Efficient Global Optimization of dynamic power cable configurations for floating offshore wind turbines. Offshore Mech. Arctic Eng. 2024. (submitted). [Google Scholar]
- Nehme, R.; Dethoor, X. Oil Offloading Lines and Fiber Optic Cable Package. Presented at the Offshore Technology Conference, Houston, TX, USA, 4–7 May 2015; 2015; p. OTC-25885-MS. [Google Scholar] [CrossRef]
- Cunliffe, N.; McCarthy, T.J. Application of Genetic Algorithms for the Automated Design of Offshore Riser Systems. In Proceedings of the Seventh International Conference on the Application of Artificial Intelligence to Civil and Structural Engineering, Egmond-Aan-Zee, The Netherlands, 2–4 September 2003; p. 35. [Google Scholar] [CrossRef]
- Vieira, L.T. Projeto Ótimo de Risers via Algoritmos Genéticos (Optimal Riser Design via Genetic Algorithms). Ph.D. Thesis, COPPE/UFRJ, Rio de Janeiro, Brazil, 2004. [Google Scholar]
- Vieira, L.T. Otimização de Sistemas de Risers para Exploração de Petróleo Offshore Através de Algoritmos Genéticos Paralelos (Optimization of Riser Systems for Offshore Oil Exploration Using Parallel Genetic Algorithms). Ph.D. Thesis, Universidade Federal do Rio de Janeiro, COPPE, Rio de Janeiro, Brazil, 2008. [Google Scholar]
- Laddé, S. Multi-Objective Optimization of Steel Lazy Wave Riser Design Through Genetic Algorithms. Master’s Thesis, TU Delft, Delft, The Netherlands, 2011. [Google Scholar]
- Cardoso, P.H.D.S. Otimização da Configuração Geométrica de Risers Rígidos (Optimization of the Geometric Configuration of Rigid Risers). Master’s Thesis, Pontifícia Universidade Católica do Rio de Janeiro, Rio de Janeiro, Brazil, 2019. [Google Scholar] [CrossRef]
- Vieira, I.N.; de Lima, B.S.L.P.; Jacob, B.P. Bio-inspired algorithms for the optimization of offshore oil production systems. Int. J. Numer. Methods Eng. 2012, 91, 1023–1044. [Google Scholar] [CrossRef]
- Vieira, I.N.; Silva, A.J.M.; Lima, B.; Jacob, B.P. A Comparative Study Applied to Risers Optimization Using Bio-Inspired Algorithms. Int. J. Model. Simul. Pet. Ind. 2009, 3, 12. [Google Scholar]
- Wang, A.; Yang, H.; Li, H. Multi-objective Optimization for Dynamic Umbilical Installation Using Non-Dominated Sorting Genetic Algorithm. In Proceedings of the 30th International Conference on Ocean, Offshore and Arctic Engineering (OMAE); Volume 4: Pipeline and Riser Technology, Rotterdam, The Netherlands, 19–24 June 2011; American Society of Mechanical Engineers (ASME): New York, NY, USA, 2011; pp. 121–129. [Google Scholar] [CrossRef]
- Yang, H.; Wang, A.; Li, H. Multi-objective optimization for deepwater dynamic umbilical installation analysis. Sci. China Phys. Mech. Astron. 2012, 55, 1445–1453. [Google Scholar] [CrossRef]
- Yang, Z.; Yan, J.; Sævik, S.; Lu, Q.; Ye, N.; Chen, J.; Yue, Q. Integrated optimisation design of a dynamic umbilical based on an approximate model. Mar. Struct. 2021, 78, 102995. [Google Scholar] [CrossRef]
- Yan, J.; Su, Q.; Li, R.; Xu, J.; Lu, Q.; Yang, Z. Optimization Design Method of the Umbilical Cable Global Configuration Based on Representative Fatigue Conditions. IEEE J. Ocean. Eng. 2023, 48, 188–198. [Google Scholar] [CrossRef]
- Riggs, H.R. Automated Preliminary Design of Flexible Riser Configurations. In Proceedings of the Eighth International Conference on Offshore Mechanics and Arctic Engineering, The Hague, the Netherlands, 19–23 March 1989; American Society of Mechanical Engineers: New York, NY, USA, 1989; pp. 503–510. [Google Scholar]
- Riggs, H.R.; Leraand, T. Efficient Static Analysis and Design of Flexible Risers. J. Offshore Mech. Arctic Eng. 1991, 113, 235–240. [Google Scholar] [CrossRef]
- Larsen, C.M.; Hanson, T. Optimization of Catenary Risers. J. Offshore Mech. Arctic Eng. 1999, 121, 90–94. [Google Scholar] [CrossRef]
- Vieira, L.T.; de Lima Bde, S.L.P.; Evsukoff, A.G.; Jacob, B.P. Application of Genetic Algorithms to the Synthesis of Riser Configurations. In Proceedings of the 22nd International Conference on Offshore Mechanics and Arctic Engineering, Cancun, Mexico, 8–13 June 2003; Offshore Technology; Ocean Space Utilization. American Society of Mechanical Engineers (ASME): New York, NY, USA, 2003; Volume 1, pp. 391–396. [Google Scholar] [CrossRef]
- Cunliffe, N.; Baxter, C.; McCarthy, T.; Trim, A. Evolutionary Design of Marine Riser Systems. In Proceedings of the 23rd International Conference on Offshore Mechanics and Arctic Engineering, Parts A and B, Vol. 1, Vancouver, BC, Canada, 20–25 June 2004; American Society of Mechanical Engineers: New York, NY, USA, 2004; pp. 733–739. [Google Scholar] [CrossRef]
- de Rodrigues, G.J.O. Ferramentas Computacionais para Otimização e Síntese de Sistemas Híbridos de Risers Baseados no Conceito de Bóia de Subsuperfície (Computational Tools for the Optimisation and Synthesis of Hybrid Risers Based on the Subsurface Buoy Concept). Ph.D. Thesis, Universidade Federal do Rio de Janeiro, COPPE, Rio de Janeiro, Brazil, 2004. [Google Scholar]
- Roveri, F.E.; de Arruda Martins, C.; Balena, R. Parametric Analysis of a Lazy-Wave Steel Riser. In Proceedings of the 24th International Conference on Offshore Mechanics and Arctic Engineering: Volume 1, Parts A and B, Halkidiki, Greece, 12–17 June 2005; The American Society of Mechanical Engineers Digital Collection (ASMEDC): New York, NY, USA, 2005; pp. 289–295. [Google Scholar] [CrossRef]
- de Lima, B.S.L.P.; Jacob, B.P.; Ebecken, N.F.F. A hybrid fuzzy/genetic algorithm for the design of offshore oil production risers. Int. J. Numer. Meth. Eng. 2005, 64, 1459–1482. [Google Scholar] [CrossRef]
- Tanaka, R.L.; de Martins, C.A. A Genetic Algorithm Approach to Steel Riser Optimization. In Proceedings of the 25th International Conference on Offshore Mechanics and Arctic Engineering, Hamburg, Germany, 28 May–2 June 2006; Offshore Technology; Offshore Wind Energy; Ocean Research Technology; LNG Specialty Symposium. American Society of Mechanical Engineers (ASME): New York, NY, USA, 2006; Volume 1, pp. 271–277. [Google Scholar] [CrossRef]
- Tanaka, R.L.; de Martins, C.A.; de Takafuji, F.C.M. Steel riser optimization with simulated annealing. In Proceedings of the 13th Rio Oil & Gas Expo and Conference, Rio de Janeiro, Brazil, 11–15 September 2006. [Google Scholar]
- Tanaka, R.L.; de Martins, C.A. Dynamic Optimization of Steel Risers. In Proceedings of the Sixteenth (2007) International Offshore and Polar Engineering Conference, Lisbon, Portugal, 1–6 July 2007; The International Society of Offshore and Polar Engineers (ISOPE): Cupertino, CA, USA, 2007. [Google Scholar]
- Vieira, I.N.; de Lima, B.S.L.P.; Jacob, B.P. Optimization of Steel Catenary Risers for Offshore Oil Production Using Artificial Immune System. In Artificial Immune Systems; Bentley, P.J., Lee, D., Jung, S., Eds.; Springer: Berlin/Heidelberg, Germany, 2008; pp. 254–265. [Google Scholar]
- Yang, H.; Jiang, R.; Li, H. Optimization Design of Deepwater Steel Catenary Risers Using Genetic Algorithm. Computational Structural Engineering; Springer: Dordrecht, The Netherlands, 2009. [Google Scholar] [CrossRef]
- Tanaka, R.L. Otimização da configuração de risers rígidos (Optimising the Configuration of Rigid Risers). Doutorado em Engenharia de Controle e Automação Mecânica, Universidade de São Paulo, São Paulo, Brazil, 2009. [CrossRef]
- Andrade, E.Q.d.; Aguiar, L.L.d.; Senra, S.F.; Siqueira, E.F.N.; Torres, A.L.F.L.; Mourelle, M.M. Optimization Procedure of Steel Lazy Wave Riser Configuration for Spread Moored FPSOs in Deepwater Offshore Brazil. In Proceedings of the Offshore Technology Conference (OTC), Houston, TX, USA, 3–6 May 2010; p. OTC-20777-MS. [Google Scholar] [CrossRef]
- de Pina, A.A.; Albrecht, C.H.; de Lima, B.S.L.P.; Jacob, B.P. Tailoring the particle swarm optimization algorithm for the design of offshore oil production risers. Optim. Eng. 2011, 12, 215–235. [Google Scholar] [CrossRef]
- de Pina, A.A.; de Lima, B.S.L.P.; Albrecht, C.H.; Jacob, B.P. Parameter Selection and Convergence Analysis of the PSO Algorithm Applied to the Design of Risers. In Proceedings of the EngOpt 2008-International Conference on Engineering Optimization, Rio de Janeiro, Brazil, 1–5 June 2008. [Google Scholar]
- Zheng, W.; Yang, H. Dynamic Optimization of Steel Catenary Risers Based on Reliability Using Metamodel; American Society of Mechanical Engineers Digital Collection, New York, NY, USA, 2010; pp. 223–228. [CrossRef]
- Yang, H.Z.; Zheng, W. Metamodel approach for reliability-based design optimization of a steel catenary riser. J. Mar. Sci. Technol. 2011, 16, 202–213. [Google Scholar] [CrossRef]
- Tanaka, R.L.; Martins, C.d.A. Parallel Dynamic Optimization of Steel Risers. J. Offshore Mech. Arctic Eng. 2011, 133, 011302. [Google Scholar] [CrossRef]
- Arruda, M.D.S.V.; de Martins, M.A.L.; Lages, E.N. Otimização dos flutuadores de risers com catenária em configuração complexa (Optimization of complex configuration of catenary risers buoyancy modules). Semin. Ciências Exatas E Tecnol. 2012, 33, 149–164. [Google Scholar] [CrossRef][Green Version]
- de Martins, M.A.L. Otimização da Configuração de Risers Verticais Complacentes (Compliant Vertical Access Risers Optimization). Master’s Thesis, Universidade Federal de Alagoas, Maceió, Brazil, 2011. [Google Scholar]
- de Martins, M.A.L.; Lages, E.N.; Silveira, E.S.S. Compliant vertical access riser assessment: DOE analysis and dynamic response optimization. Appl. Ocean Res. 2013, 41, 28–40. [Google Scholar] [CrossRef]
- Voie, P.E.; Sødahl, N. Optimisation of Steel Catenary Risers. In Proceedings of the 32nd International Conference on Ocean, Offshore and Arctic Engineering; Volume 4A: Pipeline and Riser Technology, Nantes, France, 9–15 June 2013; American Society of Mechanical Engineers: New York, NY, USA, 2013; p. V04AT04A011. [Google Scholar] [CrossRef]
- Nariño, G.A.R.; Martha, L.F.; de Menezes, I.F.M. Otimização de Risers em Catenária com Amortecedores Hidrodinâmicos (Optimization of Catenary Risers with Hydrodynamic Dampers). In Proceedings of the XXXV Iberian Latin-American Congress on Computational Methods in Engineering, Fortaleza, CE, Brazil, 23–26 November 2014; Pontifícia Universidade Católica do Rio de Janeiro: Rio de Janeiro, Brazil, 2014. [Google Scholar] [CrossRef]
- Chen, J.; Yan, J.; Yang, Z.; Yue, Q.; Tang, M. Flexible Riser Configuration Design for Extremely Shallow Water with Surrogate-Model-Based Optimization. J. Offshore Mech. Arctic Eng. 2016, 138, 041701. [Google Scholar] [CrossRef]
- Yang, Z.; Yan, J.; Sævik, S.; Zhen, L.; Ye, N.; Chen, J.; Yue, Q. Multi-Objective Optimization Design of Flexible Risers Based on Bi-Scale Response Surface Models. In Proceedings of the 37th International Conference on Ocean, Offshore and Arctic Engineering (OMAE); Volume 5: Pipelines, Risers, and Subsea Systems, Madrid, Spain, 17–22 June 2018; American Society of Mechanical Engineers: New York, NY, USA, 2018; p. V005T04A018. [Google Scholar] [CrossRef]
- Diakonova, E.; Bonnet, G.; Brouard, Y. SLWR Innovative Design Methodology Supporting Concept Engineering Through to Project Cost Optimisation. In Proceedings of the Offshore Technology Conference, Houston, TX, USA, 6–9 May 2019; p. D021S018R004. [Google Scholar] [CrossRef]
- Cardoso, P.H.S.; Casaprima, N.A.G.; Senhora, F.V.; Menezes, I.F.M. Optimization of catenary risers with hydrodynamic dampers. Ocean Eng. 2019, 184, 134–142. [Google Scholar] [CrossRef]
- Ai, S.; Xu, Y.; Kang, Z.; Yan, F. Performance comparison of stress-objective and fatigue-objective optimisation for steel lazy wave risers. Ships Offshore Struct. 2019, 14, 534–544. [Google Scholar] [CrossRef]
- Bhowmik, S.; Noiray, G.; Naik, H. Riser Design Automation with Machine Learning. In Proceedings of the Abu Dhabi International Petroleum Exhibition & Conference, Abu Dhabi, United Arab Emirates, 11–14 November 2019; SPE Society of Petroleum Engineers: Richardson, TX, USA, 2019; p. D041S106R003. [Google Scholar] [CrossRef]
- Bhowmik, S.; Naik, H.; Noiray, G. Lazy Wave Steel Catenary Riser Design Optimisation in Digital Field Twin. In Proceedings of the Offshore Technology Conference Asia, Kuala Lumpur, Malaysia, 2–6 November 2020; OnePetro: Richardson, TX, USA, 2020; p. D012S001R074. [Google Scholar] [CrossRef]
- Lal, M.; Sebastian, A.; Wang, F.; Lu, X. Steel Lazy Wave Riser Optimization Using Artificial Intelligence Tool. In Proceedings of the 39th International Conference on Ocean, Offshore and Arctic Engineering, Virtual, Online, 3–7 August 2020; American Society of Mechanical Engineers (ASME): New York, NY, USA, 2020; Volume 4. [Google Scholar] [CrossRef]
- Ogbeifun, A.M.; Oterkus, S.; Race, J.; Naik, H.; Moorthy, D.; Bhowmik, S.; Ingram, J. A tabular optimisation technique for steel lazy wave riser. IOP Conf. Ser. Mater. Sci. Eng. 2021, 1052, 012022. [Google Scholar] [CrossRef]
- Lal, M.; Sebastian, A.; Rana, Y. Steel Lazy Wave Riser Optimization Using Artificial Neural Networks and Genetic Algorithm. In Proceedings of the International Conference on Offshore Mechanics and Arctic Engineering, Volume 4: Pipelines, Risers, and Subsea Systems, Virtual, Online, 21–30 June 2021; American Society of Mechanical Engineers: New York, NY, USA, 2021; p. V004T04A034. [Google Scholar] [CrossRef]
- Elsas, J.H.; Casaprima, N.A.G.; Cardoso, P.H.S.; Menezes, I.F.M. Bayesian optimization of riser configurations. Ocean Eng. 2021, 236, 109402. [Google Scholar] [CrossRef]
- Abam, J.T.; Pu, Y.; Hu, Z. Cost-Effective Optimal Solutions for Steel Catenary Riser Using Artificial Neural Network. In Volume 3: Materials Technology; Pipelines, Risers, and Subsea Systems; American Society of Mechanical Engineers: Hamburg, Germany, 2022; p. V003T04A031. [Google Scholar] [CrossRef]
- Abam, J.T.; Pu, Y.; Hu, Z. Weight Optimisation of Steel Catenary Riser Using Genetic Algorithm and Finite Element Analysis; American Society of Mechanical Engineers Digital Collection: Hamburg, Germany, 2022; p. 9. [Google Scholar] [CrossRef]
- Lal, M.; Yenduri, A.; Sebastian, A. Steel Lazy Wave Riser Optimization Using Particle Swarm Optimization Algorithm. In Volume 3: Materials Technology; Pipelines, Risers, and Subsea Systems; American Society of Mechanical Engineers: Melbourne, Australia, 2023; p. V003T04A048. [Google Scholar] [CrossRef]
- Jakobsen, E.A. Performance Efficiency and Reliability Analysis of Offshore Wind Power Plants: Case Study of Utsira Nord. Master’s Thesis, NTNU, Trondheim, Norway, 2023. [Google Scholar]
- Petrie, H.E.; Eide, C.H.; Haflidason, H.; Watton, T. A conceptual geological model for offshore wind sites in former ice stream settings: The Utsira Nord site, North Sea. JGS 2022, 179, jgs2021-163. [Google Scholar] [CrossRef]
- Cheynet, E.; Li, L.; Jiang, Z. Metocean Conditions at Two Norwegian Sites for Development of Offshore Wind Farms. Renew. Energy 2024, 224, 120184. [Google Scholar] [CrossRef]
- Reistad, M.; Magnusson, A.K.; Haver, S.; Gudmestad, O.T.; Kvamme, D. How severe wave conditions are possible on the Norwegian Continental Shelf? Mar. Struct. 2005, 18, 428–450. [Google Scholar] [CrossRef]
- Liang, G.; Jiang, Z.; Merz, K. Dynamic analysis of a dual spar floating offshore wind farm with shared moorings in extreme environmental conditions. Mar. Struct. 2023, 90, 103441. [Google Scholar] [CrossRef]
- Asplin, L.; Albretsen, J.; Johnsen, I.A.; Sandvik, A.D. The hydrodynamic foundation for salmon lice dispersion modeling along the Norwegian coast. Ocean Dyn. 2020, 70, 1151–1167. [Google Scholar] [CrossRef]
- Jackson, C.R.; Alpers, W. North Sea and Skagerrak Strait; Office of Naval Research: Alexandria, VA, USA, 2004. [Google Scholar]
- Petrie, H.E. An Integrated Geological Characterization of Marine Ground Conditions in the North Sea. Ph.D. Thesis, The University of Bergen, Bergen, Norway, 2023. [Google Scholar]
- Hansen, T.D. Utsira Nord Floating Wind Farm–Optimalisation of Marine Operations Related to Inter-Array Cable Installation. Master’s Thesis, University of Stavanger, Stavanger, Norway, 2023. [Google Scholar]
- von Schlanbusch, F. Driving Factors for Levelized Cost of Energy in Floating Wind Farms. Master’s Thesis, The University of Bergen, Bergen, Norway, 2022. [Google Scholar]
- Jodar Hernandez, P. Optimization of Marine Operations for the Assembly and Installation of a New Floating Wind Concept. Master’s Thesis, University of Stavanger, Stavanger, Norway, 2023. [Google Scholar]
- Høines, I. Modelling Analysis of Maintenance Logistics Optimization for a Floating Wind Park: A Case Study for Utsira Nord. Master’s Thesis, University of Stavanger, Stavanger, Norway, 2022. [Google Scholar]
- Austreim, A.H. Hvilke drift og vedlikeholds oppgaver må gjennomføres under vann på den flytende vindparken Utsira Nord, og hva vil være kostnaden? (Which Operation and Maintenance Tasks Must Be Carried Out Underwater at the Utsira Nord Floating Wind Farm, and What Will the Cost Be?). Master’s Thesis, University of Stavanger, Stavanger, Norway, 2023. [Google Scholar]
- Olsson, E.S. Potential Conflicts in Offshore Wind Development–a Qualitative Study of Utsira Nord; Norwegian University of Life Sciences, Aas, Norway, 2021.
- Wang, W. Significance of Offshore Wind Farm Sound on Marine Populations. Master’s Thesis, NTNU, Trondheim, Norway, 2019. [Google Scholar]
- Tjøsvoll, S.E.G. What Socioeconomic Dynamics Will Offshore Wind Affect? A Regional Case Study of Utsira North. Master’s Thesis, University of Stavanger, Stavanger, Norway, 2022. [Google Scholar]
- Anda, L.M. Communication and Stakeholder Management in Renewable Energy Development; Case Study Utsira Nord Floating Wind Offshore Energy Development. Master’s Thesis, University of Stavanger, Stavanger, Norway, 2022. [Google Scholar]
- Oliveira, R.V. Whispers in the Wind: Ethical dimensions of social conflict in offshore wind. J. Phys. Conf. Ser. 2023, 2626, 012070. [Google Scholar] [CrossRef]
- Flermoen, P. Motvind eller medvind for norske havvindutbygginger (Headwind or Downwind for Norwegian Offshore Wind Developments). Master’s Thesis, NTNU, Trondheim, Norway, 2023. (In Norwegian). [Google Scholar]
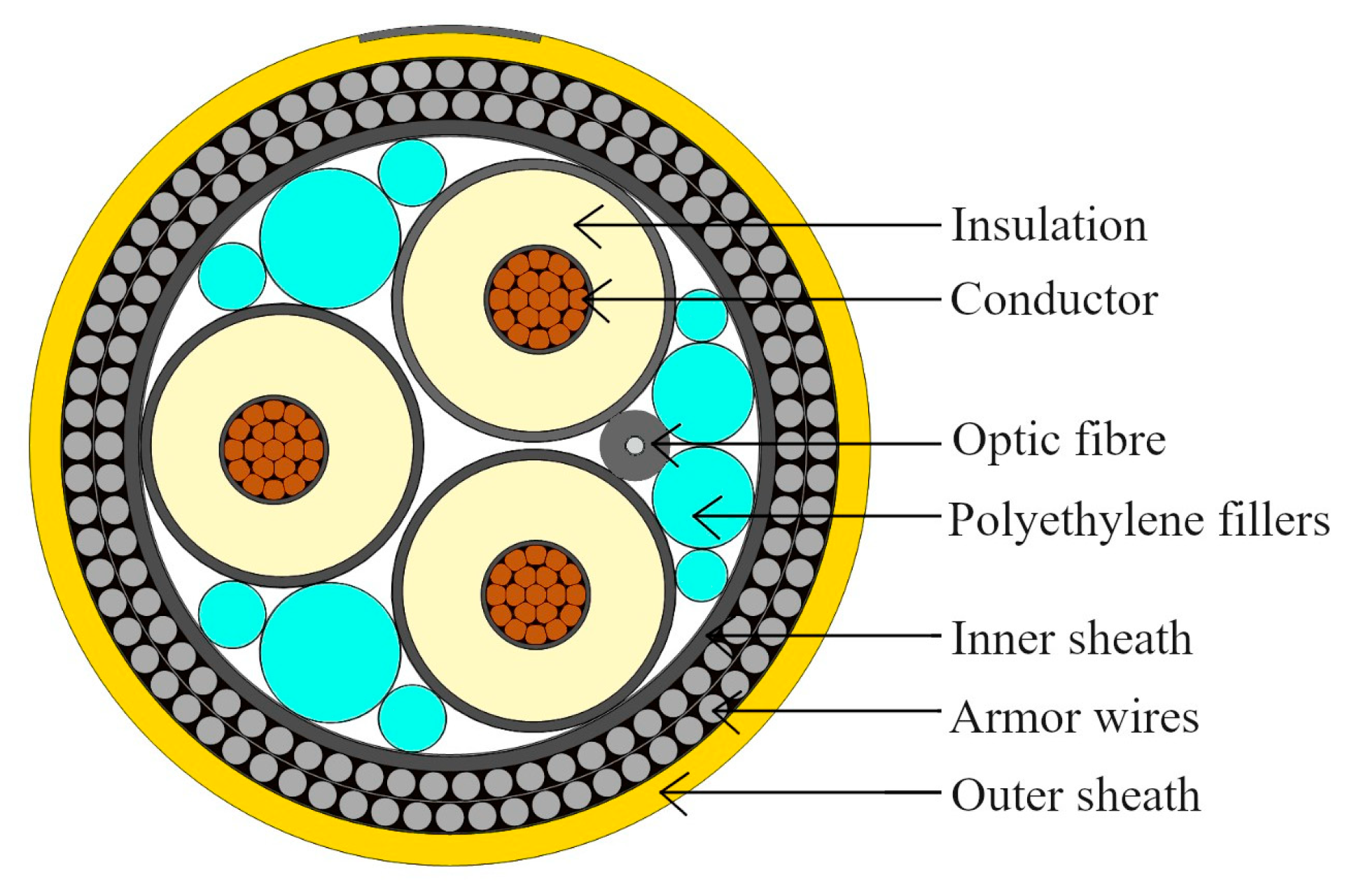
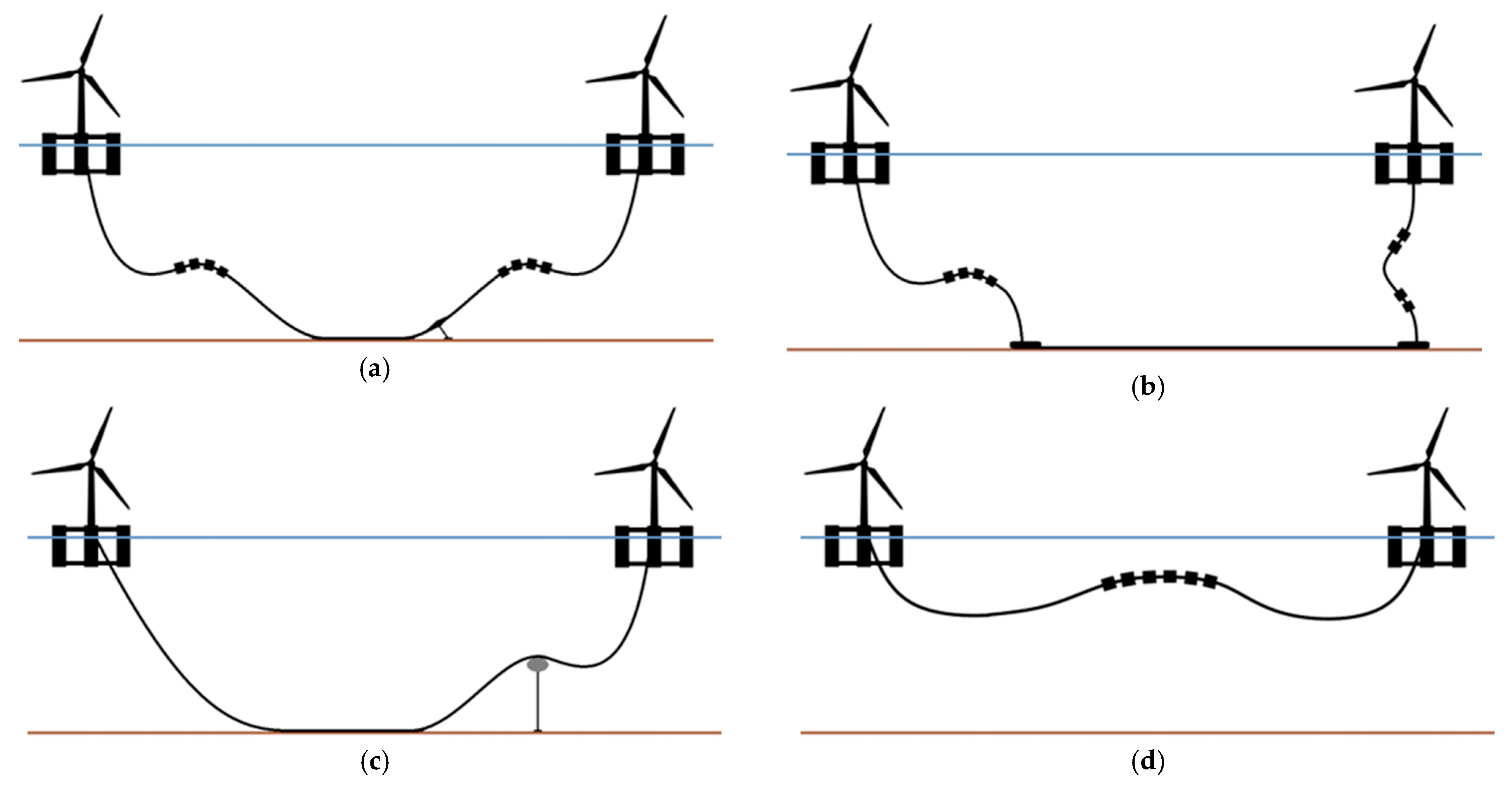
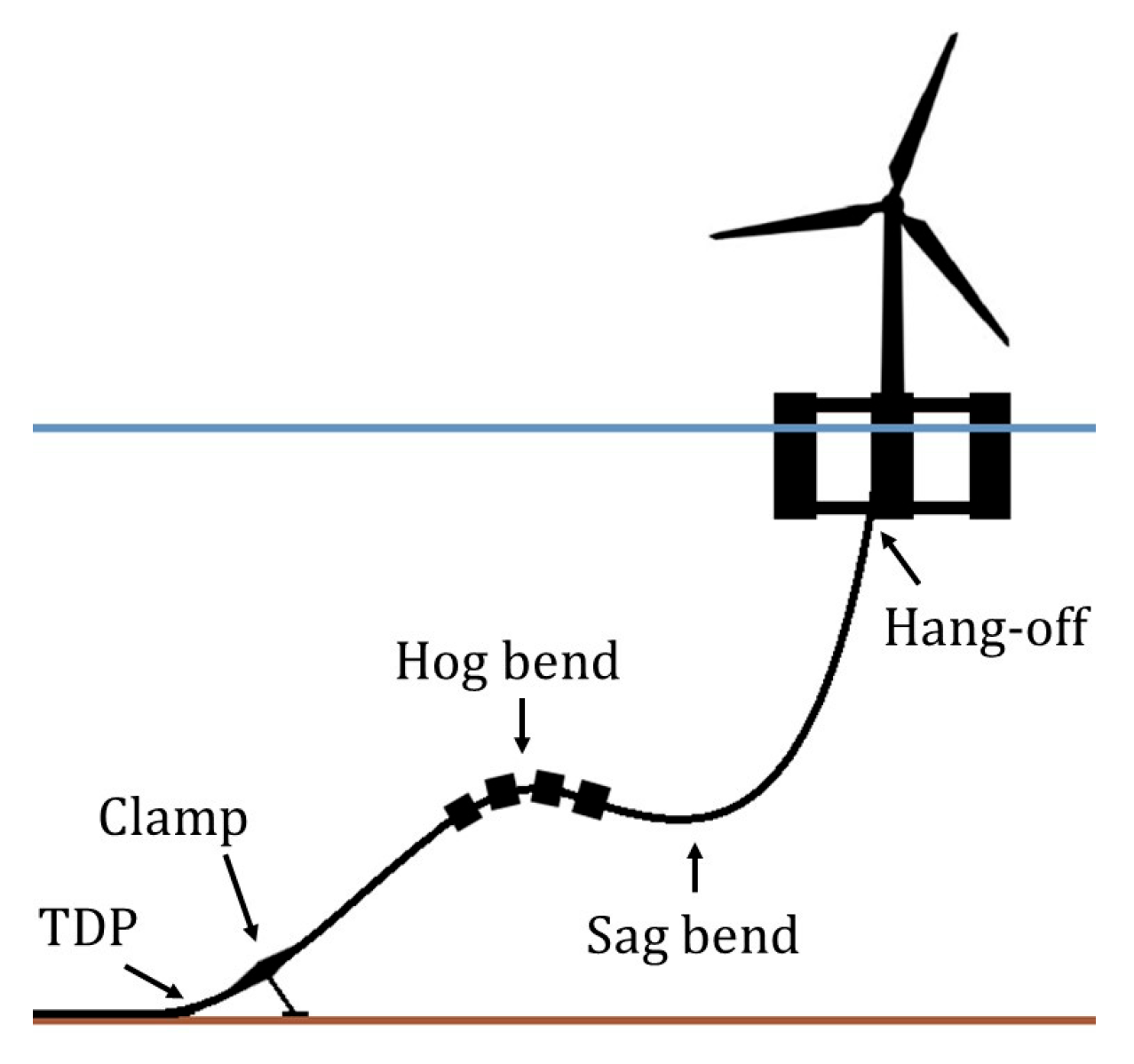
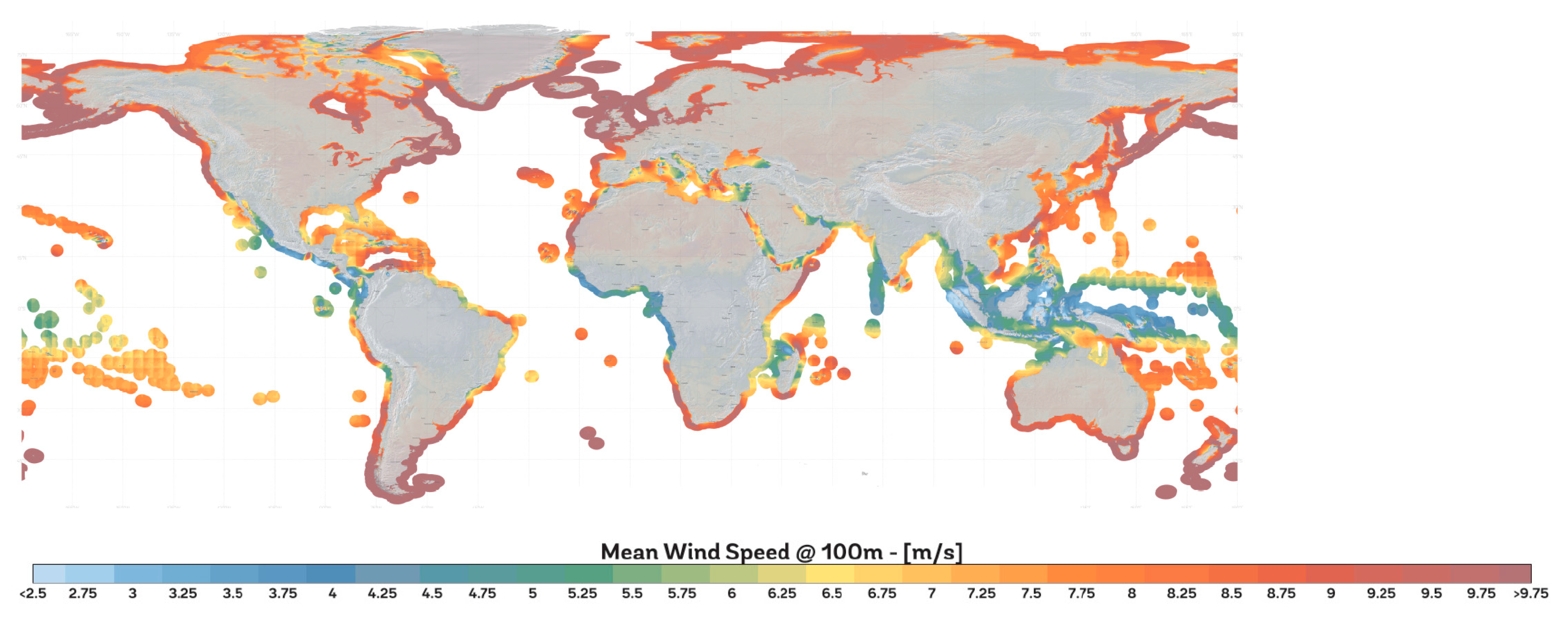
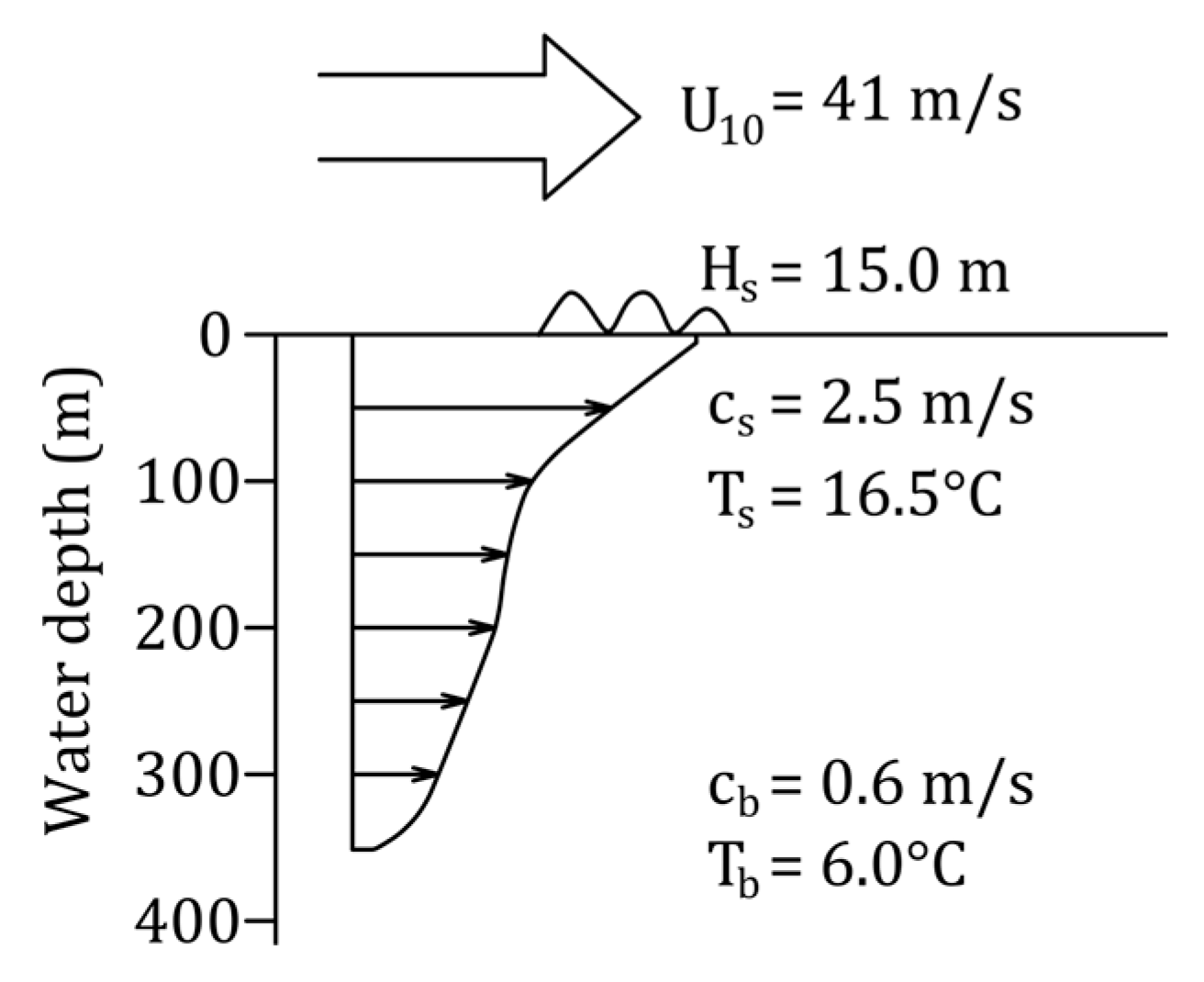
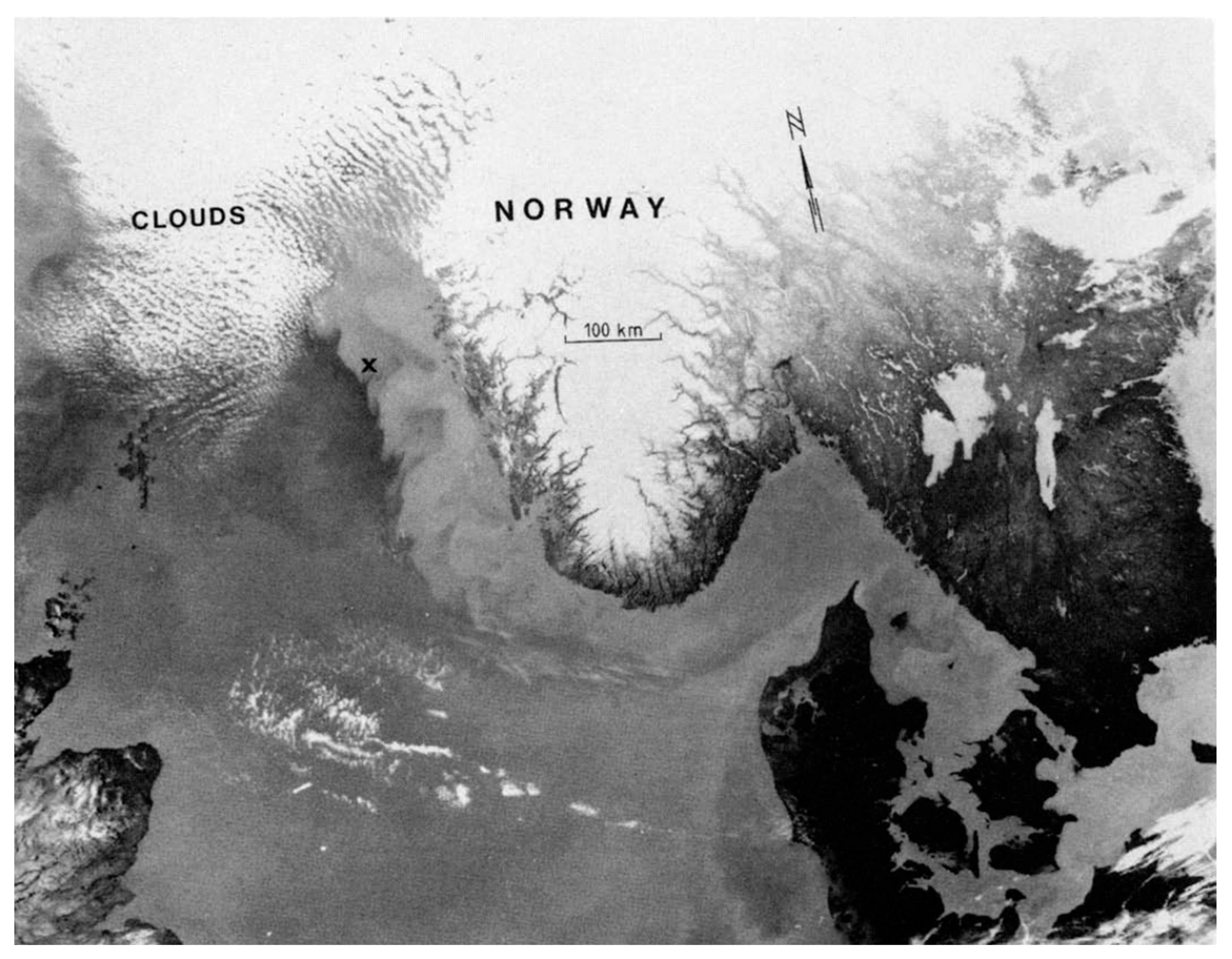
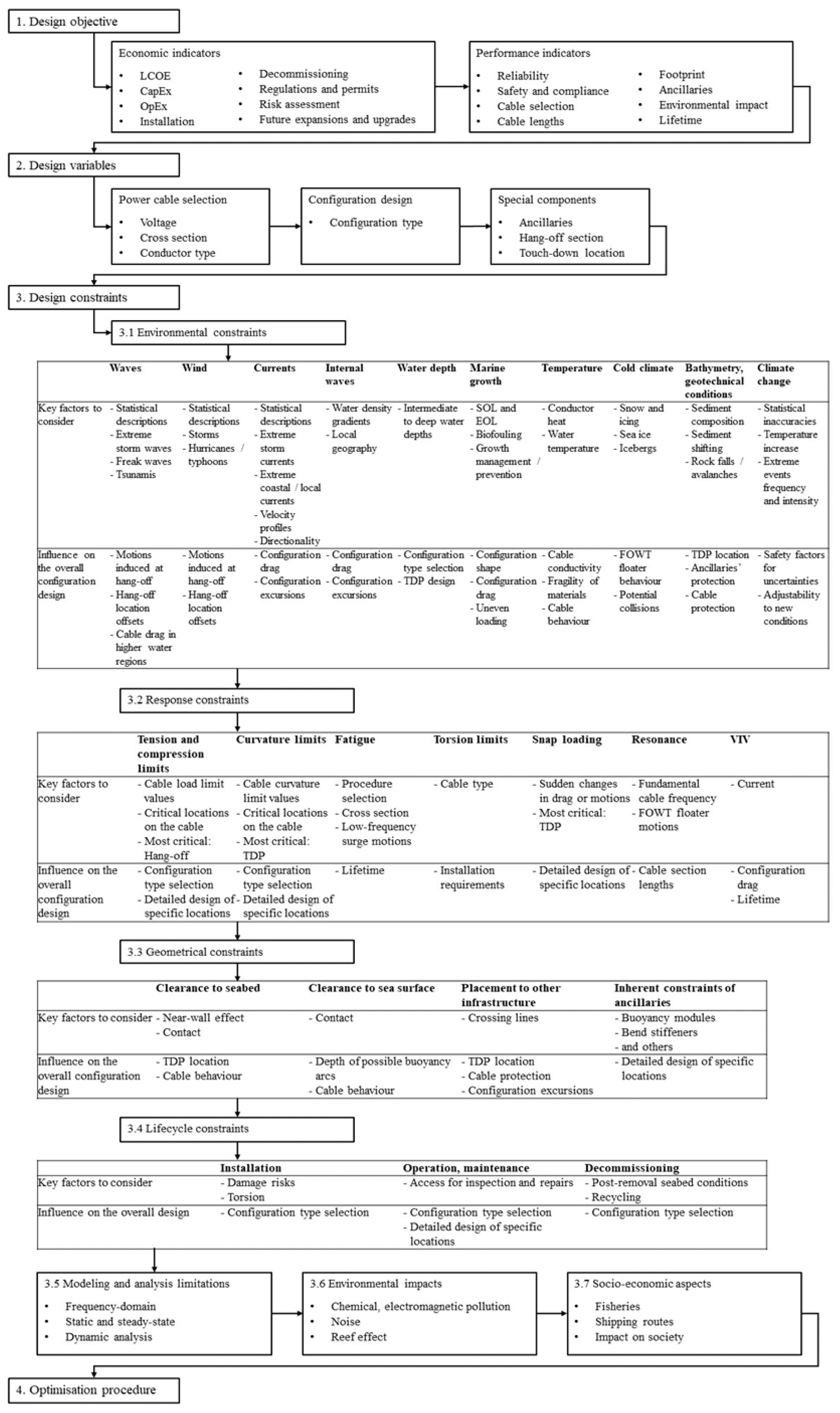
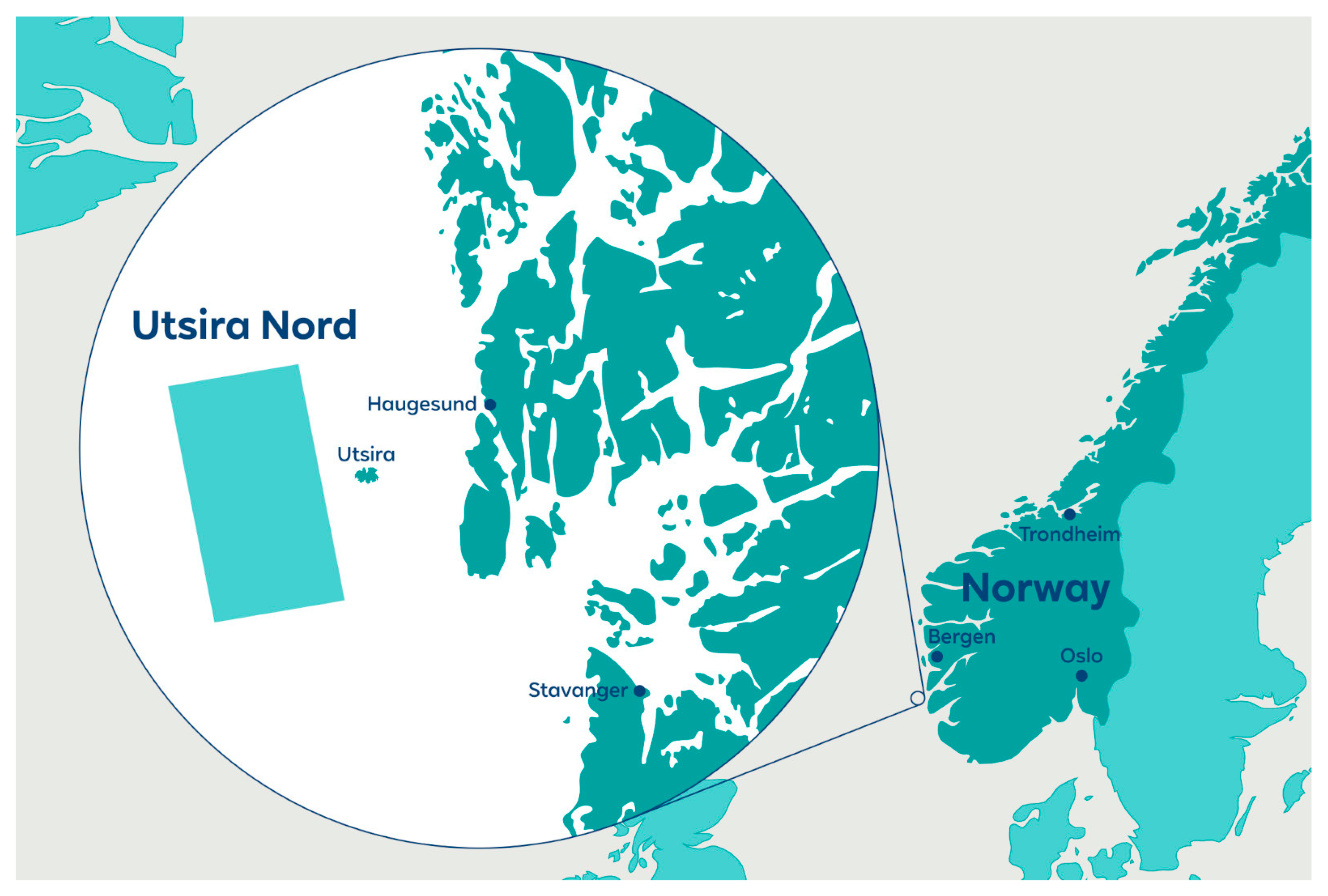
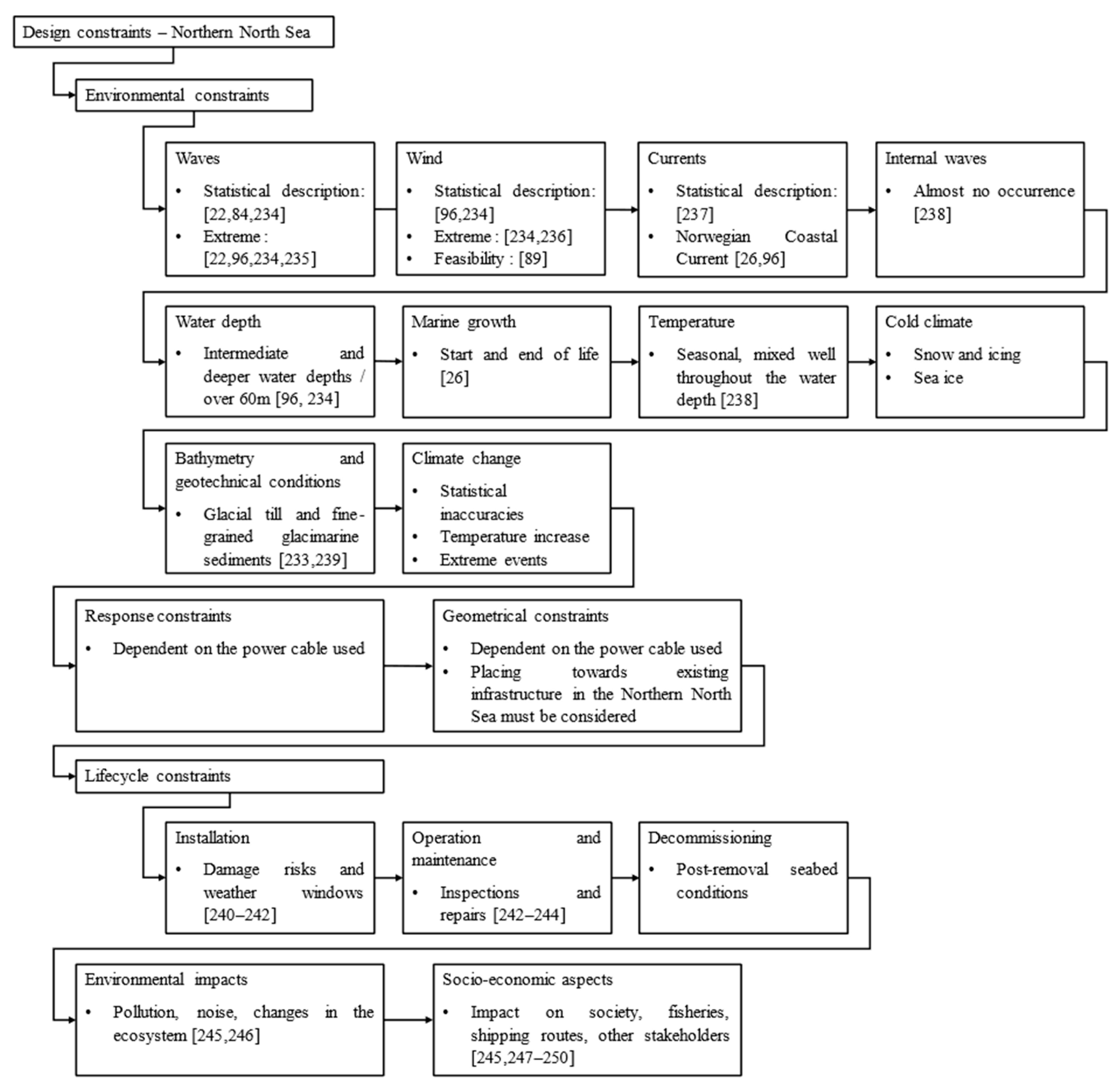
| Reference | Fylling and Berthelsen 2012 [173] | Poirette et al., 2017 [177] | Rentschler et al., 2019 [55] | Ahmad et al., 2023 [51] |
|---|---|---|---|---|
| Optimization technique | Non-Linear Programming by Quadratic Lagrangian (NLPQL) algorithm | Derivative-free Sequential Quadratic Approximation (SQA) algorithm | Genetic algorithm (GA) | Fitness criteria |
| Objective function | Minimize the material costs of spar, mooring, and cable | Minimize the material costs of power cable configuration | Minimize cable section lengths, buoyancy section lengths | Fittest configuration considering cable tension, curvature, and length |
| Design variables | Cable and mooring fairlead vertical position, line direction, pretension, section lengths and diameters; weight of possible buoy; FOWT spar height, diameter, damper plate diameter | Cable and buoyancy section length, cable and buoyancy diameter, length, and diameter of the base of the bend stiffener at hang-off | Cable length, buoyancy section lengths, and positions | Cable length, attached subsea buoy number, and spacing |
| Cable max. curvature, tension, min. static horizontal pretension; mooring max. tension, min. fatigue life, static horizontal pretension; FOWT max. inclination, max. draught, min. and max. heave and pitch period, max. nacelle acceleration | Cable max. allowable curvature, min. and max. tension, max. elongation | Cable max. accumulated fatigue damage, max. allowable curvature, min. breaking load | Cable max. effective tension, min. bend radius |
| Cable and mooring max. horizontal offset; mooring max. slope angle at the anchor | Cable min. seabed clearance, min. sea surface clearance; bounded location of the buoyancy section | Cable seabed clearance; hang-off section dependent on simulation setup | Cable min. seabed clearance, max. horizontal excursion |
| Multidirectional conditions with wave spectrum, wind spectrum, wind profile, and constant current (no current profile provided) | Unidirectional ultimate limit states (ULS) with wave spectrum, constant wind speed, wind profile, and constant current throughout depth | Multidirectional conditions with wave spectrum, constant wind speed throughout height | Unidirectional load cases with wave spectrum, wind spectrum, wind profile, and constant current profile |
| Modeling and analysis method | Quasi-static mooring line analysis: frequency domain | Finite Element Analysis (FEA), dynamic | Finite Element Analysis (FEA), dynamic | Finite Element Analysis (FEA); steady-state (static), dynamic |
| Cable configuration type | Lazy wave | Lazy wave | Lazy wave | Suspended |
| Other components included? | Yes: FOWT spar, mooring | No | No | No |
| Reference | Schnepf et al., 2023 [178] | Schnepf and Giljarhus [179,180] | ||
| Optimization technique | Sequential Least Squares Programming (SLSQP) algorithm | Efficient Global Optimization (EGO) | ||
| Objective function | Minimize cable section lengths, buoyancy section length | Minimize cable bending | ||
| Design variables | Cable length, buoyancy section length, and position | Cable section lengths, buoyancy section length, and tether location | ||
| Response constraints | Cable min. and max. tension, tether min. and max. tension | Min. and max. tether tension | ||
| Geometrical constraints | Cable min. and max. section lengths, min. and max. curvature, min. and max. hang-off angles, min. seabed clearance | Cable hang-off angle, seabed clearance | ||
| Environmental constraints | Unidirectional load cases with wave spectrum, wind spectrum, wind profile, and constant current profile | Unidirectional constant current profile, hang-off location displacement | ||
| Modeling and analysis method | Finite Element Analysis (FEA), steady-state (static) | Finite Element Analysis (FEA), steady-state (static) | ||
| Cable configuration type | Tethered-wave | Tethered-wave | ||
| Other components included? | No | No | ||
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Schnepf, A.; Gudmestad, O.T. Key Parameters for Design Analysis and Optimization of Dynamic Inter-Array Power Cable Configurations in Floating Offshore Wind Farms. J. Mar. Sci. Eng. 2025, 13, 875. https://doi.org/10.3390/jmse13050875
Schnepf A, Gudmestad OT. Key Parameters for Design Analysis and Optimization of Dynamic Inter-Array Power Cable Configurations in Floating Offshore Wind Farms. Journal of Marine Science and Engineering. 2025; 13(5):875. https://doi.org/10.3390/jmse13050875
Chicago/Turabian StyleSchnepf, Anja, and Ove Tobias Gudmestad. 2025. "Key Parameters for Design Analysis and Optimization of Dynamic Inter-Array Power Cable Configurations in Floating Offshore Wind Farms" Journal of Marine Science and Engineering 13, no. 5: 875. https://doi.org/10.3390/jmse13050875
APA StyleSchnepf, A., & Gudmestad, O. T. (2025). Key Parameters for Design Analysis and Optimization of Dynamic Inter-Array Power Cable Configurations in Floating Offshore Wind Farms. Journal of Marine Science and Engineering, 13(5), 875. https://doi.org/10.3390/jmse13050875








