An LES Investigation of Flow Field Around the Cuboid Artificial Reef at Different Angles of Attack
Abstract
1. Introduction
2. Methodology
3. Results and Discussions
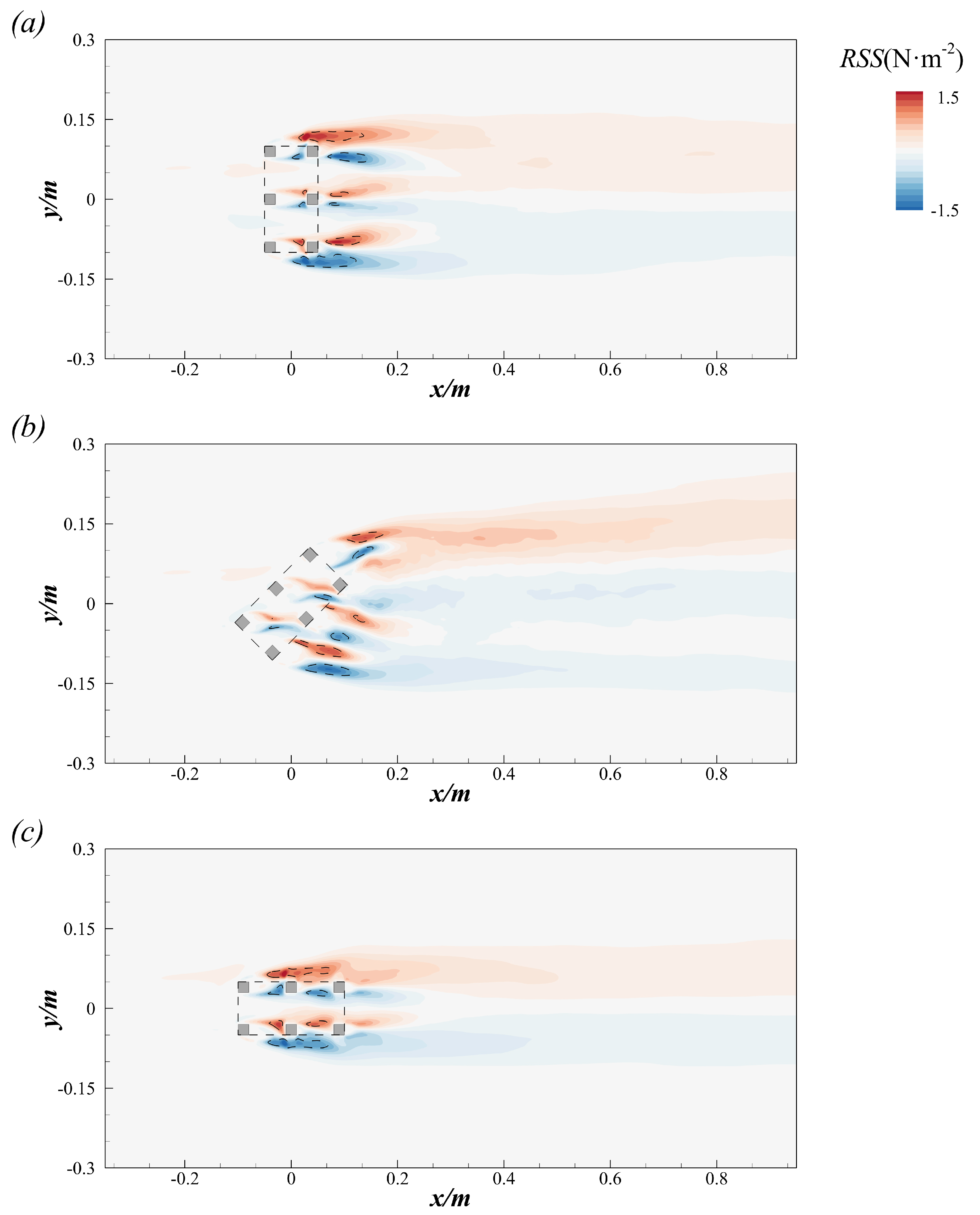
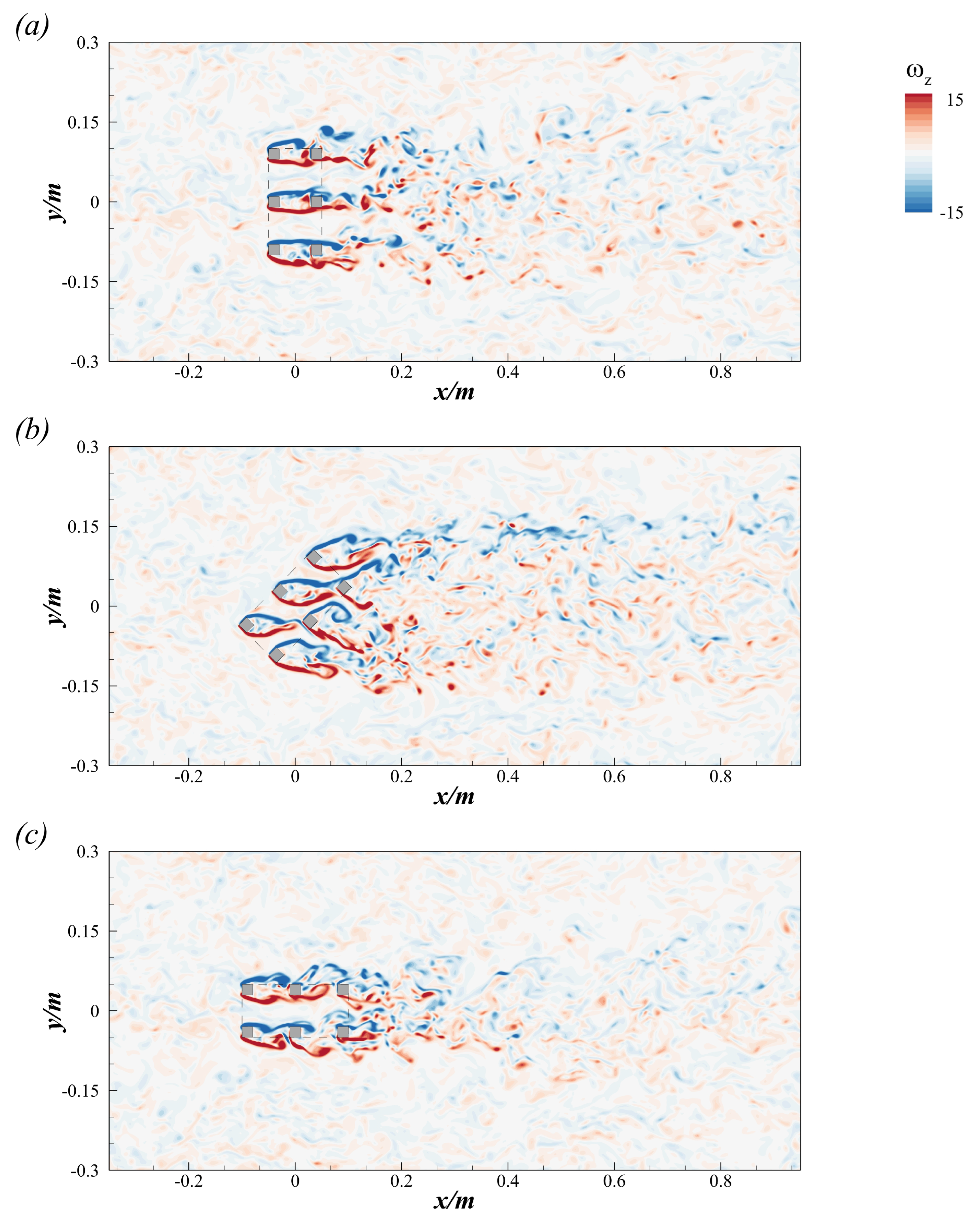
3.1. Time-Averaged Flow and Flow Separation
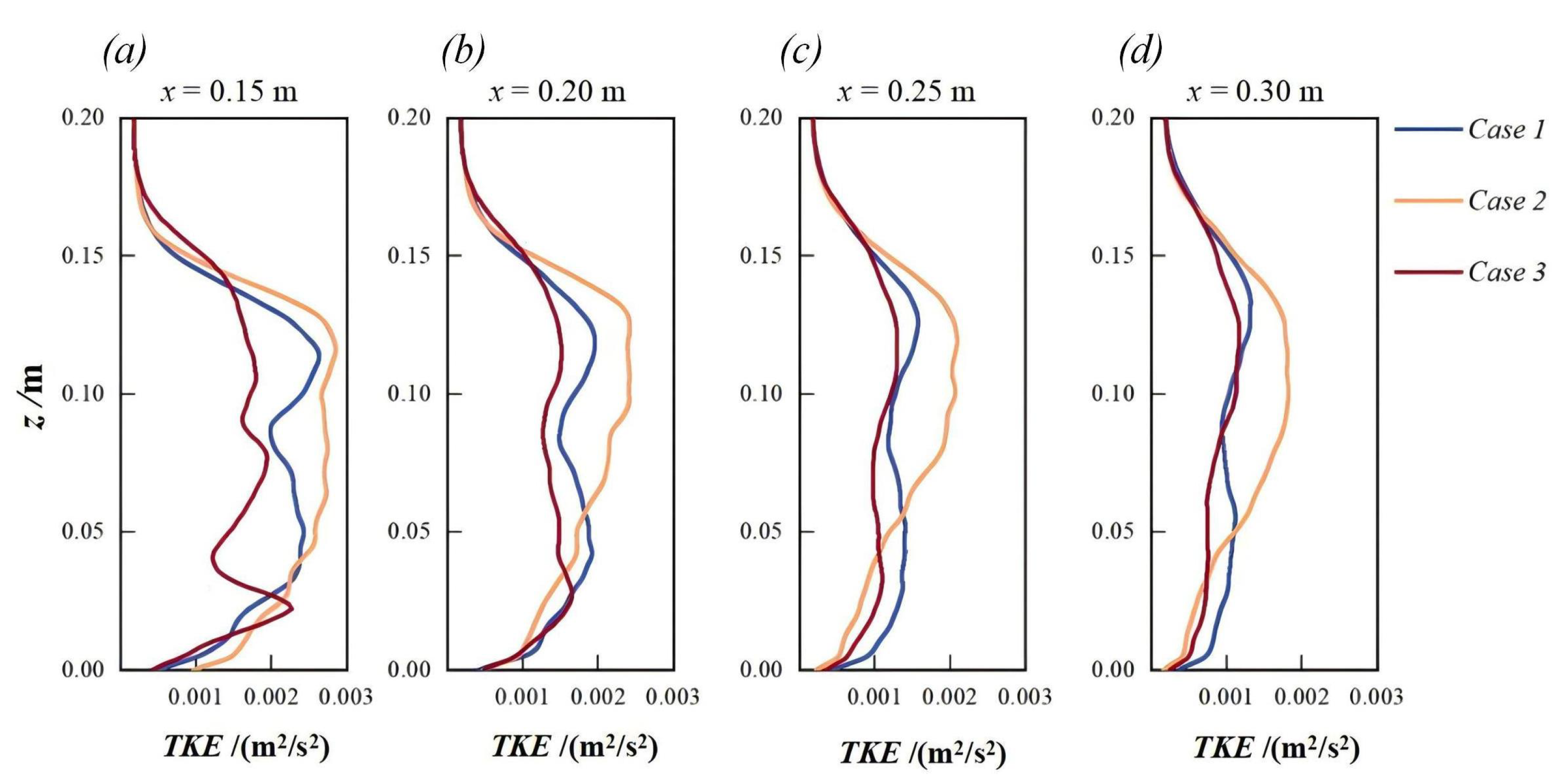
3.2. Turbulence Parameters
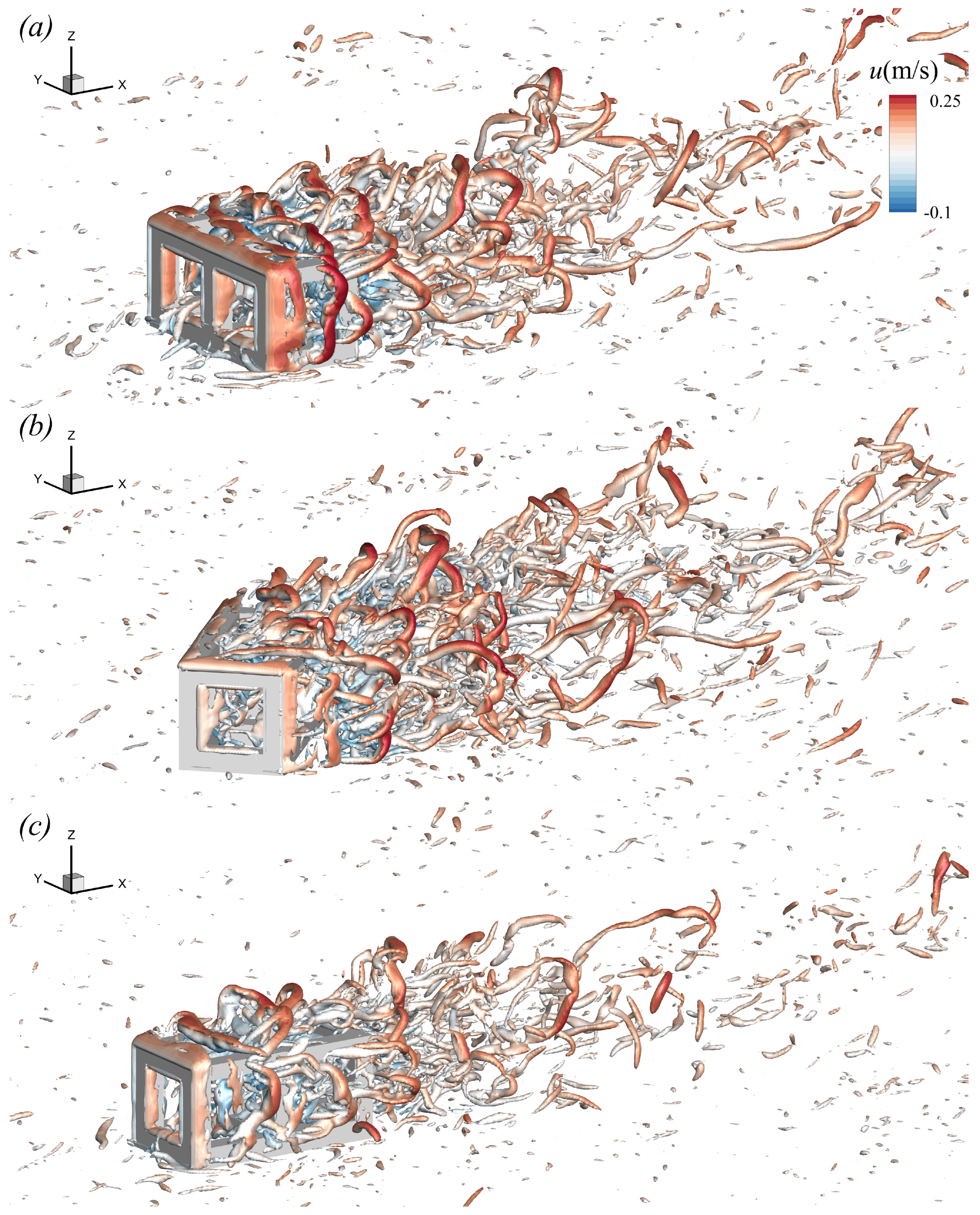
3.3. Instantaneous Flow Structures
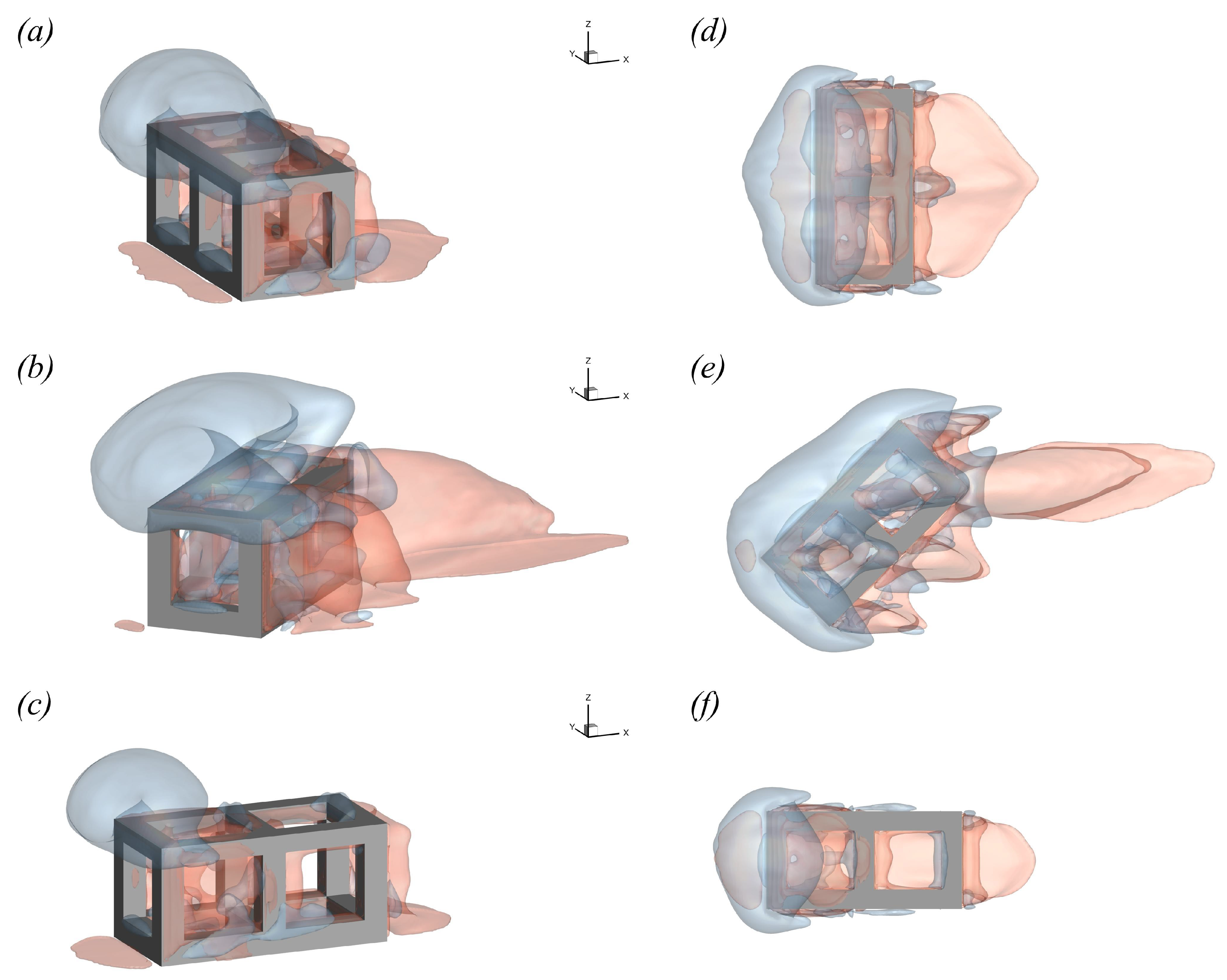
3.4. Upwelling and Wake Region Parameters
4. Conclusions
- (1)
- Time-averaged velocity distribution and streamline plots indicated that flow separation occurs inside and downstream of the CAR. Case 2 has the maximum length of the recirculation bubble, 2.5 times and 2.9 times greater than that of Case 1 and Case 3, respectively.
- (2)
- The high-level region of the Reynolds shear stress () is located where flow separation occurs. This region extends the most in both the streamwise and spanwise directions when the inflow angle is 45°. Additionally, Case 2 exhibits higher levels of turbulence kinetic energy () compared with Case 1 and Case 3 at most vertical locations downstream of the CAR.
- (3)
- The instantaneous flow around the CAR is characterized by significant shear layers prevailing from the leading edge of square cylinders. Isosurfaces of the Q-criterion reveal various types of turbulent structures, including torn horseshoe vortices and hairpin vortices, with the latter formed from the rollers shed by the shear layer.
- (4)
- Case 2, with an inflow angle of , exhibits the largest volumes for both the upwelling and wake regions. Compared with Case 2, and in Case 1 () decrease by approximately 35% and 15%, respectively. Case 3 () shows the smallest volumes for both regions, with reductions of approximately 64% and 21% for and , respectively, compared with Case 2.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Au, D. The application of histo-cytopathological biomarkers in marine pollution monitoring: A review. Mar. Pollut. Bull. 2004, 48, 817–834. [Google Scholar] [CrossRef] [PubMed]
- Hoegh-Guldberg, O. Coral reef ecosystems and anthropogenic climate change. Reg. Environ. Change 2011, 11, 215–227. [Google Scholar] [CrossRef]
- Wu, W.; Yang, Z.; Tian, B.; Huang, Y.; Zhou, Y.; Zhang, T. Impacts of coastal reclamation on wetlands: Loss, resilience, and sustainable management. Estuar. Coast. Shelf Sci. 2018, 210, 153–161. [Google Scholar] [CrossRef]
- Guo, X.; Fan, N.; Zheng, D.; Fu, C.; Wu, H.; Zhang, Y.; Song, X.; Nian, T. Predicting impact forces on pipelines from deep-sea fluidized slides: A comprehensive review of key factors. Int. J. Mining Sci. Technol. 2024, 34, 211–225. [Google Scholar] [CrossRef]
- Lee, M.O.; Otake, S.; Kim, J.K. Transition of artificial reefs (ARs) research and its prospects. Ocean Coast. Manag. 2018, 154, 55–65. [Google Scholar] [CrossRef]
- Lima, J.S.; Zalmon, I.R.; Love, M. Overview and trends of ecological and socioeconomic research on artificial reefs. Mar. Environ. Res. 2019, 145, 81–96. [Google Scholar] [CrossRef]
- Bull, A.S.; Love, M.S. Worldwide oil and gas platform decommissioning: A review of practices and reefing options. Ocean Coast. Manag. 2019, 168, 274–306. [Google Scholar] [CrossRef]
- Simon, T.; Pinheiro, H.T.; Joyeux, J.C. Target fishes on artificial reefs: Evidences of impacts over nearby natural environments. Sci. Total Environ. 2011, 409, 4579–4584. [Google Scholar] [CrossRef] [PubMed]
- Walles, B.; Troost, K.; van den Ende, D.; Nieuwhof, S.; Smaal, A.C.; Ysebaert, T. From artificial structures to self-sustaining oyster reefs. J. Sea Res. 2016, 108, 1–9. [Google Scholar] [CrossRef]
- Paxton, A.B.; Peterson, C.H.; Taylor, J.C.; Adler, A.M.; Pickering, E.A.; Silliman, B.R. Artificial reefs facilitate tropical fish at their range edge. Commun. Biol. 2019, 2, 168. [Google Scholar] [CrossRef] [PubMed]
- Liu, T.L.; Su, D.T. Numerical analysis of the influence of reef arrangements on artificial reef flow fields. Ocean Eng. 2013, 74, 81–89. [Google Scholar] [CrossRef]
- Kim, D.; Woo, J.; Na, W.B. Intensively stacked placement models of artificial reef sets characterized by wake and upwelling regions. Mar. Technol. Soc. J. 2017, 51, 60–70. [Google Scholar] [CrossRef]
- Santiago Caamaño, L.; Lamas Galdo, M.I.; Carballo, R.; López, I.; Cartelle Barros, J.J.; Carral, L. Numerical and experimental analysis of the velocity field inside an artificial reef. Application to the Ares-Betanzos estuary. J. Mar. Sci. Eng. 2022, 10, 1827. [Google Scholar] [CrossRef]
- Liu, Y.; Zhao, Y.P.; Dong, G.H.; Guan, C.T.; Cui, Y.; Xu, T.J. A study of the flow field characteristics around star-shaped artificial reefs. J. Fluids Struct. 2013, 39, 27–40. [Google Scholar] [CrossRef]
- Jiao, L.; Yan-Xuan, Z.; Pi-Hai, G.; Chang-Tao, G. Numerical simulation and PIV experimental study of the effect of flow fields around tube artificial reefs. Ocean Eng. 2017, 134, 96–104. [Google Scholar] [CrossRef]
- Wu, J.; Zhang, S.; Sun, M.; Chen, Y. Experiment on the distribution of different artificial reef models for Paralichthys olivaceus. Mar. Fish. 2004, 26, 394–398. [Google Scholar]
- Kim, D.; Woo, J.; Yoon, H.S.; Na, W.B. Efficiency, tranquillity and stability indices to evaluate performance in the artificial reef wake region. Ocean Eng. 2016, 122, 253–261. [Google Scholar] [CrossRef]
- Wang, X.; Liu, X.; Tang, Y.; Zhao, F.; Luo, Y. Numerical analysis of the flow effect of the menger-type artificial reefs with different void space complexity indices. Symmetry 2021, 13, 1040. [Google Scholar] [CrossRef]
- Kim, D.; Woo, J.; Yoon, H.S.; Na, W.B. Wake lengths and structural responses of Korean general artificial reefs. Ocean Eng. 2014, 92, 83–91. [Google Scholar] [CrossRef]
- Maslov, D.; Pereira, E.; Duarte, D.; Miranda, T.; Ferreira, V.; Tieppo, M.; Cruz, F.; Johnson, J. Numerical analysis of the flow field and cross section design implications in a multifunctional artificial reef. Ocean Eng. 2023, 272, 113817. [Google Scholar] [CrossRef]
- Shu, A.; Qin, J.; Rubinato, M.; Sun, T.; Wang, M.; Wang, S.; Wang, L.; Zhu, J.; Zhu, F. An experimental investigation of turbulence features induced by typical artificial M-shaped unit reefs. Appl. Sci. 2021, 11, 1393. [Google Scholar] [CrossRef]
- Jiang, Z.; Liang, Z.; Zhu, L.; Liu, Y. Numerical simulation of effect of guide plate on flow field of artificial reef. Ocean Eng. 2016, 116, 236–241. [Google Scholar] [CrossRef]
- Liao, J.C. A review of fish swimming mechanics and behaviour in altered flows. Philos. Trans. R. Soc. B Biol. Sci. 2007, 362, 1973–1993. [Google Scholar] [CrossRef]
- Fang, H.; Liu, Y.; Stoesser, T. Influence of boulder concentration on turbulence and sediment transport in open-channel flow over submerged boulders. J. Geophys. Res. Earth Surf. 2017, 122, 2392–2410. [Google Scholar] [CrossRef]
- Muhawenimana, V.; Wilson, C.; Ouro, P.; Cable, J. Spanwise cylinder wake hydrodynamics and fish behavior. Water Resour. Res. 2019, 55, 8569–8582. [Google Scholar] [CrossRef]
- Yusuf, S.N.A.; Asako, Y.; Sidik, N.A.C.; Mohamed, S.B.; Japar, W.M.A.A. A short review on rans turbulence models. CFD Lett. 2020, 12, 83–96. [Google Scholar]
- Zhao, C.; Ouro, P.; Stoesser, T.; Dey, S.; Fang, H. Response of flow and saltating particle characteristics to bed roughness and particle spatial density. Water Resour. Res. 2022, 58, e2021WR030847. [Google Scholar] [CrossRef]
- Jiang, Z.; Liang, Z.; Liu, Y.; Tang, Y.; Huang, L. Particle image velocimetry and numerical simulations of the hydrodynamic characteristics of an artificial reef. Chin. J. Oceanol. Limnol. 2013, 31, 949–956. [Google Scholar] [CrossRef]
- Kim, D.; Woo, J.; Na, W.B.; Yoon, H.S. Flow and structural response characteristics of a box-type artificial reef. J. Korean Soc. Coast. Ocean Eng. 2014, 26, 113–119. [Google Scholar] [CrossRef]
- Kim, D.; Jung, S.; Na, W.B. Evaluation of turbulence models for estimating the wake region of artificial reefs using particle image velocimetry and computational fluid dynamics. Ocean Eng. 2021, 223, 108673. [Google Scholar] [CrossRef]
- Jalalabadi, R.; Stoesser, T.; Ouro, P.; Luo, Q.; Xie, Z. Free surface flow over square bars at different Reynolds numbers. J. Hydro-Environ. Res. 2021, 36, 67–76. [Google Scholar] [CrossRef]
- He, K.; Su, X.; Gao, G.; Krajnović, S. Evaluation of LES, IDDES and URANS for prediction of flow around a streamlined high-speed train. J. Wind Eng. Ind. Aerodyn. 2022, 223, 104952. [Google Scholar] [CrossRef]
- Sheidani, A.; Salavatidezfouli, S.; Stabile, G.; Rozza, G. Assessment of URANS and LES methods in predicting wake shed behind a vertical axis wind turbine. J. Wind Eng. Ind. Aerodyn. 2023, 232, 105285. [Google Scholar] [CrossRef]
- Ouro, P.; Juez, C.; Franca, M. Drivers for mass and momentum exchange between the main channel and river bank lateral cavities. Adv. Water Resour. 2020, 137, 103511. [Google Scholar] [CrossRef]
- Luo, Q.; Dolcetti, G.; Stoesser, T.; Tait, S. Water surface response to turbulent flow over a backward-facing step. J. Fluid Mech. 2023, 966, A18. [Google Scholar] [CrossRef]
- Guo, X.; Luo, Q.; Stoesser, T.; Hajaali, A.; Liu, X. Evolution of high-density submarine turbidity current and its interaction with a pair of parallel suspended pipes. Phys. Fluids 2023, 35, 086608. [Google Scholar] [CrossRef]
- Liu, Y.; Stoesser, T.; Fang, H.; Papanicolaou, A.; Tsakiris, A.G. Turbulent flow over an array of boulders placed on a rough, permeable bed. Comput. Fluids 2017, 158, 120–132. [Google Scholar] [CrossRef]
- Alzabari, F.; Wilson, C.A.; Ouro, P. Hydrodynamics of in-stream leaky barriers for natural flood management. Water Resour. Res. 2024, 60, e2024WR038117. [Google Scholar] [CrossRef]
- Guo, X.; Liu, X.; Zheng, T.; Zhang, H.; Lu, Y.; Li, T. A mass transfer-based LES modelling methodology for analyzing the movement of submarine sediment flows with extensive shear behavior. Coastal Eng. 2024, 191, 104531. [Google Scholar] [CrossRef]
- Zhang, J.; Zhu, L.; Liang, Z.; Sun, L.; Nie, Z.; Wang, J.; Xie, W.; Jiang, Z. Numerical study of efficiency indices to evaluate the effect of layout mode of artificial reef unit on flow field. J. Mar. Sci. Eng. 2021, 9, 770. [Google Scholar] [CrossRef]
- Gong, Y.; Stoesser, T.; Mao, J.; McSherry, R. LES of flow through and around a finite patch of thin plates. Water Resour. Res. 2019, 55, 7587–7605. [Google Scholar] [CrossRef]
- Luo, Q.; Stoesser, T.; Cameron, S.; Nikora, V.; Zampiron, A.; Patella, W. Meandering of instantaneous large-scale structures in open-channel flow over longitudinal ridges. Environ. Fluid Mech. 2023, 23, 829–846. [Google Scholar] [CrossRef]
- Liu, Y.; Tang, Z.; Huang, L.; Stoesser, T.; Fang, H. On the role of the Froude number on flow, turbulence, and hyporheic exchange in open-channel flow through boulder arrays. Phys. Fluids 2024, 36, 095141. [Google Scholar] [CrossRef]
- Dai, J.; Mao, J.q.; Gong, Y.Q.; Gao, H. Effect of triangular baffle designs on flow dynamics and sediment transport within standard box culverts. J. Hydrodyn. 2025, 1–13. [Google Scholar] [CrossRef]
- Nicoud, F.; Ducros, F. Subgrid-scale stress modelling based on the square of the velocity gradient tensor. Flow Turbul. Combust. 1999, 62, 183–200. [Google Scholar] [CrossRef]
- Tan, S.f.; Wang, X.z.; Zhang, M.; Liu, C.g.; Wang, A.m.; Xu, X.f.; Xu, P. Flow field effect of cuboid frame artificial reef. Chin. J. Appl. Mech. 2025. online first (In Chinese) [Google Scholar]
- Rodi, W.; Constantinescu, G.; Stoesser, T. Large-Eddy Simulation in Hydraulics; CRC Press: Boca Raton, FL, USA, 2013. [Google Scholar]
- Zheng, Y.; Kuang, C.; Zhang, J.; Gu, J.; Chen, K.; Liu, X. Current and turbulence characteristics of perforated box-type artificial reefs in a constant water depth. Ocean Eng. 2022, 244, 110359. [Google Scholar] [CrossRef]
- Farzadkhoo, M.; Kingsford, R.T.; Suthers, I.M.; Felder, S. Flow hydrodynamics drive effective fish attraction behaviour into slotted fishway entrances. J. Hydrodyn. 2023, 35, 782–802. [Google Scholar] [CrossRef]
- Wu, Y.; Liu, G.; Yang, J.; Xu, J.; Ke, S.; Li, D.; Chen, X.; Shi, X.; Lin, C. Avoidance behavior of grass carp (Ctenopharyngodon idella) shoals to low-frequency sound stimulation. Aquat. Sci. 2025, 87, 21. [Google Scholar] [CrossRef]
- Marriner, B.A.; Baki, A.B.; Zhu, D.Z.; Cooke, S.J.; Katopodis, C. The hydraulics of a vertical slot fishway: A case study on the multi-species Vianney-Legendre fishway in Quebec, Canada. Ecol. Eng. 2016, 90, 190–202. [Google Scholar] [CrossRef]
- Quaranta, E.; Katopodis, C.; Revelli, R.; Comoglio, C. Turbulent flow field comparison and related suitability for fish passage of a standard and a simplified low-gradient vertical slot fishway. River Res. Appl. 2017, 33, 1295–1305. [Google Scholar] [CrossRef]
- Palau-Salvador, G.; Stoesser, T.; Rodi, W. LES of the flow around two cylinders in tandem. J. Fluids Struct. 2008, 24, 1304–1312. [Google Scholar] [CrossRef]
- McSherry, R.J.; Chua, K.V.; Stoesser, T. Large eddy simulation of free-surface flows. J. Hydrodyn. Ser. B 2017, 29, 1–12. [Google Scholar] [CrossRef]
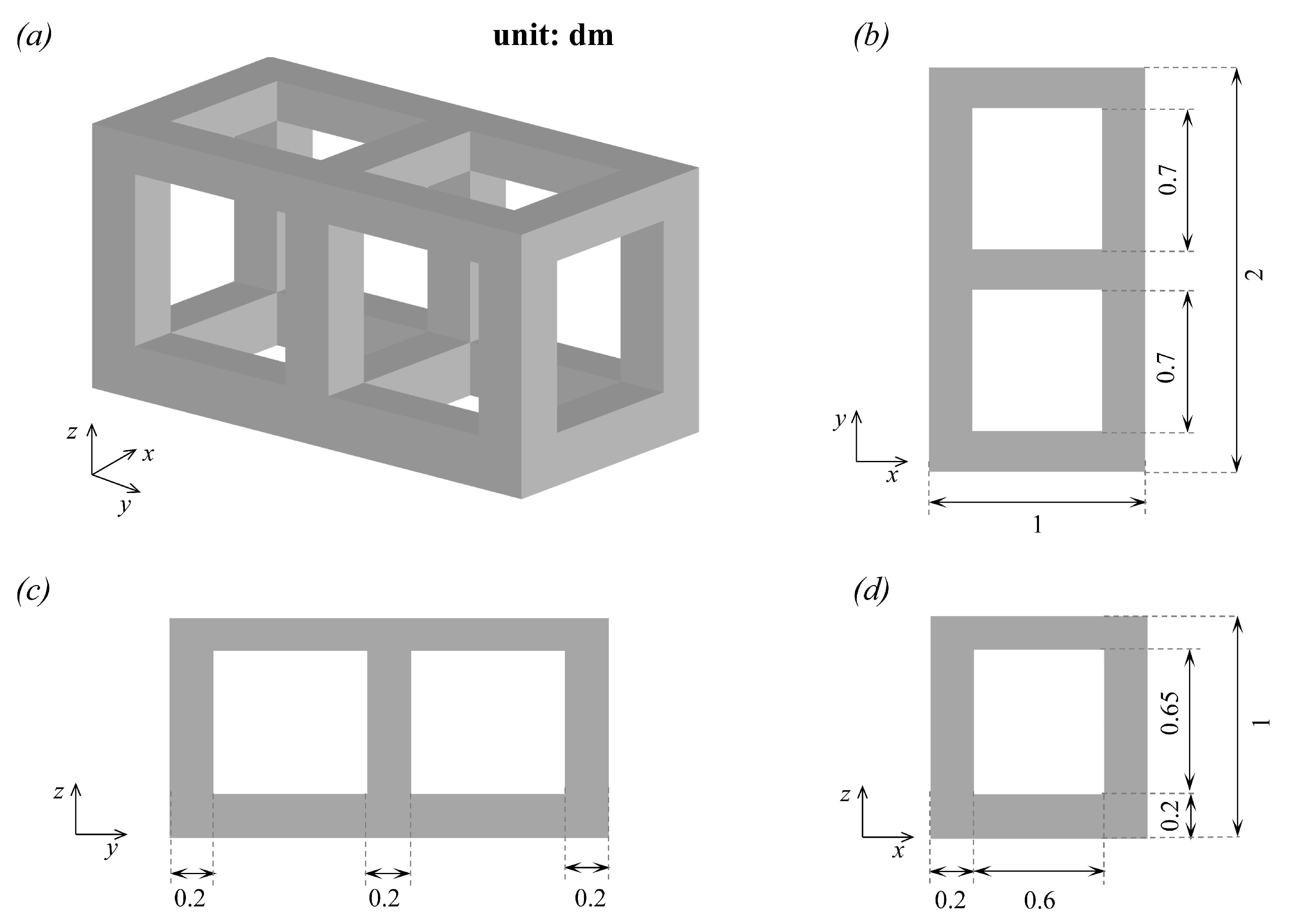
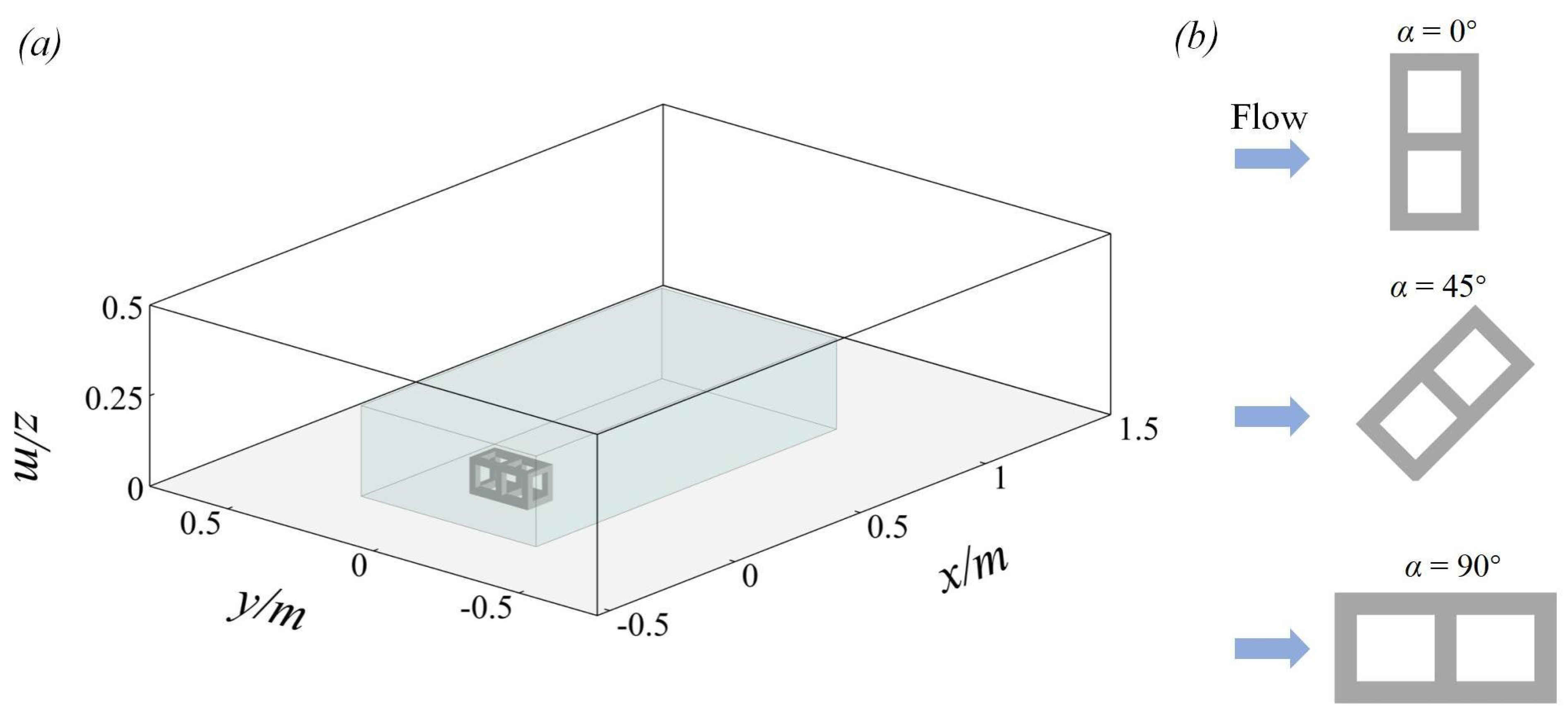
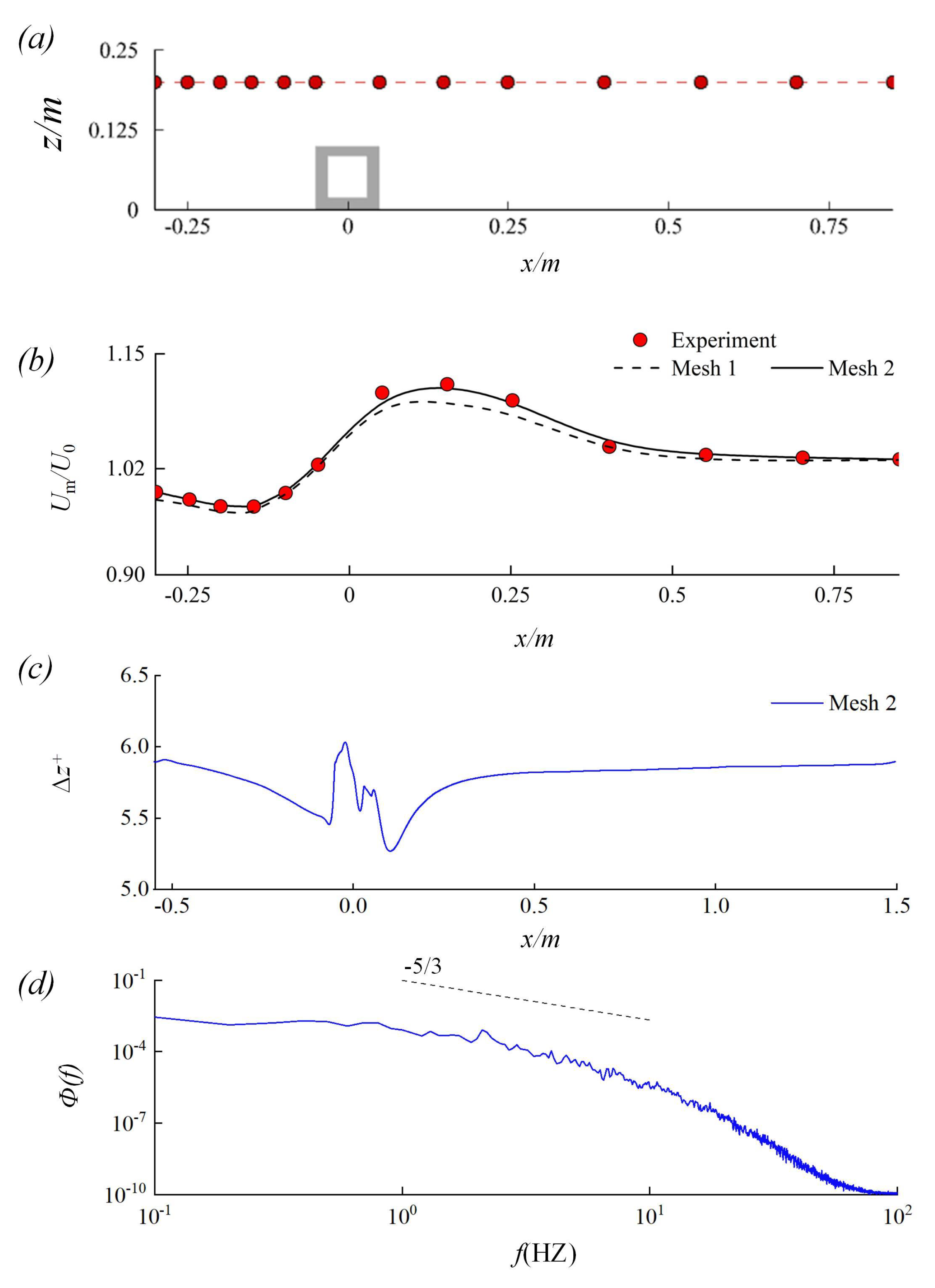

| Case | (m/s) | h (m) | (°) |
|---|---|---|---|
| 1 | 0.22 | 0.5 | 0 |
| 2 | 0.22 | 0.5 | 45 |
| 3 | 0.22 | 0.5 | 90 |
| Mesh | ||
|---|---|---|
| 1 | 0.004 | 0.002 |
| 2 | 0.002 | 0.002 |
| Case | (°) | (m3) | (m3) |
|---|---|---|---|
| 1 | 0 | ||
| 2 | 45 | ||
| 3 | 90 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Dai, J.; Xu, Q.; Gong, Y.; Lu, Y.; Liu, X.; Mao, J. An LES Investigation of Flow Field Around the Cuboid Artificial Reef at Different Angles of Attack. J. Mar. Sci. Eng. 2025, 13, 463. https://doi.org/10.3390/jmse13030463
Dai J, Xu Q, Gong Y, Lu Y, Liu X, Mao J. An LES Investigation of Flow Field Around the Cuboid Artificial Reef at Different Angles of Attack. Journal of Marine Science and Engineering. 2025; 13(3):463. https://doi.org/10.3390/jmse13030463
Chicago/Turabian StyleDai, Jie, Qianshun Xu, Yiqing Gong, Yang Lu, Xinbo Liu, and Jingqiao Mao. 2025. "An LES Investigation of Flow Field Around the Cuboid Artificial Reef at Different Angles of Attack" Journal of Marine Science and Engineering 13, no. 3: 463. https://doi.org/10.3390/jmse13030463
APA StyleDai, J., Xu, Q., Gong, Y., Lu, Y., Liu, X., & Mao, J. (2025). An LES Investigation of Flow Field Around the Cuboid Artificial Reef at Different Angles of Attack. Journal of Marine Science and Engineering, 13(3), 463. https://doi.org/10.3390/jmse13030463








