Mechanical Behavior of Marine Soft Soil with Different Water Contents Under Cyclic Loading
Abstract
:1. Introduction
2. Analysis Methods
2.1. Geotechnical Dynamic Triaxial Test Analysis Method
2.2. Energy Loss Analysis Method
2.3. Discrete Particle Flow Analysis Method
2.4. Analytical Method Validation
3. Results
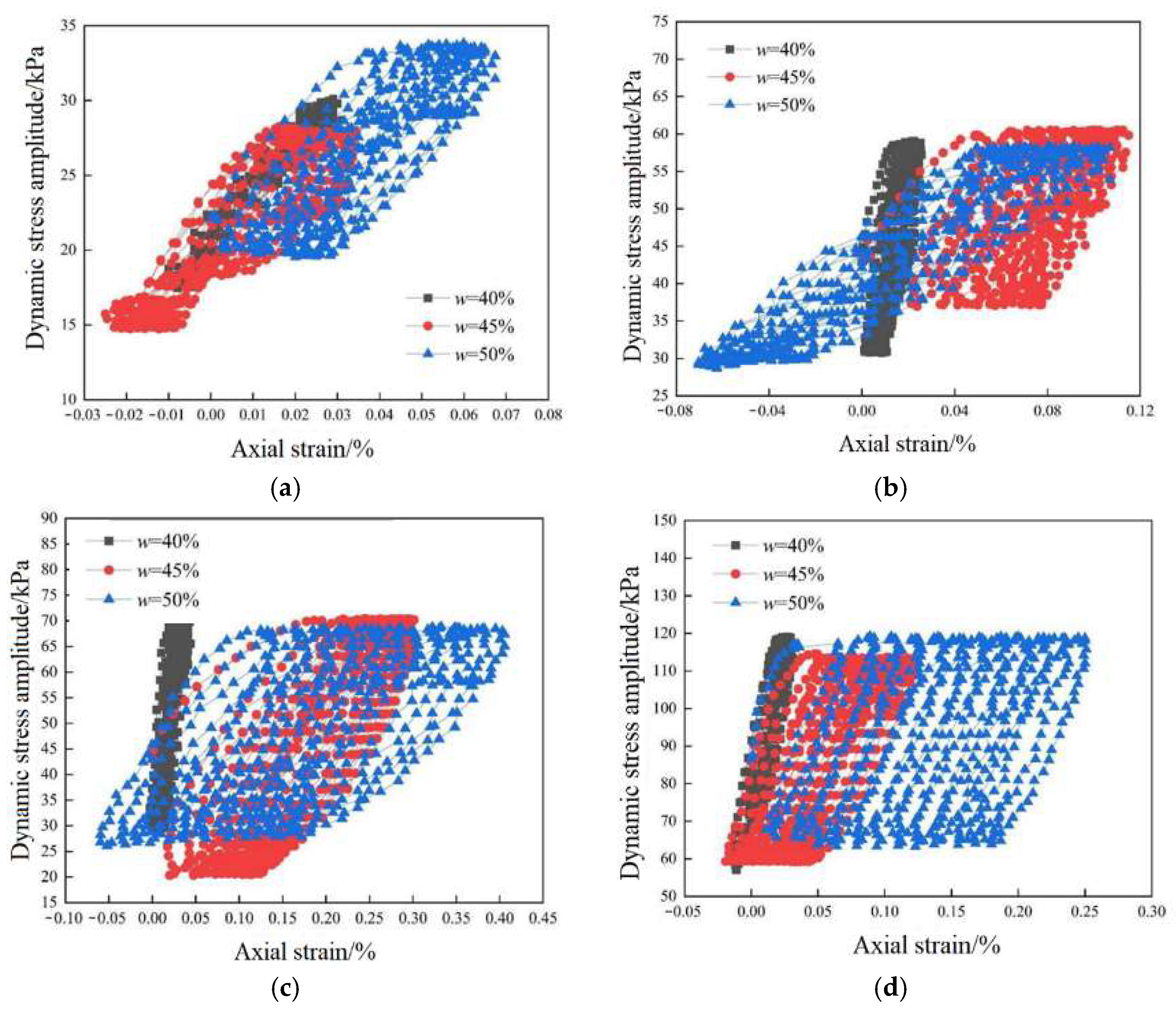
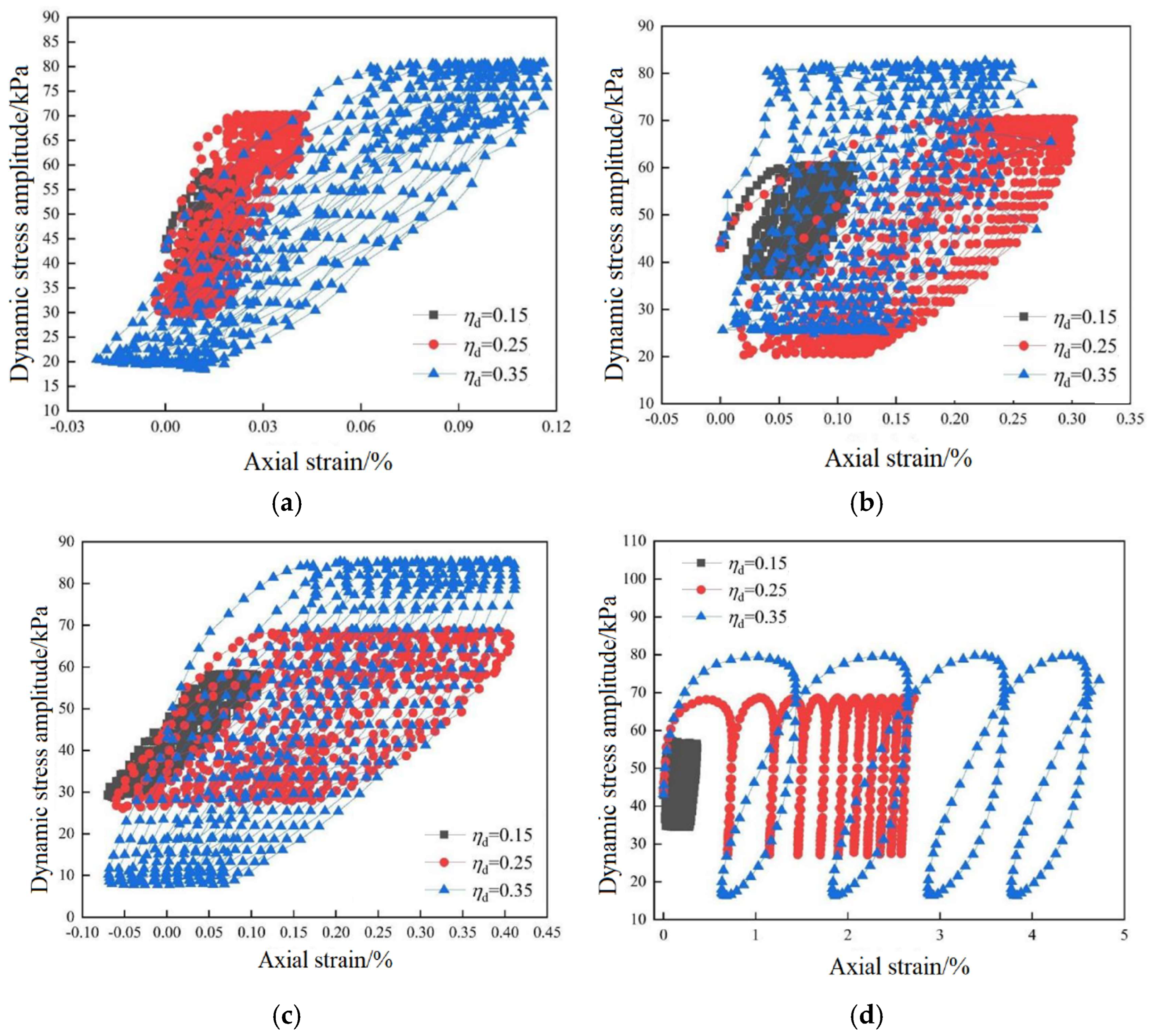
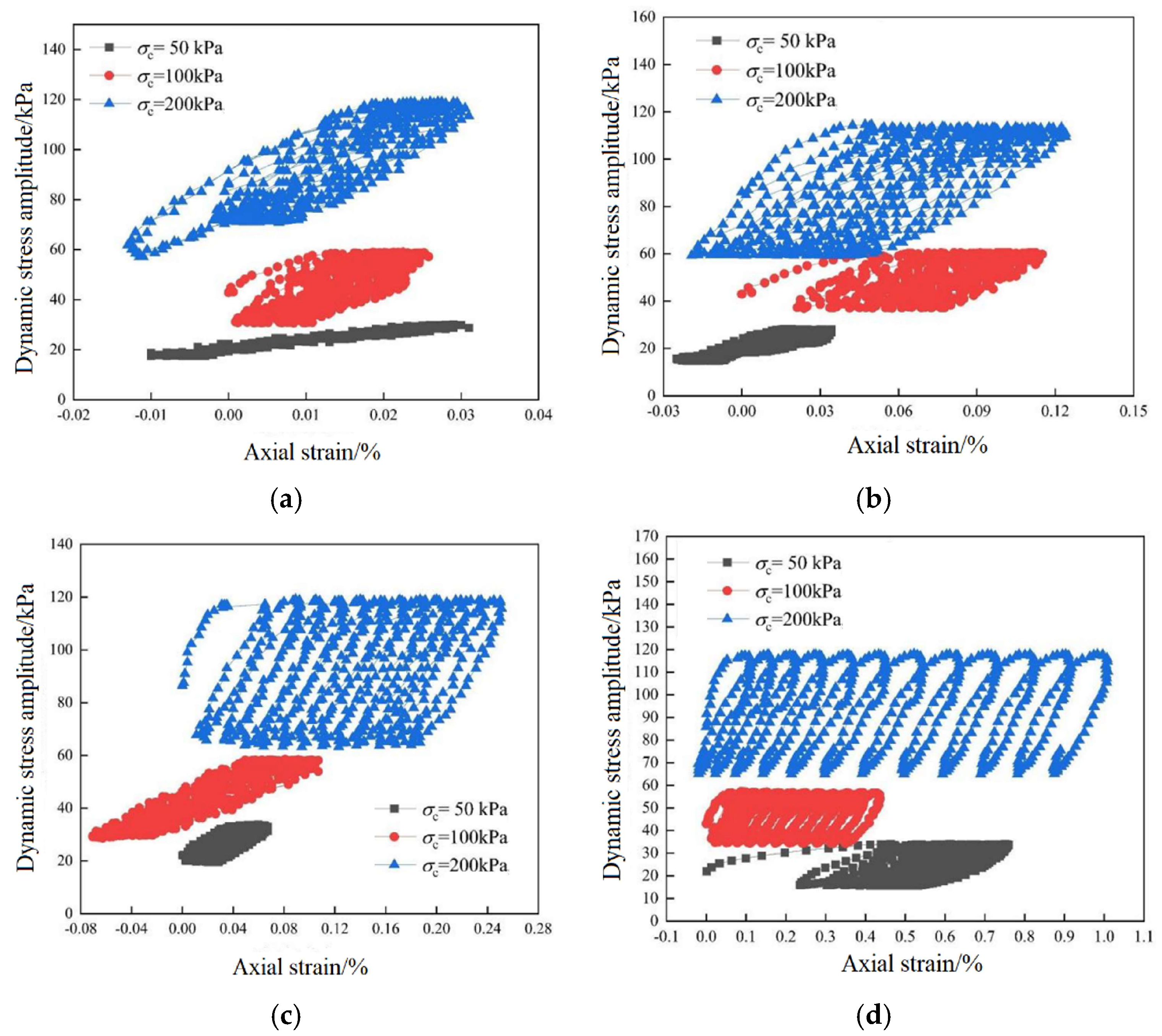
3.1. Morphological Characteristics of Stress–Strain Hysteresis Curves in Marine Soft Soils
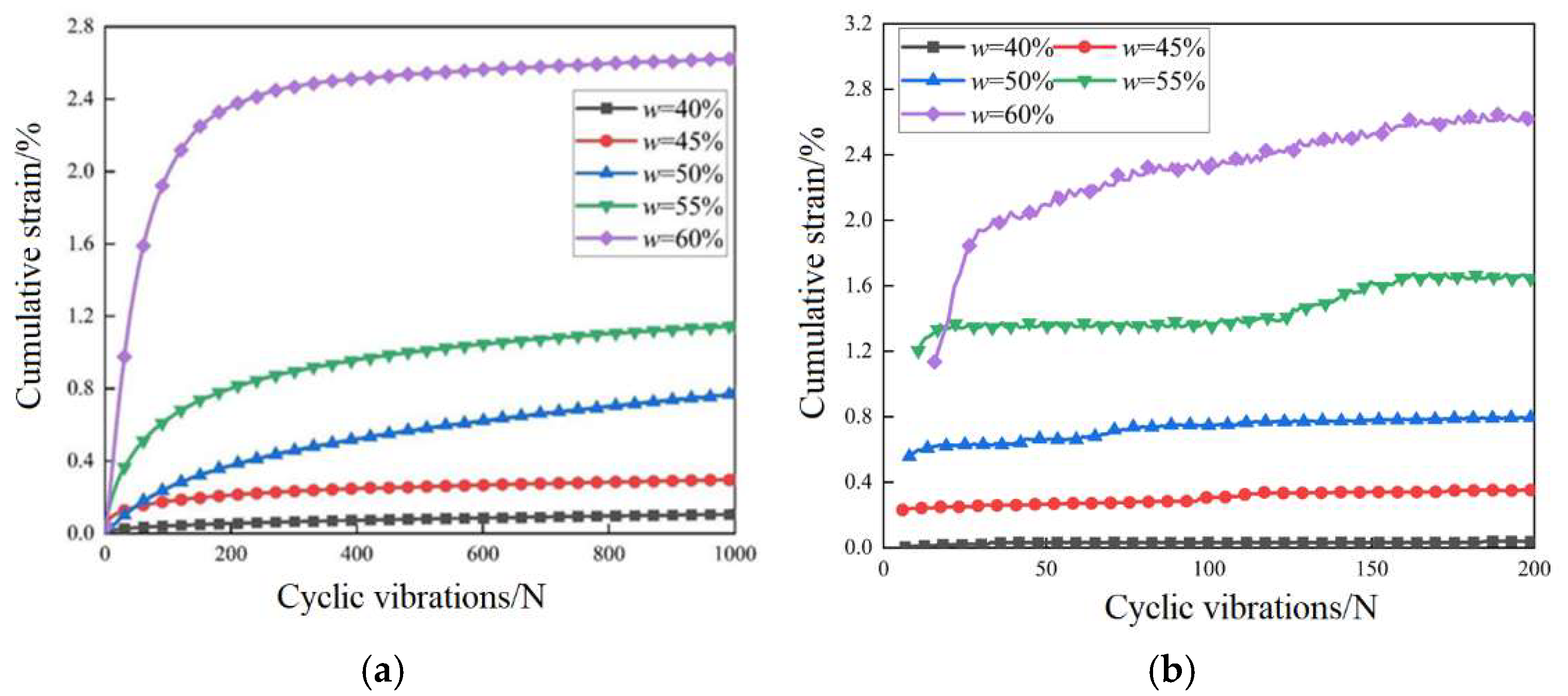
3.2. Characterization of Energy Loss in Marine Soft Soil
- (1)
- Effect of water content on energy loss: At low water content, friction and particle contact in the marine soft soil are stronger, leading to higher energy dissipation and greater soil stiffness, with energy loss primarily occurring through friction between soil particles. As water content increases to a medium level, the water gradually fills the pore spaces within the soil, enhancing the lubricating effect and causing the energy dissipation mechanism to shift toward viscous dissipation. At high water content, the soil becomes softer, and the proportion of water increases, potentially reaching a higher level of energy loss. In this condition, the soil may exhibit behavior akin to liquefaction, and the energy dissipation mechanism becomes more complex. However, the energy loss may decrease as the deformation mode becomes more water-dominated, reducing friction and, thus, frictional dissipation.
- (2)
- Effect of perimeter pressure on energy loss: When the perimeter pressure is 50 kPa, the larger pore spaces in the soil result in more noticeable water effects, leading to more significant soil deformation and higher energy dissipation. As water content increases, energy loss rises more gradually, but water content still plays an important role. At 100 kPa perimeter pressure, the soil is more compacted, enhancing particle contact and increasing stiffness, which reduces energy loss. The stronger contact force and higher stiffness limit soil deformation, leading to more efficient energy transfer but less energy dissipation compared to lower perimeter pressures. For soil with medium water content, the effect of perimeter pressure on energy dissipation becomes more pronounced. At 200 kPa perimeter pressure, the soil becomes denser, and particle contact reaches its maximum, resulting in increased stiffness and significantly reduced energy dissipation. At this point, energy is primarily transferred through elastic deformation, with minimal energy loss, particularly at higher water content.
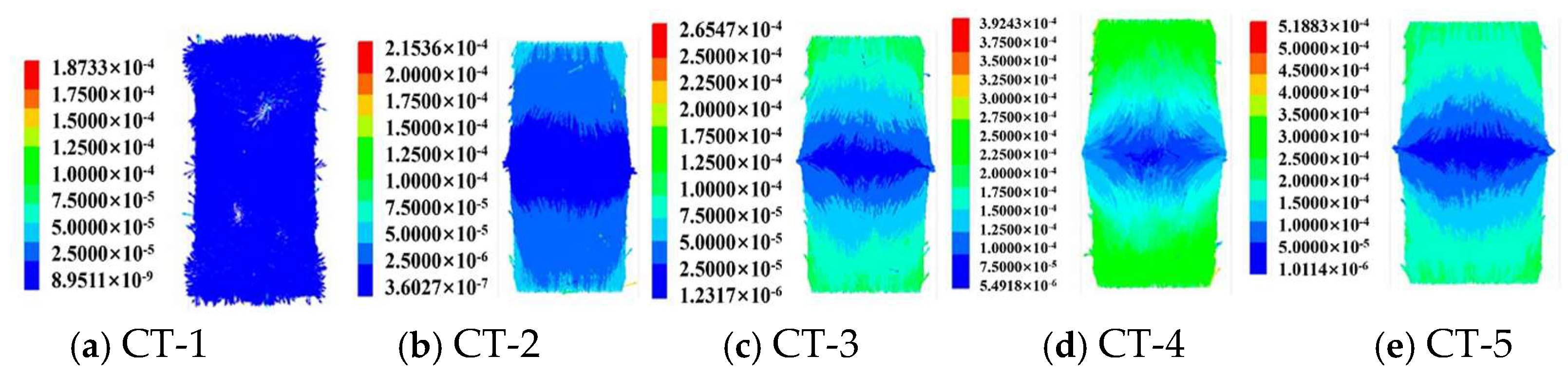
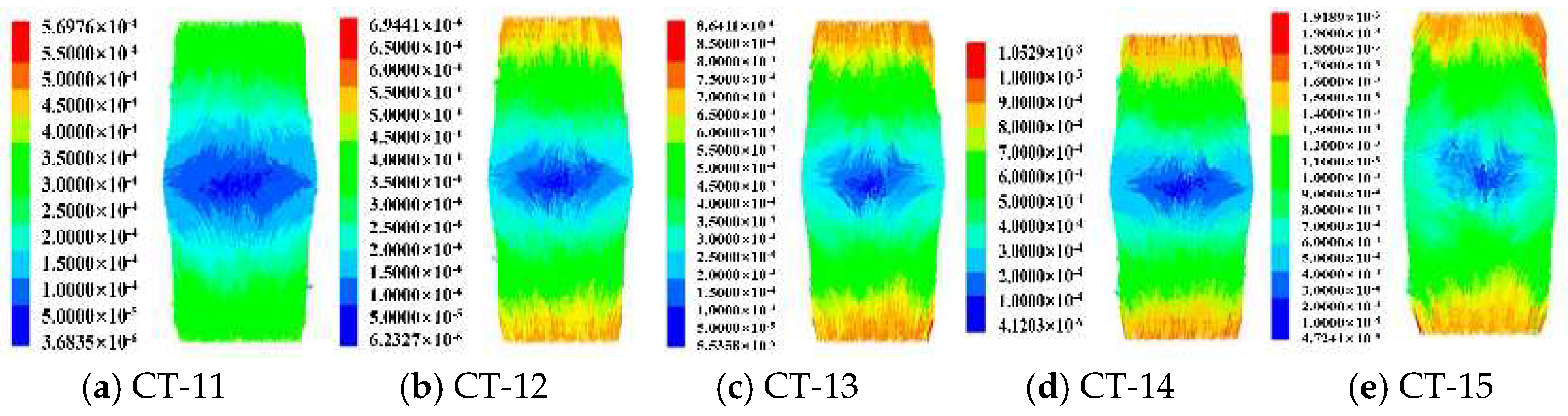
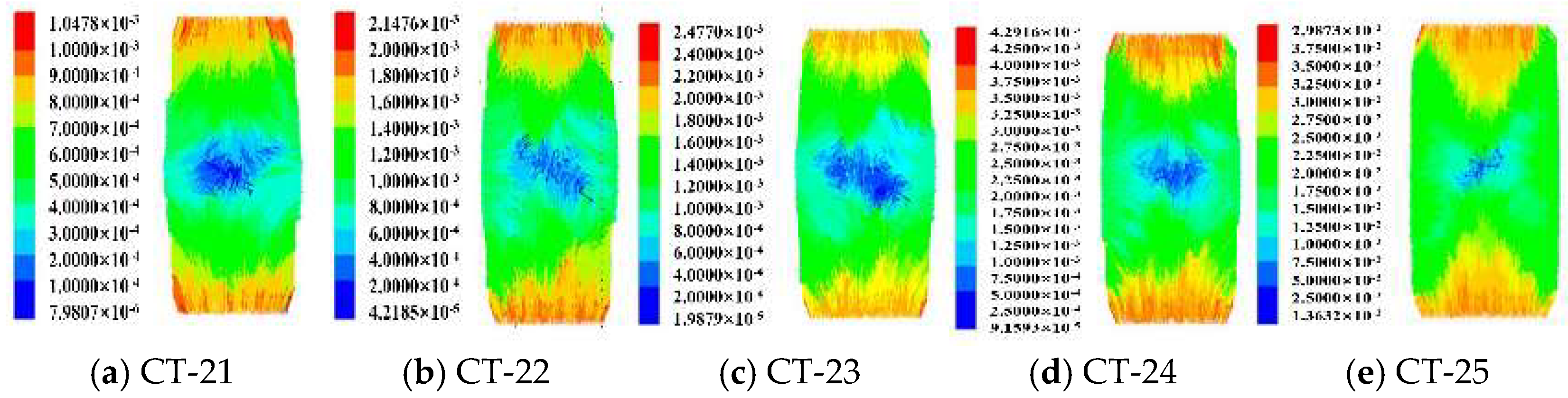
3.3. Displacement Fields
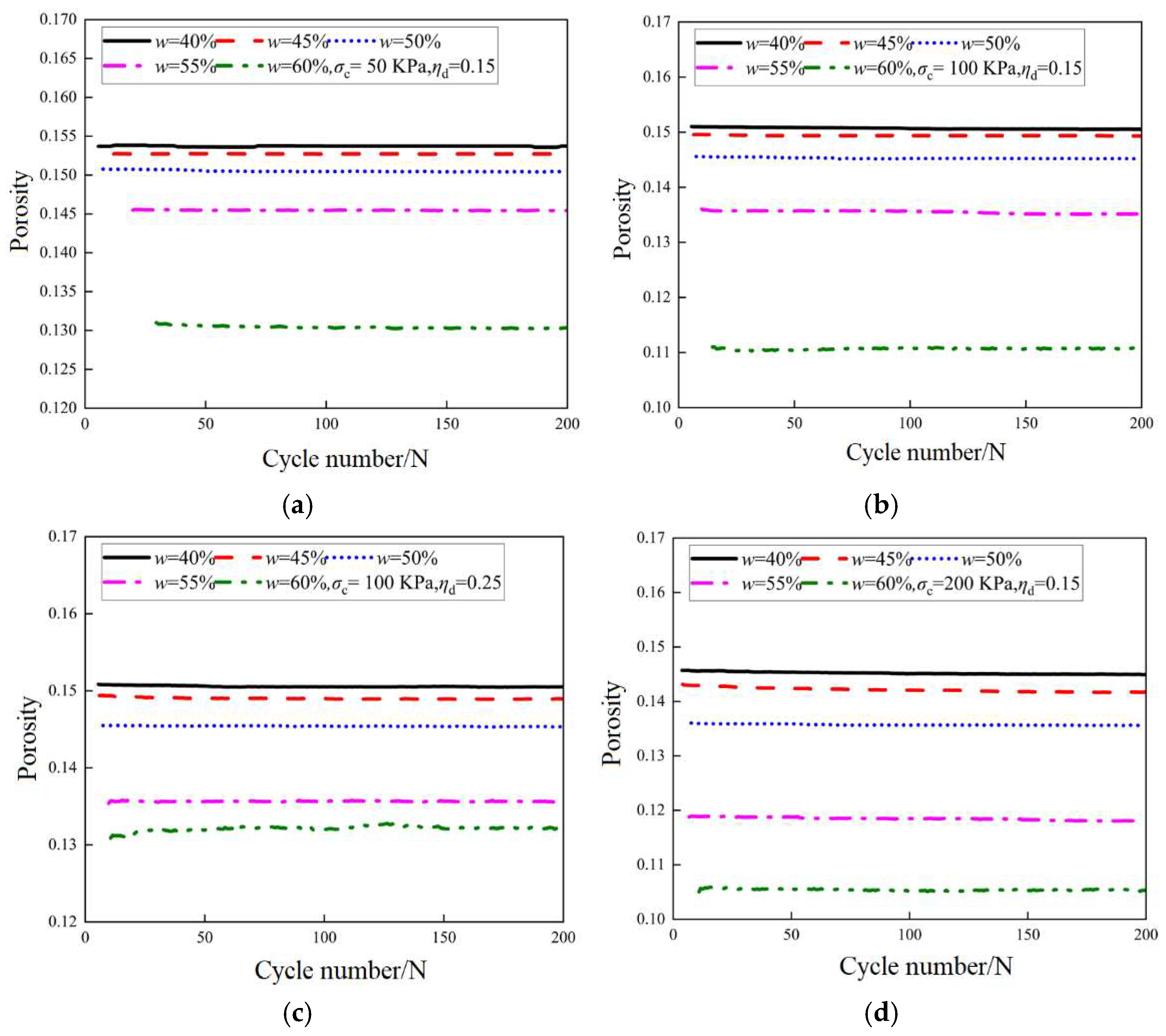
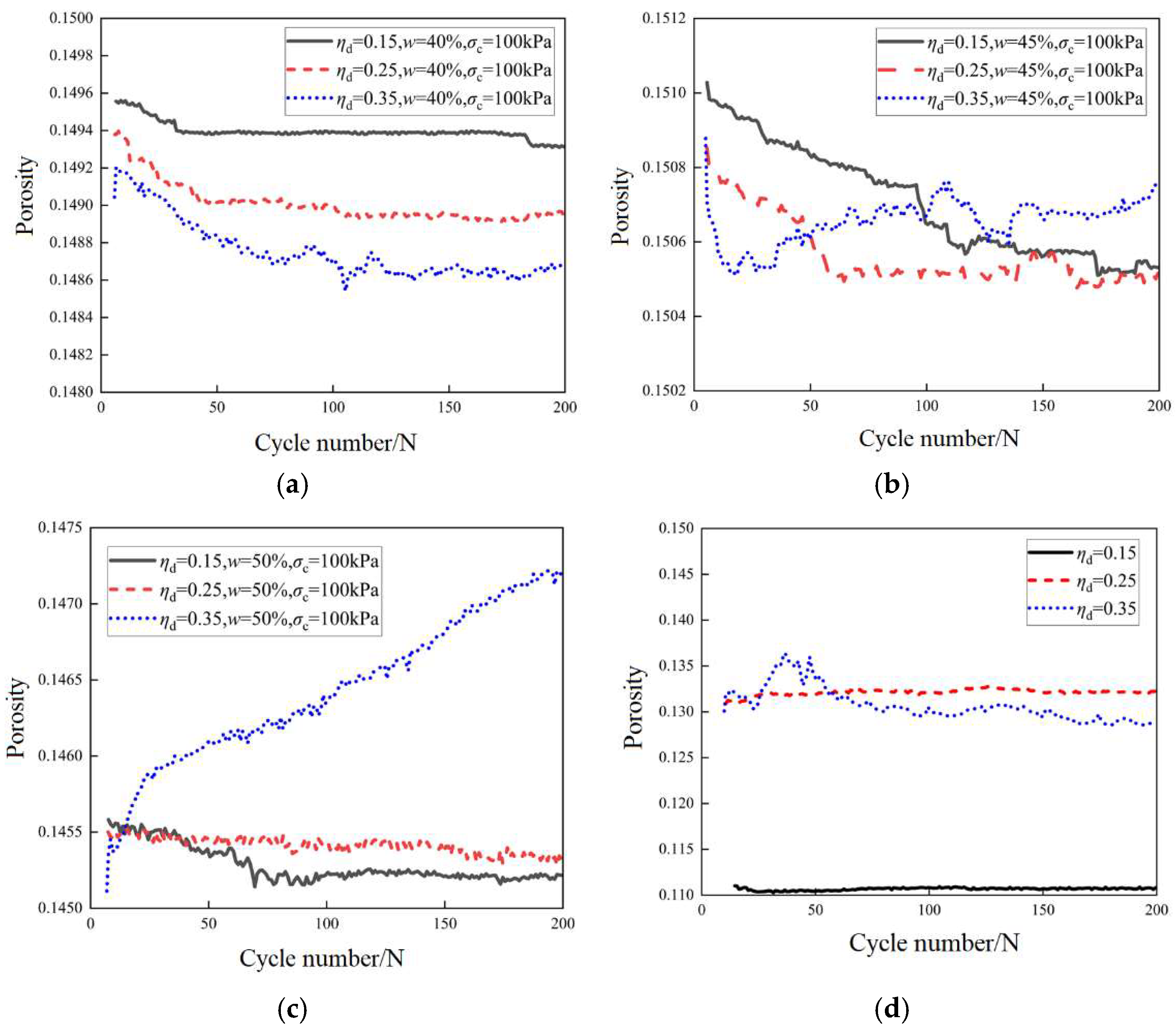
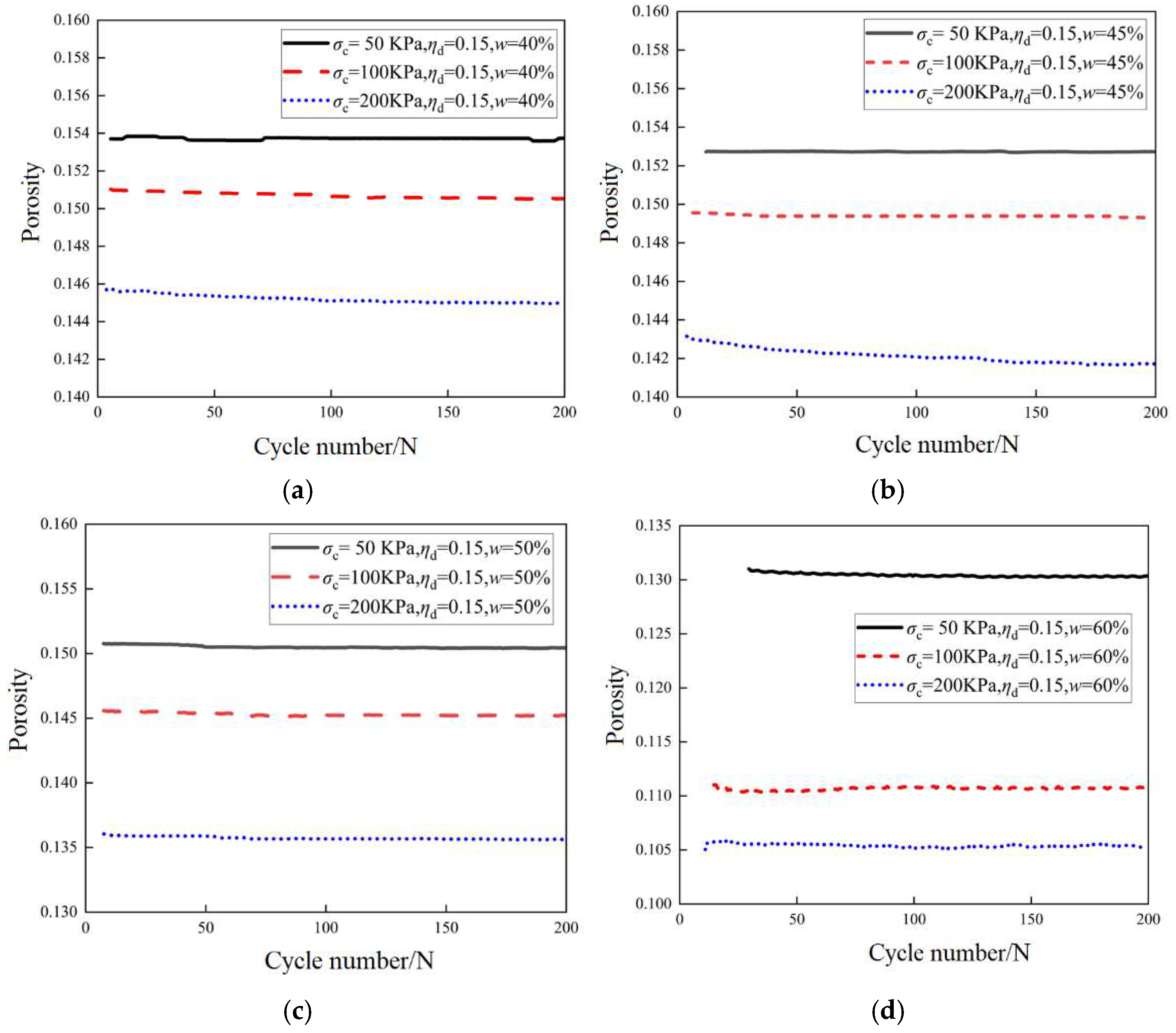
3.4. Porosity Evolution
4. Conclusions
- (1)
- Strain behavior and energy dissipation: A detailed analysis of the first 10 cycles of the hysteresis curve reveals that the strain range of the specimen increases significantly with higher dynamic stress ratios, confining pressures, and water content. The hysteresis loop extends and tilts toward the axial strain direction, with a corresponding increase in the overall loop area, indicating enhanced energy dissipation. This trend suggests that higher dynamic stress ratios, confining pressures, and water content exacerbate strain softening in marine soft soil during the initial cycles.
- (2)
- Particle displacement and shear band formation: At lower water contents, increased dynamic stress ratios lead to greater particle displacement but do not result in significant shear band formation. However, as water content increases to 50%, particle displacement becomes more pronounced, and initial shear bands begin to form, indicating that moderate water content provides sufficient lubrication while facilitating orderly particle movement. Further increases in water content to 60% result in wider and more pronounced shear zones, signifying extensive specimen damage. Although higher confining pressure enhances particle displacement, it does not lead to marked shear band formation, likely due to the stabilizing effect of high confining pressure, which promotes more uniform stress distribution and suppresses shear band development.
- (3)
- Energy dissipation: As water content increases, energy dissipation in marine soft soil accelerates at lower confining pressures but increases more gradually at higher confining pressures. At higher perimeter pressures, energy dissipation stabilizes, and the influence of water content on energy loss diminishes. Comparison of curves at different pressures shows that at lower pressures, energy dissipation increases more significantly, whereas at higher pressures, the rate of energy loss levels off. Additionally, higher dynamic stress ratios lead to greater energy dissipation in marine soft soils.
- (4)
- Porosity and moisture content: At low moisture content, porosity is minimally affected by the dynamic stress ratio, indicating that insufficient moisture prevents substantial changes in particle interactions. As moisture content reaches moderate levels, however, porosity becomes more responsive to changes in dynamic stress ratio, likely due to moisture-induced lubrication that reduces direct particle contact and promotes pore formation. At high moisture content, porosity change becomes more pronounced, especially with increasing dynamic stress ratio. This is due to increased lubrication between particles, which reduces friction and expands pore spaces. Conversely, higher confining pressure typically reduces porosity by promoting tighter particle packing and reducing pore space.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Kim, A.R.; Chang, I.; Cho, G.C.; Shim, S.H. Strength and Dynamic Properties of Cement-Mixed Korean Marine Clays. KSCE J. Civ. Eng. 2018, 22, 1150–1161. [Google Scholar] [CrossRef]
- Huancollo, H.J.M.; Saboya, F.; Tibana, S.; McCartney, J.S.; Borges, R.G. Thermal Triaxial Tests to Evaluate Improvement of Soft Marine Clay through Thermal Consolidation. Geotech. Test. J. 2023, 46, 579–597. [Google Scholar] [CrossRef]
- Emmanuel, E.; Anggraini, V.; Raghunandan, M.E.; Asadi, A.; Bouazza, A. Improving the engineering properties of a soft marine clay with forsteritic olivine. Eur. J. Environ. Civ. Eng. 2019, 26, 519–546. [Google Scholar] [CrossRef]
- Nguyen, T.N.; Nguyen, T.D.; Bui, T.S. Geotechnical Properties of Soft Marine Soil at Chan May Port, Vietnam. Inz. Miner.-J. Pol. Miner. Eng. Soc. 2021, 2, 207–215. [Google Scholar] [CrossRef]
- Wu, K.; Xu, W.; Liu, Y.; Liu, D.; Dou, Z.; Liu, Z.; Chen, R.; Hao, D. Mechanical properties of marine soft soil and its influence on urban underground track transport engineering. J. Water Resour. Archit. Eng. 2024, 22, 122–131. [Google Scholar]
- Viens, L.; Bonilla, L.F.; Spica, Z.J.; Nishida, K.; Yamada, T.; Shinohara, M. Nonlinear earthquake response of marine sediments with distributed acoustic sensing. Geophys. Res. Lett. 2022, 49, e2022GL100122. [Google Scholar] [CrossRef]
- Lu, Y.; Fu, W.; Xue, D. Deformation Characteristics of Soft Marine Soil Tested under Cyclic Loading with Low Frequency. Adv. Civ. Eng. 2020, 2020, 8875315. [Google Scholar] [CrossRef]
- Qiao, F.; Bo, J.S.; Qi, W.H.; Wang, L.; Chang, C.Y.; Zhang, Z.P.; Wang, J. Study on the dynamic characteristics of soft soil. RSC Adv. 2020, 10, 4630–4639. [Google Scholar] [CrossRef] [PubMed]
- Ding, Z.; Kong, B.W.; Wei, X.J.; Zhang, M.Y.; Xu, B.L.; Zhao, F.J. Laboratory Testing to Research the Micro-Structure and Dynamic Characteristics of Frozen-Thawed Marine Soft Soil. J. Mar. Sci. Eng. 2019, 7, 85. [Google Scholar] [CrossRef]
- Cai, H.; Zhang, Q.; Ye, G.L. Numerical simulation on undrained cylic triaxial test of soft marine clay considering end restrictions of soil specimen. Ocean Eng. 2021, 216, 108100. [Google Scholar] [CrossRef]
- Tong, J.H.; Wang, Q.; Zhang, W.K.; Guo, L.; Ni, D.Y.; Zhai, J.L.; Yuan, D.Y.; Wang, H.Z. Post-cyclic undrained behavior of marine soft clay subjected to partially drained cyclic loading. Mar. Georesources Geotechnol. 2023, 42, 898–911. [Google Scholar] [CrossRef]
- Wang, Y.; Qi, Z.; Wei, T.Z.; Bao, J.J.; Zhang, X.; Zhou, Y.S. Numerical Study on the Responses of Suction Pile Foundations under Horizontal Cyclic Loading Considering the Soil Stiffness Degradation. J. Mar. Sci. Eng. 2023, 11, 2336. [Google Scholar] [CrossRef]
- Yang, J.Q.; Cui, Z.D.; Xi, B.L.; Song, W.L. Experimental study on cyclic triaxial behaviors of saturated soft soil considering time intermittent and variable confining pressure. Soil Dyn. Earthq. Eng. 2024, 179, 108508. [Google Scholar] [CrossRef]
- Zhou, J.; Liu, C.J.; Zhu, K.F.; Shen, P.P. Macroscopic behaviour and mesoscopic mechanism of shear strength characteristics of pile-soil interface under cyclic loading. Ocean Eng. 2024, 297, 116998. [Google Scholar] [CrossRef]
- Zhao, L.Y.; Shan, Z.G.; Wang, M.Y.; Ni, W.D.; Jin, H.X.; Shi, L. Undrained deformation behavior of marine clay under two-way cyclic loading. Mar. Georesources Geotechnol. 2023, 42, 465–474. [Google Scholar] [CrossRef]
- Wang, X.Y.; Sun, H.; Niu, F.J. Microstructure study of natural marine clay in loading and unloading processes. Eng. Geol. 2023, 327, 107363. [Google Scholar] [CrossRef]
- Li, F.; Zhang, K.Y.; Luo, X.J. Micro-study of soil’s inherent anisotropy with PFC simulation. Appl. Mech. Mater. 2013, 275–277, 383–386. [Google Scholar]
- Cao, B. Sensitivity analysis of loess triaxial mesoscopic parameters based on PFC3D. In Proceedings of the 6th International Conference on Energy Science and Chemical Engineering, Dali, China, 17–19 July 2020; Volume 565, p. 012070. [Google Scholar]
- Lin, C.-X.; Ling, D.-S.; Zhong, S.-Y. Application of particle flow code numerical simulation in research of geotechnical behavior of lunar soil. J. Zhejiang Univ. (Eng. Sci.) 2015, 49, 1679–1691. [Google Scholar]
- GB/T50123-2019; Standard for Geotechnical Testing Method. National Standard of the People’s Republic of China: Beijing, China, 2019.
- ASTM D7181-11; Method for Consolidated Drained Triaxial Compression Test for Soils. American Society for Testing and Materials: Conshohocken, PA, USA, 2011.
- Xiao, W.; Wu, K.; Xu, W.; Liu, Y.; Lu, H.; Chen, R. Experiment and analysis on dynamic characteristics of marine soft clay. Mar. Georesources Geotechnol. 2024, 5, 1–22. [Google Scholar] [CrossRef]
- Ni, X.; Yin, X.; Cai, Z. Study on the meso-mechanism of consolidation of soft soil based on PFC-COMSOL. J. Hebei Univ. Eng. (Nat. Sci. Ed.) 2017, 34, 30–36. [Google Scholar]
- Ji, S.T.; Karlovsek, J. Optimized differential evolution algorithm for solving DEM material calibration problem. Eng. Comput. 2023, 39, 2001–2016. [Google Scholar] [CrossRef]
- Geng, X. Analysis of Microscopic Damage Mechanism of Marine Silt Cement-solidified Soil Based on PFC3D. Master’s Thesis, Wuhan University of Engineering, Wuhan, China, 2022. [Google Scholar]
- Tang, Y.; Chen, H.J.; Xiong, L.X.; Xu, Z.Y. Conventional triaxial loading and unloading test and PFC numerical simulation of rock with single fracture. Arch. Civ. Eng. 2024, 70, 233–254. [Google Scholar] [CrossRef]

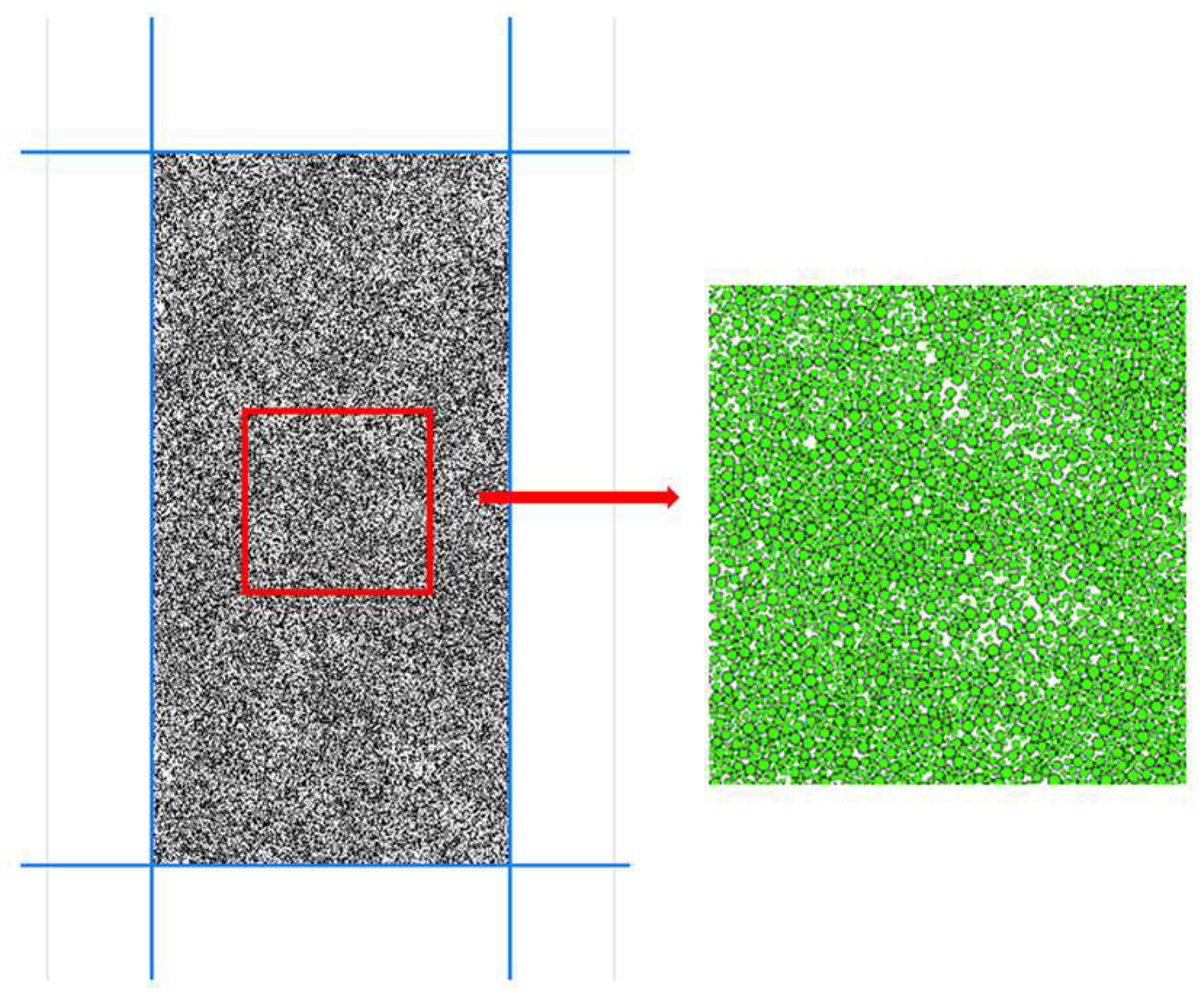


| Liquid Limit (%) | Plastic Limit (%) | Plasticity Index (%) | Specific Gravity (kg/m3) | Particle Composition (%) | ||
|---|---|---|---|---|---|---|
| Sand Grains (0.02–2 mm) | Powder Grain (0.002–0.02 mm) | Clay Grain (<0.002 mm) | ||||
| 63.62 | 37.37 | 26.25 | 1.69 | 18.8% | 58.5% | 22.7% |
| Specimen Number | Water Content (%) | Surrounding Pressure (kPa) | Deviator Stress (kPa) | Dynamic Stress (kPa) | Dynamic Stress Ratio |
|---|---|---|---|---|---|
| CT-1 | 40 | 50 | 22 | 15 | 0.15 |
| CT-2 | 100 | 43 | 30 | 0.15 | |
| CT-3 | 100 | 43 | 50 | 0.25 | |
| CT-4 | 100 | 43 | 70 | 0.35 | |
| CT-5 | 200 | 86 | 60 | 0.15 | |
| CT-6 | 45 | 50 | 22 | 15 | 0.15 |
| CT-7 | 100 | 43 | 30 | 0.15 | |
| CT-8 | 100 | 43 | 50 | 0.25 | |
| CT-9 | 100 | 43 | 70 | 0.35 | |
| CT-10 | 200 | 86 | 60 | 0.15 | |
| CT-11 | 50 | 50 | 22 | 15 | 0.15 |
| CT-12 | 100 | 43 | 30 | 0.15 | |
| CT-13 | 100 | 43 | 50 | 0.25 | |
| CT-14 | 100 | 43 | 70 | 0.35 | |
| CT-15 | 200 | 86 | 60 | 0.15 | |
| CT-16 | 55 | 50 | 22 | 15 | 0.15 |
| CT-17 | 100 | 43 | 30 | 0.15 | |
| CT-18 | 100 | 43 | 50 | 0.25 | |
| CT-19 | 100 | 43 | 70 | 0.35 | |
| CT-20 | 200 | 86 | 60 | 0.15 | |
| CT-21 | 60 | 50 | 22 | 15 | 0.15 |
| CT-22 | 100 | 43 | 30 | 0.15 | |
| CT-23 | 100 | 43 | 50 | 0.25 | |
| CT-24 | 100 | 43 | 70 | 0.35 | |
| CT-25 | 200 | 86 | 60 | 0.15 |
| Water Content | Coefficient of Friction | Linear Contact Effective Modulus (MPa) | Stiffness Ratio | Normal Bond Strength (kPa) | Tangential Bond Strength (kPa) |
|---|---|---|---|---|---|
| 40% | 0.17 | 50 | 2 | 30 | 30 |
| 45% | 0.15 | 40 | 2 | 25 | 25 |
| 50% | 0.14 | 20 | 2 | 20 | 20 |
| 55% | 0.13 | 10 | 2 | 10 | 10 |
| 60% | 0.11 | 8 | 2 | 1 | 1 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Liu, Y.; Zhang, H.; Sun, Y.; Wu, K.; Xiao, W. Mechanical Behavior of Marine Soft Soil with Different Water Contents Under Cyclic Loading. J. Mar. Sci. Eng. 2024, 12, 2307. https://doi.org/10.3390/jmse12122307
Liu Y, Zhang H, Sun Y, Wu K, Xiao W. Mechanical Behavior of Marine Soft Soil with Different Water Contents Under Cyclic Loading. Journal of Marine Science and Engineering. 2024; 12(12):2307. https://doi.org/10.3390/jmse12122307
Chicago/Turabian StyleLiu, Yajun, Heng Zhang, Yindong Sun, Ke Wu, and Wenbin Xiao. 2024. "Mechanical Behavior of Marine Soft Soil with Different Water Contents Under Cyclic Loading" Journal of Marine Science and Engineering 12, no. 12: 2307. https://doi.org/10.3390/jmse12122307
APA StyleLiu, Y., Zhang, H., Sun, Y., Wu, K., & Xiao, W. (2024). Mechanical Behavior of Marine Soft Soil with Different Water Contents Under Cyclic Loading. Journal of Marine Science and Engineering, 12(12), 2307. https://doi.org/10.3390/jmse12122307





