Effects on the Potential for Seepage Failure Under a Geotextile Mattress with Floating Plate
Abstract
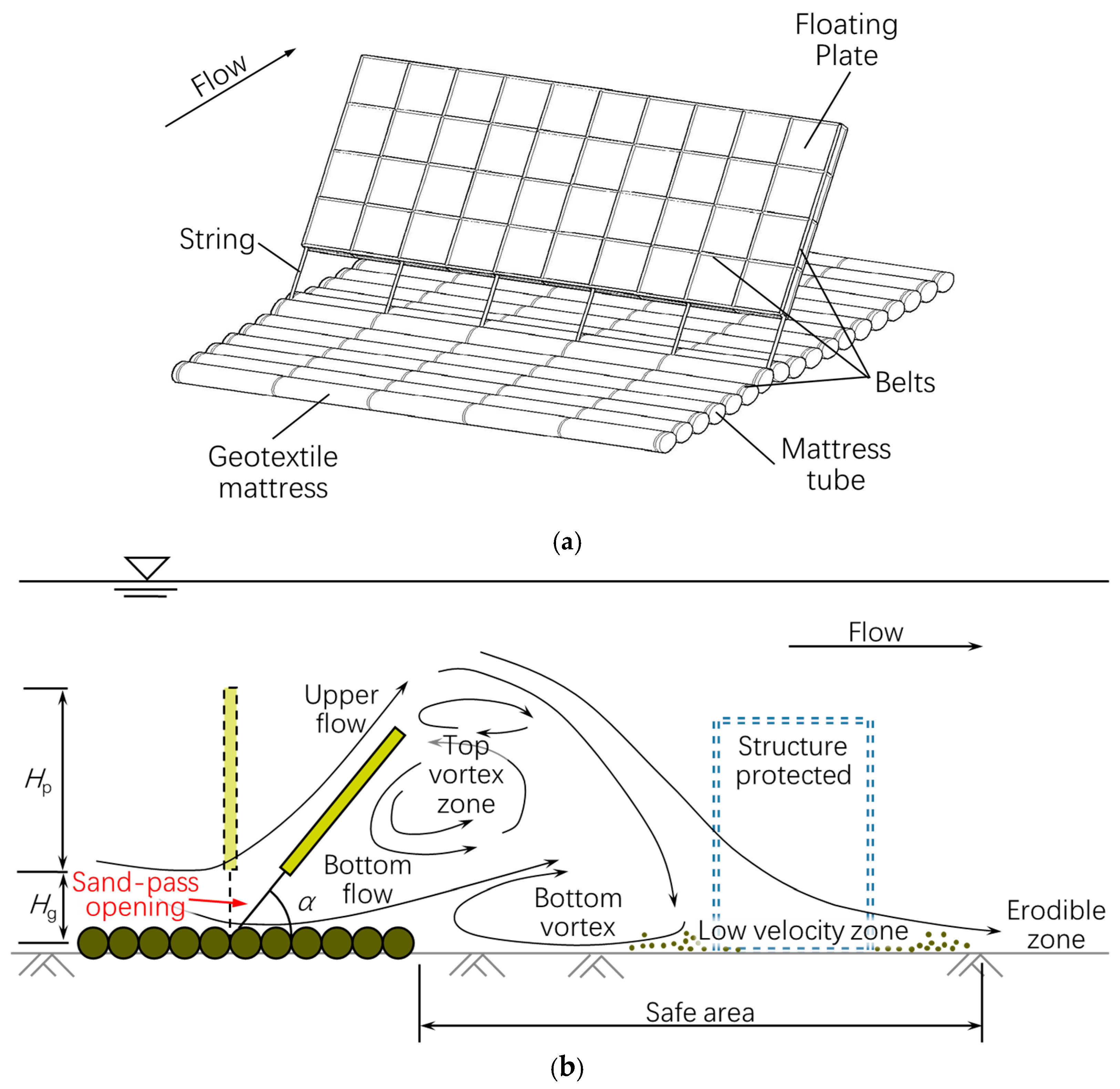
1. Introduction
2. Experiments on the Variation of the Sloping Curtain
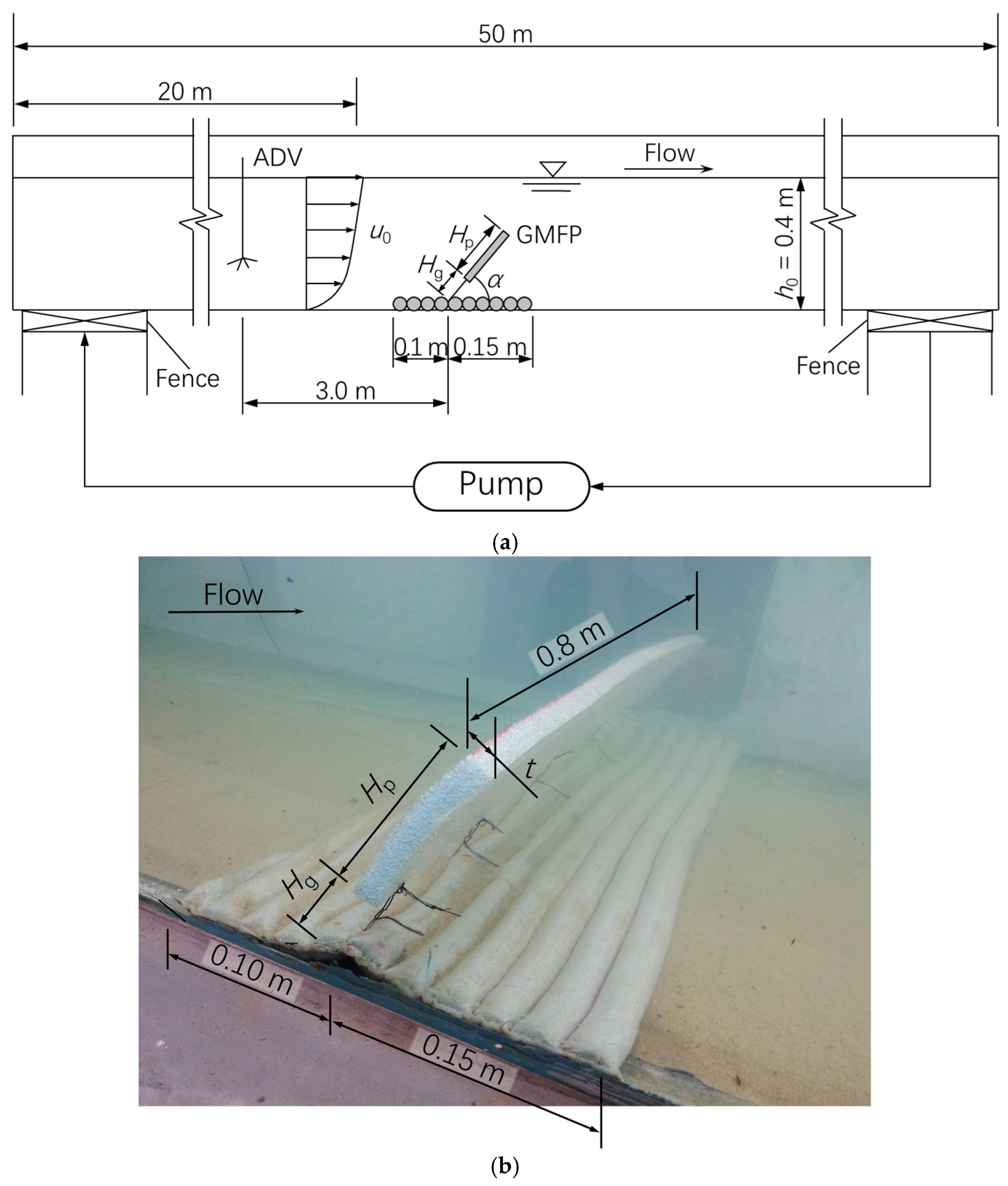
2.1. Experimental Setup
2.2. Experiment Cases
2.3. Experiment Results
3. Numerical Model
3.1. Governing Equations
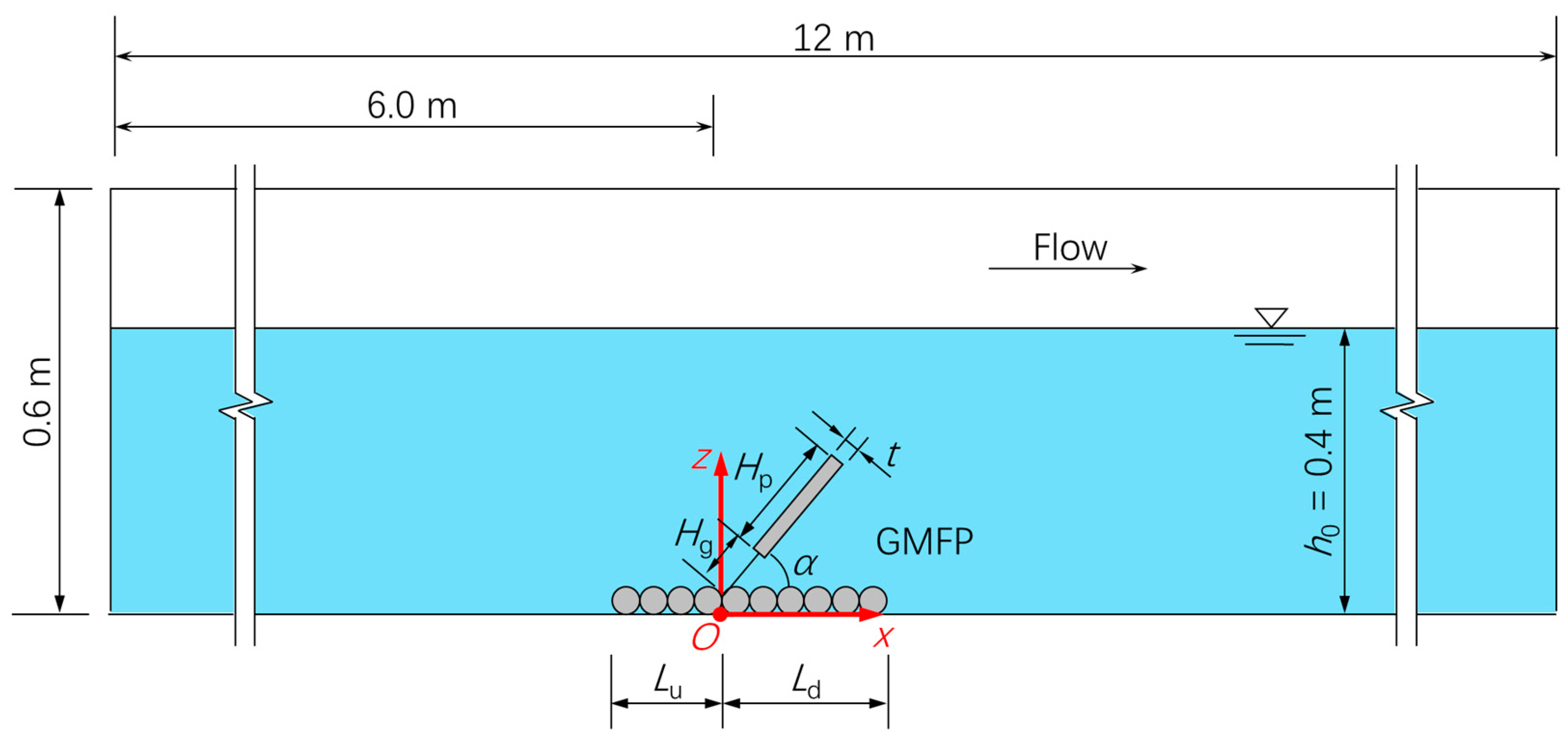
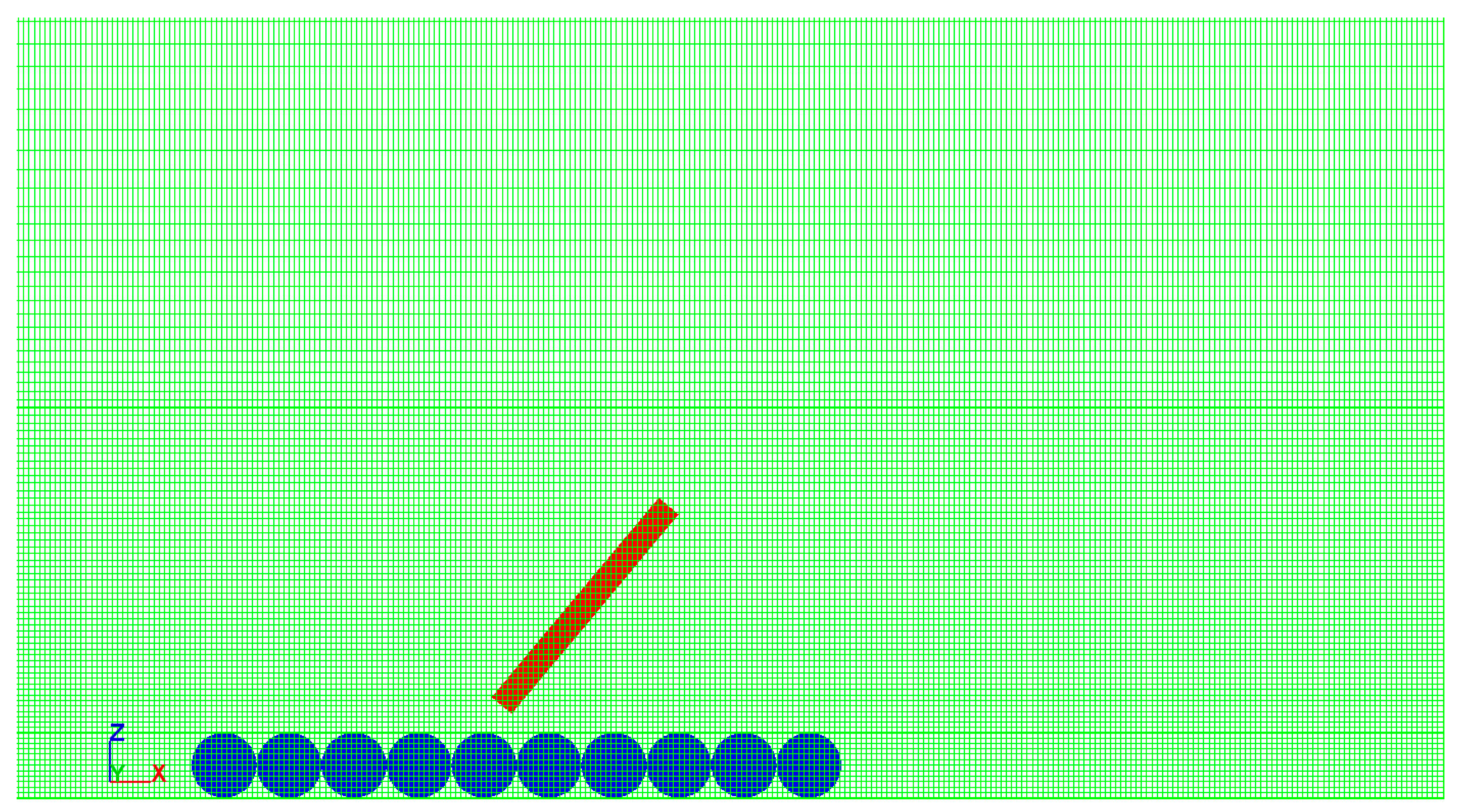
3.2. Model Setup
3.3. Model Validation
3.3.1. Validation of Bed Pressure Distribution
3.3.2. Validation of Flow Pattern near the GMFP
4. Effects on the Averaged Hydraulic Gradient Under the GMFP
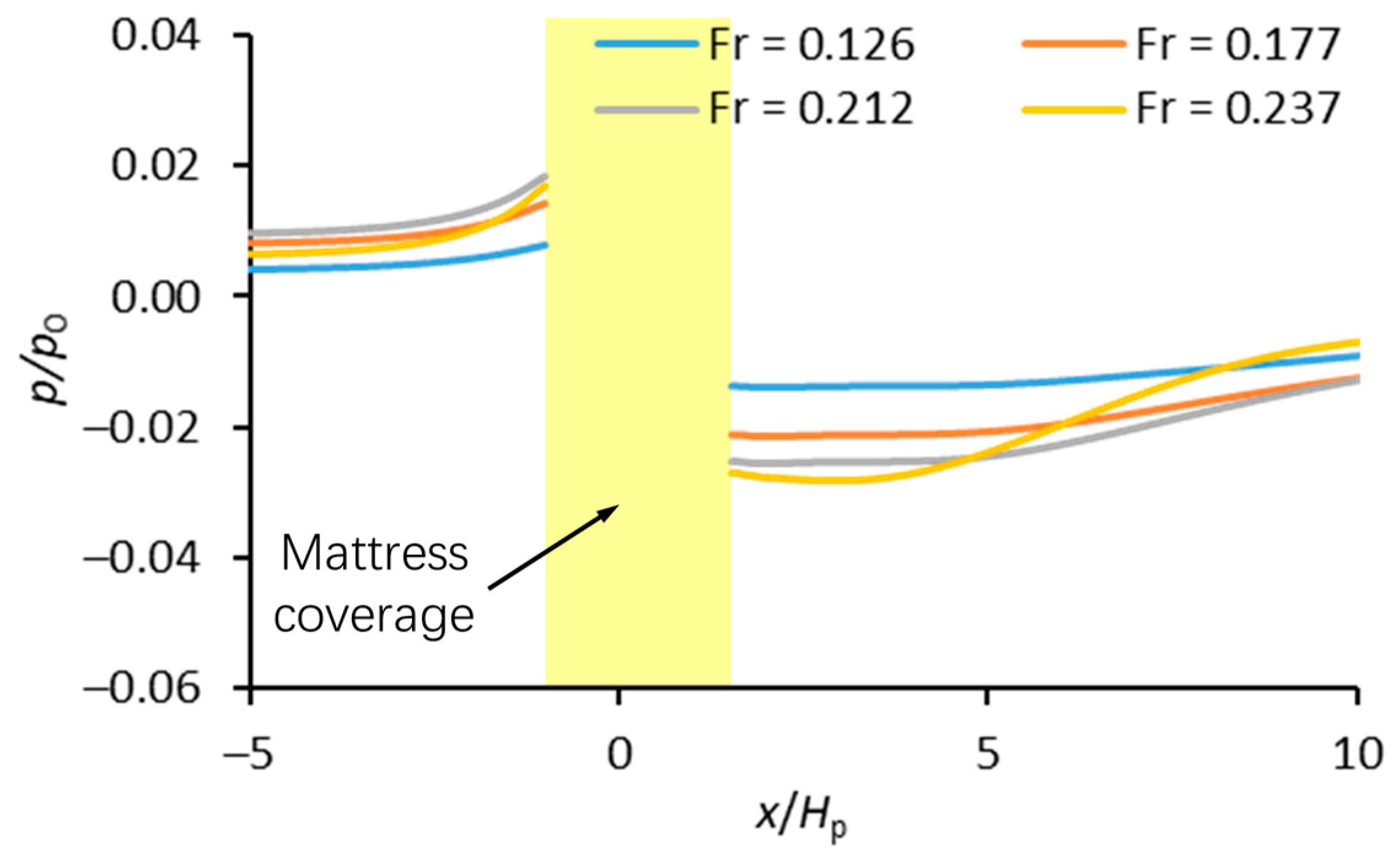
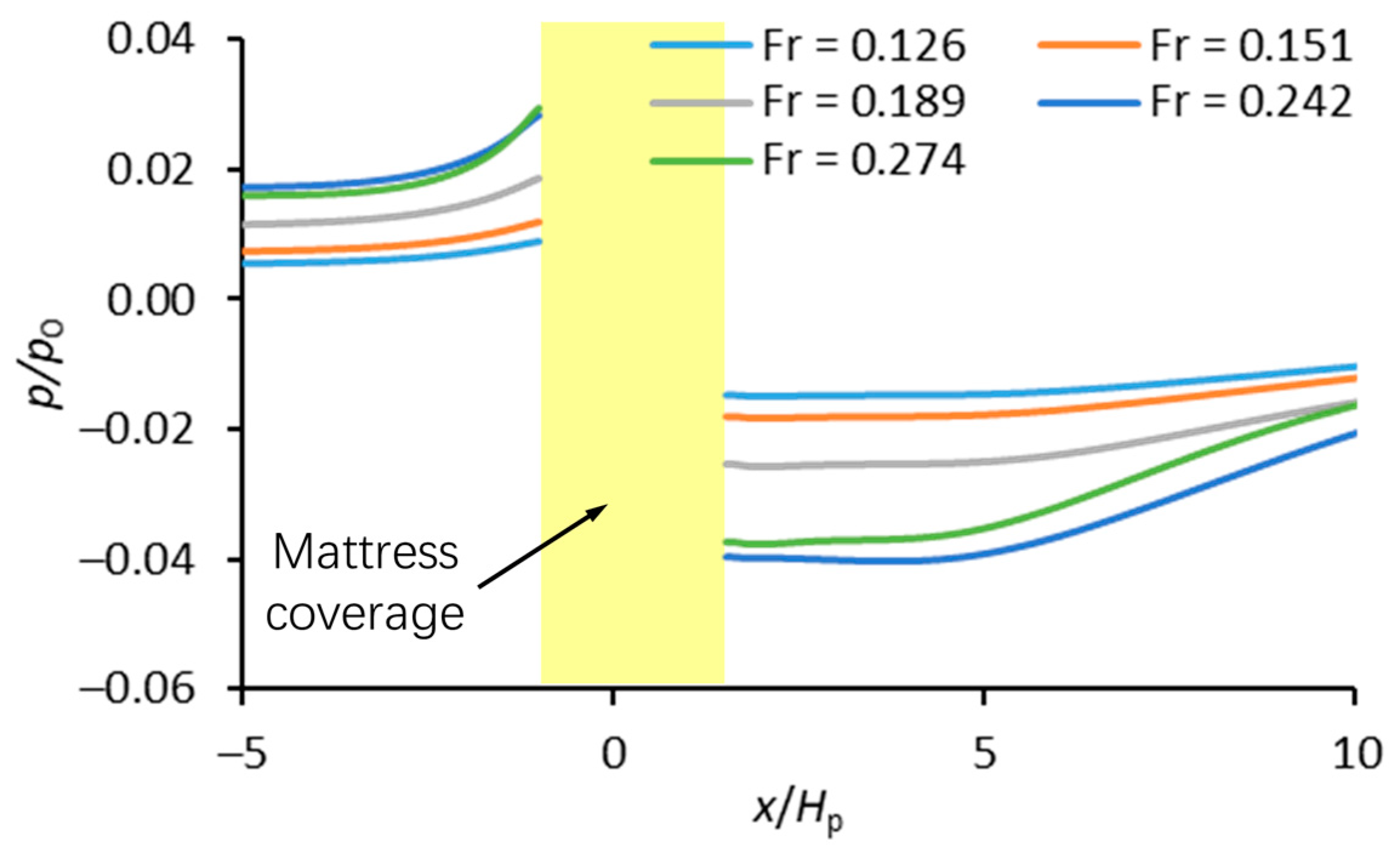
4.1. Effects of the Flow Velocity and the Buoyancy of the Floating Plate
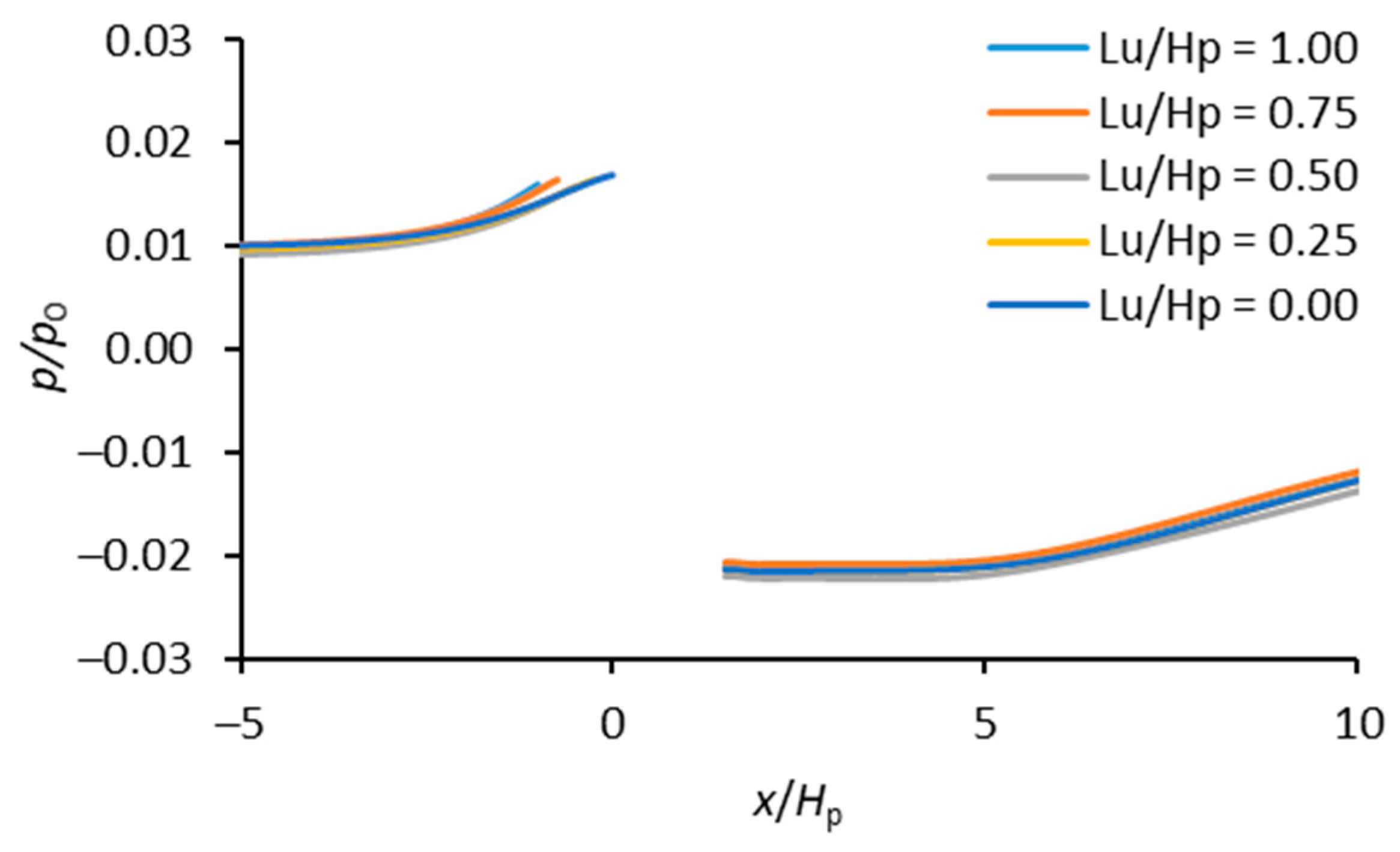
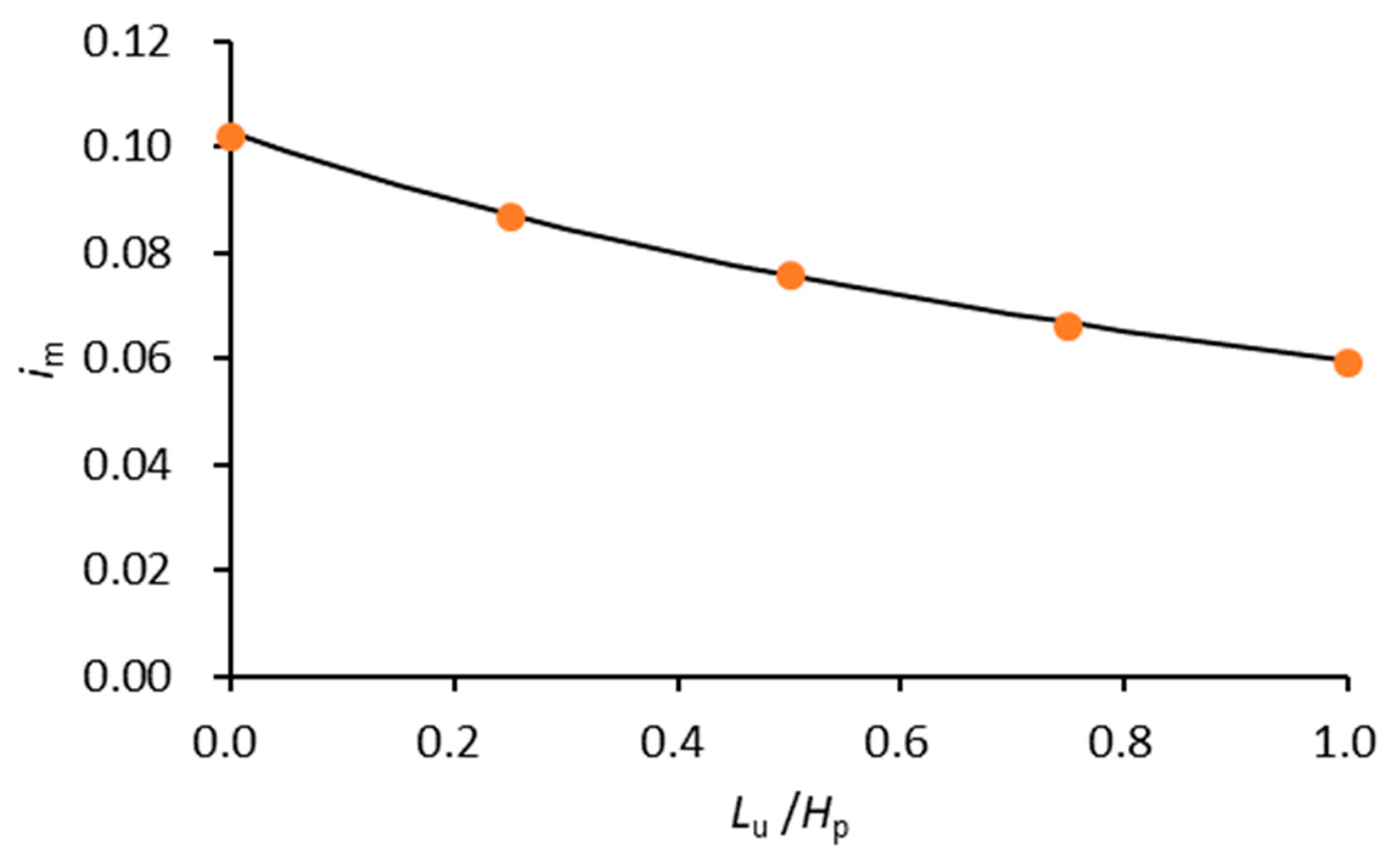
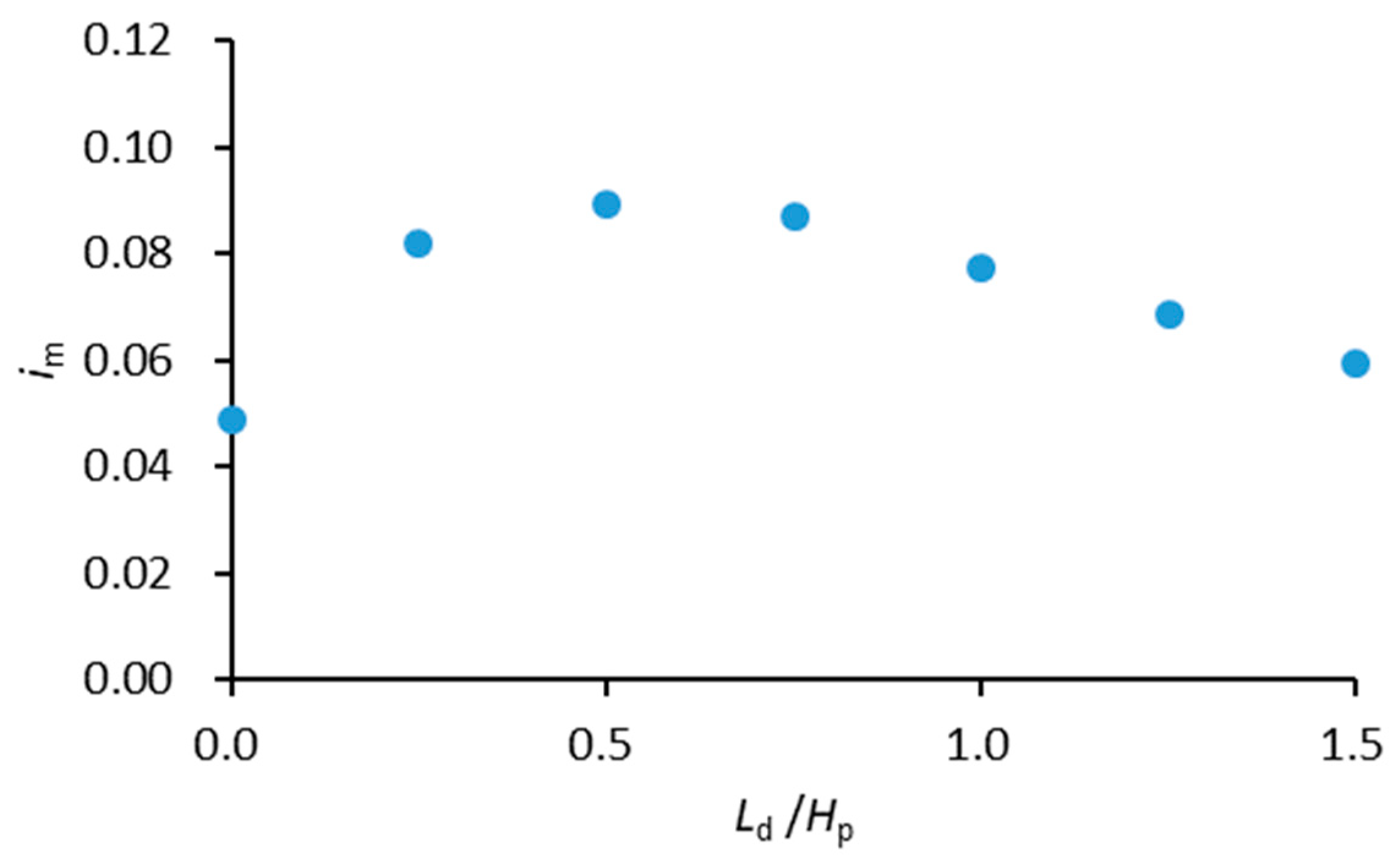
4.2. Effects of the Length of Geotextile Mattress
- The distribution pattern of bed pressure. When the mattress on the leeside of the floating plate was shortened, the pressure drop adjacent to the downstream edge of the mattress became more remarkable. The amplitude of bed pressure fluctuation also increased with the decrease in the downstream mattress length. This phenomenon can be associated with the variation of local flow pattern as the downstream mattress was shortened. A detailed discussion can be referred to in Section 5.2.
- The bed pressure on the downstream edge of the mattress. The bed pressure on the downstream edge of the mattress almost remained constant when Ld/Hp ≥ 1.0. With a further decrease in the downstream mattress length, the bed pressure on the downstream edge of the mattress gradually increased, but the increase was not remarkable for Ld/Hp ≥ 0.5. When Ld/Hp < 0.5, the rise of bed pressure on the downstream edge of the mattress became significant. This variation pattern can influence the effect of the downstream mattress length on the average seepage hydraulic gradient.
5. Discussion and Analysis
5.1. Effects of the Flow Velocity
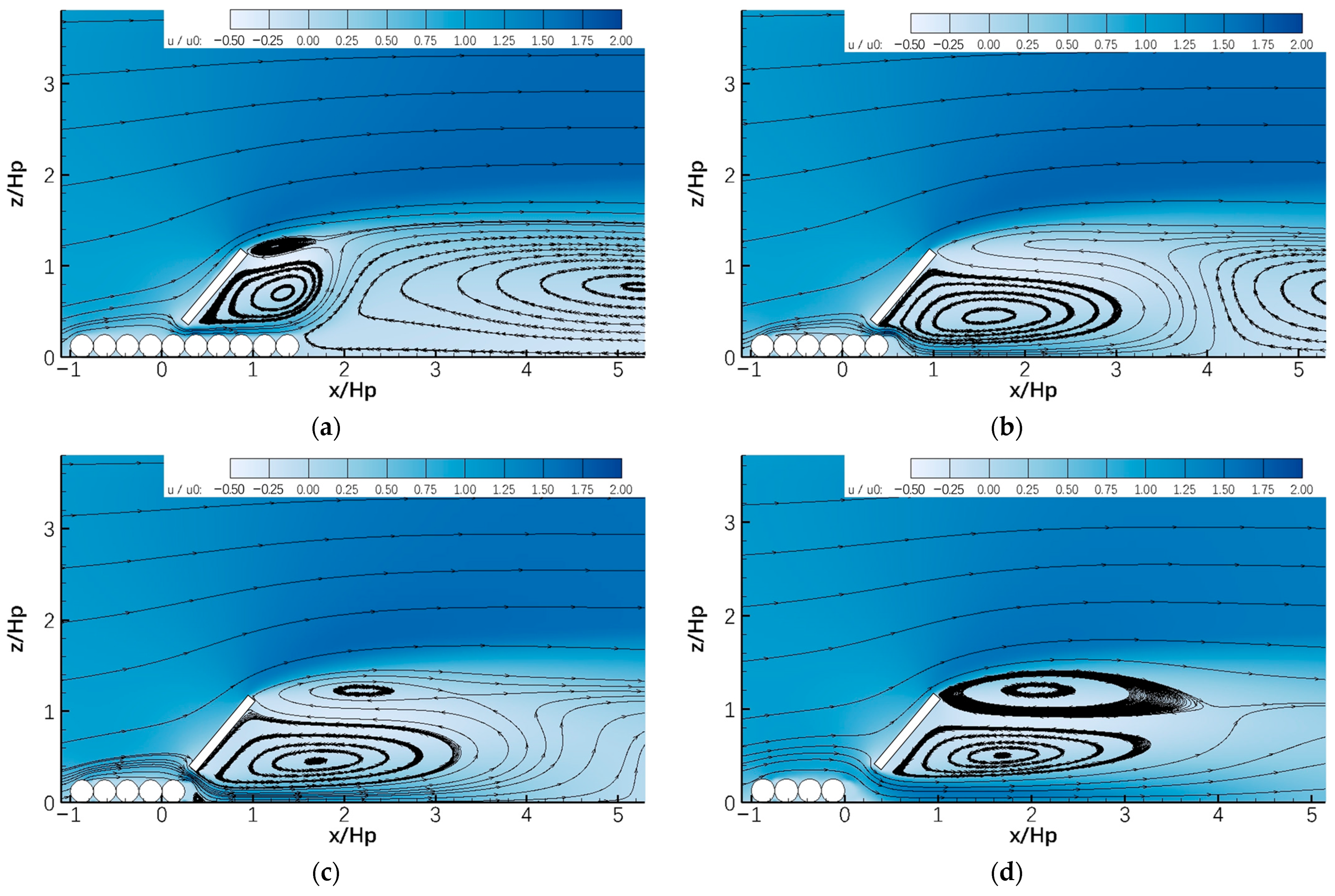
5.2. Effects of the Length of the Mattress Downstream of the Floating Plate
6. Conclusions
- The sloping angle of the floating plate descends almost linearly with the increase in the Froude number within the tested range of parameters. The prediction equation of a similar structure coincides well with the test results.
- The average hydraulic gradient increases nearly linearly with the Froude number for small Froude numbers, and then reaches a plateau. This variation pattern can be attributed to the coupled effects of the rising Froude number, which can intensify the blockage effect of the GMFP, and the decreasing sloping angle, which can weaken the blockage effect.
- The average hydraulic gradient is approximately inversely proportional to the mattress length upstream of the floating plate. The effect of the upstream mattress length on the bed pressure distribution is minimal.
- The average hydraulic gradient increases with the decreasing mattress length downstream of the floating plate when the downstream mattress length is over 0.5 times of the plate height. With the further drop in the downstream mattress length, the average hydraulic gradient gradually drops.
- The effect of the downstream mattress length can be explained by the variation in the vortices in the top vortex zone on the leeside of the GMFP. When the downstream mattress is shortened, the mattress can fail to provide enough friction to slow down the bottom flow through the sand-pass opening, and thus the vortices in the top vortex zone can become excessively developed, pushing the bottom vortex to the leeside.
- The shortened downstream mattress can increase the risk of the GMFP of overturning and slipping, although the average hydraulic gradient decreases.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
Appendix A. Brief Introduction to Some Specialized Terms
References
- Sumer, B.M. Flow–structure–seabed interactions in coastal and marine environments. J. Hydraul. Res. 2014, 52, 1–13. [Google Scholar] [CrossRef]
- Kuang, C.; Zheng, Y.; Gu, J.; Zou, Q.; Han, X. Experimental study on backflow patterns induced by a bilateral groin pair with different spacing. Appl. Sci. 2021, 11, 1486. [Google Scholar] [CrossRef]
- Zhang, R.; Chen, Y.; Yao, P.; Stive, M.J.F.; Zeng, J. Numerical simulations of effects of the layout of permeable pile groin systems on longshore currents. J. Mar. Sci. Eng. 2023, 11, 1823. [Google Scholar] [CrossRef]
- Jiang, L.; Zhang, J.; Tong, L.; Guo, Y.; He, R.; Sun, K. Wave motion and seabed response around a vertical structure sheltered by submerged breakwaters with Fabry–Pérot Resonance. J. Mar. Sci. Eng. 2022, 10, 1797. [Google Scholar] [CrossRef]
- Guo, L.; Qu, K.; Wang, X.; Huang, J.X. Numerical study on performance of submerged permeable breakwater under impacts of multi-directional focused wave groups. Ocean Eng. 2024, 302, 117665. [Google Scholar] [CrossRef]
- Mohammadnia, M.; Pak, A. A new method for designing cube armours for low-crested and submerged breakwaters. Ocean Eng. 2023, 281, 114769. [Google Scholar] [CrossRef]
- Wang, W.; Yan, J.; Chen, S.; Liu, J.; Jin, F.; Wang, B. Gridded cemented riprap for scour protection around monopile in the marine environment. Ocean Eng. 2023, 272, 113876. [Google Scholar] [CrossRef]
- Tang, Z.; Melville, B.; Shamseldin, A.Y.; Singhal, N.; Guan, D.; Stolte, A. Performance of riprap armour at vibrating offshore wind turbine monopile foundations. Coast. Eng. 2023, 186, 104392. [Google Scholar] [CrossRef]
- Chiew, Y.M. Mechanics of local scour around submarine pipelines. J. Hydraul. Eng. 1990, 116, 515–529. [Google Scholar] [CrossRef]
- Zhang, Z.; Shi, B.; Guo, Y.; Yang, L. Numerical investigation on critical length of impermeable plate below underwater pipeline under steady current. Sci. China Technol. Sci. 2013, 56, 1232–1240. [Google Scholar] [CrossRef]
- Zhu, Y.; Xie, L.; Su, T.-C. Scour protection effects of a geotextile mattress with floating plate on a pipeline. Sustainability 2020, 12, 3482. [Google Scholar] [CrossRef]
- Xie, L.; Huang, W.; Yu, Y. Experimental study of sediment trapping by geotextile mattress installed with sloping curtain. Geosynth. Int. 2013, 20, 389–395. [Google Scholar] [CrossRef]
- Li, Y.; Yu, G. Experimental investigation on flow characteristics at leeside of suspended flexible curtain for sedimentation enhancement. China Ocean Eng. 2009, 23, 565–576. [Google Scholar]
- Wang, H.; Si, F.; Lou, G.; Yang, W.; Yu, G. Hydrodynamic characteristics of a suspended curtain for sediment trapping. J. Waterw. Port Coast. Ocean Eng. 2015, 141, 04014030. [Google Scholar] [CrossRef]
- Xie, L.; Zhu, Y.; Li, Y.; Su, T.-C. Experimental study on bed pressure around geotextile mattress with sloping plate. PLoS ONE 2019, 14, e0211312. [Google Scholar] [CrossRef] [PubMed]
- Zhu, Y.; Xie, L.; Su, T.-C. Flow characteristics on the leeside of a geotextile mattress with floating plate. Ocean Eng. 2022, 265, 112624. [Google Scholar] [CrossRef]
- Flow Science Inc. FLOW-3D Documentation; Release 10.1.0; Flow Science, Inc.: Santa Fe, NM, USA, 2012; pp. 76–206. [Google Scholar]
- Li, L.; Zheng, J.; Peng, Y.; Zhang, J.; Wu, X. Numerical investigation of flow motion and performance of a horizontal axis tidal turbine subjected to a steady current. China Ocean Eng. 2015, 29, 209–222. [Google Scholar] [CrossRef]
- Zhang, J.; Gao, P.; Zheng, J.; Wu, X.; Peng, Y.; Zhang, T. Current-induced seabed scour around a pile-supported horizontal-axis tidal stream turbine. J. Mar. Sci. Technol. 2015, 23, 929–936. [Google Scholar]
- Hirt, C.W.; Nichols, B.D. Volume of fluid (VOF) method for the dynamics of free boundaries. J. Comput. Phys. 1981, 39, 201–225. [Google Scholar] [CrossRef]
- Zheng, Z.; Hu, Z.; Xie, X.; Huang, W. Local scour around the monopile: A microscopic perspective using CFD-DEM. Ocean Eng. 2024, 299, 117318. [Google Scholar] [CrossRef]
- Groynes. Available online: https://www.coastalwiki.org/wiki/Groynes (accessed on 28 October 2024).
- Groin. Available online: https://www.britannica.com/technology/groin (accessed on 28 October 2024).
- Riprap: Merriam-Webster.com Dictionary. Available online: https://www.merriam-webster.com/dictionary/riprap (accessed on 28 October 2024).
- A New Specification for Geotextile Grout Filled Mattresses. Available online: https://geosyntheticsmagazine.com/2021/04/01/a-new-specification-for-geotextile-grout-filled-mattresses (accessed on 28 October 2024).
- Seepage in Civil Engineering: Definition, Effects, Remedial Measures. Available online: https://testbook.com/civil-engineering/seepage-in-civil-engineering (accessed on 28 October 2024).
- Piping Failure in Hydraulic Structures. Available online: https://www.elementaryengineeringlibrary.com/civil-engineering/soil-mechanics/piping-failure-in-hydraulic-structures (accessed on 28 October 2024).
- RNG k-Epsilon Model. Available online: https://www.cfd-online.com/Wiki/RNG_k-epsilon_model (accessed on 28 October 2024).






| Case | Fr | FB (N/m) |
|---|---|---|
| 001 | 0.126 | 9.8 |
| 002 | 0.177 | 9.8 |
| 003 | 0.212 | 9.8 |
| 004 | 0.237 | 9.8 |
| 005 | 0.126 | 14.7 |
| 006 | 0.189 | 14.7 |
| 007 | 0.243 | 14.7 |
| 008 | 0.274 | 14.7 |
| Group | Case | Fr | FB (N/m) | Lu/Hp | Ld/Hp |
|---|---|---|---|---|---|
| A | A01 | 0.126 | 9.8 | 1.00 | 1.50 |
| A02 | 0.177 | 9.8 | 1.00 | 1.50 | |
| A03 | 0.212 | 9.8 | 1.00 | 1.50 | |
| A04 | 0.237 | 9.8 | 1.00 | 1.50 | |
| A11 | 0.126 | 14.7 | 1.00 | 1.50 | |
| A12 | 0.151 | 14.7 | 1.00 | 1.50 | |
| A13 | 0.189 | 14.7 | 1.00 | 1.50 | |
| A14 | 0.243 | 14.7 | 1.00 | 1.50 | |
| A15 | 0.274 | 14.7 | 1.00 | 1.50 | |
| B | A02 | 0.177 | 9.8 | 1.00 | 1.50 |
| B01 | 0.177 | 9.8 | 0.75 | 1.50 | |
| B02 | 0.177 | 9.8 | 0.50 | 1.50 | |
| B03 | 0.177 | 9.8 | 0.25 | 1.50 | |
| B04 | 0.177 | 9.8 | 0.00 | 1.50 | |
| B11 | 0.177 | 9.8 | 1.00 | 1.25 | |
| B12 | 0.177 | 9.8 | 1.00 | 1.00 | |
| B13 | 0.177 | 9.8 | 1.00 | 0.75 | |
| B14 | 0.177 | 9.8 | 1.00 | 0.50 | |
| B15 | 0.177 | 9.8 | 1.00 | 0.25 | |
| B16 | 0.177 | 9.8 | 1.00 | 0.00 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zhu, Y.; Wang, Q.; Wu, G.; Li, Y.; Xie, L. Effects on the Potential for Seepage Failure Under a Geotextile Mattress with Floating Plate. J. Mar. Sci. Eng. 2024, 12, 1975. https://doi.org/10.3390/jmse12111975
Zhu Y, Wang Q, Wu G, Li Y, Xie L. Effects on the Potential for Seepage Failure Under a Geotextile Mattress with Floating Plate. Journal of Marine Science and Engineering. 2024; 12(11):1975. https://doi.org/10.3390/jmse12111975
Chicago/Turabian StyleZhu, Yehui, Qiyun Wang, Guokai Wu, Yanhong Li, and Liquan Xie. 2024. "Effects on the Potential for Seepage Failure Under a Geotextile Mattress with Floating Plate" Journal of Marine Science and Engineering 12, no. 11: 1975. https://doi.org/10.3390/jmse12111975
APA StyleZhu, Y., Wang, Q., Wu, G., Li, Y., & Xie, L. (2024). Effects on the Potential for Seepage Failure Under a Geotextile Mattress with Floating Plate. Journal of Marine Science and Engineering, 12(11), 1975. https://doi.org/10.3390/jmse12111975






