On the Digital Twin of The Ocean Cleanup Systems—Part I: Calibration of the Drag Coefficients of a Netted Screen in OrcaFlex Using CFD and Full-Scale Experiments
Abstract
1. Introduction
- The cleanup system catches plastic at the sea surface. Trawling is mostly performed close to the sea bed or at mid-water depth. That means that the netting, ropes, and floaters of the cleanup system are exposed to cyclic wave-induced forces for longer time periods than fishing rigs. In turn, that leads to increased wear and tear, as has been verified during the System 002 test campaign.
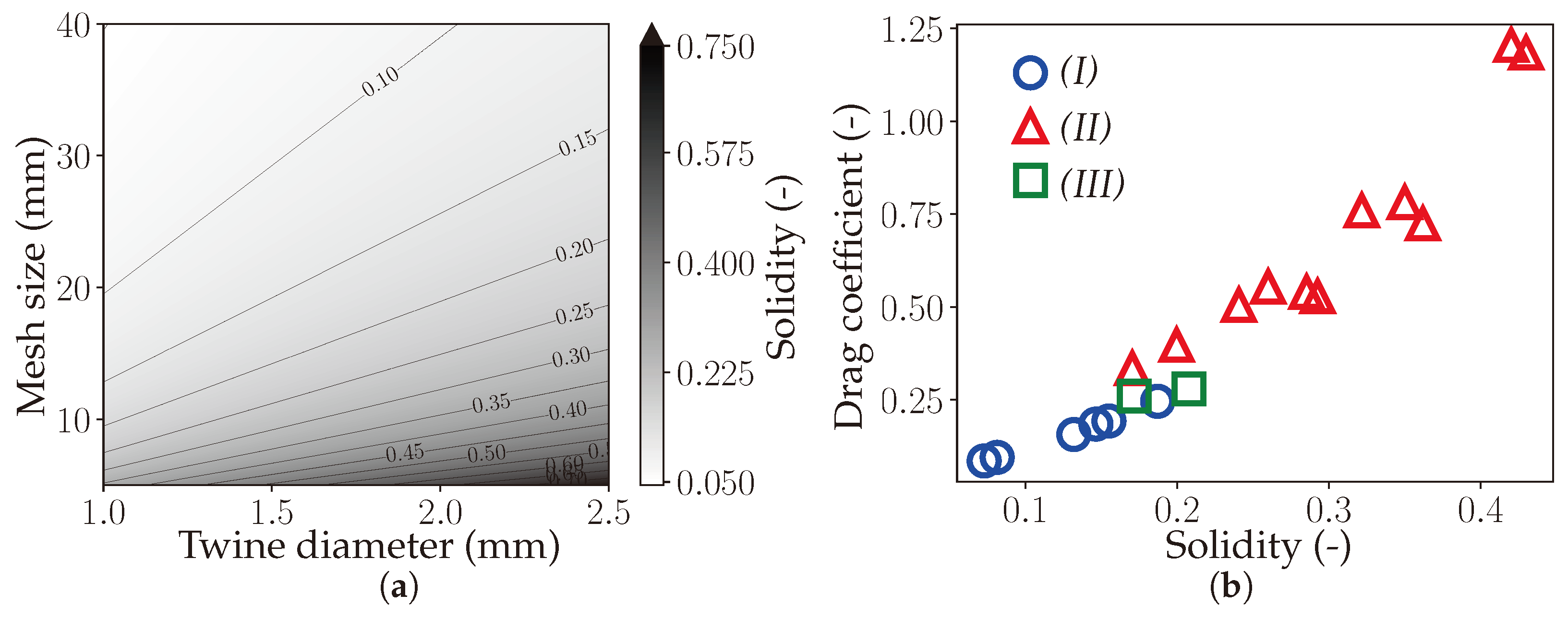
- The cleanup system netting of System 002 has a mesh size of 10 mm to catch also mesoplastics, which are plastics with sizes between 0.5 cm and 5 cm. The netting used in the trawling has normally a mesh size larger than 10 mm. That means that the drag force on a 800 m-long system (System 002) or 2 km-long system (System 03) is considerably larger than from trawling. As a consequence, understanding this force and the variables influencing it is particularly important for the cleanup application.
- The wingspan of System 002 adopts a “U-shape” during operation, and the netting draft is 3 m. That means that the bottom of the system is completely open, allowing fish to easily escape. Additionally, in the retention zone’s bottom, there are openings to allow marine life to escape. This emphasizes that the purpose is to capture plastics, not fish. Therefore, since the netting configuration is different from fishing nets, it is valuable to understand how this new application of netting performs in water, how it interacts with marine life, and how the offshore operation around it must be efficient to make it work. All these points were thoroughly studied and monitored within The Ocean Cleanup, and the present work is part of it.
- An important difference between catching plastic and fishing is how dispersed the plastic is at the ocean’s surface, in comparison to a dense school of fish. There is a large amount of plastic floating in the GPGP, but it circulates on a massive area of the North Pacific Ocean. That means that the area density of the catch that the cleanup system encounters is much lower than in the fishing case. As a consequence, the cleanup system needs to sweep a much larger area to match a fish catch with a plastic catch. Additionally, The Ocean Cleanup objective is to get rid of 90% of the floating plastic in the GPGP by 2040, but it is not the objective of the fisheries to deplete fish to that level. Therefore, the energy invested in cleaning is larger than in fishing, making the efficiency and the hydrodynamic performance of the cleanup system even more relevant.
- OrcaFlex is used for the dynamic analysis of offshore marine systems, including floating structures, moorings, and risers. We used it for simulating the behavior of our complex ocean cleanup system under different environmental conditions such as waves, currents, and winds. It provides a comprehensive set of features for modeling the geometric structures of the system and its dynamic response, as well as that of the lines to tow the system. It is based on Morison’s equation [30] and potential flow to model the forces on a structure generated by the fluid flow, such as the forces on a mooring line due to waves. It takes into account the inertia and drag forces of the fluid, as well as the added mass of the structure.
- AquaSim [31,32] is used to predict the behavior of aquatic ecosystems and the impacts of environmental stressors. It uses numerical methods to solve the flow around fishing farms and the resulting loads. It is a Finite-Element Analysis (FEA) program, which allows further details on the physics of interest. On the one hand, AS is ideal to increase the level of accuracy of the drag calculation on the cleanup system. On the other hand, the twine-by-twine calculation increases the computational time, specially in irregular waves. Since AS includes a formula to account for the shielding effects, it is seen, from the perspective of the present work, as an initial approximation to that effect.
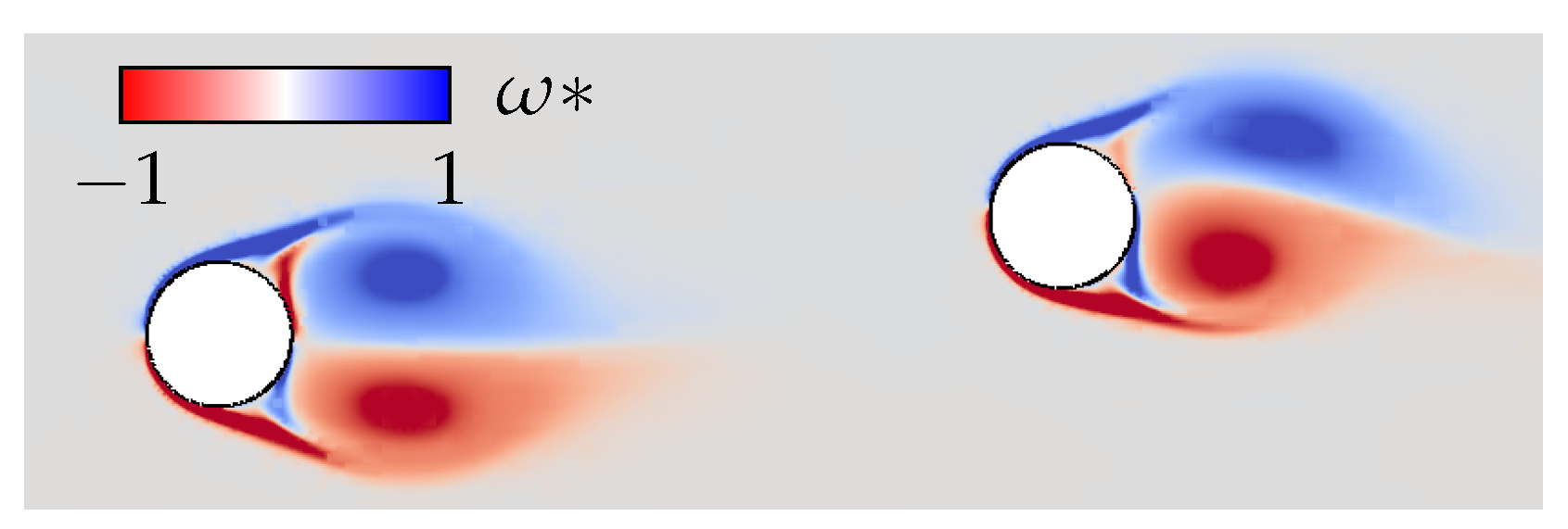
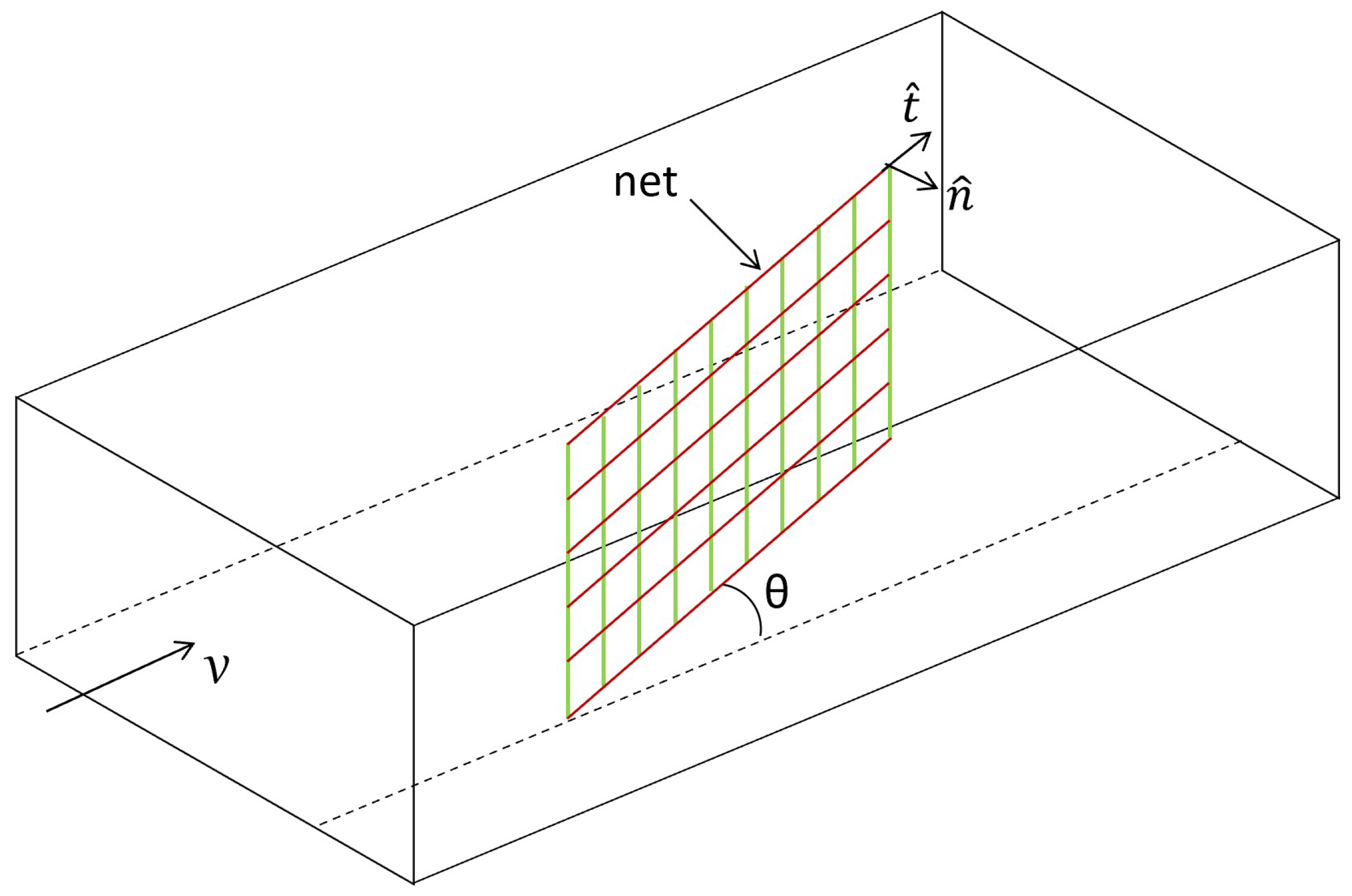
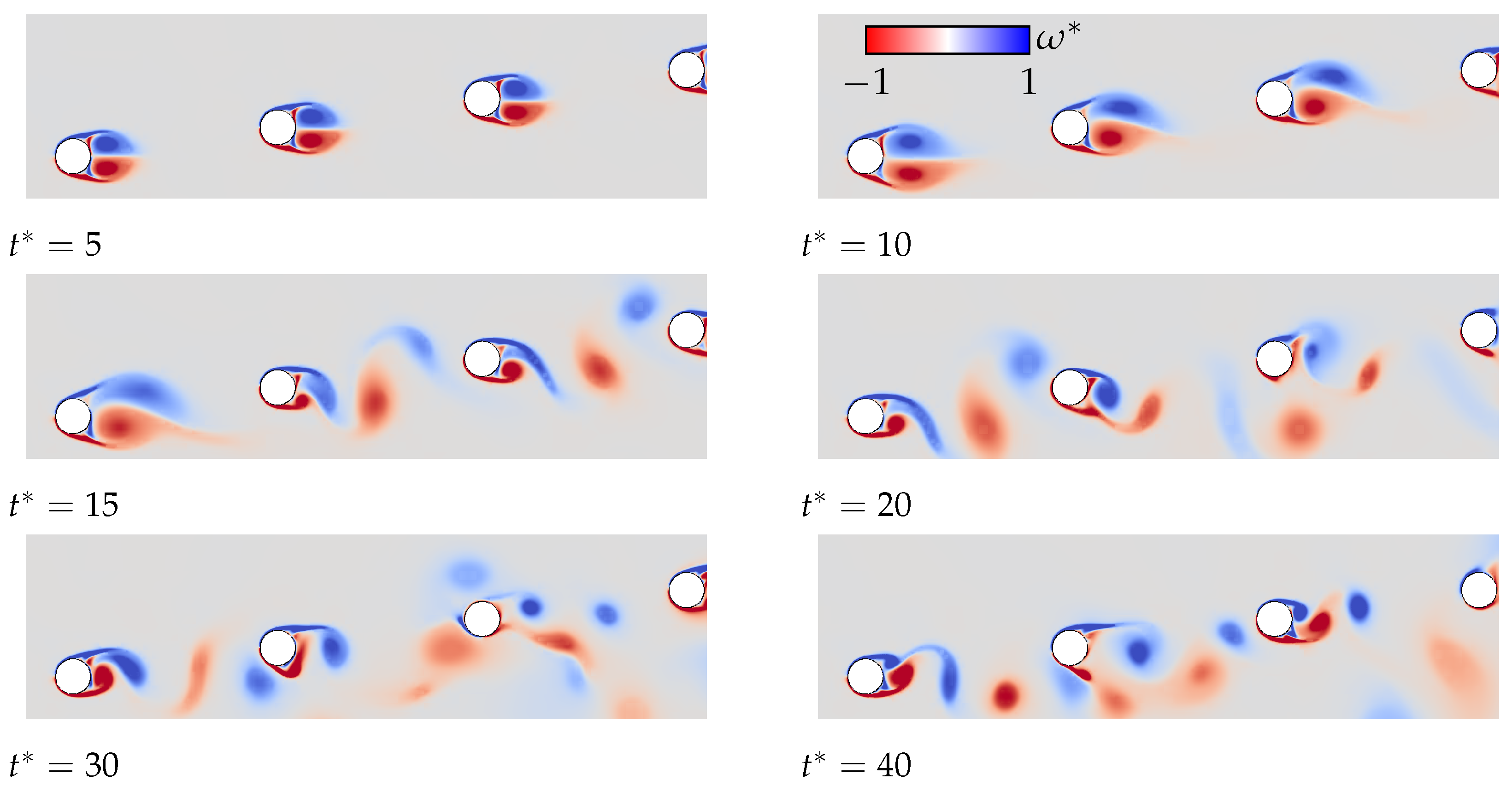
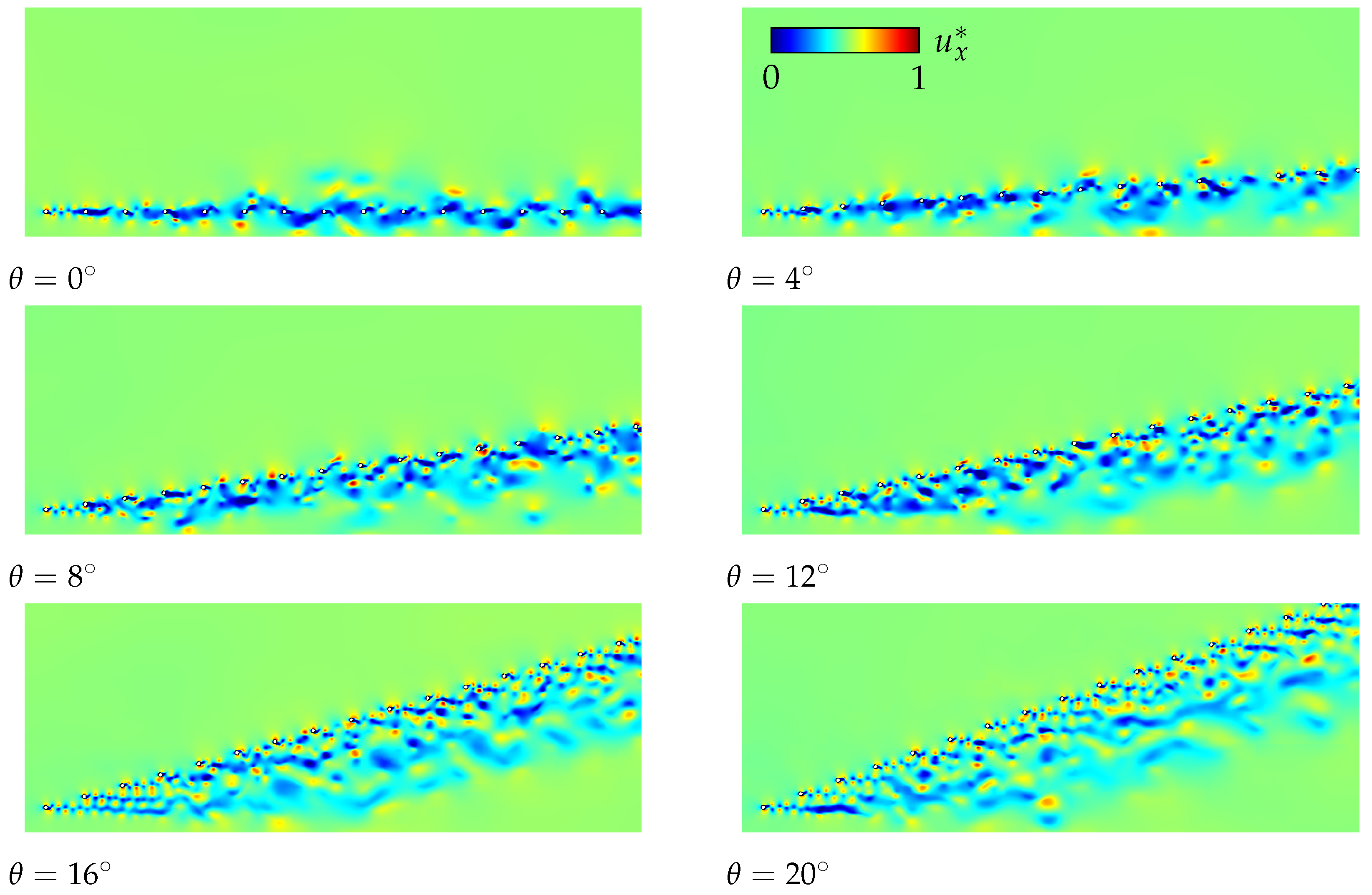
- Basilisk [33,34,35] is a Direct Numerical Simulation (DNS) solver, which offers an accurate insight into the flow around the twines, especially if they are in tandem at low . For this purpose, we highlight the cross-sectional flow features impacting the drag coefficient around individual twines to trigger the shielding effect due to the various angles of attack of the net.
2. Materials
2.1. GPGP Measured Data
- The mean tension was calculated for periods of 3 h, for each of the towing lines. The sum of the mean values for each towline provides the combined towline tension, which is the variable to be validated in the present work.
- The error associated with the combined towline tension was calculated. Taking the mean tension for a specific time period introduces an error because the true mean value, for a specific metocean condition, can be anywhere between the extreme values of the dynamic response. Therefore, the difference between the calculated mean and the maximum tension for the 3 h period was taken as the associated error.
- The RLM has a high accuracy, typically between 0.5% and 2.0% of the measured load. The associated error described in the previous point is typically higher than the error associated with each instant measurement.
- A Doppler Velocity Logger (DVL) supplies the of the vessel, which is registered on a computer on board the vessel.
- The Speed Over Ground (SOG) of the vessel combined with the sea surface current velocity estimation, from the X-band radar of the vessel.
- The DVL on the vessels has an accuracy of 1.5%.
- Since two sources of data are considered to calculate the average value, an error of half the difference between the two measured values is introduced.
- Finally, the maximum between the previous two errors is taken as the speed-through-water-associated error.
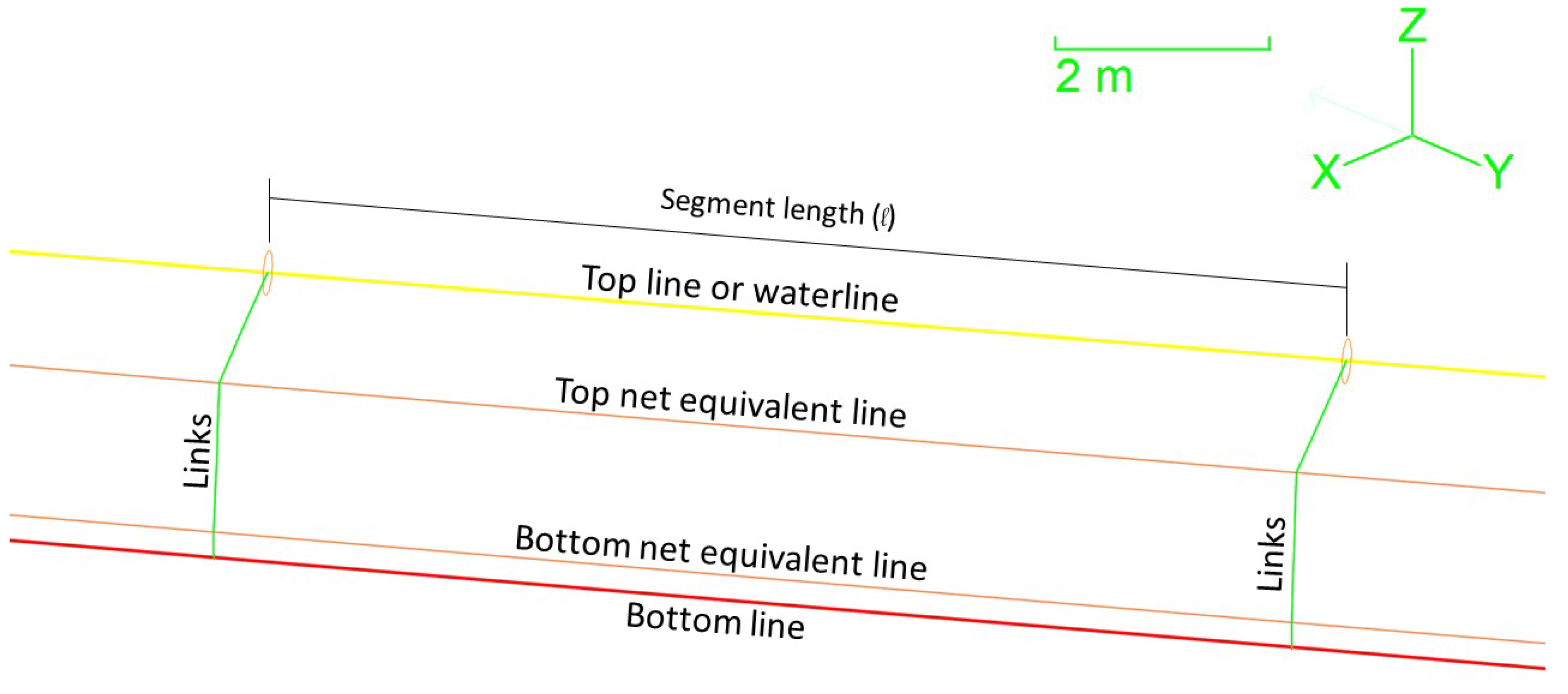
2.2. OrcaFlex
2.3. AquaSim
2.4. Basilisk
- Continuity equation:
- Combined momentum equations:
3. Method
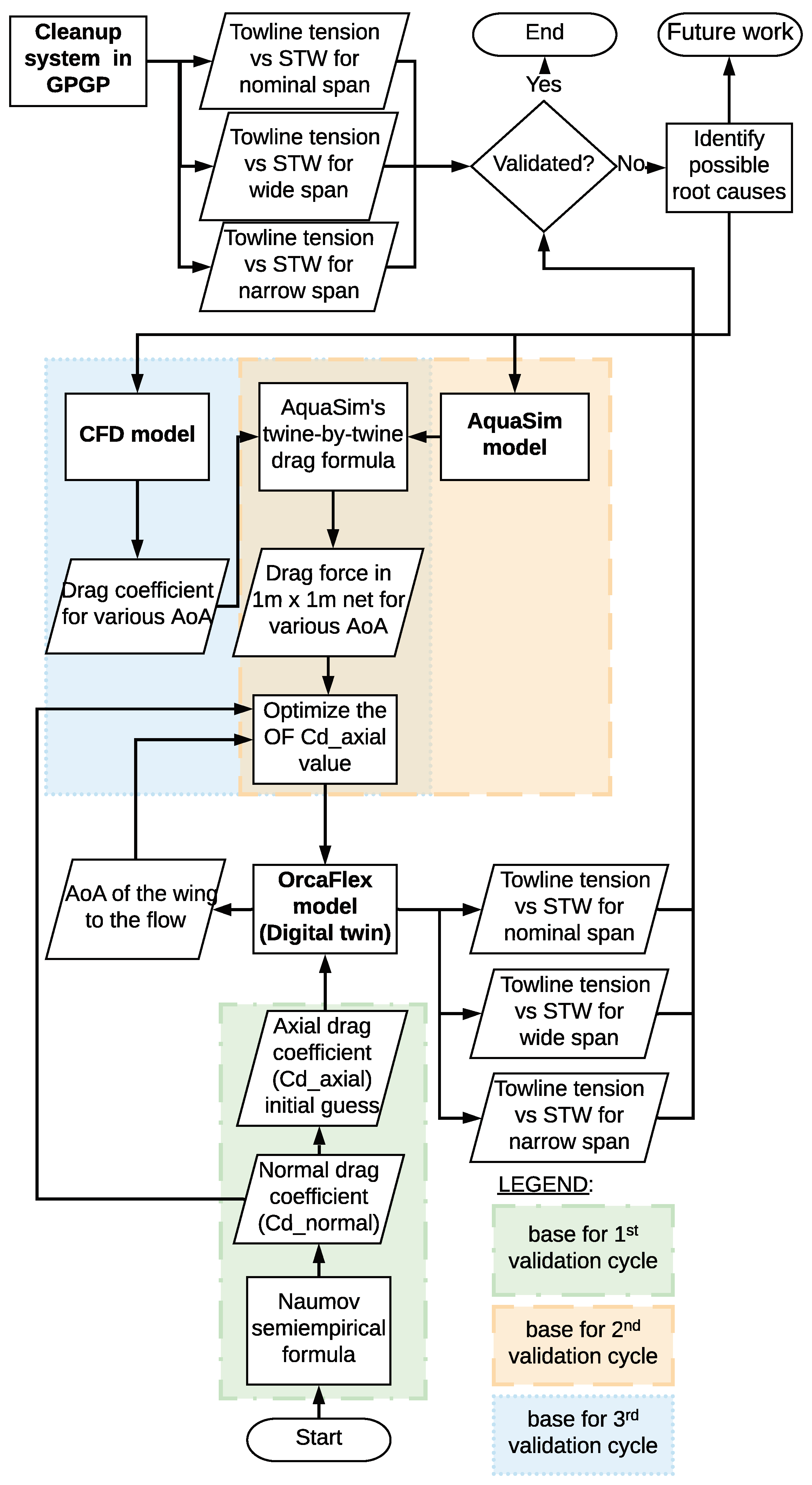
3.1. Overview of the Method
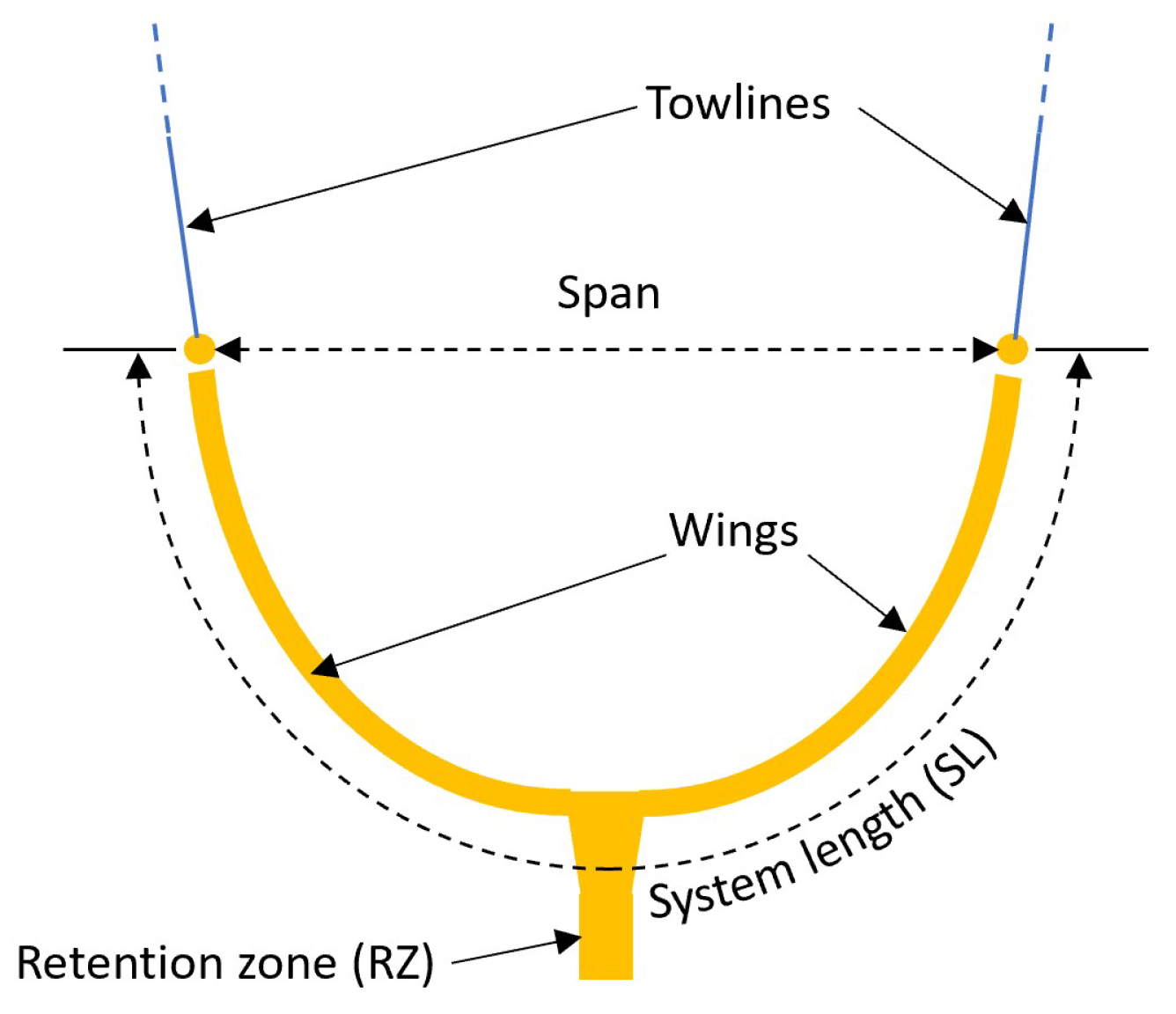
- The variable to validate is the combined towline tension, defined as the sum of the tensions in each towline, for various speeds through water . The span is the distance between the wing’s extremities as depicted in Figure 8. The figure also shows the System Length (), towlines, wings, and Retention Zone (RZ). The System Ratio () is defined as the ratio between the span and the system length ().Three span values are considered as shown in Table 1 for System 002’s length equal to 800 m:
- The OF model validation was performed in three cycles:
- First cycle: Simulation based on the initial estimation of the OF axial drag coefficient . This step is detailed in Section 3.2.
- Second cycle: Triggered by discrepancies between the model and the GPGP data, especially for a narrow span, we used the AS model of a 1 m × 1 m piece of the system’s net at multiple . Then, the difference between the drag forces on a 1 m × 1 m section of the net from OrcaFlex and AquaSim was calculated and written as:Then, the Root Mean Square (RMS) of the set of differences for a group of , corresponding to a certain span, can be calculated:where is the total number of angles of attack for the optimization case. The optimization objective is to obtain the value that produces the minimum RMS for the specific optimization case. In that way, the OF drag force will be close to the AS drag force. With this optimization, the accuracy of a twine-by-twine calculation and the shielding effect can be included in OF, leading to more-accurate load estimations. Further details about the OrcaFlex modeling are seen in Section 3.2.
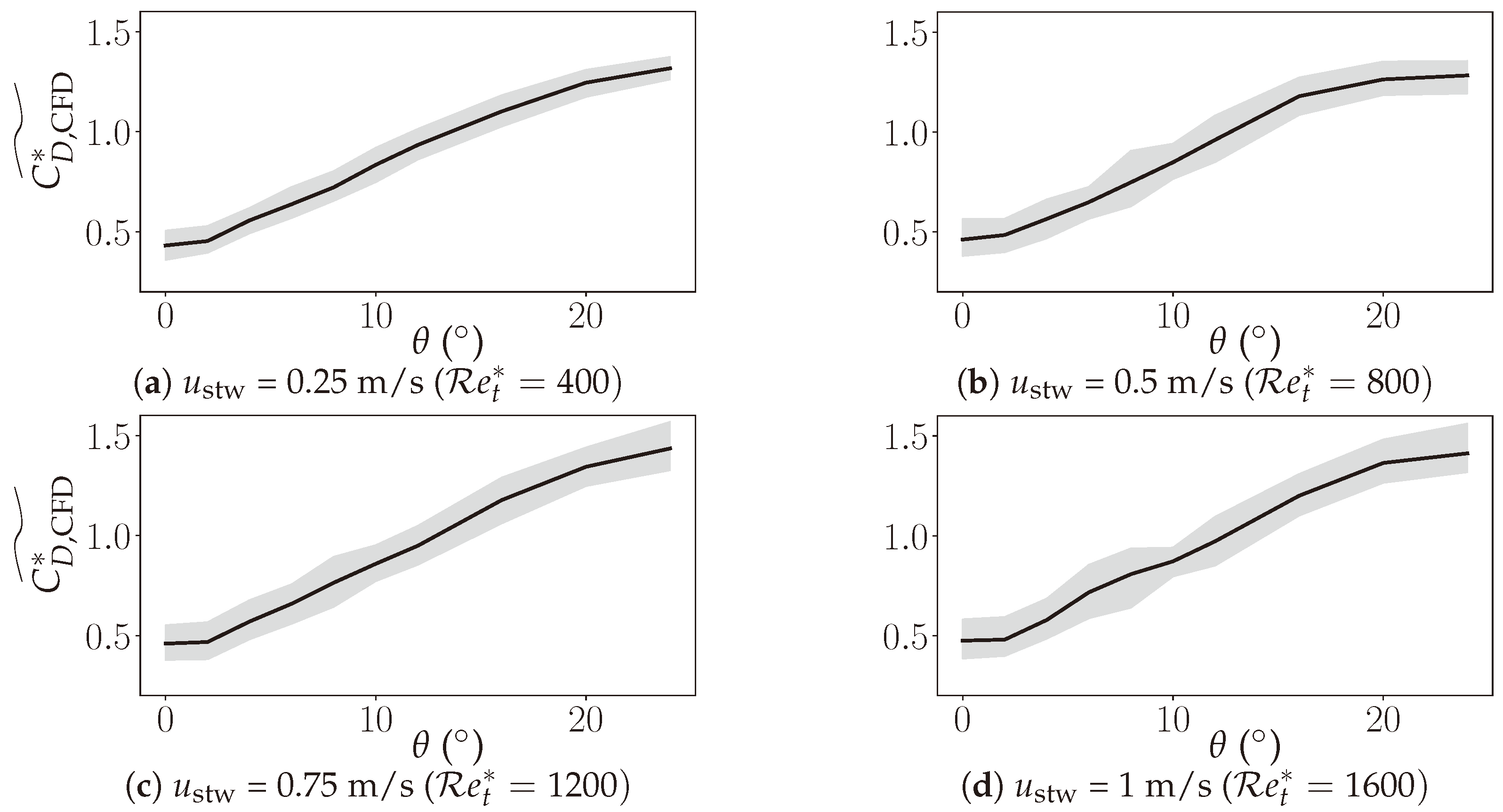
- Third cycle: Triggered by the necessity to verify the AS results, using a CFD model, a two dimensional piece of the net was simulated for various to quantify the effect of the vortex shedding on the twines in tandem on the average drag coefficient. This gives a piecewise definition of the average drag coefficients of the net. They are given as a function of and and used in the AS drag force Equation (28), replacing the AS drag coefficients. Then, the same optimization (Equations (20) and (21)) can be carried out one more time.
3.2. OrcaFlex Drag Force Calculation
- Due to the way the net is modeled, since potential flow and one-way coupling were considered, there was no option to directly include or capture what is called the shielding effect between the twines of a submerged net. In contrast, the drag calculation in the software AquaSim and Basilisk does include this effect.
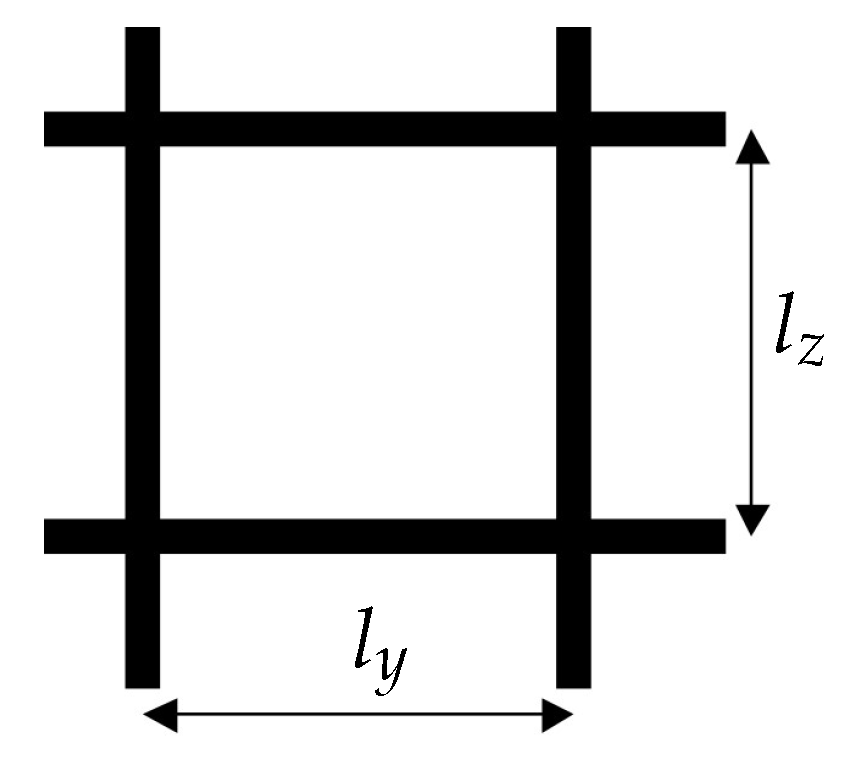
- Due to the way the net was modeled, it was not possible to calculate the drag twine-by-twine and actually differentiate between horizontal and vertical twines. In contrast, the drag calculation in the software AquaSim does include this option.
- The effect of the underwater cross-section shape of the net has on the drag force was not included in the model. Both OF normal drag coefficients were considered equal. That is, . This effect can be included by considering the torsion of the drag equivalent line, but that considerably increases the computation time.
3.3. AquaSim Drag Force Calculation
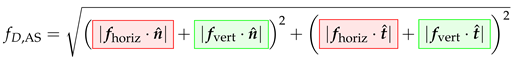

3.4. Comparing GPGP Data with Simulation Results
3.5. Problem Setup for CFD Model
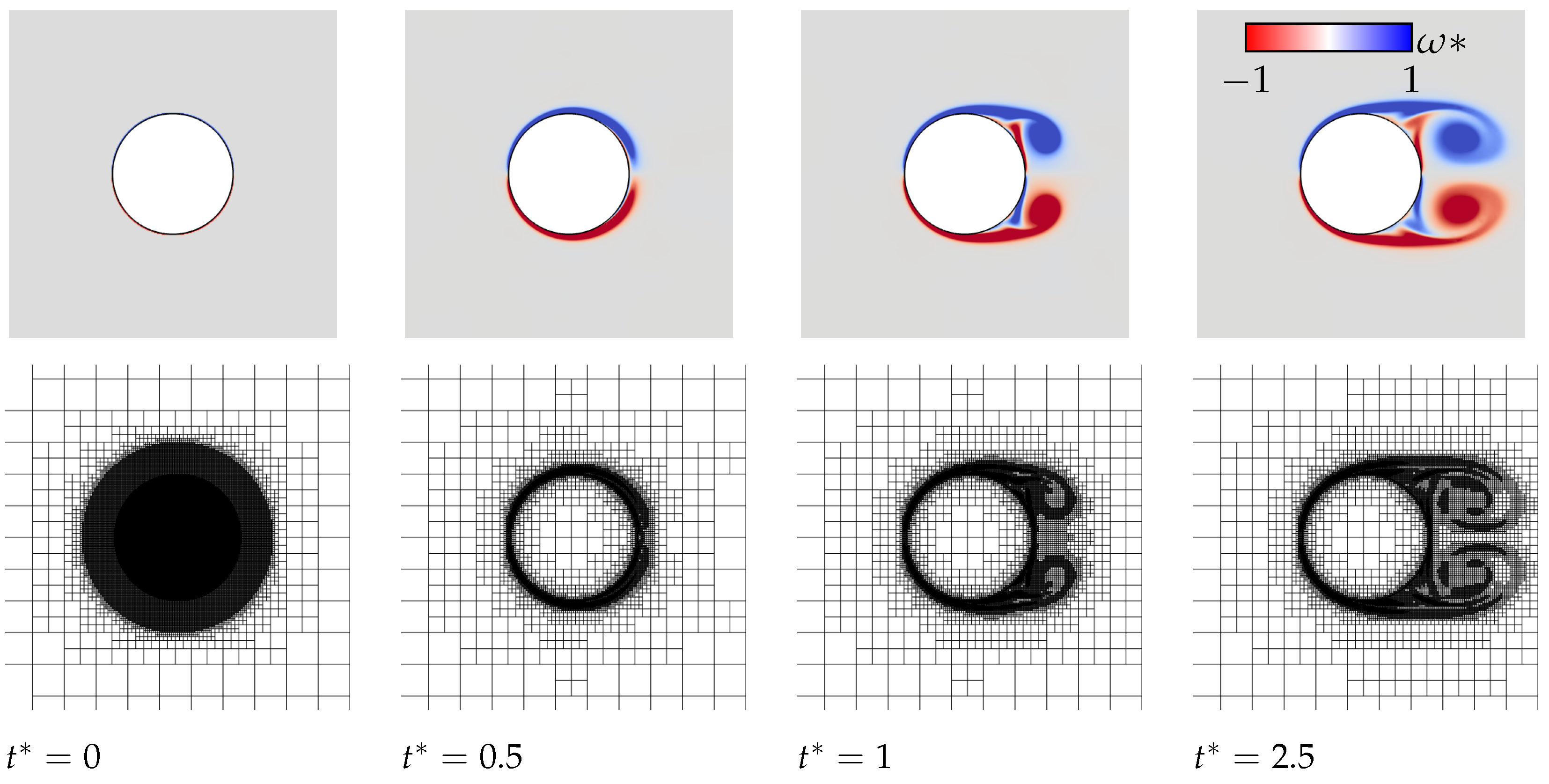
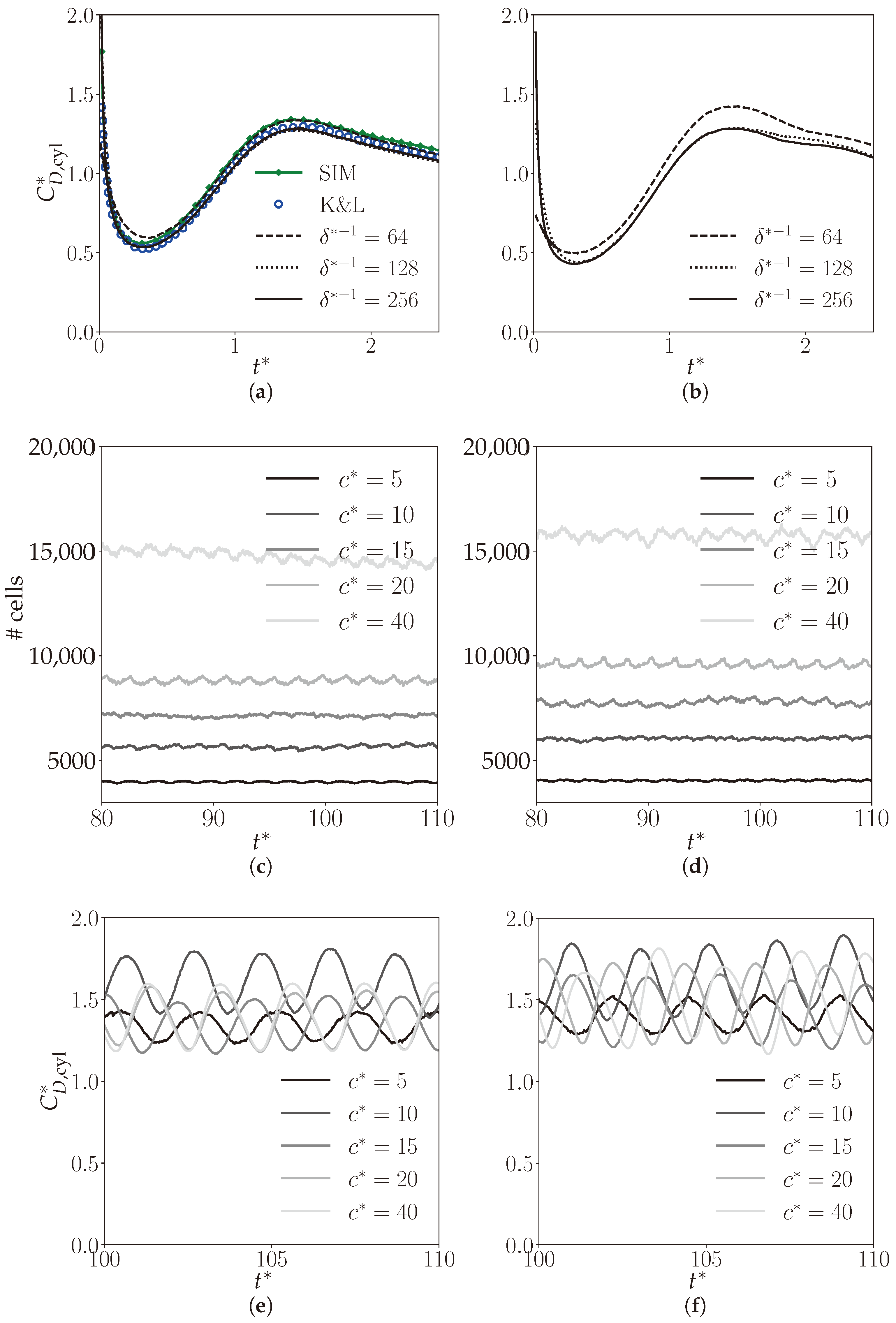
3.5.1. Space Convergence on Flow Past a Circular Cylinder
3.5.2. Convergence on the Drag Coefficient of Multiple Twines in Tandem at Various Angles of Attack
4. Results
4.1. First Validation Cycle
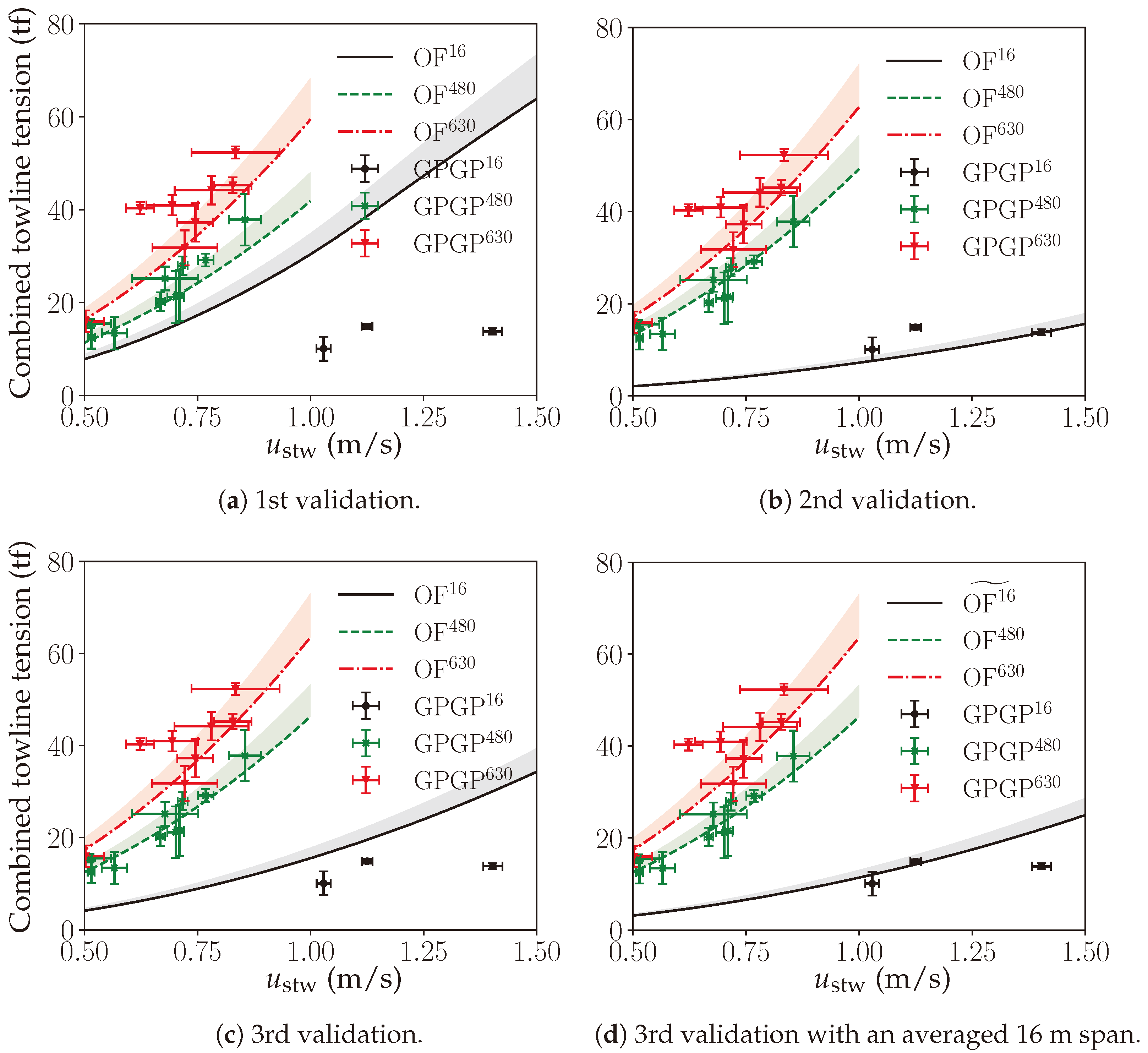
- The axial drag coefficient led to a DT estimation larger than the measured data in the narrow span. Specifically, the average deviation between the 15% increase area and real-world data was about 276%. The main phenomenon that was not captured by OF in this case was the shielding effect (cf. Section 2.3 for an explanation on this effect).
- The axial drag coefficient produced a 15% area that was close to the GPGP data points for the nominal span. Nevertheless, the majority of the data points were still outside of the area, and the computed mean deviation was about −3% (minus sign meaning an underestimation of the ground truth). The expectation was that the second and third validation cycle curves will comply even better with the validation criteria. The reason behind this expectation is that was not optimized for the angles of attack to the flow and the twine-by-twine calculation had not been included yet.
- The axial drag coefficient produced a 15% area that was close to the GPGP data points for a wide span. Even some data points were inside the area, and the calculated mean deviation was just 1%. That means that the OF model might be considered as validated at this cycle for a wide span. Nevertheless, the expectation was that the 2nd and 3rd validation cycle curves would comply with the validation criteria in a more-conservative manner, following the same reasoning as for the nominal span.
4.2. Second Validation Cycle
4.2.1. Optimization Cases
4.2.2. Findings Based on Second validation Cycle Results
- The optimized for a narrow span was definitely more accurate than the first cycle value. That demonstrated the influence of the shielding effect on the drag coefficients. Nevertheless, the 15% increase area was still below the data around m/s and m/s. This means that the DT was slightly underestimating for narrow span. The mean deviation in this case was −10%.
- The optimized also produced a more-accurate and more-conservative nominal span curve, in comparison with the first cycle, since all data points between m/s and 1.0 m/s were inside the 15% increase area or below the curve. Having points below the curve might mean that the second cycle curve was slightly overestimating the towline tension for nominal span. Indeed, the mean deviation was about 14%.
- The optimized led to the majority of the data points falling inside the 15% increase area. The mean deviation was computed as 6%. Then, the DT can be considered as validated and sufficiently overestimating for a wide span. This also means that the AS calculation was enough to calibrate the OF axial drag coefficient and to obtain a proper estimation of the towline tension in a wide span.
4.3. Third Validation Cycle
- The optimized for a narrow span produced more-accurate results than the first cycle. The results were also more conservative than the second cycle ones. Nevertheless, the curve in no waves was above all points. This means that the DT was overestimating, specifically by a mean deviation of 97%. This overestimation might be due to a missing phenomenon or effect related to the flexibility of the net and the shape it adopts under water. In spite of the overestimation and from an engineering perspective, the 3rd cycle curve was preferred over the 2nd cycle curve. A better option, again from an engineering perspective and to obtain more-accurate results, is to average both curves. That averaged result is plotted in Figure 19d. In terms of validation, it can be said that the DT had not been validated yet for the narrow span. The validation for narrow span depends on future studies. Nevertheless, the validation effort brought the 276% deviation of the 1st cycle to about a −10% deviation after the 2nd cycle and to about 97% after the 3rd cycle. If the averaging option was applied, the combined deviation was about 43%.
- The optimized produced a more-accurate nominal span curve than for the second cycle, since almost all GPGP data points were inside the 15% increase area curve. The mean deviation in this case was just 7%. This also means that the DT was validated for a nominal span.
- The optimized led to the majority of the GPGP data points falling inside the 15% increase area, and the mean deviation was calculated to be 8%. Then, the DT at the third cycle can be considered as validated for a wide span. Since the wide span curve was considered as validated already on the second cycle, this result also means that the CFD calculation verified the AS calculation for a wide span.
4.4. Calibrated Drag Coefficients for System 002
4.5. Boundaries for the Application of the Results
5. Discussion and Conclusions
5.1. Objective-Related Conclusions
- The accuracy of the DT on the towline tension estimation of the ocean cleanup System 002 in wide span () was improved from having several GPGP data outside of the 15% increase area. Moreover, the DT for the wide span was validated against the GPGP data, with sufficient overestimation (respectively, 6% and 8% mean deviations), at the 2nd and 3rd validation cycles.
- The accuracy of the DT estimation of the towline tension of the cleanup System 002 in the nominal span () was improved from from having most of the GPGP data outside of the 15% increase area. Moreover, the OF model (i.e., the DT) for the nominal span was validated against the GPGP data at the third validation cycle, with a mean deviation of 7%.
- The accuracy of the DT on the towline tension estimation of the ocean cleanup System 002 in the narrow span ( 0.02) was improved. An initial deviation of 276% with respect to the GPGP data was reduced in the 2nd cycle to −10% and in the 3rd cycle to 97%. In spite of the OF model for the narrow span having not been validated yet and that more GPGP data points are required for a more-robust validation, a combined deviation of 43% can be considered for engineering and design purposes, once the average of the 2nd and 3rd cycle curves is taken.
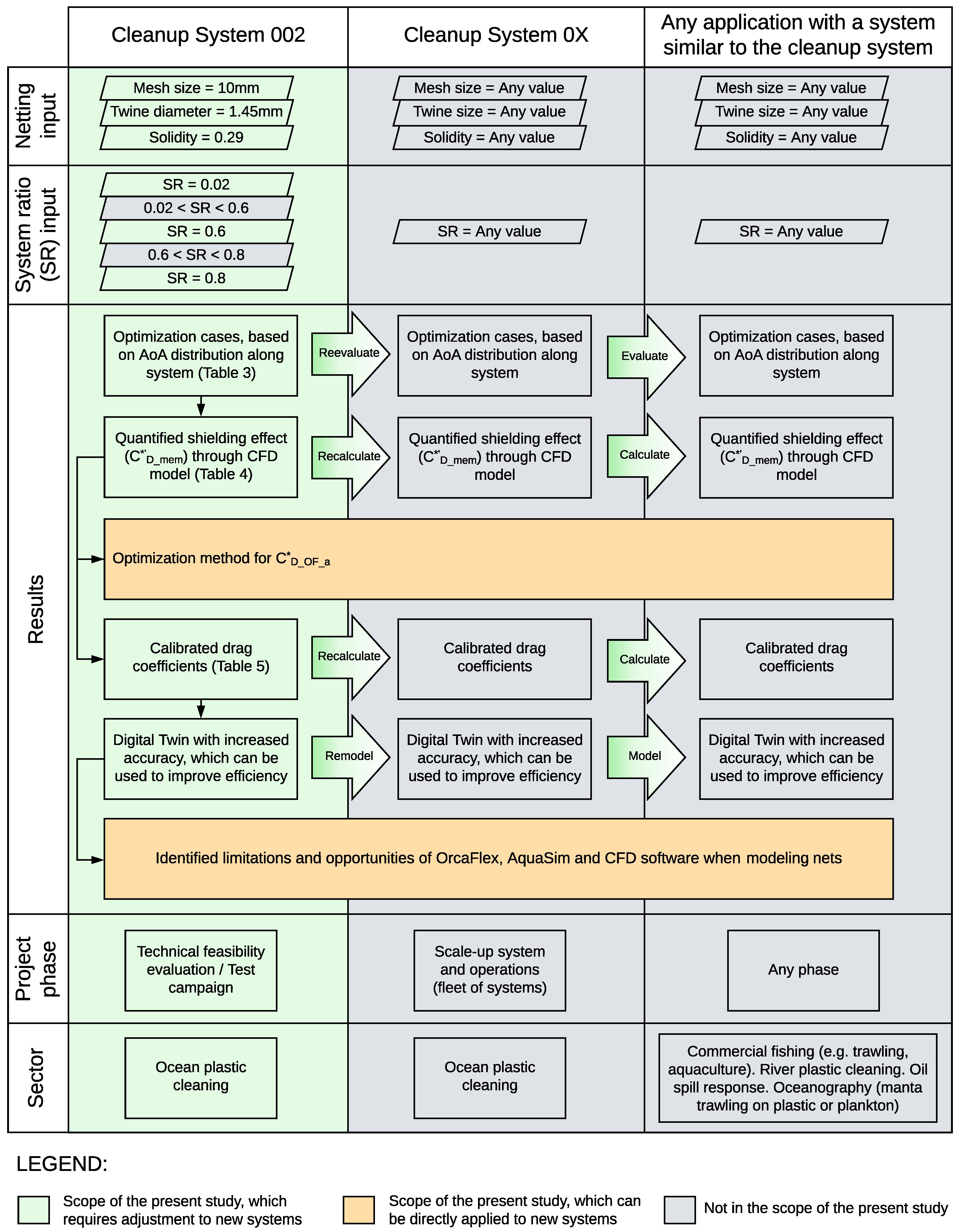
- The results obtained for System 002 can be either directly applied to System 03 and future systems, if the netting and system ratio inputs are the same, or easily reevaluated, recalculated, and remodeled in case the inputs are different (cf. Figure 21). Therefore, considering the same input, System 03 DT can be also considered as validated for wide and nominal spans.
- The previous conclusions have important implications for The Ocean Cleanup development. First, the DT of System 002 and System 03, provided that the latter has the same net and SR characteristics of the former, can be used to improve the efficiency of the operations. As an example, the towing configuration can be dynamically optimized through a trip, to sail with a large span when the plastic area density is high (plastic hot-spots) and in a short span in low-density areas. The under-designing and over-designing of the cleanup system were minimized, further reducing capital expenditure, but also operational expenditure by limiting the case of under-designed systems experiencing failures during operation. The maneuverability of the system can also be improved. As an example, the effect of optimizing the netting sizes can be accurately estimated. That will also improve efficiency since the system will reach and be swept through plastic hot-spots faster. Ultimately, all these improvements will bring the KPIs to values that are below the projected targets.
5.2. Wide-Ranging Conclusions
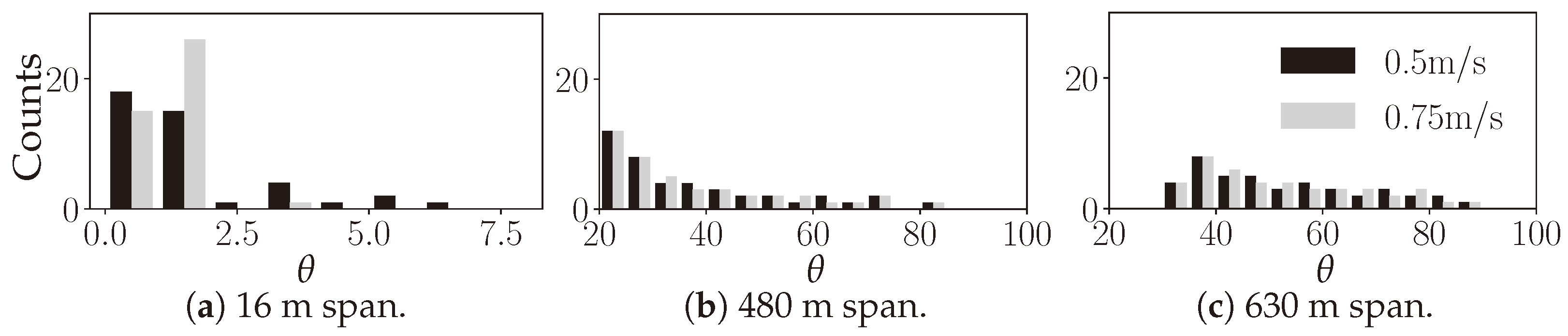
- The quantification of the shielding effect is considerably different if it is performed with the AS calculation or with the CFD model. On top of that, neither the Basilisk model nor AS produce a towline tension curve that accurately fits the GPGP data for a narrow span. Therefore, both calculation methods should be investigated to find the source of the difference.
- Through the validation cycles, it was demonstrated that the semi-empirical formula of Naumov et al. [11] can be used to obtain drag coefficients that produce sufficiently accurate towline tension estimations when the net being towed experiences a flow that is close to perpendicular along most of its length. In terms of , 50% or more of the angles found along the length are distributed between 45° and 90°. The same applies for the AS calculation and the CFD-derived drag coefficients. Furthermore, additional (future) GPGP data for a narrow span need to be included to strengthen the validation.
- Through the validation cycles, it was demonstrated that the AS drag calculation was consistent with the CFD drag calculation until approximately . Below that angle, which is close to parallel flow, AS seemed to underestimate the real drag and the CFD model seemed to overestimate it. Therefore, a more in-depth investigation of AS Equation (8) and of the CFD model to identify missing physical phenomena is required. In that way, the root causes of the respective deviations can be identified.
- As clearly depicted in the schematic of Figure 21, it is possible to adapt and apply the results of the present study to different systems, utilized in sectors ranging from river plastic cleaning to oceanography, through to trawling, aquaculture, oil spill response, and marine ecology. In particular, the optimization method of the OrcaFlex drag coefficient can be directly applied to any of these applications, as well as the lessons on the limitations and opportunities of the software. That should allow running OrcaFlex simulations that produce accurate estimations on motions, loads, maneuverability, operability, survivability, and steering strategies, among others. This can be combined with the modeled data on the catch itself (plastic, fish, plankton, oil, etc.) to obtain accurate estimations on catch rates. Then, considering the invested energy or input, this would lead to efficiency estimations. Ultimately, this allows developing optimization actions to improve the efficiency of the system.Additionally, the present study can also be utilized by other applications or sectors as a guideline to develop the following activities:
- To understand the hydrodynamic drag force experienced by submerged nets and the previous research related to it.
- To validate a model using the materials, knowledge, and method presented in this study.
6. Directions of Further Research
6.1. Multi-Scale Numerical Approach
6.2. How to Hydrodynamically Choose a Net for Our KPIs?
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Tauti, M. The force acting on the plane net in motion through the water. Nippon Suisan Gakkaishi 1934, 3, 1–4. [Google Scholar] [CrossRef]
- Christensen, B. Hydrodynamic modeling of fishing nets. In Proceedings of the OCEAN 75, Okinawa, Japan, 20 July 1975–18 January 1976; pp. 484–490. [Google Scholar] [CrossRef]
- Berteaux, H.O. Buoy Engineering (Ocean Engineering, A Wiley Series); John Wiley and Sons: Hoboken, NJ, USA, 1976. [Google Scholar]
- Laws, E.; Livesey, J. Flow through screens. Annu. Rev. Fluid Mech. 1978, 10, 247–266. [Google Scholar] [CrossRef]
- Rudi, H.; Aarsnes, J.; Dahle, L. Environmental forces on a floating cage system, mooring considerations. In Aquaculture Engineering Technologies for the Future; Hemisphere Publishing Corporation: London, UK, 1988; pp. 97–122. [Google Scholar]
- Rudi, H.; Løland, G.; Furunes, L. Model Tests with Net Enclosures. Forces on and Flow through Single Nets and Cage Systems; MTC: Trondheim, Norway, 1988. [Google Scholar]
- Løland, G. Current Forces on and Flow through Fish Farms. Ph.D. Thesis, University of Trondheim, Trondheim, Norway, 1993. [Google Scholar]
- Takagi, T.; Suzuki, K.; Hiraishi, T. Modeling of net for calculation method of dynamic fishing net shape. Fish. Sci. 2002, 68, 1857–1860. [Google Scholar] [CrossRef] [PubMed][Green Version]
- Balash, C.; Colbourne, B.; Bose, N.; Raman-Nair, W. Aquaculture net drag force and added mass. Aquac. Eng. 2009, 41, 14–21. [Google Scholar] [CrossRef]
- Zhao, Y.P.; Li, Y.C.; Dong, G.H.; Gui, F.K.; Teng, B. Numerical simulation of the effects of structure size ratio and mesh type on three-dimensional deformation of the fishing-net gravity cage in current. Aquac. Eng. 2007, 36, 285–301. [Google Scholar] [CrossRef]
- Naumov, V.; Velikanov, N.; Kikot, A.; Bojarinova, N. The hydrodynamic drag coefficient of flat netting at a cross-section flow. In Proceedings of the Contributions on the Theory of Fishing Gears and Related Marine Systems: Proceeding of the 11th International Workshop on Methods for the Development and Evaluation of Maritime Technologies, Rostock, Germany, 9–12 October 2013; Volume 8, p. 73. [Google Scholar]
- Zhao, Y.; Guan, C.; Bi, C.; Liu, H.; Cui, Y. Experimental investigations on hydrodynamic responses of a semi-submersible offshore fish farm in waves. J. Mar. Sci. Eng. 2019, 7, 238. [Google Scholar] [CrossRef]
- Cheng, H.; Li, L.; Aarsæther, K.G.; Ong, M.C. Typical hydrodynamic models for aquaculture nets: A comparative study under pure current conditions. Aquac. Eng. 2020, 90, 102070. [Google Scholar] [CrossRef]
- Cheng, H.; Ong, M.C.; Li, L.; Chen, H. Development of a coupling algorithm for fluid-structure interaction analysis of submerged aquaculture nets. Ocean. Eng. 2022, 243, 110208. [Google Scholar] [CrossRef]
- Simonsen, K.; Tsukrov, I.; Baldwin, K.; Swift, M.; Patursson, O. Modeling flow through and around a net panel using computational fluid dynamics. In Proceedings of the OCEANS 2006, Boston, MA, USA, 18–21 September 2006; pp. 1–5. [Google Scholar] [CrossRef]
- Zhao, Y.P.; Bi, C.W.; Dong, G.H.; Gui, F.K.; Cui, Y.; Guan, C.T.; Xu, T.J. Numerical simulation of the flow around fishing plane nets using the porous media model. Ocean Eng. 2013, 62, 25–37. [Google Scholar] [CrossRef]
- Gansel, L.C.; Jensen, Ø.; Lien, E.; Endresen, P.C. Forces on Nets with Bending Stiffness: An Experimental Study on the Effects of Flow Speed and Angle of Attack. In Proceedings of the International Conference on Offshore Mechanics and Arctic Engineering, Rio de Janeiro, Brazil, 1–6 June 2012; pp. 69–76. [Google Scholar] [CrossRef]
- Thierry, B.N.N.; Tang, H.; Achile, N.P.; Xu, L.; Zhou, C.; Hu, F. Experimental and Numerical Investigations of the Hydrodynamic Characteristics, Twine Deformation, and Flow Field Around the Netting Structure Composed of Two Types of Twine Materials for Midwater Trawls. J. Ocean Univ. China 2021, 20, 1215–1235. [Google Scholar] [CrossRef]
- Tang, H.; Bruno Thierry, N.N.; Pandong, A.N.; Sun, Q.; Xu, L.; Hu, F.; Zou, B. Hydrodynamic and turbulence flow characteristics of fishing nettings made of three twine materials at small attack angles and low Reynolds numbers. Ocean Eng. 2022, 249, 110964. [Google Scholar] [CrossRef]
- Mi, S.; Wang, M.; Avital, E.J.; Williams, J.J.; Chatjigeorgiou, I.K. An implicit Eulerian–Lagrangian model for flow-net interaction using immersed boundary method in OpenFOAM. Ocean Eng. 2022, 264, 112843. [Google Scholar] [CrossRef]
- Yu, S.; Qin, H.; Li, P.; Gong, F.; Tian, Y. Experimental study on drag characteristics of the practical rigid net under different current conditions. Front. Mar. Sci. 2023, 10. [Google Scholar] [CrossRef]
- Endresen, P.C.; Moe Føre, H. Numerical Modelling of Drag and Lift Forces on Aquaculture Nets: Comparing New Numerical Load Model With Physical Model Test Results. In Proceedings of the International Conference on Offshore Mechanics and Arctic Engineering, Hamburg, Germany, 5–10 June 2022; Volume 4, p. V004T05A002. [Google Scholar] [CrossRef]
- Wang, G.; Cui, Y.; Guan, C.; Gong, P.; Wan, R. Effects of Inclination Angles on the Hydrodynamics of Knotless Net Panels in Currents. J. Mar. Sci. Eng. 2023, 11, 1148. [Google Scholar] [CrossRef]
- Liu, W.; Tang, H.; You, X.; Dong, S.; Xu, L.; Hu, F. Effect of Cutting Ratio and Catch on Drag Characteristics and Fluttering Motions of Midwater Trawl Codend. J. Mar. Sci. Eng. 2021, 9, 256. [Google Scholar] [CrossRef]
- Guo, G.; You, X.; Hu, F.; Yamazaki, R.; Zhuang, X.; Wu, Q.; Lan, G.; Huang, L. Hydrodynamic characteristics of fine-mesh minnow netting for sampling nets. Ocean Eng. 2023, 281, 114738. [Google Scholar] [CrossRef]
- Egger, M.; Nijhof, R.; Quiros, L.; Leone, G.; Royer, S.J.; McWhirter, A.C.; Kantakov, G.; Radchenko, V.; Pakhomov, E.; Hunt, B.; et al. A spatially variable scarcity of floating microplastics in the eastern North Pacific Ocean. Environ. Res. Lett. 2020, 15, 114056. [Google Scholar] [CrossRef]
- Egger, M.; Schilt, B.; Wolter, H.A.; Mani, T.; Vries, R.d.; Zettler, E.R.; Niemann, H.; Egger, M.; Schilt, B.; Wolter, H.A.; et al. Pelagic distribution of plastic debris (>500 µm) and marine organisms in the upper layer of the North Atlantic Ocean. Sci. Rep. 2022, 12, 13465. [Google Scholar] [CrossRef]
- Chong, F.; Spencer, M.; Maximenko, N.; Hafner, J.; McWhirter, A.C.; Helm, R.R. High concentrations of floating neustonic life in the plastic-rich North Pacific Garbage Patch. PLoS Biol. 2023, 21. [Google Scholar] [CrossRef]
- Orcina. OrcaFlex. 1986–2022. Available online: https://www.orcina.com/ (accessed on 17 September 2023).
- Morison, J.; Johnson, J.; Schaaf, S. The Force Exerted by Surface Waves on Piles. J. Pet. Technol. 1950, 2, 149–154. [Google Scholar] [CrossRef]
- Reichert, P. AQUASIM—A tool for simulation and data analysis of aquatic systems. Water Sci. Technol. 1994, 30, 21–30. [Google Scholar] [CrossRef]
- Reichert, P. Design techniques of a computer program for the identification of processes and the simulation of water quality in aquatic systems. Environ. Softw. 1995, 10, 199–210. [Google Scholar] [CrossRef]
- Popinet, S.; Collaborators. Basilisk. 2013–2023. Available online: http://basilisk.fr (accessed on 17 September 2023).
- Popinet, S. Gerris: A tree-based adaptive solver for the incompressible Euler equations in complex geometries. J. Comput. Phys. 2003, 190, 572–600. [Google Scholar] [CrossRef]
- Popinet, S. An accurate adaptive solver for surface-tension-driven interfacial flows. J. Comput. Phys. 2009, 228, 5838–5866. [Google Scholar] [CrossRef]
- Berstad, A.; Walaunet, J.; Heimstad, L. Loads From Currents and Waves on Net Structures. Proc. Int. Conf. Offshore Mech. Arct. Eng. OMAE 2012, 7, 95–104. [Google Scholar] [CrossRef]
- Wachs, A. Particle-scale computational approaches to model dry and saturated granular flows of non-Brownian, non-cohesive, and non-spherical rigid bodies. Acta Mech. 2019, 230, 1919–1980. [Google Scholar] [CrossRef]
- Wu, Y.; Yang, B. An Overview of Numerical Methods for Incompressible Viscous Flow with Moving Particles. Arch. Comput. Methods Eng. 2019, 26, 1255–1282. [Google Scholar] [CrossRef]
- Uhlmann, M.; Derksen, J.; Wachs, A.; Wang, L.P.; Moriche, M. Efficient methods for particle-resolved direct numerical simulation. In Modeling Approaches and Computational Methods for Particle-Laden Turbulent Flows; Subramaniam, S., Balachandar, S., Eds.; Computation and Analysis of Turbulent Flows; Academic Press: Cambridge, MA, USA, 2023; pp. 147–184. [Google Scholar] [CrossRef]
- Selçuk, C.; Ghigo, A.R.; Popinet, S.; Wachs, A. A fictitious domain method with distributed Lagrange multipliers on adaptive quad/octrees for the direct numerical simulation of particle-laden flows. J. Comput. Phys. 2021, 430, 109954. [Google Scholar] [CrossRef]
- Glowinski, R.; Pan, T.; Périaux, J. Distributed Lagrange multiplier methods for incompressible viscous flow around moving rigid bodies. Comput. Methods Appl. Mech. Eng. 1998, 151, 181–194. [Google Scholar] [CrossRef]
- Glowinski, R.; Pan, T.; Hesla, T.; Joseph, D. A distributed Lagrange multiplier/fictitious domain method for particulate flows. Int. J. Multiph. Flow 1999, 25, 755–794. [Google Scholar] [CrossRef]
- Patankar, N.; Singh, P.; Joseph, D.; Glowinski, R.; Pan, T. A new formulation of the distributed Lagrange multiplier/fictitious domain method for particulate flows. Int. J. Multiph. Flow 2000, 26, 1509–1524. [Google Scholar] [CrossRef]
- Glowinski, R.; Pan, T.; Hesla, T.; Joseph, D.; Periaux, J. A fictitious domain approach to the direct numerical simulation of incompressible viscous flow past moving rigid bodies: Application to particulate flow. J. Comput. Phys. 2001, 169, 363–426. [Google Scholar] [CrossRef]
- Wachs, A. PeliGRIFF, a parallel DEM-DLM/FD direct numerical simulation tool for 3D particulate flows. J. Eng. Math. 2011, 71, 131–155. [Google Scholar] [CrossRef]
- Wachs, A.; Hammouti, A.; Vinay, G.; Rahmani, M. Accuracy of Finite Volume/Staggered Grid Distributed Lagrange Multiplier/Fictitious Domain simulations of particulate flows. Comput. Fluids 2015, 115, 154–172. [Google Scholar] [CrossRef]
- Bouard, R.; Coutanceau, M. The early stage of development of the wake behind an impulsively started cylinder for 40 < Re < 104. J. Fluid Mech. 1980, 101, 583–607. [Google Scholar] [CrossRef]
- Koumoutsakos, P.; Leonard, A. High-resolution simulations of the flow around an impulsively started cylinder using vortex methods. J. Fluid Mech. 1995, 296, 1–38. [Google Scholar] [CrossRef]
- Mohaghegh, F.; Udaykumar, H. Comparison of sharp and smoothed interface methods for simulation of particulate flows II: Inertial and added mass effects. Comput. Fluids 2017, 143, 103–119. [Google Scholar] [CrossRef]
- Anderson, T.B.; Jackson, R. Fluid Mechanical Description of Fluidized Beds. Equations of Motion Ind. Eng. Chem. Fundamen. 1967, 6, 527–539. [Google Scholar] [CrossRef]
- Kawaguchi, T.; Tanaka, T.; Tsuji, Y. Numerical simulation of two-dimensional fluidized beds using the discrete element method (comparison between the two- and three-dimensional models). Powder Technol. 1998, 96, 129–138. [Google Scholar] [CrossRef]
- Tsuji, T.; Yabumoto, K.; Tanaka, T. Spontaneous structures in three-dimensional bubbling gas-fluidized bed by parallel DEM–CFD coupling simulation. Powder Technol. 2008, 184, 132–140. [Google Scholar] [CrossRef]
- Esteghamatian, A.; Euzenat, F.; Hammouti, A.; Lance, M.; Wachs, A. A stochastic formulation for the drag force based on multiscale numerical simulation of fluidized beds. Int. J. Multiph. Flow 2018, 99, 363–382. [Google Scholar] [CrossRef]
- Cundall, P.A.; Strack, O.D. Discrete numerical model for granular assemblies. Geotechnique 1979, 29, 47–65. [Google Scholar] [CrossRef]
- Cundall, P. Formulation of a three-dimensional distinct element model - Part I. A scheme to detect and represent contacts in a system composed of many polyhedral blocks. Int. J. Rock Mech. Min. Sci. Geomech. Abstr. 1988, 25, 107–116. [Google Scholar] [CrossRef]
- Kong, Y.; Zhao, J.; Li, X. Assessing the performance of flexible barrier subjected to impacts of typical geophysical flows: A unified computational approach based on coupled CFD/DEM. In Proceedings of the EGU General Assembly Conference Abstracts, Online, 4–8 May 2020. [Google Scholar] [CrossRef]
- Kong, Y.; Guan, M.; Li, X.; Zhao, J.; Yan, H. How Flexible, Slit and Rigid Barriers Mitigate Two-Phase Geophysical Mass Flows: A Numerical Appraisal. J. Geophys. Res. Earth Surf. 2021, 127, e2021JF006587. [Google Scholar] [CrossRef]
- Li, X.; Zhao, J.; Kwan, J.S. Assessing debris flow impact on flexible ring net barrier: A coupled CFD-DEM study. Comput. Geotech. 2020, 128, 103850. [Google Scholar] [CrossRef]
- Tang, H.; Xu, L.; Hu, F. Hydrodynamic characteristics of knotted and knotless purse seine netting panels as determined in a flume tank. PLoS ONE 2018, 13, e0192206. [Google Scholar] [CrossRef]
- Gansel, L.C.; Plew, D.R.; Endresen, P.C.; Olsen, A.I.; Misimi, E.; Guenther, J.; Jensen, Ø. Drag of Clean and Fouled Net Panels - Measurements and Parameterization of Fouling. PLoS ONE 2015, 10, e0131051. [Google Scholar] [CrossRef]
- Tsukrov, I.; Drach, A.; DeCew, J.; Robinson Swift, M.; Celikkol, B. Characterization of geometry and normal drag coefficients of copper nets. Ocean Eng. 2011, 38, 1979–1988. [Google Scholar] [CrossRef]
 Port-side vessel.
Port-side vessel.  Starboard-side vessel.
Starboard-side vessel.  Port-side tow bar and towing bridle, connected to a towing line.
Port-side tow bar and towing bridle, connected to a towing line.  Starboard-side tow bar and towing bridle, connected to a towing line.
Starboard-side tow bar and towing bridle, connected to a towing line.  Port-side wingspan.
Port-side wingspan.  Starboard-side wingspan.
Starboard-side wingspan.  Retention opening.
Retention opening.  Retention zone.
Retention zone.  Extraction pick-up line. (a) Aerial view of System 002 towed by two vessels. (b) Underwater view of the retention zone made of nets.
Extraction pick-up line. (a) Aerial view of System 002 towed by two vessels. (b) Underwater view of the retention zone made of nets.
 Port-side vessel.
Port-side vessel.  Starboard-side vessel.
Starboard-side vessel.  Port-side tow bar and towing bridle, connected to a towing line.
Port-side tow bar and towing bridle, connected to a towing line.  Starboard-side tow bar and towing bridle, connected to a towing line.
Starboard-side tow bar and towing bridle, connected to a towing line.  Port-side wingspan.
Port-side wingspan.  Starboard-side wingspan.
Starboard-side wingspan.  Retention opening.
Retention opening.  Retention zone.
Retention zone.  Extraction pick-up line. (a) Aerial view of System 002 towed by two vessels. (b) Underwater view of the retention zone made of nets.
Extraction pick-up line. (a) Aerial view of System 002 towed by two vessels. (b) Underwater view of the retention zone made of nets.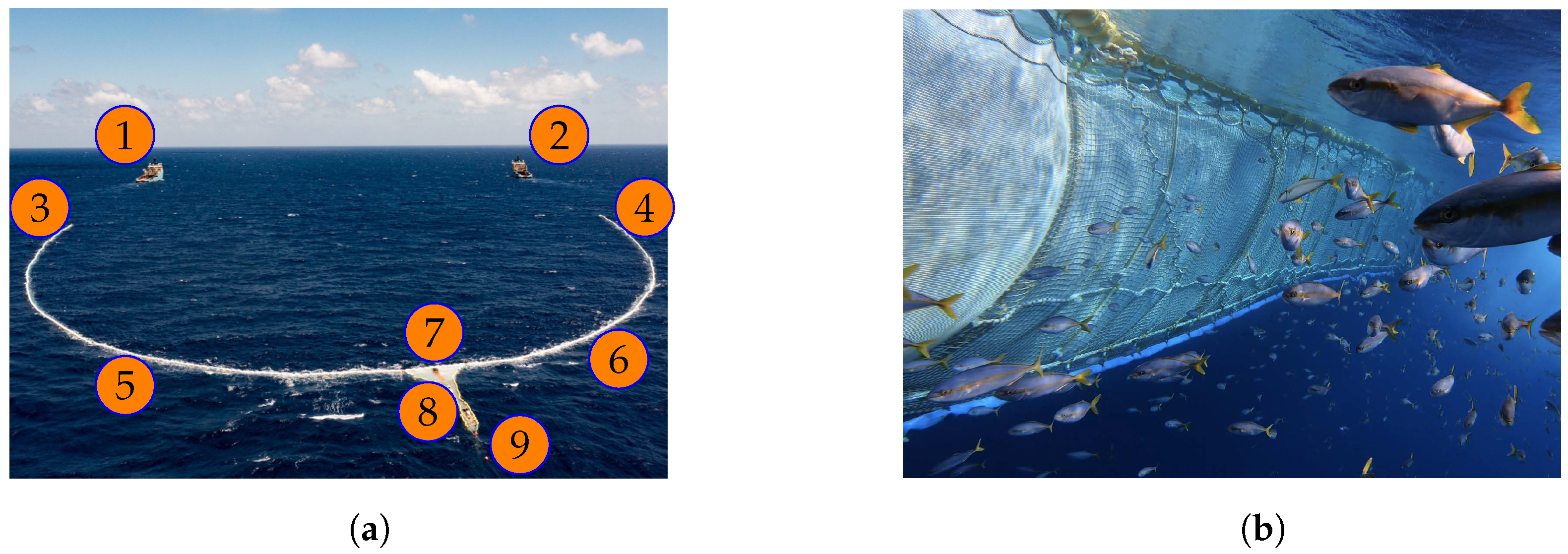





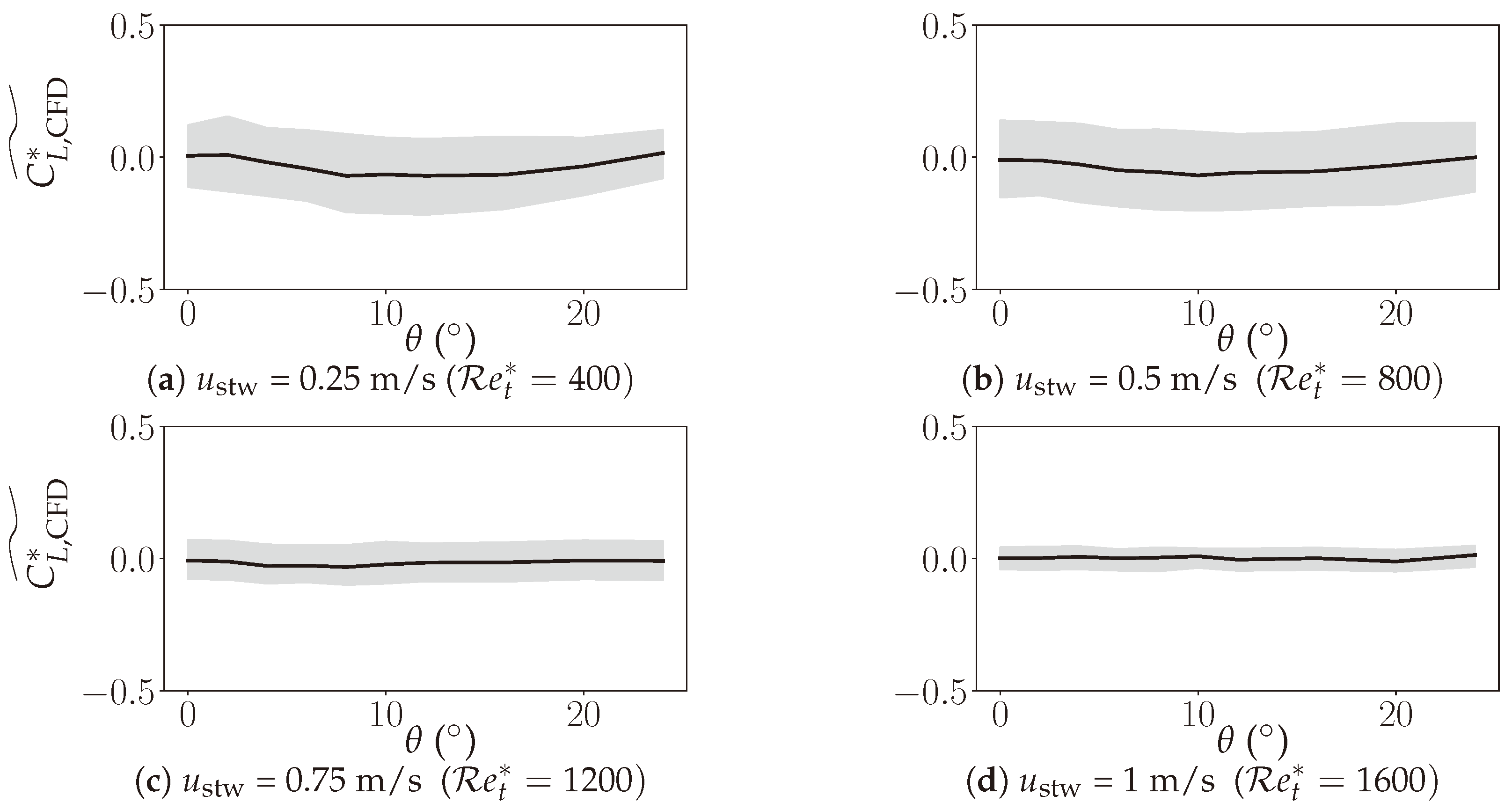
| Span Type | Span (m) | System Ratio | System Status |
|---|---|---|---|
| Narrow | ≈16 | 0.02 | Towing behind one vessel |
| Nominal | ≈480 | 0.6 | Optimal performance in operation |
| Wide | ≈630 | 0.8 | Maximize plastic catch |
| Sea State | (m/s) | ||||||
|---|---|---|---|---|---|---|---|
| a.n.e.p. (†) | (m) | (s) | 0.1 | 0.25 | 0.5 | 0.75 | 1.0 |
| p25 | 1.7 | 10.8 | 3.83 | 1.74 | 1.19 | 1.09 | 1.11 |
| p50 | 2.3 | 11.9 | 4.72 | 2.02 | 1.30 | 1.15 | 1.13 |
| p75 | 3.0 | 11.9 | 6.77 | 2.62 | 1.54 | 1.28 | 1.19 |
| p90 | 4.1 | 13.1 | 9.56 | 3.37 | 1.81 | 1.41 | 1.26 |
| Optimization Case | Span Type | Angles |
|---|---|---|
| 1 | Narrow | |
| 2 | Nominal | |
| 3 | Wide |
| () | 2nd Cycle | 3rd Cycle |
|---|---|---|
| 90 | 1.5999 | 1.5967 |
| 45 | 1.5999 | 1.5589 |
| 30 | 1.5999 | 1.3822 |
| 20 | 1.5589 | 1.2649 |
| 10 | 0.5639 | 0.8492 |
| 5 | 0.2005 | 0.6076 |
| 3 | 0.0933 | 0.5253 |
| 2 | 0.0508 | 0.4849 |
| 1 | 0.0180 | 0.4732 |
| 0 | 0.0 | 0.4614 |
| Span Type | 1st Cycle | 2nd Cycle | 3rd Cycle | 3rd Cycle—Averaged |
|---|---|---|---|---|
| Narrow | 0.208 | 0.010 | 0.082 | 0.046 |
| Nominal | 0.208 | 0.332 | 0.285 | 0.285 |
| Wide | 0.208 | 0.301 | 0.324 | 0.324 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Gonzalez Jimenez, M.A.; Rakotonirina, A.D.; Sainte-Rose, B.; Cox, D.J. On the Digital Twin of The Ocean Cleanup Systems—Part I: Calibration of the Drag Coefficients of a Netted Screen in OrcaFlex Using CFD and Full-Scale Experiments. J. Mar. Sci. Eng. 2023, 11, 1943. https://doi.org/10.3390/jmse11101943
Gonzalez Jimenez MA, Rakotonirina AD, Sainte-Rose B, Cox DJ. On the Digital Twin of The Ocean Cleanup Systems—Part I: Calibration of the Drag Coefficients of a Netted Screen in OrcaFlex Using CFD and Full-Scale Experiments. Journal of Marine Science and Engineering. 2023; 11(10):1943. https://doi.org/10.3390/jmse11101943
Chicago/Turabian StyleGonzalez Jimenez, Martin Alejandro, Andriarimina Daniel Rakotonirina, Bruno Sainte-Rose, and David James Cox. 2023. "On the Digital Twin of The Ocean Cleanup Systems—Part I: Calibration of the Drag Coefficients of a Netted Screen in OrcaFlex Using CFD and Full-Scale Experiments" Journal of Marine Science and Engineering 11, no. 10: 1943. https://doi.org/10.3390/jmse11101943
APA StyleGonzalez Jimenez, M. A., Rakotonirina, A. D., Sainte-Rose, B., & Cox, D. J. (2023). On the Digital Twin of The Ocean Cleanup Systems—Part I: Calibration of the Drag Coefficients of a Netted Screen in OrcaFlex Using CFD and Full-Scale Experiments. Journal of Marine Science and Engineering, 11(10), 1943. https://doi.org/10.3390/jmse11101943







