Formation Control of Unmanned Surface Vehicles Using Fixed-Time Non-Singular Terminal Sliding Mode Strategy
Abstract
:1. Introduction
2. Preliminaries and Problem Formulation
2.1. Preliminaries
2.2. Problem Formulation
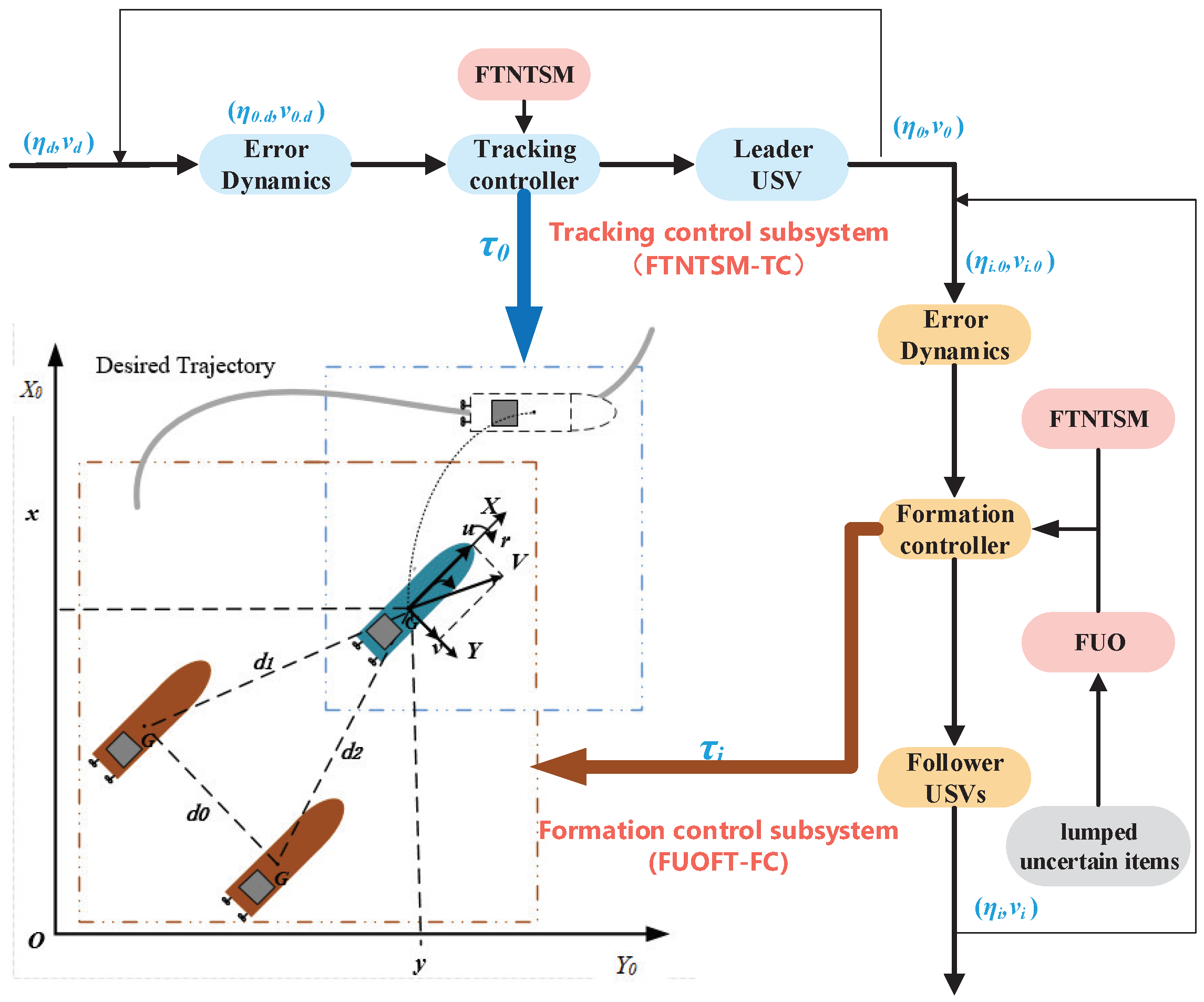
3. Design of Formation Control Strategy
3.1. Design of Tracking Control Subsystem and Stability Analysis
3.2. Design of Formation Control Subsystem and Stability Analysis
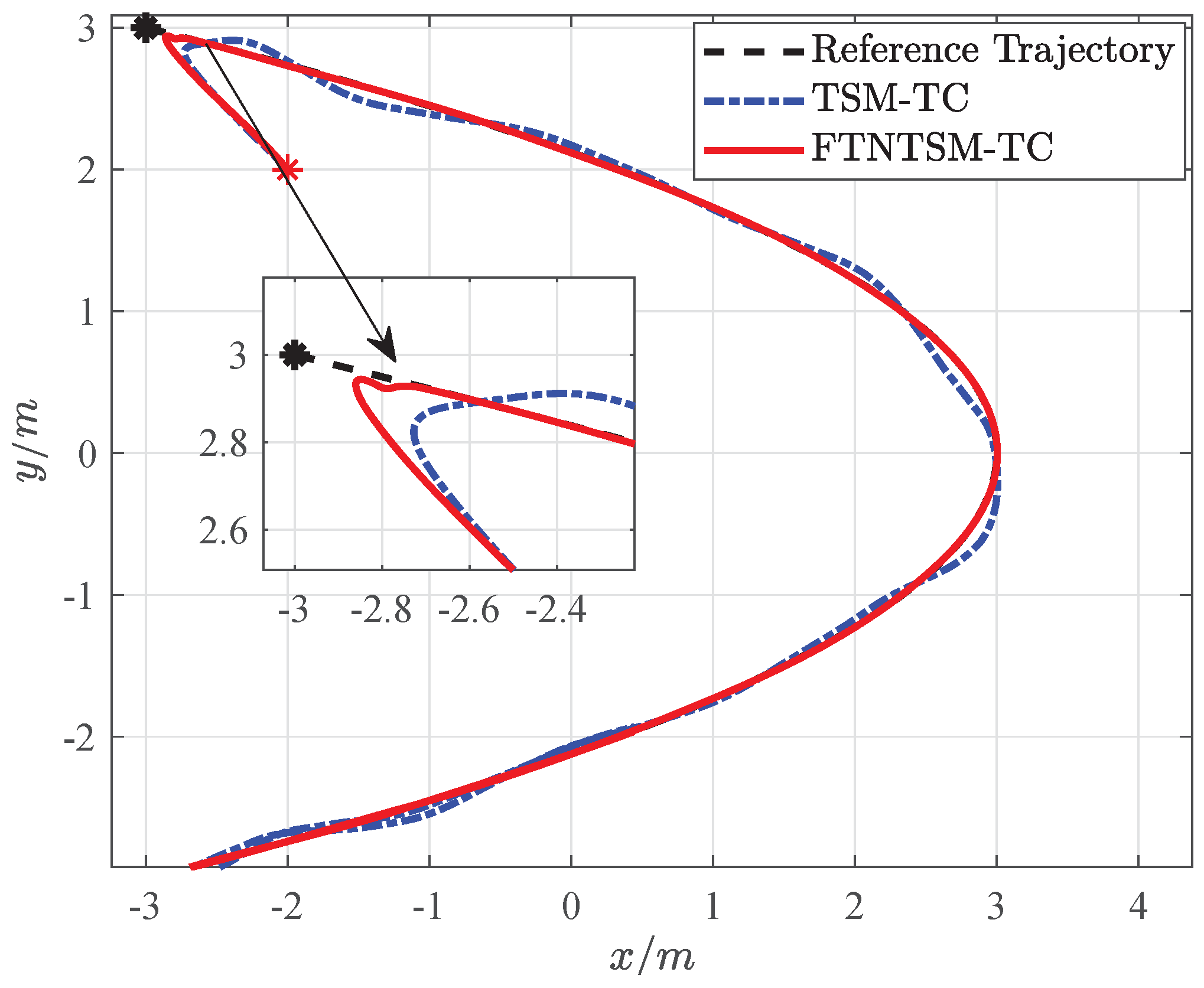
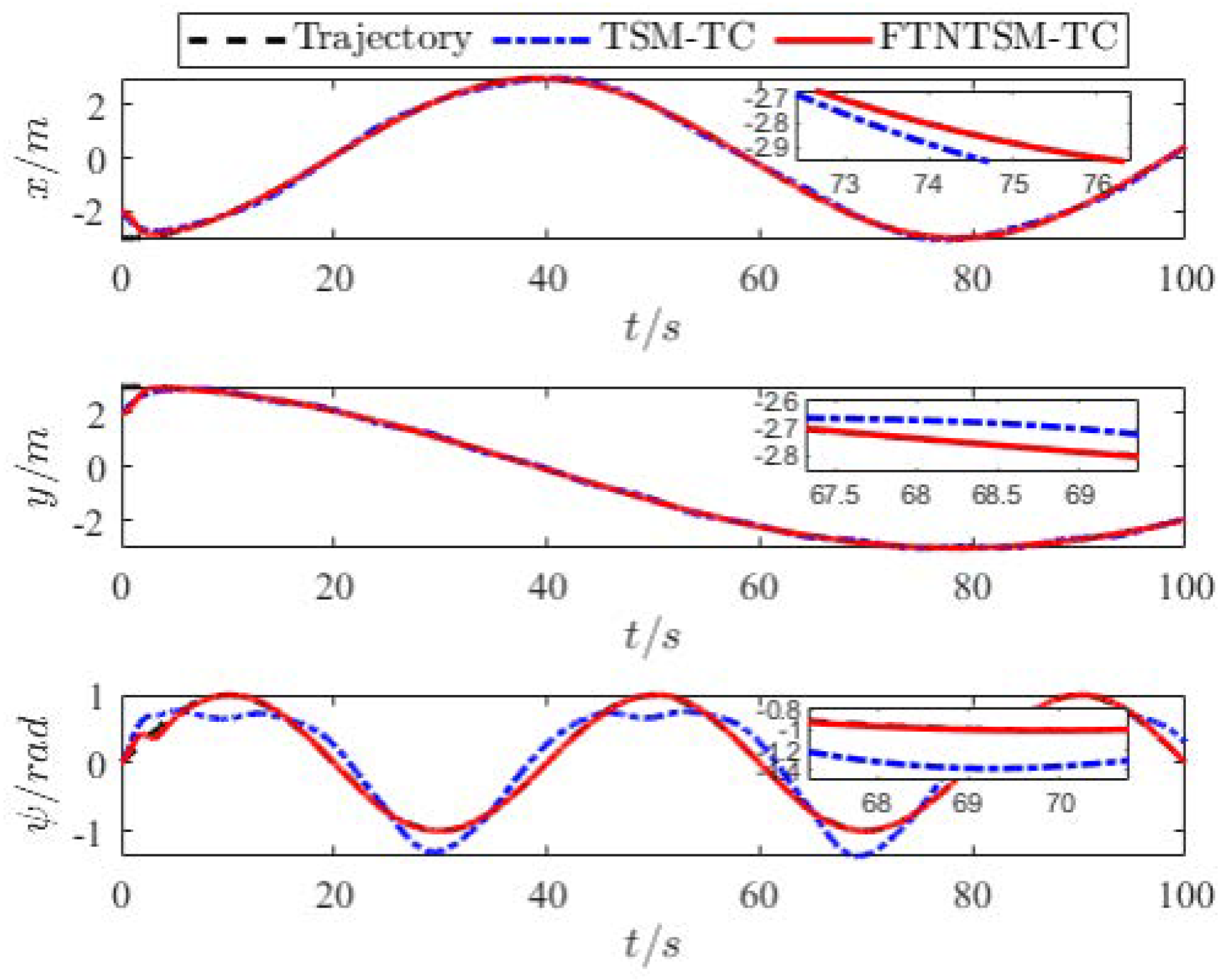
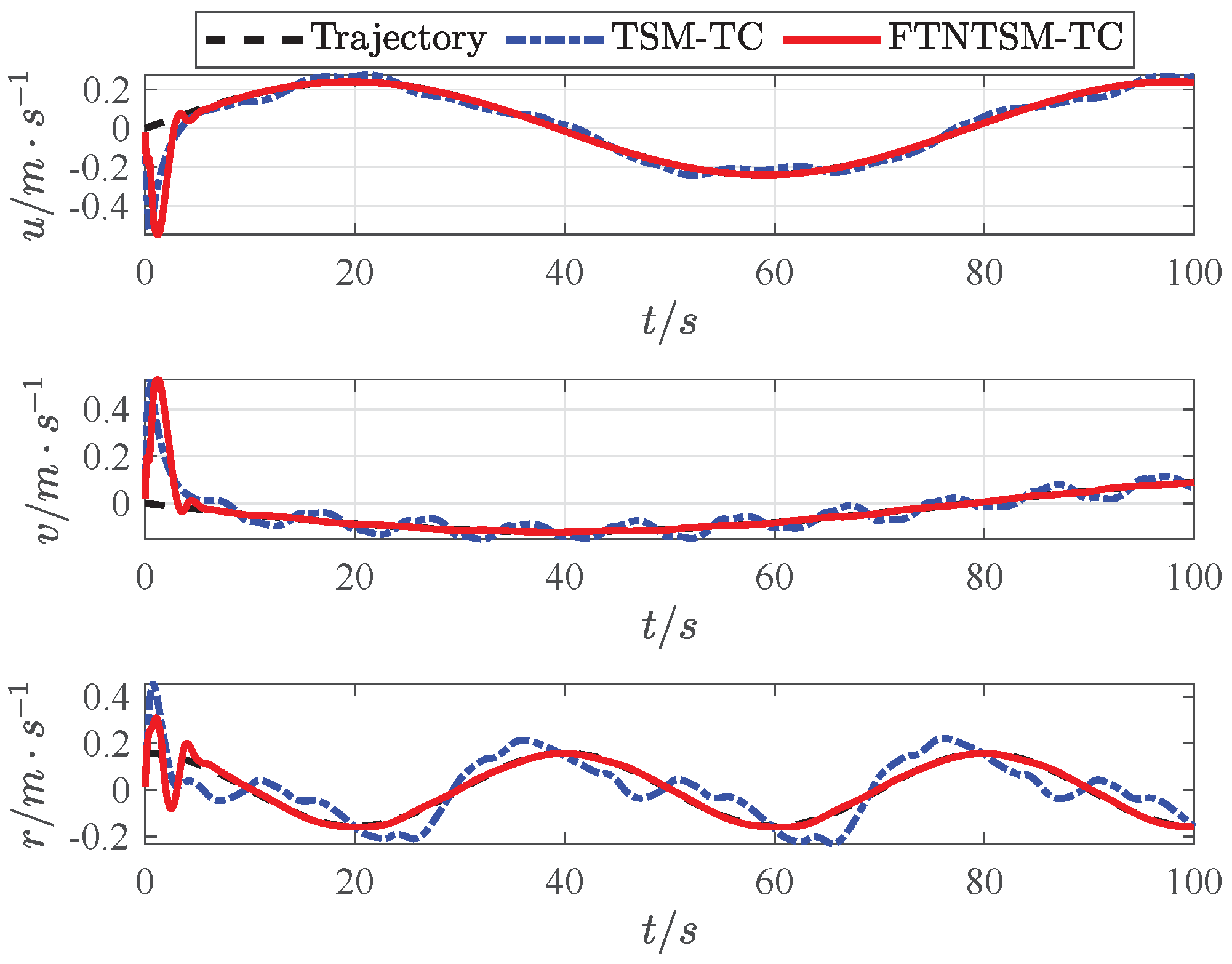
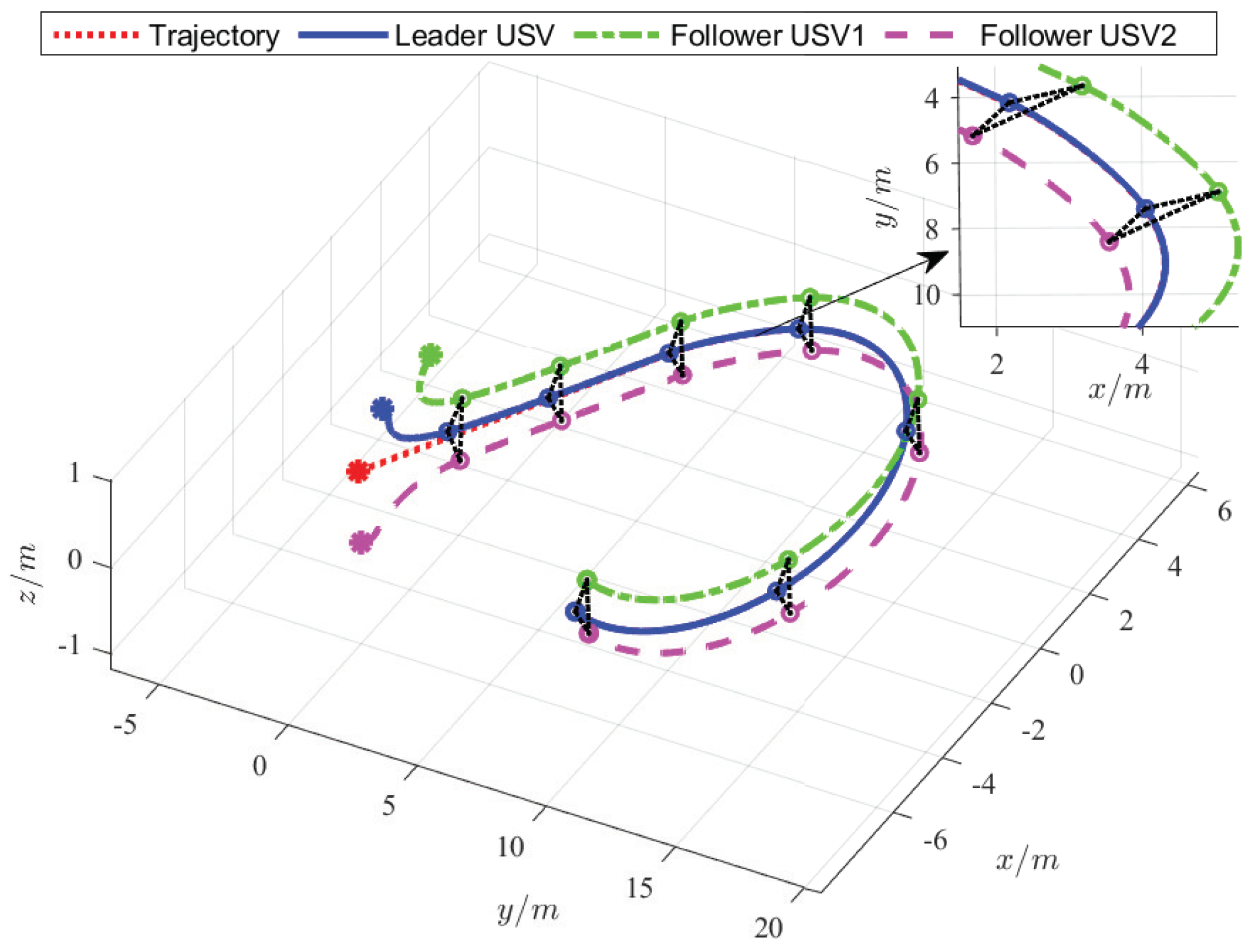
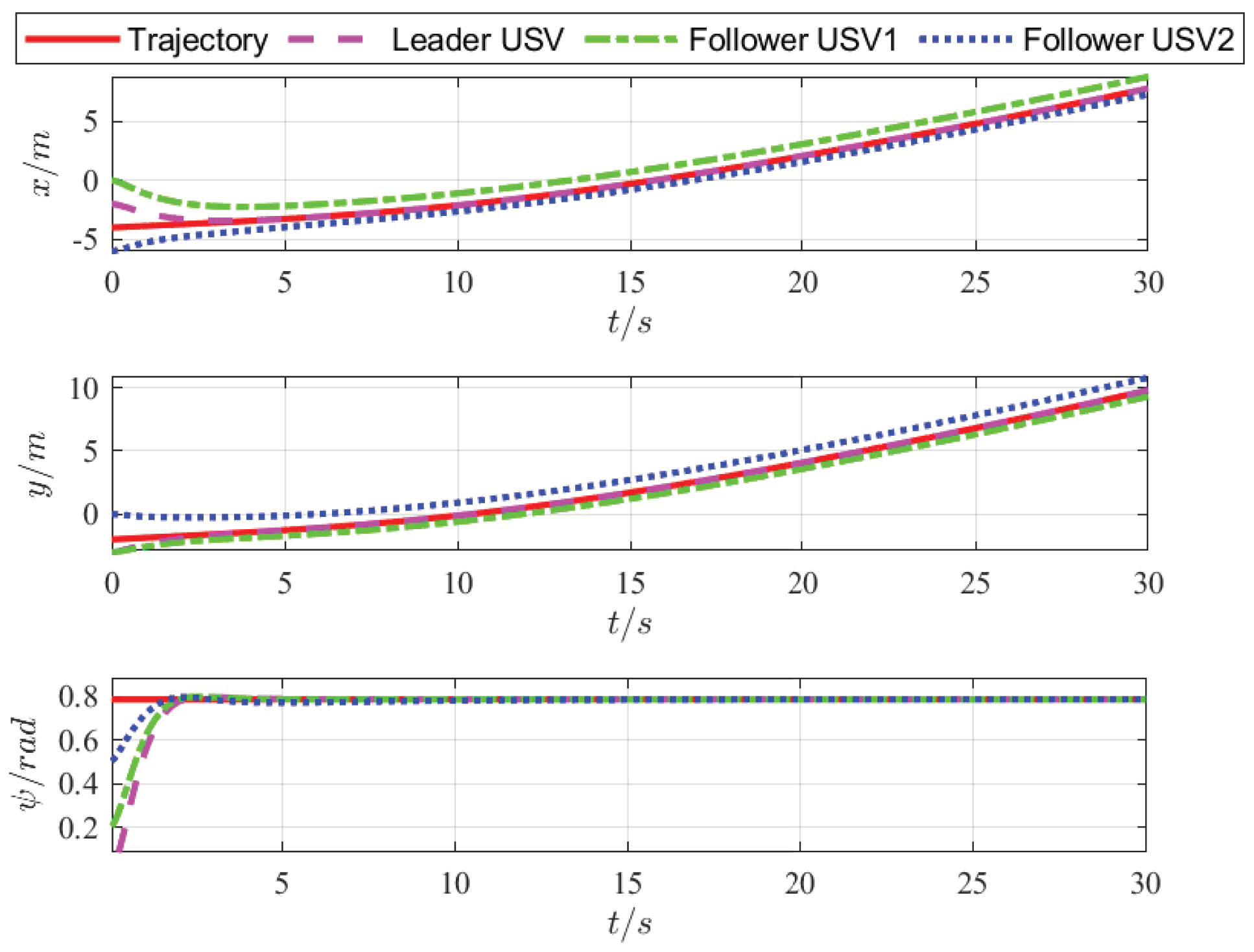
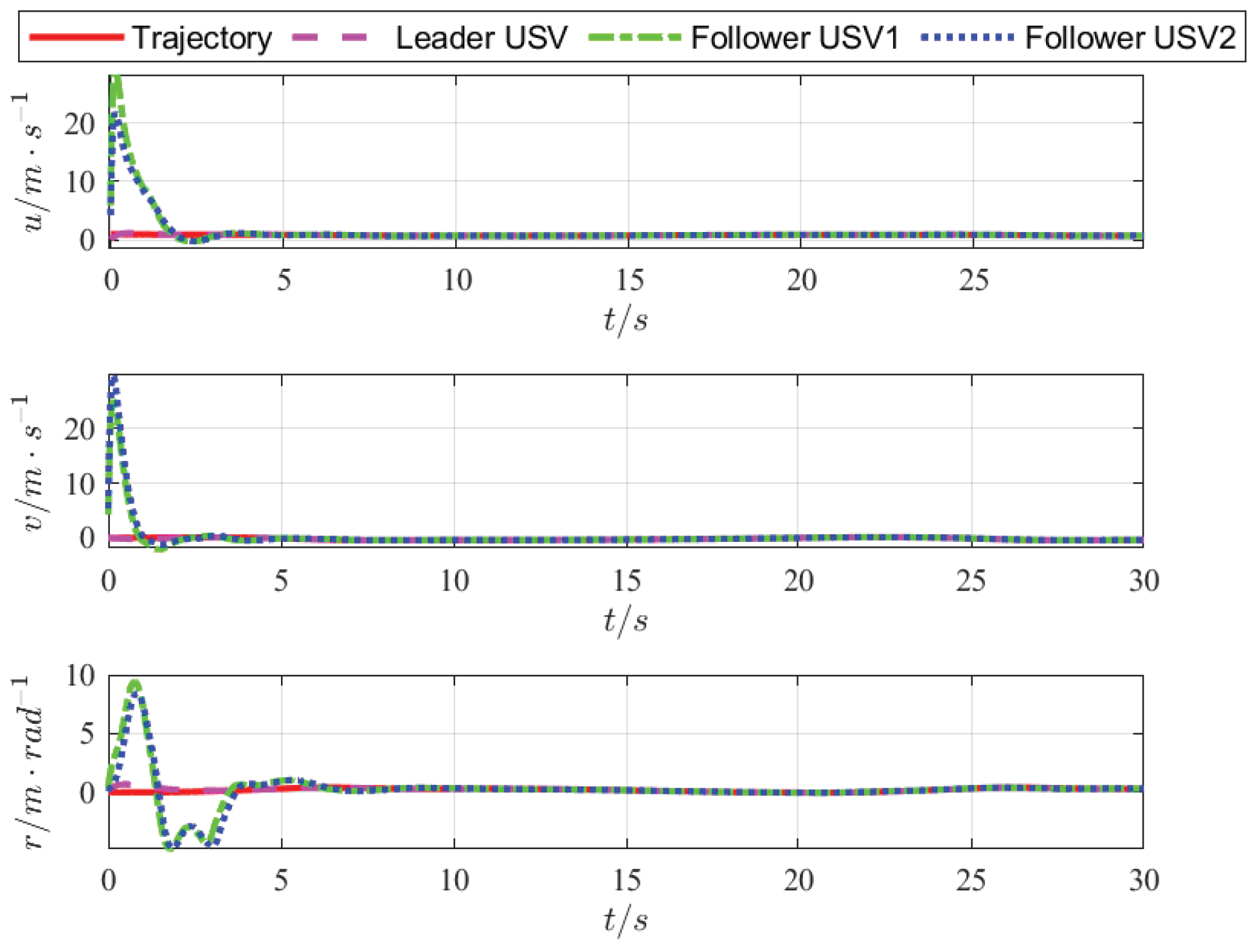
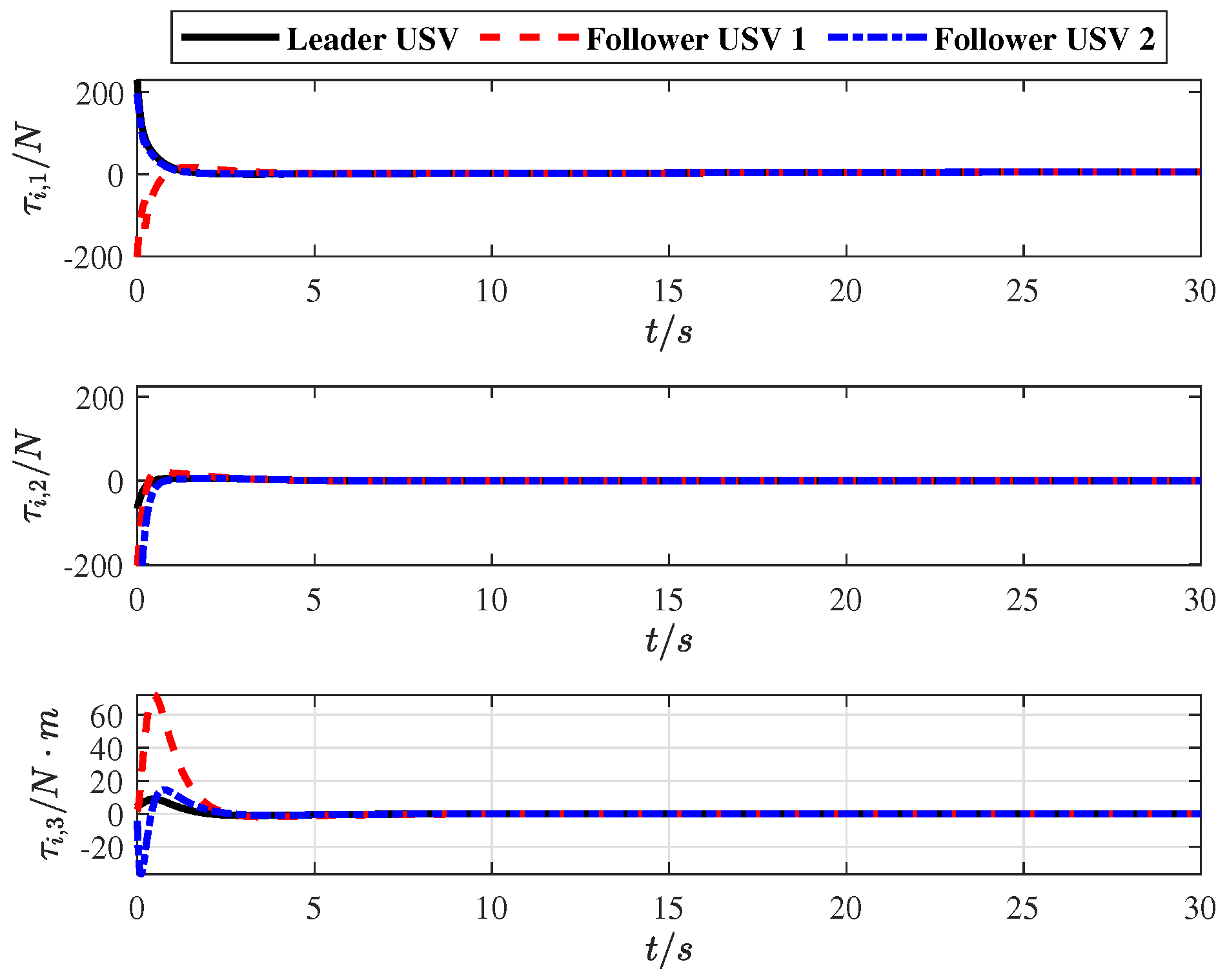
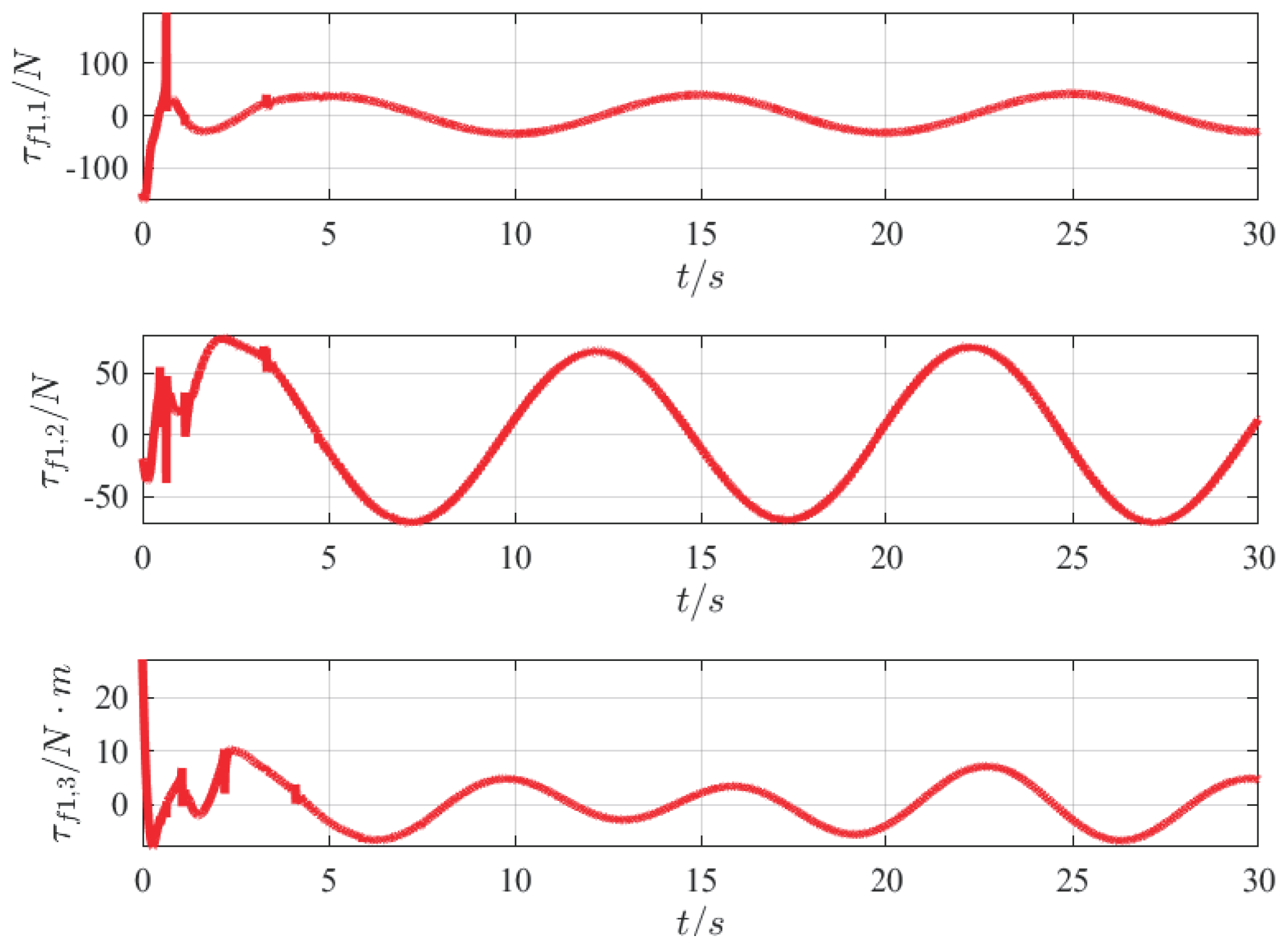
4. Simulation and Discussion
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Chen, D.; Zhang, J.D.; Li, Z.K. A novel fixed-time trajectory tracking strategy of unmanned surface vessel based on the fractional sliding mode control method. Electronics 2022, 11, 726. [Google Scholar] [CrossRef]
- Jin, X.Z.; Er, M.J. Dynamic collision avoidance scheme for unmanned surface vehicles under complex shallow sea Environments. Ocean Eng. 2020, 218, 108102. [Google Scholar] [CrossRef]
- Li, J.J.; Chen, X. Event-triggered control for bipartite consensus and formation of multiple wheeled mobile robots with non-holonomic constraint. Asian J. Control 2022, 24, 1795–1807. [Google Scholar] [CrossRef]
- Ghommam, J.; Saad, M. Adaptive leader-follower formation control of underactuated surface vessels under asymmetric range and bearing constraints. IEEE Trans. Veh. Technol. 2018, 67, 852–865. [Google Scholar] [CrossRef]
- Zhang, P.; de Queiroz, M. 3D multi-agent formation control with rigid body maneuvers. Asian J. Control 2019, 21, 1088–1099. [Google Scholar] [CrossRef]
- Song, R.; Liu, Y.; Bucknall, R. A multi-layered fast marching method for unmanned surface vehicle path planning in a time-variant maritime environment. Ocean Eng. 2017, 129, 301–317. [Google Scholar] [CrossRef]
- Balch, T.; Arkin, R.C. Behavior-based formation control for multi-robot teams. IEEE Trans. Robot. Autom. 1998, 14, 926–939. [Google Scholar] [CrossRef]
- Zhang, C. Synchronization and tracking of multi-spacecraft formation attitude control using adaptive sliding mode. Asian J. Control 2019, 21, 832–846. [Google Scholar] [CrossRef]
- Qin, D. Formation control of mobile robot systems incorporating primal-dual neural network and distributed predictive approach. J. Frankl. Inst. 2020, 357, 12454–12472. [Google Scholar] [CrossRef]
- Fu, M. A cross-coupling control approach for coordinated formation of surface vessels with uncertain disturbances. Asian J. Control 2018, 20, 2370–2379. [Google Scholar] [CrossRef]
- Glotzbach, T.; Schneider, M.; Otto, P. Cooperative line of sight target tracking for heterogeneous unmanned marine vehicle teams: From theory to practice. Robot. Auton. Syst. 2015, 67, 53–60. [Google Scholar] [CrossRef]
- Zong, Q.; Shao, S.K. Decentralized finite-time attitude syn-chronization on for multiple rigid spacecraft via a novel disturbance observer. ISA Trans. 2016, 65, 150–163. [Google Scholar] [PubMed]
- Wang, J.; Luo, X.; Wang, L.; Zuo, Z.; Guan, X. Integral sliding mode control using a disturbance observer for vehicle platoons. IEEE Trans. Ind. Electron. 2020, 67, 6639–6648. [Google Scholar] [CrossRef]
- Xing, M.; Deng, F. Tracking control for stochastic multi-agent systems based on hybrid event-triggered mechanism. Asian J. Control 2019, 21, 2352–2363. [Google Scholar] [CrossRef]
- Chu, Z.; Meng, F.; Zhu, D.; Luo, C. Fault reconstruction using a terminal sliding mode observer for a class of second-order MIMO uncertain nonlinear systems. ISA Trans. 2020, 97, 67–75. [Google Scholar] [CrossRef]
- Xu, G.-H.; Li, M.; Chen, J.; Lai, Q.; Zhao, Z.-W. Formation tracking control for multi-agent networks with fixed time convergence via terminal sliding mode control approach. Sensors 2021, 21, 1416. [Google Scholar] [CrossRef]
- Asl, S.B.F.; Moosapour, S.S. Adaptive backstepping fast terminal sliding mode controller design for ducted fan engine of thrust-vectored aircraft. Aerosp. Sci. Technol. 2017, 71, 521–529. [Google Scholar]
- Pai, M.C. Observer-based adaptive sliding mode control for nonlinear uncertain state-delayed systems. Int. J. Control Autom. Syst. 2009, 7, 536–544. [Google Scholar] [CrossRef]
- Grochmal, T.R.; Lynch, A.F. Precision tracking of a rotating shaft with magnetic bearings by nonlinear decoupled disturbance observers. IEEE Trans. Control Syst. Technol. 2007, 15, 1112–1121. [Google Scholar] [CrossRef]
- Wang, N.; He, H.K. Dynamics-level finite-Time fuzzy monocular visual servo of an unmanned surface vehicle. IEEE Trans. Ind. Electron. 2020, 67, 9648–9658. [Google Scholar] [CrossRef]
- Wang, N.; Ahn, C.K. Hyperbolic-tangent LOS guidance-based finite-time path following of underactuated marine vehicles. IEEE Trans. Ind. Electron. 2020, 99, 8566–8575. [Google Scholar] [CrossRef]
- Wang, N.; Deng, Z.C. Finite-time fault estimator based fault-tolerance control for a surface vehicle with input saturations. IEEE Trans. Ind. Inform. 2020, 16, 1172–1181. [Google Scholar] [CrossRef]
- Wang, N.; Karimi, H.R. Successive waypoints tracking of an underactuated surface vehicle. IEEE Trans. Ind. Inform. 2020, 16, 898–908. [Google Scholar] [CrossRef]
- Wang, N.; Pan, X.X. Path following of autonomous underactuated ships: A translation–rotation cascade control approach. IEEE/ASME Trans. Mechatron. 2019, 24, 2583–2593. [Google Scholar] [CrossRef]
- Wang, N.; Su, S.F. Finite-time unknown observer-based interactive trajectory tracking control of asymmetric underactuated surface vehicles. IEEE Trans. Control Syst. Technol. 2021, 29, 794–803. [Google Scholar] [CrossRef]
- Liu, W.; Zhang, K.; Jiang, B.; Yan, X. Adaptive fault-tolerant formation control for quadrotors with actuator faults. Asian J. Control 2020, 22, 1317–1326. [Google Scholar] [CrossRef]
- Wang, N.; Lv, S.; Er, M.J. Fast and accurate trajectory tracking control of an autonomous surface vehicle with unmodeled dynamics and disturbances. IEEE Trans. Intell. Veh. 2017, 1, 230–243. [Google Scholar] [CrossRef]
- Zhang, J.X.; Yang, G.H. Fault-tolerant fixed-time trajectory tracking control of autonomous surface vessels with specified accuracy. IEEE Trans. Ind. Electron. 2020, 67, 4889–4899. [Google Scholar] [CrossRef]
- Wang, N.; Qian, C.; Sun, J.C. Adaptive robust finite-time trajectory tracking control of fully actuated marine surface vehicles. IEEE Trans. Control Syst. Technol. 2015, 24, 1454–1462. [Google Scholar] [CrossRef]
- Wang, N.; Guo, S.; Yin, J. Composite trajectory tracking control of unmanned surface vehicles with disturbances and uncertainties. In Proceedings of the 2018 IEEE International Conference on Real-time Computing and Robotics (RCAR), Kandima, Maldives, 1–5 August 2018; pp. 491–496. [Google Scholar]
- Polyakov, A. Nonlinear feedback design for fixed-time stabilization of linear control systems. IEEE Trans. Automat. Contr. 2011, 57, 2106–2110. [Google Scholar] [CrossRef]
- Zuo, Z.; Tie, L. Distributed robust finite-time nonlinear consensus protocols for multi-agent systems. Int. J. Syst. Sci. 2016, 47, 1366–1375. [Google Scholar] [CrossRef]
- Zuo, Z. Nonsingular fixed-time consensus tracking for second-order multi-agent networks. Automatica 2015, 54, 305–309. [Google Scholar] [CrossRef]
- Zuo, Z.; Tian, B.; Defoort, M. Fixed-time consensus tracking for multiagent systems with high-order integrator dynamics. IEEE Trans. Autom. Contr. 2017, 63, 563–570. [Google Scholar] [CrossRef]
- Wang, N.; Li, H. Leader-follower formation control of surface vehicles: A fixed-time control approach. ISA Trans. 2020, 124, 356–364. [Google Scholar] [CrossRef]
- Fu, J.; Wang, J. Fixed-time coordinated tracking for second-order multi-agent systems with bounded input uncertainties. Syst. Control Lett. 2016, 93, 1–12. [Google Scholar] [CrossRef]
- Geng, Z.Y.; Liu, Y.F. Finite-time formation control for linear multi-agent systems: A motion planning approach. Syst. Control Lett. 2015, 85, 54–60. [Google Scholar]
- Yuri, B.; Shtessel, I.A.; Shkolnikov, A.L. Smooth second-order sliding modes: Missile guidance application. Automatica 2007, 43, 1470–1476. [Google Scholar]
- Skjetne, R.; Smogeli, Ø.; Fossen, T.I. Modeling, identification, and adaptive maneuvering of Cybership II: A complete design with experiments. IFAC Proc. Vol. 2004, 37, 203–208. [Google Scholar] [CrossRef]




| Parameter | Value | Parameter | Value |
|---|---|---|---|
| Parameters | Values | Parameters | Values | Parameters | Values |
|---|---|---|---|---|---|
| m | 23.8000 | −0.8612 | −2.0 | ||
| 1.7600 | −36.2823 | −10.0 | |||
| 0.460 | 0.1079 | 0.0 | |||
| −0.7225 | 0.1052 | 0.0 | |||
| −1.3274 | 5.0437 | −1.0 | |||
| −5.8664 |
| Parameters | Values | Parameters | Values |
|---|---|---|---|
| 7 | 9 | ||
| 3 | 9 | ||
| 2 | 5 | ||
| 0.03 | 5 | ||
| 3 | 7 | ||
| 3 |
| Parameters | Values | Parameters | Values |
|---|---|---|---|
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Er, M.J.; Li, Z. Formation Control of Unmanned Surface Vehicles Using Fixed-Time Non-Singular Terminal Sliding Mode Strategy. J. Mar. Sci. Eng. 2022, 10, 1308. https://doi.org/10.3390/jmse10091308
Er MJ, Li Z. Formation Control of Unmanned Surface Vehicles Using Fixed-Time Non-Singular Terminal Sliding Mode Strategy. Journal of Marine Science and Engineering. 2022; 10(9):1308. https://doi.org/10.3390/jmse10091308
Chicago/Turabian StyleEr, Meng Joo, and Zhongkun Li. 2022. "Formation Control of Unmanned Surface Vehicles Using Fixed-Time Non-Singular Terminal Sliding Mode Strategy" Journal of Marine Science and Engineering 10, no. 9: 1308. https://doi.org/10.3390/jmse10091308
APA StyleEr, M. J., & Li, Z. (2022). Formation Control of Unmanned Surface Vehicles Using Fixed-Time Non-Singular Terminal Sliding Mode Strategy. Journal of Marine Science and Engineering, 10(9), 1308. https://doi.org/10.3390/jmse10091308






