Estimation of CO2 Separation Performances through CHA-Type Zeolite Membranes Using Molecular Simulation
Abstract
:1. Introduction
2. Theory
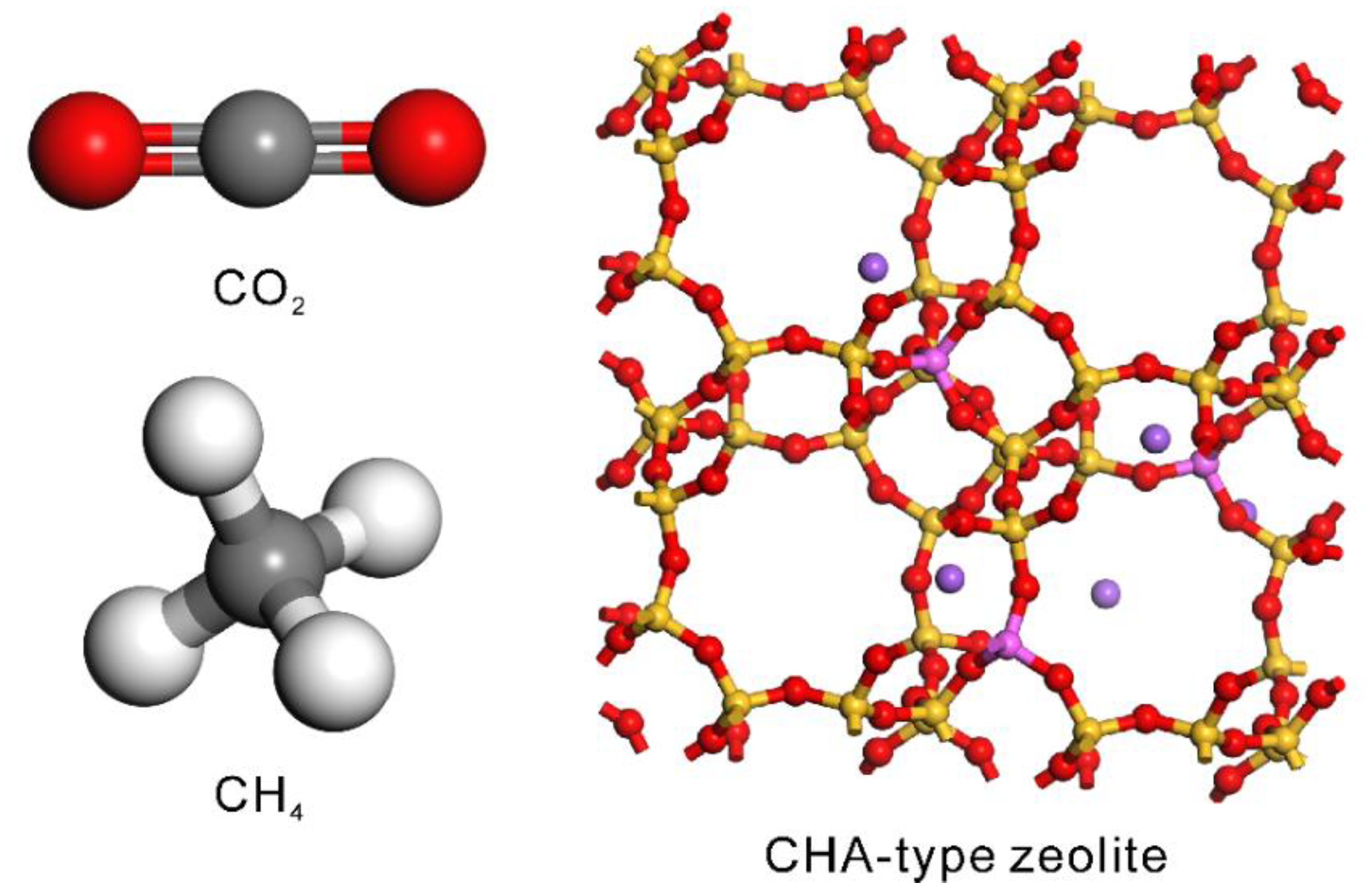
2.1. Molecular Simulation
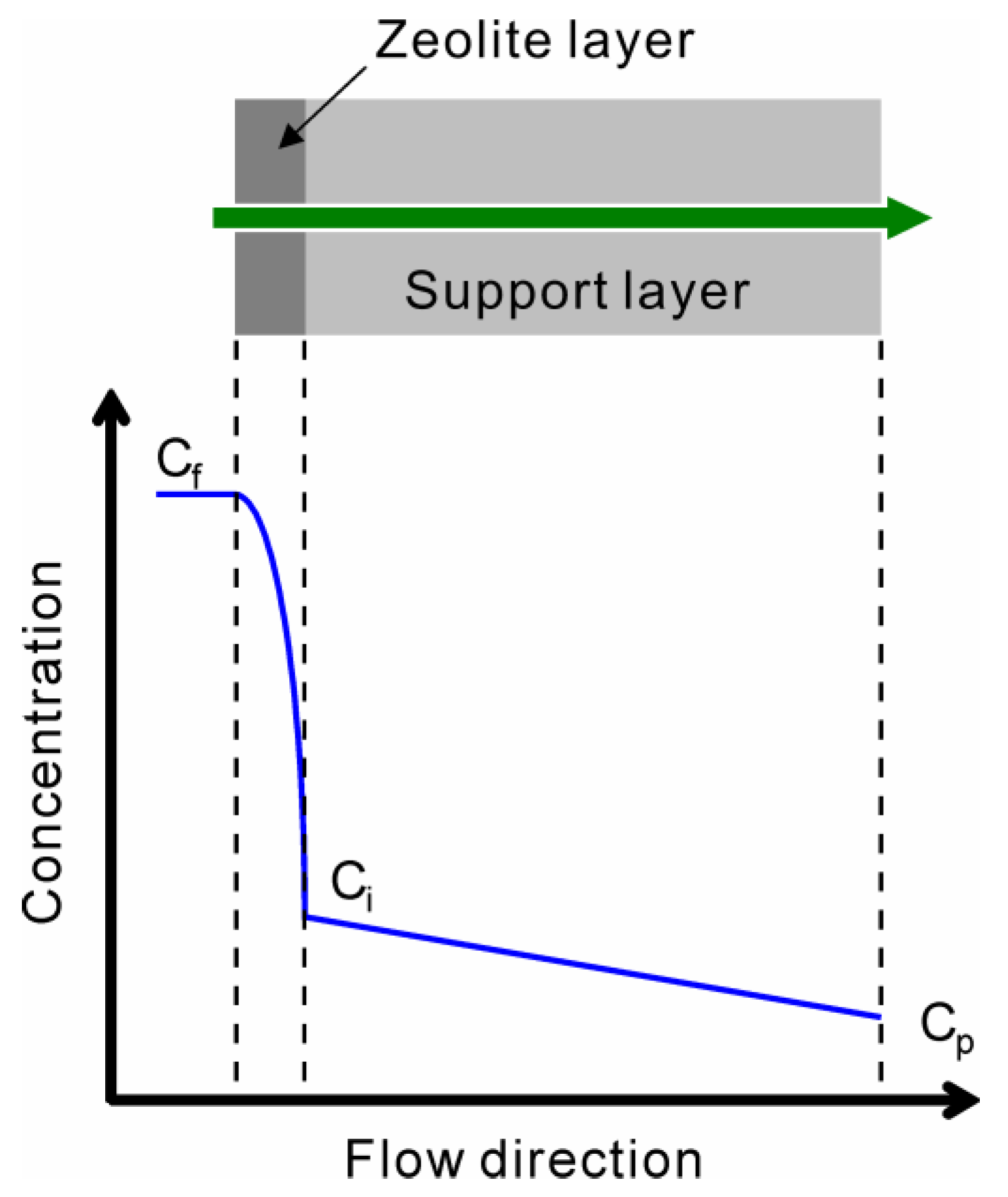
2.2. Gas permeation through Zeolite Layer
2.3. Mass Transfer in the Support Tube
3. Methods
3.1. Adsorption on Zeolites
3.2. Diffusion in Zeolite
4. Results and Discussion
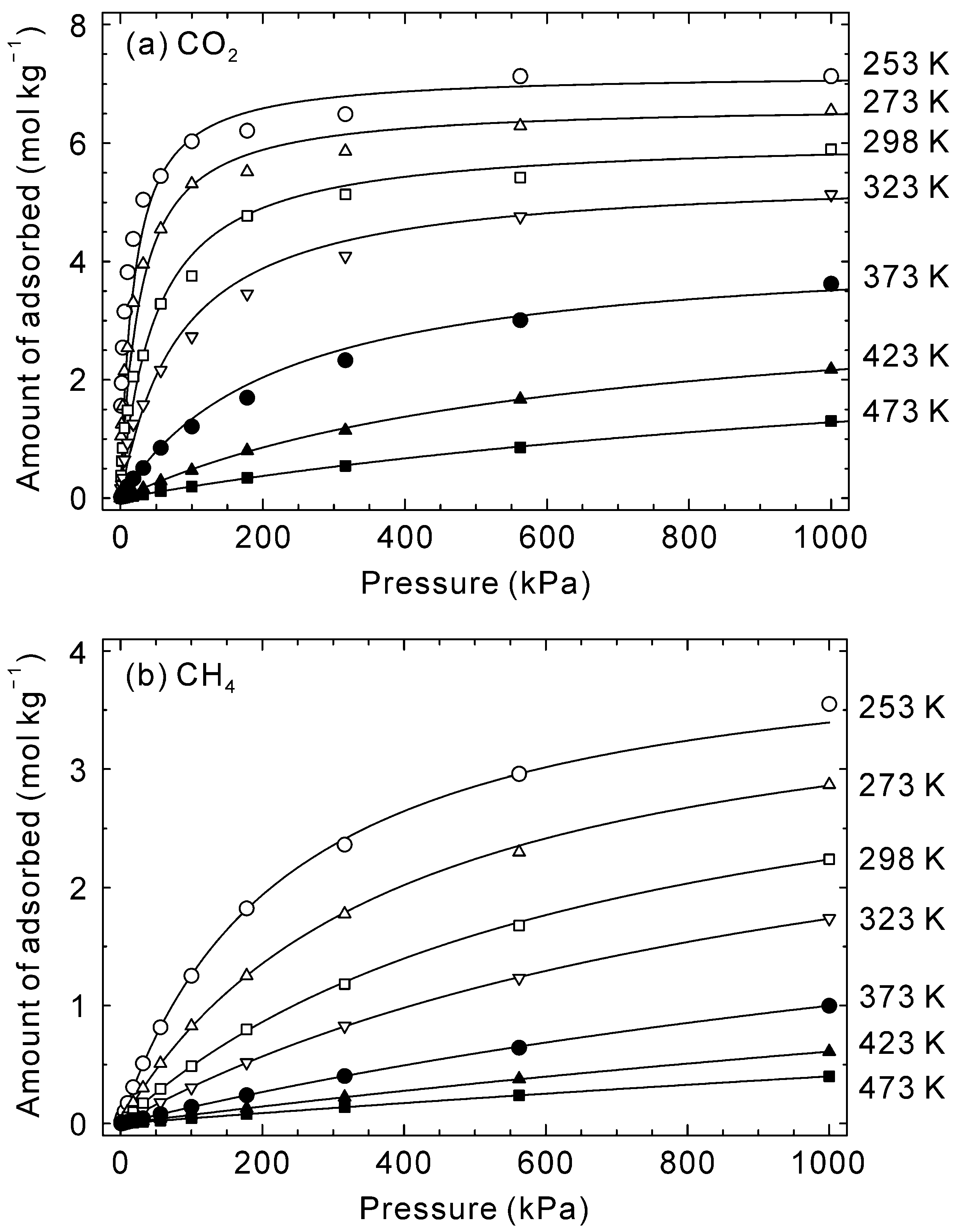
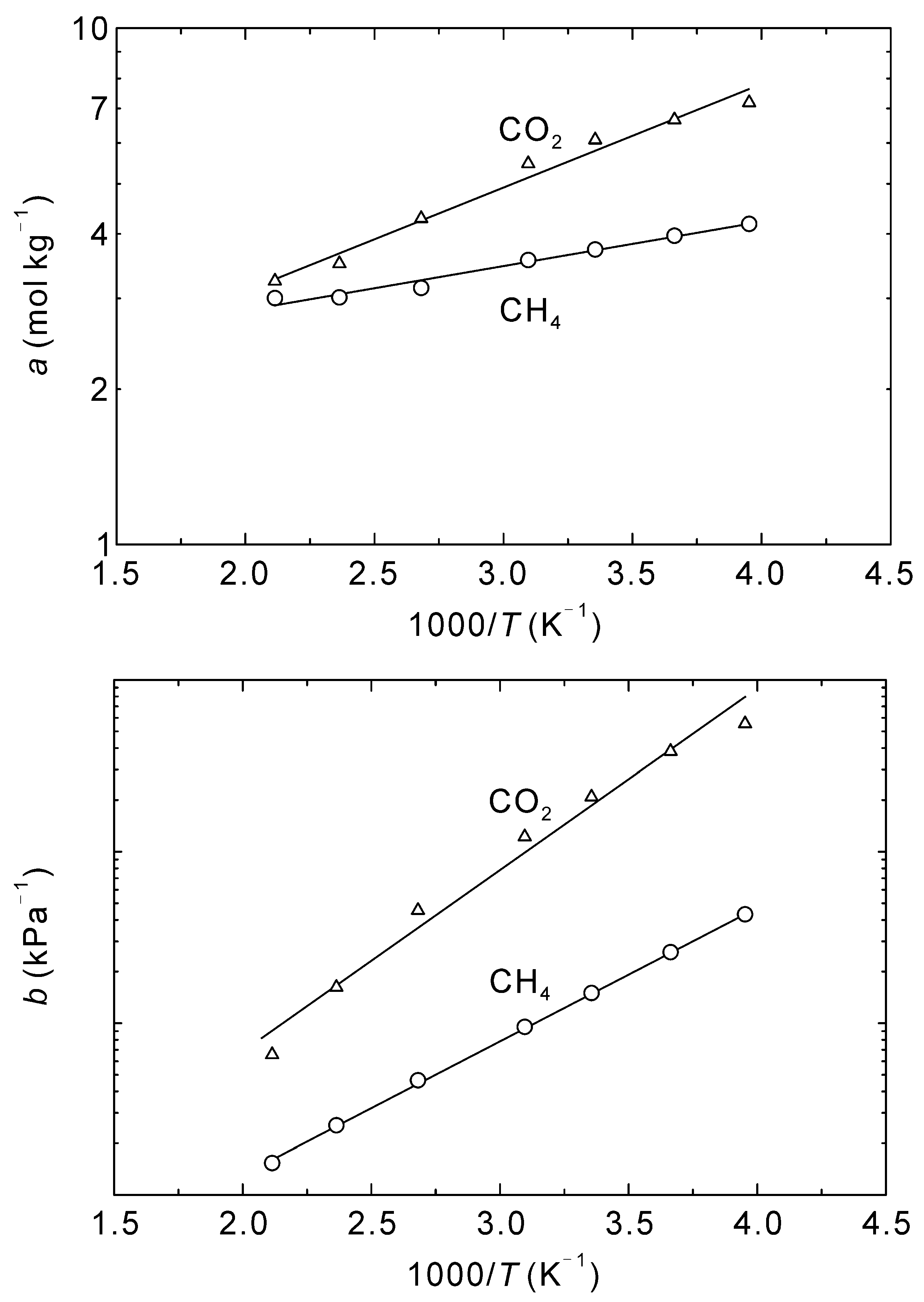
4.1. Adsorption Isotherms
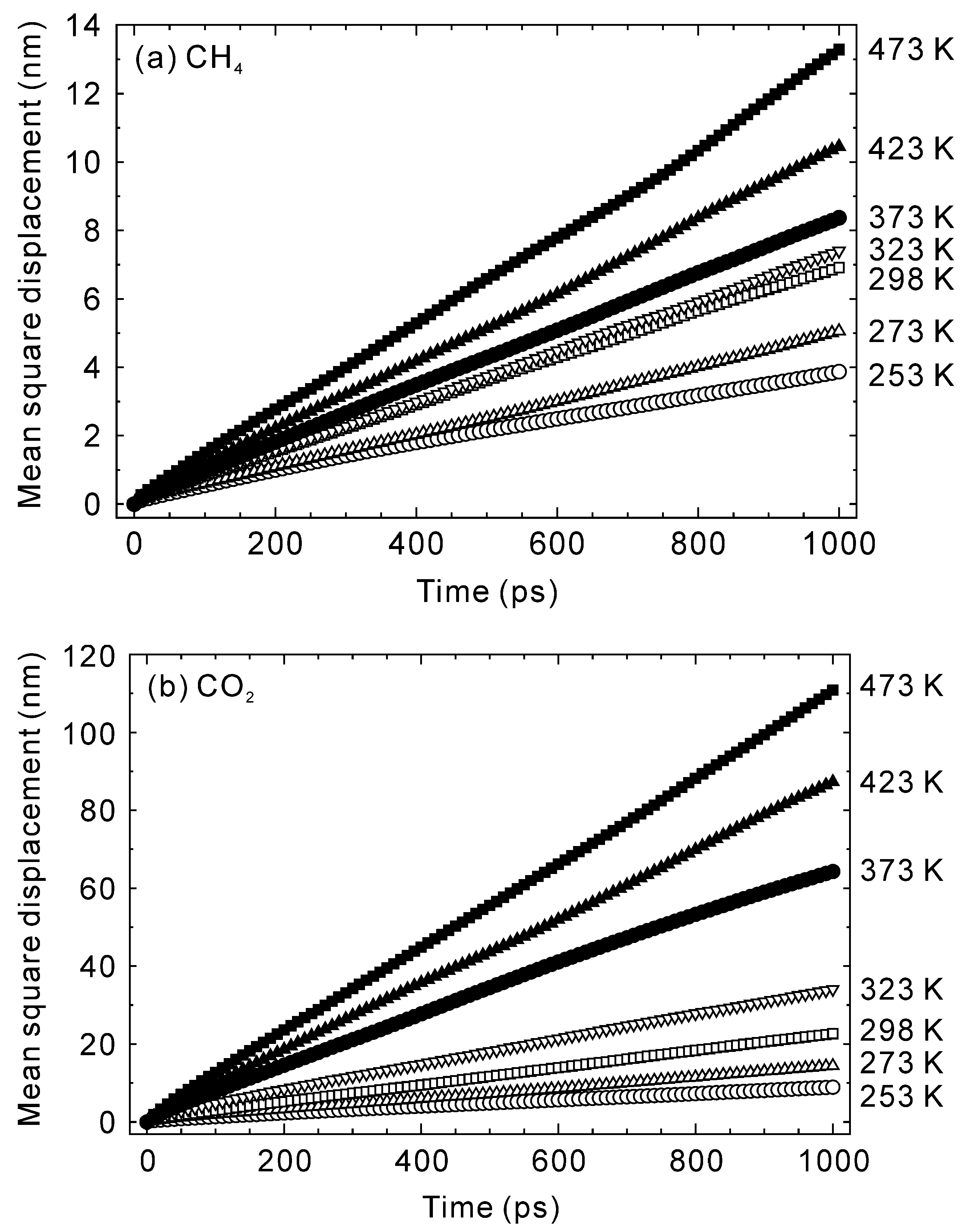
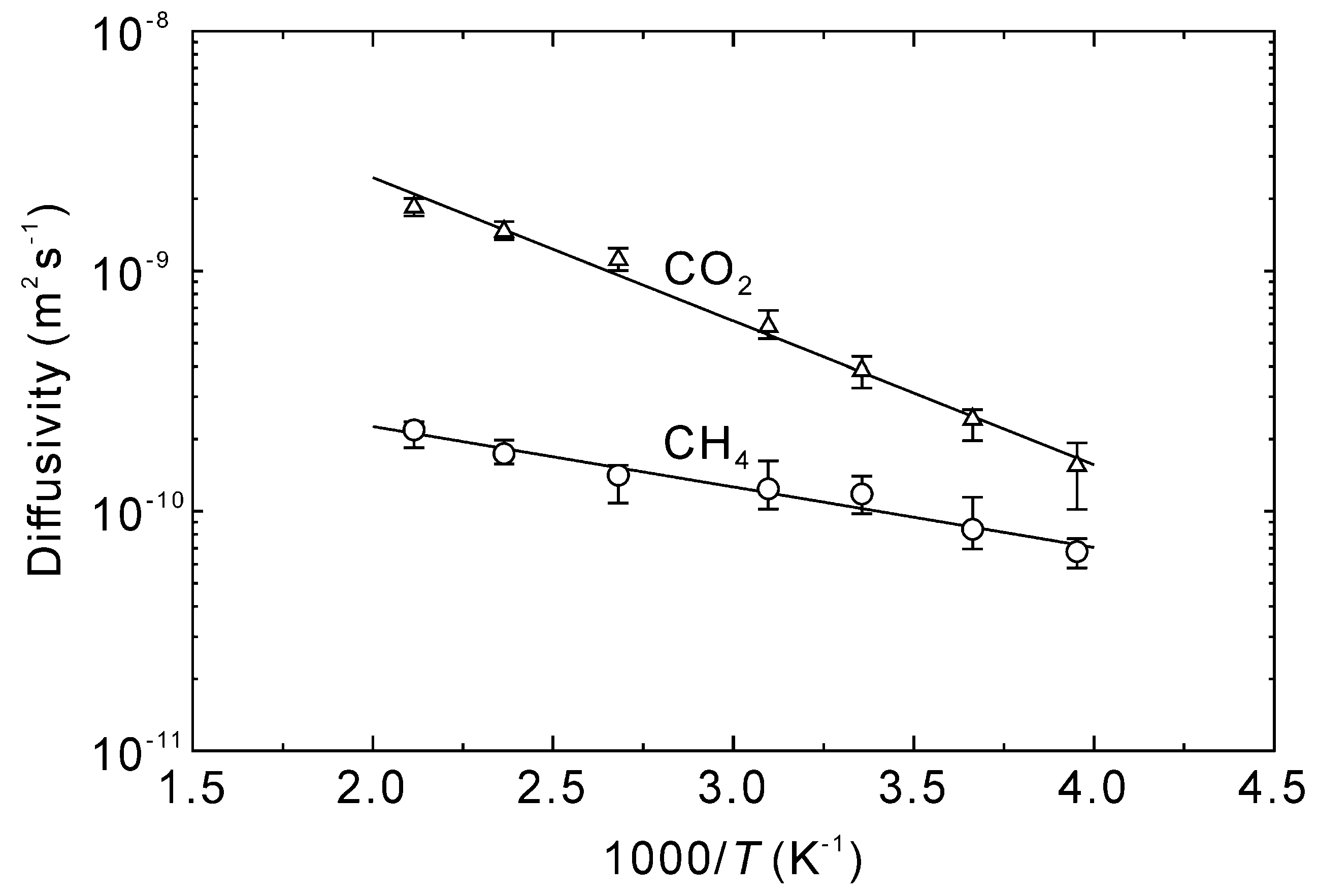
4.2. Diffusivities
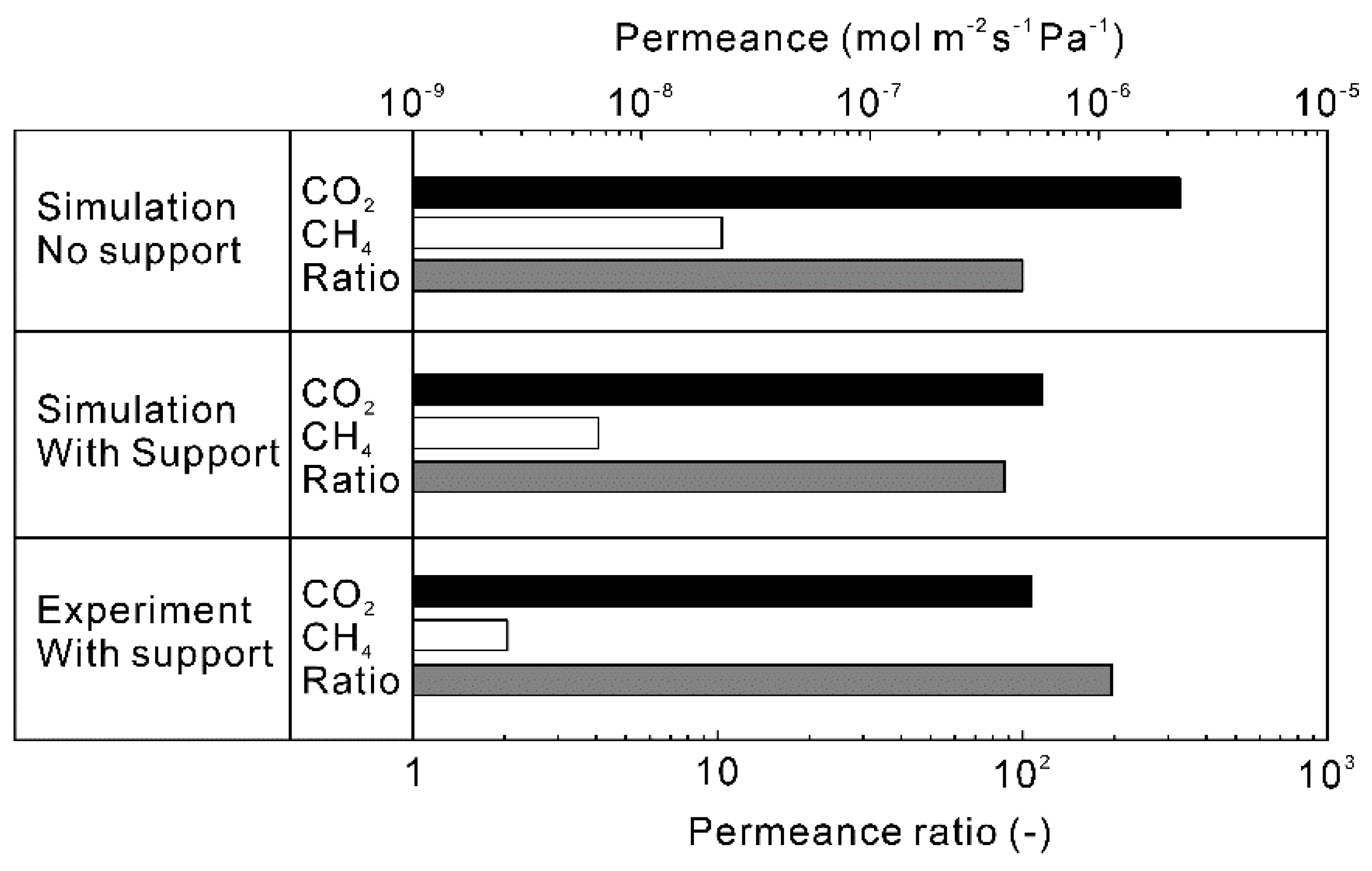
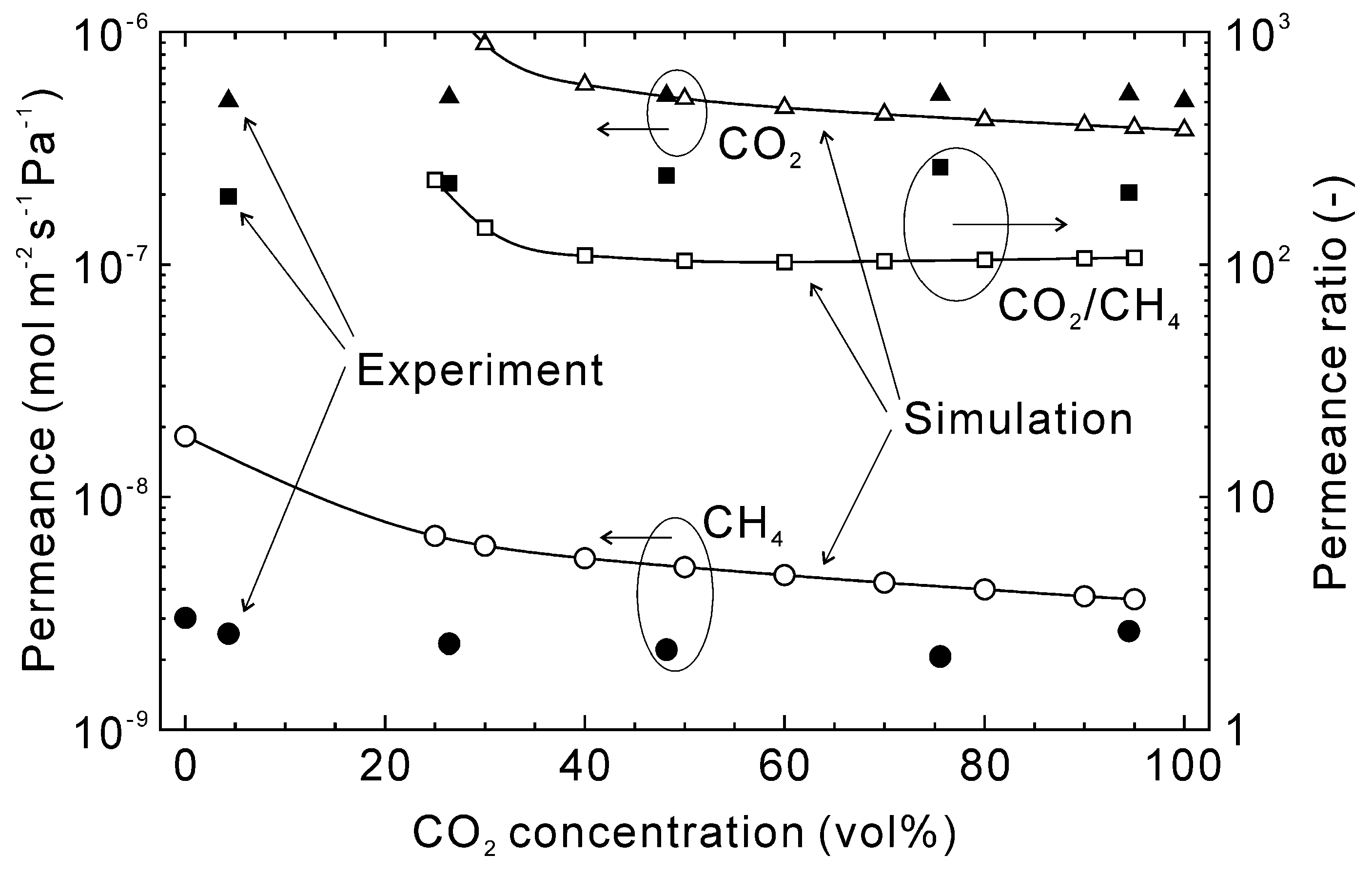
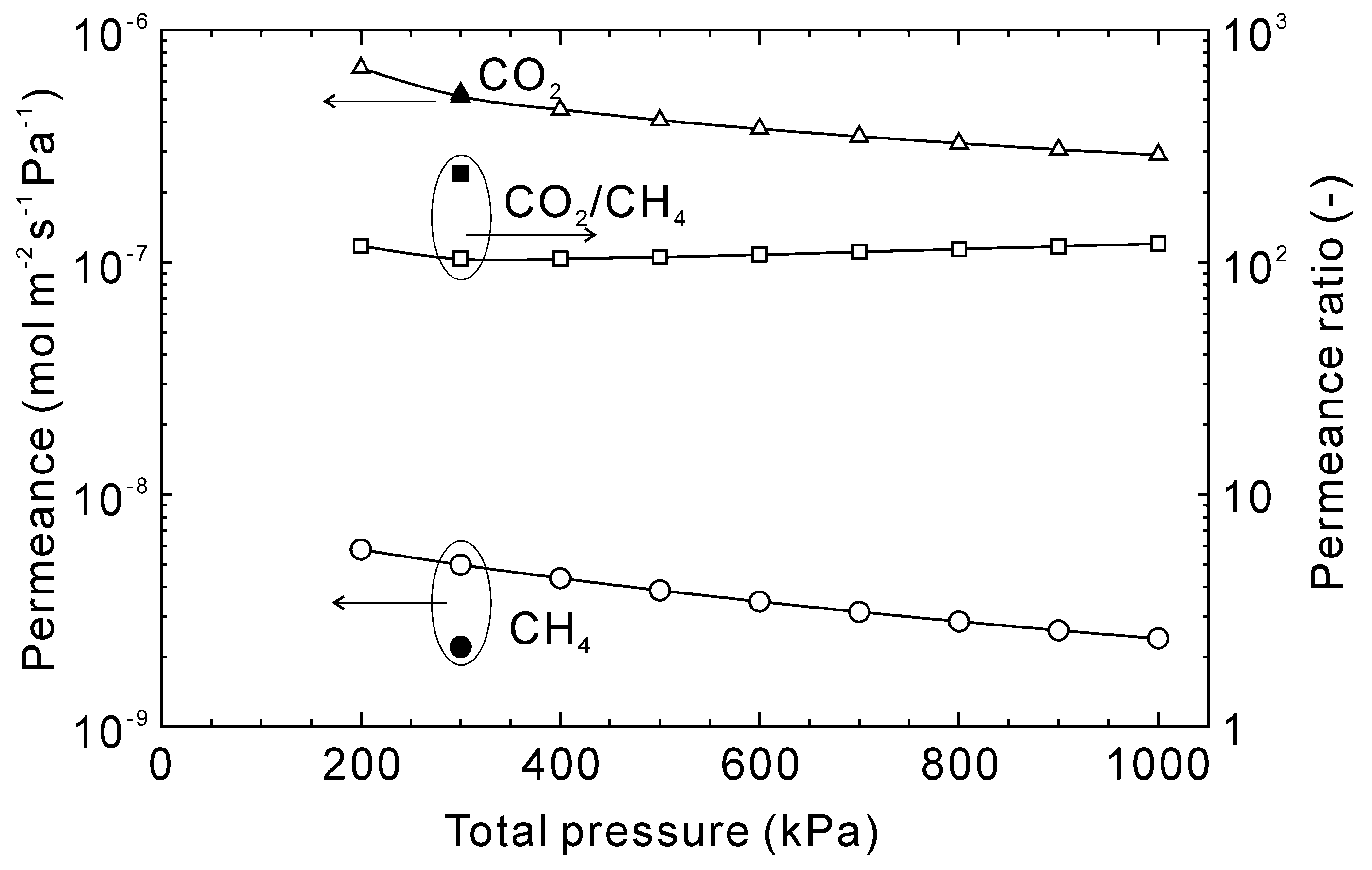
4.3. CO2 Separation Performance
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Data Availability Statement
Conflicts of Interest
Nomenclature
| a | Amount adsorbed at saturation (mol kg−1) |
| b | Langmuir constant (Pa−1) |
| B | Mobility as defined by Equation (11) |
| C | Concentration (mol m−3) |
| D | Diffusivity (m2 s−1) |
| E | Activation energy (kJ mol−1) |
| J | Permeation flux (mol m−2 s−1 Pa−1) |
| kb | Force constant for bond-stretching (kJ mol−1 m−2) |
| kθ | Force constant for angle-bending (kJ mol−1 rad−2) |
| L | Thickness (m) |
| M | Molecular mass (g mol−1) |
| p | Partial pressure (Pa) |
| Q | Partial atomic charge (e) |
| q | Amount of adsorbed (mol kg−1) |
| r | Distance (m) |
| R | Gas constant (=8.314 J K−1 mol−1) |
| T | Temperature (K) |
| V | Diffusion volume (cm3) |
| Symbols | |
| Γ | Thermodynamic factor defined by Equation (14) |
| δ | Kronecker delta (dimensionless) |
| ε | Porosity (dimensionless) |
| ε0 | Vacuum permittivity (=8.85 × 10−12 F m−1) |
| Φ | Interaction potential energy (kJ mol−1) |
| ϕ | Depth of potential (kJ mol−1) |
| θ | Binding angle (rad) |
| Θ | Surface coverage (dimensionless) |
| ρ | Density of zeolite (kg m−3) |
| σ | Distance at zero-potential energy (m) |
References
- Geus, E.R.; den Ester, M.J.; van Bekkum, H. Synthesis and characterization of zeolite (MFI) membranes on porous ceramic supports. J. Chem. Soc. Faraday Trans. 1992, 80, 3101–3109. [Google Scholar] [CrossRef]
- Geus, E.R.; van Bekkum, H.; Bakker, W.J.W.; Moulijn, J.A. High-temperature stainless steel supported zeolite (MFI) membranes: Preparation, module construction, and permeation experiments. Microp. Mater. 1993, 1, 131–147. [Google Scholar] [CrossRef]
- Kapteijn, F.; Bakker, W.J.W.; Zheng, G.; Poppe, J.; Moulijn, J.A. Permeation and separation of light hydrocarbons through a silicalite-1 membrane: Application of the generalized Maxwell-Stefan equations. Chem. Eng. J. 1995, 57, 145–153. [Google Scholar]
- Bakker, W.J.W.; van den Broeke, L.P.J.; Kapteijn, F.; Moulijn, J.A. Temperature dependence of one-component permeation through a silicalite-1 membrane. AIChE J. 1997, 43, 2203–2214. [Google Scholar] [CrossRef]
- van den Broeke, L.P.J.; Bakker, W.J.W.; Kapteijn, F.; Moulijn, J.A. Binary permeation through a silicalite-1 membrane. AIChE J. 1999, 45, 976–985. [Google Scholar] [CrossRef]
- Okamoto, K.; Kita, H.; Horii, K.; Tanaka, K. Zeolite NaA membrane: Permeation single-gas permeation, and pervaporation and vapor permeation of water/organic liquid mixtures. Ind. Eng. Chem. Res. 2001, 40, 163–175. [Google Scholar] [CrossRef]
- Kondo, M.; Komori, M.; Kita, H.; Okamoto, K. Tubular-type pervaporation module with zeolite NaA membrane. J. Membr. Sci. 1997, 133, 133–141. [Google Scholar] [CrossRef]
- Morigami, Y.; Kondo, M.; Abe, J.; Kita, H.; Okamoto, K. The first large-scale pervaporation plant using tubular-type module with zeolite NaA membrane. Sep. Purif. Technol. 2001, 25, 251–260. [Google Scholar] [CrossRef]
- Parasetya, N.; Himma, N.F.; Sutrisna, P.D.; Wenten, I.G. A review on emerging organic-containing microporous material membranes for carbon capture and separation. Chem. Eng. Sci. 2020, 391, 123575. [Google Scholar] [CrossRef]
- Han, Y.; Ho, W.S.W. Polymeric membranes for CO2 separation and capture. J. Membr. Sci. 2021, 628, 119244. [Google Scholar] [CrossRef]
- Hu, L.; Clark, K.; Alebrahim, T.; Lin, H. Mixed matrix membranes for post-combustion carbon capture: From materials design to membrane engineering. J. Membr. Sci. 2022, 644, 120140. [Google Scholar] [CrossRef]
- Usman, M.; Khan, M.Y.; Anjum, T.; Khan, A.L.; Hoque, B.; Helal, A.; Hakeem, A.S.; Al-Maythalony, B.A. Controlled covalent functionalization of ZIF-90 for selective CO2 capture and separation. Membranes 2022, 12, 1055. [Google Scholar] [CrossRef] [PubMed]
- Hasegawa, Y.; Tanak, T.; Watanabe, K.; Jeong, B.H.; Kusakabe, K.; Morooka, S. Separation of CO2-CH4 and CO2-N2 systems using ion-exchanged FAU-type zeolite membranes with different Si/Al ratios. Korean J. Chem. Eng. 2002, 19, 309–313. [Google Scholar] [CrossRef]
- Kusakabe, K.; Kuroda, T.; Uchino, K.; Hasegawa, Y.; Morooka, S. Gas permeation properties of ion-exchanged faujasite-type zeolite membranes. AIChE J. 1999, 45, 1220–1226. [Google Scholar] [CrossRef]
- Hasegawa, Y.; Watanabe, K.; Kusakabe, K.; Morooka, S. The separation of CO2 using T-type zeolite membranes ion-exchanged with alkali metal cations. Sep. Purif. Technol. 2001, 22–23, 319–325. [Google Scholar] [CrossRef]
- Hasegawa, Y.; Kusakabe, K.; Morooka, S. Effect of temperature on the gas permeation properties of NaY-type zeolite formed on the inner surface of a porous support tube. Chem. Eng. Sci. 2001, 56, 4273–4281. [Google Scholar] [CrossRef]
- Poshusta, J.C.; Tuan, V.A.; Falconer, J.L.; Noble, R.D. Synthesis and permeation properties of SAPO-34 tubular membranes. Ind. Eng. Chem. Res. 1998, 37, 3924–3929. [Google Scholar] [CrossRef]
- Poshusta, J.C.; Tuan, V.A.; Pape, E.A.; Noble, R.D.; Falconer, J.L. Separation of light gas mixtures using SAPO-34 membranes. AIChE J. 2000, 46, 779–789. [Google Scholar] [CrossRef]
- Crreon, M.A.; Li, S.; Falconer, J.L.; Noble, R.D. Alumina-supported SAPO-34 membranes for CO2/CH4 separation. J. Am. Chem. Soc. 2008, 130, 5412–5413. [Google Scholar] [CrossRef]
- Tomita, T.; Nakayama, K.; Sakai, H. Gas separation characteristics of RRR type zeolite membrane. Micropor. Mesopor. Mater. 2004, 68, 71–75. [Google Scholar] [CrossRef]
- van den Bergh, J.; Zhu, W.; Gascon, J.; Moulijn, J.A.; Kapteijn, F. Separation and permeation characteristics of a DD3R zeolite membrane. J. Membr. Sci. 2008, 316, 35–45. [Google Scholar] [CrossRef]
- Wang, B.; Hu, N.; Wang, H.; Zheng, Y.; Zhou, R. Improved AlPO-18 membranes for light gas separation. J. Mater. Chem. A 2015, 3, 12205–12212. [Google Scholar] [CrossRef]
- Wang, B.; Gao, F.; Zhang, F.; Xing, W.; Zhou, R. Highly permeable and oriented AlPO-18 membrane prepared using directly synthesized nanosheets for CO2/CH4 separation. J. Mater. Chem. A 2019, 7, 13164–13172. [Google Scholar] [CrossRef]
- Zhan, T.; Wu, T.; Shi, Y.; Chen, X.; Li, Y.; Zhu, M.; Kumakiri, I.; Chen, X.; Kita, H. Influence of synthesis parameters on the preparation of AlPO-18 membranes by single DIPEA for CO2/CH4 separation. J. Membr. Sci. 2020, 601, 117853. [Google Scholar] [CrossRef]
- Imasaka, S.; Itakura, M.; Yano, K.; Fujita, S.; Okada, M.; Hasegawa, Y.; Abe, C.; Araki, S.; Yamamoto, H. Rapid preparation of high-silica CHA-type zeolite membranes and their separation properties. Sep. Purif. Technol. 2018, 199, 298–303. [Google Scholar] [CrossRef]
- Hasegawa, Y.; Abe, C.; Ikeda, T.; Sato, K.; Imasaka, S.; Itakura, M.; Yano, K. Influence of the synthesis parameters on the morphology and dehydration performance of high-silica chabazite membranes. Adv. Porus Mater. 2016, 4, 134–143. [Google Scholar] [CrossRef]
- Hasegawa, Y.; Abe, C.; Ikeda, A. Pervaporative dehydration of organic solvents using high-silica CHA-type zeolite membrane. Membranes 2021, 11, 229. [Google Scholar] [CrossRef]
- Hasegawa, Y.; Abe, C.; Natsui, M.; Ikeda, A. Gas permeation properties of high-silica CHA-type zeolite membrane. Membranes 2021, 11, 249. [Google Scholar] [CrossRef]
- Talu, O.; Myers, A.L. Reference potentials for adsorption of helium, argon, methane, and krypton in high-silica zeolites. Colloids Surf. A 2001, 187–188, 83–93. [Google Scholar] [CrossRef]
- Bai, P.; Tsapatsis, M.; Siepmann, J.I. TraPPE-zeo: Transferable potentials for phase equilibria force field for all-silica zeolites. J. Phys. Chem. C 2013, 117, 24375–24387. [Google Scholar] [CrossRef]
- Rahmati, M.; Modarress, H. Selectivity of new siliceous zeolites for separation of methane and carbon dioxide by Monte Carlo simulation. Micropor. Mesopor. Mater. 2013, 176, 168–177. [Google Scholar] [CrossRef]
- Pham, T.D.; Xiong, R.; Dandler, S.I.; Lobo, R.F. Experimental and computational studies on the adsorption of CO2 and N2 on pure silica zeolites. Micropor. Mesopor. Mater. 2014, 185, 157–166. [Google Scholar] [CrossRef]
- Vujic, B.; Lyubartsev, A.P. Transferable forcefield for modelling of CO2, N2, O2, and Ar in all-silica and Na+ exchanged zeolites. Modelling Simul. Mater. Sci. Eng. 2016, 24, 045002. [Google Scholar] [CrossRef]
- Hasegawa, Y.; Abe, C. Prediction of adsorption and diffusion behaviors of CO2 and CH4 in all-silica zeolites using molecular simulation. Membranes 2021, 11, 392. [Google Scholar] [CrossRef] [PubMed]
- Poling, B.E.; Prausnitz, J.M.; O’Connell, J.P. The Properties of Gases and Liquids, 5th ed.; McGraw Hill: New York, NY, USA, 2000. [Google Scholar]
- Harris, J.G.; Yung, K.H. Carbon dioxide’s liquid-vapor coexistence curve and critical properties as predicted by a simple molecular model. J. Phys. Chem. 1995, 99, 12021–12024. [Google Scholar] [CrossRef]
- Martin, M.G.; Siepmann, J.I. Transferable potentials for phase equilibria. 1. United-atom description of n-alkanes. J. Phys. Chem. B 1998, 102, 2569–2577. [Google Scholar] [CrossRef]
- Baerlocher, C.; McCusker, L.B.; Olson, D.H. Atlas of Zeolite Framework Types, 6th ed.; Elsevier: Amsterdam, The Netherlands, 2007. [Google Scholar]
- Pham, T.D.; Liu, Q.; Lobo, R.F. Carbon dioxide and nitrogen adsorption on cation exchanged SSZ-13 zeolites. Langmuir 2013, 29, 832–839. [Google Scholar] [CrossRef]
- Maghsoudi, H.; Soltanieh, M.; Bozorgzadeh, H.; Mohamadalizadeh, A. Adsorption isotherms and ideal selectivities of hydrogen sulfide and carbon dioxide over methane for the Si-CHA zeolite: Comparison of carbon dioxide and methane adsorption with the all-silica DD3R zeolite. Adsorption 2013, 19, 1045–1053. [Google Scholar] [CrossRef]
- Breck, D.W.D. Zeolite Molecular Sieves; Wiley: New York, NY, USA, 1974. [Google Scholar]
- Krishna, R.; van Baten, J.M.; Garcia-Perez, E.; Calero, S. Diffusion of CH4 and CO2 in MFI, CHA and DDR zeolites. Chem. Phys. Lett. 2006, 429, 219–224. [Google Scholar] [CrossRef]
- Sladek, K.J.; Gilliland, E.R.; Baddour, R.F. Diffusion on surfaces. II. Correlation of diffusivities of physically and chemically adsorbed species. Ind. Eng. Chem. Fundam. 1974, 13, 100–105. [Google Scholar] [CrossRef]

| Molecule | Element | σ (nm) | ε/k (K) | q (e) | Ref. |
|---|---|---|---|---|---|
| CH4 | C | 0.3730 | 148.0 | 0 | [33] |
| H | --- | --- | 0 | ||
| CO2 | C | 0.2757 | 28.1 | 0.6512 | [32] |
| O | 0.3033 | 80.5 | −0.3256 | ||
| Zeolite | Si | 0.2970 | 32.0 | 1.413 | [29] |
| Al | 0.3140 | 24.0 | 1.072 | ||
| O(Si–O–Si) | 0.3011 | 52.0 | −0.7065 | ||
| O(Si–O–Al) | 0.3011 | 55.0 | −0.8712 | ||
| Na | 0.3230 | 234.1 | 1.000 |
| Unit | CO2 | CH4 | |
|---|---|---|---|
| ai* | mol kg−1 | 1.24 | 1.92 |
| Ea | kJ mol−1 | 3.8 | 1.6 |
| bi* | kPa−1 | 5.53 × 10−6 | 3.57 × 10−6 |
| Eb | kJ mol−1 | 20.0 | 15.0 |
| Unit | CO2 | CH4 | |
|---|---|---|---|
| Di* | m2 s−1 | 3.8 × 10−8 | 7.2 × 10−9 |
| Ed | kJ mol−1 | 11.4 | 4.8 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Hasegawa, Y.; Natsui, M.; Abe, C.; Ikeda, A.; Lundin, S.-T.B. Estimation of CO2 Separation Performances through CHA-Type Zeolite Membranes Using Molecular Simulation. Membranes 2023, 13, 60. https://doi.org/10.3390/membranes13010060
Hasegawa Y, Natsui M, Abe C, Ikeda A, Lundin S-TB. Estimation of CO2 Separation Performances through CHA-Type Zeolite Membranes Using Molecular Simulation. Membranes. 2023; 13(1):60. https://doi.org/10.3390/membranes13010060
Chicago/Turabian StyleHasegawa, Yasuhisa, Mayumi Natsui, Chie Abe, Ayumi Ikeda, and Sean-Thomas B. Lundin. 2023. "Estimation of CO2 Separation Performances through CHA-Type Zeolite Membranes Using Molecular Simulation" Membranes 13, no. 1: 60. https://doi.org/10.3390/membranes13010060
APA StyleHasegawa, Y., Natsui, M., Abe, C., Ikeda, A., & Lundin, S.-T. B. (2023). Estimation of CO2 Separation Performances through CHA-Type Zeolite Membranes Using Molecular Simulation. Membranes, 13(1), 60. https://doi.org/10.3390/membranes13010060









