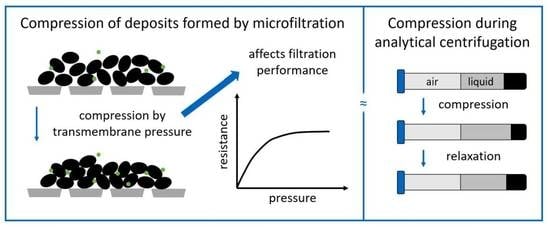
Determination of Compressibility and Relaxation Behavior of Yeast Cell Sediments by Analytical Centrifugation and Comparison with Deposit Formation on Membrane Surfaces
Abstract
:1. Introduction
2. Materials and Methods
2.1. Preparation of the Cell Suspension
2.2. Analytical Methods
2.3. Compressibility Analysis
2.4. Dead-End Filtration
2.5. Data Handling and Statistics
3. Results and Discussion
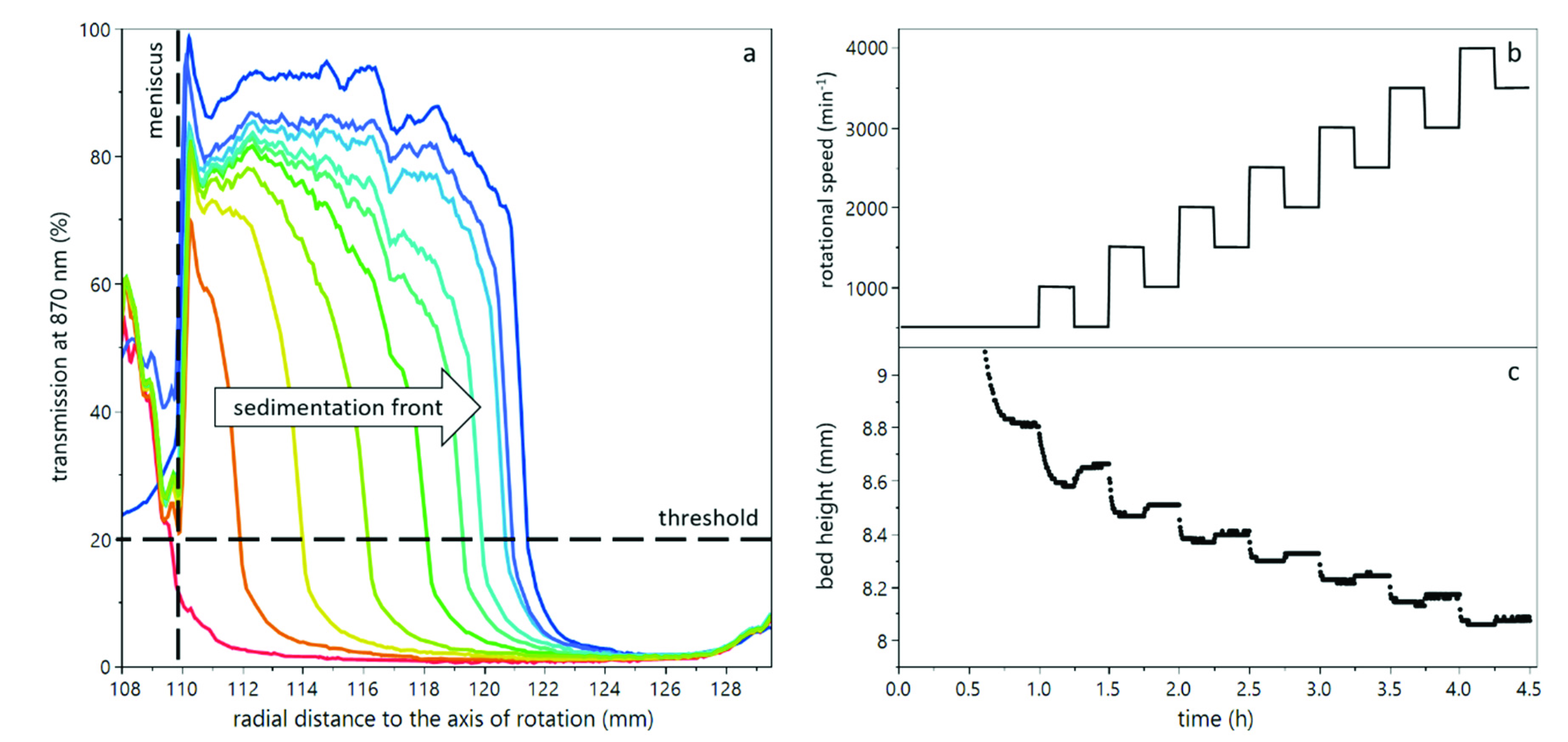
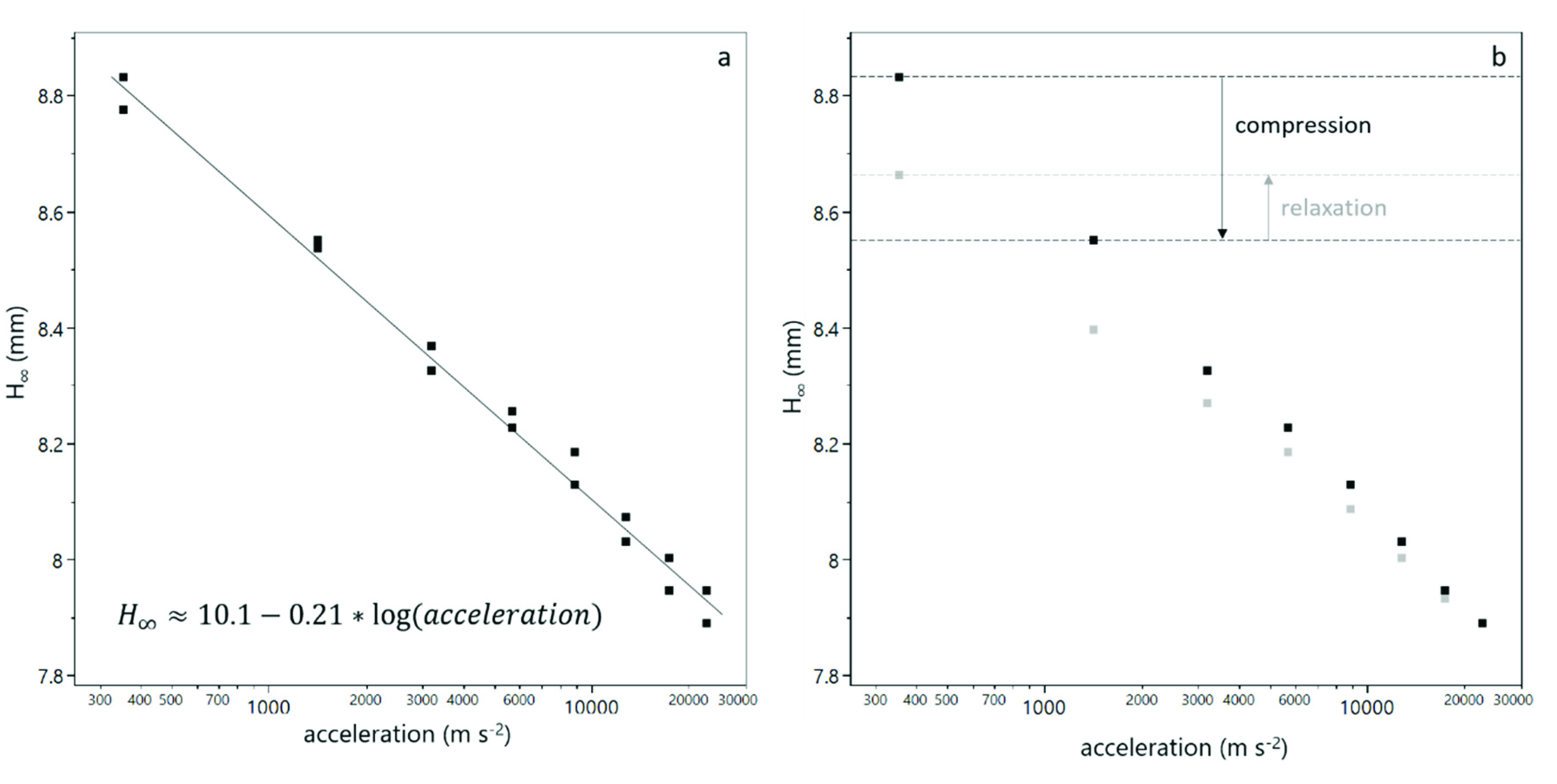
3.1. Compression of Yeast Deposits during Analytical Centrifugation
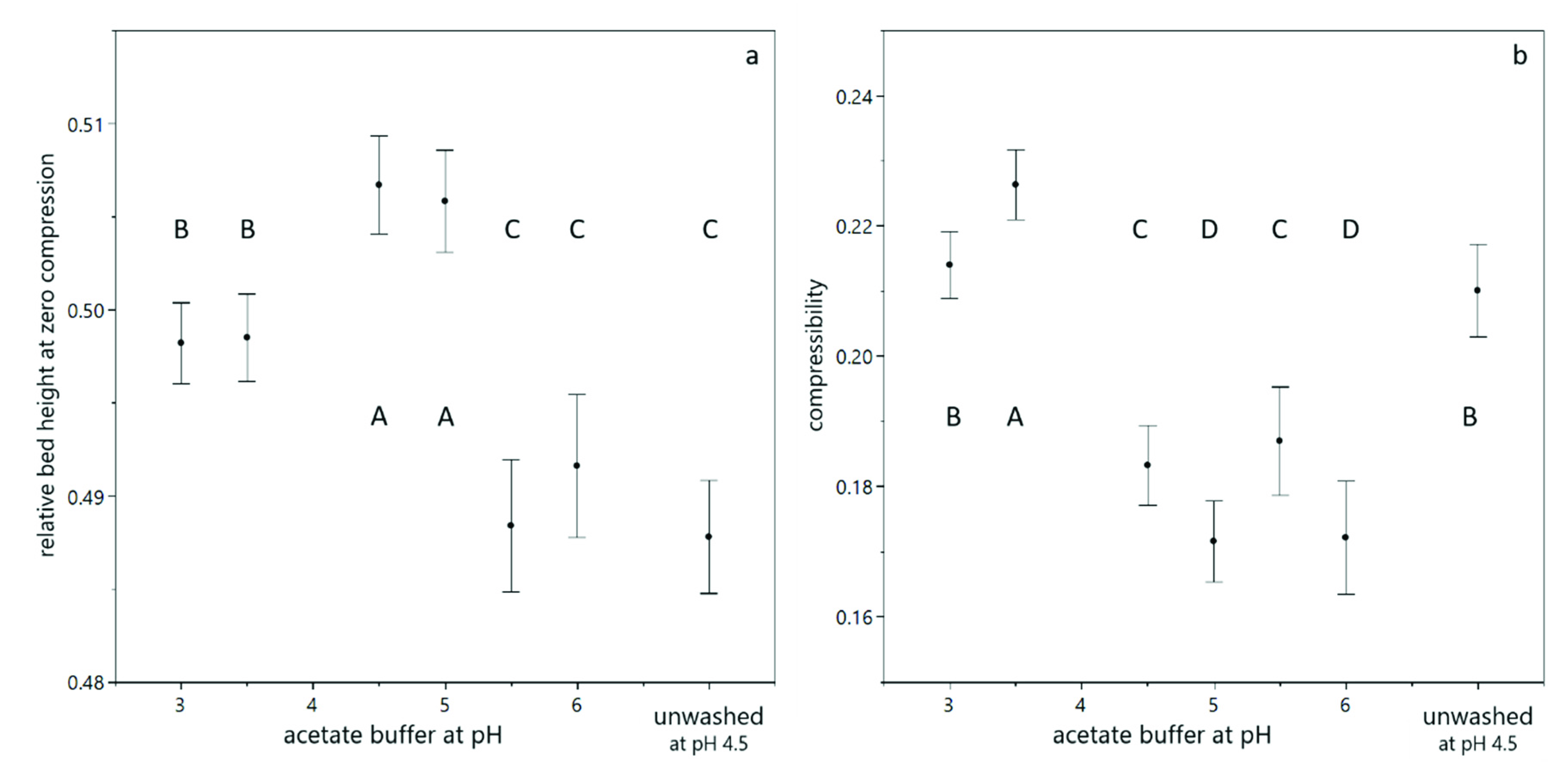
3.2. Deposit Layer Formation and Filtration Performance
3.3. Comments on the Applicability of Analytical Centrifugation
4. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Belfort, G.; Davis, R.H.; Zydney, A.L. The behavior of suspensions and macromolecular solutions in crossflow microfiltration. J. Membr. Sci. 1994, 96, 1–58. [Google Scholar] [CrossRef]
- Tang, C.Y.; Chong, T.H.; Fane, A.G. Colloidal interactions and fouling of NF and RO membranes: A review. Adv. Colloid Interface Sci. 2011, 164, 126–143. [Google Scholar]
- Mendret, J.; Guigui, C.; Schmitz, P.; Cabassud, C. In situ dynamic characterisation of fouling under different pressure conditions during dead-end filtration: Compressibility properties of particle cakes. J. Membr. Sci. 2009, 333, 20–29. [Google Scholar] [CrossRef]
- Wu, D.; Howell, J.A.; Field, R.W. Critical flux measurement for model colloids. J. Membr. Sci. 1999, 152, 89–98. [Google Scholar] [CrossRef]
- Taheri, A.H.; Sim, L.N.; Krantz, W.B.; Fane, A.G. Ultrafiltration with intermittent relaxation using colloidal silica and humic acid as model foulants. Sep. Purif. Technol. 2019, 212, 262–272. [Google Scholar] [CrossRef]
- Weinberger, M.E.; Kulozik, U. Effect of low-frequency pulsatile crossflow microfiltration on flux and protein transmission in milk protein fractionation. Sep. Sci. Technol. 2021, 56, 1112–1127. [Google Scholar] [CrossRef]
- Weinberger, M.E.; Kulozik, U. Pulsatile crossflow improves microfiltration fractionation of cells and proteins. J. Membr. Sci. 2021, 629, 119295. [Google Scholar] [CrossRef]
- Hwang, K.-J.; Sz, P.-Y. Filtration characteristics and membrane fouling in cross-flow microfiltration of BSA/dextran binary suspension. J. Membr. Sci. 2010, 347, 75–82. [Google Scholar] [CrossRef]
- Hwang, K.-J.; Wang, Y.-T.; Iritani, E.; Katagiri, N. Effects of porous gel particle compression properties on microfiltration characteristics. J. Membr. Sci. 2009, 341, 286–293. [Google Scholar] [CrossRef]
- Bacchin, P.; Aimar, P.; Field, R.W. Critical and sustainable fluxes: Theory, experiments and applications. J. Membr. Sci. 2006, 281, 42–69. [Google Scholar] [CrossRef] [Green Version]
- Loginov, M.; Lebovka, N.; Vorobiev, E. Multistage centrifugation method for determination of filtration and consolidation properties of mineral and biological suspensions using the analytical photocentrifuge. Chem. Eng. Sci. 2014, 107, 277–289. [Google Scholar] [CrossRef]
- Ye, Y.; Chen, V. Reversibility of heterogeneous deposits formed from yeast and proteins during microfiltration. J. Membr. Sci. 2005, 265, 20–28. [Google Scholar] [CrossRef]
- Iritani, E.; Katagiri, N.; Inagaki, G. Compression and expansion properties of filter cake accompanied with step change in applied pressure in membrane filtration. Sep. Purif. Technol. 2018, 198, 3–9. [Google Scholar] [CrossRef]
- Hartinger, M.; Heidebrecht, H.-J.; Schiffer, S.; Dumpler, J.; Kulozik, U. Milk protein fractionation by means of spiral-wound microfiltration membranes: Effect of the pressure adjustment mode and temperature on flux and protein permeation. Foods 2019, 8, 180. [Google Scholar] [CrossRef] [Green Version]
- Loginov, M.; Zierau, A.; Kavianpour, D.; Lerche, D.; Vorobiev, E.; Gésan-Guiziou, G.; Mahnic-Kalamiza, S.; Sobisch, T. Multistep centrifugal consolidation method for characterization of filterability of aggregated concentrated suspensions. Sep. Purif. Technol. 2017, 183, 304–317. [Google Scholar] [CrossRef]
- Howell, J.A.; Field, R.W.; Wu, D. Yeast cell microfiltration: Flux enhancement in baffled and pulsatile flow systems. J. Membr. Sci. 1993, 80, 59–71. [Google Scholar] [CrossRef]
- Kuberkar, V.T.; Davis, R.H. Microfiltration of protein-cell mixtures with crossflushing or backflushing. J. Membr. Sci. 2001, 183, 1–14. [Google Scholar] [CrossRef]
- Mores, W.D.; Davis, R.H. Yeast foulant removal by backpulses in crossflow microfiltration. J. Membr. Sci. 2002, 208, 389–404. [Google Scholar] [CrossRef]
- Redkar, S.G.; Davis, R.H. Crossflow microfiltration of yeast suspensions in tubular filters. Biotechnol. Prog. 1993, 9, 625–634. [Google Scholar] [CrossRef]
- Ní Mhurchú, J.; Foley, G. Dead-end filtration of yeast suspensions: Correlating specific resistance and flux data using artificial neural networks. J. Membr. Sci. 2006, 281, 325–333. [Google Scholar] [CrossRef] [Green Version]
- Hughes, D.; Field, R.W. Crossflow filtration of washed and unwashed yeast suspensions at constant shear under nominally sub-critical conditions. J. Membr. Sci. 2006, 280, 89–98. [Google Scholar] [CrossRef]
- McCarthy, A.A.; Gilboy, P.; Walsh, P.K.; Foley, G. Characterisation of cake compressibility in dead-end microfiltration of microbial suspensions. Chem. Eng. Commun. 1999, 173, 79–90. [Google Scholar] [CrossRef]
- Foley, G. A review of factors affecting filter cake properties in dead-end microfiltration of microbial suspensions. J. Membr. Sci. 2006, 274, 38–46. [Google Scholar] [CrossRef]
- Buscall, R.; White, L.R. The consolidation of concentrated suspensions. Part 1—The theory of sedimentation. J. Chem. Soc. Faraday Trans. 1 Phys. Chem. Condens. Phases 1987, 83, 873–891. [Google Scholar] [CrossRef]
- Haller, N.; Kulozik, U. Separation of Whey Protein Aggregates by Means of Continuous Centrifugation. Food Bioprocess Technol. 2019, 12, 1052–1067. [Google Scholar] [CrossRef]
- Weinberger, M.E.; Andlinger, D.J.; Kulozik, U. A novel approach for characterisation of stabilising bonds in milk protein deposit layers on microfiltration membranes. Int. Dairy J. 2021, 118, 105044. [Google Scholar] [CrossRef]
- Lavaisse, L.M.; Hollmann, A.; Nazareno, M.A.; Disalvo, E.A. Zeta potential changes of Saccharomyces cerevisiae during fermentative and respiratory cycles. Colloids Surf. B Biointerfaces 2019, 174, 63–69. [Google Scholar] [CrossRef]
- Bouhid de Aguiar, I.; Schroën, K.; Meireles, M.; Bouchoux, A. Compressive resistance of granular-scale microgels: From loose to dense packing. Colloids Surf. A Physicochem. Eng. Asp. 2018, 553, 406–416. [Google Scholar] [CrossRef]




Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Weinberger, M.E.; Kulozik, U. Determination of Compressibility and Relaxation Behavior of Yeast Cell Sediments by Analytical Centrifugation and Comparison with Deposit Formation on Membrane Surfaces. Membranes 2022, 12, 603. https://doi.org/10.3390/membranes12060603
Weinberger ME, Kulozik U. Determination of Compressibility and Relaxation Behavior of Yeast Cell Sediments by Analytical Centrifugation and Comparison with Deposit Formation on Membrane Surfaces. Membranes. 2022; 12(6):603. https://doi.org/10.3390/membranes12060603
Chicago/Turabian StyleWeinberger, Maria E., and Ulrich Kulozik. 2022. "Determination of Compressibility and Relaxation Behavior of Yeast Cell Sediments by Analytical Centrifugation and Comparison with Deposit Formation on Membrane Surfaces" Membranes 12, no. 6: 603. https://doi.org/10.3390/membranes12060603
APA StyleWeinberger, M. E., & Kulozik, U. (2022). Determination of Compressibility and Relaxation Behavior of Yeast Cell Sediments by Analytical Centrifugation and Comparison with Deposit Formation on Membrane Surfaces. Membranes, 12(6), 603. https://doi.org/10.3390/membranes12060603