Heat to Hydrogen by RED—Reviewing Membranes and Salts for the RED Heat Engine Concept
Abstract
:1. Introduction
2. Performance Parameters of the RED Stack
3. The Solution Regeneration Unit
| Working Fluid / () | Regeneration Unit | RED Stack | (W) | (V) | H2 () | Ref. |
|---|---|---|---|---|---|---|
| Experimental Work | ||||||
| NH4HCO3 | air stripping + adsorption | 2.42 | no | [55,65] | ||
| NH4HCO3 | air vs. vapour stripping + absorption/ condensation | no | [43] | |||
| NH4HCO3 1.5/0.2 | distillation column | 20 cell pairs Selemion CMV/AMV 10.5 × 7.5 cm 130 m 500 m | 0.33 | 3.07 | no | [57] |
| NH4HCO3 | ||||||
| 1.5/0 | 20 cell pairs | yes | [37] | |||
| Theoretical work | ||||||
| NaCl 2/0.01 | MD = 5 m | [51] | ||||
| NH4HCO 2.4–2.6/ 0.01–0.075 | stripping + adsorption | 4.8–8.6 | no | [65] | ||
| NH4HCO 2.0/0.5 | vapour stripping + adsorption/ condensation | no | [43] | |||
| NaCl 3/0.05 | MED | 1000 cell pairs Fujifilm Type 10 25 × 100 cm 150 m 125 m | 1.9–4.3 | no | [53] | |
| NaCl 1–5 | MD | no | [66] | |||
| NaCl 5/0.05 | MED | 930 cell pairs Fujifilm 10 × 10 cm 120 m | no | [63] | ||
| NaCl 2–5/ 0.01–0.2 | MED | 50 cell pairs 10 × 10 (10 × 88) cm | 5.4 (2.9) | no | [67] | |
| KNO3 | (1) salt precipitation (2) water evaporation | (1) 43–93 cell pairs (2) 15–18 cell pairs Fumatech FAS-50/FKS-50 13 × 9 cm 155 m 50 m | (1) 0.2–1.0 (2) 3.2–6.5 | 1.33 | (1) 1.1 (2) 2.6 | [52] |
| NH4HCO3 0.05–2/0.01 | distillation column | 5 cell pairs 300 m 120 m | 0.84 | no | [68] | |
| various sol.limit/0.05 | (1) MED (2) thermolytic salt (NH4HCO3) | 10 cell pairs Fujifilm 10 × 10 cm 270 m 125 m | (1) 7.5 (2) 7.7 | no | [30] | |
4. Membranes and RED Stack Design
| Membrane | Preparation Technique | () | Area (cm2) | IEC () | Water Uptake (%) | FCD ( H2O) | Area Resistance ( cm2) | Feed Solution | () | Ref. | |
|---|---|---|---|---|---|---|---|---|---|---|---|
| Fuji CEMT1- PPyCS-0.05 | Surface polymerization on commercial membrane | 122 | 18 | 1.7 | 47.4 | 3.5 | 2.12 | NaCl 4 M/ 0.5 M | - | 1.5 | [88] |
| SPES-P SPES-D | Phase inversion | 83 63 | 207 | 1.15 1.19 | 67.2 28.0 | 1.7 4.3 | 1.4 1.9 | NaCl 4 M/ 0.1 M | <0.5 <0.8 | 3.64 3.92 | [92] |
| PCEM PAM | Pore filling of porous polyethylene by single impregnation in a roll-to-roll process | 16 17 | 19.6 | 1.80 1.81 | 49.5 39.3 | - | 0.42 0.40 | NaCl 0.5 M/ 0.017 M | 0.957 0.924 | 1.95 1.46 | [100] |
| PErC(5)QPS- QPPO | Chemical crosslinking of polyethylene support | 51 | 25 | 1.2 | 37 | - | 0.69 | NaCl 0.599 M/ 0.00856 M | - | 1.82 | [103] |
| UTFCS- 5/CMX | Spin coating on ceramic support | 45 | - | - | - | - | 1.2 | sea/ river water | 0.886 | 0.036 | [104] |
| AEM | Chloromethylation and quaternization of the grafted copolymer films | - | - | 1.1 2.9 | - | - | 0.6 | - | - | 0.8– 0.9 | [105] |
| PAEM- AA25 | Pore filling of polyethylene by photoinduced radical polymerization | 17 | 19.6 | 1.67 | 93.72 | - | 0.323 | NaCl 0.5 M/ 0.017 M | 0.955 | 1.50 | [99] |
| PPO-PVA PDDA-PVA | Solution casting and solvent evaporation | 50 55 | - | 1.58–1.91 0.97–1.50 | 46–93 100–148 | 1.74–4.2 1.0–1.2 | 1.30–1.54 0.71–1.32 | NaCl 0.5 M/ 0.017 M | 0.810–0.873 0.420-0.595 | 0.25–0.46 0.21–0.46 | [106] |
| PDDA-PVA | Solution casting and solvent evaporation | 55 | 36 | 1.0–1.54 | 171–179 | - | 0.76–1.34 | NaCl 0.5 M/ 0.017 M | 0.42–0.62 | 0.34–0.58 | [91] |
| CJMA- 2–7.5 | Layer-by-layer deposition of polyelectrolyte | 102.7 | 36 | - | - | - | 3.1 | NaCl 0.51 M/ 0.017 M | 0.91 | <0.39 | [87] |
| E2C1- DMA0.5 | Pore-filled polyethylene by the addition of electrolytes | 25 | 19.6 | 1.40 | - | 8 | 0.754 | NaCl 0.5 M/ 0.017 M | 0.938 | 1.524 | [107] |
| PAES-ABCO PAES-IMD PAES-TMA | Solution casting Solvent evaporation Quaternization | 64–70 59–64 58–70 | 34 | 1.2–1.48 1.19–1.48 1.17–1.45 | 11–17 8–13 15–30 | 10.55–12.62 13.31–16.40 6.68–9.06 | 1.59–3.82 1.65–3.86 1.45–3.53 | NaCl 0.5 M/ 0.017 M | 0.935–0.972 0.944–0.986 0.916–0.966 | 1.16 1.2 1.14 | [95] |
| sPVA (2–10%) | Hybrid membrane by solution casting and solvent evaporation | 50 | 36 | 1.6–2.05 | 45–75 | 2.0–4.5 | 1.3–2.1 | NaCl 0.5 M/ 0.017 M | 0.80–0.86 | 0.3–0.462 | [108] |
| SPPO-(0.1–0.8) O-MWCNT | Blending | 47–70 | 36 | 1.77–2.28 | 37.6–42.6 | 4.6–5.5 | 0.45–0.67 | NaCl 0.5 M/ 0.017 M | 0.899–0.953 | 0.37–0.48 | [109] |
| A-SPPO | Ion channel alignment by pulse electric field | 80-91 | 20 | 0.91-1.06 | - | - | 0.86 | NaCl 0.599 M/ 0.017 M | 0.962 | 1.34 | [110] |
| KIER | Pore filling | 26–27 | 19.6 | 1.42–2.6 | 21.7–26.9 | 6.5–9.8 | 0.28–0.72 | NaCl 0.58 M/ 0.017 M | 0.918–0.992 | <2.5 | [101] |
| sPPO-SiO2– SO3H | Solvent evaporation | 30 | - | 0.78–1.18 | 21–34 | 2.6–94.7 | 0.85–1.87 | NaCl 0.5 M/ 0.017 M | 0.791–0.865 | 1.3 | [96] |
| Fe2O3– SO4/sPPO | Two-step phase inversion | 30–150 | 36 | 0.98–1.42 | 16–58 | 2.0–6.4 | 0.82–2.26 | NaCl 0.5 M/ 0.017 M | 0.771–0.923 | 0.62–1.4 | [97] |
| Fe2O3– SO4/sPPO | Solution casting Solvent evaporation | 100 | 36 | 0.87-1.40 | 20–26 | 3.4–5.4 | 0.87–2.26 | NaCl 0.5M/ 0.017M | 0.686–0.877 | 1.30 | [98] |
| Flat Ridges Waves Pillars | Solution casting/ Solvent evaporation | 190 199 200 212 | 100 | - | - | - | 2.55 3.16 2.94 3.20 | NaCl 0.507 M/ 0.017 M | 0.905 0.896 0.895 0.901 | 1.10 1.10 1.25 1.30 | [111] |
| SPEEK PECH | Solution casting Solvent evaporation | 33–130 | 100 | 1.23–1.76 | 23–54 | 3.4–5.3 | 0.82–2.05 | NaCl 0.507 M/ 0.017 M | 0.891–0.953 | 1.07–1.28 | [93] |
| PECH | Solution casting/ amination reaction | 33–130 | 100 | 1.31–1.88 | 32.2–53.5 | 3.4–4.1 | 0.82–2.05 | NaCl 0.507 M/ 0.017 M | 0.792–0.903 | 0.90–1.27 | [94] |
5. Potential Salts for REDHE
- The solubility in water defines the maximum concentration difference achievable; therefore, the maximum driving force for energy generation. A salt with high solubility and a high temperature dependency of the solubility is favorable for use in a REDHE. For precipitation as the solution regeneration step, a high temperature dependency of the solubility is crucial for maximizing the power output [30,52]. NaCl has a moderate solubility at room temperature, and the temperature dependency of the solubility is low. This is sub-optimal for use in a REDHE.
- The dissolution enthalpy change of a salt plays a significant role in the heat requirement of solution regeneration via precipitation, and thus for the process efficiency. Salts can have a positive or negative enthalpy change of dissolution, increasing or decreasing the heat requirement, respectively [130,131].
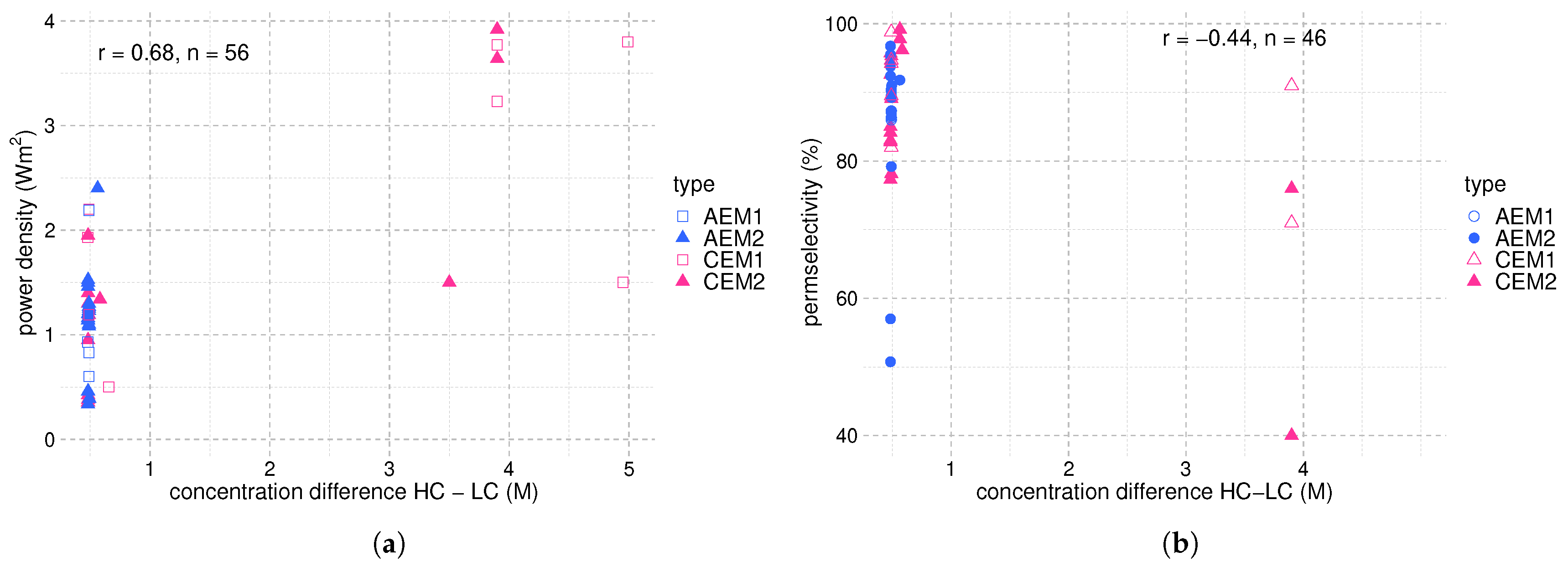
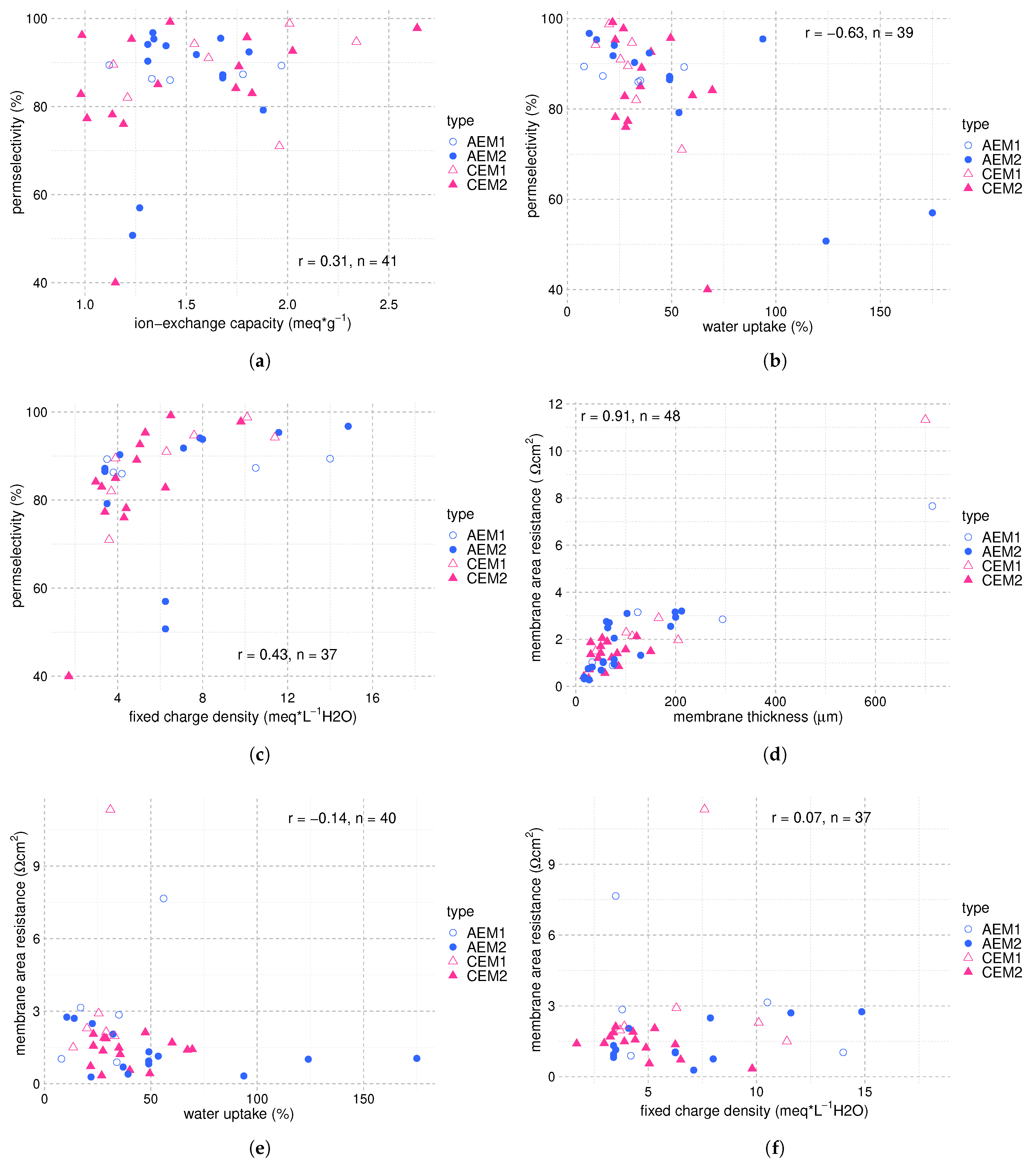
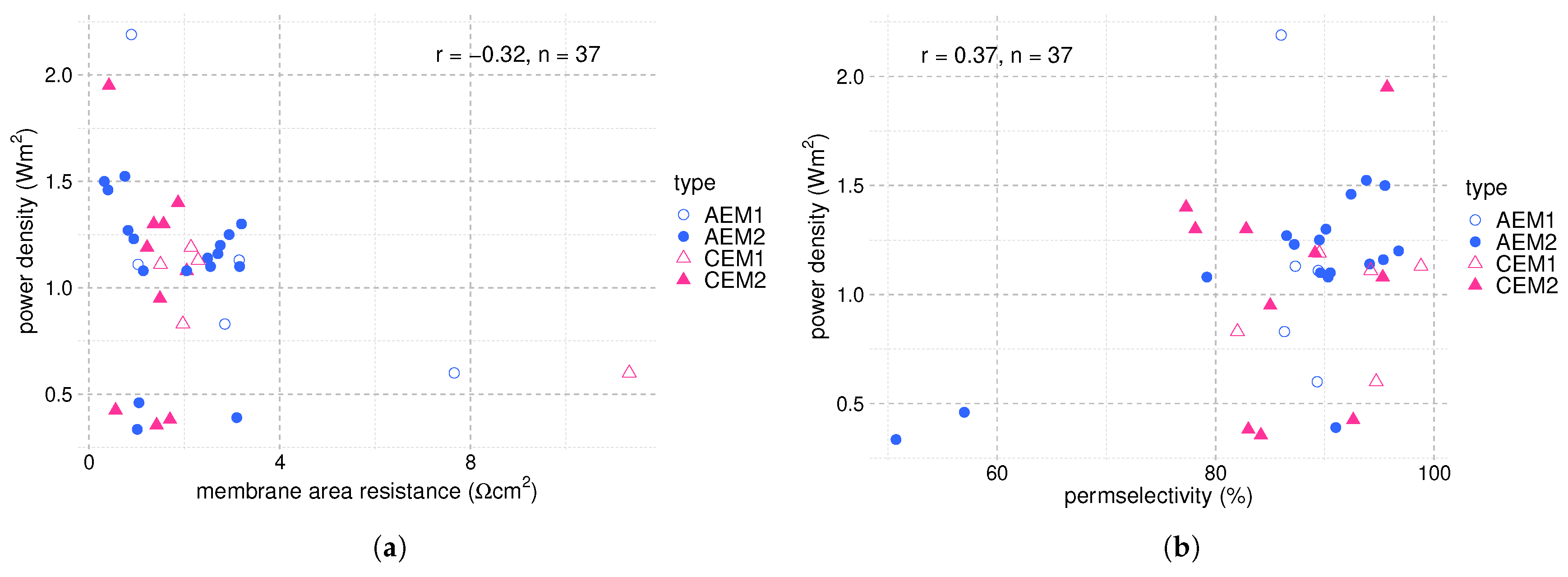
- The affinity and mobility of ions in the IEM determine their permselectivity; therefore, it influences the achievable power output. Affinity and mobility are functions of ion properties like hydrated radius and hydration energy.
6. Conclusions
- possibility for green hydrogen production,
- free choice of electrolyte due to closed-loop operation,
- free choice of solvent,
- mitigation of membrane fouling,
- no pre-treatment required,
- possible use of low-grade waste heat for solution regeneration.
Author Contributions
Funding
Conflicts of Interest
References
- Micale, G.; Cipollina, A.; Tamburini, A. Salinity gradient energy. In Sustainable Energy from Salinity Gradients; Elsevier: Cambridge, UK, 2016; pp. 1–17. [Google Scholar]
- Logan, B.E.; Elimelech, M. Membrane-based processes for sustainable power generation using water. Nature 2012, 488, 313–319. [Google Scholar] [CrossRef] [PubMed]
- Kingsbury, R.S.; Chu, K.; Coronell, O. Energy storage by reversible electrodialysis: The concentration battery. J. Membr. Sci. 2015, 495, 502–516. [Google Scholar] [CrossRef]
- Boon, N.; Van Roij, R. ‘Blue energy’from ion adsorption and electrode charging in sea and river water. Mol. Phys. 2011, 109, 1229–1241. [Google Scholar] [CrossRef] [Green Version]
- Isaacs, J.D.; Seymour, R.J. The ocean as a power resource. Int. J. Environ. Stud. 1973, 4, 201–205. [Google Scholar] [CrossRef]
- Wick, G.; Schmitt, W. Prospects for renewable energy from the sea. Mar. Technol. Soc. J. 1977, 11, 16–21. [Google Scholar]
- Post, J.W. Blue Energy: Electricity Production from Salinity Gradients by Reverse Electrodialysis; Wageningen University: Wageningen, The Netherlands, 2009. [Google Scholar]
- Enerdata. Global Energy Statistical Yearbook-2019 Edition; Enerdata: Grenoble, France, 2020. [Google Scholar]
- Pattle, R. Production of electric power by mixing fresh and salt water in the hydroelectric pile. Nature 1954, 174, 660. [Google Scholar] [CrossRef]
- Lacey, R. Energy by reverse electrodialysis. OcEng 1980, 7, 1–47. [Google Scholar] [CrossRef]
- Papapetrou, M. Reverse Electrodialysis Power Production-Progress in the development of an innovative system. In Proceedings of the 4th International Conference on Ocean Energy, Dublin, Ireland, 17 October 2012. [Google Scholar]
- Tedesco, M.; Cipollina, A.; Tamburini, A.; van Baak, W.; Micale, G. Modelling the Reverse ElectroDialysis process with seawater and concentrated brines. Desalin. Water Treat. 2012, 49, 404–424. [Google Scholar] [CrossRef] [Green Version]
- Loeb, S. Osmotic power plants. Science 1975, 189, 654. [Google Scholar] [CrossRef] [Green Version]
- Post, J.W.; Veerman, J.; Hamelers, H.V.; Euverink, G.J.; Metz, S.J.; Nymeijer, K.; Buisman, C.J. Salinity-gradient power: Evaluation of pressure-retarded osmosis and reverse electrodialysis. J. Membr. Sci. 2007, 288, 218–230. [Google Scholar] [CrossRef] [Green Version]
- Krakhella, K.W.; Morales, M.; Bock, R.; Seland, F.; Burheim, O.S.; Einarsrud, K.E. Electrodialytic Energy Storage System: Permselectivity, Stack Measurements and Life-Cycle Analysis. Energies 2020, 13, 1247. [Google Scholar] [CrossRef] [Green Version]
- Scialdone, O.; Guarisco, C.; Grispo, S.; D’Angelo, A.; Galia, A. Investigation of electrode material–Redox couple systems for reverse electrodialysis processes. Part I: Iron redox couples. J. Electroanal. Chem. 2012, 681, 66–75. [Google Scholar] [CrossRef]
- Tedesco, M.; Cipollina, A.; Tamburini, A.; Micale, G.; Helsen, J.; Papapetrou, M. REAPower: Use of desalination brine for power production through reverse electrodialysis. Desalin. Water Treat. 2015, 53, 3161–3169. [Google Scholar] [CrossRef] [Green Version]
- Tufa, R.A.; Curcio, E.; van Baak, W.; Veerman, J.; Grasman, S.; Fontananova, E.; Di Profio, G. Potential of brackish water and brine for energy generation by salinity gradient power-reverse electrodialysis (SGP-RE). RSC Adv. 2014, 4, 42617–42623. [Google Scholar] [CrossRef] [Green Version]
- Tedesco, M.; Brauns, E.; Cipollina, A.; Micale, G.; Modica, P.; Russo, G.; Helsen, J. Reverse electrodialysis with saline waters and concentrated brines: A laboratory investigation towards technology scale-up. J. Membr. Sci. 2015, 492, 9–20. [Google Scholar] [CrossRef] [Green Version]
- Tedesco, M.; Cipollina, A.; Tamburini, A.; Micale, G. Towards 1 kW power production in a reverse electrodialysis pilot plant with saline waters and concentrated brines. J. Membr. Sci. 2017, 522, 226–236. [Google Scholar] [CrossRef] [Green Version]
- Tedesco, M.; Scalici, C.; Vaccari, D.; Cipollina, A.; Tamburini, A.; Micale, G. Performance of the first reverse electrodialysis pilot plant for power production from saline waters and concentrated brines. J. Membr. Sci. 2016, 500, 33–45. [Google Scholar] [CrossRef] [Green Version]
- Jalili, Z.; Krakhella, K.W.; Einarsrud, K.E.; Burheim, O.S. Energy generation and storage by salinity gradient power: A model-based assessment. J. Energy Storage 2019, 24, 100755. [Google Scholar] [CrossRef]
- Tamburini, A.; Cipollina, A.; Papapetrou, M.; Piacentino, A.; Micale, G. Salinity gradient engines. In Sustainable Energy from Salinity Gradients; Elsevier: Cambridge, UK, 2016; pp. 219–256. [Google Scholar]
- Loeb, S. Method and Apparatus for Generating Power Utilizing Pressure-Retarded-Osmosis. U.S. Patent 3,906,250, 16 September 1975. [Google Scholar]
- Loeb, S. Method and Apparatus for Generating Power Utilizing Reverse Electrodialysis. U.S. Patent 4,171,409, 16 October 1979. [Google Scholar]
- Bijmans, M.; Burheim, O.; Bryjak, M.; Delgado, A.; Hack, P.; Mantegazza, F.; Tenisson, S.; Hamelers, H. CAPMIX-Deploying Capacitors for Salt Gradient Power Extraction. Energy Procedia 2012, 20, 108–115. [Google Scholar] [CrossRef] [Green Version]
- Achilli, A.; Childress, A.E. Pressure retarded osmosis: From the vision of Sidney Loeb to the first prototype installation. Desalination 2010, 261, 205–211. [Google Scholar] [CrossRef]
- Rattner, A.S.; Garimella, S. Energy harvesting, reuse and upgrade to reduce primary energy usage in the USA. Energy 2011, 36, 6172–6183. [Google Scholar] [CrossRef]
- Horenburg, P. Transforming Waste Heat into Electricity. Steam Expansion Engine Makes Efficient and Flexible Use of Low-Temperature Heat with ORC Technology; FIZ Karlsruhe: Eggenstein-Leopoldshafen, Germany, 2011. [Google Scholar]
- Tamburini, A.; Tedesco, M.; Cipollina, A.; Micale, G.; Ciofalo, M.; Papapetrou, M.; Van Baak, W.; Piacentino, A. Reverse electrodialysis heat engine for sustainable power production. Appl. Energy 2017, 206, 1334–1353. [Google Scholar] [CrossRef]
- Haywood, R.W. Analysis of Engineering Cycles: Power, Refrigerating and Gas Liquefaction Plant; Elsevier Science: Amsterdam, The Netherlands, 2012. [Google Scholar]
- Ni, M.; Leung, M.K.; Leung, D.Y. Energy and exergy analysis of hydrogen production vby a proton exchange membrane (PEM) electrolyzer plant. Energy Convers. Manag. 2008, 49, 2748–2756. [Google Scholar] [CrossRef]
- Kumar, S.S.; Himabindu, V. Hydrogen production by PEM water electrolysis—A review. Mater. Sci. Energy Technol. 2019, 2, 442–454. [Google Scholar]
- Lamb, J.J.; Burheim, O.S.; Pollet, B.G. Hydrogen Fuel Cells and Water Electrolysers. In Micro-Optics and Energy; Springer: Cham, Switzerland, 2020; pp. 61–71. [Google Scholar]
- Lamb, J.J.; Hillestad, M.; Rytter, E.; Bock, R.; Nordgård, A.S.; Lien, K.M.; Burheim, O.S.; Pollet, B.G. Traditional routes for hydrogen production and carbon conversion. In Hydrogen, Biomass and Bioenergy: Integration Pathways for Renewable Energy Applications; Elsevier Science: London, UK, 2020; p. 21. [Google Scholar]
- Lamb, J.J.; Pollet, B.G.; Burheim, O.S. Energy-smart buildings design: Construction and monitoring of buildings for improved energy efficiency. Energy Storage 2020. [Google Scholar] [CrossRef]
- Hatzell, M.C.; Ivanov, I.; Cusick, R.D.; Zhu, X.; Logan, B.E. Comparison of hydrogen production and electrical power generation for energy capture in closed-loop ammonium bicarbonate reverse electrodialysis systems. Phys. Chem. Chem. Phys. 2014, 16, 1632–1638. [Google Scholar] [CrossRef] [PubMed]
- Rytter, E.; Hillestad, M.; Austbø, B. Thermochemical production of fuels. In Hydrogen, Biomass and Bioenergy: Integration Pathways for Renewable Energy Applications; Elsevier Science: London, UK, 2020; p. 89. [Google Scholar]
- Borgschulte, A. The hydrogen grand challenge. Front. Energy Res. 2016, 4, 11. [Google Scholar] [CrossRef] [Green Version]
- Boyano, A.; Blanco-Marigorta, A.; Morosuk, T.; Tsatsaronis, G. Exergoenvironmental analysis of a steam methane reforming process for hydrogen production. Energy 2011, 36, 2202–2214. [Google Scholar] [CrossRef]
- Rand, D.A. A journey on the electrochemical road to sustainability. J. Solid State Electrochem. 2011, 15, 1579–1622. [Google Scholar] [CrossRef]
- Acar, C.; Dincer, I. Comparative assessment of hydrogen production methods from renewable and non-renewable sources. Int. J. Hydrogen Energy 2014, 39, 1–12. [Google Scholar] [CrossRef]
- Vassallo, F.; Giacalone, F.; Scargiali, F.; Tamburini, A.; Cipollina, A.; Micale, G. Regeneration units for thermolytic salts applications in water & power production: State of the art, experimental and modelling assessment. Desalination 2021, 504, 114965. [Google Scholar]
- Giacalone, F.; Olkis, C.; Santori, G.; Cipollina, A.; Brandani, S.; Micale, G. Novel solutions for closed-loop reverse electrodialysis: Thermodynamic characterisation and perspective analysis. Energy 2019, 166, 674–689. [Google Scholar] [CrossRef]
- Burheim, O.S. Engineering Energy Storage; Elsevier Science: London, UK, 2017. [Google Scholar]
- Davydov, D.; Nosova, E.; Loza, S.; Achoh, A.; Korzhov, A.; Sharafan, M.; Melnikov, S. Use of the Microheterogeneous Model to Assess the Applicability of Ion-Exchange Membranes in the Process of Generating Electricity from a Concentration Gradient. Membranes 2021, 11, 406. [Google Scholar] [CrossRef] [PubMed]
- Kristiansen, K.R.; Barragán, V.M.; Kjelstrup, S. Thermoelectric Power of Ion Exchange Membrane Cells Relevant to Reverse Electrodialysis Plants. Phys. Rev. Appl. 2019, 11. [Google Scholar] [CrossRef] [Green Version]
- Ramon, G.Z.; Feinberg, B.J.; Hoek, E.M. Membrane-based production of salinity-gradient power. Energy Environ. Sci. 2011, 4, 4423–4434. [Google Scholar] [CrossRef]
- Rumble, J.R. (Ed.) CRC Handbook of Chemistry and Physics, 102nd Edition (Internet Version 2021), 102nd ed.; CRC Press: Boca Raton, FL, USA, 2021. [Google Scholar]
- Raka, Y.D.; Karoliussen, H.; Lien, K.M.; Burheim, O.S. Opportunities and challenges for thermally driven hydrogen production using reverse electrodialysis system. Int. J. Hydrogen Energy 2020, 45, 1212–1225. [Google Scholar] [CrossRef]
- Micari, M.; Cipollina, A.; Giacalone, F.; Kosmadakis, G.; Papapetrou, M.; Zaragoza, G.; Micale, G.; Tamburini, A. Towards the first proof of the concept of a Reverse ElectroDialysis-Membrane Distillation Heat Engine. Desalination 2019, 453, 77–88. [Google Scholar] [CrossRef]
- Krakhella, K.W.; Bock, R.; Burheim, O.S.; Seland, F.; Einarsrud, K.E. Heat to H2: Using waste heat for hydrogen production through reverse electrodialysis. Energies 2019, 12, 3428. [Google Scholar] [CrossRef] [Green Version]
- Ortega-Delgado, B.; Giacalone, F.; Catrini, P.; Cipollina, A.; Piacentino, A.; Tamburini, A.; Micale, G. Reverse electrodialysis heat engine with multi-effect distillation: Exergy analysis and perspectives. Energy Convers. Manag. 2019, 194, 140–159. [Google Scholar] [CrossRef]
- Zhu, X.; He, W.; Logan, B.E. Influence of solution concentration and salt types on the performance of reverse electrodialysis cells. J. Membr. Sci. 2015, 494, 154–160. [Google Scholar] [CrossRef] [Green Version]
- Bevacqua, M.; Carubia, A.; Cipollina, A.; Tamburini, A.; Tedesco, M.; Micale, G. Performance of a RED system with ammonium hydrogen carbonate solutions. Desalin. Water Treat. 2016, 57, 23007–23018. [Google Scholar] [CrossRef] [Green Version]
- Kim, D.H.; Park, B.H.; Kwon, K.; Li, L.; Kim, D. Modeling of power generation with thermolytic reverse electrodialysis for low-grade waste heat recovery. Appl. Energy 2017, 189, 201–210. [Google Scholar] [CrossRef]
- Luo, X.; Cao, X.; Mo, Y.; Xiao, K.; Zhang, X.; Liang, P.; Huang, X. Power generation by coupling reverse electrodialysis and ammonium bicarbonate: Implication for recovery of waste heat. Electrochem. Commun. 2012, 19, 25–28. [Google Scholar] [CrossRef]
- Mercer, S.M.; Jessop, P.G. “Switchable water”: Aqueous solutions of switchable ionic strength. Chemsuschem Chem. Sustain. Energy Mater. 2010, 3, 467–470. [Google Scholar] [CrossRef]
- Han, G.; Ge, Q.; Chung, T.S. Conceptual demonstration of novel closed-loop pressure retarded osmosis process for sustainable osmotic energy generation. Appl. Energy 2014, 132, 383–393. [Google Scholar] [CrossRef]
- McCutcheon, J.R.; McGinnis, R.L.; Elimelech, M. Desalination by ammonia—Carbon dioxide forward osmosis: Influence of draw and feed solution concentrations on process performance. J. Membr. Sci. 2006, 278, 114–123. [Google Scholar] [CrossRef]
- McGinnis, R.L.; Elimelech, M. Global Challenges in Energy and Water Supply: The Promise of Engineered Osmosis. Environ. Sci. Technol. 2008, 42, 8625–8629. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Elimelech, M.; Phillip, W.A. The future of seawater desalination: Energy, technology, and the environment. Science 2011, 333, 712–717. [Google Scholar] [CrossRef]
- Hu, J.; Xu, S.; Wu, X.; Wu, D.; Jin, D.; Wang, P.; Leng, Q. Theoretical simulation and evaluation for the performance of the hybrid multi-effect distillation—Reverse electrodialysis power generation system. Desalination 2018, 443, 172–183. [Google Scholar] [CrossRef]
- Hu, J.; Xu, S.; Wu, X.; Wu, D.; Jin, D.; Leng, Q. Multi-stage reverse electrodialysis: Strategies to harvest salinity gradient energy. Energy Convers. Manag. 2019, 183, 803–815. [Google Scholar] [CrossRef]
- Bevacqua, M.; Tamburini, A.; Papapetrou, M.; Cipollina, A.; Micale, G.; Piacentino, A. Reverse electrodialysis with NH4HCO3-water systems for heat-to-power conversion. Energy 2017, 137, 1293–1307. [Google Scholar] [CrossRef]
- Long, R.; Li, B.; Liu, Z.; Liu, W. Hybrid membrane distillation-reverse electrodialysis electricity generation system to harvest low-grade thermal energy. J. Membr. Sci. 2017, 525, 107–115. [Google Scholar] [CrossRef]
- Palenzuela, P.; Micari, M.; Ortega-Delgado, B.; Giacalone, F.; Zaragoza, G.; Alarcón-Padilla, D.C.; Cipollina, A.; Tamburini, A.; Micale, G. Performance analysis of a RED-MED salinity gradient heat engine. Energies 2018, 11, 3385. [Google Scholar] [CrossRef] [Green Version]
- Kim, D.H.; Park, B.H.; Kwon, K.; Li, L.; Kim, D. Power Generation with Thermolytic Reverse Electrodialysis for Low-Grade Waste Heat Recovery. Organic Rankine Cycle Technology for Heat Recovery; IntechOpen: London, UK, 2018; p. 167. [Google Scholar]
- Strathmann, H. Electrodialysis, a mature technology with a multitude of new applications. Desalination 2010, 264, 268–288. [Google Scholar] [CrossRef]
- Geise, G.M.; Hickner, M.A.; Logan, B.E. Ionic resistance and permselectivity tradeoffs in anion exchange membranes. Acs Appl. Mater. Interfaces 2013, 5, 10294–10301. [Google Scholar] [CrossRef]
- Khan, M.I.; Zheng, C.; Mondal, A.N.; Hossain, M.M.; Wu, B.; Emmanuel, K.; Wu, L.; Xu, T. Preparation of anion exchange membranes from BPPO and dimethylethanolamine for electrodialysis. Desalination 2017, 402, 10–18. [Google Scholar] [CrossRef] [Green Version]
- Geise, G.M.; Lee, H.S.; Miller, D.J.; Freeman, B.D.; McGrath, J.E.; Paul, D.R. Water purification by membranes: The role of polymer science. J. Polym. Sci. Part B Polym. Phys. 2010, 48, 1685–1718. [Google Scholar] [CrossRef]
- Wei, B.; Pan, J.; Feng, J.; Chen, C.; Liao, S.; Yu, Y.; Li, X. Highly conductive and permselective anion exchange membranes for electrodialysis desalination with series-connected dications appending flexible hydrophobic tails. Desalination 2020, 474, 114184. [Google Scholar] [CrossRef]
- Mei, Y.; Tang, C.Y. Recent developments and future perspectives of reverse electrodialysis technology: A review. Desalination 2018, 425, 156–174. [Google Scholar] [CrossRef]
- Avci, A.H.; Tufa, R.A.; Fontananova, E.; Di Profio, G.; Curcio, E. Reverse Electrodialysis for energy production from natural river water and seawater. Energy 2018, 165, 512–521. [Google Scholar] [CrossRef]
- Avci, A.H.; Sarkar, P.; Tufa, R.A.; Messana, D.; Argurio, P.; Fontananova, E.; Di Profio, G.; Curcio, E. Effect of Mg2+ ions on energy generation by Reverse Electrodialysis. J. Membr. Sci. 2016, 520, 499–506. [Google Scholar] [CrossRef]
- Ortiz-Imedio, R.; Gomez-Coma, L.; Fallanza, M.; Ortiz, A.; Ibañez, R.; Ortiz, I. Comparative performance of Salinity Gradient Power-Reverse Electrodialysis under different operating conditions. Desalination 2019, 457, 8–21. [Google Scholar] [CrossRef]
- Mehdizadeh, S.; Yasukawa, M.; Kuno, M.; Kawabata, Y.; Higa, M. Evaluation of energy harvesting from discharged solutions in a salt production plant by reverse electrodialysis (RED). Desalination 2019, 467, 95–102. [Google Scholar] [CrossRef]
- Veerman, J.; Saakes, M.; Metz, S.; Harmsen, G. Reverse electrodialysis: Performance of a stack with 50 cells on the mixing of sea and river water. J. Membr. Sci. 2009, 327, 136–144. [Google Scholar] [CrossRef] [Green Version]
- Daniilidis, A.; Vermaas, D.A.; Herber, R.; Nijmeijer, K. Experimentally obtainable energy from mixing river water, seawater or brines with reverse electrodialysis. Renew. Energy 2014, 64, 123–131. [Google Scholar] [CrossRef]
- Vermaas, D.A.; Saakes, M.; Nijmeijer, K. Doubled power density from salinity gradients at reduced intermembrane distance. Environ. Sci. Technol. 2011, 45, 7089–7095. [Google Scholar] [CrossRef] [PubMed]
- Oh, Y.; Jeong, Y.; Han, S.J.; Kim, C.S.; Kim, H.; Han, J.H.; Hwang, K.S.; Jeong, N.; Park, J.S.; Chae, S. Effects of divalent cations on electrical membrane resistance in reverse electrodialysis for salinity power generation. Ind. Eng. Chem. Res. 2018, 57, 15803–15810. [Google Scholar] [CrossRef]
- Besha, A.T.; Tsehaye, M.T.; Aili, D.; Zhang, W.; Tufa, R.A. Design of Monovalent Ion Selective Membranes for Reducing the Impacts of Multivalent Ions in Reverse Electrodialysis. Membranes 2020, 10, 7. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Galama, A.; Daubaras, G.; Burheim, O.; Rijnaarts, H.; Post, J. Seawater electrodialysis with preferential removal of divalent ions. J. Membr. Sci. 2014, 452, 219–228. [Google Scholar] [CrossRef]
- Fontananova, E.; Messana, D.; Tufa, R.; Nicotera, I.; Kosma, V.; Curcio, E.; Van Baak, W.; Drioli, E.; Di Profio, G. Effect of solution concentration and composition on the electrochemical properties of ion exchange membranes for energy conversion. J. Power Sources 2017, 340, 282–293. [Google Scholar] [CrossRef]
- Güler, E.; van Baak, W.; Saakes, M.; Nijmeijer, K. Monovalent-ion-selective membranes for reverse electrodialysis. J. Membr. Sci. 2014, 455, 254–270. [Google Scholar] [CrossRef]
- Gao, H.; Zhang, B.; Tong, X.; Chen, Y. Monovalent-anion selective and antifouling polyelectrolytes multilayer anion exchange membrane for reverse electrodialysis. J. Membr. Sci. 2018, 567, 68–75. [Google Scholar] [CrossRef]
- Tufa, R.A.; Piallat, T.; Hnat, J.; Fontananova, E.; Paidar, M.; Chanda, D.; Curcio, E.; di Profio, G.; Bouzek, K. Salinity gradient power reverse electrodialysis: Cation exchange membrane design based on polypyrrole-chitosan composites for enhanced monovalent selectivity. Chem. Eng. J. 2020, 380, 122461. [Google Scholar] [CrossRef]
- Rijnaarts, T.; Huerta, E.; van Baak, W.; Nijmeijer, K. Effect of divalent cations on RED performance and cation exchange membrane selection to enhance power densities. Environ. Sci. Technol. 2017, 51, 13028–13035. [Google Scholar] [CrossRef]
- Hong, J.G.; Zhang, B.; Glabman, S.; Uzal, N.; Dou, X.; Zhang, H.; Wei, X.; Chen, Y. Potential ion exchange membranes and system performance in reverse electrodialysis for power generation: A review. J. Membr. Sci. 2015, 486, 71–88. [Google Scholar] [CrossRef]
- Hong, J.G.; Park, T.W. Electrochemical characterizations and reverse electrodialysis performance of hybrid anion exchange membranes for salinity gradient energy. J. Electroanal. Chem. 2018, 817, 134–140. [Google Scholar] [CrossRef]
- Avci, A.H.; Rijnaarts, T.; Fontananova, E.; Di Profio, G.; Vankelecom, I.F.; De Vos, W.M.; Curcio, E. Sulfonated polyethersulfone based cation exchange membranes for reverse electrodialysis under high salinity gradients. J. Membr. Sci. 2020, 595, 117585. [Google Scholar] [CrossRef]
- Güler, E.; Elizen, R.; Vermaas, D.A.; Saakes, M.; Nijmeijer, K. Performance-determining membrane properties in reverse electrodialysis. J. Membr. Sci. 2013, 446, 266–276. [Google Scholar] [CrossRef]
- Güler, E.; Zhang, Y.; Saakes, M.; Nijmeijer, K. Tailor-made anion-exchange membranes for salinity gradient power generation using reverse electrodialysis. ChemSusChem 2012, 5, 2262–2270. [Google Scholar] [CrossRef] [PubMed]
- Cho, D.H.; Lee, K.H.; Kim, Y.M.; Park, S.H.; Lee, W.H.; Lee, S.M.; Lee, Y.M. Effect of cationic groups in poly (arylene ether sulfone) membranes on reverse electrodialysis performance. Chem. Commun. 2017, 53, 2323–2326. [Google Scholar] [CrossRef] [PubMed]
- Hong, J.G.; Glabman, S.; Chen, Y. Effect of inorganic filler size on electrochemical performance of nanocomposite cation exchange membranes for salinity gradient power generation. J. Membr. Sci. 2015, 482, 33–41. [Google Scholar] [CrossRef]
- Hong, J.G.; Chen, Y. Evaluation of electrochemical properties and reverse electrodialysis performance for porous cation exchange membranes with sulfate-functionalized iron oxide. J. Membr. Sci. 2015, 473, 210–217. [Google Scholar] [CrossRef]
- Hong, J.G.; Chen, Y. Nanocomposite reverse electrodialysis (RED) ion-exchange membranes for salinity gradient power generation. J. Membr. Sci. 2014, 460, 139–147. [Google Scholar] [CrossRef]
- Yang, S.; Kim, W.S.; Choi, J.; Choi, Y.W.; Jeong, N.; Kim, H.; Nam, J.Y.; Jeong, H.; Kim, Y.H. Fabrication of photocured anion-exchange membranes using water-soluble siloxane resins as cross-linking agents and their application in reverse electrodialysis. J. Membr. Sci. 2019, 573, 544–553. [Google Scholar] [CrossRef]
- Yang, S.; Choi, Y.W.; Choi, J.; Jeong, N.; Kim, H.; Nam, J.Y.; Jeong, H. R2R Fabrication of Pore-Filling Cation-Exchange Membranes via One-Time Impregnation and Their Application in Reverse Electrodialysis. ACS Sustain. Chem. Eng. 2019, 7, 12200–12213. [Google Scholar] [CrossRef]
- Kim, H.K.; Lee, M.S.; Lee, S.Y.; Choi, Y.W.; Jeong, N.J.; Kim, C.S. High power density of reverse electrodialysis with pore-filling ion exchange membranes and a high-open-area spacer. J. Mater. Chem. A 2015, 3, 16302–16306. [Google Scholar] [CrossRef] [Green Version]
- Luo, T.; Abdu, S.; Wessling, M. Selectivity of ion exchange membranes: A review. J. Membr. Sci. 2018, 555, 429–454. [Google Scholar] [CrossRef]
- Lee, Y.J.; Cha, M.S.; Oh, S.G.; So, S.; Kim, T.H.; Ryoo, W.S.; Hong, Y.T.; Lee, J.Y. Reinforced anion exchange membrane based on thermal cross-linking method with outstanding cell performance for reverse electrodialysis. RSC Adv. 2019, 9, 27500–27509. [Google Scholar] [CrossRef] [Green Version]
- Jung, D.H.; Han, E.D.; Kim, B.H.; Seo, Y.H. Ultra-thin ion exchange film on the ceramic supporter for output power improvement of reverse electrodialysis. Sci. Rep. 2019, 9, 17440. [Google Scholar] [CrossRef]
- Golubenko, D.V.; Van der Bruggen, B.; Yaroslavtsev, A.B. Novel anion exchange membrane with low ionic resistance based on chloromethylated/quaternized-grafted polystyrene for energy efficient electromembrane processes. J. Appl. Polym. Sci. 2020, 137, 48656. [Google Scholar] [CrossRef]
- Hong, J.G.; Park, T.W.; Dhadake, Y. Property evaluation of custom-made ion exchange membranes for electrochemical performance in reverse electrodialysis application. J. Electroanal. Chem. 2019, 850, 113437. [Google Scholar] [CrossRef]
- Choi, J.; Yang, S.; Jeong, N.J.; Kim, H.; Kim, W.S. Fabrication of an anion-exchange membrane by pore-filling using catechol–1, 4-diazabicyclo-[2, 2, 2] octane coating and its application to reverse electrodialysis. Langmuir 2018, 34, 10837–10846. [Google Scholar] [CrossRef]
- Zhang, H.; Jiang, D.; Zhang, B.; Hong, J.G.; Chen, Y. A novel hybrid poly (vinyl alcohol)(PVA)/poly (2, 6-dimethyl-1, 4-phenylene oxide)(PPO) membranes for reverse electrodialysis power system. Electrochim. Acta 2017, 239, 65–73. [Google Scholar] [CrossRef] [Green Version]
- Tong, X.; Zhang, B.; Chen, Y. Fouling resistant nanocomposite cation exchange membrane with enhanced power generation for reverse electrodialysis. J. Membr. Sci. 2016, 516, 162–171. [Google Scholar] [CrossRef] [Green Version]
- Lee, J.Y.; Kim, J.H.; Lee, J.H.; Kim, S.; Moon, S.H. Morphologically aligned cation-exchange membranes by a pulsed electric field for reverse electrodialysis. Environ. Sci. Technol. 2015, 49, 8872–8877. [Google Scholar] [CrossRef]
- Güler, E.; Elizen, R.; Saakes, M.; Nijmeijer, K. Micro-structured membranes for electricity generation by reverse electrodialysis. J. Membr. Sci. 2014, 458, 136–148. [Google Scholar] [CrossRef]
- Kingsbury, R.; Zhu, S.; Flotron, S.; Coronell, O. Microstructure determines water and salt permeation in commercial ion-exchange membranes. ACS Appl. Mater. Interfaces 2018, 10, 39745–39756. [Google Scholar] [CrossRef]
- Pearson, K. VII. Note on regression and inheritance in the case of two parents. Proc. R. Soc. Lond. 1895, 58, 240–242. [Google Scholar]
- Taraldsen, G. Confidence in Correlation. Tech. Rep. 2020. [Google Scholar] [CrossRef]
- Jin, D.; Xi, R.; Xu, S.; Wang, P.; Wu, X. Numerical simulation of salinity gradient power generation using reverse electrodialysis. Desalination 2021, 512, 115132. [Google Scholar] [CrossRef]
- Dlugolkecki, P.; Ogonowski, P.; Metz, S.J.; Saakes, M.; Nijmeijer, K.; Wessling, M. On the resistances of membrane, diffusion boundary layer and double layer in ion exchange membrane transport. J. Membr. Sci. 2010, 349, 369–379. [Google Scholar] [CrossRef]
- Kim, H.; Choi, J.; Jeong, N.; Jung, Y.G.; Kim, H.; Kim, D.; Yang, S. Correlations between properties of pore-filling ion exchange membranes and performance of a reverse electrodialysis stack for high power density. Membranes 2021, 11, 609. [Google Scholar] [CrossRef] [PubMed]
- Długołeçki, P.; Gambier, A.; Nijmeijer, K.; Wessling, M. Practical potential of reverse electrodialysis as process for sustainable energy generation. Environ. Sci. Technol. 2009, 43, 6888–6894. [Google Scholar] [CrossRef] [PubMed]
- Pawlowski, S.; Sistat, P.; Crespo, J.G.; Velizarov, S. Mass transfer in reverse electrodialysis: Flow entrance effects and diffusion boundary layer thickness. J. Membr. Sci. 2014, 471, 72–83. [Google Scholar] [CrossRef]
- Post, J.W.; Hamelers, H.V.; Buisman, C.J. Energy recovery from controlled mixing salt and fresh water with a reverse electrodialysis system. Environ. Sci. Technol. 2008, 42, 5785–5790. [Google Scholar] [CrossRef]
- La Cerva, M.; Di Liberto, M.; Gurreri, L.; Tamburini, A.; Cipollina, A.; Micale, G.; Ciofalo, M. Coupling CFD with a one-dimensional model to predict the performance of reverse electrodialysis stacks. J. Membr. Sci. 2017, 541, 595–610. [Google Scholar] [CrossRef] [Green Version]
- Vermaas, D.A.; Saakes, M.; Nijmeijer, K. Power generation using profiled membranes in reverse electrodialysis. J. Membr. Sci. 2011, 385, 234–242. [Google Scholar] [CrossRef]
- Tsai, T.C.; Liu, C.W.; Yang, R.J. Power generation by reverse electrodialysis in a microfluidic device with a nafion ion-selective membrane. Micromachines 2016, 7, 205. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Kingsbury, R.; Coronell, O. Modeling and validation of concentration dependence of ion exchange membrane permselectivity: Significance of convection and Manning’s counter-ion condensation theory. J. Membr. Sci. 2021, 620, 118411. [Google Scholar] [CrossRef]
- Tedesco, M.; Hamelers, H.; Biesheuvel, P. Nernst-Planck transport theory for (reverse) electrodialysis: III. Optimal membrane thickness for enhanced process performance. J. Membr. Sci. 2018, 565, 480–487. [Google Scholar] [CrossRef] [Green Version]
- Chanda, S.; Tsai, P.A. Renewable Power Generation by Reverse Electrodialysis Using an Ion Exchange Membrane. Membranes 2021, 11, 830. [Google Scholar] [CrossRef]
- Chanda, S.; Tsai, P.A. Numerical simulation of renewable power generation using reverse electrodialysis. Energy 2019, 176, 531–543. [Google Scholar] [CrossRef]
- Fontananova, E.; Zhang, W.; Nicotera, I.; Simari, C.; van Baak, W.; Di Profio, G.; Curcio, E.; Drioli, E. Probing membrane and interface properties in concentrated electrolyte solutions. J. Membr. Sci. 2014, 459, 177–189. [Google Scholar] [CrossRef]
- Raka, Y.D.; Bock, R.; Karoliussen, H.; Wilhelmsen, Ø.; Stokke Burheim, O. The influence of concentration and temperature on the membrane resistance of ion exchange membranes and the levelised cost of hydrogen from reverse electrodialysis with ammonium bicarbonate. Membranes 2021, 11, 135. [Google Scholar] [CrossRef] [PubMed]
- Criss, C.M.; Millero, F.J. Modeling the Heat Capacities of Aqueous 1-1 Electrolyte Solutions with Pitzer’s Equations. J. Phys. Chem. 1996, 100, 1288–1294. [Google Scholar] [CrossRef]
- Hubert, N.; Solimando, R.; Pere, A.; Schuffenecker, L. Dissolution enthalpy of NaC1 in water at 25 °C, 45 °C and 60 °C. Determination of the Pitzer’s parameters of the HzO-NaC1 system and the molar dissolution enthalpy at infinite dilution of NaC1 in water between 25 °C and 100 °C. Thermochim. Acta 1997, 294, 157–163. [Google Scholar] [CrossRef]
- Rumble, J.R. Aqueous solubility of inorganic compounds at various tempratures. In CRC Handbook of Chemistry and Physics, 101st ed.; CRC Press/Taylor & Francis: Boca Raton, FL, USA, 2020. [Google Scholar]
- Yllö, A.; Zhang, C. Experimental and molecular dynamics study of the ionic conductivity in aqueous LiCl electrolytes. Chem. Phys. Lett. 2019, 729, 6–10. [Google Scholar] [CrossRef]
- Wu, X.; Gong, Y.; Xu, S.; Yan, Z.; Zhang, X.; Yang, S. Electrical Conductivity of Lithium Chloride, Lithium Bromide, and Lithium Iodide Electrolytes in Methanol, Water, and Their Binary Mixtures. J. Chem. Eng. Data 2019, 64, 4319–4329. [Google Scholar] [CrossRef]
- Tansel, B. Significance of thermodynamic and physical characteristics on permeation of ions during membrane separation: Hydrated radius, hydration free energy and viscous effects. Sep. Purif. Technol. 2012, 86, 119–126. [Google Scholar] [CrossRef]
- Marcus, Y. Thermodynamics of solvation of ions. Part 6—The standard partial molar volumes of aqueous ions at 298.15 K. J. Chem. Soc. Faraday Trans. 1993, 89, 713–718. [Google Scholar] [CrossRef]
- Strathmann, H. Ion-Exchange Membrane Separation Processes; Elsevier: Heidelberg, Germany, 2004. [Google Scholar]
- Geise, G.M.; Cassady, H.J.; Paul, D.R.; Logan, B.E.; Hickner, M.A. Specific ion effects on membrane potential and the permselectivity of ion exchange membranes. Phys. Chem. Chem. Phys. 2014, 16, 21673–21681. [Google Scholar] [CrossRef] [PubMed]
- Epsztein, R.; Shaulsky, E.; Qin, M.; Elimelech, M. Activation behavior for ion permeation in ion-exchange membranes: Role of ion dehydration in selective transport. J. Membr. Sci. 2019, 580, 316–326. [Google Scholar] [CrossRef]
- Yang, L.; Tang, C.; Ahmad, M.; Yaroshchuk, A.; Bruening, M.L. High selectivities among monovalent cations in dialysis through cation-exchange membranes coated with polyelectrolyte multilayers. ACS Appl. Mater. Interfaces 2018, 10, 44134–44143. [Google Scholar] [CrossRef]
- Sata, T.; Yamaguchi, T.; Kawamura, K.; Matsusaki, K. Transport numbers of various anions relative to chloride ions in modified anion-exchange membranes during electrodialysis. J. Chem. Soc. Faraday Trans. 1997, 93, 457–462. [Google Scholar] [CrossRef]
- Xu, T.; Liu, Z.; Yang, W. Fundamental studies of a new series of anion exchange membranes: Membrane prepared from poly (2, 6-dimethyl-1, 4-phenylene oxide)(PPO) and triethylamine. J. Membr. Sci. 2005, 249, 183–191. [Google Scholar] [CrossRef]

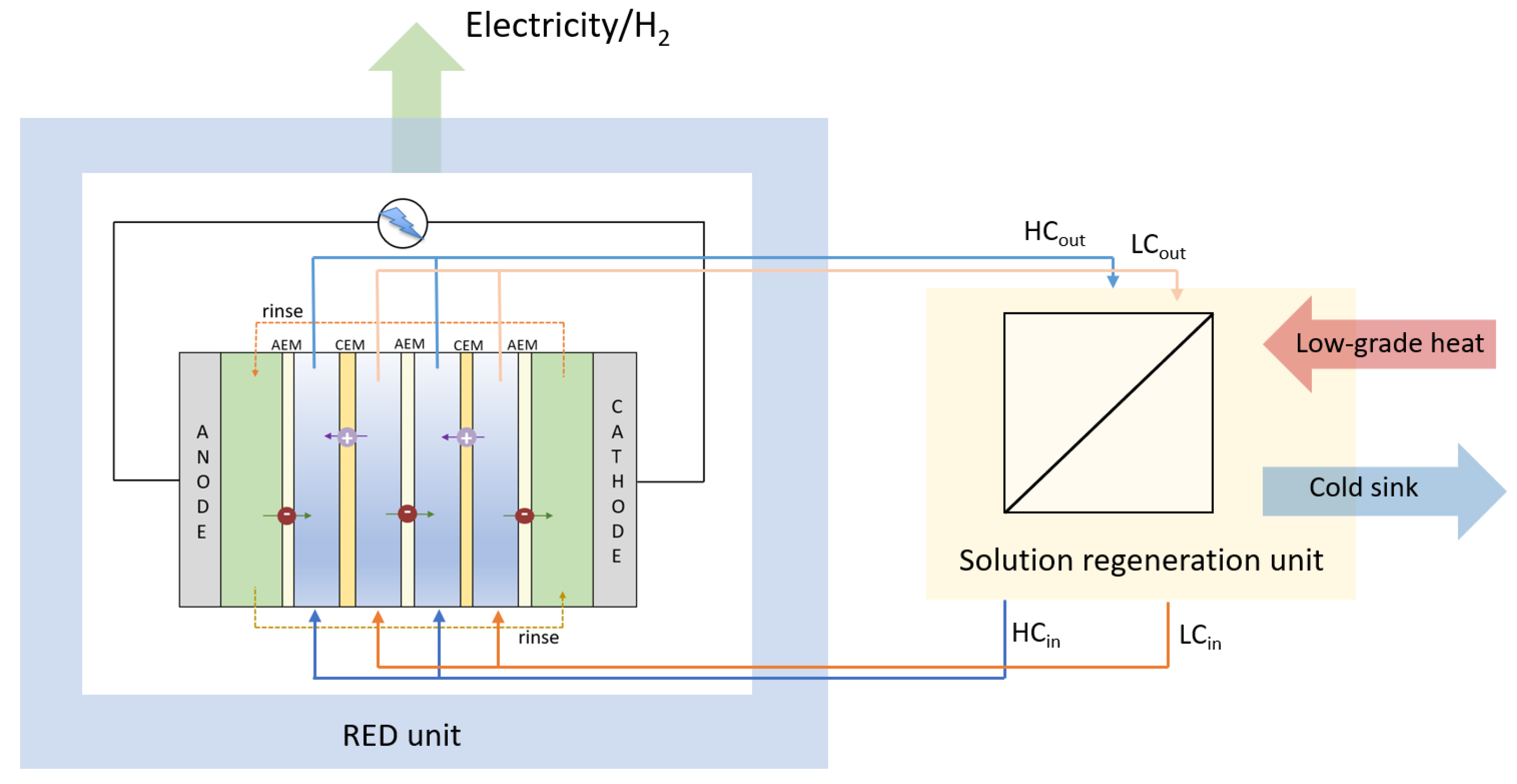

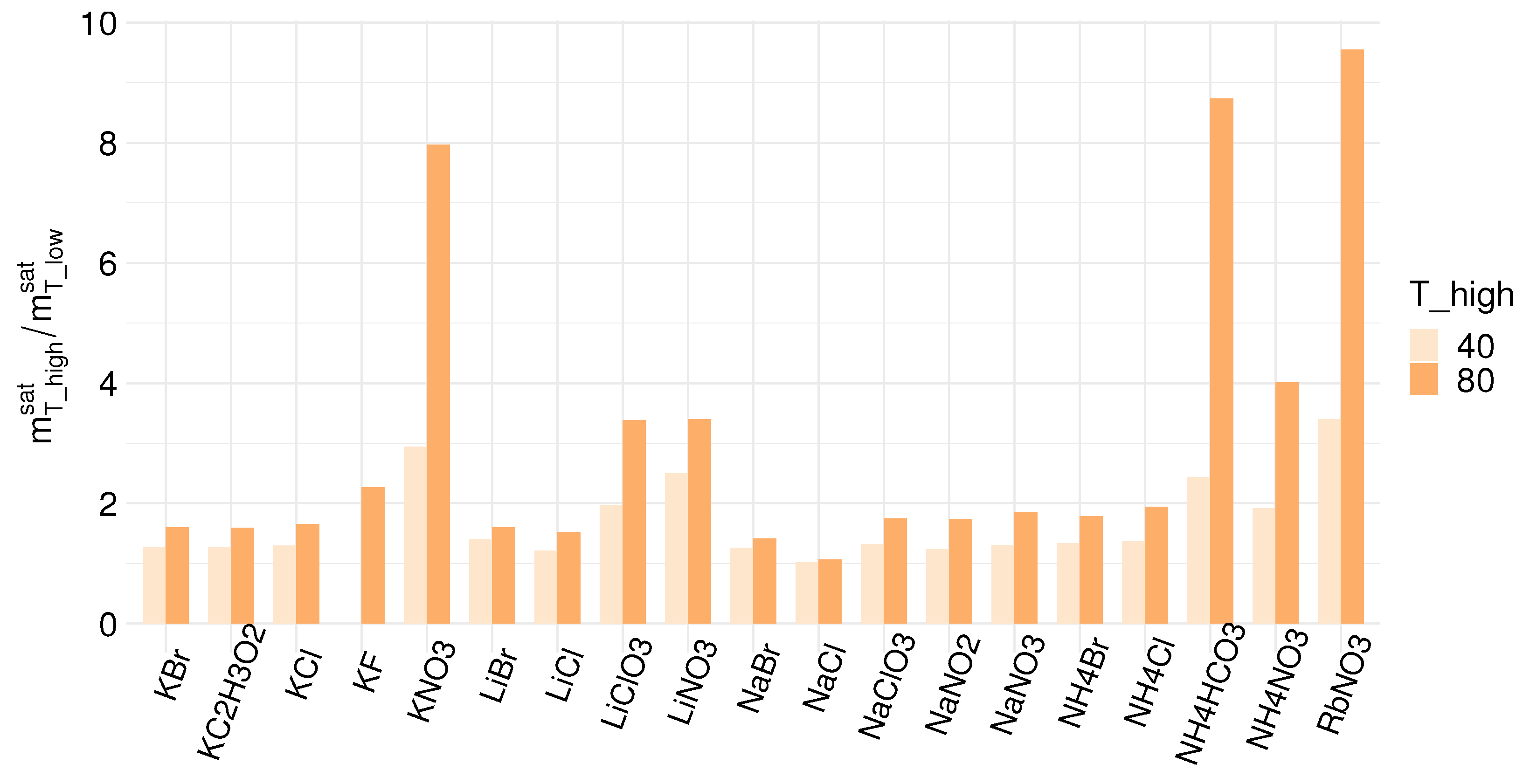
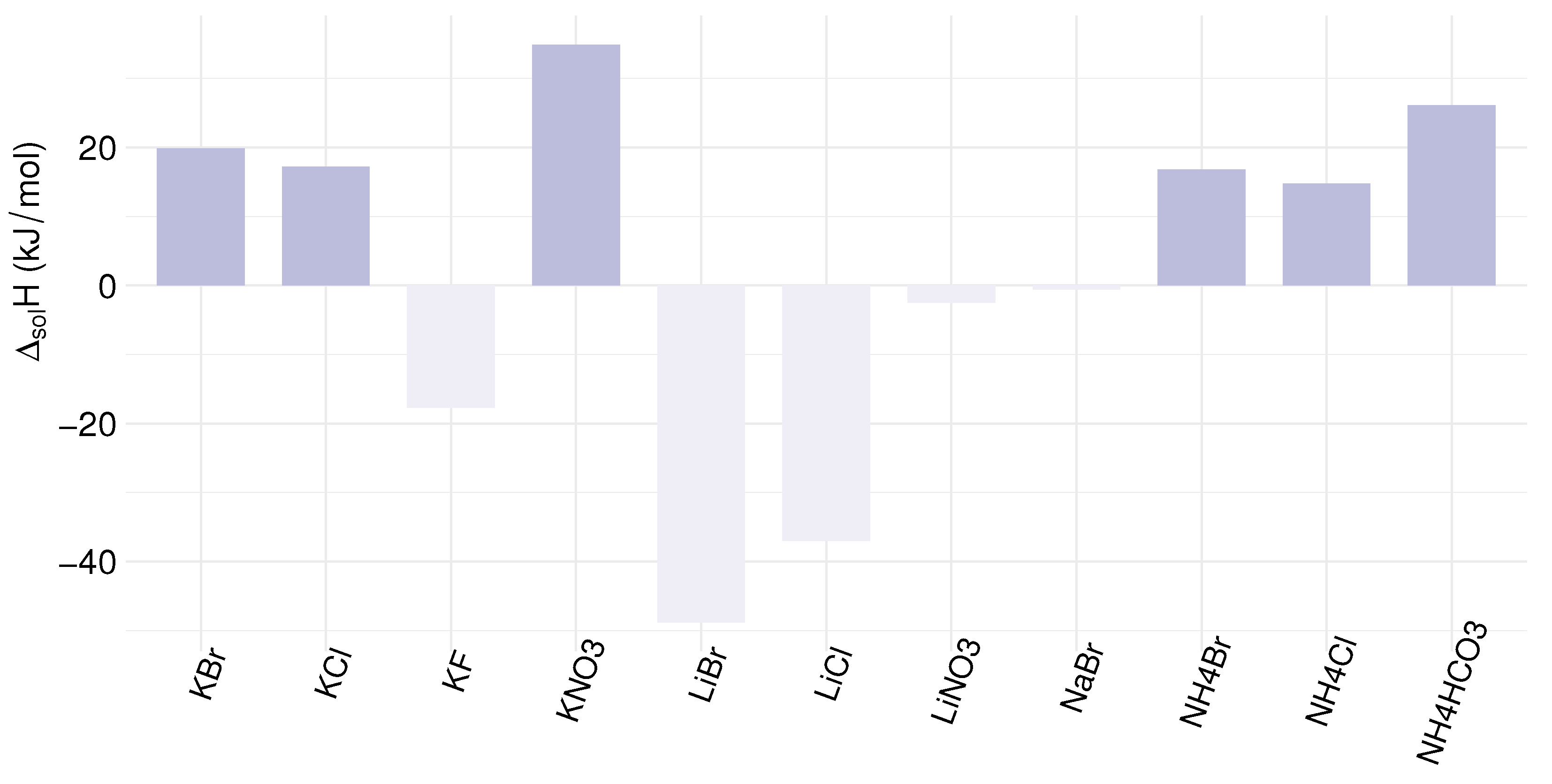
| Application | Rinse Solution | T | Membrane | Ref. | |||||||
|---|---|---|---|---|---|---|---|---|---|---|---|
| (V) | () | () | (C) | () | () | () | |||||
| NaCl, 25 cell pairs, 10 × 10 cm | 3.48–4.10 | 12.8–32.4 | 0.38–1.41 | 0.3 M C6FeK4N6 0.3 M C6FeK3N6 2.5 M NaCl | 0.68 | 10–60 | Fuji-AEM-80045 Fuji-CEM-80050 | 129 114 | 270 | 20–40 | [75,76] |
| 1.5 M/0.02 M NH4HCO3 20 cell pairs, 10.5 × 7.5 cm | 3.07 | 25 | 0.33 | 0.1 M C6FeK4N6 0.1 M C6FeK3N6 | 0.88 | amb. | Selemion AMV Selemion CMV | 130 | 500 | 48 | [57] |
| (a) 0.14 M/3.6 M NaCl (b) 0.0015 M/1.5 M NH4HCO3 10 cell pairs 8 × 8 cm | (a) 1.08 (b) - | (a) 0.62 (b) 0.32 | - | 0.6 M NaCl | - | amb. | PCCell PC-SA PCCell PC-SK | - | 500 | 0.6 (HC) 1.2 (LC) | [54] |
| (a) 0.66 M/0.0036 M NaCl (b) 5 M/0.1 M NaCl (c) 5 M/1 M NaCl 20 cell pairs 6.3 × 32 cm | (a) 4.11 (b) 2.63 (c) 0.88 | (a) - (b) 2.0 (c) 0.25 | (a) 0.5 (b) - (c) - | 0.05 M C6FeK4N6 0.05 M C6FeK3N6 0.25 M NaCl | (a) - (b) 0.68 (c) 0.46 | 24 | Fumatech FAS-50 Fumatech FKS-50 | 50 | 270 | 12 | [77] |
| brine/brackish water 125 cell pairs 44 × 44 cm | 15.4 | 1.2 | 1.6 | 0.3 M FeCl2 0.3 M FeCl3 2.5 M NaCl | - | 26 | Fujifilm: AEM 80045-01 CEM 80045-04 | 120 | 280 | 480 | [21] |
| brine/brackish water 10 cell pairs 8 × 11 cm | 2.1 | 4.5 | 0.5 | 3 M NaCl | - | 20 | Neosepta AMX Neosepta CMX | - | 200 | - | [78] |
| 0.02 M/0.5 M NaCl 50 cell pairs 10 × 10 cm | - | 17 | 0.93 | 0.05 M C6FeK4N6 0.05 M C6FeK3N6 1 M NaCl | - | 25 | Fumasep FAD Fumasep FKD | 82 | 200 | 42 | [79] |
| 0.01/5 M NaCl 5 cell pairs 10 × 10 cm | - | - | 3.8 | 0.1 M C6FeK4N6 0.1 M C6FeK3N6 0.5 M NaCl | - | 25 | Neosepta ACS Neosepta CMS | - | 100 | 1.5 | [80] |
| 0.507 M/0.017 M NaCl 5 cell pairs 10 × 10 cm | - | - | ≤ 2.2 | 0.025 M C6FeK4N6 0.025 M C6FeK3N6 0.25 M NaCl | - | 25 | Fumatech FAS Fumatech FKS | 30–40 | 60–485 | 0.06–15 | [81] |
| 5 M/0.05 M NaCl 1 cell pair 13 × 9 cm | (a) 0.115 (b) 0.118 | - | (a) 1.5 (b) 2.0 | 0.5 M FeCl2 0.5 M FeCl3 1.0 M NaCl | 0.5–0.7 0.7–0.8 | (a) 25 (b) 40 | Fumatech FAS-50 Fumatech FKS-50 | 50 | 155 | 0.42 | [15] |
| Compound | Formula | (g/mol) | Aqueous Solubility (mol/kg) | (kJ/mol) | ||
|---|---|---|---|---|---|---|
| at 10 C | at 40 C | at 80 C | ||||
| Ammonium Bromide | NH4Br | 97.94 | 6.86 | 9.16 | 12.28 | 16.78 |
| Ammonium Chloride | NH4Cl | 53.49 | 6.27 | 8.58 | 12.15 | 14.78 |
| Ammonium Bicarbonate | NH4HCO3 | 79.06 | 2.01 | 4.89 | 17.54 | 26.09 |
| Lithium Bromide | LiBr | 86.85 | 17.34 | 24.25 | 27.79 | −48.83 |
| Lithium Chloride | LiCl | 42.39 | 17.41 | 21.17 | 26.58 | −37.03 |
| Lithium Nitrate | LiNO3 | 68.95 | 8.74 | 21.85 | 29.71 | −2.51 |
| Potassium Bromide | KBr | 119.0 | 5.00 | 6.39 | 8.01 | 19.87 |
| Potassium Chloride | KCl | 74.55 | 4.15 | 5.37 | 6.87 | 17.22 |
| Potassium Fluoride | KF | 58.10 | 11.38 | 24.55 | 25.82 | −17.73 |
| Potassium Nitrate | KNO3 | 101.1 | 2.11 | 6.22 | 16.84 | 34.89 |
| Sodium Bromide | NaBr | 102.9 | 8.25 | 10.36 | 11.64 | −0.60 |
| Sodium Chloride | NaCl | 58.44 | 6.11 | 6.22 | 6.49 | 3.88 |
| Ion | Hydrated Radius (nm) | Hydration Energy (kJ/mol) | Mobility in Water () |
|---|---|---|---|
| Na+ | 0.358 | −365 | 5.19 |
| Li+ | 0.382 | −475 | 4.01 |
| K+ | 0.331 | −295 | 7.19 |
| NH4+ | 0.331 | −285 | 7.63 |
| Cl− | 0.332 | −340 | 7.91 |
| F− | 0.352 | −465 | 5.70 |
| NO3− | 0.335 | −300 | 7.40 |
| Br− | 0.330 | −315 | 8.09 |
| HCO3− | 0.439 | −335 | - |
| Component | Key Parameters | Determined by | |
|---|---|---|---|
| RED stack | Membrane properties | Permselectivity and electrical resistance | - ion-exchange capacity - water uptake - fixed charge density |
| Ion Characeristics | Affinity and mobility, open circuit potential | - hydration energy - hydrated radius - conductivity of solution - chemical potential of salt and water - activity coefficient ratio | |
| Hydrodynamic Design | hydrodynamic losses/ pressure drop | - flow channel dimensions - spacer selection - manifolding system - dead spots in flow channels | |
| Regeneration unit | Evaporation - higher H2 output - less membrane area required Precipitation - less heat required | Restored salinity gradient, heat requirement | - salt solubility - temperature dependency of solubility - dissolution enthalpy change |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zimmermann, P.; Solberg, S.B.B.; Tekinalp, Ö.; Lamb, J.J.; Wilhelmsen, Ø.; Deng, L.; Burheim, O.S. Heat to Hydrogen by RED—Reviewing Membranes and Salts for the RED Heat Engine Concept. Membranes 2022, 12, 48. https://doi.org/10.3390/membranes12010048
Zimmermann P, Solberg SBB, Tekinalp Ö, Lamb JJ, Wilhelmsen Ø, Deng L, Burheim OS. Heat to Hydrogen by RED—Reviewing Membranes and Salts for the RED Heat Engine Concept. Membranes. 2022; 12(1):48. https://doi.org/10.3390/membranes12010048
Chicago/Turabian StyleZimmermann, Pauline, Simon Birger Byremo Solberg, Önder Tekinalp, Jacob Joseph Lamb, Øivind Wilhelmsen, Liyuan Deng, and Odne Stokke Burheim. 2022. "Heat to Hydrogen by RED—Reviewing Membranes and Salts for the RED Heat Engine Concept" Membranes 12, no. 1: 48. https://doi.org/10.3390/membranes12010048
APA StyleZimmermann, P., Solberg, S. B. B., Tekinalp, Ö., Lamb, J. J., Wilhelmsen, Ø., Deng, L., & Burheim, O. S. (2022). Heat to Hydrogen by RED—Reviewing Membranes and Salts for the RED Heat Engine Concept. Membranes, 12(1), 48. https://doi.org/10.3390/membranes12010048









