A New Stochastic Split-Step θ-Nonstandard Finite Difference Method for the Developed SVIR Epidemic Model with Temporary Immunities and General Incidence Rates
Abstract
:1. Introduction
- are differentiable functions, where s.t.
- are exist and positive where and
- and
- and
- and
- Investigating the existence and uniqueness of the positive global solution of the model (3);
- Establishing the sufficient conditions for the extinction and persistence of disease;
- Designing a novel numerical method to approximate the solution of our model and comparing its performance with another method. This new method can be used to approximate the solution of other stochastic delayed epidemic models;
- Discussing the effect of the length of immunity periods, parameter values of the incidence rates and noise on the dynamics of the model.
2. Stochastic Model Analysis
2.1. Existence and Uniqueness of Positive Global Solution
2.2. Extinction of Disease
2.3. Persistence of Disease in Mean
- where
3. Numerical Model Analysis
3.1. Split-Step -Milstein Scheme
3.2. Stochastic Split-Step -Nonstandard Finite Difference Method
Convergence Analysis of Split-Step -Nonstandard Finite Difference Method
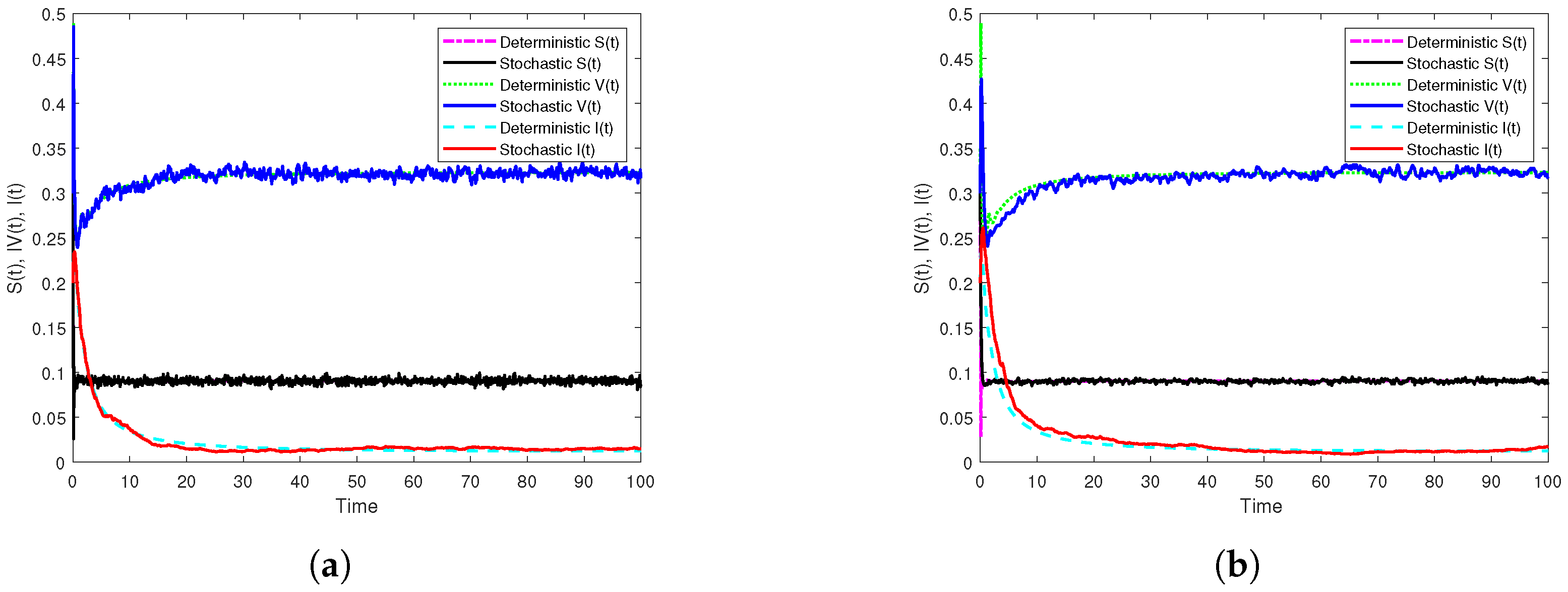
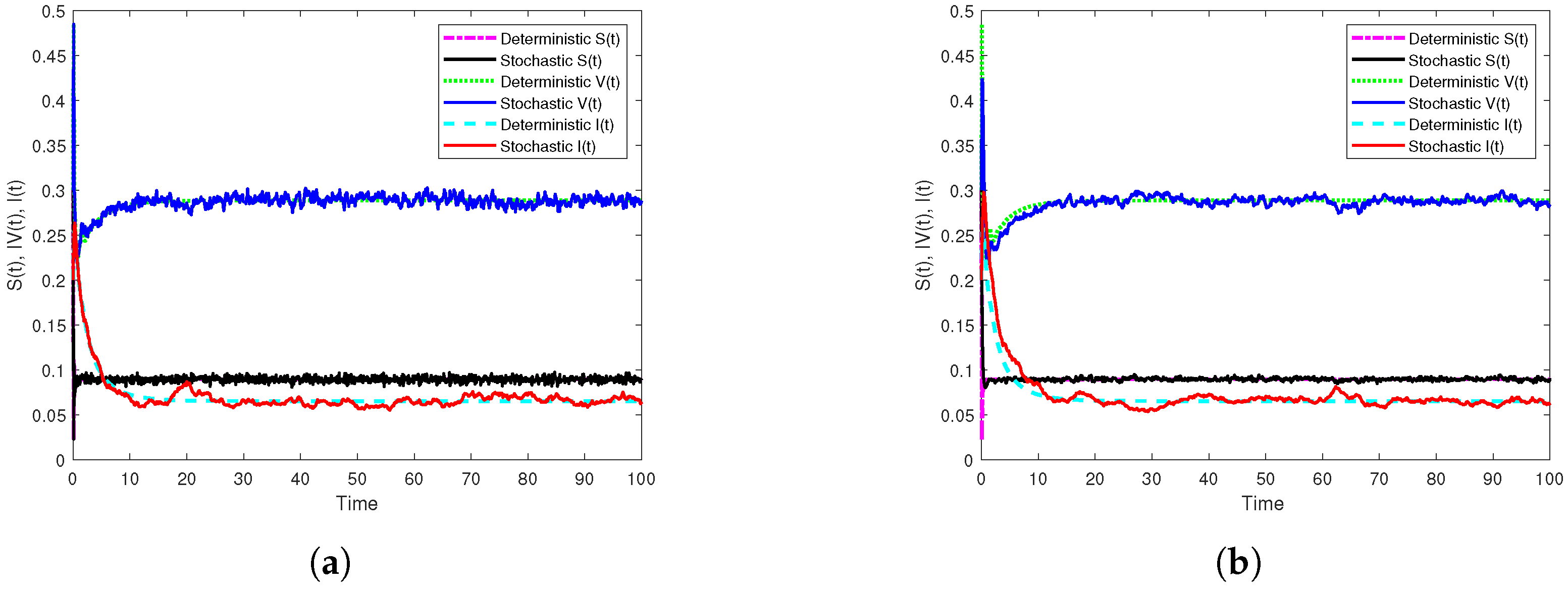
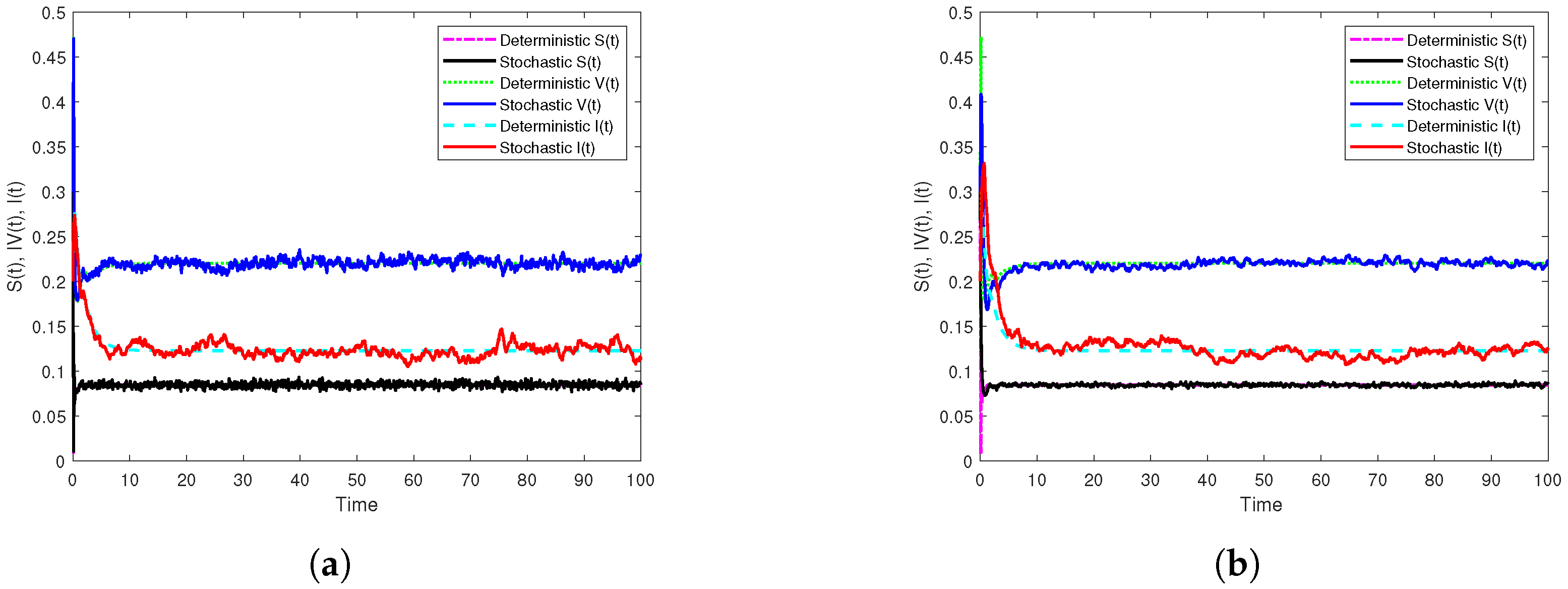
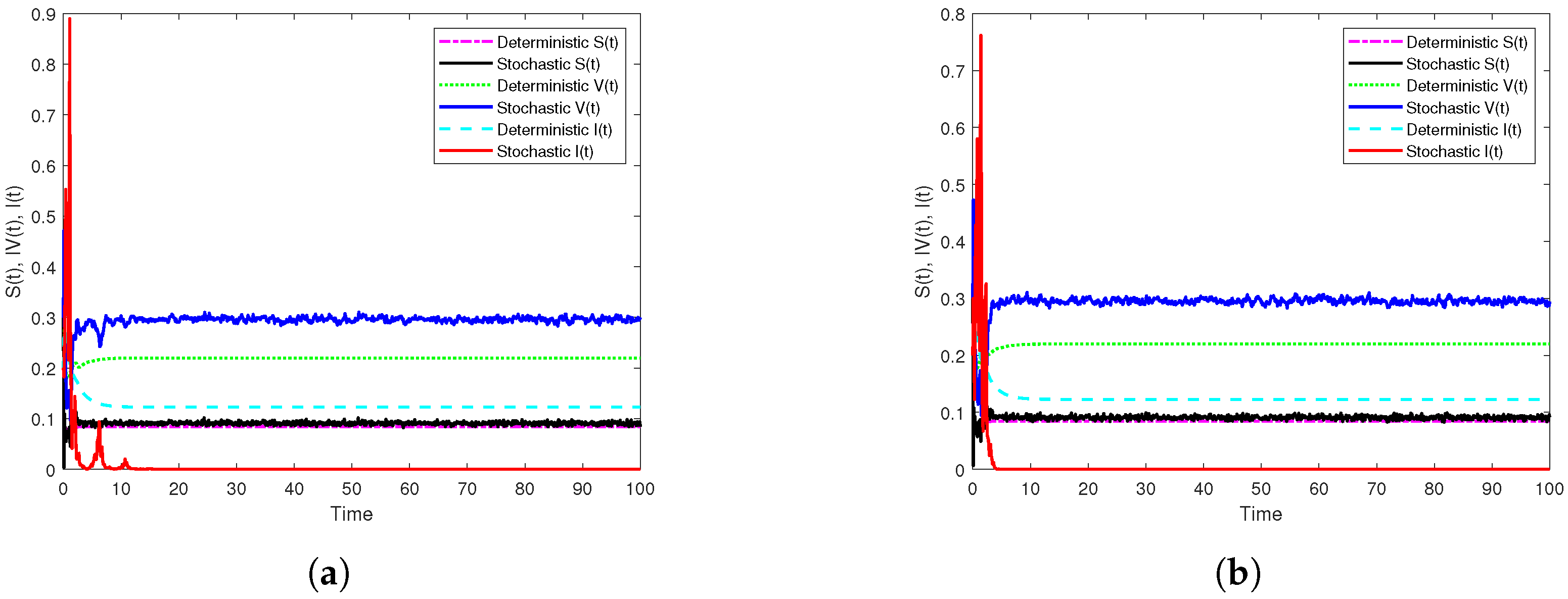
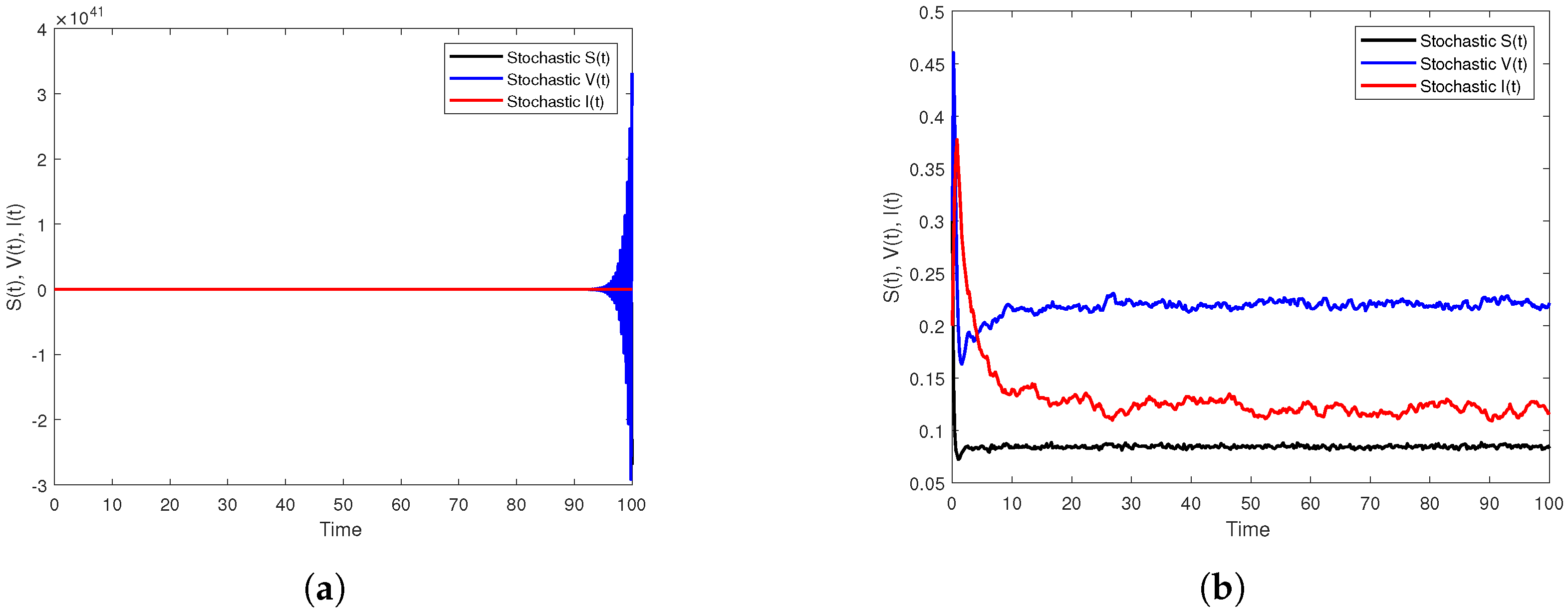
3.3. Illustration and Discussion
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Adnani, J.; Hattaf, K.; Yousfi, N. Stability Analysis of a Stochastic SIR Epidemic Model with Specific Nonlinear Incidence Rate. Int. J. Stoch. Anal. 2013, 2013, 431257. [Google Scholar] [CrossRef] [Green Version]
- Jehad, A.; Ghada, A.; Shah, H.; Elissa, N.; Hasib, K. Stochastic dynamics of influenza infection: Qualitative analysis and numerical results. Math. Biosci. Eng. 2022, 19, 10316–10331. [Google Scholar]
- Shah, H.; Elissa, N.; Hasib, K.; Haseena, G.; Sina, E.; Shahram, R.; Mohammed, K. On the Stochastic Modeling of COVID-19 under the Environmental White Noise. J. Funct. Spaces. 2022, 2022, 4320865. [Google Scholar]
- Miaomiao, G.; Daqing, J.; Tasawar, H. Stationary distribution and periodic solution of stochasticchemostat models with single-species growthon two nutrients. Int. J. Biomath. 2019, 12, 1950063. [Google Scholar]
- Liu, Q.; Jiang, D.; Shi, N.; Hayat, T.; Alsaedi, A. Asymptotic behavior of a stochastic delayed SEIR epidemic model with nonlinear incidence. Phys. A. 2016, 462, 870–882. [Google Scholar] [CrossRef]
- Tailei, Z.; Zhidong, T. Global asymptotic stability of a delayed SEIRS epidemic model with saturation incidence. Chaos Solitons Fractals 2008, 37, 1456–1468. [Google Scholar]
- Rui, X.; Zhien, M. Global stability of a SIR epidemic model with nonlinear incidence rate and time delay. Nonlinear Anal. Real World Appl. 2009, 10, 3175–3189. [Google Scholar]
- Hattaf, K.; Mahrouf, M.; Adnani, J.; Yousfi, N. Qualitative analysis of a stochastic epidemic model with specific functional response and temporary immunity. Phys. A. 2018, 490, 591–600. [Google Scholar] [CrossRef]
- Pitchaimani, M.; Brasanna, D.M. Stochastic dynamical probes in a triple delayed SICR model with general incidence rate and immunization strategies. Chaos Solitons Fractals 2021, 143, 110540. [Google Scholar]
- Xu, C.; Li, X. The threshold of a stochastic delayed SIRS epidemic model with temporary immunity and vaccination. Chaos Solitons Fractals 2018, 111, 227–234. [Google Scholar] [CrossRef]
- Zhang, X.; Jiang, D.; Hayat, T.; Ahmad, B. Dynamical behavior of a stochastic SVIR epidemic model with vaccination. Phys. A. 2017, 483, 94–108. [Google Scholar] [CrossRef]
- Xianning, L.; Yasuhiro, T.; Shingo, I. SVIR epidemic models with vaccination strategies. J. Theoret. Biol. 2008, 253, 1–11. [Google Scholar]
- Li, F.; Zhang, S.Q.; Meng, X.Z. Dynamics analysis and numerical simulations of a delayed stochastic epidemic model subject to a general response function. Comput. Appl. Math. 2019, 38, 95. [Google Scholar] [CrossRef]
- Hattaf, K.; Yousfi, N.; Tridane, A. Stability analysis of a virus dynamics model with general incidence rate and two delays. Appl. Math. Comput. 2013, 221, 514–521. [Google Scholar] [CrossRef]
- Wang, J.; Zhang, J.; Jin, Z. Analysis of an SIR model with bilinear incidence rate. Nonlinear Anal. Real World Appl. 2010, 11, 2390–2402. [Google Scholar] [CrossRef]
- Liu, X.; Yang, L. Stability analysis of an SEIQV epidemic model with saturated incidence rate. Nonlinear Anal. Real World Appl. 2012, 13, 2671–2679. [Google Scholar] [CrossRef]
- Zhao, Y.; Jiang, D. The threshold of a stochastic SIRS epidemic model with saturated incidence. Appl. Math. Lett. 2014, 34, 90–93. [Google Scholar] [CrossRef]
- Cantrell, R.; Cosner, C. On the dynamics of predator-prey models with the Beddington–DeAngelis functional response. J. Math. Anal. Appl. 2001, 257, 206–222. [Google Scholar] [CrossRef]
- Zhou, X.; Cui, J. Global stability of the viral dynamics with Crowley–Martin functional response. Bull. Korean Math. Soc. 2011, 48, 555–574. [Google Scholar] [CrossRef] [Green Version]
- Anderson, R.; Garnett, G. Low-efficacy HIV vaccines: Potential for community-based intervention programmes. Lancet 1996, 348, 1010–1013. [Google Scholar] [CrossRef]
- Chaves, S.; Gargiullo, P.; Zhang, J.; Civen, R.; Guris, D.; Mascola, L.; Seward, J. Loss of vaccine-induced immunity to varicella over time. N. Engl. J. Med. 2007, 356, 1121–1129. [Google Scholar] [CrossRef] [PubMed]
- Wendelboe, A.; Van Rie, A.; Salmaso, S.; Englund, J. Duration of immunity against pertussis after natural infection or vaccination. Pediatr. Infect. Dis. J. 2005, 24, 58–61. [Google Scholar] [CrossRef] [PubMed]
- Craig, M.P. An evolutionary epidemiological mechanism, with applications to type a influenza. Theor. Popul. Biol. 1987, 31, 422–452. [Google Scholar]
- Loubet, P.; Laureillard, D.; Martin, A.; Larcher, R.; Sotto, A. Why promoting a COVID-19 vaccine booster dose? Anaesth Crit Care Pain Med. 2021, 40, 100967. [Google Scholar] [CrossRef] [PubMed]
- Mohamed, F.; Roger, P.; Idriss, S.; Regragui, T. A stochastic analysis for a triple delayed SIQR epidemic model with vaccination and elimination strategies. J. Appl. Math. Comput. 2020, 64, 781–805. [Google Scholar]
- Mao, X. Stochastic Differential Equations and Their Applications; Horwood: Chichester, UK, 1997. [Google Scholar]
- Chunyan, J.; Daqing, J. Threshold behaviour of a stochastic SIR model. Appl. Math. Model. 2014, 38, 5067–5079. [Google Scholar]
- Qian, G.; Wenwen, X.; Taketomo, M. Convergence and Stability of the Split-Step θ-Milstein Method for Stochastic Delay Hopfield Neural Networks. Abstr. Appl. Anal. 2013, 2013, 169214. [Google Scholar]
- Zain, U.; Nigar, A.; Samina, Y.; Sayed, F.; Kottakkaran, S. Numerical investigations of stochastic HIV/AIDS infection model. Alex. Eng. J. 2021, 60, 5341–5363. [Google Scholar]
- Raza, A.; Arif, M.S.; Rafiq, M. A reliable numerical analysis for stochastic dengue epidemic model with incubation period of virus. Adv. Differ. Equ. 2019, 2019, 32. [Google Scholar] [CrossRef]

| Parameter | Biological Meaning |
|---|---|
| The recruitment rate and natural rate of population | |
| The rate at which susceptible individuals are moved into the vaccination process | |
| The transmission coefficient between the two compartments and | |
| The disease transmission rate when the vaccinees contact with infected individuals before obtaining the immunity against the disease | |
| The recovery rate of infected individuals | |
| The average rate for the vaccinees to obtain immunity and move into recovery compartment | |
| The length of the immunity period of the recovered infected individuals | |
| The length of the immunity period of recovered vaccinated individuals |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Alkhazzan, A.; Wang, J.; Nie, Y.; Hattaf, K. A New Stochastic Split-Step θ-Nonstandard Finite Difference Method for the Developed SVIR Epidemic Model with Temporary Immunities and General Incidence Rates. Vaccines 2022, 10, 1682. https://doi.org/10.3390/vaccines10101682
Alkhazzan A, Wang J, Nie Y, Hattaf K. A New Stochastic Split-Step θ-Nonstandard Finite Difference Method for the Developed SVIR Epidemic Model with Temporary Immunities and General Incidence Rates. Vaccines. 2022; 10(10):1682. https://doi.org/10.3390/vaccines10101682
Chicago/Turabian StyleAlkhazzan, Abdulwasea, Jungang Wang, Yufeng Nie, and Khalid Hattaf. 2022. "A New Stochastic Split-Step θ-Nonstandard Finite Difference Method for the Developed SVIR Epidemic Model with Temporary Immunities and General Incidence Rates" Vaccines 10, no. 10: 1682. https://doi.org/10.3390/vaccines10101682
APA StyleAlkhazzan, A., Wang, J., Nie, Y., & Hattaf, K. (2022). A New Stochastic Split-Step θ-Nonstandard Finite Difference Method for the Developed SVIR Epidemic Model with Temporary Immunities and General Incidence Rates. Vaccines, 10(10), 1682. https://doi.org/10.3390/vaccines10101682






