A Novel Analytical Method of Inductance Identification for Direct Drive PMSM with a Stator Winding Fault Considering Spatial Position of the Shorted Turns
Abstract
:1. Introduction
2. Structure and Parameters of the DDPMSM
3. Inductance Identification and Inductance Analytical Expressions
3.1. SAM
3.2. The Proposed NAM
3.2.1. Inductances Identification of Fault Coils in the Slot Considering Spatial Position
3.2.2. Inductances’ Identification of Branches Considering Spatial Position
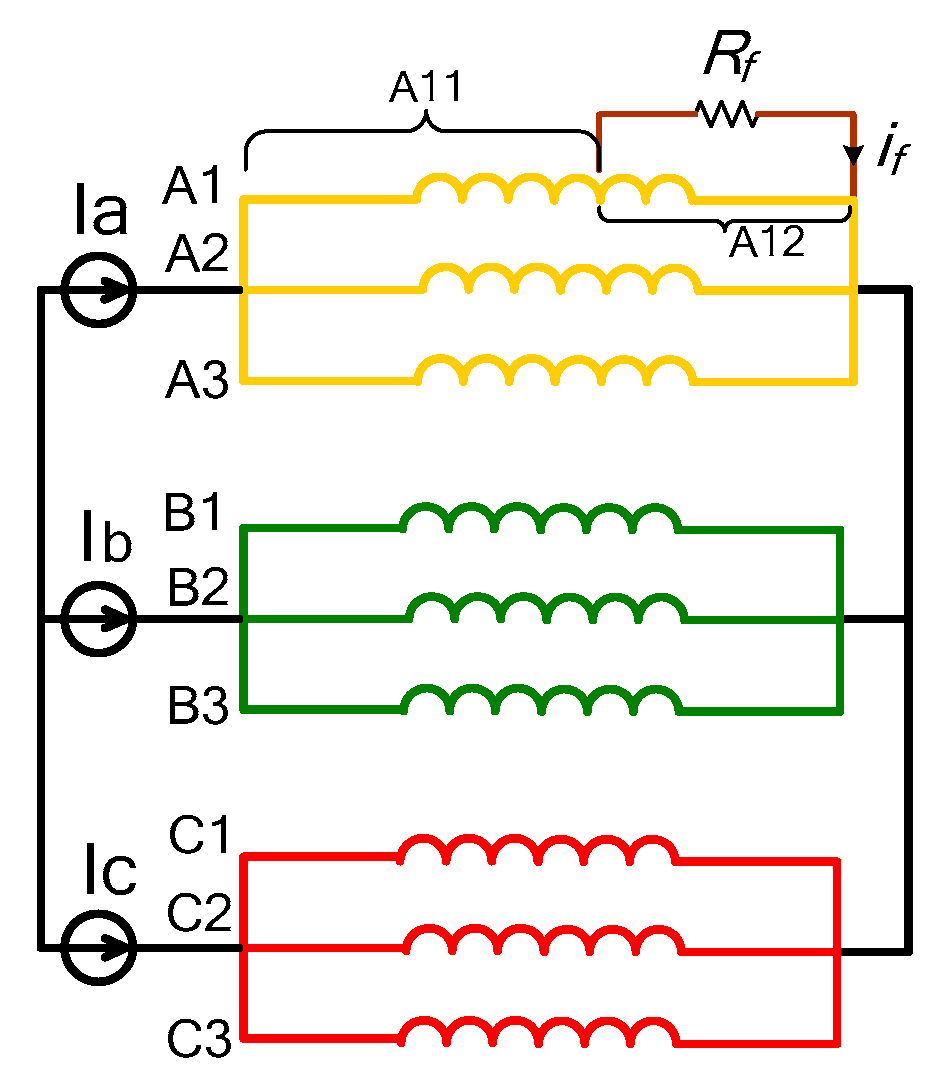
4. Model of DDPMSM with SWF
5. Validation of the Proposed Method by Comparing Inductance and Fault Current
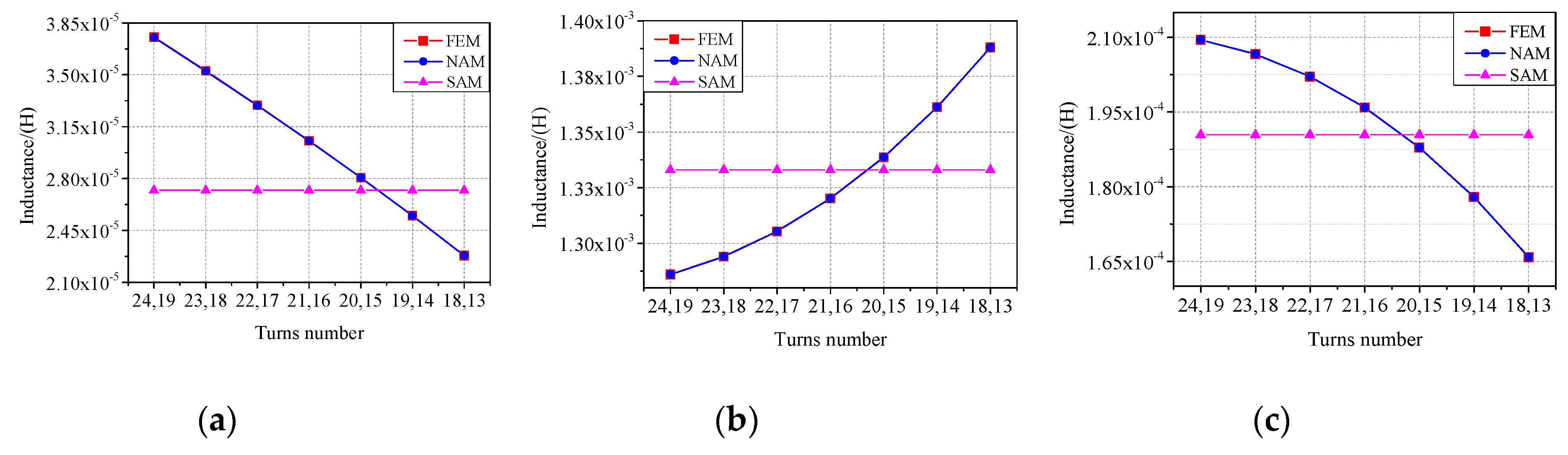
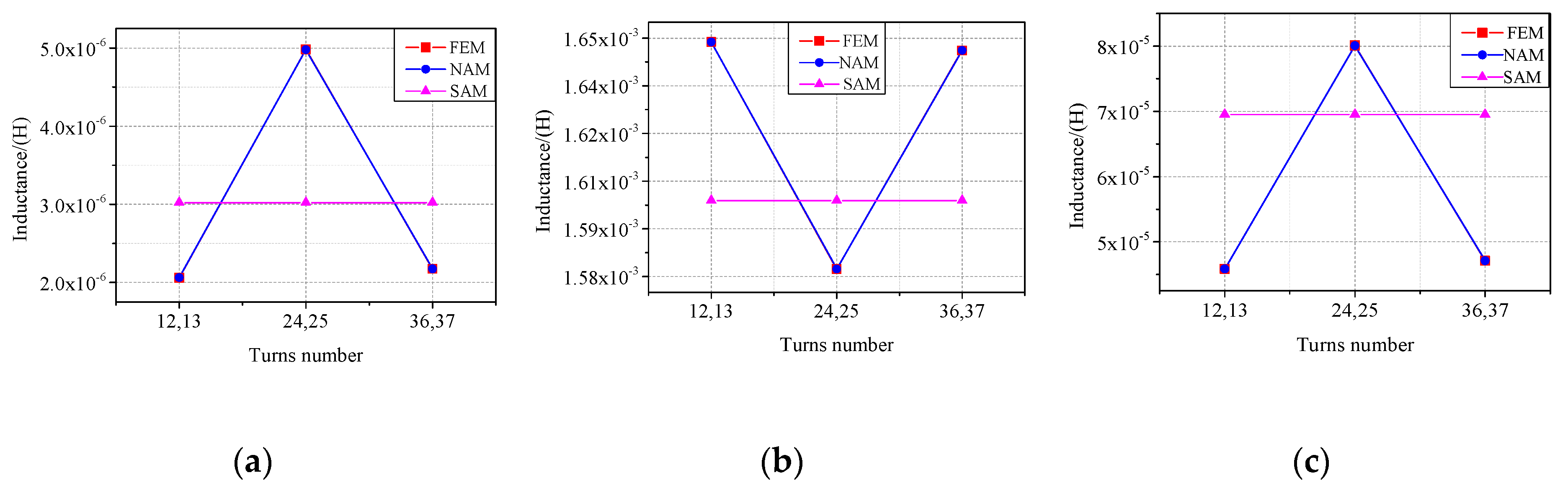
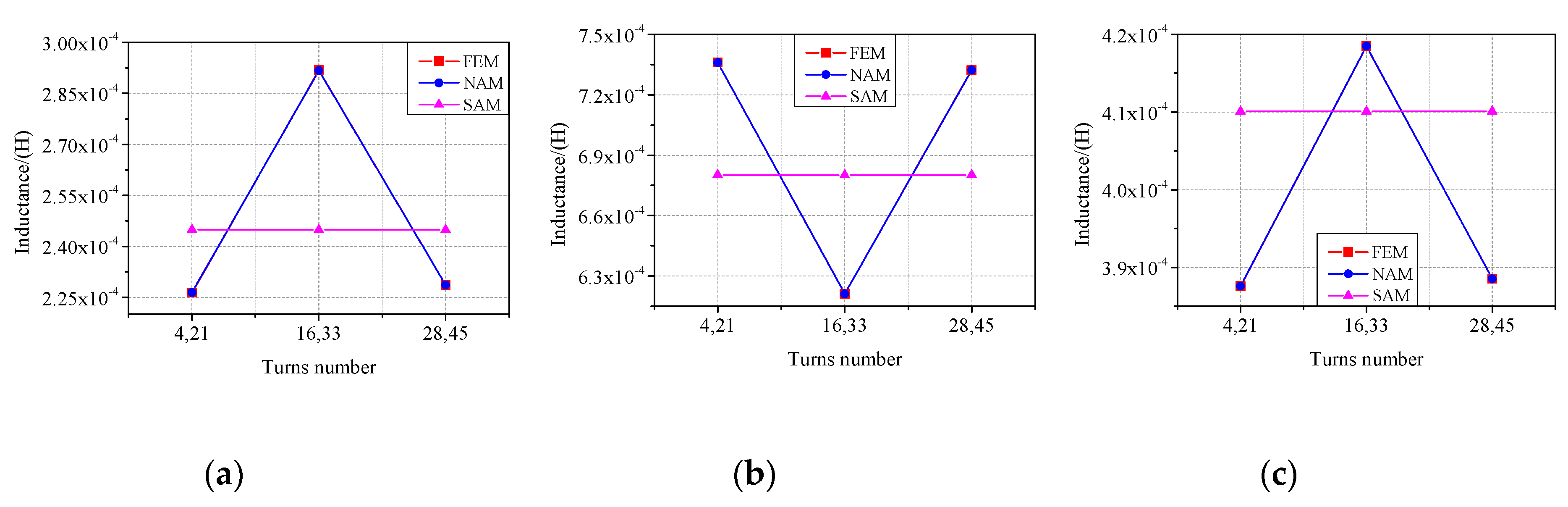
5.1. Inductance Comparisons
5.1.1. Inductances Identification of Faulty Coils in the Slot
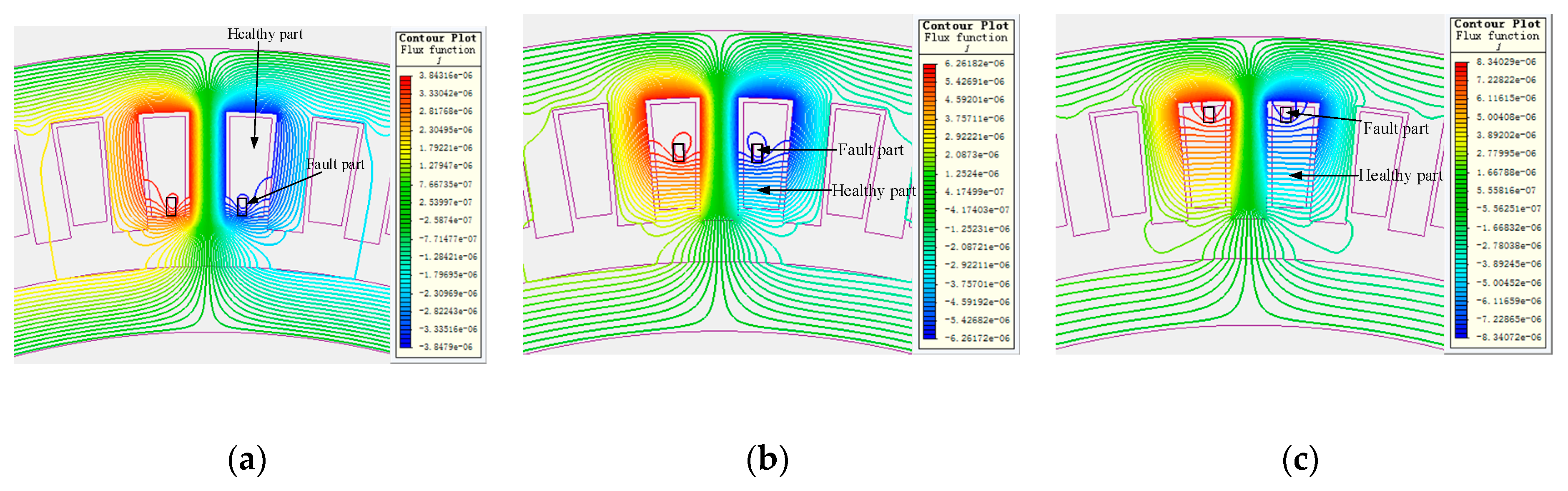
ITSCF in the Same Layer
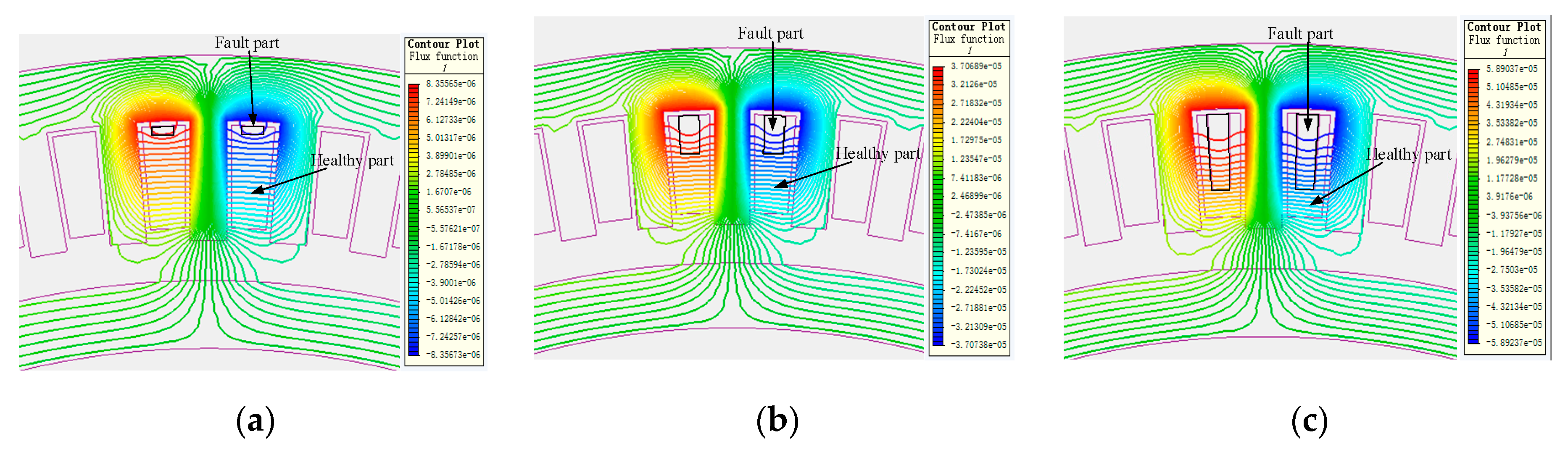
ITSCF in the Different Layers
5.1.2. Inductances Identification of Fault Branches
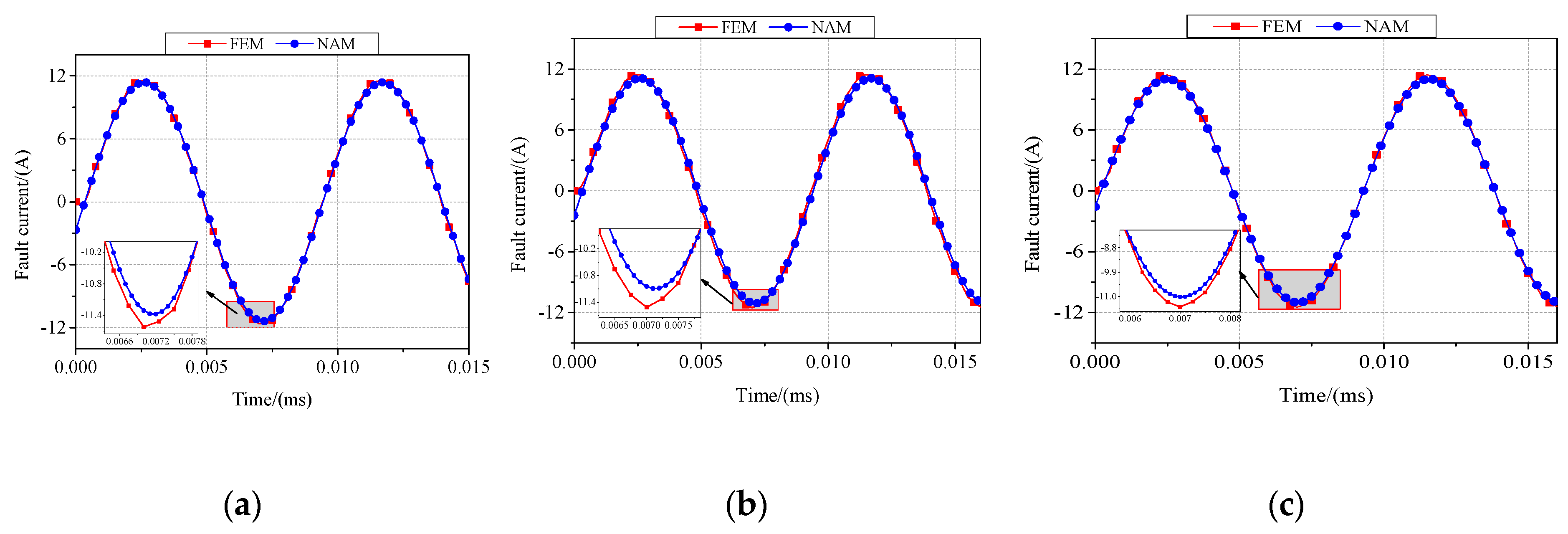
5.2. Fault Current Comparisons
5.2.1. ITSCF in the Same Layer
5.2.2. Inter Turn Short Circuit Fault in the Different Layers
6. Conclusions
Author Contributions
Funding
Conflicts of Interest
References
- Tang, R.Y. Modern Permanent Magnet Machines Theory and Machines; China Machine Press: Beijing, China, 1997; pp. 310–311. [Google Scholar]
- Xia, C.L.; Ji, B.N.; Yan, Y. Smooth Speed Control for Low-Speed High-Torque Permanent–Magnet Synchronous Motor Using Proportional Integral Resonant Controller. IEEE Trans. Ind. Electron. 2015, 62, 2123–2134. [Google Scholar] [CrossRef]
- Donato, G.D.; Capponi, F.G.; Borocci, G. Ω-shaped axial-flux permanent-magnet machine for direct-drive applications with constrained shaft height. IEEE Trans. Ind. Appl. 2015, 51, 3050–3058. [Google Scholar] [CrossRef]
- Choi, G.; Jahns, T.M. PM Synchronous Machine Drive Response to Asymmetrical Short-Circuit Faults. IEEE Trans. Ind. Appl. 2016, 52, 2167–2185. [Google Scholar] [CrossRef]
- Vaseghi, B.; Nahid-mobarakh, B.; Takorabet, N. Inductance Identification and Study of PM Motor with Winding Turn Short Circuit Fault. IEEE Trans. Magn. 2011, 47, 978–981. [Google Scholar] [CrossRef]
- Bi, D.Q.; Wang, X.H.; Wang, W.J. Improved Transient Simulation of Salient-Pole Synchronous Generators with Internal and Ground Faults in the Stator Winding. IEEE Trans. Energy Convers. 2005, 20, 128–134. [Google Scholar] [CrossRef]
- Sim, J.H.; Ahn, D.G.; Kim, D.Y. Three-Dimensional Equivalent Magnetic Circuit Network Method for Precise and Fast Analysis of PM-Assisted Claw-Pole Synchronous Motor. IEEE Trans. Ind. Appl. 2018, 54, 160–171. [Google Scholar] [CrossRef]
- Leboeuf, N.; Boileau, T.; Nahid-Mobarakeh, B. Inductance Calculations in Permanent-Magnet Motors under Fault Conditions. IEEE Trans. Magn. 2012, 48, 2605–2616. [Google Scholar] [CrossRef]
- Gao, Y.T.; Qu, R.H.; Li, D.W. Improved hybrid method to calculate inductances of permanent magnet synchronous machines with skewed stators based on winding function theory. Chin. J. Electr. Eng. 2016, 2, 52–61. [Google Scholar]
- Xu, W.; Sun, G.Y.; Wen, G.L. Equivalent Circuit Derivation and Performance Analysis of a Single-Sided Linear Induction Motor Based on the Winding Function Theory. IEEE Trans. Veh. Technol. 2012, 61, 1515–1525. [Google Scholar] [CrossRef]
- Vaseghi, B.; Takorabet, N.; Meibody-Tabar, F. Fault Analysis and Parameter Identification of Permanent-Magnet Motors by the Finite-Element Method. IEEE Trans. Magn. 2009, 45, 3290–3295. [Google Scholar] [CrossRef]
- Mohammed, O.A.; Liu, Z.; Liu, S. Internal Short Circuit Fault Diagnosis for PM Machines Using FE-Based Phase Variable Model and Wavelets Analysis. IEEE Trans. Magn. 2007, 43, 1729–1732. [Google Scholar] [CrossRef]
- Vaseghi, B.; Takorabet, N.; Meibody-Tabar, F. Analytical circuit-based model of PMSM under stator inter-turn short-circuit fault validated by time-stepping finite element analysis. In Proceedings of the XIX International Conference on Electrical Machines—ICEM 2010, Rome, Italy, 6–8 September 2010. [Google Scholar]
- Leboeuf, N.; Boileau, T.; Nahid-Mobarakeh, B. Estimating Permanent-Magnet Motor Parameters under Inter-Turn Fault Conditions. IEEE Trans. Magn. 2012, 48, 963–966. [Google Scholar] [CrossRef]
- HÄRSJÖ, J. Modeling and Analysis of PMSM with Turn-To-Turn Fault. Ph.D. Thesis, Chalmers University of Technology, Göteborg, Sweden, 2016. [Google Scholar]


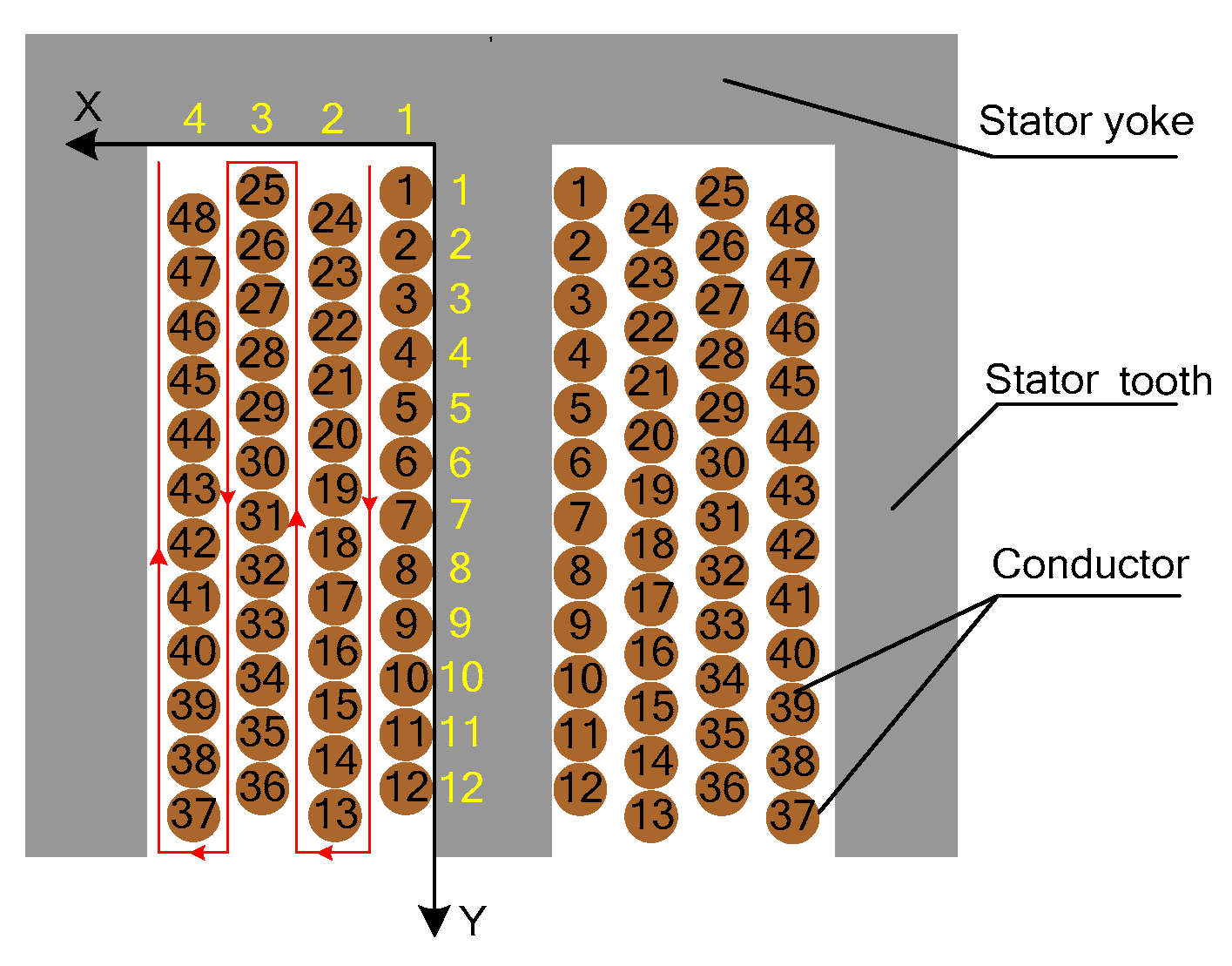
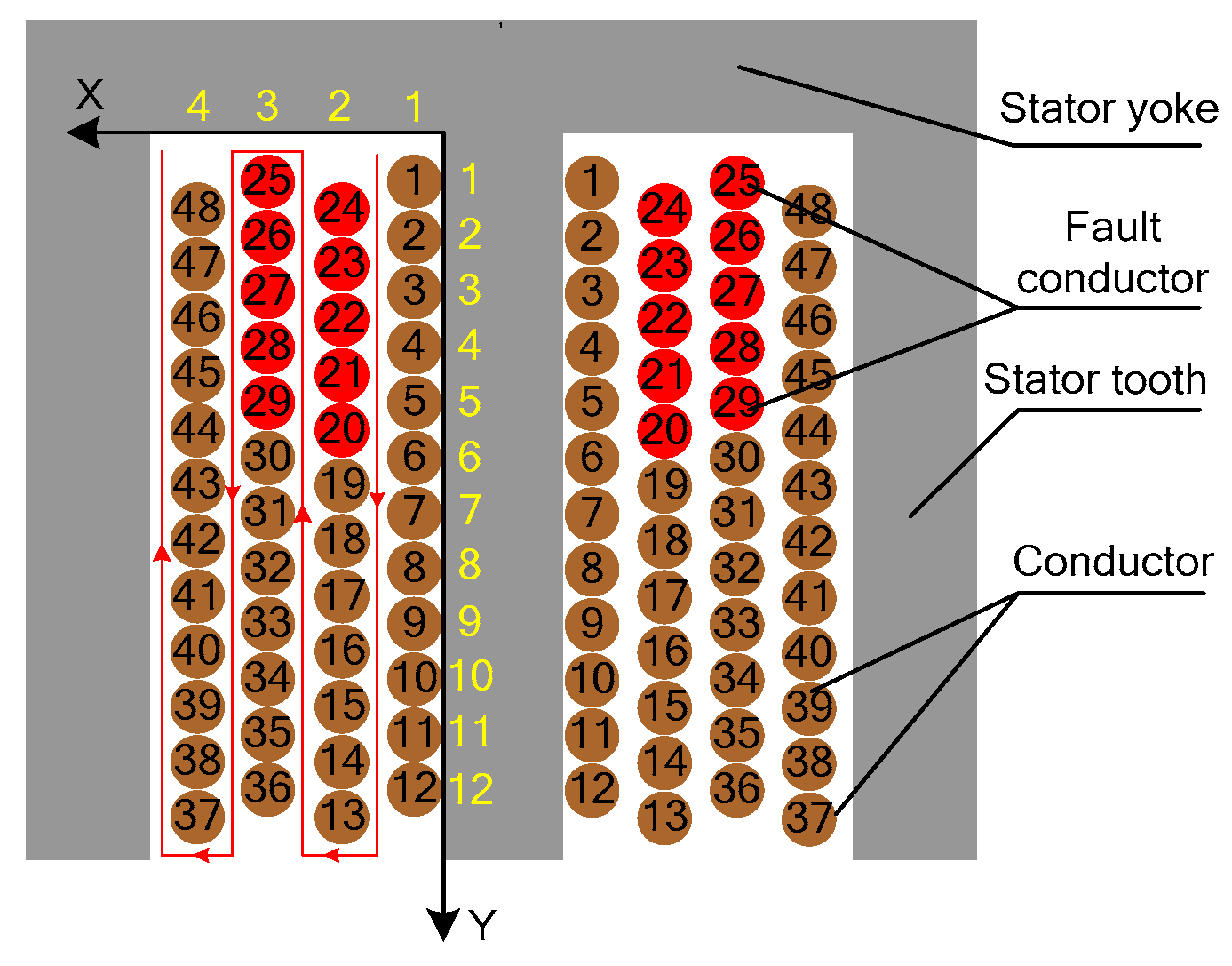
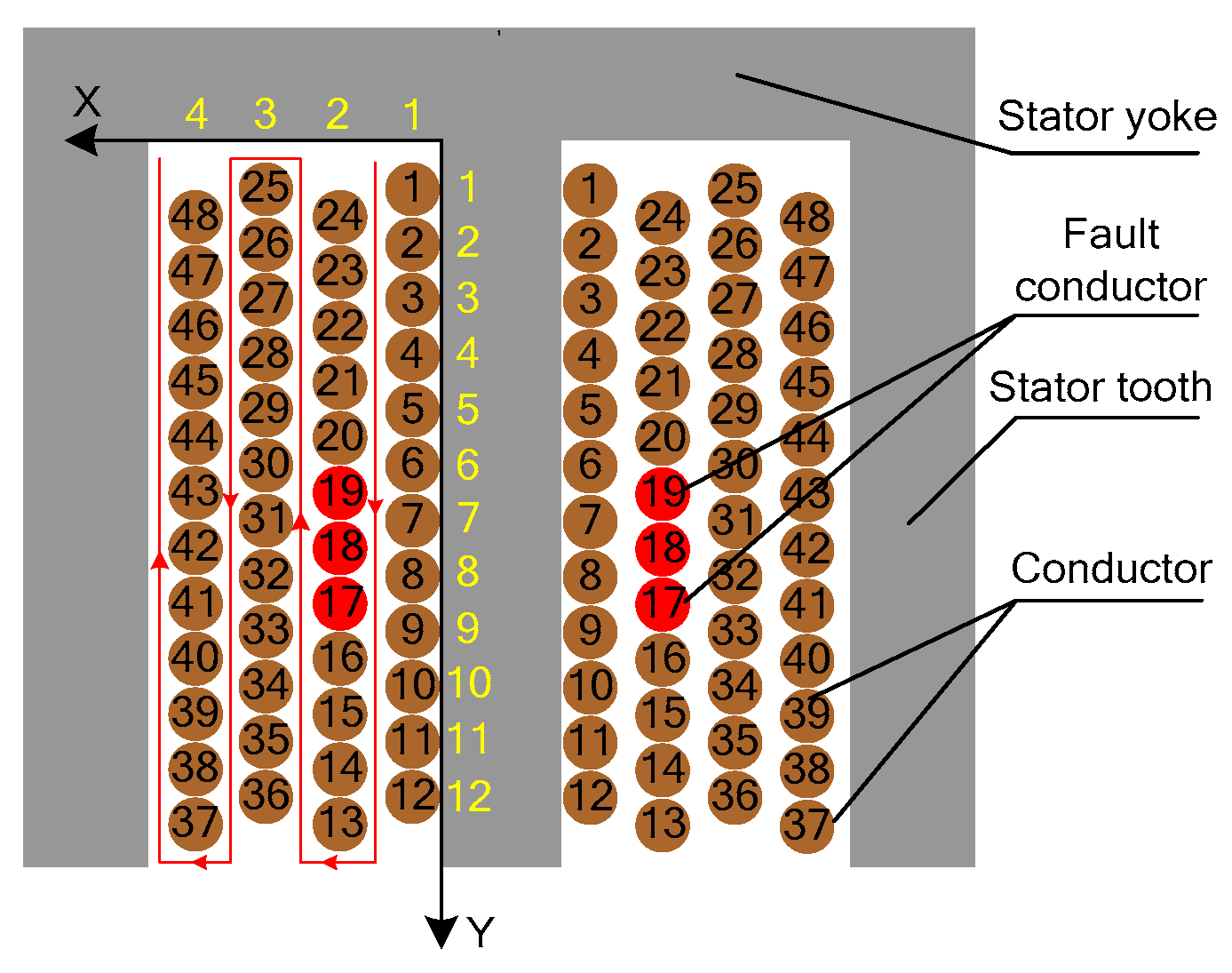
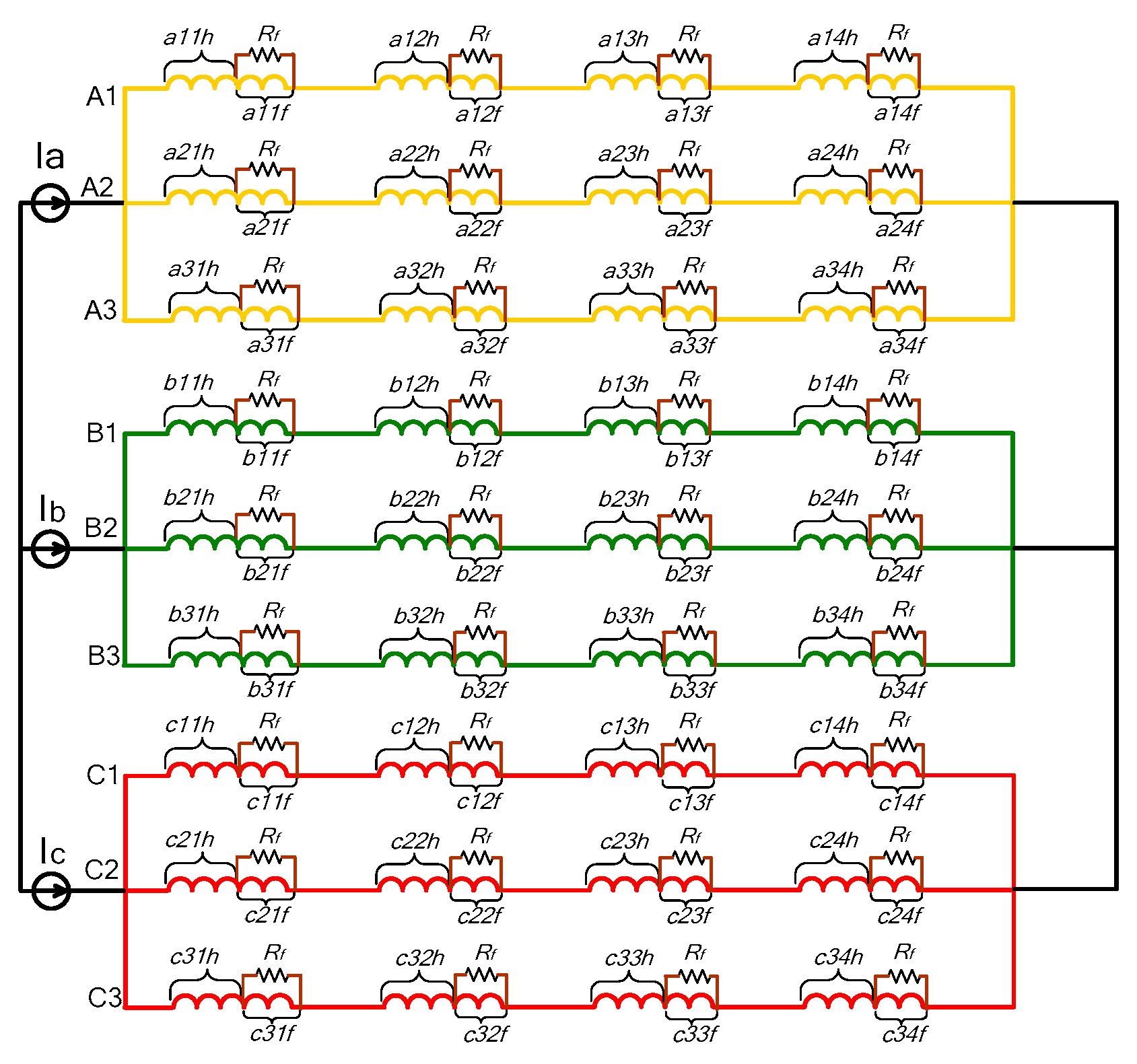





| Items (Unit) | Value | Items (Unit) | Value |
|---|---|---|---|
| Out diameter of stator (mm) | 360 | Axial length (mm) | 150 |
| Inner diameter of stator (mm) | 300 | Rated power (kW) | 10 |
| Inner diameter of rotor (mm) | 265 | Rated speed (rpm) | 200 |
| Air-gap length (mm) | 1.2 | Number of phases | 3 |
| Wire diameter of winding (mm) | 1.3 | Number of coils | 36 |
| Area of slot (mm2) | 154 | Coil turns | 48 |
| Thickness of PM (mm) | 5.3 | Parallel-circuits per phase | 3 |
| Pole-arc coefficient | 0.83 | Slot-pole combination | 72–66 |
| Turns | 2 Turns (Y = 1–2) | 6 Turns (Y = 1–6) | 10 Turns (Y = 1–10) | |
|---|---|---|---|---|
| Layer | ||||
| Layer1 | 5.07339 10−6 | 3.842 10−5 | 8.76368 10−5 | |
| Layer2 | 4.89856 10−6 | 3.7532 10−5 | 8.6111 10−5 | |
| Layer3 | 4.89909 10−6 | 3.75409 10−5 | 8.62187 10−5 | |
| Layer4 | 5.07488 10−6 | 3.84441 10−5 | 8.79202 10−5 | |
| Method | FEM | NAM | Error_1 | SAM | Error_2 | |
|---|---|---|---|---|---|---|
| Branch | ||||||
| A1 | 0.006961 | 0.006962 | 3.08822 10−5 | 0.00696 | −0.00024 | |
| A2 | 0.006962 | 0.006962 | 6.95848 10−6 | 0.006961 | −0.00013 | |
| A3_healthy | 0.006736 | 0.006736 | × 10−5 | 0.005033 | −0.25275 | |
| A3_fault | 0.000227 | 0.000227 | × 10−7 | 2.2 × 10−5 | −0.90304 | |
| B1 | 0.006976 | 0.006976 | −4.12819 10−8 | 0.006978 | 0.000282 | |
| B2 | 0.006978 | 0.006978 | −5.6927 10−6 | 0.006979 | 0.00014 | |
| B3 | 0.006976 | 0.006976 | −1.44934 10−6 | 0.006971 | −0.00065 | |
| C1 | 0.006975 | 0.006975 | −4.33412 10−7 | 0.006976 | 0.000212 | |
| C2 | 0.006976 | 0.006976 | −5.9959 10−7 | 0.006977 | 0.000129 | |
| C3 | 0.006976 | 0.006976 | 2.41647 10−6 | 0.006972 | −0.00062 | |
© 2019 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Gao, C.; Gao, M.; Si, J.; Su, P.; Hu, Y. A Novel Analytical Method of Inductance Identification for Direct Drive PMSM with a Stator Winding Fault Considering Spatial Position of the Shorted Turns. Appl. Sci. 2019, 9, 3599. https://doi.org/10.3390/app9173599
Gao C, Gao M, Si J, Su P, Hu Y. A Novel Analytical Method of Inductance Identification for Direct Drive PMSM with a Stator Winding Fault Considering Spatial Position of the Shorted Turns. Applied Sciences. 2019; 9(17):3599. https://doi.org/10.3390/app9173599
Chicago/Turabian StyleGao, Caixia, Mengzhen Gao, Jikai Si, Peng Su, and Yihua Hu. 2019. "A Novel Analytical Method of Inductance Identification for Direct Drive PMSM with a Stator Winding Fault Considering Spatial Position of the Shorted Turns" Applied Sciences 9, no. 17: 3599. https://doi.org/10.3390/app9173599
APA StyleGao, C., Gao, M., Si, J., Su, P., & Hu, Y. (2019). A Novel Analytical Method of Inductance Identification for Direct Drive PMSM with a Stator Winding Fault Considering Spatial Position of the Shorted Turns. Applied Sciences, 9(17), 3599. https://doi.org/10.3390/app9173599





