Numerical Approach for Studying the Evolution of the Degrees of Coherence of Partially Coherent Beams Propagation through an ABCD Optical System
Abstract
1. Introduction
2. Theory
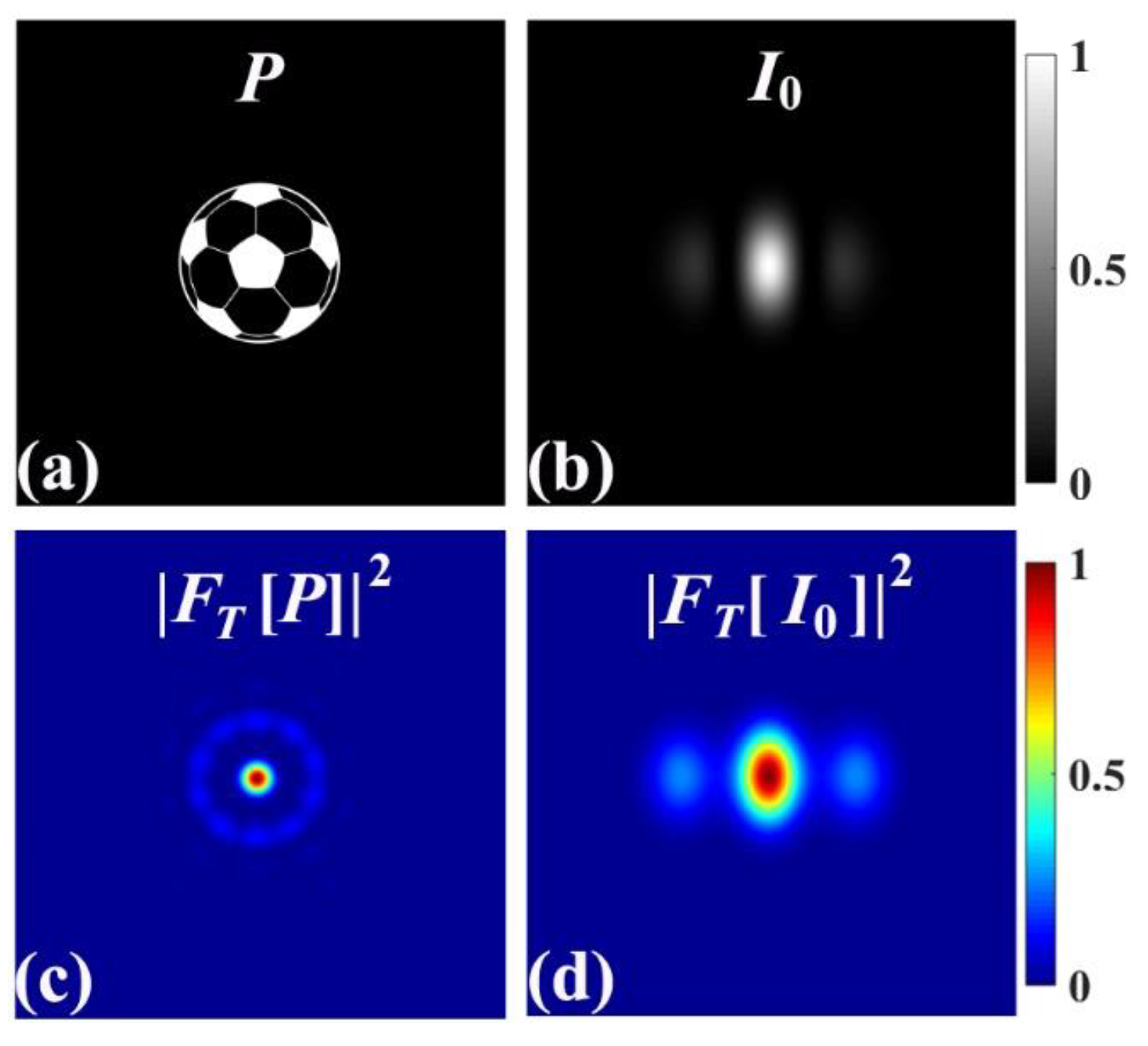
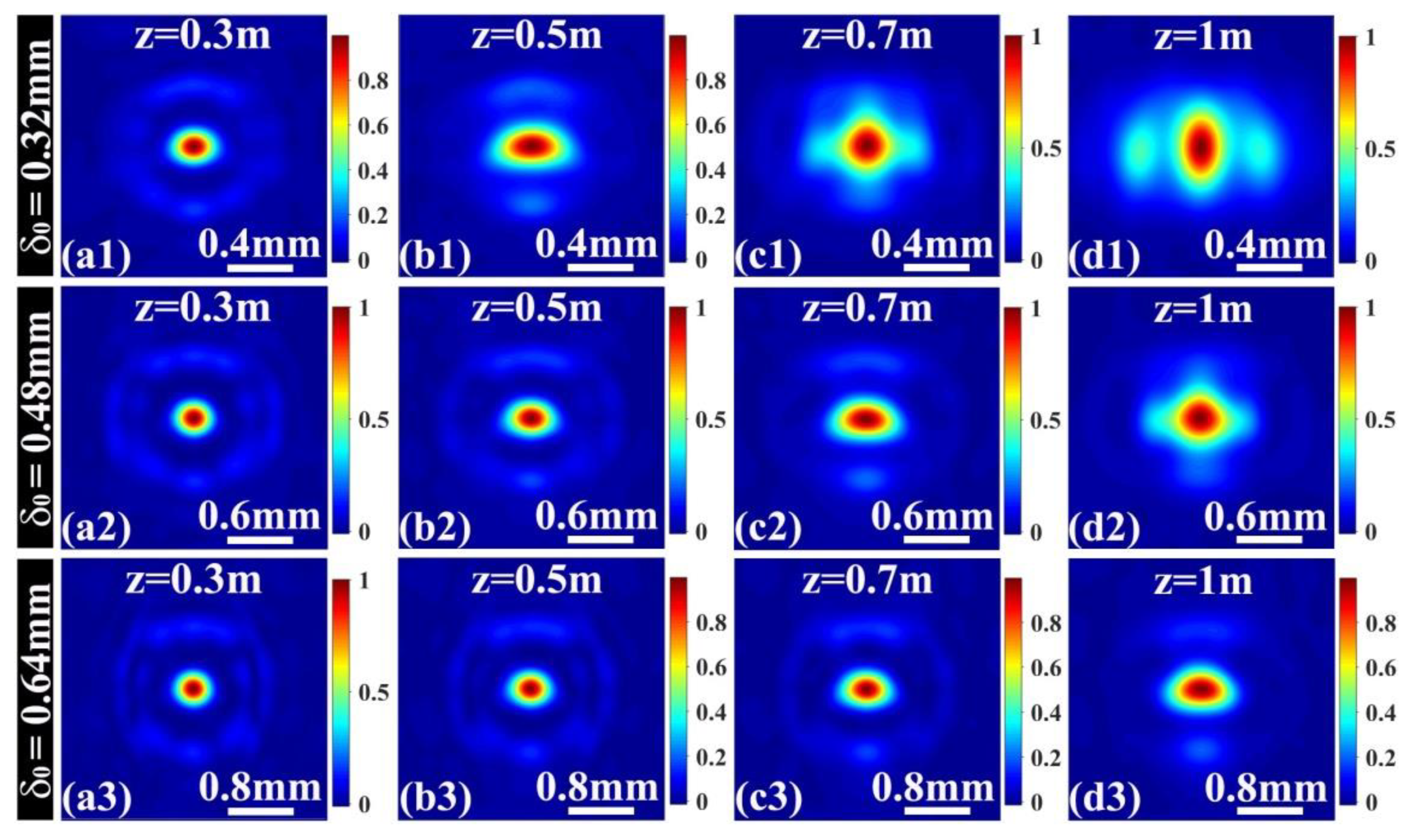
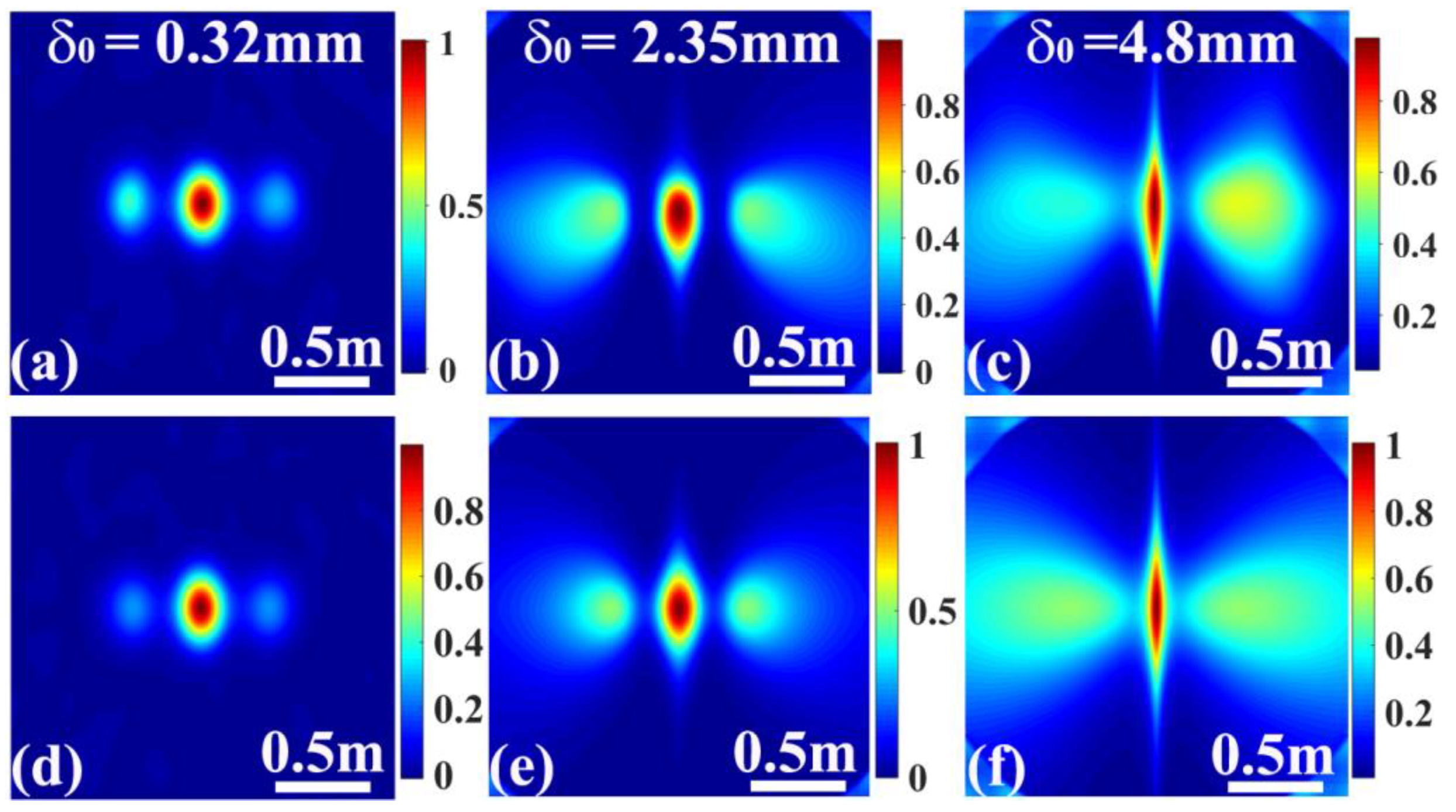
3. Simulation Results
4. Conclusions
Author Contributions
Funding
Conflicts of Interest
References
- Mandel, L.; Wolf, E. Optical Coherence and Quantum Optics; Cambridge University Press: New York, NY, USA, 1995; ISBN 9780521417112. [Google Scholar]
- Wolf, E. Introduction to the Theory of Coherence and Polarization of Light; Cambridge University Press: New York, NY, USA, 2007; ISBN 9780521822114. [Google Scholar]
- Cai, Y.; Wang, F.; Zhao, C.; Zhu, S.; Wu, G.; Dong, Y. Partially Coherent Vector Beams: From Theory to Experiment. In Vectorial Optical Fields: Fundamentals and Applications; Zhen, Q., Ed.; World Scientific: Singapore, 2013; ISBN 9789814449885. Chapter 7; pp. 221–273. [Google Scholar]
- Gbur, G.; Visser, T.D. The Structure of Partially Coherent Fields. Prog. Opt. 2010, 55, 285–341. [Google Scholar]
- Karamata, B.; Lambelet, P.; Laubscher, M.; Salathé, R.; Lasser, T. Spatially Incoherent Illumination as a Mechanism for Cross-Talk Suppression in Wide-Field Optical Coherence Tomography. Opt. Lett. 2004, 29, 736–738. [Google Scholar] [CrossRef]
- Zhao, C.; Cai, Y.; Lu, X.; Eyyuboğlu, H.T. Radiation Force of Coherent and Partially Coherent Flat-Topped Beams on a Rayleigh Particle. Opt. Express 2009, 17, 1753–1765. [Google Scholar] [CrossRef] [PubMed]
- Kato, Y.; Mima, K.; Miyanaga, N.; Arinaga, S.; Kitagawa, Y.; Nakatsuka, M.; Yamanaka, C. Random Phasing of High-Power Lasers for Uniform Target Acceleration and Plasma-Instability Suppression. Phys. Rev. Lett. 1984, 53, 1057–1060. [Google Scholar] [CrossRef]
- Douglass, K.M.; Sieben, C.; Archetti, A.; Lambert, A.; Manley, S. Super-Resolution Imaging of Multiple Cells by Optimised Flat-Field Epi-Illumination. Nat. Photonics 2016, 10, 705–708. [Google Scholar] [CrossRef] [PubMed]
- Van Dijk, T.; Fischer, D.G.; Visser, T.D.; Wolf, E. Effects of Spatial Coherence on the Angular Distribution of Radiant Intensity Generated by Scattering on a Sphere. Phys. Rev. Lett. 2010, 104, 173902. [Google Scholar] [CrossRef] [PubMed]
- Cai, Y.; Chen, Y.; Wang, F. Generation and Propagation of Partially Coherent Beams with Nonconventional Correlation Functions: A Review [Invited]. J. Opt. Soc. Am. A 2014, 31, 2083–2096. [Google Scholar] [CrossRef]
- Liang, C.; Wu, G.; Wang, F.; Li, W.; Cai, Y.; Ponomarenko, S.A. Overcoming the Classical Rayleigh Diffraction Limit by Controlling Two-Point Correlations of Partially Coherent Light Source. Opt. Express 2017, 25, 28352–28362. [Google Scholar] [CrossRef]
- Brown, D.P.; Brown, T.G. Partially Correlated Azimuthal Vortex Illumination: Coherence and Correlation Measurements and Effects in Imaging. Opt. Express 2008, 16, 20418–20426. [Google Scholar] [CrossRef]
- Wang, F.; Chen, Y.; Liu, X.; Cai, Y.; Ponomarenko, S.A. Self-Reconstruction of Partially Coherent Light Beams Scattered by Opaque Obstacles. Opt. Express 2016, 24, 23735–23746. [Google Scholar] [CrossRef]
- Liu, X.; Peng, X.; Liu, L.; Wu, G.; Zhao, C.; Wang, F.; Cai, Y. Self-Reconstruction of the Degree of Coherence of a Partially Coherent Vortex Beam Obstructed by an Opaque Obstacle. Appl. Phys. Lett. 2017, 110, 181104. [Google Scholar] [CrossRef]
- Gori, F.; Santarsiero, M. Devising Genuine Spatial Correlation Functions. Opt. Lett. 2007, 32, 3531–3533. [Google Scholar] [CrossRef]
- Gori, F.; Ramírez-Sánchez, V.; Santarsiero, M.; Shirai, T. On Genuine Cross-Spectral Density Matrices. J. Opt. A Pure Appl. Opt. 2009, 11, 085706. [Google Scholar] [CrossRef]
- Lajunen, H.; Saastamoinen, T. Propagation Characteristics of Partially Coherent Beams with Spatially Varying Correlations. Opt. Lett. 2011, 36, 4104–4106. [Google Scholar] [CrossRef]
- Sahin, S.; Korotkova, O. Light Sources Generating Far Fields with Tunable Flat Profiles. Opt. Lett. 2012, 37, 2970–2972. [Google Scholar] [CrossRef]
- Wang, F.; Liu, X.; Yuan, Y.; Cai, Y. Experimental Generation of Partially Coherent Beams with Different Complex Degrees of Coherence. Opt. Lett. 2013, 38, 1814–1816. [Google Scholar] [CrossRef]
- Ma, L.; Ponomarenko, S.A. Optical Coherence Gratings and Lattices. Opt. Lett. 2014, 39, 6656–6659. [Google Scholar] [CrossRef]
- Chen, Y.; Norrman, A.; Ponomarenko, S.A.; Friberg, A.T. Coherence Lattices in Surface Plasmon Polariton Fields. Opt. Lett. 2018, 43, 3429–3432. [Google Scholar] [CrossRef]
- Chen, Y.; Ponomarenko, S.A.; Cai, Y. Experimental Generation of Optical Coherence Lattices. Appl. Phys. Lett. 2016, 109, 061107. [Google Scholar] [CrossRef]
- Liu, X.; Wu, T.; Liu, L.; Zhao, C.; Cai, Y. Experimental Determination of the Azimuthal and Radial Mode Orders of a Partially Coherent LGpl Beam. Chin. Opt. Lett. 2017, 15, 030002. [Google Scholar]
- Hyde, M.; Bose-Pillai, S.; Voelz, D.G.; Xiao, X. Generation of Vector Partially Coherent Optical Sources Using Phase-Only Spatial Light Modulators. Phys. Rev. Appl. 2016, 6, 064030. [Google Scholar] [CrossRef]
- Chriki, R.; Nixon, M.; Pal, V.; Tradonsky, C.; Barach, G.; Friesem, A.A.; Davidson, N. Manipulating the Spatial Coherence of a Laser Source. Opt. Express 2015, 23, 12989–12997. [Google Scholar] [CrossRef]
- Lehtolahti, J.; Kuittinen, M.; Turunen, J.; Tervo, J. Coherence Modulation by Deterministic Rotating Diffusers. Opt. Express 2015, 23, 10453–10466. [Google Scholar] [CrossRef]
- Hyde, M.; Bose-Pillai, S.; Wood, R.A. Synthesis of Non-Uniformly Correlated Partially Coherent Sources Using a Deformable Mirror. Appl. Phys. Lett. 2017, 111, 101106. [Google Scholar] [CrossRef]
- Wu, T.; Liang, C.; Wang, F.; Cai, Y. Shaping the Intensity and Degree of Coherence of a Partially Coherent Beam by a 4f Optical System with an Amplitude Filter. J. Opt. 2017, 19, 124010. [Google Scholar] [CrossRef]
- Visser, T.D.; Agrawal, G.P.; Milonni, P.W. Fourier Processing with Partially Coherent Fields. Opt. Lett. 2017, 42, 4600–4602. [Google Scholar] [CrossRef]
- Liang, C.; Mi, C.; Wang, F.; Zhao, C.; Cai, Y.; Ponomarenko, S.A. Vector Optical Coherence Lattices Generating Controllable Far-Field Beam Profiles. Opt. Express 2017, 25, 9872–9885. [Google Scholar] [CrossRef]
- Zhu, S.; Wang, J.; Liu, X.; Cai, Y.; Li, Z. Generation of Arbitrary Radially Polarized Array Beams by Manipulating Correlation Structure. Appl. Phys. Lett. 2016, 109, 161904. [Google Scholar] [CrossRef]
- Mei, Z.; Korotkova, O. Sources for Random Arrays with Structured Complex of Coherence. Opt. Lett. 2018, 43, 2676–2679. [Google Scholar] [CrossRef]
- Liang, C.; Zhu, X.; Mi, C.; Peng, X.; Wang, F.; Cai, Y.; Ponomarenko, S.A. High-Quality Partially Coherent Bessel Beam Array Generation. Opt. Lett. 2018, 43, 3188–3191. [Google Scholar] [CrossRef]
- Liu, X.; Liu, L.; Peng, X.; Liu, L.; Wang, F.; Gao, Y.; Cai, Y. Partially coherent vortex beam with periodical coherence properties. J. Quant. Spectrosc. Radiat. Transf. 2019, 222–223, 138–144. [Google Scholar] [CrossRef]
- Carter, W.H.; Wolf, E. Coherence and Radiometry with Quasihomogeneous Planar Sources. J. Opt. Soc. Am. 1977, 67, 785–796. [Google Scholar] [CrossRef]
- Raghunathan, S.B.; Visser, T.D.; Wolf, E. Far-Zone Properties of Electromagnetic Beams Generated by Quasi-Homogeneous Sources. Opt. Commun. 2013, 295, 11–16. [Google Scholar] [CrossRef]
- Voelz, D.; Xiao, X.; Korotkova, O. Numerical Modeling of Schell-Model Beams with Arbitrary Far-Field Patterns. Opt. Lett. 2015, 40, 352–355. [Google Scholar] [CrossRef]
- Goodman, J.W. Statistical Optics, 2nd ed.; Wiley: New York, NY, USA, 1985; ISBN 9781119009450. [Google Scholar]
- Dainty, J.C. Laser Speckle and Related Phenomena; Spring-Verlag: Berlin, Germany, 1975; ISBN 9783662432051. [Google Scholar]
- Wang, F.; Korotkova, O. Convolution Approach for Beam Propagation in Random Media. Opt. Lett. 2016, 41, 1546–1549. [Google Scholar] [CrossRef]
- Schmidt, J.D. Numerical Simulation of Optical Wave Propagation with Examples in MATLAB; SPIE Press: Washington, DC, USA, 2010; ISBN 9780819483263. [Google Scholar]
- Wang, F.; Cai, Y.; Eyyuboğlu, H.T.; Baykal, Y.; Çil, C.Z. Partially Coherent Elegant Hermite-Gaussian Beams. Appl. Phys. B 2010, 100, 617–626. [Google Scholar] [CrossRef]
- Pauwels, J.; Verschaffelt, G. Speckle Reduction in Laser Projection Using Microlens-Array Screens. Opt. Express 2017, 25, 3180–3195. [Google Scholar] [CrossRef]

© 2019 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Ma, P.; Kacerovská, B.; Khosravi, R.; Liang, C.; Zeng, J.; Peng, X.; Mi, C.; Monfared, Y.E.; Zhang, Y.; Wang, F.; et al. Numerical Approach for Studying the Evolution of the Degrees of Coherence of Partially Coherent Beams Propagation through an ABCD Optical System. Appl. Sci. 2019, 9, 2084. https://doi.org/10.3390/app9102084
Ma P, Kacerovská B, Khosravi R, Liang C, Zeng J, Peng X, Mi C, Monfared YE, Zhang Y, Wang F, et al. Numerical Approach for Studying the Evolution of the Degrees of Coherence of Partially Coherent Beams Propagation through an ABCD Optical System. Applied Sciences. 2019; 9(10):2084. https://doi.org/10.3390/app9102084
Chicago/Turabian StyleMa, Pujuan, Barbora Kacerovská, Raha Khosravi, Chunhao Liang, Jun Zeng, Xiaofeng Peng, Chenkun Mi, Yashar E. Monfared, Yuping Zhang, Fei Wang, and et al. 2019. "Numerical Approach for Studying the Evolution of the Degrees of Coherence of Partially Coherent Beams Propagation through an ABCD Optical System" Applied Sciences 9, no. 10: 2084. https://doi.org/10.3390/app9102084
APA StyleMa, P., Kacerovská, B., Khosravi, R., Liang, C., Zeng, J., Peng, X., Mi, C., Monfared, Y. E., Zhang, Y., Wang, F., & Cai, Y. (2019). Numerical Approach for Studying the Evolution of the Degrees of Coherence of Partially Coherent Beams Propagation through an ABCD Optical System. Applied Sciences, 9(10), 2084. https://doi.org/10.3390/app9102084








