Comparison Between Longitudinal and Transverse Shaking of Culvert–Frame Combined Underground Structure
Abstract
1. Introduction
2. Shaking Table Test
2.1. Testing System
2.2. Longitudinal and Transverse Equivalent Design of the Model Structure
2.3. Instrumentation
2.4. Earthquake Motions and Testing Cases
3. Comparisons of Soil Acceleration
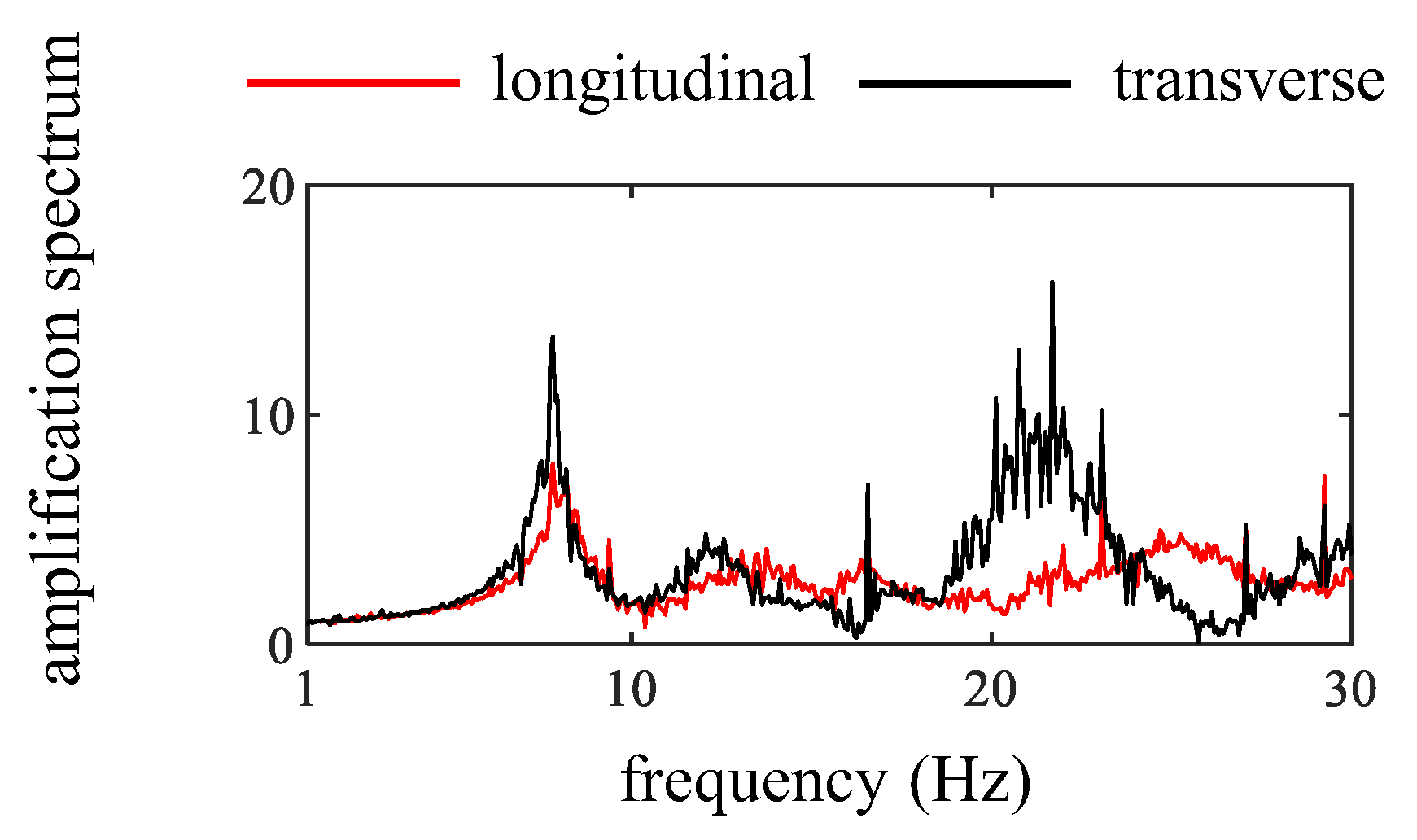
3.1. Acceleration Amplification Spectra
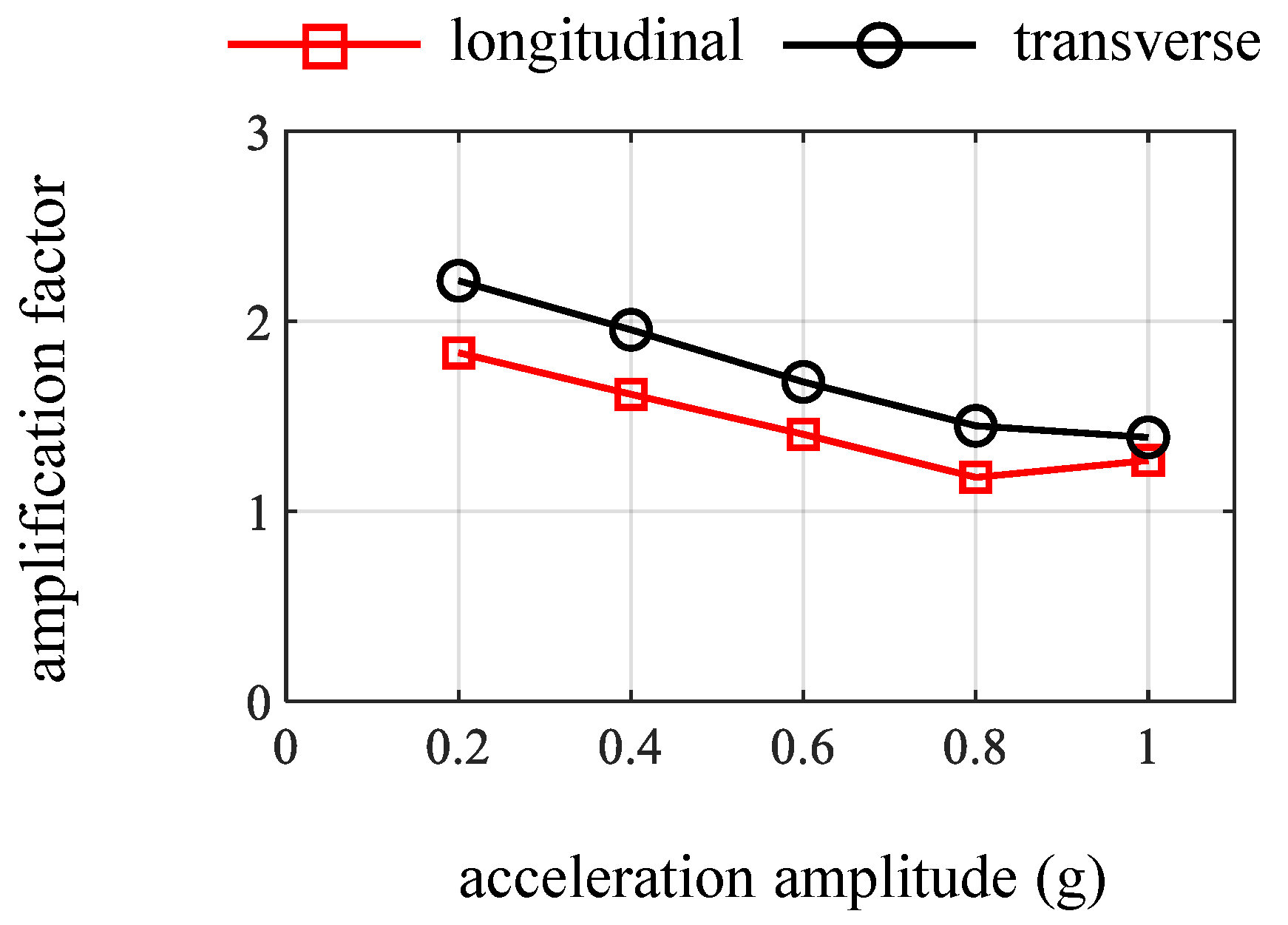
3.2. Peak Acceleration Amplification Factors
4. Comparisons of Box Frame Acceleration
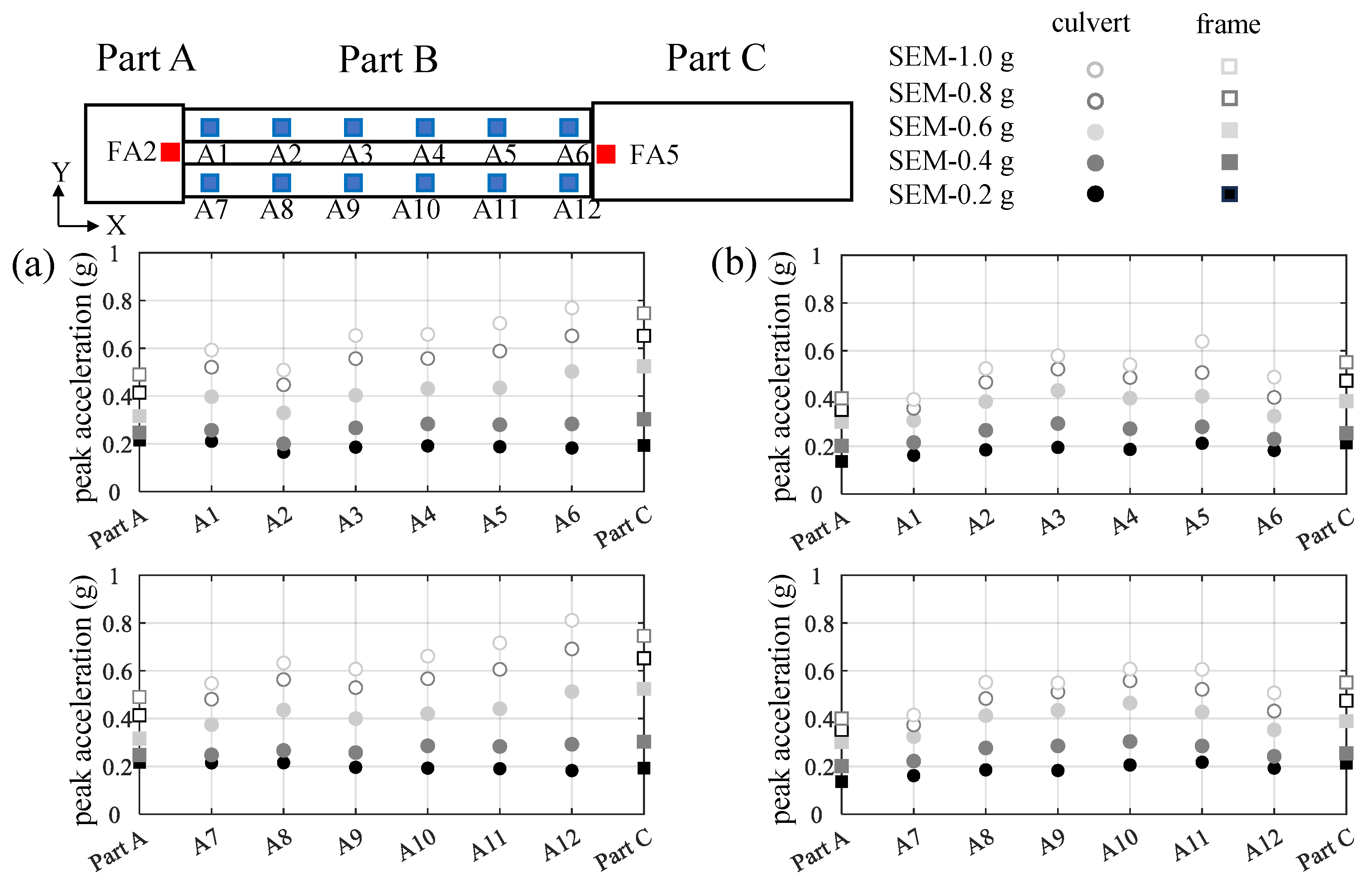
4.1. Peak Acceleration Amplification Factors of Frames
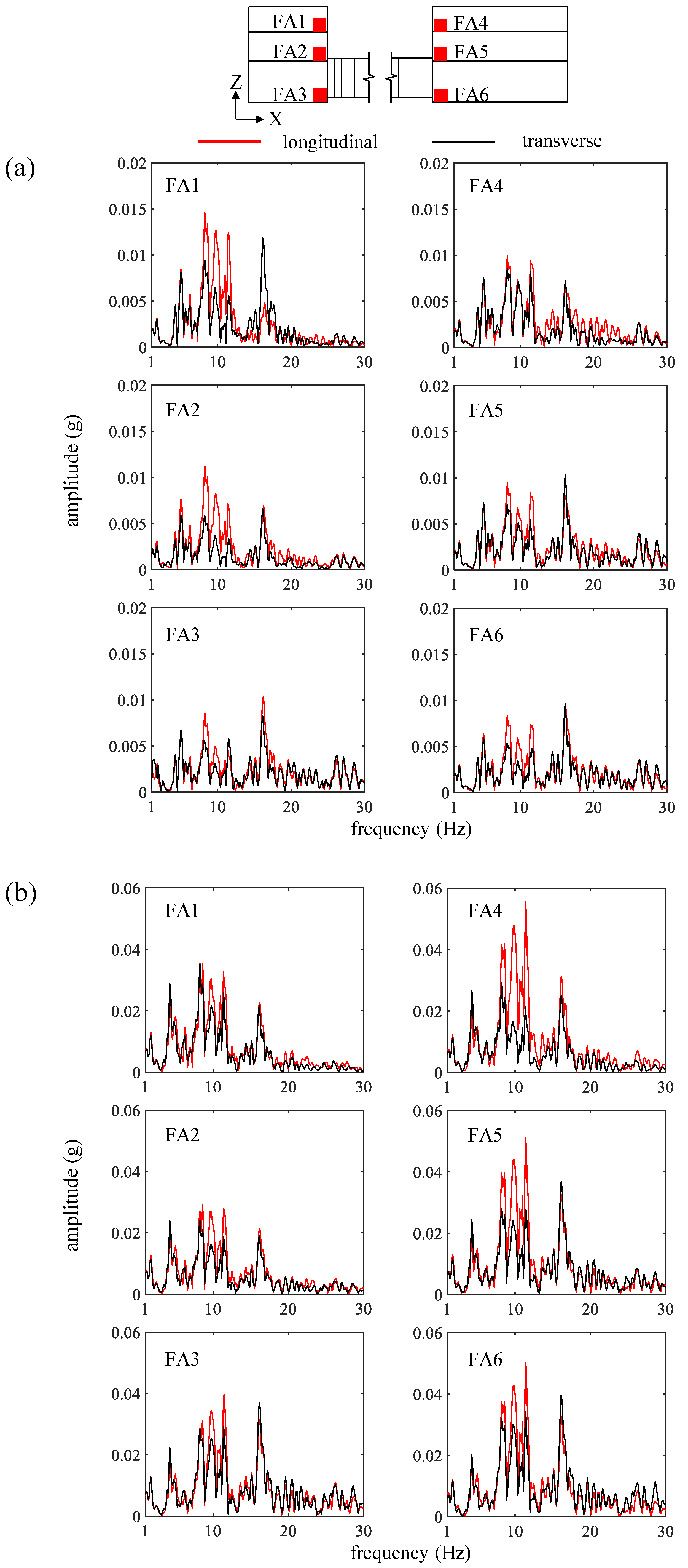
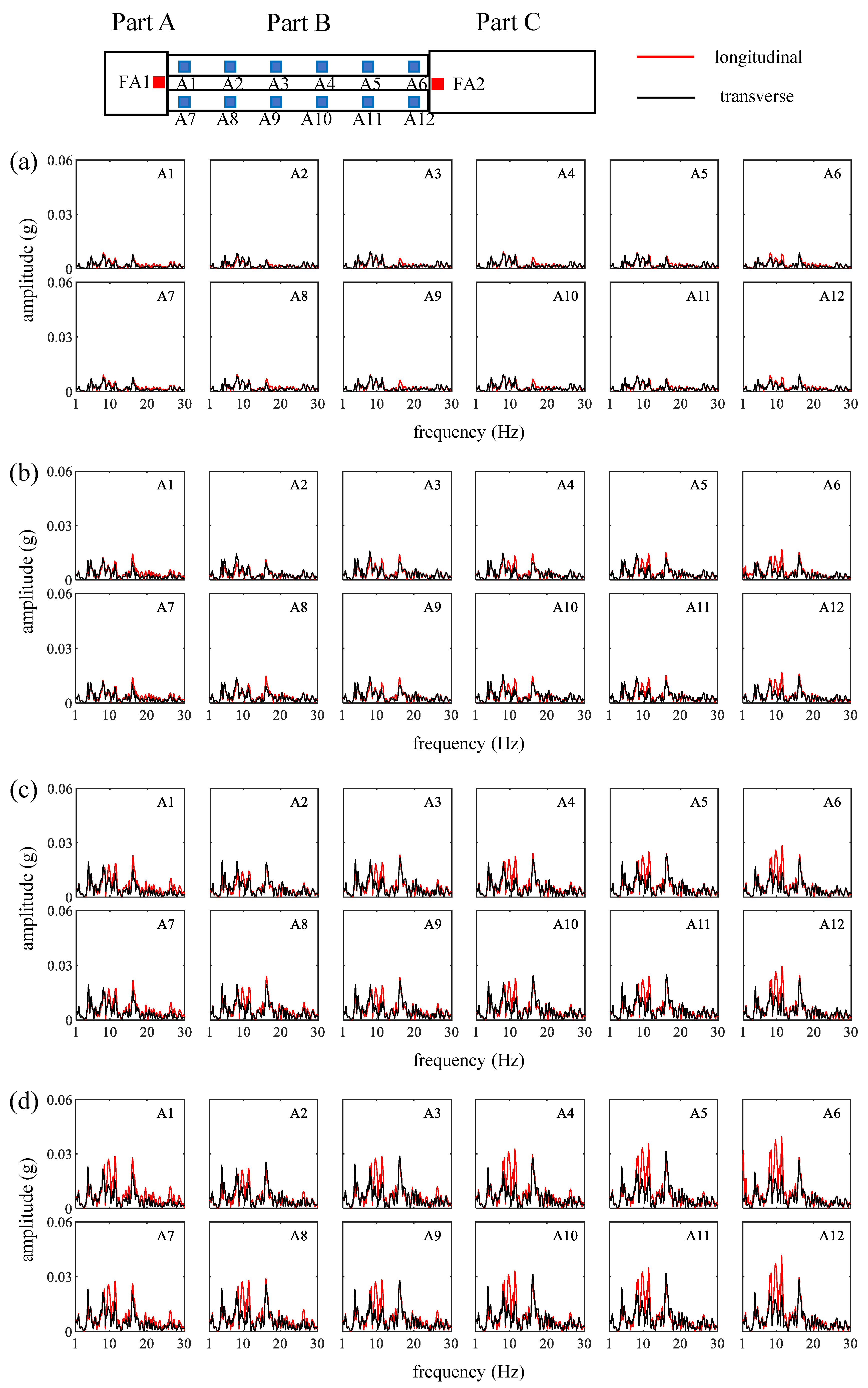
4.2. Acceleration Fourier Spectra of Frames
5. Comparisons of Culvert Acceleration
5.1. Peak Acceleration of Culverts
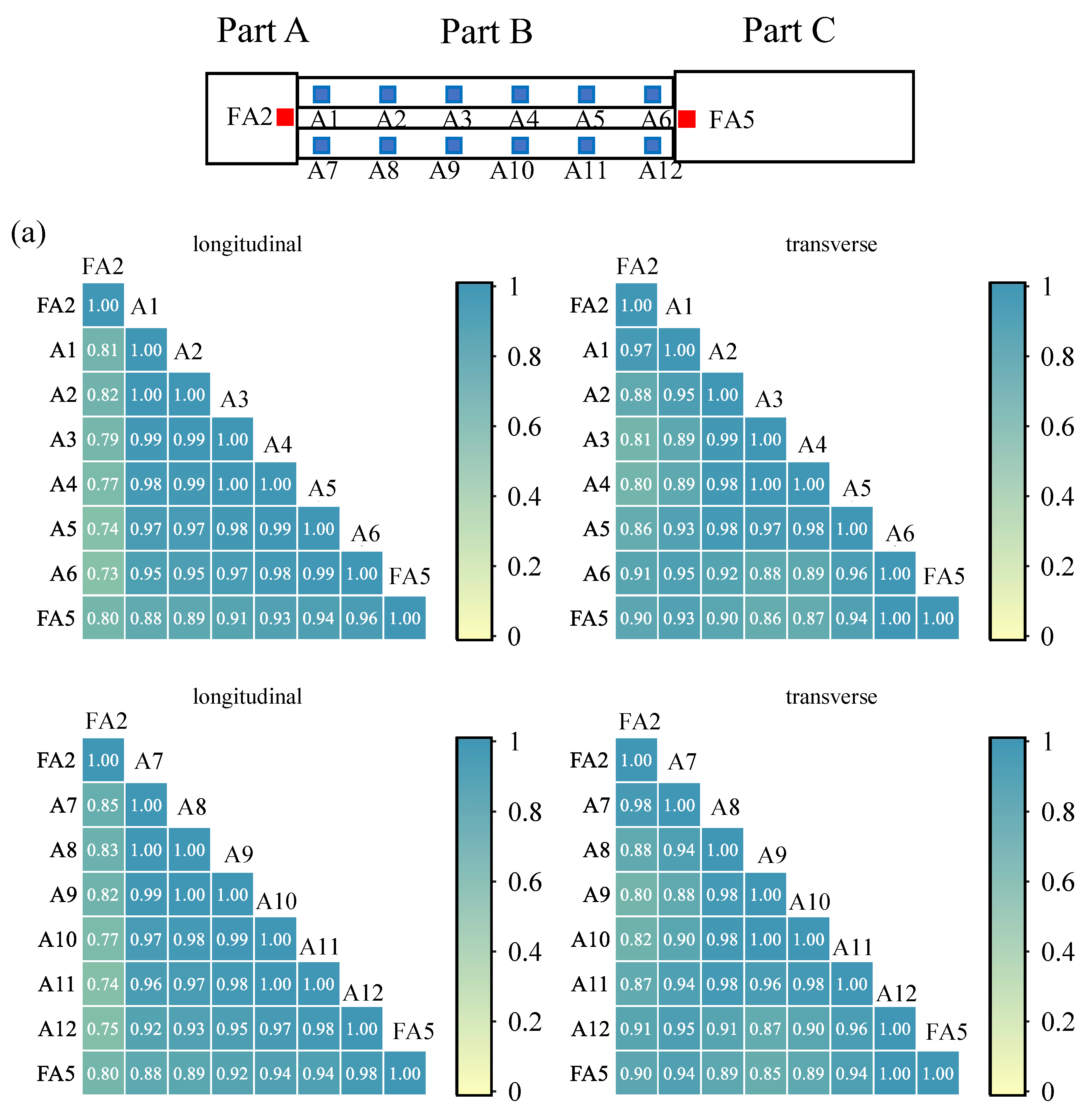
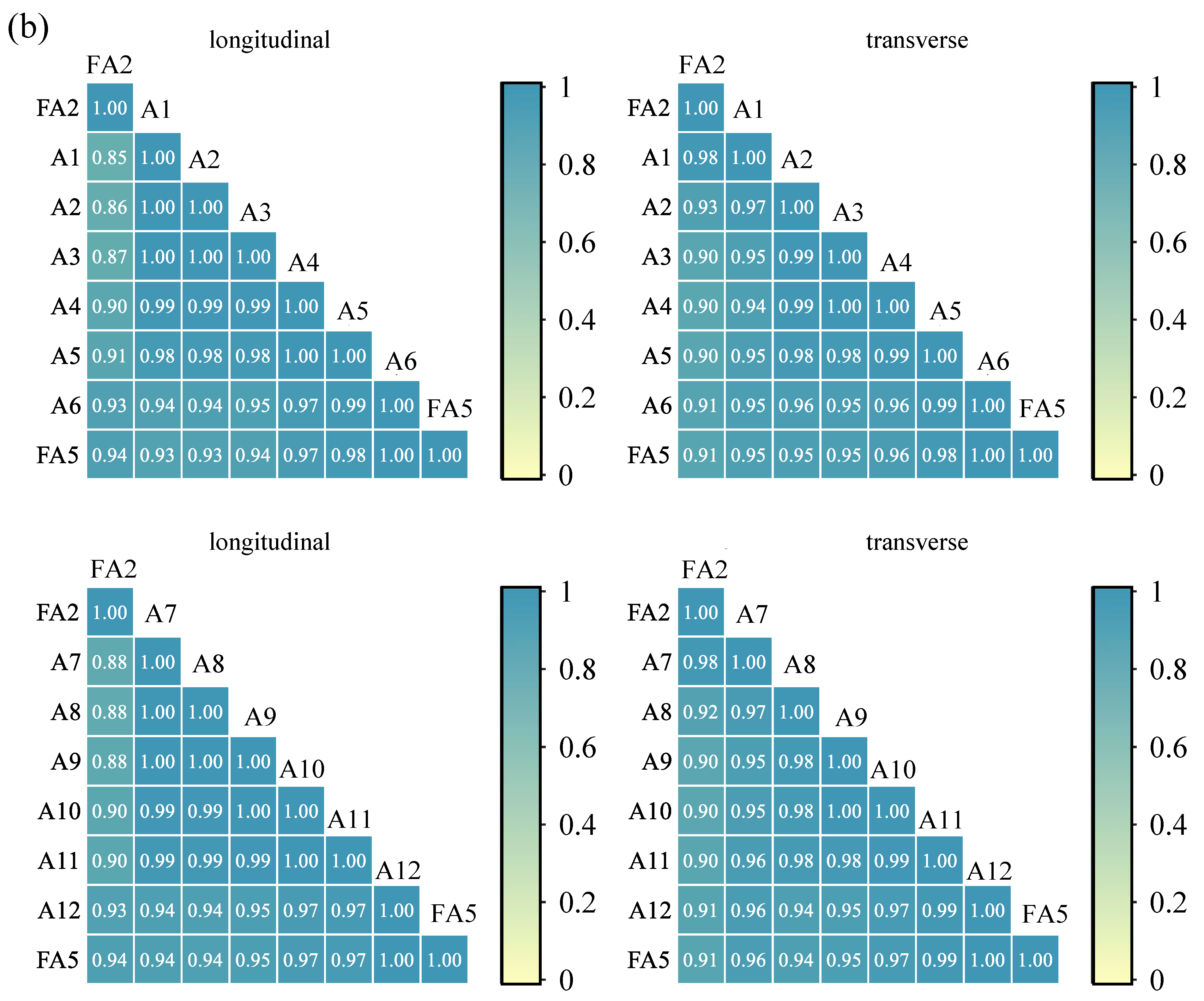
5.2. Acceleration Correlation Coefficient of Culverts
5.3. Acceleration Fourier Spectra of Culverts
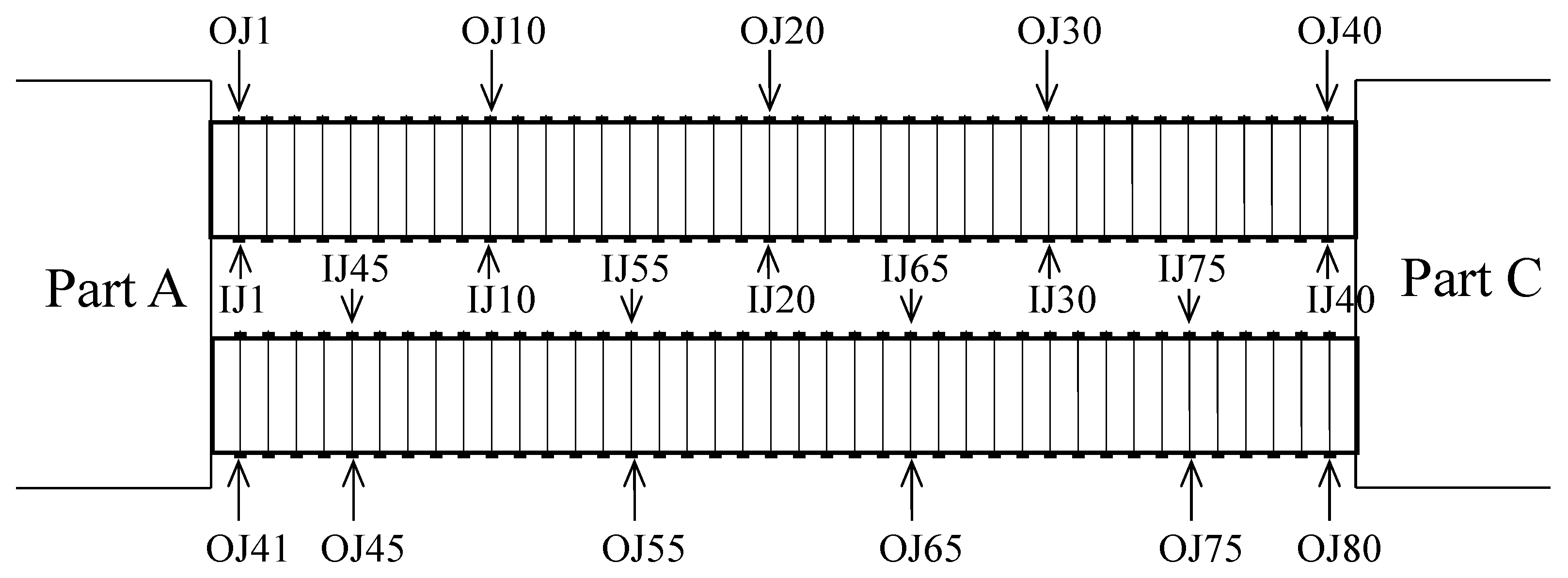
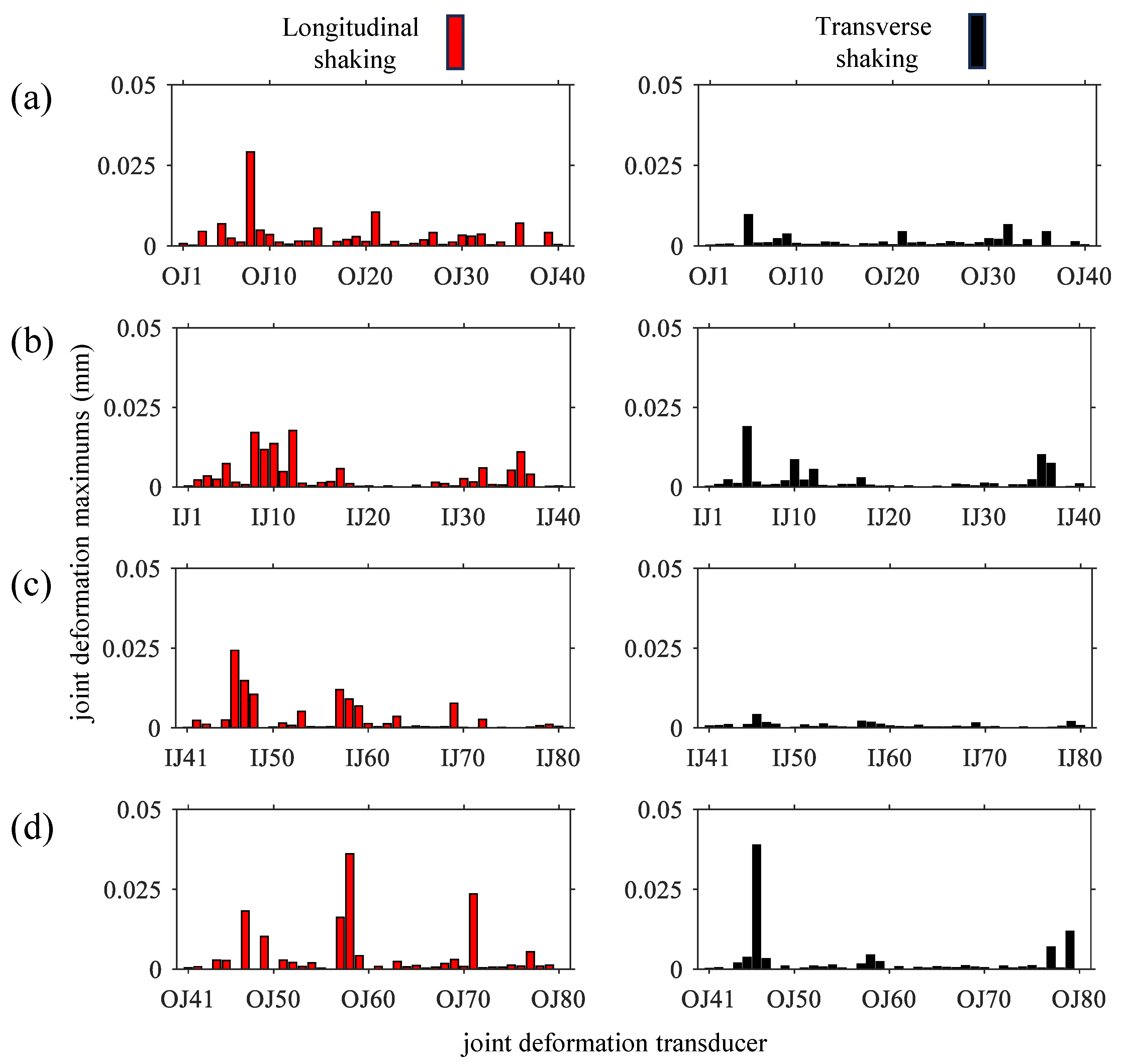
6. Culvert Joint Deformation
7. Conclusions
- (1)
- The ground surface exhibits the same dominant frequency but different amplitudes under the longitudinal and transverse excitation in the WN case. From both time and frequency domain perspectives, the acceleration responses of the model soil are greater under transverse excitations than under longitudinal excitations.
- (2)
- Under transverse excitations, different floors of the frames (Part A and Part B) have more consistent peak accelerations than those under longitudinal excitation. The longitudinal excitations enlarge the acceleration component in the frequency range of 8 Hz to 12 Hz compared with the transverse excitations.
- (3)
- The peak accelerations of culverts increase from the end near Part A to the end near Part C under longitudinal excitations. The peak accelerations in the center of the culvert are significantly greater than those at both ends under transverse excitations. Moreover, correlation coefficients of the accelerograms statistically quantify the discrepant acceleration responses in the center of the culvert under transverse excitations. The acceleration component in the frequency range of 8 Hz to 12 Hz under longitudinal excitations is greater than that under transverse excitations.
- (4)
- The distribution of culvert joint deformations is spiky, i.e., several culvert joints have deformations much larger than the average value. The culvert joint deformations are greater under longitudinal excitations than under transverse excitations. The spiky culvert joint deformations tend to occur at the ends of culverts under transverse excitations, while there is no significant trend of spiky deformation locations under longitudinal excitations. The joints at culvert ends have greater deformations because the frames’ restrained effect on the culverts is negatively correlated to the distance. The enhancement of the joints at culvert ends is necessary.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- He, J.; Liao, S.; Gong, Z.; Tang, C.; Liu, M.; Li, Y. The Design and Construction of a Large Composite Subway Station in Limited Space: An Exemplary Application of OCUE Method in Xuzhou, China. IOP Conf. Ser. Earth Environ. Sci. 2021, 703, 012024. [Google Scholar] [CrossRef]
- Iegupov, V.; Strizhelchik, G.; Kupreychyk, A.; Ubiyvovk, A. Geological Hazards During Construction and Operation of Shallow Subway Stations and Tunnels by the Example of the Kharkiv Metro (1968–2018). Int. J. Georesour. Environ. 2018, 4, 187–200. [Google Scholar] [CrossRef]
- Yang, Y.-F.; Liao, S.-M.; Liu, M.-B.; Wu, D.-P.; Pan, W.-Q.; Li, H. A New Construction Method for Metro Stations in Dense Urban Areas in Shanghai Soft Ground: Open-Cut Shafts Combined with Quasi-Rectangular Jacking Boxes. Tunn. Undergr. Space Technol. 2022, 125, 104530. [Google Scholar] [CrossRef]
- Zhuang, H.; Chen, G.; Hu, Z.; Qi, C. Influence of Soil Liquefaction on the Seismic Response of a Subway Station in Model Tests. Bull. Eng. Geol. Environ. 2016, 75, 1169–1182. [Google Scholar] [CrossRef]
- Liu, Z.; Chen, Z.; Liang, S.; Li, C. Isolation Mechanism of a Subway Station Structure with Flexible Devices at Column Ends Obtained in Shaking-Table Tests. Tunn. Undergr. Space Technol. 2020, 98, 103328. [Google Scholar] [CrossRef]
- Wang, J.; Yang, J.; Zhuang, H.; Ma, G.; Sun, Y. Seismic Responses of a Large Unequal-Span Underground Subway Station in Liquefiable Soil Using Shaking Table Test. J. Earthq. Eng. 2021, 26, 8446–8467. [Google Scholar] [CrossRef]
- Xu, C.; Zhang, Z.; Li, Y.; Du, X. Seismic Response and Failure Mechanism of Underground Frame Structures Based on Dynamic Centrifuge Tests. Earthq. Eng. Struct. Dyn. 2021, 50, 2031–2048. [Google Scholar] [CrossRef]
- Zhang, Z.; Yu, H.; Wang, Z.; Song, Y.; Liu, S.; Jiang, W. Shaking Table Test for Near-Valley Subway Station—Part I: Seismic Site Effects. Soil Dyn. Earthq. Eng. 2023, 172, 108064. [Google Scholar] [CrossRef]
- Yu, H.; Wang, Z.; Zhang, Z.; Song, Y.; Liu, S.; Jiang, W. Shaking Table Test for Near-Valley Subway Station—Part II: Seismic Response of the Station. Soil Dyn. Earthq. Eng. 2023, 172, 108065. [Google Scholar] [CrossRef]
- Maleska, T.; Beben, D.; Vaslestad, J.; Sergei Sukuvara, D. Application of EPS Geofoam below Soil–Steel Composite Bridge Subjected to Seismic Excitations. J. Geotech. Geoenviron. Eng. 2024, 150, 04024115. [Google Scholar] [CrossRef]
- Maleska, T.; Beben, D. Effect of the Soil Cover Depth on the Seismic Response in a Large-Span Thin-Walled Corrugated Steel Plate Bridge. Soil Dyn. Earthq. Eng. 2023, 166, 107744. [Google Scholar] [CrossRef]
- Maleska, T.; Beben, D.; Nowacka, J. Seismic Vulnerability of a Soil-Steel Composite Tunnel—Norway Tolpinrud Railway Tunnel Case Study. Tunn. Undergr. Space Technol. 2021, 110, 103808. [Google Scholar] [CrossRef]
- Han, J.; Guo, Z.; El Naggar, M.H.; Li, L.; Wang, X.; Du, X. Response of Buried Pipeline to Non-Uniform Longitudinal and Transverse Ground Motions. J. Earthq. Eng. 2023, 28, 799–818. [Google Scholar] [CrossRef]
- Yatsumoto, H.; Mitsuyoshi, Y.; Sawamura, Y.; Kimura, M. Evaluation of Seismic Behavior of Box Culvert Buried in the Ground through Centrifuge Model Tests and Numerical Analysis. Undergr. Space 2019, 4, 147–167. [Google Scholar] [CrossRef]
- Saito, K.; Yamane, K.; Koizumi, A. A Study on the Seismic Behavior of Shield Tunnel Including Shaft in Longitudinal Direction. J. Tunn. Eng. 2006, 16, 121–132. [Google Scholar] [CrossRef]
- Towhata, I.; Kawamata, Y.; Nakayama, M.; Yasuda, S. E-Defense Shaking Test on Large Model of Underground Shaft and Tunnels. In Proceedings of the Geotechnical Aspects of Underground Construction in Soft Ground, Seoul, Republic of Korea, 25–27 August 2014; Yoo, C., Park, S.W., Kim, B., Ban, H., Eds.; CRC Press-Taylor & Francis Group: Boca Raton, FL, USA, 2014; pp. 31–40. [Google Scholar]
- Kawamata, Y.; Nakayama, M.; Towhata, I.; Yasuda, S. Dynamic Behaviors of Underground Structures in E-Defense Shaking Experiments. Soil Dyn. Earthq. Eng. 2016, 82, 24–39. [Google Scholar] [CrossRef]
- Ma, X.; Wang, G.; Wu, J.; Ji, Q. Experimental Study on the Seismic Response of Subway Station in Soft Ground. J. Earthq. Tsunami 2017, 11, 1750020. [Google Scholar] [CrossRef]
- Zhuang, H.; Wang, X.; Miao, Y.; Yao, E.; Chen, S.; Ruan, B.; Chen, G. Seismic Responses of a Subway Station and Tunnel in a Slightly Inclined Liquefiable Ground through Shaking Table Test. Soil Dyn. Earthq. Eng. 2019, 116, 371–385. [Google Scholar] [CrossRef]
- Zhang, J.; Yuan, Y.; Yu, H. Shaking Table Tests on Discrepant Responses of Shaft-Tunnel Junction in Soft Soil under Transverse Excitations. Soil Dyn. Earthq. Eng. 2019, 120, 345–359. [Google Scholar] [CrossRef]
- Chen, Q.; Zhang, T.; Hong, N.; Huang, B. Seismic Performance of a Subway Station-Tunnel Junction Structure: A Shaking Table Investigation and Numerical Analysis. KSCE J. Civ. Eng. 2021, 25, 1653–1669. [Google Scholar] [CrossRef]
- Wang, X.; El Naggar, H.M.; Xu, C.; Zhong, Z.; Du, X. Seismic Response of Subway Station-Tunnel with Expansion Joint Connection in Liquefiable Soil. Tunn. Undergr. Space Technol. 2025, 159, 106477. [Google Scholar] [CrossRef]
- Zhang, J.; Yuan, Y.; Bao, Z.; Yu, H.; Bilotta, E. Shaking Table Tests on the Intersection of Cross Passage and Twin Tunnels. Soil Dyn. Earthq. Eng. 2019, 124, 136–150. [Google Scholar] [CrossRef]
- Zhang, J.; Yuan, Y.; Bao, Z.; Yu, H.; Bilotta, E. Shaking Table Tests on Shaft-Tunnel Junction under Longitudinal Excitations. Soil Dyn. Earthq. Eng. 2020, 132, 106055. [Google Scholar] [CrossRef]
- Wang, J.-N. Seismic Design of Tunnels A Simple State-of-the-Art Design Approach; Parsons Brinckerhoff Quade and Douglas Inc.: New York, NY, USA, 1993. [Google Scholar]
- Shiba, Y.; Kawashima, K.; Obinata, N.; Kano, T. An Evaluation Method of Longitudinal Stiffness of Shield Tunnel Linings for Application to Seismic Response Analyses. Doboku Gakkai Ronbunshu 1988, 1988, 319–327. [Google Scholar] [CrossRef]
- Kramer, S.L. Geotechnical Earthquake Engineering, 1st ed.; Pearson: Upper Saddle River, NJ, USA, 1996; ISBN 978-0-13-374943-4. [Google Scholar]
- Abuhajar, O.; El Naggar, H.; Newson, T. Experimental and Numerical Investigations of the Effect of Buried Box Culverts on Earthquake Excitation. Soil Dyn. Earthq. Eng. 2015, 79, 130–148. [Google Scholar] [CrossRef]
- Tsinidis, G.; Heron, C.; Madabhushi, G.; Pitilakis, K. Calibration of Strain Gauged Square Tunnels for Centrifuge Testing. Geotech. Geol. Eng. 2016, 34, 911–921. [Google Scholar] [CrossRef]
- Tsinidis, G.; Pitilakis, K.; Madabhushi, G.; Heron, C. Dynamic Response of Flexible Square Tunnels: Centrifuge Testing and Validation of Existing Design Methodologies. Géotechnique 2015, 65, 401–417. [Google Scholar] [CrossRef]
- Tsinidis, G.; Rovithis, E.; Pitilakis, K.; Chazelas, J.L. Seismic Response of Box-Type Tunnels in Soft Soil: Experimental and Numerical Investigation. Tunn. Undergr. Space Technol. 2016, 59, 199–214. [Google Scholar] [CrossRef]
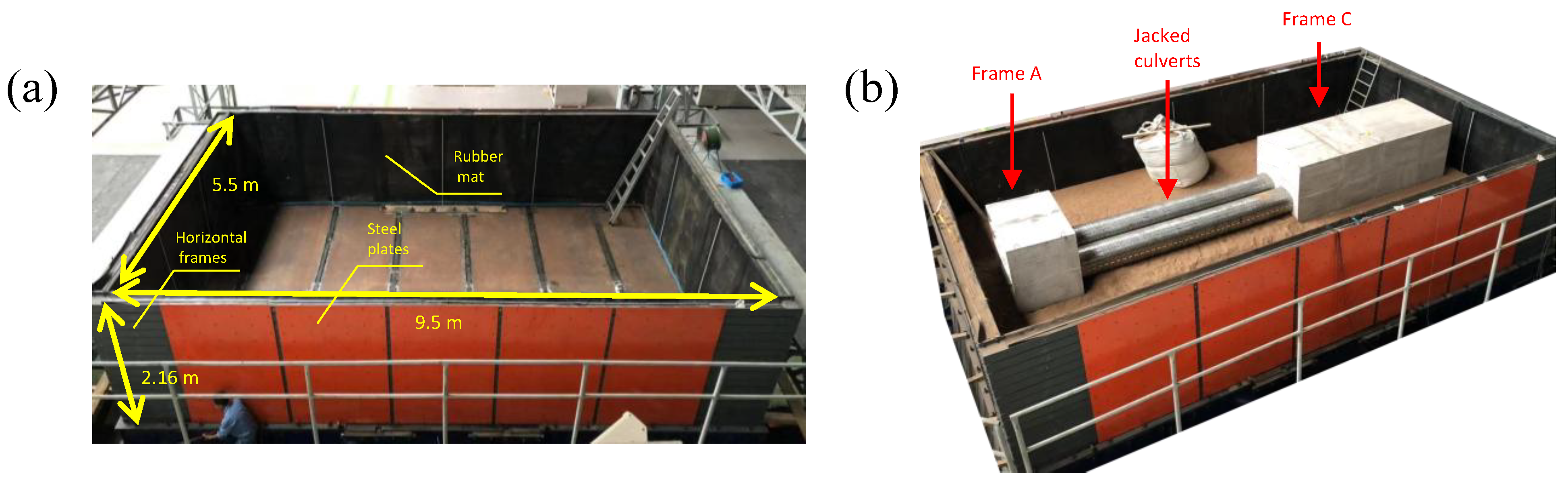
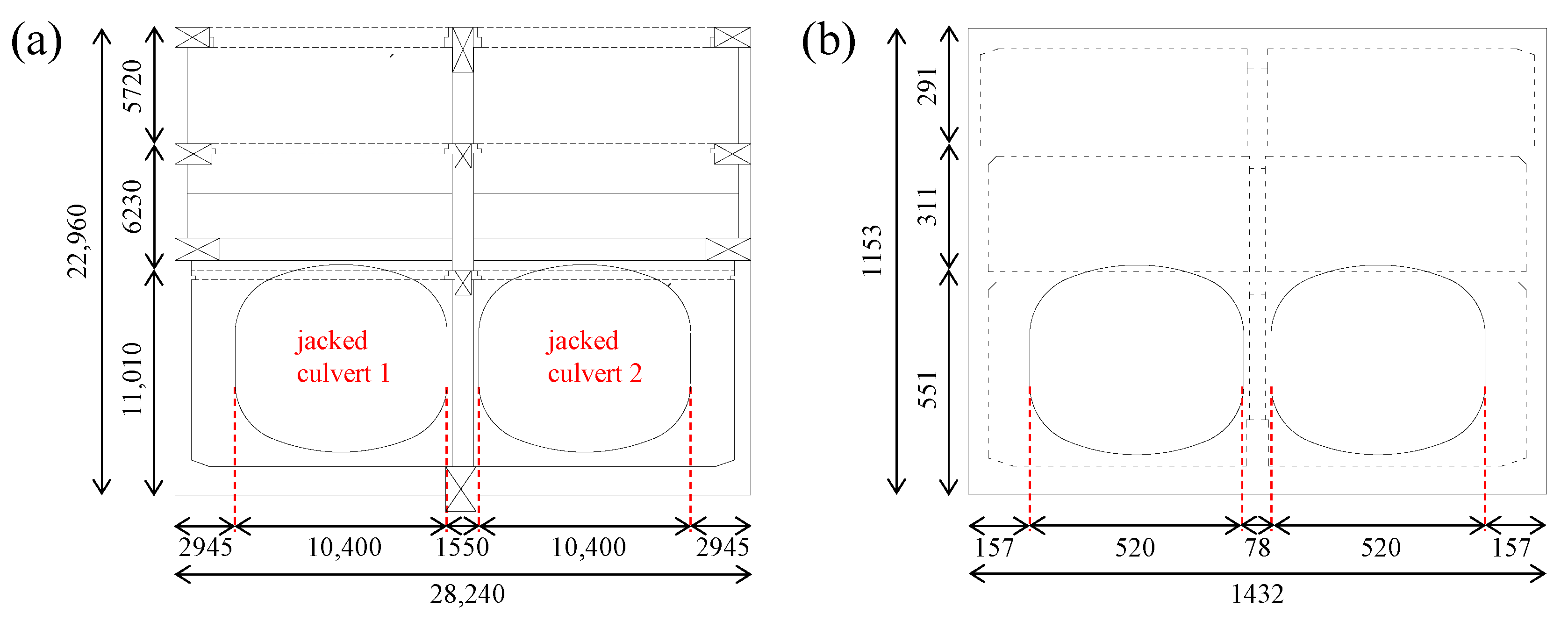

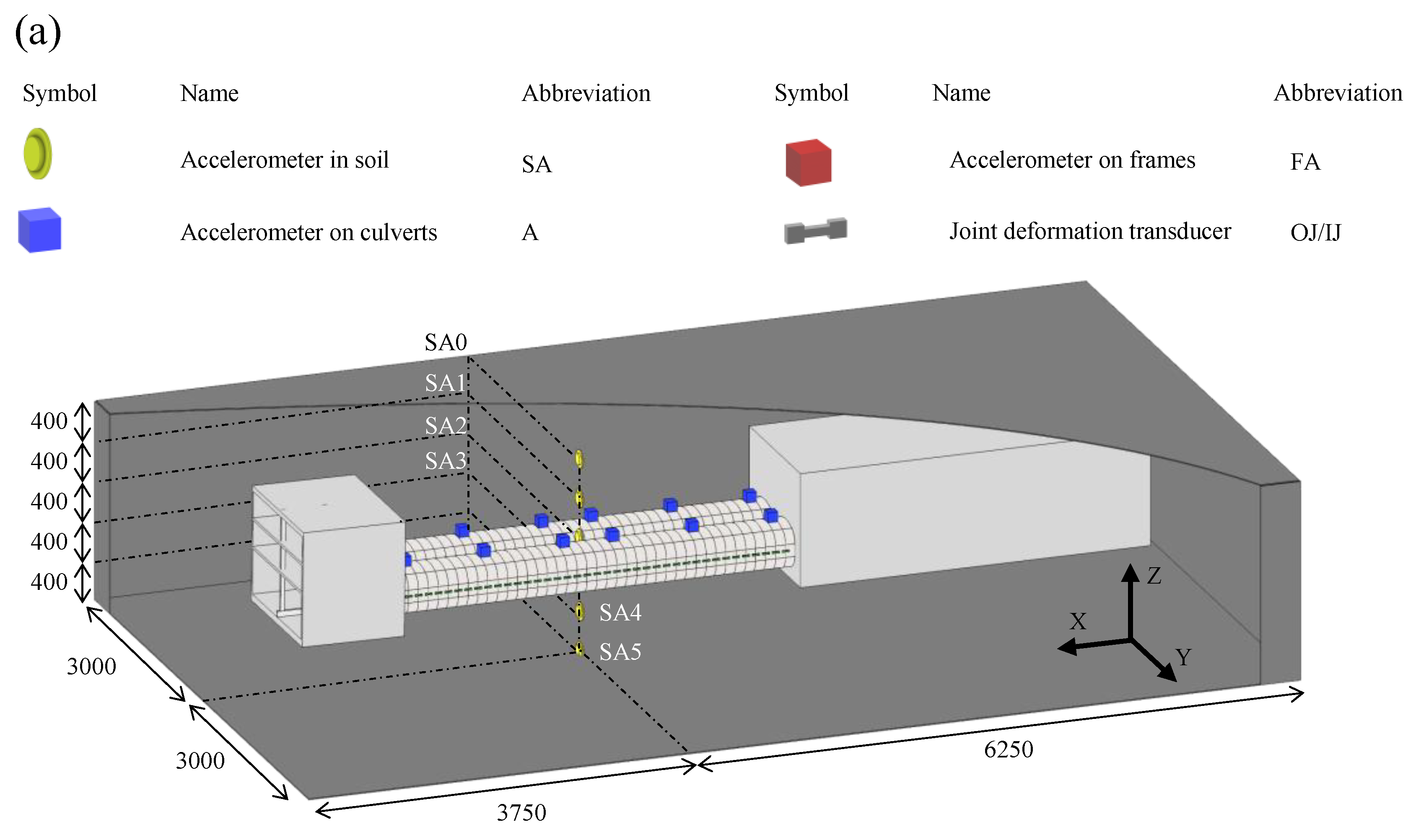
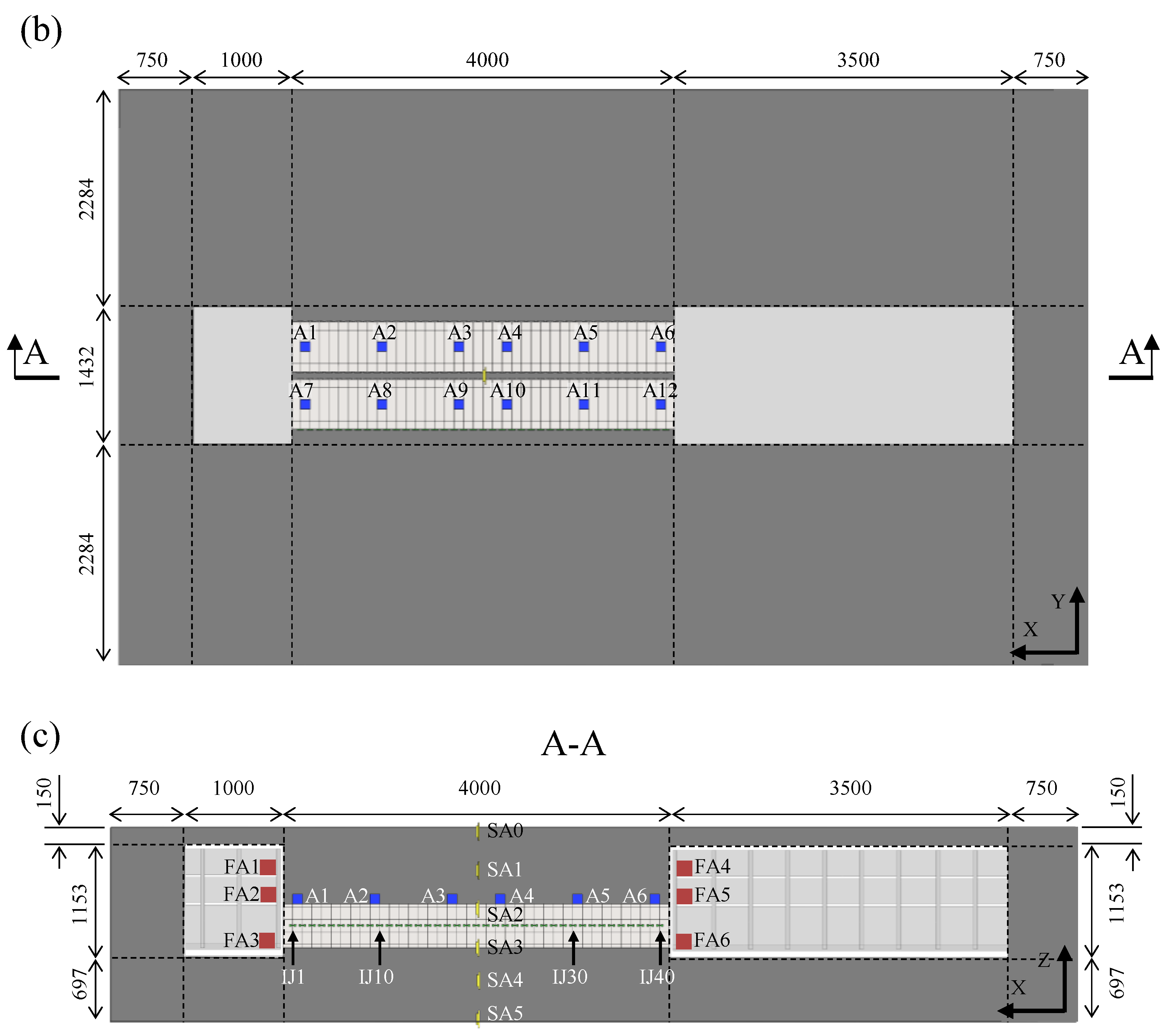




| Variables | Similitude Relations | Similitude Ratios |
|---|---|---|
| Displacement | 1/20 | |
| Density | 1/1.95 | |
| Shear modulus | 1/39 | |
| Acceleration | 1 | |
| Time | 1/4.47 |
| Properties | Depth (m) | Density (kg/m3) | Shear Modulus (Mpa) |
|---|---|---|---|
| Prototype soil | 40 | 1802 | 72 |
| Model soil | 2 | 694 | 2.84 |
| Prototype | Model | |
|---|---|---|
| Tensile stiffness of each culvert ring (N) | ||
| Number of longitudinal bolts | ||
| Tensile stiffness of each bolt (N) | ||
| Equivalent tensile stiffness of each joint (N) | ||
| Relative tensile stiffness | 68.1 |
| Prototype | Model | |
|---|---|---|
| Bending stiffness of each culvert ring (N × m2) | ||
| Equivalent bending stiffness of each joint (N × m2) | ||
| Relative bending stiffness | 64.0 |
| Prototype | Model | |
|---|---|---|
| Tensile stiffness of the frame (N) | ||
| Number of longitudinal bolts | ||
| Tensile stiffness of each bolt (N) | ||
| Equivalent tensile stiffness of the culvert (N) | ||
| Relative tensile stiffness |
| Symbol | Earthquake Wave | Acceleration Amplitude (g) | Shaking Direction | |
|---|---|---|---|---|
| Case 1 | WN-0.05g | white noise | 0.05 | X and Y |
| Case 2 | SEM-0.2g | synthetic earthquake motion | 0.2 | X and Y |
| Case 3 | SEM-0.4g | synthetic earthquake motion | 0.4 | X and Y |
| Case 4 | SEM-0.6g | synthetic earthquake motion | 0.6 | X and Y |
| Case 5 | SEM-0.8g | synthetic earthquake motion | 0.8 | X and Y |
| Case 6 | SEM-1.0g | synthetic earthquake motion | 1.0 | X and Y |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Yuan, Y.; Lan, X.; Wu, W.; Wang, X. Comparison Between Longitudinal and Transverse Shaking of Culvert–Frame Combined Underground Structure. Appl. Sci. 2025, 15, 5164. https://doi.org/10.3390/app15095164
Yuan Y, Lan X, Wu W, Wang X. Comparison Between Longitudinal and Transverse Shaking of Culvert–Frame Combined Underground Structure. Applied Sciences. 2025; 15(9):5164. https://doi.org/10.3390/app15095164
Chicago/Turabian StyleYuan, Yong, Xuzhao Lan, Weifeng Wu, and Xiuzhi Wang. 2025. "Comparison Between Longitudinal and Transverse Shaking of Culvert–Frame Combined Underground Structure" Applied Sciences 15, no. 9: 5164. https://doi.org/10.3390/app15095164
APA StyleYuan, Y., Lan, X., Wu, W., & Wang, X. (2025). Comparison Between Longitudinal and Transverse Shaking of Culvert–Frame Combined Underground Structure. Applied Sciences, 15(9), 5164. https://doi.org/10.3390/app15095164







