Strength Deterioration Pattern and Stability Evaluation of Open−Pit Mine Slopes in Cold Regions Under Freeze–Thaw Cycles
Abstract
1. Introduction
2. Technical Approach for Strength Degradation and Stability Evaluation of Slope Rock Mass Under Freeze–Thaw Cycles
3. Engineering Overview and Rock Mass Temperature Variation Characteristics with Depth
3.1. Engineering Overview
3.2. The Relationship Between the Temperature of the Rock Mass and Its Burial Depth
4. Impact of Burial Depth and Freeze–Thaw Cycles on Rock Mechanical Properties
4.1. Specimen Preparation and Testing Protocol
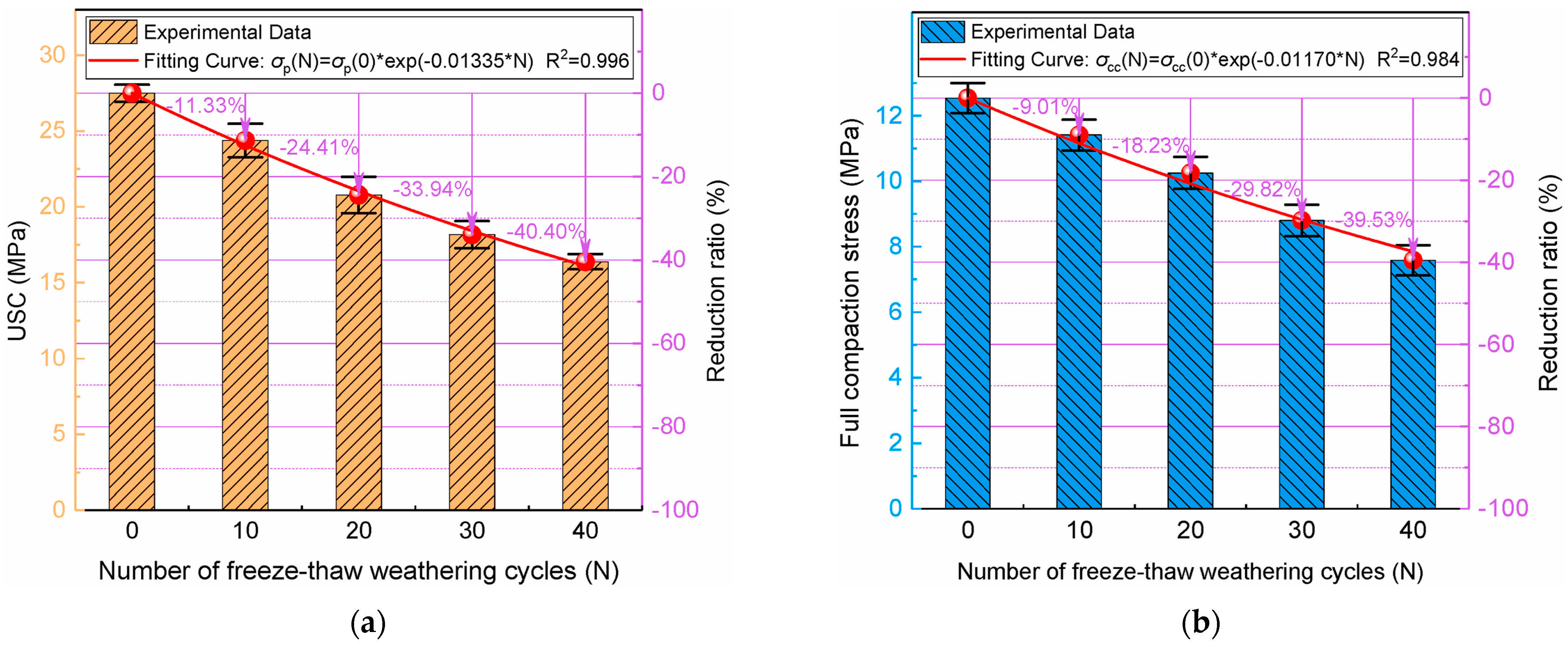
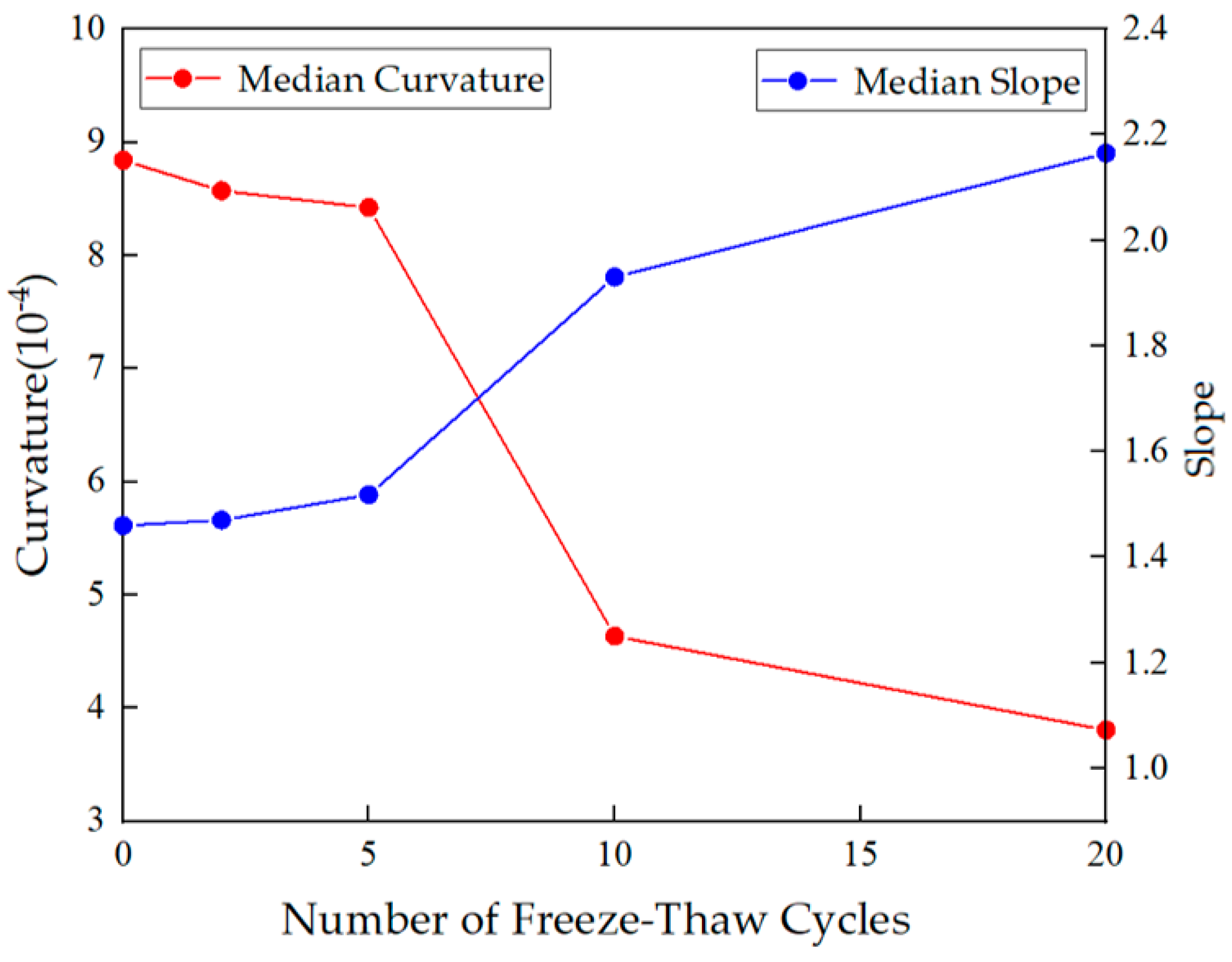
4.2. Experimental Results and Analysis
5. Evolution of Rock Mass Mechanical Parameters Under Freeze–Thaw Cycles
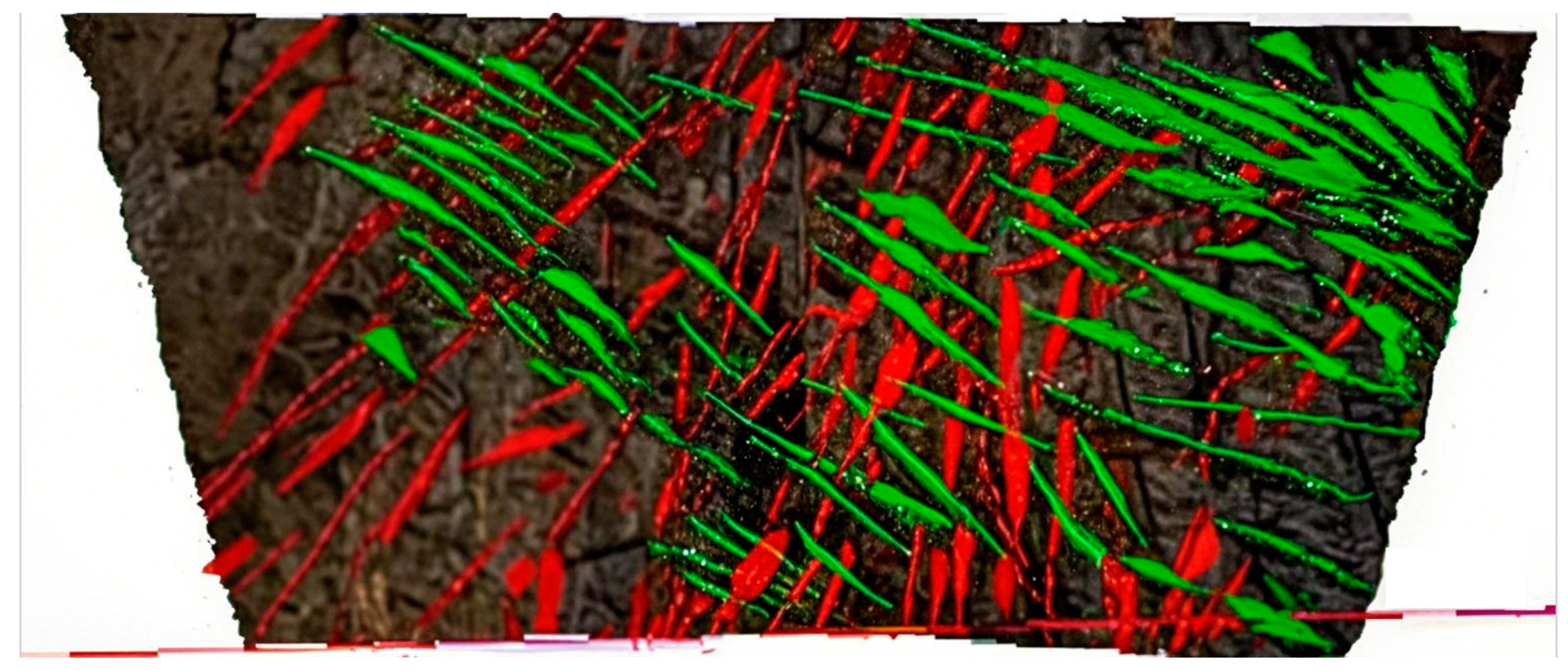
5.1. Structural Geometry Parameters and Rock Mass Quality Quantification
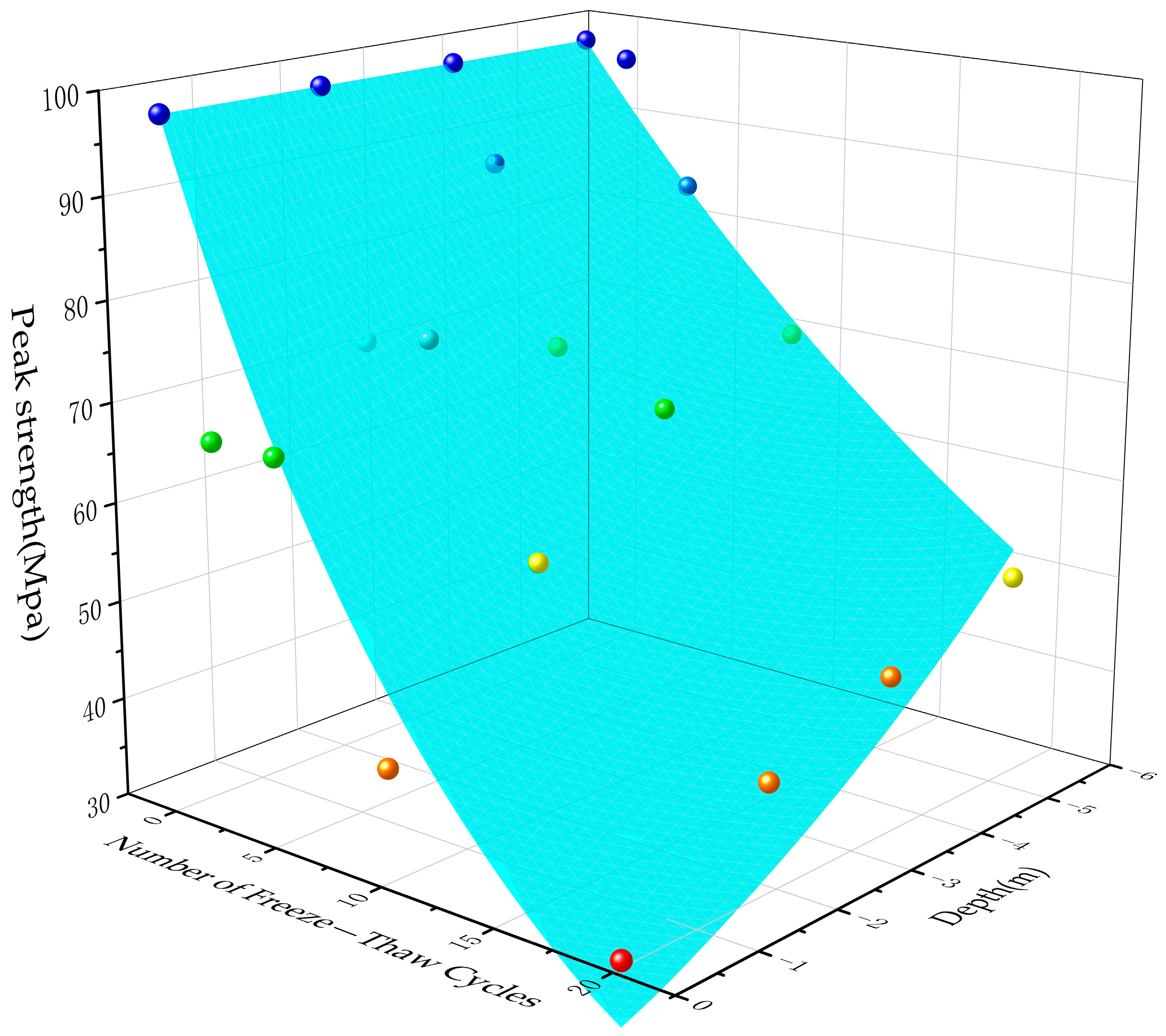
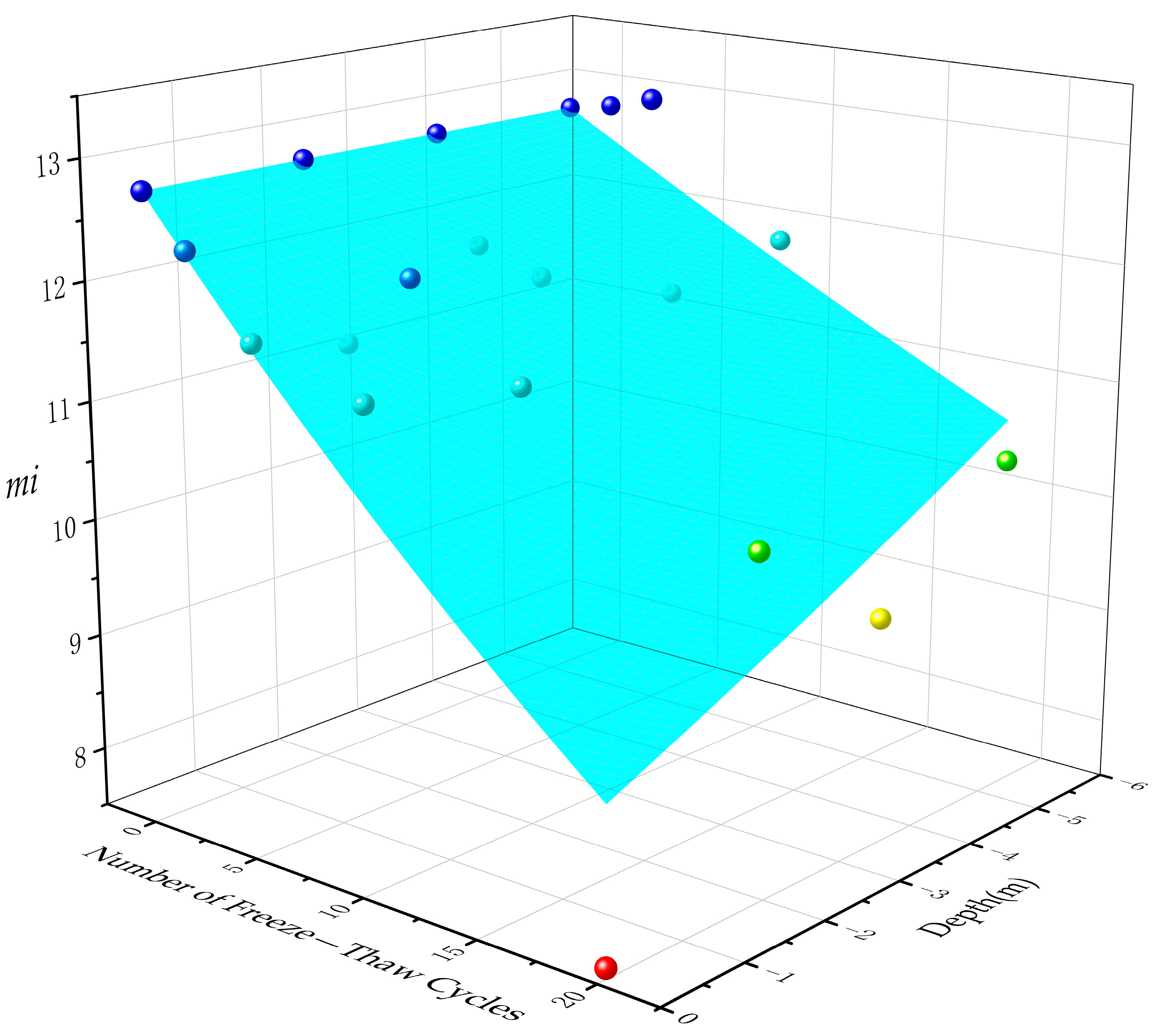
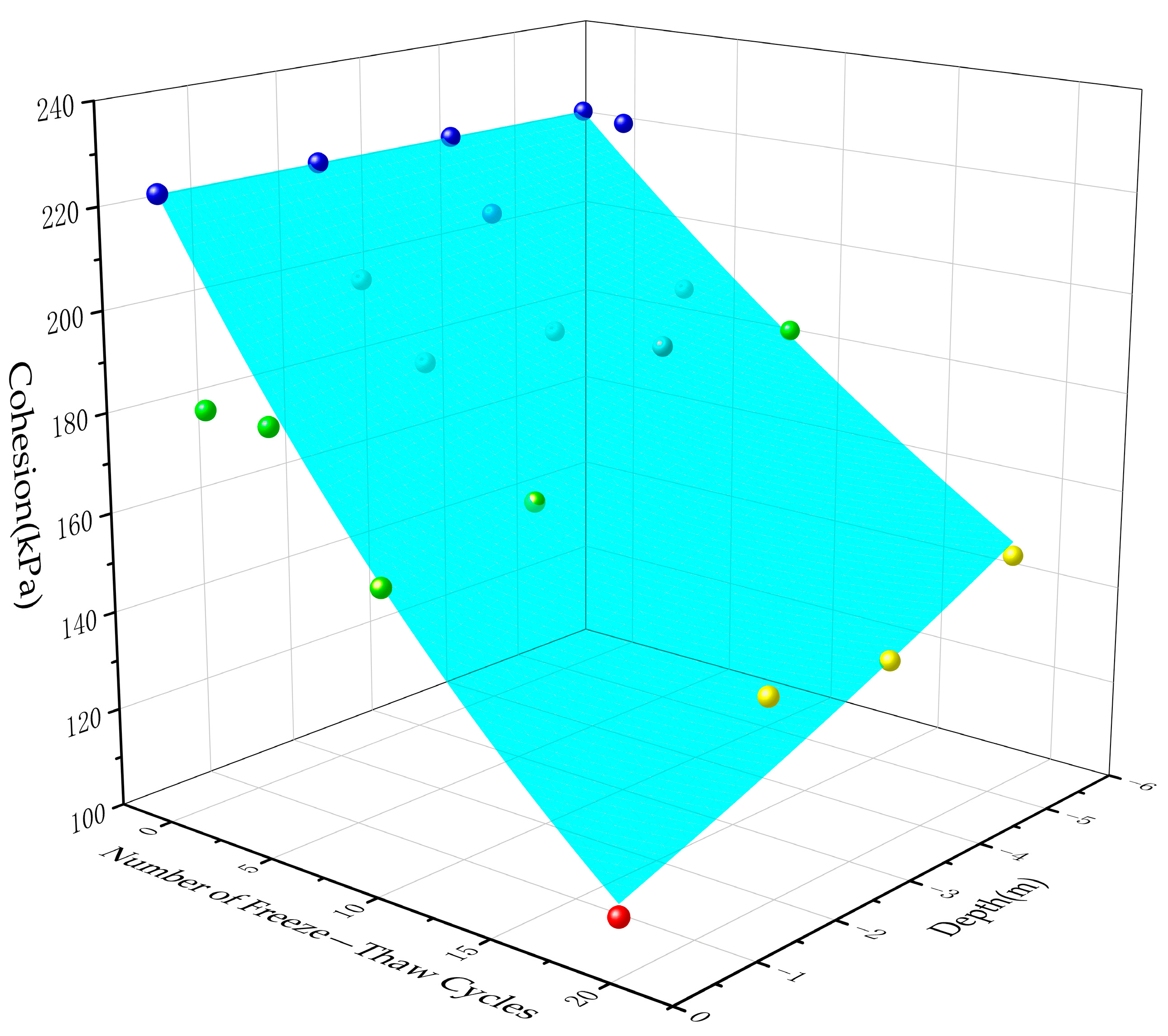
5.2. Quantification of Rock Mass Mechanical Parameters Under Freeze–Thaw Cycles
6. Variation in Slope Safety Factor Under Freeze–Thaw Cycles
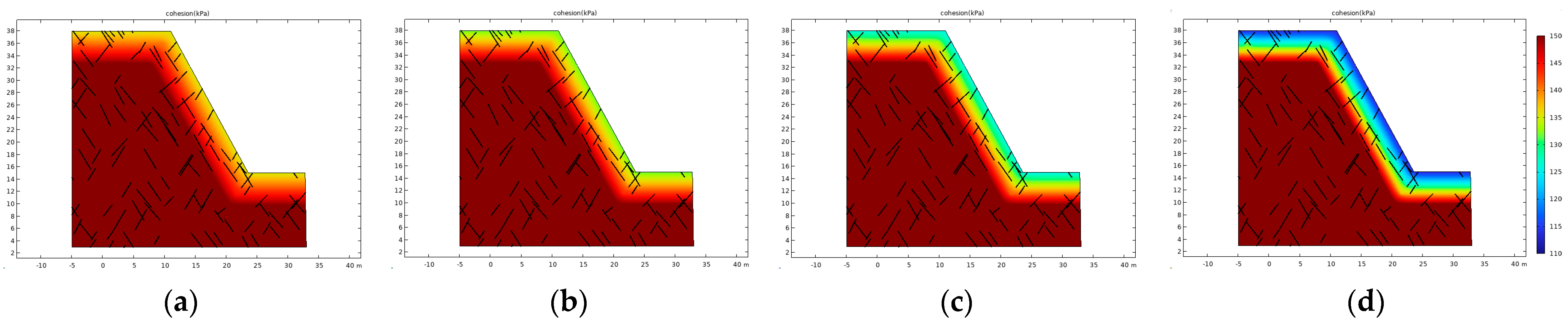
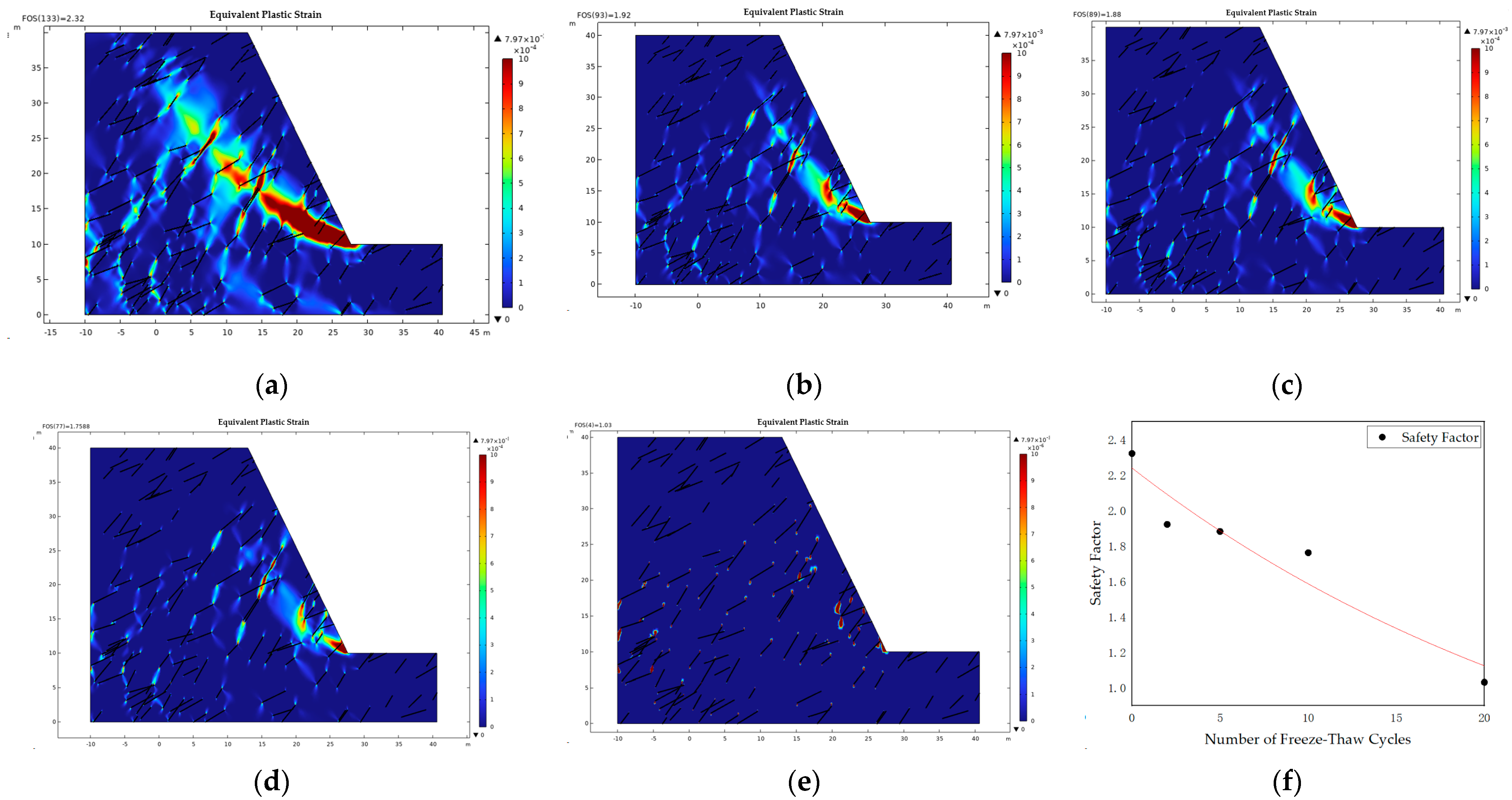
6.1. Variation Pattern of Safety Factor in Slopes with Weak Joints Under Freeze–Thaw Cycles—Jinbao Open−Pit Mine
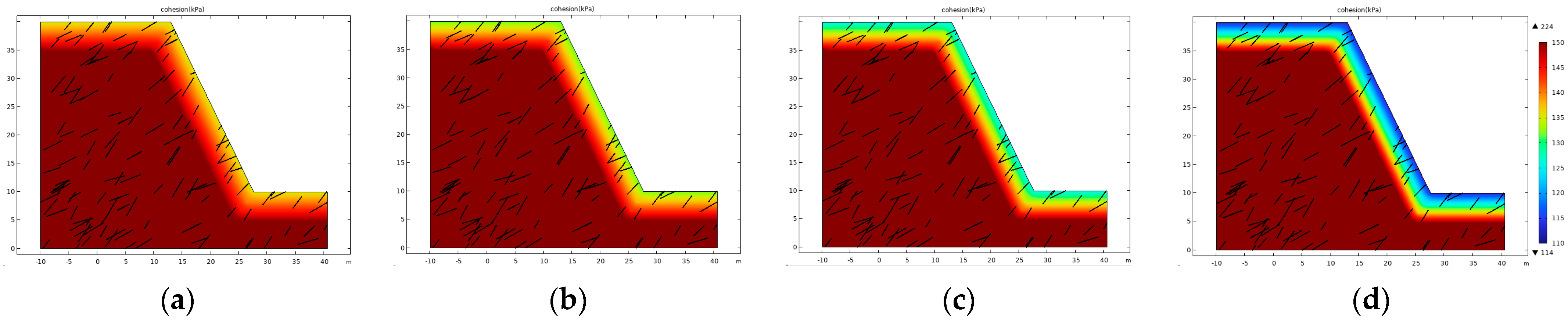
6.2. Variation Pattern of Safety Factor in Slopes with Weak Joints Under Freeze–Thaw Cycles—Wushan Open−Pit Mine
7. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
Appendix A

Appendix B
References
- Matsuoka, N.; Sakai, H. Rockfall activity from an alpine cliff during thawing periods. Geomorphology 1999, 28, 309–328. [Google Scholar] [CrossRef]
- Kodama, J.; Goto, T.; Fujii, Y.; Hagan, P. The effects of water content, temperature and loading rate on strength and failure process of frozen rocks. Int. J. Rock Mech. Min. Sci. 2013, 62, 1–13. [Google Scholar] [CrossRef]
- Zhang, H.; Meng, X.; Yang, G. A study on mechanical properties and damage model of rock subjected to freeze−thaw cycles and confining pressure. Cold Reg. Sci. Technol. 2020, 174, 103056. [Google Scholar] [CrossRef]
- Li, J.; Zhou, K.; Liu, W.; Zhang, Y. Analysis of the effect of freeze–thaw cycles on the degradation of mechanical parameters and slope stability. Bull. Eng. Geol. Environ. 2018, 77, 573–580. [Google Scholar] [CrossRef]
- Zhang, H.M.; Yang, G.S. Experimental study on freeze−thaw cycles and tensile characteristics of rocks. J. Xi’an Univ. Sci. Technol. 2012, 32, 691–695. [Google Scholar]
- Walbert, C.; Eslami, J.; Beaucour, A.L.; Bourges, A.; Noumowe, A. Evolution of the mechanical behaviour of limestone subjected to freeze–thaw cycles. Environ. Earth Sci. 2015, 74, 6339–6351. [Google Scholar] [CrossRef]
- Bayram, F. Predicting mechanical strength loss of natural stones after freeze–thaw in cold regions. Cold Reg. Sci. Technol. 2012, 83, 98–102. [Google Scholar] [CrossRef]
- Zhang, J.; Deng, H.; Taheri, A.; Ke, B.; Liu, C.; Yang, X. Degradation of physical and mechanical properties of sandstone subjected to freeze−thaw cycles and chemical erosion. Cold Reg. Sci. Technol. 2018, 155, 37–46. [Google Scholar] [CrossRef]
- Ren, J.X.; Zhang, L.; Zhang, K. Macro−meso experimental study on triaxial compression failure characteristics of freeze−thaw fractured sandstone. Sci. Technol. Eng. 2023, 23, 7056–7063. [Google Scholar]
- Yu, J.; Chen, X.; Li, H.; Zhou, J.W.; Cai, Y.Y. Effect of freeze−thaw cycles on mechanical properties and permeability of red sandstone under triaxial compression. J. Mt. Sci. 2015, 12, 218–231. [Google Scholar] [CrossRef]
- Yu, J.; Fu, G.F.; Chen, X.; Guo, X. Experimental study on mechanical properties of sandstone under triaxial unloading confining pressure after freeze−thaw cycles. Chin. J. Rock Mech. Eng. 2015, 34, 2001–2009. [Google Scholar]
- Chen, G.Q.; Guo, F.; Wang, J.C.; Zhou, Y.X. Experimental study on triaxial creep characteristics of quartz sandstone after freeze−thaw cycles. Rock Soil Mech. 2017, 38, 203–210. [Google Scholar]
- Gao, F.; Cao, S.; Zhou, K.; Lin, Y.; Zhu, L. Damage characteristics and energy−dissipation mechanism of frozen–thawed sandstone subjected to loading. Cold Reg. Sci. Technol. 2020, 169, 102920. [Google Scholar] [CrossRef]
- Wang, Y.; Gao, S.H.; Li, C.H.; Han, J.Q. Energy dissipation and damage evolution for dynamic fracture of marble subjected to freeze−thaw and multiple level compressive fatigue loading. Int. J. Fatigue 2021, 142, 105927. [Google Scholar] [CrossRef]
- Wen, L.; Li, X.B.; Su, W. Study on physico−mechanical properties of hard rocks in metal mine slopes under freeze−thaw cycles. J. Min. Saf. Eng. 2015, 32, 689–696. [Google Scholar]
- Zhang, Y.; Huang, R.; Zhao, L.; Fu, R.; Pei, X. Study on TSMR system for rock mass quality evaluation of Tianshan highway slopes. Chin. J. Rock Mech. Eng. 2010, 29, 617–623. [Google Scholar]
- Tan, H.H.; Li, B.; Li, J.X.; Yuan, W.; Li, Z.H. Stability analysis of dolomite slopes under freeze−thaw cycles. Sci. Technol. Eng. 2020, 20, 13825–13832. [Google Scholar]
- Xiao, Y.G. Study on Mining−Induced Response and Multi−Field Coupled Time−Dependent Disaster Process of High−Altitude Slope Rock Mass. Doctoral dissertation, University of Science and Technology Beijing, Beijing, China, 2021.
- Zerradi, Y.; Souissi, M.; Larabi, A. Application of the deterministic block theory to the slope stability design of an open−pit mine in Morocco. Min. Miner. Depos. 2023, 17, 53–60. [Google Scholar] [CrossRef]
- Sdvyzhkova, O.; Moldabayev, S.; Babets, D.; Başçetin, A.; Asylkhanova, G.; Nurmanova, A.; Prykhodko, V. Numerical modelling of the pit wall stability while optimizing its boundaries to ensure the ore mining completeness. Min. Miner. Depos. 2024, 18, 1–10. [Google Scholar] [CrossRef]
- Russo, G. A new rational method for calculating the GSI. Tunn. Undergr. Space Technol. 2009, 24, 103–111. [Google Scholar] [CrossRef]
- Hoek, E.; Brown, E.T. The Hoek–Brown failure criterion and GSI–2018 edition. J. Rock Mech. Geotech. Eng. 2019, 11, 445–463. [Google Scholar] [CrossRef]
- Sonmez, H.; Ulusay, R. Modifications to the geological strength index (GSI) and their applicability to stability of slopes. Int. J. Rock Mech. Min. 1999, 36, 743–760. [Google Scholar] [CrossRef]
- Gao, F.; Xiong, X.; Xu, C.; Zhou, K. Mechanical property deterioration characteristics and a new constitutive model for rocks subjected to freeze−thaw weathering process. Int. J. Rock Mech. Min. Sci. 2021, 140, 104642. [Google Scholar] [CrossRef]











| No. | Freeze–Thaw Temperature (°C) | Corresponding Burial Depth (m) | Freeze–Thaw Cycles | No. of Specimens | |
|---|---|---|---|---|---|
| Uniaxial Compression | Brazilian Splitting | ||||
| 1 | −2~7 | 0.10 | 0, 2, 5, 10, 20 | 5 | 5 |
| 2 | −5~10 | 1.88 | 5 | 5 | |
| 3 | −10~14 | 3.50 | 5 | 5 | |
| 4 | −20~22 | 5.27 | 5 | 5 | |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zhang, P.; Gao, N.; Yan, W.; Hou, J.; Liu, H. Strength Deterioration Pattern and Stability Evaluation of Open−Pit Mine Slopes in Cold Regions Under Freeze–Thaw Cycles. Appl. Sci. 2025, 15, 4853. https://doi.org/10.3390/app15094853
Zhang P, Gao N, Yan W, Hou J, Liu H. Strength Deterioration Pattern and Stability Evaluation of Open−Pit Mine Slopes in Cold Regions Under Freeze–Thaw Cycles. Applied Sciences. 2025; 15(9):4853. https://doi.org/10.3390/app15094853
Chicago/Turabian StyleZhang, Penghai, Ning Gao, Wanni Yan, Jun Hou, and Honglei Liu. 2025. "Strength Deterioration Pattern and Stability Evaluation of Open−Pit Mine Slopes in Cold Regions Under Freeze–Thaw Cycles" Applied Sciences 15, no. 9: 4853. https://doi.org/10.3390/app15094853
APA StyleZhang, P., Gao, N., Yan, W., Hou, J., & Liu, H. (2025). Strength Deterioration Pattern and Stability Evaluation of Open−Pit Mine Slopes in Cold Regions Under Freeze–Thaw Cycles. Applied Sciences, 15(9), 4853. https://doi.org/10.3390/app15094853





