Numerical Simulation of Rock-Breaking Mechanism by Spherical Tooth Impact in Granite Formation
Abstract
1. Introduction
2. Model and Methodology
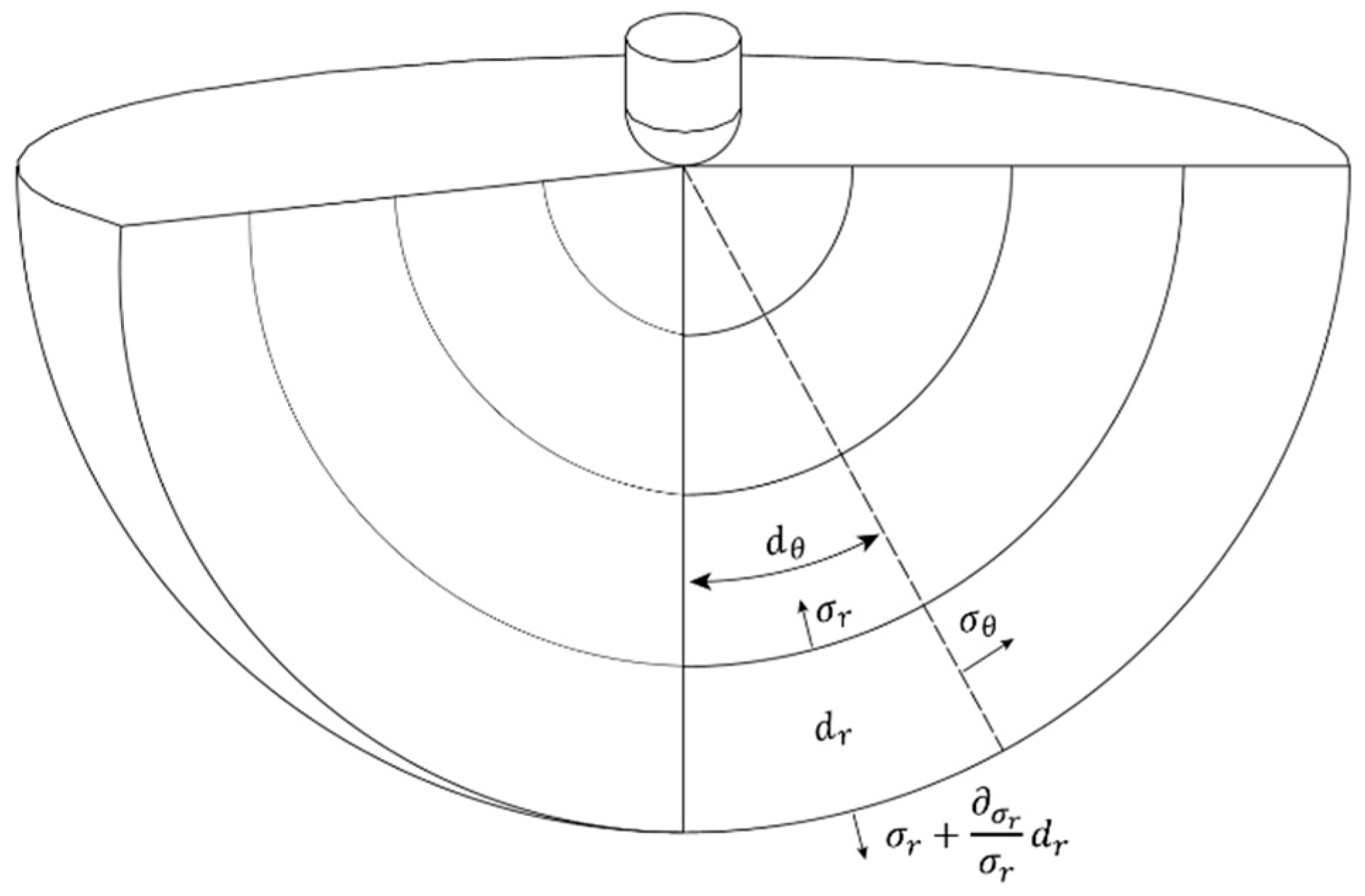
2.1. Spherical Tooth Impact Model
2.2. Simulation Settings
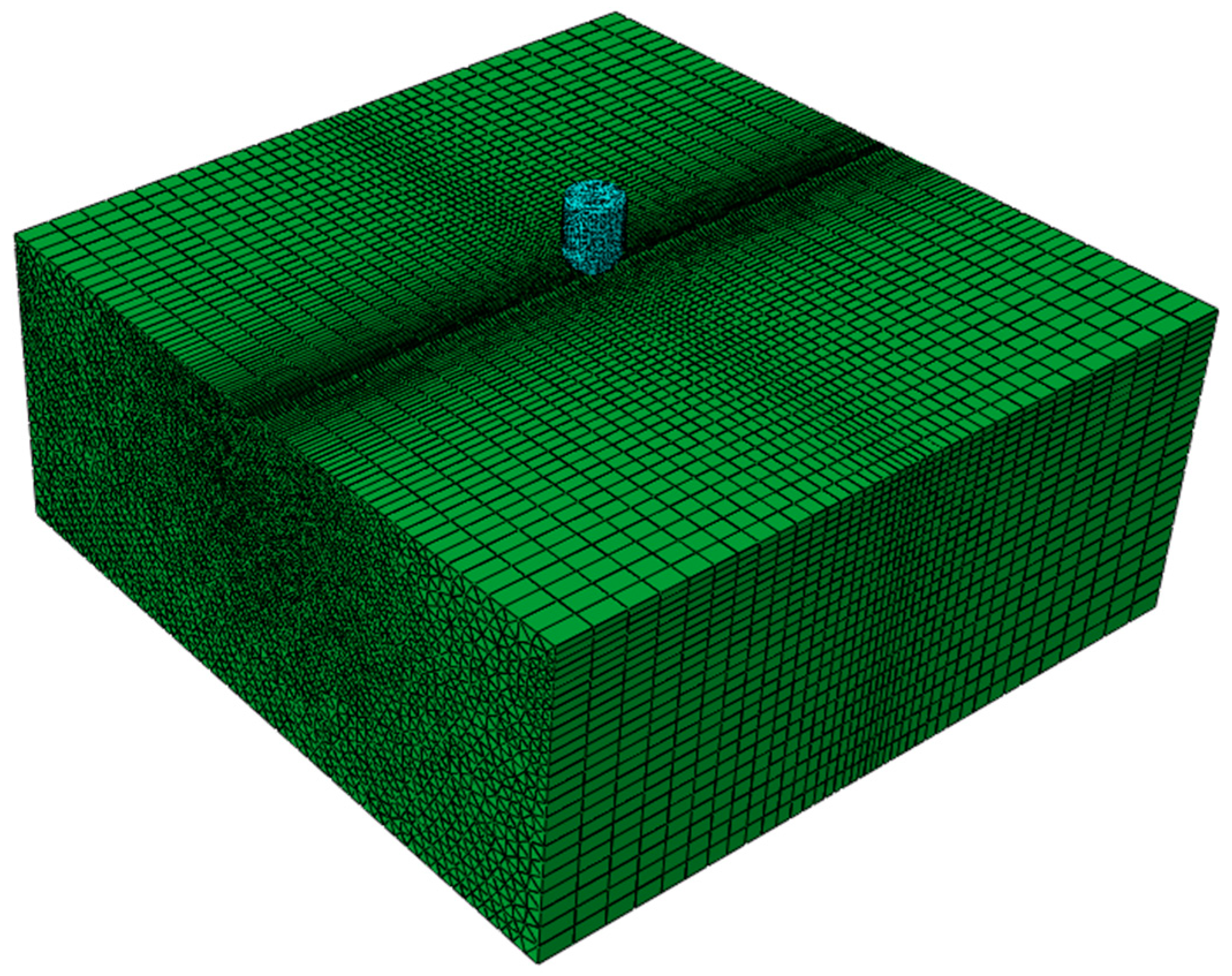
2.2.1. Simulation Model
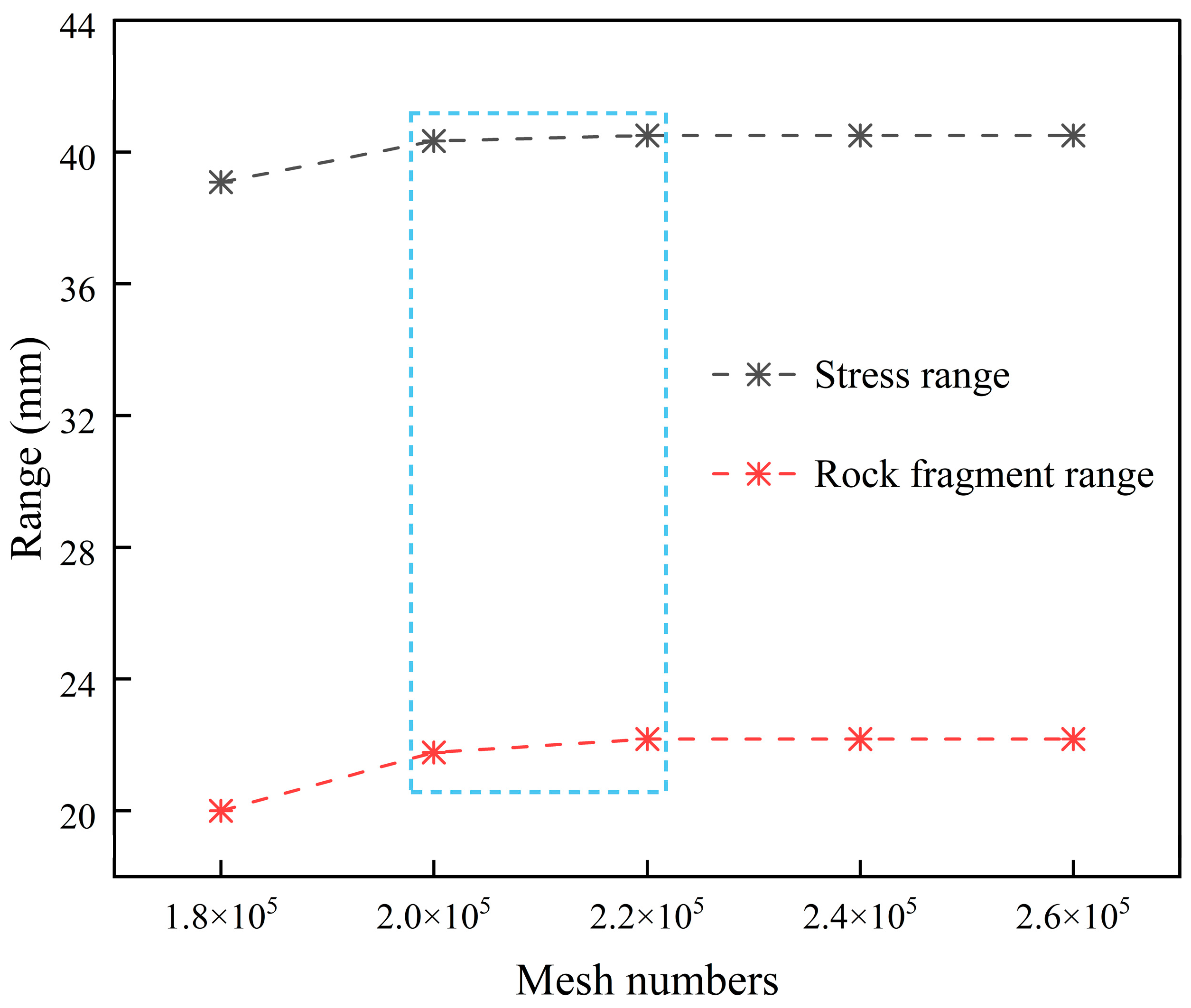
2.2.2. Grid and Independence Verification
2.2.3. Simulation Parameter Setting
3. Results and Discussion
3.1. Simulation Correctness Verification
3.2. Study on the Influencing Factors of Single-Tooth Broken Rock
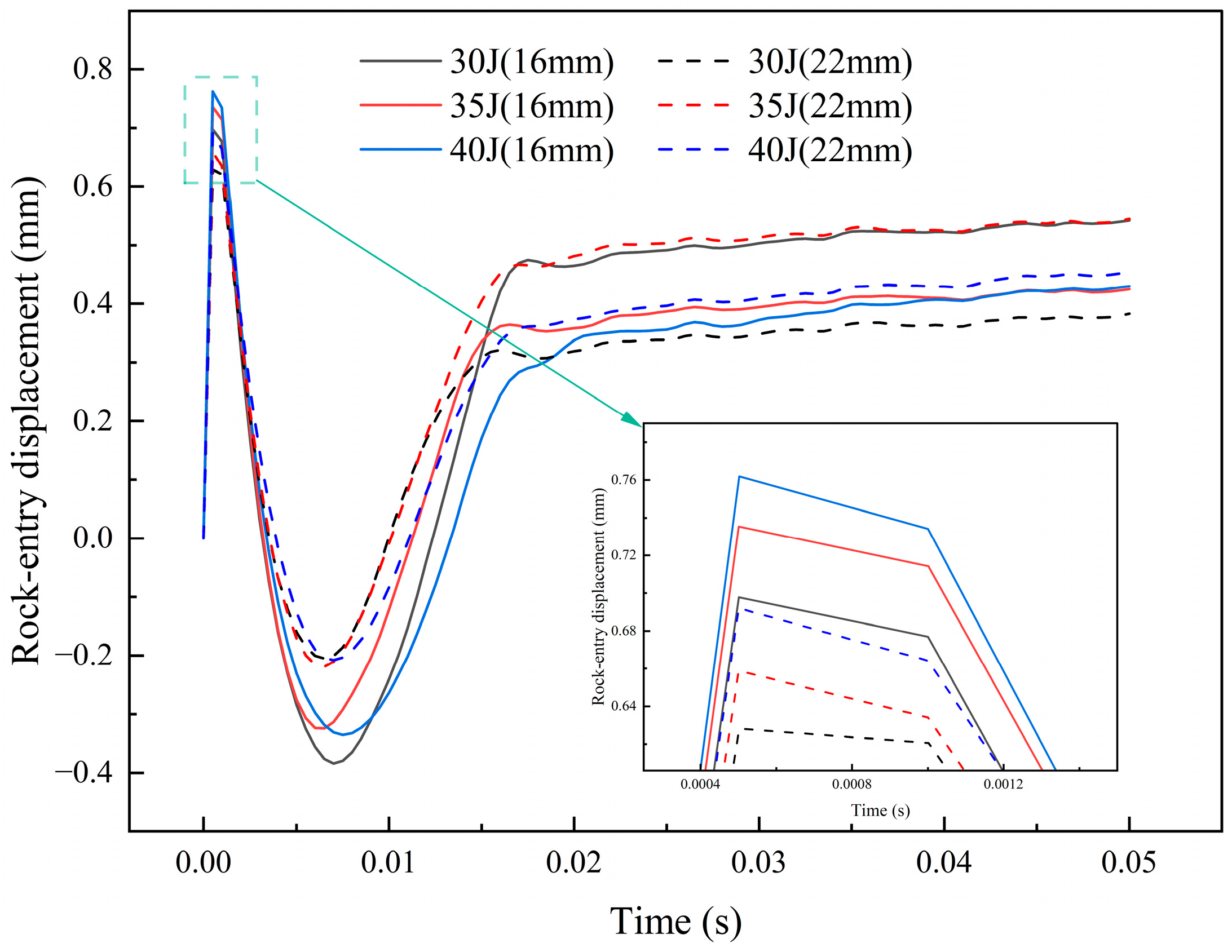
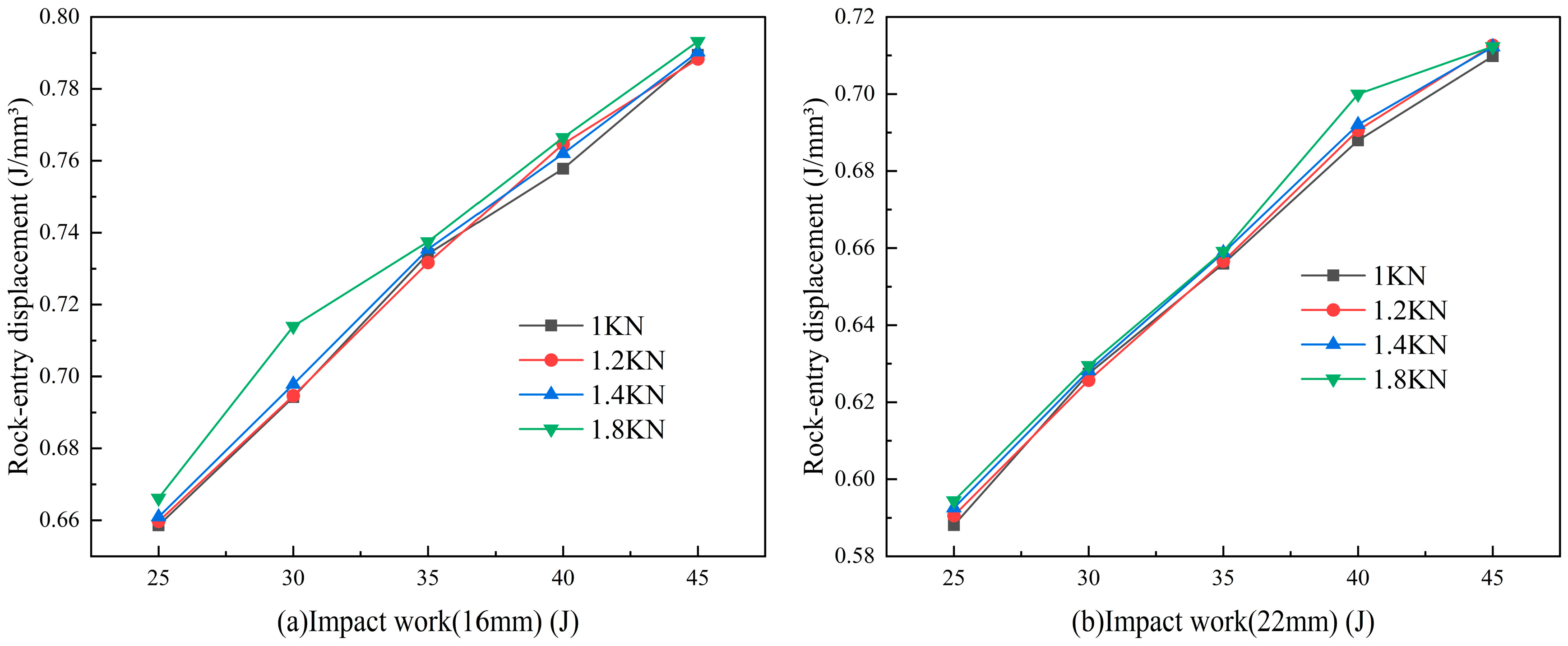
3.2.1. Spherical Tooth into Rock Displacement
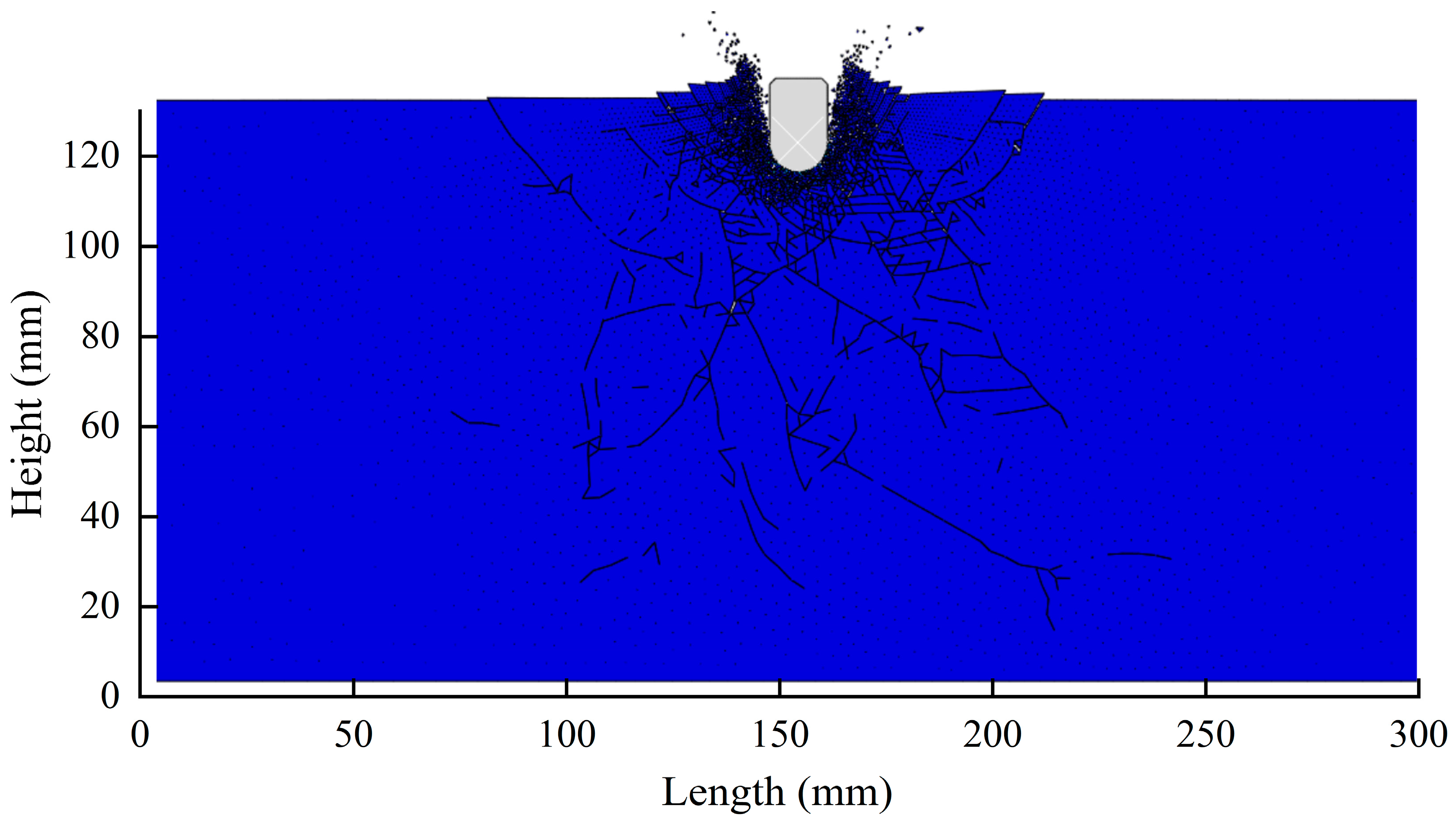
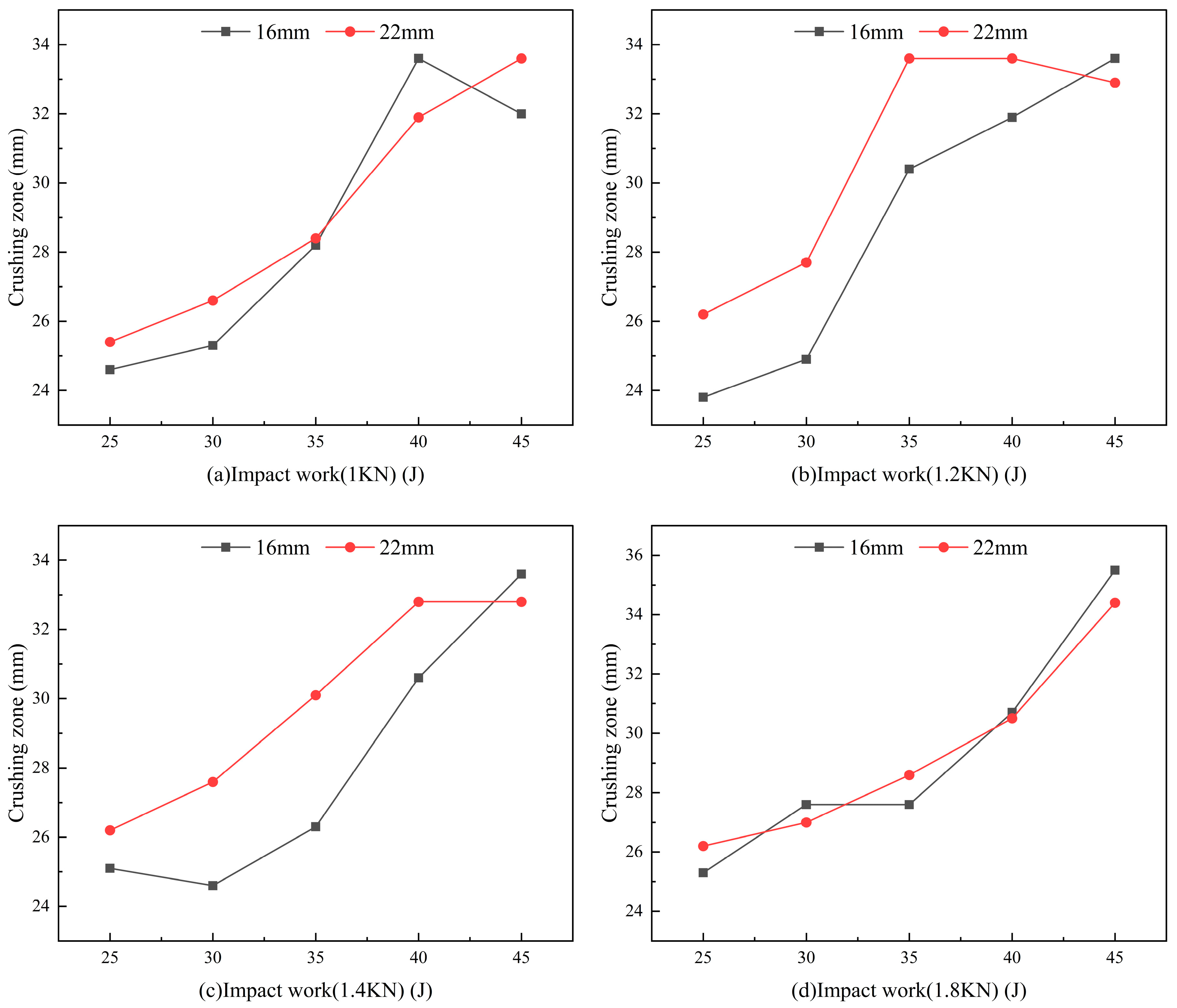
3.2.2. Rock Fragmentation Range
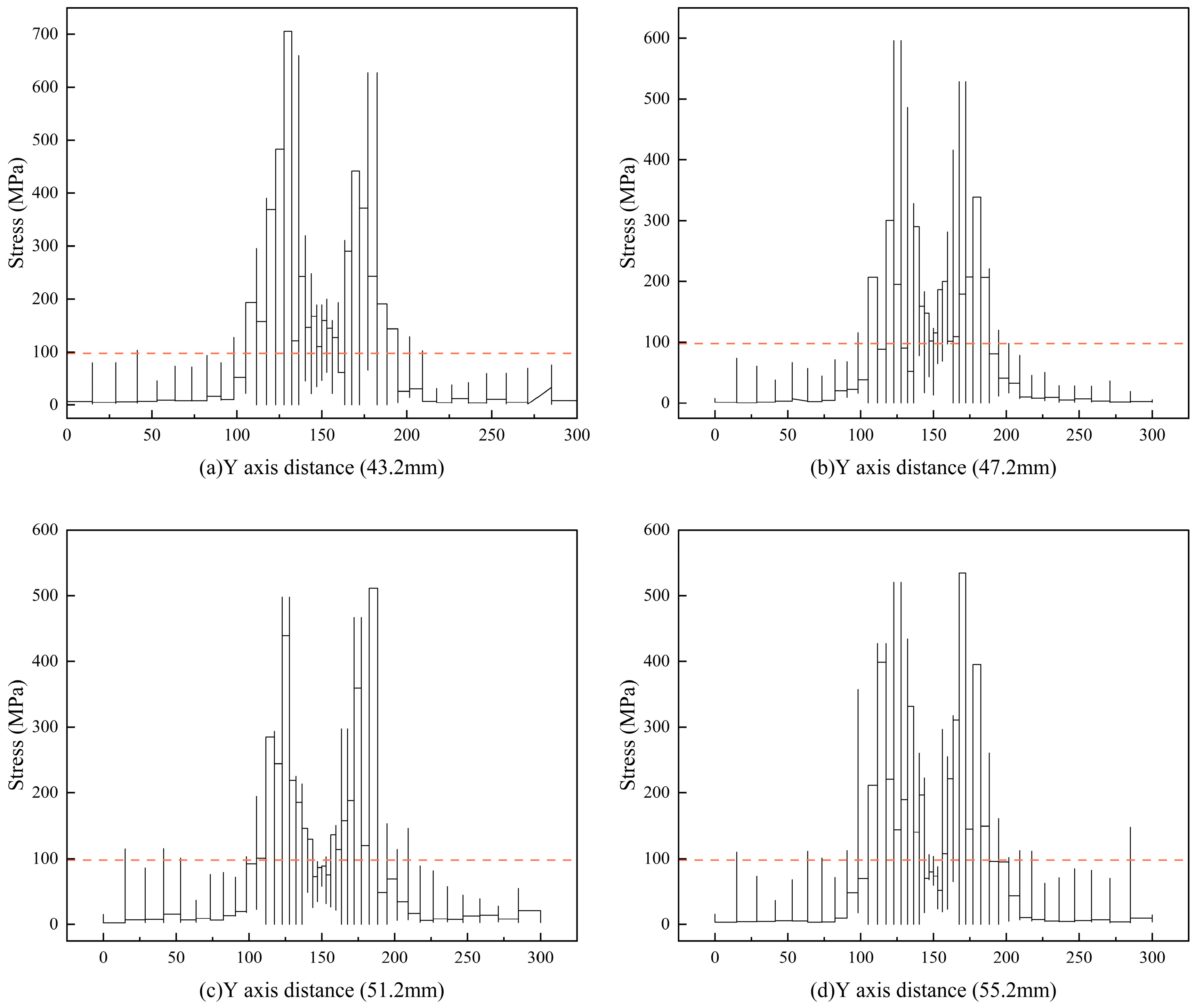
3.2.3. Influence Range of Rock Stress
3.2.4. Single Spherical Tooth Crushing Specific Work
3.3. Layout Optimization Research
3.3.1. Optimization of Pitch of 22 mm Spherical Tooth Ring
3.3.2. Spacing Optimization of 22 mm Spherical Teeth
4. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Bo, K.; Ji, F.; Zhao, Z.; Fan, L.; Wang, M. Optimum Design of Large-Diameter Reverse Circulation Drill Bit for Drilling Rescue Wells Using Orthogonal Experimental Method and CFD Simulation. Energies 2023, 16, 3913. [Google Scholar] [CrossRef]
- He, J.; Zhao, Z.; Yin, Q.; Luo, Y.; Gan, X. Design and Optimisation on Rapid Rescue Well-Drilling Technology with Large-Diameter Pneumatic Hammers. Int. J. Min. Reclam. Environ. 2020, 34, 19–33. [Google Scholar] [CrossRef]
- Zhao, J.; Hao, S. Application of pneumatic DTH hammer in drilling rescue of mine accidents. Coal Geol. Explor. 2022, 50, 24–34. [Google Scholar]
- Kivade, S.B. Experimental Investigations on Penetration Rate of Percussive Drill. Procedia Earth Planet. Sci. 2015, 11, 89–99. [Google Scholar] [CrossRef]
- Souissi, S.; Miled, K.; Hamdi, E.; Sellami, H. Numerical Modeling of Rock Damage during Indentation Process with Reference to Hard Rock Drilling. Int. J. Geomech. 2017, 17, 04017002. [Google Scholar] [CrossRef]
- Weddfelt, K.; Saadati, M.; Larsson, P.-L. On the Load Capacity and Fracture Mechanism of Hard Rocks at Indentation Loading. Int. J. Rock Mech. Min. Sci. 2017, 100, 170–176. [Google Scholar] [CrossRef]
- Zhang, H.; Huang, G.; Song, H.; Kang, Y. Experimental Investigation of Deformation and Failure Mechanisms in Rock under Indentation by Digital Image Correlation. Eng. Fract. Mech. 2012, 96, 667–675. [Google Scholar] [CrossRef]
- Qin, Z.; Baolin, L.; Mingtao, J.; Tong, L.; Guoxin, W.; Jun, Z. Experiment Research on Impact Fragmentation Mechanism of Single-Indenter under Low Power Condition. Procedia Eng. 2014, 73, 186–193. [Google Scholar] [CrossRef][Green Version]
- Ajibose, O.K.; Wiercigroch, M.; Akisanya, A.R. Experimental Studies of the Resultant Contact Forces in Drillbit–Rock Interaction. Int. J. Mech. Sci. 2015, 91, 3–11. [Google Scholar] [CrossRef]
- Wang, S.Y.; Sloan, S.W.; Liu, H.Y.; Tang, C.A. Numerical Simulation of the Rock Fragmentation Process Induced by Two Drill Bits Subjected to Static and Dynamic (Impact) Loading. Rock Mech. Rock Eng. 2011, 44, 317–332. [Google Scholar] [CrossRef]
- Zhu, X.; Yue, Y.; Wong, P.; Zhang, Y.; Tan, J. Optimum Water Quality Monitoring Network Design for Bidirectional River Systems. Int. J. Environ. Res. Public Health 2018, 15, 195. [Google Scholar] [CrossRef]
- Chen, L.H.; Labuz, J.F. Indentation of Rock by Wedge-Shaped Tools. Int. J. Rock Mech. Min. Sci. 2006, 43, 1023–1033. [Google Scholar] [CrossRef]
- Wu, C.M.L. Nopainear Thermal and Mechanical Analysis of Step-Tapered Laminated Plate under Uniform in-Plane Load. Compos. Struct. 1992, 22, 33–45. [Google Scholar]
- Lundberg, B.; Collet, P. Optimal Wave Shape with Respect to Efficiency in Percussive Drilling with Detachable Drill Bit. Int. J. Impact Eng. 2015, 86, 179–187. [Google Scholar] [CrossRef]
- Lundberg, B.; Collet, P. Optimal Wave with Respect to Efficiency in Percussive Drilling with Integral Drill Steel. Int. J. Impact Eng. 2010, 37, 901–906. [Google Scholar] [CrossRef]
- Song, H.; Wu, Z.; Wang, A.; Sun, W.; Liu, H.; Lou, Y.; Zuo, Y. Study on the Microscale Tensile Properties of Lower Cambrian Niutitang Formation Shale Based on Digital Images. Geofluids 2020, 2020, 8828965. [Google Scholar] [CrossRef]
- Song, H.; Shi, H.; Chen, Z.; Li, G.; Ji, R.; Chen, H. Numerical Study on Impact Energy Transfer and Rock Damage Mechanism in Percussive Drilling Based on High Temperature Hard Rocks. Geothermics 2021, 96, 102215. [Google Scholar] [CrossRef]
- Song, H.; Shi, H.; Li, G.; Chen, Z.; Li, X. Numerical Simulation of the Energy Transfer Efficiency and Rock Damage in Axial-Torsional Coupled Percussive Drilling. J. Pet. Sci. Eng. 2021, 196, 107675. [Google Scholar] [CrossRef]
- Li, H.; Liu, S.; Chang, H. Experimental Research on the Influence of Working Parameters on the Drilling Efficiency. Tunn. Undergr. Space Technol. 2020, 95, 103174. [Google Scholar] [CrossRef]
- Song, H.; Shi, H.; Ji, Z.; Wu, X.; Li, G.; Zhao, H.; Wang, G.; Liu, Y.; Hou, X. The Percussive Process and Energy Transfer Efficiency of Percussive Drilling with Consideration of Rock Damage. Int. J. Rock Mech. Min. Sci. 2019, 119, 1–12. [Google Scholar] [CrossRef]
- Yang, Y.; Chen, C.; Wen, L.; Chen, X.; Liang, H.; Liu, R.; Guan, X.; Luo, B.; Xie, C. Characteristics of Buried Structures in Northern Longmenshan Mountains and Its Significance to Oil and Gas Exploration in the Sichuan Basin. Nat. Gas Ind. B 2019, 6, 175–182. [Google Scholar] [CrossRef]
- Liu, H.Y.; Kou, S.Q.; Lindqvist, P.-A.; Tang, C.A. Numerical Simulation of the Rock Fragmentation Process Induced by Indenters. Int. J. Rock Mech. Min. Sci. 2002, 39, 491–505. [Google Scholar] [CrossRef]
- Saksala, T. Numerical Study of the Influence of Hydrostatic and Confining Pressure on Percussive Drilling of Hard Rock. Comput. Geotech. 2016, 76, 120–128. [Google Scholar]
- Li, D.; Cheng, J.; Leung, V.C.M. Adaptive Spectrum Sharing for Half-Duplex and Full-Duplex Cognitive Radios: From the Energy Efficiency Perspective. IEEE Trans. Commun. 2018, 66, 5067–5080. [Google Scholar] [CrossRef]
- Jiang, H.; Cai, Z.; Zhao, H. Numerical Study of Hard Rock Breakage under Indenter Impact by the Hybrid FDEM. Eng. Fract. Mech. 2020, 233, 107068. [Google Scholar] [CrossRef]
- Saadati, M.; Forquin, P.; Weddfelt, K.; Larsson, P.; Hild, F. A Numerical Study of the Influence from Pre-existing Cracks on Granite Rock Fragmentation at Percussive Drilling. Num. Anal. Meth. Geomech. 2015, 39, 558–570. [Google Scholar] [CrossRef]
- Cook, W.; Hood, M.; Tsai, F. Observations of Crack Growth in Hard Rock Loaded by an Indenter. Int. J. Rock Mech. Min. Sci. Geomech. Abstr. 1984, 21, 97–107. [Google Scholar]
- Souissi, S.; Hamdi, E.; Sellami, H. Microstructure Effect on Hard Rock Damage and Fracture During Indentation Process. Geotech. Geol. Eng. 2015, 33, 1539–1550. [Google Scholar] [CrossRef]
- Yang, Q.; Zhou, J. Pneumatic DTH Hammer Tooth Crushing and Optimization of Teeth Arrangement Based on ABAQUS. Drill. Eng. 2024, 51, 40–50. [Google Scholar] [CrossRef]
- Hill, R.; Storåkers, B.; Zdunek, A.B. A Theoretical Study of the Brinell Hardness Test. Proc. R. Soc. Lond. A 1989, 423, 301–330. [Google Scholar] [CrossRef]
- Jiang, H.; Meng, D. 3D Numerical Modelling of Rock Fracture with a Hybrid Finite and Cohesive Element Method. Eng. Fract. Mech. 2018, 199, 280–293. [Google Scholar] [CrossRef]
- Wu, Z.; Ma, L.; Fan, L. Investigation of the Characteristics of Rock Fracture Process Zone Using Coupled FEM/DEM Method. Eng. Fract. Mech. 2018, 200, 355–374. [Google Scholar] [CrossRef]
- Li, S.; Chen, Z.; Li, W.; Yan, T.; Bi, F.; Tong, Y. An FE Simulation of the Fracture Characteristics of Blunt Rock Indenter Under Static and Harmonic Dynamic Loadings Using Cohesive Elements. Rock Mech. Rock Eng. 2023, 56, 2935–2947. [Google Scholar] [CrossRef]




| Item | Parameter and Unit | Unit | Value |
|---|---|---|---|
| Rock and Cohesion Unit | Density () | kg·m−3 | 2700 |
| Elastic modulus () | MPa | 60,000 | |
| Poisson’s ratio () | 0.24 | ||
| Displacement at failure () | mm | 0.004 | |
| Nominal stress of normal-only mode () | MPa | 40 | |
| Nominal stress in the first direction () | MPa | 120 | |
| Nominal stress in the second direction () | MPa | 120 | |
| Spherical Tooth | Density () | kg·m−3 | 15,000 |
| Elastic modulus () | MPa | 588,000 | |
| Poisson’s ratio () | 0.22 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zhou, J.; Li, K.; Wu, H.; Dong, Y.; Xia, B. Numerical Simulation of Rock-Breaking Mechanism by Spherical Tooth Impact in Granite Formation. Appl. Sci. 2025, 15, 3649. https://doi.org/10.3390/app15073649
Zhou J, Li K, Wu H, Dong Y, Xia B. Numerical Simulation of Rock-Breaking Mechanism by Spherical Tooth Impact in Granite Formation. Applied Sciences. 2025; 15(7):3649. https://doi.org/10.3390/app15073649
Chicago/Turabian StyleZhou, Jing, Kunkun Li, Hao Wu, Yuan Dong, and Bairu Xia. 2025. "Numerical Simulation of Rock-Breaking Mechanism by Spherical Tooth Impact in Granite Formation" Applied Sciences 15, no. 7: 3649. https://doi.org/10.3390/app15073649
APA StyleZhou, J., Li, K., Wu, H., Dong, Y., & Xia, B. (2025). Numerical Simulation of Rock-Breaking Mechanism by Spherical Tooth Impact in Granite Formation. Applied Sciences, 15(7), 3649. https://doi.org/10.3390/app15073649





