Topological and Fractal Analysis of Nanostructured Metal–Dielectric Films
Abstract
1. Introduction
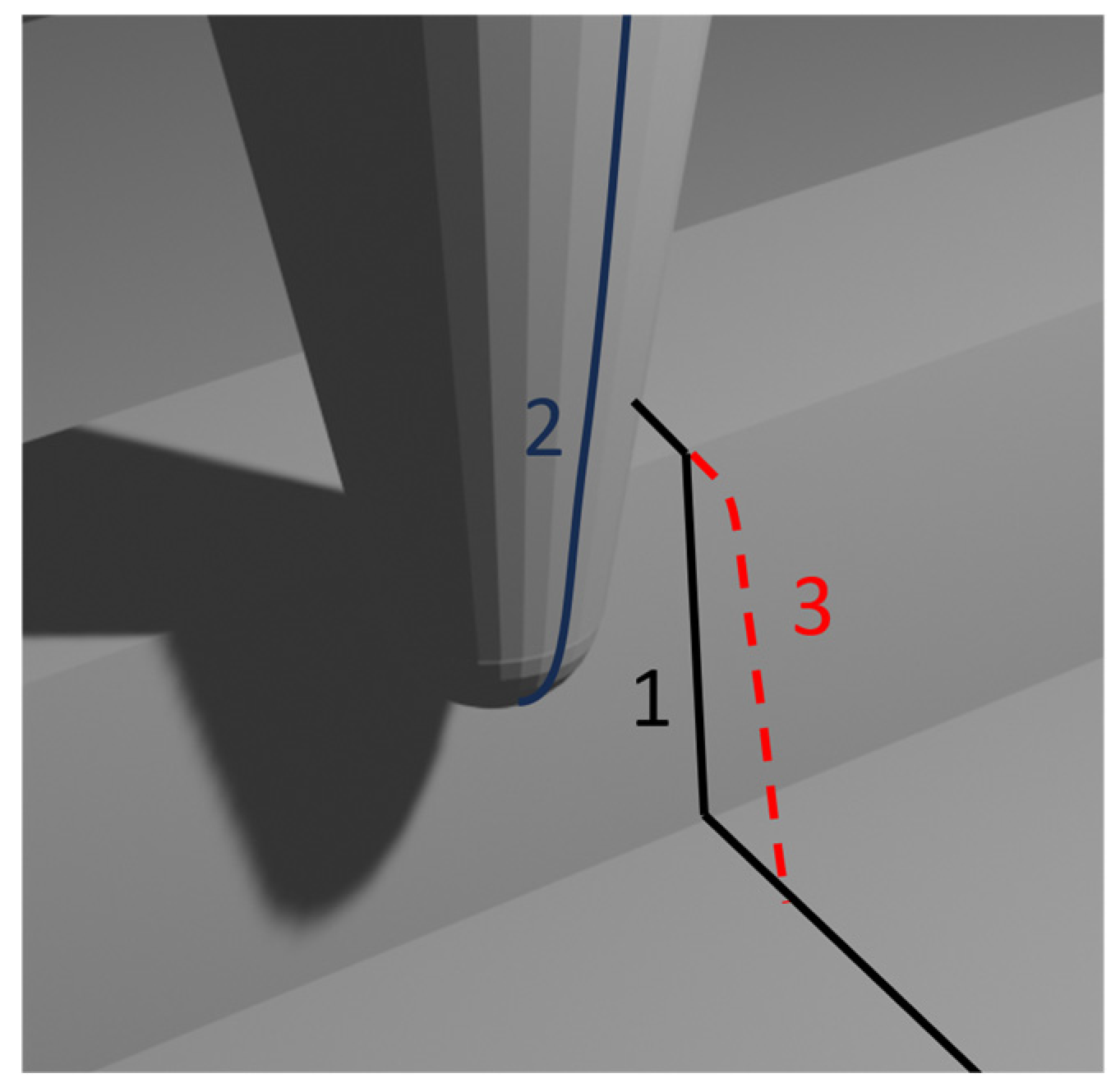
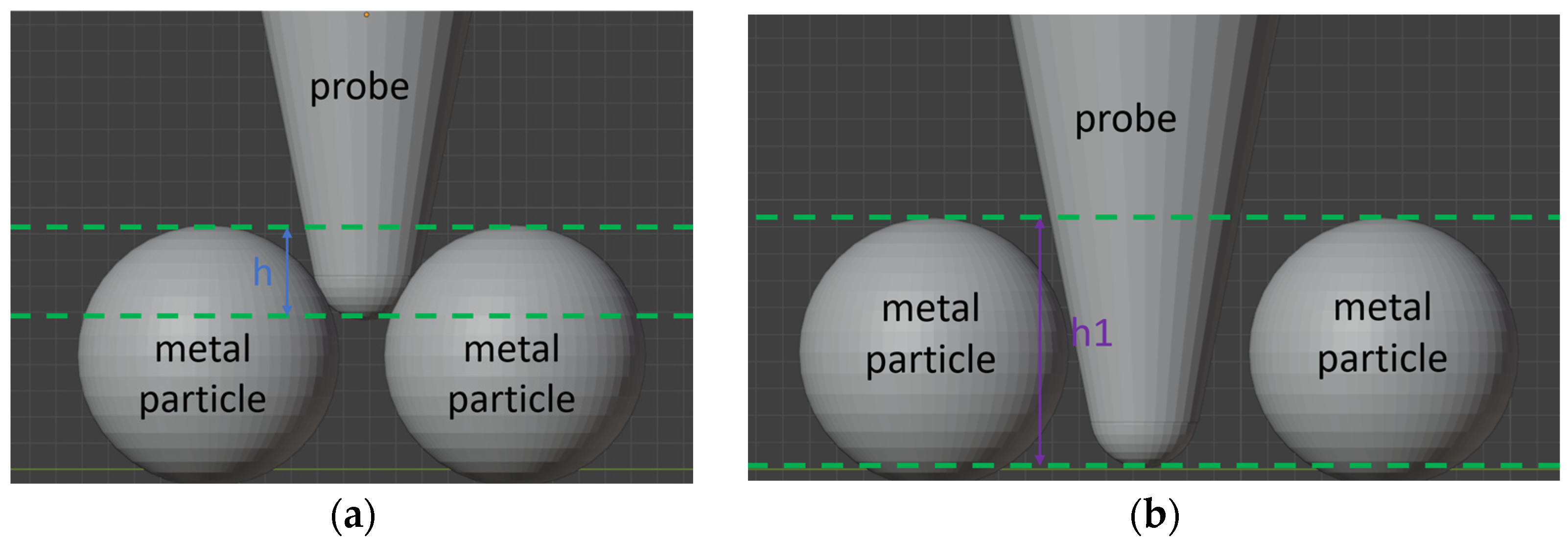
2. Materials and Methods
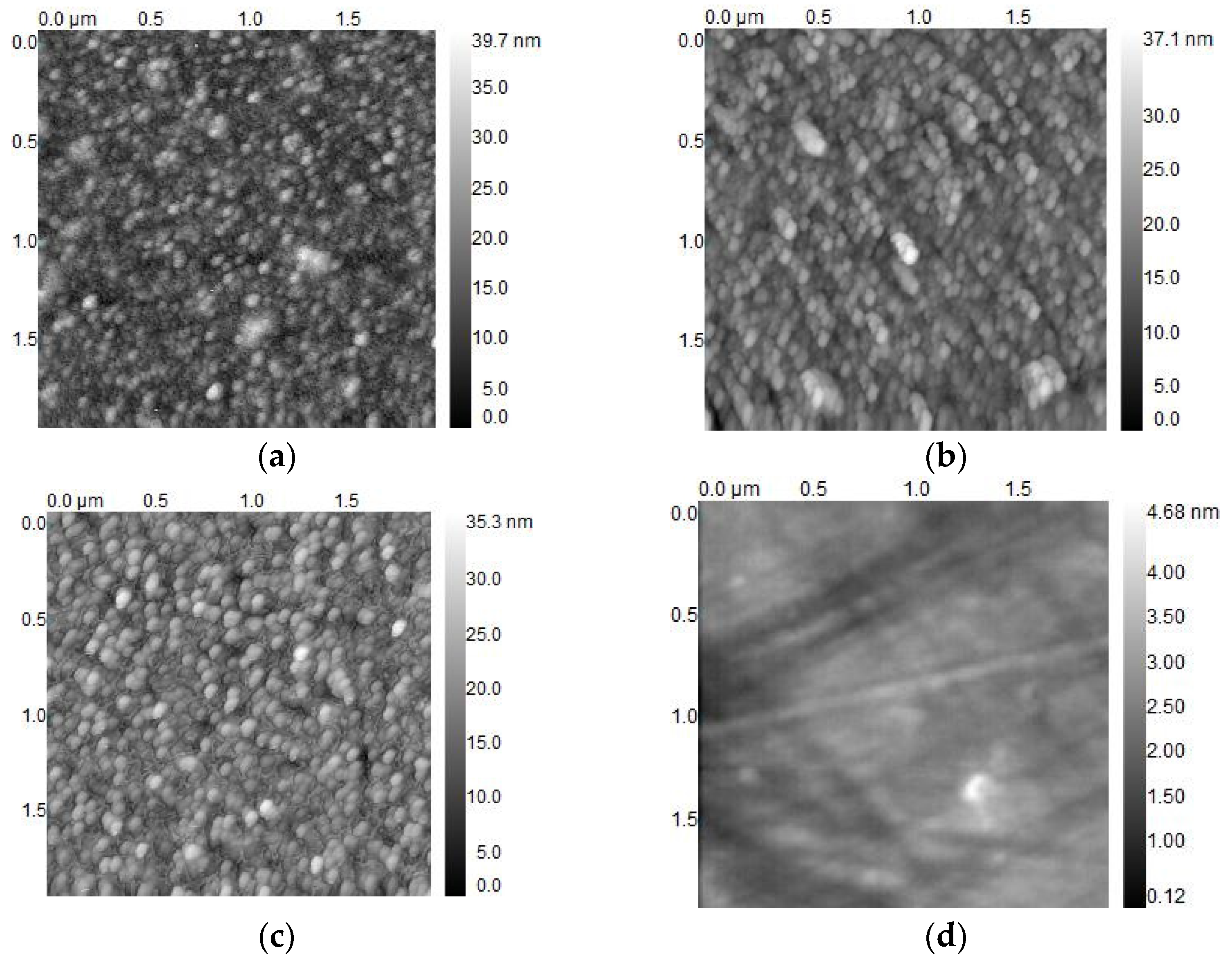
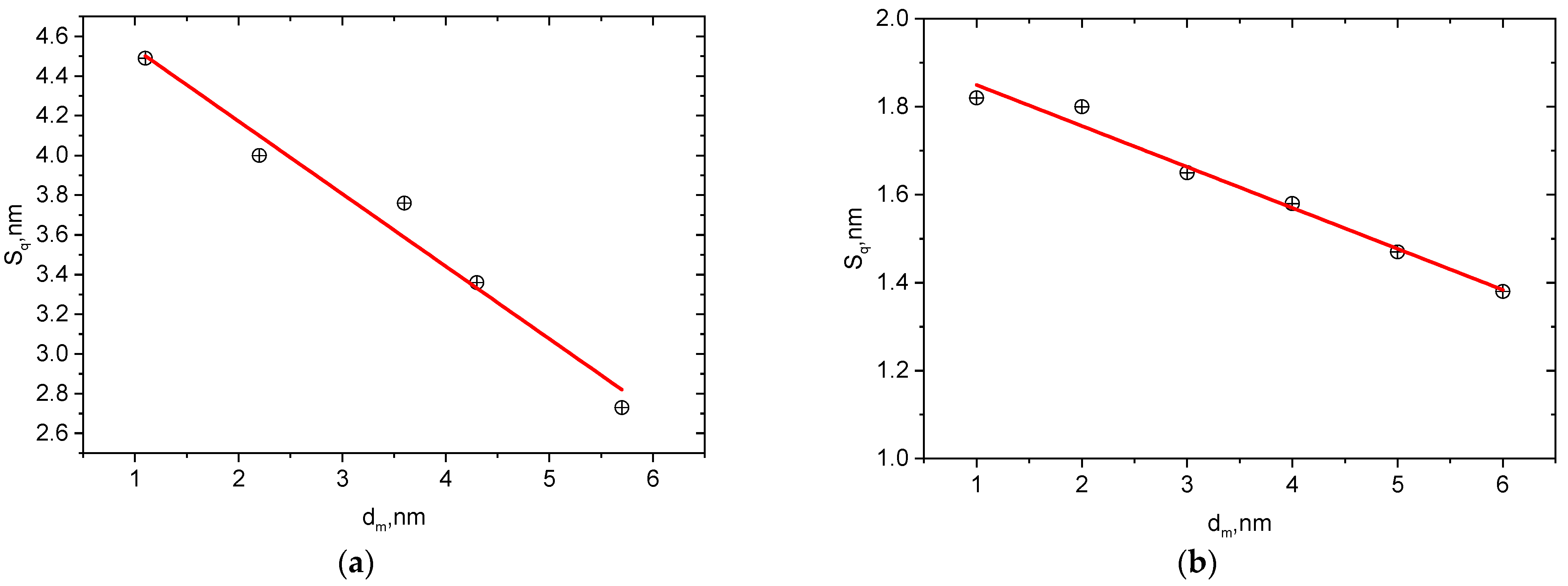
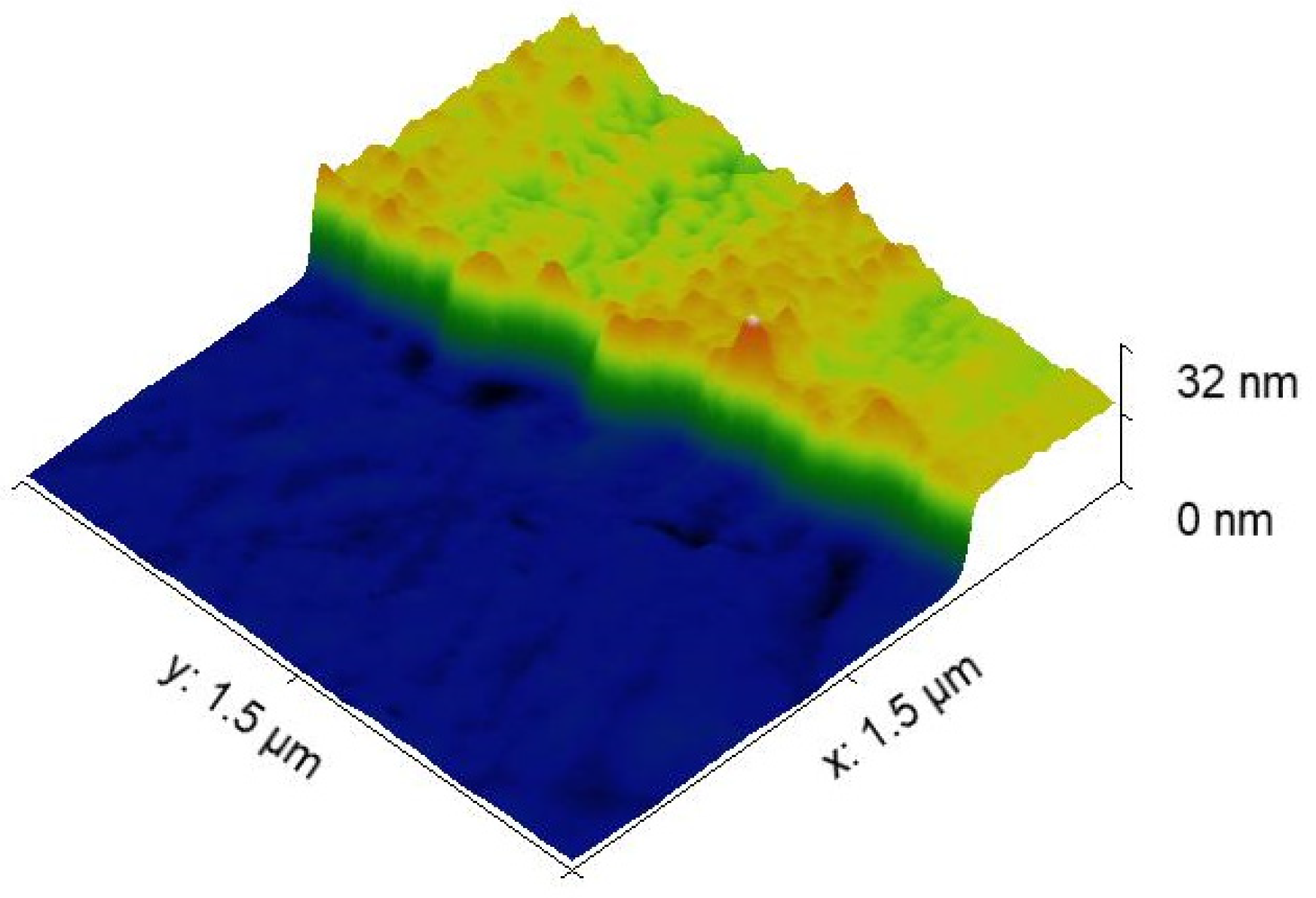
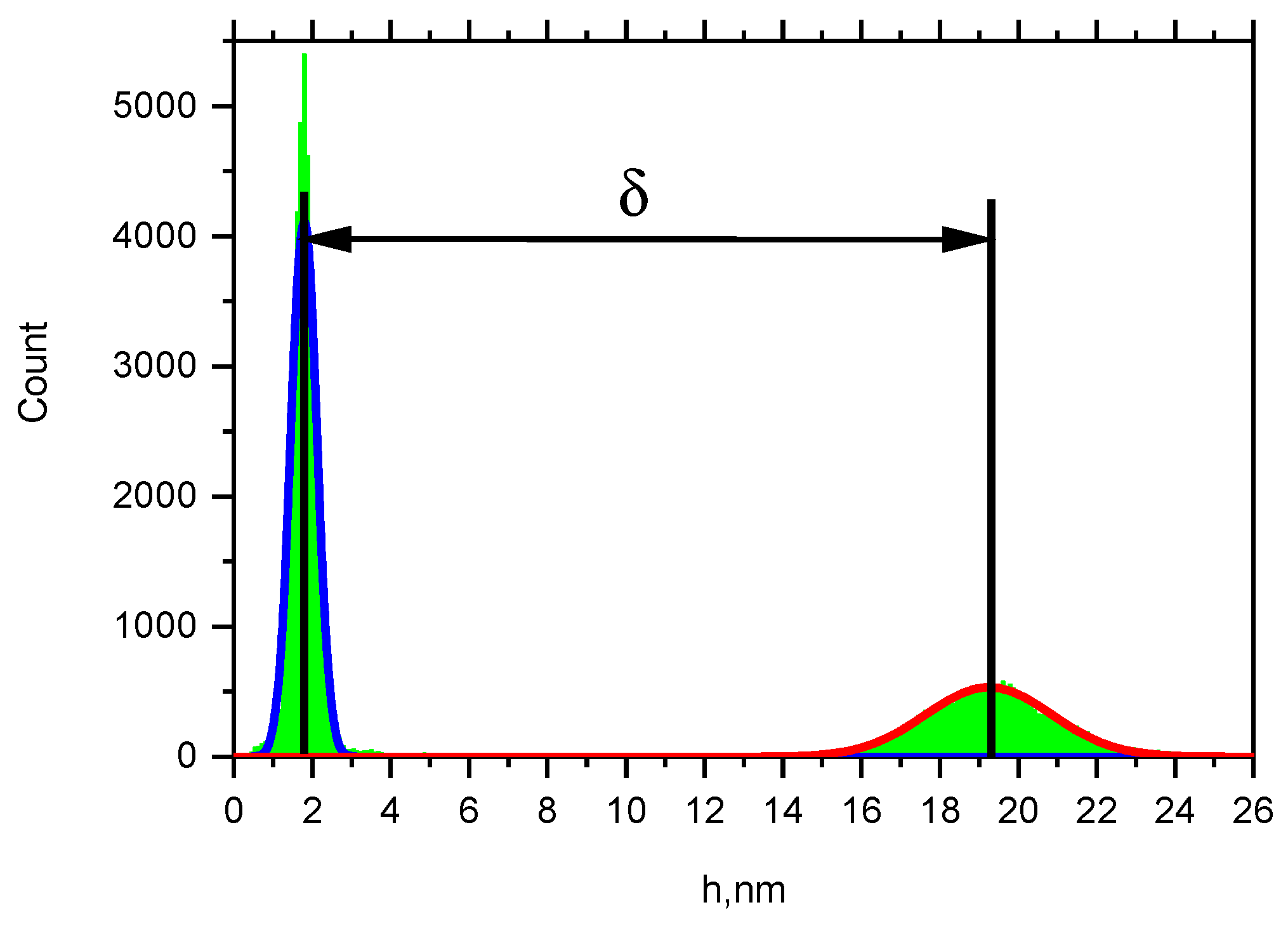
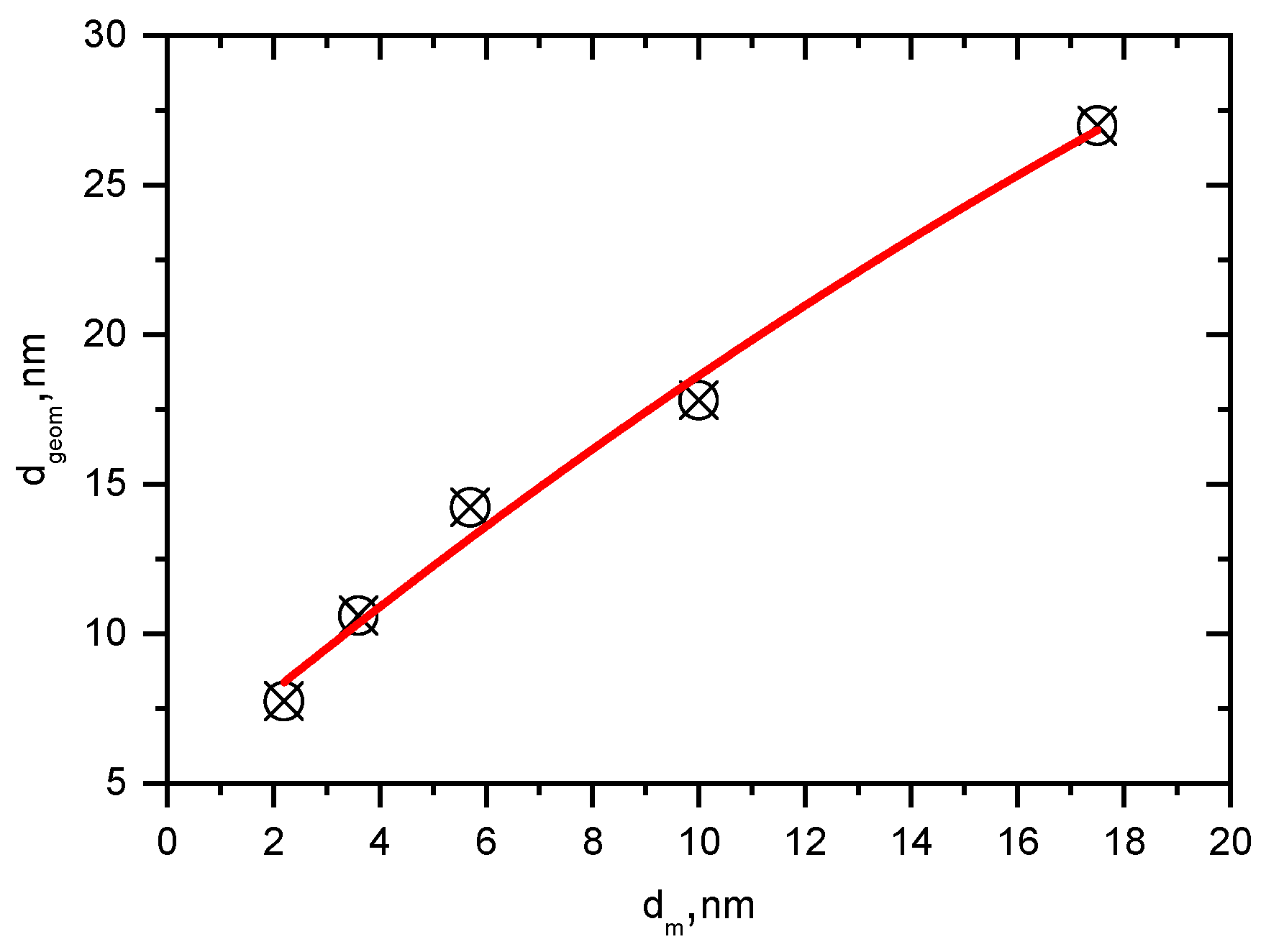
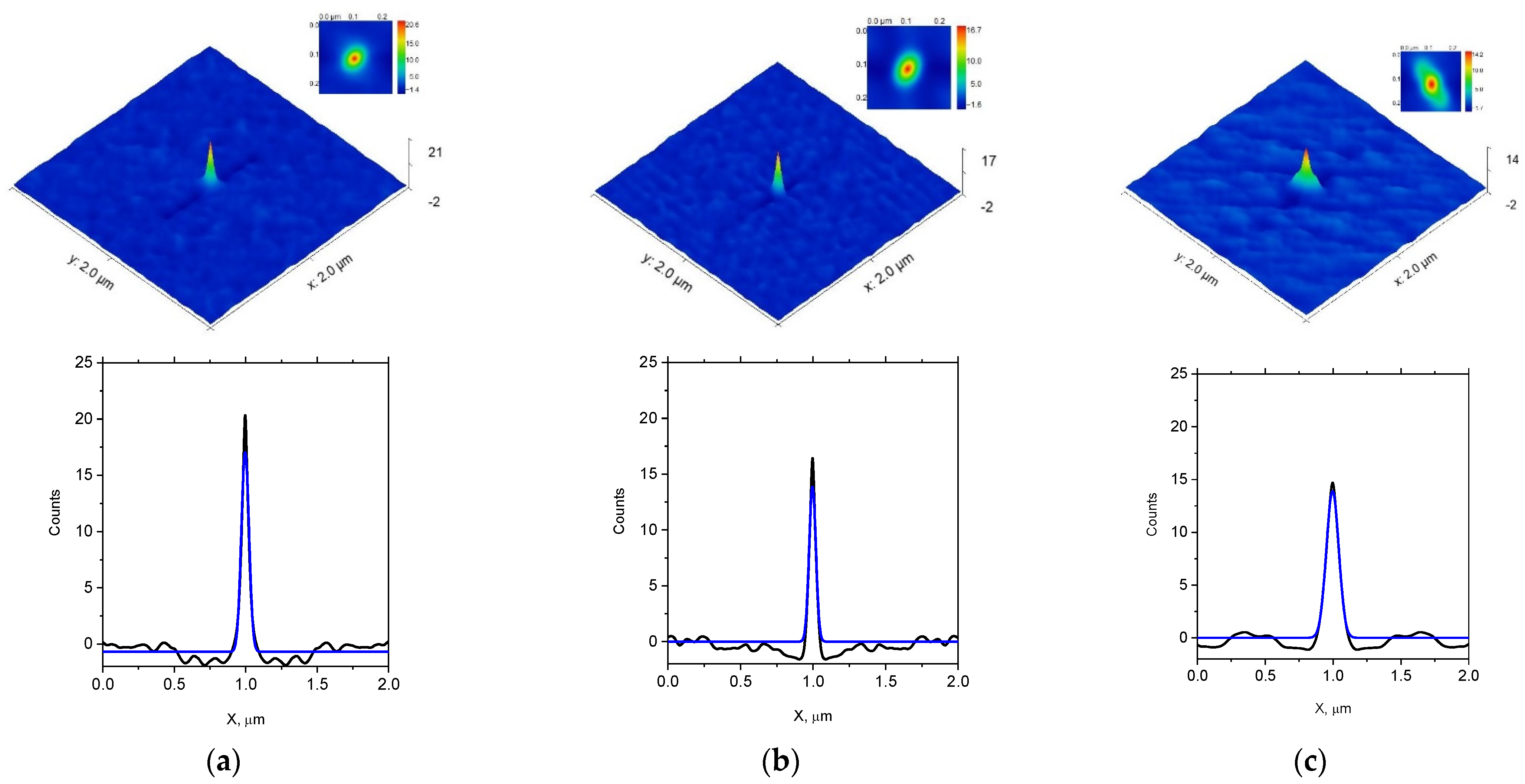
3. Results and Discussion
4. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Niguma, R.; Matsuyama, T.; Wada, K.; Okamoto, K. Novel Plasmonic Metamaterials Based on Metal Nano-Hemispheres and Metal-Dielectric Composites. Photonics 2024, 11, 356. [Google Scholar] [CrossRef]
- Ren, J.; Liang, D.; Liu, H.; Yang, Y.; Li, A.; Sun, Y.; Wang, C. High-temperature thermal stable solar selective absorbing coating based on the dielectric-metal-dielectric structure. Mater. Today Phys. 2023, 34, 101092. [Google Scholar] [CrossRef]
- Klym, H.; Karbovnyk, I.; Piskunov, S.; Popov, A.I. Positron annihilation lifetime spectroscopy insight on free volume conversion of nanostructured MgAl2O4 ceramics. Nanomaterials 2021, 11, 3373. [Google Scholar] [CrossRef]
- Karbovnyk, I.; Borshchyshyn, I.; Vakhula, Y.; Lutsyuk, I.; Klym, H.; Bolesta, I. Impedance characterization of Cr3+, Y3+ and Zr4+ activated forsterite nanoceramics synthesized by sol–gel method. Ceram. Int. 2016, 42, 8501–8504. [Google Scholar] [CrossRef]
- Nur-E-Alam, M.; Basher, M.K.; Vasiliev, M.; Das, N. Physical vapor-deposited silver (Ag)-based metal-dielectric nanocomposites for thin-film and coating applications. Appl. Sci. 2021, 11, 6746. [Google Scholar] [CrossRef]
- Klym, H.; Ingram, A.; Shpotyuk, O.; Karbovnyk, I. Influence of CsCl addition on the nanostructured voids and optical properties of 80GeS2-20Ga2S3 glasses. Opt. Mater. 2016, 59, 39–42. [Google Scholar] [CrossRef]
- Wang, W.; Qi, L. Light management with patterned micro-and nanostructure arrays for photocatalysis, photovoltaics, and optoelectronic and optical devices. Adv. Funct. Mater. 2019, 29, 1807275. [Google Scholar] [CrossRef]
- Cheng, P.; An, Y.; Jen, A.K.Y.; Lei, D. New Nanophotonics Approaches for Enhancing the Efficiency and Stability of Perovskite Solar Cells. Adv. Mater. 2024, 36, 2309459. [Google Scholar] [CrossRef]
- Nur-E-Alam, M.; Lonsdale, W.; Vasiliev, M.; Alameh, K. Application-Specific oxide-based and metal–dielectric thin-film materials prepared by radio frequency magnetron sputtering. Materials 2019, 12, 3448. [Google Scholar] [CrossRef]
- Chlebus, R.; Chylek, J.; Ciprian, D.; Hlubina, P. Surface plasmon resonance based measurement of the dielectric function of a thin metal film. Sensors 2018, 18, 3693. [Google Scholar] [CrossRef]
- Willey, R.R.; Stenzel, O. Designing optical coatings with incorporated thin metal films. Coatings 2023, 13, 369. [Google Scholar] [CrossRef]
- Kravets, V.; Poperenko, L.; Kudryavtsev, Y.; Kovanzhi, P. Optical properties and electron characteristics of noble-metal-dielectric oxide nanostructures with covered graphene layer. Opt. Mater. X 2023, 19, 100256. [Google Scholar] [CrossRef]
- Eldabagh, N.; Micek, M.; DePrince, A.E., III; Foley, J.J., IV. Resonance Energy Transfer Mediated by Metal–Dielectric Composite Nanostructures. J. Phys. Chem. C 2018, 122, 18256–18265. [Google Scholar] [CrossRef]
- Yue, D.; Zhang, W.; Wang, P.; Zhang, Y.; Teng, Y.; Yin, J.; Feng, Y. Constructing asymmetric gradient structures to enhance the energy storage performance of PEI-based composite dielectrics. Mater. Horiz. 2024, 11, 726–736. [Google Scholar] [CrossRef] [PubMed]
- Ge, S.; Liu, S.; Feng, W.; Bao, Z.; Liu, Y.; Xue, Y.; Cheng, B. Achieving high energy storage performance and thermal stability concurrently in the cost-cutting Al2O3/Ba0. 6Sr0. 4Ti0. 95Ce0.05O3/ZrO2 composite films for energy storage applications. Ceram. Int. 2023, 49, 9155–9164. [Google Scholar] [CrossRef]
- Katyal, J.; Badoni, V. Localized surface plasmon resonance and field enhancement of Au, Ag, Al and Cu nanoparticles having isotropic and anisotropic nanostructure. Mater. Today Proc. 2021, 44, 5012–5017. [Google Scholar] [CrossRef]
- Coello, V.; Abdulkareem, M.U.A.; Garcia-Ortiz, C.E.; Sosa-Sánchez, C.T.; Téllez-Limón, R.; Peña-Gomar, M. Plasmonic Coupled Modes in a Metal–Dielectric Periodic Nanostructure. Micromachines 2023, 14, 1713. [Google Scholar] [CrossRef]
- Gentile, A.; Ruffino, F.; Grimaldi, M.G. Complex-morphology metal-based nanostructures: Fabrication, characterization, and applications. Nanomaterials 2016, 6, 110. [Google Scholar] [CrossRef]
- Gompf, B.; Dressel, M.; Berrier, A. Impedance spectroscopy and equivalent circuits of metal-dielectric composites around the percolation threshold. Appl. Phys. Lett. 2018, 113, 243104. [Google Scholar] [CrossRef]
- Chen, H.; Wang, F.; Li, K.; Woo, K.C.; Wang, J.; Li, Q.; Sun, L.-D.; Zhang, X.; Lin, H.-Q.; Yan, C.H. Plasmonic percolation: Plasmon-manifested dielectric-to-metal transition. ACS Nano 2012, 6, 7162–7171. [Google Scholar] [CrossRef]
- Karbovnyk, I.; Klym, H.; Chalyy, D.; Zhydenko, I.; Lukashevych, D. Impedance analysis of PEDOT: PSS/CNT composites below percolation threshold. Appl. Nanosci. 2022, 12, 1263–1266. [Google Scholar] [CrossRef]
- Leong, E.S.P.; Wu, S.; Zhang, N.; Loh, W.W.; Khoo, E.H.; Si, G.Y.; Dai, H.T.; Liu, Y.J. Optical properties of ultrafine line and space polymeric nanogratings coated with metal and metal–dielectric–metal thin films. Nanotechnology 2014, 25, 055203. [Google Scholar] [CrossRef] [PubMed]
- Hedl, E.; Bregović, V.B.; Rakić, I.Š.; Bergmann, A.; Sancho-Parramon, J. Evolution of optical properties of Au thin films with thermal annealing. Opt. Mater. 2024, 150, 115129. [Google Scholar] [CrossRef]
- Cattin, L.; Jouad, E.; Stephant, N.; Louarn, G.; Morsli, M.; Hssein, M.; Mouchaal, Y.; Thouiri, S.; Addou, M.; Khelil, A.; et al. Dielectric/metal/dielectric alternative transparent electrode: Observations on stability/degradation. J. Phys. D Appl. Phys. 2017, 50, 375502. [Google Scholar] [CrossRef]
- Zhang, C.; Ji, C.; Park, Y.B.; Guo, L.J. Thin-metal-film-based transparent conductors: Material preparation, optical design, and device applications. Adv. Opt. Mater. 2021, 9, 2001298. [Google Scholar] [CrossRef]
- Vafaei, M.; Moradi, M.; Bordbar, G.H. Realization of epsilon-near-zero metamaterial stack based on dielectric-semiconductor-metal multilayers. Plasmonics 2019, 14, 1929–1937. [Google Scholar] [CrossRef]
- Pogosov, V.V. On the specific behavior of the work function and surface potential of an asymmetric metal-dielectric nanosandwich. Low Temp. Phys. 2024, 50, 342–349. [Google Scholar] [CrossRef]
- Bolesta, I.; Velgosh, S.; Datsiuk, Y.; Karbovnyk, I.; Lesivtsiv, V.; Kulay, T.; Popov, A.I.; Bellucci, S.; Cestelli Guidi, M.; Marcelli, A. Optical, Infrared and Electron-Microscopy Studies of (Cdi)n Metallic Clusters in Layered CdI2 Crystals. Radiat. Meas. 2007, 42, 851–854. [Google Scholar] [CrossRef]
- Bellucci, S.; Bolesta, I.; Guidi, M.C.; Karbovnyk, I.; Lesivciv, V.; Micciulla, F.; Pastore, R.; Popov, A.; Velgosh, S. Cadmium clusters in CdI2 layered crystals: The influence on the optical properties. J. Phys. Condens. Matter 2007, 19, 395015. [Google Scholar] [CrossRef]
- Cheng, Y.L.; Peng, W.F.; Huang, C.J.; Chen, G.S.; Fang, J.S. Reliability Characteristics of Metal-Insulator-Semiconductor Capacitors with Low-Dielectric-Constant Materials. Molecules 2023, 28, 1134. [Google Scholar] [CrossRef]
- Yakovkin, I.; Reshetnyak, V. Controlling plasmon resonance of gold and silver nanoparticle arrays with help of liquid crystal. Photonics 2023, 10, 1088. [Google Scholar] [CrossRef]
- Cueva, A.; Carretero, E. Comparison of the Optical Properties of Different Dielectric Materials (SnO2, ZnO, AZO, or SiAlNx) Used in Silver-Based Low-Emissivity Coatings. Coatings 2023, 13, 1709. [Google Scholar] [CrossRef]
- Li, M.; Li, Y.; Zou, N.; Wu, J.; Wei, W.; Cao, G.; Yang, J. Optimizing the design of highly linearly polarized white LED with multilayer metal-dielectric grating structure. Opt. Mater. 2024, 147, 114684. [Google Scholar] [CrossRef]
- Sardar, M.R.; Faisal, M. Numerical analysis of highly sensitive twin-core, gold-coated, D-shaped photonic crystal fiber based on surface plasmon resonance sensor. Sensors 2023, 23, 5029. [Google Scholar] [CrossRef] [PubMed]
- Lu, L.; Luo, Z.; Xu, T.; Yu, L. Cooperative plasmonic effect of Ag and Au nanoparticles on enhancing performance of polymer solar cells. Nano Lett. 2013, 13, 59–64. [Google Scholar] [CrossRef]
- Alkhalayfeh, M.A.; Aziz, A.A.; Pakhuruddin, M.Z.; Katubi, K.M.M. Plasmonic effects of Au@ Ag nanoparticles in buffer and active layers of polymer solar cells for efficiency enhancement. Materials 2022, 15, 5472. [Google Scholar] [CrossRef] [PubMed]
- Mitra, S.; Basak, M. Diverse bio-sensing and therapeutic applications of plasmon enhanced nanostructures. Mater. Today 2022, 57, 225–261. [Google Scholar] [CrossRef]
- Badshah, M.A.; Koh, N.Y.; Zia, A.W.; Abbas, N.; Zahra, Z.; Saleem, M.W. Recent developments in plasmonic nanostructures for metal enhanced fluorescence-based biosensing. Nanomaterials 2020, 10, 1749. [Google Scholar] [CrossRef]
- Alberti, G.; Zanoni, C.; Magnaghi, L.R.; Biesuz, R. Gold and silver nanoparticle-based colorimetric sensors: New trends and applications. Chemosensors 2021, 9, 305. [Google Scholar] [CrossRef]
- Pryjmaková, J.; Kaimlová, M.; Hubáček, T.; Švorčík, V.; Siegel, J. Nanostructured materials for artificial tissue replacements. Int. J. Mol. Sci. 2020, 21, 2521. [Google Scholar] [CrossRef]
- Wei, H.; Eilers, H. From silver nanoparticles to thin films: Evolution of microstructure and electrical conduction on glass substrates. J. Phys. Chem. Solids 2009, 70, 459–465. [Google Scholar] [CrossRef]
- Bolesta, I.M.; Borodchuk, A.V.; Kushnir, A.A.; Kolych, I.I.; Syworotka, I.I. Morphology and absorption spectra of ultra-thin films of silver. J. Phys. Stud. 2011, 15, 4703. [Google Scholar] [CrossRef]
- Bolesta, I.; Kolych, I.; Kushnir, A.; Karbovnyk, I.; Collins, J.; Gamernyk, R.; Luchechko, A.; Rykhlyuk, S. Local fields in nanostructured silver films. J. Nanophotonics 2014, 8, 083087. [Google Scholar] [CrossRef]
- Gwyddion. Available online: https://gwyddion.net/ (accessed on 5 July 2024).
- Lončarić, M.; Sancho-Parramon, J.; Pavlović, M.; Zorc, H.; Dubček, P.; Turkovic, A.; Bernstorff, S.; Jakopic, G.; Haase, A. Optical and structural characterization of silver island films on glass substrates. Vacuum 2009, 84, 188–192. [Google Scholar] [CrossRef]
- Xu, G.; Tazawa, M.; Jin, P.; Nakao, S. Surface plasmon resonance of sputtered Ag films: Substrate and mass thickness dependence. Appl. Phys. A 2005, 80, 1535–1540. [Google Scholar] [CrossRef]
- Bihun, R.I.; Stasyuk, Z.V.; Gavrylukh, M.V.; Leonov, D.S. Influence of Silicon Sublayers on the Optical Properties of Silver Thin Films. Met. Adv. Technol. 2019, 41, 1567–1574. [Google Scholar] [CrossRef]
- Bihun, R.; Koman, B. Nanoscale Metal Film Electronics. Traditions and New Scientific Strategies in the Context of Global Transformation of Society: Scientific Monograph; Part 1. “Physical and mathematical sciences”; Baltija Publishing: Riga, Latvia, 2024; pp. 1–33. [Google Scholar] [CrossRef]
- Lee, G.J.; Lee, Y.; Jung, B.-Y.; Jung, S.; Hwangbo, C.K.; Kim, J.; Yoon, C. Microstructural and Nonlinear Optical Properties of Thin Silver Films Near the Optical Percolation Threshold. J. Korean Phys. Soc. 2007, 51, 1555. [Google Scholar] [CrossRef]
- Degarmo, E.P.; Black, J.; Kohser, R.A. Materials and Processes in Manufacturing, 9th ed.; Wiley: New York, NY, USA, 2003. [Google Scholar]
- Lutter, L.; Serpell, C.; Tuite, M.; Serpell, L.; Xue, W.-F. Three-dimensional reconstruction of individual helical nano-filament structures from atomic force microscopy topographs. Biomol. Concepts 2020, 11, 102–115. [Google Scholar] [CrossRef]
- Bellotti, R.; Picotto, G.B.; Ribotta, L. AFM Measurements and Tip Characterization of Nanoparticles with Different Shapes. Nanomanufacturing Metrol. 2022, 5, 127–138. [Google Scholar] [CrossRef]
- Marques-Moros, F.; Forment-Aliaga, A.; Pinilla-Cienfuegos, E. Mirror effect in atomic force microscopy profiles enables tip reconstruction. Sci. Rep. 2020, 10, 18911. [Google Scholar] [CrossRef]
- Arildsen, T.; Oxvig, C.S.; Pedersen, P.S.; Ostergaard, J.; Larsen, T. Reconstruction Algorithms in Undersampled AFM Imaging. IEEE J. Sel. Top. Signal Process. 2016, 10, 31–46. [Google Scholar] [CrossRef]
- Liu, H.; Wang, B.; Leong, E.S.P.; Yang, P.; Zong, Y.; Si, G.; Maier, S.A. Enhanced Surface Plasmon Resonance on a Smooth Silver Film with a Seed Growth Layer. ACS Nano 2010, 4, 3139–3146. [Google Scholar] [CrossRef]
- Liang, S.; Schwartzkopf, M.; Roth, S.V.; Müller-Buschbaum, P. State of the art of ultra-thin gold layers: Formation fundamentals and applications. Nanoscale Adv. 2022, 4, 2533–2560. [Google Scholar] [CrossRef] [PubMed]
- Jardim, S.; António, J.; Mora, C. Graphical Image Region Extraction with K-Means Clustering and Watershed. J. Imaging 2022, 8, 163. [Google Scholar] [CrossRef]
- Xu, X.; Xu, S.; Jin, L.; Song, E. Characteristic analysis of Otsu threshold and its applications. Pattern Recognit. Lett. 2011, 32, 956–961. [Google Scholar] [CrossRef]
- Klym, H.; Kushnir, O.; Karbovnyk, I. Surface crystallization of GeSe2 in the 80GeSe2–20Ga2Se3 glasses caused by thermal annealing: Experimental study and statistical analysis. Appl. Nanosci. 2023, 13, 7445–7454. [Google Scholar] [CrossRef]
- Zbilut, J.P.; Marwan, N. The Wiener–Khinchin theorem and recurrence quantification. Phys. Lett. A 2008, 372, 6622–6626. [Google Scholar] [CrossRef]
- Gong, Y.; Misture, S.T.; Gao, P.; Mellott, N.P. Surface Roughness Measurements Using Power Spectrum Density Analysis with Enhanced Spatial Correlation Length. J. Phys. Chem. C 2016, 120, 22358–22364. [Google Scholar] [CrossRef]
- Ifeachor, E.C.; Jervis, B.W. Digital Signal Processing: A Practical Approach; Pearson Education: London, UK, 2002. [Google Scholar]
- Schroeder, M. Fractals, Chaos, Power Laws: Minutes from an Infinite Paradise; Courier Corporation: New York, NY, USA, 2009. [Google Scholar]




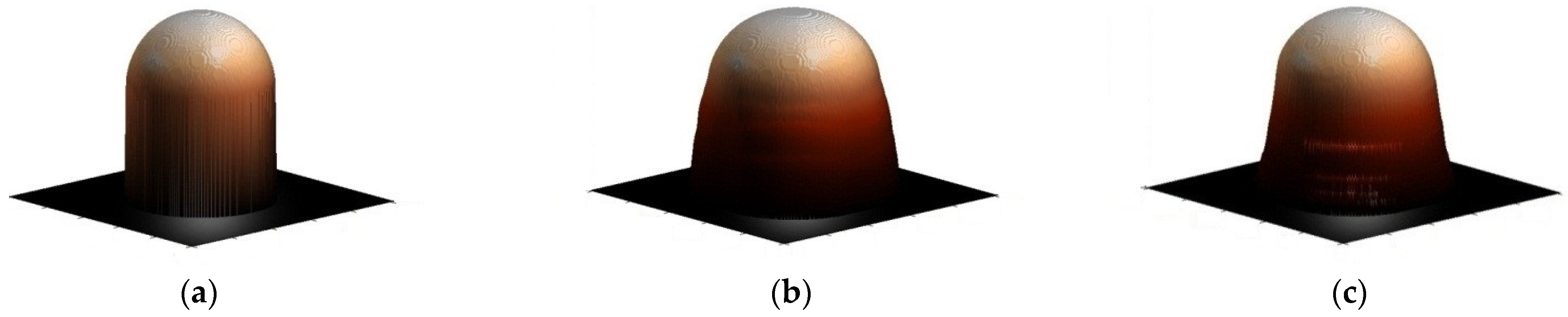
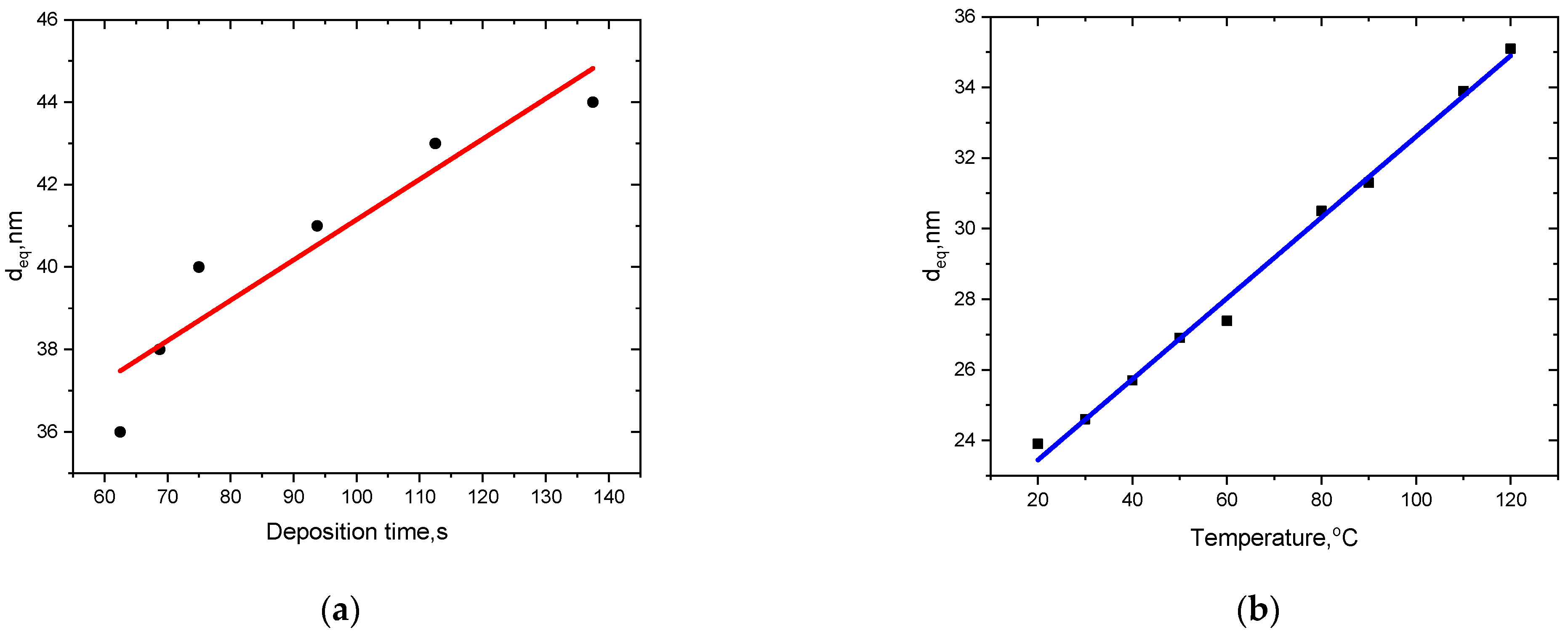



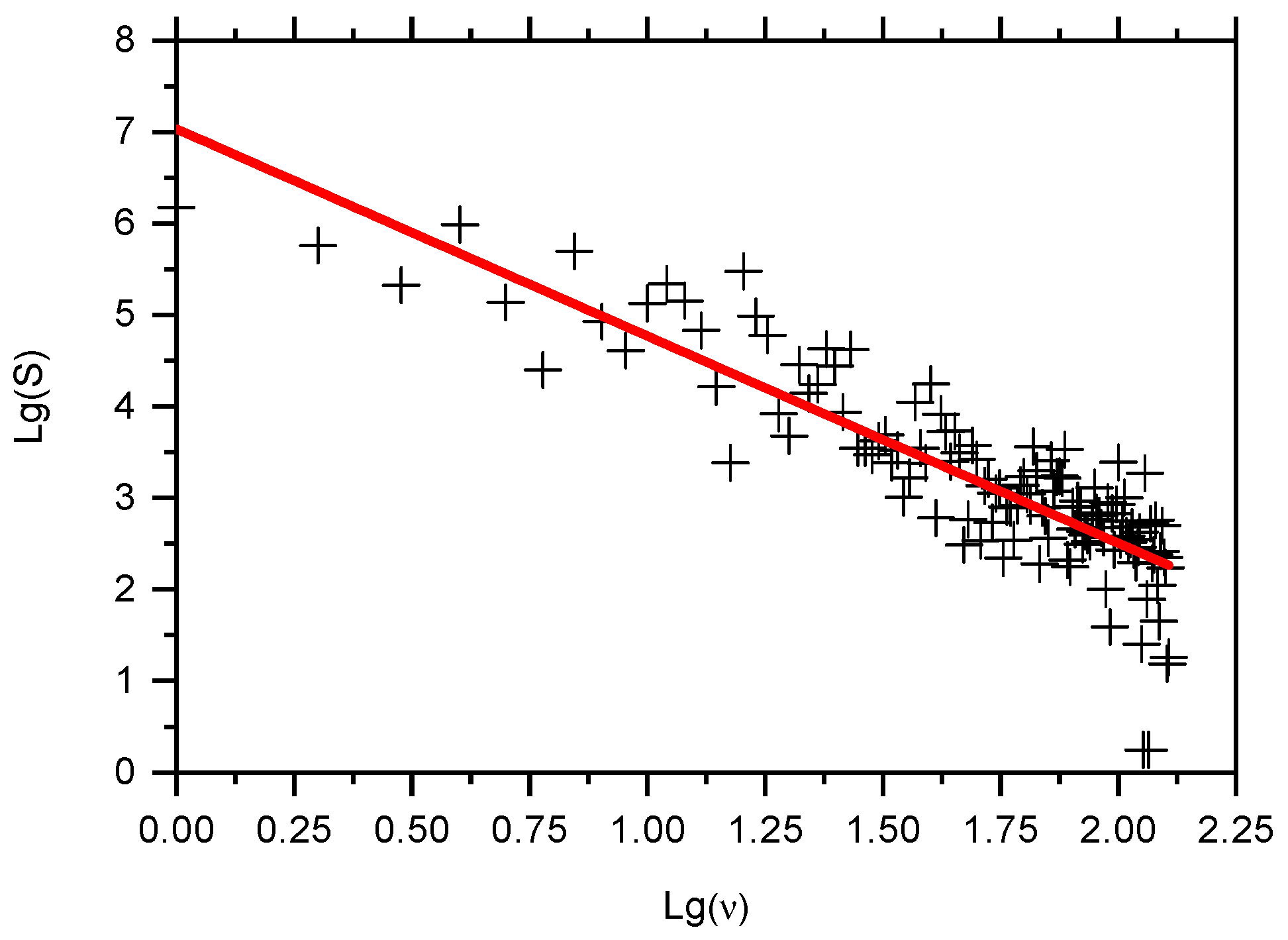

| dm (±0.1), nm | a (±2), nm | b (±2), nm | c (±1), nm |
|---|---|---|---|
| 1.0 | 40 | 30 | 31 |
| 1.1 | 45 | 32 | 32 |
| 1.2 | 47 | 33 | 32 |
| 1.5 | 49 | 33 | 35 |
| 1.8 | 53 | 34 | 34 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Bolesta, I.; Kushnir, O.; Karbovnyk, I.; Klym, H.; Konuhova, M.; Popov, A.I. Topological and Fractal Analysis of Nanostructured Metal–Dielectric Films. Appl. Sci. 2025, 15, 3250. https://doi.org/10.3390/app15063250
Bolesta I, Kushnir O, Karbovnyk I, Klym H, Konuhova M, Popov AI. Topological and Fractal Analysis of Nanostructured Metal–Dielectric Films. Applied Sciences. 2025; 15(6):3250. https://doi.org/10.3390/app15063250
Chicago/Turabian StyleBolesta, Ivan, Oleksii Kushnir, Ivan Karbovnyk, Halyna Klym, Marina Konuhova, and Anatoli I. Popov. 2025. "Topological and Fractal Analysis of Nanostructured Metal–Dielectric Films" Applied Sciences 15, no. 6: 3250. https://doi.org/10.3390/app15063250
APA StyleBolesta, I., Kushnir, O., Karbovnyk, I., Klym, H., Konuhova, M., & Popov, A. I. (2025). Topological and Fractal Analysis of Nanostructured Metal–Dielectric Films. Applied Sciences, 15(6), 3250. https://doi.org/10.3390/app15063250








