Gain-Scheduled Disturbance Observer-Based Saturated Controllers for Non-Linear First-Order System †
Abstract
1. Introduction
1.1. The Integral Action Versus Automatic Reset
1.2. Directions of Further Development
1.3. Modern and Postmodern Model-Based Control
- The design of the stabilizing controller gain ;
- The analysis of stability limits of ;
- The design of the DOBs or ARCs with the filter time constant ;
- The analysis of stable parameters of the loops extended by ARCs and DOBs;
- The robustness analysis of the loops with stabilizing controller and with DOBs or ARCs.
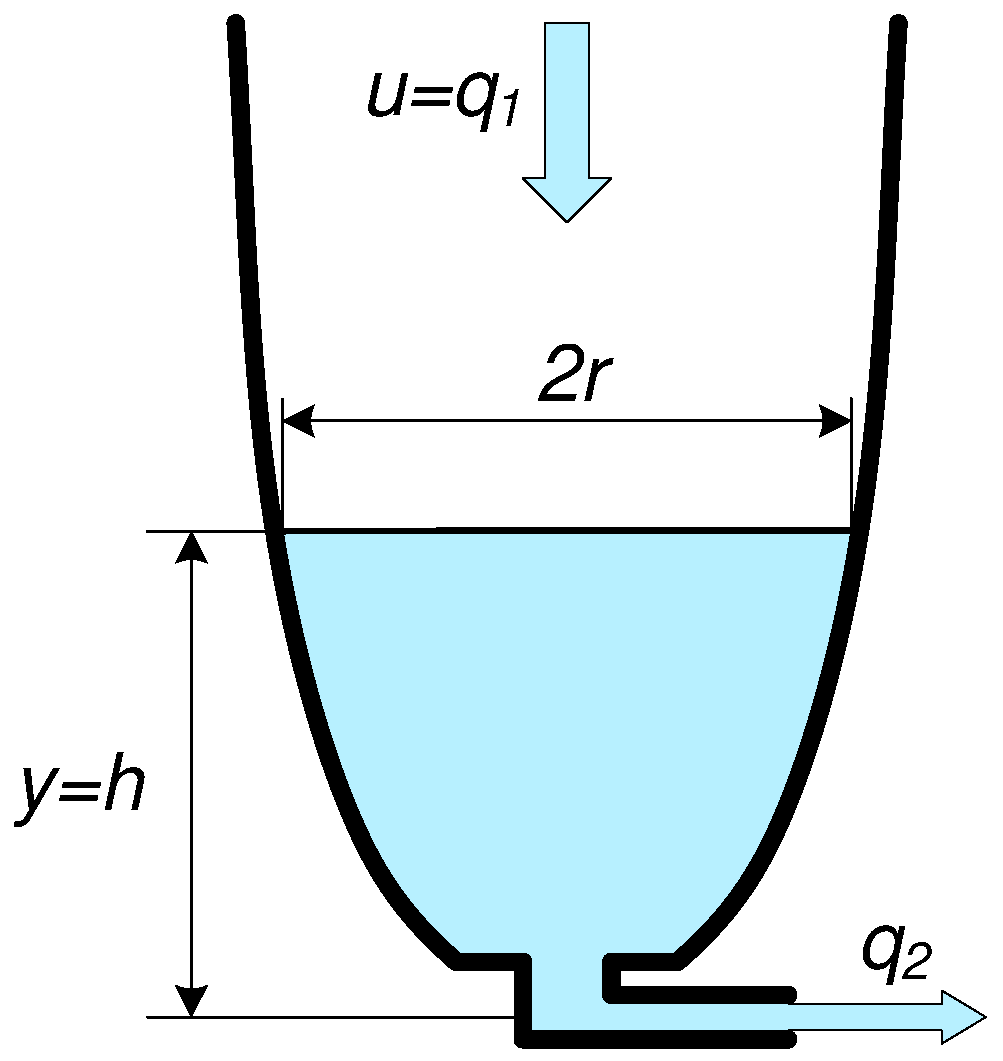
1.4. Process Modeling and Gain-Scheduled Control
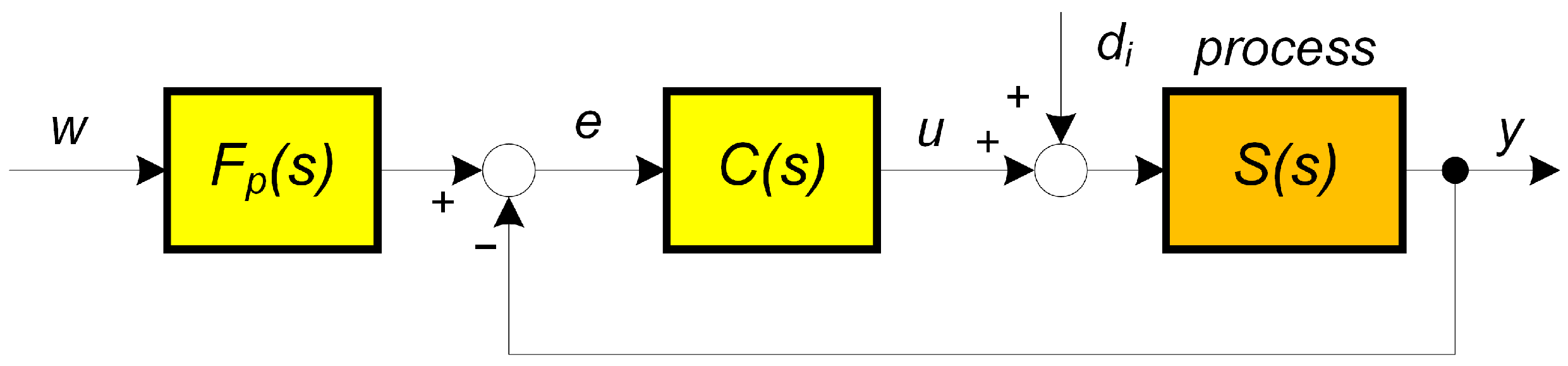
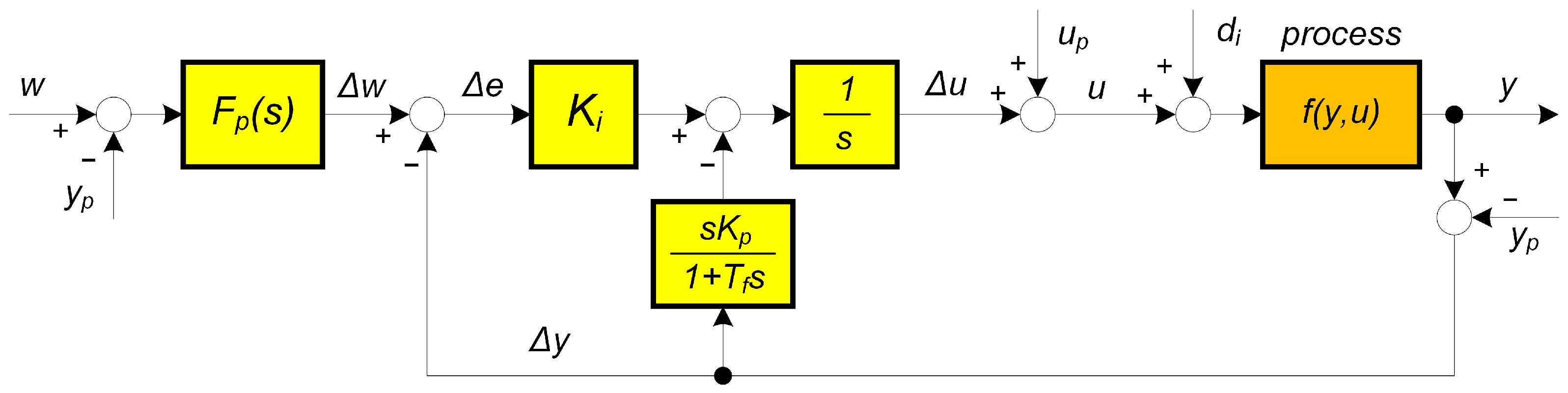
2. Generalized Constrained Non-Linear Controller Design
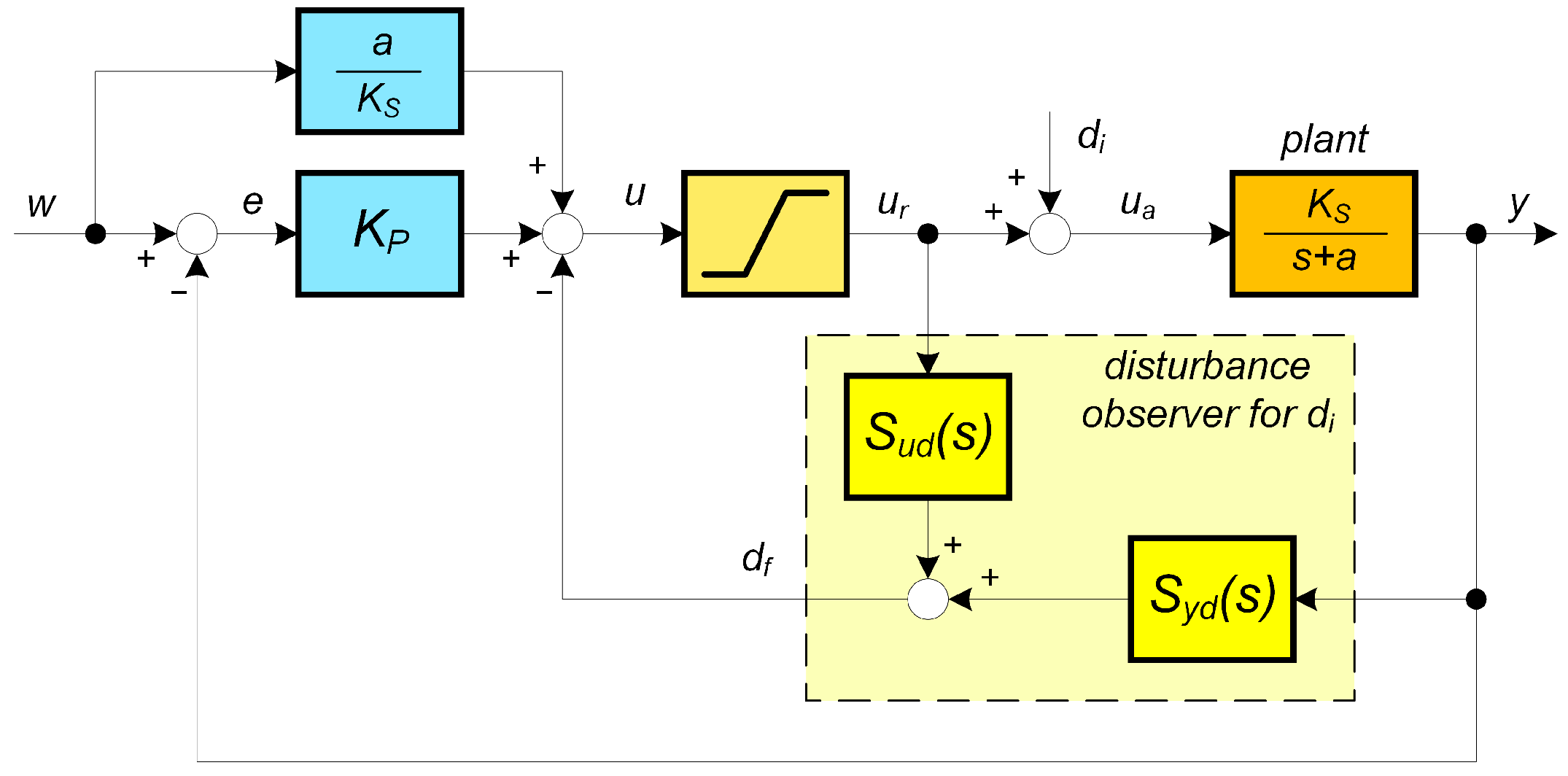
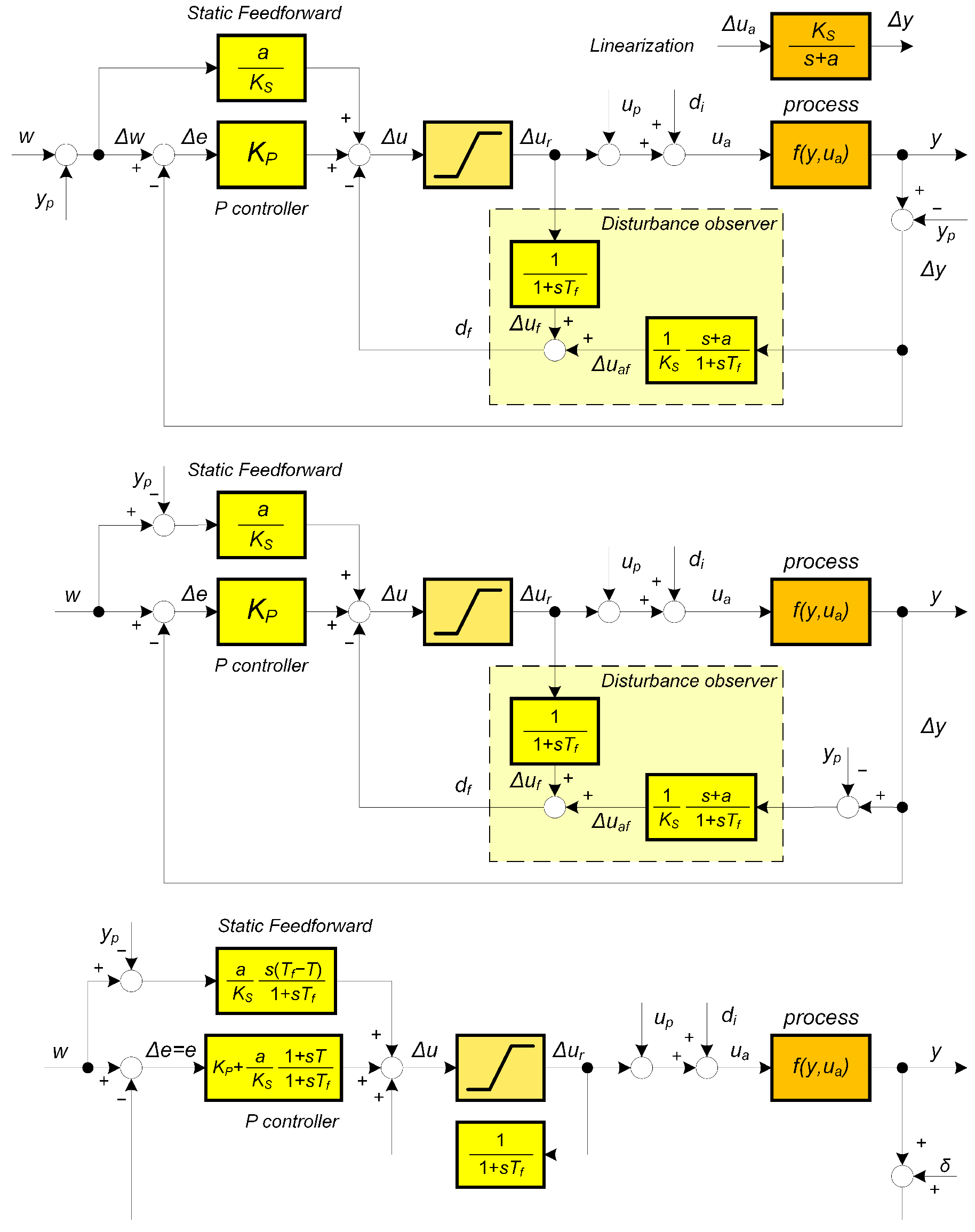
2.1. Input Disturbance Reconstruction and Compensation
2.2. Selection of the Gain-Scheduling Parameter
2.3. Gain-Scheduled GS-ARC0 Based on Ultralocal Models
- The simplest reconstruction of with is achieved for a sufficiently large , i.e., when approaching the steady-states. The disturbance reconstruction and compensation is reduced to a positive feedback from the saturation non-linearity to the offset of the stabilizing controller;
- The saturation limits when calculating the output from correspond to those of u reduced by the offset (18) added to the controller output, if
- If the output correction by the offset according to (18) is omitted, the controller output with the saturation limit defined for u compensates for an equivalent disturbance . As with ADRC, such a cumulative disturbance can then be referred to as a “lumped” or ”total” disturbance.
- As will be shown below, simplifications based on ultralocal models with can only guarantee stable processes for limited tuning parameters and .
2.4. Stability and Overshoot of GS-ARC0 Controller
2.5. GS-DOB0 Controller Based on Ultralocal Models
2.6. GS-ARC1 Generalized for Local Model
2.7. Nominal GS-DOB1 Tuning
2.8. Overview of the Design Options Considered
3. Illustrative Example
3.1. Preliminary Models and Analysis of the Controller Settings
3.2. Performance Evaluation for Unconstrained Control
3.3. Responses with Unconstrained 1DoF Controllers
- The corresponding to the local ARC1 models is larger than in the case of the simpler ultralocal ARC0 models.
- If you choose a smaller filter time constant , the overshoots are relatively large when using ARCs, while for they are about the same as when using more complex DOBs.
- Smaller values of for can apparently be achieved with DOBs. But even here, the DOB0s based on the ultralocal model result in less deviations from monotonicity () than the DOB1s.
- With the exception of ARCs, which correspond to , none of the options considered leads to excessive control effort with .
3.4. Offset Impact
3.5. Choice of the Scheduling Parameter and Stability of Transients
3.6. 2DoF Unconstrained Ultralocal Control
3.7. Impact of the Measurement Noise
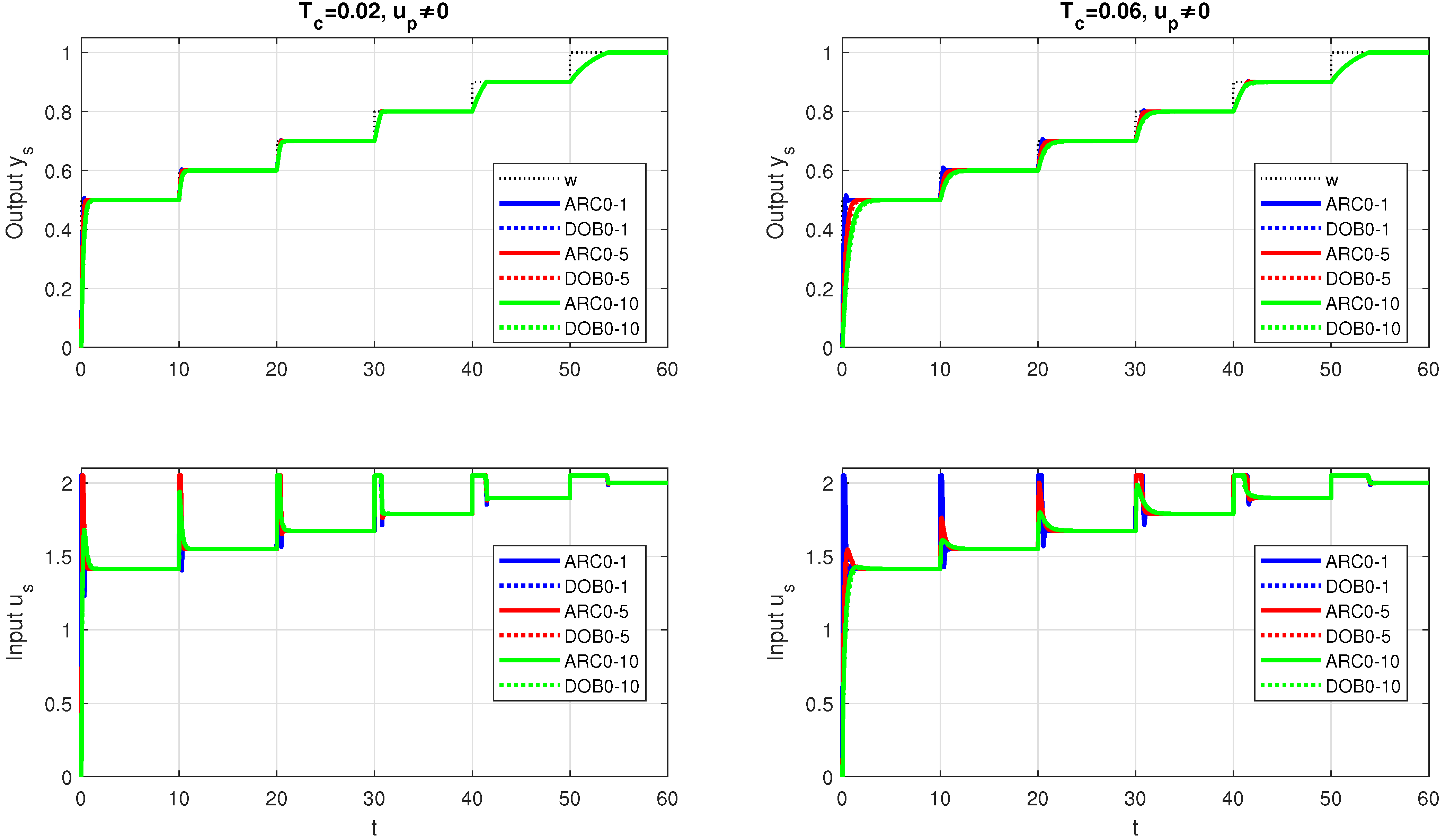
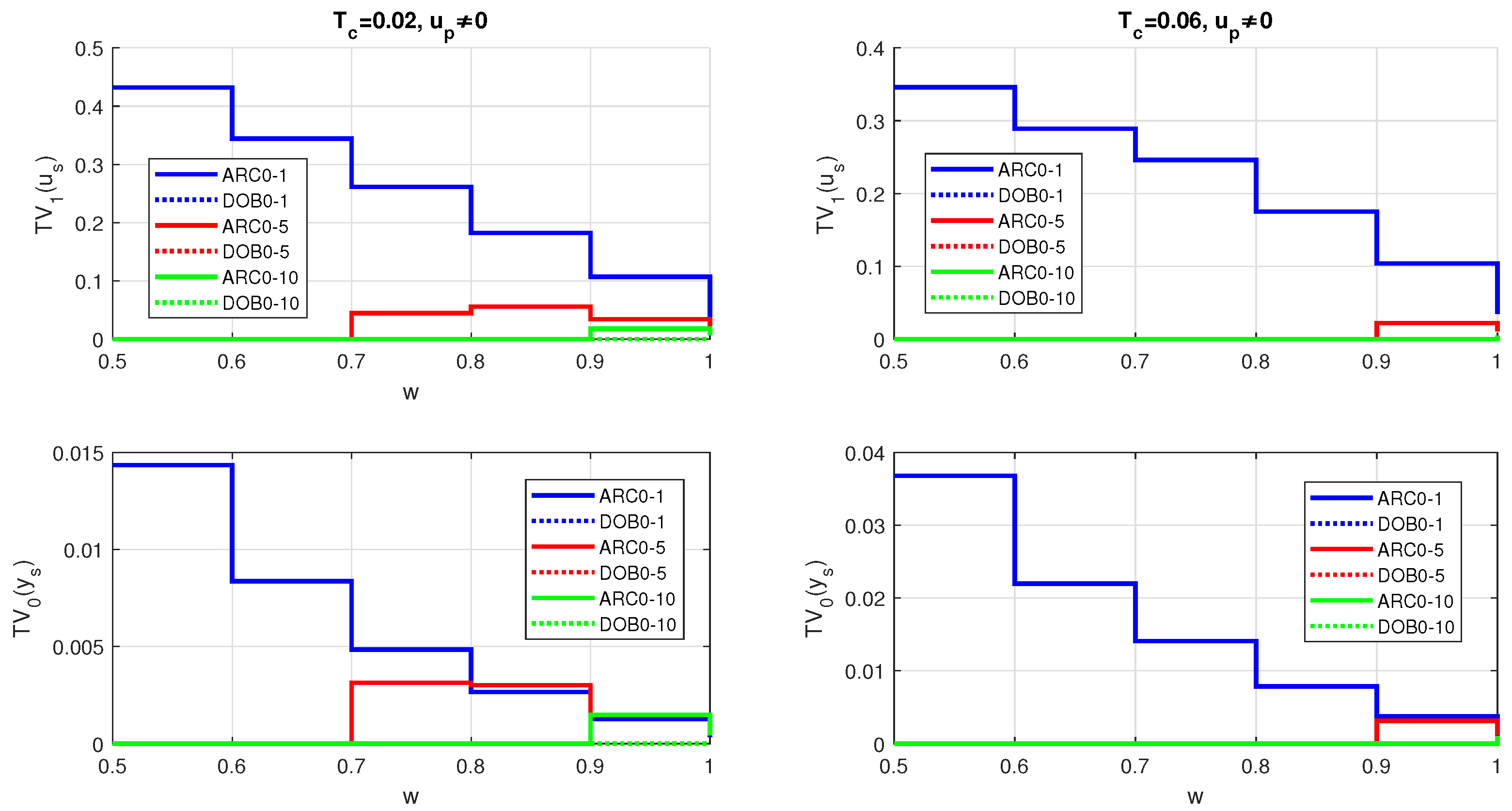
3.8. Constrained Control
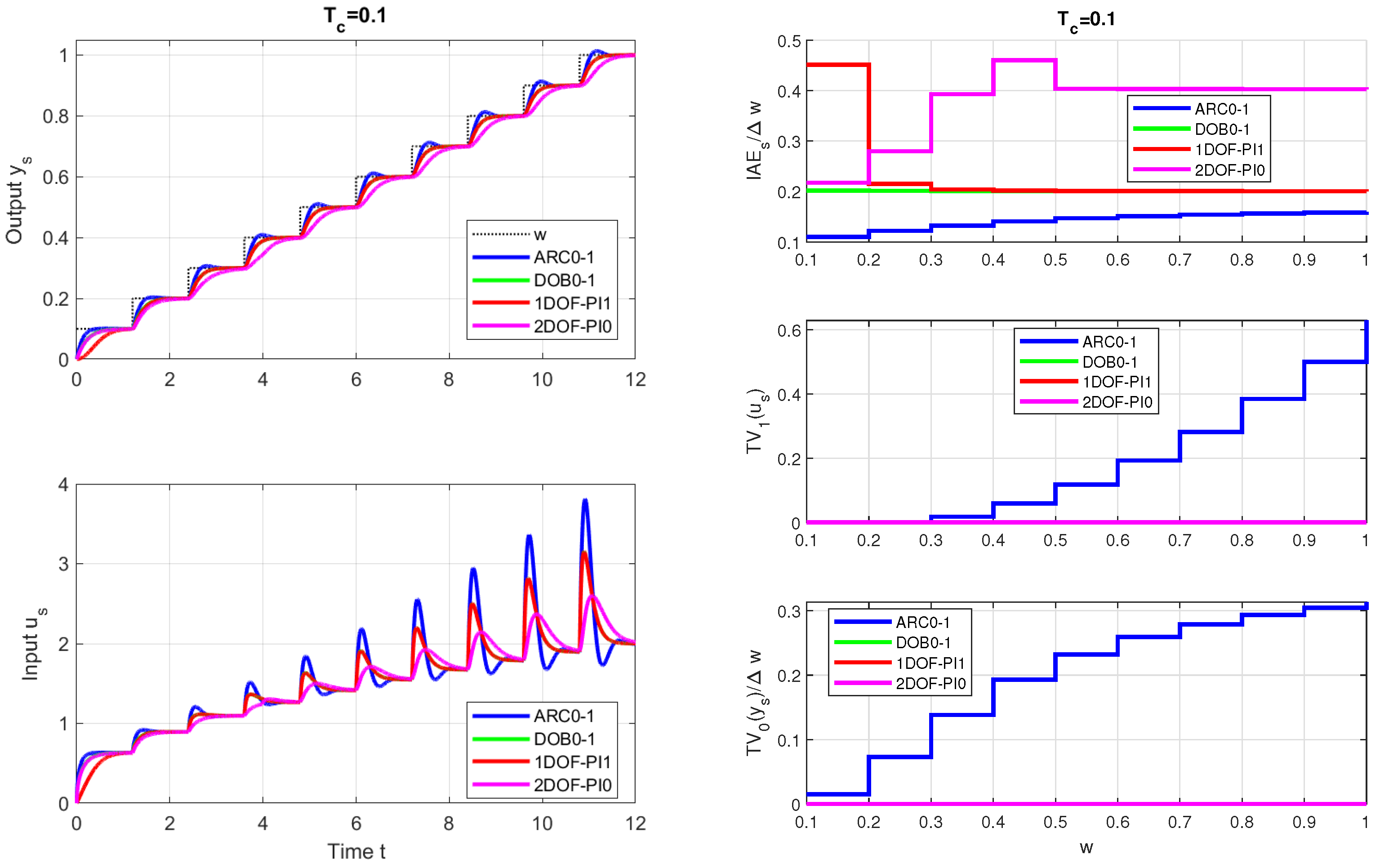
3.9. Comparison with Gain-Scheduled PI Control
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
Abbreviations
| 1P | One-Pulse, response with two monotonic segments (one extreme point) |
| 1DoF | One Degree of Freedom |
| 2DoF | Two Degrees of Freedom |
| AR | Automatic Reset |
| ARC | Automatic Reset Controller |
| ADRC | Active Disturbance Rejection Control |
| DOB | Disturbance Observer |
| DOBC | Disturbance Observer-Based Control |
| ESO | Extended State Observer |
| GS | Gain-Scheduled |
| HO | Higher-Order |
| HO-AR | Higher-Order Automatic Reset |
| Integral of Absolute Error | |
| IMC | Internal Model Control |
| I/O | Input–Output |
| MCT | Modern Control Theory |
| MFC | Model-Free Control |
| P | Proportional |
| PI | Proportional–Integral |
| PID | Proportional–Integral–Derivative |
| SISO | Single-Input Single-Output |
| TV | Total Variation |
| TV0 | Deviation from Monotonicity |
| TV1 | Deviation from 1P Shape |
References
- Bennett, S. Development of the PID controller. Control Syst. IEEE 1993, 13, 58–62. [Google Scholar]
- Bennet, S. A Brief History of Automatic Control. IEEE Control Syst. 1996, 16, 17–25. [Google Scholar]
- Bennett, S. The Past of PID Controllers. In Proceedings of the IFAC Workshop on Digital Control: Past, Present and Future of PID Control, Terrassa, Spain, 5–7 April 2000; IFAC Proceedings Volumes. Volume 33, pp. 1–11. [Google Scholar]
- Skogestad, S. PID is the Future of Advanced Control. In Proceedings of the 4th IFAC Conference on Advances in Proportional-Integral-Derivative Control PID 2024, Almería, Spain, 12–14 June 2024. IFAC-PapersOnLine. [Google Scholar]
- Huba, M.; Bistak, P.; Vrancic, D. Practice-oriented controller design of a simple nonlinear system. In Proceedings of the 18th IFAC Int. Conf. on Programmable Devices and Embedded Systems—PDES 2024, Brno, Czech Republic, 19–21 June 2024. [Google Scholar]
- Kaminer, I.; Pascoal, A.; Khargonekar, P.; Coleman, E. A Velocity Algorithm for the Implementation of Gain-scheduled Controllers. Automatica 1995, 31, 1185–1191. [Google Scholar] [CrossRef]
- Glattfelder, A.; Schaufelberger, W. Control Systems with Input and Output Constraints; Springer: London, UK, 2003. [Google Scholar]
- Ziegler, J.G.; Nichols, N.B. Optimum settings for automatic controllers. Trans. ASME 1942, 64, 759–768. [Google Scholar] [CrossRef]
- Åström, K.J.; Hägglund, T. Advanced PID Control; ISA: Research Triangle Park, NC, USA, 2006. [Google Scholar]
- Huba, M.; Chamraz, S.; Bisták, P.; Vrančić, D. Making the PI and PID Controller Tuning Inspired by Ziegler and Nichols Precise and Reliable. Sensors 2021, 18, 6157. [Google Scholar] [CrossRef]
- Åström, K.J.; Hägglund, T. PID Controllers: Theory, Design, and Tuning, 2nd ed.; Instrument Society of America: Research Triangle Park, NC, USA, 1995. [Google Scholar]
- Kothare, M.V.; Campo, P.J.; Morari, M.; Nett, C.N. A Unified Framework for the Study of Anti-windup Designs. Automatica 1994, 30, 1869–1883. [Google Scholar] [CrossRef]
- Hanus, R.; Kinnaert, M.; Henrotte, J. Conditioning technique, a general anti-windup and bumpless transfer method. Automatica 1987, 23, 729–739. [Google Scholar] [CrossRef]
- Hanus, R.; Peng, Y. Conditioning technique for controllers with time delays. IEEE Trans. Aututomatic Control 1992, 37, 689–692. [Google Scholar] [CrossRef]
- Huba, M.; Vrančić, D.; Bisták, P. PID Control with Higher Order Derivative Degrees for IPDT Plant Models. IEEE Access 2021, 9, 2478–2495. [Google Scholar] [CrossRef]
- Huba, M.; Bistak, P.; Vrancic, D. Series PID Control with Higher-Order Derivatives for Processes Approximated by IPDT Models. IEEE TASE 2024, 21, 4406–4418. [Google Scholar] [CrossRef]
- Huba, M.; Bistak, P.; Vrancic, D. Parametrization and Optimal Tuning of Constrained Series PIDA Controller for IPDT Models. Mathematics 2023, 11, 4229. [Google Scholar] [CrossRef]
- Zhao, D.; Gao, C.; Li, J.; Fu, H.; Ding, D. PID control and PI state estimation for complex networked systems: A survey. Int. J. Syst. Sci. 2025, 1–16. [Google Scholar] [CrossRef]
- Kumar, V.; Hote, Y.V. New approach of series-PID controller design based on modern control theory: Simulations and real-time validation. Ifac J. Syst. Control 2025, 31, 100295. [Google Scholar] [CrossRef]
- Åström, K.J.; Panagopoulos, H.; Hägglund, T. Design of PI Controllers based on Non-Convex Optimization. Automatica 1998, 34, 585–601. [Google Scholar] [CrossRef]
- Kallannan, J.; Baskaran, A.; Dey, N.; Ashour, A. Bio-Inspired Algorithms in PID Controller Optimization; CRC Press: Boca Raton, FL, USA, 2018. [Google Scholar]
- Valluru, S.K.; Singh, M. Optimization Strategy of Bio-Inspired Metaheuristic Algorithms Tuned PID Controller for PMBDC Actuated Robotic Manipulator. Procedia Comput. Sci. 2020, 171, 2040–2049. [Google Scholar] [CrossRef]
- Dutta, P.; Pal, S.; Kumar, A.; Cengiz, K. Artificial Intelligence for Cognitive Modeling: Theory and Practice; Chapman and Hall/CRC: New York, NY, USA, 2023. [Google Scholar]
- Sahoo, A.; Mishra, S.; Acharya, D.; Chakraborty, S.; Swain, S. A comparative evaluation of a set of bio-inspired optimization algorithms for design of two-DOF robust FO-PID controller for magnetic levitation plant. Electr. Eng. 2023, 105, 3033–3054. [Google Scholar] [CrossRef]
- Luenberger, D. Observers for multivariable systems. IEEE Trans. Autom. Control 1966, 11, 190–197. [Google Scholar] [CrossRef]
- Brogan, W.L. Modern Control Theory; Prentice Hall: Upper Saddle River, NJ, USA, 1991. [Google Scholar]
- Ackermann, J. Abtastregelung; Springer: Berlin, Germany, 1988. [Google Scholar]
- Ogata, K. Modern Control Engineering, 5th ed.; Prentice Hall: Upper Saddle River, NJ, USA, 2010. [Google Scholar]
- Huba, M.; Gao, Z. Uncovering Disturbance Observer and Ultra-Local Plant Models in Series PI Controllers. Symmetry 2022, 14, 640. [Google Scholar] [CrossRef]
- Huba, M. Disturbance Observer in PID Controllers for First-Order Time-Delayed Systems. In Proceedings of the 13th IFAC Symposium Advances in Control Education, Hamburg, Germany, 24–27 July 2022. [Google Scholar]
- Huba, M.; Zakova, K.; Bistak, P.; Hypiusova, M.; Tapak, P. Seeking a unique view to control of simple models. IFAC-PapersOnLine 2019, 52, 91–96. [Google Scholar] [CrossRef]
- Gao, Z. On the centrality of disturbance rejection in automatic control. Isa Trans. 2014, 53, 850–857. [Google Scholar] [CrossRef]
- Ohishi, K. A new servo method in mechantronics. Trans. Jpn. Soc. Elect. Eng. 1987, 107-D, 83–86. [Google Scholar]
- Ohishi, K.; Nakao, M.; Ohnishi, K.; Miyachi, K. Microprocessor-Controlled DC Motor for Load-Insensitive Position Servo System. IEEE Trans. Ind. Electron. 1987, IE-34, 44–49. [Google Scholar]
- Sariyildiz, E.; Oboe, R.; Ohnishi, K. Disturbance Observer-Based Robust Control and Its Applications: 35th Anniversary Overview. IEEE Trans. Ind. Electron. 2020, 67, 2042–2053. [Google Scholar] [CrossRef]
- Morari, M.; Zafiriou, E. Robust Process Control; Prentice Hall: Englewood Cliffs, NJ, USA, 1989. [Google Scholar]
- Smith, O. Closser control of loops with dead time. Chem. Eng. Prog. 1957, 53, 217–219. [Google Scholar]
- Huba, M.; Bisták, P.; Vrančić, D.; Zakova, K. Asymmetries in the Disturbance Compensation Methods for the Stable and Unstable First Order Plants. Symmetry 2020, 12, 1595. [Google Scholar] [CrossRef]
- Åström, K.J.; Hang, C.C.; Lim, B.C. A new Smith predictor for controlling a process with an integrator and long dead-time. IEEE Trans. Autom. Control 1994, 39, 343–345. [Google Scholar] [CrossRef]
- Hölzl, S.L. The case for the Smith-Åström predictor. J. Process. Control 2023, 128, 103026. [Google Scholar] [CrossRef]
- Wu, Z.; Gao, Z.; Li, D.; Chen, Y.; Liu, Y. On transitioning from PID to ADRC in thermal power plants. Control. Theory Technol. 2021, 19, 3–18. [Google Scholar] [CrossRef]
- Lin, P.; Wu, Z.; Fei, Z.; Sun, X.M. A Generalized PID Interpretation for High-Order LADRC and Cascade LADRC for Servo Systems. IEEE Trans. Ind. Electron. 2022, 69, 5207–5214. [Google Scholar] [CrossRef]
- Sun, Y.; Su, Z.G.; Sun, L.; Zhao, G. Time-Delay Active Disturbance Rejection Control of Wet Electrostatic Precipitator in Power Plants. IEEE Trans. Autom. Sci. Eng. 2022, 20, 2748–2760. [Google Scholar] [CrossRef]
- Wang, R.; Li, X.; Zhang, J.; Zhang, J.; Li, W.; Liu, Y.; Fu, W.; Ma, X. Speed Control for a Marine Diesel Engine Based on the Combined Linear-Nonlinear Active Disturbance Rejection Control. Math. Probl. Eng. 2018, 2018, 7641862. [Google Scholar] [CrossRef]
- Madonski, R.; Herbst, G.; Stankovic, M. ADRC in output and error form: Connection, equivalence, performance. Control Theory Technol. 2023, 21, 56–71. [Google Scholar] [CrossRef]
- Stankovic, M.; Ting, H.; Madonski, R. From PID to ADRC and back: Expressing error-based active disturbance rejection control schemes as standard industrial 1DOF and 2DOF controllers. Asian J. Control 2024, 26, 2796–2806. [Google Scholar] [CrossRef]
- Amokrane, S.B.; Laidouni, M.Z.; Adli, T.; Madonski, R.; Stankovic, M. Active disturbance rejection control for unmanned tracked vehicles in leader–follower scenarios: Discrete-time implementation and field test validation. Mechatronics 2024, 97, 103114. [Google Scholar] [CrossRef]
- Wang, L. Disturbance Observer-based PID Control with Constraints: Design and Robustness Analysis. In Proceedings of the 4th IFAC Conference on Advances in Proportional-Integral-Derivative Control PID 2024, Almería, Spain, 12–14 June 2024. IFAC-PapersOnLine. [Google Scholar]
- Oldenbourg, R.; Sartorius, H. Dynamik selbsttätiger Regelungen, 2nd ed.; R.Oldenbourg-Verlag: München, Germany, 1951. [Google Scholar]
- Khalil, H. Nonlinear Systems, 2nd ed.; Prentice Hall: Upper Saddle River, NJ, USA, 1996. [Google Scholar]
- Vidyasagar, M. Nonlinear System Analysis, 2nd ed.; Prentice Hall: Englewood Cliffs, NJ, USA, 1993. [Google Scholar]
- Slotine, J.; Li, W. Applied Nonlinear Control; Prentice Hall: Englewood Cliffs, NJ, USA, 1991. [Google Scholar]
- Isidori, A. Nonlinear Control Systems, 3rd ed.; Springer: London, UK, 1995. [Google Scholar]
- Conte, G.; Moog, C.; Perdon, A. Algebraic Methods for Nonlinear Control Systems, 2nd ed.; Springer: London, UK, 2007. [Google Scholar]
- Halás, M. An algebraic framework generalizing the concept of transfer functions to nonlinear systems. Automatica 2008, 44, 1181–1190. [Google Scholar] [CrossRef]
- Halás, M.; Kotta, Ü. A transfer function approach to the realisation problem of nonlinear systems. Int. J. Control 2012, 85, 320–331. [Google Scholar] [CrossRef]
- Baumann, W.; Rugh, W. Feedback Control of Nonlinear Systems by Extended Linearization. IEEE Trans. AC 1986, 31, 40–46. [Google Scholar] [CrossRef]
- Wang, J.; Rugh, W. Feedback Linearization Families for Nonlinear Systems. IEEE Trans. AC 1987, 32, 935–940. [Google Scholar] [CrossRef]
- Wang, J.; Rugh, W. Parametrized Linear Systems and Linearization Families for Nonlinear Systems. IEEE Trans. CS 1987, 34, 650–657. [Google Scholar]
- Rugh, W.J. Analytical Framework for Gain Scheduling. IEEE Control Syst. Mag. 1991, 11, 79–84. [Google Scholar]
- Klatt, K.; Engell, S. Nichtlinearer Reglerentwurf mit Gain-Scheduling Techniken. In Entwurf Nichtlinearer Regelungen; Engell, S., Ed.; R.Oldenbourg Verlag: München, Germany, 1995; pp. 93–121. [Google Scholar]
- Lawrence, D.; Rugh, W. Gain Scheduling Dynamic Linear Controllers for a Nonlinear Plant. Automatica 1995, 31, 381–390. [Google Scholar] [CrossRef]
- Tepljakov, A.; Petlenkov, E.; Belikov, J. Gain and order scheduled fractional-order PID control of fluid level in a multi-tank system. In Proceedings of the ICFDA’14 International Conference on Fractional Differentiation and Its Applications 2014, Catania, Italy, 23–25 June 2014; pp. 1–6. [Google Scholar]
- Samad, T. A Survey on Industry Impact and Challenges Thereof [Technical Activities]. IEEE Control Syst. Mag. 2017, 37, 17–18. [Google Scholar]
- Grimholt, C.; Skogestad, S. Optimal PID control of double integrating processes. IFAC-PapersOnLine 2016, 49, 127–132. [Google Scholar] [CrossRef]
- Leith, D.; Leithead, W. Gain-scheduled controller design: An analytic framework directly incorporating non-equilibrium plant dynamics. Int. J. Control 1998, 70, 249–269. [Google Scholar] [CrossRef]
- Huba, M.; Vrančič, D. Comparing filtered PI, PID and PIDD2 control for the FOTD plants. IFAC-PapersOnLine 2018, 51, 954–959. [Google Scholar] [CrossRef]
- Han, J. From PID to Active Disturbance Rejection Control. Ind. Electron. IEEE Trans. 2009, 56, 900–906. [Google Scholar] [CrossRef]
- Umeno, T.; Hori, Y. Robust speed control of dc servomotors using modern two-degree-of-freedom controller design. IEEE Trans. Ind. Electr. 1991, 38, 363–368. [Google Scholar] [CrossRef]
- Gao, H.; Chen, Z.; Sun, M.; Huang, J.; Wang, Z.; Chen, Z. An efficient fast altitude control for hypersonic vehicle. Control Eng. Pract. 2020, 100, 104426. [Google Scholar] [CrossRef]
- Huba, M. Gain Scheduled PI Level Control of a Tank with Variable Cross Section. Ifac Proc. Vol. 2003, 36, 53–58. [Google Scholar] [CrossRef]
- Shinskey, F. How good are Our Controllers in Absolute Performance and Robustness. Meas. Control. 1990, 23, 114–121. [Google Scholar] [CrossRef]
- Huba, M. Performance measures, performance limits and optimal PI control for the IPDT plant. J. Process. Control 2013, 23, 500–515. [Google Scholar] [CrossRef]
- Huba, M.; Sovišová, D.; Spurná, N. Digital Time-Optimal Control of Nonlinear Second-Order System. Prepr. 10th Ifac World Congr. 1987, 8, 29–34. [Google Scholar] [CrossRef]
- Jin, H.; Song, J.; Lan, W.; Gao, Z. On the characteristics of ADRC: A PID interpretation. Sci. China Inf. Sci. 2020, 63, 1869–1919. [Google Scholar] [CrossRef]
- Lin, P.; Sun, X.M.; Fei, Z. A Generalized Interpretation of Three Types of Disturbance-Based Controllers for Perturbed Integral Systems in Frequency Domain. IEEE Trans. Circuits Syst. Ii Express Briefs 2021, 68, 1328–1332. [Google Scholar] [CrossRef]
- Wen, S.X.; Pan, Z.R.; Liu, K.Z.; Sun, X.M. Practical Anti-windup for Open-Loop Stable Systems Under Magnitude and Rate Constraints: Application to Turbofan Engines. IEEE Trans. Ind. Electron. 2023, 70, 4128–4137. [Google Scholar] [CrossRef]
- Cao, H.; Deng, Y.; Zuo, Y.; Li, H.; Wang, J.; Liu, X.; Lee, C.H.T. Unified Interpretation of Active Disturbance Rejection Control for Electrical Drives. IEEE Trans. Circuits Syst. Ii Express Briefs 2024, 71, 3433–3437. [Google Scholar] [CrossRef]
- Xue, W.; Madonski, R.; Lakomy, K.; Gao, Z.; Huang, Y. Add-on Module of Active Disturbance Rejection for Set-Point Tracking of Motion Control Systems. IEEE Trans. Ind. Appl. 2017, 53, 4028–4040. [Google Scholar] [CrossRef]
- Herbst, G.; Madonski, R. Interlude: A Look Around. In Active Disturbance Rejection Control: From Principles to Practice; Springer Nature: Cham, Switzerland, 2025; pp. 103–117. [Google Scholar]
- Isermann, R. Digital Control Systems. Volume 1, 2nd ed.; Springer: Berlin, Germany, 1989. [Google Scholar]
- Kumar, M.; Hote, Y.V. Robust PIDD2 Controller Design for Perturbed Load Frequency Control of an Interconnected Time-Delayed Power Systems. IEEE Trans. Control Syst. Tech. 2021, 29, 2662–2669. [Google Scholar] [CrossRef]
- Kumar, M.; Hote, Y.V. Real-Time Performance Analysis of PIDD2 Controller for Nonlinear Twin Rotor TITO Aerodynamical System. J. Intell. Robot. Syst. Theory Appl. 2021, 101, 1–16. [Google Scholar] [CrossRef]
- Kumar, M.; Hote, Y.V. PIDD2 Controller Design Based on Internal Model Control Approach for a Non-Ideal DC-DC Boost Converter. In Proceedings of the 2021 IEEE Texas Power and Energy Conference, TPEC 2021, College Station, TX, USA, 2–5 February2021. [Google Scholar]
- Visioli, A.; Sánchez-Moreno, J. Design of PIDA Controllers for High-order Integral Processes. In Proceedings of the 2023 IEEE 28th International Conference on Emerging Technologies and Factory Automation (ETFA), Sinaia, Romania, 12–15 September 2023; pp. 1–8. [Google Scholar]
- Campregher, F.; Milanesi, M.; Schiavo, M.; Visioli, A. Generalized Haalman tuning of PIDA controllers. In Proceedings of the 4th IFAC Conference on Advances in Proportional-Integral-Derivate Control PID 2024, Almería, Spain, 12–14 June 2024; IFAC-PapersOnLine. Volume 58, pp. 406–411. [Google Scholar]
- Schiavo, M.; Beschi, M.; Satué, M.G.; Arahal, M.R.; Visioli, A. PIDA Control of Heat Exchangers. In Proceedings of the 2024 IEEE 29th International Conference on Emerging Technologies and Factory Automation (ETFA), Padova, Italy, 10–13 September 2024; pp. 1–8. [Google Scholar]
- Boskovic, M.C.; Sekara, T.B.; Stojic, D.M.; Rapaic, M.R. Novel tuning rules for PIDC controllers in automatic voltage regulation systems under constraints on robustness and sensitivity to measurement noise. Int. J. Electr. Power Energy Syst. 2024, 157, 109791. [Google Scholar] [CrossRef]
- Huba, M.; Bistak, P.; Vrancic, D.; Sun, M. PID versus Model-Based Control for the Double Integrator Plus Dead-Time: Noise Attenuation and Robustness Aspects. Mathematics 2025, 13, 664. [Google Scholar] [CrossRef]


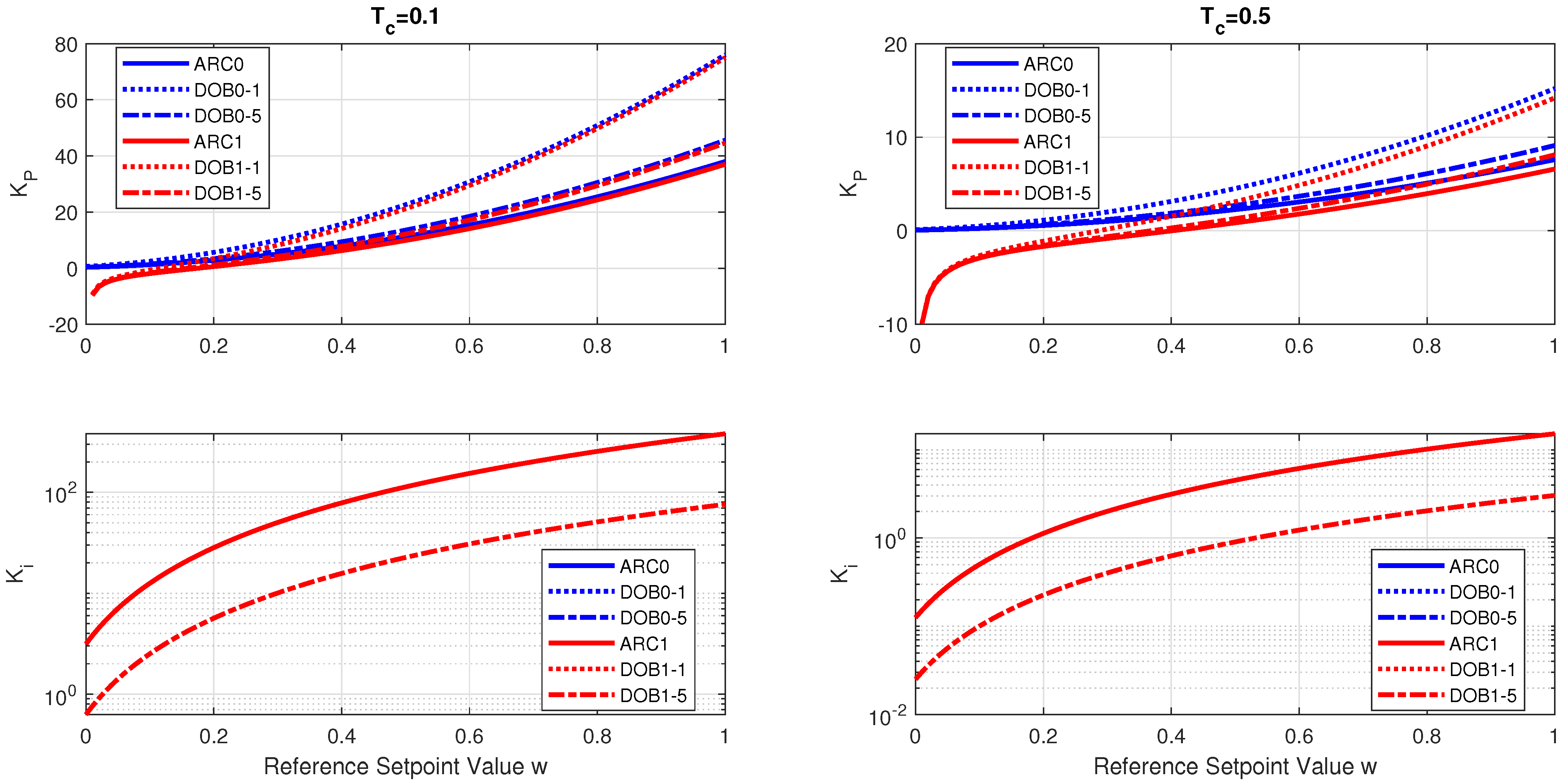

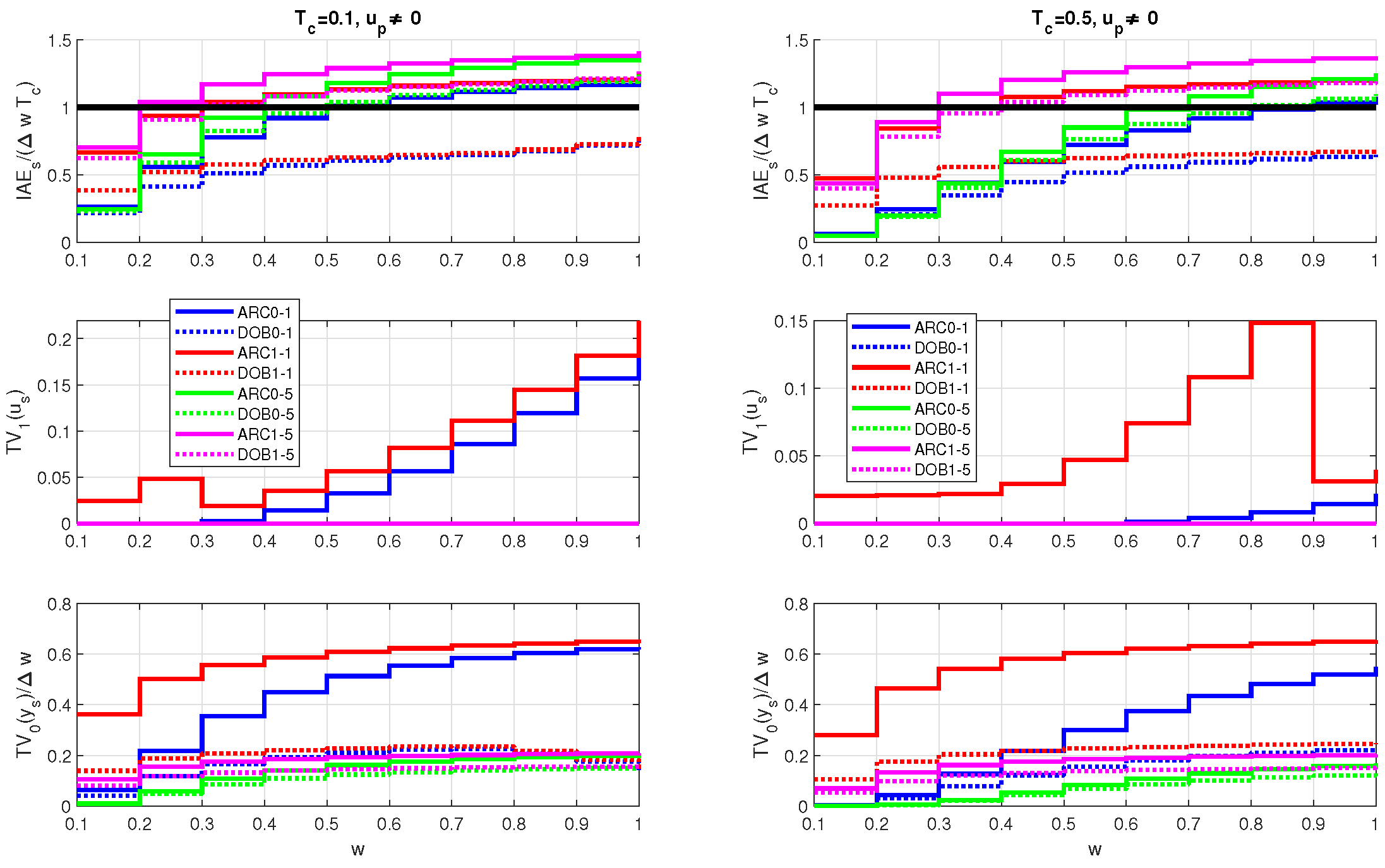

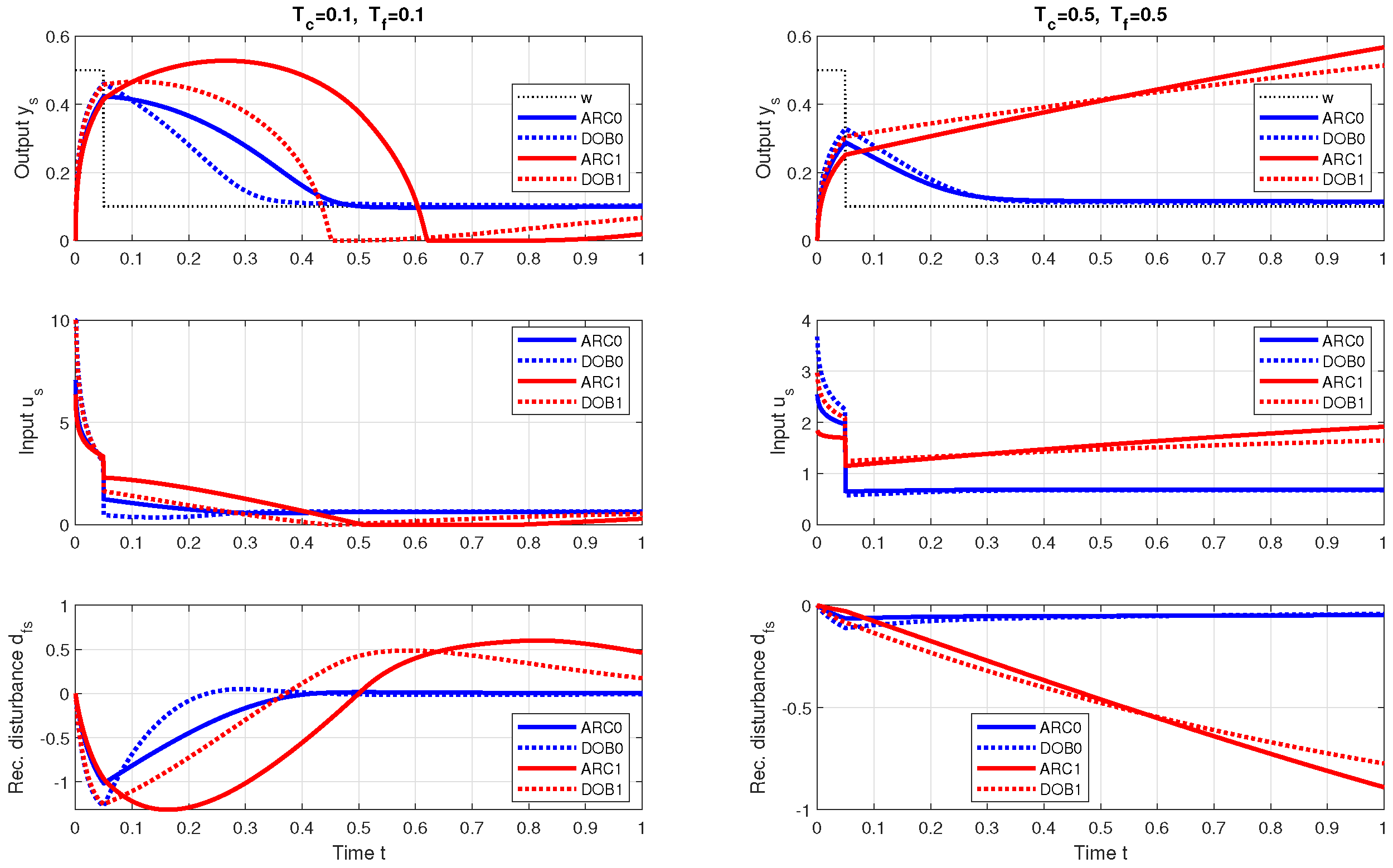
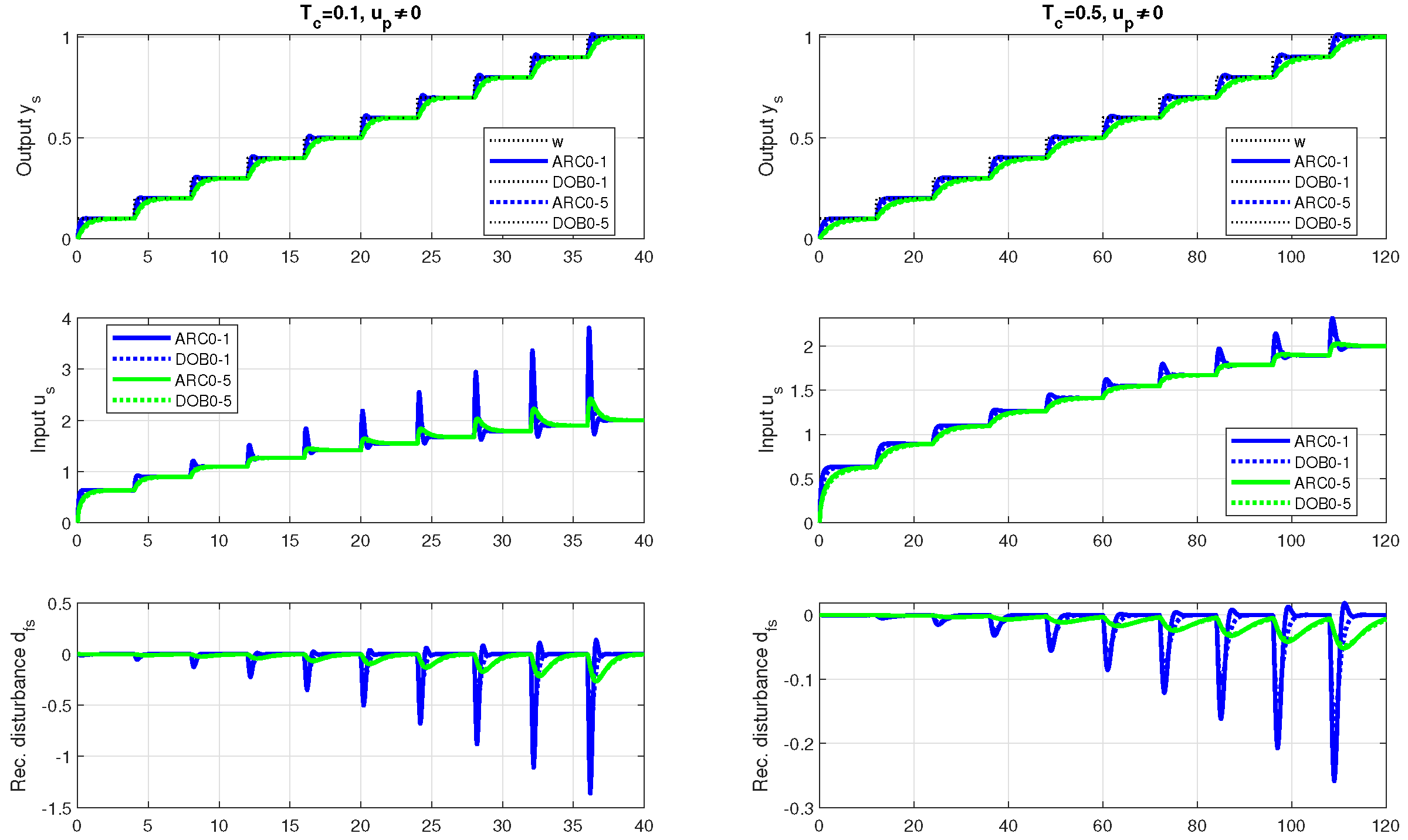


| ARC0 | Automatic Reset Controller based on ultralocal linear process model |
| ARC1 | Automatic Reset Controller based on local linear process model |
| DOB0 | Stabilizing P controller with Disturbance Observer based on ultralocal linear process model |
| DOB1 | Stabilizing P controller with Disturbance Observer based on local linear process model |
| − | ARC0 | DOB0 | ARC1 | DOB1 |
|---|---|---|---|---|
| ARC0-1, | ||||||||||
|---|---|---|---|---|---|---|---|---|---|---|
| w | 0.1 | 0.2 | 0.3 | 0.4 | 0.5 | 0.6 | 0.7 | 0.8 | B | 1 |
| 0.011935 | 0.013234 | 0.014865 | 0.015264 | 0.015883 | 0.016313 | 0.016681 | 0.016897 | 0.016894 | 0.017535 | |
| 0.17787 | 0.18706 | 0.19206 | 0.1947 | 0.19134 | 0.19496 | 0.1966 | 0.19815 | 0.19652 | 0.20149 | |
| 32.616486 | 76.042108 | 135.58941 | 208.66087 | 297.53243 | 404.31701 | 531.64265 | 668.94032 | 825.90369 | 1011.4014 | |
| DOB0-1, | ||||||||||
| w | 0.1 | 0.2 | 0.3 | 0.4 | 0.5 | 0.6 | 0.7 | 0.8 | 0.9 | 1 |
| 0.021189 | 0.021393 | 0.021954 | 0.021669 | 0.02128 | 0.021412 | 0.021776 | 0.021572 | 0.021252 | 0.021844 | |
| 0.35134 | 0.35656 | 0.35706 | 0.35482 | 0.349 | 0.35141 | 0.34833 | 0.34973 | 0.34757 | 0.35408 | |
| 66.142893 | 153.40691 | 273.39205 | 420.57951 | 599.70507 | 816.62546 | 1074.0832 | 1351.1266 | 1669.2539 | 2043.106 | |
| ARC0-5, | ||||||||||
| w | 0.1 | 0.2 | 0.3 | 0.4 | 0.5 | 0.6 | 0.7 | 0.8 | 0.9 | 1 |
| 0.050488 | 0.050709 | 0.050805 | 0.05057 | 0.050332 | 0.050285 | 0.050795 | 0.050626 | 0.050296 | 0.050531 | |
| 0.1594 | 0.1537 | 0.1507 | 0.149 | 0.1474 | 0.1459 | 0.1453 | 0.1469 | 0.1443 | 0.1480 | |
| 32.671235 | 76.303127 | 136.11613 | 209.4762 | 298.72178 | 407.01276 | 535.46824 | 673.1578 | 831.65296 | 1019.1571 | |
| DOB0-5, | ||||||||||
| w | 0.1 | 0.2 | 0.3 | 0.4 | 0.5 | 0.6 | 0.7 | 0.8 | 0.9 | 1 |
| 0.060213 | 0.060506 | 0.060351 | 0.060243 | 0.059976 | 0.059897 | 0.060629 | 0.060474 | 0.059885 | 0.060328 | |
| 0.207 | 0.1944 | 0.1879 | 0.183 | 0.1796 | 0.1772 | 0.1753 | 0.1768 | 0.1738 | 0.1778 | |
| 39.373144 | 91.735237 | 163.59209 | 251.72539 | 358.95352 | 489.12914 | 643.37008 | 808.78953 | 999.21409 | 1224.51 | |
| ARC0-1, | ||||||||||
|---|---|---|---|---|---|---|---|---|---|---|
| w | 0.1 | 0.2 | 0.3 | 0.4 | 0.5 | 0.6 | 0.7 | 0.8 | 0.9 | 1 |
| 0.051801 | 0.056311 | 0.059723 | 0.063522 | 0.067422 | 0.070043 | 0.07228 | 0.074821 | 0.076368 | 0.077478 | |
| 0.1009 | 0.1001 | 0.1015 | 0.1066 | 0.1087 | 0.1112 | 0.1136 | 0.1149 | 0.1170 | 0.1162 | |
| 19.7342 | 44.5925 | 79.5527 | 125.406 | 179.2605 | 248.6903 | 319.8459 | 407.0494 | 503.2121 | 606.7853 | |
| DOB0-1, | ||||||||||
| w | 0.1 | 0.2 | 0.3 | 0.4 | 0.5 | 0.6 | 0.7 | 0.8 | 0.9 | 1 |
| 0.10119 | 0.10221 | 0.10244 | 0.10206 | 0.10195 | 0.10208 | 0.10179 | 0.10218 | 0.10135 | 0.10153 | |
| 0.2014 | 0.1935 | 0.1909 | 0.1949 | 0.1942 | 0.194 | 0.1949 | 0.1937 | 0.1965 | 0.1921 | |
| 39.874588 | 89.572495 | 159.52872 | 251.36843 | 359.19898 | 498.2467 | 640.76059 | 815.39492 | 1008.0409 | 1215.4798 | |
| ARC0-5, | ||||||||||
| w | 0.1 | 0.2 | 0.3 | 0.4 | 0.5 | 0.6 | 0.7 | 0.8 | 0.9 | 1 |
| 0.2493 | 0.25272 | 0.25237 | 0.25132 | 0.25136 | 0.2507 | 0.25026 | 0.25041 | 0.2503 | 0.25063 | |
| 0.0842 | 0.0727 | 0.0685 | 0.0687 | 0.0682 | 0.0678 | 0.0685 | 0.0674 | 0.0684 | 0.0668 | |
| 19.6364 | 44.6244 | 79.6069 | 125.5128 | 179.3979 | 248.8807 | 320.0882 | 407.3516 | 503.6140 | 607.2546 | |
| DOB0-5, | ||||||||||
| w | 0.1 | 0.2 | 0.3 | 0.4 | 0.5 | 0.6 | 0.7 | 0.8 | 0.9 | 1 |
| 0.29585 | 0.3027 | 0.30267 | 0.30177 | 0.30176 | 0.30105 | 0.30052 | 0.30063 | 0.30048 | 0.30086 | |
| 0.1181 | 0.0982 | 0.0891 | 0.0876 | 0.0855 | 0.0841 | 0.0842 | 0.0824 | 0.0834 | 0.0809 | |
| 23.6752 | 53.6179 | 95.5950 | 150.6905 | 215.3628 | 298.7598 | 384.2280 | 488.9660 | 604.5095 | 728.9076 | |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Huba, M.; Bistak, P.; Vrancic, D. Gain-Scheduled Disturbance Observer-Based Saturated Controllers for Non-Linear First-Order System. Appl. Sci. 2025, 15, 2812. https://doi.org/10.3390/app15052812
Huba M, Bistak P, Vrancic D. Gain-Scheduled Disturbance Observer-Based Saturated Controllers for Non-Linear First-Order System. Applied Sciences. 2025; 15(5):2812. https://doi.org/10.3390/app15052812
Chicago/Turabian StyleHuba, Mikulas, Pavol Bistak, and Damir Vrancic. 2025. "Gain-Scheduled Disturbance Observer-Based Saturated Controllers for Non-Linear First-Order System" Applied Sciences 15, no. 5: 2812. https://doi.org/10.3390/app15052812
APA StyleHuba, M., Bistak, P., & Vrancic, D. (2025). Gain-Scheduled Disturbance Observer-Based Saturated Controllers for Non-Linear First-Order System. Applied Sciences, 15(5), 2812. https://doi.org/10.3390/app15052812







