Effects of Installation Angle on Energy Harvesting Performance of Airfoil-Based Piezoelectric Energy Harvester
Abstract
1. Introduction
2. Airfoil-Based Flutter Energy Harvester System Design and Control Equations
2.1. Conceptual Designing
2.2. Governing Equations
3. Multi-Physical Coupled Fields Simulation Analyses
3.1. Equations for Rigid Body Motion and Solution Methodology
3.2. Aerodynamic Performance
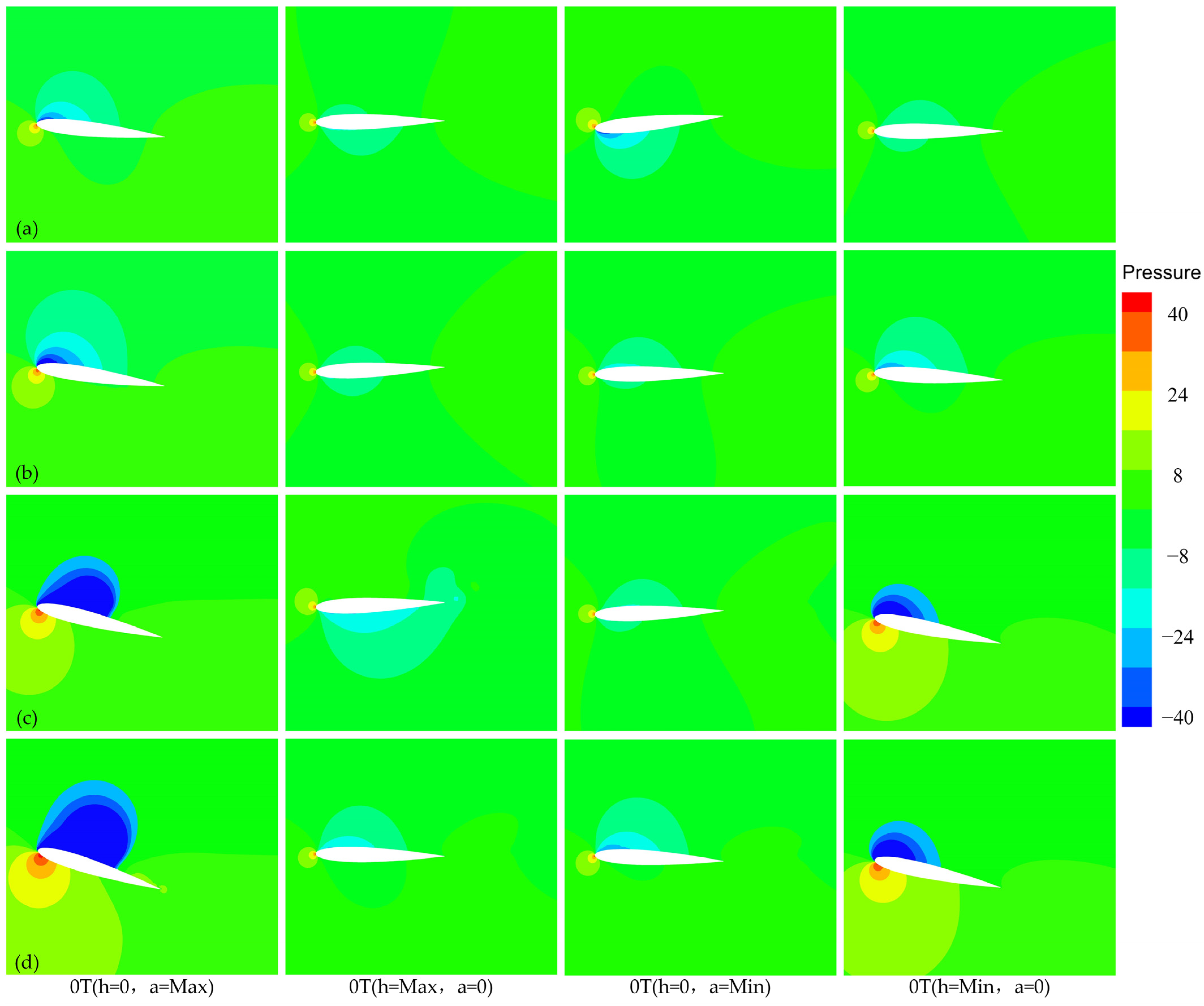
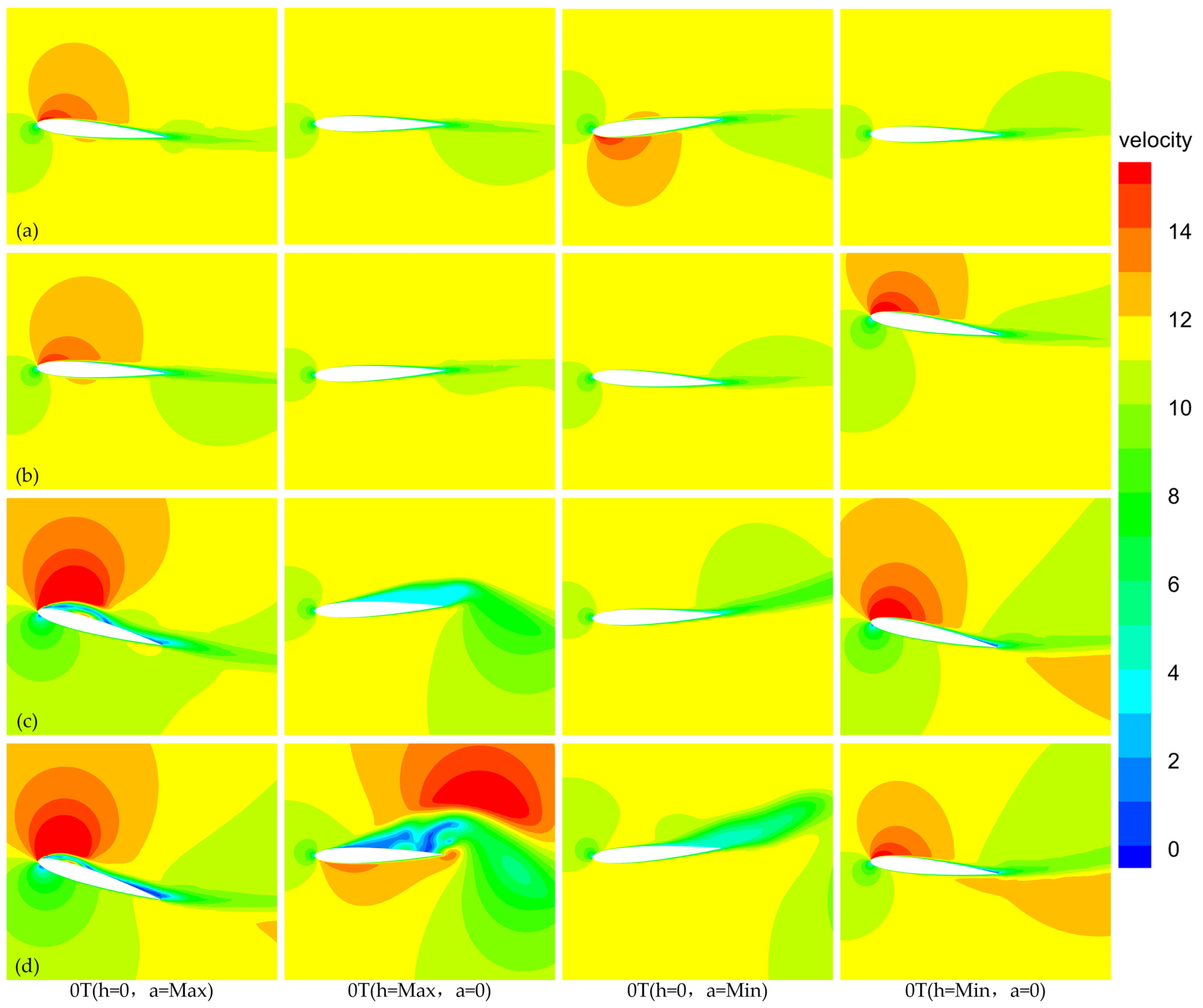
3.3. Visualized Analysis
4. Experimental Investigation of Aeroelastic Vibration and Harvesting Performance
4.1. Experimental Setup
4.2. Experimental Validation
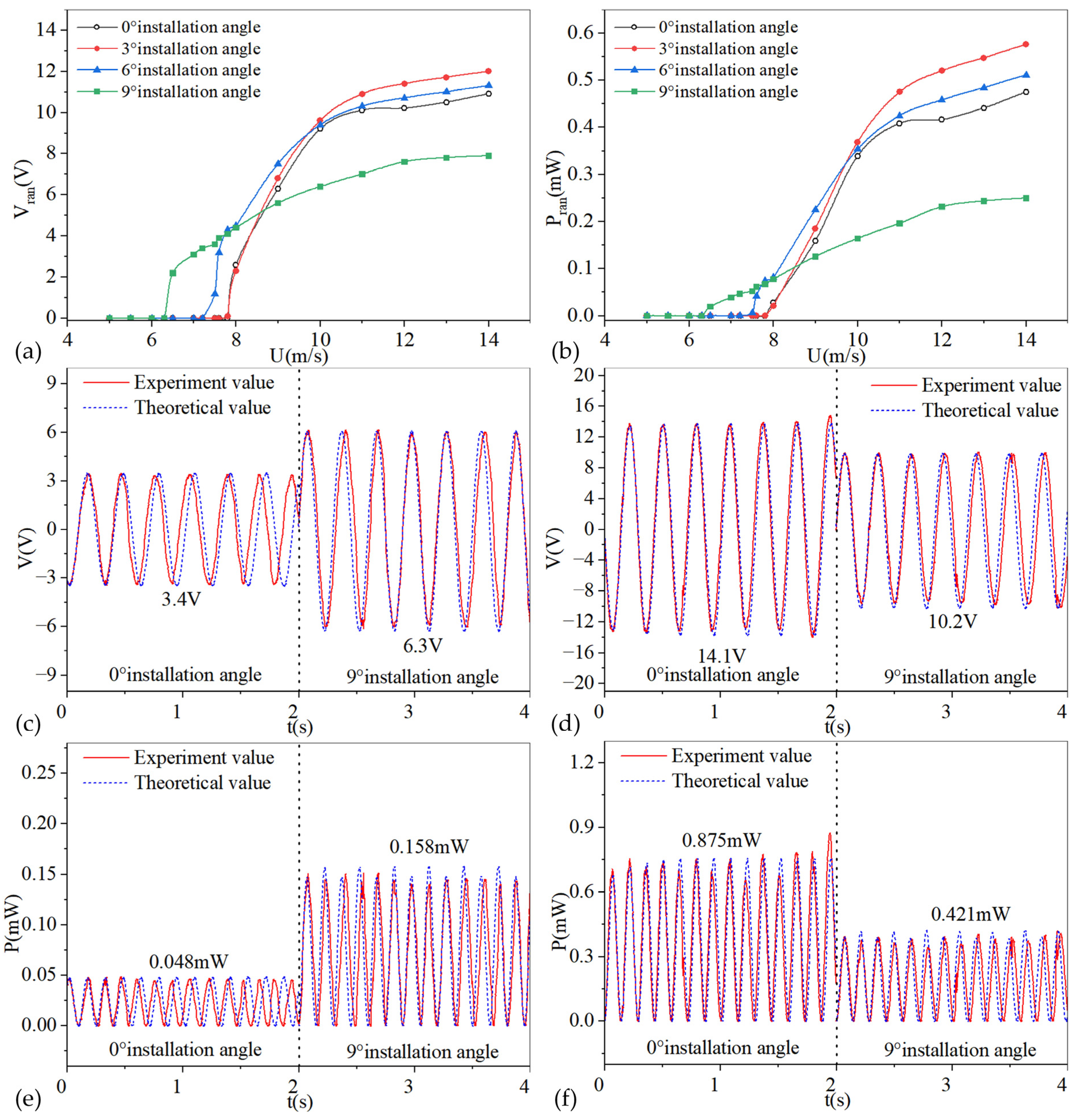
4.3. Aeroelastic Vibration and Harvesting Performance
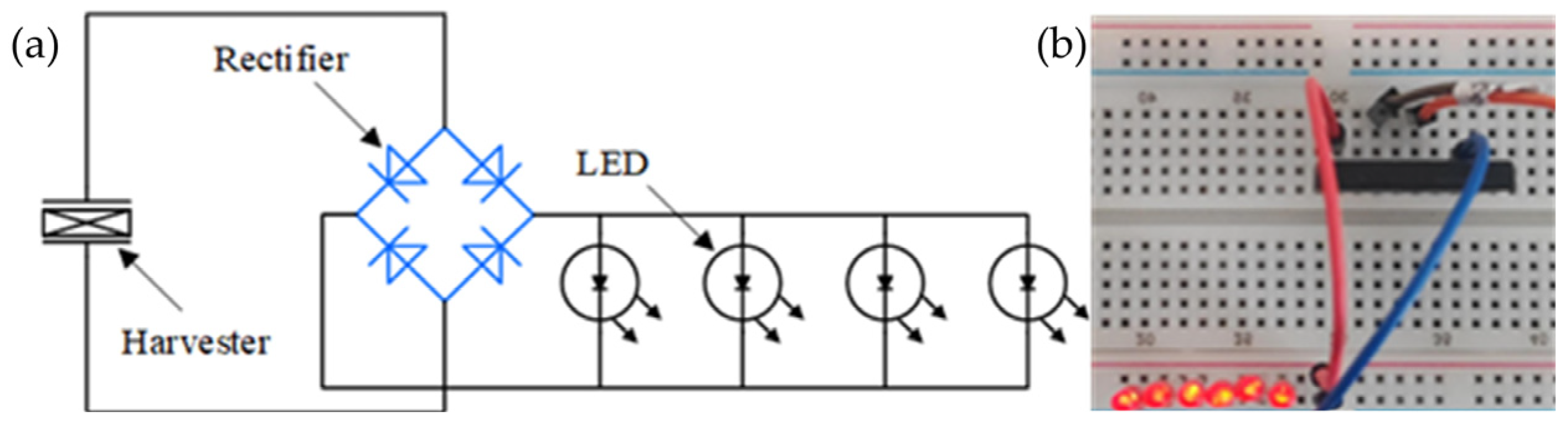
4.4. Field Harvesting Performance
5. Conclusions
- (1)
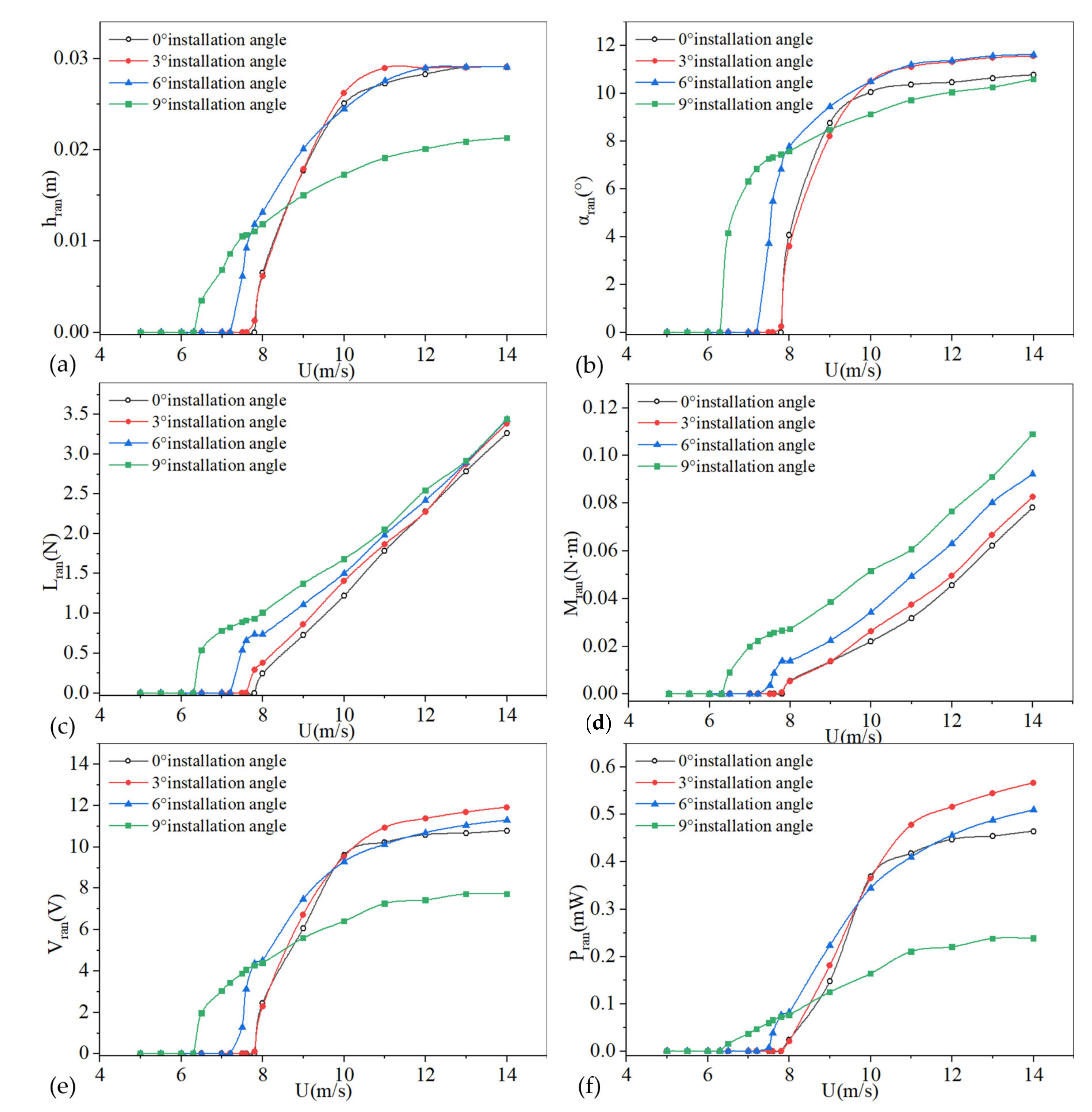
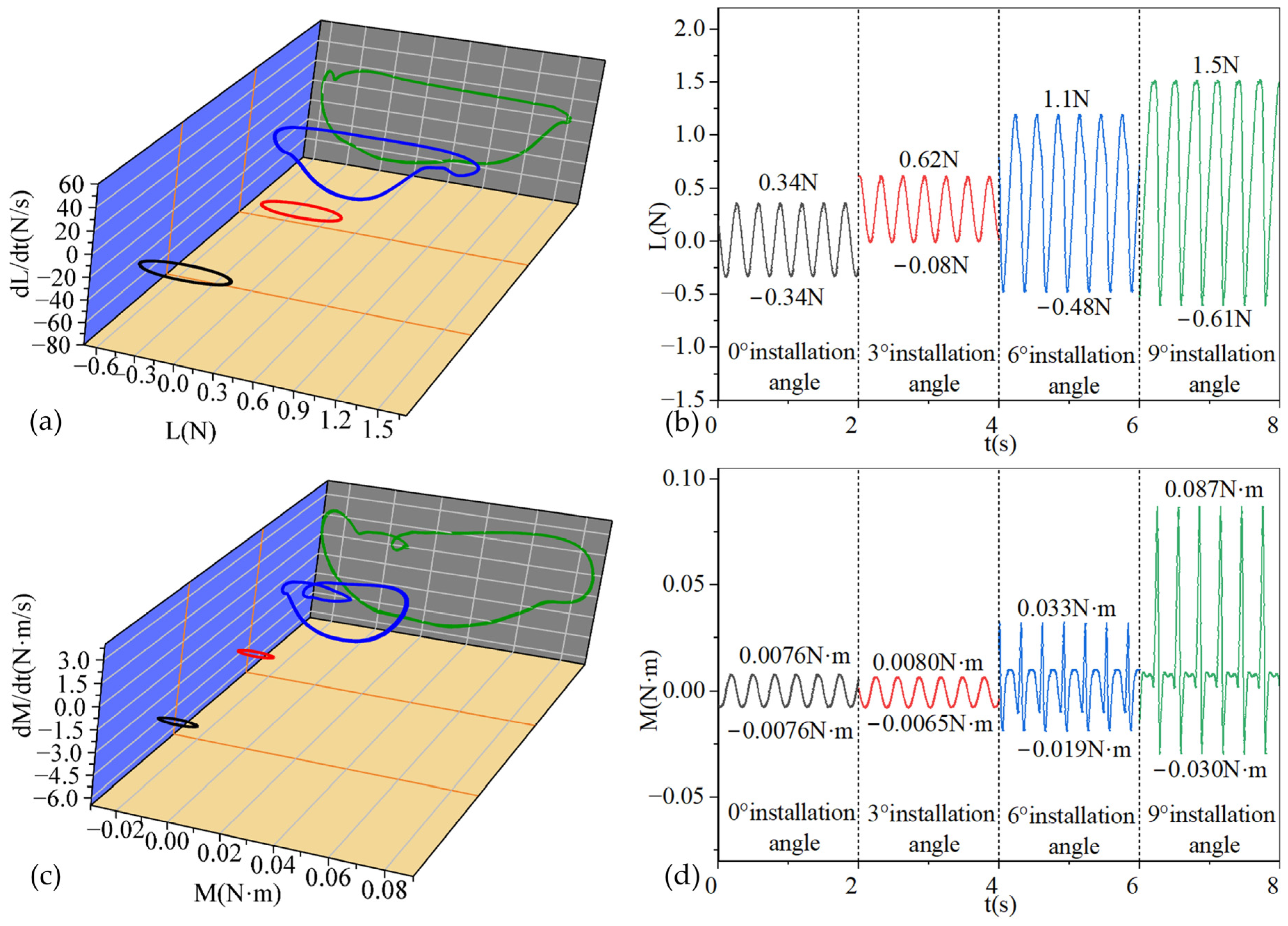
- The installation angle is a crucial parameter influencing the critical flutter velocity of the airfoil-based piezoelectric energy harvester system. A clear inverse relationship exists between the installation angle and critical flutter velocity, with the latter decreasing from 7.8 m/s at 0° to 6.3 m/s at 9°. This systematic reduction in critical velocity can be attributed to enhanced initial lift generation at higher installation angles, which facilitates the earlier onset of flutter oscillations.
- (2)
- Moderate installation angles (3° and 6°) yield optimal energy harvesting performance. The system achieves maximum efficiency at a 3° installation angle, producing a peak output voltage of 12.0 V and power output of 0.58 mW, marking a substantial improvement over the baseline configuration at 0° (10.9 V, 0.48 mW). This enhanced performance stems from the development of favorable asymmetric flow field characteristics at moderate angles, enabling more efficient energy extraction from the airflow.
- (3)
- At higher installation angles, particularly at 9°, significant performance degradation occurs. Although this configuration achieves the lowest critical flutter velocity, it exhibits markedly reduced performance metrics (7.9 V voltage, 0.25 mW power). This deterioration is primarily due to the premature aerodynamic stall and persistent flow separation, highlighting a fundamental trade-off between reduced critical flutter velocity and maintained aerodynamic stability.
- (4)
- Installation angle adjustment represents an effective and practical approach to optimizing airfoil-based flutter energy harvesters, particularly in low-speed wind conditions. However, successful implementation necessitates careful consideration of the balance between reducing critical velocity and avoiding stall conditions. The results suggest that optimal performance can be achieved with moderate installation angles around 3° to 6°, providing valuable guidance for practical applications.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Tang, R.; Wang, Q.; Gu, Y.; Wang, J.; You, Z.; Zhao, Y. Numerical study of wake induced vibration(WIV) and energy harvesting characteristics under different stable conditions. Energy 2024, 289, 129908. [Google Scholar] [CrossRef]
- Wang, J.; Sun, S.; Tang, L.; Hu, G.; Liang, J. On the use of metasurface for Vortex-Induced vibration suppression or energy harvesting. Energy Convers. Manag. 2021, 235, 113991. [Google Scholar] [CrossRef]
- Gu, Y.; Yurchenko, D.; Wang, J.; Li, S.; Wei, S.; Tang, R. Numerical analysis of reverse wake-induced vibration at low Reynolds number. Phys. Fluids 2023, 35. [Google Scholar] [CrossRef]
- Tian, H.; Shan, X.; Cao, H.; Xie, T. Enhanced performance of airfoil-based piezoaeroelastic energy harvester: Numerical simu-lation and experimental verification. Mech. Syst. Signal Process. 2022, 162, 108065. [Google Scholar] [CrossRef]
- Goyaniuk, L.; Poirel, D.; Benaissa, A.; Amari, H. The energy extraction potential from pitch-heave coupled flutter. J. Sound Vib. 2023, 555, 117714. [Google Scholar] [CrossRef]
- Chai, Y.; Gao, W.; Ankay, B.; Li, F.; Zhang, C. Aeroelastic analysis and flutter control of wings and panels: A review. Int. J. Mech. Syst. Dyn. 2021, 1, 5–34. [Google Scholar] [CrossRef]
- Yuan, W.; Zhang, X. Numerical Stabilization for Flutter Analysis Procedure. Aerospace 2023, 10, 302. [Google Scholar] [CrossRef]
- Ma, X.; Zhou, S. A review of flow-induced vibration energy harvesters. Energy Convers. Manag. 2023, 254, 115223. [Google Scholar] [CrossRef]
- Ma, X.; Li, Z.; Zhang, H.; Zhou, S. Dynamic modeling and analysis of a tristable vortex-induced vibration energy harvester. Mech. Syst. Signal Process. 2023, 187, 109924. [Google Scholar] [CrossRef]
- Li, J.; Wang, G.; Yang, P.; Wen, Y.; Zhang, L.; Song, R.; Hou, C. An orientation-adaptive electromagnetic energy harvester scavenging for wind-induced vibration. Energy 2024, 286, 129578. [Google Scholar] [CrossRef]
- Ali, A.; Ali, S.; Shaukat, H.; Khalid, E.; Behram, L.; Rani, H.; Altabey, W.; Kouritem, S.; Noori, M. Advancements in piezoelectric wind energy harvesting: A review. Results Eng. 2024, 21, 101777. [Google Scholar] [CrossRef]
- Naqvi, A.; Ali, A.; Altabey, W.A.; Kouritem, S.A. Energy harvesting from fluid flow using piezoelectric materials: A review. Energies 2022, 15, 7424. [Google Scholar] [CrossRef]
- Miao, G.; Fang, S.; Wang, S.; Zhou, S. A low-frequency rotational electromagnetic energy harvester using a magnetic plucking mechanism. Appl. Energy 2022, 305, 117838. [Google Scholar] [CrossRef]
- Fan, B.; Fang, J.W.; Zhao, J.C.; Li, C.; Wang, J.; Lv, M.M. Bionic flutter wing piezoelectric-electromagnetic composite energy harvesting system. Energy Convers. Manag. 2022, 271, 116319. [Google Scholar] [CrossRef]
- Chen, Y.; Cheng, Y.; Jie, Y.; Cao, X.; Wang, N.; Wang, Z.L. Energy harvesting and wireless power transmission by a hybridized electromagnetic–triboelectric nanogenerator. Energy Environ. Sci. 2019, 12, 2678–2684. [Google Scholar] [CrossRef]
- Tcho, I.-W.; Kim, W.-G.; Kim, J.-K.; Kim, D.-W.; Yun, S.-Y.; Son, J.-H.; Choi, Y.-K. A flutter-driven triboelectric nanogenerator for harvesting energy of gentle breezes with a rear-fixed fluttering film. Nano Energy 2022, 98, 107197. [Google Scholar] [CrossRef]
- Wang, J.; Geng, L.; Ding, L.; Zhu, H.; Yurchenko, D. The state-of-the-art review on energy harvesting from flow-induced vi-brations. Appl. Energy 2020, 267, 114902. [Google Scholar] [CrossRef]
- Lensvelt, R.; Fey, R.H.; Mestrom, R.M.C.; Nijmeijer, H. Design and numerical analysis of an electrostatic energy harvester with impact for frequency up-conversion. J. Comput. Nonlinear Dyn. 2020, 15, 051005. [Google Scholar] [CrossRef]
- Sezer, N.; Koç, M. A comprehensive review on the state-of-the-art of piezoelectric energy harvesting. Nano Energy 2021, 80, 105567. [Google Scholar] [CrossRef]
- Sitharthan, R.; Yuvaraj, S.; Padmanabhan, S.; Holm-Nielsen, J.B.; Sujith, M.; Rajesh, M.; Prabaharan, N. Piezoelectric energy harvester converting wind aerodynamic energy into electrical energy for microelectronic application. IET Renew. Power Gener. 2021, 15, 1968–1975. [Google Scholar] [CrossRef]
- Li, Z.; Zhou, S.; Yang, Z. Recent progress on flutter-based wind energy harvesting. Int. J. Mech. Syst. Dyn. 2022, 2, 82–98. [Google Scholar] [CrossRef]
- Li, S.; Chen, Z.; He, X.; Ye, Y.; Wan, S.; Dong, L. High performance hybrid omnidirectional wind energy harvester based on flutter for wireless sensing and hydrogen production applications. Nano Energy 2024, 132, 110403. [Google Scholar] [CrossRef]
- Rong, Z.; Cao, B.; Hu, J. Stability analysis on an aeroelastic system for design of a flutter energy harvester. Aerosp. Sci. Technol. 2017, 60, 203–209. [Google Scholar] [CrossRef]
- Elahi, H.; Eugeni, M.; Gaudenzi, P. Design and performance evaluation of a piezoelectric aeroelastic energy harvester based on the limit cycle oscillation phenomenon. Acta Astronaut. 2019, 157, 233–240. [Google Scholar] [CrossRef]
- Tian, H.; Shan, X.; Cao, H.; Song, R.; Xie, T. A method for investigating aerodynamic load models of piezoaeroelastic energy harvester. J. Sound Vib. 2021, 502, 116084. [Google Scholar] [CrossRef]
- Shan, X.; Tian, H.; Cao, H.; Xie, T. Enhancing performance of a piezoelectric energy harvester system for concurrent flutter and vortex-induced vibration. Energies 2020, 13, 3101. [Google Scholar] [CrossRef]
- Li, X.; Wang, X.; Tian, H.; Wang, C.; Liu, B. Experimental research of symmetrical airfoil-based piezoelectric energy harvester excited by vortex-induced flutter coupling. Appl. Sci. 2022, 12, 12514. [Google Scholar] [CrossRef]
- Wan, C.; Tian, H.; Shan, X.; Xie, T. Enhanced performance of airfoil-based piezoelectric energy harvester under coupled flutter and vortex-induced vibration. Int. J. Mech. Sci. 2023, 241, 107979. [Google Scholar] [CrossRef]
- Tian, H.; Shan, X.; Wang, H.; Li, X.; Su, Y.; Wang, J. Enhanced piezoelectric energy harvesting performance using trailing-edge flap. Ocean Eng. 2023, 285, 115443. [Google Scholar] [CrossRef]
- Iqbal, M.; Khan, F.U. Hybrid vibration and wind energy harvesting using combined piezoelectric and electromagnetic con-version for bridge health monitoring applications. Energy Convers. Manag. 2018, 172, 611–618. [Google Scholar] [CrossRef]
- Li, Z.; Zhou, S.; Li, X. A piezoelectric–electromagnetic hybrid flutter-based wind energy harvester: Modeling and nonlinear analysis. Int. J. Non-Linear Mech. 2022, 144, 104051. [Google Scholar] [CrossRef]
- Tian, H.; Shan, X.; Li, X.; Wang, J. Enhanced airfoil-based flutter piezoelectric energy harvester via coupling magnetic force. Appl. Energy 2023, 340, 120979. [Google Scholar] [CrossRef]
- Chen, Z.; Shi, Z.; Chen, S.; Yao, Z. Stall flutter suppression of NACA 0012 airfoil-based based on steady blowing. J. Fluids Struct. 2022, 109, 103472. [Google Scholar] [CrossRef]
- Menter, F.R. 2-EQUATION eddy-viscosity turbulence models for engineering applications. AIAA J. 1994, 32, 1598–1605. [Google Scholar] [CrossRef]
- Menter, F.R. A comparison of some recent eddy-viscosity turbulence models. J. Fluids Eng. 1996, 118, 514–519. [Google Scholar] [CrossRef]
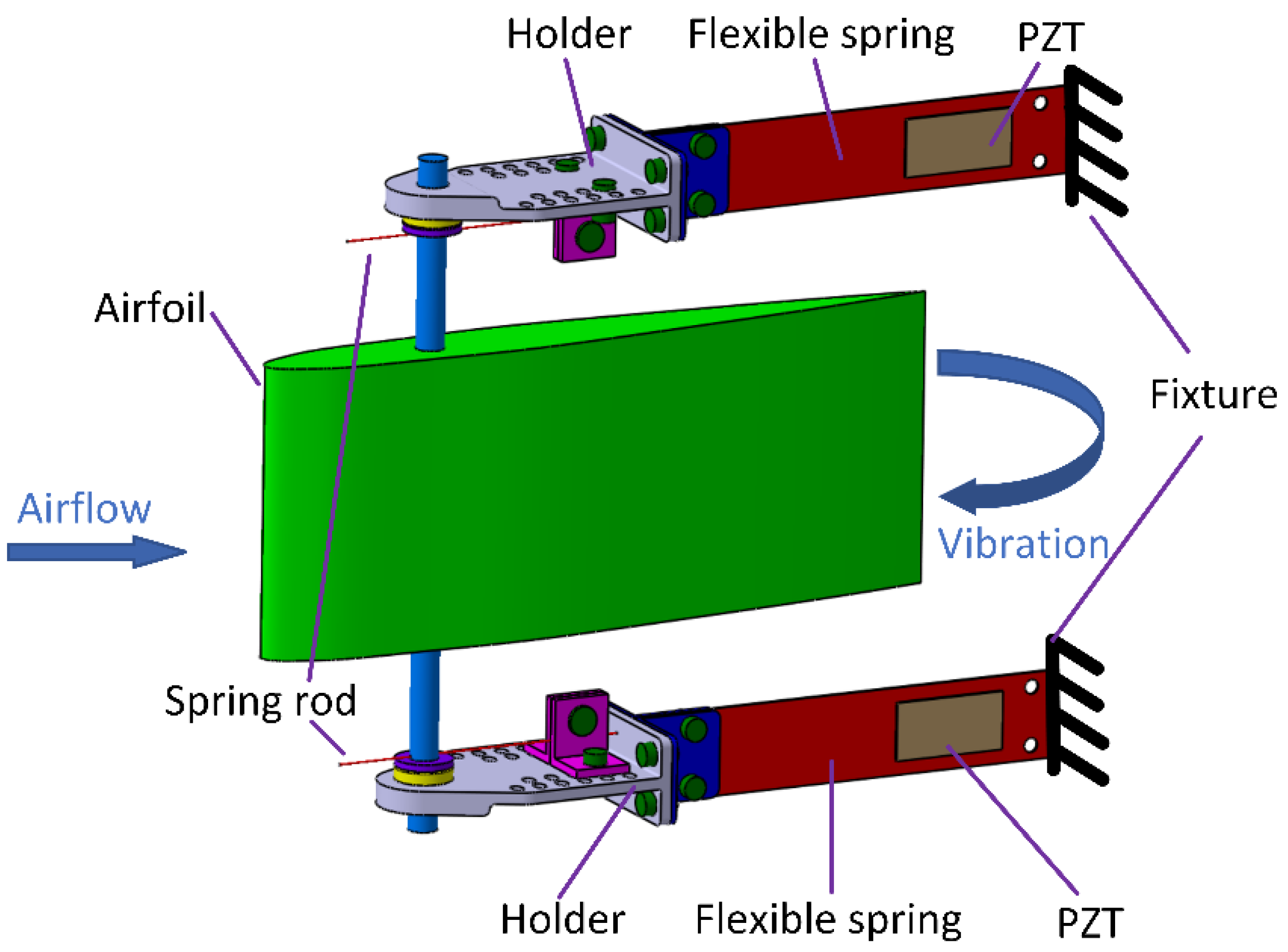







| Parameters | Flexural Spring | Piezoelectric Patch | Holder | Spring Rod |
|---|---|---|---|---|
| Materials | Spring steel | PZT-5H | Aluminum alloy | Glass fiber |
| Density (kg/m3) | 7850 | 7500 | 2810 | 2500 |
| Young’s modulus (GPa) | 210 | 66 | 72 | 73 |
| Poisson’s ratio | 0.29 | 0.3 | 0.33 | 0.22 |
| Length (mm) | 180 | 40 | 90 | 50 |
| Width/Radius (mm) | 30 | 20 | 60 | 0.3 |
| Thickness (mm) | 0.8 | 0.2 | 4 | - |
| Properties | Value |
|---|---|
| Span, (m) (kg) | 0.1 0.6 |
| (kg) | 0.18 |
| Semi-chord, (m) | 0.125 |
| Position of the airfoil-based pitch relative to the semi-chord, | 0.5 |
| 0.335 | |
| (N/m) | 240 |
| (0.28 Nm) | 0.28 |
| (kg/s) | 0.056 |
| (kg/s) | 0.056 |
| Resistance, (KΩ) | 250 |
| (F) | 0.0000007 |
| electromechanical coefficient of the harvester, (N/V) | 2.7 × 10−4 |
| Mesh | Elements | A,rms (m) | Change Rate | Cl,rms | Change Rate |
|---|---|---|---|---|---|
| M1 | 57,344 | 0.0164 | --- | 0.5445 | --- |
| M2 | 81,097 | 0.0167 | 1.80% | 0.5594 | 2.66% |
| M3 | 114,689 | 0.0168 | 0.63% | 0.5628 | 0.61% |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Tang, R.; Li, D.; Wei, Y.; Li, E.; You, Z. Effects of Installation Angle on Energy Harvesting Performance of Airfoil-Based Piezoelectric Energy Harvester. Appl. Sci. 2025, 15, 1366. https://doi.org/10.3390/app15031366
Tang R, Li D, Wei Y, Li E, You Z. Effects of Installation Angle on Energy Harvesting Performance of Airfoil-Based Piezoelectric Energy Harvester. Applied Sciences. 2025; 15(3):1366. https://doi.org/10.3390/app15031366
Chicago/Turabian StyleTang, Rongjiang, Dianliang Li, Yanhong Wei, Enze Li, and Zeyu You. 2025. "Effects of Installation Angle on Energy Harvesting Performance of Airfoil-Based Piezoelectric Energy Harvester" Applied Sciences 15, no. 3: 1366. https://doi.org/10.3390/app15031366
APA StyleTang, R., Li, D., Wei, Y., Li, E., & You, Z. (2025). Effects of Installation Angle on Energy Harvesting Performance of Airfoil-Based Piezoelectric Energy Harvester. Applied Sciences, 15(3), 1366. https://doi.org/10.3390/app15031366






