Efficient Method for Identifying Key Errors Based on 21-Geometric-Error Measurement of Three Linear Axes of Machine Tools
Abstract
1. Introduction
2. Sensitivity Analysis for 21 Geometric Errors of Three Linear Axes of Machine Tools
3. Experimental Results and Analysis
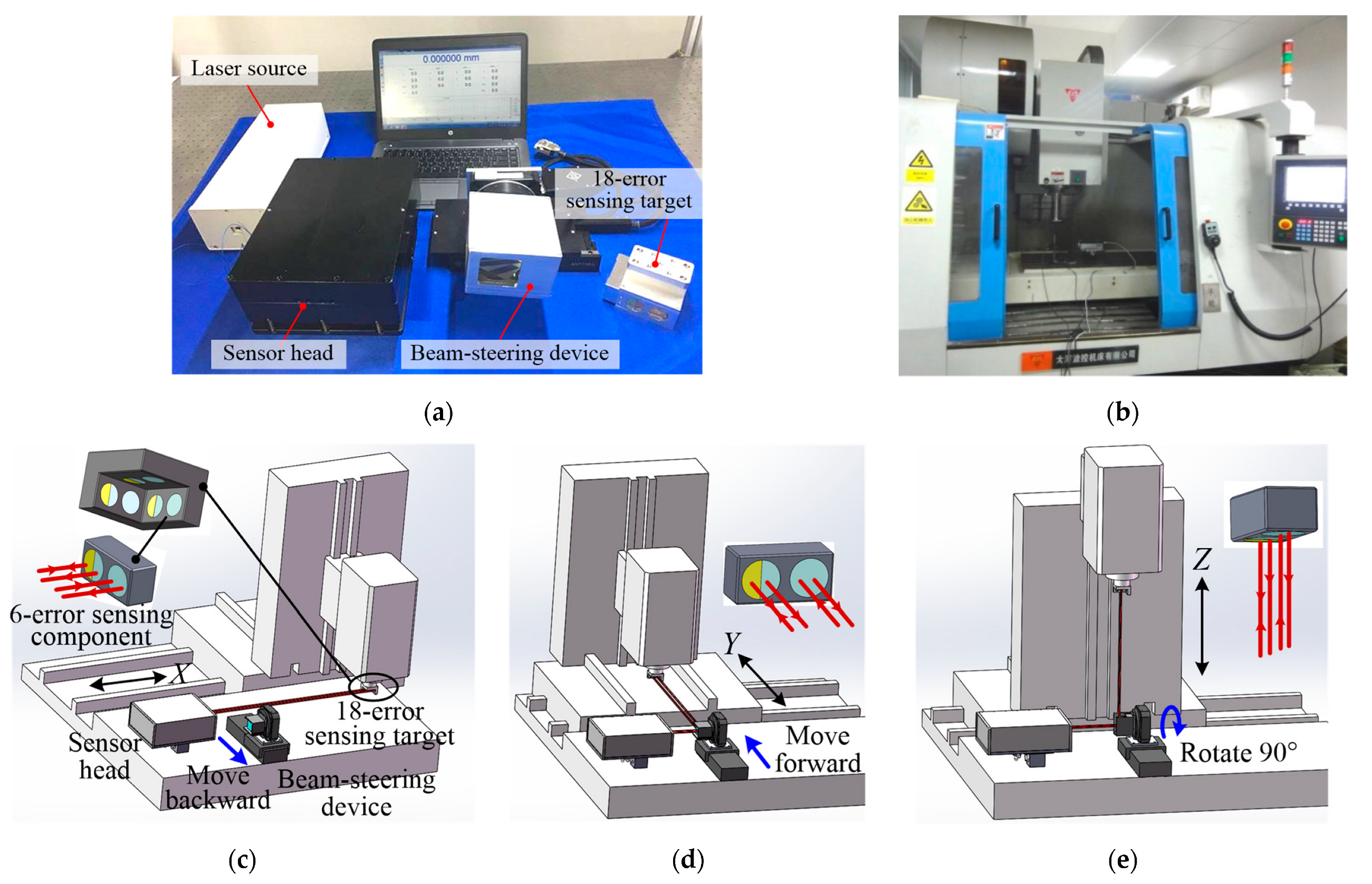
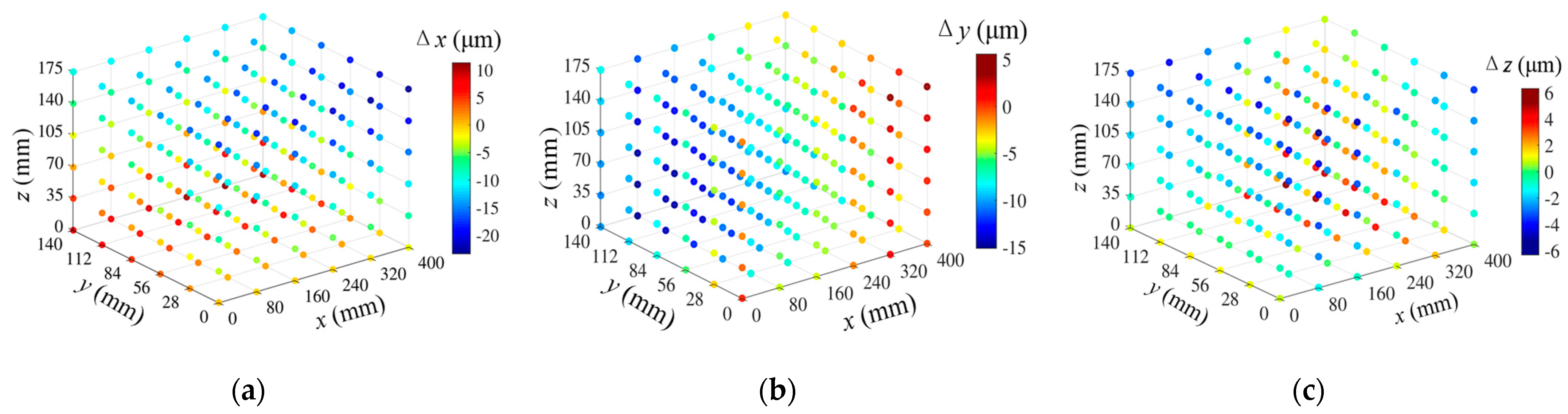
3.1. Measurement Experiments of 21 Geometric Errors
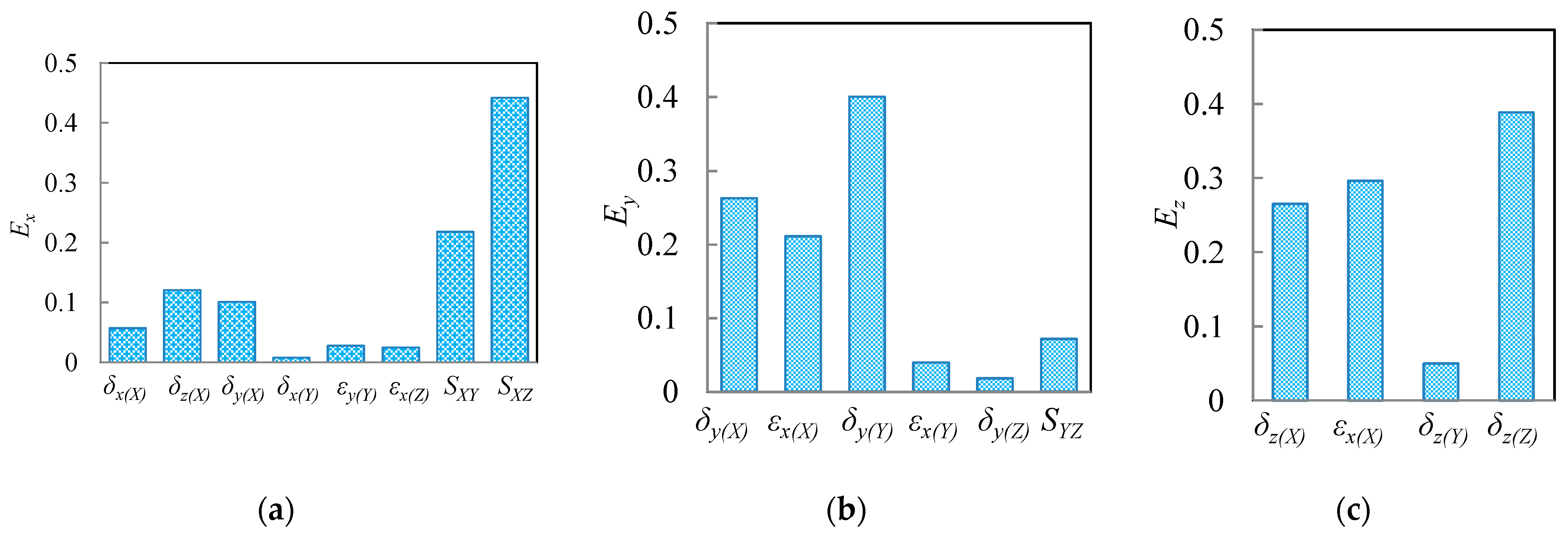
3.2. Sensitivity Analysis of 21 Geometric Errors
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Hong, C.; Ibaraki, S.; Matsubara, A. Influence of position-dependent geometric errors of rotary axes on a machining test of cone frustum by five-axis machine tools. Precis. Eng. 2011, 35, 1–11. [Google Scholar] [CrossRef]
- Khan, A.W.; Chen, W. A methodology for systematic geometric error compensation in five-axis machine tools. Int. J. Adv. Manuf. Technol. 2011, 53, 615–628. [Google Scholar] [CrossRef]
- Zhu, S.; Ding, G.; Qin, S.; Jiang, L.; Zhuang, L.; Yan, K. Integrated geometric error modeling, identification and compensation of CNC machine tools. Int. J. Adv. Manuf. Technol. 2012, 52, 24–29. [Google Scholar] [CrossRef]
- Gao, W.; Ibaraki, S.; Donmez, M.A.; Kono, D.; Mayer, J.R.R.; Chen, Y.L.; Szipka, K.; Archenti, A.; Linares, J.M.; Suzuki, N. Machine tool calibration: Measurement, modeling, and compensation of machine tool errors. Int. J. Mach. Tools Manuf. 2023, 187, 104017. [Google Scholar] [CrossRef]
- Ramesh, R.; Mannan, M.A.; Poo, A.N. Error compensation in machine tools—A review Part I: Geometric, cutting-force induced and fixture-dependent errors. Int. J. Mach. Tools Manuf. 2000, 9, 1235–1256. [Google Scholar] [CrossRef]
- Li, H.; Zhang, P.; Deng, M.; Xiang, S.; Du, Z.; Yang, J. Volumetric error measurement and compensation of three-axis machine tools based on laser bidirectional sequential step diagonal measuring method. Meas. Sci. Technol. 2020, 31, 055201. [Google Scholar] [CrossRef]
- Lee, K.I.; Yang, S.H. Accuracy evaluation of machine tools by modeling spherical deviation based on double ball-bar measurements. Int. J. Mach. Tools Manuf. 2013, 75, 46–54. [Google Scholar] [CrossRef]
- Slamani, M.; Mayer, R.; Balazinski, M. Concept for the integration of geometric and servo dynamic errors for predicting volumetric errors in five-axis high-speed machine tools: An application on a XYC three-axis motion trajectory using programmed end point constraint measurements. Int. J. Adv. Manuf. Technol. 2012, 65, 1669–1679. [Google Scholar] [CrossRef]
- Bringmann, B.; Küng, A.; Knapp, W. A measuring artefact for true 3D machine testing and calibration. CIRP Ann. 2005, 54, 471–474. [Google Scholar] [CrossRef]
- Chen, H.; Jiang, B.; Shi, Z.; Sun, Y.; Song, H.; Tang, L. Uncertainty modeling of the spatial coordinate error correction system of the CMM based on laser tracer multi-station measurement. Meas. Sci. Technol. 2019, 2, 025007. [Google Scholar] [CrossRef]
- Lee, C.; Kim, G.H.; Lee, S.K. Design and construction of a single unit multi-function optical encoder for a six-degree-of-freedom motion error measurement in an ultraprecision linear stage. Meas. Sci. Technol. 2011, 22, 105901. [Google Scholar] [CrossRef]
- Cai, Y.; Wang, L.; Liu, Y.; Li, C.; Fan, K.C. Accuracy improvement of linear stages using on-machine geometric error measurement system and error transformation model. Opt. Express 2022, 30, 7539–7550. [Google Scholar] [CrossRef] [PubMed]
- Liu, W.; Yu, Z.; Duan, F.; Hu, H.; Fu, X.; Bao, R. Robust five-degree-of-freedom measurement system with self-compensation and air turbulence protection. Opt. Express 2023, 31, 4652–4666. [Google Scholar] [CrossRef] [PubMed]
- Yu, K.; Zhu, J.; Yuan, W.; Zhou, Q.; Xue, G.; Wu, G.; Wang, X.; Li, X. Two-channel six degrees of freedom grating-encoder for precision-positioning of sub-components in synthetic-aperture optics. Opt. Express 2021, 29, 21113–21128. [Google Scholar] [CrossRef] [PubMed]
- Zhou, S.; Le, V.; Xiong, S.; Yang, Y.; Ni, K.; Zhou, Q.; Wu, G. Dual-comb spectroscopy resolved three-degree-of-freedom sensing. Photonics Res. 2021, 9, 243–251. [Google Scholar] [CrossRef]
- Li, D.; Feng, P.; Zhang, J.; Yu, D.; Wu, Z. An identification method for key geometric errors of machine tool based on matrix differential and experimental test. Proc. Inst. Mech. Eng. C J. Mech. Eng. Sci. 2014, 228, 3141–3155. [Google Scholar] [CrossRef]
- Cheng, G.; Ge, S.R. Error model and analysis of 3-RPS symmetrical parallel robot leg with three degree-of-freedom. J. China Univ. Min. Technol. 2009, 38, 50–55. [Google Scholar]
- Yang, Y.; Zhu, M.; Li, H.; Du, Z.; Yang, J. Volumetric error modelling and compensation for batch of vertical machining centers based on sensitivity analysis. J. Mech. Eng. 2020, 56, 204–212. [Google Scholar]
- Cui, C.; Feng, Q.; Zhang, B.; Zhao, Y. System for simultaneously measuring 6DOF geometric motion errors using a polarization maintaining fiber-coupled dual-frequency laser. Opt. Express 2016, 24, 6735–6748. [Google Scholar] [CrossRef] [PubMed]
- Zheng, F.; Feng, Q.; Zhang, B.; Li, J. A method for simultaneously measuring 6DOF geometric motion errors of linear and rotary axes using lasers. Sensors 2019, 19, 1764. [Google Scholar] [CrossRef] [PubMed]
- Zheng, F.; Feng, Q.; Zhang, B.; Li, J.; Zhao, Y. A high-precision laser method for directly and quickly measuring 21 geometric motion errors of three linear axes of computer numerical control machine tools. Int. J. Adv. Manuf. Technol. 2020, 109, 1285–1296. [Google Scholar] [CrossRef]
- Du, Z.; Fan, K.; Yang, J. Technique and Application in Error Compensation for CNC Machine Tools, 1st ed.; China Machine Press: Being, China, 2020; pp. 63–65. [Google Scholar]

| Geometric Errors | X-Axis | Y-Axis | Z-Axis |
|---|---|---|---|
| Position error | δx(X) | δy(Y) | δz(Z) |
| Horizontal straightness | δy(X) | δx(Y) | δy(Z) |
| Vertical straightness | δz(X) | δz(Y) | δx(Z) |
| Yaw | εz(X) | εz(Y) | εx(Z) |
| Pitch | εy(X) | εx(Y) | εy(Z) |
| Roll | εx(X) | εy(Y) | εz(Z) |
| Squareness error | SXY, SZX, SYZ | ||
| Translational Error (μm) | Angular Error (″) | |||||
|---|---|---|---|---|---|---|
| X-Axis | δx(X) | δy(X) | δz(X) | εz(X) | εy(X) | εx(X) |
| 2.57 | 6.94 | 3.49 | 7.03 | 4.72 | 6.24 | |
| Y-Axis | δx(Y) | δy(Y) | δz(Y) | εz(Y) | εy(Y) | εx(Y) |
| 0.35 | 10.59 | 0.66 | 1.86 | 1.31 | 1.15 | |
| Z-Axis | δx(Z) | δy(Z) | δz(Z) | εz(Z) | εy(Z) | εx(Z) |
| 1.13 | 0.46 | 5.12 | 1.53 | 0.47 | 1.42 | |
| Squareness error | SXY: 20.59″; SYZ: 12.71″; SZX: 2.11″ | |||||
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zheng, F.; Zhang, B.; Zhao, Y.; Li, J.; Long, F.; Feng, Q. Efficient Method for Identifying Key Errors Based on 21-Geometric-Error Measurement of Three Linear Axes of Machine Tools. Appl. Sci. 2024, 14, 2982. https://doi.org/10.3390/app14072982
Zheng F, Zhang B, Zhao Y, Li J, Long F, Feng Q. Efficient Method for Identifying Key Errors Based on 21-Geometric-Error Measurement of Three Linear Axes of Machine Tools. Applied Sciences. 2024; 14(7):2982. https://doi.org/10.3390/app14072982
Chicago/Turabian StyleZheng, Fajia, Bin Zhang, Yuqiong Zhao, Jiakun Li, Fei Long, and Qibo Feng. 2024. "Efficient Method for Identifying Key Errors Based on 21-Geometric-Error Measurement of Three Linear Axes of Machine Tools" Applied Sciences 14, no. 7: 2982. https://doi.org/10.3390/app14072982
APA StyleZheng, F., Zhang, B., Zhao, Y., Li, J., Long, F., & Feng, Q. (2024). Efficient Method for Identifying Key Errors Based on 21-Geometric-Error Measurement of Three Linear Axes of Machine Tools. Applied Sciences, 14(7), 2982. https://doi.org/10.3390/app14072982








