Shaking Table Testing and Numerical Study on Aseismic Measures of Twin-Tube Tunnel Crossing Fault Zone with Extra-Large Section
Abstract
1. Introduction
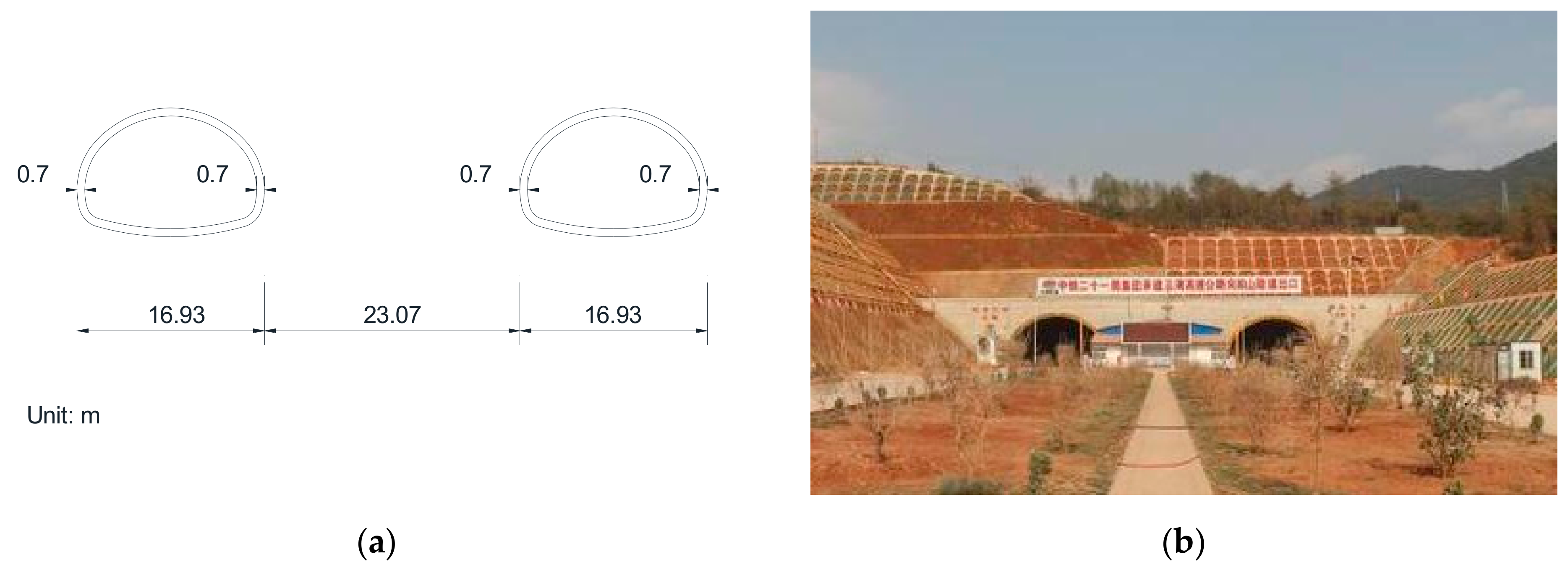
2. Case Study Tunnel
3. Shake Table Model Tests
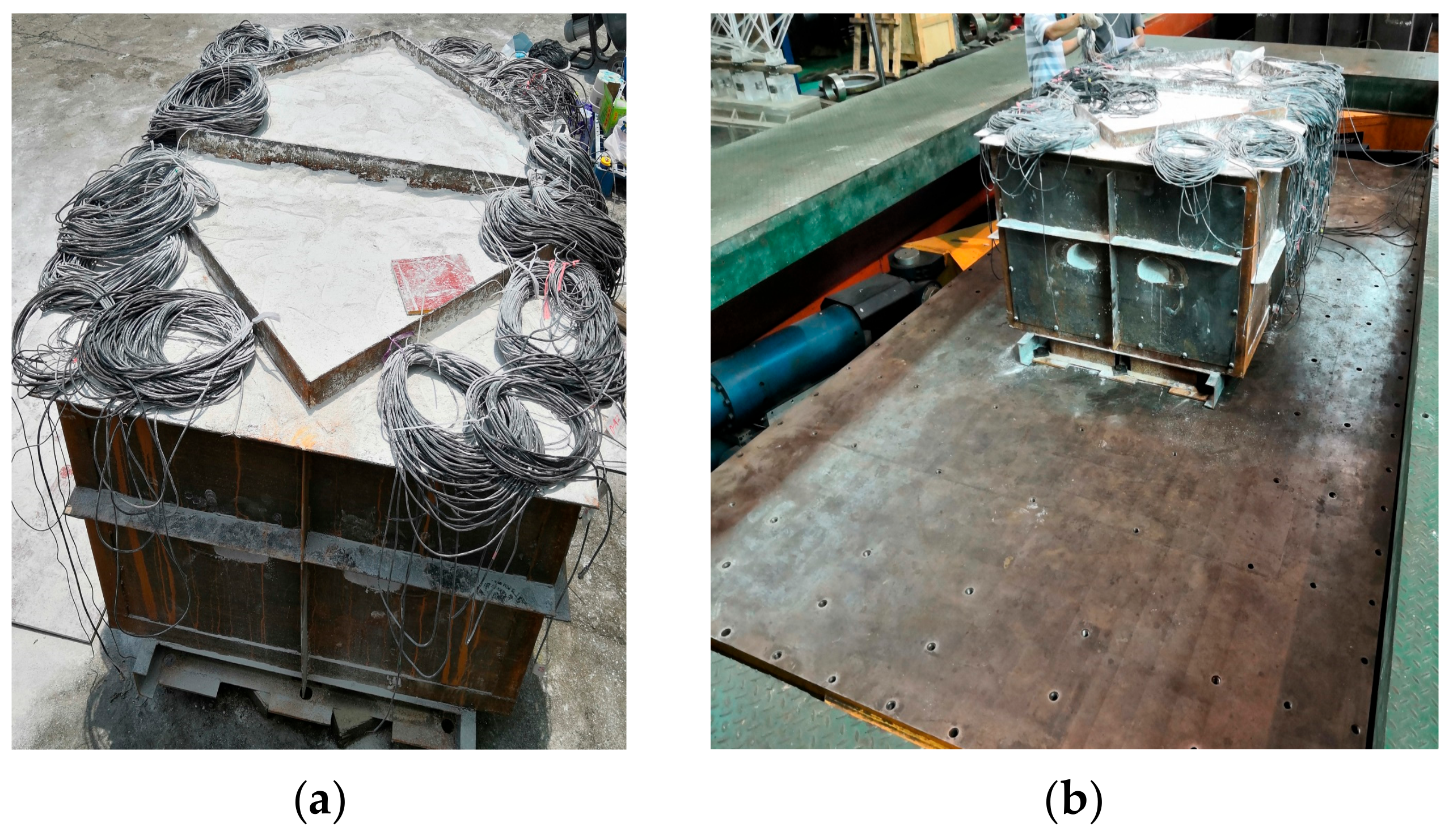
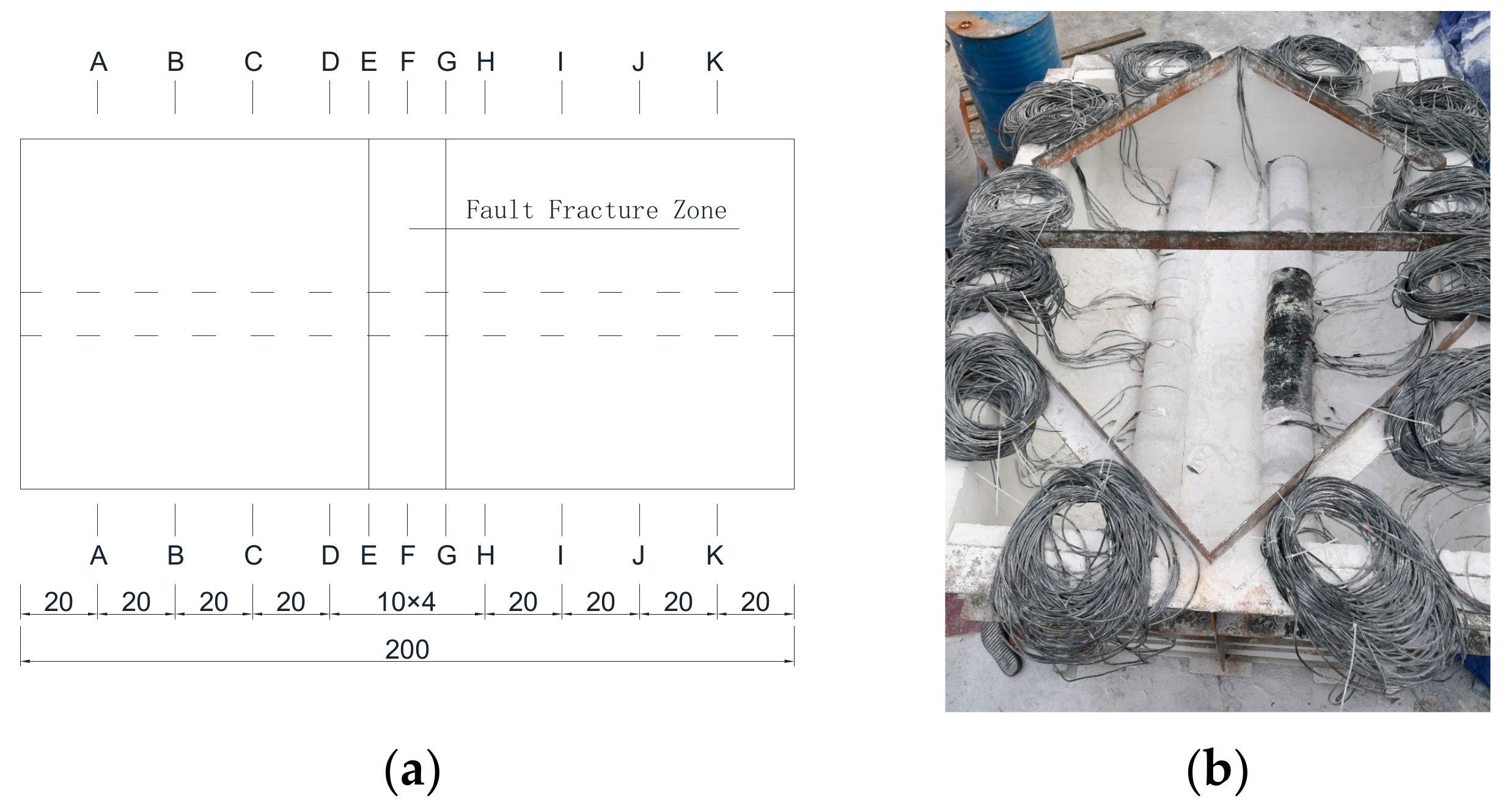
3.1. Model Design and Fabrication
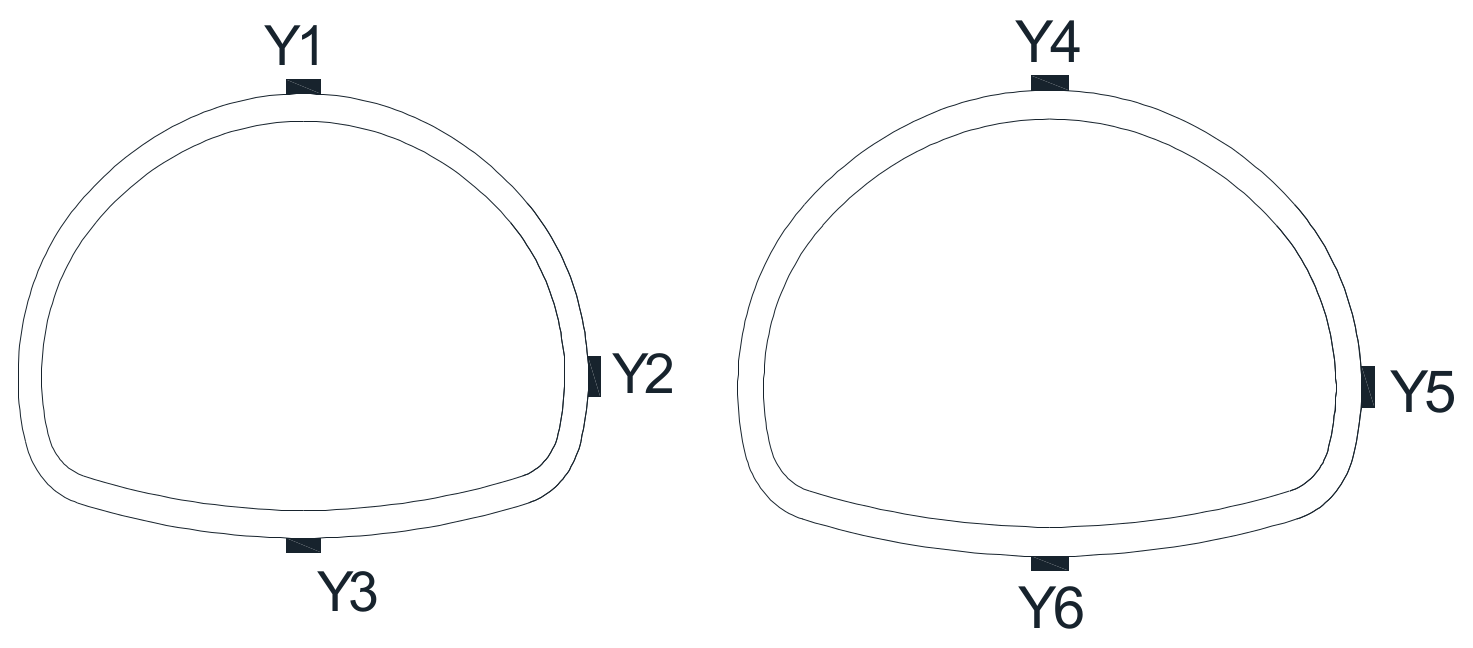
3.2. Instrumentation and Measurement
3.3. Loading Protocols
3.4. Results and Analysis
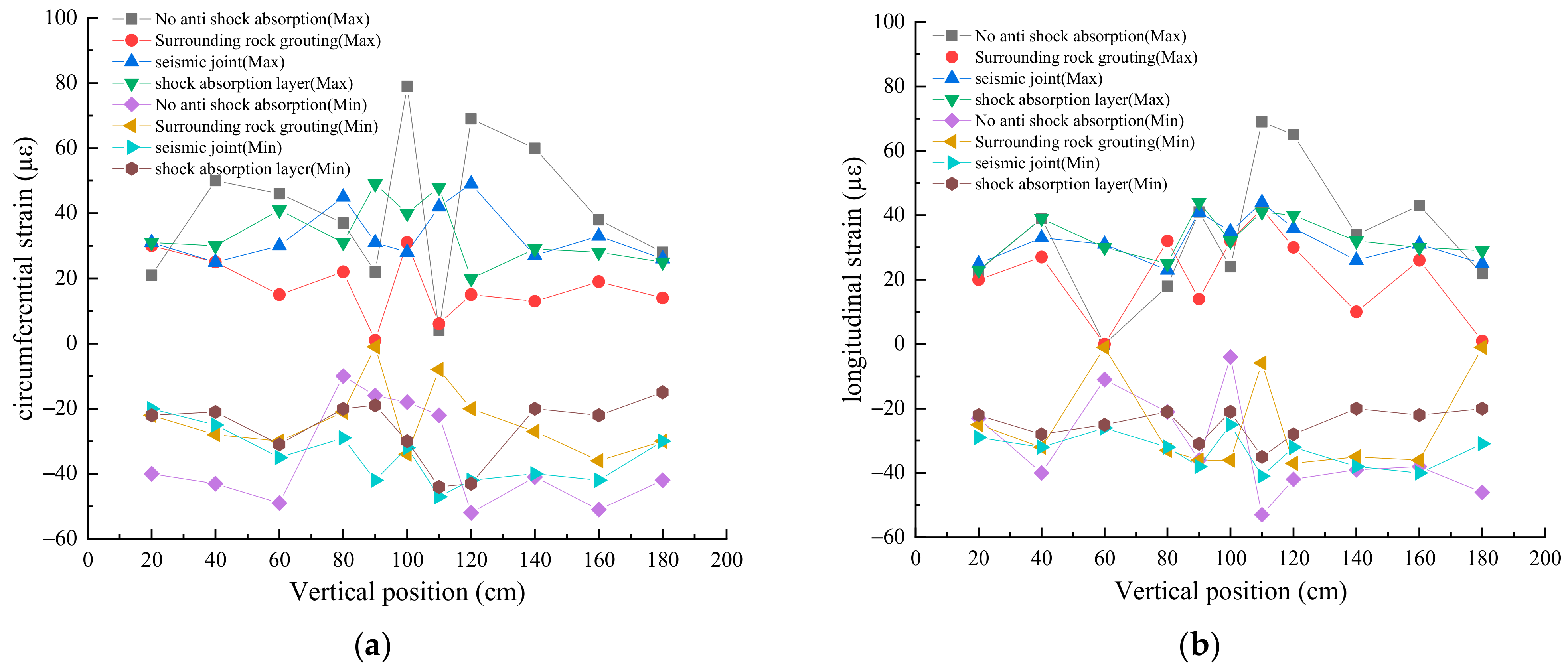
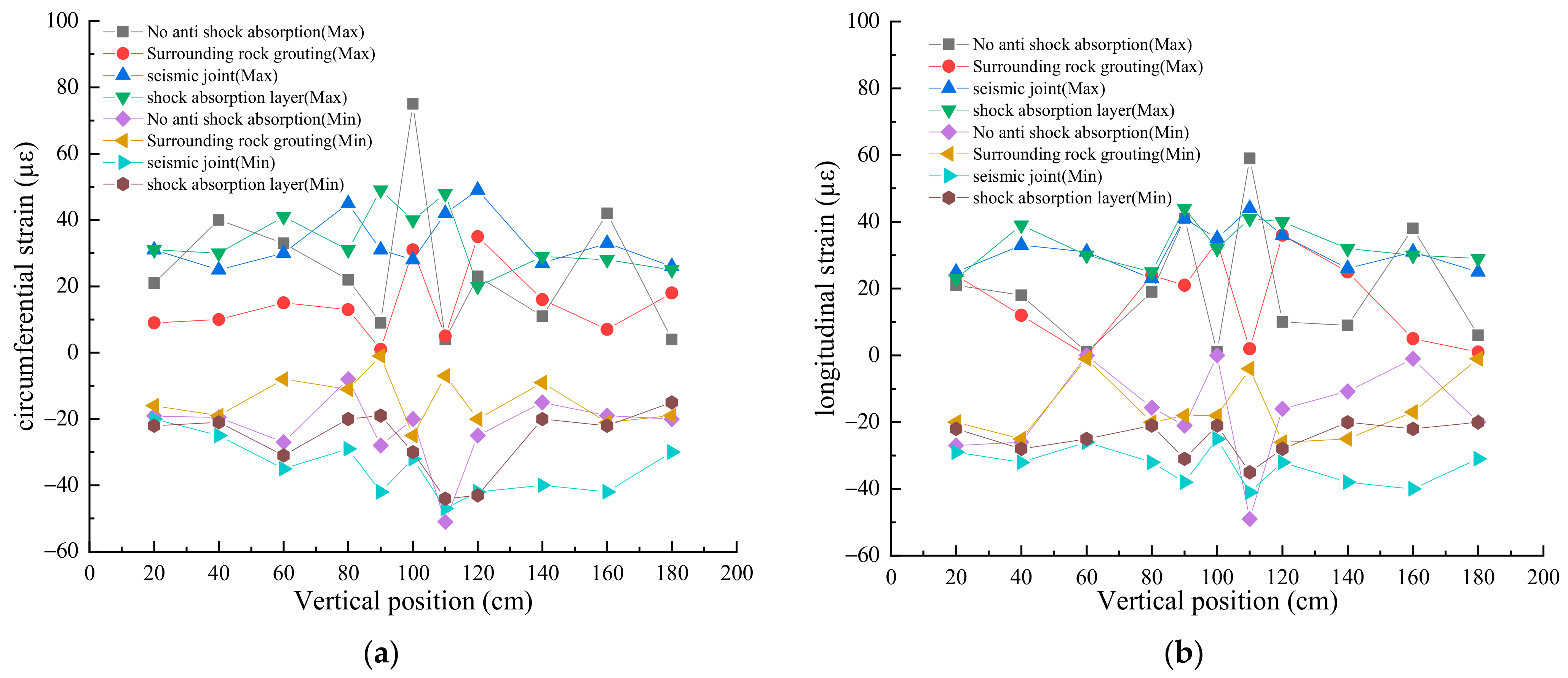
3.4.1. Strain Analysis
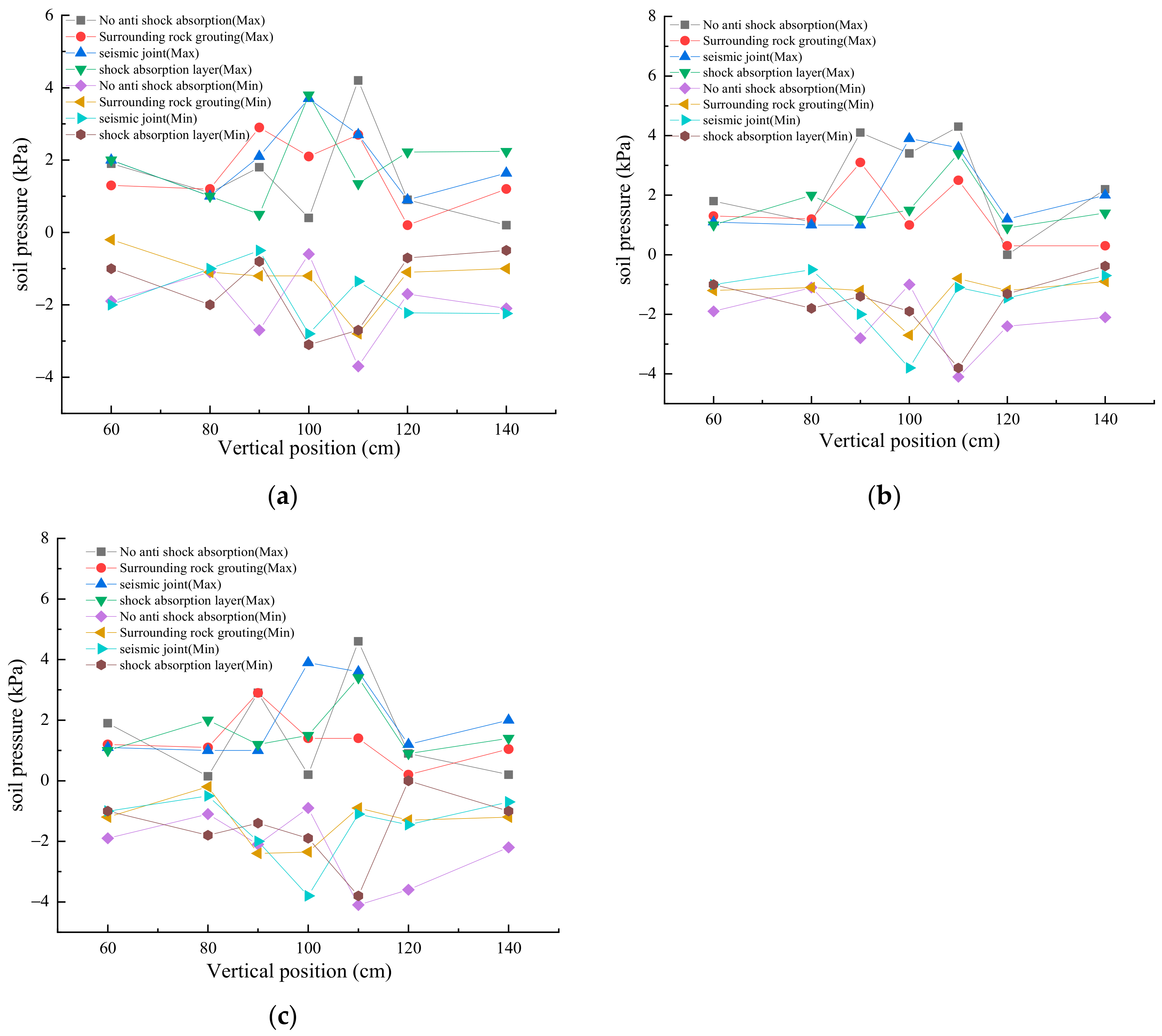
3.4.2. Earth Pressure Analysis
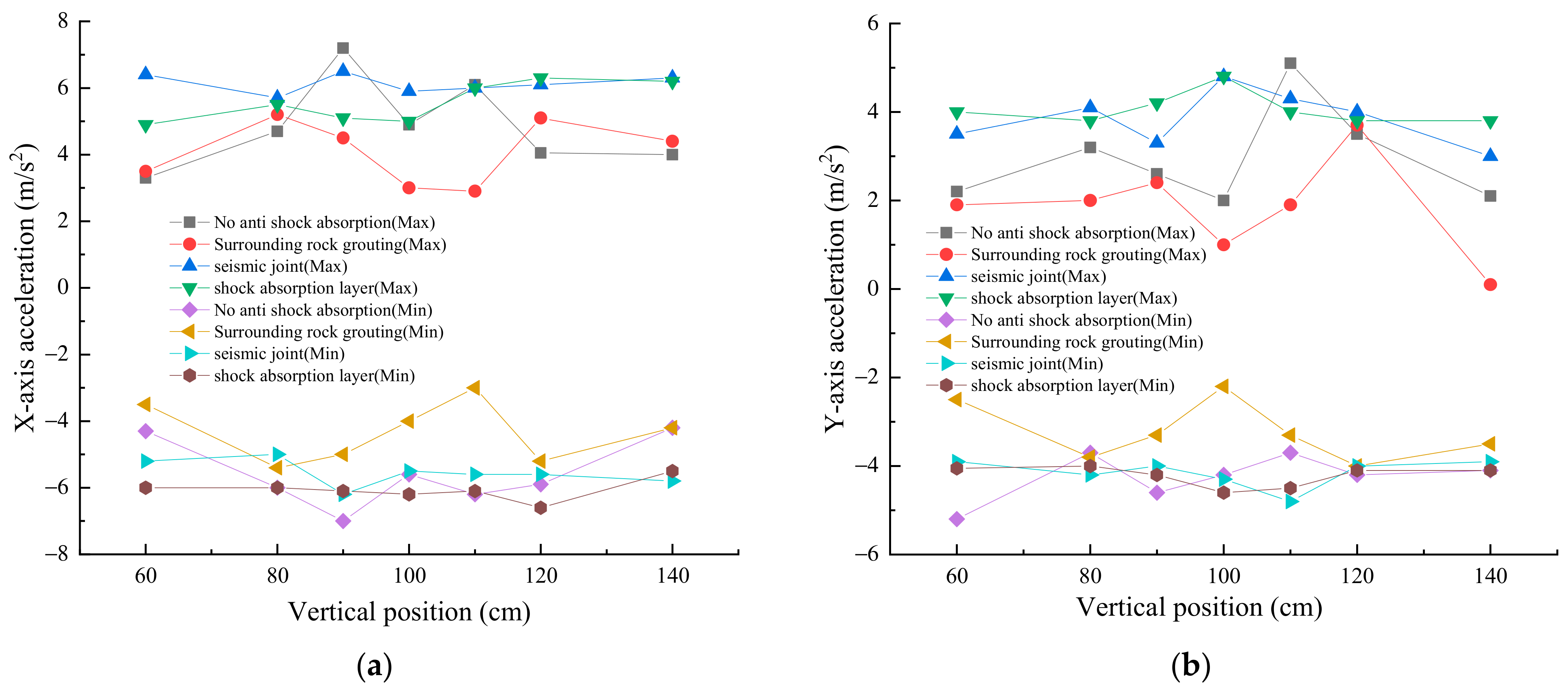
3.4.3. Acceleration Analysis
3.4.4. Discussion
4. Numerical Simulation
4.1. Theoretical Basis
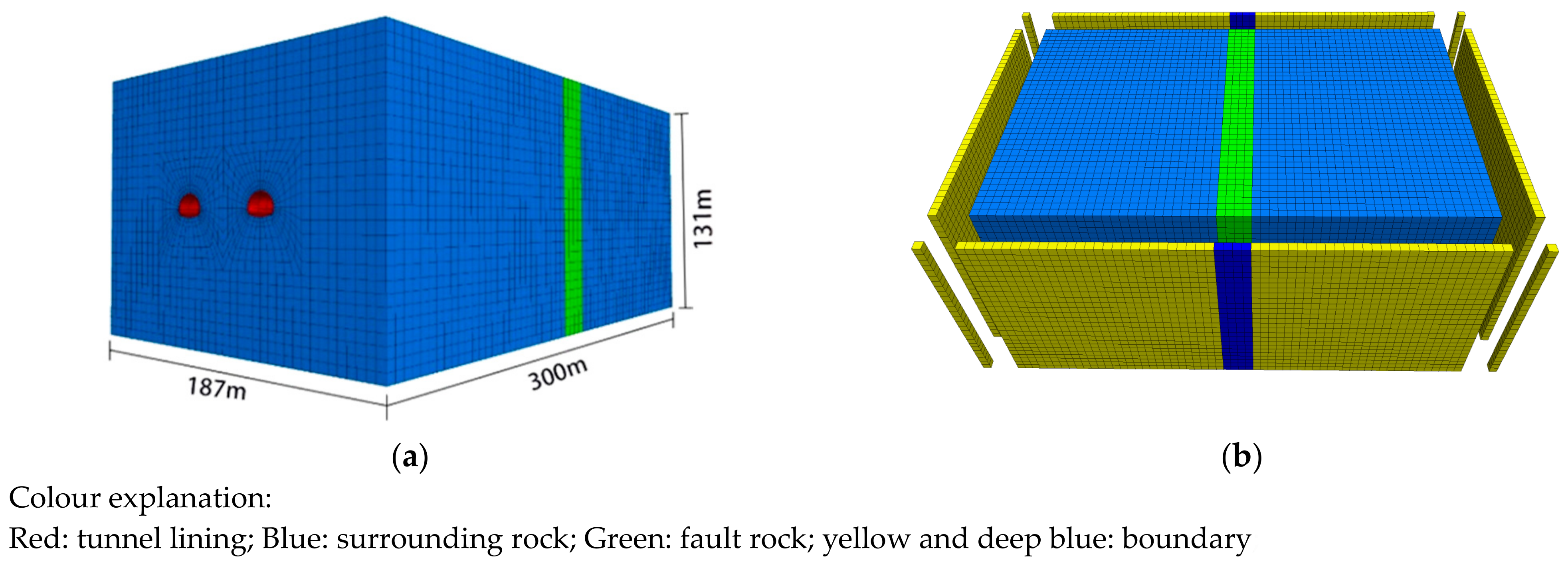
4.2. Computational Model
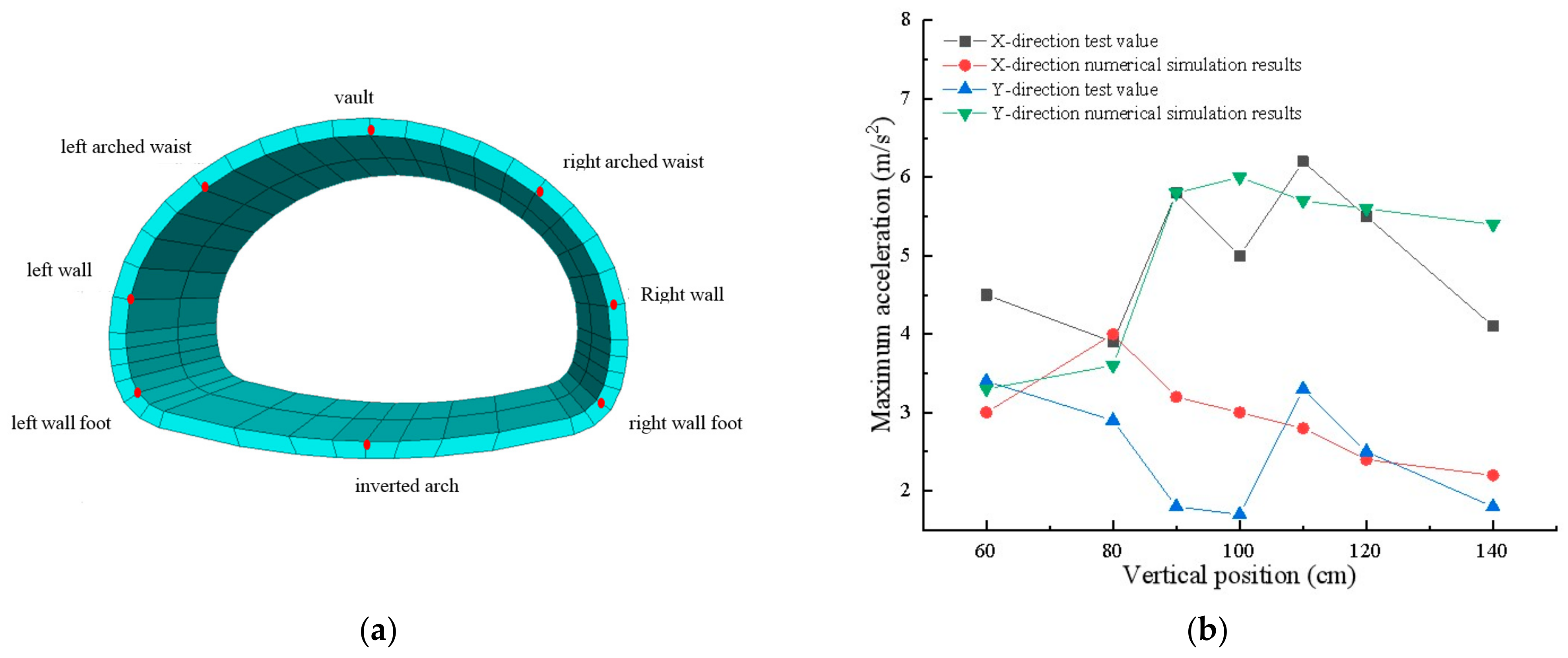
4.3. Validation of Numerical Model
4.4. Parametric Study of Controls
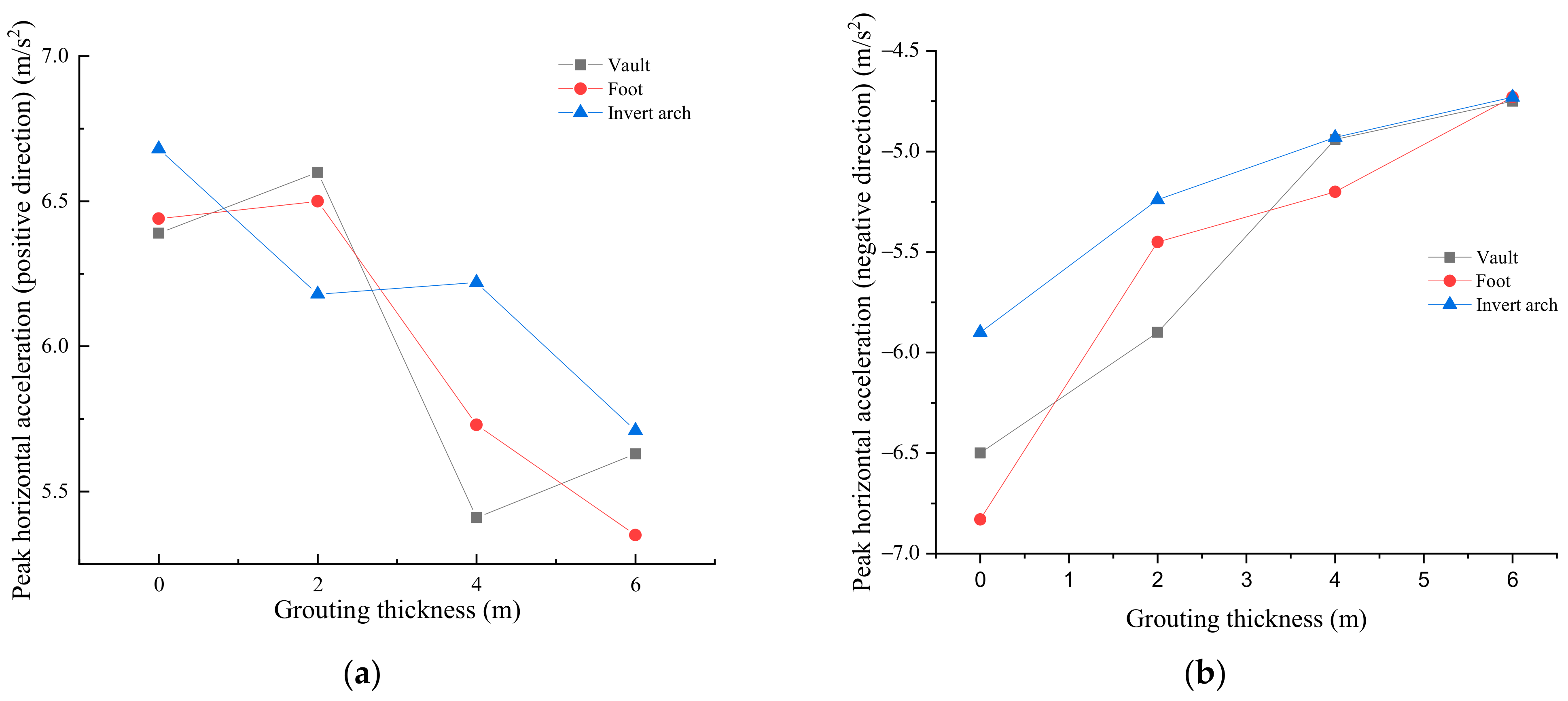
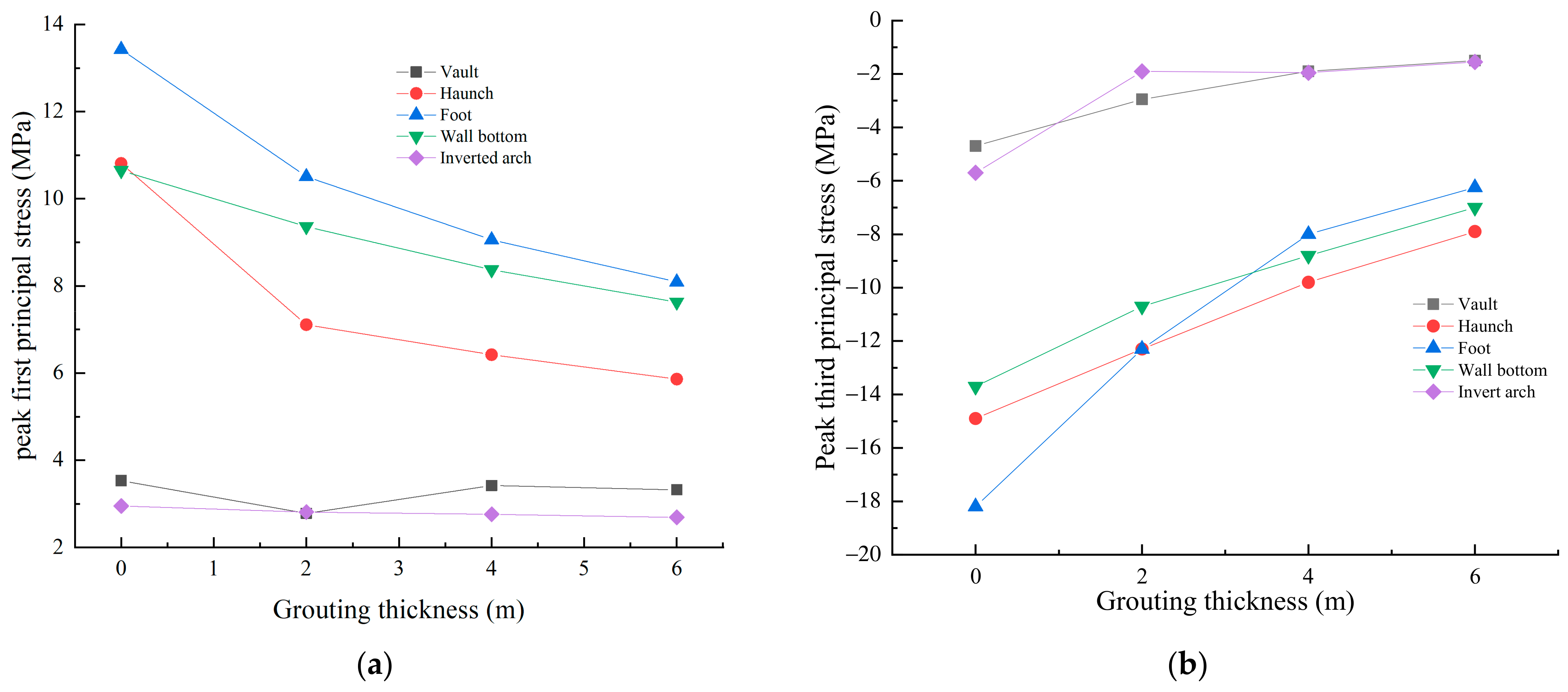
4.4.1. Grouting Layer Thickness
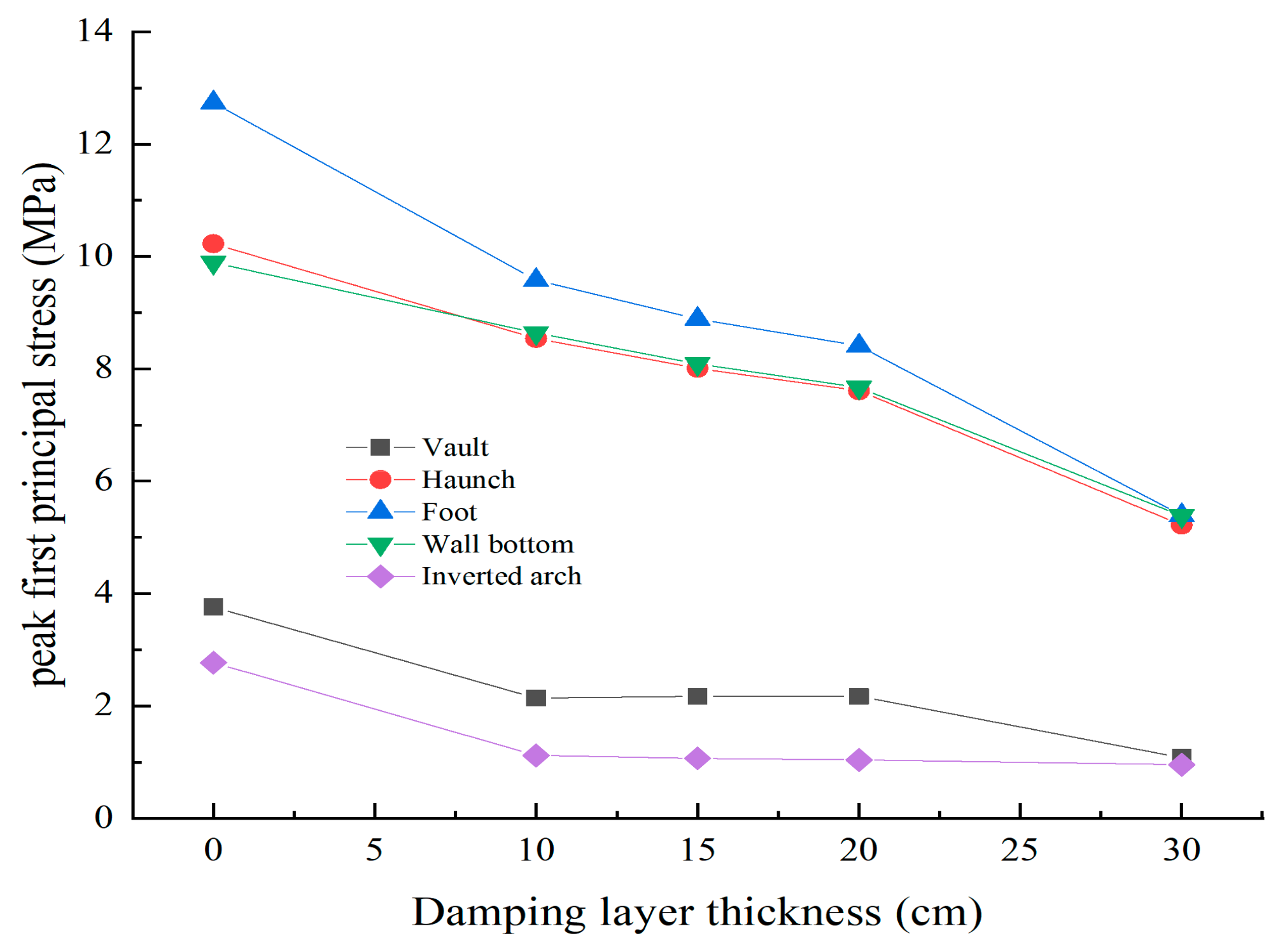
4.4.2. Shock Absorbing Layer Thickness
4.4.3. Overall Analysis
5. Conclusions
- (1)
- Under the excitation of three waveforms, strain, earth pressure, and acceleration all showed a tendency to be slightly higher along the longitudinal direction of the tunnel at the fault section and its surroundings. The most significant amplification effect was observed at the interface between the fault zone and the surrounding rock. This amplification effect extends from the boundary of the surrounding rock to the entire surrounding rock, with a maximum extension distance of 10 m. It is recommended that the minimum length of seismic fortification at both ends of the fault section in tunnels with large spans should be extended by 10 to 20 m beyond that specified for tunnels with the standard section in the Chinese industry standard (JTG D70-2004).
- (2)
- In general, the variation in the anti-vibration effect under different waveform excitations remained largely consistent, affirming the accuracy of the model test results. Following the implementation of seismic joints and shock-absorbing layers, there were changes in the positions of the maximum seismic response peaks at fault interface sections. Grouting reinforcement of the surrounding rock, installation of seismic joints, and incorporation of shock-absorbing layers all demonstrated noticeable seismic effects. The seismic impact of seismic joints and shock-absorbing layers was comparable, whereas the reinforcement effect of grouting on the surrounding rock surpassed that of the other two measures.
- (3)
- As the thickness of the grouting layer increased, there was a significant decrease in both the acceleration and the peak value of principal stress in the tunnel structure. The influence of the grouting layer on stress response was more pronounced than on acceleration, with the maximum reduction in principal stress reaching up to 73%. When the grouting layer thickness reached 6 m, the tunnel principal stress complied with the code requirements. At this thickness, the acceleration response peak value at the fracture zone position approached that of the intact section of the surrounding rock, the longitudinal distribution curve of acceleration became gentler, the amplification effect of lining acceleration at the fracture zone position diminished significantly, and the impact of further increasing the grouting layer thickness became negligible. It was recommended that the grouting layer thickness be maintained at approximately 6 m.
- (4)
- The horizontal acceleration of the lining at the fault of an extra-large section tunnel increased with the thickness of the shock-absorbing layer. When the shock-absorbing layer thickness was 30 cm, the acceleration amplification reached 2.52 times, representing the largest difference. The installation of a shock-absorbing layer could significantly reduce the first principal stress value of the lining structure at the fault fracture zone, with the maximum reduction rate nearing 62%. Initially, the reduction rate slightly increased with the thickness of the shock-absorbing layer and gradually stabilized. Once the shock-absorbing layer thickness exceeded 20 cm, the effectiveness of further increasing the thickness became limited. It was recommended to maintain the shock-absorbing layer thickness at 20 cm.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Editorial Department of China Journal of Highway and Transport. Review on China’s Traffic Tunnel Engineering Research: 2022. China J. Highw. Transp. 2022, 35, 1–40. (In Chinese) [Google Scholar]
- Gong, J.; Tang, G.; Wang, W.; Fan, L. As of the End of 2021, Statistics on the Situation of Railway Tunnels in China and an Overview of the Design and Construction of the Gaoligongshan Tunnel. Tunn. Constr. 2022, 42, 508–517. (In Chinese) [Google Scholar]
- Hong, K.; Feng, H. Development Trends and Views of Highway Tunnels in China over the Past Decade. China J. Highw. Transp. 2020, 33, 62–76. (In Chinese) [Google Scholar]
- Khan, M.S.; Khan, Z.A.; Sadique, M.R.; Alam, M.M. Seismic Behaviour of Double Arched Tunnel: A Review. IOP Conf. Ser. Earth Environ. Sci. 2021, 796, 012043. [Google Scholar] [CrossRef]
- JTG 2232-2019; Ministry of Transport of the People’s Republic of China. Specification for Seismic Design of Highway Tunnels. China Communication Press: Beijing, China, 2022. (In Chinese)
- GB/T 51336-2018; Ministry of Housing and Urban Rural Development of the People’s Republic of China, State Administration for Market Regulation. Standard for Seismic Design of Underground Structures. China Architecture & Building Press: Beijing, China, 2019. (In Chinese)
- GB 50111-2006; The Ministry of Railways of the People’s Republic of China. Code for Seismic Design of Railway Engineering. China Planning Press: Beijing, China, 2009. (In Chinese)
- Asakura, T.; Shiba, Y.; Matsuoka, S.; Oya, T.; Yashiro, K. Damage to mountain tunnels by earthquake and its mechanism. Doboku Gakkai Ronbunshu. 2000, 659, 27–38. [Google Scholar] [CrossRef] [PubMed]
- Xu, H.; Li, T.; Xia, L.; Zhao, J.X.; Wang, D. Shaking table tests on seismic measures of a model mountain tunnel. Tunn. Undergr. Space Technol. Inc. Trenchless Technol. Res. 2016, 60, 197–209. [Google Scholar] [CrossRef]
- Tsinidis, G.; de Silva, F.; Anastasopoulos, I.; Bilotta, E.; Bobet, A.; Hashash, Y.M.; He, C.; Kampas, G.; Knappett, J.; Madabhushi, G.; et al. Seismic behaviour of tunnels: From experiments to analysis. Tunn. Undergr. Space Technol. Inc. Trenchless Technol. Res. 2020, 99, 103334. [Google Scholar] [CrossRef]
- Prasad, S.K.; Towhata, I.; Chandradhara, G.P.; Nanjundaswamy, P. Shaking table tests in earthquake geotechnical engineering. Curr. Sci. Geotech. Earthq. Hazards 2004, 87, 1398–1404. [Google Scholar]
- Liu, J.; Liu, X.; Wang, Z.; Zhao, D. Dynamic Centrifuge Model Test of a Soil-structure Interaction System. China Civ. Eng. J. 2010, 43, 114–121. (In Chinese) [Google Scholar] [CrossRef]
- Xie, H.T. Numerical Analysis on Influence of Cross Section Shape on Earthquake Resistant Capability of Shallow-Buried Tunnel. Appl. Mech. Mater. 2013, 405, 1292–1296. [Google Scholar] [CrossRef]
- Salemi, A.; Mikaeil, R.; Haghshenas, S.S. Integration of Finite Difference Method and Genetic Algorithm to Seismic analysis of Circular Shallow Tunnels (Case Study: Tabriz Urban Railway Tunnels). KSCE J. Civ. Eng. 2018, 22, 1978–1990. [Google Scholar] [CrossRef]
- Momenzadeh, M.; Koopialipoor, M.; Tootoonchi, H.; Khalili, F.; Khorami, S.; Khorami, S. Investigation of Seismic Reliability Index in Shallow Underground Tunnels by Combining Three Methods of Surface Response, Hasofer–Lind and Finite Element Method. Geotech. Geol. Eng. 2019, 37, 3903–3914. [Google Scholar] [CrossRef]
- An, D.; Chen, Z.; Cui, G. Research on Seismic Performance of Fiber Concrete Lining Structure of Urban Shallow-Buried Rectangular Tunnel in Strong Earthquake Area. KSCE J. Civ. Eng. 2021, 25, 2748–2757. [Google Scholar] [CrossRef]
- Wang, J.; Hu, Y.; Fu, B.; Shan, H.; Wei, H.; Cui, G. Study on Antiseismic Effect of Different Thicknesses of Shock Absorption Layer on Urban Shallow Buried Double Arch Rectangular Tunnel. Shock. Vib. 2022, 2022, 4863756. [Google Scholar] [CrossRef]
- Liu, S.; Liu, S.; Lu, S.; Ma, F.; Pei, G. Seismic Behaviour of Shallow Tunnelling Method Tunnels Accounting for Primary Lining Effects. Buildings 2022, 13, 20. [Google Scholar] [CrossRef]
- Zhang, W.; Han, L.; Feng, L.; Ding, X.; Wang, L.; Chen, Z.; Liu, H.; Aljarmouzi, A.; Sun, W. Study on seismic behaviors of a double box utility tunnel with joint connections using shaking table model tests. Soil Dyn. Earthq. Eng. 2020, 136, 106118. [Google Scholar] [CrossRef]
- Liang, J.; Xu, A.; Ba, Z.; Chen, R.; Zhang, W.; Liu, M. Shaking table test and numerical simulation on ultra-large diameter shield tunnel passing through soft-hard stratum. Soil Dyn. Earthq. Eng. 2021, 147, 106790. [Google Scholar] [CrossRef]
- Zhang, S.; Yang, Y.; Yuan, Y.; Li, C.; Qiu, J. Experimental investigation of seismic performance of shield tunnel under near-field ground motion. Structures 2022, 43, 1407–1421. [Google Scholar] [CrossRef]
- JTG D70-2004. Ministry of Transport of the People’s Republic of China. Code for Design of Road Tunnel. China Communication Press: Beijing, China, 2004. (In Chinese)
- ALMahdi, F.; Fahjan, Y.; Doğangün, A. Critical remarks on Rayleigh damping model considering the explicit scheme for the dynamic response analysis of high rise buildings. Adv. Struct. Eng. 2021, 24, 1955–1971. [Google Scholar] [CrossRef]
- Gu, Y.; Liu, J.-B.; Du, Y.-X. 3D consistent viscous-spring artificial boundary and viscous-spring boundary element. Eng. Mech. 2007, 24, 31–37. (In Chinese) [Google Scholar]
- Liu, J.; Lu, Y. A direct method for analysis of dynamic soil-structure interaction. China Civ. Eng. J. 1998, 31, 55–64. (In Chinese) [Google Scholar]
- Zhang, B.; Li, S.; Yang, X.; Wang, X. Research on seismic wave input with three-dimensional viscoelastic artificial boundary. Rock Soil Mech. 2009, 30, 774–778. (In Chinese) [Google Scholar]
- Zhou, Q.; Zhou, J.; Ma, H.; Li, X.; Zhang, L. An improved algorithm for the optimization of the primary tensioning force of the steel pipe arch rib section. J. Transp. Eng. 2020, 20, 93–99. (In Chinese) [Google Scholar]
- Zhou, Q.; Zhou, J.; Feng, P.; Xin, L.; Jing, S.; Zheng, G. Full-Scale Experimental Study on Temperature Field of Large-Diameter CFST Arch Bridges under Strong Radiation and Large Daily Ambient Temperature Difference. J. Civ. Struct. Health Monit. 2022, 12, 1247–1263. [Google Scholar] [CrossRef]
- Lai, J.; He, S.; Qiu, J.; Chen, J.; Wang, L.; Wang, K.; Wang, J. Characteristics of seismic disasters and aseismic measures of tunnels in Wenchuan earthquake. Environ. Earth Sci. 2017, 76, 1–19. [Google Scholar] [CrossRef]

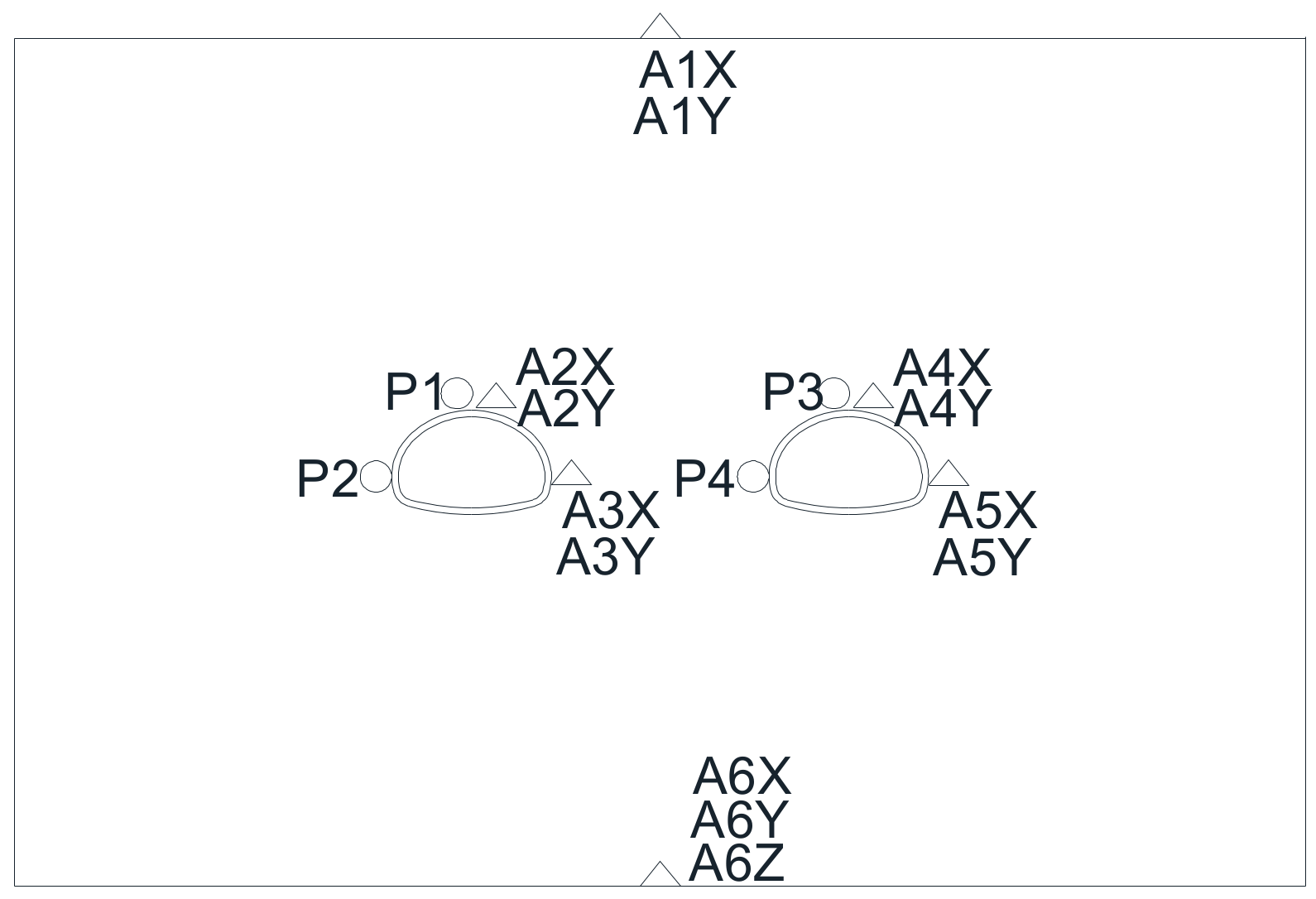




| Physical Parameters | Similarity Relationships | Physical Parameters | Similarity Relationships |
|---|---|---|---|
| Stress σ | 1/137.5 | Time T | 1/11.72604 |
| Strain ε | 1/1.375 | Frequency f | 1/0.08528 |
| Elastic modulus E | 1/100 | Velocity v | 1/11.72604 |
| Poisson’s ratio μ | 1 | Accelerate a | 1/0.727263 |
| Density ρ | 1/1.375 | gravitational acceleration g | 1 |
| Length L | 1/100 | Cohesive force c | 1/137.5 |
| Displacement u | 1/137.5 | Damping C | 1/1612.33 |
| Force F | 1/1375000 | Stiffness K | 1/10000 |
| Model | Setting Conditions | Proposed Research Content | Model Overall Size |
|---|---|---|---|
| Model 1 | The fault has a dip of 90 degrees and a width of 20 m, and the tunnel is a two-hole tunnel with a small clearance distance. The left tunnel does not have any anti-vibration measures and is supported by general V surrounding rock conditions. In contrast, grouting is used to reinforce the surrounding rock in the right tunnel. The grouting is carried out at intervals of the entire ring with a thickness of 4 m. Different reinforcement lengths are set before and after the longitudinal upper fault, with lengths of 25 m and 35 m, respectively. | (1) Seismic response characteristics of an extra-large section tunnel. (2) Strengthening effect of grouting reinforcement on surrounding rock in fault-related areas. | 137 cm × 200 cm × 91 cm based on similarity ratio calculation. |
| Model 2 | The fault has a dip of 90 degrees and a width of 20 m, and the tunnel is a two-hole tunnel with a small clearance distance. Both the left and right tunnels are supported by Class V surrounding rock. The left tunnel is equipped with seismic joints, while the right tunnel is provided with shock absorption layers. Different reinforcement ranges are set before and after the longitudinal upper fault, with lengths of 25 m and 35 m, respectively. | (1) Establishing the strengthening effect of seismic joints. (2) Establishing the strengthening effect of the shock absorption layer. |
| No. | Loading Model | Input Seismic Wave | Seismic Input Mode | Model Loading Intensity |
|---|---|---|---|---|
| 1 | Model 1 and Model 2 | White noise | Bottom synchronous input X, Y, Z direction vibration | Load seismic wave peak 0.10 A |
| 2 | Model 1 and Model 2 | El-Centro wave | Bottom input X direction vibration | Load seismic wave peak 0.25 A |
| 3 | Model 1 and Model 2 | Taft wave | Bottom input X direction vibration | Load seismic wave peak 0.25 A |
| 4 | Model 1 and Model 2 | Artificial wave | Bottom input X direction vibration | Load seismic wave peak 0.25 A |
| 5 | Model 1 and Model 2 | White noise | Bottom synchronous input X, Y, Z direction vibration | Load seismic wave peak 0.10 A |
| 6 | Model 1 and Model 2 | El-Centro wave | Bottom input X direction vibration | Load seismic wave peak 0.5 A |
| 7 | Model 1 and Model 2 | Taft wave | Bottom input X direction vibration | Load seismic wave peak 0.5 A |
| 8 | Model 1 and Model 2 | Artificial wave | Bottom input X vibration | Load seismic wave peak 0.5 A |
| 9 | Model 1 and Model 2 | White noise | Bottom synchronous input X, Y, Z direction vibration | Load seismic wave peak 0.10 A |
| 10 | Model 1 and Model 2 | El-Centro wave | Bottom input X direction vibration | Load seismic wave peak 0.75 A |
| 11 | Model 1 and Model 2 | Taft wave | Bottom input X direction vibration | Load seismic wave peak 0.75 A |
| 12 | Model 1 and Model 2 | Artificial wave | Bottom input X direction vibration | Load seismic wave peak 0.75 A |
| 13 | Model 1 and Model 2 | White noise | Bottom synchronous input X, Y, Z direction vibration | Load seismic wave peak 0.10 A |
| 14 | Model 1 and Model 2 | El-Centro wave | Bottom input X direction vibration | Load seismic wave peak 1 A |
| 15 | Model 1 and Model 2 | Taft wave | Bottom input X direction vibration | Load seismic wave peak 1 A |
| 16 | Model 1 and Model 2 | Artificial wave | Bottom input X direction vibration | Load seismic wave peak 1 A |
| Anti-Shock Measures | Maximum Reduction Ratio (%) | |||||
|---|---|---|---|---|---|---|
| Waveform | Circumferential Strain | Longitudinal Strain | Earth Pressure | X-Axis Acceleration | Y-Axis Acceleration | |
| Surrounding rock grouting | El-Centro wave | −54.8% | −45% | −40% | −27.8% | −56.6% |
| Taft wave | −60.8% | −53.6% | −34% | −27.8% | −27.5% | |
| Artificial wave | −53.3% | −47% | −41.4% | −28% | −43.8% | |
| Average | −56.3% | −48.5% | −38.5% | −27.9% | −42.6% | |
| Seismic joints | El-Centro wave | −47.9% | −30.64% | −24.3% | −27.8% | −9.4% |
| Taft wave | −38% | −36.2% | −9.3% | −11.4% | −7.7% | |
| Artificial wave | −34.7% | −30.5% | −15.2% | −17.3% | −15.8% | |
| Average | −40.2% | −32.4% | −16.3% | −18.8% | −11.0% | |
| Shock absorbing layer | El-Centro wave | −30.1% | −24.2% | −16.2% | −12.5% | −9.4% |
| Taft wave | −38% | −36.2 | −20.9% | −12.5% | −11.5% | |
| Artificial wave | −34.7% | −28.6 | −26.1% | −12.5% | −15.8% | |
| Average | −34.3% | −29.7% | −21.1% | −12.5% | −12.2% | |
| Parameters | Grouting Thickness of Surrounding Rock | Shock Absorbing Layer Thickness | ||||||
|---|---|---|---|---|---|---|---|---|
| 2 m | 4 m | 6 m | 10 cm | 15 cm | 20 cm | 30 cm | ||
| Horizontal acceleration | Maximum reduction ratio/% | −20% | −24% | −31% | 17% | 21% | 23% | 46% |
| Appearance position | Arch foot | Arch foot | Arch foot | Arch foot | Inverted arch | Arch foot | Inverted arch | |
| Principal stress | Maximum reduction ratio/% | −68% | −65% | −73% | −60% | −61% | −62% | −65% |
| Appearance position | Inverted arch | Inverted arch | Inverted arch | Inverted arch | Inverted arch | Inverted arch | Inverted arch | |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zhao, F.; Liang, B.; Zhao, N.; Jiang, B. Shaking Table Testing and Numerical Study on Aseismic Measures of Twin-Tube Tunnel Crossing Fault Zone with Extra-Large Section. Appl. Sci. 2024, 14, 2391. https://doi.org/10.3390/app14062391
Zhao F, Liang B, Zhao N, Jiang B. Shaking Table Testing and Numerical Study on Aseismic Measures of Twin-Tube Tunnel Crossing Fault Zone with Extra-Large Section. Applied Sciences. 2024; 14(6):2391. https://doi.org/10.3390/app14062391
Chicago/Turabian StyleZhao, Fengbing, Bo Liang, Ningyu Zhao, and Bolin Jiang. 2024. "Shaking Table Testing and Numerical Study on Aseismic Measures of Twin-Tube Tunnel Crossing Fault Zone with Extra-Large Section" Applied Sciences 14, no. 6: 2391. https://doi.org/10.3390/app14062391
APA StyleZhao, F., Liang, B., Zhao, N., & Jiang, B. (2024). Shaking Table Testing and Numerical Study on Aseismic Measures of Twin-Tube Tunnel Crossing Fault Zone with Extra-Large Section. Applied Sciences, 14(6), 2391. https://doi.org/10.3390/app14062391






