Analysis of Vehicle-Bridge Coupling Vibration Characteristics of Curved Girder Bridges
Abstract
1. Introduction
2. Vehicle-Bridge Coupled Vibration of Curved Girder Bridges
2.1. Automotive Dynamic Analysis Model
2.2. Vibration Equation of Vehicle Model
- Formation of stiffness matrix
- Formation of damping matrix
- Formation of quality matrix
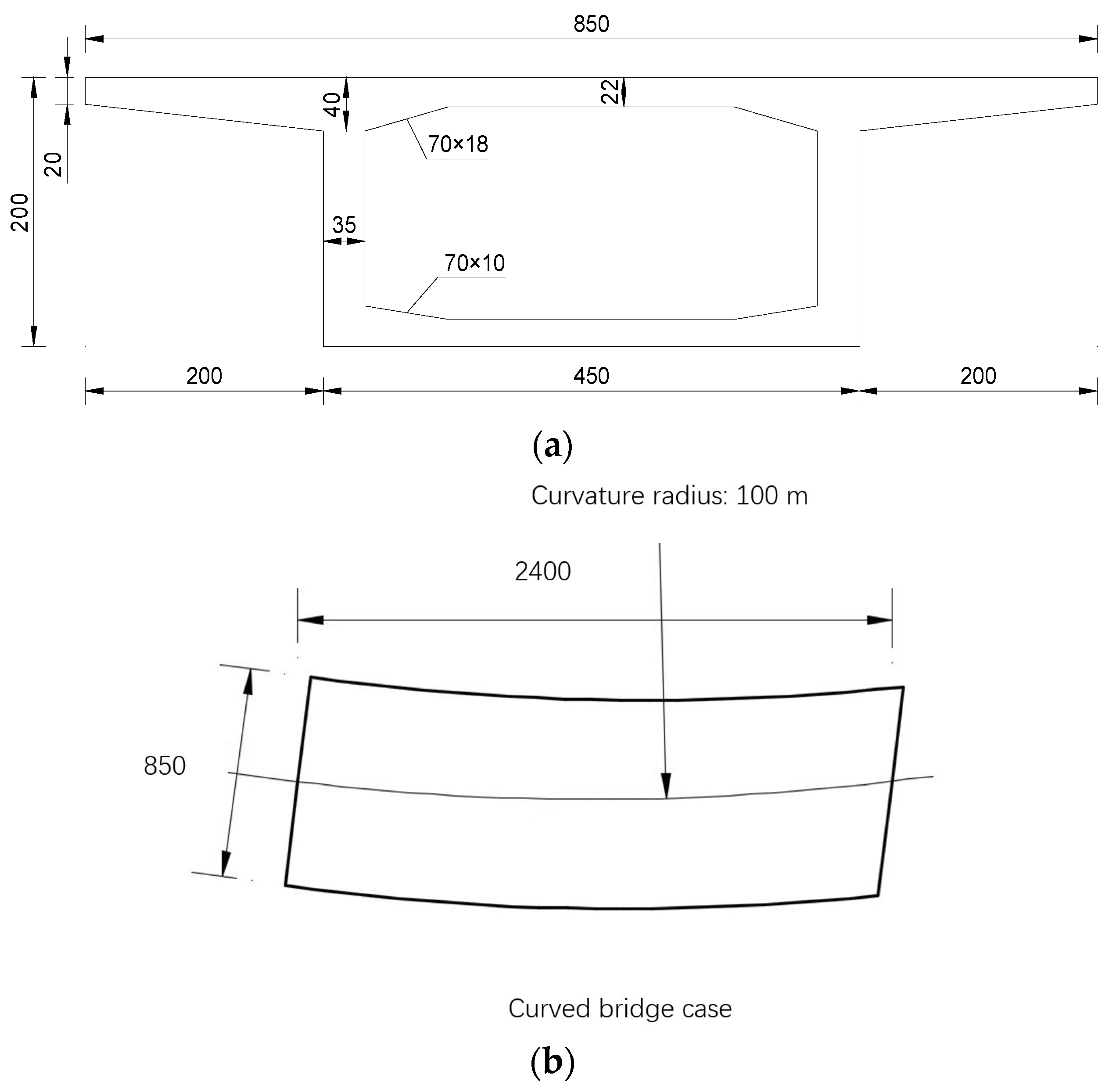
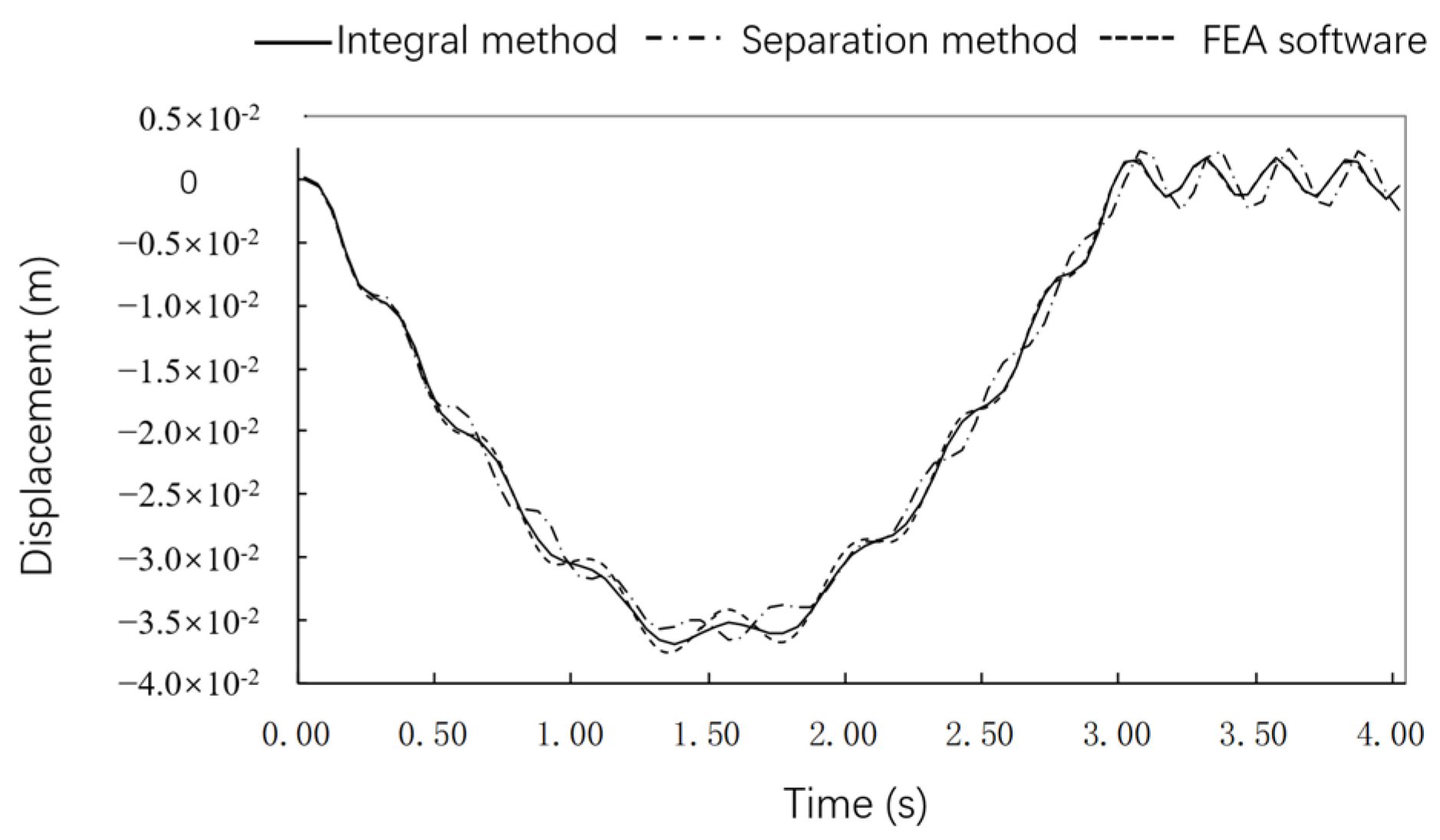
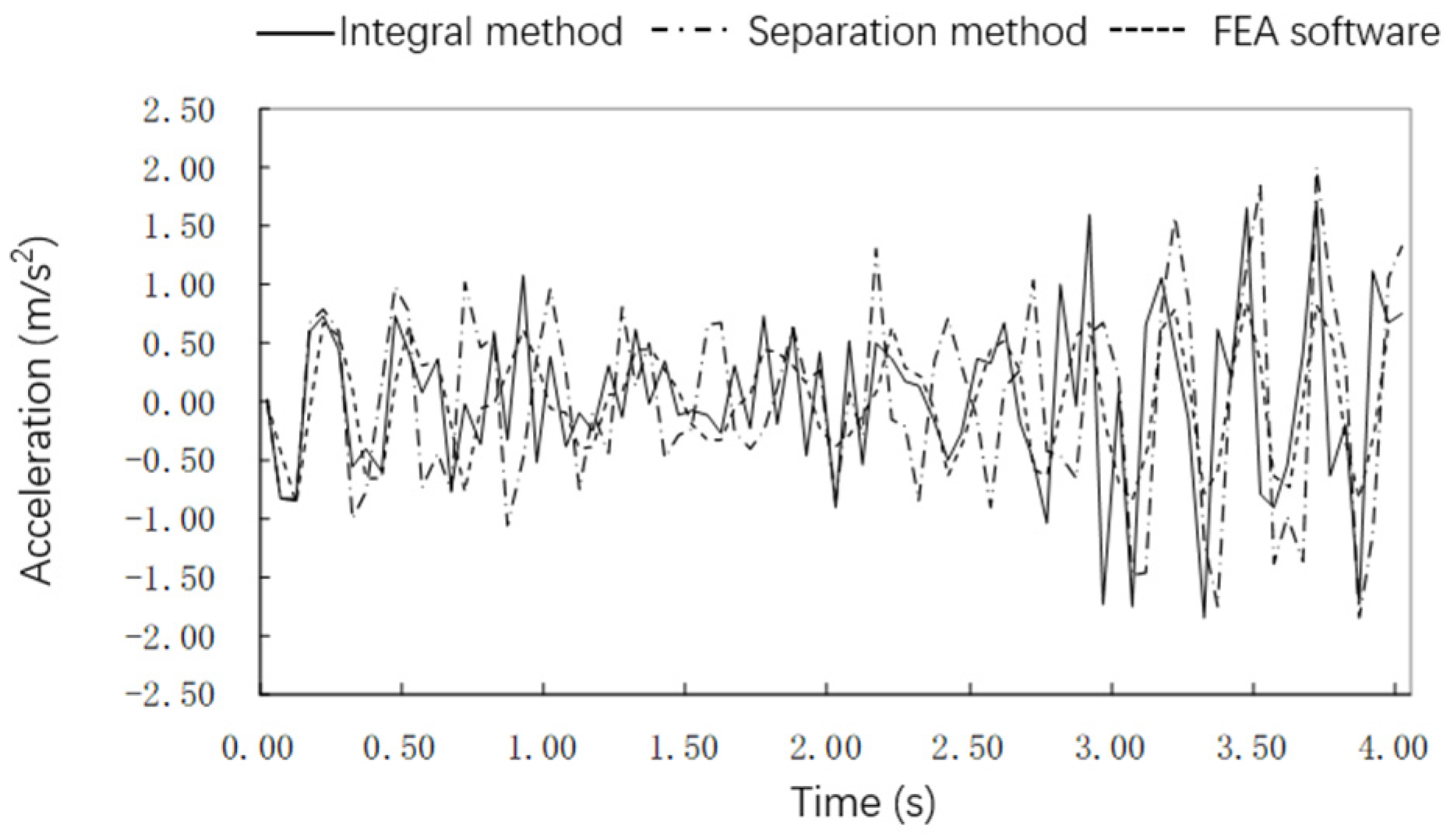
3. Verification of Programs
4. Spatial Vibration Analysis of the Coupled Time-Varying System of Vehicle-Curved Girder Bridge
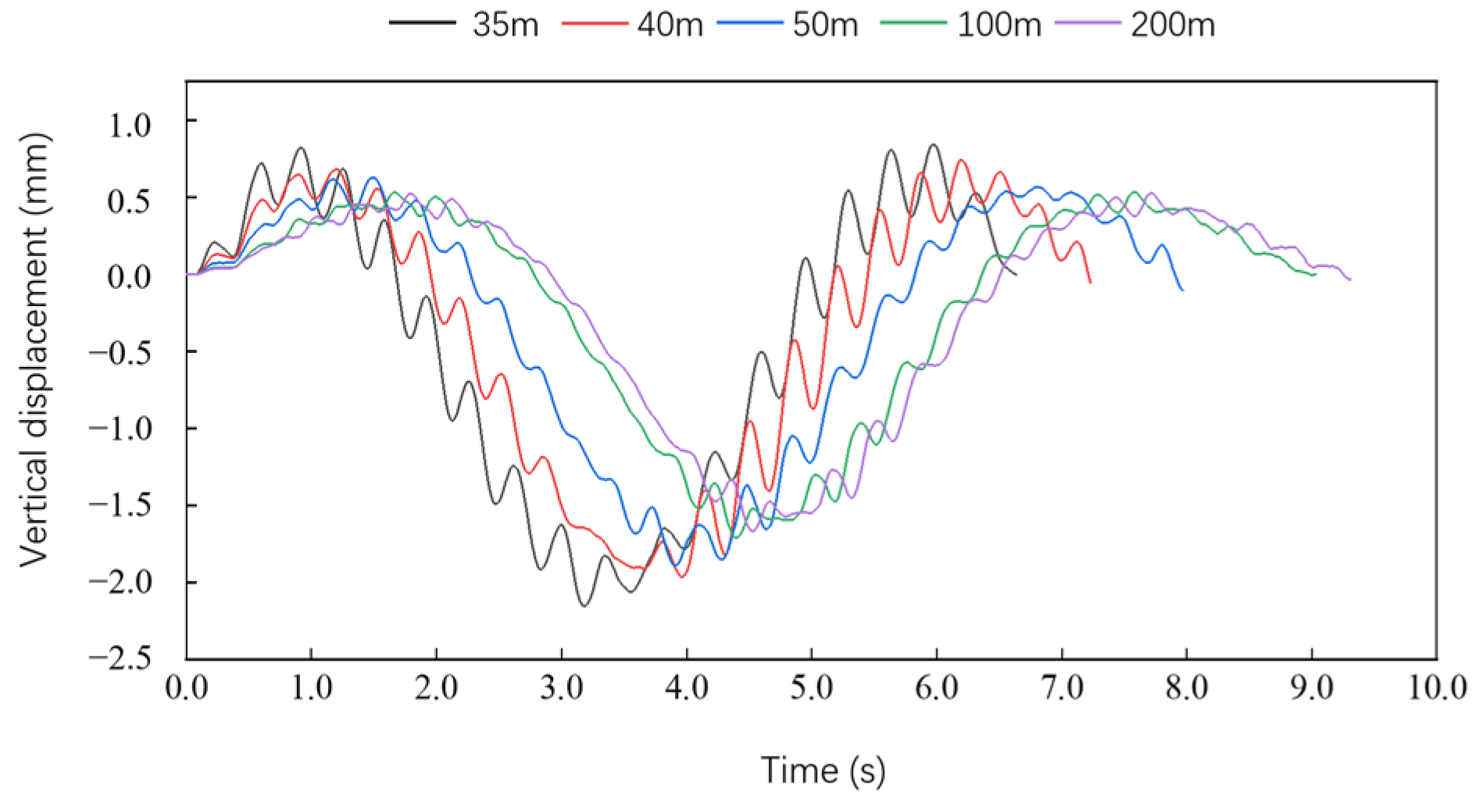
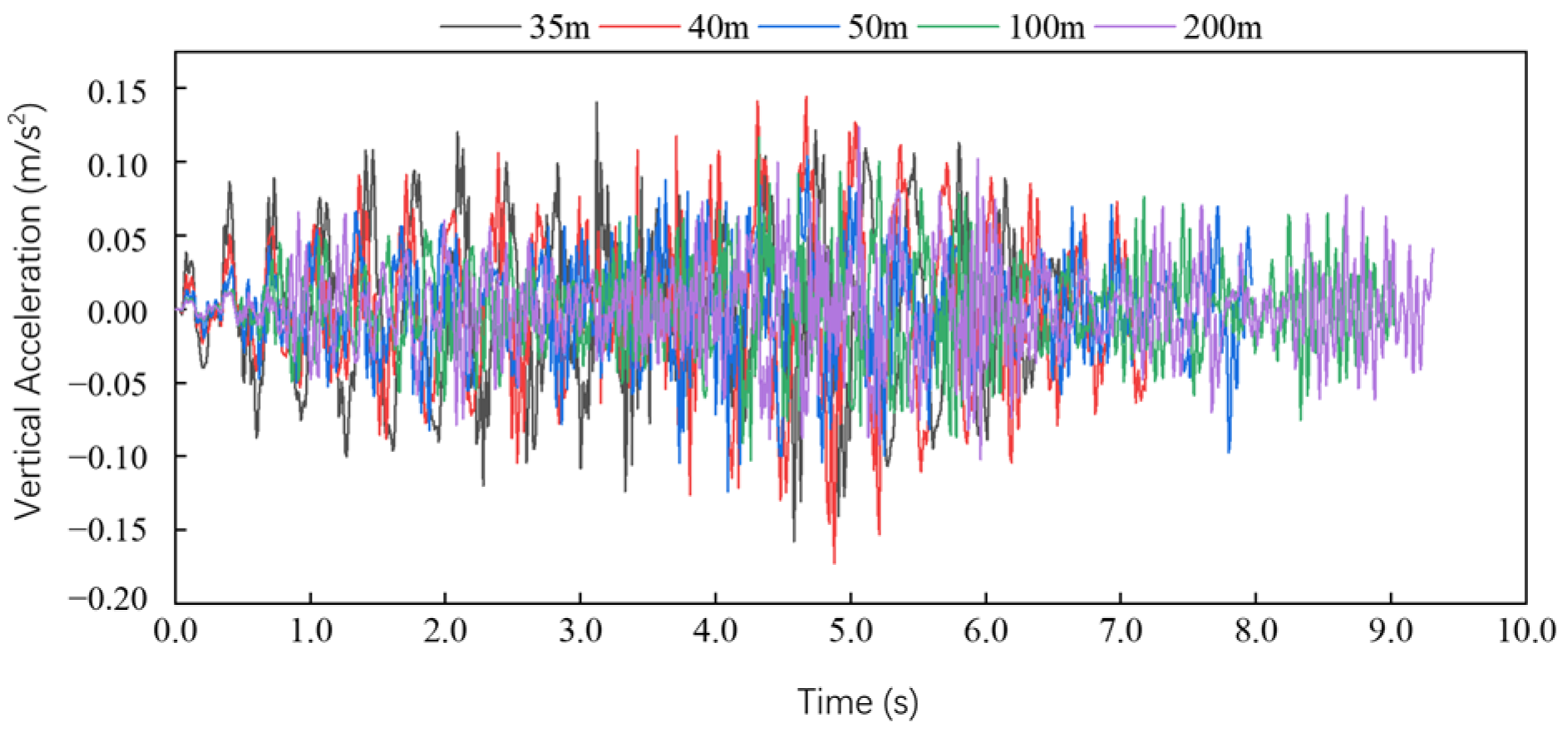
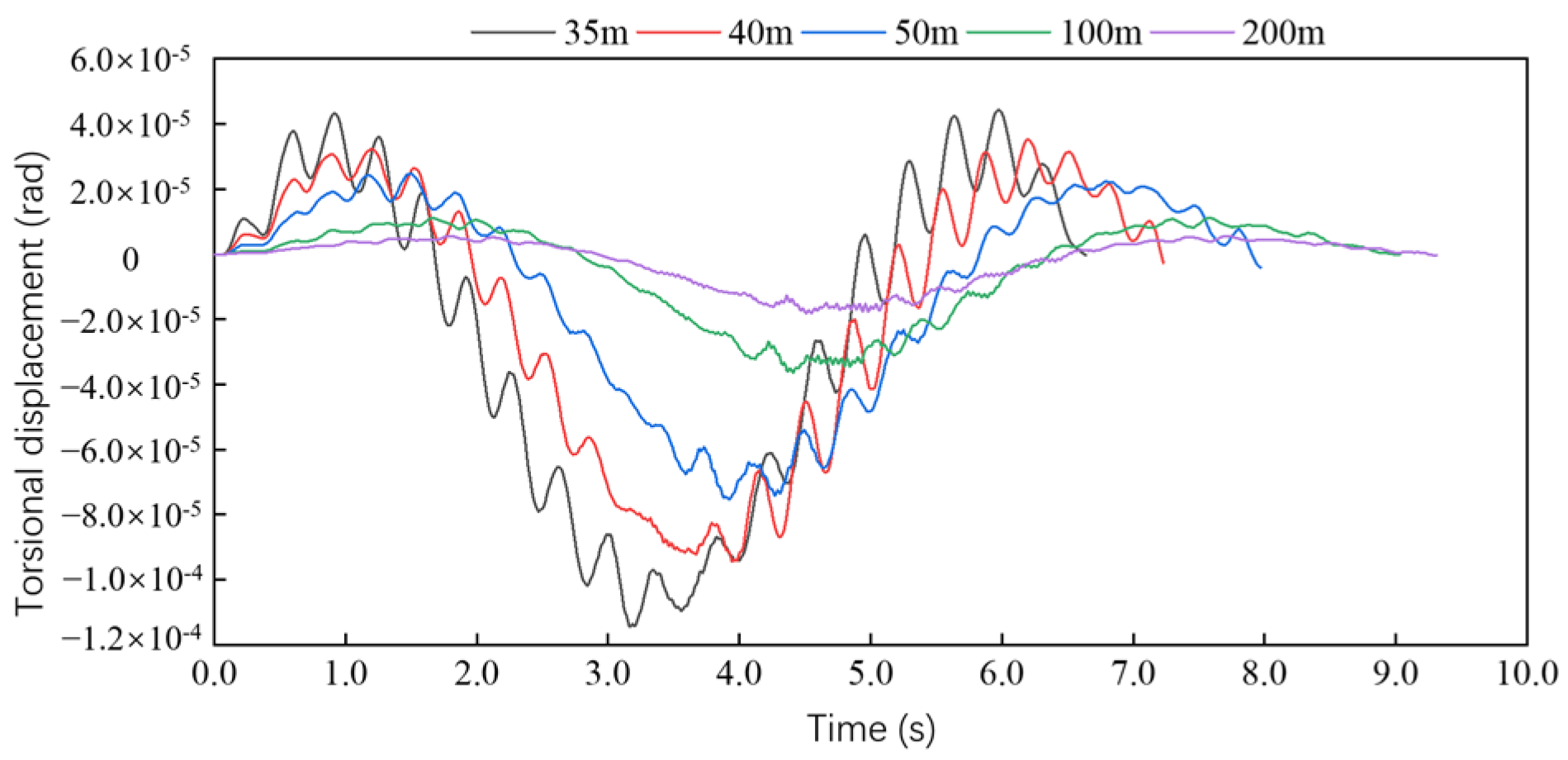
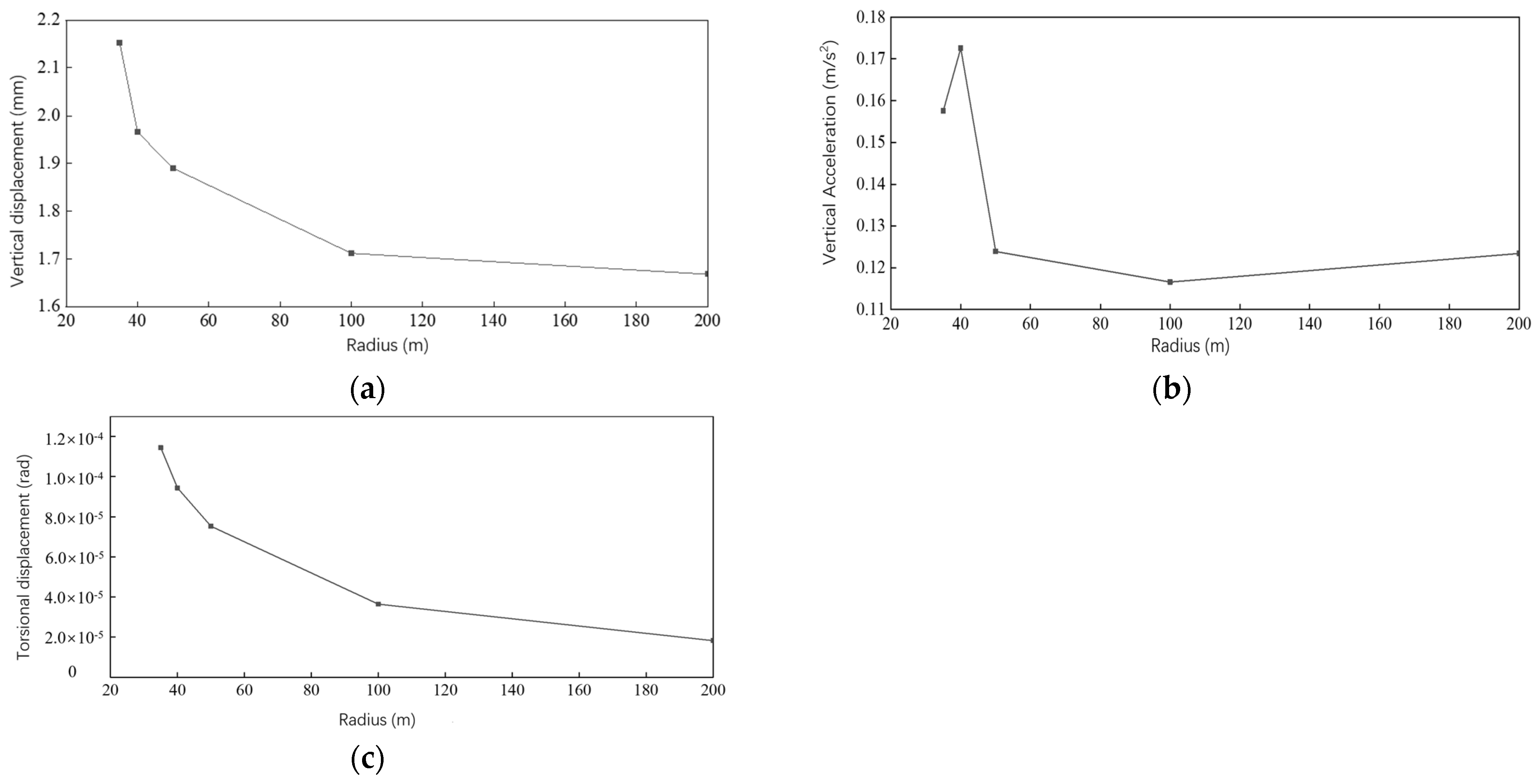
4.1. Impact Analysis of Curvature Radius
4.2. Analysis of the Influence of Constraint Methods
4.3. Analysis of the Impact of Vehicle Types
5. Conclusions
- (1)
- Through comparison with the results of general finite element program calculations, it was shown that the overall and separate methods in the program Cmck had good agreement with the results via general FEA software, indicating that the calculation program based on the two methods of overall and separate vehicle-bridge coupled vibration analysis can effectively analyze the vibration.
- (2)
- As the radius of curvature increased, the dynamic response of the bridge showed a gradual decreasing trend. The peak vertical displacement decreased by 22.3%, the peak vertical acceleration decreased by 28.9%, and the peak torsional angular displacement decreased by 83.9%. Within the radius of curvature less than 100m, the decrease in the dynamic response of the bridge was more pronounced; compared to the vertical dynamic response, the torsional response was more sensitive to the radius of curvature.
- (3)
- Different bridge constraints significantly affected bridges’ dynamic responses. Bridges’ vertical and torsional dynamic responses under rectangular coordinate constraints increased significantly compared to polar coordinates. As the axle weight of vehicles decreased, the mid-span vertical and torsional dynamic responses decreased while the lateral dynamic response gradually increased.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Zhou, L.; Liu, Z.W.; Yan, X.F.; Li, Q.F. Design Theory and Analysis Methods for Curved Girder Bridges; People’s Transportation Press: Beijing, China, 2018. [Google Scholar]
- Liu, S.; De Corte, W.; Ding, H.; Taerwe, L. A comparison of the mechanical properties of curved box girders with corrugated steel webs to curved box girders with concrete webs. Adv. Struct. Eng. 2022, 25, 2103–2120. [Google Scholar] [CrossRef]
- Zhu, L.; Wang, J.-J.; Li, M.-J.; Chen, C.; Wang, G.-M. Finite beam element with 22 DOF for curved composite box girders considering torsion, distortion, and biaxial slip. Arch. Civ. Mech. Eng. 2020, 20, 101. [Google Scholar] [CrossRef]
- Nadi, A.; Raghebi, M. Finite element model of circularly curved Timoshenko beam for in-plane vibration analysis. FME Trans. 2021, 49, 615–626. [Google Scholar] [CrossRef]
- Zhou, Y.; Zhang, K.; Miao, F.; Yang, P. Dynamic performance of straddle monorail curved girder bridge. Comput. Model. Eng. Sci. 2022, 130, 1669–1682. [Google Scholar] [CrossRef]
- Eshkevari, S.S.; Matarazzo, T.J.; Pakzad, S.N. Simplified vehicle-bridge interaction for medium to long-span bridges subject to random traffic load. J. Civ. Struct. Health Monit. 2020, 10, 693–707. [Google Scholar] [CrossRef]
- Liu, Y.; Yan, W.; Yin, X.; Wang, L.; Han, Y. Vibration analysis of the continuous beam bridge considering the action of jump impacting. Adv. Struct. Eng. 2022, 25, 2627–2640. [Google Scholar] [CrossRef]
- Gara, F.; Nicoletti, V.; Carbonari, S.; Ragni, L.; Dall’asta, A. Dynamic monitoring of bridges during static load tests: Influence of the dynamics of trucks on the modal parameters of the bridge. J. Civ. Struct. Health Monit. 2020, 10, 197–217. [Google Scholar] [CrossRef]
- Cantero, D.; Hester, D.; Brownjohn, J. Evolution of bridge frequencies and modes of vibration during truck passage. Eng. Struct. 2017, 152, 452–464. [Google Scholar] [CrossRef]
- Zakeri, J.A.; Feizi, M.M.; Shadfar, M.; Naeimi, M. Sensitivity analysis on dynamic response of railway vehicle and ride index over curved bridges. Proc. Inst. Mech. Eng. Part K J. Multi-Body Dyn. 2017, 231, 266–277. [Google Scholar] [CrossRef]
- Ma, Q.Q.; Zhang, J. Study on Vehicle-Bridge Coupled Vibration of Small-Radius-Curved Trough Girder Bridge. Bridge Constr. 2018, 48, 24–28. [Google Scholar]
- Guo, F.; Cai, H.; Li, H.F. Impact coefficient analysis on long-span beam bridge. J. Vibroeng. 2021, 23, 436–448. [Google Scholar] [CrossRef]
- Liu, S.; Yan, S.M.; Liu, C.J.; Wang, X. Analysis of Vehicle-Bridge Coupling Response of Curved CFST Composite Truss Bridge. Technol. Highw. Transp. 2023, 39, 89–98. [Google Scholar]
- Zhou, R.-J.; Wang, Y.; Chen, H. Analysis of vehicle-bridge coupled vibration of long-span curved truss bridge in service. J. Vib. Eng. 2022, 35, 103–112. [Google Scholar]
- Li, Z.; Li, Z.D.; Chen, D.H.; Xu, S.; Zhang, Y. Theoretical analysis and experimental study of vehicle-bridge coupled vibration for highway bridges. Arch. Appl. Mech. 2023, 94, 21–37. [Google Scholar] [CrossRef]
- Shi, Y.; Fang, S.T.; Tian, Q.Y.; Chen, W. Spatial analysis of vehicle-bridge coupled vibration response between curved continuous rigid-frame bridge and motor vehicle. Appl. Mech. Mater. 2012, 1802, 2456–2461. [Google Scholar] [CrossRef]
- Guo, F.; Cai, H.; Li, H.F. Impact coefficient analysis of curved box girder bridge based on vehicle-bridge coupling. Math. Probl. Eng. 2022, 2022, 8628479. [Google Scholar] [CrossRef]
- Cao, H.T.; Chen, D.H.; Zhang, Y.S.; Wang, H.; Chen, H. Finite Element Analysis of Curved Beam Elements Employing Trigonometric Displacement Distribution Patterns. Buildings 2023, 13, 2239. [Google Scholar] [CrossRef]
- Nallasivam, K.; Dutta, A.; Talukdar, S. Dynamic analysis of horizontally curved thin-walled box-girder bridge due to moving vehicle. Shock. Vib. 2007, 14, 65–84. [Google Scholar] [CrossRef]
- Zhang, X.; Chen, E.L.; Li, L.Y.; Si, C. Development of the dynamic response of curved bridge deck pavement under vehicle-bridge interactions. Int. J. Struct. Stab. Dyn. 2022, 22, 1720–1735. [Google Scholar] [CrossRef]
- Zeng, Q.Y.; Guo, X.R. Theory and Application of Vibration Analysis for Time-Varying System of Train-Bridge; China Railway Press: Beijing, China, 1999. [Google Scholar]
- Luo, X.T. Dynamic Response Analysis of a Long Span Cable-Stayed Bridge Subjected to Automobiles. Master’s Thesis, Beijing Jiaotong University, Beijing, China, 2018. [Google Scholar]
- Zong, Z.H.; Xue, C.; Yang, Z.G. Vehicle load model for highway bridges in Jiangsu province based on WIM. J. Southeast Univ. 2020, 50, 143–152. [Google Scholar]
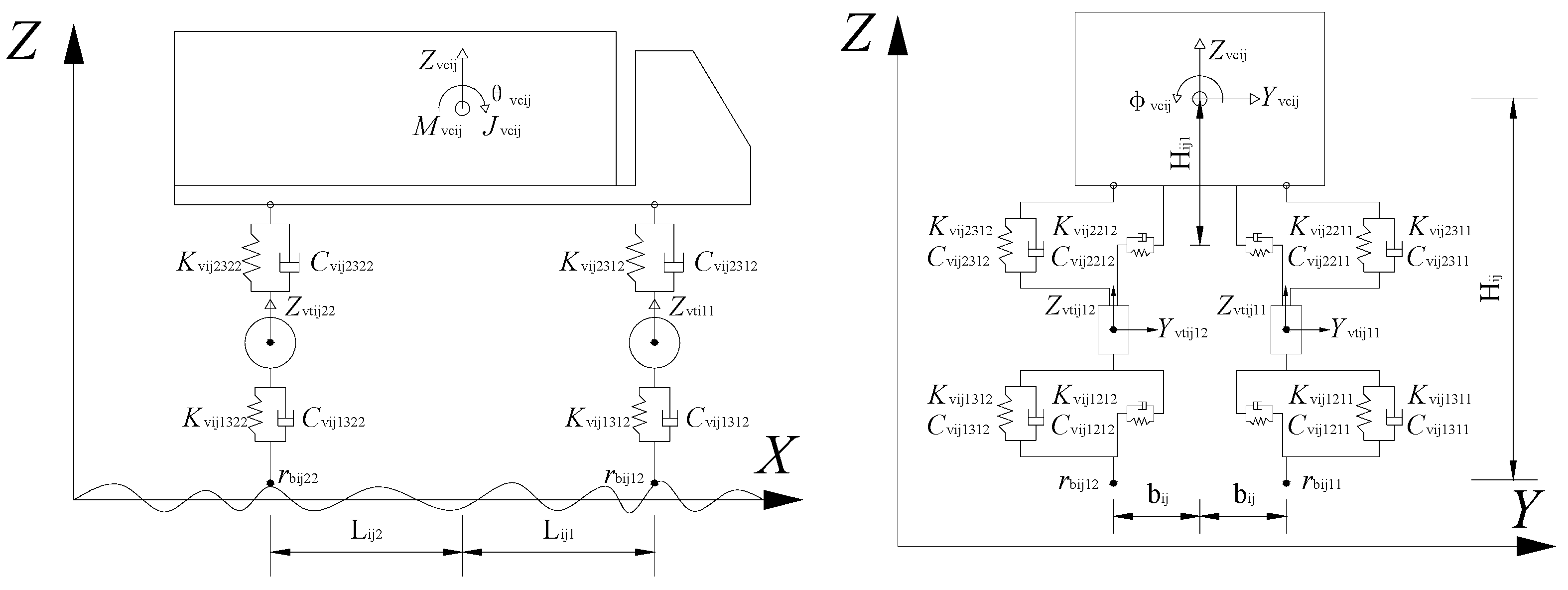





| Parameter | Description |
|---|---|
| The rigid mass of the vehicle body of the j car on the i lane; The vertical rotational inertia of the vehicle body of the j car on the i lane. | |
| The lateral inertia of the vehicle body; The longitudinal rotational inertia of the vehicle body. | |
| The lateral stiffness coefficient of secondary suspension. | |
| The vertical stiffness coefficient of secondary suspension. | |
| The lateral damping constant of each wheel. | |
| The vertical damping constant of each wheel. | |
| The vehicle’s unsprung mass. | |
| The lateral stiffness coefficient of the vehicle’s wheel. | |
| The vertical stiffness coefficient of the vehicle’s wheel. | |
| The lateral damping constant of the vehicle’s wheel. | |
| The vertical damping constant of the vehicle’s wheel. | |
| The longitudinal distance from the vehicle’s first and second axles to the center of gravity of the car body. | |
| The distance from the vehicle body to the secondary suspension. The distance from the rigid body of the vehicle to the ground. |
| Radius (m) | Vertical Displacement (mm) | Vertical Acceleration (m/s2) | Torsional Displacement (rad) |
|---|---|---|---|
| 35 | 2.153 | 0.158 | 0.114 × 10−3 |
| 40 | 1.966 | 0.173 | 0.944 × 10−4 |
| 50 | 1.890 | 0.124 | 0.753 × 10−4 |
| 100 | 1.712 | 0.117 | 0.364 × 10−4 |
| 200 | 1.668 | 0.123 | 0.183 × 10−4 |
| Constraints Arrangement | Vertical Displacement (mm) | Vertical Acceleration (m/s2) | Torsional Displacement (rad) |
|---|---|---|---|
| Polar coordinate | 1.890 | 0.124 | 0.753 × 10−4 |
| Rectangular coordinate | 5.756 | 0.363 | 0.113 × 10−3 |
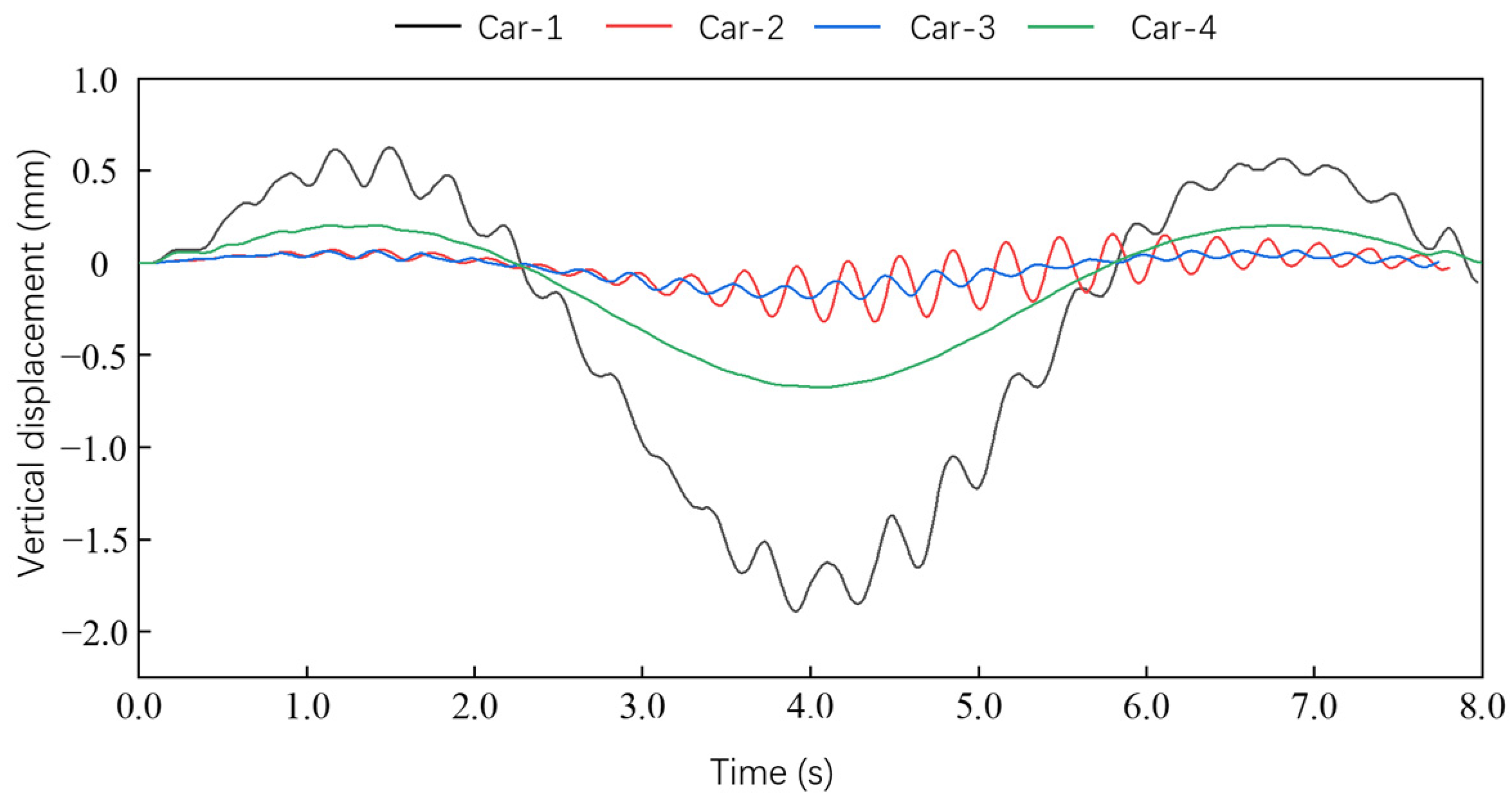
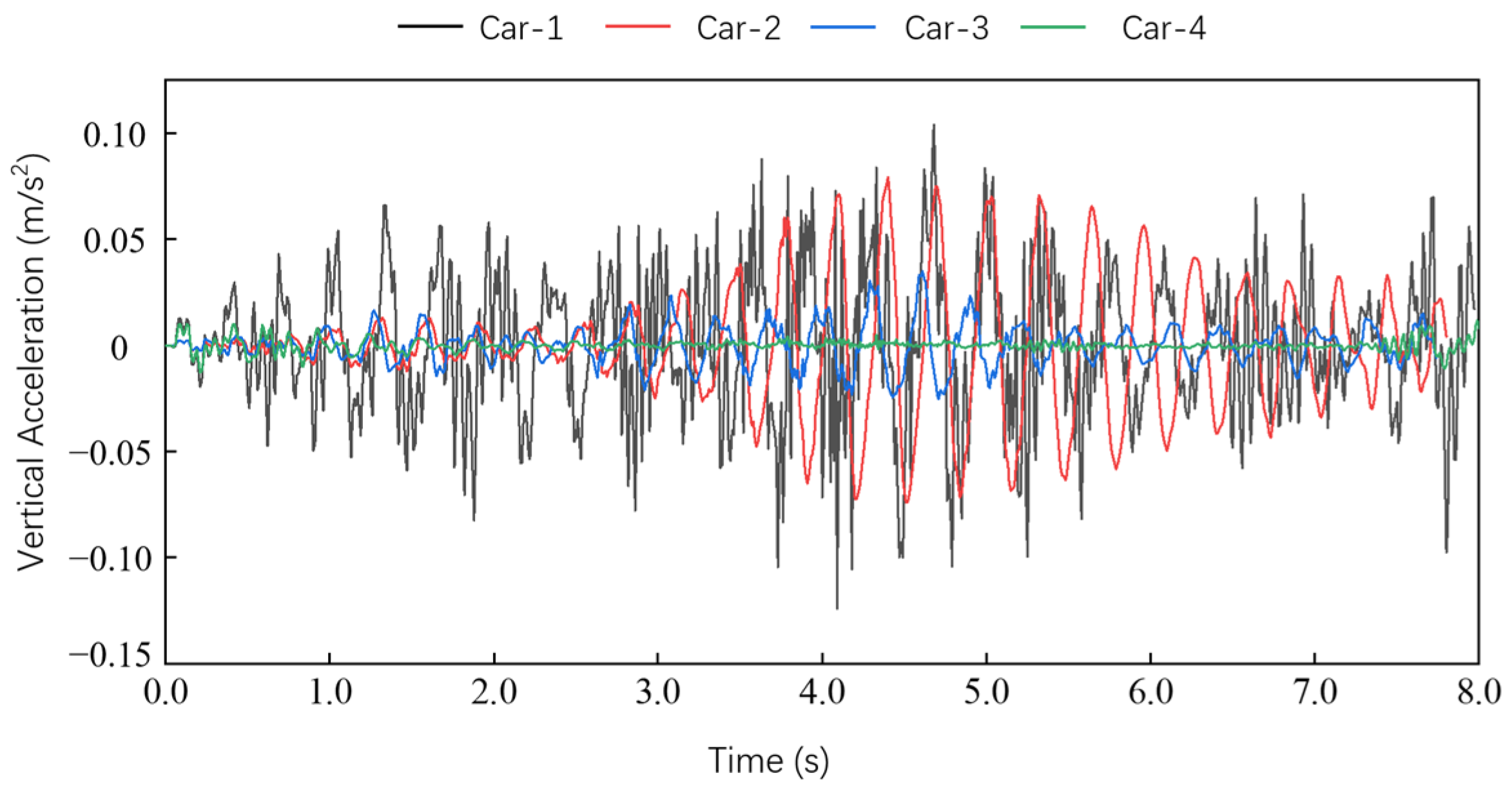
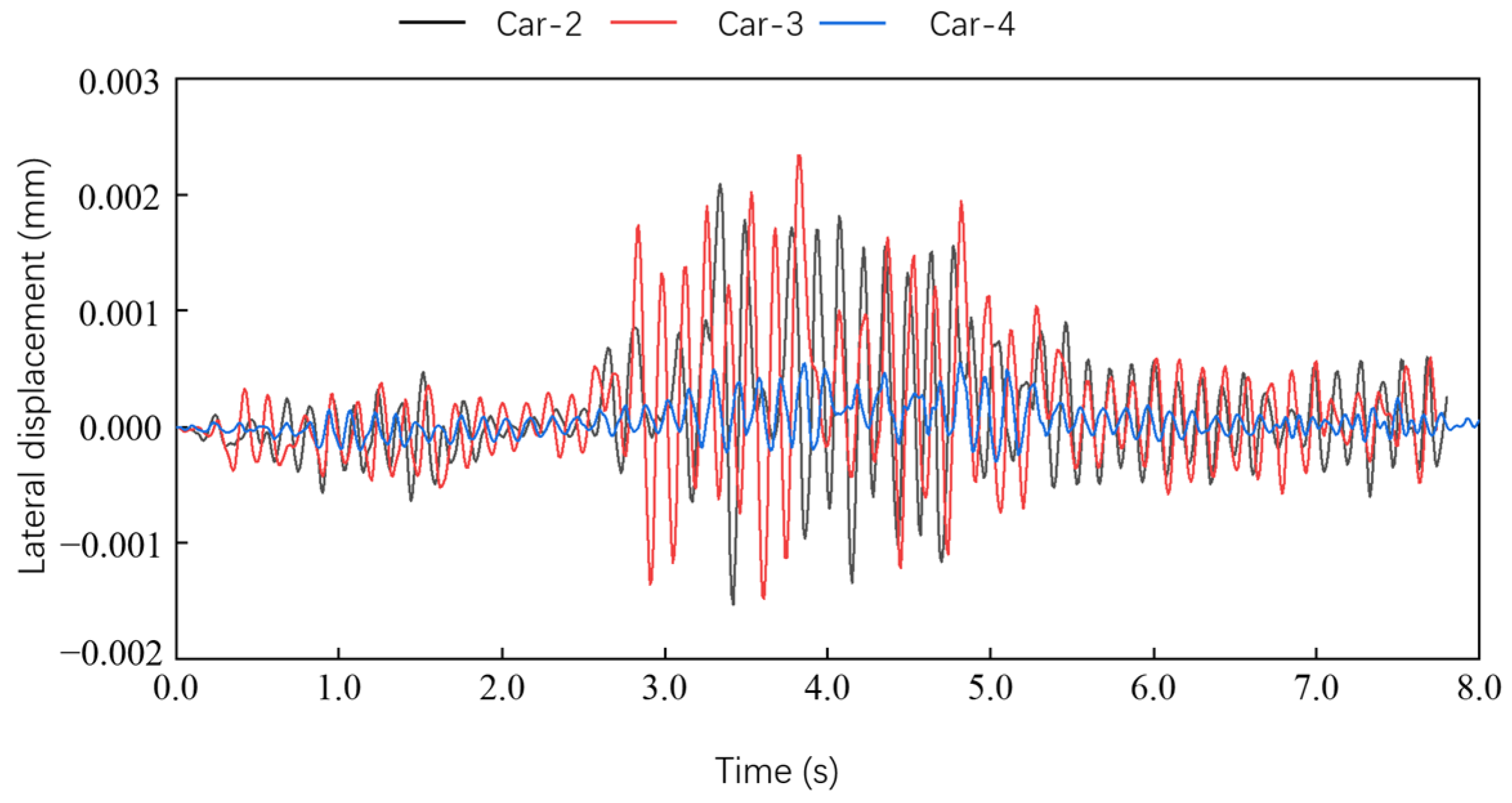
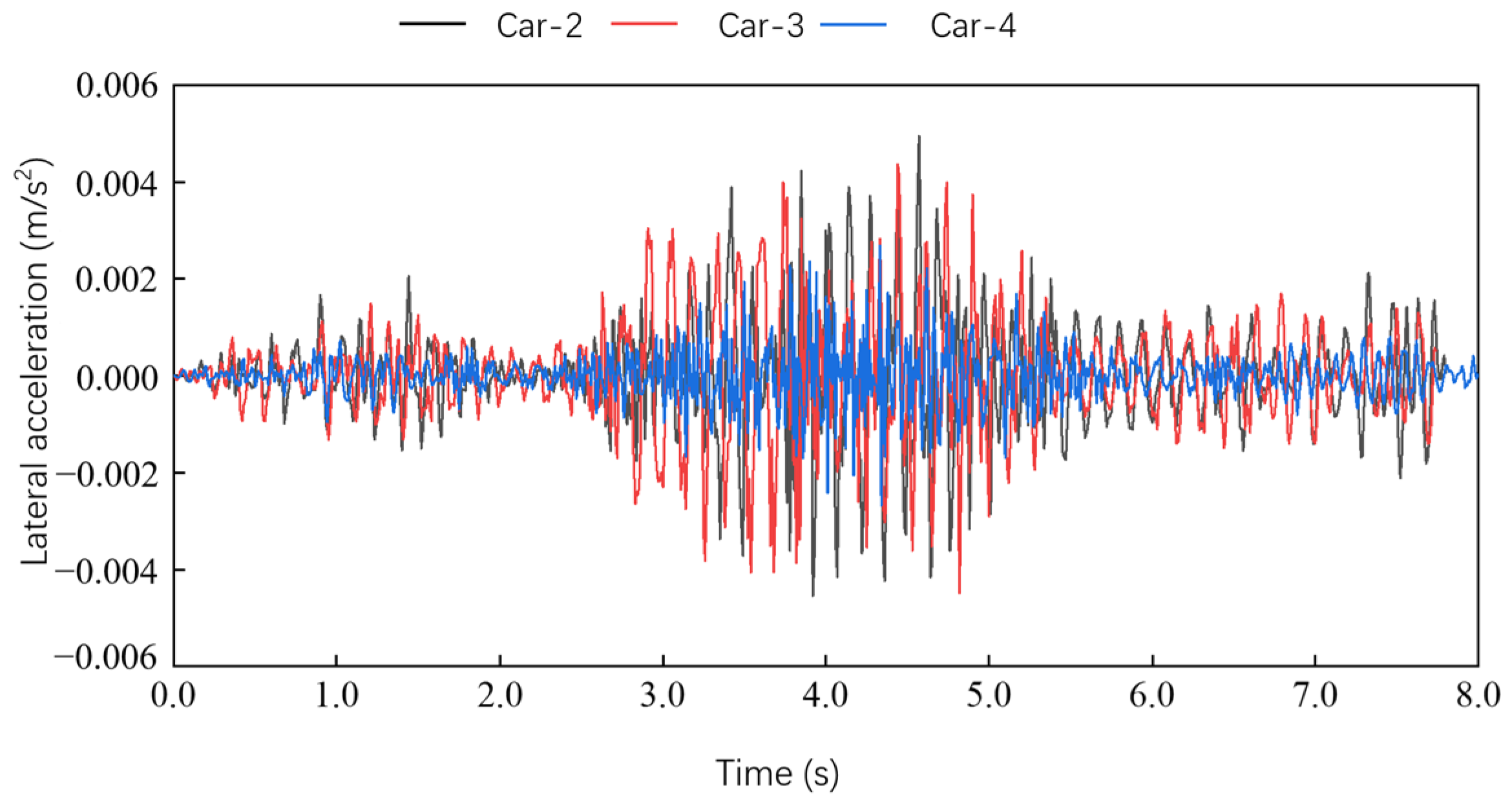
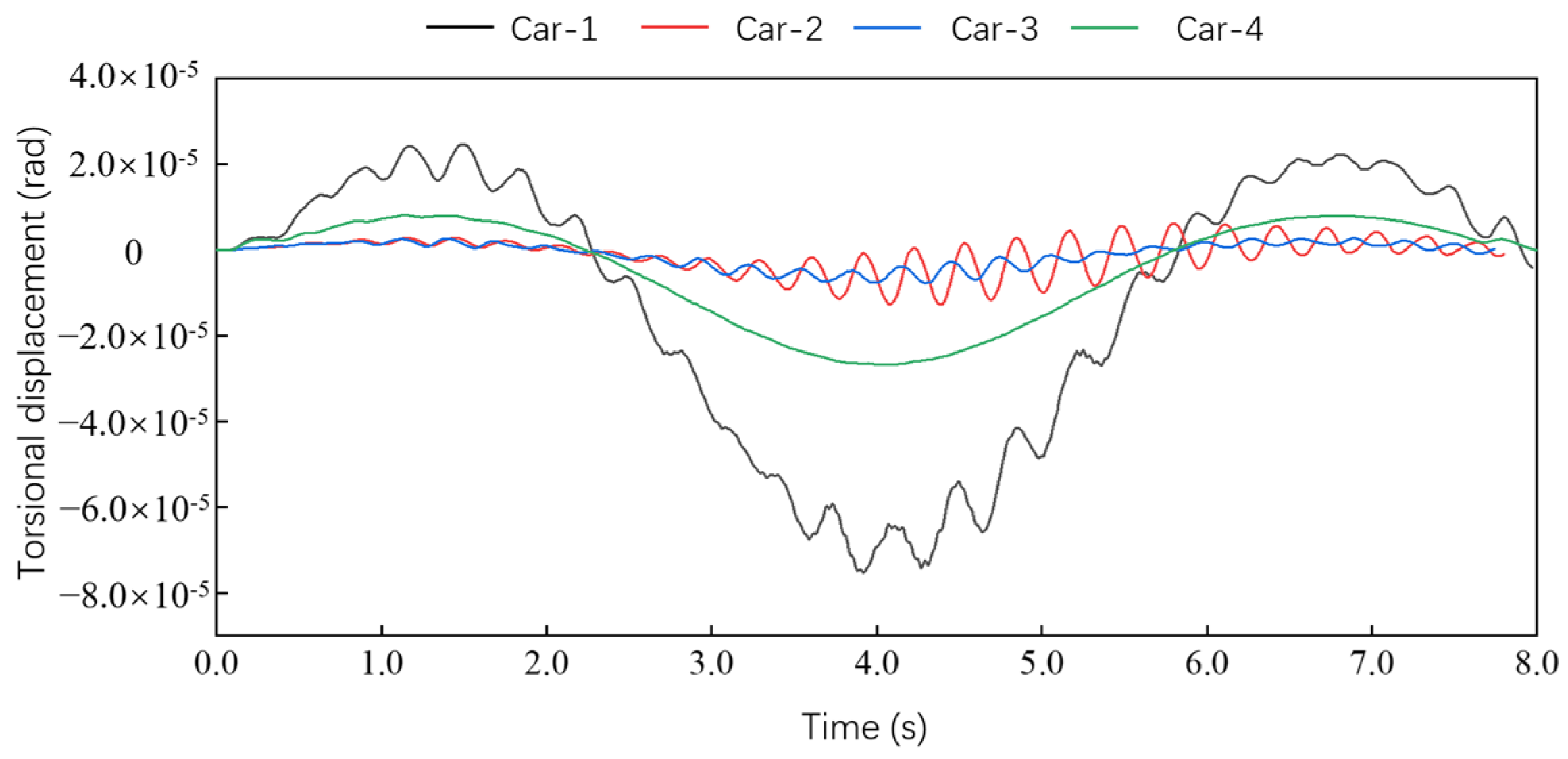
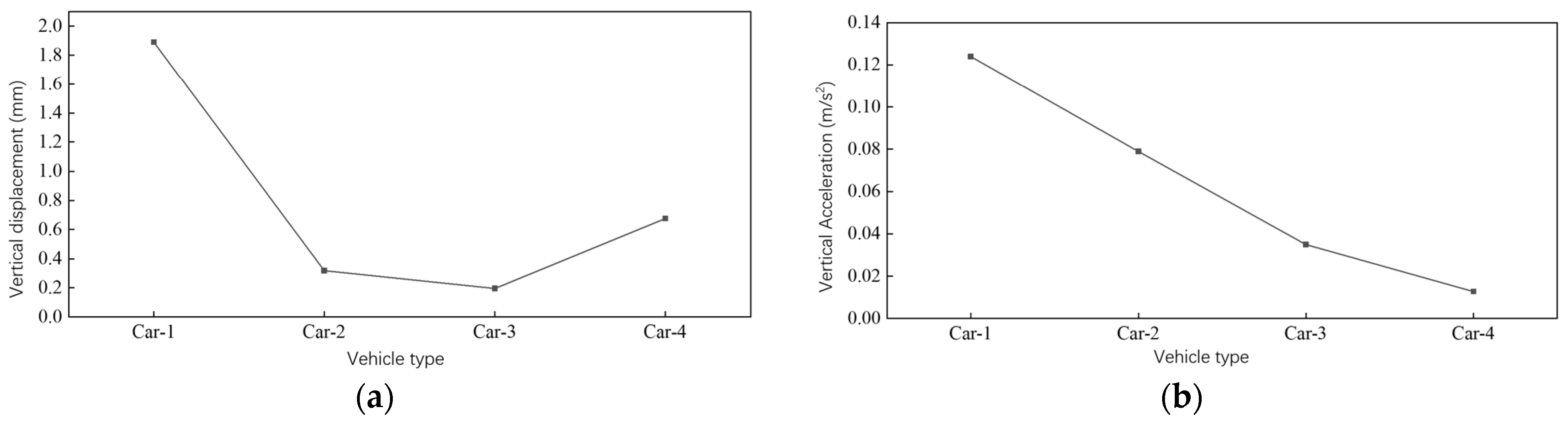
| Vehicle Type | Vertical Displacement (mm) | Vertical Acceleration (m/s2) | Lateral Displacement (mm) | Lateral Acceleration (m/s2) | Torsional Displacement (rad) |
|---|---|---|---|---|---|
| Car-1 | 1.890 | 0.124 | -- | -- | 0.753 × 10−4 |
| Car-2 | 0.318 | 0.079 | 3.449 | 0.747 | 0.128 × 10−4 |
| Car-3 | 0.196 | 0.035 | 5.898 | 1.017 | 0.774 × 10−5 |
| Car-4 | 0.675 | 0.013 | 1.913` | 0.034 | 0.269 × 10−4 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Cao, H.; Lu, Y.; Chen, D. Analysis of Vehicle-Bridge Coupling Vibration Characteristics of Curved Girder Bridges. Appl. Sci. 2024, 14, 2021. https://doi.org/10.3390/app14052021
Cao H, Lu Y, Chen D. Analysis of Vehicle-Bridge Coupling Vibration Characteristics of Curved Girder Bridges. Applied Sciences. 2024; 14(5):2021. https://doi.org/10.3390/app14052021
Chicago/Turabian StyleCao, Hengtao, Yao Lu, and Daihai Chen. 2024. "Analysis of Vehicle-Bridge Coupling Vibration Characteristics of Curved Girder Bridges" Applied Sciences 14, no. 5: 2021. https://doi.org/10.3390/app14052021
APA StyleCao, H., Lu, Y., & Chen, D. (2024). Analysis of Vehicle-Bridge Coupling Vibration Characteristics of Curved Girder Bridges. Applied Sciences, 14(5), 2021. https://doi.org/10.3390/app14052021





