A Two-Time-Scale Turbulence Model and Its Application in Free Shear Flows
Abstract
1. Introduction
2. The WFC Model of Turbulence
3. The k–ε–τ Model of Turbulence
- The production and destruction terms of in Equation (6) cannot balance each other properly with two different time scales (i.e., in the production term of and in the other), and this imbalance causes the model to become instable and overshoot the turbulence viscosity in shear flows, particularly in plane and round jets. This, therefore, necessitated the use of the same time scale in both terms in the -equation for shear flows.
- The -equation in Equation (7), as it is, cannot account for shear flows, as was also warned by Wu et al. [5]. Hence, a production related term associated with the constant has been added to the -equation, and its coefficient has been tuned to give the best result for free shear flows, provided that coefficients satisfy the relations derived below.
3.1. Grid Turbulence Decay
3.2. Return to Equilibrium
3.3. Constraint for the Coefficient of Mean Strain Term
3.4. Local Equilibrium
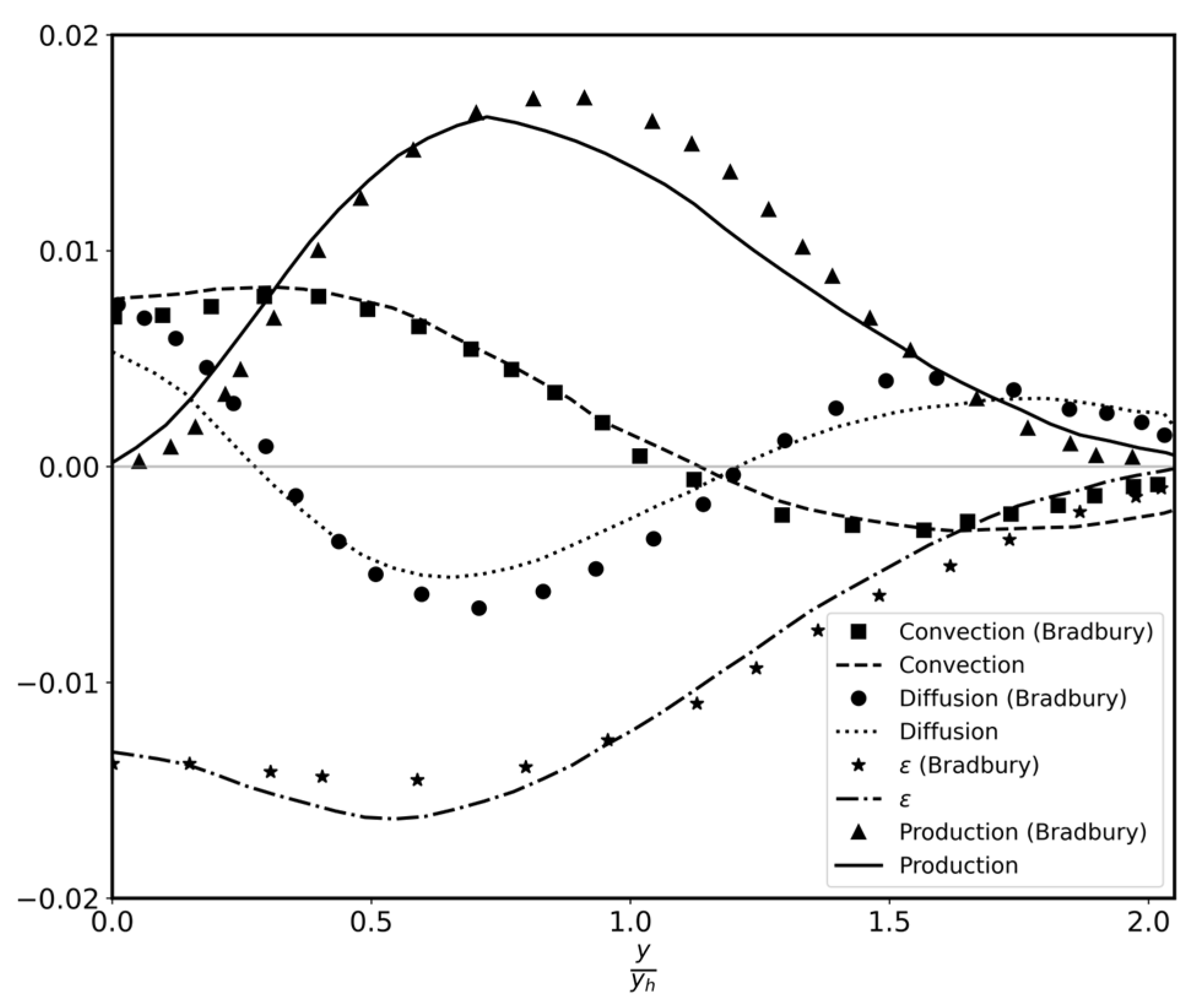
4. Model Validation
- U-momentum
- Continuity
- Scalar entity
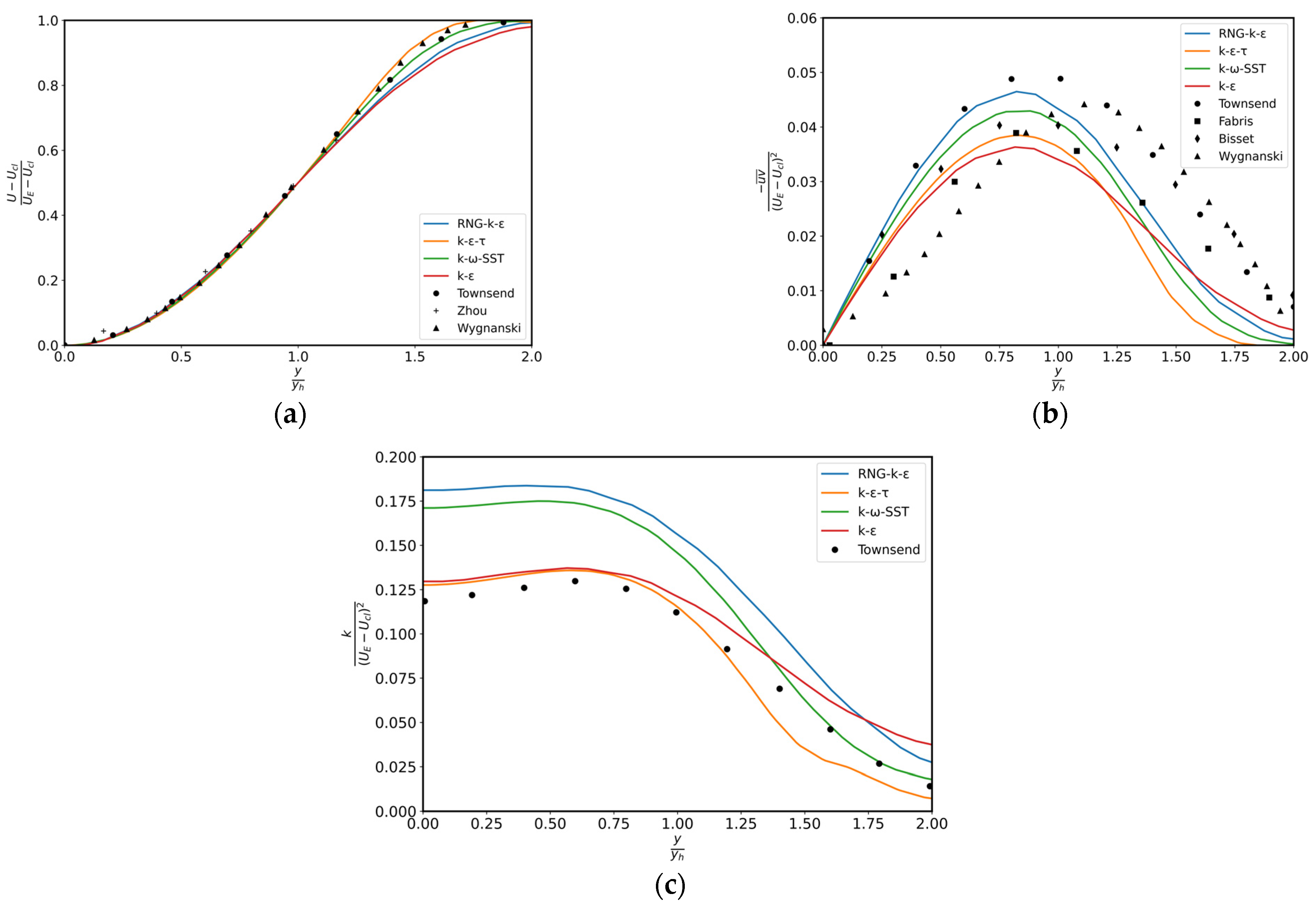
4.1. Plane Jet Results
4.2. Round Jet Results
| Investigator | Spreading Rate | Remarks | |
|---|---|---|---|
| Hussein and George [45] | 0.094 | 0.021 | moving HW |
| Wygnanski and Fiedler [48] | 0.086 | 0.0165 | HWA |
| Rodi [49] | 0.086 | 0.0186 | HWA |
| Capp [50] | 0.095 | - | LDA |
| Panchapakesan and Lumley [51] | 0.096 | 0.021 | moving HW |
| Taulbee et al. [52] | 0.094–0.102 | 0.021 | LDA-HWA |
| model | 0.120 | 0.025 | |
| model | 0.121 | 0.028 | |
| model | 0.088 | 0.023 | |
| model | 0.089 | 0.027 |
4.3. Plane Far Wake Results
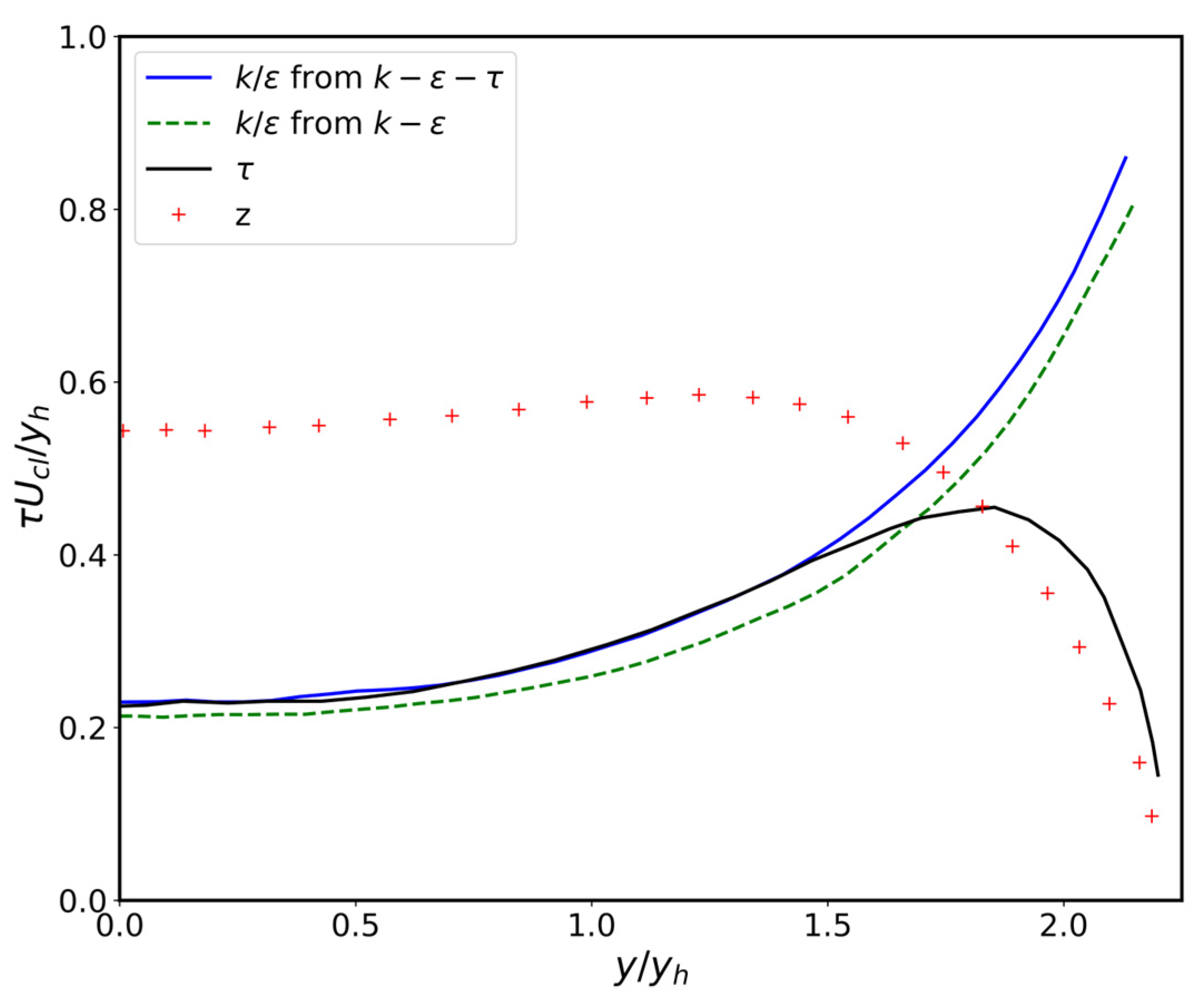
4.4. Turbulence Viscosity Relation
5. Conclusions
- Consistent with the measurements, the three-equation model (utilizing identical parameters across all three cases) estimates a spread rate of 0.109 for the plane jet;
- The model estimates the round jet spreading rate of 0.089, which is over 23% better than the k–ε and SST k–ω models and consistent with the experimental data;
- The parameter for the spreading of the plane wake is estimated to be 0.081, which is approximately 6% more accurate than the k–ε model and 4% more accurate than the SST k–ω model;
- Time scales and behave quite similar to each other in most parts of the jet, as expected (in non-equilibrium situations, as in the compression stroke of an IC engine, these two will differ considerably);
- For the turbulence viscosity, several options, such as the geometric and arithmetic averages with a weighting factor, were investigated and shown to have no significant advantage over the traditional one for the types of flows tested.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Argyropoulos, C.D.; Markatos, N.C. Recent Advances on the Numerical Modelling of Turbulent Flows. Appl. Math. Model 2015, 39, 693–732. [Google Scholar] [CrossRef]
- Klein, T.S.; Craft, T.J.; Iacovides, H. The Development and Application of Two-Time-Scale Turbulence Models for Non-Equilibrium Flows. Int. J. Heat Fluid Flow 2018, 71, 334–352. [Google Scholar] [CrossRef]
- Klein, T.S.; Craft, T.J.; Iacovides, H. Assessment of the Performance of Different Classes of Turbulence Models in a Wide Range of Non-Equilibrium Flows. Int. J. Heat Fluid Flow 2015, 51, 229–256. [Google Scholar] [CrossRef]
- Nie, X.; Chen, Z.; Zhu, Z. Assessment of Low-Reynolds Number k-ε Models in Prediction of a Transitional Flow with Coanda Effect. Appl. Sci. 2023, 13, 1783. [Google Scholar] [CrossRef]
- Wu, C.-T.; Ferziger, J.-H.; Chapman, D.-R. Simulation and Modeling of Homogeneous, Compressed Turbulence. In Proceedings of the 5th Symposium on Turbulent Shear Flows, Ithaca, NY, USA, 7–9 August 1985; pp. 17.13–17.19. [Google Scholar]
- Le Penven, L.; Serre, G. A Generalized κ-ε Model for Compressed Turbulence. In Proceedings of the Eurotherm 15, Toulouse, France, 4–6 December 1991. [Google Scholar]
- Hamlington, P.E.; Ihme, M. Modeling of Non-Equilibrium Homogeneous Turbulence in Rapidly Compressed Flows. Flow Turbul. Combust. 2014, 93, 93–124. [Google Scholar] [CrossRef]
- Kim, S.W.; Chen, C.P. A Multiple-Time-Scale Turbulence Model Based on Variable Partitioning of the Turbulent Kinetic Energy Spectrum. Numer. Heat Transf. Part B Fundam. 1990, 16, 193–211. [Google Scholar] [CrossRef]
- Hanjalic, K.; Launder, B.; Schiestel, R. Multiple-Time-Scale Concepts in Turbulent Transport Modeling. In Proceedings of the Turbulent Shear Flows 2, London, UK, 4 July 1980; Springer: Berlin/Heidelberg, Germany, 1980; pp. 36–49. [Google Scholar]
- Chitta, V.; Dhakal, T.P.; Walters, D.K. Development and Application of a New Four-Equation Eddy-Viscosity Model for Flows With Transition, Curvature and Rotation Effects. In Proceedings of the Fluids Engineering Division Summer Meeting, Incline Village, NV, USA, 7 July 2013; ASME: New York, NY, USA, 2013; pp. 1–10. [Google Scholar]
- Grunloh, T.P. Four Equation K-Omega Based Turbulence Model with Algebraic Flux for Supercritical Flows. Ann. Nucl. Energy 2019, 123, 210–221. [Google Scholar] [CrossRef]
- Zeierman, S.; Wolfshtein, M. Turbulent Time Scale for Turbulent-Flow Calculations. AIAA J. 1986, 24, 1606–1610. [Google Scholar] [CrossRef]
- Catris, S.; Aupoix, B. Towards a Calibration of the Length-Scale Equation. Int. J. Heat Fluid Flow 2000, 21, 606–613. [Google Scholar] [CrossRef]
- Chen, C.J.; Singh, K. Development of a Two-Scale Turbulence Model and Prediction of Buoyant Shear Flows. In Proceedings of the AIAA/ASME Thermophsics and Heat Transfer Conference, Seattle, WA, USA, 16–18 June 1990; HTD Publication: Washington, DC, USA, 1990. [Google Scholar]
- Jaw, S.Y.; Hwang, R.R. A Two-Scale Low-Reynolds Number Turbulence Model. Int. J. Numer. Methods Fluids 2000, 33, 695–710. [Google Scholar] [CrossRef]
- Morgan, B.E.; Schilling, O.; Hartland, T.A. Two-Length-Scale Turbulence Model for Self-Similar Buoyancy-, Shock-, and Shear-Driven Mixing. Phys. Rev. E 2018, 97, 013104. [Google Scholar] [CrossRef]
- Lumley, J.L. Some Comments on Turbulence. Phys. Fluids A 1992, 4, 203–211. [Google Scholar] [CrossRef]
- Goldberg, U.C. Exploring a Three-Equation r-k-ϵ Turbulence Model. J. Fluids Eng. Trans. ASME 1996, 118, 795–799. [Google Scholar] [CrossRef]
- Baldwin, B.S.; Barth, T.J. A One-Equation Turbulence Transport Model for High Reynolds Number Wall-Bounded Flow; Ames Research Center: Moffett Field, CA, USA, 1990. [Google Scholar]
- Cotton, M.A.; Ismael, J.O. A Strain Parameter Turbulence Model and Its Application to Homogeneous and Thin Shear Flows. Int. J. Heat Fluid Flow 1998, 19, 326–337. [Google Scholar] [CrossRef]
- Billard, F.; Laurence, D. A Robust K-ε-V2−/k Elliptic Blending Turbulence Model Applied to near-Wall, Separated and Buoyant Flows. Int. J. Heat Fluid Flow 2012, 33, 45–58. [Google Scholar] [CrossRef]
- Wilcox, D.C. Multiscale Model for Turbulent Flows. AIAA J. 1988, 26, 1311–1320. [Google Scholar] [CrossRef]
- Duranti, S.; Pittaluga, F. Navier-Stokes Prediction of Internal Flows with a Three-Equation Turbulence Model. AIAA J. 2000, 38, 1098–1100. [Google Scholar] [CrossRef]
- Chen, C.P.; Guo, K. A Non-Isotropic Multiple-Scale Turbulence Model. Appl. Math. Mech. 1991, 12, 981–991. [Google Scholar] [CrossRef]
- Nagano, Y.; Hattori, H. Improvement of an LRN Two-Equation Turbulence Model Reflecting Multi-Time Scales. Int. J. Heat Fluid Flow 2015, 51, 221–228. [Google Scholar] [CrossRef]
- Ertesvag, I.S.; Byggstoyl, S.; Magnussen, B.F. An “Eddy-Dissipation” Reynolds-Stress Turbulence Model Closed by an Equation Related to the Turbulent Transport Timescale. In Proceedings of the 7th Symposium on Turbulent Shear Flows, Stanford, CA, USA, 23 August 1989; PSU: State College, PA, USA, 1989; Volume 2, pp. 17.3.1–17.3.6. [Google Scholar]
- Ma, T.; Lucas, D.; Jakirlić, S.; Fröhlich, J. Progress in the Second-Moment Closure for Bubbly Flow Based on Direct Numerical Simulation Data. J. Fluid Mech. 2020, 883, A9. [Google Scholar] [CrossRef]
- Lopez, M.; Walters, D.K. Prediction of Transitional and Fully Turbulent Flow Using an Alternative to the Laminar Kinetic Energy Approach. J. Turbul. 2016, 17, 253–273. [Google Scholar] [CrossRef]
- Launder, B.E.; Spalding, D.B. The Numerical Computation of Turbulent Flows. Comput. Methods Appl. Mech. Eng. 1974, 3, 269–289. [Google Scholar] [CrossRef]
- Yakhot, V.; Orszag, S.A.; Thangam, S.; Gatski, T.B.; Speziale, C.G. Development of Turbulence Models for Shear Flows by a Double Expansion Technique. Phys. Fluids A 1992, 4, 1510–1520. [Google Scholar] [CrossRef]
- Menter, F.R. Two-Equation Eddy-Viscosity Turbulence Models for Engineering Applications. AIAA J. 1994, 32, 1598–1605. [Google Scholar] [CrossRef]
- Shih, T.-H.; Liou, W.W.; Shabbir, A.; Yang, Z.; Zhu, J. A New K-ϵ Eddy Viscosity Model for High Reynolds Number Turbulent Flows. Comput. Fluids 1995, 24, 227–238. [Google Scholar] [CrossRef]
- Moukalled, F.; Mangani, L.; Darwish, M. The Finite Volume Method in Computational Fluid Dynamics; Fluid Mechanics and Its Applications; Springer International Publishing: Cham, Switzerland, 2016; Volume 113, ISBN 978-3-319-16873-9. [Google Scholar]
- Bailly, C.; Comte-Bellot, G. Turbulence; Experimental Fluid Mechanics; Springer International Publishing: Cham, Switzerland, 2015; ISBN 978-3-319-16159-4. [Google Scholar]
- Bradbury, L.J.S. The Structure of a Self-Preserving Turbulent Plane Jet. J. Fluid Mech. 1965, 23, 31–64. [Google Scholar] [CrossRef]
- Gutmark, E.; Wygnanski, I. The Planar Turbulent Jet. J. Fluid Mech. 1976, 73, 465–495. [Google Scholar] [CrossRef]
- Miller, D.R.; Comings, E.W. Static Pressure Distribution in the Free Turbulent Jet. J. Fluid Mech. 1957, 3, 985. [Google Scholar] [CrossRef]
- Robins, A. The Structure and Development of a Plane Turbulent Free Jet. Ph.D. Thesis, University of London, London, UK, 1973. [Google Scholar]
- Van der Hegge Zijnen, B.G. Measurements of the Velocity Distribution in a Plane Turbulent Jet of Air. Appl. Sci. Res. Sect. A 1958, 7, 256–276. [Google Scholar] [CrossRef]
- Heskestad, G. Hot-Wire Measurements in a Plane Turbulent Jet. J. Appl. Mech. Trans. ASME 1964, 32, 721–734. [Google Scholar] [CrossRef]
- Salerno, S. RANS and LES Simulations of a Turbulent Plane Jet. Master’s Thesis, Politecnico di Milano, Milan, Italy, 2018. [Google Scholar]
- Ramaprian, B.R.; Chandrasekhara, M.S. LDA Measurements in Plane Turbulent Jets. J. Fluids Eng. Trans. ASME 1985, 107, 264–271. [Google Scholar] [CrossRef]
- Everitt, K.W.; Robins, A.G. The Development and Structure of Turbulent Plane Jets. J. Fluid Mech. 1978, 88, 563–583. [Google Scholar] [CrossRef]
- Rodi, W. A Review of Experimental Data of Uniform Density Free Turbulent Boundary Layers; Launder, B.E., Ed.; Academic Press: Cambridge, MA, USA, 1975. [Google Scholar]
- Hussein, H.J.; George, W.K. Measurement of Small Scale Turbulence in an Axisymmetric Jet Using Moving Hot-Wires. In Proceedings of the 7th Symposium on Turbulent Shear Flows, Stanford, CA, USA, 23 August 1989; PSU: State College, PA, USA, 1989; Volume 2, pp. 30.2.1–30.2.6. [Google Scholar]
- Hussein, H.J.; Capp, S.P.; George, W.K. Velocity Measurements in a High-Reynolds-Number, Momentum-Conserving, Axisymmetric, Turbulent Jet. J. Fluid Mech. 1994, 258, 31–75. [Google Scholar] [CrossRef]
- Rodi, W.; Spalding, D.B. A Two-Parameter Model of Turbulence, and Its Application to Free Jets. Wärme Stoffübertrag. 1970, 3, 85–95. [Google Scholar] [CrossRef]
- Wygnanski, I.; Fiedler, H. Some Measurements in the Self-Preserving Jet. J. Fluid Mech. 1969, 38, 577–612. [Google Scholar] [CrossRef]
- Rodi, W. The Prediction of Free Turbulent Boundary Layers by Use of a Two-Equation Model of Turbulence. Ph.D. Thesis, University of London, London, UK, 1972. [Google Scholar]
- Capp, S.P. Experimental Investigation of the Turbulent Axisymmetric Jet. Ph.D. Thesis, State University of New York, New York, NY, USA, 1983. [Google Scholar]
- Panchapakesan, N.; Lumley, J.L. Turbulence measurements in axisymmetric jets of air and helium. Part 1. Air jet. J. Fluid Mech. 1993, 246, 197–223. [Google Scholar] [CrossRef]
- Taulbee, D.B.; Hussein, H.; Capp, S. Round Jet—Experiment and Inferences on Turbulence Modeling. In Proceedings of the 6th Symposium on Turbulent Shear Flows, Toulouse, France, 7–9 September 1987; pp. 10-5-1–10-5-6. [Google Scholar]
- Newman, B.G. Turbulent Jets and Wakes in a Pressure Gradient; DDC: Virginia Beach, VA, USA, 1967. [Google Scholar]
- Zhou, Y.; Antonia, R.A.; Tsang, W.K. The Effect of Reynolds Number on a Turbulent Far-Wake. Exp. Fluids 1998, 25, 118–125. [Google Scholar] [CrossRef]
- Wygnanski, I.; Champagne, F.; Marasli, B. On the Large-Scale Structures in Two-Dimensional, Small-Deficit, Turbulent Wakes. J. Fluid Mech. 1986, 168, 31. [Google Scholar] [CrossRef]
- Louchez, P.R.; Kawall, J.G.; Keffer, J.F. Investigation of the Detailed Spread Characteristics of Plane Turbulent Wakes. In Proceedings of the Fifth Symposium on Turbulent Shear Flows, New York, NY, USA, 9 August 1985; Cornell University: Ithaca, NY, USA; pp. 98–109. [Google Scholar]
- Townsend, A.A. The Fully Developed Turbulent Wake of a Circular Cylinder. Aust. J. Sci. Res. Ser. A Phys. Sci. 1949, 2, 451–468. [Google Scholar]
- Sreenivasan, K.R.; Narasimha, R. Equilibrium Parameters for Two-Dimensional Turbulent Wakes. J. Fluids Eng. Trans. ASME 1982, 104, 167–169. [Google Scholar] [CrossRef]
- Fabris, G. Conditional sampling study of the turbulent wake of a cylinder. Part 1. J. Fluid Mech. 1979, 94, 673–709. [Google Scholar] [CrossRef]
- Bisset, D.K.; Antonia, R.A.; Browne, L.W.B. Spatial organization of large structures in the turbulent far wake of a cylinder. J. Fluid Mech. 1990, 218, 439–461. [Google Scholar] [CrossRef]

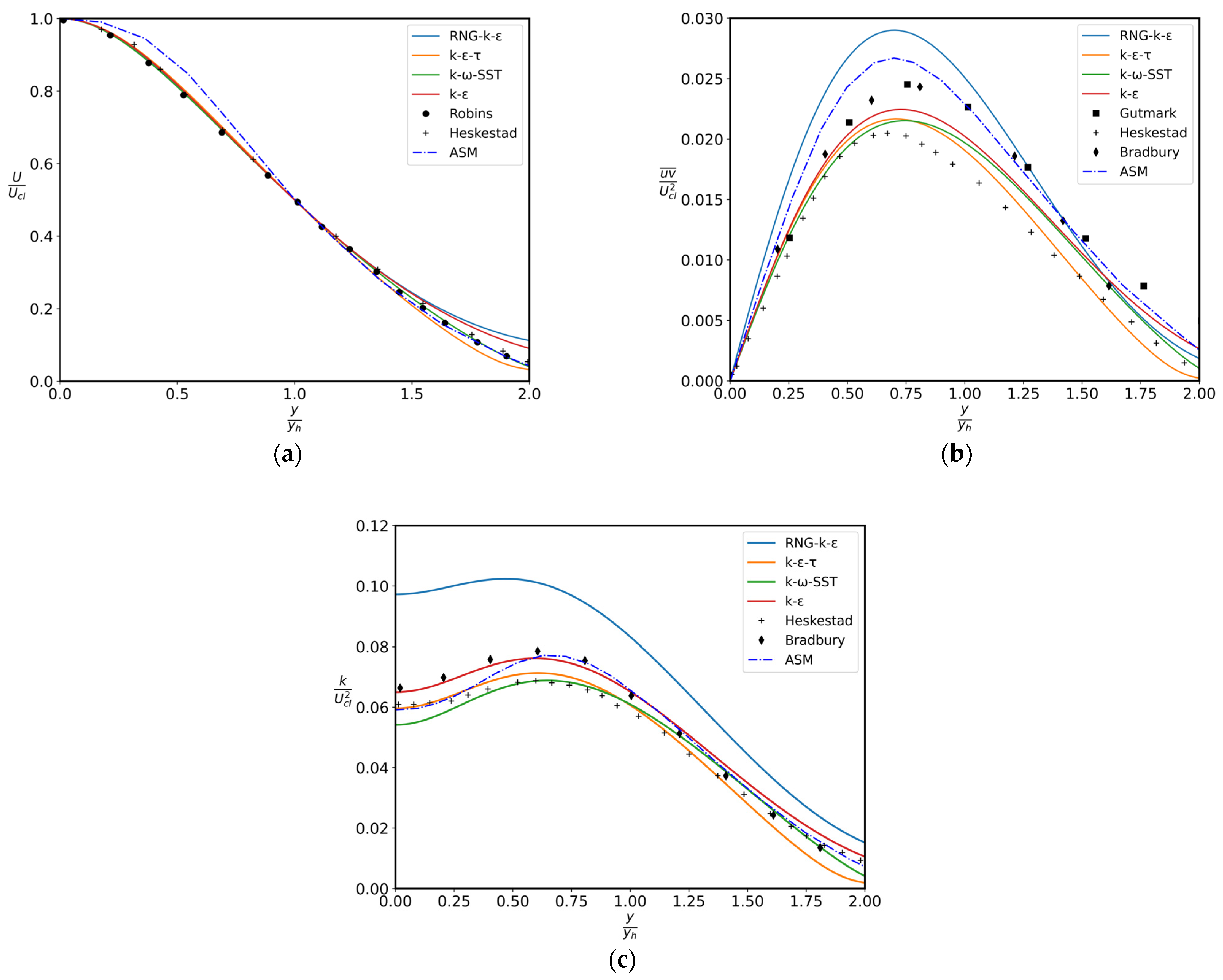

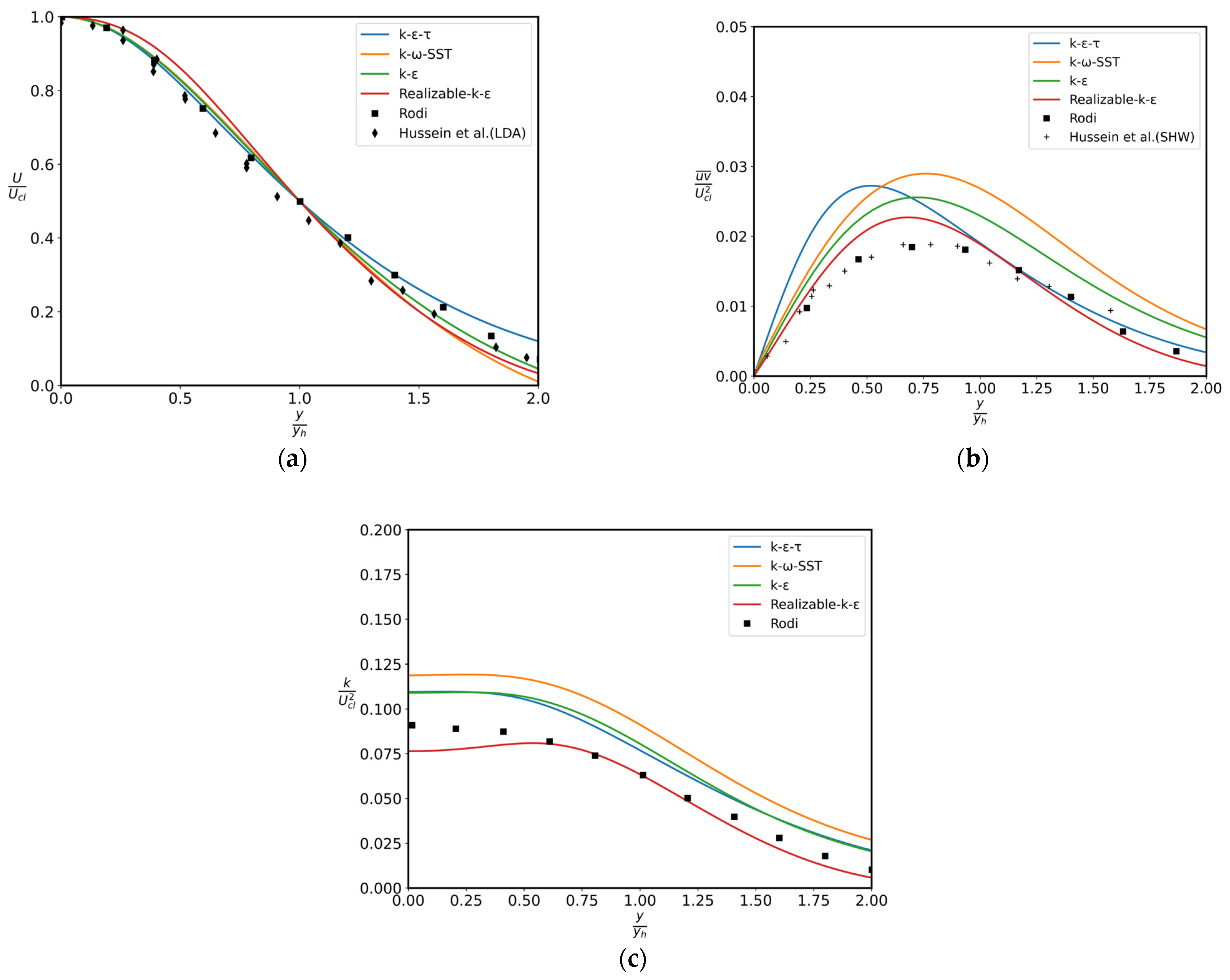
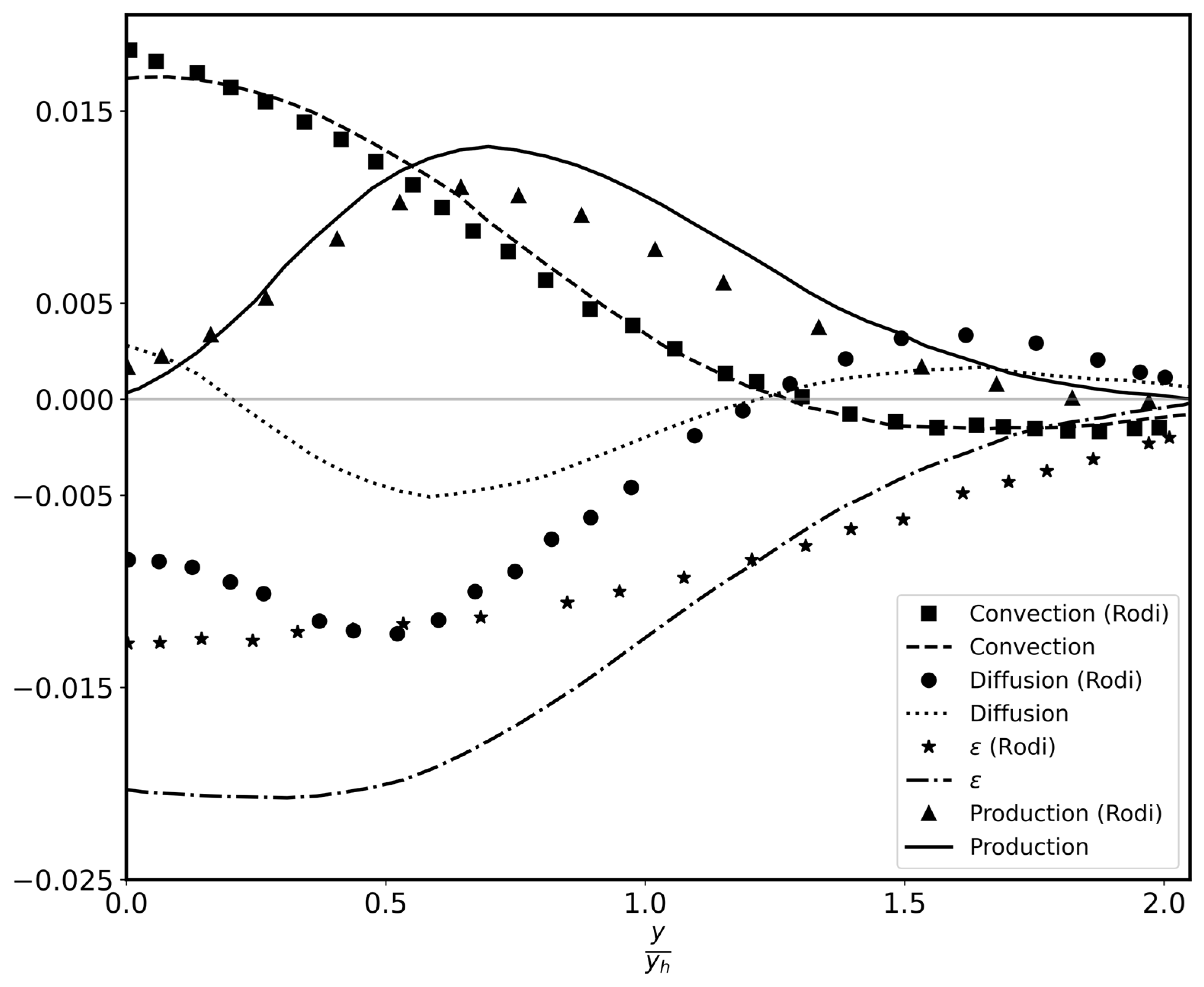

| 0.75 | 1.05 | 0.67 | 1.054 | 1.1 | 0.59 | 0.83 | 1.0 | 1.2 | 1.1 |
| Investigator | Spreading Rate | Remarks | |
|---|---|---|---|
| Bradbury [35] | 0.109 | 0.024 | HWA |
| Gutmark and Wygnanski [36] | 0.11 | 0.024 | HWA |
| Miller and Comings [37] | 0.097 | 0.025 | CTA |
| Van der Hegge Zijnen [39] | 0.095 | - | HWA |
| Heskestad [40] | 0.11 | 0.020 | HWA |
| Everitt and Robins [43] | 0.09–0.11 | 0.019 | CTA |
| Ramaprian and Chandrasekhara [42] | 0.112 | 0.02 | LDA |
| k–ε model | 0.108 | 0.022 | |
| SST k–ω model | 0.113 | 0.0215 | |
| RNG k–ε model | 0.117 | 0.029 | |
| k–ε–τ model | 0.109 | 0.0216 |
| Investigator | Spreading Parameter | |
|---|---|---|
| Everitt and Robins [43] | 0.096 | 0.037 |
| Ermshaus (from Ramaprian and Chandrasekhara [42]) | 0.089 | - |
| Wygnanski et al. [55] | 0.082 | 0.048 |
| Townsend [57] | 0.098 | 0.051 |
| Sreenivasan and Narasimha [58] | 0.092 | - |
| k–ε model | 0.077 | 0.036 |
| model | 0.079 | 0.042 |
| model | 0.099 | 0.046 |
| k–ε–τ model | 0.081 | 0.039 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Gul, M.Z.; Yangaz, M.U.; Sen, S. A Two-Time-Scale Turbulence Model and Its Application in Free Shear Flows. Appl. Sci. 2024, 14, 1133. https://doi.org/10.3390/app14031133
Gul MZ, Yangaz MU, Sen S. A Two-Time-Scale Turbulence Model and Its Application in Free Shear Flows. Applied Sciences. 2024; 14(3):1133. https://doi.org/10.3390/app14031133
Chicago/Turabian StyleGul, Mehmet Zafer, Murat Umut Yangaz, and Serhat Sen. 2024. "A Two-Time-Scale Turbulence Model and Its Application in Free Shear Flows" Applied Sciences 14, no. 3: 1133. https://doi.org/10.3390/app14031133
APA StyleGul, M. Z., Yangaz, M. U., & Sen, S. (2024). A Two-Time-Scale Turbulence Model and Its Application in Free Shear Flows. Applied Sciences, 14(3), 1133. https://doi.org/10.3390/app14031133







