Variance of the Infection Number of Heterogeneous Malware Spread in Network
Abstract
1. Introduction
2. Literature Review
3. Methodology
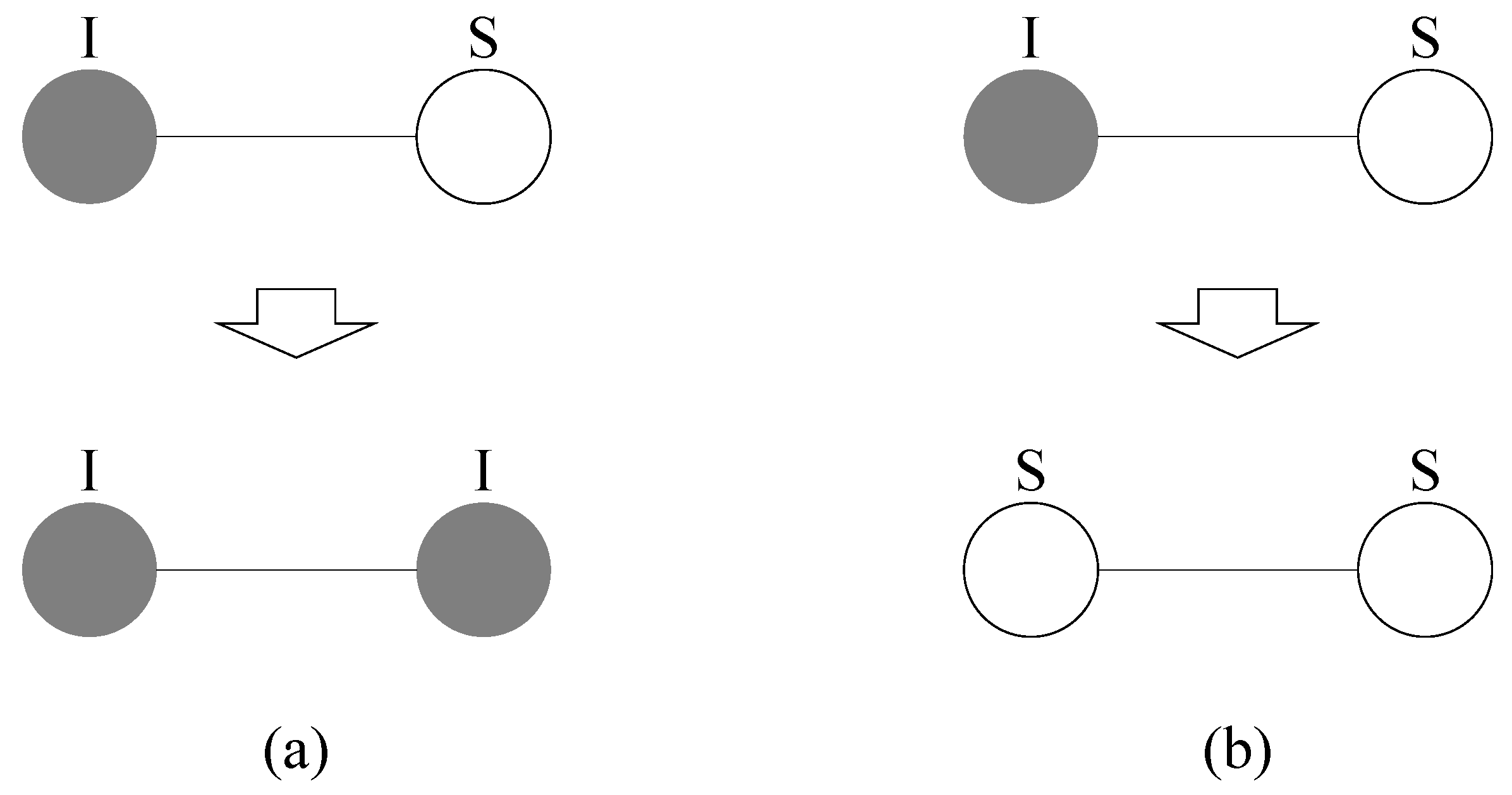
3.1. Theory Framework
3.2. Variance of Infection Fraction for Bipartite Graph
3.3. Simulation Method
4. Results
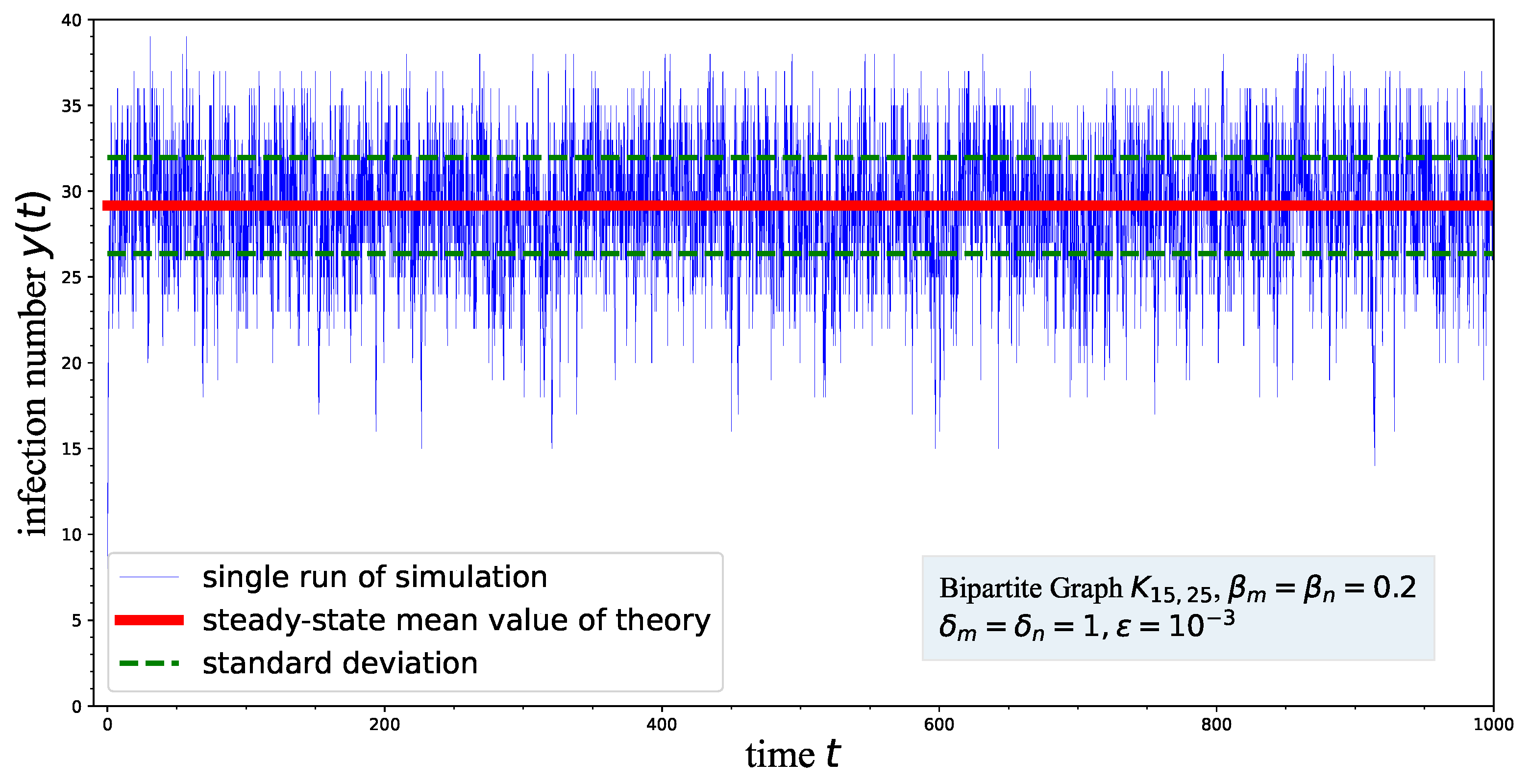
4.1. Time Evolution
4.2. Variance of Infection Fraction in Bipartite Graph
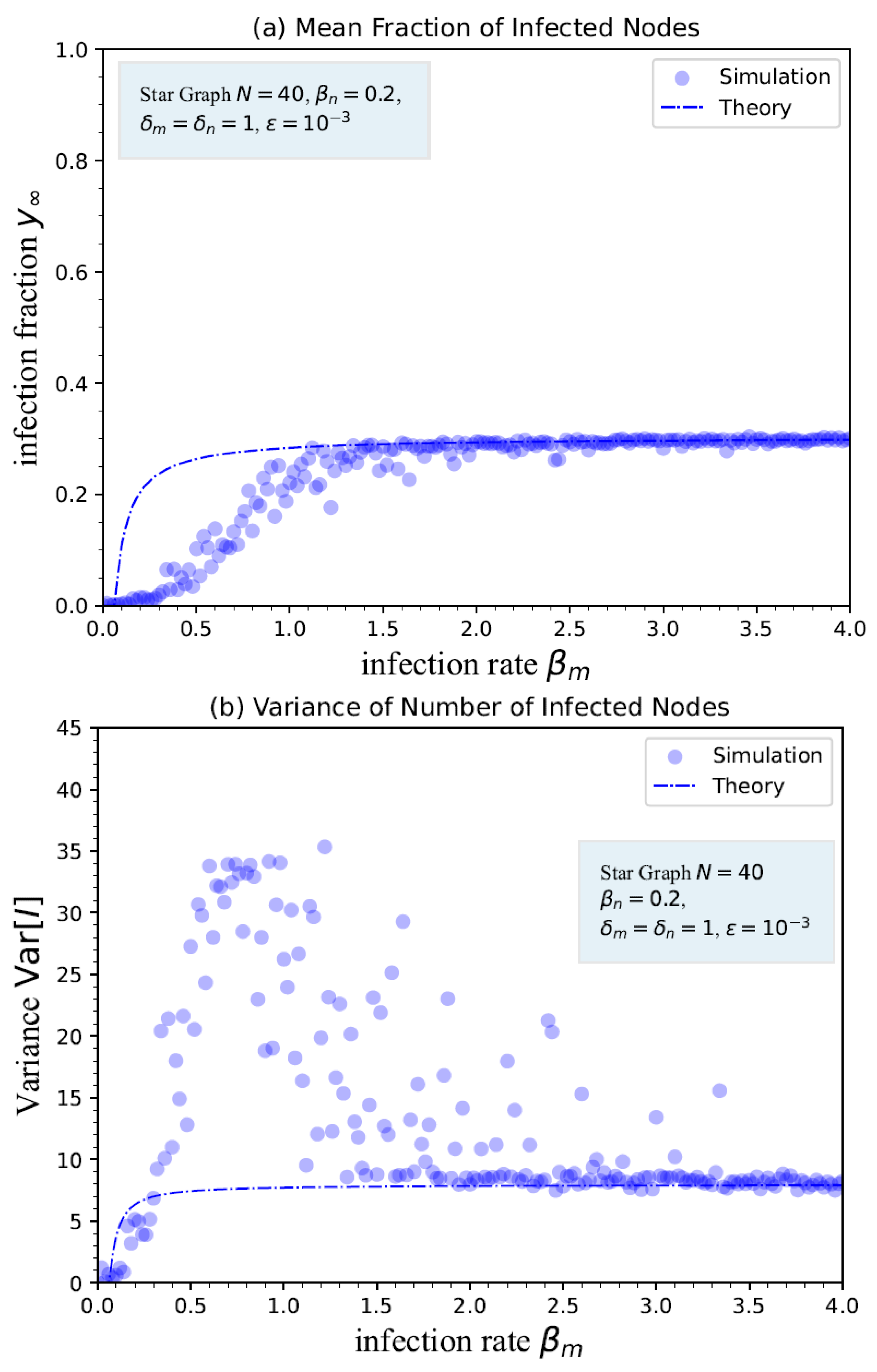
4.3. Variance of Infection Fraction in Star Graph
5. Discussion
6. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
Abbreviations
| SIS | Susceptible Infected Susceptible |
| NIMFA | N-Intertwined Mean-field Approximation |
| HMFA | Heterogeneous Mean-field Approximation |
Appendix A. Proof of Corollary 1
References
- Pastor-Satorras, R.; Castellano, C.; Van Mieghem, P.; Vespignani, A. Epidemic processes in complex networks. Rev. Mod. Phys. 2015, 87, 925–979. [Google Scholar] [CrossRef]
- de Arruda, G.F.; Rodrigues, F.A.; Moreno, Y. Fundamentals of spreading processes in single and multilayer complex networks. Phys. Rep. 2018, 756, 1–59. [Google Scholar] [CrossRef]
- Pastor-Satorras, R.; Vespignani, A. Epidemic dynamics and endemic states in complex networks. Phys. Rev. E 2001, 63, 066117. [Google Scholar] [CrossRef]
- Chakrabarti, D.; Wang, Y.; Wang, C.; Leskovec, J.; Faloutsos, C. Epidemic thresholds in real networks. ACM Trans. Inf. Syst. Secur. 2008, 10, 1:1–1:26. [Google Scholar] [CrossRef]
- Van Mieghem, P.; Omic, J.; Kooij, R. Virus Spread in Networks. IEEE/ACM Trans. Netw. 2009, 17, 1–14. [Google Scholar] [CrossRef]
- Van Mieghem, P.; Omic, J. In-Homogeneous Virus Spread in Networks. arXiv 2014, arXiv:1306.2588. [Google Scholar]
- Jiao, L.; Yin, H.; Guo, D.; Lyu, Y. Heterogeneous Malware Spread Process in Star Network. In Proceedings of the 2017 IEEE 37th International Conference on Distributed Computing Systems Workshops (ICDCSW), Atlanta, GA, USA, 5–8 June 2017; pp. 265–269. [Google Scholar] [CrossRef]
- Wang, K.; Guo, D.; Quan, W. Analyzing NDN NACK on Interest Flooding Attack via SIS Epidemic Model. IEEE Syst. J. 2020, 14, 1862–1873. [Google Scholar] [CrossRef]
- Cui, S.; Liu, F.; Jardón-Kojakhmetov, H.; Cao, M. Discrete-time layered-network epidemics model with time-varying transition rates and multiple resources. Automatica 2024, 159, 111303. [Google Scholar] [CrossRef]
- Pagliara, R.; Leonard, N.E. Adaptive Susceptibility and Heterogeneity in Contagion Models on Networks. IEEE Trans. Autom. Control 2021, 66, 581–594. [Google Scholar] [CrossRef]
- Ganesh, A.; Massoulie, L.; Towsley, D. The effect of network topology on the spread of epidemics. In Proceedings of the IEEE 24th Annual Joint Conference of the IEEE Computer and Communications Societies, Miami, FL, USA, 13–17 March 2005; Volume 2, pp. 1455–1466. [Google Scholar] [CrossRef]
- Omic, J.; Van Mieghem, P. Epidemic Spreading in Networks—Variance of the Number of Infected Nodes; Technical Report Report 20090707; Quantum and Computer Engineering Department, Delft University of Technology: Delft, The Netherlands, 2009. [Google Scholar]
- Van Mieghem, P. Performance Analysis of Communications Networks and Systems; Cambridge University Press: New York, NY, USA, 2006. [Google Scholar] [CrossRef]
- Van Mieghem, P.; Cator, E. Epidemics in networks with nodal self-infection and the epidemic threshold. Phys. Rev. E 2012, 86, 016116. [Google Scholar] [CrossRef]
- Li, C.; van de Bovenkamp, R.; Van Mieghem, P. Susceptible-infected-susceptible model: A comparison of N-intertwined and heterogeneous mean-field approximations. Phys. Rev. E 2012, 86, 026116. [Google Scholar] [CrossRef]
- Cator, E.; Van Mieghem, P. Second-order mean-field susceptible-infected-susceptible epidemic threshold. Phys. Rev. E 2012, 85, 056111. [Google Scholar] [CrossRef]
- Ward, J.A.; Tapper, A.; Simon, P.L.; Mann, R.P. Micro-scale foundation with error quantification for the approximation of dynamics on networks. Commun. Phys. 2022, 5, 71. [Google Scholar] [CrossRef]
- Ortega, E.; Machado, D.; Lage-Castellanos, A. Dynamics of epidemics from cavity master equations: Susceptible-infectious-susceptible models. Phys. Rev. E 2022, 105, 024308. [Google Scholar] [CrossRef] [PubMed]
- Han, L.; Lin, Z.; Yin, Q.; Tang, M.; Guan, S.; Boguñá, M. Non-Markovian epidemic spreading on temporal networks. Chaos Solitons Fractals 2023, 173, 113664. [Google Scholar] [CrossRef]
- Kuga, K.; Tanimoto, J. Effects of void nodes on epidemic spreads in networks. Sci. Rep. 2022, 12, 3957. [Google Scholar] [CrossRef] [PubMed]


Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Guo, D.; Jiao, L.; Jiao, J.; Meng, K. Variance of the Infection Number of Heterogeneous Malware Spread in Network. Appl. Sci. 2024, 14, 3972. https://doi.org/10.3390/app14103972
Guo D, Jiao L, Jiao J, Meng K. Variance of the Infection Number of Heterogeneous Malware Spread in Network. Applied Sciences. 2024; 14(10):3972. https://doi.org/10.3390/app14103972
Chicago/Turabian StyleGuo, Dongchao, Libo Jiao, Jian Jiao, and Kun Meng. 2024. "Variance of the Infection Number of Heterogeneous Malware Spread in Network" Applied Sciences 14, no. 10: 3972. https://doi.org/10.3390/app14103972
APA StyleGuo, D., Jiao, L., Jiao, J., & Meng, K. (2024). Variance of the Infection Number of Heterogeneous Malware Spread in Network. Applied Sciences, 14(10), 3972. https://doi.org/10.3390/app14103972





