Review of the Main Cable Shape Control of the Suspension Bridge
Abstract
1. Introduction
2. The Development of the Shape-Finding Calculation Theory for Main Cables
2.1. Analytical Methods
- (1)
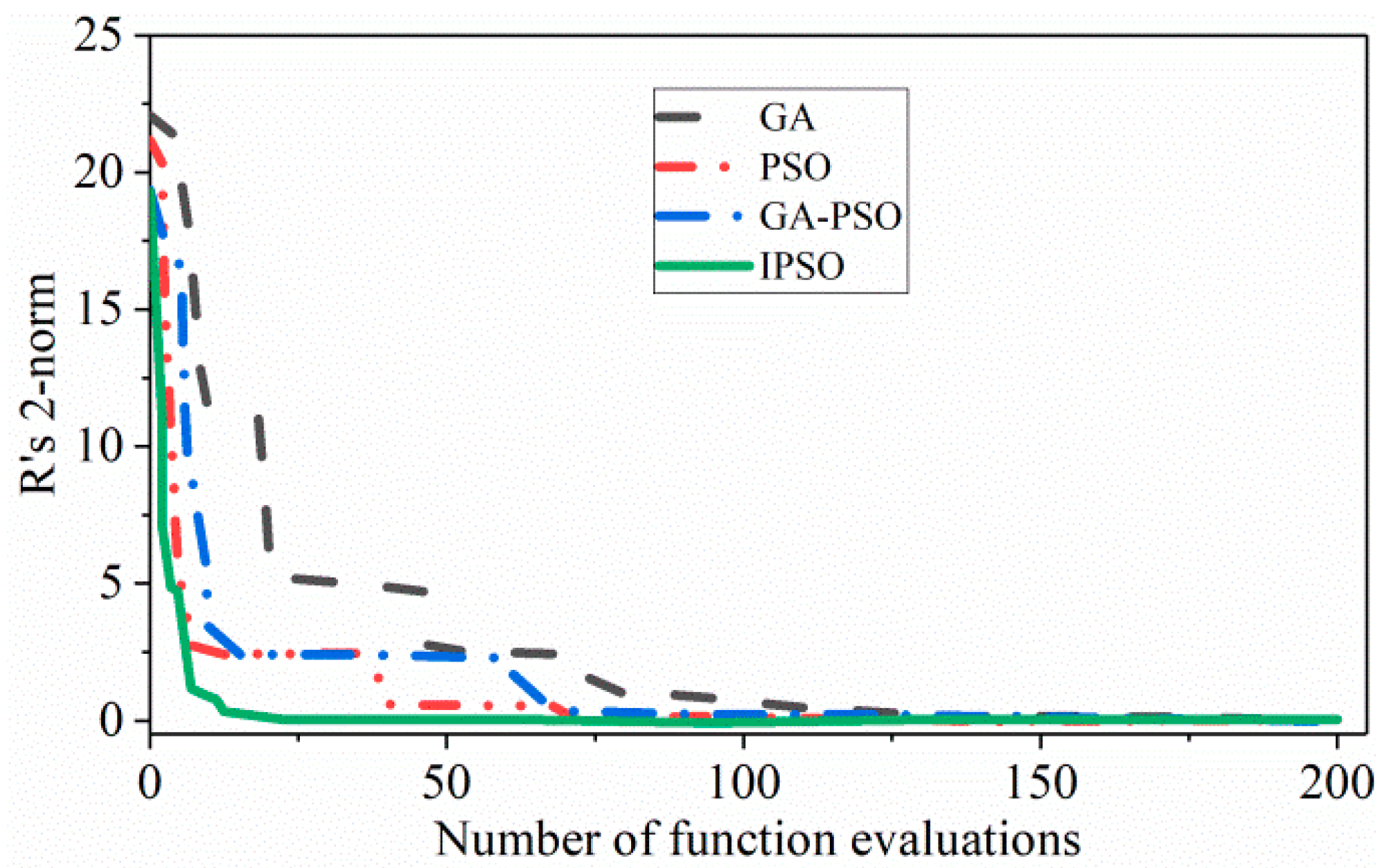
- The optimization of calculation methods
- (2)
- The refined analysis
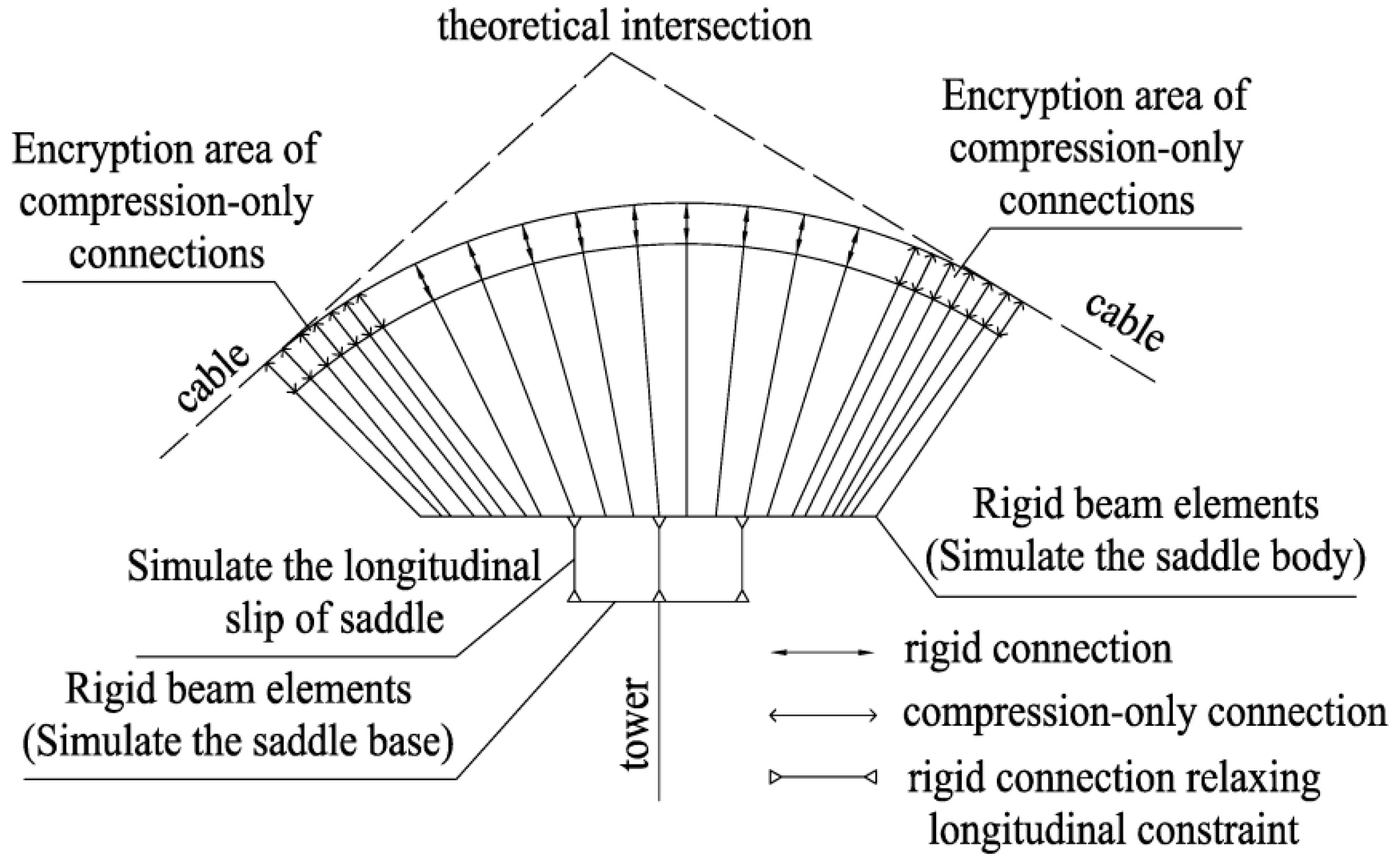
2.2. Finite Element Method (FEM)
- (1)
- The optimization of calculation methods
- (2)
- The refined analysis
2.3. The Combined Method
3. Construction Control Technology of the Main Cable
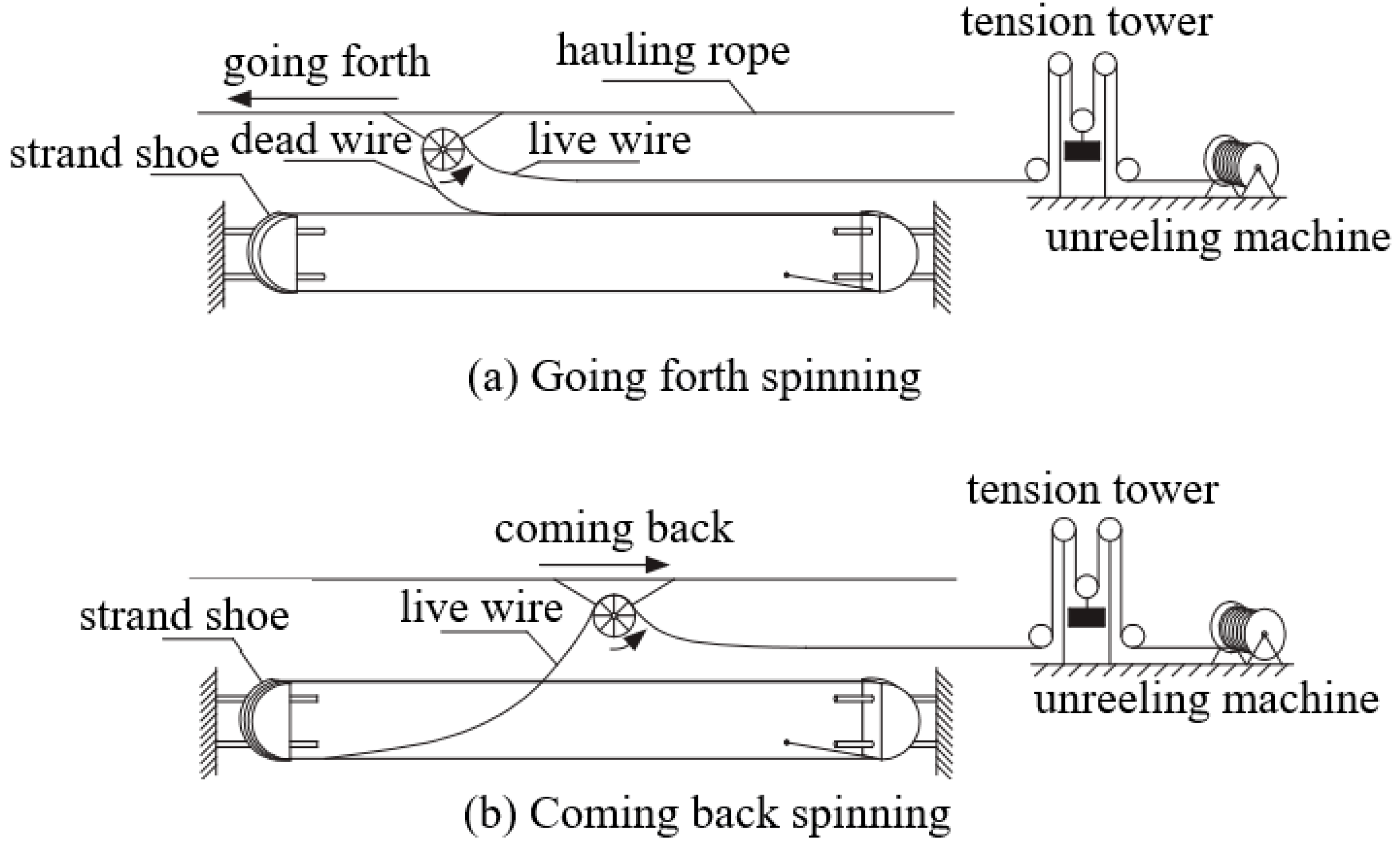
3.1. Construction Methods
- (1)
- AS method
- (2)
- PPWS method
3.2. Sag Control of Cable Strands
- (1)
- Sag adjustment method
- (2)
- Environmental conditions for the sag adjustment
- (3)
- Allowable deviation of the sag adjustment
3.3. Other Control Technologies
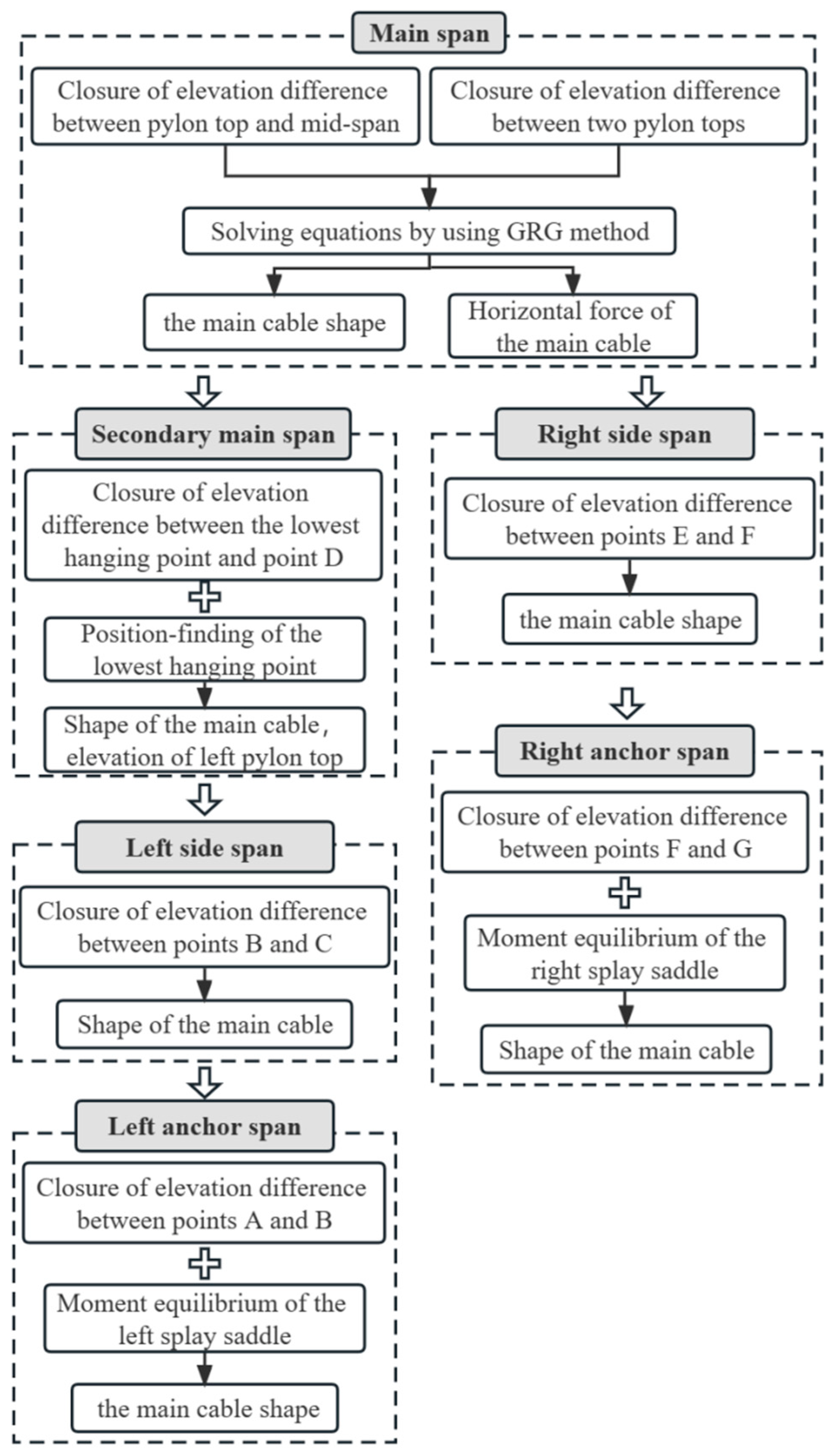
4. Construction Control Analysis of the Main Cable Shape
- (1)
- Parameter sensitivity analysis
- (2)
- Construction process analysis method
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Li, T.; Liu, Z. An improved continuum model for determining the behavior of suspension bridges during construction. Autom. Constr. 2021, 127, 103715. [Google Scholar] [CrossRef]
- Wang, X.; Wang, Y.; Zhu, P.; Zhang, X.; Wang, H.; He, Y. Experimental and numerical investigations of uhss wire main cables for suspension bridges. Structures 2022, 38, 1582–1594. [Google Scholar] [CrossRef]
- Wang, Y.G. Virtual trial assembly of steel structure based on bim platform. Autom. Constr. 2022, 141, 104395. [Google Scholar] [CrossRef]
- Zhou, X.; Zhang, X. Thoughts on the development of bridge technology in china. Engineering 2019, 5, 1120–1130. [Google Scholar] [CrossRef]
- Kim, H.S.; Kim, Y.J.; Chin, W.J.; Yoon, H. Development of highly efficient construction technologies for super long span bridge. Engineering 2013, 5, 629–636. [Google Scholar] [CrossRef]
- Apaydin, N.M.; Bas, S. Long-span orthotropic steel deck bridges of turkey. IOP Conf. Ser. Mater. Sci. Eng. 2018, 419, 012023. [Google Scholar] [CrossRef]
- Wang, S.; Zhou, Z.; Gao, Y.; Huang, Y. Analytical calculation method for the preliminary analysis of self-anchored suspension bridges. Math. Probl. Eng. 2015, 2015, 918649. [Google Scholar] [CrossRef]
- Thai, H.T. Advanced analysis of multi-span suspension bridges. J. Constr. Steel Res. 2013, 90, 29–41. [Google Scholar] [CrossRef]
- Son, Y.; Lee, C.; Yoo, D.; Kim, J.; Choi, J. Cheon-sa bridge—The first sea crossing multi-span suspension bridge. Struct. Eng. Int. 2021, 31, 431–434. [Google Scholar] [CrossRef]
- Wang, R. Study of Key Construction scheme for long-span suspension bridge in harsh mountainous region of plateau. Bridge Constr. 2019, 49, 108–113. [Google Scholar]
- Jia, L.; Lin, Z.; Xiao, R.; Jiang, Y. Parameter effects on the mechanical performance of triple-tower four-span suspension bridges. Adv. Struct. Eng. 2018, 21, 256–269. [Google Scholar] [CrossRef]
- Sun, J.Y.; Yin, C.Z.; Ma, Z.B. Object-oriented computer-aided analysis for construction control of anchored suspension bridge. AMM 2014, 543–547, 3977–3981. [Google Scholar] [CrossRef]
- Sun, Y.M.; He, X.D.; Li, W.D. Influential parameter study on the main-cable state of self-anchored suspension bridge. KEM 2014, 619, 99–108. [Google Scholar] [CrossRef]
- Hanaor, A. Prestressed pin-jointed structures—Flexibility analysis and prestress design. Comput. Struct. 1988, 28, 757–769. [Google Scholar] [CrossRef]
- Kim, H.K.; Lee, M.J.; Chang, S.P. Non-linear shape-finding analysis of a self-anchored suspension bridge. Eng. Struct. 2002, 24, 1547–1559. [Google Scholar] [CrossRef]
- Jung, M.R.; Min, D.J.; Kim, M.Y. Nonlinear analysis methods based on the unstrained element length for determining initial shaping of suspension bridges under dead loads. Comput. Struct. 2013, 128, 272–285. [Google Scholar] [CrossRef]
- Zhang, W.; Yang, C.; Tian, G.; Liu, Z. Analytical assessment of main cable shape for three-pylon suspension bridge with unequal main-span lengths: Thermal effect consideration. J. Bridge Eng. 2020, 25, 04019136. [Google Scholar] [CrossRef]
- Buonopane, S.; Billington, D. Theory and history of suspension bridge design from 1823 to 1940. J. Struct. Eng. 1993, 119, 954–977. [Google Scholar] [CrossRef]
- Ahmadi-Kashani, K.; Bell, A.J. The analysis of cables subject to uniformly distributed loads. Eng. Struct. 1988, 10, 174–184. [Google Scholar] [CrossRef]
- Jung, M.R.; Min, D.J.; Kim, M.Y. Simplified analytical method for optimized initial shape analysis of self-anchored suspension bridges and its verification. Math. Probl. Eng. 2015, 2015, 923508. [Google Scholar] [CrossRef]
- Tang, M.L. 3D Geometric Nonlinear Analysis of Long-Span Suspension Bridge and Its Software Development. Ph.D. Thesis, Southwest Jiaotong University, Chengdu, China, 2003. [Google Scholar]
- Zheng, J.J.; Ding, S.; Zhou, H.B.; Zhang, J.L.; Piao, J.M. Least squares calculation with Marquardt correction for spatial cable shape of suspension bridge. J. Beijing Jiaotong Univ. 2016, 40, 26–30. [Google Scholar]
- Wu, Y.X.; Zhou, J.T.; Sun, M.; Tian, Z.S.; Wang, Z. Geometry calculation method for main cables of completed suspension bridge with irregular spatial cable planes. Bridge Constr. 2020, 50, 37–43. [Google Scholar]
- Deng, X.K.; Xu, G.Y. New method for calculating main cable of suspension bridge. J. China Railw. Soc. 2019, 41, 133–141. [Google Scholar]
- Liu, C.; Gao, Z. A method for determining cable shape of a self-anchored suspension bridge based on an overall mechanical analysis. J. Tongji Univ. (Nat. Sci.) 2020, 48, 1–6. [Google Scholar]
- Liu, Z.; Zhan, H.P.; Zhu, Y. Modified force density method for form-finding of main cable of suspension bridges. J. Tongji Univ. (Nat. Sci.) 2022, 50, 351–358. [Google Scholar]
- Chen, Z.; Cao, H.; Ye, K.; Zhu, H.; Li, S. Improved particle swarm optimization-based form-finding method for suspension bridge installation analysis. J. Comput. Civ. Eng. 2015, 29, 04014047. [Google Scholar] [CrossRef]
- Cao, H.; Zhou, Y.-L.; Chen, Z.; Wahab, M.A. Form-finding analysis of suspension bridges using an explicit iterative approach. Struct. Eng. Mech. 2017, 62, 85–95. [Google Scholar] [CrossRef]
- Cao, H.; Qian, X.; Chen, Z.; Zhu, H. Layout and size optimization of suspension bridges based on coupled modelling approach and enhanced particle swarm optimization. Eng. Struct. 2017, 146, 170–183. [Google Scholar] [CrossRef]
- Wang, S.; Zhou, Z.; Wen, D.; Huang, Y. New method for calculating the preoffsetting value of the saddle on suspension bridges considering the influence of more parameters. J. Bridge Eng. 2016, 21, 06016010. [Google Scholar] [CrossRef]
- Gao, Q.F.; Hong, N.D.; Guo, B.Q.; Liu, Y.; Ma, Q.L. Calculation method for length of main cable at saddle in long-span suspension bridge. J. Harbin Inst. Technol. 2020, 52, 57–62. [Google Scholar]
- Luo, L.F.; Shan, D.S.; Chen, F.M.; Chen, P.Y. High-precision calculation method for configuration of completed suspension bridges with pin-connected cable clamps. Eng. Mech. 2021, 38, 133–144. [Google Scholar]
- Deng, X.; Deng, H. Improved algorithm to determine the composite circular curve splay saddle position. Struct. Eng. Int. 2022, 33, 141–146. [Google Scholar] [CrossRef]
- Li, T.; Liu, Z.; Zhang, W. Analysis of suspension bridges in construction and completed status considering the pylon saddles. Eur. J. Environ. Civ. En. 2022, 26, 4280–4295. [Google Scholar] [CrossRef]
- Sun, Y.; Zhu, H.P.; Xu, D. A specific rod model based efficient analysis and design of hanger installation for self-anchored suspension bridges with 3d curved cables. Eng. Struct. 2016, 110, 184–208. [Google Scholar] [CrossRef]
- Ozdemir, H. A finite element approach for cable problems. Int. J. Solids Struct. 1979, 15, 427–437. [Google Scholar] [CrossRef]
- Yang, Y.B.; Tsay, J.Y. Two-node catenary cable element with rigid-end effect and cable shape analysis. Int. J. Str. Stab. Dyn. 2011, 11, 563–580. [Google Scholar] [CrossRef]
- Chung, K.S.; Cho, J.Y.; Park, J.I.; Chang, S.-P. Three-dimensional elastic catenary cable element considering sliding effect. J. Eng. Mech. 2011, 137, 276–283. [Google Scholar] [CrossRef]
- Editorial Department of China Journal of Highway and Transport. An academic research summary on china highway and transport: 2012. China J. Highw. Transp. 2012, 25, 2–50. [Google Scholar]
- Kim, K.S.; Lee, H.S. Analysis of target configurations under dead loads for cable-supported bridges. Comput. Struct. 2001, 79, 2681–2692. [Google Scholar] [CrossRef]
- Kim, H.K.; Kim, M.Y. Efficient combination of a tcud method and an initial force method for determining initial shapes of cable-supported bridges. Int. J. Steel Struct. 2012, 12, 157–174. [Google Scholar] [CrossRef]
- Kim, M.Y.; Jung, M.R.; Attard, M.M. Unstrained length-based methods determining an optimized initial shape of 3-dimensional self-anchored suspension bridges. Comput. Struct. 2019, 217, 18–35. [Google Scholar] [CrossRef]
- Sun, Y.; Zhu, H.P.; Xu, D. New method for shape finding of self-anchored suspension bridges with three-dimensionally curved cables. J. Bridge Eng. 2015, 20, 04014063. [Google Scholar] [CrossRef]
- Coda, H.B.; de Oliveira Silva, A.P.; Paccola, R.R. Alternative active nonlinear total lagrangian truss finite element applied to the analysis of cable nets and long span suspension bridges. Lat. Am. J. Solids Struct. 2020, 17, e268. [Google Scholar] [CrossRef]
- Wang, H.; Qin, S. Shape finding of suspension bridges with interacting matrix. Eur. J. Environ. Civ. Eng. 2016, 20, 831–840. [Google Scholar] [CrossRef]
- Li, C.; He, J.; Zhang, Z.; Liu, Y.; Ke, H.; Dong, C.; Li, H. An improved analytical algorithm on main cable system of suspension bridge. Appl. Sci. 2018, 8, 1358. [Google Scholar] [CrossRef]
- Zhou, Y.; Chen, S. Iterative nonlinear cable shape and force finding technique of suspension bridges using elastic catenary configuration. J. Eng. Mech. 2019, 145, 04019031. [Google Scholar] [CrossRef]
- Zhu, W.; Ge, Y.; Fang, G.; Cao, J. A novel shape finding method for the main cable of suspension bridge using nonlinear finite element approach. Appl. Sci. 2021, 11, 4644. [Google Scholar] [CrossRef]
- Chen, Z.; Cao, H.; Zhu, H. An iterative calculation method for suspension bridge’s cable system based on exact catenary theory. Balt. J. Road Bridge Eng. 2013, 8, 196–204. [Google Scholar] [CrossRef]
- Fan, L.C.; Pan, Y.R.; Du, G.H. Study on the fine method of calculating the erection-parameters of long-span suspension bridges. China Civil Eng. J. 1999, 32, 20–25. [Google Scholar]
- Xu, J.L. Construction Control of Long-Span Bridges; China Communications Press: Beijing, China, 2000. [Google Scholar]
- Zhou, G.; Li, A.; Li, J.; Duan, M.; Xia, Z.; Zhu, L. Determination and implementation of reasonable completion state for the self-anchored suspension bridge with extra-wide concrete girder. Appl. Sci. 2019, 9, 2576. [Google Scholar] [CrossRef]
- Luo, X.H.; Xiao, R.C.; Xiang, H.F. Saddle-cable elements for nonlinear analysis of suspension bridges. China Civil Eng. J. 2005, 38, 47–53. [Google Scholar]
- Qi, D.C. A Refined Analysis Method of Main Cable for Long-Span Suspension Bridge. Ph.D. Thesis, Southwest Jiaotong University, Chengdu, China, 2012. [Google Scholar]
- Li, J.; Feng, D.; Li, A.; Yuan, H. Determination of reasonable finished state of self-anchored suspension bridges. J. Cent. South Univ. 2016, 23, 209–219. [Google Scholar] [CrossRef]
- Zhang, W.; Li, T.; Shi, L.; Liu, Z.; Qian, K. An iterative calculation method for hanger tensions and the cable shape of a suspension bridge based on the catenary theory and finite element method. Adv. Struct. Eng. 2019, 22, 1566–1578. [Google Scholar] [CrossRef]
- Matsuzaki, M.; Uchikawa, C.; Mitamura, T. Advanced fabrication and erection techniques for long suspension bridge cables. J. Constr. Eng. M. 1990, 116, 112–129. [Google Scholar] [CrossRef]
- Konishi, I. Latest developments on prefabricated parallel wire strand in japan. Ann. Ny. Acad. Sci. 1980, 352, 55–70. [Google Scholar] [CrossRef]
- Liu, X.H.; Xian, J.P.; Jin, C.; Li, S.; Guo, R. Construction techniques for main cable erection of suspension bridge by air spinning method. Technol. Highw. Transp. 2021, 37, 94–99. [Google Scholar]
- Moon, J.; Jeong, S.; Choi, H. Yi sun-sin bridge: Several unique features on the cable erection procedure. In Proceedings of the 18th Congress of IABSE: Innovative Infrastructures—Towards Human Urbanism, Seoul, Republic of Korea, 19–21 September 2012. [Google Scholar]
- Kim, J.; Lee, M.; Kim, J.; Choi, J. The recent cable and deck erection methods applied in various types of suspension bridges in korea. In Proceedings of the IABSE Conference—Structural Engineering: Providing Solutions to Global Challenges, Geneva, Switzerland, 23–25 September 2015. [Google Scholar]
- Yoo, H.; Seo, J.W.; Lee, S.H.; Park, Y.H. High-strength prefabricated parallel wire strand for ulsan harbor bridge and its mass production system in Korea. Struct. Eng. Int. 2014, 24, 293–297. [Google Scholar] [CrossRef]
- Kim, J.; Chung, K.; Yoon, J.; Lee, S. Erection of catwalk rope and main cable of jeokgeum bridge. In Proceedings of the 37th IABSE Symposium: Engineering for Progress, Nature and People, Madrid, Spain, 3–5 September 2014. [Google Scholar]
- Chen, Y.; Wei, W.; Dai, J. The key quality control technology of main cable erection in long-span suspension bridge construction. IOP Conf. Ser. Earth Environ. Sci. 2017, 61, 012124. [Google Scholar] [CrossRef]
- Zhang, W.; Liu, Z.; Xu, S. Jindong bridge: Suspension bridge with steel truss girder and prefabricated RC deck slabs in China. Struct. Eng. Int. 2019, 29, 315–318. [Google Scholar] [CrossRef]
- JTG/T 3650-2020; Technical Specifications for Construction of Highway Bridges and Culverts. Standards Press of China: Beijing, China, 2020.
- Zhang, J.Q.; Xu, Y.; Xian, Z.H. Study on grey forecasting control for construction of suspension bridge cables. J. Xi’an Highw. Univ. 1997, 17, 51–55. [Google Scholar]
- He, J.; Li, C.; Ke, H.; Liu, Y.; Zhang, Y.; Dong, C.; Li, H.; Zhang, Z. A simplified calculation method of length adjustment of datum strand for the main cable with small sag. Adv. Civ. Eng. 2019, 2019, 6075893. [Google Scholar] [CrossRef]
- Tan, H.M.; Yuan, S.H.; Xiao, R.C. The adjustment of datum strand of long-span suspension bridges. China Railw. Sci. 2010, 31, 38–43. [Google Scholar]
- Wang, Z.B. Construction monitoring techniques for superstructure of Ying Wuzhou Yangtze River Bridge in Wuhan. Bridge Constr. 2018, 48, 100–105. [Google Scholar]
- Deng, X.K.; Sun, J. Improved method for calculation of datum strand adjustment of suspension bridge. J. Chongqing Jiaotong Univ. (Nat. Sci.) 2021, 40, 90–95. [Google Scholar]
- Li, H.; Xian, Z.Q.; Shen, L.C.; Wen, W.; Dong, T. Method of adjusting cable strand sagging for suspension bridge of Runyang Bridge. Bridge Constr. 2004, 4, 36–39. [Google Scholar]
- Lu, W.; Gan, H.; Yu, Y.Q.; Tang, M.L.; Li, R.Z.; Qian, J.N. The adjustment technique for strand of main cable of Xi Houmen Bridge. Steel Construction. 2010, 25, 74–78. [Google Scholar]
- Huang, C.; Wang, Y.; Xu, S.; Shou, W.; Peng, C.; Lv, D. Vision-based methods for relative sag measurement of suspension bridge cables. Buildings 2022, 12, 667. [Google Scholar] [CrossRef]
- Tang, M.L.; Xu, G.T.; Li, C.; Tan, F.L.; Tang, Z.B.; Tan, G.Y.; Chen, X.Y.; Dong, J.H.; Zhang, X.B.; Wang, H.L. A Method Erection Method of Main Cable Strand Mark of Suspension Bridge Based on Multi-Standard Wire; SIPO: Beijing, China, 2019. [Google Scholar]
- Zhang, W.; Tian, G.; Liu, Z. Analytical study of uniform thermal effects on cable configuration of a suspension bridge during construction. J. Bridge Eng. 2019, 24, 04019104. [Google Scholar] [CrossRef]
- Matsumoto, K.; Arturo Linan Panting, C.; Kitratporn, N.; Takeuchi, W.; Nagai, K.; Iwasaki, E. Performance assessment using structural analysis and spatial measurement of a damaged suspension bridge: Case study of twantay bridge, myanmar. J. Bridge Eng. 2018, 23, 05018008. [Google Scholar] [CrossRef]
- Wang, X.; Zhao, Q.; Xi, R.; Li, C.; Li, G.; Li, L. Review of bridge structural health monitoring based on gnss: From displacement monitoring to dynamic characteristic identification. IEEE Access 2021, 9, 80043–80065. [Google Scholar] [CrossRef]
- Wang, C.; Hua, X.; Huang, Z.; Tang, Y.; Chen, Z. Post-critical behavior of galloping for main cables of suspension bridges in construction phases. J. Fluid. Struct. 2021, 101, 103205. [Google Scholar] [CrossRef]
- Birdsall, B. Discussion of “Advanced fabrication and erection techniques for long suspension bridge cables” by minora matsuzaki, chihiko uchikawa, and takeshi mitamura (march, 1990, vol. 116, no. 1). J. Constr. Eng. Manag. 1992, 118, 200–205. [Google Scholar] [CrossRef]
- Bi, X.D.; Feng, Y.X.; Yang, M. Technical improvement and construction of main cable strand erection of Ma Anshan Bridge. J. Highw. Transp. Res. Dev. (Appl. Technol.) 2014, 10, 215–218. [Google Scholar]
- Li, H. The main cable erection technology for preventing the main cable of suspension bridge from bulging and twisting. Chin. Overseas Architect. 2015, 177–178. [Google Scholar] [CrossRef]
- Liao, C.; Zhang, N.L.; Yi, J.W. The main suspension cable erection technology of Aizhai Bridge. Constr. Technol. 2013, 42, 5–8. [Google Scholar]
- Feng, C.B. Control techniques for superstructure construction of Wu Fengshan Yangtze River Bridge. Bridge Constr. 2020, 50, 99–104. [Google Scholar]
- Xu, T.; Zhang, Y.S.; Zeng, X. Influence of construction error on suspender length of suspension bridges. J. Chongqing Jiaotong Univ. (Nat. Sci.) 2013, 32, 915–917. [Google Scholar]
- Zhao, Y. Research on Main Cable Control and Poisson Effect Involved in Suspension Bridge. Master’s Thesis, Chang’an University, Xi’an, China, 2016. [Google Scholar]
- Zhao, J.; Xue, H.J.; Zhou, Z.B.; Dai, Z.F.; Gao, M. A Method to Control the Manufacture of Cable Strands by Using a Ruler Wire; SIPO: Beijing, China, 2010. [Google Scholar]
- Dan, Q.L. Alignment Controlling Theory and Application of Bridge Structure Formed in Stages Based on Unstressed State Control Method. Ph.D. Thesis, Southwest Jiaotong University, Chengdu, China, 2017. [Google Scholar]
- Wang, X.; Frangopol, D.M.; Dong, Y.; Lei, X.; Zhang, Y. Novel technique for configuration transformation of 3d curved cables of suspension bridges: Application to the dongtiao river bridge. J. Perform. Constr. Facil. 2018, 32, 04018045. [Google Scholar] [CrossRef]
- Zhang, W.; Yang, C.; Chang, J. Cable shape and construction parameters of triple-tower double-cable suspension bridge with two asymmetrical main spans. J. Bridge Eng. 2021, 26, 04020127. [Google Scholar] [CrossRef]
- Li, B. Control techniques for cable system construction of Wu Fengshan Yangtze River Bridge. Bridge Constr. 2021, 51, 119–126. [Google Scholar]
- Cho, T.; Kim, T.S. Probabilistic risk assessment for the construction phases of a bridge construction based on finite element analysis. Finite Elem. Anal. Des. 2008, 44, 383–400. [Google Scholar] [CrossRef]
- Li, J.; Li, A.; Feng, M.Q. Sensitivity and reliability analysis of a self-anchored suspension bridge. J. Bridge Eng. 2013, 18, 703–711. [Google Scholar] [CrossRef]
- An, X.; Gosling, P.D.; Zhou, X. Analytical structural reliability analysis of a suspended cable. Struct. Saf. 2016, 58, 20–30. [Google Scholar] [CrossRef]
- Wang, X.; Wang, H.; Sun, Y.; Mao, X.; Tang, S. Process-independent construction stage analysis of self-anchored suspension bridges. Autom. Constr. 2020, 117, 103227. [Google Scholar] [CrossRef]
- Dang, N.S.; Rho, G.T.; Shim, C.S. A master digital model for suspension bridges. Appl. Sci. 2020, 10, 7666. [Google Scholar] [CrossRef]







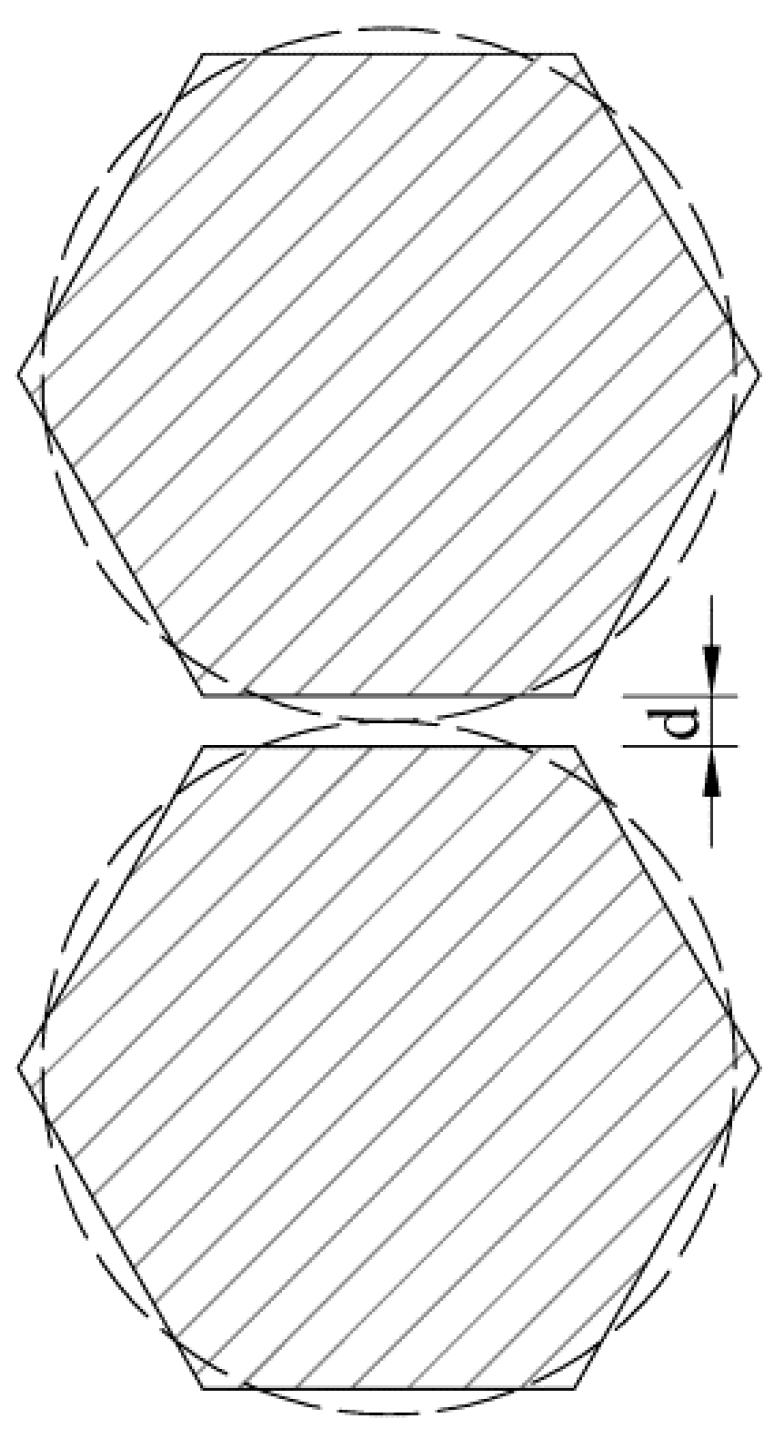

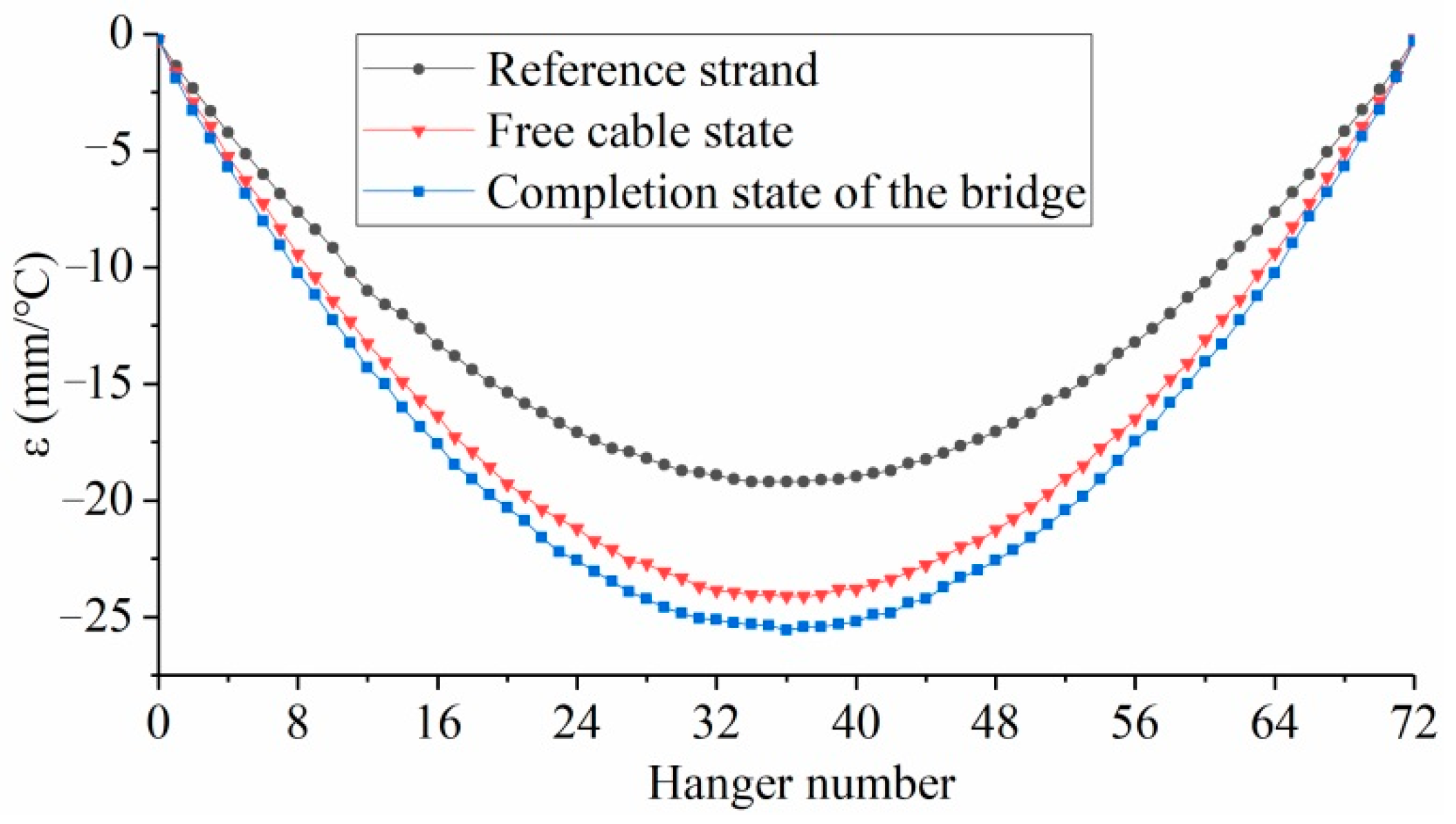

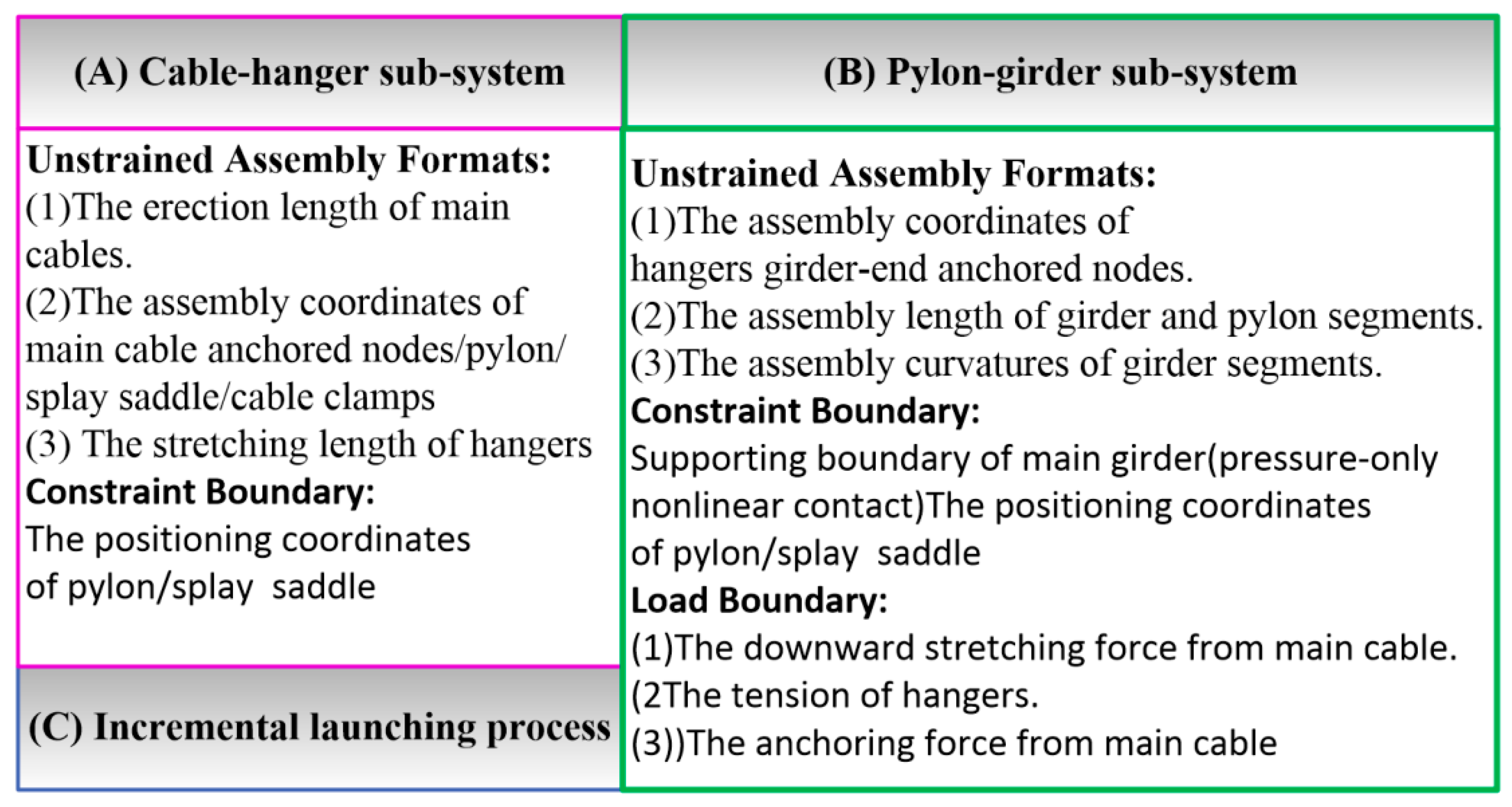
| Item Compared | AS Approach [57,59,63] | PPWS Approach [57,58,62,64] |
|---|---|---|
| production | on-site strand forming; steel wire needs joint length; steel wire crossing is inevitable; quality is difficult to control | factory-production; uniform length; controllable quality. |
| erection | larger affected by weather; slower erection; no need for large equipment; more temporary equipment | fast erection; large capacity requirements for transport and traction equipment |
| forced condition | the small bending radius of the anchor head leads to larger secondary stress; anchor connection with the large and complex force | uniform cable force; small secondary stress; simple anchor connection structure |
| Subjects | Allowable Deviation (mm) | ||
|---|---|---|---|
| strands elevation | reference strand | mid-span of the main span | ±l/20,000 |
| mid-span of side span | ±l/10,000 | ||
| upstream and downstream elevation difference | 10 | ||
| general strands | relative to reference strand | −5~+10 | |
| Bridge | Allowable Height Difference between General Strands and Reference Strand |
|---|---|
| Runyang Bridge [72], Ma Anshan Bridge [81] | 0~+5 mm |
| a self-anchored suspension bridge in China [82] | 0~15 mm |
| Aizhai Bridge [83] | −5 mm~+5 mm |
| Wu Fengshan Yangtze River Bridge [84] | −5 mm~+10 mm |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Huang, P.; Li, C. Review of the Main Cable Shape Control of the Suspension Bridge. Appl. Sci. 2023, 13, 3106. https://doi.org/10.3390/app13053106
Huang P, Li C. Review of the Main Cable Shape Control of the Suspension Bridge. Applied Sciences. 2023; 13(5):3106. https://doi.org/10.3390/app13053106
Chicago/Turabian StyleHuang, Pingming, and Chongjin Li. 2023. "Review of the Main Cable Shape Control of the Suspension Bridge" Applied Sciences 13, no. 5: 3106. https://doi.org/10.3390/app13053106
APA StyleHuang, P., & Li, C. (2023). Review of the Main Cable Shape Control of the Suspension Bridge. Applied Sciences, 13(5), 3106. https://doi.org/10.3390/app13053106





