Calculation of the Insertion Loss of Barriers on Rigid Ground in the Time Domain
Abstract
:1. Introduction
2. Theory
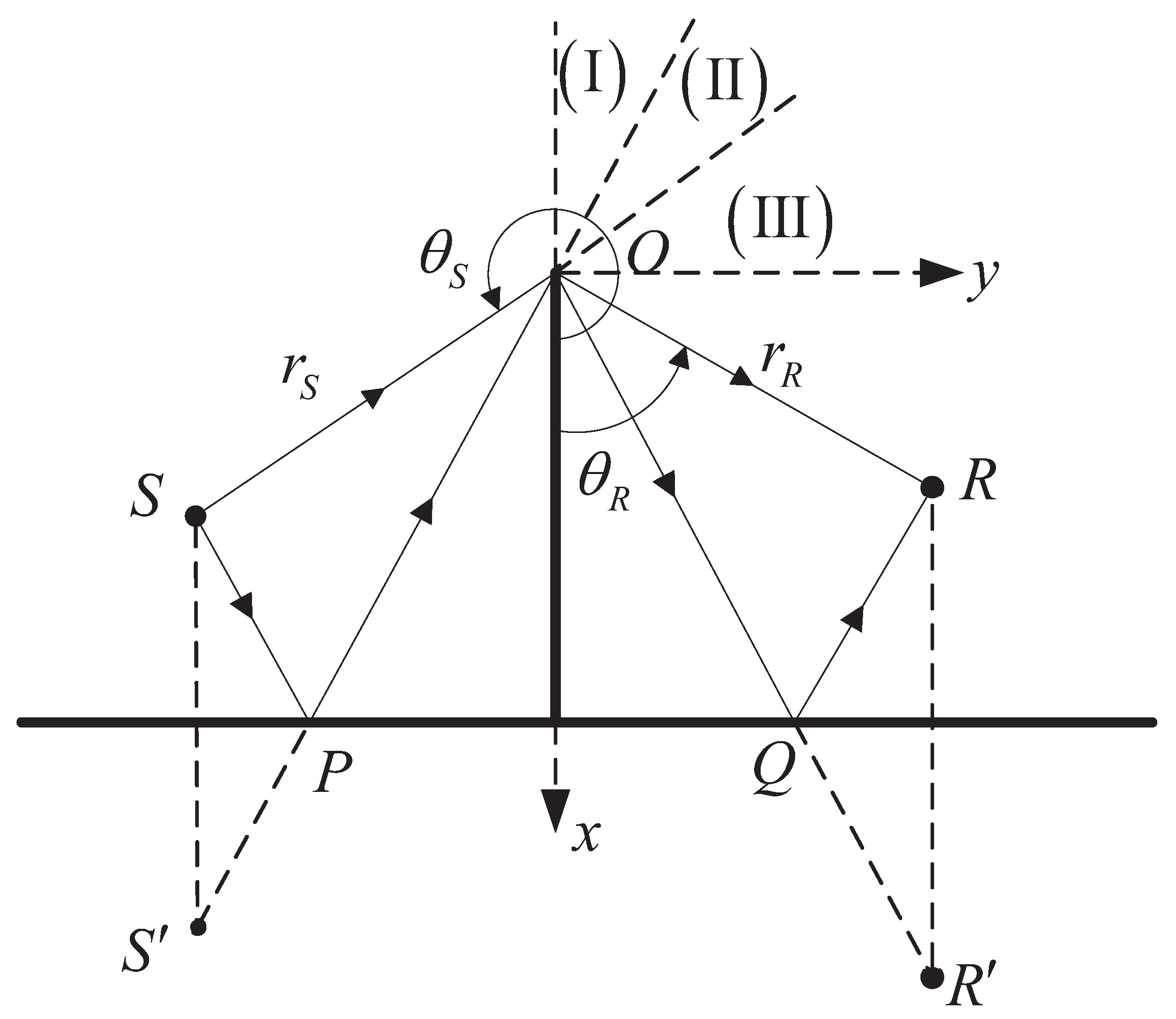
2.1. The Secondary Edge Source Model
2.2. Estimation of the Insertion Loss via the Image Method
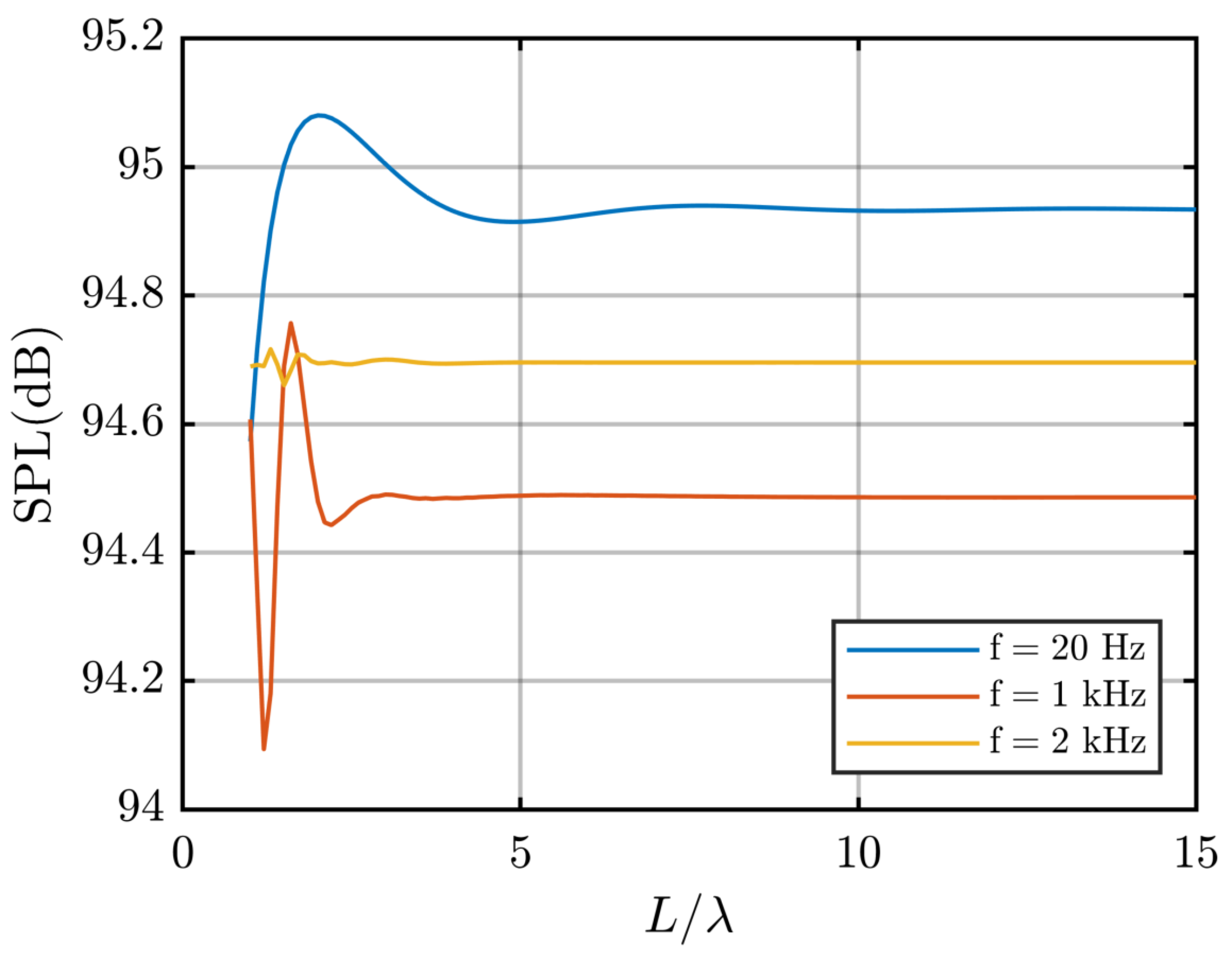
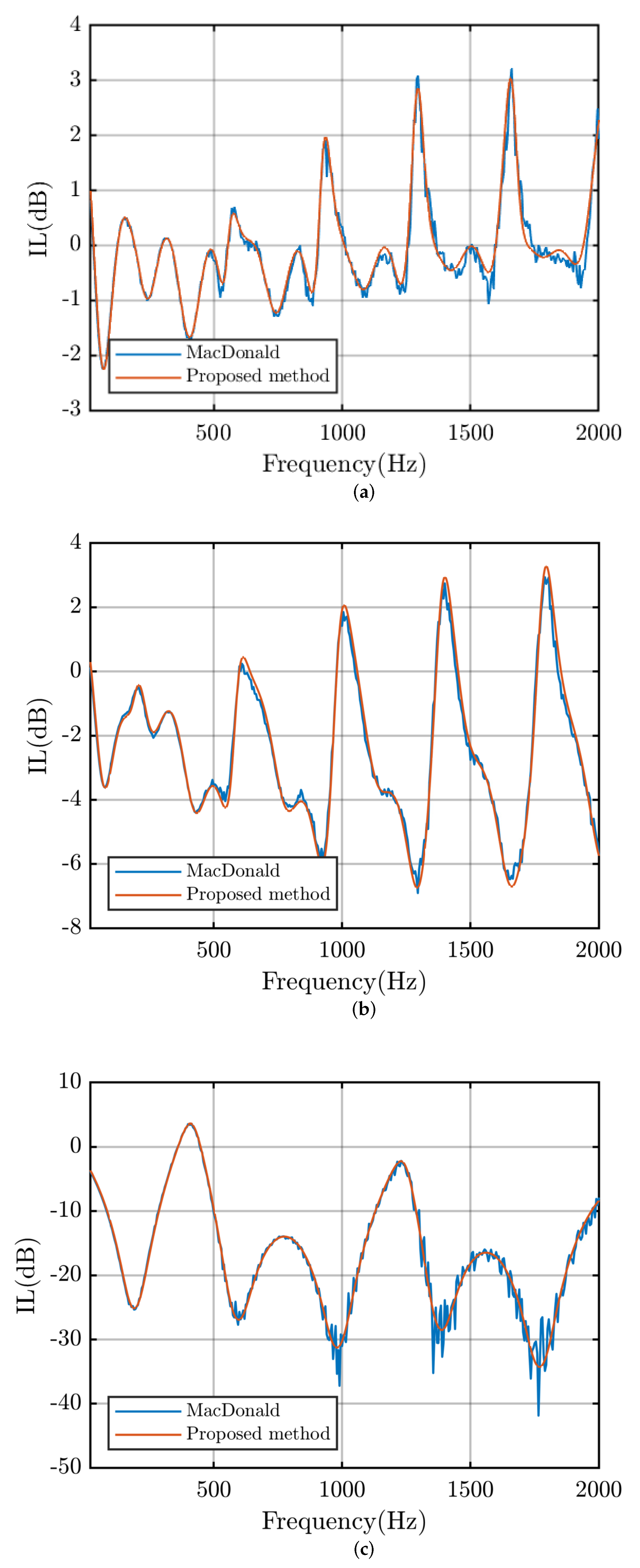
3. Simulation
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Pardo-Quiles, D.; Rodríguez, J.V.; Molina-García-Pardo, J.M.; Juan-Llácer, L. Traffic Noise Mitigation Using Single and Double Barrier Caps of Different Shapes for an Extended Frequency Range. Appl. Sci. 2020, 10, 5746. [Google Scholar] [CrossRef]
- Tang, S.K. Noise screening effects of balconies on a building facade. J. Acoust. Soc. Am. 2005, 118, 213–221. [Google Scholar] [CrossRef] [PubMed]
- Sommerfeld, A. Mathematische Theorie der Diffraction. Math. Ann. 1896, 47, 317–374. [Google Scholar] [CrossRef]
- Carslaw, H.S. Diffraction of Waves by a Wedge of any Angle. Proc. Lond. Math. Soc. 1920, 2, 291–306. [Google Scholar] [CrossRef]
- Macdonald, H.M. A Class of Diffraction Problems. Proc. Lond. Math. Soc. 1915, 2, 410–427. [Google Scholar] [CrossRef] [Green Version]
- Hadden, W.J.; Pierce, A.D. Sound diffraction around screens and wedges for arbitrary point source locations. J. Acoust. Soc. Am. 1981, 69, 1266–1276. [Google Scholar] [CrossRef]
- Zhao, S.; Qiu, X.; Cheng, J. An integral equation method for calculating sound field diffracted by a rigid barrier on an impedance ground. J. Acoust. Soc. Am. 2015, 138, 1608–1613. [Google Scholar] [CrossRef]
- Huang, X.; Zou, H.; Qiu, X. Effects of the Top Edge Impedance on Sound Barrier Diffraction. Appl. Sci. 2020, 10, 6042. [Google Scholar] [CrossRef]
- Biot, M.A.; Tolstoy, I. Formulation of Wave Propagation in Infinite Media by Normal Coordinates with an Application to Diffraction. J. Acoust. Soc. Am. 1957, 29, 381–391. [Google Scholar] [CrossRef]
- Medwin, H.; Childs, E.; Jebsen, G.M. Impulse studies of double diffraction: A discrete Huygens interpretation. J. Acoust. Soc. Am. 1982, 72, 1005–1013. [Google Scholar] [CrossRef]
- Svensson, U.P.; Fred, R.I.; Vanderkooy, J. An analytic secondary source model of edge diffraction impulse responses. J. Acoust. Soc. Am. 1999, 106, 2331–2344. [Google Scholar] [CrossRef] [Green Version]
- Svensson, U.P.; Calamia, P.T. Edge-Diffraction Impulse Responses Near Specular-Zone and Shadow-Zone Boundaries. Acta Acust. United Acust. 2006, 92, 501–512. [Google Scholar]
- Keller, J.B. Geometrical Theory of Diffraction. J. Acoust. Soc. Am. 1962, 52, 116–130. [Google Scholar] [CrossRef] [PubMed]
- Pathak, P.H.; Carluccio, G.; Albani, M. The Uniform Geometrical Theory of Diffraction and Some of Its Applications. IEEE Antennas Propag. Mag. 2013, 55, 41–69. [Google Scholar] [CrossRef]
- Ufimtsev, P.Y. Fundamentals of the Physical Theory of Diffraction, 2nd ed.; Wiley: Hoboken, NJ, USA, 2014. [Google Scholar] [CrossRef]
- Seznec, R. Diffraction of sound around barriers: Use of the boundary elements technique. J. Sound Vib. 1980, 73, 195–209. [Google Scholar] [CrossRef]
- Fard, S.M.B.; Peters, H.; Kessissoglou, N.; Marburg, S. Three-dimensional analysis of a noise barrier using a quasi-periodic boundary element method. J. Acoust. Soc. Am. 2015, 137, 3107–3114. [Google Scholar] [CrossRef] [PubMed]
- Monazzam, M.R.; Abbasi, M.; Yazdanirad, S. Performance Evaluation of T-Shaped Noise Barriers Covered with Oblique Diffusers Using Boundary Element Method. Arch. Acoust. 2019, 44, 521–531. [Google Scholar] [CrossRef]
- Kook, J.; Koo, K.; Hyun, J.; Jensen, J.S.; Wang, S. Acoustical topology optimization for Zwicker’s loudness model—Application to noise barriers. Comput. Meth. Appl. Mech. Eng. 2012, 237-240, 130–151. [Google Scholar] [CrossRef]
- Reiter, P.; Wehr, R.; Ziegelwanger, H. Simulation and measurement of noise barrier sound-reflection properties. Appl. Acoust. 2017, 123, 133–142. [Google Scholar] [CrossRef]
- Guo, J.; He, Y.; Wang, M.Y. Level-set based topology optimization on acoustic balcony ceiling design of a simplified urban building for noise reduction. J. Acoust. Soc. Am. 2020, 148, 3980–3991. [Google Scholar] [CrossRef]
- L’Espérance, A.; Nicolas, J.; Daigle, G.A. Insertion loss of absorbent barriers on ground. J. Acoust. Soc. Am. 1989, 86, 1060–1064. [Google Scholar] [CrossRef]
- Buret, M.; Li, K.M.; Attenborough, K. Diffraction of sound from a dipole source near to a barrier or an impedance discontinuity. J. Acoust. Soc. Am. 2003, 113, 2480–2494. [Google Scholar] [CrossRef] [PubMed]
- Li, K.M.; Wong, H.Y. A review of commonly used analytical and empirical formulae for predicting sound diffracted by a thin screen. Appl. Acoust. 2005, 66, 45–76. [Google Scholar] [CrossRef]

Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Gu, J.; Feng, X.; Shen, Y. Calculation of the Insertion Loss of Barriers on Rigid Ground in the Time Domain. Appl. Sci. 2022, 12, 2018. https://doi.org/10.3390/app12042018
Gu J, Feng X, Shen Y. Calculation of the Insertion Loss of Barriers on Rigid Ground in the Time Domain. Applied Sciences. 2022; 12(4):2018. https://doi.org/10.3390/app12042018
Chicago/Turabian StyleGu, Jun, Xuelei Feng, and Yong Shen. 2022. "Calculation of the Insertion Loss of Barriers on Rigid Ground in the Time Domain" Applied Sciences 12, no. 4: 2018. https://doi.org/10.3390/app12042018
APA StyleGu, J., Feng, X., & Shen, Y. (2022). Calculation of the Insertion Loss of Barriers on Rigid Ground in the Time Domain. Applied Sciences, 12(4), 2018. https://doi.org/10.3390/app12042018





