Design of a New TBM Integrated Cutter System Based on Analysis of Mechanical Properties and Dynamic Characteristics

Abstract

During the rock-breaking process, severe vibration frequently leads to cutter system failures. The traditional cutter system is complicated in structure, and the cutter-changing operation is highly dependent on the manual operation, resulting in a low construction efficiency and a high risk. Thus, this paper designed an integrated cutter system with a simple structure, which lays a theoretical foundation for popularizing the cutter-changing robot. In the statics performance experiment, we built an integrated cutter system finite element model, calculated the stress value under a nominal load; manufactured the scaled cutter system based on the similarity principle, obtained the measured stress value through the load experiment; and verified the accuracy of this cutter system’s finite element model. In the dynamics experiment, we calculated the cutter system and each components dynamic response based on the concentrated mass method and the Newmark method, obtained the measured vertical vibration displacement through a scaled loading experiment and verified the correctness of the vertical dynamics model, and set the real disc cutter linear cutting load as the external excitation to verify the feasibility of the new cutter system design scheme.

1. Introduction

The disc cutter bears a great alternating load in the tunnel boring machine (TBM) rock-breaking process. The violent vibration causes the failure of the cutter system fastener and high consumption of the cutter, which requires monitoring and replacement frequently. It is challenging to improve the tunneling efficiency [1,2]. At the same time, the cutter replacement process is highly dependent on the manual operation; various safety hazards often threaten the life and safety of operators [3]. Therefore, it is imperative to use the robot to replace the manual cutter detection and replacement operation.

Factors such as the complex structure and multiple disassembly and assembly operation steps of the traditional cutter system restrict the cutter-changing actions, which makes the cutter-changing robot difficult to popularize and use. Some universities and companies first optimized the structure based on traditional industrial robots to achieve an automatic cutter replacement [4,5]. However, this scheme is difficult to apply due to the limitation of operational space and the load ratio of industrial robots in TBMs. Therefore, researchers try to design a new structure of cutter changing robots. Based on the KUKA’s 6-DOF industrial manipulator, the Bouygues Group [6] developed a cutter changing robot for the shield machine, designed a new cutter holder, and developed the corresponding end-effector. Huo [7,8] et al. of Dalian University of Technology proposed a 6-DOF cutter-changing robot and carried out a cutter-changing range analysis, joint trajectory planning, and motion control simulation. In addition, a hybrid curve trajectory planning method based on the trapezoidal velocity curve and quintic polynomial curve was proposed, and a variable structure PID controller based on dynamic model compensation was designed.

Universities and companies also try to improve the cutter system design scheme. In 2015, French companies BOUYGUES [9] combined with companies NFM proposed a connecting rod cutter system, which has simple disassembly actions, but it is easy to be stuck in the movement process and has a low reliability. In 2018, the Robot Innovation Center of the German Artificial Intelligence Research and Development Center [10] developed a slider cutter system, which has a simple structure and less disassembly actions, but it is hard to manufacture. Two French researchers, Derycke Jean-Noel and Rubrecht Sebastien [11], invented an integrated rotating-panel TBM cutter system, which has a simple structure and few disassembly actions, but has disadvantages such as excessive stress on the rotating panel and easy sticking of the rotating panel.

The study of the cutting cutter system in China started late. In 2018, Hongrun Construction Co., Ltd. [4] cooperated with universities to develop an integrated cutter system that uses an eccentric circular mechanism to lock the cutter. The cutter can be locked and unlocked by grasping the cutter counterclockwise, but it is difficult for the cutter-changing robot to grasp accurately. Meng [12] et al. of Dalian University of Technology proposed an integrated cutter system based on the topological structure theory of the planar mechanism, and the solution space is obtained for the six-bar single-DoF, which further improves the anti-loosening effect.

In summary, the research on the integrated cutter system has achieved certain results. However, there are still shortcomings, mainly reflected in the number of disassembly steps, locking capacity, and cutter system reliability. Based on the above research results, aiming at simplifying the cutter system structure and reducing the disassembly and assembly steps, this paper innovatively proposes an integrated new TBM cutter system design scheme.

2. Mechanical Performance Experiment of New TBM Integrated Cutter System

2.1. Structure Design of New TBM Cutter System

Traditional TBM cutter system mainly has three types: double wedge blocks cutter system, upper and lower wedge blocks cutter system, and rear-installed cutter system. The main structure defect of the above traditional cutter system is a complex structure and has numerous parts. The disc cutter disassembly steps are complicated, which will significantly reduce the disc cutter replacement efficiency.

This paper obtains inspiration from the automatic firearm’s mechanism and optimizes the traditional cutter system’s structure. We obtain a sliding block swing mechanism and evolve it into a fastening mechanism. Supplementing the cutter holder, high-strength bolts, disc cutter, wedge, shift fork, cutter side panels, and other components can get the complete design scheme of the wedge swing type new cutter system, as shown in Figure 1.

The cutter system can be divided into the fastening module and supporting module according to the function. The main function of the fastening module is to fix the disc cutter tightly in the cutter system to ensure that it can reliably carry out a rock-breaking operation, and the main components are high-strength bolts, long shaft, shift fork, wedge, cushion block, fastening flange, etc. The main function of the support module is to limit the relative position between the cutter system’s two sides and provide a gripper for the operator or the cutter-changing robot when replacing the disc cutter. The main components are the grasping lever, side panels, cutter holder, cutter fixing bolts, etc.

The cutter system installation process begins when the operator or the cutter-changing robot places the entire cutter system into the cutter holder by holding the grasping lever. Just rotate the high-strength bolt and drive the shift fork to move up. At this time, the wedge will swing in the direction of the cutter holder side panel around the long axis, and the wedge top will extend into the groove inside the cutter holder to limit the displacement.

Conversely, when the cutter system needs to be removed, just rotate the high-strength bolt in the opposite direction and drive the shift fork to move down. At this time, the wedge will swing in the direction of the disc cutter around the long axis and the wedge top will leave the groove inside the cutter holder. So that the operator or the cutter-changing robot can lift the entire cutter system from the cutter holder through the grasping lever.

The core advantage of the new TBM integrated cutter system is that it simplifies and integrates the structure of the cutter system, greatly reduces the operation steps, improves the disc cutter replacement efficiency, and provides the possibility for the cutter-changing robot popularization. The disassembly performance comparison between the new and the traditional cutter system is shown in

Table 1. Disassembly performance comparison of various cutter systems.

Cutter System Design Scheme	Number of Disassembly Parts	Number of Disassembly Procedures
Double wedge blocks cutter system	11	>15
Upper and lower wedge blocks cutter system	17	>25
Rear-installed cutter system	5	>7
New TBM integrated cutter system	0	2

.

At the same time, the overall weight of the new TBM integrated cutter system is significantly reduced compared with the traditional cutter system because of the emphasis on the lightweight design at the beginning of the design process (the total weight of the new cutter system is only 354.4 kg, which is significantly less than that of the traditional cutter system). The smaller weight is helpful for the operation of the operator or the cutter-change robot, which can improve the construction efficiency.

2.2. Finite Element Stress Calculation of New TBM Integrated Cutter System

The stress distribution rule of the cutter system under a nominal load can be obtained by using the finite element simulation calculation before the statics loading experiment. For the stress concentration part, the actual stress value can be measured by the statics loading experiment to verify the finite element calculation results.

In the finite element simulation process, a proper mesh size can form a balance between the computing efficiency, storage space, and calculation accuracy. Therefore, the finite element mesh convergence analysis of the cutter system should be carried out firstly to obtain the optimal mesh size.

After calculating and comparing the cutter system stress values under 5 groups of different mesh sizes (20 mm, 10 mm, 7 mm, 5 mm, and 3 mm), it is found that the stress values can obtain the highest stability and maintain a high precision under a 5 mm mesh size. Therefore, the finite element mesh size of the entire cutter system was set as 5 mm.

Take the real TBM working condition as an example, the disc cutter’s nominal load is a 315 KN vertical load, a 31.5 KN rolling load, and a 47.25 KN lateral load. However, the vertical load is obviously greater than the load in the other two directions (more than one order of magnitude). In the real rock-breaking process, the vertical load is the most important load which the disc cutter borne. Therefore, in order to simplify the calculation process, this paper ignored the rolling load and lateral load and instead just applied the vertical load (315 kN) to the model for the calculation of the finite element.

According to the assembly relationship between the TBM cutter head and cutter system, the holder is set as completely fixed in the finite element model, and the disc cutter and fastening devices are fixed as a whole part. Contact is set between the disc cutter shaft and the side panel circular groove surface, and the friction coefficient of this surface is set as 0.1.

Apply the nominal load to the disc cutter blade and start the finite element simulation. On this basis, we set other groups of different value loads and applied them to the finite element model in turn. The finite element model rationality can be verified by comparing the maximum stress position under different load values.

We calculate the cutter system’s stress by using finite element software. According to the results, the cutter system’s calculated maximum stress 131.92 MPa occurs at the apex of the shift fork, at point Max, shown in Figure 2.

2.3. Similarity Theory and Scaled Cutter System

In equipment development and testing, prototypes are often scaled down to obtain similar models for various performance tests. The similarity model has the advantages of a low cost, short test cycle, and an ease to control, which helps form the empirical formula quickly [13,14]. We carried out statics and dynamic experiments on the new TBM cutter system based on the similarity theory in this paper.

The establishment of similar structural parameters and quality attributes between the scaled cutter system and the prototype cutter system is a prerequisite for accurately simulating the real working conditions and loading of the TBM cutter system [15]. Therefore, select the similar parameters of the TBM prototype cutter system as follows:

(a)

Structural parameters: the side length l of a single cutter holder.

(b)

Physical properties: material density ρ, Poisson’s ratio μ, and elastic modulus E.

Based on the dimensional analysis method, and comprehensively considering the performance requirements and manufacturing costs of the scaled cutter system, as well as the structure and working range of the test bench, the similarity ratio of the cutter system’s structural parameters is formulated as 1:4. Since the materials used in the machining the scaled tool system are the same as those of a real TBM cutter system, the elastic modulus E similarity ratio in the material characteristic quantity is C_E = 1, Poisson’s ratio similarity ratio is C_μ = 1, and the density similarity ratio is C_ρ = 1.

Combining the Buckingham theorem, the similar constants of the related physical quantities of the scaled cutter system are shown in

Table 2. Similarity constant of physical quantity of scale cutter system.

Classification	Physical Quantity	Similar Relationship	Scaled Ratio
Material characteristics	Stress σ	Cσ = CE	1:1
	Elastic modulus E	CE = 1	1:1
	Poisson’s ratio μ	Cμ = 1	1:1
	Density ρ	Cρ = 1	1:1
Geometric quantity	Side length l	Cl	1:1
Load quantity	Loading force F	CF = CE$C_l^2$	1:4
	Moment M	CM = CE$C_l^3$	1:16
	Surface load P	CP = CE	1:1
Dynamic quantity	Mass m	Cm = Cρ$C_l^3$	1:16
	Stiffness k	Ck = CE Cl	1:1
	Damping c	Cc = $C_l^2$(CE Cρ)1/2	1:4

.

Table 2 shows a square relationship between the loading force F and the side length l of the cutter system. Therefore, the loading force F should be scaled according to the square of the scale ratio, that is, 1:16. Since the nominal load of the real size disc cutter is 315 KN, the equivalent load applied to the scaled cutter system in the loading test is 19.69 KN.

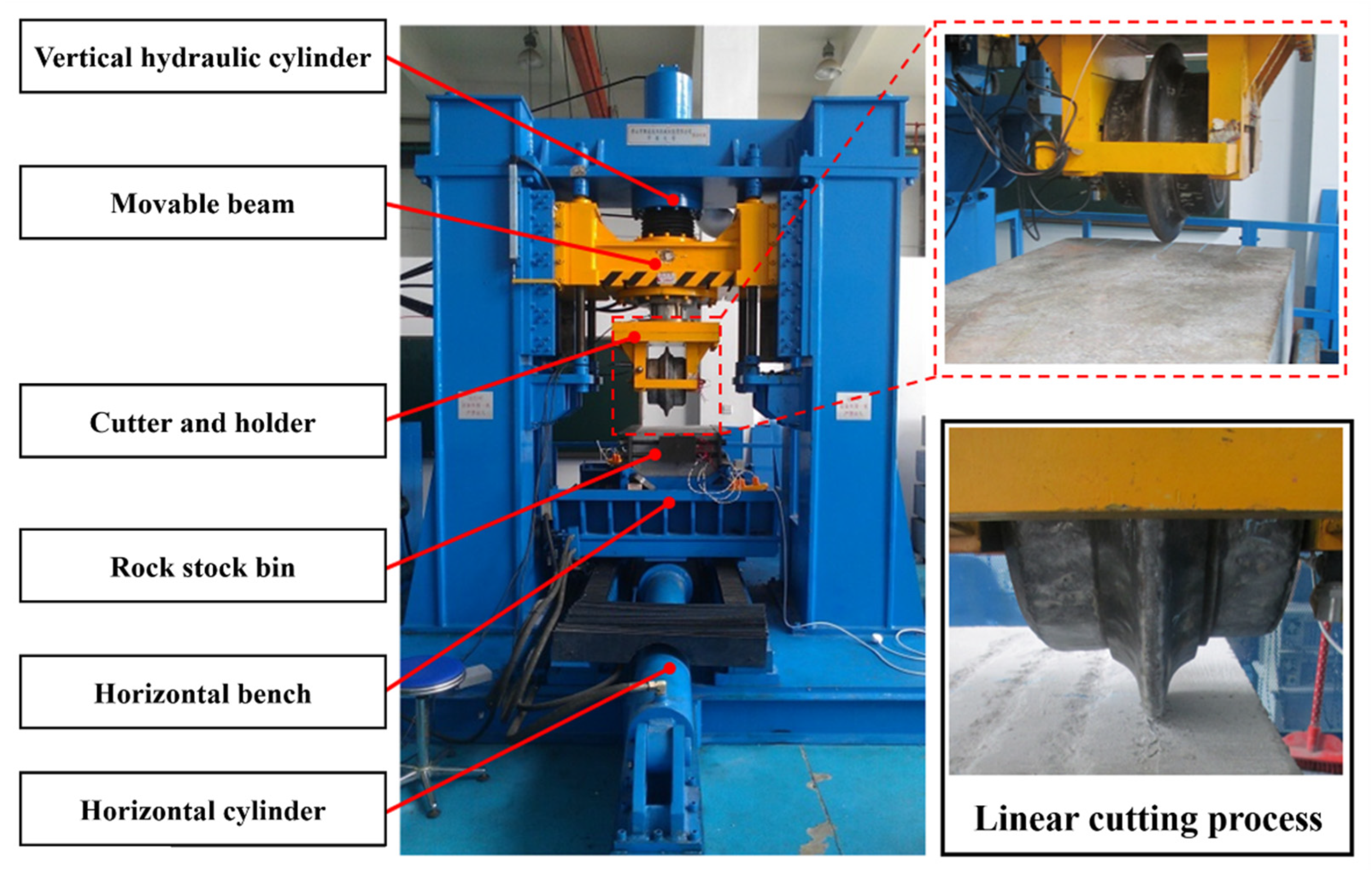
2.4. The Scheme Design of Scaled Loading Experiment

In this scaled loading experiment, arrange the strain sensors on the cutter system’s key positions and apply the equivalent load to the scaled cutter system, which can obtain the measured stress values of the cutter system. The accuracy of the finite element model can be verified by the error between the calculated and measured stress values.

According to the finite element stress calculation results, the stress will concentrate in two points when the nominal load is applied to the cutter system: one point is the wedge top that contacts the cutter holder inside the groove, another is the circular groove top on the side panel that contacts the cutter shaft. Therefore, this paper takes these two points as the key positions for the stress measurement.

To reduce the error and avoid the negative impact of the accuracy which is caused by the strain sensor quality, this experiment arranges the strain sensors symmetrically on the left and right sides of the cutter system. Strain sensors #1, #2, and #5 are used to measure the stress at two key positions on the cutter system’s left side, and strain sensors #3, #4, and #6 are on the right side. Finally, the stress data of the left and right sides are compared with each other to verify the accuracy.

Considering the arrangement operability, strain sensors #1 and #2 are arranged in the plane area around the wedge top of the cutter system’s left side to simultaneously measure the stress value and are used as the comparison verification to reduce the error. Strain sensors #3 and #4 are arranged in the same way. At the same time, strain sensors #5 and #6 are arranged, respectively, at circular grooves at the top of the left and right side of the cutter system’s panels to simultaneously measure the stress value. A detailed sensor arrange schematic diagram is shown as Figure 3a.

The critical experimental devices in this statics performance experiment mainly include a test bench, wireless strain sensors, fixtures, and a hydraulic loader, shown in Figure 3b. The fixtures are used to limit the cutter system’s displacement, which divides into upper, middle, and lower parts. Wireless strain sensors are used to collect the strain data. To maximize the accuracy, arrange the strain gauges on six key points, and set 4 sets of load values, specifically 5 kN, 10 kN, 15 kN, and 20 kN.

The critical experimental devices used in this experiment are as follows:

(1) A hydraulic servo actuator; (2) a control system; (3) two computers; (4) a wireless gateway; (5) four strain test nodes; (6) and testing software. Part of the experimental test instruments is shown in Figure 4.

2.5. Processing and Analysis of Experimental Data

The statics performance experiment has obtained the strain value ε on the measured key points of the cutter system, and the elastic modulus E of the cutter holder material is known, so that stress value σ can be converted by Equation (1).

$$\sigma =E\epsilon$$

(1)

Strain data are collected 12 times, respectively. The average strain value ε is obtained after processing, and then we plugged it into Equation (1) to obtain the average stress value σ of the two parts, which can be compared with the finite element calculation results.

We applied the load to the scaled cutter system separately according to 4 sets of the load values, collected the strain values of the six strain sensors 10 times, and the average values at each sensor in each loading. The maximum error between the measured and calculated stress is 8.35% through comparison and calculation. Thus, the accuracy of the finite element model and the static properties of the new cutter system is proved.

3. Multi-DoF Coupled Dynamic Model of New TBM Integrated Cutter System

Due to a complex structure and connection relations, and the difficulty in predicting the vibration characteristics of the cutter system, it often leads to abnormal damage, deformation, and other faults to disc cutters and their holders [16,17]. The load will cause significant damage to the cutter body and holder’s fastening bolts, even the whole cutter system [18].

To build an accurate multi-DoF coupled dynamic model of the TBM new cutter system, establish an equivalent dynamic model based on the concentrated mass method, obtain the dynamic equation, and calculate the effective stiffness and damping of each node through the formula method and finite element method.

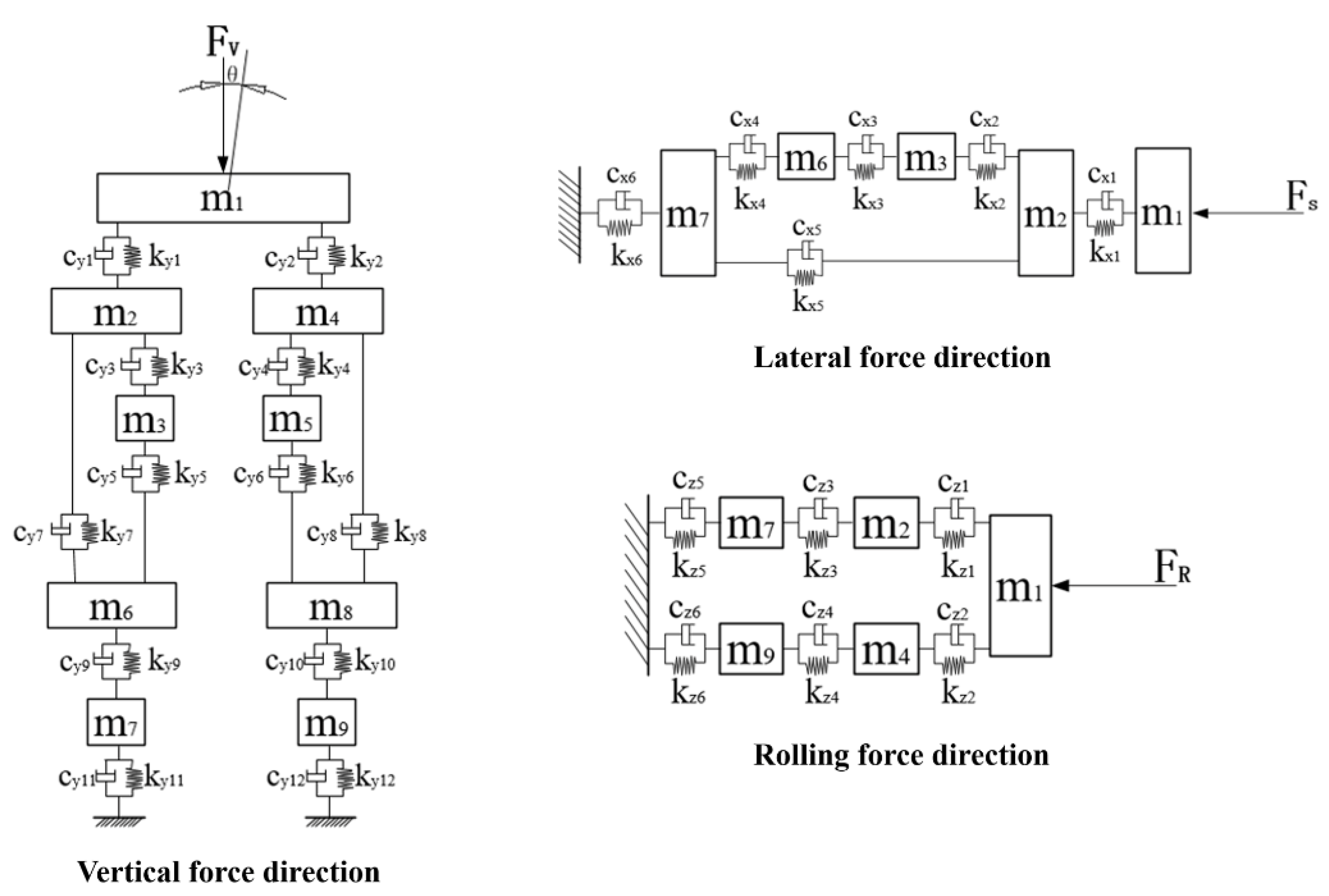
3.1. Equivalent Dynamic Model of New Integrated TBM Cutter System

Since the reaction force loss on the cutter ring in the transmission process of the cutter ring-cutter body-bearing-shaft is negligible, this section studies the disc cutter and holder’s vibration characteristics based on the concentrated mass method. The cutter ring, bearing, cutter body and cutter shaft are equivalented to one mass point, the two cutter side panels are equivalented to two mass points, the two ears are equivalented to two mass points, and the two shift forks are equivalented to two mass points, and the cutter holder wall panel is equivalented to two mass points. Considering the stiffness and damping of the parts simultaneously, establish a multi-DoF coupled cutter system vibration model, and its dynamic equivalent model is shown in Figure 5.

3.2. Dynamic Differential Equations of Vibration System

In this section, the dynamic differential equation of the cutter system will be established based on the equivalent dynamic model. The dynamic differential equation of each component of the cutter system can be expressed by Equation (2):

$$\left[M\right]\left\{\ddot{x}\left(t\right)\right\}+\left[C\right]\left\{\dot{x}\left(t\right)\right\}+\left[K\right]\left\{x\left(t\right)\right\}=\left\{F\left(t\right)\right\}$$

(2)

where [M], [C], and [K] are the mass, damping, and stiffness matrices, which can be obtained by the concentrated mass method, empirical formula, and finite element calculation, respectively.

This cutter system has 20-DoF. Establish a dynamic equation by means of the concentrated mass method and Newton’s second law in this section. According to the balanced relationship between the inertia force, elastic deformation force, damping force, and external excitation, establish the dynamic differential equations of each part in a vertical, lateral, rolling, and swing direction successively, and obtain the dynamic differential equations of this cutter system after a simultaneous establishment.

1.

Vertical direction

$$\{\begin{array}{c}{m}_1{\ddot{y}}_1+\left(c_{y1}+c_{y2}\right){\dot{y}}_1-c_{y1}{\dot{y}}_2-c_{y2}{\dot{y}}_4+\left(k_{y1}+k_{y2}\right)y_1-k_{y1}{y}_2-k_{y2}{y}_4=F_v\hfill \\ m_2{\ddot{y}}_2+\left(c_{y1}+c_{y3}+c_{y7}\right){\dot{y}}_2-c_{y1}{\dot{y}}_1-c_{y3}{\dot{y}}_3-c_{y7}{\dot{y}}_6+\left(k_{y1}+k_{y3}+k_{y7}\right)y_2-\hfill \\ k_{y1}{y}_1-k_{y3}{y}_3-k_{y7}{y}_6=0\\ m_3{\ddot{y}}_3+\left(c_{y3}+c_{y5}\right){\dot{y}}_3-c_{y3}{\dot{y}}_2-c_{y5}{\dot{y}}_6+\left(k_{y3}+k_{y5}\right)y_3-k_{y3}{y}_2-k_{y5}{y}_6=0\hfill \\ m_4{\ddot{y}}_4+\left(c_{y2}+c_{y4}+c_{y8}\right){\dot{y}}_4-c_{y2}{\dot{y}}_1-c_{y4}{\dot{y}}_5-c_{y8}{\dot{y}}_8+\left(k_{y2}+k_{y4}+k_{y8}\right)y_4-\hfill \\ k_{y2}{y}_1-k_{y4}{y}_5-k_{y8}{y}_8=0\\ m_5{\ddot{y}}_5+\left(c_{y4}+c_{y6}\right){\dot{y}}_5-c_{y4}{\dot{y}}_4-c_{y6}{\dot{y}}_8+\left(k_{y4}+k_{y6}\right)y_5-k_{y4}{y}_4-k_{y6}{y}_8=0\hfill \\ m_6{\ddot{y}}_6+\left(c_{y5}+c_{y7}+c_{y9}\right){\dot{y}}_6-c_{y5}{\dot{y}}_3-c_{y7}{\dot{y}}_2-c_{y9}{\dot{y}}_7+\left(k_{y5}+k_{y7}+k_{y9}\right)y_6-\hfill \\ k_{y5}{y}_3-k_{y7}{y}_2-k_{y9}{y}_7=0\\ m_7{\ddot{y}}_7+\left(c_{y9}+c_{y11}\right){\dot{y}}_7-c_{y9}{\dot{y}}_6+\left(k_{y9}+k_{y11}\right)y_7-k_{y9}{y}_6=0\hfill \\ m_8{\ddot{y}}_8+\left(c_{y6}+c_{y8}+c_{y10}\right){\dot{y}}_8-c_{y6}{\dot{y}}_5-c_{y8}{\dot{y}}_4-c_{y10}{\dot{y}}_9+\left(k_{y6}+k_{y8}+k_{y10}\right)y_8-\hfill \\ k_{y6}{y}_5-k_{y8}{y}_4-k_{y10}{y}_9=0\\ m_9{\ddot{y}}_9+\left(c_{y10}+c_{y12}\right){\dot{y}}_9-c_{y10}{\dot{y}}_8+\left(k_{y10}+k_{y12}\right)y_9-k_{y10}{y}_8=0\hfill \end{array}$$

(3)

2.

Lateral direction

$$\{\begin{array}{c}{m}_1{\ddot{x}}_1+c_{x1}{\dot{x}}_1-c_{x1}{\dot{x}}_2+k_{x1}{x}_1-k_{x1}{x}_2=F_s\hfill \\ m_2{\ddot{x}}_2+\left(c_{x1}+c_{x2}+c_{x5}\right){\dot{x}}_2-c_{x1}{\dot{x}}_1-c_{x2}{\dot{x}}_3-c_{x5}{\dot{x}}_7+\hfill \\ \left(k_{x1}+k_{x2}+k_{x5}\right)x_2-k_{x1}{x}_1-k_{x2}{x}_3-k_{x5}{x}_7=0\\ m_3{\ddot{x}}_3+\left(c_{x2}+c_{x3}\right){\dot{x}}_3-c_{x2}{\dot{x}}_2-c_{x3}{\dot{x}}_6+\left(k_{x2}+k_{x3}\right)x_3-k_{x2}{x}_2-k_{x3}{x}_6=0\hfill \\ m_6{\ddot{x}}_6+\left(c_{x3}+c_{x4}\right){\dot{x}}_6-c_{x3}{\dot{x}}_3-c_{x4}{\dot{x}}_7+\left(k_{x3}+k_{x4}\right)x_6-k_{x3}{x}_3-k_{x4}{x}_7=0\hfill \\ m_7{\ddot{x}}_7+\left(c_{x4}+c_{x5}+c_{x6}\right){\dot{x}}_7-c_{x5}{\dot{x}}_2-c_{x4}{\dot{x}}_6+\hfill \\ \left(k_{x4}+k_{x5}+k_{x6}\right)x_7-k_{x5}{x}_2-k_{x4}{x}_6=0\end{array}$$

(4)

3.

Rolling direction

$$\{\begin{array}{c}{m}_1{\ddot{z}}_1+\left(c_{z1}+c_{z2}\right){\dot{z}}_1-c_{z1}{\dot{z}}_2-c_{z2}{\dot{z}}_4+\left(k_{z1}+k_{z2}\right)z_1-k_{z1}{z}_2-k_{z2}{z}_4=F_R\hfill \\ m_2{\ddot{z}}_2+\left(c_{z1}+c_{z3}\right){\dot{z}}_2-c_{z1}{\dot{z}}_1-c_{z3}{\dot{z}}_7+\left(k_{z1}+k_{z3}\right)z_1-k_{z1}{z}_1-k_{z3}{z}_7=0\hfill \\ m_4{\ddot{z}}_4+\left(c_{z2}+c_{z4}\right){\dot{z}}_4-c_{z2}{\dot{z}}_1-c_{z4}{\dot{z}}_9+\left(k_{z2}+k_{z4}\right)z_4-k_{z2}{z}_1-k_{z4}{z}_9=0\hfill \\ m_7{\ddot{z}}_7+\left(c_{z3}+c_{z5}\right){\dot{z}}_7-c_{z3}{\dot{z}}_2+\left(k_{z3}+k_{z5}\right)z_7-k_{z3}{z}_2=0\hfill \\ m_9{\ddot{z}}_9+\left(c_{z4}+c_{z6}\right){\dot{z}}_9-c_{z4}{\dot{z}}_4+\left(k_{z4}+k_{z6}\right)z_9-k_{z4}{z}_4=0\hfill \end{array}$$

(5)

4.

Swing direction

$$J\ddot{{\theta }_1}+C_{\theta }\dot{{\theta }_1}=M$$

(6)

In the above four equations, m_i is the equivalent mass of each mass block, where m₁ is the equivalent mass of the disk cutter; m₂ and m₄ are the equivalent mass of the left and right side panel, respectively; m₆ and m₈ are the equivalent mass of the left and right side of the wedge, respectively; m₃ and m₅ are the equivalent mass of the left and right shift forks and high strength bolts (bolts and shift forks can be regarded as a whole particle through thread connection), respectively; and m₇ and m₉ are the equivalent mass of the left and right inside of cutter holder, respectively.

y_i (i = 1, 2, 3, 4, 5, 6, 7, 8, 9) is the displacement of the mass block in a vertical direction;

x_i (i = 1, 2, 3, 6, 7) is the displacement of the mass block in a lateral direction;

z_i (i = 1, 2, 4, 7, 9) is the displacement of the mass block in a rolling direction;

k_yi (i = 1, 2, 3, 4, 5, 6, 7, 8, 9, 10, 11, 12) is equivalent stiffness of the mass block in a vertical direction;

k_xi (i = 1, 2, 3, 4, 5, 6) is the equivalent stiffness of the mass block in a lateral direction;

k_zi (i = 1, 2, 3, 4, 5, 6) is the equivalent stiffness of the mass block in a rolling direction;

c_yi (i = 1, 2, 3, 4, 5, 6, 7, 8, 9, 10, 11, 12) is the equivalent damping of the mass block in a vertical direction;

c_xi (i = 1, 2, 3, 4, 5, 6) is the equivalent damping of the mass block in a lateral direction;

c_zi (i = 1, 2, 3, 4, 5, 6) is the equivalent damping of the mass block in a rolling direction;

θ₁ is the swing angle of the cutter ring;

J is the rotational inertia in the rolling direction of the disc cutter.

3.3. Structural Dynamic Parameters of the Vibration Model

The 19-inch disc cutter, the most widely used in engineering, is used for the modeling. The cutter’s bearing greatly influences the overall stiffness, so it cannot be solved simply as a single node. Therefore, the cutter is divided into three nodes: the cutter body Z₁, the bearing Z₂, and the cutter shaft Z₃, and then calculate the stiffness, respectively. Then, the overall stiffness of the cutter is equivalent by series.

3.3.1. Equivalent Mass of the Cutter System Components

This section obtained the equivalent mass of each node by using SolidWorks software based on the above dynamic differential equations, as shown in

Table 3. Nodes equivalent mass of cutter system components.

Node	m1 (kg)	m2 (kg)	m3 (kg)	m4 (kg)	m5 (kg)
Equivalent Mass	141.17	77.19	4.57	77.19	4.57
Node	m6 (kg)	m7 (kg)	m8 (kg)	m9 (kg)	m10 (kg)
Equivalent Mass	5.51	156.74	5.51	156.74	0.96

.

3.3.2. Equivalent Stiffness of the Cutter System Components

This section mainly studies the vibration characteristics of the TBM new cutter system, and the components which have the greatest influence on the cutter system will be regarded as a whole, such as a cutter ring, bearing, and cutter shaft. The other components’ influence is too small to be ignored. The stiffness of the W1 cutter body can obtain the stiffness of the whole cutter, the W2 bearing (including the bearing outer ring and bearing half roller), and the W3 cutter shaft (including the cutter shaft and bearing the inner ring) in series.

The stiffness k of the whole disc cutter can be expressed in Equation (7):

$$\frac{1}{k}=\frac{1}{k_{W1}}+\frac{1}{k_{W2}}+\frac{1}{k_{W3}}$$

(7)

This section uses equations to calculate the stiffness of W2, and uses the FEA method to calculate the stiffness of W1 and W3, considering the low accuracy in using equations to calculate the nonstandard parts.

(1)

Equivalent stiffness of node W1

Fix the cutter body’s internal surface, apply a vertical load and lateral load, respectively, on the cutter ring in finite element software, and calculate the cutter body’s deformation results as shown in Figure 6. From the definition of the stiffness and FEA method, the equivalent radial stiffness k_yW1 and axial stiffness k_xW1 of node W1 can be obtained as Equations (8) and (9):

$$k_{yW1}=\frac{F_{ymax}}{{\delta }_{ymax}}=9.06\times {10}^9\left(N/m\right)$$

(8)

$$k_{xW1}=\frac{F_{xmax}}{{\delta }_{xmax}}=1.98\times {10}^9\left(N/m\right)$$

(9)

(2)

Equivalent stiffness of node W2

The bearing is a standard part, and the equivalent radial stiffness k_yW2 and axial stiffness k_xW2 of node W2 can be obtained by Equations (10) and (11):

$$k_{yW2}=1.08\times {10}^9\left(N/m\right)$$

(10)

$$k_{xW2}=2.07\times {10}^9\left(N/m\right)$$

(11)

(3)

Equivalent stiffness of node W3

Fix both ends of the cutter shaft and apply a vertical load during the vertical deformation analysis. Since the lateral load will transfer to the cutter shaft through the end cover, fix the cutter shaft through the side with no end cover and apply the lateral load to the side near the end cover; the calculated deformation results of the cutter body are shown in Figure 7. From the definition of the stiffness and FEA method, the equivalent radial stiffness k_yW3 and axial stiffness k_xW3 of node W3 can be obtained as Equations (12) and (13):

$$k_{yW3}=\frac{F_{ymax}}{{\delta }_{ymax}}=3.02\times {10}^9\left(N/m\right)$$

(12)

$$k_{xW3}=\frac{F_{xmax}}{{\delta }_{xmax}}=6.69\times {10}^9\left(N/m\right)$$

(13)

The vertical and lateral equivalent stiffness of the cutter at node m₁ can be obtained as Equations (14) and (15):

$$k_{y1}=k_{y2}=\frac{1}{2}\times \frac{1}{\frac{1}{k_{yW1}}+\frac{1}{k_{yW2}}+\frac{1}{k_{yW3}}}=3.66\times {10}^8\left(N/m\right)$$

(14)

$$k_{x1}=k_{x2}=\frac{1}{\frac{1}{k_{xW1}}+\frac{1}{k_{xW2}}+\frac{1}{k_{xW3}}}=1.82\times {10}^8\left(N/m\right)$$

(15)

The rolling direction equivalent stiffness of the cutter at node m₁ can be obtained as Equation (16):

$$k_{z1}=k_{z2}=3.24\times {10}^9\left(N/m\right)$$

(16)

The equivalent stiffness of other nodes can be calculated by using the above method, as shown in

Table 4. Vertical direction equivalent stiffness of each node.

Node	ky1	ky2	ky3	ky4	ky5	ky6
Stiffness (N/m)	3.66 × 108	3.66 × 108	2.09 × 109	2.09 × 109	5.64 × 108	5.64 × 108
Node	ky7	ky8	ky9	ky10	ky11	ky12
Stiffness (N/m)	1.52 × 109	1.52 × 109	1.47 × 109	1.47 × 109	2.62 × 1010	2.62 × 1010

,

Table 5. Lateral direction equivalent stiffness of each node.

Node	kx1	kx2	kx3	kx4	kx5	kx6
Stiffness (N/m)	1.82 × 108	5.71 × 108	1.12 × 108	4.62 × 109	5.71 × 108	3.99 × 1010

and

Table 6. Rolling direction equivalent stiffness of each node.

Node	kz1	kz2	kz3	kz4	kz5	kz6
Stiffness (N/m)	3.24 × 109	3.24 × 109	2.38 × 109	2.38 × 109	1.5 × 109	1.5 × 109

.

3.3.3. Equivalent Damping of the Cutter System Components

This section uses equations to calculate the damping of W₂, and uses the empirical formula in the mechanical design manual to calculate the stiffness of W₁ and W₃, which is shown as Equation (17):

$$c=2\xi \sqrt{m_e{k}_e}$$

(17)

In Equation (17), c is the damping of the structural parts and ξ is the damping ratio. In the elastic stage of the steel structure, ξ is generally 0.02~0.05, so we set it to 0.02 in this paper. m_e and k_e are the equivalent mass and equivalent stiffness of each component, respectively.

(1)

Equivalent damping of node W1

The equivalent radial damping c_yW1 and axial damping c_xW1 of node W1 can be obtained as Equations (18) and (19):

$$c_{yW1}=3.25\times {10}^4\left(Ns/m\right)$$

(18)

$$c_{xW1}=1.52\times {10}^4\left(Ns/m\right)$$

(19)

(2)

Equivalent damping of node W2

The equivalent radial damping k_yW2 and axial damping c_xW2 of node W2 can be obtained as Equations (20) and (21):

$$c_{yW2}=6.57\times {10}^3\left(Ns/m\right)$$

(20)

$$c_{xW2}=6.74\times {10}^3\left(Ns/m\right)$$

(21)

(3)

Equivalent damping of node W3

The equivalent radial damping c_yW3 and axial damping c_xW3 of node W3 can be obtained as Equations (22) and (23):

$$c_{yW3}=9.80\times {10}^3\left(Ns/m\right)$$

(22)

$$c_{xW3}=1.46\times {10}^4\left(Ns/m\right)$$

(23)

The vertical and lateral equivalent damping of the cutter at node m₁ can be obtained as Equations (24) and (25):

$$c_{y1}=c_{y2}=\frac{1}{2}\times \frac{1}{\frac{1}{c_{yW1}}+\frac{1}{c_{yW2}}+\frac{1}{c_{yW3}}}=1.75\times {10}^3\left(Ns/m\right)$$

(24)

$$c_{x1}=c_{x2}=\frac{1}{\frac{1}{c_{xW1}}+\frac{1}{c_{xW2}}+\frac{1}{c_{xW3}}}=3.54\times {10}^3\left(Ns/m\right)$$

(25)

The rolling direction equivalent damping of the cutter at node m₁ can be obtained as Equation (26):

$$c_{z1}=c_{z2}=2.71\times {10}^4\left(Ns/m\right)$$

(26)

The calculated each note’s damping results are shown in

Table 7. Vertical direction equivalent damping of each node.

Node	cy1	cy2	cy3	cy4	cy5	cy6
Damping (Ns/m)	1.75 × 103	1.75 × 103	1.61 × 104	1.61 × 104	2.03 × 103	2.03 × 103
Node	cy7	cy8	cy9	cy10	cy11	cy12
Damping (Ns/m)	1.37 × 104	1.37 × 104	3.60 × 103	3.60 × 103	8.11 × 104	8.11 × 104

,

Table 8. Lateral direction equivalent damping of each node.

Node	cx1	cx2	cx3	cx4	cx5	cx6
Damping (Ns/m)	3.54 × 103	8.40 × 103	9.05 × 102	6.38 × 103	8.40 × 103	1.00 × 105

and

Table 9. Rolling direction equivalent damping of each node.

Node	cz1	cz2	cz3	cz4	cz5	cz6
Damping (Ns/m)	2.71 × 104	2.71 × 104	1.71 × 104	1.71 × 104	1.94 × 104	1.94 × 104

.

During the rock-breaking process, the cutter will be subjected to the lateral force due to the rotation of the cutterhead and the squeezing of the blade on the rock, resulting in a torsional pendulum vibration along the Y-axis. The calculation equation for the overturning damping is shown as Equation (27):

$$c_{\theta }=\frac{c_{br}\left(l_1+l_2\right)}{2}$$

(27)

where c_br is the radial damping of the bearing, l₁ and l₂ are the distances from the left and right bearing outer ring to the center of the cutter shaft, respectively, and their lengths are both 0.0375 m. Finally, the c_θ value is 246.53 Nm²/s.

4. Dynamic Characteristics Analysis of New TBM Integrated Cutter System

This chapter will study the dynamic characteristics and load transfer rule of each component of the new TBM cutter system, based on the regular sinusoidal load as the external excitation. To analyze the vibration response rule, use the Newmark method as the main calculation method, meanwhile combine the equivalent stiffness and damping of each component. Calculate the theoretical vertical vibration displacement of the scaled cutter system based on similarity theory, measure the actual vertical vibration displacement through the load experiment, set the actual cutter load obtained from the linear cutting experiment as the external excitation, and study the more realistic vibration response of each component of the cutter system, which ensures that the cutter system can work well under real rock-breaking conditions.

4.1. Vibration Response Solution of Cutter System

4.1.1. Numerical Solution of Differential Equations

There are two main methods for solving dynamic differential equations: the analytical method and numerical method. The analytical method gives the exact solution of the equation. However, the existence of the initial value makes the solution process harder and more complicated when solving the cutter system’s dynamic response. The numerical method obtains the approximate solution of the equation. It is easier to program and solve, even though it is less accurate than the analytical method. Currently, the numerical methods for solving dynamic differential equations mainly include Euler, Newmark, Runge-kutta, Gill, etc. [19,20]. The Newmark method is a direct integration method mainly used to solve the structural dynamic response. It has the characteristics of a fast solving speed and a high accuracy [21]. This section uses the Newmark method to solve the cutter system’s dynamic equation and obtain each component’s time-domain dynamic response.

When α and β, which are the control parameters in the Newmark numerical method, are equal to 0.5 and 0.25, the solution is unconditionally stable and is not affected by the time step Δt. Combined with the dynamic differential equation of the cutter system, the solution process based on the Newmark method is shown in Figure 8.

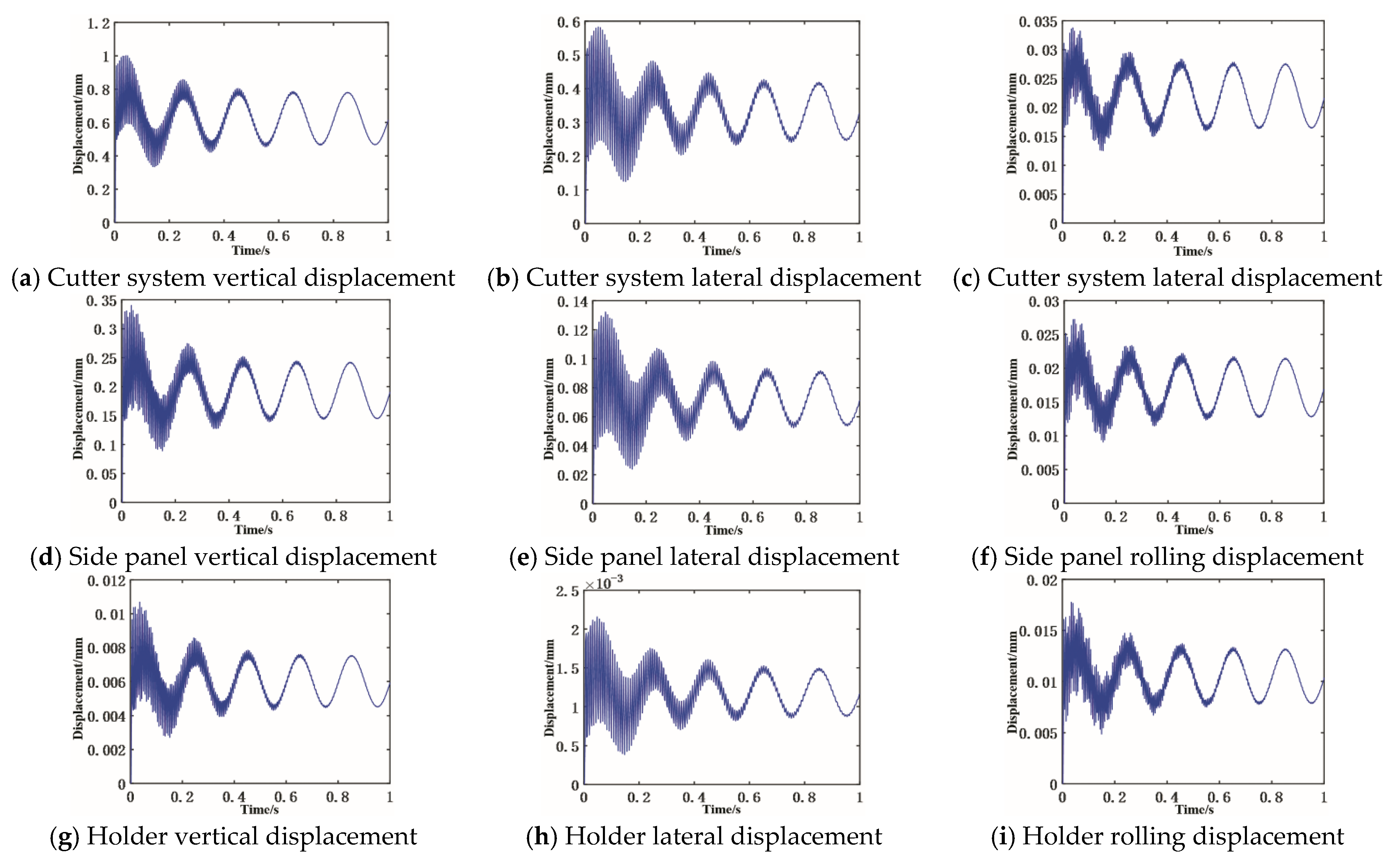
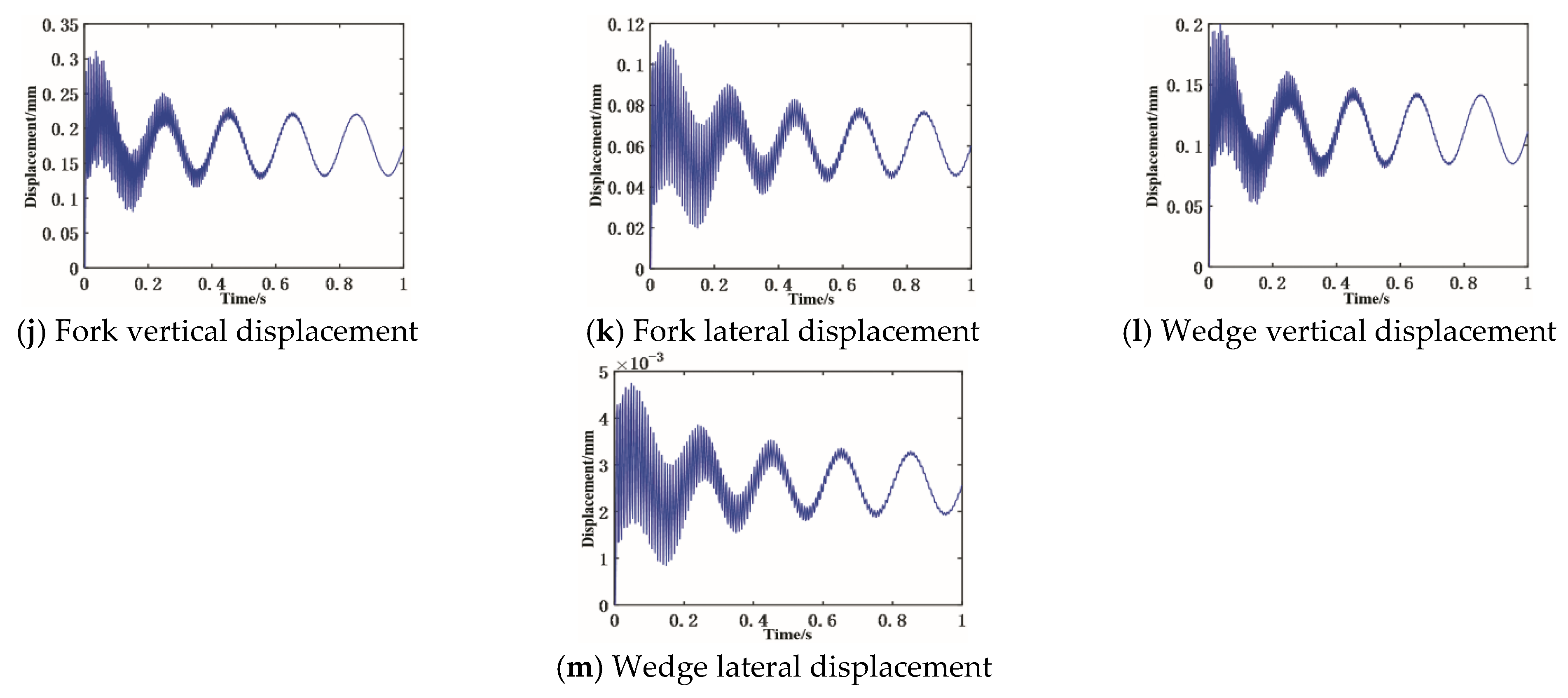
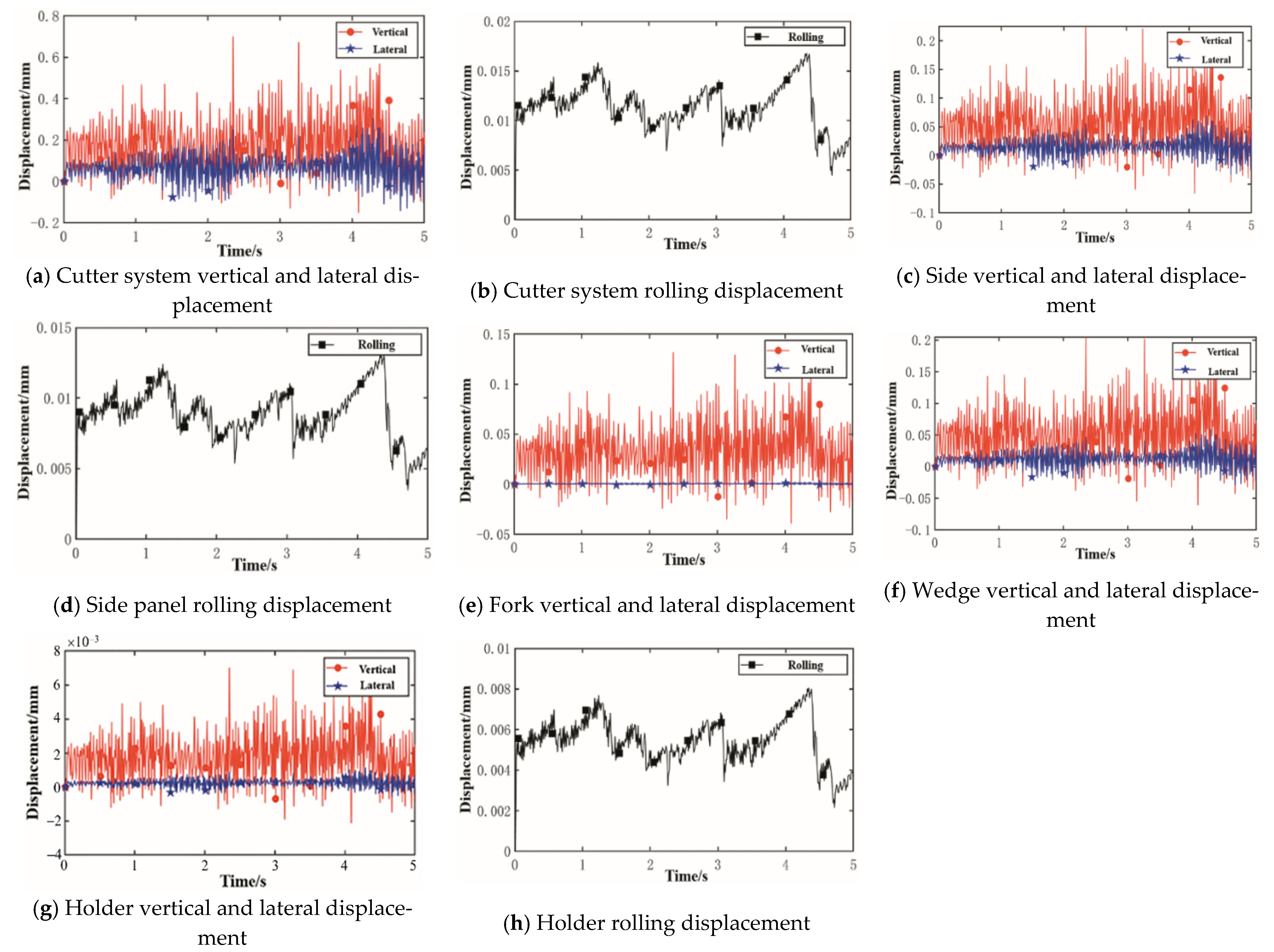
4.1.2. Vibration Response of Each Component

Set the cutters’ nominal load as the mean value of external sinusoidal excitation, set the excitation’s vibration frequency as 5 Hz, and set the vibration amplitude as 25% of the mean value, based on the actual cutters’ impact load. Solve the cutter system’s each component vibration displacement response through the Newmark method, as shown in Figure 9.

The vibration displacement response curve of the disc cutter and holder’s each mass block shows that:

(1)

The new cutter system’s each component response will oscillate in the initial loading stage. In this stage, each component acceleration changes dramatically under the load action, which leads to the reduction in the system’s stability. Under the common influence of internal and external excitation, each component vibration displacement response is the same as that of external load excitation, and the vibration displacement response changes sinusoidal after the stability.

(2)

Order the mean value and amplitude of each component vibration displacement. In the vertical direction: cutter system > disc cutter side panel > shift fork > wedge > cutter holder; in the lateral direction: cutter system > disc cutter side panel > shift fork > wedge > cutter holder; and in the rolling direction: cutter system > cutter side panel > cutter holder. The above shows that each component vibration amplitude decreases gradually along the force transmission direction. Therefore, in the design process, those parts that are prone to failure due to the vibration influence should be placed as far back as possible.

(3)

Order the mean value and amplitude of most of the components’ vibration displacement in different directions: vertical direction > lateral direction > rolling direction, which is caused by the order: vertical load > lateral load > rolling load. The rock-breaking process is mainly carried out by a vertical load, which is one order of magnitude higher than the lateral and rolling load. Therefore, the vertical direction should have a high stiffness during the cutter system design, to ensure the system’s reliability in the rock-breaking process.

4.2. Scaled Vibration Experiment of Cutter System

To measure the vibration displacement of the cutter structure’s key components under the vertical low-frequency load, carry out a vibration characteristic experiment through a dynamic hydraulic loading device in this section to verify the dynamic model’s accuracy.

To avoid the influence of different sample machining errors on the measured data, the vibration loading experiment and the statics experiment use the same scaled cutter system and fixture.

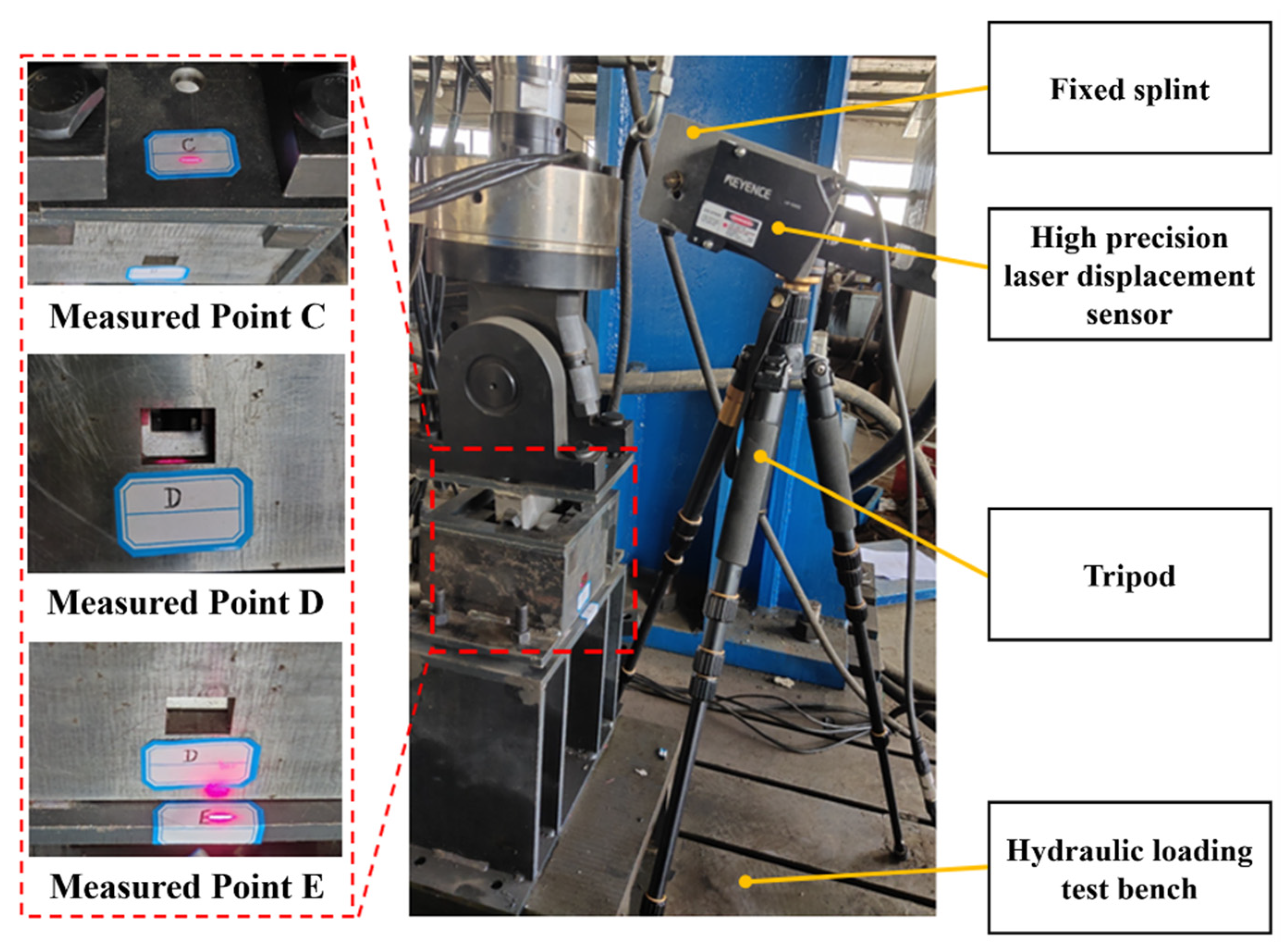
4.2.1. Vibration Loading Experiment Scheme Design

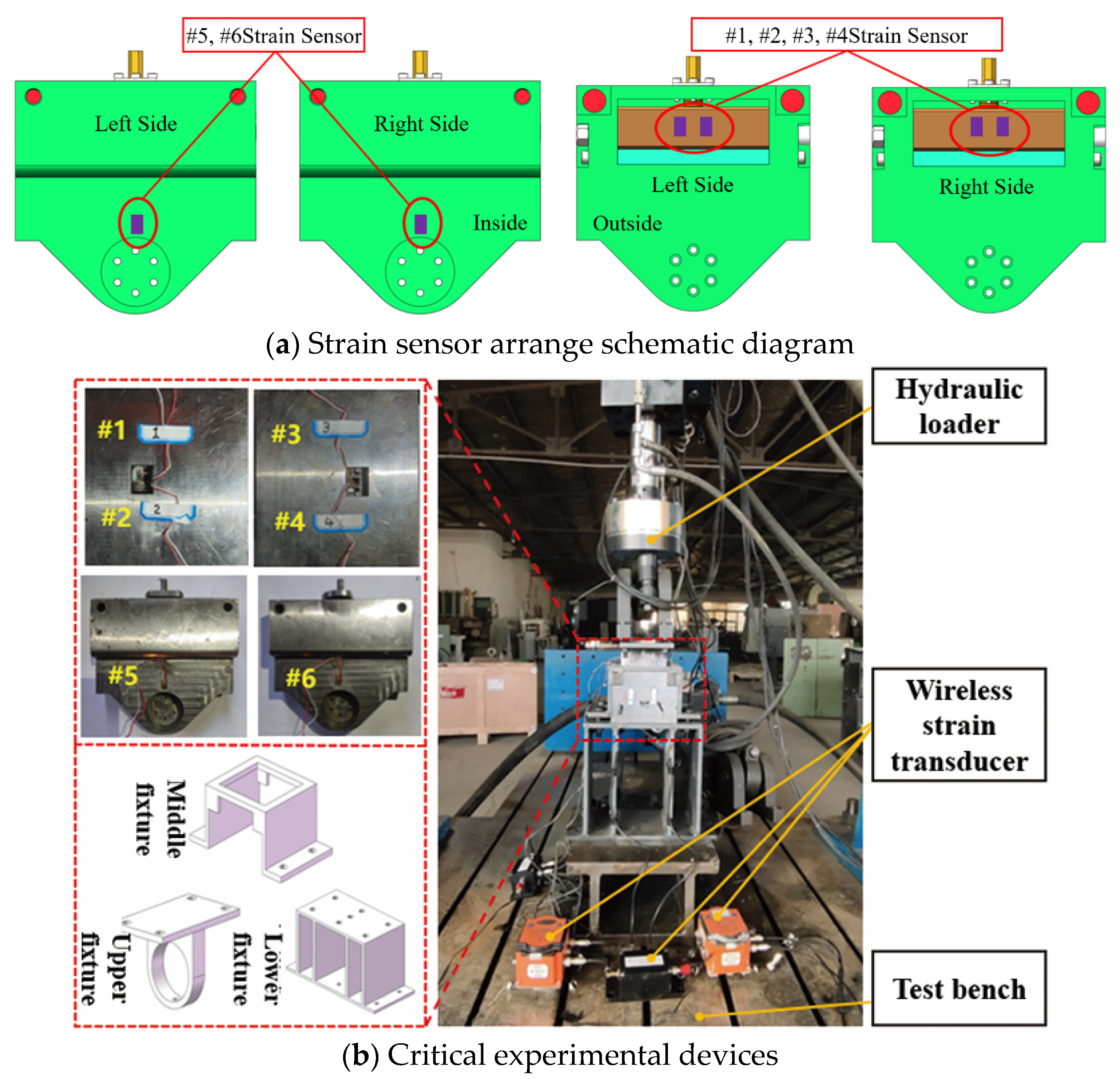
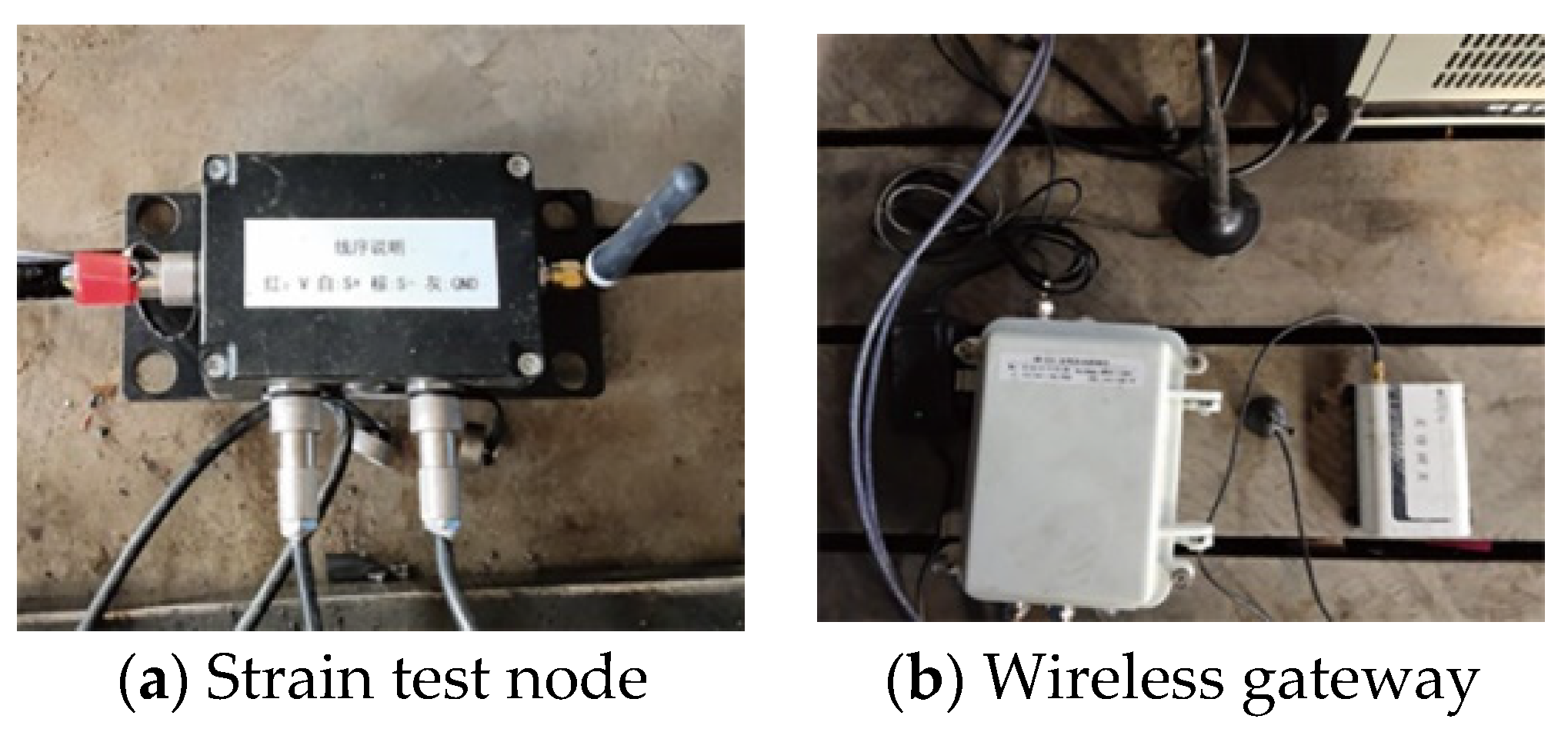
(1)

Critical experimental devices

Figure 10a shows the critical devices of the vibration loading experiment. Number one is the Keyence high-precision displacement sensor (LK-G405), it can measure the displacement of the key points; number two is a laptop computer for the data collection; number three is the processing display for converting the laser pulse signal into the displacement data and displaying it in real-time; number four is a tripod, used to adjust the position and angle of the sensor; and number five is the gantry hydraulic actuator test bench for providing the vibration loads. The overall measurement scheme is shown in Figure 10b.

(2)

Experimental scheme

Fix the scaled cutter system on the test bench, clamp the laser displacement sensor, and adjust the distance between the sensor and measured points to 400 mm.

To improve the vibration displacement measurement accuracy, the measured points should be selected reasonably. Set the point C on the upper fixture’s upper surface, as the cutter is directly connected to the upper fixture through bolts; set the point D on the lower surface in the rectangular groove on the middle of the cutter system’s side panel; and set the point E on the upper surface of the test bench, to eliminate the interference of the test bench vibration to the cutter and holder, which is shown in Figure 11.

According to the schematic design, the experimental steps are as follows: start the laser displacement sensor and irradiate the laser spot on the measured points; start the hydraulic loader and apply the load (average load is 19.6 kN, vibration amplitude is ±20%, frequency is 5 Hz). Use the computer and processing monitor to record the displacement and vibration load data.

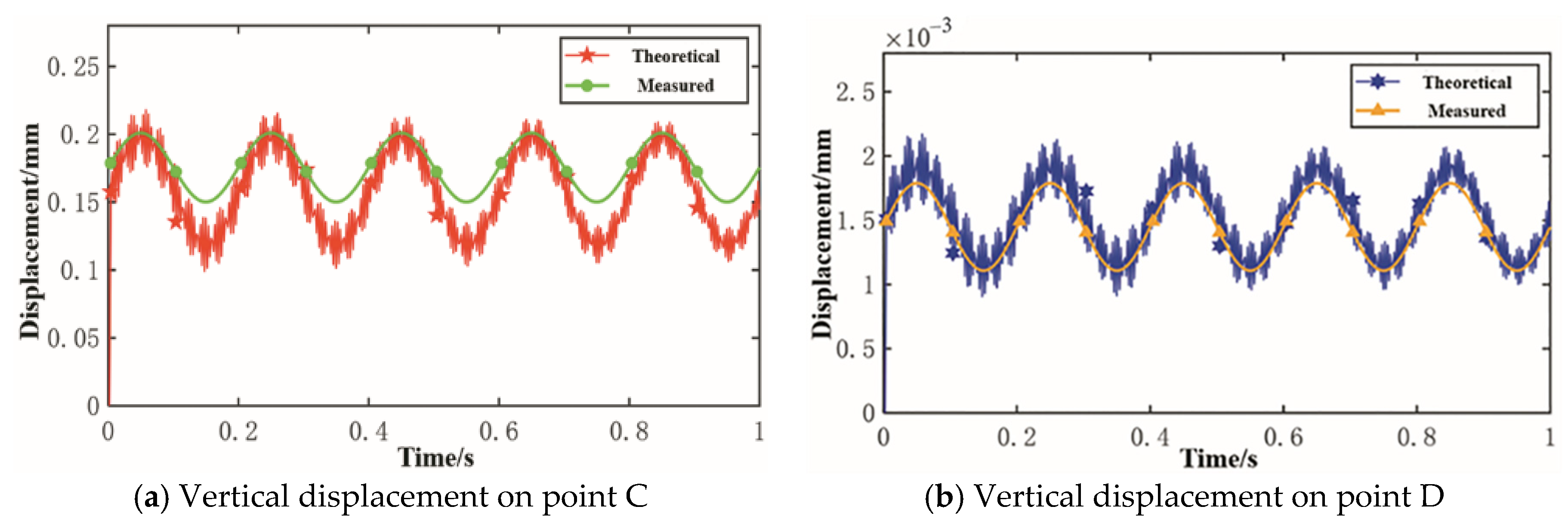
4.2.2. Experimental Results and Comparative Analysis

It is necessary to collect the vibration average value and vibration amplitude data of the 10 cycles, to reduce the measurement error. Use d_C, d_D, and d_E to represent the vertical vibration displacements on point C, point D, and point E. The test bench can also vibrate under external excitation and the vertical displacement of point E can be subtracted from point C and point D to eliminate the influence of test bench vibration. Use d_(C–E) and d_(D–E) to represent the vertical vibration displacement relative to the earth. Detailed displacement data are shown in Figure 12 and

Table 10. Vibration displacement data of measured points.

Parameter	lC/mm	lD/mm	lE/mm	dC/mm
Mean value of vibration	0.450393	0.261204	0.259630	0.414589
Amplitude of vibration	0.042180	0.014945	0.014575	0.038827
Parameter	dD/mm	dE/mm	d(C–E)/mm	d(D–E)/mm
Mean value of vibration	0.240440	0.238991	0.175598	0.001449
Amplitude of vibration	0.013757	0.013417	0.025411	0.000341

. It can be concluded that the relative errors between the measured and theory average vertical displacement values are 9.99% on point C and 5.18% on point D, which can verify the vertical dynamic model’s accuracy.

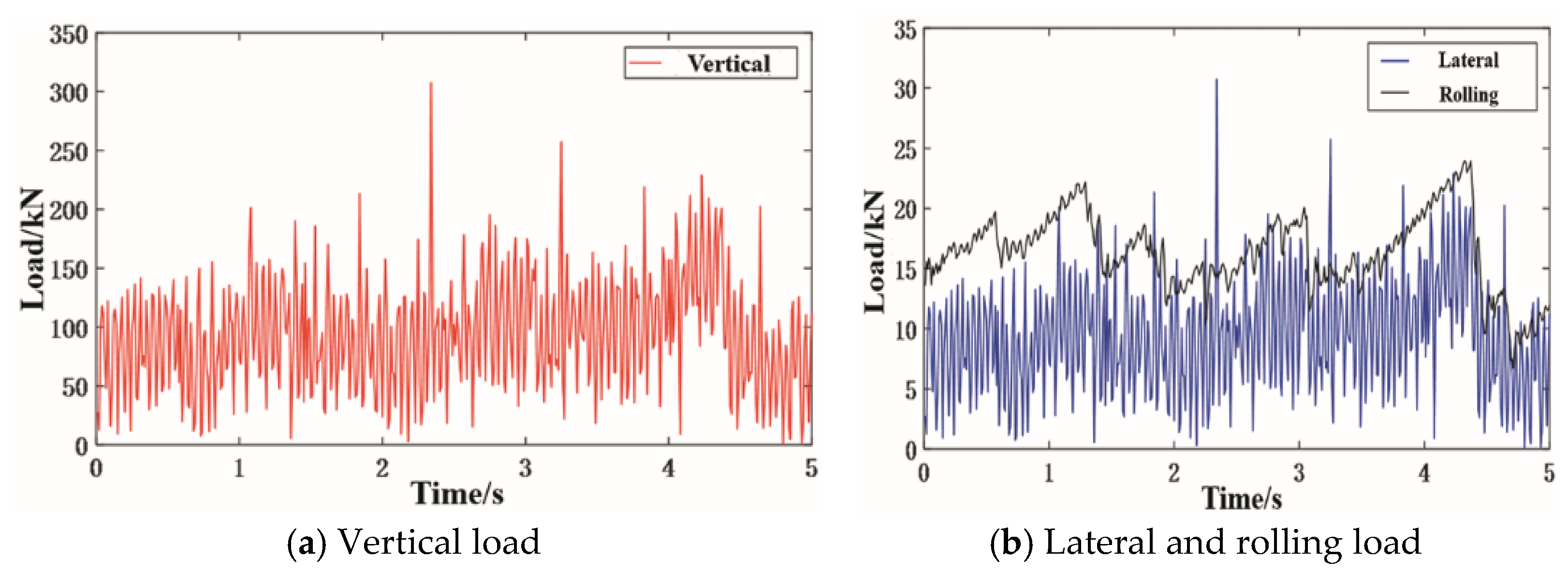
4.3. Dynamic Response of Cutter System Based on Measured Load

Use the actual cutter load obtained from the previous linear cutting experiment as the external excitation to obtain a more real cutter system dynamic response rule of each internal component during the rock-breaking process. The critical experimental devices are shown in Figure 13. The vertical hydraulic cylinder presses the cutter against the rock with great pressure. Meanwhile, the test bench moves horizontally under the horizontal hydraulic cylinder action. Collect the load data in real-time during the rock-breaking process with a triaxial force transducer, which is shown in Figure 14.

Use the Newmark method to solve each component’s vertical, lateral, and rolling vibration displacement responses under the above external excitation, shown in Figure 15 and

Table 11. Displacement response of each component of the system under measured load.

Direction	Parameter	Cutter System	Side Panel	Fork	Wedge	Holder
Vertical	RMS/mm	0.2300	0.0753	0.0688	0.0442	0.0024
	Amplitude/mm	0.7003	0.2246	0.2051	0.1320	0.0070
Lateral	RMS/mm	0.0977	0.0223	0.0188	8.0318 × 10−4	3.6542 × 10−4
	Amplitude/mm	0.3055	0.0701	0.0592	0.0025	0.0011
Rolling	RMS/mm	0.0116	0.0090	/	/	0.0055
	Amplitude/mm	0.0168	0.0131	/	/	0.0081

. The root mean square (RMS) value and amplitude of the components’ vibration displacement decrease along the force transmission path, the maximum value is less than 1 mm, and both appear in the vertical direction. This proves that the disc cutter mainly relies on the vertical impact load to break the rock, and it is necessary to increase the cutter ring’s surface hardness through the heat treatment operation.

5. Conclusions

In this paper, a new TBM integrated cutter system with a simple structure and few disassembly steps is designed, which lays a theoretical foundation for the TBM cutter-changing robot popularization.

(1)

In this paper, the statics performance experiment is carried out first. The finite element method is used to calculate the new cutter system stress distribution under the nominal load, and different values of the load are applied to calculate the position of the maximum stress point, so as to verify the accuracy of the finite element model. A new scaled cutter system is manufactured based on the similarity theory, and the statics performance experiment is carried out. It is found that the relative error between the calculated stress values and the measured stress values is less than 10%, which further verified the accuracy of the finite element model.

(2)

Then, the dynamic characteristics is analyzed. The multi-DoF equivalent mechanical model of the new cutter system and the dynamic differential equations in each direction are established by the concentrated mass method. The vibration response of each cutter system component is solved by the Newmark method. The vibration test of the scale sample is carried out based on the hydraulic loading test bench. It is found that the relative error between the theoretical mean value of the vertical vibration displacement and what is measured is less than 10%, which verifies the correctness of the vertical dynamics model which is established. The real load in the disc cutter linear cutting experiment is used as the external excitation to analyze the dynamic response of each component. The maximum vibration RMS and amplitude of the system are 0.2300 mm and 0.7003 mm, respectively. Additionally, the feasibility of the new cutter system’s design scheme during the real rock breaking process is verified finally.