Determination of the Length of the Rock Bolts for Tunnels with Consideration of the Nonlinear Rheological Behavior of Squeezing Rock
Abstract
:1. Introduction
2. Visco-Elastic-Plastic Constitutive Model for Squeezing Rock
2.1. Derivation of the Komamura-Huang-Bingham Model
2.2. Strain Integration Scheme of the Rheological Model
3. Numerical Model for the Tunnel with Rock Bolts
4. Sensitivity Analyses
4.1. Mechanical Responses of the Rock Bolts
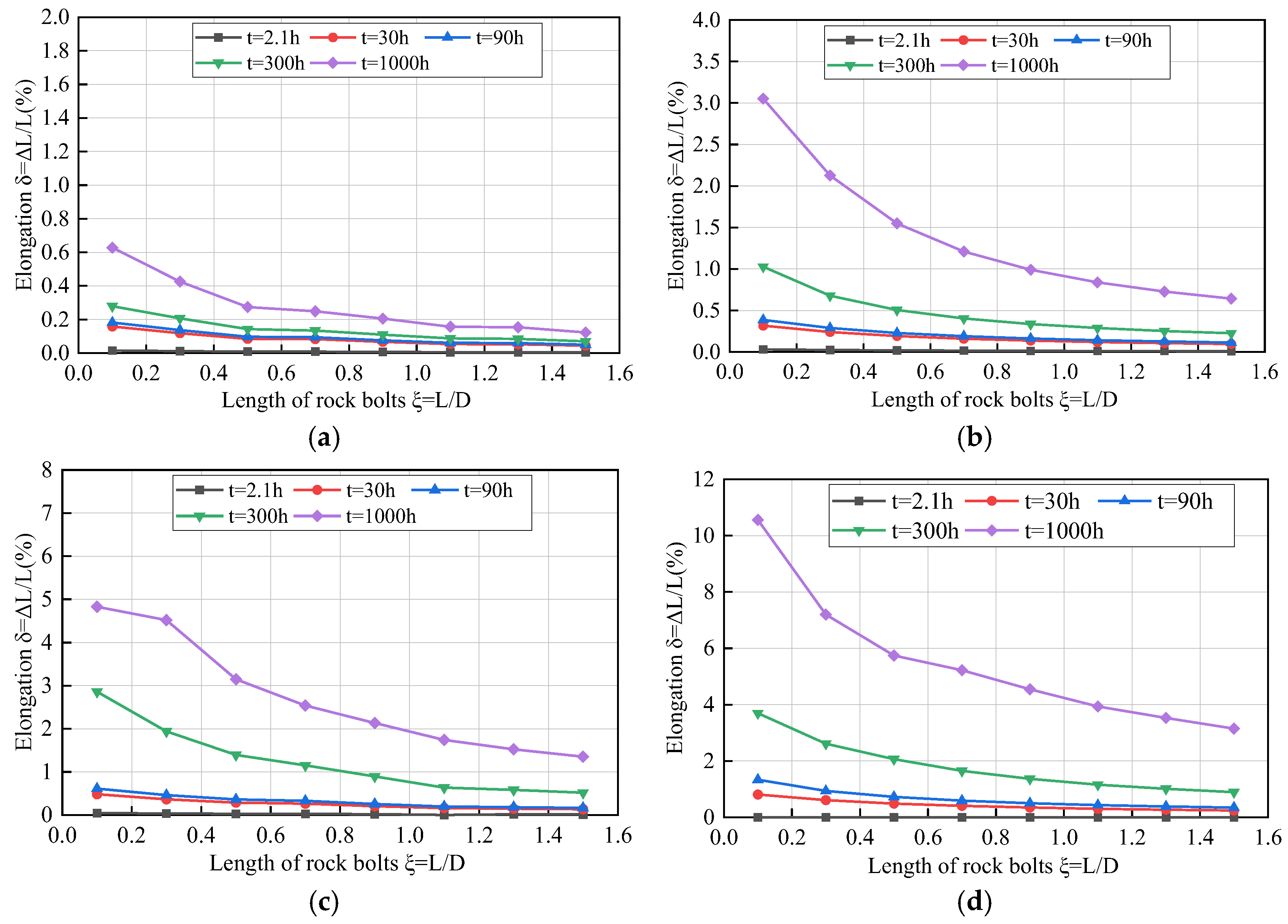
4.1.1. Elongation
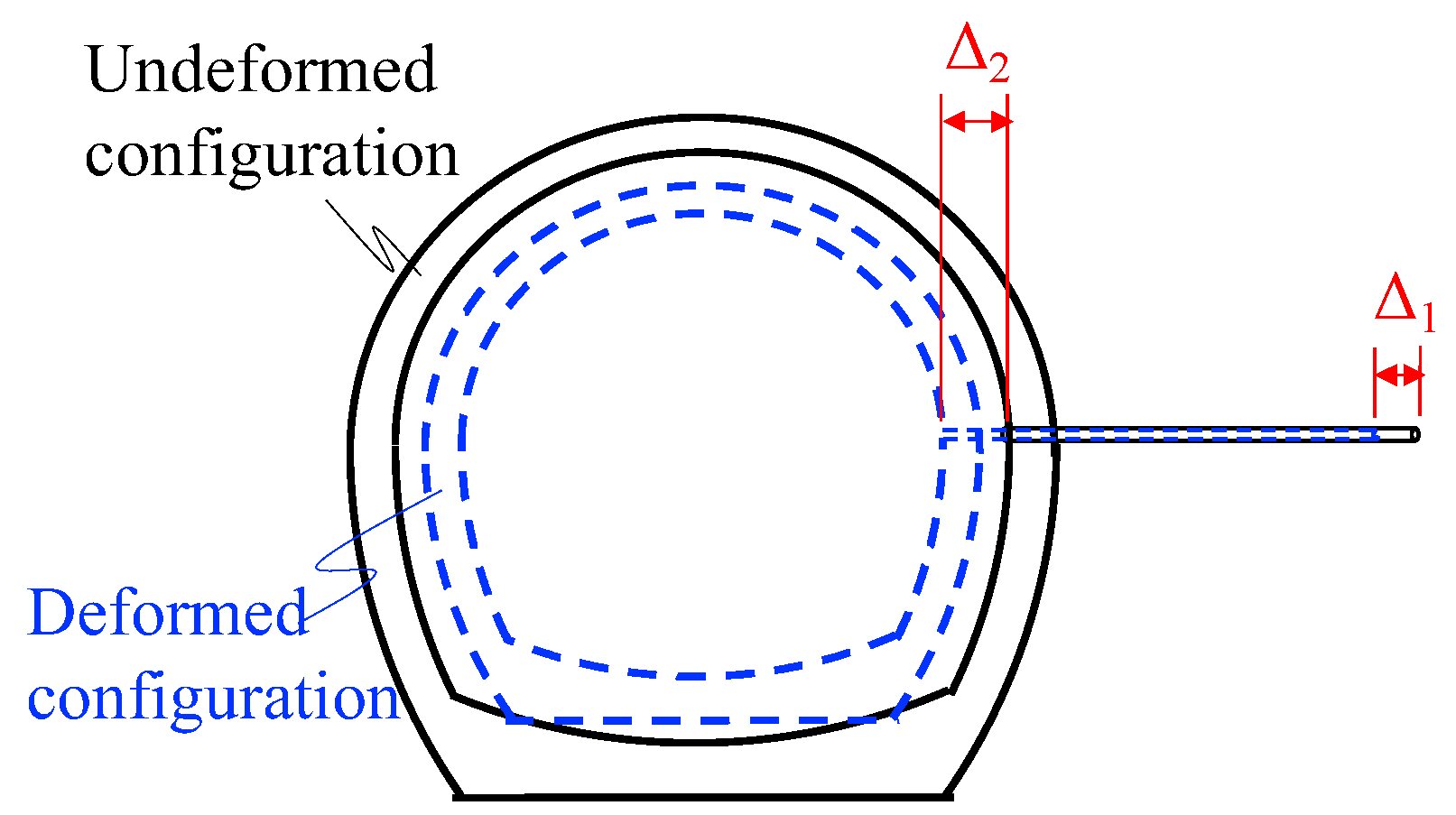
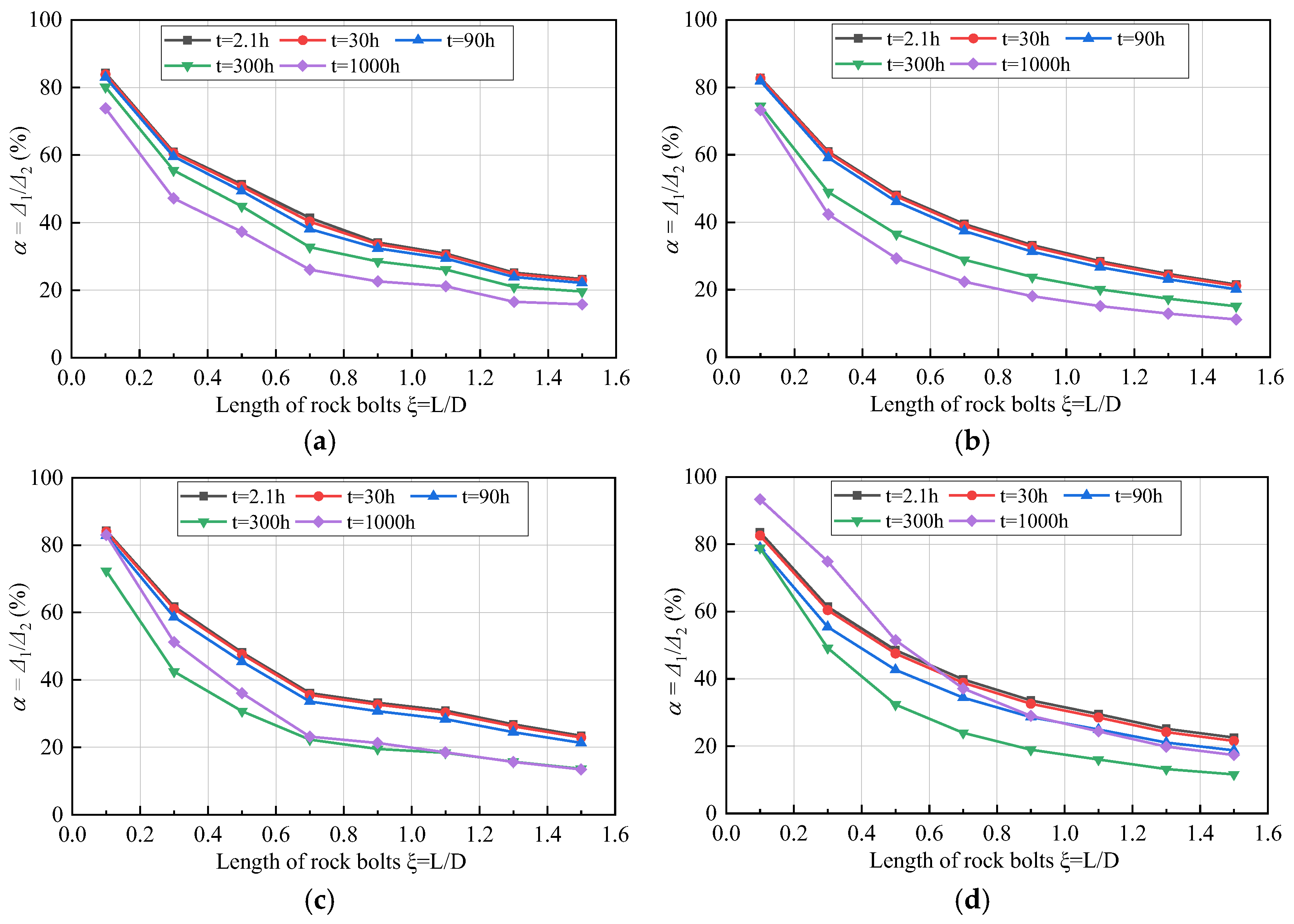
4.1.2. Displacement Ratio
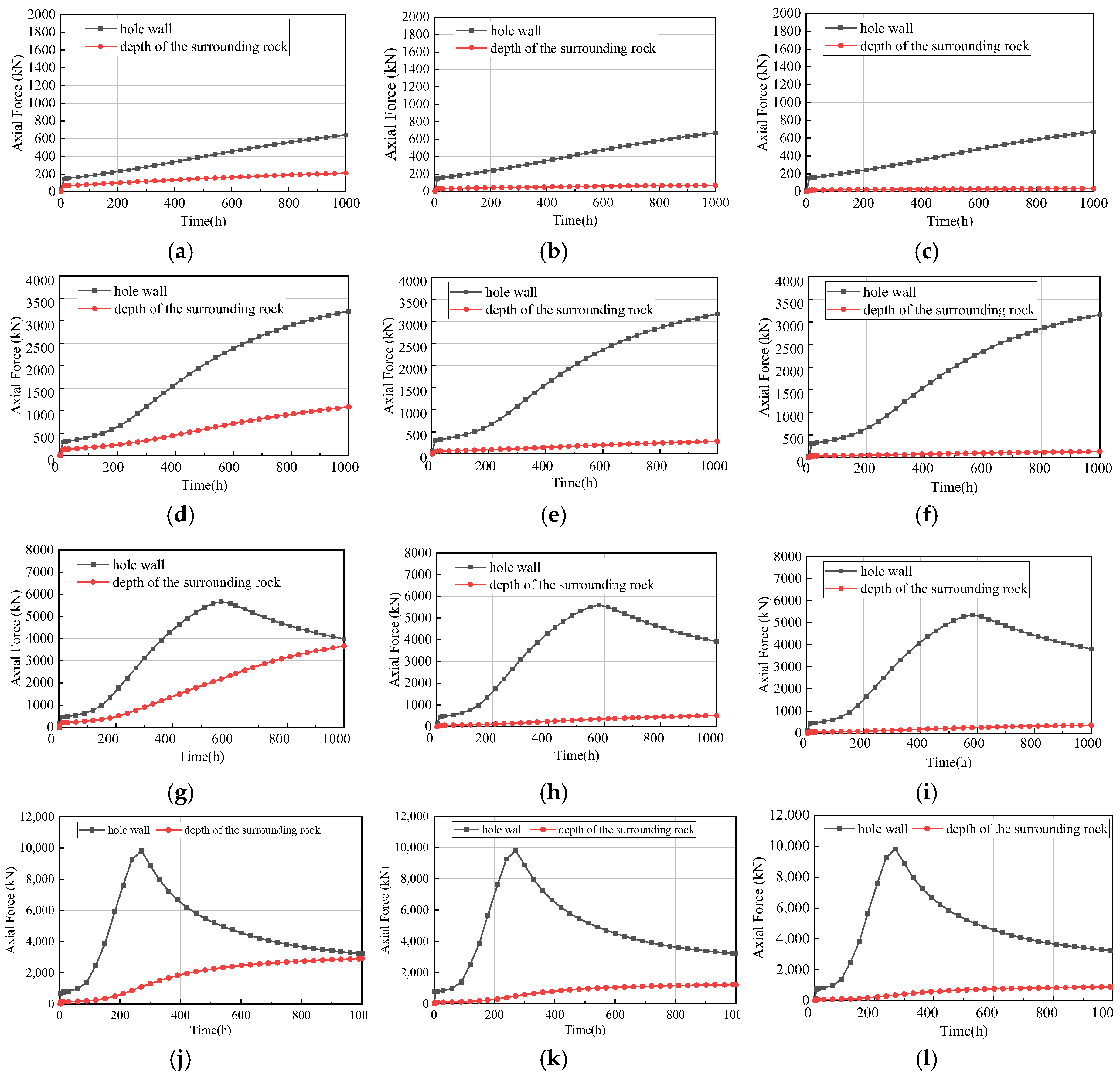
4.1.3. Axial Forces
4.2. Deformation of Surrounding Rock Mass
4.2.1. Plastic Zones
4.2.2. Displacements
5. Discussion
6. Conclusions
- (1)
- When the bolt length exceeds 9 m, the elongation sensitivity to increasing the bolt length is very low. Therefore, keeping the bolt length as long as possible is not advised. Economically, there is an optimal value for the length of the bolt. When the bolt exceeds the threshold, the reinforcement effect of the bolt on the rock mass is insignificant. Such a threshold is approximately equal to the diameter of the investigated tunnel, as in the case of the lateral pressure coefficient being equal to 0.7. As for cases of different lateral pressure coefficients, the optimal length of the bolts must be scrutinized.
- (2)
- In case of few and very severe squeezing problems, corresponding to the Classes A and D, the mechanical response of the tunnels, if only bolts were used for primary support, is insensitive to the selection of rock bolt length. The disturbance effect of the bolt on the rock mass is insignificant. Even with the increase of time, the elongation of the same rock bolt length hardly changes. Even as time increases, the surrounding rock softens, and the strength decreases. As a consequence, a shorter bolt will gradually lose its function, and the anchoring end to the tunnel wall will loosen. Therefore, the anchoring capacity of the bolt will gradually decrease.
- (3)
- The size of the plastic zone is dependent on the in situ stress conditions and the time duration after the tunnel excavation, and, however, independent of the length of the bolts.
- (1)
- Notably, the development of constitutive models of rock has been a hot topic for several decades. Each model has advantages and disadvantages. The associated flow rule used in this paper may lead to excessive dilation. In order to improve this situation, carrying out true triaxial experiments of rocks may provide a way to obtain the yield and the potential surface, resulting in a non-associated flow rule.
- (2)
- It is emphasized that a small strain continuum model was used to model large deformations in this paper. The comparison of the displacement obtained from the simulation and the monitoring on site indicates that the error remains acceptable. However, using a finite strain continuum is a more logical choice for the future work.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Sun, X.; Chen, F.; Miao, C.; Song, P.; Li, G.; Zhao, C.; Xia, X. Physical modeling of deformation failure mechanism of surrounding rocks for the deep-buried tunnel in soft rock strata during the excavation. Tunn. Undergr. Space Technol. 2018, 74, 247–261. [Google Scholar] [CrossRef]
- Lei, M.; Liu, J.; Lin, Y.; Shi, C.; Liu, C. Deformation characteristics and influence factors of a shallow tunnel excavated in soft clay with high plasticity. Adv. Civ. Eng. 2019, 2019, 7483628. [Google Scholar] [CrossRef]
- Guan, K.; Zhu, W.; Wei, J.; Liu, X.; Niu, L.; Wang, X. A finite strain numerical procedure for a circular tunnel in strain–softening rock mass with large deformation. Int. J. Rock Mech. Min. Sci. 2018, 112, 266–280. [Google Scholar] [CrossRef]
- Luo, Y.; Chen, J.; Chen, Y.; Diao, P.; Qiao, X. Longitudinal deformation profile of a tunnel in weak rock mass by using the back analysis method. Tunn. Undergr. Space Technol. 2018, 71, 478–493. [Google Scholar] [CrossRef]
- Zhou, H.; Qu, C.K.; Hu, D.W.; Zhang, C.Q.; Azhar, M.U.; Shen, Z.; Chen, J. In situ monitoring of tunnel deformation evolutions from auxiliary tunnel in deep mine. Eng. Geol. 2017, 221, 10–15. [Google Scholar] [CrossRef]
- Pan, Y.; Chen, L.; Wang, J.; Ma, H.; Cai, S.; Pu, S.; Duan, J.; Gao, L.; Li, E. Research on deformation prediction of tunnel surrounding rock using the model combining firefly algorithm and nonlinear auto-regressive dynamic neural network. Eng. Comput. 2019, 37, 1443–1453. [Google Scholar] [CrossRef]
- Liu, W.; Chen, J.; Chen, L.; Luo, Y.; Shi, Z.; Wu, Y. Nonlinear deformation behaviors and a new approach for the classification and prediction of large deformation in tunnel construction stage: A case study. Eur. J. Environ. Civ. Eng. 2020, 26, 2008–2036. [Google Scholar] [CrossRef]
- Chen, J.; Liu, W.; Chen, L.; Luo, Y.; Li, Y.; Gao, H.; Zhong, D. Failure mechanisms and modes of tunnels in monoclinic and soft–hard interbedded rocks: A case study. KSCE J. Civ. Eng. 2020, 24, 1357–1373. [Google Scholar] [CrossRef]
- Sun, X.; Zhang, B.; Gan, L.; Tao, Z.; Zhao, C. Application of constant resistance and large deformation anchor cable in soft rock highway tunnel. Adv. Civ. Eng. 2019, 2019, 4347302. [Google Scholar] [CrossRef]
- Sun, J.; Jiang, Y.; Wang, B.; Fan, Y. Nonlinear Rheological Properties and Yielding Support Technology for Large Squeezing Deformation of Soft Rock Tunnel with High Ground Stress. Tunn. Constr. 2021, 41, 1627–1633. (In Chinese) [Google Scholar]
- Desai, C.S.; Sane, S.; Jenson, J. Constitutive modeling including creep-and rate-dependent behavior and testing of glacial tills for prediction of motion of glaciers. Int. J. Geomech. 2011, 11, 465–476. [Google Scholar] [CrossRef]
- Yang, S.Q.; Xu, P.; Xu, T. Nonlinear visco–elastic and accelerating creep model for coal under conventional triaxial compression. Geomech. Geophys. Geo-Energy Geo-Resour. 2015, 1, 109–120. [Google Scholar] [CrossRef]
- Sun, J.; Pan, X. Research on large squeezing deformation and its nonlinear rheological mechanical characteristics of tunnel with weak surrounding rocks. Chin. J. Rock Mech. Eng. 2012, 31, 1957–1968. (In Chinese) [Google Scholar]
- Liu, H.Z.; Xie, H.Q.; He, J.D.; Xiao, M.L.; Zhuo, L. Nonlinear creep damage constitutive model for soft rocks. Mech. Time-Depend. Mater. 2017, 21, 73–96. [Google Scholar] [CrossRef]
- Cao, P.; Youdao, W.; Yixian, W.; Haiping, Y.; Bingxiang, Y. Study on nonlinear damage creep constitutive model for highstress soft rock. Environ. Earth Sci. 2016, 75, 900. [Google Scholar] [CrossRef]
- Al-Rub, R.K.A.; Darabi, M.K.; Kim, S.M.; Little, D.N.; Glover, C.J. Mechanistic–based constitutive modeling of oxidative aging in aging–susceptible materials and its effect on the damage potential of asphalt concrete. Constr. Build. Mater. 2013, 41, 439–454. [Google Scholar] [CrossRef]
- Chen, B.-R.; Zhao, X.-J.; Feng, X.-T.; Zhao, H.-B.; Wang, S.-Y. Time-dependent damage constitutive model for the marble in the Jinping II hydropower station in China. Bull. Eng. Geol. Environ. 2013, 73, 499–515. [Google Scholar] [CrossRef]
- Chen, L.; Wang, C.P.; Liu, J.F.; Liu, Y.M.; Liu, J.; Su, R.; Wang, J. A damage–mechanism–based creep model considering temperature effect in granite. Mech. Res. Commun. 2014, 56, 76–82. [Google Scholar] [CrossRef]
- Mazotti, C.; Savoia, M. Nonlinear creep damage model for concrete under uniaxial compression. J. Eng. Mech. 2003, 129, 1065–1075. [Google Scholar] [CrossRef]
- Zhao, Y.-L.; Cao, P.; Wang, W.-J.; Wan, W.; Liu, Y.-K. Viscoelasto-plastic rheological experiment under circular increment step load and unload and nonlinear creep model of soft rocks. J. Cent. South Univ. Technol. 2009, 16, 488–494. [Google Scholar] [CrossRef]
- Sun, J. Rock rheological mechanics and its advance in engineering applications. Chin. J. Rock Mech. Eng. 2007, 26, 1081–1106. (In Chinese) [Google Scholar]
- Zienkiewicz, O.C.; Cormeau, I.C. Visco-plasticity-plasticity and creep in elastic solids-a unified numerical solution approach. Int. J. Numer. Methods Eng. 2010, 8, 821–845. [Google Scholar] [CrossRef]
- Wang, L.G.; He, F.; Liu, X.F.; Yu, Y.J. Nonlinear creep model and stability analysis of rock. Chin. J. Rock Mech. Eng. 2004, 23, 1640–1642. (In Chinese) [Google Scholar]
- Al-Ajmi, A.M.; Zimmerman, R.W. Relation between the Mogi and the Coulomb failure criteria. Int. J. Rock Mech. Min. Sci. 2005, 42, 431–439. [Google Scholar] [CrossRef]
- Cai, W.; Zhu, H.; Liang, W.; Zhang, L.; Wu, W. A New Version of the Generalized Zhang–Zhu Strength Criterion and a Discussion on Its Smoothness and Convexity. Rock Mech. Rock Eng. 2021, 54, 4265–4281. [Google Scholar] [CrossRef]
- Cai, W.; Zhu, H.; Liang, W. Three–dimensional tunnel face extrusion and reinforcement effects of underground excavations in deep rock masses. Int. J. Rock Mech. Min. Sci. 2022, 150, 104999. [Google Scholar] [CrossRef]
- Ma, X.; Haimson, B.C. Failure characteristics of two porous sandstones subjected to true triaxial stresses. J. Geophys. Res. Solid Earth 2016, 121, 6477–6498. [Google Scholar] [CrossRef]
- Mogi, K. Effect of the intermediate principal stress on rock failure. J. Geophys. Res. 1967, 72, 5117–5131. [Google Scholar] [CrossRef]
- Li, J.J.; Zheng, B.L.; Xu, C.Y. Numerical analyses of creep behavior for prestressed anchor rods. Chin. Q. Mech. 2007, 28, 124–128. (In Chinese) [Google Scholar]
- Han, W.; Luan, H.; Liu, C. Assessment of bolt reinforcement effect based on analytic hierarchy process. Geotech. Geol. Eng. 2019, 37, 803–812. [Google Scholar] [CrossRef]
- Wang, H.; Xiao, G.; Jiang, M.; Crosta, G.B. Investigation of rock bolting for deeply buried tunnels via a new efficient hybrid DEM-Analytical model. Tunn. Undergr. Space Technol. 2018, 82, 366–379. [Google Scholar] [CrossRef]
- Wang, W.; Song, Q.; Xu, C.; Gong, H. Mechanical behaviour of fully grouted GFRP rock bolts under the joint action of pre-tension load and blast dynamic load. Tunn. Undergr. Space Technol. 2018, 73, 82–91. [Google Scholar] [CrossRef]
- Sun, J. Rheological Behavior of Geomaterials and Its Engineering Applications; Architecture and Building Press: Beijing, China, 1999; pp. 123–167. (In Chinese) [Google Scholar]
- Pan, X. Study on Squeezing Large Deformation Rheological Mechanical Characteristics of Tunnel Surrounding Rocks. Ph.D. Thesis, Tongji University, Shanghai, China, 2011. [Google Scholar]
- Das, R.; Sirdesai, N.N.; Singh, T.N. Analysis of deformational behavior of circular underground opening in soft ground using three–dimensional physical model. In 51st US Rock Mechanics/Geomechanics Symposium; OnePetro: Richardson, TX, USA, 2017. [Google Scholar]
- Das, R.; Singh, P.K.; Kainthola, A.; Panthee, S.; Singh, T.N. Numerical analysis of surface subsidence in asymmetric parallel highway tunnels. J. Rock Mech. Geotech. Eng. 2017, 9, 170–179. [Google Scholar] [CrossRef]
- Sun, J.; Qin, Y.; Li, N. Nonlinear Rheological Features and Anchoring Technology for Soft Rock Tunnel with Large Squeezing Deformation. Tunn. Constr. 2019, 39, 337–347. (In Chinese) [Google Scholar]
- Hoek, E.; Marinos, P. Predicting tunnel squeezing in weak heterogeneous rock masses. Tunn. Tunn. Int. 2000, 32, 45–51. [Google Scholar]

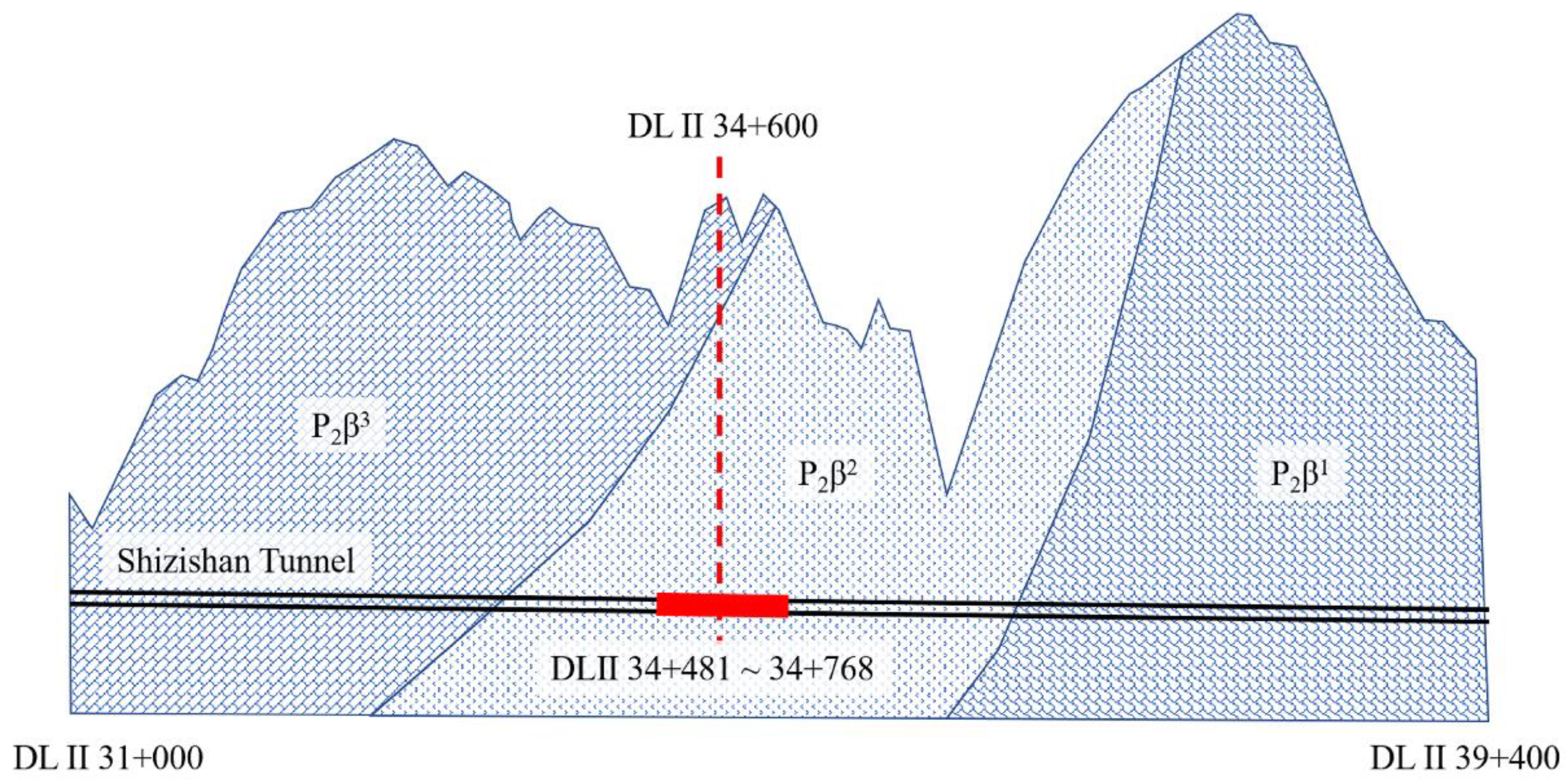
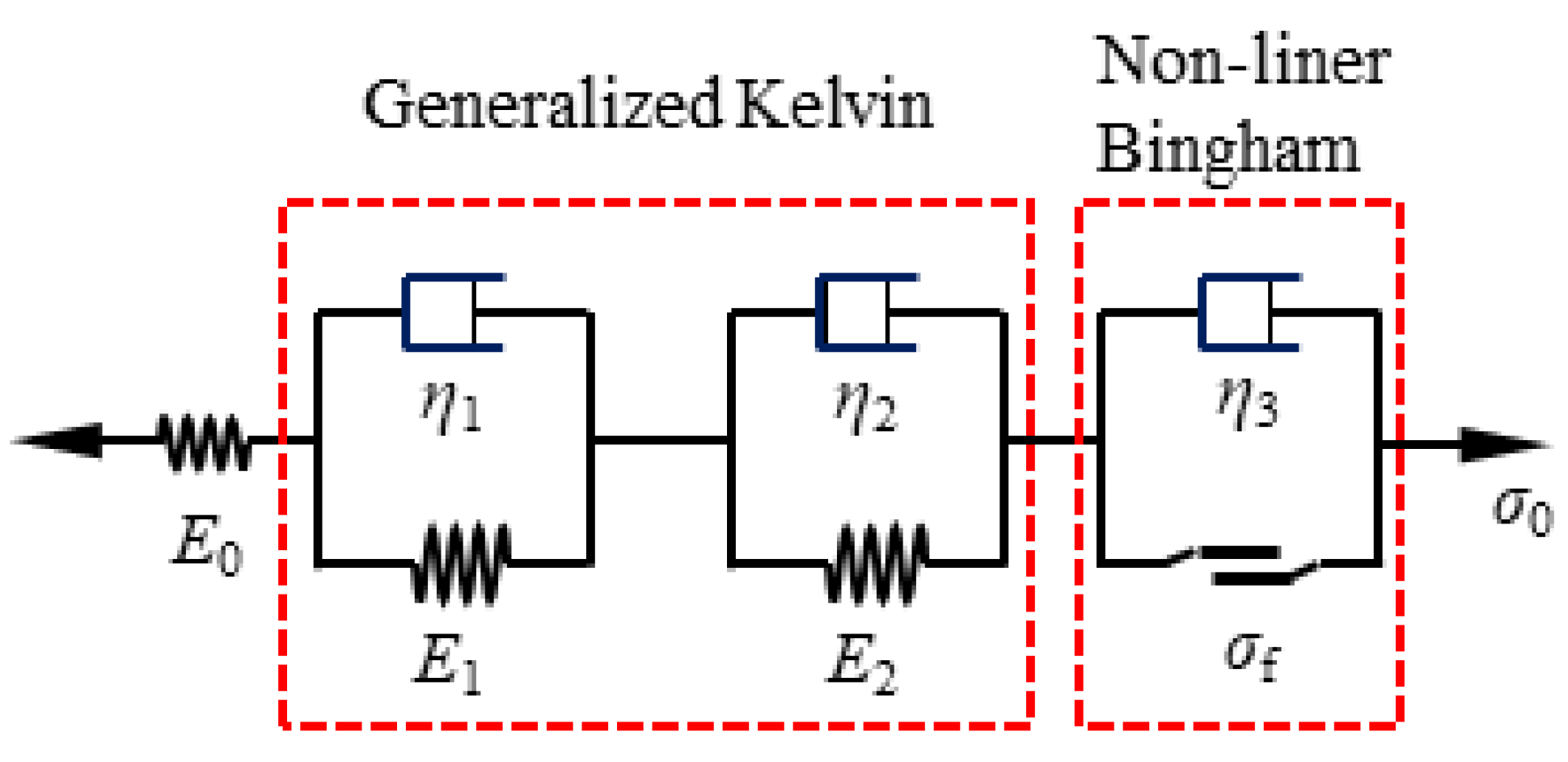
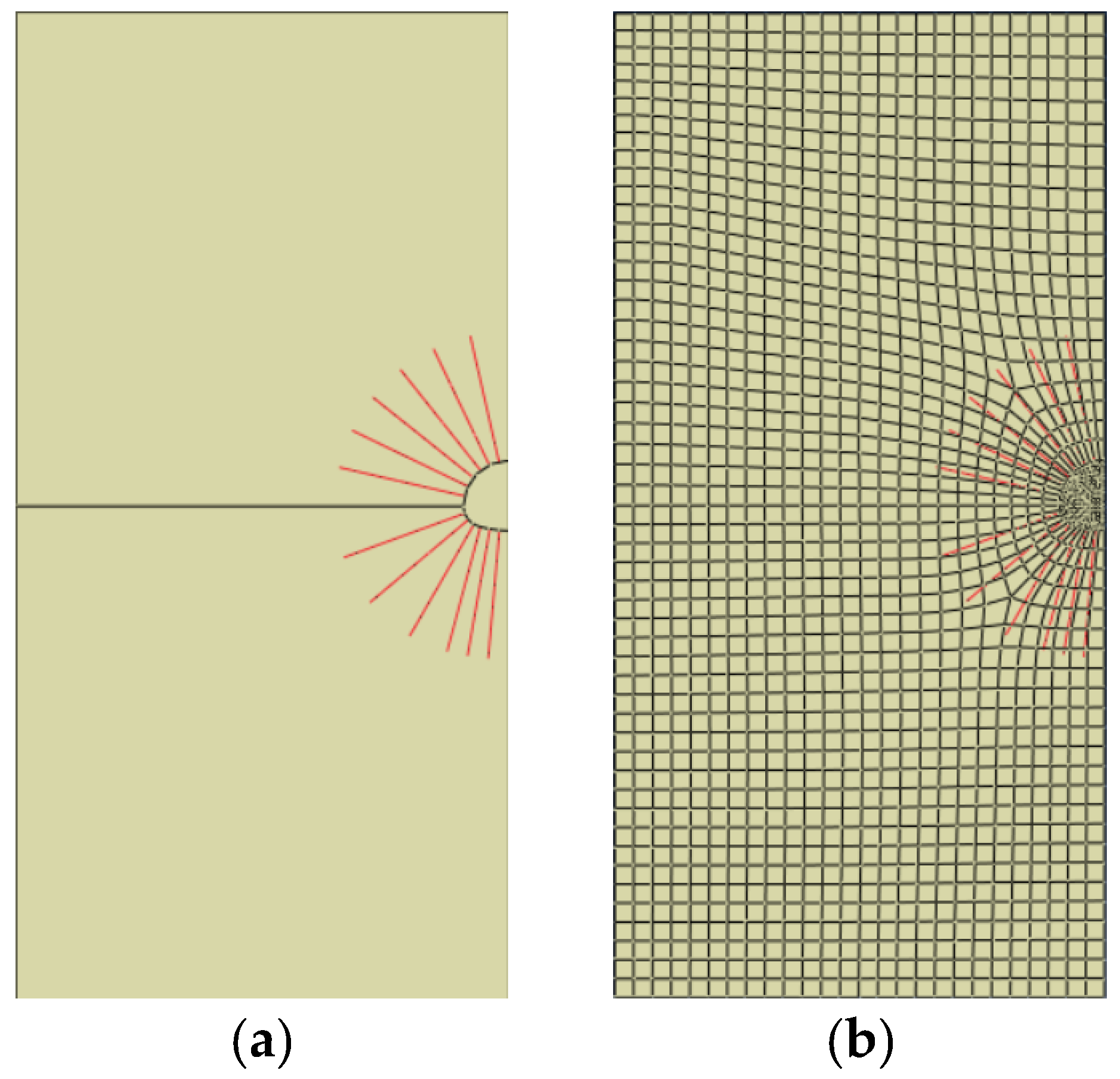
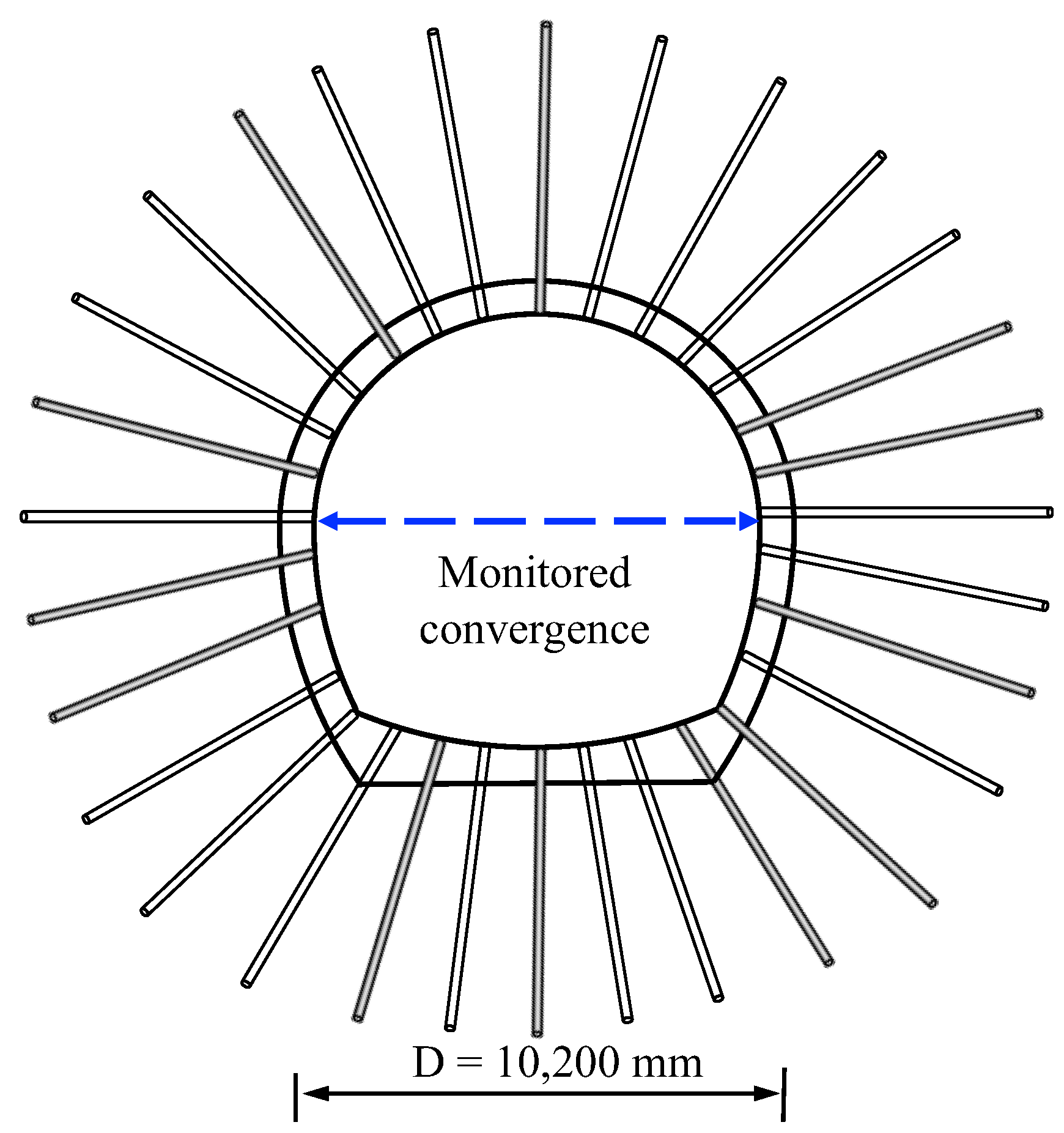

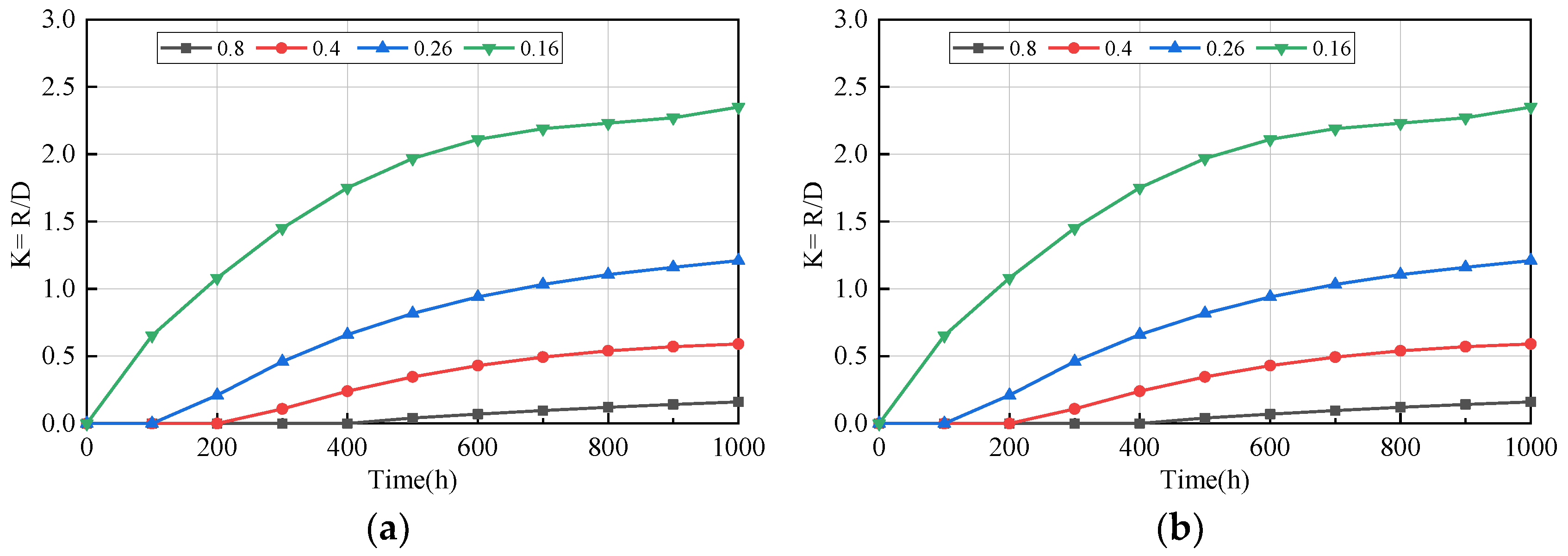
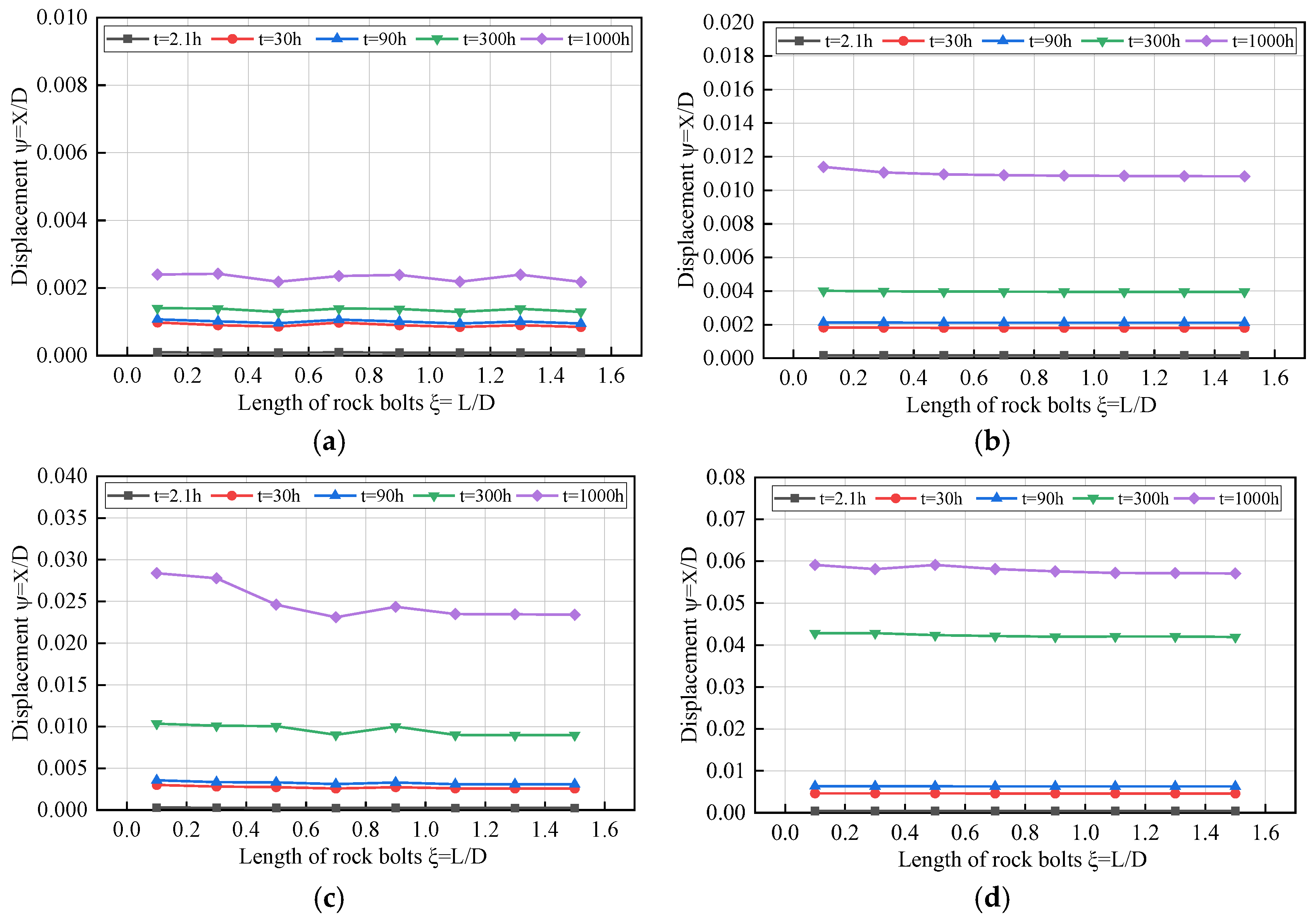
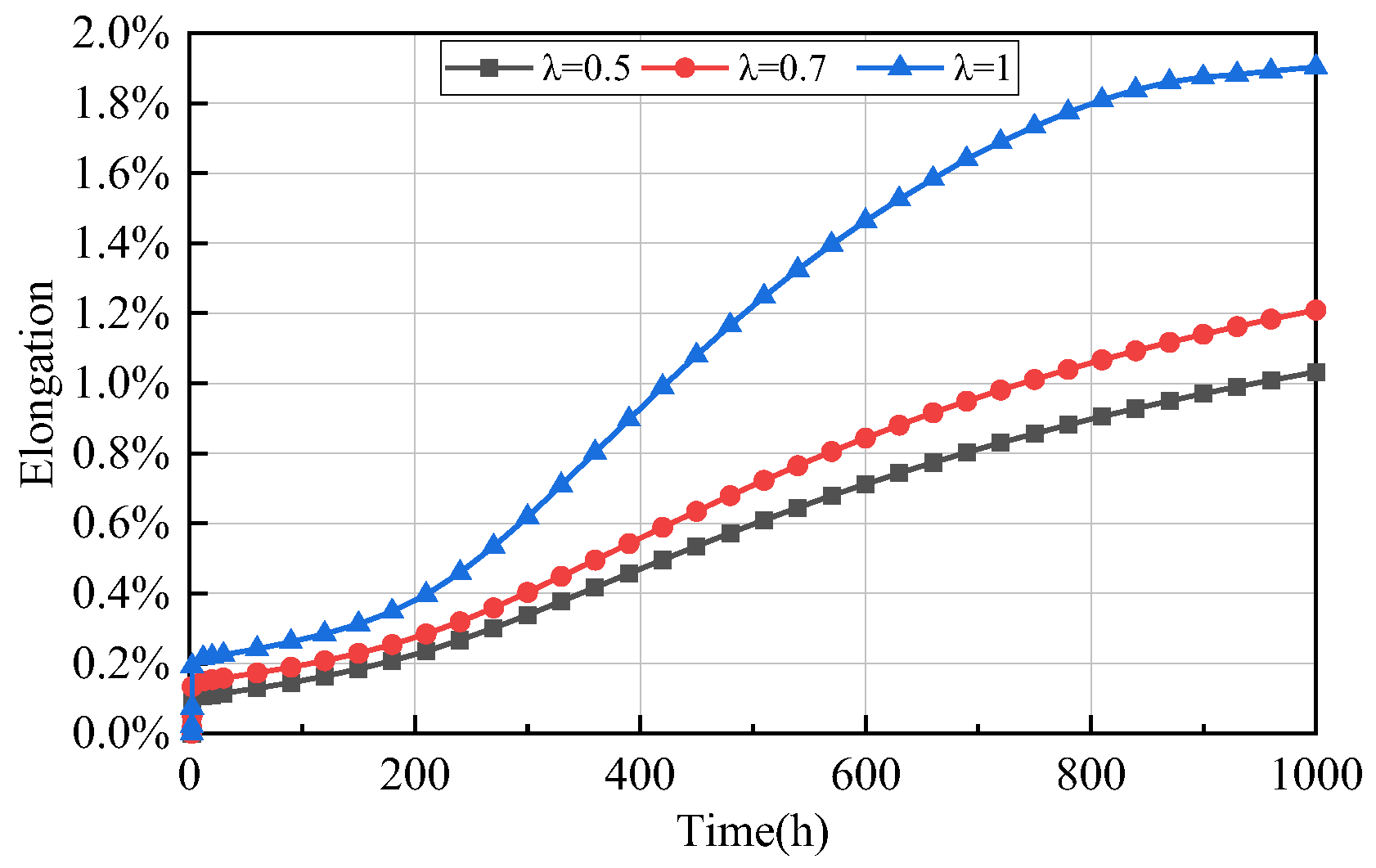
| Tunnel Depth (m) | Strata Type | Lithology | Elasticity Modulus (GPa) | Triaxial Test | Direct Shear Stress Test | Uniaxial Compressive Strength (MPa) | ||
|---|---|---|---|---|---|---|---|---|
| Cohesion (MPa) | Internal Friction Angle (°) | Cohesion (MPa) | Internal Friction Angle (°) | |||||
| 460~490 | Mudstone | J1f | 7.44 | 13.93 | 20.84 | 3.81 | 33.18 | 4.0 |
| μ | C (MPa) | φ (°) | A | T (h) | |||||||
|---|---|---|---|---|---|---|---|---|---|---|---|
| 3.5 | 0.35 | 1 | 33 | 8.32 | 11,200 | 9.16 | 10,400 | 0.3 | 0.6 | 3.8 | 1600 |
| Classes | A | B | C | D | E |
|---|---|---|---|---|---|
| Degree of squeezing problems | Few | Minor | Severe | Very severe | Extreme |
| Strength-to-stress ratios β |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Jiang, Y.; Li, N.; Jiang, H.-H.; Zhou, M.-L.; Zhang, J.-L. Determination of the Length of the Rock Bolts for Tunnels with Consideration of the Nonlinear Rheological Behavior of Squeezing Rock. Appl. Sci. 2022, 12, 8560. https://doi.org/10.3390/app12178560
Jiang Y, Li N, Jiang H-H, Zhou M-L, Zhang J-L. Determination of the Length of the Rock Bolts for Tunnels with Consideration of the Nonlinear Rheological Behavior of Squeezing Rock. Applied Sciences. 2022; 12(17):8560. https://doi.org/10.3390/app12178560
Chicago/Turabian StyleJiang, Yu, Ning Li, Hao-Hong Jiang, Ming-Liang Zhou, and Jiao-Long Zhang. 2022. "Determination of the Length of the Rock Bolts for Tunnels with Consideration of the Nonlinear Rheological Behavior of Squeezing Rock" Applied Sciences 12, no. 17: 8560. https://doi.org/10.3390/app12178560
APA StyleJiang, Y., Li, N., Jiang, H.-H., Zhou, M.-L., & Zhang, J.-L. (2022). Determination of the Length of the Rock Bolts for Tunnels with Consideration of the Nonlinear Rheological Behavior of Squeezing Rock. Applied Sciences, 12(17), 8560. https://doi.org/10.3390/app12178560







