Subduction Evolution Controlled Himalayan Orogenesis: Implications from 3-D Subduction Modeling
Abstract
:Featured Application
Abstract
1. Introduction
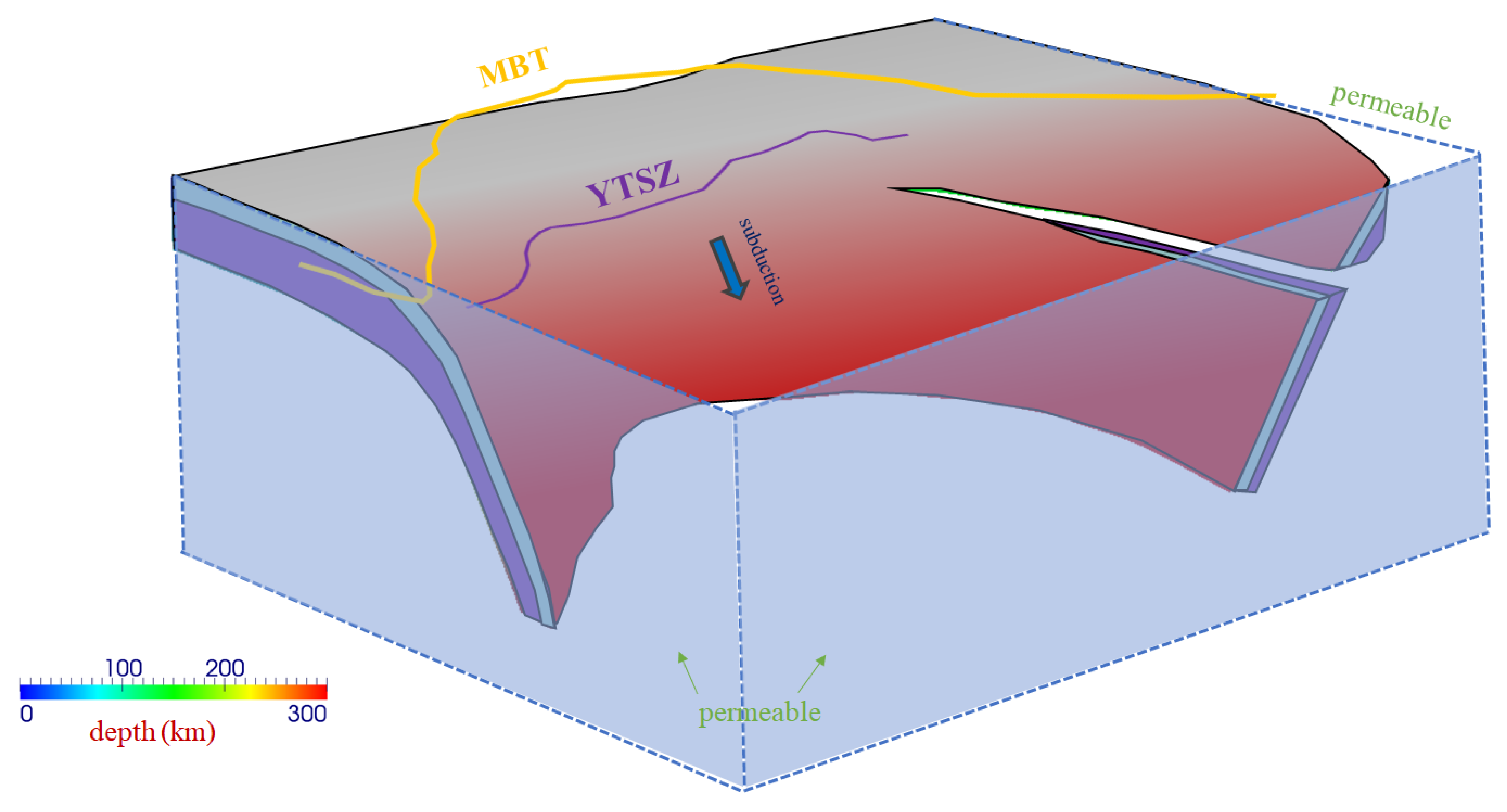
2. Materials and Methods
3. Results
4. Discussion
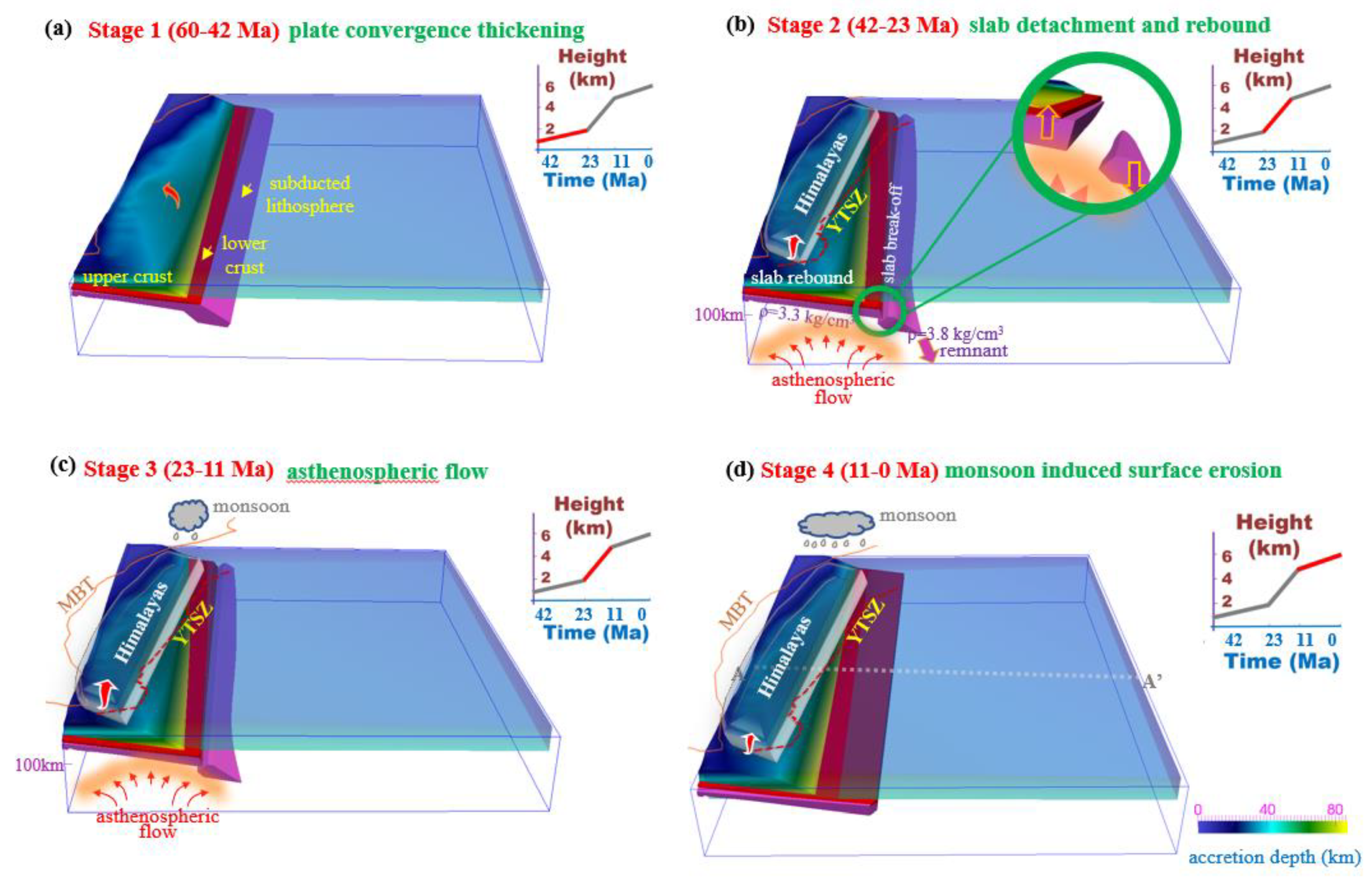
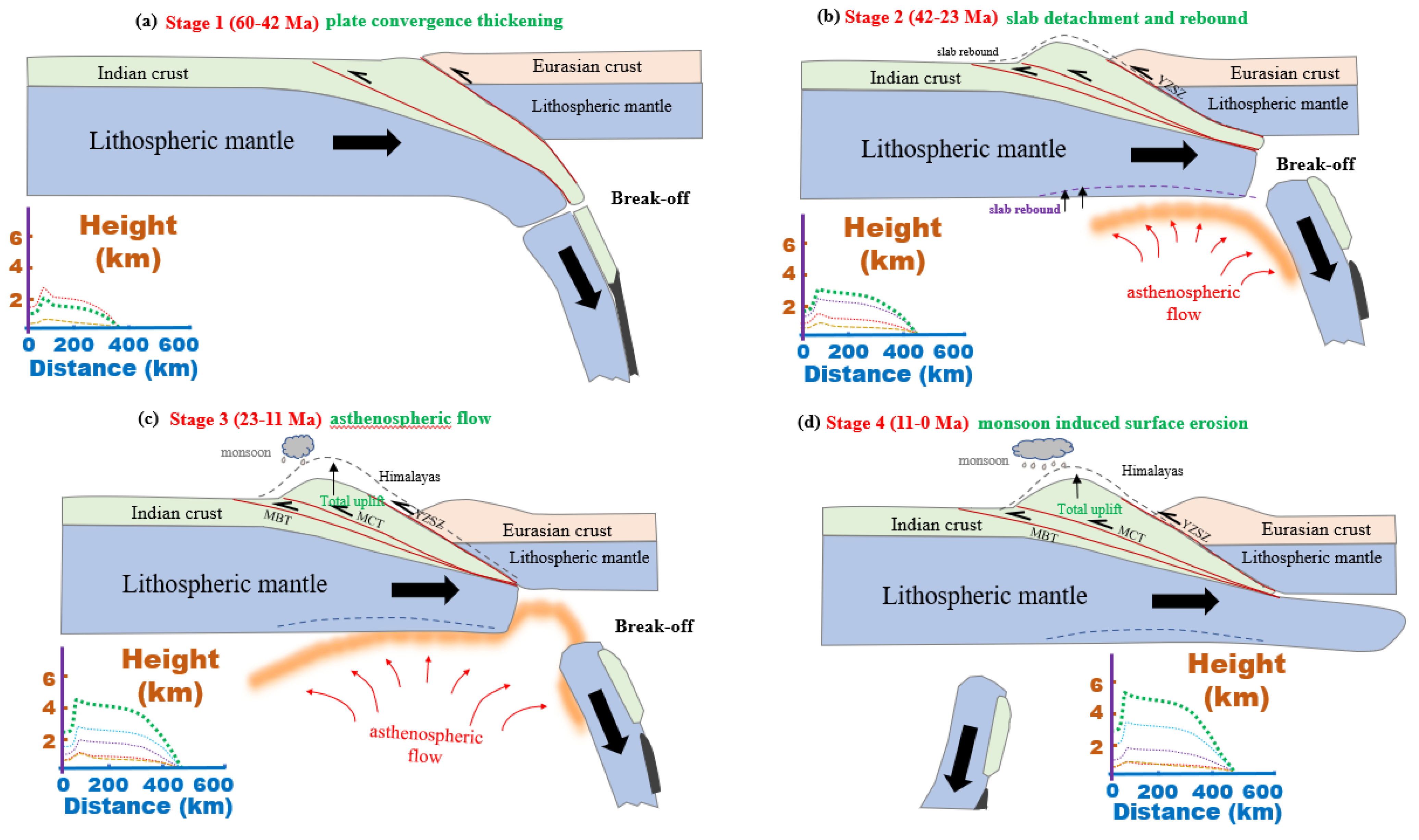
4.1. 3-D Numerical Modeling and Subduction Evolution
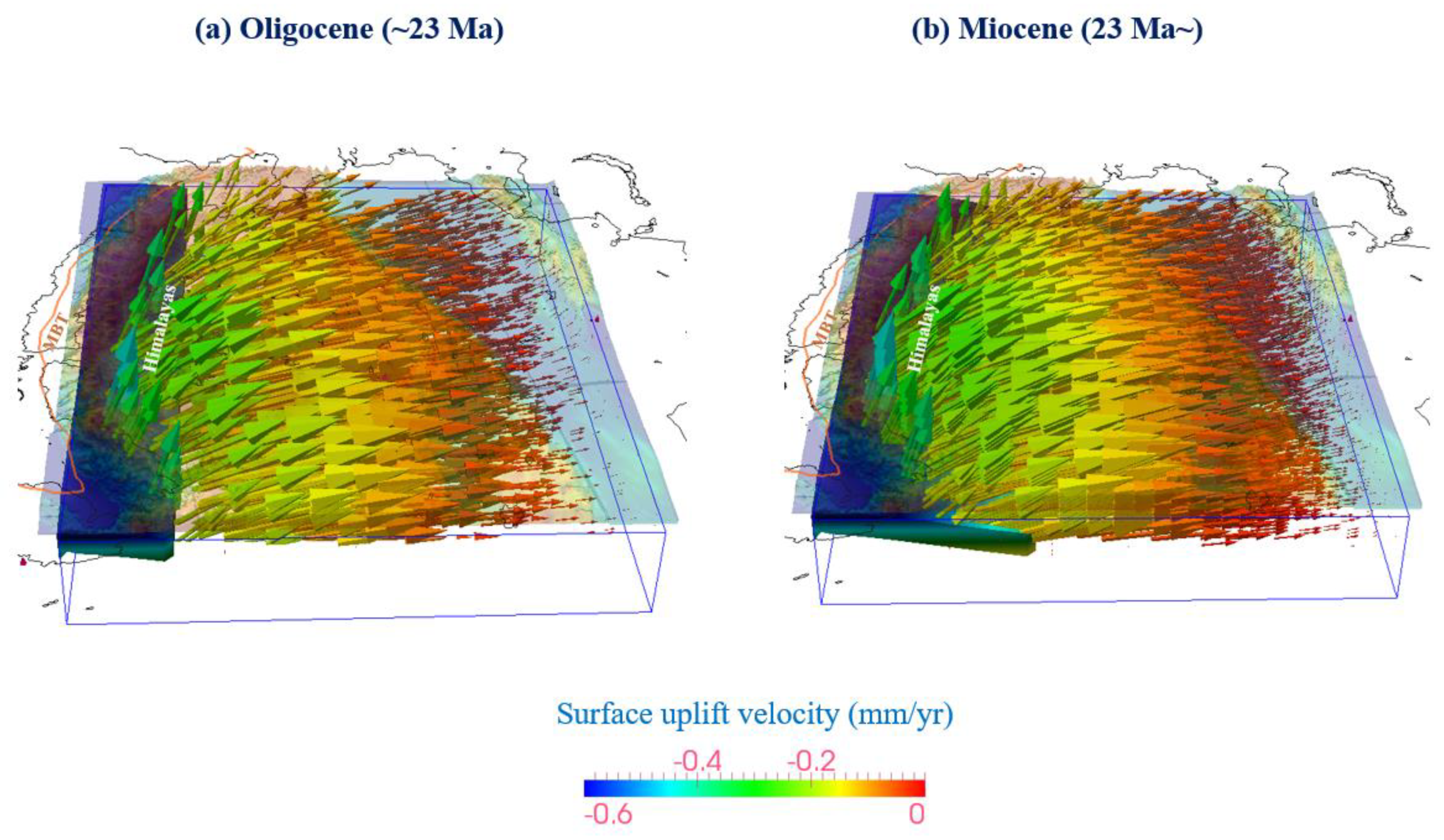
4.2. Slab Detachment and Asthenospheric Flow
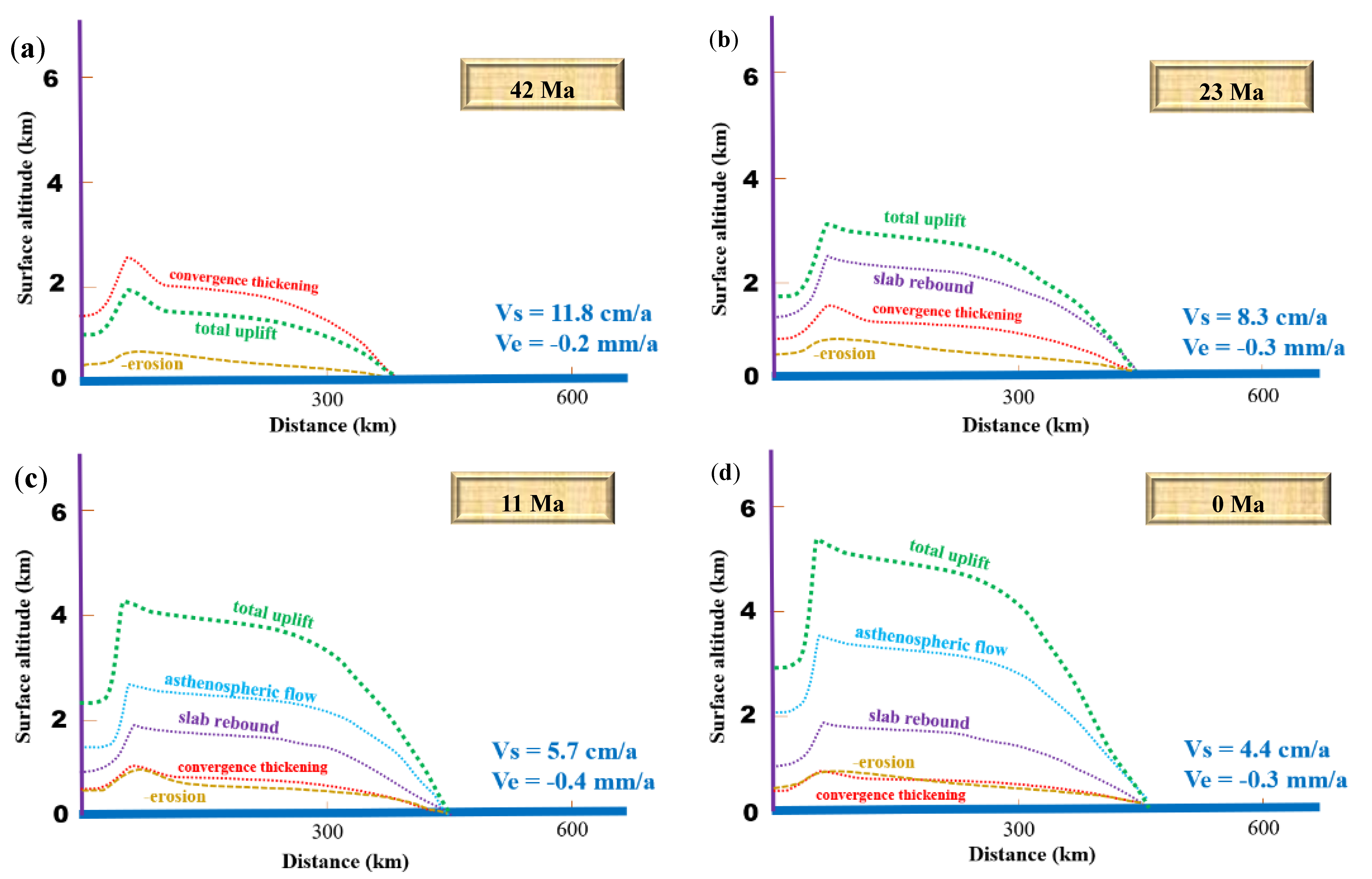
4.3. Orogenic Uplift and Surface Erosion
5. Conclusions
Supplementary Materials
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- DeCelles, P.G.; Kapp, P.; Gehrels, G.E.; Ding, L. Paleocene-Eocene foreland basin evolution in the Himalaya of southern Tibet and Nepal: Implications for the age of initial India-Asia collision. Tectonics 2014, 33, 824–849. [Google Scholar] [CrossRef]
- Takada, Y.; Matsu’ura, M. A unified interpretation of vertical movement in Himalaya and horizontal deformation in Tibet on the basis of elastic and viscoelastic dislocation theory. Tectonophysics 2004, 383, 105–131. [Google Scholar] [CrossRef]
- Ding, L.; Maksatbek, S.; Cai, F.; Wang, H.; Song, P.; Ji, W.; Xu, Q.; Zhang, L.; Muhammad, Q.; Upendra, B. Processes of initial collision and suturing between India and Asia. Sci. China Earth Sci. 2017, 60, 635–651. [Google Scholar] [CrossRef]
- Ding, L.; Spicer, R.; Yang, J.; Xu, Q.; Cai, F.; Li, S.; Lai, Q.; Wang, H.; Spicer, T.; Yue, Y. Quantifying the rise of the Himalaya orogen and implications for the South Asian monsoon. Geology 2017, 45, 215–218. [Google Scholar] [CrossRef] [Green Version]
- Dey, S.; Thiede, R.C.; Biswas, A.; Chauhan, N.; Chakravarti, P.; Jain, V. Implications of the ongoing rock uplift in NW Himalayan interiors. Earth Surf. Dyn. 2021, 9, 463–485. [Google Scholar] [CrossRef]
- Li, Y.; Wang, C.; Dai, J.; Xu, G.; Hou, Y.; Li, X. Propagation of the deformation and growth of the Tibetan–Himalayan orogen: A review. Earth-Sci. Rev. 2015, 143, 36–61. [Google Scholar] [CrossRef]
- Wang, W.; Pandit, M.K.; Zhao, J.-H.; Chen, W.-T.; Zheng, J.-P. Slab break-off triggered lithosphere-asthenosphere interaction at a convergent margin: The Neoproterozoic bimodal magmatism in NW India. Lithos 2018, 296, 281–296. [Google Scholar] [CrossRef]
- Jolivet, L.; Faccenna, C.; Becker, T.; Tesauro, M.; Sternai, P.; Bouilhol, P. Mantle flow and deforming continents: From India-Asia convergence to Pacific subduction. Tectonics 2018, 37, 2887–2914. [Google Scholar] [CrossRef]
- Webb, A.A.G.; Guo, H.; Clift, P.D.; Husson, L.; Müller, T.; Costantino, D.; Yin, A.; Xu, Z.; Cao, H.; Wang, Q. The Himalaya in 3D: Slab dynamics controlled mountain building and monsoon intensification. Lithosphere 2017, 9, 637–651. [Google Scholar] [CrossRef]
- Ji, Y.; Yan, R.; Zeng, D.; Xie, C.; Zhu, W.; Qu, R.; Yoshioka, S. Slab dehydration in Sumatra: Implications for fast and slow earthquakes and arc magmatism. Geophys. Res. Lett. 2021, 48, e2020GL090576. [Google Scholar] [CrossRef]
- Tackley, P.; Xie, S. STAG3D: A code for modeling thermo-chemical multiphase convection in Earth’s mantle. In Computational Fluid and Solid Mechanics 2003; Elsevier: Amsterdam, The Netherlands, 2003; pp. 1524–1527. [Google Scholar]
- Ji, Y.; Yoshioka, S.; Matsumoto, T. Three-dimensional numerical modeling of temperature and mantle flow fields associated with subduction of the Philippine Sea plate, southwest Japan. J. Geophys. Res. Solid Earth 2016, 121, 4458–4482. [Google Scholar] [CrossRef]
- Yoshioka, S.; Murakami, K. Temperature distribution of the upper surface of the subducted Philippine Sea Plate along the Nankai Trough, southwest Japan, from a three-dimensional subduction model: Relation to large interplate and low-frequency earthquakes. Geophys. J. Int. 2007, 171, 302–315. [Google Scholar] [CrossRef] [Green Version]
- Hayes, G.P.; Moore, G.L.; Portner, D.E.; Hearne, M.; Flamme, H.; Furtney, M.; Smoczyk, G.M. Slab2, a comprehensive subduction zone geometry model. Science 2018, 362, 58–61. [Google Scholar] [CrossRef] [PubMed]
- Yoshii, T. Regionality of group velocities of Rayleigh waves in the Pacific and thickening of the plate. Earth Planet. Sci. Lett. 1975, 25, 305–312. [Google Scholar] [CrossRef]
- Grose, C.J.; Afonso, J.C. Comprehensive plate models for the thermal evolution of oceanic lithosphere. Geochem. Geophys. Geosystems 2013, 14, 3751–3778. [Google Scholar] [CrossRef]
- Argus, D.F.; Gordon, R.G.; DeMets, C. Geologically current motion of 56 plates relative to the no-net-rotation reference frame. Geochem. Geophys. Geosystems 2011, 12. [Google Scholar] [CrossRef] [Green Version]
- DeMets, C.; Gordon, R.G.; Argus, D.F. Geologically current plate motions. Geophys. J. Int. 2010, 181, 1–80. [Google Scholar] [CrossRef] [Green Version]
- Vance, D.; Bickle, M.; Ivy-Ochs, S.; Kubik, P.W. Erosion and exhumation in the Himalaya from cosmogenic isotope inventories of river sediments. Earth Planet. Sci. Lett. 2003, 206, 273–288. [Google Scholar] [CrossRef]
- Lenard, S.J.; Lave, J.; France-Lanord, C.; Aumaitre, G.; Bourles, D.L.; Keddadouche, K. Steady erosion rates in the Himalayas through late Cenozoic climatic changes. Nat. Geosci. 2020, 13, 448–452. [Google Scholar] [CrossRef]
- Pedersen, V.K.; Egholm, D.L. Glaciations in response to climate variations preconditioned by evolving topography. Nature 2013, 493, 206–210. [Google Scholar] [CrossRef]
- Guan, Q.; Zhu, D.-C.; Zhao, Z.-D.; Dong, G.-C.; Zhang, L.-L.; Li, X.-W.; Liu, M.; Mo, X.-X.; Liu, Y.-S.; Yuan, H.-L. Crustal thickening prior to 38 Ma in southern Tibet: Evidence from lower crust-derived adakitic magmatism in the Gangdese Batholith. Gondwana Res. 2012, 21, 88–99. [Google Scholar] [CrossRef]
- Shellnutt, J.G.; Lee, T.-Y.; Brookfield, M.E.; Chung, S.-L. Correlation between magmatism of the Ladakh Batholith and plate convergence rates during the India–Eurasia collision. Gondwana Res. 2014, 26, 1051–1059. [Google Scholar] [CrossRef]
- Mugnier, J.-L.; Huyghe, P. Ganges basin geometry records a pre-15 Ma isostatic rebound of Himalaya. Geology 2006, 34, 445–448. [Google Scholar] [CrossRef] [Green Version]
- Guo, Z.; Wilson, M. Late Oligocene–early Miocene transformation of postcollisional magmatism in Tibet. Geology 2019, 47, 776–780. [Google Scholar] [CrossRef]
- Replumaz, A.; Funiciello, F.; Reitano, R.; Faccenna, C.; Balon, M. Asian collisional subduction: A key process driving formation of the Tibetan Plateau. Geology 2016, 44, 943–946. [Google Scholar] [CrossRef]
- Zou, Y.; Tian, X.; Yu, Y.; Pan, F.B.; Wang, L.; He, X. Seismic evidence for the existence of an entrained mantle flow coupling the northward advancing Indian plate under Tibet. Earth Planet. Phys. 2019, 3, 62–68. [Google Scholar] [CrossRef]
- Govin, G.; Najman, Y.; Copley, A.; Millar, I.; Van der Beek, P.; Huyghe, P.; Grujic, D.; Davenport, J. Timing and mechanism of the rise of the Shillong Plateau in the Himalayan foreland. Geology 2018, 46, 279–282. [Google Scholar] [CrossRef] [Green Version]
- Clark, M.K.; Bilham, R. Miocene rise of the Shillong Plateau and the beginning of the end for the Eastern Himalaya. Earth Planet. Sci. Lett. 2008, 269, 337–351. [Google Scholar] [CrossRef]
- Liang, S.; Gan, W.; Shen, C.; Xiao, G.; Liu, J.; Chen, W.; Ding, X.; Zhou, D. Three-dimensional velocity field of present-day crustal motion of the Tibetan Plateau derived from GPS measurements. J. Geophys. Res. Solid Earth 2013, 118, 5722–5732. [Google Scholar] [CrossRef]
- Huangfu, P.; Li, Z.-H.; Gerya, T.; Fan, W.; Zhang, K.-J.; Zhang, H.; Shi, Y. Multi-terrane structure controls the contrasting lithospheric evolution beneath the western and central–eastern Tibetan plateau. Nat. Commun. 2018, 9, 3780. [Google Scholar] [CrossRef]
- Spicer, R.A.; Su, T.; Valdes, P.J.; Farnsworth, A.; Wu, F.-X.; Shi, G.; Spicer, T.E.; Zhou, Z. Why ‘the uplift of the Tibetan Plateau’ is a myth. Natl. Sci. Rev. 2021, 8, nwaa091. [Google Scholar] [CrossRef]
- Kroon, D.; Steens, T.; Troelstra, S.R. Onset of monsoonal related upwelling in the western Arabian sea. Proc. Ocean Drill. Program Sci. Results 1991, 117, 257–263. [Google Scholar]
- Chemenda, A.I.; Burg, J.-P.; Mattauer, M. Evolutionary model of the Himalaya–Tibet system: Geopoem: Based on new modelling, geological and geophysical data. Earth Planet. Sci. Lett. 2000, 174, 397–409. [Google Scholar] [CrossRef]
- Husson, L.; Bernet, M.; Guillot, S.; Huyghe, P.; Mugnier, J.-L.; Replumaz, A.; Robert, X.; Van der Beek, P. Dynamic ups and downs of the Himalaya. Geology 2014, 42, 839–842. [Google Scholar] [CrossRef] [Green Version]
- She, Y.; Fu, G. Uplift mechanism of the highest mountains at eastern himalayan syntaxis revealed by in situ dense gravimetry. Geophys. Res. Lett. 2020, 47, e2020GL091208. [Google Scholar] [CrossRef]
- Boos, W.R.; Kuang, Z. Dominant control of the South Asian monsoon by orographic insulation versus plateau heating. Nature 2010, 463, 218–222. [Google Scholar] [CrossRef] [PubMed]
- Clift, P.D.; Hodges, K.V.; Heslop, D.; Hannigan, R.; Van Long, H.; Calves, G. Correlation of Himalayan exhumation rates and Asian monsoon intensity. Nat. Geosci. 2008, 1, 875–880. [Google Scholar] [CrossRef]
- Iaffaldano, G.; Husson, L.; Bunge, H.-P. Monsoon speeds up Indian plate motion. Earth Planet. Sci. Lett. 2011, 304, 503–510. [Google Scholar] [CrossRef]
- Chen, Q.; Freymueller, J.T.; Wang, Q.; Yang, Z.; Xu, C.; Liu, J. A deforming block model for the present-day tectonics of Tibet. J. Geophys. Res. Solid Earth 2004, 109. [Google Scholar] [CrossRef]
- Beaumont, C.; Jamieson, R.A.; Nguyen, M.; Lee, B. Himalayan tectonics explained by extrusion of a low-viscosity crustal channel coupled to focused surface denudation. Nature 2001, 414, 738–742. [Google Scholar] [CrossRef]
- Burkett, E.R.; Billen, M.I. Three-dimensionality of slab detachment due to ridge-trench collision: Laterally simultaneous boudinage versus tear propagation. Geochem. Geophys. Geosyst. 2010, 11, Q11012. [Google Scholar] [CrossRef]
- Hirth, G.; Kohlstedf, D. Rheology of the upper mantle and the mantle wedge: A view from the experimentalists. Geophys. Monogr.-Am. Geophys. Union 2003, 138, 83–106. [Google Scholar]
- Ranalli, G. Rheology of the Earth; Springer Science & Business Media: Berlin/Heidelberg, Germany, 1995. [Google Scholar]
- Stein, C.A.; Stein, S. A model for the global variation in oceanic depth and heat flow with lithospheric age. Nature 1992, 359, 123–129. [Google Scholar] [CrossRef]
- Müller, R.D.; Sdrolias, M.; Gaina, C.; Roest, W.R. Age, spreading rates, and spreading asymmetry of the world’s ocean crust. Geochem. Geophys. Geosyst. 2008, 9, Q04006. [Google Scholar] [CrossRef]
- Hacker, B.R.; Abers, G.A.; Peacock, S.M. Subduction factory 1. Theoretical mineralogy, densities, seismic wave speeds, and H2O contents. J. Geophys. Res. Solid Earth 2003, 108, 2029. [Google Scholar] [CrossRef]
- Ji, Y.; Yoshioka, S.; Manea, V.C.; Manea, M.; Matsumoto, T. Three-dimensional numerical modeling of thermal regime and slab dehydration beneath Kanto and Tohoku, Japan. J. Geophys. Res. Solid Earth 2017, 122, 332–353. [Google Scholar] [CrossRef] [Green Version]
- Pollack, H.N.; Hurter, S.J.; Johnson, J.R. Heat flow from the Earth’s interior: Analysis of the global data set. Rev. Geophys. 1993, 31, 267–280. [Google Scholar] [CrossRef]
- Li, C.-F.; Lu, Y.; Wang, J. A global reference model of Curie-point depths based on EMAG2. Sci. Rep. 2017, 7, srep45129. [Google Scholar] [CrossRef]
- Li, C.F.; Wang, J.; Lin, J.; Wang, T. Thermal evolution of the North Atlantic lithosphere: New constraints from magnetic anomaly inversion with a fractal magnetization model. Geochem. Geophys. Geosyst. 2013, 14, 5078–5105. [Google Scholar] [CrossRef] [Green Version]
- Turcotte, D.L.; Schubert, G. Geodynamics; Cambridge University Press: Cambridge, UK, 2002. [Google Scholar]
- Ji, Y.; Yoshioka, S.; Manea, V.C.; Manea, M. Seismogenesis of dual subduction beneath Kanto, central Japan controlled by fluid release. Sci. Rep. 2017, 7, 16864. [Google Scholar] [CrossRef] [Green Version]
- Suenaga, N.; Ji, Y.; Yoshioka, S.; Feng, D. Subduction thermal regime, slab dehydration, and seismicity distribution beneath Hikurangi based on 3-D simulations. J. Geophys. Res. Solid Earth 2018, 123, 3080–3097. [Google Scholar] [CrossRef]
- Ji, Y.; Yoshioka, S. Slab dehydration and earthquake distribution beneath southwestern and central Japan based on three-dimensional thermal modeling. Geophys. Res. Lett. 2017, 44, 2679–2686. [Google Scholar] [CrossRef]
- Suenaga, N.; Yoshioka, S.; Ji, Y. 3-D thermal regime and dehydration processes around the regions of slow earthquakes along the Ryukyu Trench. Sci. Rep. 2021, 11, 11251. [Google Scholar] [CrossRef] [PubMed]
- Ji, Y.; Yoshioka, S.; Banay, Y.A. Thermal state, slab metamorphism, and interface seismicity in the Cascadia subduction zone based on 3-D modeling. Geophys. Res. Lett. 2017, 44, 9242–9252. [Google Scholar] [CrossRef] [Green Version]
- Wang, K.; Hyndman, R.D.; Yamano, M. Thermal regime of the Southwest Japan subduction zone: Effects of age history of the subducting plate. Tectonophysics 1995, 248, 53–69. [Google Scholar] [CrossRef]
- Iwamori, H. Heat sources and melting in subduction zones. J. Geophys. Res. Solid Earth 1997, 102, 14803–14820. [Google Scholar] [CrossRef]
- Christensen, U.R. The influence of trench migration on slab penetration into the lower mantle. Earth Planet. Sci. Lett. 1996, 140, 27–39. [Google Scholar] [CrossRef] [Green Version]
- Trabant, C.; Hutko, A.R.; Bahavar, M.; Karstens, R.; Ahern, T.; Aster, R. Data products at the IRIS DMC: Stepping stones for research and other applications. Seismol. Res. Lett. 2012, 83, 846–854. [Google Scholar] [CrossRef]
- Wessel, P.; Smith, W.H. New, improved version of Generic Mapping Tools released. Eos Trans. Am. Geophys. Union 1998, 79, 579. [Google Scholar] [CrossRef]

Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zhu, W.; Ding, L.; Ji, Y.; Qu, R.; Zhu, Y.; Xie, C.; Zeng, D. Subduction Evolution Controlled Himalayan Orogenesis: Implications from 3-D Subduction Modeling. Appl. Sci. 2022, 12, 7413. https://doi.org/10.3390/app12157413
Zhu W, Ding L, Ji Y, Qu R, Zhu Y, Xie C, Zeng D. Subduction Evolution Controlled Himalayan Orogenesis: Implications from 3-D Subduction Modeling. Applied Sciences. 2022; 12(15):7413. https://doi.org/10.3390/app12157413
Chicago/Turabian StyleZhu, Weiling, Lin Ding, Yingfeng Ji, Rui Qu, Ye Zhu, Chaodi Xie, and Deng Zeng. 2022. "Subduction Evolution Controlled Himalayan Orogenesis: Implications from 3-D Subduction Modeling" Applied Sciences 12, no. 15: 7413. https://doi.org/10.3390/app12157413
APA StyleZhu, W., Ding, L., Ji, Y., Qu, R., Zhu, Y., Xie, C., & Zeng, D. (2022). Subduction Evolution Controlled Himalayan Orogenesis: Implications from 3-D Subduction Modeling. Applied Sciences, 12(15), 7413. https://doi.org/10.3390/app12157413





