Study on Accuracy of CFD Simulations of Wind Environment around High-Rise Buildings: A Comparative Study of k-ε Turbulence Models Based on Polyhedral Meshes and Wind Tunnel Experiments
Abstract
1. Introduction
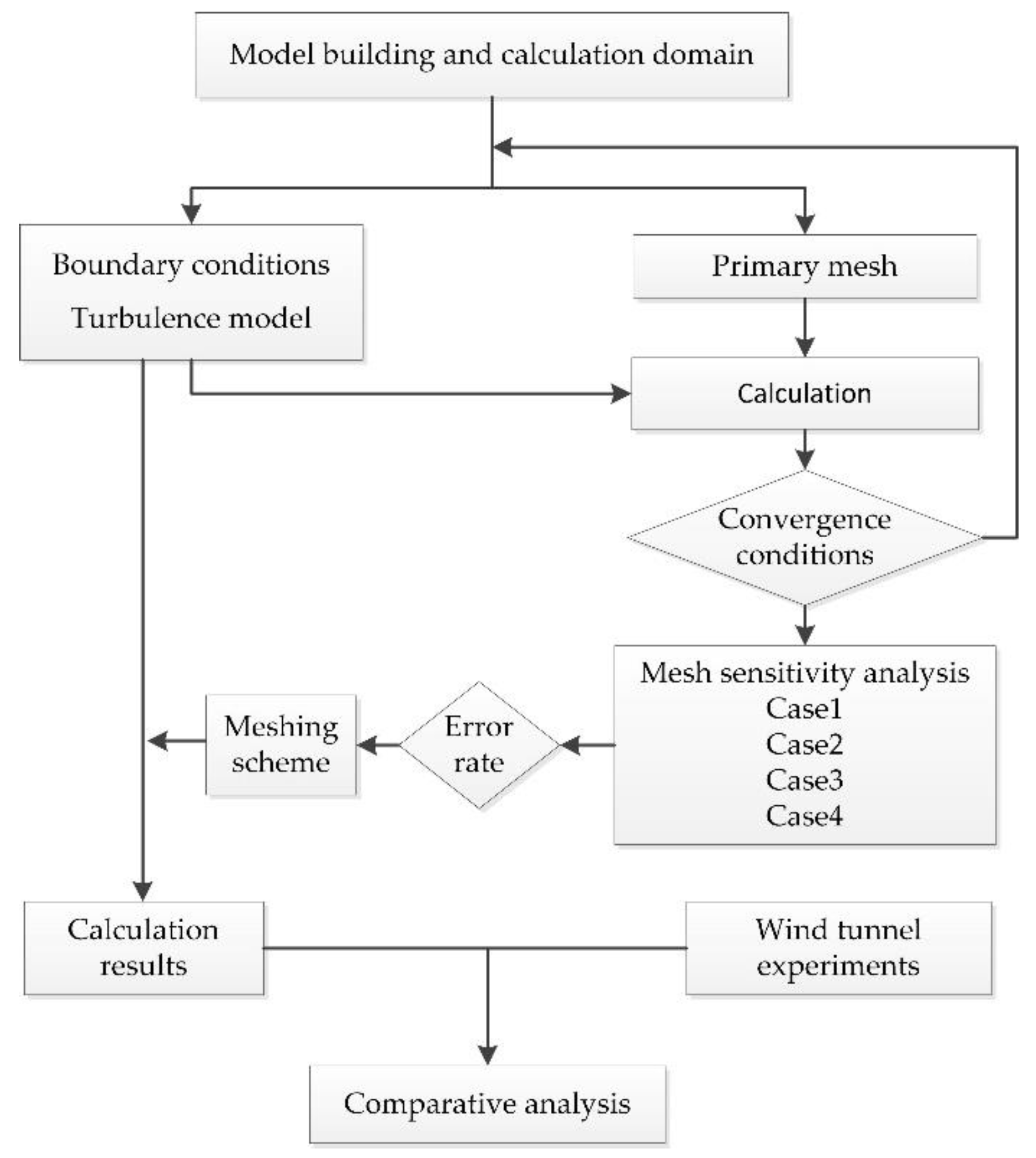
2. Method
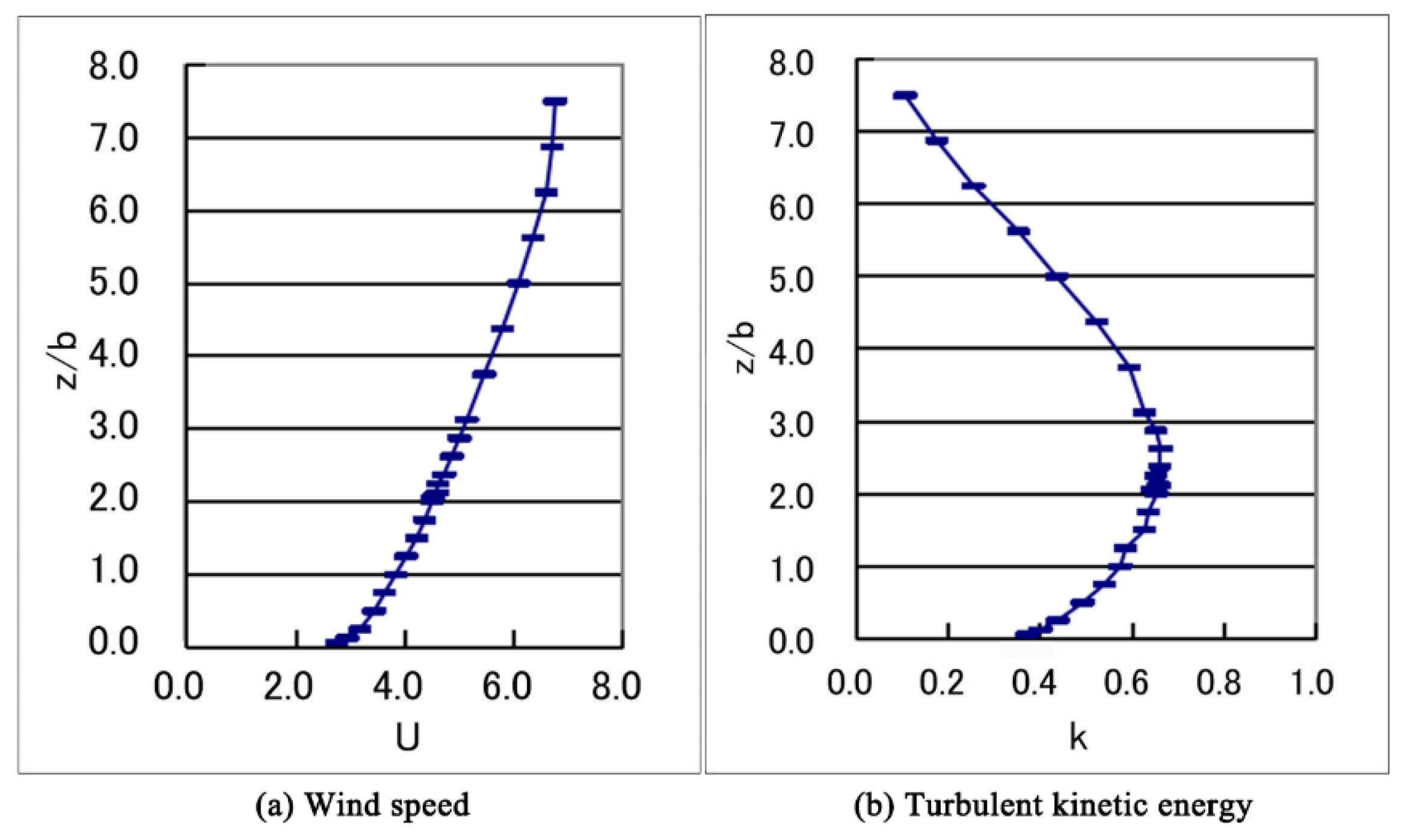
2.1. Outline of Wind Tunnel Experiment
2.2. Numerical Simulations
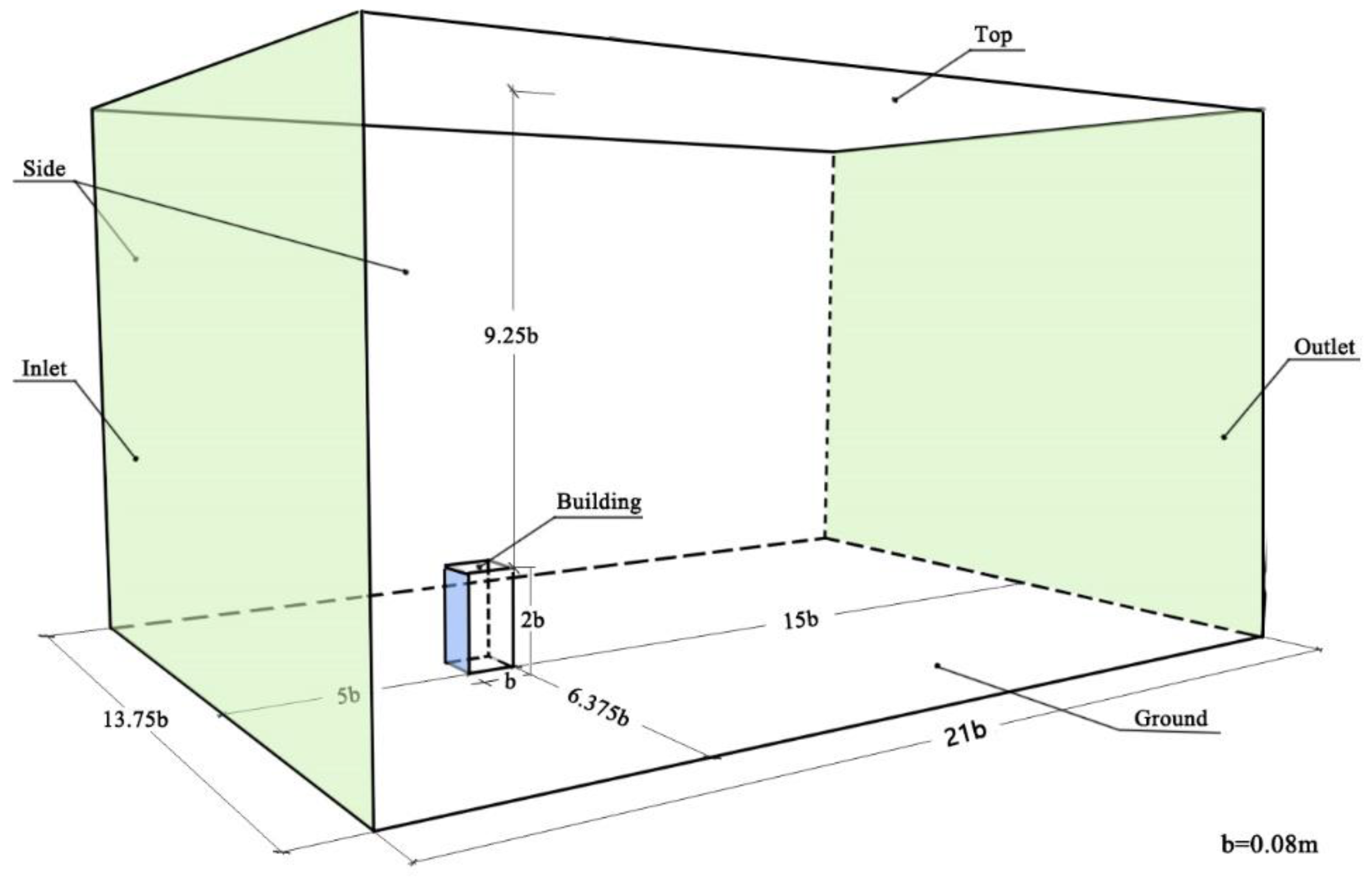
2.2.1. Model Building and Computational Domain
2.2.2. Boundary Conditions
2.2.3. Turbulence Model
2.2.4. Convergence Conditions
2.2.5. Meshing
2.3. Mesh Sensitivity Analysis
3. Results
3.1. Reattachment Length Comparison
3.2. Speed Comparison
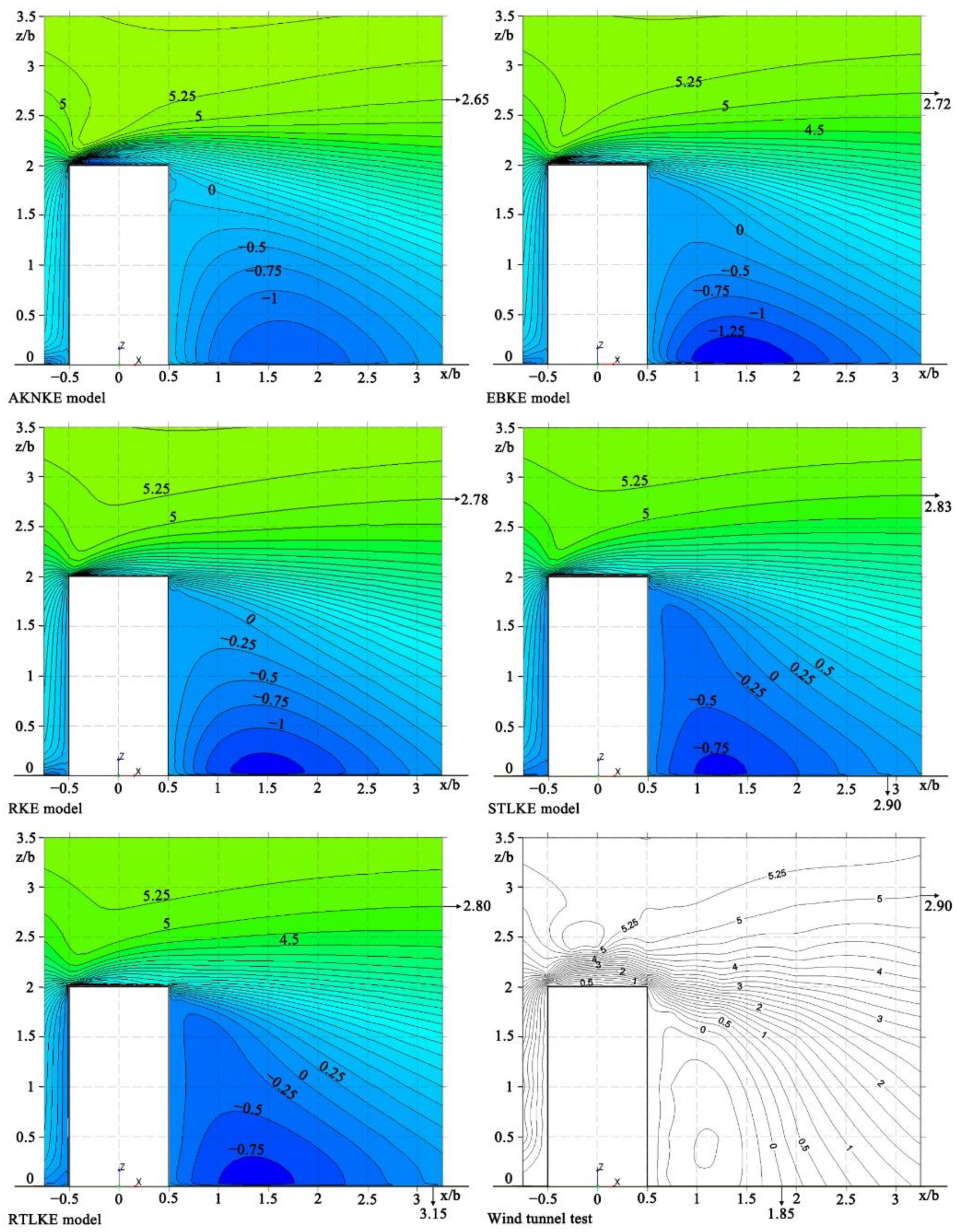
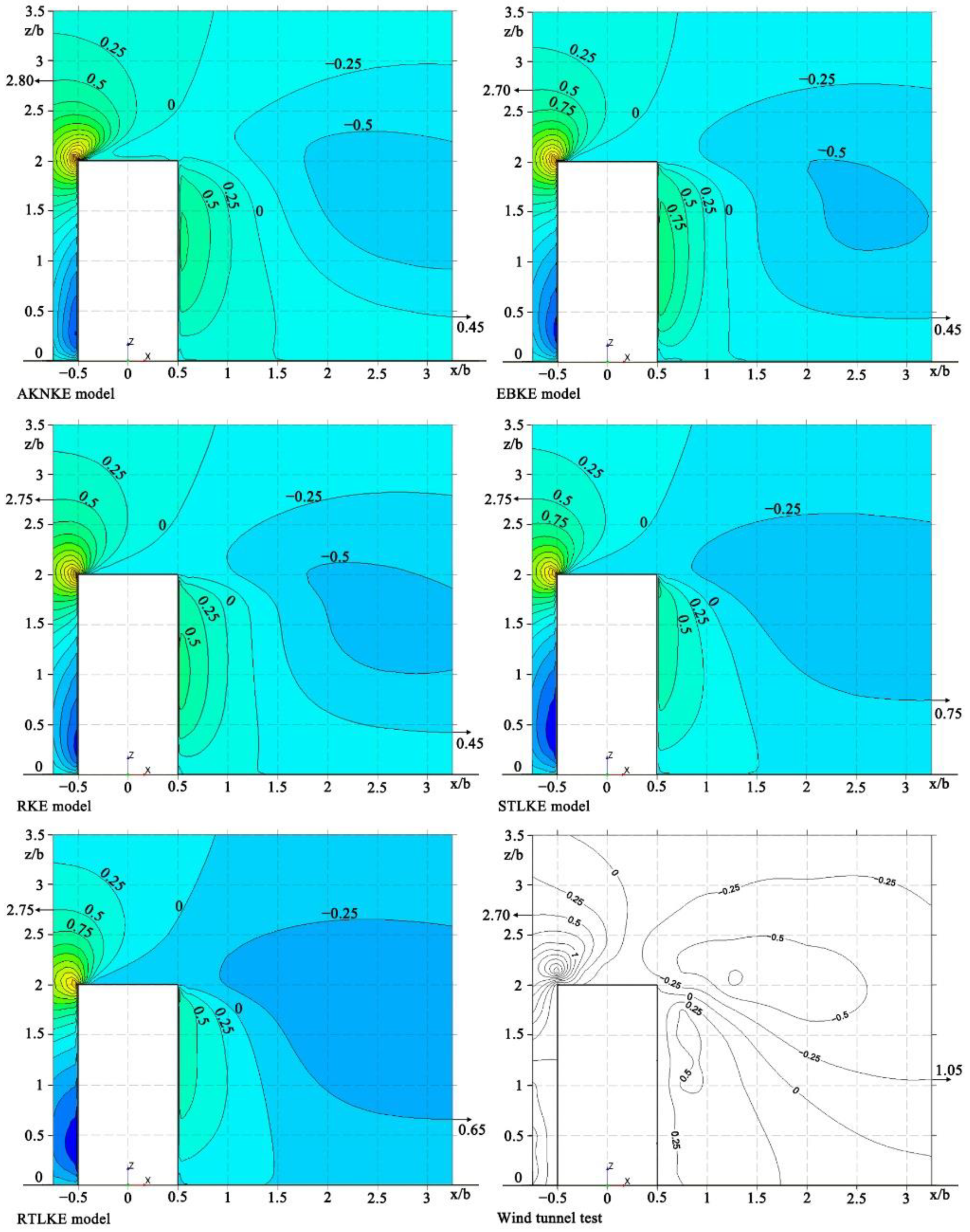
3.2.1. Comparison of Wind Speeds on the Vertical Plane at Y = 0
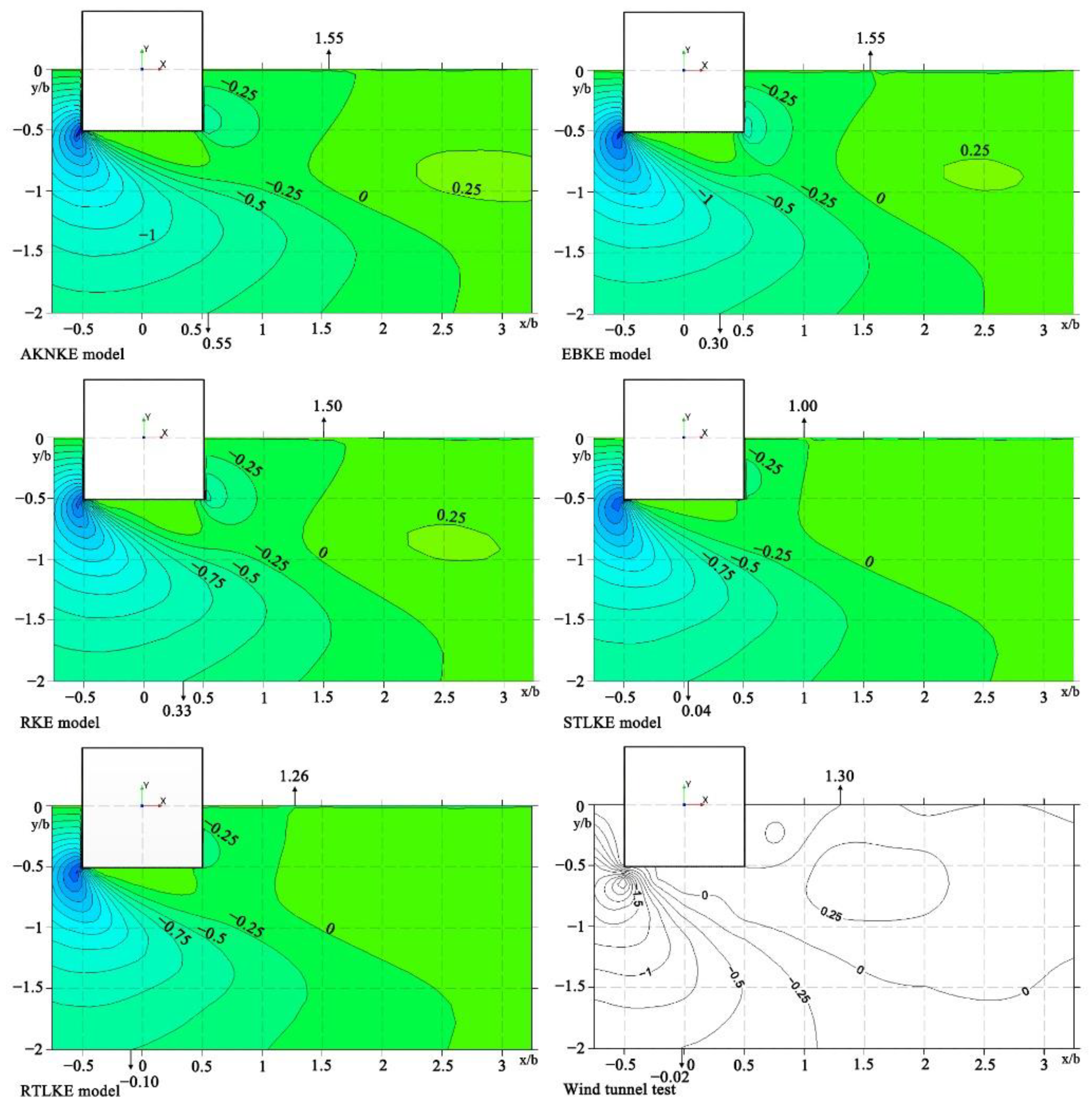
3.2.2. Comparison of Wind Speeds on the Horizontal Plane
3.3. Comparison of Turbulent Kinetic Energy
3.3.1. Comparison of Turbulent Kinetic Energy at Y = 0
3.3.2. Comparison of Turbulent Kinetic Energy at z/b = 0.125
3.3.3. Comparison of Turbulent Kinetic Energy at z/b = 1.25
4. Discussion
- (a)
- In the low-wind-speed area, the STLKE model and the RTLKE model could predict turbulence kinetic energy in a more accurate way. The RTLKE model also performed well in predicting the wind speed on the vertical and horizontal planes. The RTLKE model could be used for simulations in research studies of low wind-speed areas to predict the size of the static-wind areas around high-rise buildings, the diffusion time of pollutants around buildings, etc.
- (b)
- In the high-wind-speed area, the STLKE model and the RTLKE model could accurately predict wind speed. Between them, the STLKE model performed better in simulating the turbulence kinetic energy. Therefore, the STLKE model is recommended to be used for simulations in research studies of high wind-speed areas (e.g., windward side) of high-rise buildings to predict the damage of high wind speed to the area around buildings during a typhoon, the maximum wind-speed area around high-rise buildings, etc.
5. Conclusions
- (1)
- Under polyhedral mesh conditions, the modified k-e models applied with the wall function and the damping function improved the accuracy of the simulations but could still not perfectly match the wind tunnel experiment results. The correction of the models (e.g., optimizing the closure coefficients of the RANS turbulence model) needs to be further studied in order to significantly improve the accuracy of CFD simulations.
- (2)
- In the low-wind-speed area, the simulation results of the RTLKE model were the closest to the experimental results of the wind tunnels. In the high-wind-speed area, the simulation results of the STLKE model were the closest to the experimental results of the wind tunnels. So, these two models are recommended to be used for the simulation of wind around high-rise buildings under different circumstances.
- (3)
- In practice, it is recommended to use the STLKE model to explore high-wind-speed areas around high-rise buildings (e.g., the high-wind-speed areas around buildings during a typhoon, the maximum wind speed area around high-rise buildings, etc.). It is recommended to use the RTLKE model to explore low-wind-speed areas around high-rise buildings (e.g., the size of the static-wind area around high-rise buildings, the diffusion time of pollutants around buildings, etc.).
Supplementary Materials
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Blocken, B.; Carmeliet, J. Pedestrian Wind Environment around Buildings: Literature Review and Practical Examples. J. Therm. Envel. Build. Sci. 2004, 28, 107–159. [Google Scholar] [CrossRef]
- Mittal, H.; Sharma, A.; Gairola, A. A review on the study of urban wind at the pedestrian level around buildings. J. Build. Eng. 2018, 18, 154–163. [Google Scholar] [CrossRef]
- Uematsu, Y.; Yamada, M.; Higashiyama, H.; Orimo, T. Effects of the corner shape of high-rise buildings on the pedestrian-level wind environment with consideration for mean and fluctuating wind speeds. J. Wind Eng. Ind. Aerodyn. 1992, 44, 2289–2300. [Google Scholar] [CrossRef]
- Liu, H.; Jiang, Y.; Liang, B.; Zhu, F.; Zhang, B.; Sang, J. Studies on wind environment around high buildings in urban areas. Sci. China Ser. D Earth Sci. 2005, 48, 102–115. [Google Scholar] [CrossRef]
- Tsang, C.W.; Kwok, K.C.S.; Hitchcock, P.A. Wind tunnel study of pedestrian level wind environment around tall buildings: Effects of building dimensions, separation and podium. Build. Environ. 2012, 49, 167–181. [Google Scholar] [CrossRef]
- Cheung, J.O.P.; Liu, C.-H. CFD Simulations of Natural Ventilation Behaviour in High-Rise Buildings in Regular and Staggered Arrangements at Various Spacing. Energy Build. 2011, 43, 1149–1158. [Google Scholar] [CrossRef]
- Fang, P.; Zheng, D.; Gu, M.; Cheng, H.; Zhu, B. Numerical investigation of the wind environment around tall buildings in a central business district. Front. Earth Sci. 2019, 13, 848–858. [Google Scholar] [CrossRef]
- Tominaga, Y.; Shirzadi, M. Wind tunnel measurement of three-dimensional turbulent flow structures around a building group: Impact of high-rise buildings on pedestrian wind environment. Build. Environ. 2021, 206, 108389. [Google Scholar] [CrossRef]
- Yim, S.H.L.; Fung, J.; Lau, A.; Kot, S.C. Air ventilation impacts of the “wall effect” resulting from the alignment of high-rise buildings. Atmos. Environ. 2009, 43, 4982–4994. [Google Scholar] [CrossRef]
- Aristodemou, E.; Boganegra, L.; Mottet, L.; Pavlidis, D.; Constantinou, A.; Pain, C.; Robins, A.; Apsimon, H. How tall buildings affect turbulent air flows and dispersion of pollution within a neighbourhood. Environ. Pollut. 2017, 233, 782–796. [Google Scholar] [CrossRef]
- Liu, X.P.; Niu, J.; Kwok, K.C.S.; Wang, J.H.; Li, B.Z. Investigation of indoor air pollutant dispersion and cross-contamination around a typical high-rise residential building: Wind tunnel tests. Build. Environ. 2010, 45, 1769–1778. [Google Scholar] [CrossRef]
- Qiao, W.; Wang, Y.; Zhang, J.; Tian, W.; Tian, Y.; Yang, Q. An innovative coupled model in view of wavelet transform for predicting short-term PM10 concentration. J. Environ. Manag. 2021, 289, 112438. [Google Scholar] [CrossRef] [PubMed]
- Qiao, W.; Li, Z.; Liu, W.; Liu, E. Fastest-growing source prediction of US electricity production based on a novel hybrid model using wavelet transform. Int. J. Energy Res. 2022, 46, 1766–1788. [Google Scholar] [CrossRef]
- Paterson, D.; Apelt, C. Computation of wind flows over three-dimensional buildings. J. Wind Eng. Ind. Aerodyn. 1986, 24, 193–213. [Google Scholar] [CrossRef]
- Shirasawa, T.; Endo, Y.; Yoshie, R.; Mochida, A.; Tanaka, H. Comparison of les and durbin type k-ε model for gas diffusion in weak wind region behind a building. J. Environ. Eng. 2008, 73, 615–622. [Google Scholar] [CrossRef][Green Version]
- Tominaga, Y. Flow around a high-rise building using steady and unsteady RANS CFD: Effect of large-scale fluctuations on the velocity statistics. J. Wind Eng. Ind. Aerodyn. 2015, 142, 93–103. [Google Scholar] [CrossRef]
- Revuz, J.; Hargreaves, D.; Owen, J. On the domain size for the steady-state CFD modelling of a tall building. Wind Struct. 2012, 15, 313–329. [Google Scholar] [CrossRef]
- Kim, J.-C.; Lee, K.-S. Effect of Grid Size on Building Wind According to a Computation Fluid Dynamics Simulation. Asia Pac. J. Atmos. Sci. 2011, 47, 193–198. [Google Scholar] [CrossRef]
- Murakami, S.; Mochida, A. Three-Dimensional Numerical Simulation of Turbulent Flow Around Buildings using the k-e Turbulence Model. Build. Environ. 1989, 24, 51–64. [Google Scholar] [CrossRef]
- Tan, Y.; Li, C. Simulation of Wind Effects Embracing a Complex Shape Super High-Rise Steel TV Tower Using CFD. Adv. Mater. Res. 2011, 201–203, 2807–2813. [Google Scholar] [CrossRef]
- Behrouzi, F.; Sidik, N.A.C.; Malik, A.M.A.; Nakisa, M. Prediction of Wind Flow around High-Rise Buildings Using RANS Models. Appl. Mech. Mater. 2014, 554, 724–729. [Google Scholar] [CrossRef]
- Yoshie, R.; Mochida, A.; Tominaga, Y.; Kataoka, H.; Harimoto, K.; Nozu, T.; Shirasawa, T. Cooperative project for CFD prediction of pedestrian wind environment in the Architectural Institute of Japan. J. Wind Eng. Ind. Aerodyn. 2007, 95, 1551–1578. [Google Scholar] [CrossRef]
- Tominaga, Y.; Stathopoulos, T. Numerical simulation of dispersion around an isolated cubic building: Model evaluation of RANS and LES. Build. Environ. 2010, 45, 2231–2239. [Google Scholar] [CrossRef]
- Vardoulakis, S.; Dimitrova, R.; Richards, K.; Hamlyn, D.; Camilleri, G.; Weeks, M.; Sini, J.-F.; Britter, R.; Borrego, C.; Schatzmann, M.; et al. Numerical Model Inter-comparison for Wind Flow and Turbulence Around Single-Block Buildings. Environ. Model. Assess. 2011, 16, 169–181. [Google Scholar] [CrossRef]
- Gousseau, P.; Blocken, B.; van Heijst, G.J.F. CFD simulation of pollutant dispersion around isolated buildings: On the role of convective and turbulent mass fluxes in the prediction accuracy. J. Hazard. Mater. 2011, 194, 422–434. [Google Scholar] [CrossRef]
- Zhang, Z.P.; Zhang, W.; Chen, G.P. Numerical Simulation of Flow around High-Rise Buildings Based on Reynolds Stress Model. Appl. Mech. Mater. 2014, 580–583, 3057–3061. [Google Scholar] [CrossRef]
- Tominaga, Y.; Mochida, A.; Murakami, S.; Sawaki, S. Comparison of various revised k–ε models and LES applied to flow around a high-rise building model with 1:1:2 shape placed within the surface boundary layer. J. Wind Eng. Ind. Aerodyn. 2008, 96, 389–411. [Google Scholar] [CrossRef]
- Thordal, M.S.; Bennetsen, J.C.; Koss, H.H.H. Review for practical application of CFD for the determination of wind load on high-rise buildings. J. Wind Eng. Ind. Aerodyn. 2019, 186, 155–168. [Google Scholar] [CrossRef]
- Thordal, M.S.; Bennetsen, J.C.; Capra, S.; Koss, H.H.H. Towards a standard CFD setup for wind load assessment of high-rise buildings: Part 1—Benchmark of the CAARC building. J. Wind. Eng. Ind. Aerodyn. 2020, 205, 104283. [Google Scholar] [CrossRef]
- Thordal, M.S.; Bennetsen, J.C.; Capra, S.; Kragh, A.K.; Koss, H.H.H. Towards a standard CFD setup for wind load assessment of high-rise buildings: Part 2—Blind test of chamfered and rounded corner high-rise buildings. J. Wind. Eng. Ind. Aerodyn. 2020, 205, 104282. [Google Scholar] [CrossRef]
- Liu, J.; Niu, J. CFD simulation of the wind environment around an isolated high-rise building: An evaluation of SRANS, LES and DES models. Build. Environ. 2016, 96, 91–106. [Google Scholar] [CrossRef]
- Shirzadi, M.; Mirzaei, P.A.; Naghashzadegan, M. Improvement of k-epsilon turbulence model for CFD simulation of atmospheric boundary layer around a high-rise building using stochastic optimization and Monte Carlo Sampling technique. J. Wind Eng. Ind. Aerodyn. 2017, 171, 366–379. [Google Scholar] [CrossRef]
- Shirzadi, M.; Mirzaei, P.A.; Tominaga, Y. RANS model calibration using stochastic optimization for accuracy improvement of urban airflow CFD modeling. J. Build. Eng. 2020, 32, 101756. [Google Scholar] [CrossRef]
- Du, Y.; Mak, C.M.; Ai, Z. Modelling of pedestrian level wind environment on a high-quality mesh: A case study for the HKPolyU campus. Environ. Modell. Softw. 2018, 103, 105–119. [Google Scholar] [CrossRef]
- Nozu, T.; Tamura, T.; Takeshi, K.; Akira, K. Mesh-adaptive LES for wind load estimation of a high-rise building in a city. J. Wind Eng. Ind. Aerodyn. 2015, 144, 62–69. [Google Scholar] [CrossRef]
- Blocken, B.; Carmeliet, J. Pedestrian wind conditions at outdoor platforms in a high-rise apartment building: Generic sub-configuration validation, wind comfort assessment and uncertainty issues. Wind Struct. 2008, 11, 51–70. [Google Scholar] [CrossRef]
- Sosnowski, M.; Gnatowska, R.; Grabowska, K.; Krzywański, J.; Jamrozik, A. Numerical Analysis of Flow in Building Arrangement: Computational Domain Discretization. Appl. Sci. 2019, 9, 941. [Google Scholar] [CrossRef]
- China Academy of Building Research. Assessment Standard for Green Building, GB/T 50378-2019; Ministry of Housing and Urban-rural Development of the People’s Republic of China: Beijing, China, 2019; pp. 30–31.
- Meng, Y.; Hibi, K. Turbulent measurements of the flow field around a high-rise buildings. Wind Eng. JAWE 1998, 76, 55–64. [Google Scholar] [CrossRef]
- Mochida, A.; Tominaga, Y.; Murakami, S.; Yoshie, R.; Ishihara, T.; Ooka, R. Comparison of various k-e models and DSM applied to flow around a high rise building report on AIJ cooperative project for CFD prediction of wind environment. Wind Struct. 2002, 5, 227–244. [Google Scholar] [CrossRef]
- Franke, J.; Hellsten, A.; Schlünzen, K.; Carissimo, B. Best practice guideline for the CFD simulation of flows in the urban environment-a summary. In Proceedings of the 11th Conference on Harmonisation within Atmospheric Dispersion Modelling for Regulatory Purposes, Cambridge, UK, 2–5 July 2007. [Google Scholar]
- Blocken, B. Computational Fluid Dynamics for urban physics: Importance, scales, possibilities, limitations and ten tips and tricks towards accurate and reliable simulations. Build. Environ. 2015, 91, 219–245. [Google Scholar] [CrossRef]
- Tominaga, Y.; Mochida, A.; Yoshie, R.; Kataoka, H.; Nozu, T.; Yoshikawa, M.; Shirasawa, T. AIJ guidelines for practical applications of CFD to pedestrian wind environment around buildings. J. Wind Eng. Ind. Aerodyn. 2008, 96, 1749–1761. [Google Scholar] [CrossRef]
- Jones, W.P.; Launder, B.E. The prediction of laminarization with a two-equation model of turbulence. Int. J. Heat Mass Transf. 1972, 15, 301–314. [Google Scholar] [CrossRef]
- Wolfshtein, M. The velocity and temperature distribution in one-dimensional flow with turbulence augmentation and pressure gradient. Int. J. Heat Mass Transf. 1969, 12, 301–318. [Google Scholar] [CrossRef]
- Lien, F.S.; Chen, W.L.; Leschziner, M. Low-Reynolds-Number Eddy-Viscosity Modelling Based on Non-Linear Stress-Strain/Vorticity Relations. In Engineering Turbulence Modelling and Experiments; Rodi, W., Bergeles, G., Eds.; Elsevier: Oxford, UK, 1996; Volume 3, pp. 91–100. [Google Scholar]
- Shih, T.-H.; Liou, W.W.; Shabbir, A.; Yang, Z.; Zhu, J. A new k-ϵ eddy viscosity model for high reynolds number turbulent flows. Comput. Fluids 1995, 24, 227–238. [Google Scholar] [CrossRef]
- Abe, K.; Kondoh, T.; Nagano, Y. A new turbulence model for predicting fluid flow and heat transfer in separating and reattaching flows—II. Thermal field calculations. Int. J. Heat Mass Transf. 1995, 38, 1467–1481. [Google Scholar] [CrossRef]
- Durbin, P.A. A Reynolds stress model for near-wall turbulence. J. Fluid Mech. 1993, 249, 465–498. [Google Scholar] [CrossRef]
- Lien, F.S.; Kalitzin, G.; Durbin, P.A. RANS modeling for compressible and transitional flows. In Proceedings of the Summer Program, Stanford, CA, USA; 1998; pp. 267–286. [Google Scholar]
- Durbin, P.A. On the k-3 stagnation point anomaly. Int. J. Heat Fluid Flow 1996, 17, 89–90. [Google Scholar] [CrossRef]
- Zhang, H.; Xiong, M.; Chen, B.; Wang, Y. Influence of Tropical Cyclones on Outdoor Wind Environment in High-Rise Residential Areas in Zhejiang Province, China. Sustainability 2022, 14, 3932. [Google Scholar] [CrossRef]
- Qin, H.; Lin, P.; Lau, S.S.Y.; Song, D. Influence of site and tower types on urban natural ventilation performance in high-rise high-density urban environment. Build. Environ. 2020, 179, 106960. [Google Scholar] [CrossRef]
- Adams, E.W.; Johnston, J.P. Effects of the separating shear layer on the reattachment flow structure part 2: Reattachment length and wall shear stress. Exp. Fluids 1988, 6, 493–499. [Google Scholar] [CrossRef]
- Mochida, A.; Tominaga, Y.; Ishida, Y.; Ishihara, T.; Uehara, K.; Kataoka, H.; Kurabuchi, T.; Kobayashi, N.; Ooka, R.; Shirasawa, T.; et al. AIJ Benchmarks for Validation of CFD Simulations Applied to Pedestrian Wind Environment around Buildings; Architectural Institute of Japan: Tokyo, Japan, 2016. [Google Scholar]


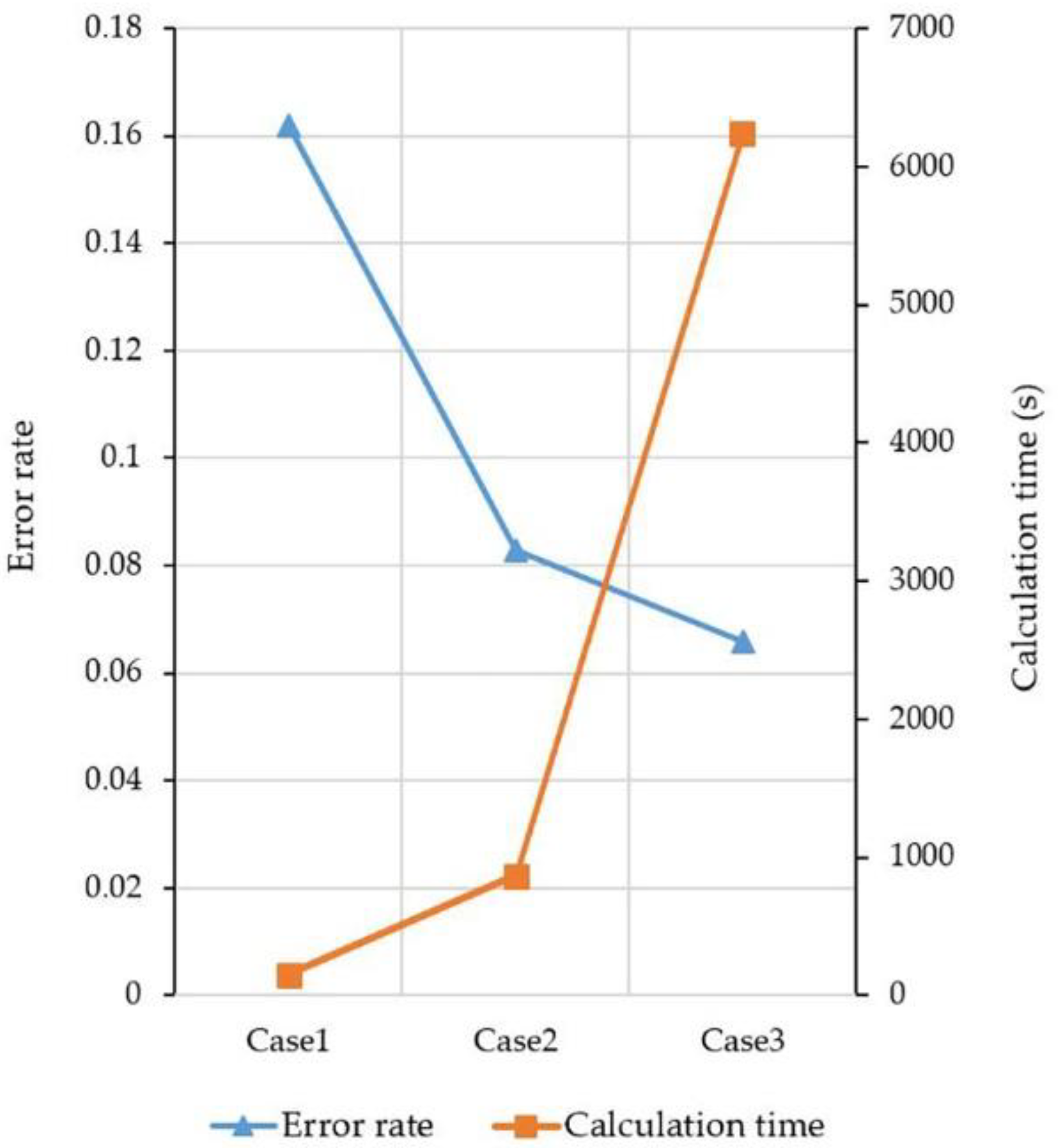
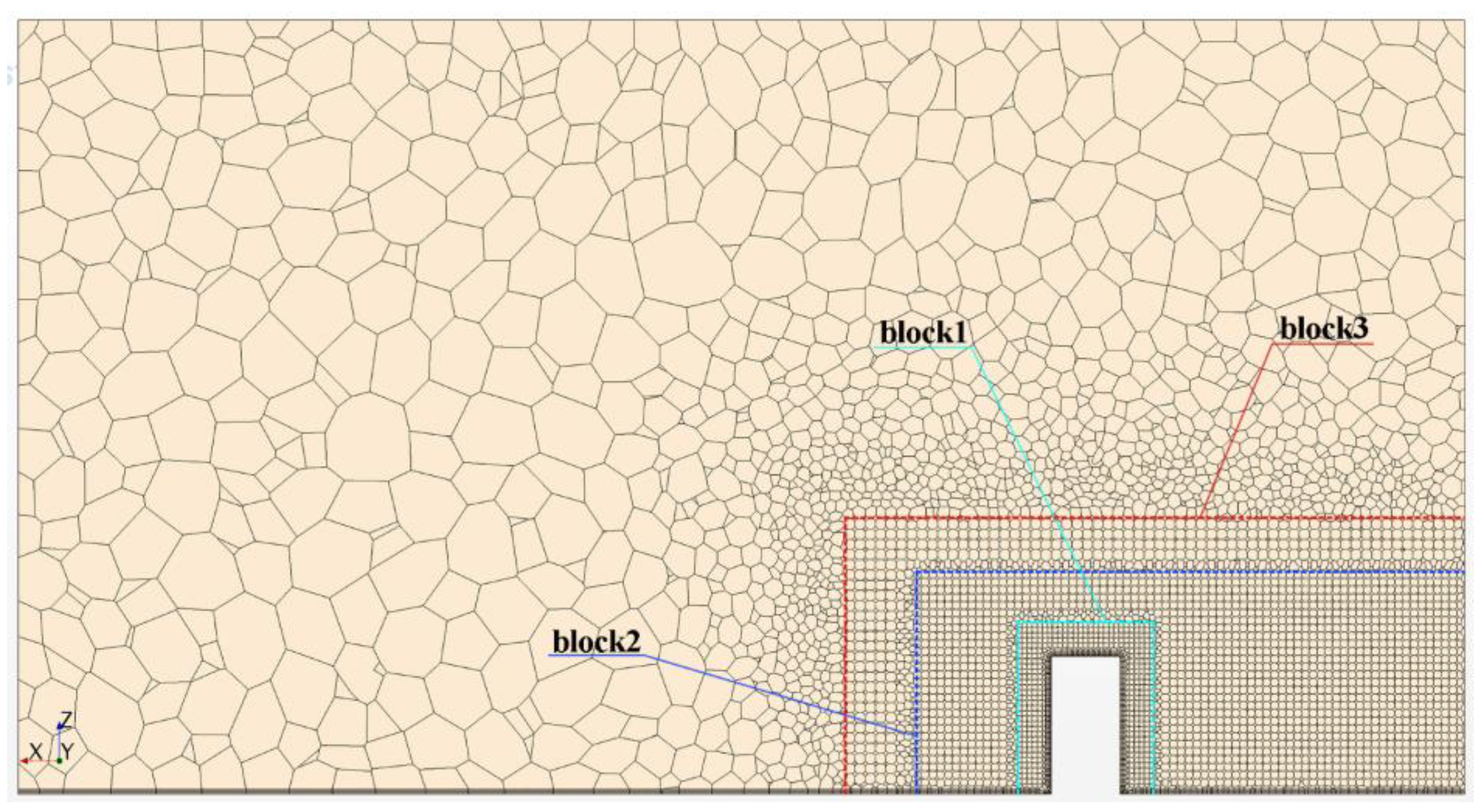




| Length (m) | Width (m) | Height (m) | Block Mesh Size/Base Mesh Size | |
|---|---|---|---|---|
| Block 1 | 0.16 | 0.16 | 0.2 | 10% |
| Block 2 | 0.64 | 0.24 | 0.26 | 15% |
| Block 3 | 0.72 | 0.4 | 0.32 | 20% |
| Base Mesh Size (m) | Architectural Mesh Size (m) | Mesh Numbers of Building Surface | Calculation Time (s) | |
|---|---|---|---|---|
| Case 1 | 0.10 | 0.01 | 977 | 152 |
| Case 2 | 0.06 | 0.006 | 2869 | 865 |
| Case 3 | 0.04 | 0.004 | 5944 | 6235 |
| Case 4 | 0.02 | 0.002 | 23,386 | 81,154 |
| SKE | STLKE | SLRNKE | RKE | RTLKE | AKNKE | EBKE | V2FKE | Wind Tunnel Experiment | |
|---|---|---|---|---|---|---|---|---|---|
| XR/b | — | 0.33 | — | 0.38 | 0.32 | 0.89 | 0.37 | 0.85 | 0.52 |
| XF/b | 2.63 | 2.38 | 2.53 | 3.05 | 2.65 | 3.55 | 3.20 | 4.35 | 1.42 |
| AKNKE | EBKE | RKE | STLKE | RTLKE | Wind Tunnel Experiment | |
|---|---|---|---|---|---|---|
| Location of contour line of 0 m/s in X-direction (low-wind-speed area) | >3.5 | >3.5 | >3.5 | 2.90 | 3.15 | 1.85 |
| Location of contour line of 5 m/s in Z-direction (high-wind-speed area) | 2.65 | 2.72 | 2.78 | 2.83 | 2.80 | 2.90 |
| AKNKE | EBKE | RKE | STLKE | RTLKE | Wind Tunnel Experiment | |
|---|---|---|---|---|---|---|
| Location of the contour line of −0.25 m/s in Z-direction (low-wind-speed area) | 0.45 | 0.45 | 0.45 | 0.75 | 0.65 | 1.05 |
| Location of the contour line of 0.5 m/s in Z-direction (high-wind-speed area) | 2.80 | 2.70 | 2.75 | 2.75 | 2.75 | 2.70 |
| AKNKE | EBKE | RKE | STLKE | RTLKE | Wind Tunnel Experiment | |
|---|---|---|---|---|---|---|
| Location of the contour line of −0.5 m/s in X-direction (low-wind-speed area) | >3.5 | 2.90 | 2.94 | 1.98 | 2.25 | 1.40 |
| Location of the contour line of 3 m/s in Y-direction (high-wind-speed area) | −1.23 | −1.35 | −1.35 | −1.45 | −1.35 | −1.65 |
| AKNKE | EBKE | RKE | STLKE | RTLKE | Wind Tunnel Experiment | |
|---|---|---|---|---|---|---|
| Location of the contour line of 0 m/s in X-direction (low-wind-speed area) | 1.55 | 1.55 | 1.50 | 1.00 | 1.26 | 1.30 |
| Location of the contour line of −0.5 m/s in X-direction (high-wind-speed area) | 0.55 | 0.30 | 0.33 | 0.04 | −0.10 | −0.02 |
| AKNKE | EBKE | RKE | STLKE | RTLKE | Wind Tunnel Experiment | |
|---|---|---|---|---|---|---|
| Location of the contour line of 0 m/s in X-direction (low-wind-speed area) | 2.15 | 1.57 | 1.80 | 1.46 | 1.63 | 1.45 |
| Location of the contour line of 4.25 m/s in Y-direction (high-wind-speed area) | −1.48 | −1.65 | −1.80 | −1.84 | −1.83 | −1.80 |
| AKNKE | EBKE | RKE | STLKE | RTLKE | Wind Tunnel Experiment | |
|---|---|---|---|---|---|---|
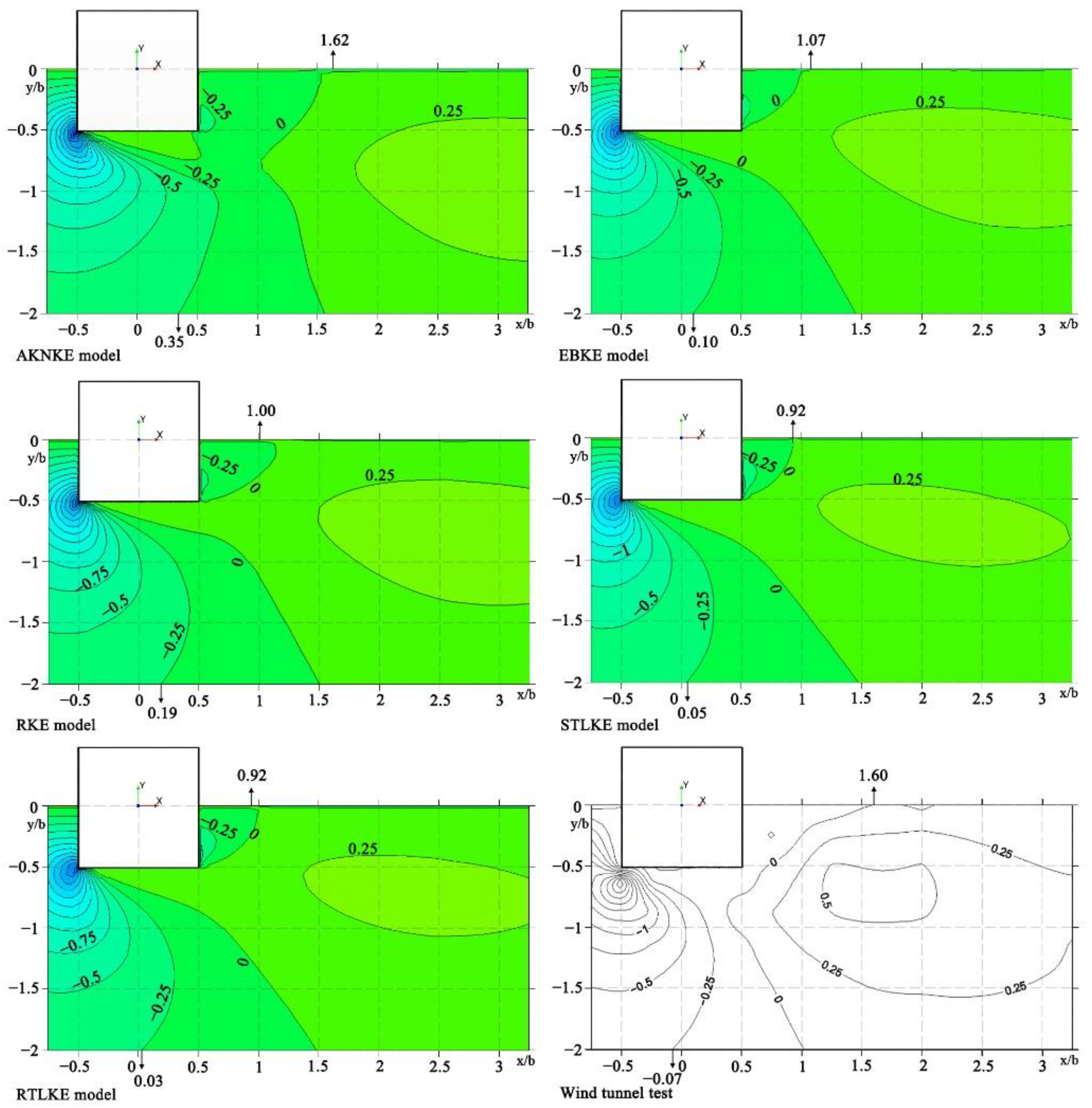
| Location of the contour line of 0 m/s in X-direction (low-wind-speed area) | 1.62 | 1.07 | 1.00 | 0.92 | 0.92 | 1.60 |
| Location of the contour line of −0.25 m/s in X-direction (high-wind-speed area) | 0.35 | 0.10 | 0.19 | 0.15 | 0.03 | −0.07 |
| Location | Recommended Models | Not-Recommended Models (Much Different from the Experiment) |
|---|---|---|
| X-velocity (Y = 0) | STLKE, RTLKE | RKE, EBKE, AKNKE |
| Z-velocity (Y = 0) | STLKE, RTLKE | RKE, EBKE, AKNKE |
| X-velocity at z/b = 0.125 | STLKE, RTLKE | RKE, EBKE, AKNKE |
| Y-velocity at z/b = 0.125 | RTLKE | STLKE, RKE, EBKE, AKNKE |
| X-velocity at z/b = 1.25; | STLKE, RKE, RTLKE, EBKE | AKNKE |
| Y-velocity at z/b = 1.25 | AKNKE | RKE, STLKE, RTLKE, EBKE |
| Total | RTLKE |
| Location | Recommended Models | Not-Recommended Models (Much Different from the Experiment) |
|---|---|---|
| X-velocity (Y = 0) | All | |
| Z-velocity (Y = 0) | All | |
| X-velocity at z/b = 0.125 | STLKE, EBKE, RKE, RTLKE, | AKNKE |
| Y-velocity at z/b = 0.125 | STLKE, RTLKE | RKE, EBKE, AKNKE |
| X-velocity at z/b = 1.25; | STLKE, RTLKE, RKE, EBKE | AKNKE |
| Y-velocity at z/b = 1.25 | STLKE, RTLKE, EBKE, RKE | AKNKE |
| Total | STLKE, RTLKE | EBKE, RKE, AKNKE |
| AKNKE | EBKE | RKE | STLKE | RTLKE | Wind Tunnel Experiment | |
|---|---|---|---|---|---|---|
| Location of the contour line of 0.5 in X-direction (low-wind-speed area) | 0.90 | 2.05 | >3.5 | 0.65 | 1.60 | 0.77 |
| Location of the contour line of 1 in Z-direction (high-wind-speed area) | _ | _ | _ | 2.25 | _ | 2.30 |
| AKNKE | EBKE | RKE | STLKE | RTLKE | Wind Tunnel Experiment | |
|---|---|---|---|---|---|---|
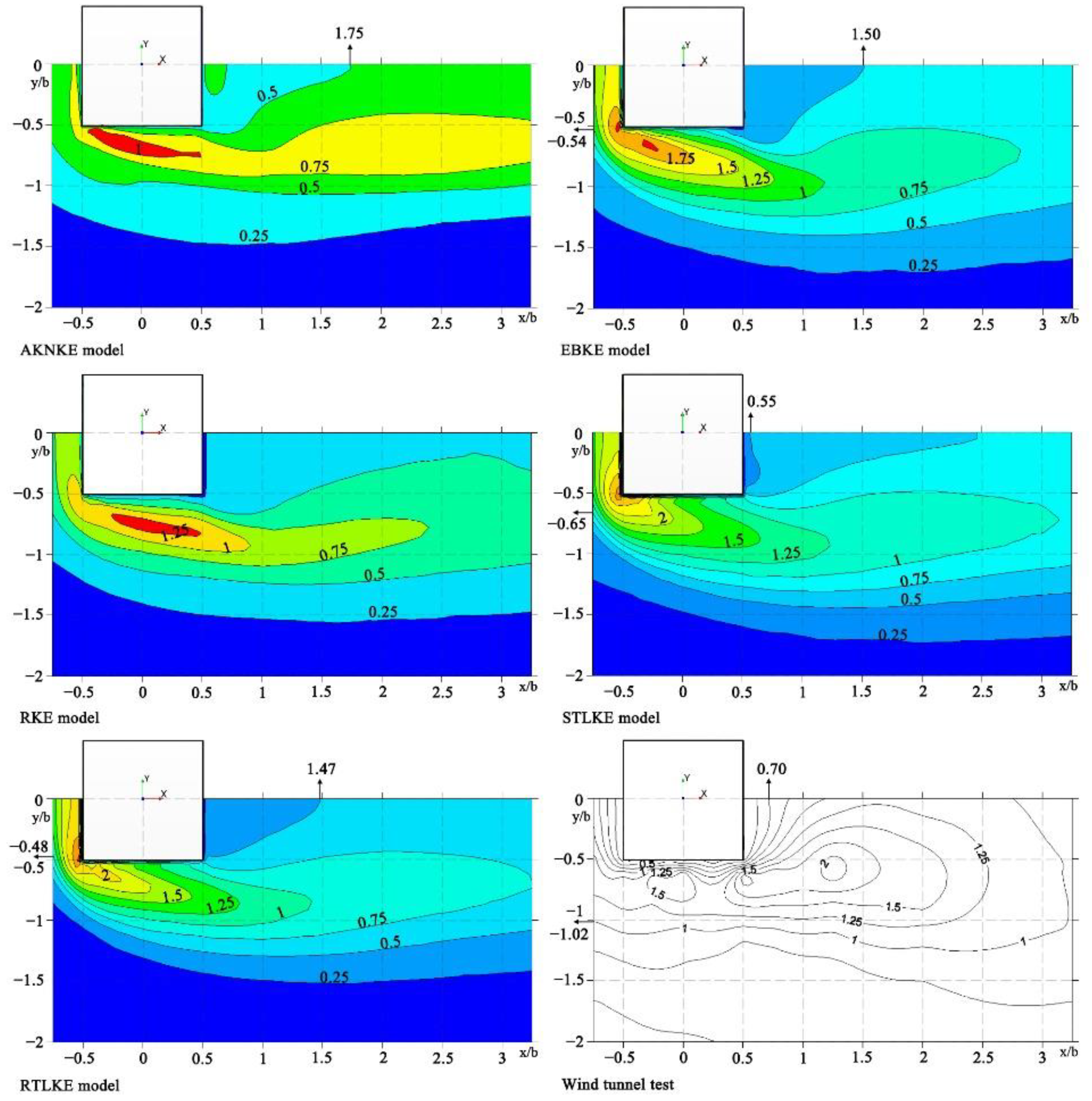
| Location of the contour line of 0.5 in X-direction (low-wind-speed area) | 1.75 | 1.50 | - | 0.55 | 1.47 | 0.70 |
| Location of the contour line of 1 in Y-direction (high-wind-speed area) | - | −0.54 | - | −0.65 | −0.48 | −1.02 |
| AKNKE | EBKE | RKE | STLKE | RTLKE | Wind Tunnel Experiment | |
|---|---|---|---|---|---|---|
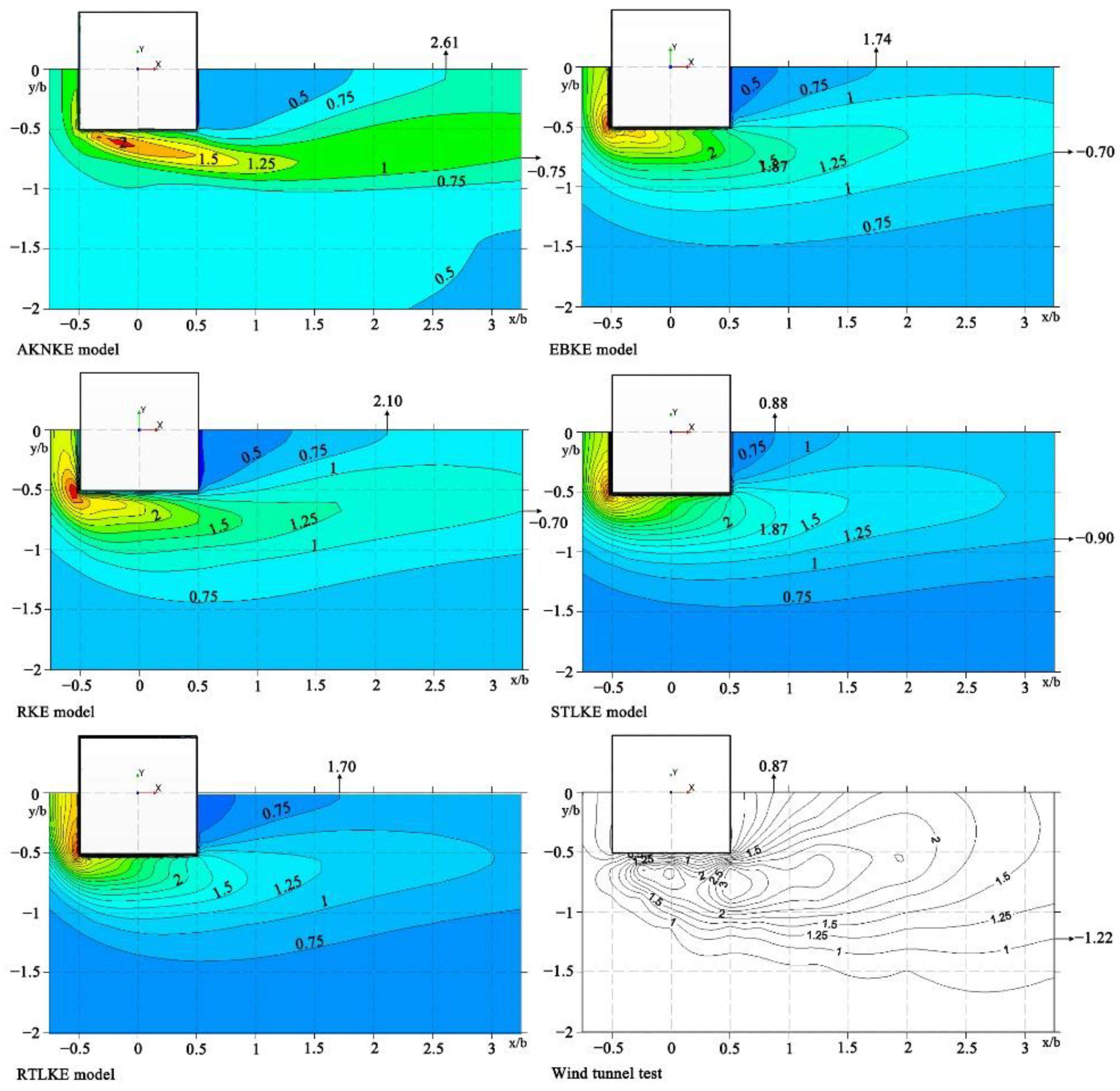
| Location of the contour line of 0.75 in X-direction (low-wind-speed area) | 2.61 | 1.74 | 2.10 | 0.88 | 1.70 | 0.87 |
| Location of the contour line of 1 in Y-direction (high-wind-speed area) | −0.75 | −0.70 | −0.70 | −0.90 | - | −1.22 |
| Location | Recommended Models | Not-Recommended Models (Much Different from the Experiment) |
|---|---|---|
| Y = 0 | STLKE, AKNKE, RTLKE | RKE, EBKE, |
| z/b = 0.125 | STLKE, AKNKE, RTLKE | EBKE, RKE |
| z/b = 1.25 | STLKE, RTLKE, EBKE | RKE, AKNKE |
| Total | STLKE, RTLKE | RKE, EBKE, AKNKE |
| Location | Recommended Models | Not-Recommended Models (Much Different from the Experiment) |
|---|---|---|
| Y = 0 | STLKE | RTLKE, RKE, AKNKE, EBKE |
| z/b = 0.125 | STLKE, EBKE, RTLKE | RKE, AKNKE |
| z/b = 1.25 | STLKE, EBKE, RKE | RTLKE, AKNKE |
| STLKE | RTLKE, RKE, AKNKE, EBKE |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Xiong, M.; Chen, B.; Zhang, H.; Qian, Y. Study on Accuracy of CFD Simulations of Wind Environment around High-Rise Buildings: A Comparative Study of k-ε Turbulence Models Based on Polyhedral Meshes and Wind Tunnel Experiments. Appl. Sci. 2022, 12, 7105. https://doi.org/10.3390/app12147105
Xiong M, Chen B, Zhang H, Qian Y. Study on Accuracy of CFD Simulations of Wind Environment around High-Rise Buildings: A Comparative Study of k-ε Turbulence Models Based on Polyhedral Meshes and Wind Tunnel Experiments. Applied Sciences. 2022; 12(14):7105. https://doi.org/10.3390/app12147105
Chicago/Turabian StyleXiong, Minghui, Bing Chen, Hua Zhang, and Yao Qian. 2022. "Study on Accuracy of CFD Simulations of Wind Environment around High-Rise Buildings: A Comparative Study of k-ε Turbulence Models Based on Polyhedral Meshes and Wind Tunnel Experiments" Applied Sciences 12, no. 14: 7105. https://doi.org/10.3390/app12147105
APA StyleXiong, M., Chen, B., Zhang, H., & Qian, Y. (2022). Study on Accuracy of CFD Simulations of Wind Environment around High-Rise Buildings: A Comparative Study of k-ε Turbulence Models Based on Polyhedral Meshes and Wind Tunnel Experiments. Applied Sciences, 12(14), 7105. https://doi.org/10.3390/app12147105







