The Effect of Subgrade Coefficient on Static Work of a Pontoon Made as a Monolithic Closed Tank
Abstract
1. Introduction
- p—load (kN/m2),
- w—deflection (m), and
- Kz—substrate stiffness (kN/m3).
- D = —plate flexural rigidity,
- —Laplacian,
- —bi-Laplacian,
- ν—Poisson’s ratio,
- h—plate thickness,
- w—plate deflection,
- αt—coefficient of thermal expansion,
- q—load perpendicular to the central surface of the plate,
- ΔT—difference in temperature between lower plate Td and upper plate Tg determined by correlation: ΔT = Td − Tg, αt is the coefficient of thermal expansion of the plate material,
- Kz—subgrade stiffness reaction.
2. Materials and Methods
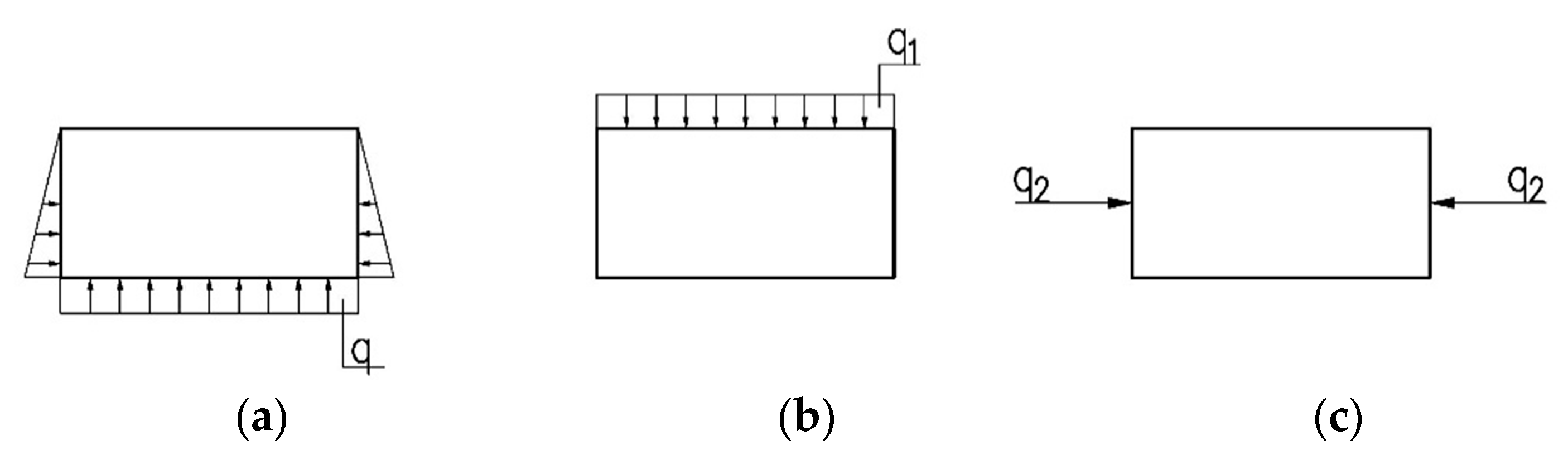
2.1. Calculation Method
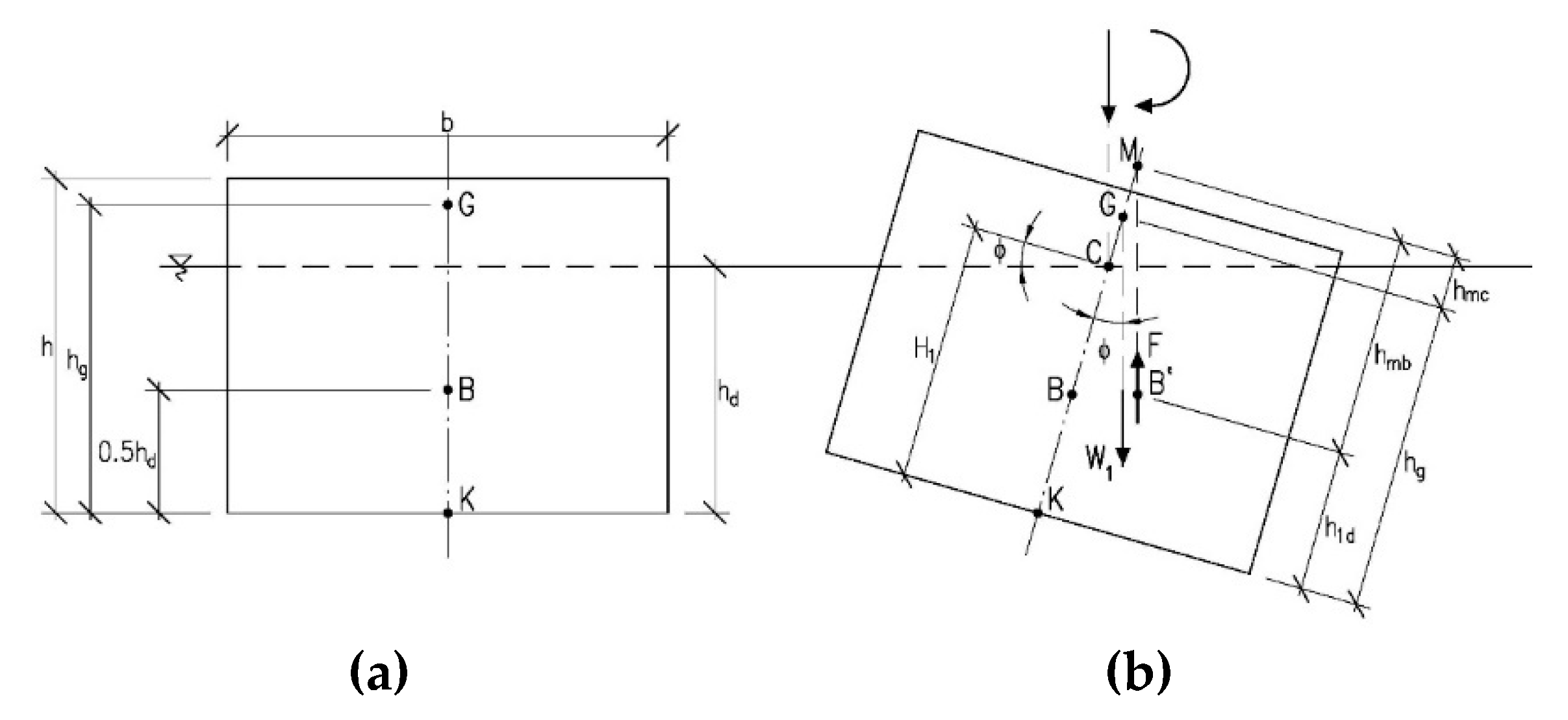
2.2. Verification of Pontoon Buoyancy and Stability
3. Results
3.1. Static Analysis of the Pontoon
3.2. Calculation Results of Buoyancy and Stability
4. Discussion
5. Conclusions
- taking into account in calculations the cooperation of the tank walls with its elastic substrate filling reduces bending moments and, in some cases, changes the sign of bending moments (Table 1). For example, the bending moment Mx813 (according to designations in Figure 4) occurring in the middle of the bottom length, above the support, which due to the hydrostatic load acting on the walls and the bottom, without taking into account its elastic substrate, was Mx813= 10.24996 qs2 while, taking into account its elastic substrate, was reduced to Mx813 = 8.16864 qs2, so it decreased by 20%;
- taking into account in calculations an internal Styrofoam filling treated as an elastic substrate reduces the values of bending moments and consequently the area that requires necessary reinforcement and thus the solution becomes more financially beneficial;
- taking into account in calculations the cooperation of the pontoon’s structure with an internal filling does not affect its buoyancy and stability;
- the pontoon presented in the article can be a ready-made, prefabricated reinforced concrete shipping element, prepared to be built in at its final location, which can be used as a float in amphibious construction.
Funding
Conflicts of Interest
References
- Wang, C.M. Great, Ideas Float on the Top. In Large Floating Structures: Technological Advances; Wang, C.M., Wang, B.T., Eds.; Springer: Berlin/Heidelberg, Germany, 2015; pp. 1–36. [Google Scholar]
- Holcombe, S. Applications and Huge Potential Demand for Amphibious Structures. In Proceedings of the First International Conference on Amphibious Architecture, Design & Engineering, Waterloo, ON, Canada, 25–28 June 2017; Volume 138. [Google Scholar]
- Nakajima, T.; Umeyama, M. A New Concept for the Safety of Low-lying Land Areas from Natural Disasters. J. Ocean Eng. Mar. Energy 2015, 1, 19–29. [Google Scholar] [CrossRef]
- Ostrowska-Wawryniuk, K.; Piątek, Ł. Lightweight Prefabricated Floating Buildings for Shallow Inland Waters. Design and Construction of The Floating Hotel Apartment in Poland. J. Water Land Dev. 2020, 44, 118–125. [Google Scholar] [CrossRef]
- Laks, I.; Walczak, Z.; Szymczak-Graczyk, A.; Ksit, B.; Mądrawski, J. Hydraulic and legal conditions for buildings in floodplains—A case study for the city of Kalisz (Poland). IOP Conf. Ser. Mater. Sci. Eng. 2019, 471, 102050. [Google Scholar] [CrossRef]
- Mazurkiewicz, B. Yacht Ports and Marinas. Design; Fundacja Promocji Przemysłu Okrętowego i Gospodarki Morskiej: Gdańsk, Poland, 2010. [Google Scholar]
- Szymczak-Graczyk, A. Floating platforms made of monolithic closed rectangular tanks. Bull. Pol. Acad. Sci. Tech. Sci. 2018, 66, 209–219. [Google Scholar] [CrossRef]
- Szymczak-Graczyk, A. Numerical Analysis of the Bottom Thickness of Closed Rectangular Tanks Used as Pontoons. Appl. Sci. 2020, 10, 8082. [Google Scholar] [CrossRef]
- Szymczak-Graczyk, A. “Numerical analysis of the impact of thermal spray insulation solutions on floor loading”. Appl. Sci. 2020, 10, 1016. [Google Scholar] [CrossRef]
- Winkler, E. Die Lehre von der Elastizität und Festigkeit; Dominicus: Prague, Czech Republic, 1867. [Google Scholar]
- Kączkowski, Z. Plates. Static Calculations; Arkady: Warszawa, Poland, 2000. [Google Scholar]
- Urbanowski, W. Case studies of bending a round plate connected to a flexible substrate of generalized properties. Zesz. Nauk. Politech. Warsz. 1956, 3, 33–61. [Google Scholar]
- Szcześniak, W.; Ataman, M. Vibration of beam resting on the inertial Vlasov-Leontiev foundation under impulse of force. Autobusy 2016, 3, 727–732. [Google Scholar]
- Wang, Y.H.; Tham, L.G.; Cheung, Y.K. Beams and plates on elastic foundations: A review. Prog. Struct. Eng. Mater. 2005, 7, 174–182. [Google Scholar] [CrossRef]
- Ryżyński, W.; Karczewski, B. Determination of calculation parameters of the layered substrate under industrial floors. Nowocz. Hale 2014, 5, 34–38. [Google Scholar]
- Korhan, O.; Ayse, T.D. Free vibration analysis of thick plates on elastic foundations using modified Vlasov model with higher order finite elements. Int. J. Econ. Manag. 2012, 19, 279–291. [Google Scholar]
- Gołaś, J. Introduction to the Theory of Plates; Opole University of Technology Publishing House: Opole, Poland, 1972. [Google Scholar]
- Donnell, L.H. Beams, Plates and Shells; McGraw-Hill: New York, NY, USA, 1976. [Google Scholar]
- Naghdi, P.M. The Theory of Shells and Plates; Handbuch der Physick: Berlin, Germany, 1972. [Google Scholar]
- Panc, V. Theries of Elastic Plates; Academia: Prague, Czech Republic, 1975. [Google Scholar]
- Timoshenko, S.; Woinowsky-Krieger, S. Theory of Plates and Coatings; Arkady: Warszawa, Poland, 1962. [Google Scholar]
- Szlilard, R. Theory and Analysis of Plates. Classical and Numerical Methods; Prentice Hall, Englewood Cliffs: Bergen, NJ, USA; Prentice-Hall: Upper Saddle River, NJ, USA, 1974. [Google Scholar]
- Ugural, A.C. Stresses in Plates and Shells; McGraw-Hill: New York, NY, USA, 1981. [Google Scholar]
- Wilde, P. Variational approach of finite differences in the theory of plate. In Proceedings of the Materials of XII Scientific Conference of the Committee of Science PZiTB and the Committee of Civil Engineering of Polish Academy of Sciences, Krynica, Poland, 12–17 September 1966. [Google Scholar]
- Tribiłło, R. Application of the generalized finite difference method for plate calculations. Arch. Inżynierii Lądowej 1975, 2, 579–586. [Google Scholar]
- Son, M.; Sang Jung, H.; Hee Yoon, H.; Sung, D.; Suck Kim, J. Numerical Study on Scale Effect of Repetitive Plate-Loading Test. Appl. Sci. 2019, 9, 4442. [Google Scholar] [CrossRef]
- Nowacki, W. Z zastosowań rachunku różnic skończonych w mechanice budowli. Arch. Mech. Stos. 1951, 3, 419–435. [Google Scholar]
- Rapp, B.E. Chapter 30—Finite Difference Method. In Microfluidics: Modelling, Mechanics and Mathematics, Micro and Nano Technologies; Rapp, B.E., Ed.; Elsevier: Amsterdam, The Netherlands, 2017; pp. 623–631. [Google Scholar] [CrossRef]
- Blazek, J. Chapter 3—Principles of Solution of the Governing Equations. In Computational Fluid Dynamics: Principles and Applications; Blazek, J., Ed.; Elsevier: Amsterdam, The Netherlands, 2015; pp. 29–72. [Google Scholar] [CrossRef]
- Sadd, M.H. Chapter 5—Formulation and Solution Strategies. In Elasticity, Theory, Applications, and Numerics; Sadd, M.H., Ed.; Academic Press, Elsevier: Cambridge, MA, USA, 2005; pp. 83–102. [Google Scholar] [CrossRef]
- Szymczak-Graczyk, A. Rectangular plates of a trapezoidal cross-section subjected to thermal load. IOP Conf. Ser. Mater. Sci. Eng. 2019, 603, 032095. [Google Scholar] [CrossRef]
- Numayr, K.S.; Haddad, R.H.; Haddad, M.A. Free vibration of composite plates using the finite difference method. Thin-Walled Struct. 2004, 42, 399–414. [Google Scholar] [CrossRef]
- Buczkowski, W.; Szymczak-Graczyk, A.; Walczak, Z. Experimental validation of numerical static calculations for a monolithic rectangular tank with walls of trapezoidal cross-section. Bull. Pol. Acad. Sci. Tech. Sci. 2017, 65, 799–804. [Google Scholar] [CrossRef]
- Z44, Zalecenia do Projektowania Marskich Konstrukcji Hydrotechnicznych, Z1–Z46; Studia i materiały, 21; Politechnika Gdańska, Katedra Budownictwa Morskiego: Gdańsk, Poland, 1997.
- AS 3962-2001: The Australian Standard: Guidelines for Design of Marinas; Standards Australia International Ltd.: Sydney, Australian, 2001.
- PN–EN 14504:2010: Inland Waterway Vessels. Floating Harbors. Requirements, Tests; Polish Standardization Committee: Warsaw, Poland, 2010.
- PN-EN 1992-1-1:2008 Eurocode 2. Design of Concrete Structures. Part 1-1. General Rules and Rules for Buildings; Polish Standardization Committee: Warsaw, Poland, 2008.
- Jiang, D.; Tan, K.H.; Wang, C.M.; Ong, K.C.G.; Bra, H.; Jin, J.; Kim, M.O. Analysis and design of floating prestressed concrete structures in shallow waters. Mar. Struct. 2018, 59, 301–320. [Google Scholar] [CrossRef]
- Seifa, M.S.; Inoue, Y. Dynamic analysis of floating bridges. Mar. Struct. 1998, 11, 29–46. [Google Scholar] [CrossRef]
- Karczewski, A.; Piątek, Ł. The Influence of the Cuboid Float’s Parameters on the Stability of a Floating Building. Pol. Marit. Res. 2020, 3, 16–21. [Google Scholar] [CrossRef]

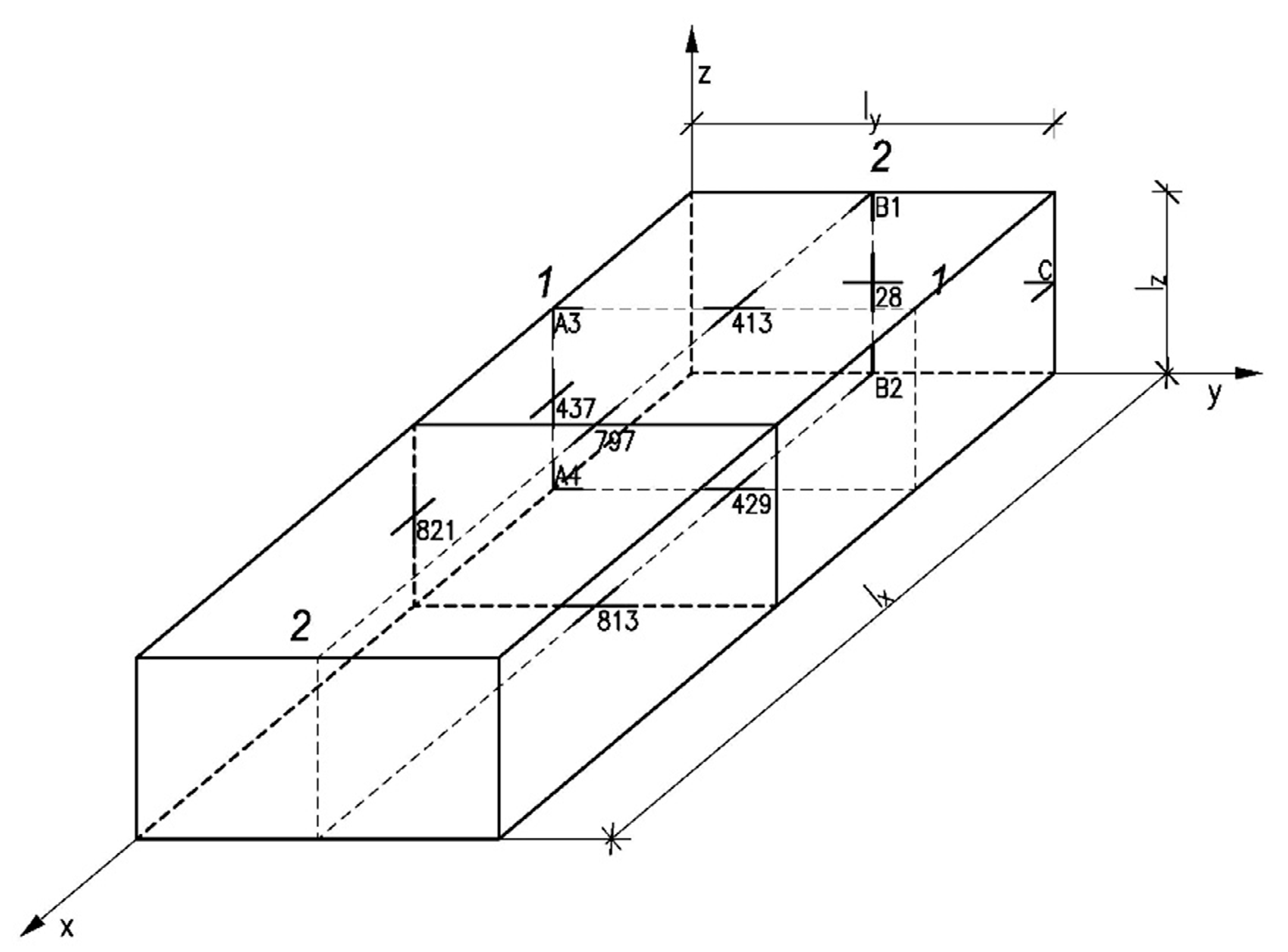
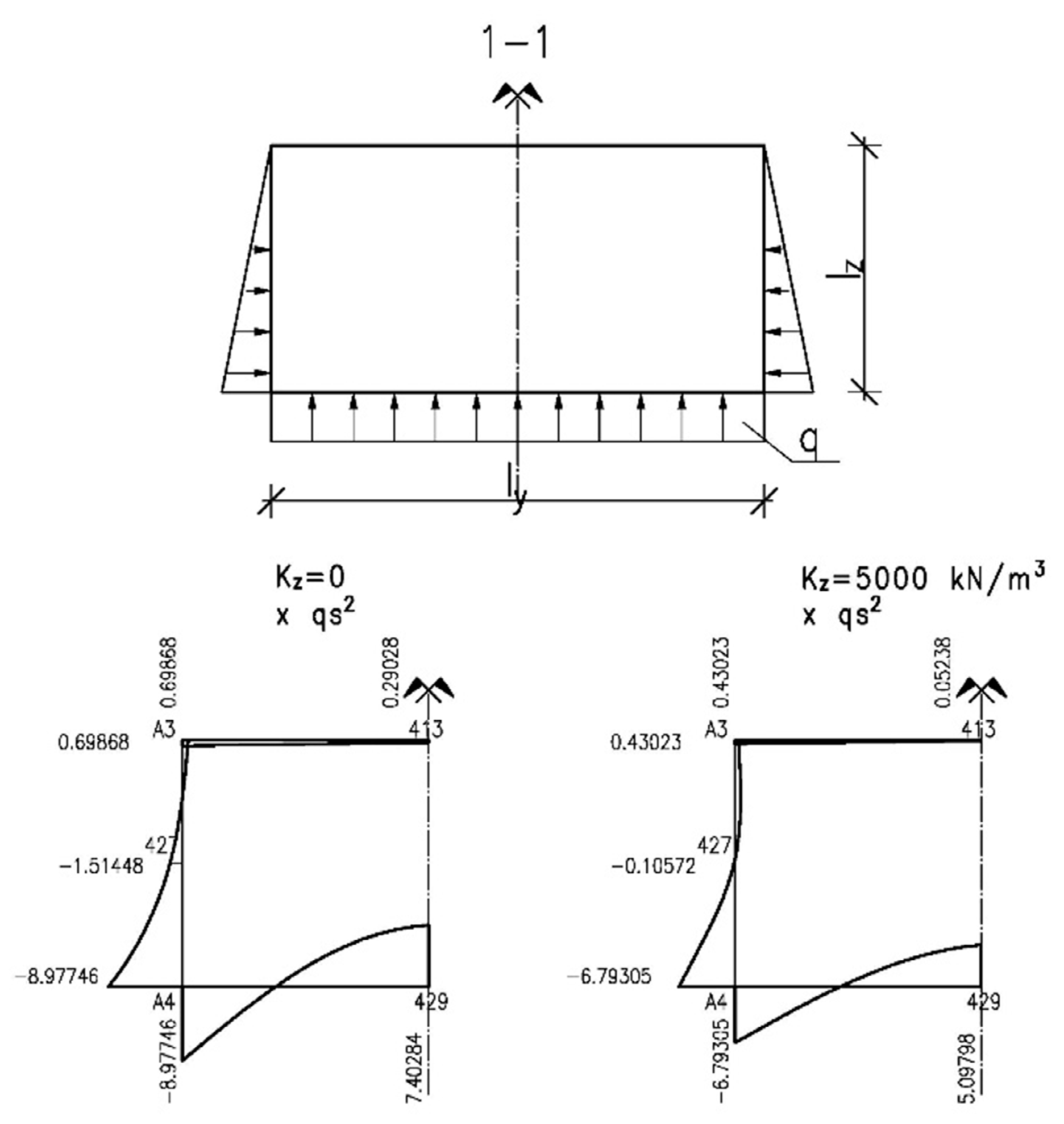
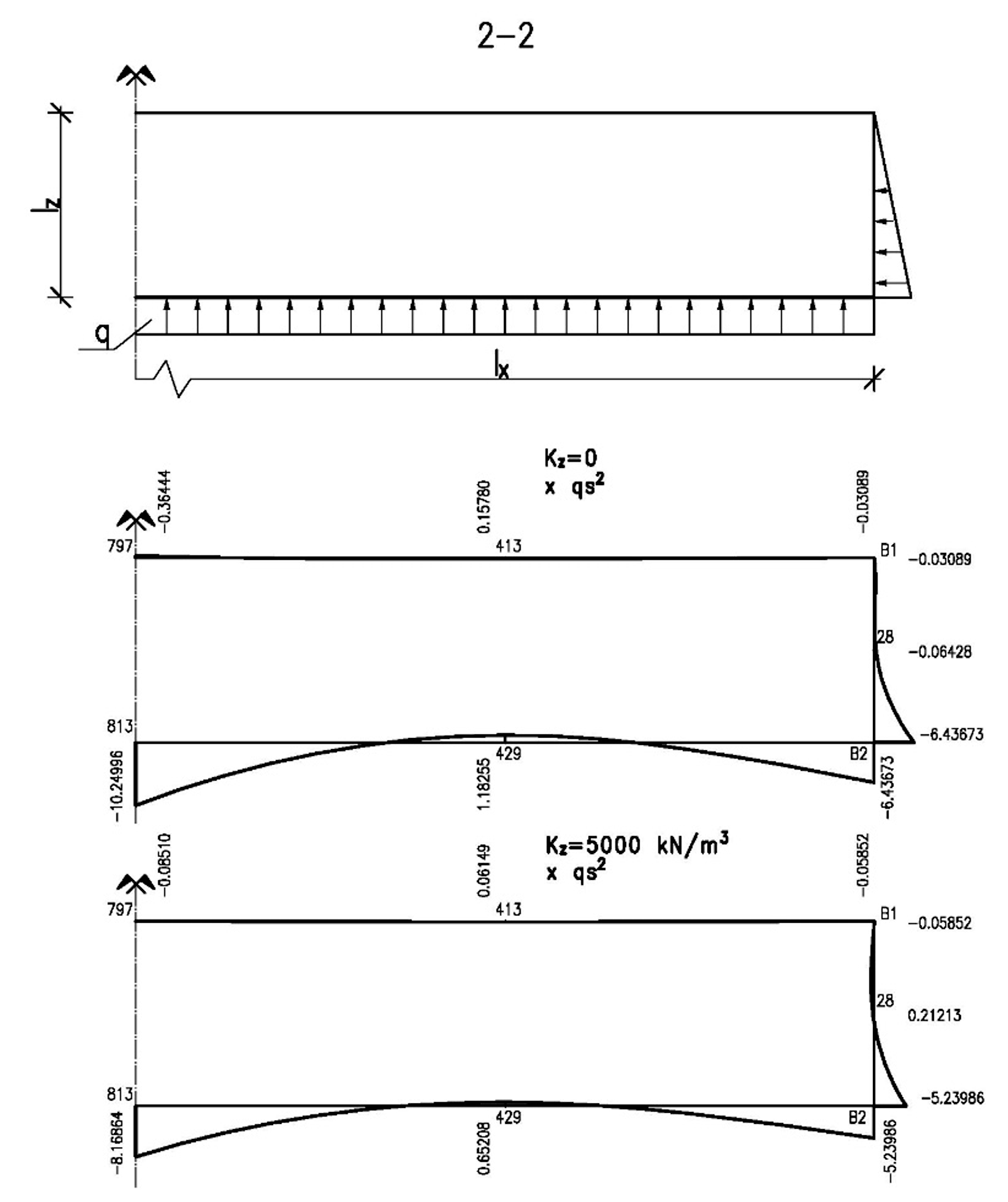
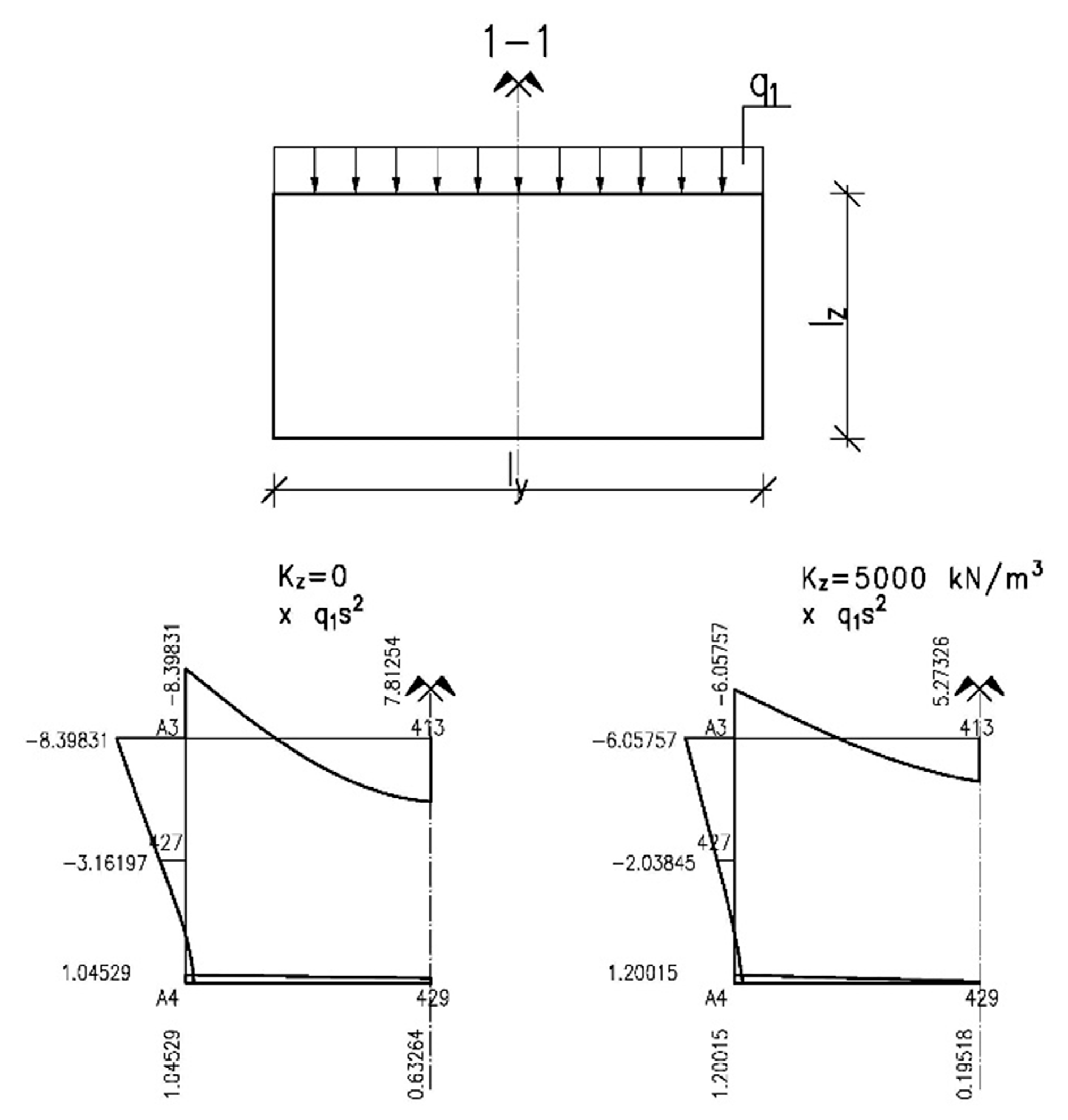


| Analyzed Value acc. Figure 4 |  |  |  | 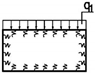 |  | 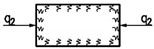 |
|---|---|---|---|---|---|---|
| K = 5000 kN/m3 | K = 5000 kN/m3 | K = 5000 kN/m3 | ||||
| MA3 | 0.69868 qs2 | 0.43023 qs2 | −8.39831q1s2 | −6.05757 q1s2 | −0.27892 q2s | −0.34647 q2s |
| MA4 | −8.97746 qs2 | −6.79305 qs2 | 1.20015 q1s2 | 1.04529 q1s2 | −0.27892 q2s | −0.34647 q2s |
| MB1 | −0.03089 qs2 | −0.05852 qs2 | −5.88475 q1s2 | −4.57988 q1s2 | −0.25756 q2s | −0.30472 q2s |
| MB2 | −6.43673 qs2 | −5.23986 qs2 | 0.42435 q1s2 | 0.47834 q1s2 | −0.25756 q2s | −0.30472 q2s |
| MC | 0.30288 qs2 | −0.12571 qs2 | 1.95192 q1s2 | 1.39644 q1s2 | −1.03379 q2s | −0.96737 q2s |
| My28 | −0.32098 qs2 | −0.18282 qs2 | −0.61660 q1s2 | −0.43870 q1s2 | 0.16188 q2s | 0.14114 q2s |
| Mz28 | −0.06428 qs2 | 0.21213 qs2 | −1.28998 q1s2 | −0.91521 q1s2 | 0.95926 q2s | 0.90710 q2s |
| My413 | 0.29028 qs2 | 0.05238 qs2 | 7.81254 q1s2 | 5.27326 q1s2 | −0.19180 q2s | −0.08074 q2s |
| Mx413 | 0.15780 qs2 | 0.06149 qs2 | 1.29175 q1s2 | 0.70420 q1s2 | −0.05392 q2s | −0.02615 q2s |
| My429 | 7.40284 qs2 | 5.09798 qs2 | 0.63264 q1s2 | 0.19518 q1s2 | −0.19180 q2s | −0.08074 q2s |
| Mx429 | 1.18255 qs2 | 0.65208 qs2 | 0.25493 q1s2 | 0.10836 q1s2 | −0.05392 q2s | −0.02615 q2s |
| Mx437 | −0.19095 qs2 | −0.10572 qs2 | −0.22838 q1s2 | −0.12710 q1s2 | 0.01971 q2s | 0.01140 q2s |
| Mz437 | −1.51448 qs2 | −0.61475 qs2 | −3.16197 q1s2 | −2.03845 q1s2 | 1.18854 q2s | 1.07084 q2s |
| Mx797 | −0.36444 qs2 | −0.08510 qs2 | −10.87368 q1s2 | −8.58140 q1s2 | 0.28786 q2s | 0.18698 q2s |
| Mx813 | −10.24996 qs2 | −8.16864 qs2 | −0.87588 q1s2 | −0.41450 q1s2 | 0.28786 q2s | 0.18698 q2s |
| Mx821 | 0.13284 qs2 | −0.22996 qs2 | 1.62748 q1s2 | 1.16812 q1s2 | −0.95842 q2s | −0.90726 q2s |
| Bottom Thickness of the Analysed Tanks (m) | Pontoon Buoyancy | Pontoon Stability with Half of the Upper Plate Loaded 1.0 kN/m2 | |||||
|---|---|---|---|---|---|---|---|
| At Self-Weight Load | At Uniform Load 3.0 kN/m2 | ||||||
| Immersion Depth hd (m) | Freeboard hf (m) | Immersion Depth hd (m) | Freeboard hf (m) | Metacentric Height hmc (m) | Tilt Angle φ (°) | Freeboard hf (m) | |
| 0.08 | 0.72 | 0.53 | 1.02 | 0.23 | 0.33 | 7.00 | 0.33 |
| 0.08 * | 0.75 * | 0.50 * | 1.05 * | 0.20 * | 0.32 * | 6.92 * | 0.30 * |
| * results for the pontoon with 5% concrete absorbability. | |||||||
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Szymczak-Graczyk, A. The Effect of Subgrade Coefficient on Static Work of a Pontoon Made as a Monolithic Closed Tank. Appl. Sci. 2021, 11, 4259. https://doi.org/10.3390/app11094259
Szymczak-Graczyk A. The Effect of Subgrade Coefficient on Static Work of a Pontoon Made as a Monolithic Closed Tank. Applied Sciences. 2021; 11(9):4259. https://doi.org/10.3390/app11094259
Chicago/Turabian StyleSzymczak-Graczyk, Anna. 2021. "The Effect of Subgrade Coefficient on Static Work of a Pontoon Made as a Monolithic Closed Tank" Applied Sciences 11, no. 9: 4259. https://doi.org/10.3390/app11094259
APA StyleSzymczak-Graczyk, A. (2021). The Effect of Subgrade Coefficient on Static Work of a Pontoon Made as a Monolithic Closed Tank. Applied Sciences, 11(9), 4259. https://doi.org/10.3390/app11094259






