Steady State Response of Linear Time Invariant Systems Modeledby Multibond Graphs
Abstract
1. Introduction
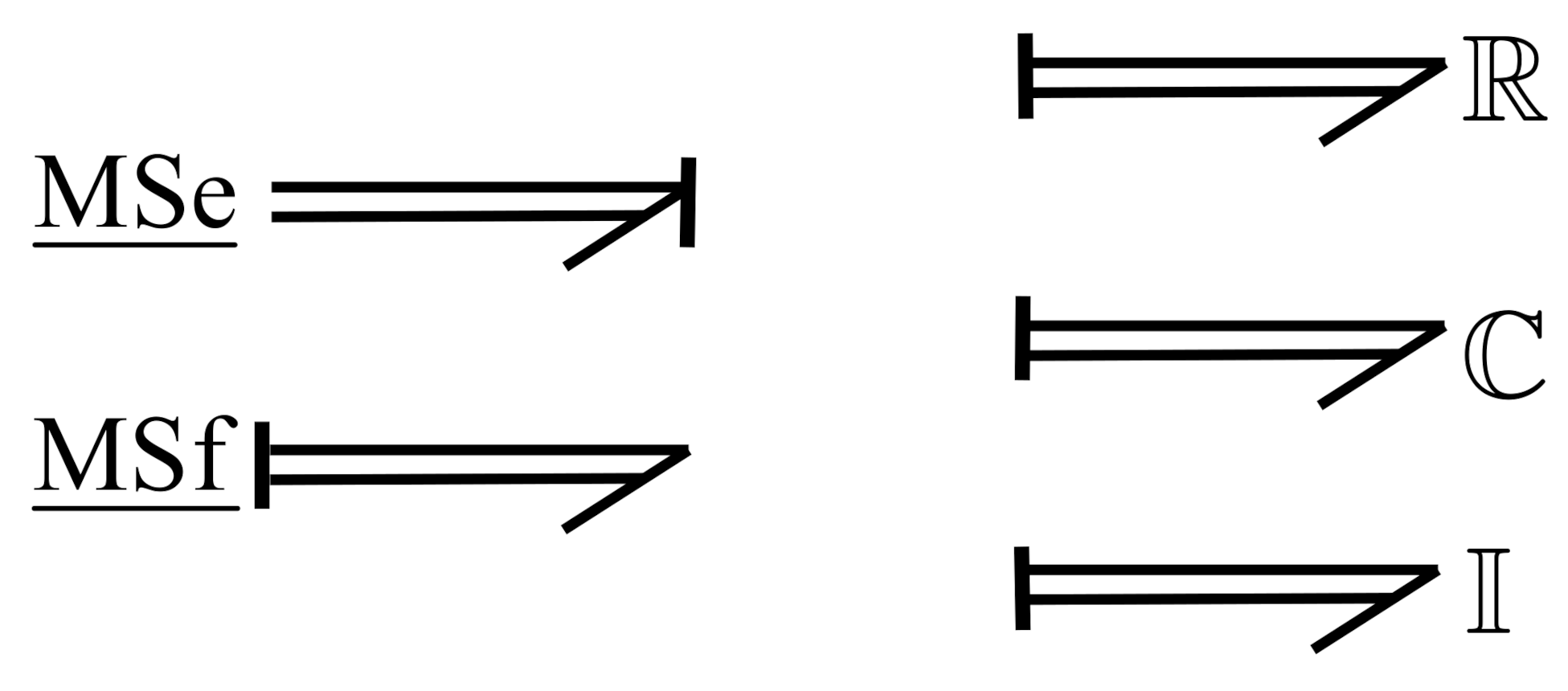
2. Modeling in a Bond Graph
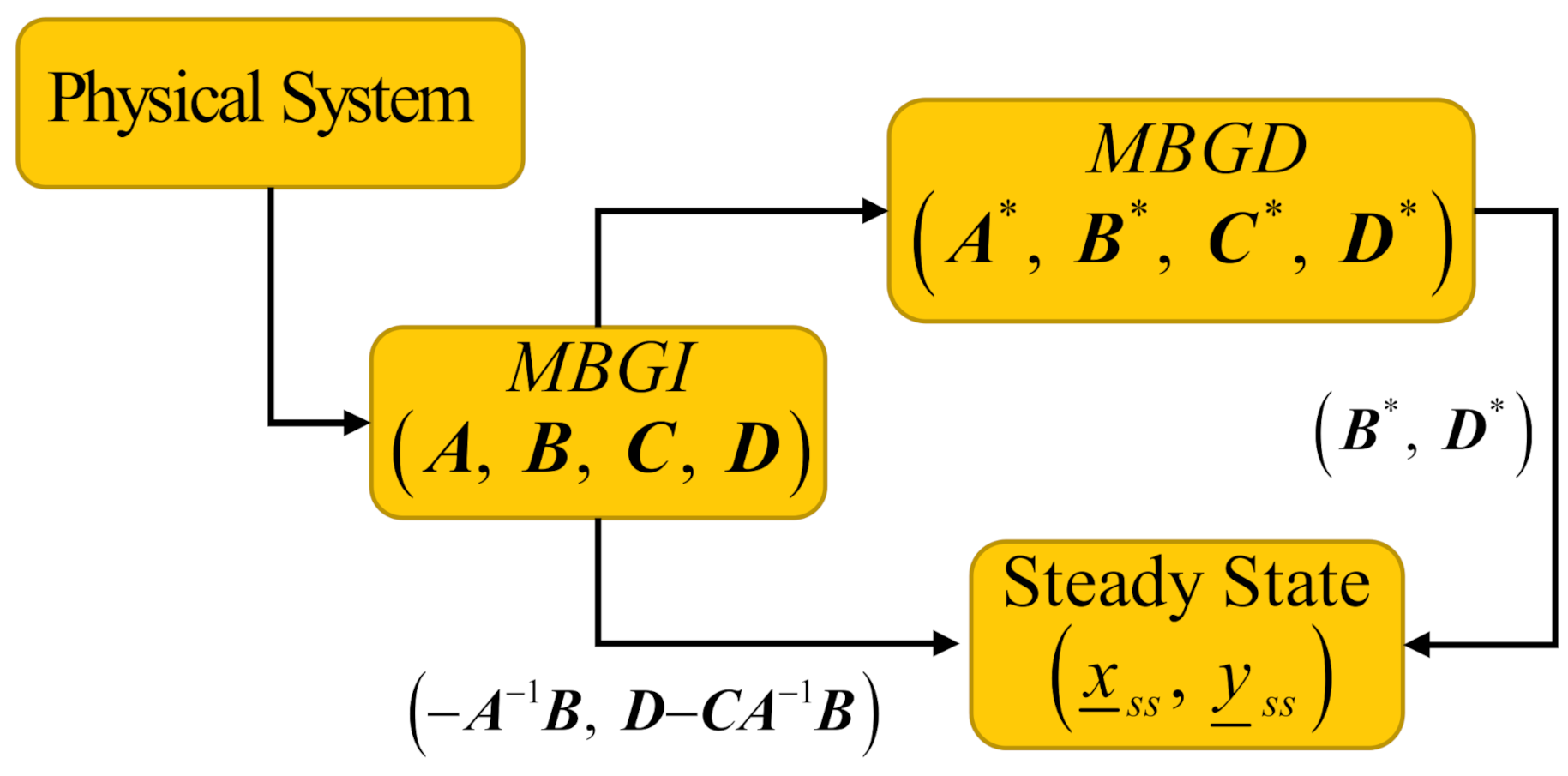
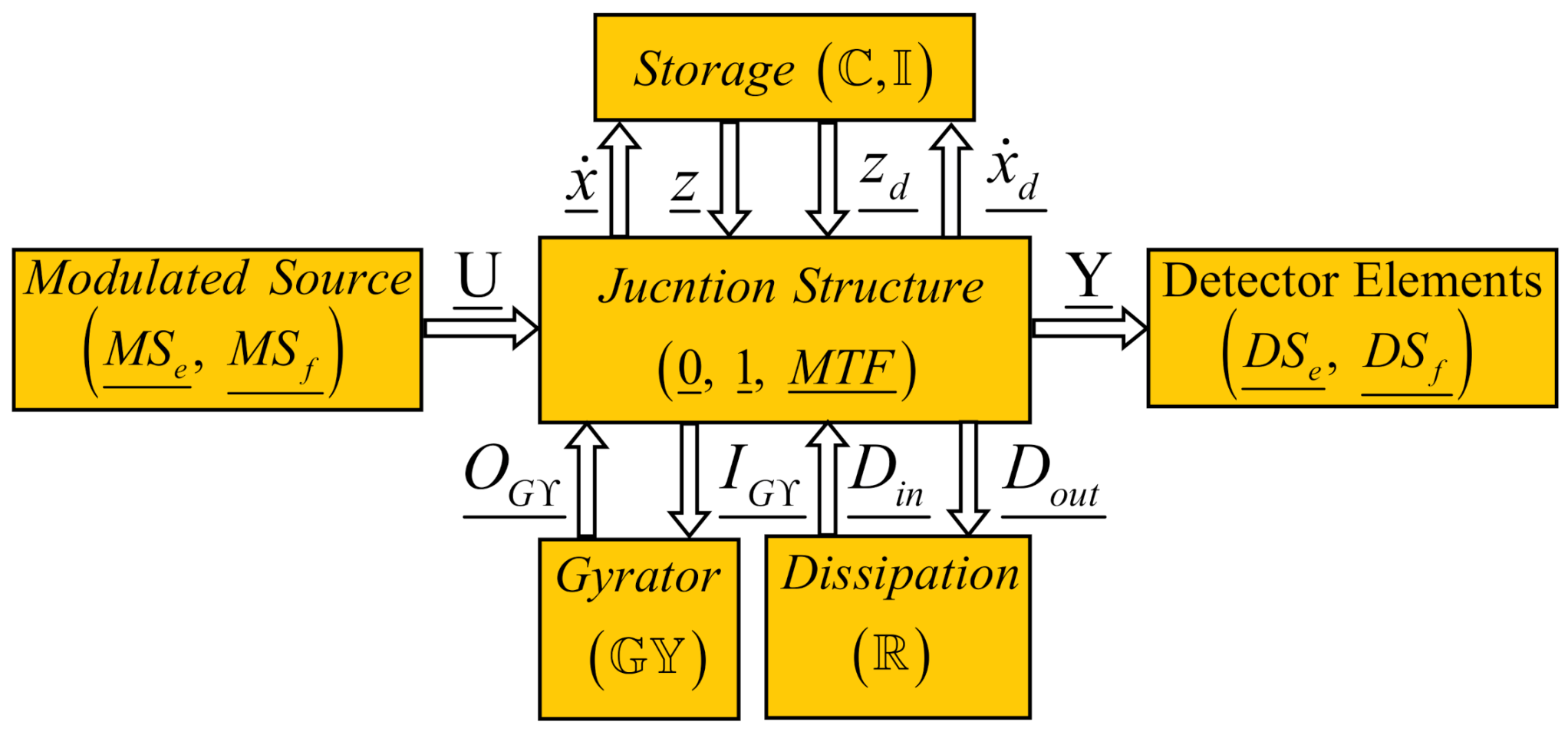
3. A Multibond Graph in an Integral Causality Assignment
- represent the effort and flow modulated multiport sources.
- denote the multiport storage elements defined by multiport capacitance and inertia, respectively.
- are the multiport dissipation elements that constitute the multiport resistors.
- represent the multiport junction structure with and multiport junctions, and the multiport transformers are denoted by .
- is the multiport gyrator.
- determine the detectors for the effort and flow, respectively.
- and represent the multiport state variables for multiport storage elements in integral and derivative causality assignments, respectively.
- and are the co-energy vectors for multiport storage elements in integral and derivative causality assignments, respectively.
- and denote the inputs and outputs of the multiport gyrators.
- and represent the relationships between the multiport junction structure and multiport dissipation elements.
- and determine the multiport inputs and outputs of the system.
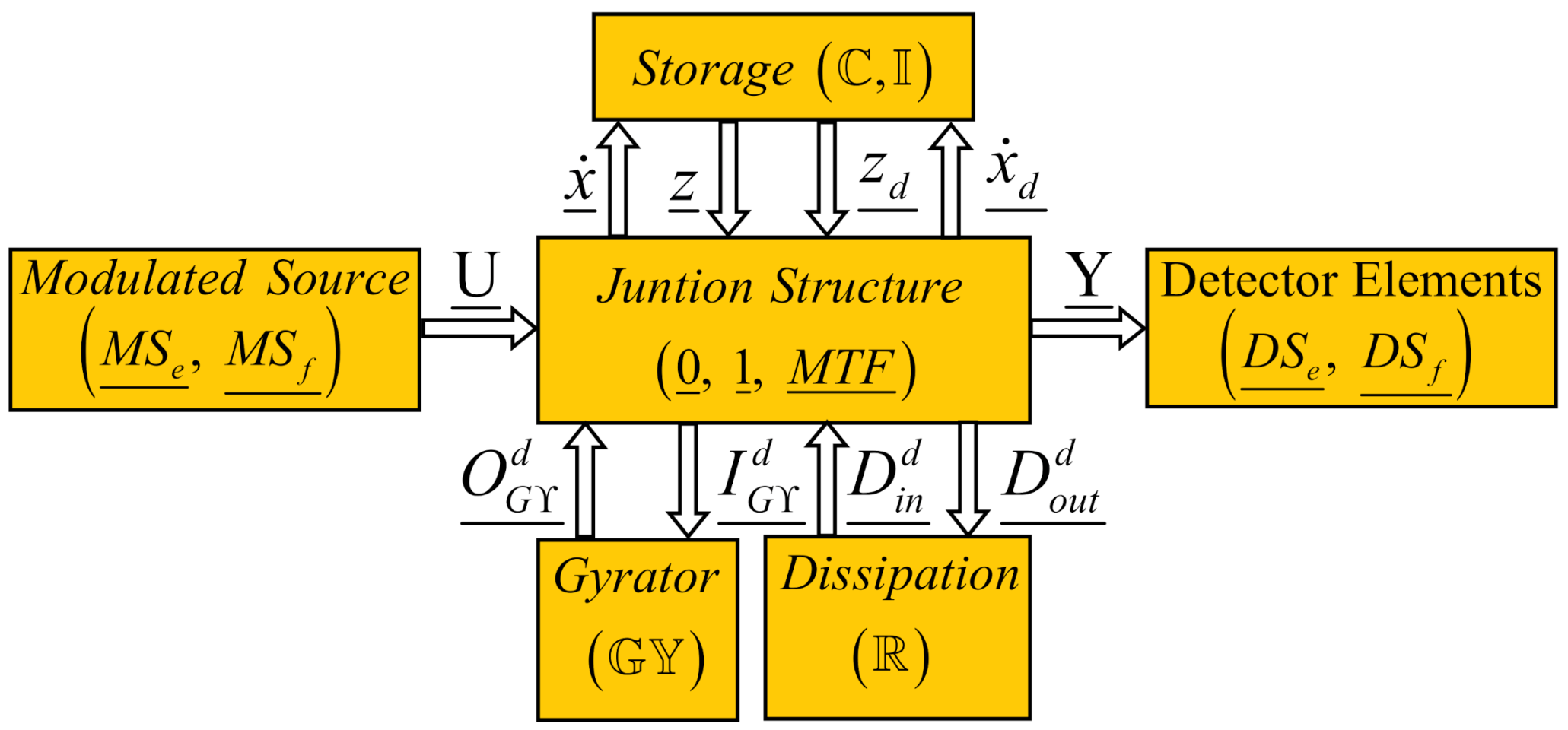
4. A Multibond Graph in a Derivative Causality Assignment
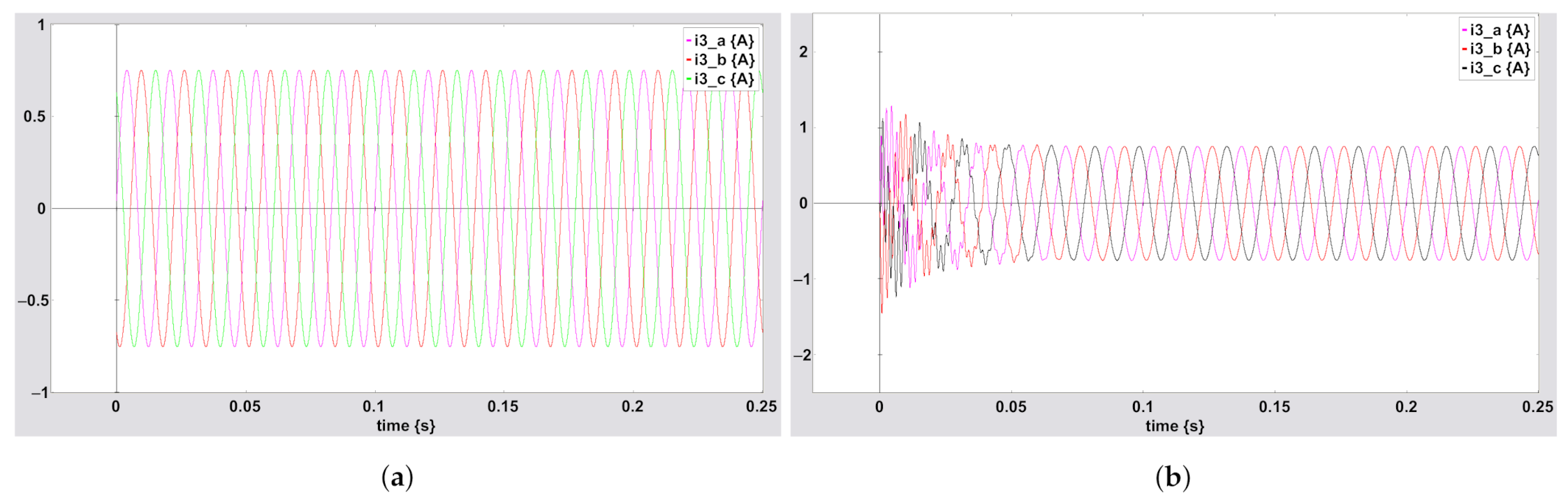
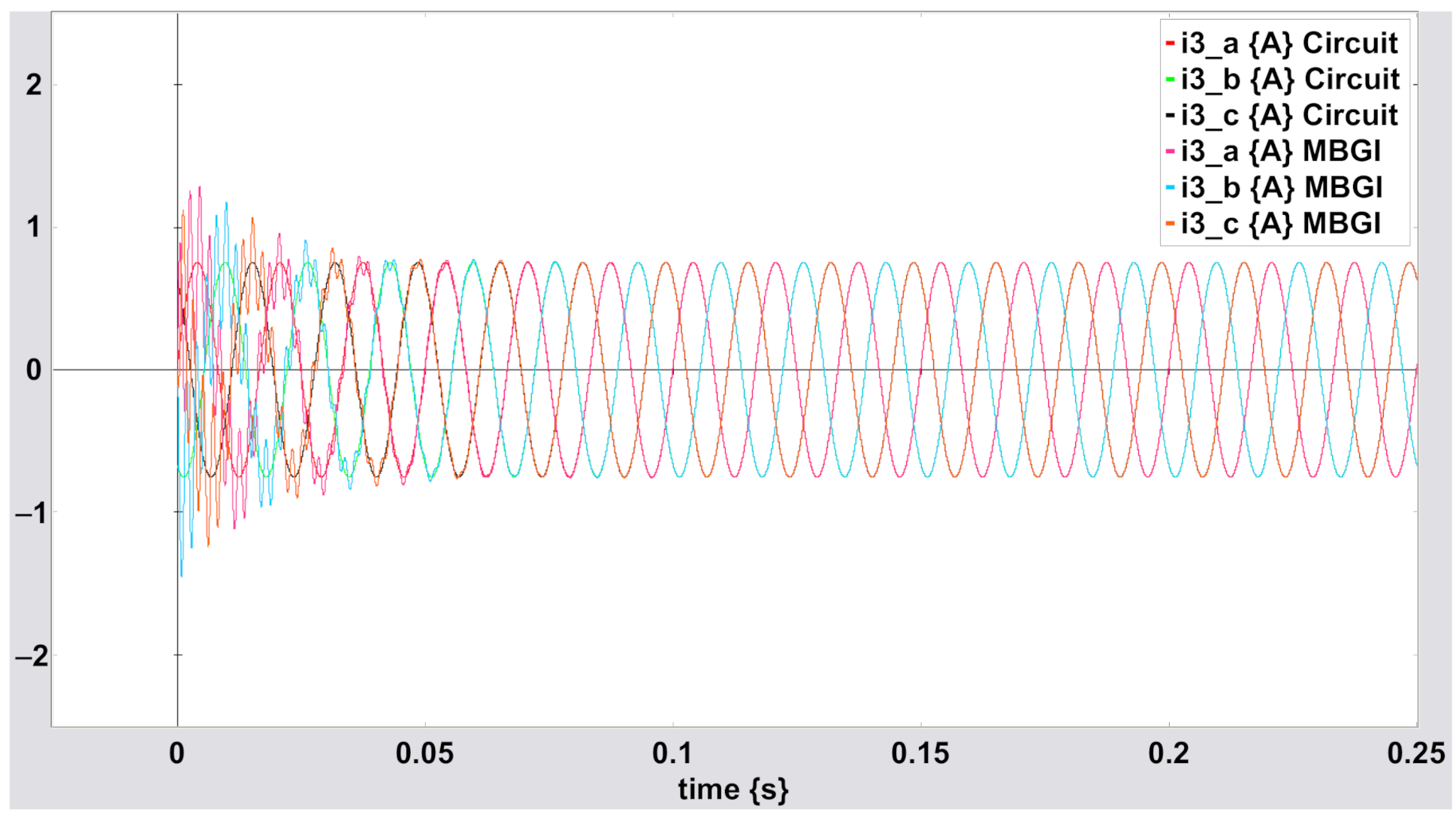
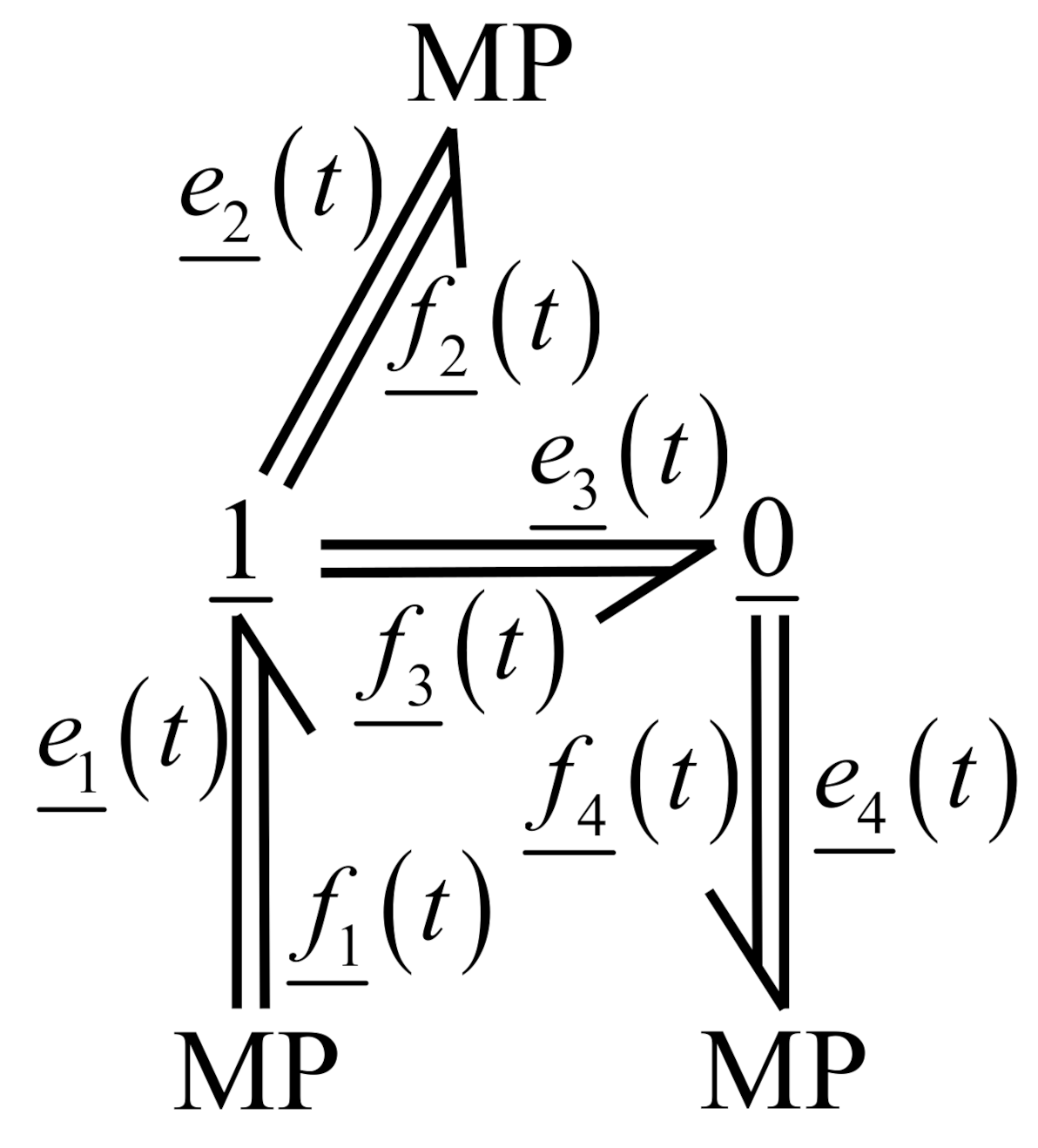
5. Case Study
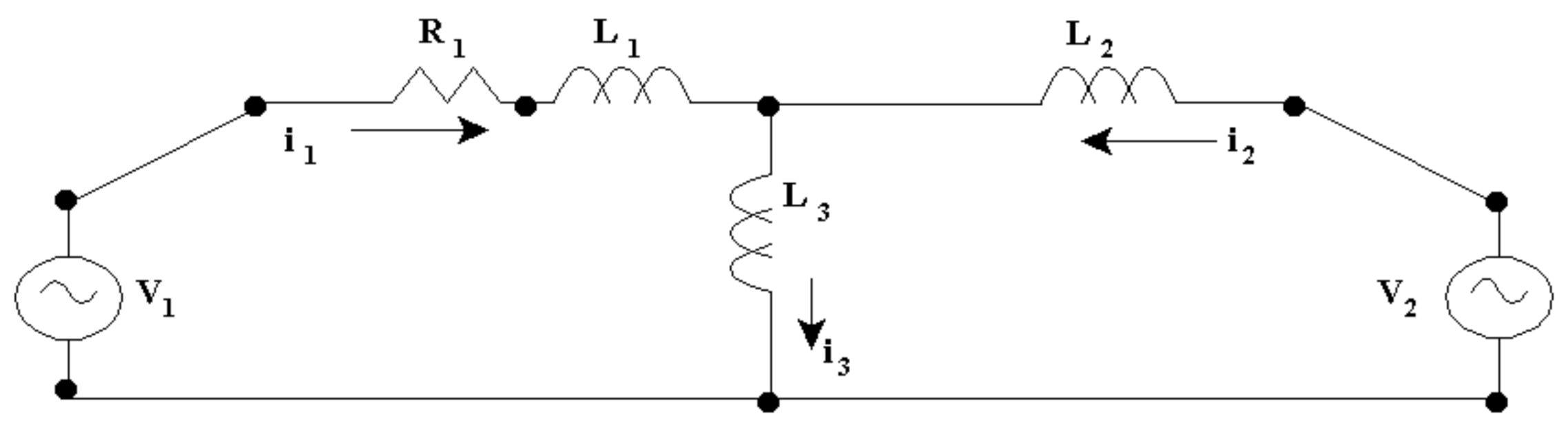
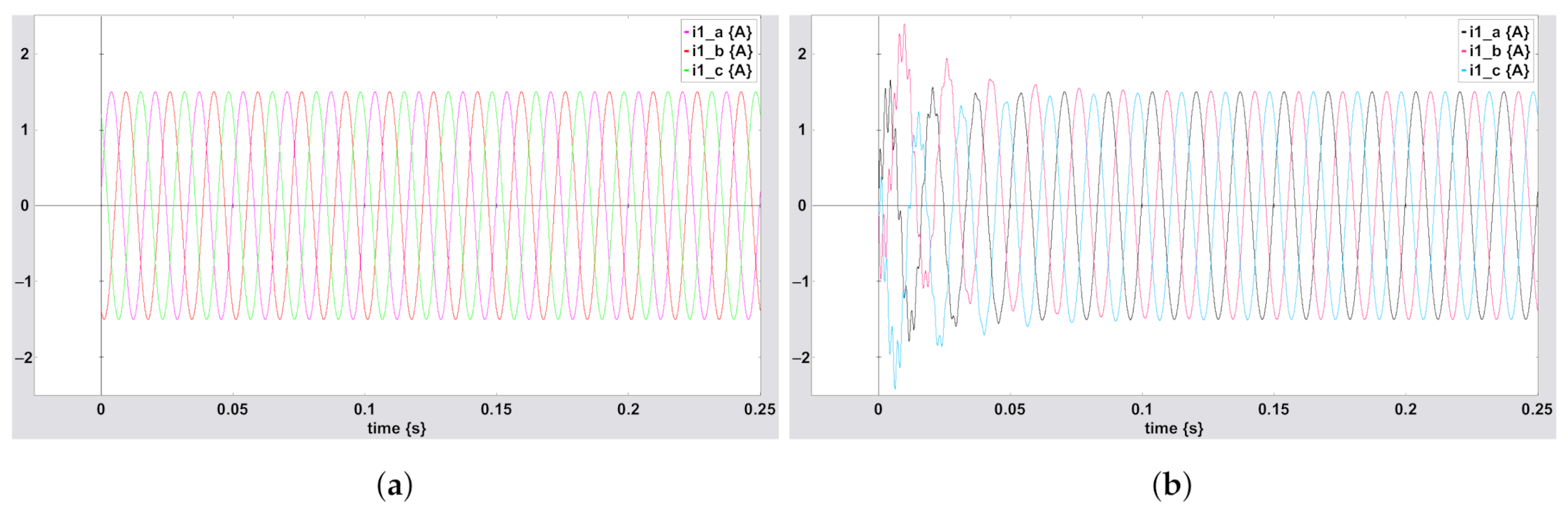
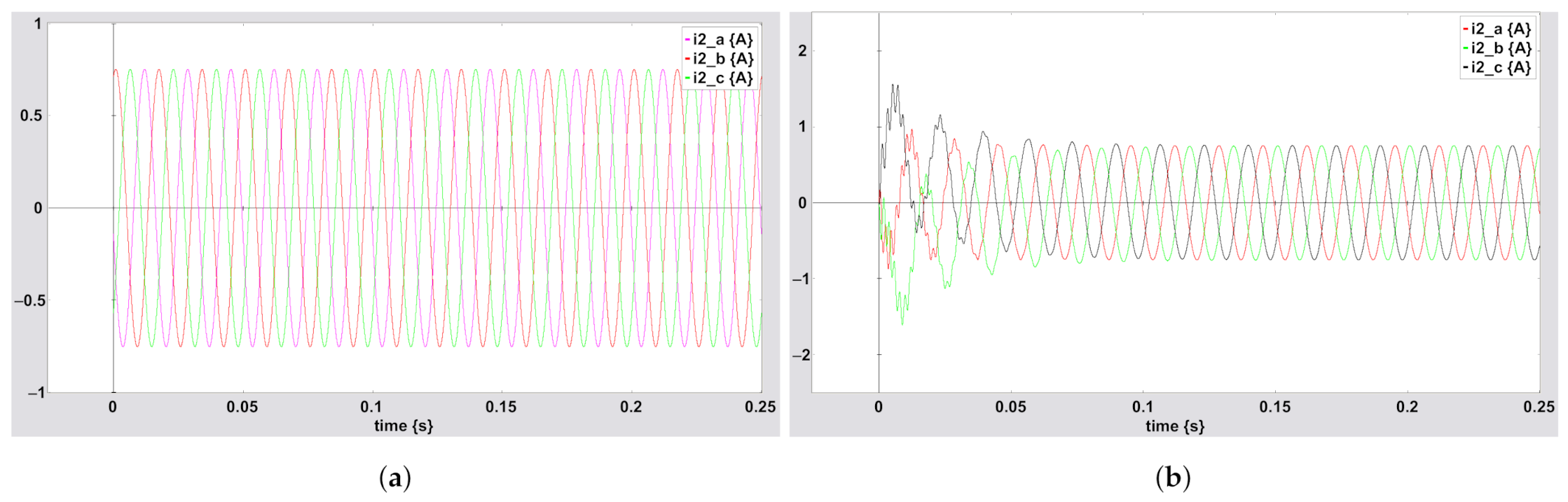
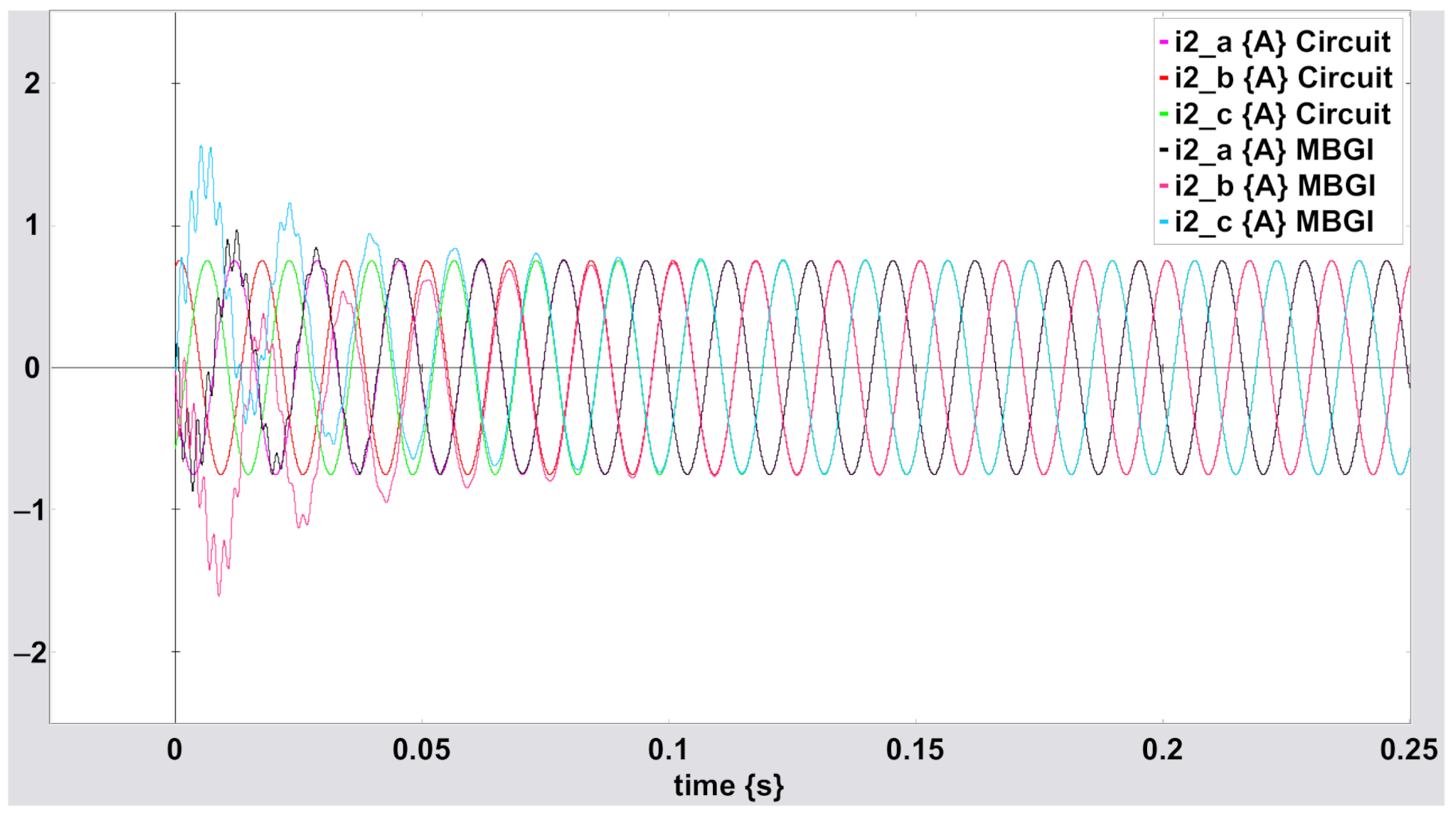
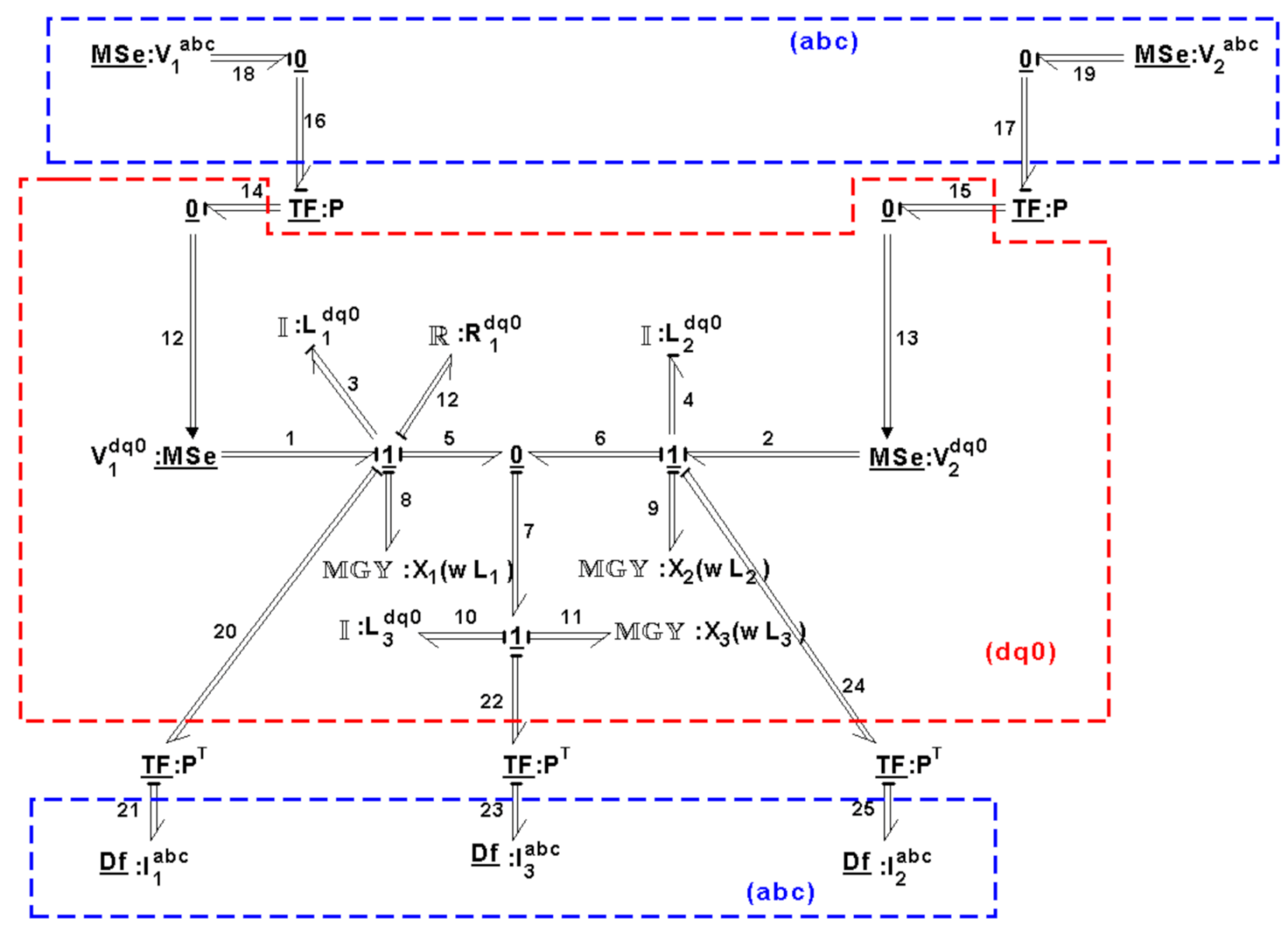
5.1. Three-Phase Electrical System
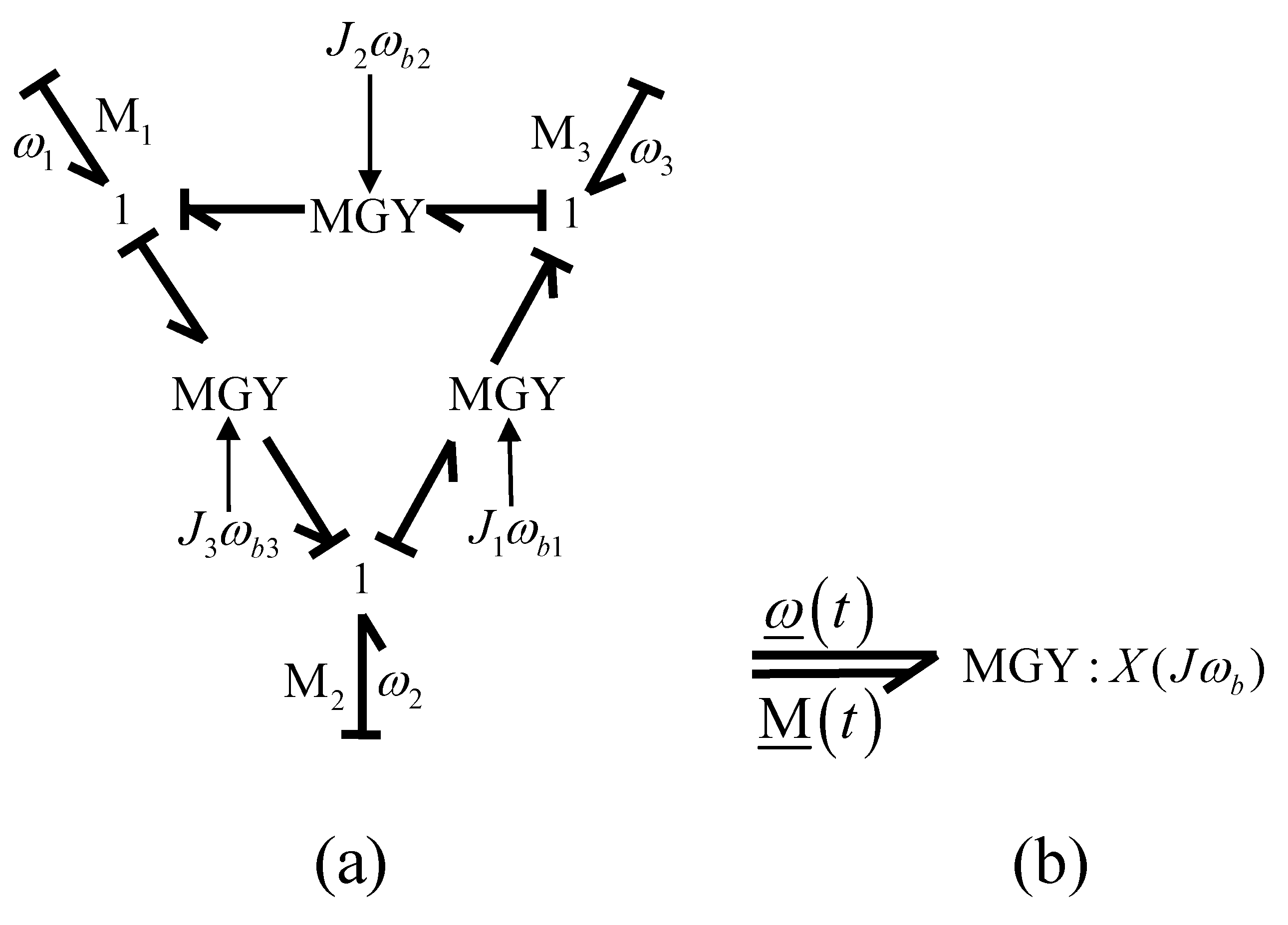
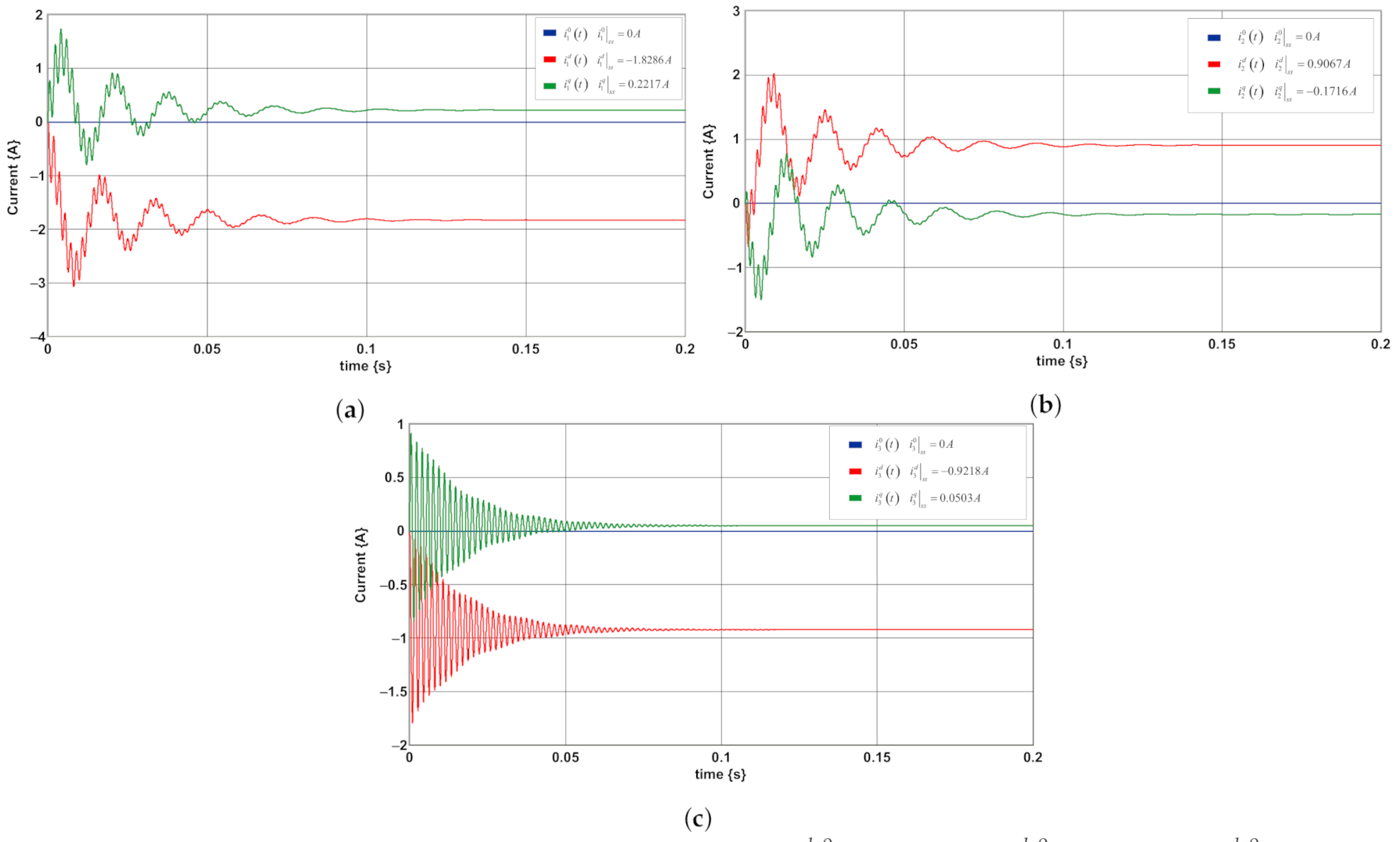
5.2. Synchronous Generator
- Stator circuits formed by three phase windings on the d-q axis where and denote the resistance and self-inductance on the d-axis circuit; and denote the resistance and self-inductance on the q-axis circuit; M is the mutual inductance between the stator and rotor; and are the supply voltages on -q axis; and the mechanical part whose elements are the mechanical torque, J the moment of inertia, and D the mechanical friction.This section is modeled by a multibond graph where , , and are effort sources that determine a multiport effort source ; the resistances on the d-q axis and the friction give a multiport resistance ; the inductances , , and J represent a multiport field with the mutual inductance M linking the inductance rotor circuit ; the relationships between the electrical circuits and the mechanical part are represented by a multiport gyrator .
- The rotor circuit formed by the resistance and inductance on the field winding and and the supply voltage for this winding .
6. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
Appendix A. Proof of Lemma 1
Appendix B. Proof of Lemma 2
Appendix C. Proof of Theorem
Appendix D. Calculation of P X
Appendix A. Mesh Current Solution

References
- Nise, N.S. Control Systems Engineering; Wiley: New York, NY, USA, 2000. [Google Scholar]
- Pagnozzi, M.; Coletta, G.; Leone, G.; Catani, V.; Esposito, L.; Fiorillo, F. A Steady State Model to Simulate Groundwater Flow in Unconfined Aquifer. Appl. Sci. 2020, 10, 2708. [Google Scholar] [CrossRef]
- Chang, C.-W.; Liu, C.-H.; Wang, C.-C. A Modified Polynomial Expansion Algorithm for Solving The Staedy-State Allen-Cahn Equation for Heat Transfer in Thin Films. Appl. Sci. 2018, 8, 983. [Google Scholar] [CrossRef]
- Senturia, S.D. Microsystem Design; Kluwer Academic Publishers: Dordrecht, The Netherlands, 2002. [Google Scholar]
- Yu, M.; Lu, H.; Wang, H.; Xiao, C.; Lan, D. Compound Fault Diganosis and Sequential Prognosis for Electric Scooter with Uncertainties. Actuators 2020, 9, 128. [Google Scholar] [CrossRef]
- Barjuei, E.S.; Caldwell, D.G.; Ortiz, J. Bond Graph Modeling and Kalman Filter Observer Design for an Industrial Back-Support Exoskeleton. Designs 2020, 4, 53. [Google Scholar] [CrossRef]
- Zrafi, R.; Ghedira, S.; Besbes, K. A Bond Graph Approach for the Modeling and Simulation of a Buck Converter. J. Low Power Electron. Appl. 2018, 8, 2. [Google Scholar] [CrossRef]
- Lin, C.; Shen, Z.; Yu, J.; Li, P.; Huo, D. Modeling and Analysis of Characteristics of a Piezoelectric-Actuated Micro-/Nano Compliant Platform Using Bond Graph Approach. Micromachines 2018, 9, 498. [Google Scholar] [CrossRef]
- Algar, A.; Codina, E.; Freire, J. Bond Graph Simulation of Error Propagation in Position Estimation of a Hydraulic Cylinder Using Los Cost Accelerometers. Energies 2018, 11, 2603. [Google Scholar] [CrossRef]
- Van der Weff, K. Kinematic and Dynamic Analysis of Mechanisms, a Finite Element Approach; Delft University Press: Delft, The Netherlands, 1977. [Google Scholar]
- Zhang, W.J.; van der Werff, K. Automatic communication from a neutral object model of mechanism to mechanism analysis programs based in a finite element approach in a software enviroment for CADCAM of mechanisms. Finite Elem. Anal. Des. 1998, 28, 209–239. [Google Scholar] [CrossRef]
- Moon, W.-K.; Busch-Vishniac, I.J. A finite-element equivalent bond graph modeling approach with application to the piezoelectric thickness vibrator. J. Acoust. Am. 1993, 93, 3496. [Google Scholar] [CrossRef]
- Pal, S.K.; Talamantes-Silva, J.; Linkens, D.A.; Howard, I.C. Bond graph and finite element analyses of temperature distribution in a hot rolling process: A comparative study. Proc. Inst. Mech. Eng. Part I J. Syst. Control 2007, 221, 653–661. [Google Scholar] [CrossRef]
- Nakhaeinejad, M.; Lee, S.; Bryant, M.D. Finite element bond graph model of rotors. In Proceedings of the 2010 Spring Simulation Multiconference, Orlando, FL, USA, 11–15 April 2010; pp. 1–6. [Google Scholar]
- Breedveld, P.C. A bond graph algorithm to determine the equilibrium state of a system. J. Franklin Inst. 1984, 318, 71–75. [Google Scholar] [CrossRef]
- Gawthrop, P.J. Bicausal bond graphs. In Proceedings of the International Conference on Bond graph modeling (ICBGM’95), Las Vegas, NV, USA, 15–18 January 1995; pp. 83–88. [Google Scholar]
- Ngwompo, R.F.; Scarvada, R.; Thomasset, D. Inversion of linear time-invariant SISO systems modeled by bond graph. J. Frankl. Inst. 1996, 333, 157–174. [Google Scholar] [CrossRef]
- Bideaux, E.; Marquis-Favre, W.; Scavarda, S. Equilibrium set investigation using bicausality. Math. Comput. Model Dynam Syst. 2006, 12, 127–140. [Google Scholar] [CrossRef]
- Gonzalez, G.; Galindo, R. Steady state determination using bond graphs for systems with singular state matrix. Proc. IMechE Part I J. Syst. Control Eng. 2011, 225, 887–901. [Google Scholar] [CrossRef]
- Gawthrop, P. Computing Biomolecular System Steady-States. IEEE Trans. Nanobiosci. 2018, 17, 36–43. [Google Scholar] [CrossRef]
- Breedveld, P.C. Multibond Graph Elements in Physical Systems Theory. J. Frankl. Inst. 1985, 319, 1–36. [Google Scholar] [CrossRef]
- Borutzky, W.; Dauphin-Tanguy, G.; Thoma, J.U. Advances in bond graph modeling: Theory, software, applications. Math. Comput. Simul. 1995, 39, 465–475. [Google Scholar] [CrossRef]
- Boruttzky, W. Bond Graphs for Modeling, Control and Fault Diagnosis of Engineering Systems, 2nd ed.; Springer: Berlin/Heidelberg, Germany, 2017. [Google Scholar]
- Behzadipour, S.; Khajepour, A. Causality in vector bond graphs and its application to modeling of multi-body dynamic. Syst. Simul. Model. Pract. Theory 2006, 14, 279–295. [Google Scholar] [CrossRef]
- Breedveld, P.C. Stability of rigid body rotation from a bond graph perspective. Simul. Model. Pract. Theory 2009, 17, 92–106. [Google Scholar] [CrossRef]
- Breedveld, P.C. Essential gyrators and equivalence rules for 3-port junction structures. J. Franklin Inst. 1984, 318, 77–89. [Google Scholar] [CrossRef]
- Breedveld, P.C. Decomposition of multiport elements in a revised multibond graph notation. J. Franklin Inst. 1984, 318, 253–273. [Google Scholar] [CrossRef]
- Golo, G.; der Schaft, A.V.; Breedveld, P.C.; Marschke, B.M. Hamiltonian formulation of bond graphs. In Nonlinear and Hybrid Systems in Automotive Control; University of Gronigen, Johann Bernoulli, Institute for Mathematics and Computer Science: Groningen, The Netherlands, 2003; Volume 19, pp. 351–372. [Google Scholar]
- Nu nez, I.; Breedveld, P.C.; Weustink, P.B.T.; Gonzalez, G. Steady-state power flow analysis of electrical power systems modeled by 2- dimensional multibond graphs. In Proceedings of the International Conference on Integrated Modeling and Analysis in Applied Control and Automation, Bergeggi, Italy, 21–23 September 2015; pp. 39–47. [Google Scholar]
- Avalos, G.G.; Ayala, G.; Gallegos, N.B.; Padilla, A. Linearization of a class of non-linear systems modeled by multibond graphs. Math. Comput. Model. Dyn. 2019, 25, 284–332. [Google Scholar] [CrossRef]
- Abbes, M.; Farhat, A.; Mami, A.; Dauphin-Tanguy, G. Pseudo bond graph model of coupled heat and mass transfers in a plastic tunnel greenhouse. Simul. Model. Pract. Theory 2010, 18, 1327–1341. [Google Scholar] [CrossRef]
- Boudon, B.; Malburet, F.; Carmona, J. Simulation of a helicopter’s main gearbox semi-active suspension with bond graphs. Multibody Syst. Dyn. 2016, 39, 60–64. [Google Scholar]
- Sarker, M.; Rideout, D.G.; Butt, S.D. Dynamic model for 3D motions of a horizontal oil well BHA with wellbore stick-slip whirl interaction. J. Pet. Sci. Eng. 2017, 157, 482–506. [Google Scholar] [CrossRef]
- Pfeifer, M.; Caspart, S.; Hampel, S.; Muller, C.; Krebs, S.; Hohmann, S. Explicit port-Hamiltonian formulation of multi-bond graphs for an automated model generation. Automatica 2020, 120, 109121. [Google Scholar] [CrossRef]
- Lee, J.-N.; Nikravesh, P.E. Steady-state analysis of multibody systems with reference to vehicle dynamics. Nonlinear Dyn. 1994, 5, 181–192. [Google Scholar]
- Hendy, H.; Rui, X.; Zhou, Q. Controller Parameters tuning based on Transfer matrix method for multibody systems. Adv. Mech. Eng. 2015. [Google Scholar] [CrossRef]
- Dauphin-Tanguy, G.; Rahmani, A.; Sueur, C. Bond graph aided design of controlled systems. Simul. Pract. Theory 1999, 7, 493–513. [Google Scholar] [CrossRef]
- Sueur, C.; Dauphin-Tamguy, G. Bond-graph Approach for Structural Analysis of MIMO Linear Systems. J. Franklin Inst. 1991, 328, 55–70. [Google Scholar] [CrossRef]
- Avalos, G.G.; Gallegos, N.B. Quasi-steady state model determination for systems with singular perturbations modeled by bond graphs. Math. Comput. Model. Dyn. 2013, 19, 483–503. [Google Scholar] [CrossRef]
- Karnopp, D.C.; Margolis, D.L.; Rosenberg, R.C. System Dynamics Modeling and Simulation of Mechatronic Systems; Wiley, John & Sons: Hoboken, NJ, USA, 2000. [Google Scholar]
- Banerjee, S. Dynamics for Engineers; Wiley: Hoboken, NJ, USA, 2005. [Google Scholar]
- Kundur, J.R. Power System Stability and Control; Mc. Graw-Hill: New York, NY, USA, 1994. [Google Scholar]
- Anderson, P.M. Power System Control and Stability; The Iowa State University Press: Ames, IA, USA, 1977. [Google Scholar]
- Zhou, K.; Doyle, J.C. Essentials of Robust Control; Prentice-Hall: Upper Saddle River, NJ, USA, 1998. [Google Scholar]


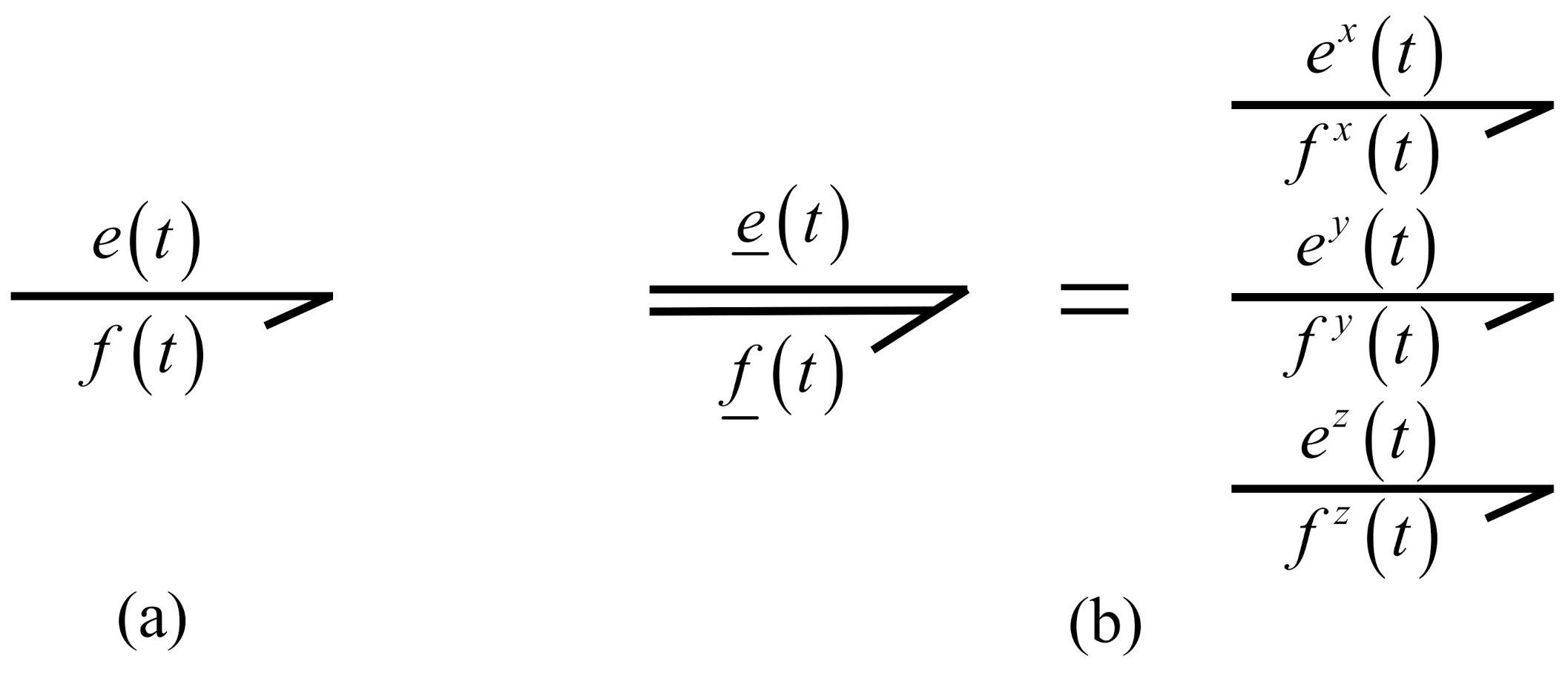


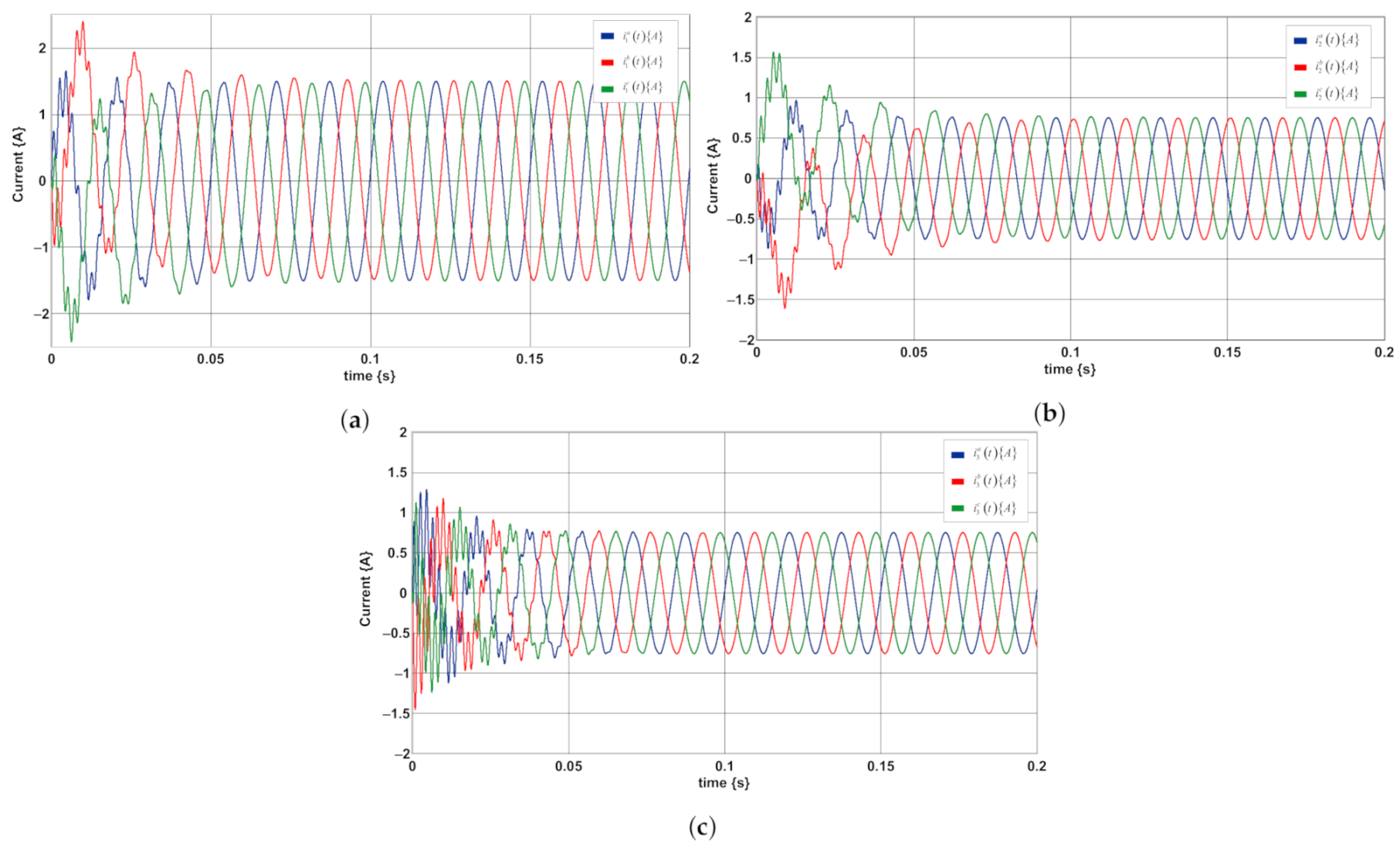

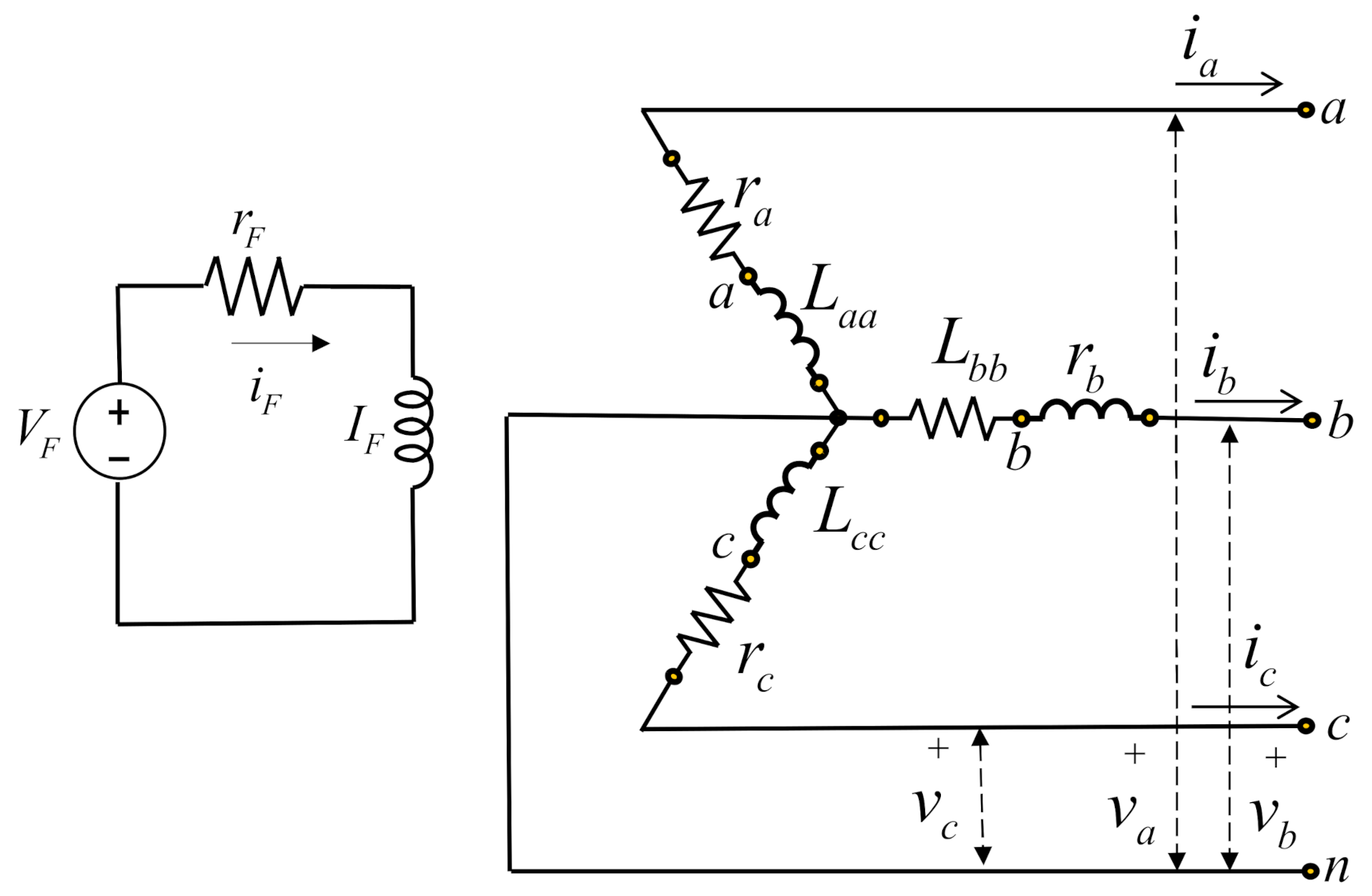
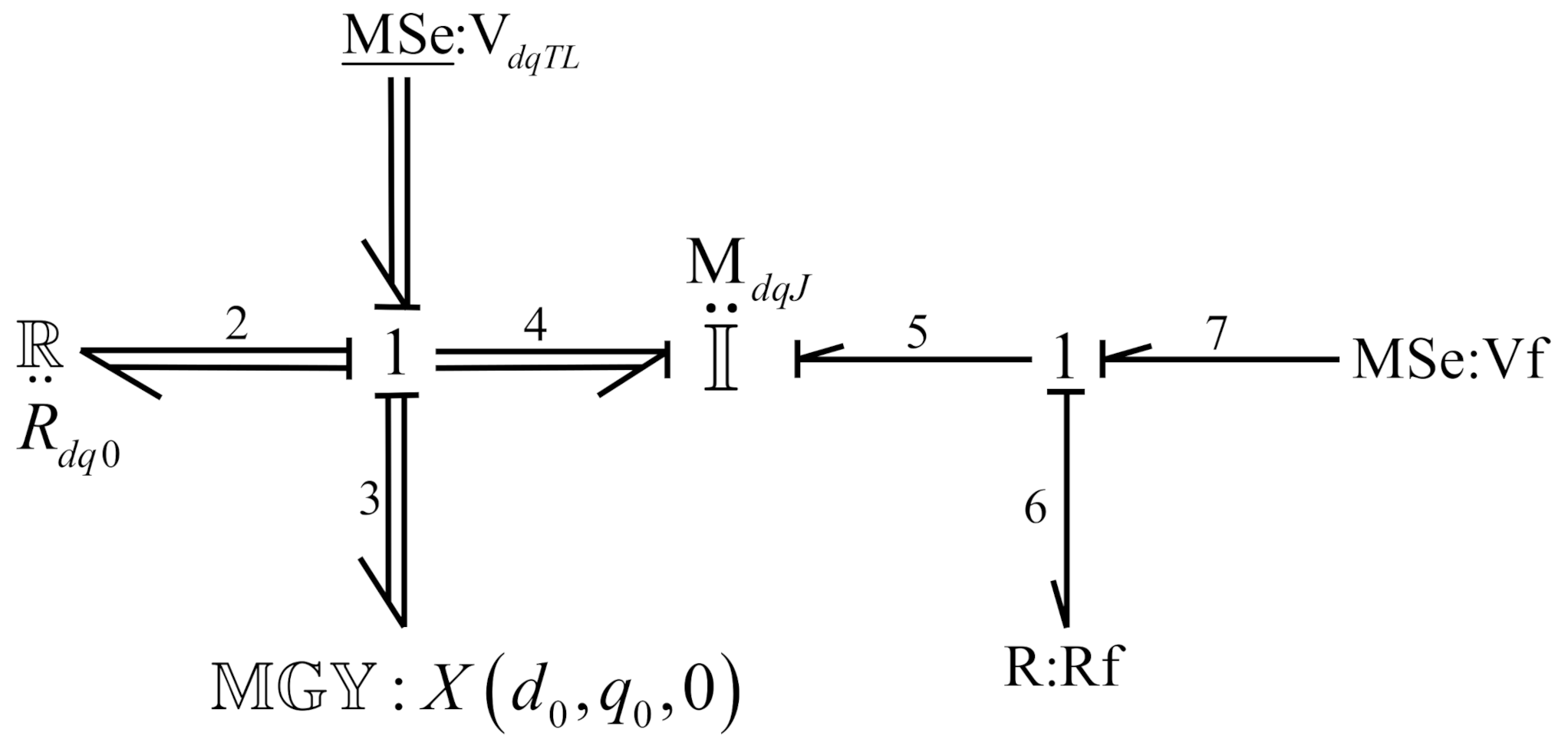

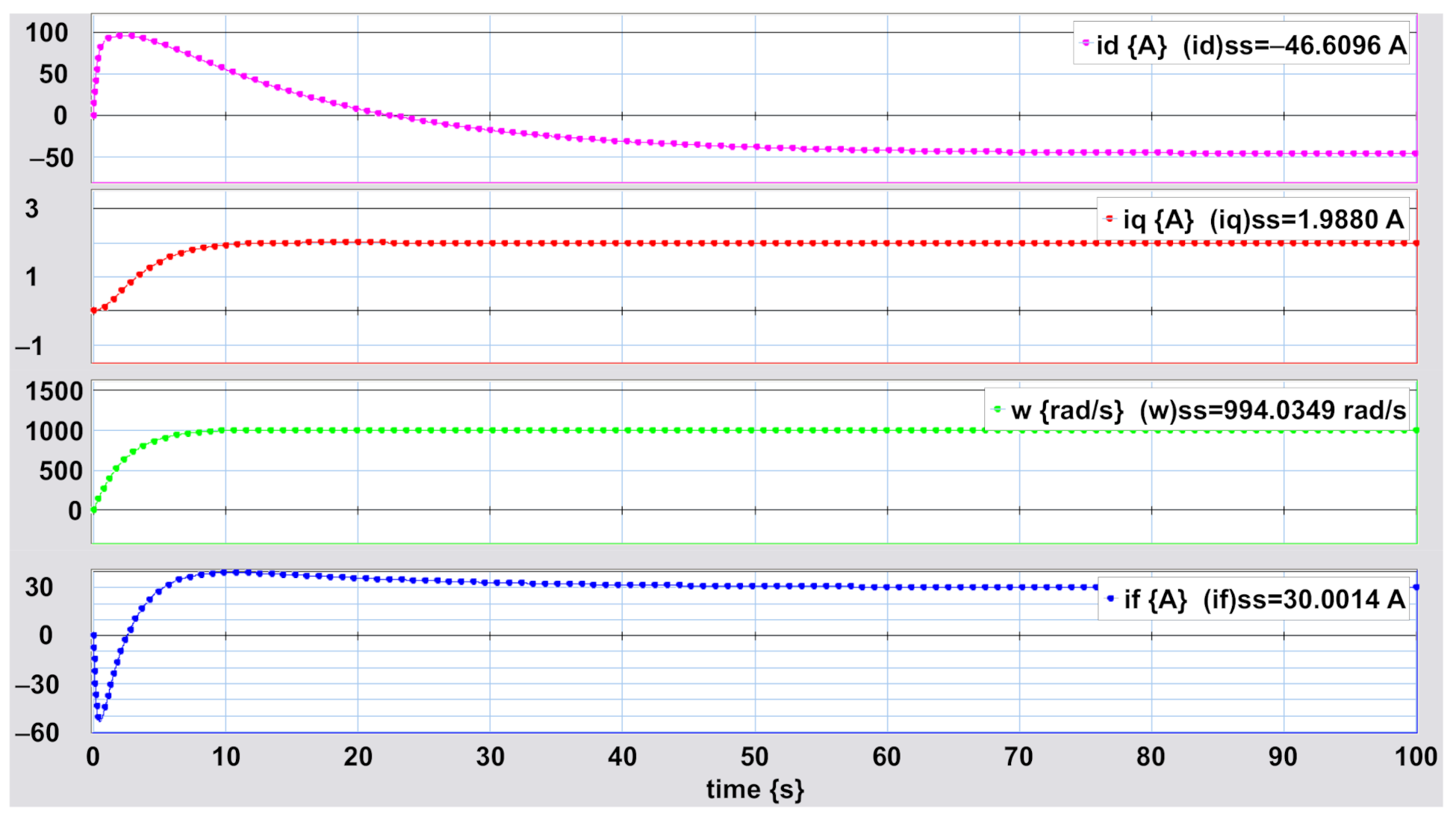
| System | Effort | Flow |
|---|---|---|
| Mechanical | Force | Ang.velocity |
| Torque | Velocity | |
| Electrical | Voltage | Current |
| Hydraulic | Pressure | Volume flow rate |
| Element | Causal Form | Causal Relation |
|---|---|---|
| Effort Source |  | |
| Flow Source |  | |
| Resistance |  | |
| Capacitance |  | |
| Inertia |  |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Gonzalez Avalos, G.; Barrera Gallegos, N.; Ayala-Jaimes, G.; Padilla Garcia, A. Steady State Response of Linear Time Invariant Systems Modeledby Multibond Graphs. Appl. Sci. 2021, 11, 1717. https://doi.org/10.3390/app11041717
Gonzalez Avalos G, Barrera Gallegos N, Ayala-Jaimes G, Padilla Garcia A. Steady State Response of Linear Time Invariant Systems Modeledby Multibond Graphs. Applied Sciences. 2021; 11(4):1717. https://doi.org/10.3390/app11041717
Chicago/Turabian StyleGonzalez Avalos, Gilberto, Noe Barrera Gallegos, Gerardo Ayala-Jaimes, and Aaron Padilla Garcia. 2021. "Steady State Response of Linear Time Invariant Systems Modeledby Multibond Graphs" Applied Sciences 11, no. 4: 1717. https://doi.org/10.3390/app11041717
APA StyleGonzalez Avalos, G., Barrera Gallegos, N., Ayala-Jaimes, G., & Padilla Garcia, A. (2021). Steady State Response of Linear Time Invariant Systems Modeledby Multibond Graphs. Applied Sciences, 11(4), 1717. https://doi.org/10.3390/app11041717








