A Durability Prediction Method for Historical Square Rebar Reinforced Concrete Buildings
Abstract
:Featured Application
Abstract
1. Introduction
- The durability prediction method of the current China standard is structured on “target service life” which is dependent on the current durability design codes and current construction technology [18,21]. However, a precise definition of “target service life” for historical RC structures has not been established in history; hence, the durability prediction relied on the current China standard cannot be employed directly.
- Compared with the current level of the construction industry, the materials properties and construction techniques in history were backward [22]; thus, historical RC buildings cannot satisfy all of the current standards’ durability design criteria. Accordingly, numerous elements and indexes from the current standards are inaccurate and unreliable for assessing the historical RC buildings.
- The theoretical models and experiments in the standard [18] are all designed for round rebar components; however, according to the existing studies, the structural configuration design [23], the bond-slip behavior between rebar and concrete [24] and the rebar corrosion induced cracking model [25] for square rebar cases are very different from the round rebar cases.
2. The Durability Prediction Method and Research Method
2.1. The Durability Prediction Method
2.2. Research Method
2.3. Research Significance and Novelties
- Fundamentally, the proposed durability prediction method accomplishes the issue that there has been no applicable and reliable method of durability evaluation for historical square rebar RC buildings.
- The durability prediction calculation methods for a single concrete component focus on the historical concrete and square rebar components. It considers the poor properties of the historical concrete and the different models of corrosion-induced cover cracking between square rebar and round rebar.
- The proposed important analysis method is improved from the existing theory on the realistic application, which is more accurate and efficient. The inclusion of the finite element model also enhances the efficiency of the evaluation works of the building.
- GB/T 51,355 standard fosters the engineers or workers to choose a limited number of structural members to represent the status of the entire structure; however, the selection is performed randomly or inaccurately only depending on the experience. The proposed method ranks the structural members according to importance, which can reduce the potential human error and improve the reliability of the durability evaluation. Additionally, the component importance ranking is highly valuable and practical for the renovations and conservations of historic buildings that have aesthetic parts as colorful drawings and patterns. When feasible, the ranks can guide engineers to reinforce only the essential components rather than all components; hence, allowing the original paintings and patterns on the components to be preserved.
3. The Method for Determination of Critical Components
3.1. The Components Importance Analysis
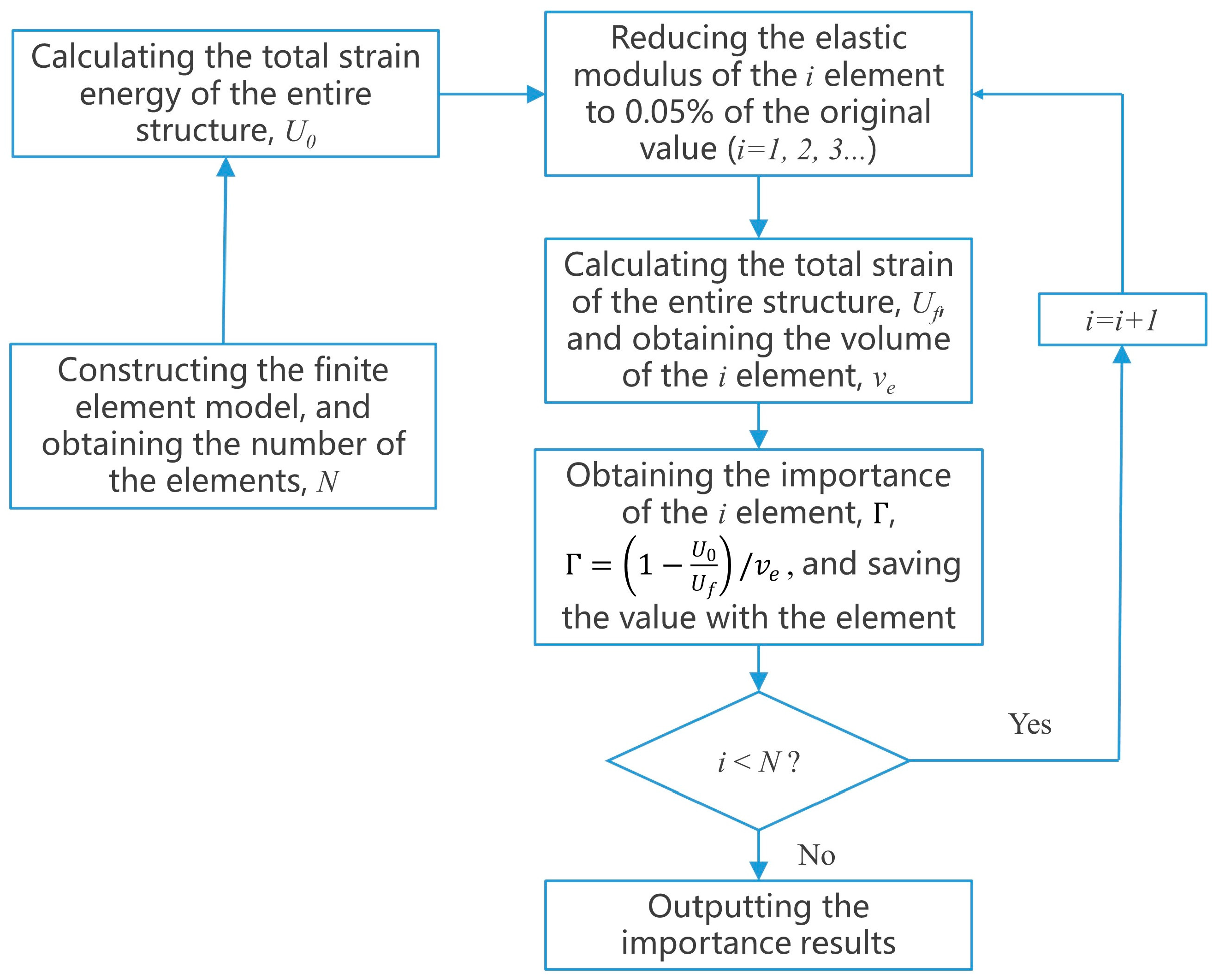
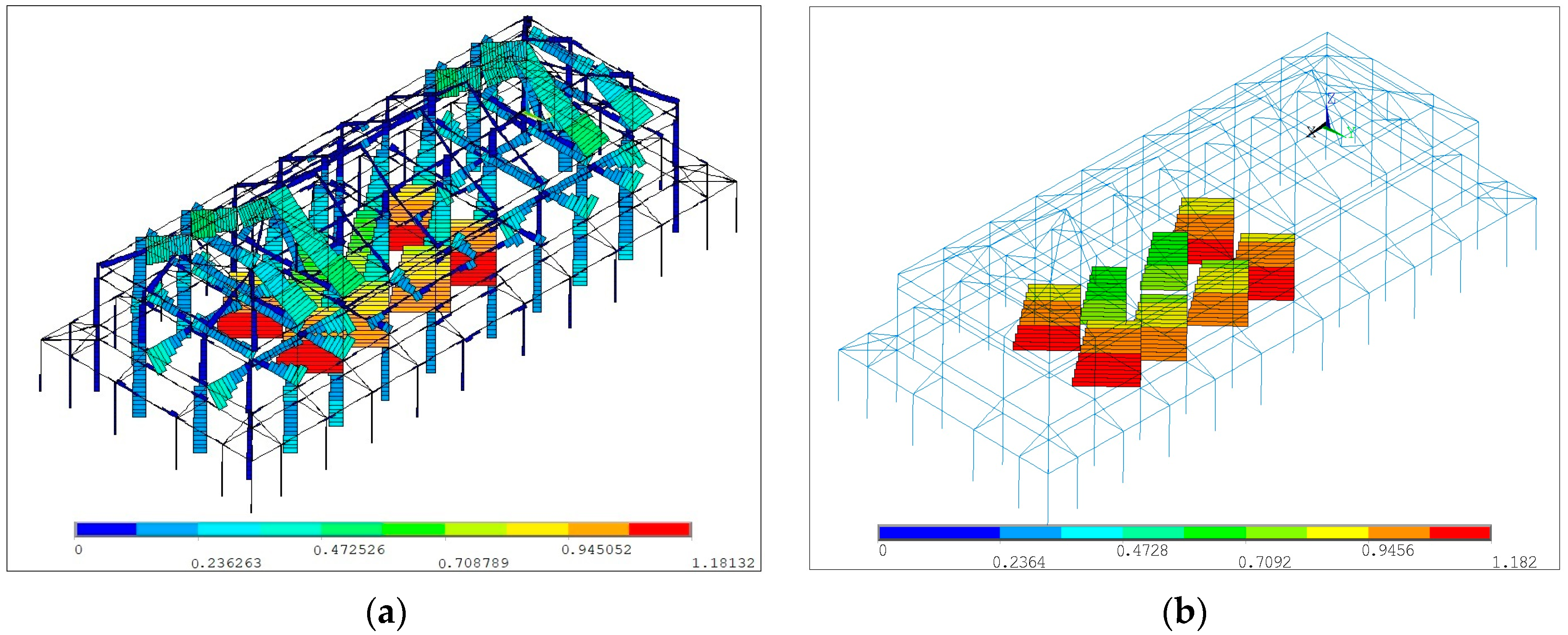
3.2. The Finite Element Method for Components Importance Analysis
3.3. The Ranks of the Components
4. The Durability Prediction Calculation Methods for Square Rebar Components
4.1. The Time before Rebar Corrosion
4.2. The Time from Corrosion Initiation to Concrete Cover Cracking
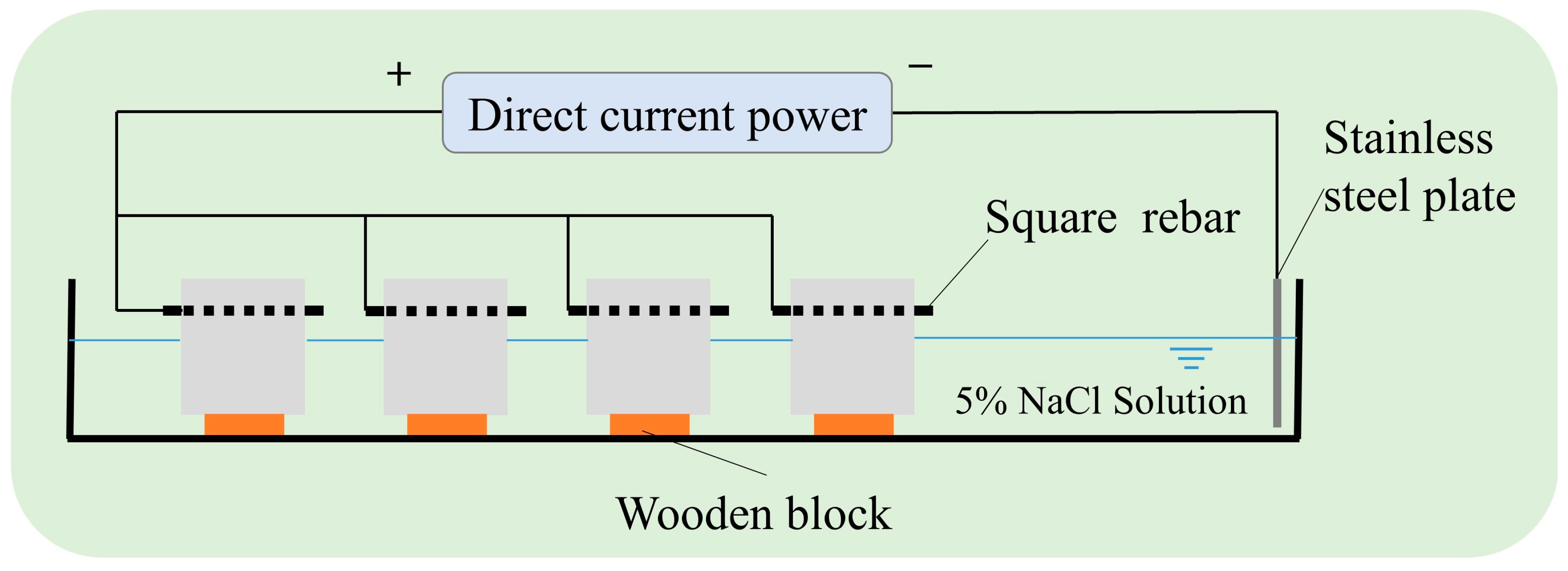
4.3. Corrosion-Induced Cover Cracking Experiments for Square Rebar Component
4.4. Experimental Results
4.5. The Calculation Method of the Critical Corrosion Depth of Square Rebar
4.6. The Durability Prediction Calculation Methods for the Square Rebar Component
5. Applications of the Proposed Method
5.1. Two Typical Historical Square Rebar Reinforced Concrete Buildings
5.2. Results of Component Importance Analysis of the Two Buildings
5.3. The Durability Prediction Results of the Two Buildings
5.4. The Reliability and Applicability of the Proposed Method
5.4.1. The Conservativeness of the Proposed Method
5.4.2. The Origin of the Proposed Calculation Methods for Single Component
5.4.3. The Realistic Evaluations of the Two Cases
The Songfengge Building
The Main Hall of the Tomb of Yu
6. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Bazant, Z.P. Physical model for steel corrosion in concrete sea structures—Theory. J. Struct. Div. ASCE 1979, 105, 1137–1153. [Google Scholar] [CrossRef]
- Rodriguez, J.; Ortega, L.M.; Casal, J.; Diez, J.M. Corrosion of reinforcement and service life of concrete structures. In Proceedings of the 7th International Conference on Durability of Building Materials and Components, London, UK, 30 May–3 June 1996; pp. 117–126. [Google Scholar]
- Alonso, C.; Andrade, C.; Rodriguez, J.; Diez, J.M. Factors controlling cracking of concrete affected by reinforcement corrosion. Mater. Struct. 1998, 31, 435–441. [Google Scholar] [CrossRef]
- Zhang, W. Corrosion Damage Prediction and Durability Evaluation of Reinforced Concrete Structures. Ph.D. Thesis, Tongji University, Shanghai, China, 1999. [Google Scholar]
- Vu, K.; Stewart, M.G.; Mullard, J. Corrosion-induced cracking: Experimental data and predictive models. ACI Struct. J. 2005, 102, 719–726. [Google Scholar]
- El Maaddawy, T.; Soudki, K. A model for prediction of time from corrosion initiation to corrosion cracking. Cem. Concr. Compos. 2007, 29, 168–175. [Google Scholar] [CrossRef]
- Lu, C.; Jin, W.; Liu, R. Reinforcement corrosion-induced cover cracking and its time prediction for reinforced concrete structures. Corros. Sci. 2011, 53, 1337–1347. [Google Scholar] [CrossRef]
- Stambaugh, N.D.; Bergman, T.L.; Srubar, W.V. Numerical service-life modeling of chloride-induced corrosion in recycled-aggregate concrete. Constr. Build. Mater. 2018, 161, 236–245. [Google Scholar] [CrossRef]
- Wang, P.; Qiao, H.; Chen, K.; Li, Y.; Feng, Q. Life prediction and long-term durability of coated steel bars in magnesium oxychloride concrete. KSCE J. Civ. Eng. 2020, 24, 2120–2131. [Google Scholar] [CrossRef]
- Liang, Y.; Wang, L. Prediction of corrosion-induced cracking of concrete cover: A critical review for thick-walled cylinder models. Ocean Eng. 2020, 213, 107688. [Google Scholar] [CrossRef]
- European Committee for Standardization. EN 1992-1-1: 2004 Eurocode 2: Design of Concrete Structures—Part 1-1: General Rules and Rules for Buildings; European Committee for Standardization: Brussels, Belgium, 2004. [Google Scholar]
- American Concrete Institute. ACI 318-08: Building Code Requirements for Structural Concrete; American Concrete Institute: Farmington Hills, MI, USA, 2008. [Google Scholar]
- Ministry of Housing and Urban-Rural Development of the People’s Republic of China. Code for Design of Concrete Structures GB 50010-2010; Ministry of Housing and Urban-Rural Development of the People’s Republic of China: Beijing, China, 2015.
- Du, Y. Engineering budget (17). Buil. Mon. Mag. 1934, 2, 48–51. [Google Scholar]
- Lee, H.E. Engineers’ Pocketbook of Reinforced Concrete; Myron, C., Ed.; Clark Publishing: London, UK, 1915. [Google Scholar]
- Alexander, N. Structural Renovation of Buildings: Methods, Details, & Design Examples; McGraw-Hill Professional: New York, NY, USA, 2000. [Google Scholar]
- Meinheit, D.F.; Felder, A.L. Vintage Steel Reinforcement in Concrete Structures; Concrete Reinforcing Steel Institute: Schaumburg, IL, USA, 2014. [Google Scholar]
- Ministry of Housing and Urban-Rural Development of the People’s Republic of China. Standard for Durability Assessment of Existing Concrete Structures; Ministry of Housing and Urban-Rural Development of the People’s Republic of China: Beijing, China, 2019.
- ACI Committee 408. ACI 408R-03: Bond and Development of Straight Reinforcing Bars in Tension; American Concrete Institute: Farmington Hills, MI, USA, 2003. [Google Scholar]
- Ministry of Housing and Urban-Rural Development of the People’s Republic of China. Concrete Structure Design Standard GB/J 10-89; China Architecture Publishing & Media Co., Ltd.: Beijing, China, 1989. [Google Scholar]
- Ministry of Housing and Urban-Rural Development of the People’s Republic of China. Standard for Design of Concrete Structures Durability GB/T 50476; China Architecture Publishing & Media Co., Ltd.: Beijing, China, 2019. [Google Scholar]
- Chun, Q.; Pan, J. Research on structural configuration design of chinese modern reinforced concrete buildings. China Cul. Herit. Sci. Res. 2015, 2, 85–90. [Google Scholar] [CrossRef]
- Chun, Q.; Van Balen, K.; Han, Y. Comparison study on calculation methods of bending behavior of Chinese reinforced concrete beams from 1912 to 1949. J. Southeast Univ. 2015, 31, 529–534. [Google Scholar] [CrossRef]
- Zhang, C.; Chun, Q.; Jin, H.; Zhang, S.; Lin, Y. Experimental and numerical research on the bond-slip behavior of square-rebar reinforced concrete during the republic of China (1912–1949). Struct. Concr. 2020, 23, 871–893. [Google Scholar] [CrossRef]
- Dong, Y.; Chun, Q.; Xu, X.; Wang, J.; Huang, S. Critical corrosion depth of rebar of Republican Period reinforced concrete structures. J. Zhejiang Univ. 2017, 51, 27–37. [Google Scholar] [CrossRef]
- Chun, Q.; Van Balen, K.; Pan, J. Experimental research on physical and mechanical performance of steel rebars in Chinese modern reinforced concrete buildings built during the Republic of China era from 1912 to 1949. Mater. Struct. 2015, 49, 3679–3692. [Google Scholar] [CrossRef]
- Chun, Q.; Balen, K.V.; Han, Y. Comparative studies on calculation methods of shear behavior of reinforced concrete beams of Chinese modern reinforced concrete buildings. J. Harbin Inst. Technol. 2016, 23, 1–8. [Google Scholar] [CrossRef]
- Zhang, C.; Chun, Q.; Lin, Y.; Jin, H.; Wang, Y. Experimental and theoretical analysis for the shear capacity of the square rebar reinforced concrete beams during the period of the Republic of China (1912–1949). Eur. J. Environ. Civ. Eng. 2021, 1–40. [Google Scholar] [CrossRef]
- Ye, L.; Lin, X.; Qu, Z.; Lu, X.; Peng, P. Evaluating method of element importance of structural system based on general ized structural stiffness. J. Archit. Civ. Eng. 2010, 27, 1–6. [Google Scholar] [CrossRef]
- Zhu, L.; Wang, N.; Jun, D. Evaluation on element importance based on objective weighting method. J. Archit. Civ. Eng. 2015, 32, 46–52. [Google Scholar] [CrossRef]
- Zhao, F. Reinforced Concrete Structure; China Engineers Association: Shanghai, China, 1935. [Google Scholar]
- Chen, Z. Simplified Reinforced Concrete Structure; World Bookstore: Shanghai, China, 1936. [Google Scholar]
- Zhang, J. Simplified Reinforced Concrete Design Method; World Bookstore: Shanghai, China, 1944. [Google Scholar]
- Yu, M.; Li, S.; Zhang, S.; Qiang, N. Model for calcualting the steel corrosion depth based on crack width. Concrete 2014, 301, 13. [Google Scholar] [CrossRef]

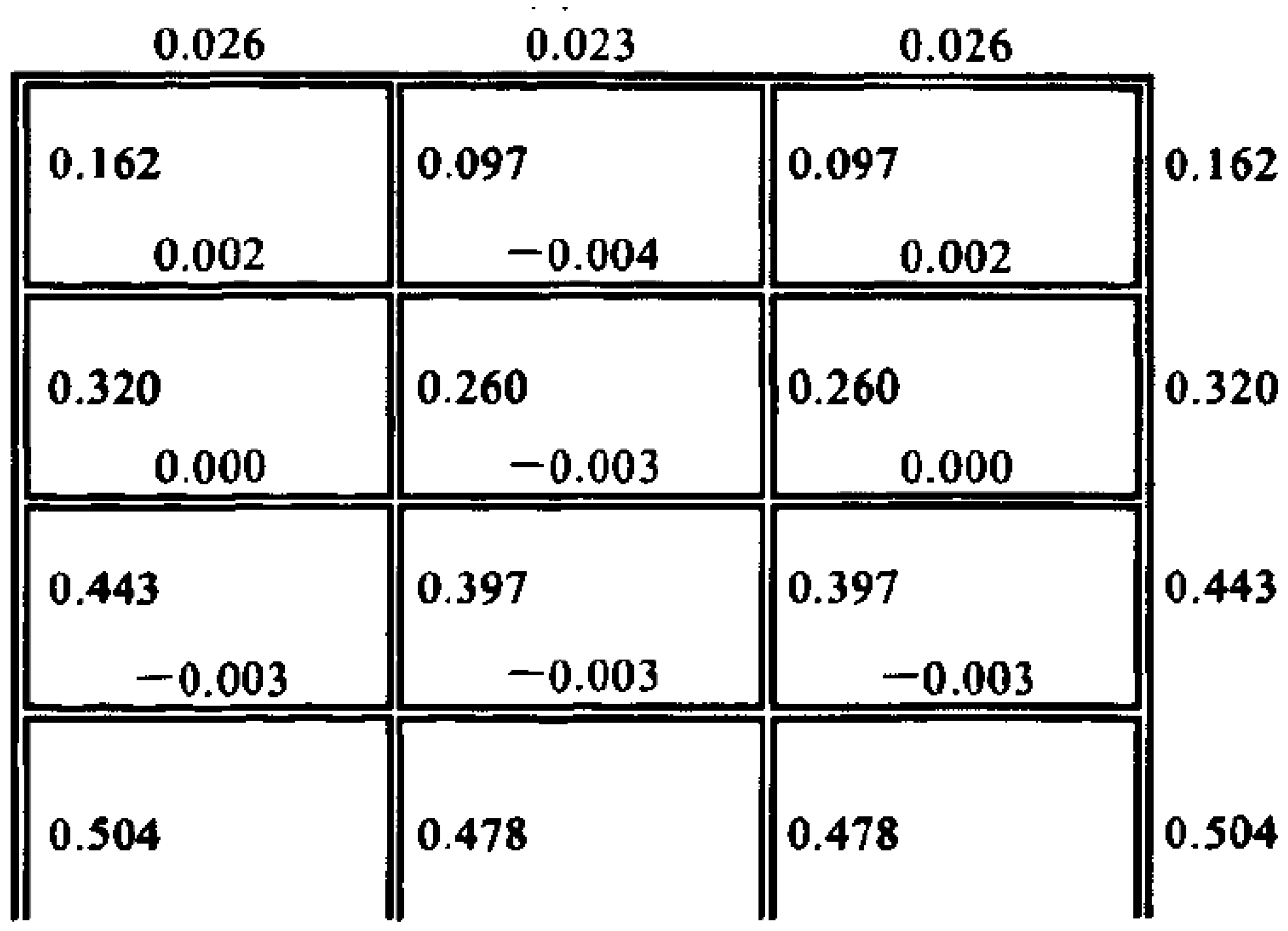
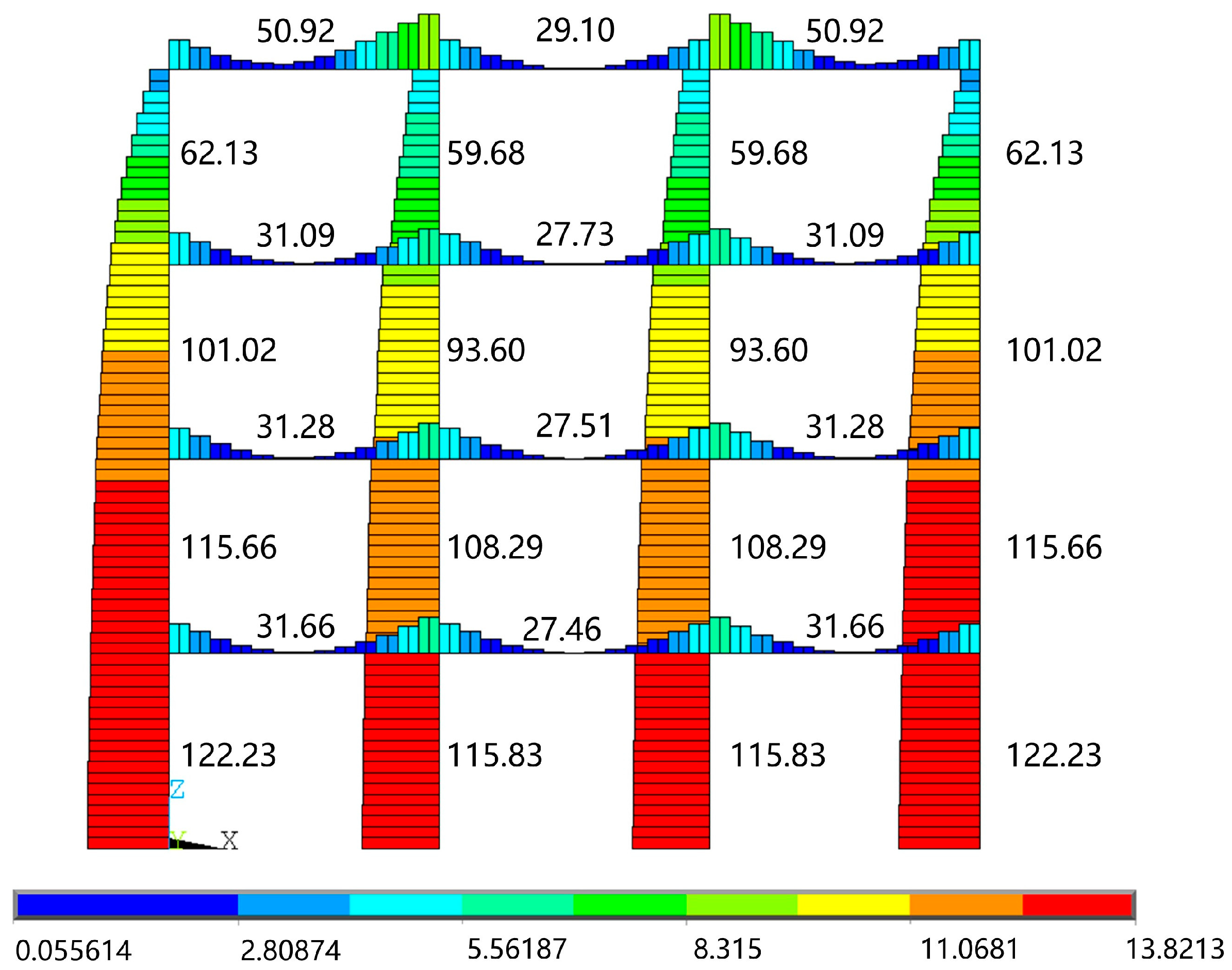





| Element Ranking Percentage | ||||
|---|---|---|---|---|
| Component ranks | Critical Components | Important Components | Ordinary Components | Secondary Components |
| 1 Cases | Items | |||||
|---|---|---|---|---|---|---|
| 2 Concrete Average Compressive Strength/MPa | 2 Average Thickness of Concrete Cover/mm | 2 Concrete Average Measured Carbonation Depth/mm | ||||
| Column | Beam | Column | Beam | Column | Beam | |
| Main Hall of the Tomb of Yu (1933) | 15.7 | 15.4 | 40 | 41 | 33 | 40 |
| No.1 Building of Zhongshan East Road (1935) | 12.4 | 11.7 | 34 | 27 | 62 | 65 |
| Dahua Cinema (1931) | 15.6 | 17.8 | 33 | 34 | 63 | 40 |
| Dacheng Factory (1935) | 17.0 | 22.9 | 35 | 34 | 45 | 40 |
| Main Hall of Nanjing Museum (1937) | 18.0 | 23.2 | 34 | 35 | 43 | 49 |
| Huangpu Hall of Jiangsu Conference Center (1931) | 14.7 | 17.6 | 35 | 33 | 45 | 54 |
| Former site of overseas Chinese Hostel (1933) | 16.5 | 14.8 | 30 | 28 | 58 | 62 |
| Lingyuan Post Office (1947) | 14.0 | 17.4 | 40 | 38 | 55 | 45 |
| Former site of National Art Museum (1925) | 14.4 | 17.1 | 35 | 33 | 60 | 71 |
| Former site of the Ordnance Special School of the National Revolutionary Army (1932) | - | 17.2 | - | 25 | - | 55 |
| Former site of Young Men’s Christian Association (1946) | 10.4 | - | 38 | - | 47 | - |
| Former site of Central Radio Equipment Co., Ltd. (1946) | - | 12.2 | - | 32 | - | 40 |
| Specimen | 1 /mm | c /mm | Crack Width /mm | Critical Corrosion Depth/mm | |||||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
| Test Value | 2 Equation (10) | 2 Equation (11) | 2 Equation (12) | 2 Equation (13) | Equation (14) | ||||||||||
| Value | 3 Error/% | Value | 3 Error/% | Value | 3 Error/% | Value | 3 Error/% | Value | 3 Error/% | ||||||
| A1 | 16 | 36 | 14.7 | 0.1 | 0.0642 | 0.0573 | −10.6 | 0.0260 | −59.5 | 0.0285 | −55.6 | 0.0159 | −75.2 | 0.08552 | 33.26 |
| A2 | 16 | 38 | 14.9 | 0.1 | 0.0900 | 0.0590 | −34.4 | 0.0264 | −70.6 | 0.0297 | −67.0 | 0.0170 | −81.1 | 0.09084 | 0.98 |
| A3 | 16 | 40 | 15.6 | 0.1 | 0.1093 | 0.0611 | −44.1 | 0.0256 | −76.6 | 0.0308 | −71.8 | 0.0182 | −83.3 | 0.09677 | −11.47 |
| A4 | 16 | 42 | 15.8 | 0.1 | 0.0951 | 0.0628 | −34.0 | 0.0261 | −72.6 | 0.0320 | −66.3 | 0.0193 | −79.7 | 0.10210 | 7.42 |
| A5 | 16 | 44 | 15.2 | 0.1 | 0.1134 | 0.0638 | −43.8 | 0.0285 | −74.9 | 0.0332 | −70.8 | 0.0201 | −82.3 | 0.10647 | −6.12 |
| B1 | 22 | 36 | 14.3 | 0.1 | 0.0639 | 0.0496 | −22.3 | 0.0225 | −64.8 | 0.0228 | −64.3 | 0.0109 | −83.0 | 0.06006 | −5.96 |
| B2 | 22 | 38 | 14.8 | 0.1 | 0.0652 | 0.0512 | −21.6 | 0.0219 | −66.5 | 0.0236 | −63.8 | 0.0118 | −82.0 | 0.06436 | −1.33 |
| B3 | 22 | 40 | 15.6 | 0.1 | 0.0673 | 0.0529 | −21.4 | 0.0206 | −69.4 | 0.0245 | −63.6 | 0.0128 | −81.0 | 0.06902 | 2.56 |
| B4 | 22 | 42 | 16.2 | 0.1 | 0.0715 | 0.0545 | −23.8 | 0.0198 | −72.3 | 0.0253 | −64.6 | 0.0137 | −80.8 | 0.07344 | 2.70 |
| B5 | 22 | 44 | 14.7 | 0.1 | 0.0860 | 0.0543 | −36.8 | 0.0241 | −71.9 | 0.0262 | −69.6 | 0.0139 | −83.8 | 0.07534 | −12.41 |
| Names | Durability Assessment Time/Years | c /mm | fcu,e /MPa | /mm | Durability Prediction by GB/T 51,355/Years | Durability Prediction by the Proposed Method/Years | ||||
|---|---|---|---|---|---|---|---|---|---|---|
| * Part 1 | * Part 2 | Total | * Part 1 | * Part 2 | Total | |||||
| Songfengge Building | 86 | 34 | 22.5 | 24 | 47 | 53 | 100 | 43 | 60 | 103 |
| Main Hall of the Tomb of Yu | 78 | 40 | 15.7 | 25 | 49 | 25 | 74 | 46 | 30 | 76 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Jin, H.; Chun, Q.; Zhang, C.; Han, Y. A Durability Prediction Method for Historical Square Rebar Reinforced Concrete Buildings. Appl. Sci. 2021, 11, 11737. https://doi.org/10.3390/app112411737
Jin H, Chun Q, Zhang C, Han Y. A Durability Prediction Method for Historical Square Rebar Reinforced Concrete Buildings. Applied Sciences. 2021; 11(24):11737. https://doi.org/10.3390/app112411737
Chicago/Turabian StyleJin, Hui, Qing Chun, Chengwen Zhang, and Yidan Han. 2021. "A Durability Prediction Method for Historical Square Rebar Reinforced Concrete Buildings" Applied Sciences 11, no. 24: 11737. https://doi.org/10.3390/app112411737
APA StyleJin, H., Chun, Q., Zhang, C., & Han, Y. (2021). A Durability Prediction Method for Historical Square Rebar Reinforced Concrete Buildings. Applied Sciences, 11(24), 11737. https://doi.org/10.3390/app112411737






