Verification Methodology for Simulation Models of the Synchronous Generator on Transients Analysis
Abstract
:1. Introduction
2. Modeling Methods for Transients Calculation
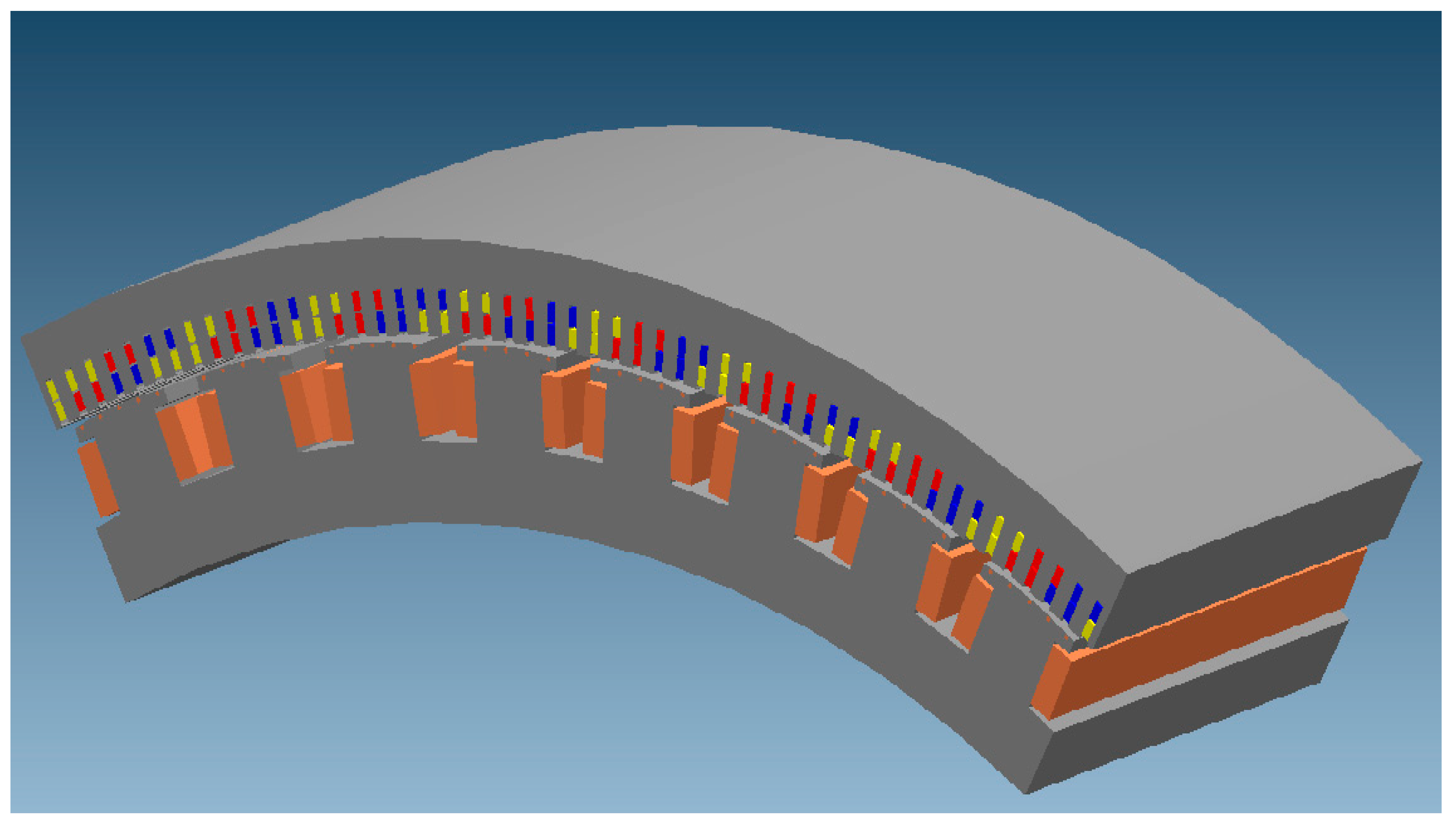
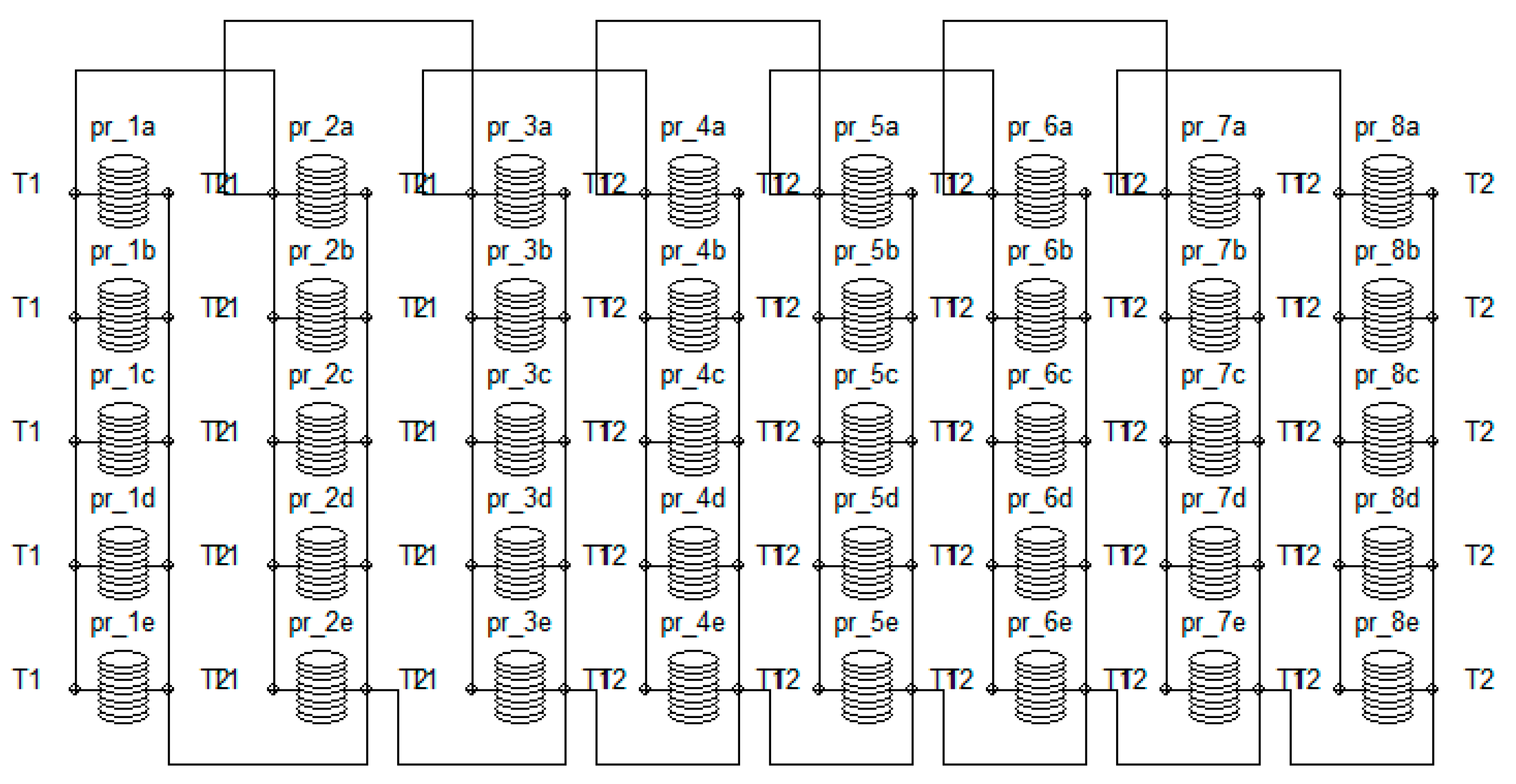
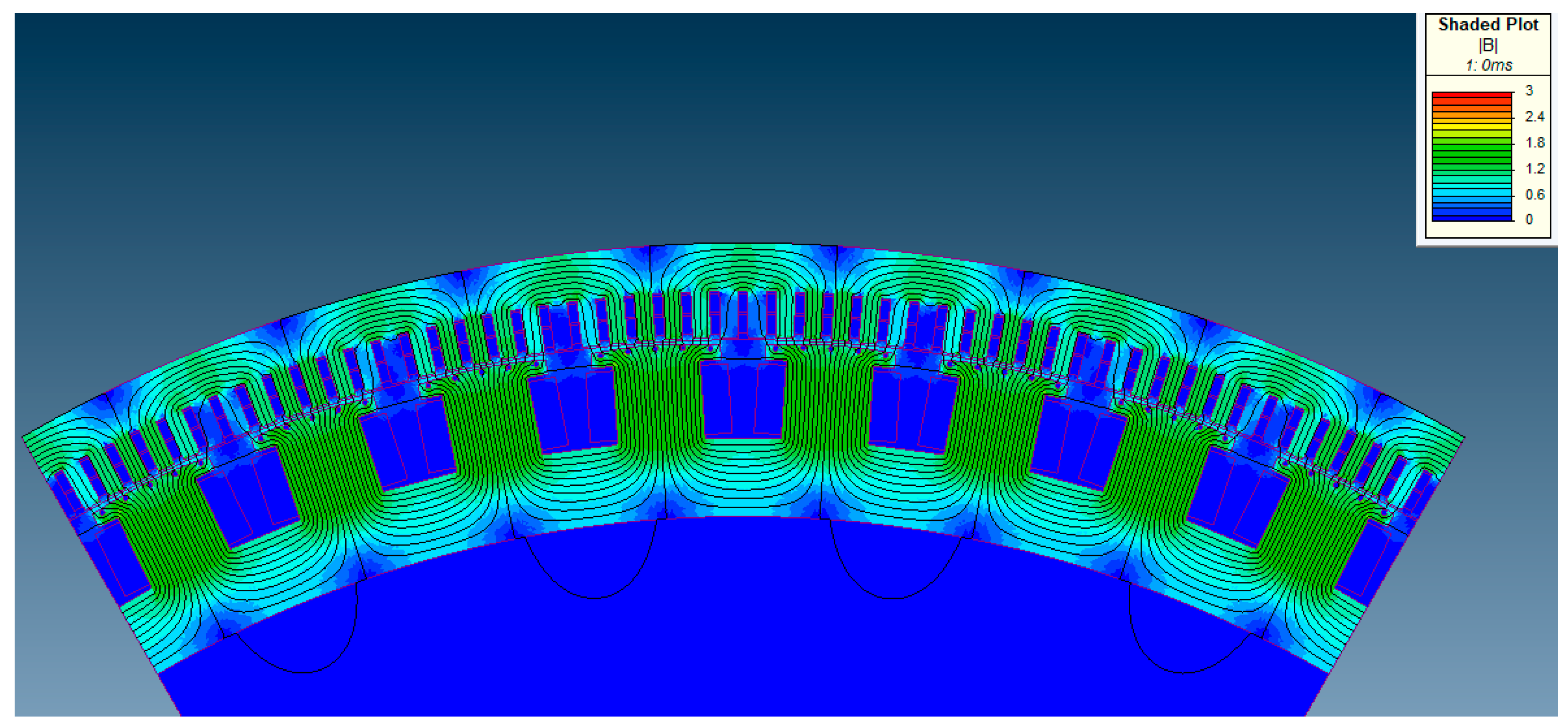
2.1. Modeling of a Sudden Three-Phase Short-Circuit Using the FEM
- all of the coils in windings have equal numbers of conductors and therefore equal resistance; windings are placed symmetrically on poles, enabling the analysis of one repeating segment of the generator;
- the ratio of the core length and the end winding length of the generator is 3.2, and allows the 2D model to be applicable;
- the influence of the skin effect, which can appear in excitation or in armature winding conductors, is neglected;
- the stray current and the eddy current are excluded because the electric conductivity of iron can also be neglected;
- embedded materials are isotropic, the hysteresis loop is neglected, and the iron permeability depends on the magnetic field strength;
- the rotor speed variations throughout the transients, due to high inertia of mass, are also neglected; and
- the sources used to feed the windings are assumed to be perfect.
- is the electric field strength in the conductors,
- is the lux density,
- is the magnetic field strength,
- is thecurrent density,
- C is the curve bounding the surface S,
- S is the plane in which the field is calculated, and
- is the unit vector normal to surface S.
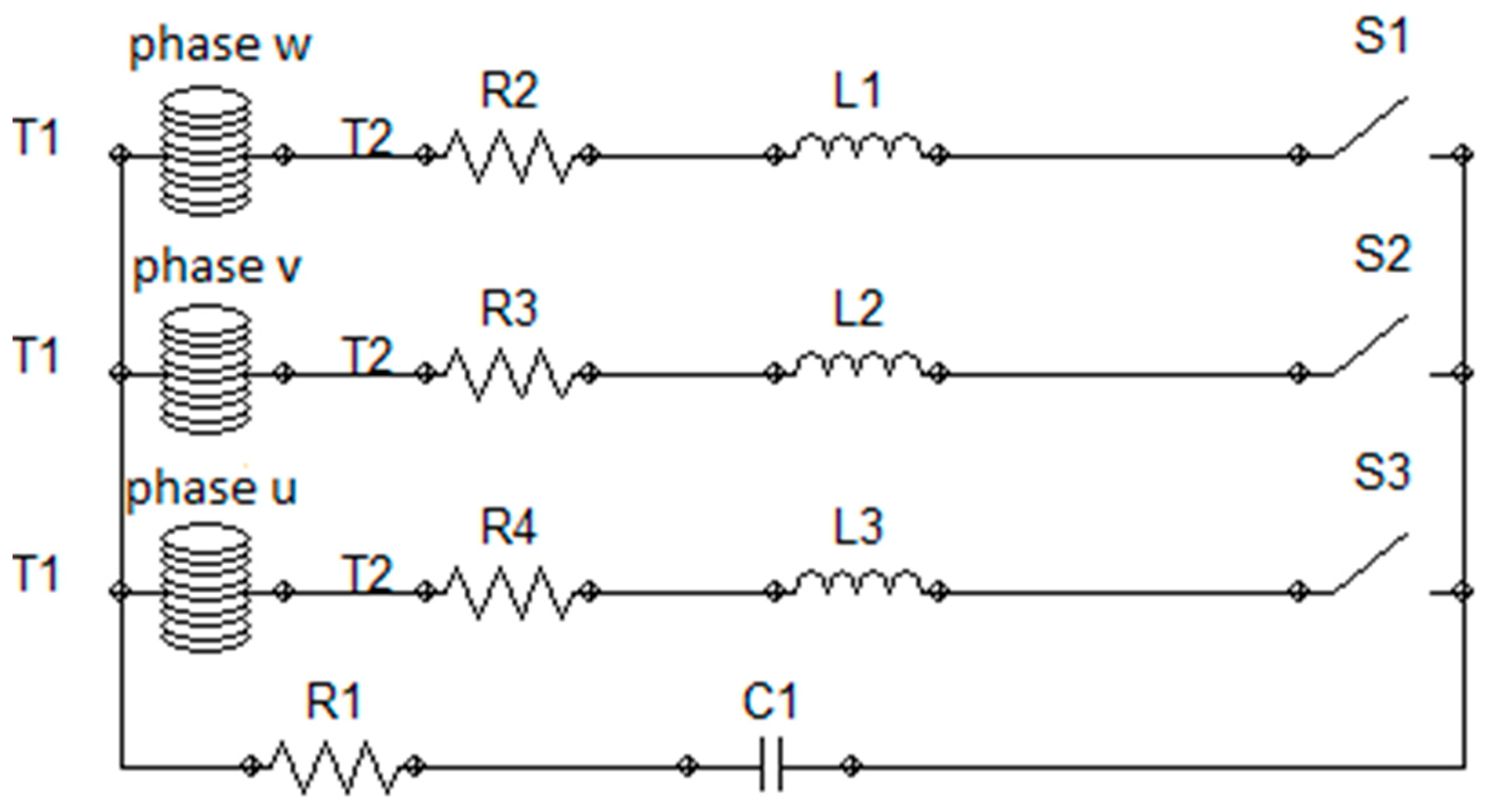
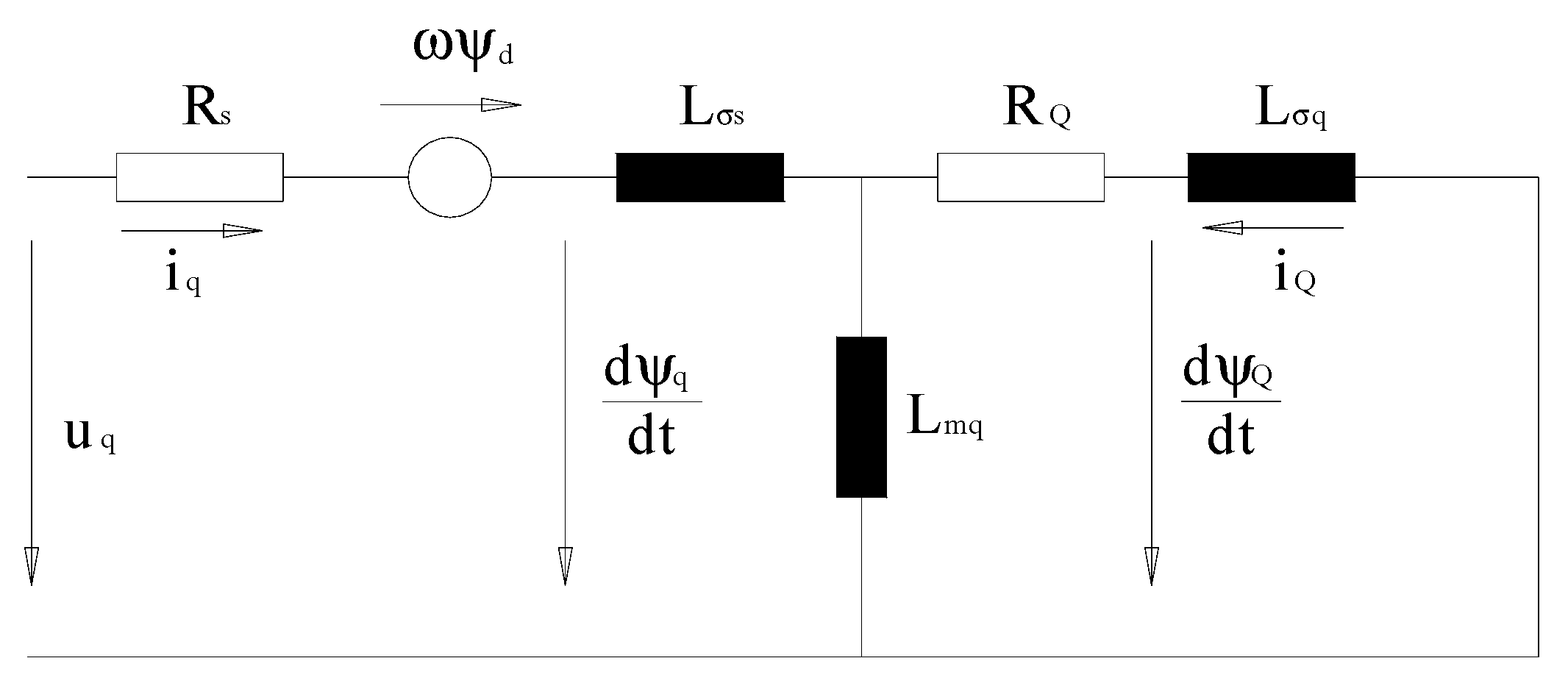
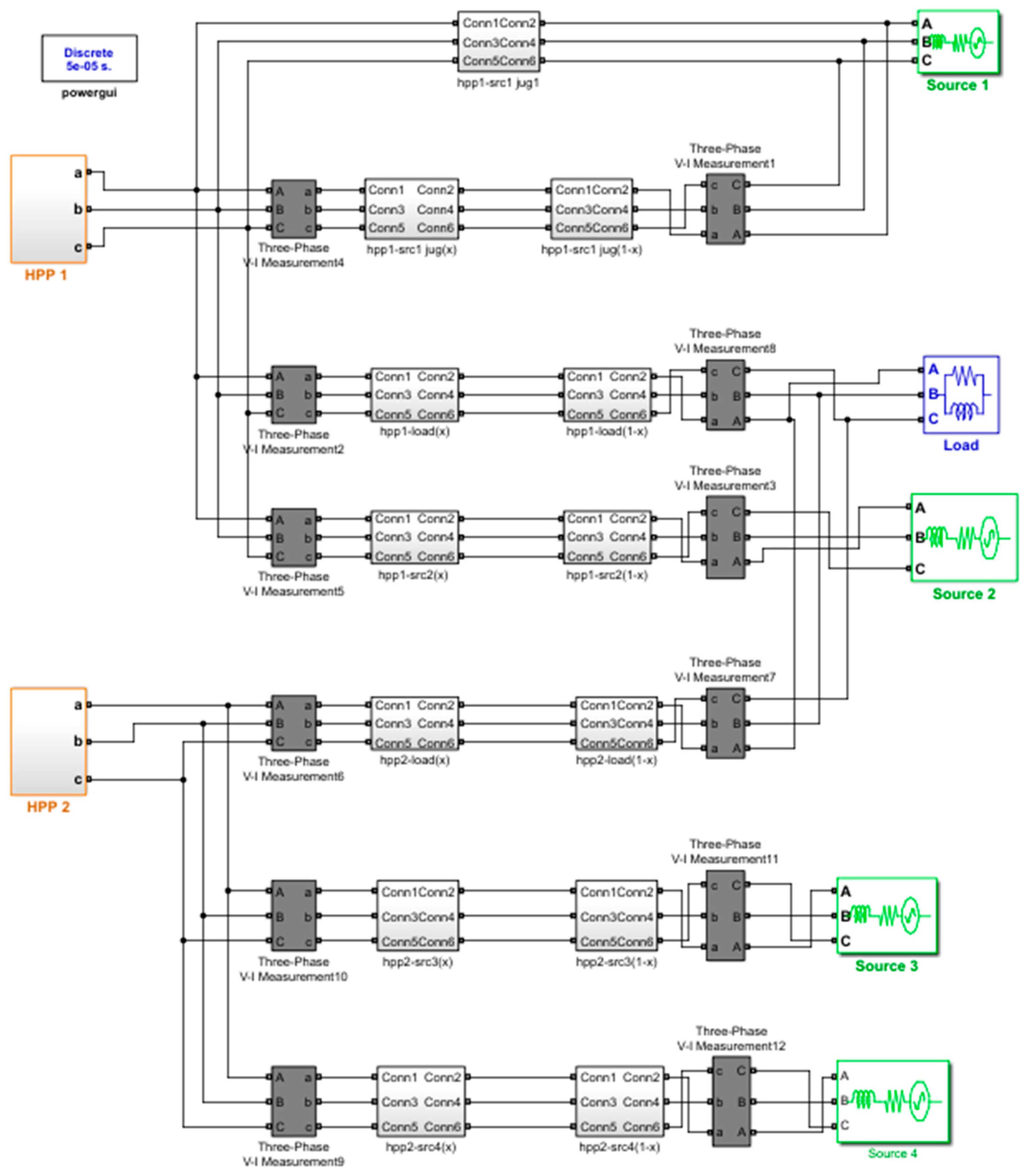
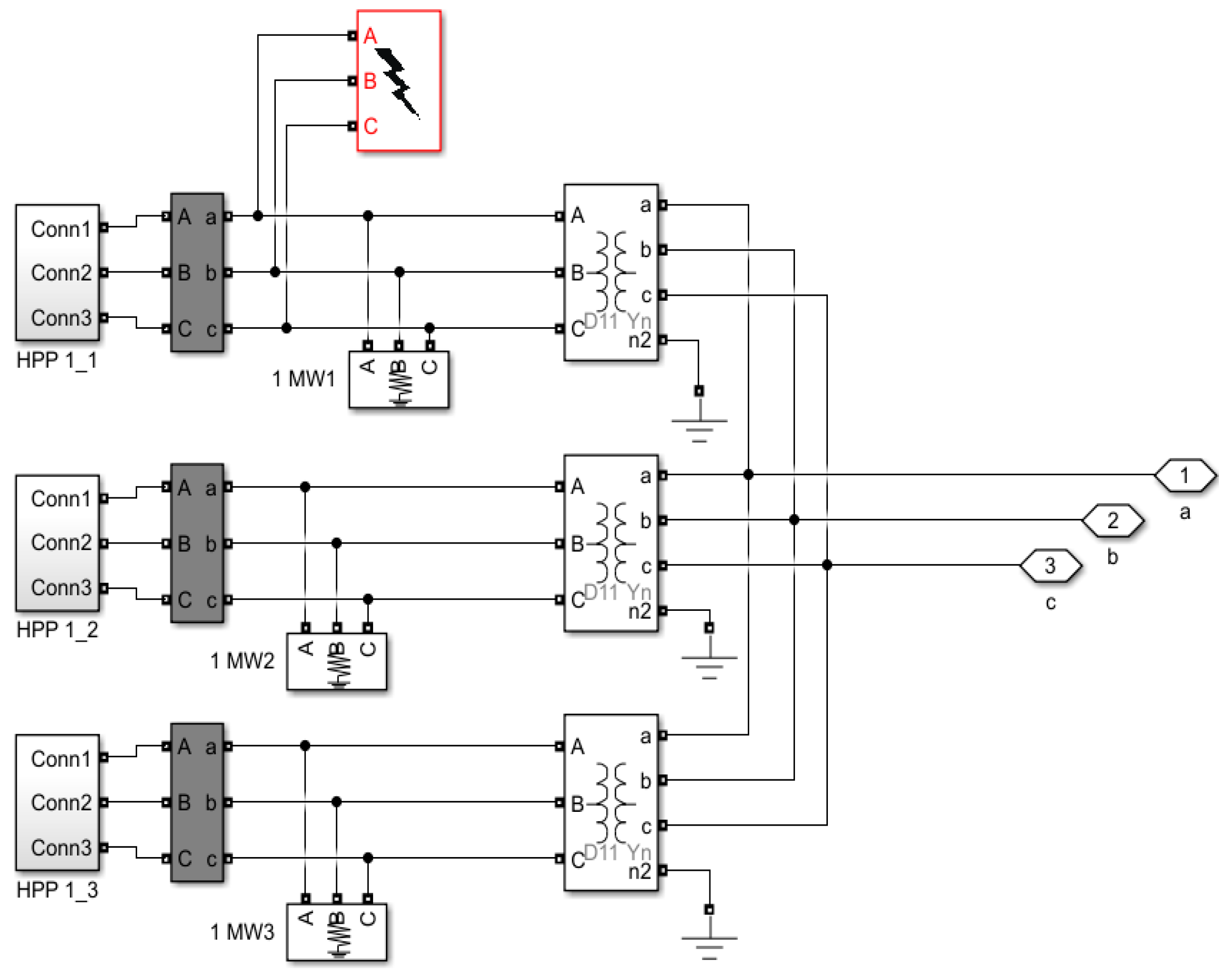
2.2. Sudden Short-Circuit by a Dynamic Model
- [U] is the matrix of voltage,
- [i] is the matrix of current,
- [R] is thematrix of resistance,
- [L] is the matrix of inductance,
- ωel is the electric angle velocity of rotor, and
- γ is the electric angle between the axis of stator phase “a” and the d-axis of rotor.
- Ψd is the magnetic flux in armature winding in d-axis,
- Ψf is the magnetic flux in excitation winding,
- ΨD is the magnetic flux in damping winding in d-axis,
- Ψq is the magnetic flux in armature winding in q-axis,
- ΨQ is the magnetic flux in damping winding in q-axis,
- ud is the transformed armature winding in d-axis,
- uf is the excitation voltage,
- uq is thetransformed armature winding in q-axis,
- kd is the linkage factor of armature winding in d-axis,
- kf is the linkage factor of excitation winding,
- kD is the linkage factor of damping winding in d-axis,
- kq is the linkage factor of armature winding in q-axis,
- kQ is the linkage factor of damping winding in q-axis,
- is the transient inductance of damping and excitation windings,
- is the transient inductance of armature and damping windings,
- is the transient inductance of excitation and damping windings,
- is the transient inductance of armature and excitation windings,
- is the transient inductance of excitation and armature windings,
- is the transient inductance of damping and armature windings,
- is the subtransient inductance of armature winding in d-axis,
- is the subtransient inductance of excitation winding,
- is the subtransient inductance of damping winding in d-axis,
- is the subtransient inductance of armature winding in q-axis,
- is the subtransient inductance of damping winding in q-axis,
- is the subtransient time constant of the armature winding at short-circuit in d-axis,
- is the subtransient time constant of the armature winding at short-circuit in q-axis,
- is the subtransient time constant of the damping winding at short-circuit in d-axis,
- is the subtransient time constant of the damping winding at short-circuit in q-axis,
- is the subtransient time constant of the excitation winding at short-circuit, and
- H is the inertia constant.
3. Transient Analysis Using a Dynamic Simulation Model
- d-axis synchronous reactance Xd = 1.06 p. u.,
- d-axis transient reactance Xd′ = 0.28 p. u.,
- d-axis subtransient reactance Xd″ = 0.192 p. u.,
- q-axis synchronous reactance Xq = 0.61 p. u.,
- q-axis subtransient reactance Xq″ = 0.176 p. u.,
- leakage reactance Xl = 0.14 p. u.,
- d-axis transient short-circuit time constant Td′ = 1.56 s,
- d-axis subtransient short-circuit time constant Td″ = 0.05 s,
- q-axis subtransient open-circuit Tqo″ = 0.09 s,
- inertia constant H = 3.2 s,
- friction factor F = 0, and
- stator resistance Rs = 0.0161 p. u.
4. Results and Discussion
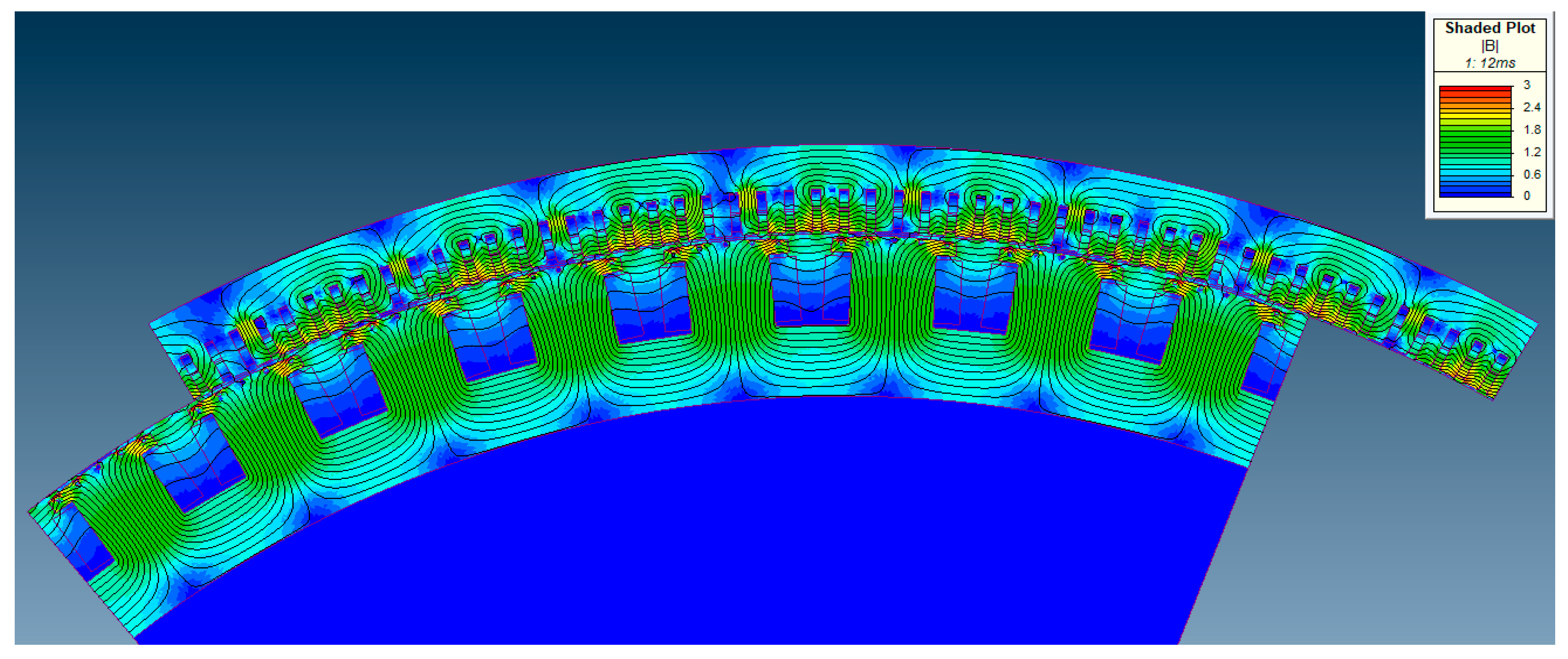
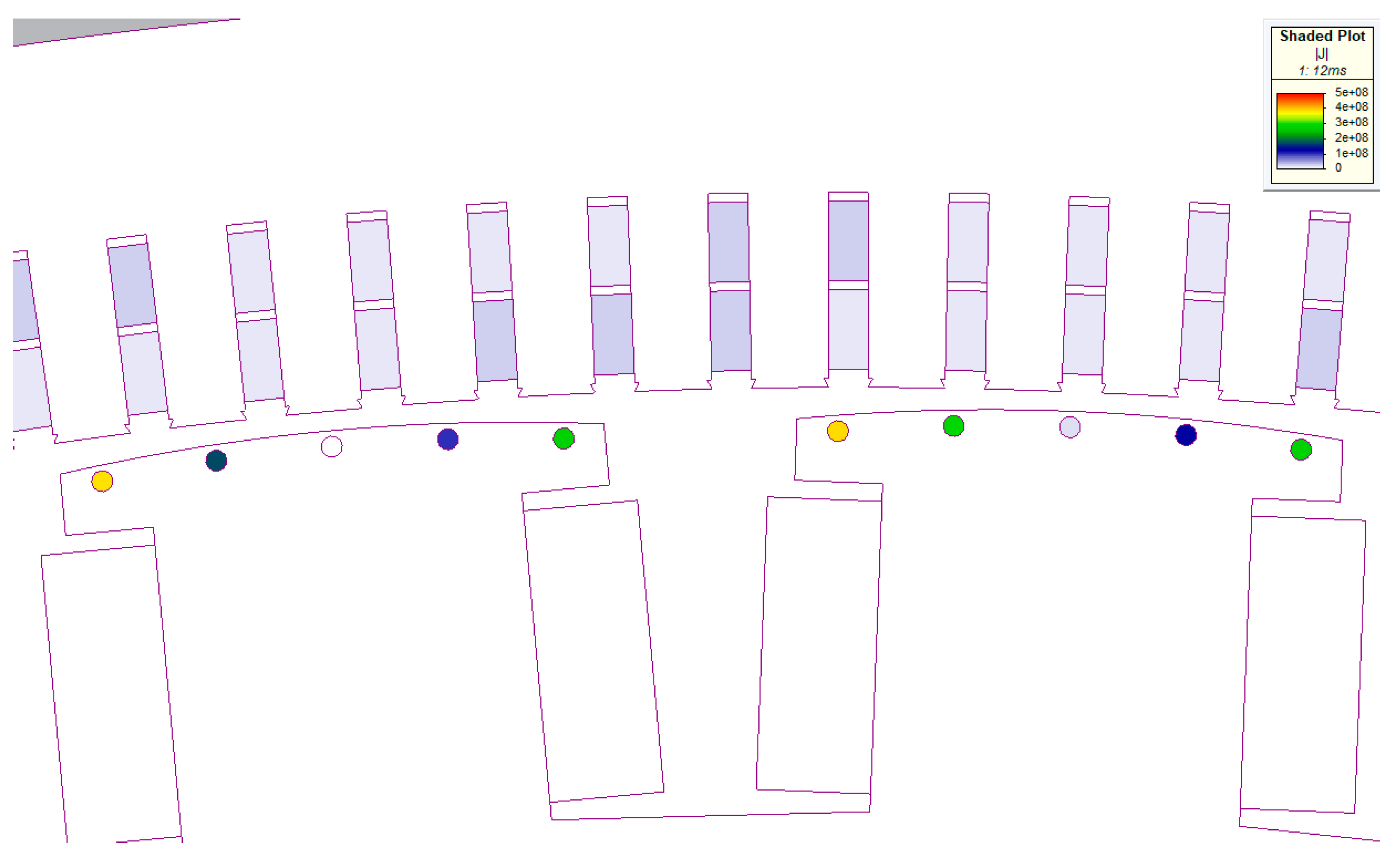
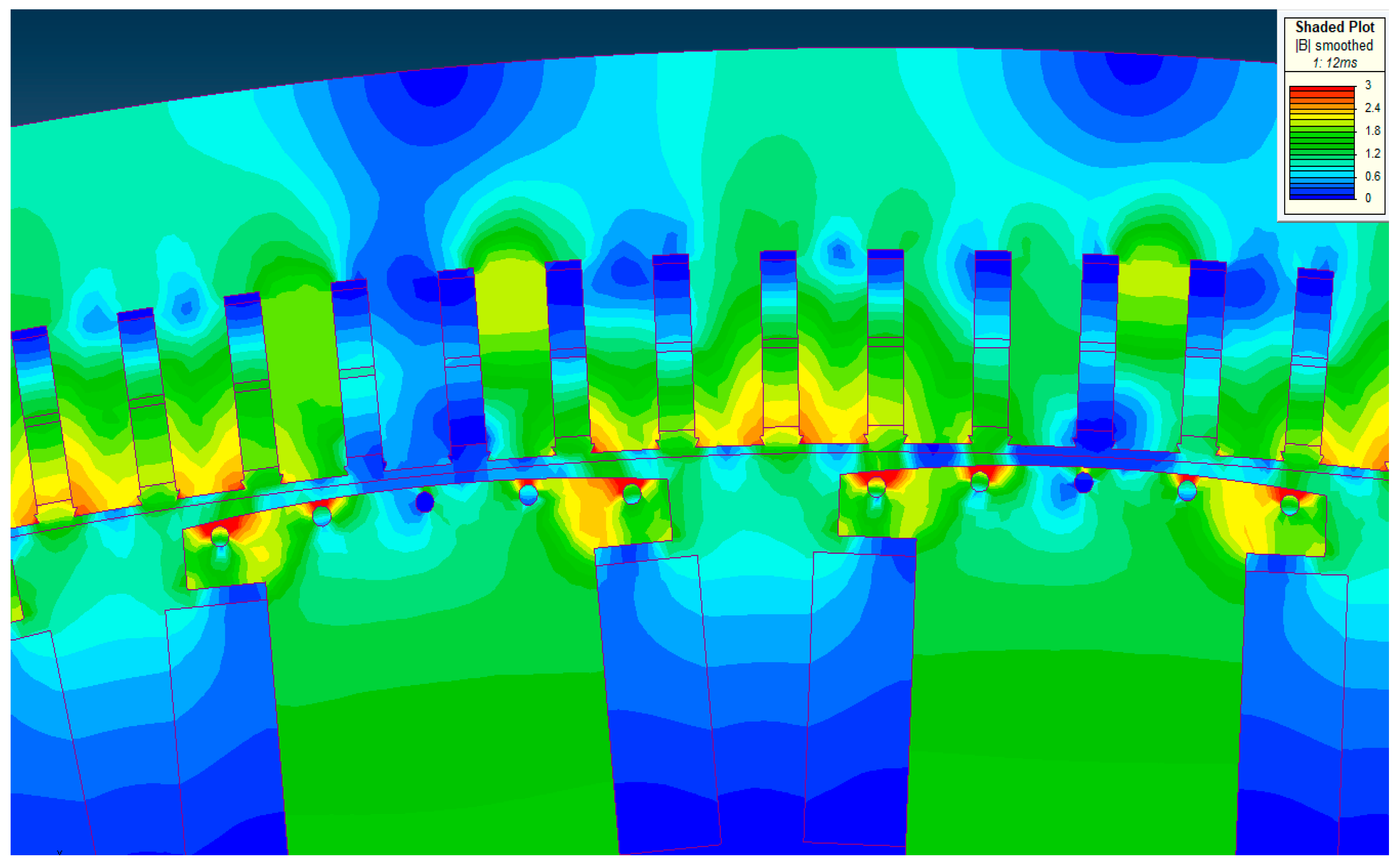
4.1. The Results of the FEM Calculation Method
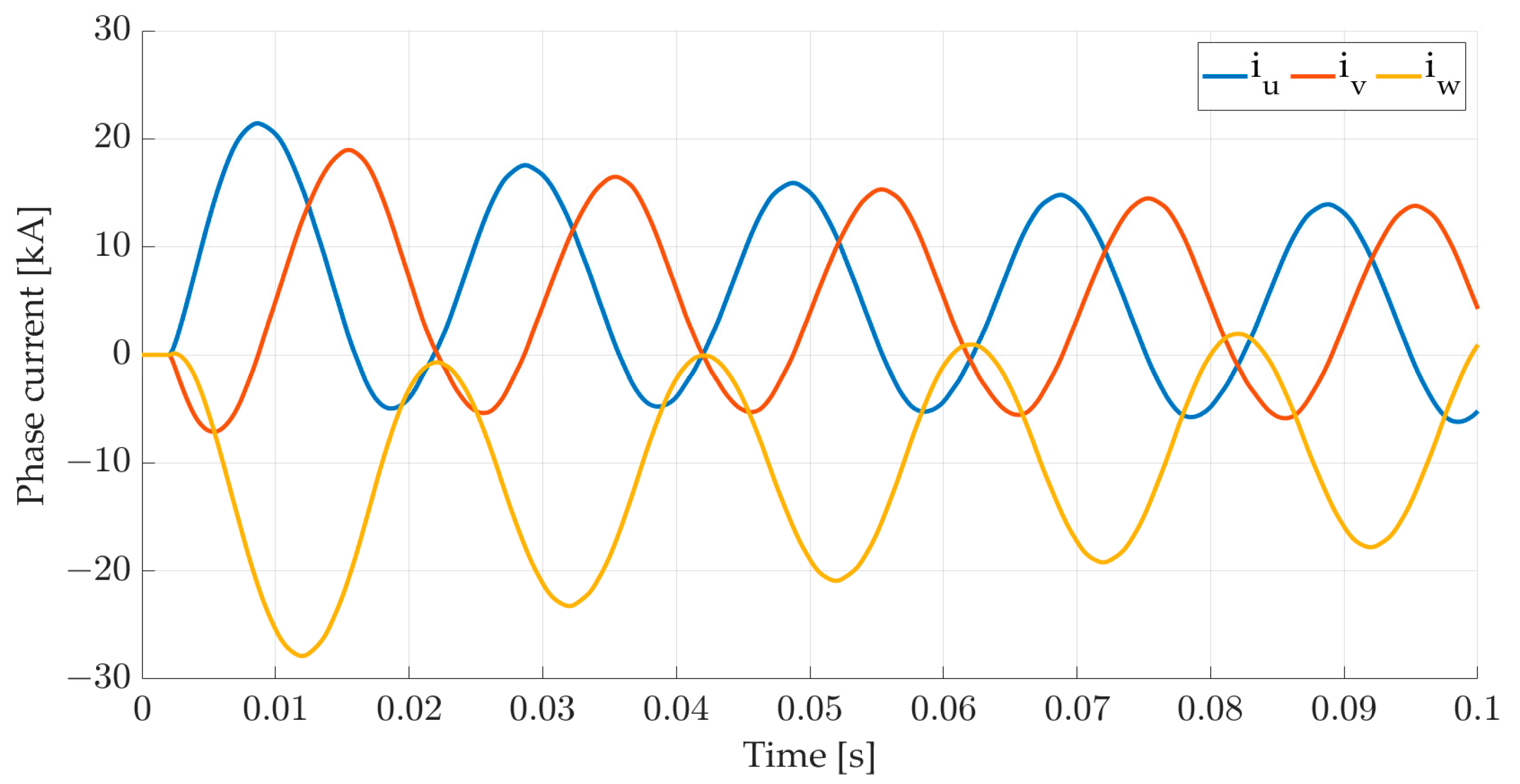
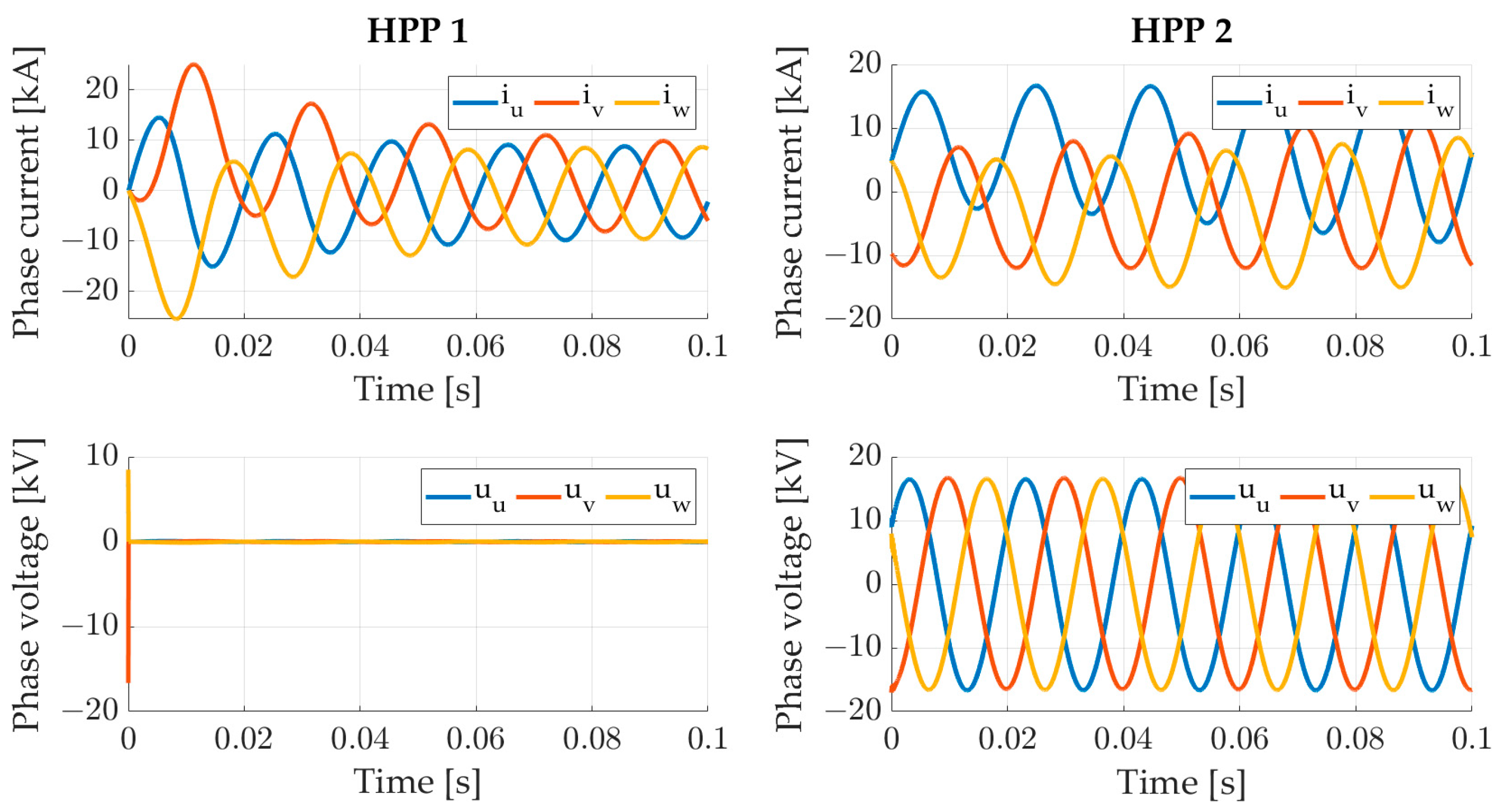
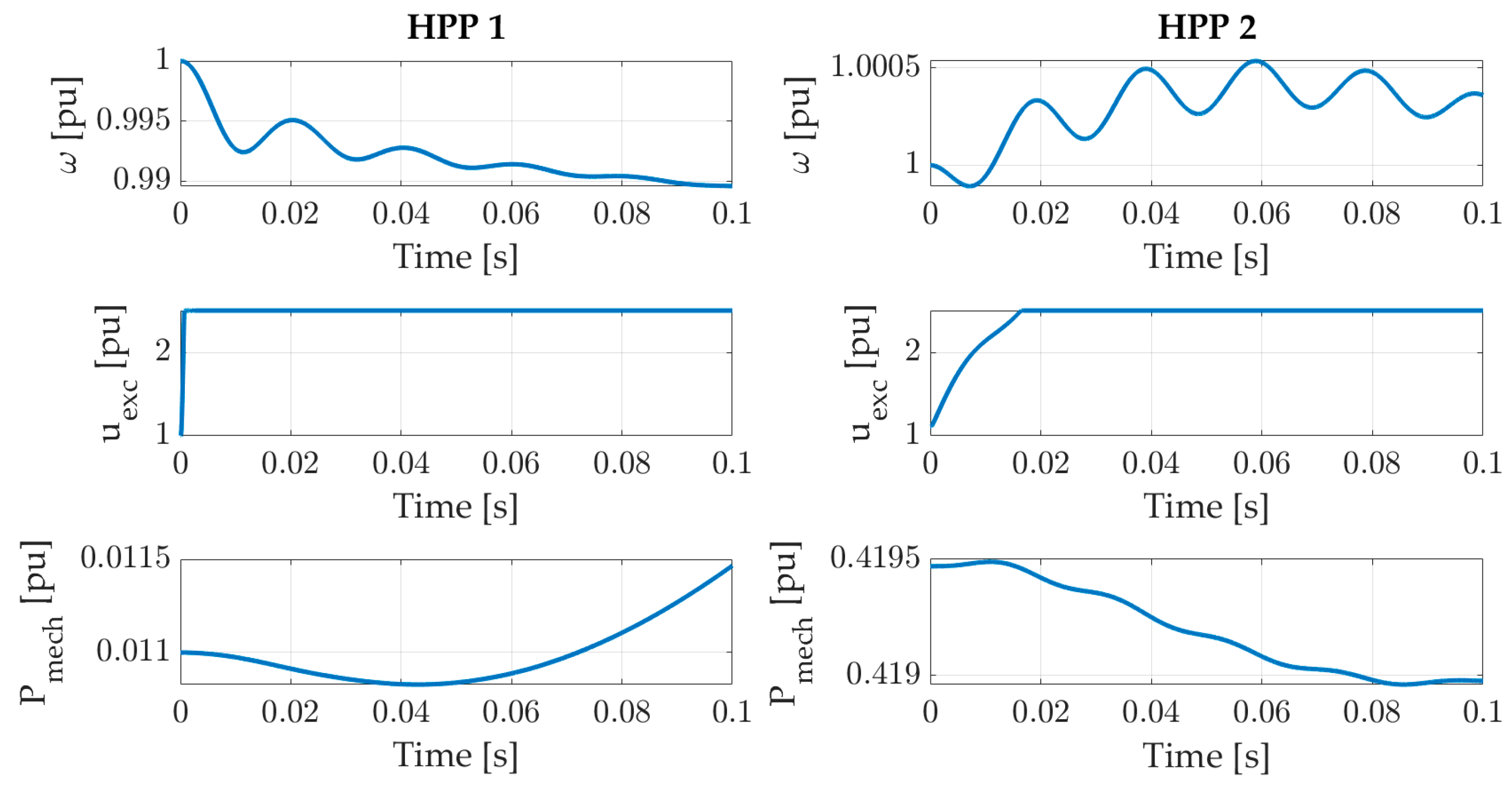
4.2. The Results of Three-Phase Fault on the Generator in the Dynamic Simulation Model
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Tomicic, B.; Stih, Z.; Car, S. The Modelling of Transients in Synchronous Generators for Wind Turbine. In Proceedings of the 2007 International Conference on Clean Electrical Power, Capri, Italy, 21–23 May 2007; pp. 591–597. [Google Scholar] [CrossRef]
- Krause, P.C. Analysis of Electric Machinery; McGraw Hill, Inc.: New York, NY, USA, 1986. [Google Scholar]
- Magnet, Version 6.5; “Tutorials”; Infolytica Corporation: Montreal, QC, Canada, 2000.
- Wamkeue, R.; Kamwa, I.; Chacha, M. Line-to-line short-circuit-based finite-element performance and parameter predictions of large hydrogenerators. IEEE Trans. Energy Convers. 2003, 18, 370–378. [Google Scholar] [CrossRef]
- Hernandez, C.; Cisneros-Gonzalez, M.; Arjona, M.A. A Gaussian Modulated Sinusoidal Pulse for Circuit-Parameter Estimation of a Synchronous Generator Using a 2D-FE Field Model. Appl. Comput. Electromagn. Soc. J. 2012, 27, 734–741. [Google Scholar] [CrossRef]
- Lidenholm, J.; Lundin, U. Estimation of Hydropower Generator Parameters through Field Simulations of Standard Tests. IEEE Trans. Energy Convers. 2010, 25, 931–939. [Google Scholar] [CrossRef]
- Kumar, T.; Kumar, R.; Jain, S.C. Finite Element Modeling of Big Turbo-Generator Rotor Vibrations using Newmark-beta Integration Method. IOP Conf. Ser. Mater. Sci. Eng. 2020, 876, 012004. [Google Scholar] [CrossRef]
- Kumar, T.; Kumar, R.; Jain, S.C. Numerical Investigation of Semi-active Torsional Vibration Control of Heavy Turbo-generator Rotor using Magnetorheological Fluid Dampers. J. Vib. Eng. Technol. 2021, 9, 967–981. [Google Scholar] [CrossRef]
- Klontz, K.W.; Miller, T.J.E.; McGilp, M.I.; Karmaker, H.; Zhong, P. Short-Circuit Analysis of Permanent-Magnet Generators. IEEE Trans. Ind. Appl. 2011, 47, 1670–1680. [Google Scholar] [CrossRef]
- Xiao, Y.; Zhou, L.; Wang, J.; Yang, R. Finite Element Computation of Transient Parameters of a Salient-Pole Synchronous Machine. Energies 2017, 10, 1015. [Google Scholar] [CrossRef] [Green Version]
- Gozdowiak, A. Faulty Synchronization of Salient Pole Synchronous Hydro Generator. Energies 2020, 13, 5491. [Google Scholar] [CrossRef]
- Marjanović, M.; Prijić, A.; Randjelović, B.; Prijić, Z. A Transient Modeling of the Thermoelectric Generators for Application in Wireless Sensor Network Nodes. Electronics 2020, 9, 1015. [Google Scholar] [CrossRef]
- Liu, T.; Wang, E.; Meng, F.; Zhang, F.; Zhao, C.; Zhang, H.; Zhao, R. Operation Characteristics and Transient Simulation of an ICE-ORC Combined System. Appl. Sci. 2020, 9, 1639. [Google Scholar] [CrossRef] [Green Version]
- Gang, L.; Dihui, Z.; Tong, Z. Influences on performance in 3D analysis of linear induction motors with different transverse m.m.f. models in winding end-regions. IET Electr. Power Appl. 2017, 11, 1424–1431. [Google Scholar] [CrossRef]
- Xiao, S.; Ge, B.; Liu, Z. Multi-loop model for fractional pole-path ratio synchronous generators with stator winding internal faults. IET Electr. Power Appl. 2020, 14, 2687–2696. [Google Scholar] [CrossRef]
- Waheed, A.; Ro, J.S. Analytical Modeling for Optimal Rotor Shape to Design Highly Efficient Line-Start Permanent Magnet Synchronous Motor. IEEE Access 2020, 8, 145672–145686. [Google Scholar] [CrossRef]
- Huangfu, Y.; Wang, S.; Qiu, J.; Zhang, H.; Wang, G.; Zhu, J. Transient Performance Analysis of Induction Motor Using Field-Circuit Coupled Finite-Element Method. IEEE Trans. Magn. 2014, 50, 873–876. [Google Scholar] [CrossRef]
- Hu, Q.L.; Xiao, K.; Zhou, Z.T.; Fan, Z.N.; Yang, Y.; Bian, Z.Y.; Li, J.C.; Yao, B. 3D Transient Electromagnetic-Temperature Field Analysis of the Loss and Heat of the Damper Bars of a Large Tubular Hydro-Generator During Short Circuit Faults. IEEE Access 2020, 8, 135963–135974. [Google Scholar] [CrossRef]
- Skok, S.; Ivanković, I.; Zbunjak, Z. Two Layer Hydropower Plant Dynamic Mathematical Modelling Using Synchronized Measurements. Int. J. Electr. Power Energy Syst. 2018, 103, 302–309. [Google Scholar] [CrossRef]

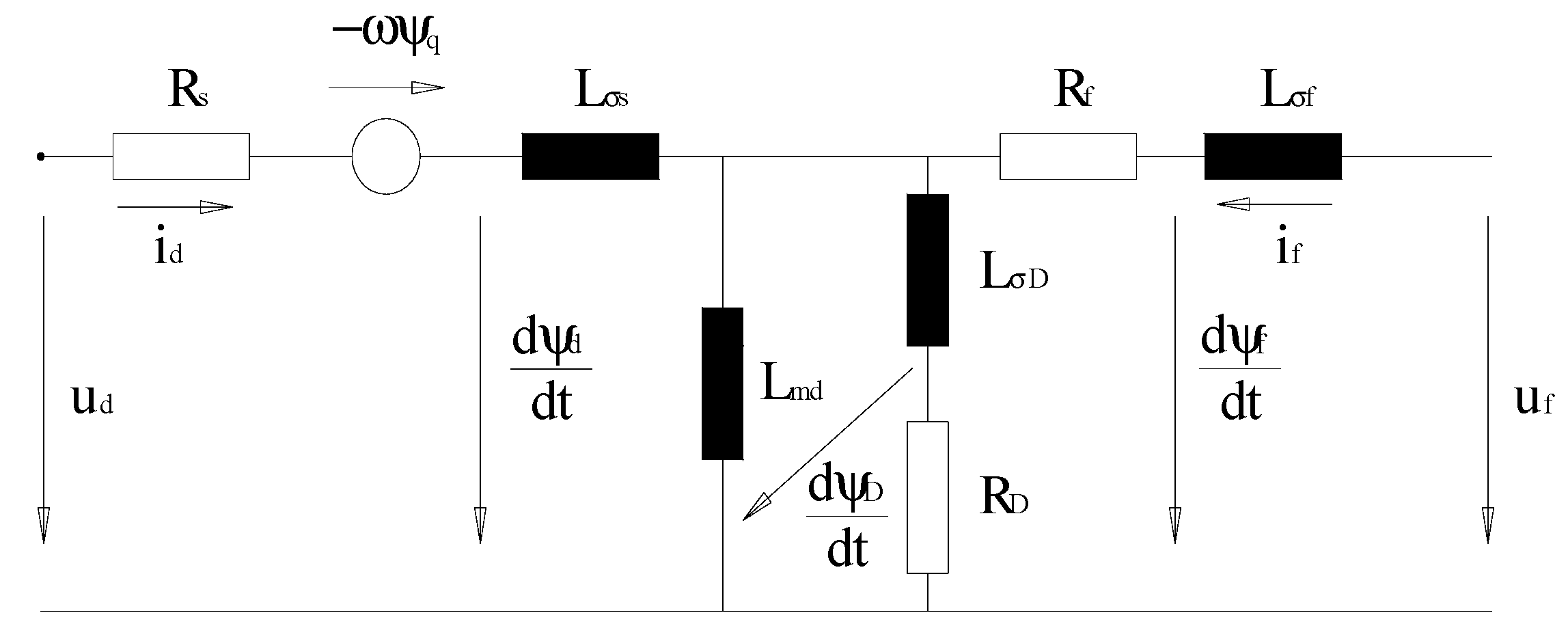


Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Tomičić, B.; Šumiga, A.; Nađ, J.; Srpak, D. Verification Methodology for Simulation Models of the Synchronous Generator on Transients Analysis. Appl. Sci. 2021, 11, 11734. https://doi.org/10.3390/app112411734
Tomičić B, Šumiga A, Nađ J, Srpak D. Verification Methodology for Simulation Models of the Synchronous Generator on Transients Analysis. Applied Sciences. 2021; 11(24):11734. https://doi.org/10.3390/app112411734
Chicago/Turabian StyleTomičić, Branko, Antonija Šumiga, Josip Nađ, and Dunja Srpak. 2021. "Verification Methodology for Simulation Models of the Synchronous Generator on Transients Analysis" Applied Sciences 11, no. 24: 11734. https://doi.org/10.3390/app112411734
APA StyleTomičić, B., Šumiga, A., Nađ, J., & Srpak, D. (2021). Verification Methodology for Simulation Models of the Synchronous Generator on Transients Analysis. Applied Sciences, 11(24), 11734. https://doi.org/10.3390/app112411734





