Miscibility Regimes in a 23Na–39K Quantum Mixture
Abstract
:1. Introduction
2. Methods
2.1. Description of an Atomic Quantum Mixture
2.2. Numerical Simulation of the Ground-State
3. Results
3.1. Experimental Setup
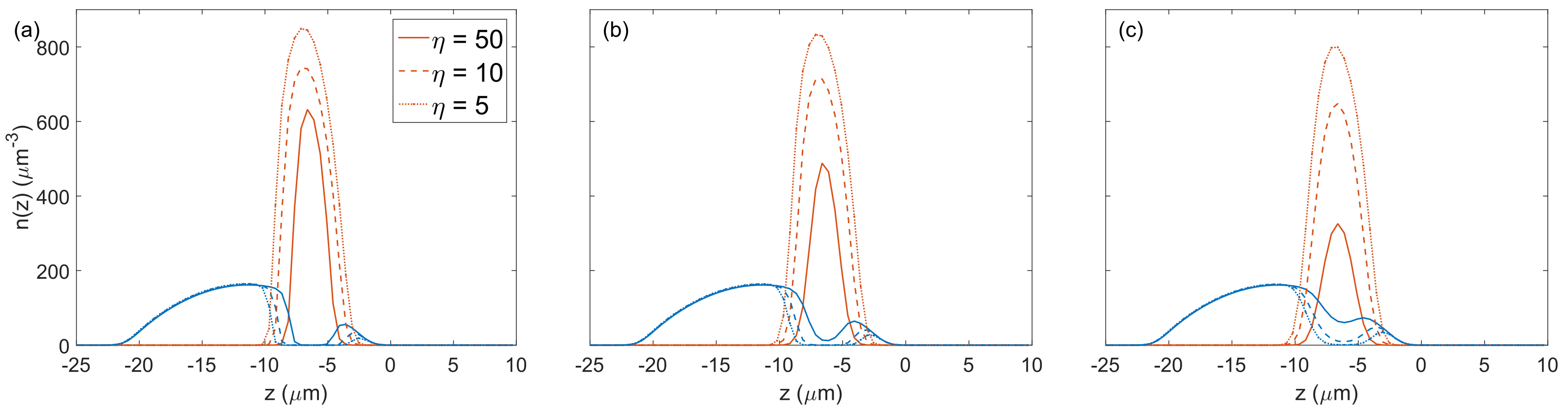
3.2. Ground-State of Na–K Mixtures
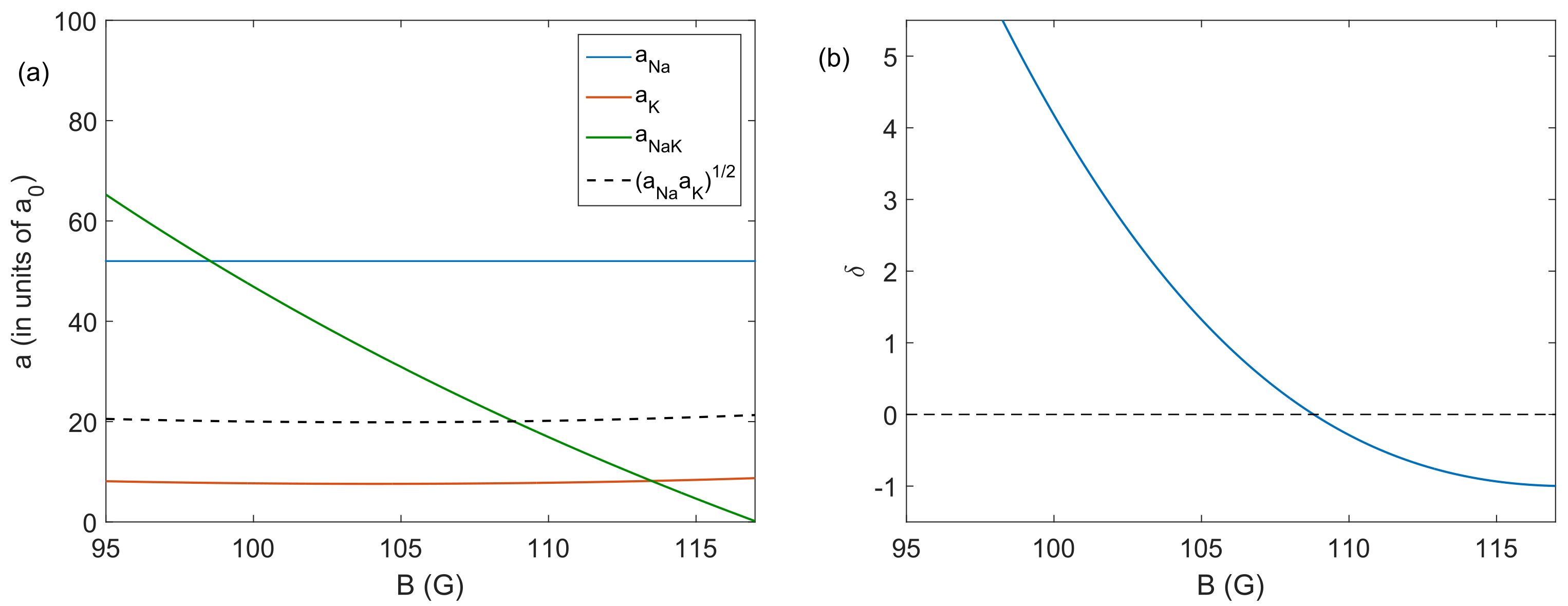
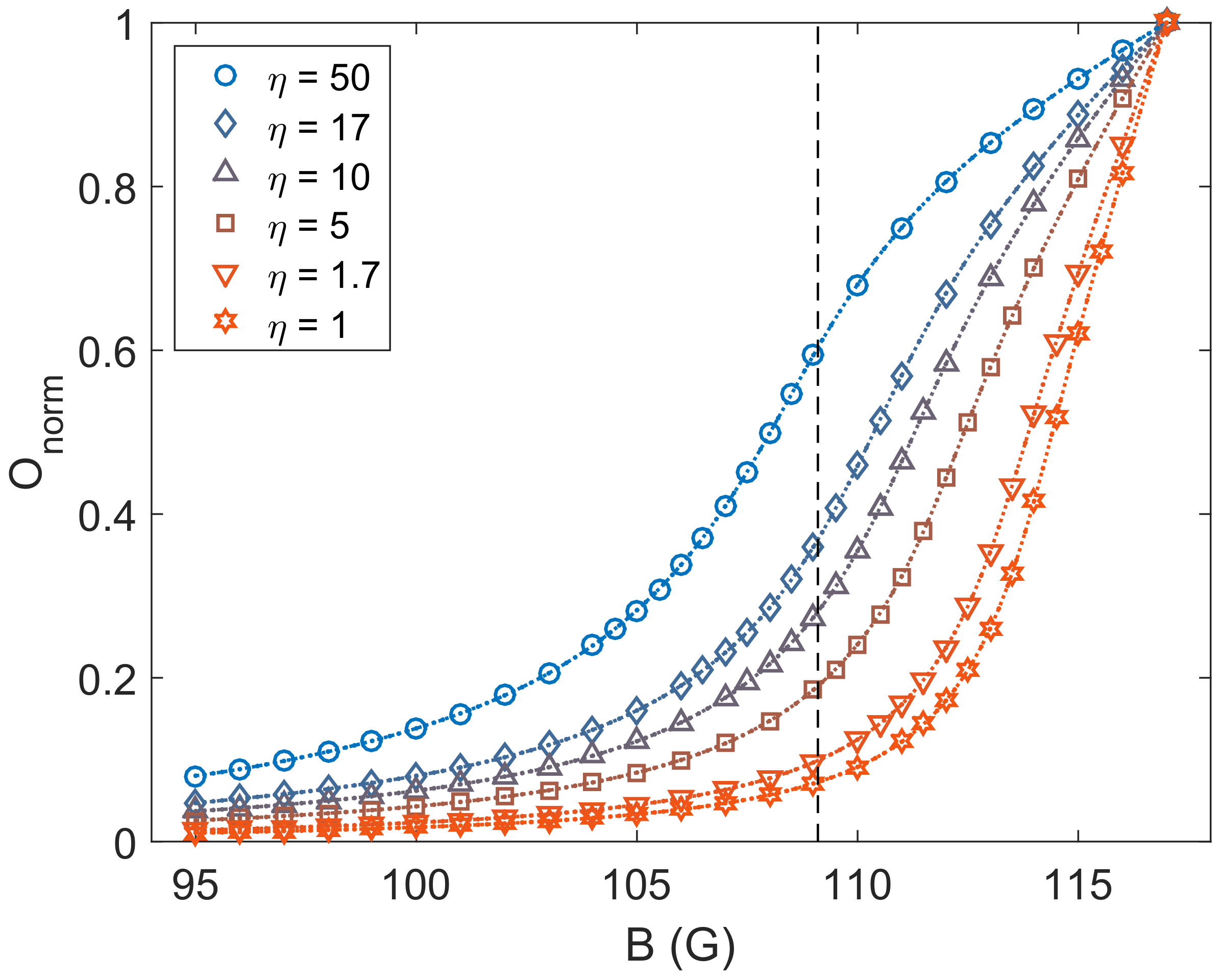
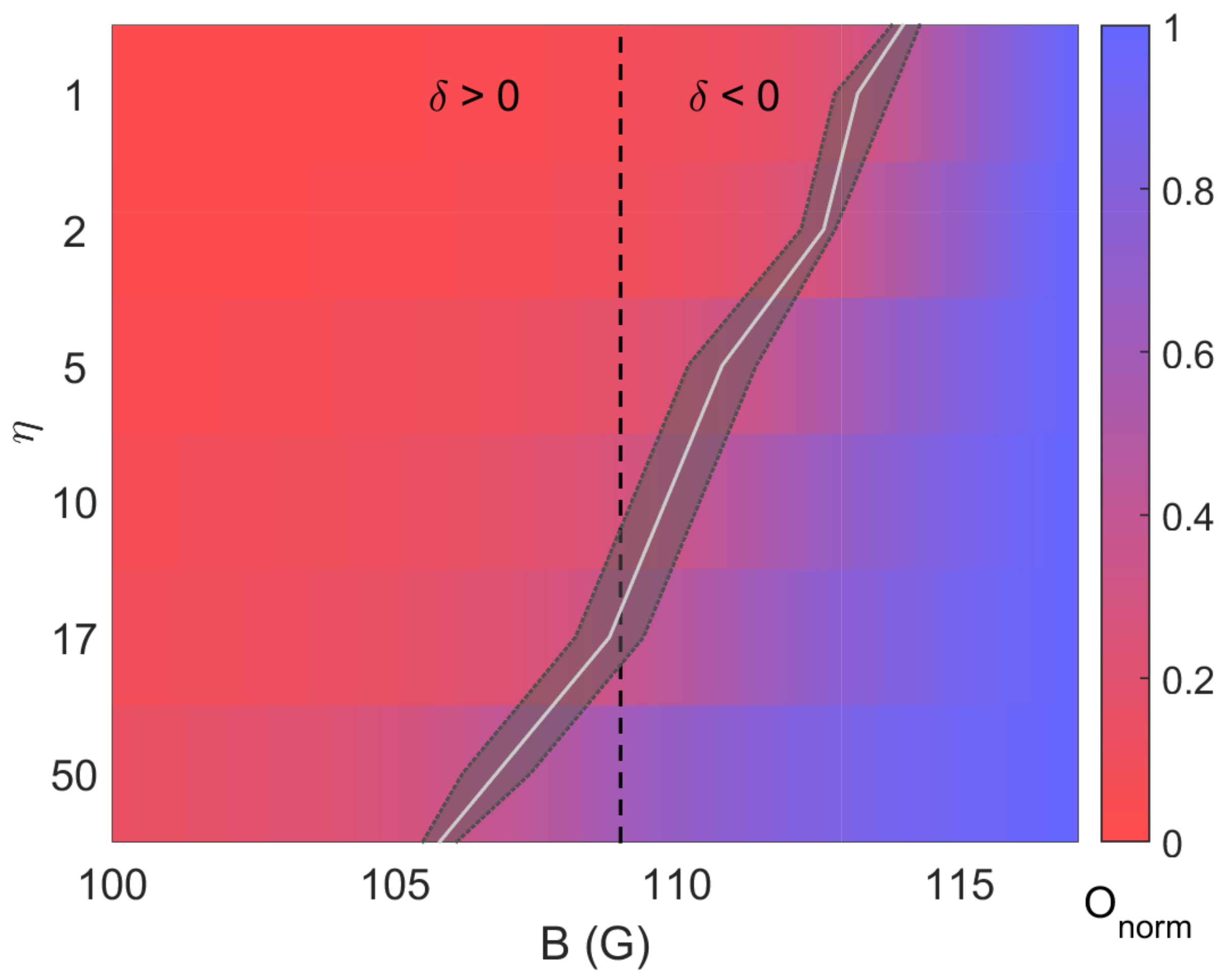
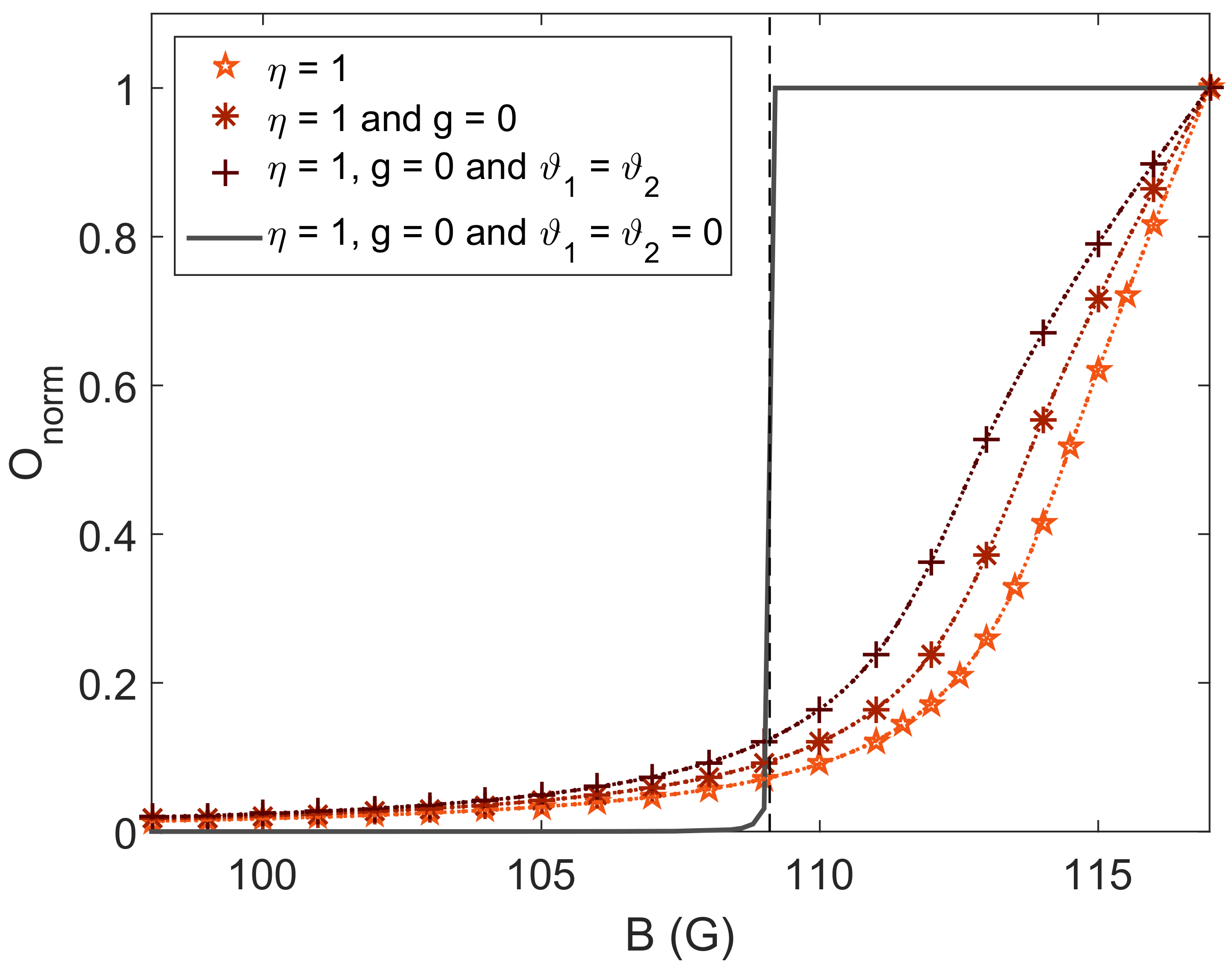
3.3. The Miscibility Phase Diagram
4. Discussion
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Graf, E.H.; Lee, D.M.; Reppy, J.D. Phase Separation and the Superfluid Transition in Liquid He3-He4 Mixtures. Phys. Rev. Lett. 1967, 19, 417–419. [Google Scholar] [CrossRef]
- Pricaupenko, L.; Treiner, J. Phase Separation of Liquid 3He-4He Mixtures: Effect of Confinement. Phys. Rev. Lett. 1995, 74, 430–433. [Google Scholar] [CrossRef] [PubMed]
- Maciołek, A.; Krech, M.; Dietrich, S. Phase diagram of a model for 3He-4He mixtures in three dimensions. Phys. Rev. E 2004, 69, 036117. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Bennemann, K.H.; Ketterson, J.B. Physics of Liquid and Solid Helium. Part I; John Wiley and Sons, Inc.: New York, NY, USA, 1978. [Google Scholar]
- Stenger, J.; Inouye, S.; Stamper-Kurn, D.; Miesner, H.J.; Chikkatur, A.; Ketterle, W. Spin domains in ground-state Bose–Einstein condensates. Nature 1998, 396, 345–348. [Google Scholar] [CrossRef] [Green Version]
- Myatt, C.J.; Burt, E.A.; Ghrist, R.W.; Cornell, E.A.; Wieman, C.E. Production of Two Overlapping Bose–Einstein Condensates by Sympathetic Cooling. Phys. Rev. Lett. 1997, 78, 586–589. [Google Scholar] [CrossRef]
- Hall, D.S.; Matthews, M.R.; Ensher, J.R.; Wieman, C.E.; Cornell, E.A. Dynamics of Component Separation in a Binary Mixture of Bose–Einstein Condensates. Phys. Rev. Lett. 1998, 81, 1539–1542. [Google Scholar] [CrossRef] [Green Version]
- Ospelkaus, S.; Ospelkaus, C.; Humbert, L.; Sengstock, K.; Bongs, K. Tuning of Heteronuclear Interactions in a Degenerate Fermi–Bose Mixture. Phys. Rev. Lett. 2006, 97, 120403. [Google Scholar] [CrossRef] [Green Version]
- Papp, S.B.; Pino, J.M.; Wieman, C.E. Tunable Miscibility in a Dual-Species Bose–Einstein Condensate. Phys. Rev. Lett. 2008, 101, 040402. [Google Scholar] [CrossRef] [Green Version]
- McCarron, D.J.; Cho, H.W.; Jenkin, D.L.; Köppinger, M.P.; Cornish, S.L. Dual-species Bose–Einstein condensate of 87Rb and 133Cs. Phys. Rev. A 2011, 84, 011603. [Google Scholar] [CrossRef] [Green Version]
- Pasquiou, B.; Bayerle, A.; Tzanova, S.M.; Stellmer, S.; Szczepkowski, J.; Parigger, M.; Grimm, R.; Schreck, F. Quantum degenerate mixtures of strontium and rubidium atoms. Phys. Rev. A 2013, 88, 023601. [Google Scholar] [CrossRef] [Green Version]
- Wang, F.; Li, X.; Xiong, D.; Wang, D. A double species 23Na and 87Rb Bose–Einstein condensate with tunable miscibility via an interspecies Feshbach resonance. J. Phys. B At. Mol. Opt. Phys. 2015, 49, 015302. [Google Scholar] [CrossRef] [Green Version]
- Wacker, L.; Jørgensen, N.B.; Birkmose, D.; Horchani, R.; Ertmer, W.; Klempt, C.; Winter, N.; Sherson, J.; Arlt, J.J. Tunable dual-species Bose–Einstein condensates of 39K and 87Rb. Phys. Rev. A 2015, 92, 053602. [Google Scholar] [CrossRef] [Green Version]
- Schulze, T.A.; Hartmann, T.; Voges, K.K.; Gempel, M.W.; Tiemann, E.; Zenesini, A.; Ospelkaus, S. Feshbach spectroscopy and dual-species Bose–Einstein condensation of 23Na-39K mixtures. Phys. Rev. A 2018, 97, 023623. [Google Scholar] [CrossRef] [Green Version]
- Altman, E.; Hofstetter, W.; Demler, E.; Lukin, M.D. Phase diagram of two-component bosons on an optical lattice. New J. Phys. 2003, 5, 113. [Google Scholar] [CrossRef]
- Isacsson, A.; Cha, M.C.; Sengupta, K.; Girvin, S. Superfluid-insulator transitions of two-species bosons in an optical lattice. Phys. Rev. B 2005, 72, 184507. [Google Scholar] [CrossRef] [Green Version]
- Bruderer, M.; Bao, W.; Jaksch, D. Self-trapping of impurities in Bose–Einstein condensates: Strong attractive and repulsive coupling. EPL (Europhys. Lett.) 2008, 82, 30004. [Google Scholar] [CrossRef] [Green Version]
- Spethmann, N.; Kindermann, F.; John, S.; Weber, C.; Meschede, D.; Widera, A. Dynamics of single neutral impurity atoms immersed in an ultracold gas. Phys. Rev. Lett. 2012, 109, 235301. [Google Scholar] [CrossRef] [Green Version]
- Cabrera, C.; Tanzi, L.; Sanz, J.; Naylor, B.; Thomas, P.; Cheiney, P.; Tarruell, L. Quantum liquid droplets in a mixture of Bose–Einstein condensates. Science 2018, 359, 301–304. [Google Scholar] [CrossRef] [Green Version]
- Semeghini, G.; Ferioli, G.; Masi, L.; Mazzinghi, C.; Wolswijk, L.; Minardi, F.; Modugno, M.; Modugno, G.; Inguscio, M.; Fattori, M. Self-bound quantum droplets of atomic mixtures in free space. Phys. Rev. Lett. 2018, 120, 235301. [Google Scholar] [CrossRef] [Green Version]
- D’Errico, C.; Burchianti, A.; Prevedelli, M.; Salasnich, L.; Ancilotto, F.; Modugno, M.; Minardi, F.; Fort, C. Observation of quantum droplets in a heteronuclear bosonic mixture. Phys. Rev. Res. 2019, 1, 033155. [Google Scholar] [CrossRef] [Green Version]
- Guo, Z.; Jia, F.; Li, L.; Ma, Y.; Hutson, J.M.; Cui, X.; Wang, D. Lee-Huang-Yang effects in the ultracold mixture of 23Na and 87Rb with attractive interspecies interactions. arXiv 2021, arXiv:2105.01277. [Google Scholar]
- Khabibullaev, P.K.; Saidov, A. Phase Separation in Soft Matter Physics: Micellar Solutions, Microemulsions, Critical Phenomena; Springer Science & Business Media: Berlin/Heidelberg, Germany, 2003; Volume 138. [Google Scholar]
- Dagotto, E. Nanoscale Phase Separation and Colossal Magnetoresistance: The Physics of Manganites and Related Compounds; Springer Science & Business Media: Berlin/Heidelberg, Germany, 2013; Volume 136. [Google Scholar]
- Pethick, C.J.; Smith, H. Bose—Einstein Condensation in Dilute Gases; Cambridge University Press: Cambridge, UK, 2008. [Google Scholar]
- Pitaevskii, L.; Stringari, S. Bose—Einstein Condensation; Oxford University Press: Oxford, UK, 2003. [Google Scholar]
- Chin, C.; Grimm, R.; Julienne, P.; Tiesinga, E. Feshbach resonances in ultracold gases. Rev. Mod. Phys. 2010, 82, 1225–1286. [Google Scholar] [CrossRef]
- Gaunt, A.L.; Schmidutz, T.F.; Gotlibovych, I.; Smith, R.P.; Hadzibabic, Z. Bose–Einstein Condensation of Atoms in a Uniform Potential. Phys. Rev. Lett. 2013, 110, 200406. [Google Scholar] [CrossRef]
- Wen, L.; Liu, W.M.; Cai, Y.; Zhang, J.M.; Hu, J. Controlling phase separation of a two-component Bose–Einstein condensate by confinement. Phys. Rev. A 2012, 85, 043602. [Google Scholar] [CrossRef] [Green Version]
- Navarro, R.; Carretero-González, R.; Kevrekidis, P.G. Phase separation and dynamics of two-component Bose–Einstein condensates. Phys. Rev. A 2009, 80, 023613. [Google Scholar] [CrossRef] [Green Version]
- Bisset, R.N.; Kevrekidis, P.G.; Ticknor, C. Enhanced quantum spin fluctuations in a binary Bose–Einstein condensate. Phys. Rev. A 2018, 97, 023602. [Google Scholar] [CrossRef] [Green Version]
- Wen, L.; Guo, H.; Wang, Y.J.; Hu, A.Y.; Saito, H.; Dai, C.Q.; Zhang, X.F. Effects of atom numbers on the miscibility-immiscibility transition of a binary Bose–Einstein condensate. Phys. Rev. A 2020, 101, 033610. [Google Scholar] [CrossRef]
- Tanatar, B.; Erkan, K. Strongly interacting one-dimensional Bose–Einstein condensates in harmonic traps. Phys. Rev. A 2000, 62, 053601. [Google Scholar] [CrossRef]
- Ma, H.; Pang, T. Condensate-profile asymmetry of a boson mixture in a disk-shaped harmonic trap. Phys. Rev. A 2004, 70, 063606. [Google Scholar] [CrossRef] [Green Version]
- Lee, K.L.; Jørgensen, N.B.; Liu, I.K.; Wacker, L.; Arlt, J.J.; Proukakis, N.P. Phase separation and dynamics of two-component Bose–Einstein condensates. Phys. Rev. A 2016, 94, 013602. [Google Scholar] [CrossRef] [Green Version]
- Cikojević, V.; Markić, L.V.; Boronat, J. Harmonically trapped Bose–Bose mixtures: A quantum Monte Carlo study. New J. Phys. 2018, 20, 085002. [Google Scholar] [CrossRef]
- Pires, R.; Ulmanis, J.; Häfner, S.; Repp, M.; Arias, A.; Kuhnle, E.D.; Weidemüller, M. Observation of Efimov Resonances in a Mixture with Extreme Mass Imbalance. Phys. Rev. Lett. 2014, 112, 250404. [Google Scholar] [CrossRef] [Green Version]
- Yao, X.C.; Chen, H.Z.; Wu, Y.P.; Liu, X.P.; Wang, X.Q.; Jiang, X.; Deng, Y.; Chen, Y.A.; Pan, J.W. Observation of Coupled Vortex Lattices in a Mass-Imbalance Bose and Fermi Superfluid Mixture. Phys. Rev. Lett. 2016, 117, 145301. [Google Scholar] [CrossRef] [Green Version]
- d’Errico, C.; Zaccanti, M.; Fattori, M.; Roati, G.; Inguscio, M.; Modugno, G.; Simoni, A. Feshbach resonances in ultracold 39K. New J. Phys. 2007, 9, 223. [Google Scholar] [CrossRef] [Green Version]
- Castilho, P.; Pedrozo-Peñafiel, E.; Gutierrez, E.; Mazo, P.; Roati, G.; Farias, K.; Bagnato, V. A compact experimental machine for studying tunable Bose–Bose superfluid mixtures. Laser Phys. Lett. 2019, 16, 035501. [Google Scholar] [CrossRef] [Green Version]
- Burchianti, A.; D’Errico, C.; Rosi, S.; Simoni, A.; Modugno, M.; Fort, C.; Minardi, F. Dual-species Bose–Einstein condensate of 41K and 87Rb in a hybrid trap. Phys. Rev. A 2018, 98, 063616. [Google Scholar] [CrossRef]
- Ota, M.; Giorgini, S.; Stringari, S. Magnetic Phase Transition in a Mixture of Two Interacting Superfluid Bose Gases at Finite Temperature. Phys. Rev. Lett. 2019, 123, 075301. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Ota, M.; Giorgini, S. Thermodynamics of dilute Bose gases: Beyond mean-field theory for binary mixtures of Bose–Einstein condensates. Phys. Rev. A 2020, 102, 063303. [Google Scholar] [CrossRef]
- Roy, A.; Ota, M.; Recati, A.; Dalfovo, F. Finite-temperature spin dynamics of a two-dimensional Bose-Bose atomic mixture. Phys. Rev. Res. 2021, 3, 013161. [Google Scholar] [CrossRef]
- Griffin, A.; Snoke, D.W.; Stringari, S. Bose—Einstein Condensation; Cambridge University Press: Cambridge, UK, 1996. [Google Scholar]
- Riboli, F.; Modugno, M. Topology of the ground state of two interacting Bose–Einstein condensates. Phys. Rev. A 2002, 65, 063614. [Google Scholar] [CrossRef] [Green Version]
- Dalfovo, F.; Stringari, S. Bosons in anisotropic traps: Ground state and vortices. Phys. Rev. A 1996, 53, 2477. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Catani, J.; Maioli, P.; De Sarlo, L.; Minardi, F.; Inguscio, M. Intense slow beams of bosonic potassium isotopes. Phys. Rev. A 2006, 73, 033415. [Google Scholar] [CrossRef] [Green Version]
- Lamporesi, G.; Donadello, S.; Serafini, S.; Ferrari, G. Compact high-flux source of cold sodium atoms. Rev. Sci. Instrum. 2013, 84, 063102. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Wu, C.H. Strongly Interacting Quantum Mixtures of Ultracold Atoms. Ph.D. Thesis, Massachusetts Institute of Technology, Cambridge, MA, USA, 2013. [Google Scholar]
- Davis, K.B.; Mewes, M.O.; Andrews, M.R.; van Druten, N.J.; Durfee, D.S.; Kurn, D.M.; Ketterle, W. Bose–Einstein Condensation in a Gas of Sodium Atoms. Phys. Rev. Lett. 1995, 75, 3969–3973. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Masuhara, N.; Doyle, J.M.; Sandberg, J.C.; Kleppner, D.; Greytak, T.J.; Hess, H.F.; Kochanski, G.P. Evaporative Cooling of Spin-Polarized Atomic Hydrogen. Phys. Rev. Lett. 1988, 61, 935–938. [Google Scholar] [CrossRef]
- Schreck, F.; Ferrari, G.; Corwin, K.L.; Cubizolles, J.; Khaykovich, L.; Mewes, M.O.; Salomon, C. Sympathetic cooling of bosonic and fermionic lithium gases towards quantum degeneracy. Phys. Rev. A 2001, 64, 011402. [Google Scholar] [CrossRef] [Green Version]
- Modugno, G.; Ferrari, G.; Roati, G.; Brecha, R.J.; Simoni, A.; Inguscio, M. Bose–Einstein condensation of potassium atoms by sympathetic cooling. Science 2001, 294, 1320–1322. [Google Scholar] [CrossRef]
- Grimm, R.; Weidemüller, M.; Ovchinnikov, Y.B. Optical dipole traps for neutral atoms. Adv. At. Mol. Opt. Phys. 2000, 42, 95–170. [Google Scholar]
- Zhan, F.; Sabbatini, J.; Davis, M.J.; McCulloch, I.P. Miscible-immiscible quantum phase transition in coupled two-component Bose–Einstein condensates in one-dimensional optical lattices. Phys. Rev. A 2014, 90, 023630. [Google Scholar] [CrossRef] [Green Version]
- Richaud, A.; Zenesini, A.; Penna, V. The mixing-demixing phase diagram of ultracold heteronuclear mixtures in a ring trimer. Sci. Rep. 2019, 9, 6908. [Google Scholar] [CrossRef] [Green Version]
- Kumar, R.K.; Muruganandam, P.; Tomio, L.; Gammal, A. Miscibility in coupled dipolar and non-dipolar Bose–Einstein condensates. J. Phys. Commun. 2017, 1, 035012. [Google Scholar] [CrossRef]
- Kasamatsu, K.; Tsubota, M.; Ueda, M. Vortex Phase Diagram in Rotating Two-Component Bose–Einstein Condensates. Phys. Rev. Lett. 2003, 91, 150406. [Google Scholar] [CrossRef] [Green Version]
- Mason, P.; Aftalion, A. Classification of the ground states and topological defects in a rotating two-component Bose–Einstein condensate. Phys. Rev. A 2011, 84, 033611. [Google Scholar] [CrossRef] [Green Version]
- Kuopanportti, P.; Huhtamäki, J.A.M.; Möttönen, M. Exotic vortex lattices in two-species Bose–Einstein condensates. Phys. Rev. A 2012, 85, 043613. [Google Scholar] [CrossRef] [Green Version]
- Takeuchi, H.; Ishino, S.; Tsubota, M. Binary Quantum Turbulence Arising from Countersuperflow Instability in Two-Component Bose–Einstein Condensates. Phys. Rev. Lett. 2010, 105, 205301. [Google Scholar] [CrossRef] [PubMed]
- Fava, E.; Bienaimé, T.; Mordini, C.; Colzi, G.; Qu, C.; Stringari, S.; Lamporesi, G.; Ferrari, G. Observation of Spin Superfluidity in a Bose Gas Mixture. Phys. Rev. Lett. 2018, 120, 170401. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Kim, J.H.; Hong, D.; Shin, Y. Observation of two sound modes in a binary superfluid gas. Phys. Rev. A 2020, 101, 061601. [Google Scholar] [CrossRef]
- Kasamatsu, K.; Tsubota, M. Modulation instability and solitary-wave formation in two-component Bose–Einstein condensates. Phys. Rev. A 2006, 74, 013617. [Google Scholar] [CrossRef] [Green Version]
- Kadokura, T.; Aioi, T.; Sasaki, K.; Kishimoto, T.; Saito, H. Rayleigh-Taylor instability in a two-component Bose–Einstein condensate with rotational symmetry. Phys. Rev. A 2012, 85, 013602. [Google Scholar] [CrossRef] [Green Version]
- Baggaley, A.W.; Parker, N.G. Kelvin-Helmholtz instability in a single-component atomic superfluid. Phys. Rev. A 2018, 97, 053608. [Google Scholar] [CrossRef] [Green Version]
- Maity, D.K.; Mukherjee, K.; Mistakidis, S.I.; Das, S.; Kevrekidis, P.G.; Majumder, S.; Schmelcher, P. Parametrically excited star-shaped patterns at the interface of binary Bose–Einstein condensates. Phys. Rev. A 2020, 102, 033320. [Google Scholar] [CrossRef]

Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Gutierrez, E.M.; de Oliveira, G.A.; Farias, K.M.; Bagnato, V.S.; Castilho, P.C.M. Miscibility Regimes in a 23Na–39K Quantum Mixture. Appl. Sci. 2021, 11, 9099. https://doi.org/10.3390/app11199099
Gutierrez EM, de Oliveira GA, Farias KM, Bagnato VS, Castilho PCM. Miscibility Regimes in a 23Na–39K Quantum Mixture. Applied Sciences. 2021; 11(19):9099. https://doi.org/10.3390/app11199099
Chicago/Turabian StyleGutierrez, Emmanuel Mercado, Gustavo Alves de Oliveira, Kilvia Mayre Farias, Vanderlei Salvador Bagnato, and Patricia Christina Marques Castilho. 2021. "Miscibility Regimes in a 23Na–39K Quantum Mixture" Applied Sciences 11, no. 19: 9099. https://doi.org/10.3390/app11199099
APA StyleGutierrez, E. M., de Oliveira, G. A., Farias, K. M., Bagnato, V. S., & Castilho, P. C. M. (2021). Miscibility Regimes in a 23Na–39K Quantum Mixture. Applied Sciences, 11(19), 9099. https://doi.org/10.3390/app11199099





