Power-Flow and Mechanical Efficiency Computation in Two-Degrees-of-Freedom Planetary Gear Units: New Compact Formulas

Abstract

The mechanical efficiency is a computed value for comparing the performance of the multi degrees-of-freedom geared transmissions of hybrid vehicles. Most of the current methods for estimating gear trains mechanical efficiency require the decomposition of gear transmissions in basic structural elements or planetary gear units (PGU). These are two degrees-of-freedom components whose mechanical efficiency has a deep influence on the overall device. The authors (E.L.E., E.P.) already evidenced that, under certain kinematic conditions, the classic Radzimovsky’s formulas, widely accepted for computing the mechanical efficiency of PGUs, are not adequate. In this paper, more general and reliable formulas for computing the mechanical efficiency are deduced. The proposed formulas herein, exploiting the concept of potential or virtual power, evidence the dependency between kinematics and efficiency. A numerical example compares our results with previous work on the subject.

1. Introduction

The multi degrees-of-freedom gear drive is a key component in hybrid vehicles. Its capability of managing power flows from different sources is of paramount importance for energy saving. For these reasons, the mechanical efficiency of planetary multi degrees-of-freedom planetary gear trains is a topic that received many contributions in recent times (e.g., [1,2,3,4,5,6,7,8,9,10,11,12,13,14]).

The analysis carried out herein offers a reliable approach toward a synthetic description of the relationship between kinematics conditions and gear train mechanical efficiency.

In this paper, the kinematic conditions governing the relationship between the algebraic signs of actual and virtual or potential powers are elucidated for the first time. Such an analysis has a significant influence on the casting of physically reliable and consistent formulas for the computation of mechanical efficiency in a two dof PGU (Planetary Gear Unit). Subsequently, two general formulas for mechanical efficiency computation in a two dof PGU are proposed. The differential unit is the building block of more complex planetary gear trains. Thus, the understanding of the two dof PGU basic mechanics influences the design of the entire planetary gear drive [15].

The approach adopted herein is based on the concept of virtual or potential power, at the base of thoughtful contributions on the mechanical efficiency of planetary gear trains [5,16,17,18,19,20,21,22]. The virtual power, a term coined by Chen and Angeles [17], or potential power, coined by Esmail [5], is the power measured by an observer on any arbitrary moving frame [23,24]. However, the use of kinematic inversion for torque and efficiency analysis on gear trains dates back to Macmillan [16]. In particular, he recognized that the torques acting on the PGU links and power losses are independent of the observer’s motion. The following quote is taken from [16]:

...our analysis is based upon an important principle relating to torques and the power lost in friction; this is the fact that magnitudes of the torques acting upon the various members of the gear are quite independent of the motion of the observer who measures them. In addition, the power lost, being determined solely by the internal torques and the relative motions of the wheels within the gear, is also independent of the observer’s motion.

Although Macmillan’s analysis was limited to one dof PGU, the importance of kinematic inversion in the gear trains mechanical efficiency analysis is well represented.

The present paper was stimulated by the discovery that, under certain kinematic conditions, the classic Radzimovsky formulas [15,25,26,27] for the computation of mechanical efficiency in a two dof gear unit are not valid anymore [28]. This situation may lead to an estimation of the overall mechanical efficiency flawed by errors.

As discussed by Pennestrì & Valentini [15], the Radzimovsky formulas consider the two dof epicyclic gear train as two single dof devices in parallel and crossed by a power-flow. These formulas are deduced after application of the general mechanical efficiency formula of a mechanical system composed of two parallel devices with a given mechanical efficiency and power-flow direction. However, with the introduction of the concept of virtual or potential power, the mechanical efficiency of an epicyclic gear train is reduced to that of an ordinary gear train. For this purpose, a kinematic inversion is introduced such that the observed motion of the gear carrier is canceled.

The orientation of power flow observed under such kinematic inversion may not be the same as one of epicyclic arrangement. This situation requires special attention and the algebraic expression of the overall mechanical efficiency should be set in a consistent manner. The new proposed formulas herein are of a general nature and do not suffer any of the mentioned pitfalls.

In this paper, the concept of virtual or potential power was applied to deduce two new general mechanical efficiency formulas that cover all the working modes of a two dof PGU:

-

Two driving members (i.e., those with positive powers) and one driven member (i.e., the one with negative power);

-

Two driven members and one driving member.

Our approach is based only on the application of mechanics first principles, namely, Willis’ formula, torque equilibrium, kinematic inversion, power balance and definition of mechanical efficiency. As a byproduct, general formulas of the power-flow ratios, for the case of a gear unit without power losses, are also deduced.

For the mechanical efficiency analysis, members with constant velocities and meshing losses only are considered. A constant mechanical efficiency is assumed for the unit working as an ordinary gear train. Moreover, the algebraic sign of the power-flow is not altered by friction.

On the basis of the previous hypotheses, two new compact formulas for the mechanical efficiency analysis of two dof PGU are deduced herein. The formulas can be adapted to any power flow arrangement within the PGU.

2. Power-Flow Ratios in a PGU

The PGU (see Figure 1) is composed of two mating gears and the gear carrier. Let us denote by x, y and z the three links of the PGU.

Angular speeds, torques and powers in a GPU are governed, respectively, by the well-known analytical conditions from Willis’ equation, torque equilibrium and power balance:

$$\begin{array}{cc}\hfill {\omega }_z+{\tau }_1{\omega }_x+{\tau }_2{\omega }_y& =0\hfill \end{array}$$

(1a)

$$\begin{array}{cc}\hfill T_x+T_y+T_z& =0\hfill \end{array}$$

(1b)

$$\begin{array}{cc}\hfill T_x{\omega }_x+T_y{\omega }_y+T_z{\omega }_z& =0\hfill \end{array}$$

(1c)

where ${\tau }_1$ and ${\tau }_2$ depend on the number of teeth ratio.

The readers will readily recognize (1a) as the Willis’ equation. In fact, setting z and x as the subscripts denoting the meshing gears and y the gear carrier, then

$${\displaystyle \frac{{\omega }_z-{\omega }_y}{{\omega }_x-{\omega }_y}}=N_{x,z}=\pm {\displaystyle \frac{\mathrm{No}.\mathrm{of}\mathrm{Teeth}\mathrm{of}\mathrm{gear}x}{\mathrm{No}.\mathrm{of}\mathrm{Teeth}\mathrm{of}\mathrm{gear}z}}$$

(2)

The comparison of (1a) with (2), yields ${\tau }_1=-N_{x,z}$ and ${\tau }_2=N_{x,z}-1$. Obviously, other subscript combinations are possible, with corresponding different analytical expressions of ${\tau }_1$ and ${\tau }_2$. The choice x, y and z for the initial indistinct labeling all the moving bodies composing the PGU is justified by the advantage of condensing all possible cases for mechanical efficiency computation in two formulas only. In other words, what is lost in apparent initial abstraction is later gained in the results’ generality.

After some algebraic manipulation of (1) one obtains the following:

$$\begin{array}{cc}\hfill {\displaystyle \frac{P_x}{P_y}}& =-{\displaystyle \frac{\left(1+{\tau }_2\right)+{\tau }_1{\displaystyle \frac{{\omega }_x}{{\omega }_y}}}{\left(1+{\tau }_1\right)+{\tau }_2{\displaystyle \frac{{\omega }_y}{{\omega }_x}}}}\hfill \end{array}$$

(3)

$$\begin{array}{cc}\hfill {\displaystyle \frac{P_y}{P_z}}& =-{\displaystyle \frac{\left(1+{\tau }_1\right)+{\tau }_2{\displaystyle \frac{{\omega }_y}{{\omega }_z}}}{\left({\tau }_1+{\tau }_2\right)+{\displaystyle \frac{{\omega }_z}{{\omega }_y}}}}\hfill \end{array}$$

(4)

where $P_x=T_x{\omega }_x$, $P_y=T_y{\omega }_y$ and $P_z=T_z{\omega }_z$ are the powers sustained by links x, y and z, respectively. The power ratios deduced by Pennestrì & Freudenstein [29] are particular cases of the previous equations.

Under ideal conditions, in a PGU, the sign of powers is established prescribing the following: two angular speeds and one torque (kinematically driven PGU) or, alternatively, one angular speed and two torques (torque driven PGU) [30].

In a two dof PGU we distinguish the following working modes:

-

Two driving links (namely x and y, $P_x>0$ and $P_y>0$) and one driving link (namely z, $P_z<0$);

-

Two driving links (namely x and y, $P_x<0$ and $P_y<0$) and one driving link (namely z, $P_z>0$).

In the current analysis, it is assumed that the presence of meshing losses does not alter the algebraic sign of actual power flows.

3. The Algebraic Sign of Virtual Power Flows

In a previous paper [28], the authors (E.L.E. and E.P. ) demonstrated that, under certain kinematic conditions, the Radzimovsky’s formulas do not hold. An analysis of power-flows algebraic signs is required to explain the limits for the Radzimovsky’s formulas and offer a more general alternative.

The following analysis will ascertain the algebraic sign correlation between the actual powers $P_x=T_x{\omega }_x$ and $P_y=T_y{\omega }_y$ and the virtual [17] or potential [5] powers, such as $P_x^y=T_x\left({\omega }_x-{\omega }_y\right)$ and $P_y^x=T_y\left({\omega }_y-{\omega }_x\right)$. For instance, the potential power corresponds to the power which would be transmitted by the gear unit, operating in a rotating reference frame at which link j appears relatively fixed and at relative angular velocity ${\omega }_u-{\omega }_j$, for link u. Therefore, the carrier potential power is measured under a kinematic inversion, making fixed the planet-carrier in the observer frame of reference. The potential power is the most important and basic principle of the mechanical efficiency analysis of differential devices).

Powers $P_x$ and $P_x^y$ have the same algebraic sign only if the following is true:

$${\displaystyle \frac{P_x^y}{P_x}}={\displaystyle \frac{{\omega }_x-{\omega }_y}{{\omega }_x}}>0$$

(5)

or

$${\displaystyle \frac{{\omega }_y}{{\omega }_x}}<1$$

(6)

Similarly, $P_y$ and $P_y^x$ have the same algebraic sign only if the following are true:

$${\displaystyle \frac{P_y^x}{P_y}}={\displaystyle \frac{{\omega }_y-{\omega }_x}{{\omega }_y}}>0$$

(7)

or

$${\displaystyle \frac{{\omega }_x}{{\omega }_y}}<1$$

(8)

There are two ranges consistent with inequality (6):

• ${\displaystyle \frac{{\omega }_y}{{\omega }_x}}<0$
• $0<{\displaystyle \frac{{\omega }_y}{{\omega }_x}}<1$

Moreover, we observe the following:

-

If ${\displaystyle \frac{{\omega }_y}{{\omega }_x}}<0$, then ${\displaystyle \frac{{\omega }_x}{{\omega }_y}}$ is also negative.

-

If $0<{\displaystyle \frac{{\omega }_y}{{\omega }_x}}<1$, then ${\displaystyle \frac{{\omega }_x}{{\omega }_y}}$ must be greater than one.

Case 1:${\omega }_x$ and ${\omega }_y$ have opposite algebraic signs.

In this case, Equations (6) and (8) are both simultaneously valid. $P_x^y$ and $P_x$, $P_y^x$ and $P_y$ have the same algebraic sign for any working mode, as depicted in the PGU block schemes shown in Figure 2 (1st working mode).

Case 2:${\omega }_x$ and ${\omega }_y$ have the same algebraic sign, or $\mathrm{sign}\left({\displaystyle \frac{{\omega }_y}{{\omega }_x}}\right)=+1$.

Under this hypothesis, Equations (6) and (8) cannot hold simultaneously.

Subcase 2a: When ${\displaystyle \frac{{\omega }_y}{{\omega }_x}}<1$, then $P_x^y$ and $P_x$ have the same algebraic sign. The described situation is depicted in Figure 3, 1st working mode.

Subcase 2b: When ${\displaystyle \frac{{\omega }_x}{{\omega }_y}}<1$, then $P_y^x$ and $P_y$ will have the same algebraic sign. The described situation is depicted in Figure 4, 1st working mode.

The previous analysis can be summarized as follows:

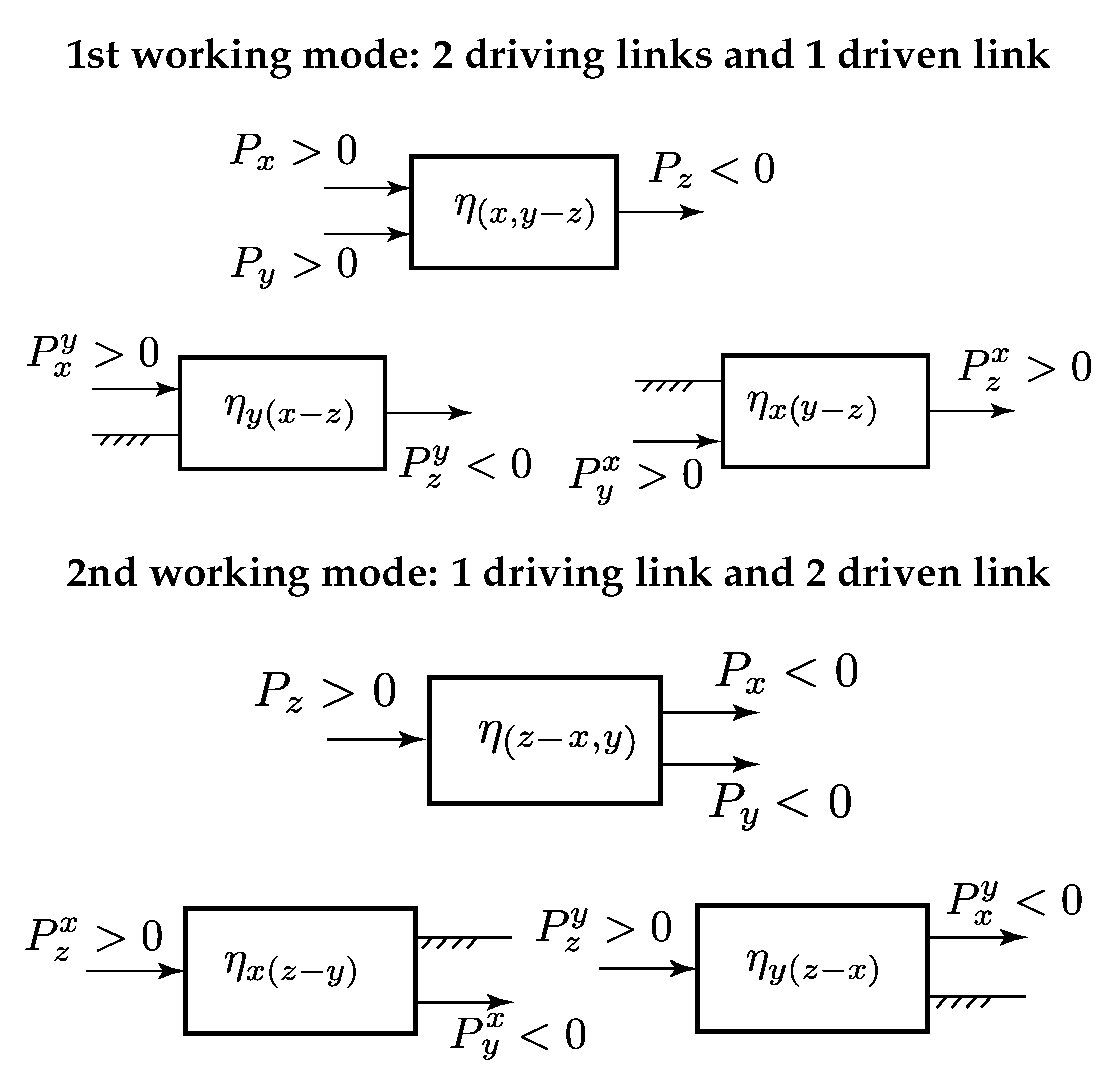
• When ${\omega }_x$ and ${\omega }_y$ do not have the same algebraic sign, the power ratios ${\displaystyle \frac{P_x^y}{P_x}}$ and ${\displaystyle \frac{P_y^x}{P_y}}$ will have simultaneously the same algebraic sign. For all the links, the direction of the virtual power-flow is the same as the actual power flow (see Figure 2).
• When ${\omega }_x$ and ${\omega }_y$ have the same algebraic sign, power ratios ${\displaystyle \frac{P_x^y}{P_x}}$ and ${\displaystyle \frac{P_y^x}{P_y}}$ cannot have simultaneously the same algebraic sign. In particular, the condition ${\displaystyle \frac{P_x^y}{P_x}}>0$ is fulfilled when ${\displaystyle \frac{{\omega }_y}{{\omega }_x}}>1$ (See Figure 3). Conversely, the condition ${\displaystyle \frac{P_y^x}{P_y}}>0$ is fulfilled when ${\displaystyle \frac{{\omega }_x}{{\omega }_y}}>1$.

4. The Modified Radzimovsky Formulas

4.1. Case x and y as Driving Links and z Driven Link

Let us consider the case of a two dof gear train with x and y as driving links (i.e., $P_x=T_x{\omega }_x>0$, $P_y=T_y{\omega }_y>0$ and $P_z=T_z{\omega }_z<0$).

Case 1:$\mathrm{sign}\left({\displaystyle \frac{{\omega }_x}{{\omega }_y}}\right)=-1$ (see Figure 2, 1st working mode)

Under kinematic inversions that fix links x and y, respectively, assuming without loss of generality $P_x^y>0$, the following relations hold (see 1st working mode of Figure 2):

$$\begin{array}{cc}\hfill T_x{\omega }_{xy}{\eta }_{y(x-z)}+T_z{\omega }_{zy}& =0\hfill \end{array}$$

(9a)

$$\begin{array}{cc}\hfill T_y{\omega }_{yx}{\eta }_{x(y-z)}+T_z{\omega }_{zx}& =0\hfill \end{array}$$

(9b)

The overall power balance condition is as follows:

$${\eta }_{(x,y-z)}={\displaystyle \frac{\left|T_z{\omega }_z\right|}{T_x{\omega }_x+T_y{\omega }_y}}$$

(9c)

Solving the system composed of the Equations (9), one obtains the following:

$${\eta }_{(x,y-z)}=\left|{\displaystyle \frac{{\omega }_z{\omega }_{yx}{\eta }_{y(x-z)}}{{\omega }_x{\omega }_{zy}-{\displaystyle \frac{{\eta }_{y(x-z)}}{{\eta }_{x(y-z)}}}{\omega }_y{\omega }_{zx}}}\right|$$

(10)

Subcase 2a: ${\displaystyle \frac{{\omega }_y}{{\omega }_x}}<1$ or $\mathrm{sgn}\left({\displaystyle \frac{{\omega }_x}{{\omega }_y}}\right)=+1$ (see Figure 3, 1st working mode)

Under kinematic inversions that fix links x and y, respectively, assuming without loss of generality $P_x^y>0$, the following relations hold:

$$\begin{array}{cc}\hfill T_x{\omega }_{xy}{\eta }_{y(x-z)}+T_z{\omega }_{zy}& =0\hfill \end{array}$$

(11a)

$$\begin{array}{cc}\hfill T_y{\omega }_{yx}+T_z{\omega }_{zx}{\eta }_{x(z-y)}& =0\hfill \end{array}$$

(11b)

Solving the system composed of the Equations (9c) and (11), one obtains the following:

$${\eta }_{(x,y-z)}=\left|{\displaystyle \frac{{\omega }_z{\omega }_{yx}{\eta }_{y(x-z)}}{{\omega }_x{\omega }_{zy}-{\eta }_{x(z-y)}{\eta }_{y(x-z)}{\omega }_y{\omega }_{zx}}}\right|$$

(12)

The analytical Expression (10) can be combined into the following formula:

$$\begin{array}{|c|}\hline {\eta }_{(x,y-z)}=\left|{\displaystyle \frac{{\omega }_z{\omega }_{yx}{\eta }_{y(x-z)}}{{\omega }_x{\omega }_{zy}-A{\omega }_y{\omega }_{zx}}}\right|\\ \hline\end{array}$$

(13)

with

$$A=\left\{\begin{array}{cc}{\displaystyle \frac{{\eta }_{y(x-z)}}{{\eta }_{x(y-z)}}}\hfill & \mathrm{when}\mathrm{sign}\left({\displaystyle \frac{{\omega }_x}{{\omega }_y}}\right)=-1\hfill \\ {\eta }_{x(z-y)}{\eta }_{y(x-z)}\hfill & \mathrm{when}\left({\displaystyle \frac{{\omega }_y}{{\omega }_x}}\right)<1\mathrm{and}\mathrm{sign}\left({\displaystyle \frac{{\omega }_x}{{\omega }_y}}\right)=+1\hfill \end{array}\right.$$

(14)

The proposed formula is more general than the one proposed by Radzimovsky (see Equation (11) of [26]).

When ${\omega }_x={\omega }_y={\omega }_z$, the Equations (9b) and (11) are identically satisfied and Formula (13) is not valid. However, under these kinematic conditions, there is no meshing power loss. Under our hypotheses, the mechanical efficiency is equal to one.

4.2. Case x and y as Driven Links and z Driving Link

Let us consider the case of a two dof gear train with x and y as driven links (i.e., $P_x=T_x{\omega }_x<0$, $P_y=T_y{\omega }_y<0$ and $P_z=T_z{\omega }_z>0$), as shown in Figure 2, 2nd working mode. In this case the overall power balance equation is the following:

$${\eta }_{(z-x,y)}={\displaystyle \frac{\left|T_x{\omega }_x+T_y{\omega }_y\right|}{T_z{\omega }_z}}$$

(15)

Case 1:$\mathrm{sign}\left({\displaystyle \frac{{\omega }_x}{{\omega }_y}}\right)=-1$ (see Figure 2, 2nd working mode)

The following relations hold:

$$\begin{array}{cc}\hfill T_z{\omega }_{zy}{\eta }_{y(z-x)}+T_x{\omega }_{xy}& =0\hfill \end{array}$$

(16a)

$$\begin{array}{cc}\hfill T_z{\omega }_{zx}{\eta }_{x(z-y)}+T_y{\omega }_{yx}& =0\hfill \end{array}$$

(16b)

Solving the system composed of the Equations (15) and (16), one obtains the following:

$${\eta }_{(z-x,y)}=\left|{\displaystyle \frac{{\eta }_{x(z-y)}{\omega }_y{\omega }_{zx}-{\eta }_{y(z-x)}{\omega }_x{\omega }_{zy}}{{\omega }_{yx}{\omega }_z}}\right|$$

(17)

Subcase 2a: ${\displaystyle \frac{{\omega }_y}{{\omega }_x}}<1$ and $\mathrm{sgn}\left({\displaystyle \frac{{\omega }_x}{{\omega }_y}}\right)=+1$ (see Figure 3, 2nd working mode)

The following relations hold:

$$\begin{array}{cc}\hfill T_z{\omega }_{zy}{\eta }_{y(z-x)}+T_x{\omega }_{xy}& =0\hfill \end{array}$$

(18a)

$$\begin{array}{cc}\hfill T_z{\omega }_{zx}+T_y{\omega }_{yx}{\eta }_{x(y-z)}& =0\hfill \end{array}$$

(18b)

Solving the system composed of the Equations (15) and (18), one obtains the following:

$${\eta }_{(z-x,y)}=\left|{\displaystyle \frac{{\omega }_y{\omega }_{zx}-{\eta }_{x(y-z)}{\eta }_{y(z-x)}{\omega }_x{\omega }_{zy}}{{\eta }_{x(y-z)}{\omega }_{yx}{\omega }_z}}\right|$$

(19)

The analytical Expressions (18) and (19) can be combined into the following formula:

$$\begin{array}{|c|}\hline {\eta }_{(z-x,y)}=\left|{\displaystyle \frac{B{\omega }_y{\omega }_{zx}-{\eta }_{y-(z-x)}{\omega }_x{\omega }_{zy}}{{\omega }_{yx}{\omega }_z}}\right|\\ \hline\end{array}$$

(20)

with

$$B=\left\{\begin{array}{cc}{\eta }_{x(z-y)}\hfill & \mathrm{when}\mathrm{sign}\left({\displaystyle \frac{{\omega }_x}{{\omega }_y}}\right)=-1\hfill \\ {\displaystyle \frac{1}{{\eta }_{x(y-z)}}}\hfill & \mathrm{when}\left({\displaystyle \frac{{\omega }_y}{{\omega }_x}}\right)<1\mathrm{and}\mathrm{sign}\left({\displaystyle \frac{{\omega }_x}{{\omega }_y}}\right)=+1\hfill \end{array}\right.$$

(21)

The proposed formula is more general than the one proposed by Radzimovsky (see Equation (25) of [26]).

The expressions of ${\eta }_{x(y-z)}$ and ${\eta }_{y(x-z)}$, for all possible combinations of x, y and z, are listed in Table 1 of reference [1] (see also Appendix A).

5. Numerical Example

We consider the case of the gear unit, shown in Figure 5, with x and y driving links and the z-driven link. In particular, ${\omega }_x=8000$ rpm and ${\omega }_y=7966.6$ rpm.

For a planet gear ratio $N_{j,i}=-1.5$,

$${\tau }_1=-{\displaystyle \frac{1}{1-N_{j,i}}}=-0.4$$

and

$${\tau }_2=-{\displaystyle \frac{N_{j,i}}{N_{j,i}-1}}=-0.6$$

Consequently, from Willis’ equation, follows ${\omega }_z=7980$ rpm.

Since $0<{\displaystyle \frac{{\omega }_x}{{\omega }_y}}<1$, the Formula (13) is applied. Considering the current value of $N_{j,i}$, and assuming

$${\eta }_{z(x-y)}=0.900$$

the entries 3a and 5a of

Table A1. Efficiencies of single epicyclic spur-gear trains. $R=N_{j,i}$: planet gear ratio.

Case		Driving	Driven	Fixed	$\mathit{\eta }$
1		i	j	k	${\eta }_{k(i-j)}$
2		j	i	k	${\eta }_{k(j-i)}$
3a	$(R<0)$ $(R>1)$	i	k	j	$\frac{{\displaystyle R{\eta }_{k(i-j)}-1}}{{\displaystyle R-1}}$
3b	$(0<R<1)$	i	k	j	$\frac{{\displaystyle R-{\eta }_{k(j-i)}}}{{\displaystyle {\eta }_{k(j-i)}\left(R-1\right)}}$
4a	$(R<0)$ $(R>1)$	k	i	j	$\frac{{\displaystyle \left(R-1\right){\eta }_{k(j-i)}}}{{\displaystyle R-{\eta }_{k(j-i)}}}$
4b	$(0<R<1)$	k	i	j	$\frac{{\displaystyle R-1}}{{\displaystyle R{\eta }_{k(i-j)}-1}}$
5a	$(R<1)$	j	k	i	$\frac{{\displaystyle R-{\eta }_{k(i-j)}}}{{\displaystyle R-1}}$
5b	$(R>1)$	j	k	i	$\frac{{\displaystyle 1-R{\eta }_{k(i-j)}}}{{\displaystyle {\eta }_{k(i-j)}\left(1-R\right)}}$
6a	$(R<1)$	k	j	i	$\frac{{\displaystyle \left(R-1\right){\eta }_{k(i-j)}}}{{\displaystyle R{\eta }_{k(i-j)}-1}}$
6b	$(R>1)$	k	j	i	$\frac{{\displaystyle R-1}}{{\displaystyle R-{\eta }_{k(j-i)}}}$

 of the Appendix A give, respectively, the following:

$$\begin{array}{cc}\hfill {\eta }_{y(x-z)}& ={\displaystyle \frac{N_{j,i}{\eta }_{z(x-y)}-1}{N_{j,i}-1}}=0.940\hfill \\ \hfill {\eta }_{x(y-z)}& ={\displaystyle \frac{N_{j,i}-{\eta }_{z(x-y)}}{N_{j,i}-1}}=0.960\hfill \end{array}$$

For the prescribed numerical data, the Formula (13) yields the following:

$${\eta }_{(x,y-z)}=\left|{\displaystyle \frac{{\omega }_z{\omega }_{yx}{\eta }_{y(x-z)}}{{\omega }_x{\omega }_{zy}-{\displaystyle \frac{{\eta }_{y(x-z)}}{{\eta }_{x(y-z)}}}{\omega }_y{\omega }_{zx}}}\right|=0.998$$

6. Conclusions

In this paper, the first novel result concerns the algebraic signs analysis of a virtual power-flow in a two dof PGU. It was shown that in a PGU, the virtual or potential powers, $P_x^y$ and $P_y^x$, cannot have always the same algebraic sign. On the basis of this observation, new formulas for the computation of mechanical efficiency in a two dof PGU were deduced. The proposed formulas maintain their range of validity, also for cases not covered by the traditional Radzimovsky’s formulas. Therefore, our approach offers a more general and compact alternative. The results herein can be extended also to other types of differential devices.

It must be observed that the current approach is limited to meshing losses only under a stationary working mode. Future work should embody in the formulas also the bearing losses. Moreover, the present treatment is based on stationary conditions; however, often, the efficiency under transient conditions is required. In this case, the time variation of kinetic energy due to inertia forces needs to be included in the power balance.