Computational Method to Optimize Design of Gripping Part of Products via Grasping Motion Simulation to Maximize Gripping Comfort
Abstract
1. Introduction
2. Materials and Methods
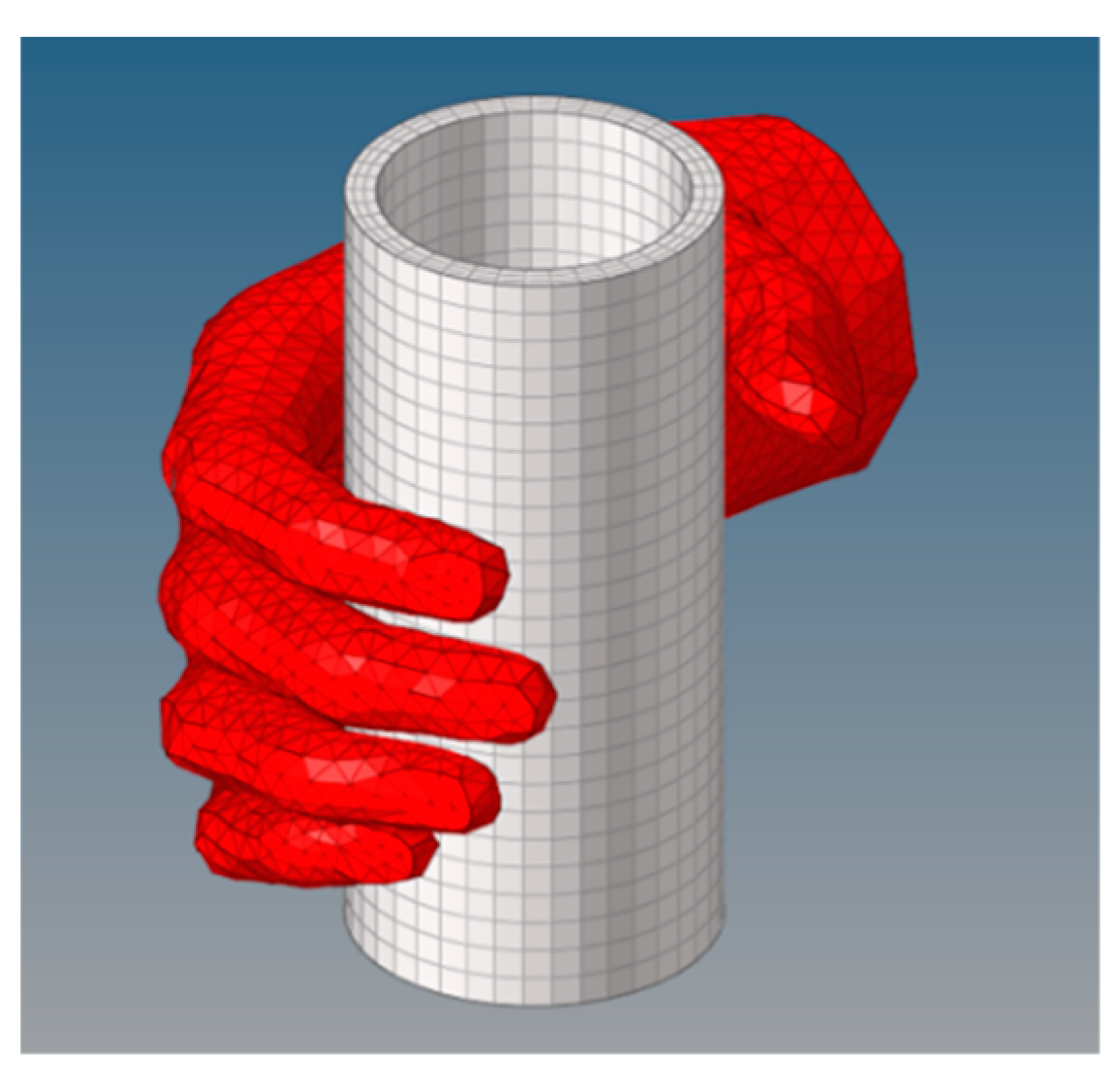
2.1. Establishment of Grasping Motion Simulation
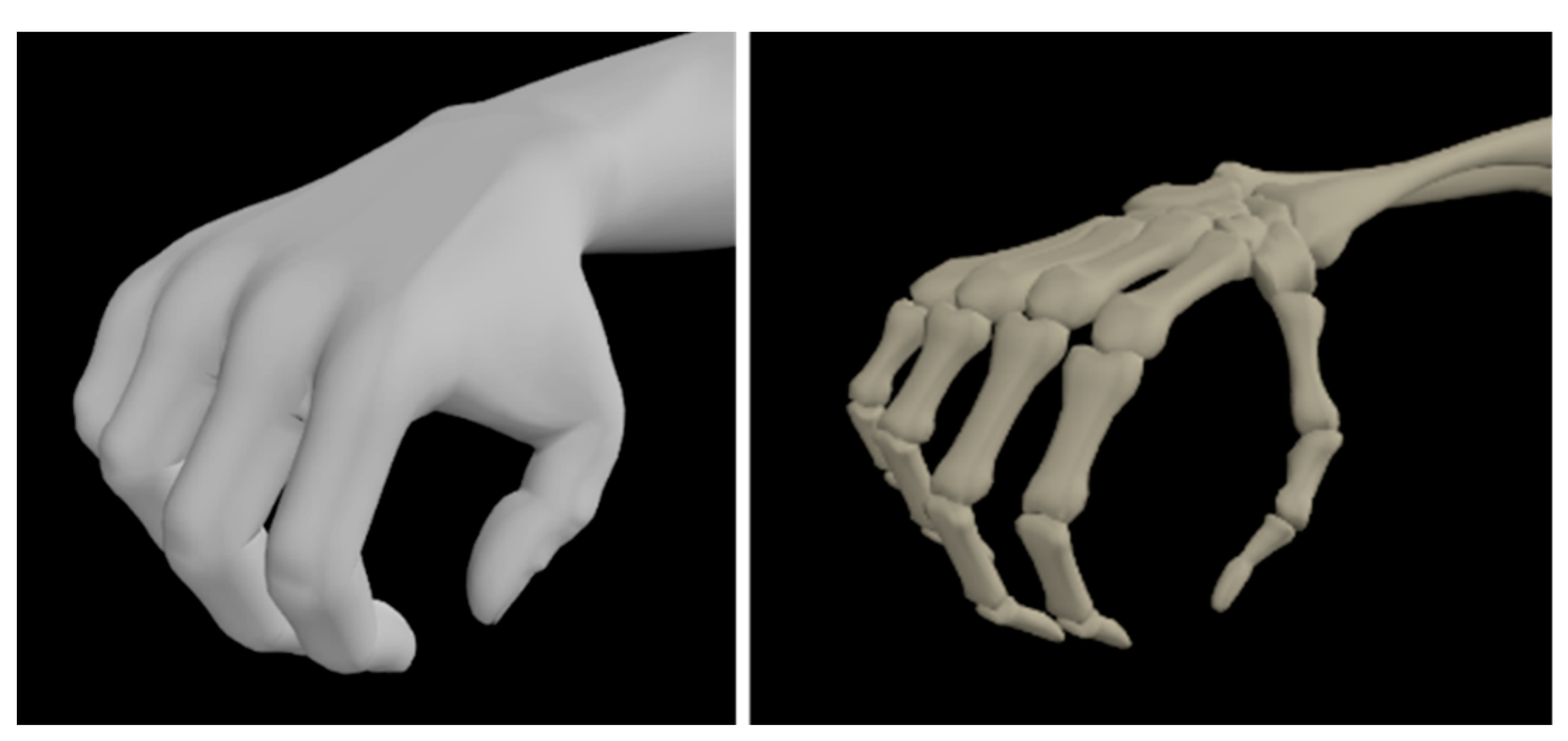
2.1.1. Construction of Finite Element Model
2.1.2. Material Properties
2.1.3. Finger Joints
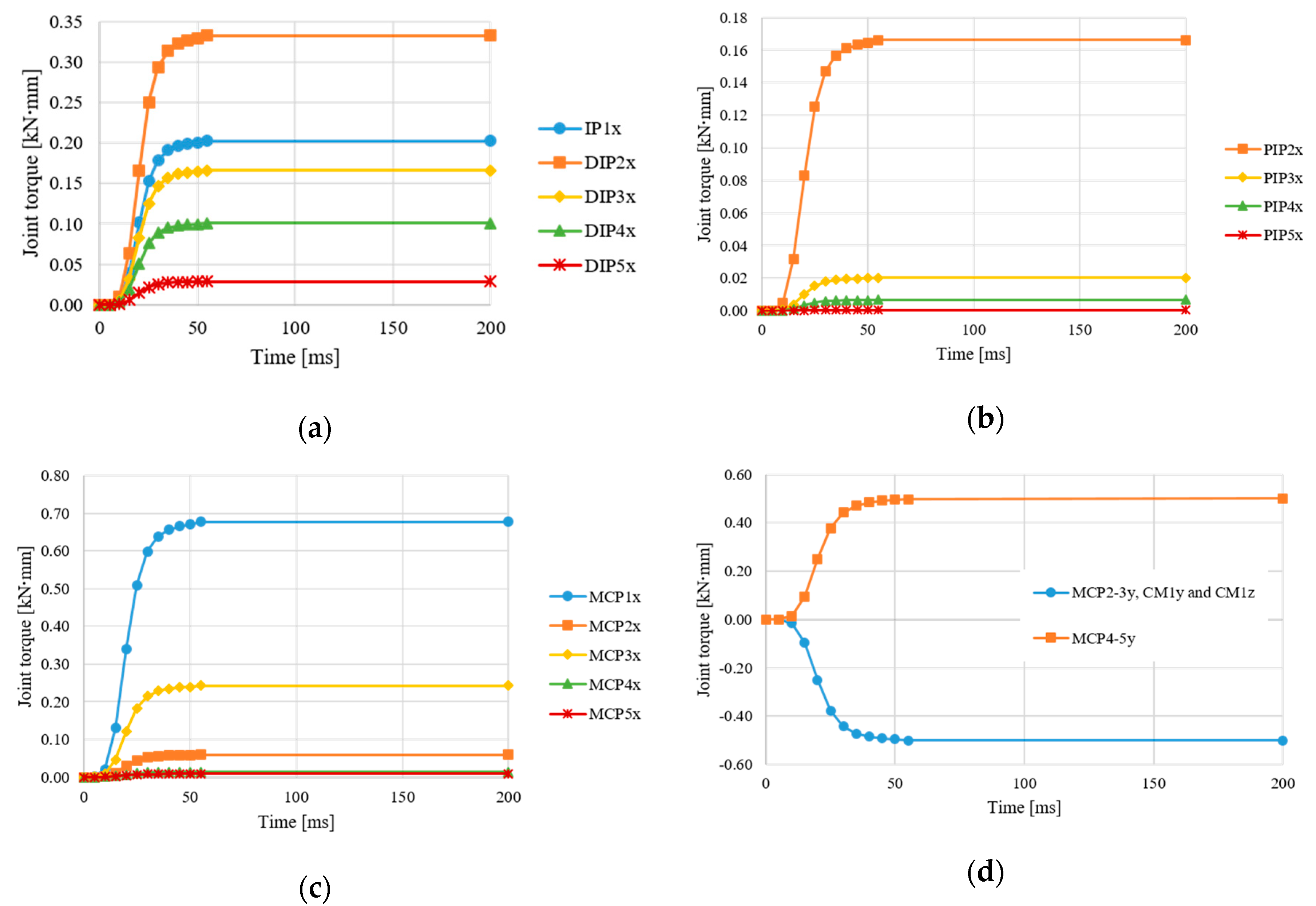
2.1.4. Calculation of Joint Torques
2.2. Validation of the Grasping Motion Simulation
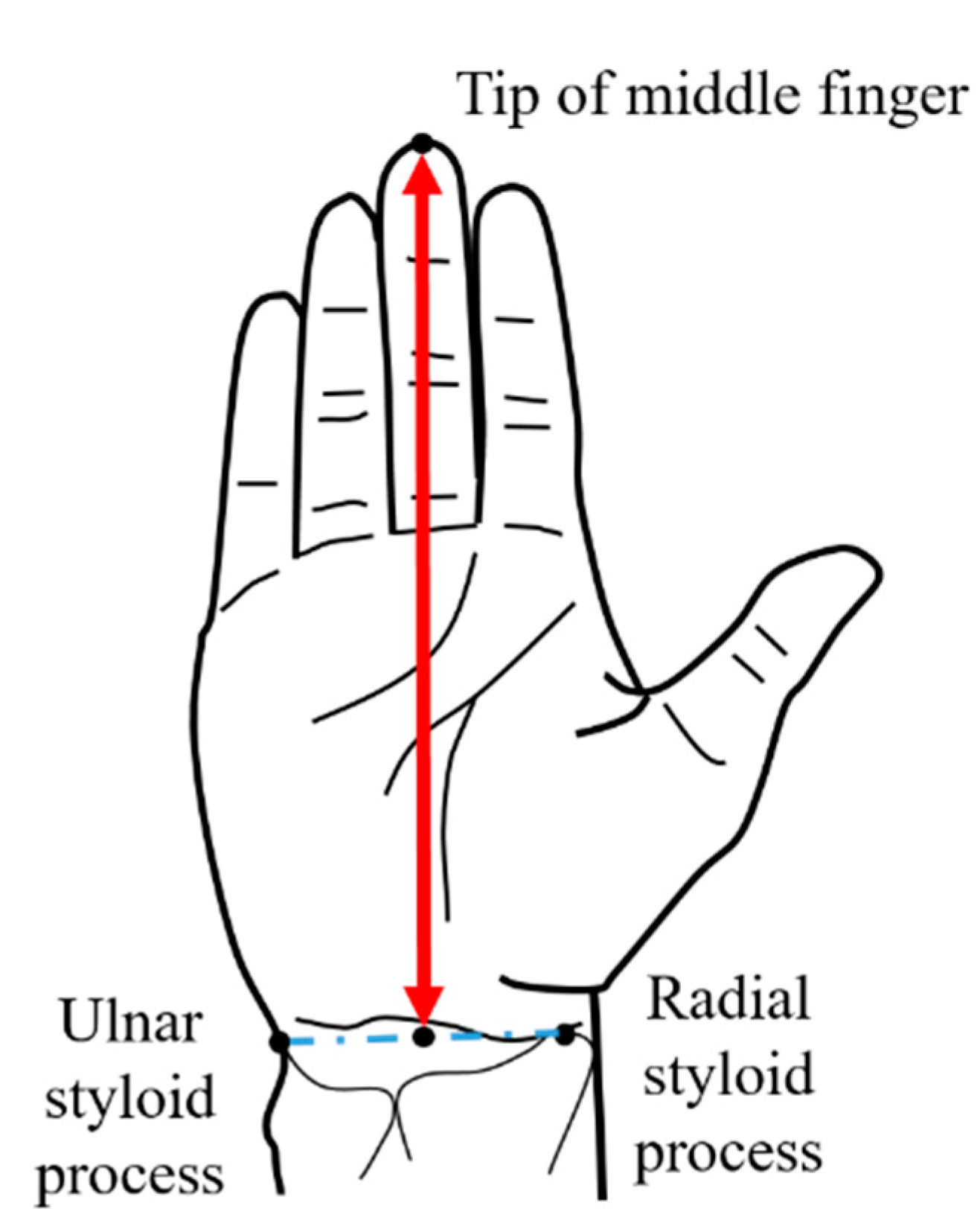
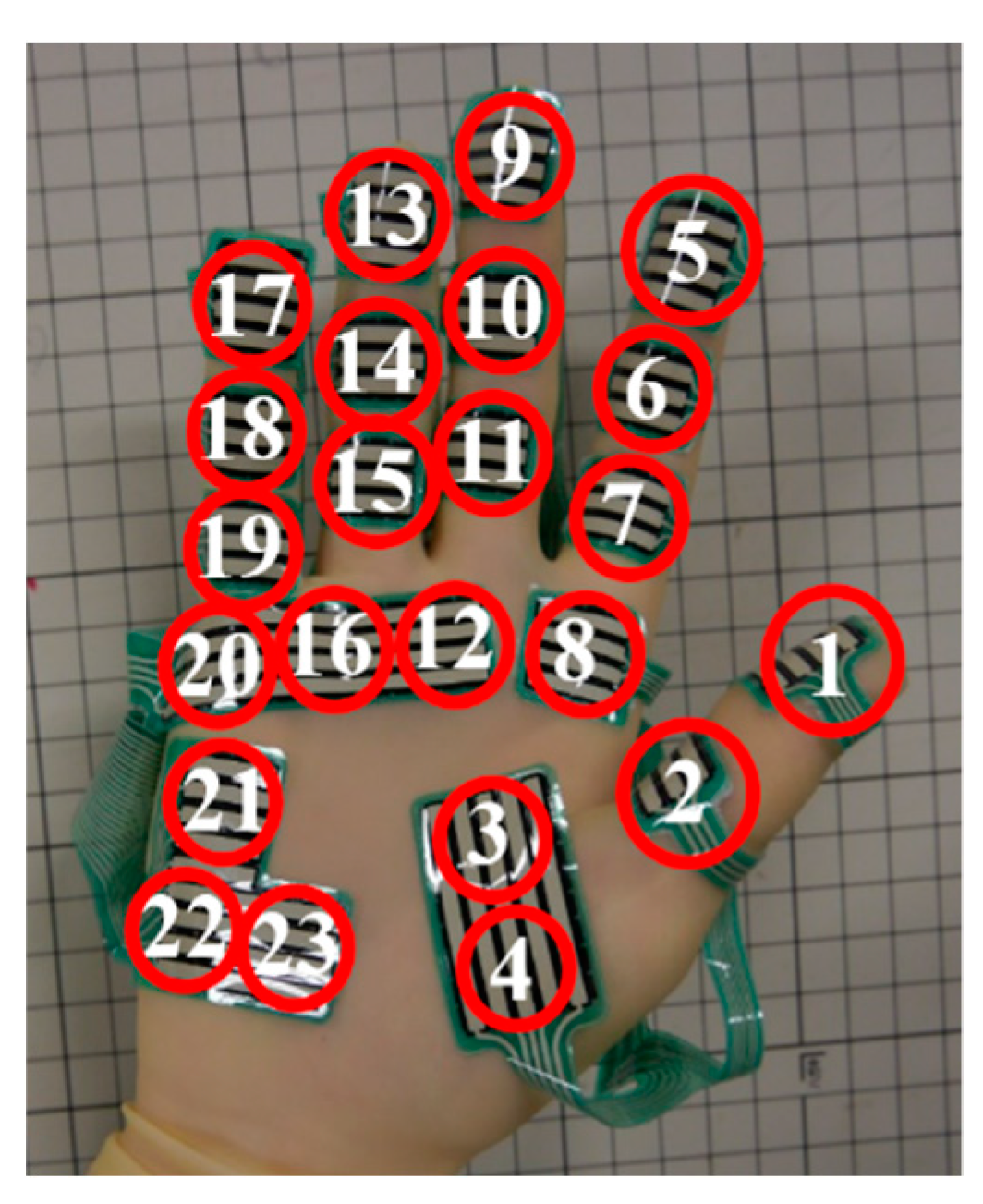
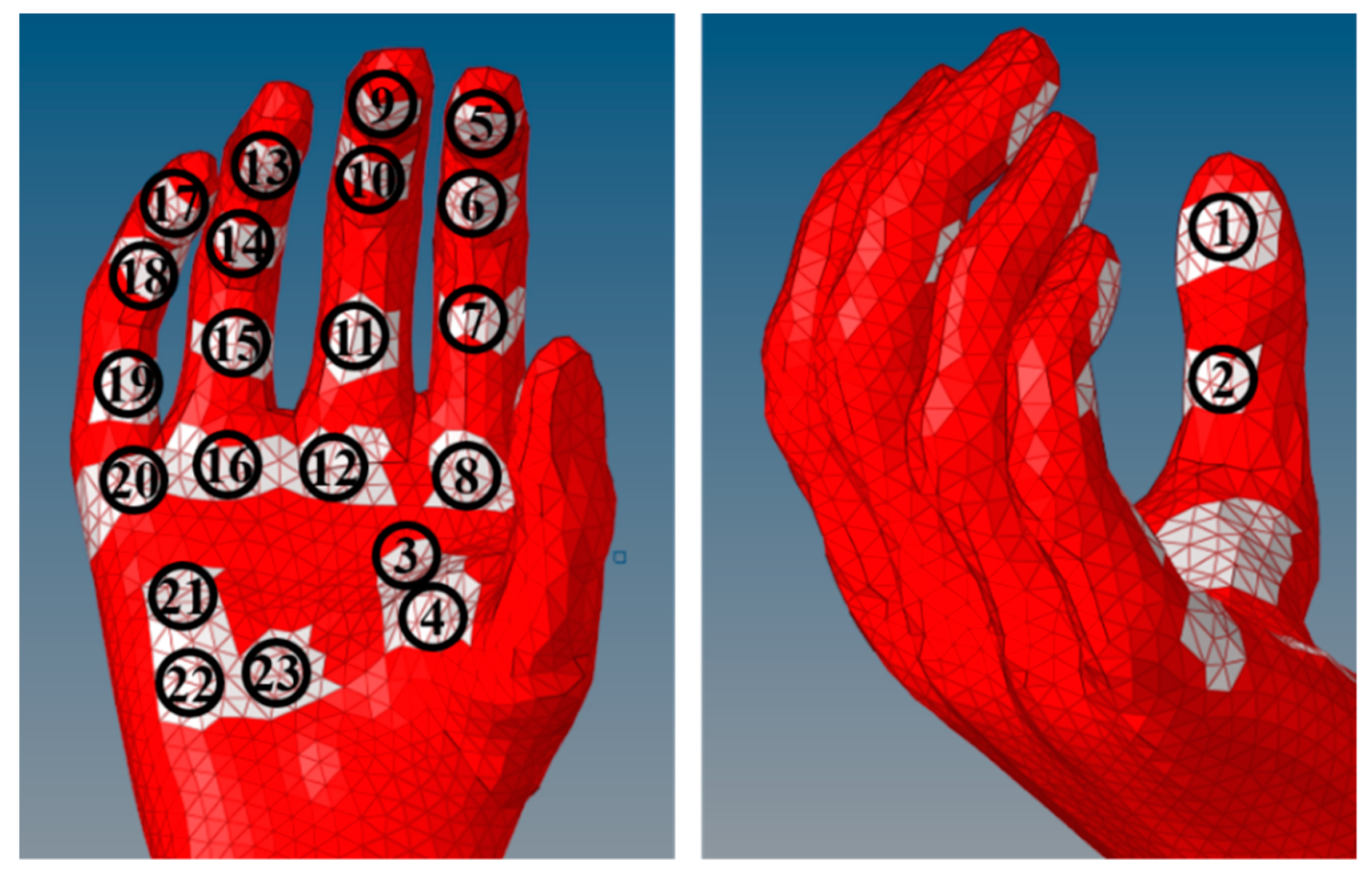
2.2.1. Evaluation Method
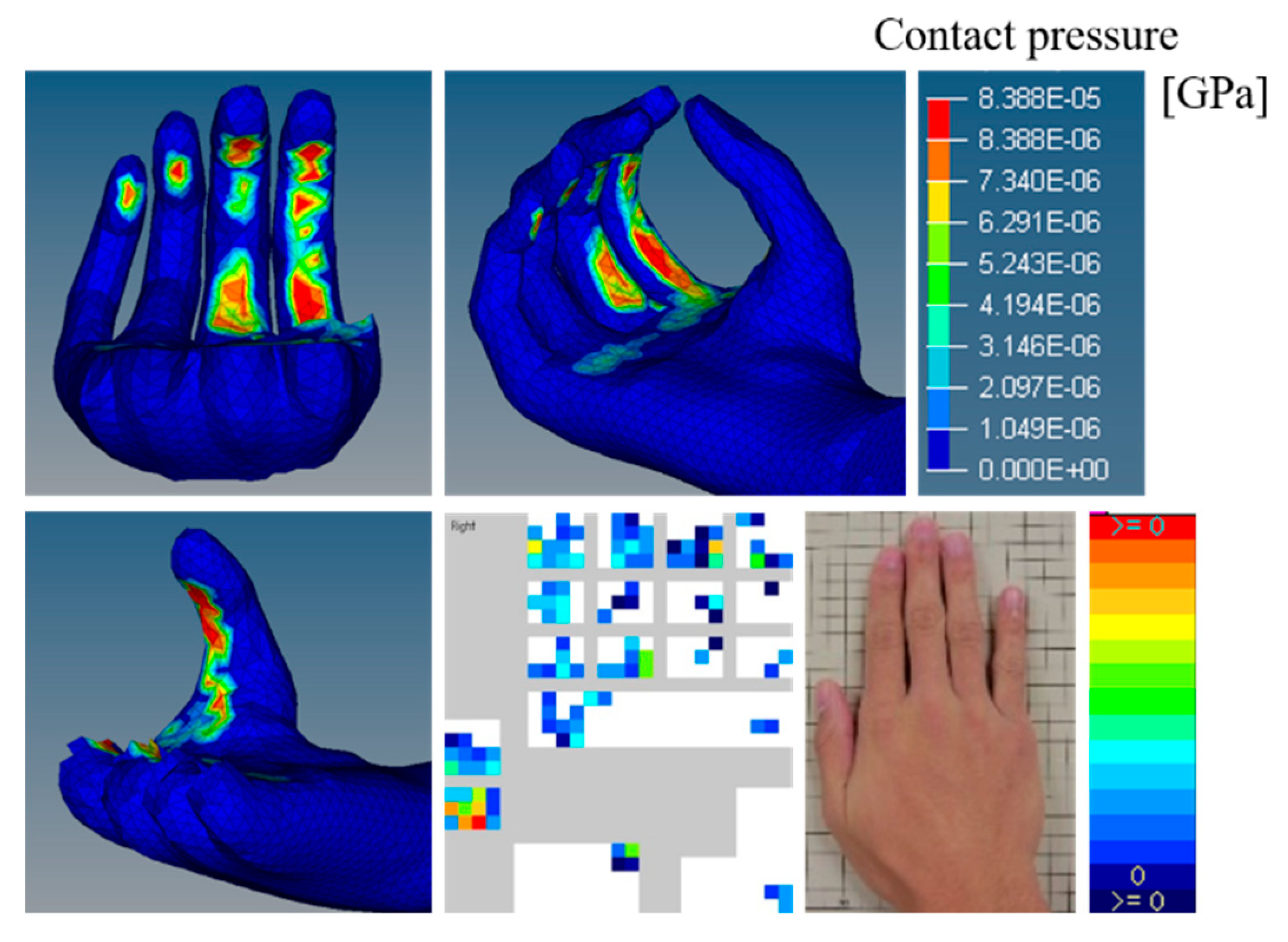
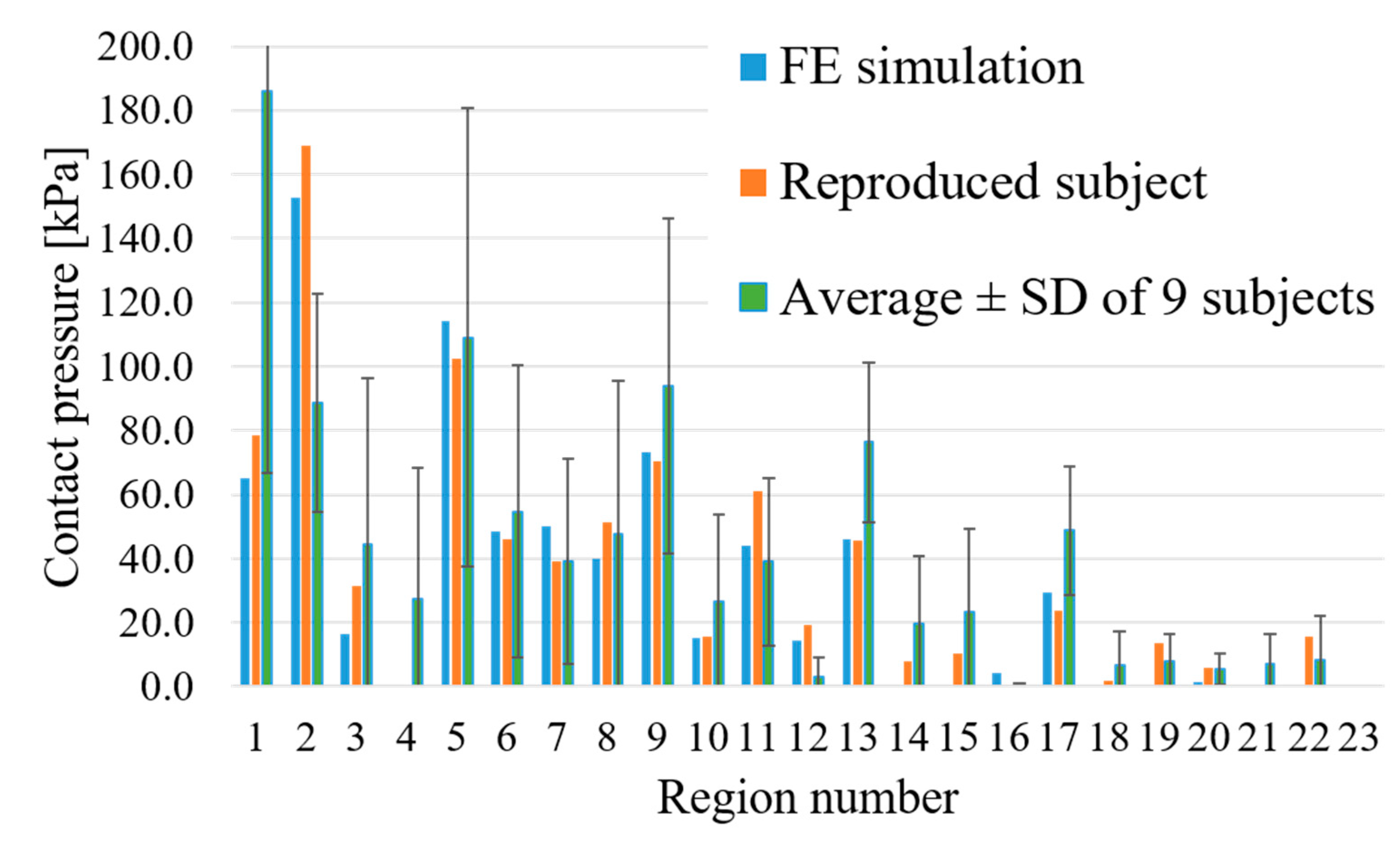
2.2.2. Evaluation Result of the Grasping Motion Simulation
2.3. Gripping Part Design Using Topology Optimization Method
2.3.1. Topology Optimization
2.3.2. Optimization Condition
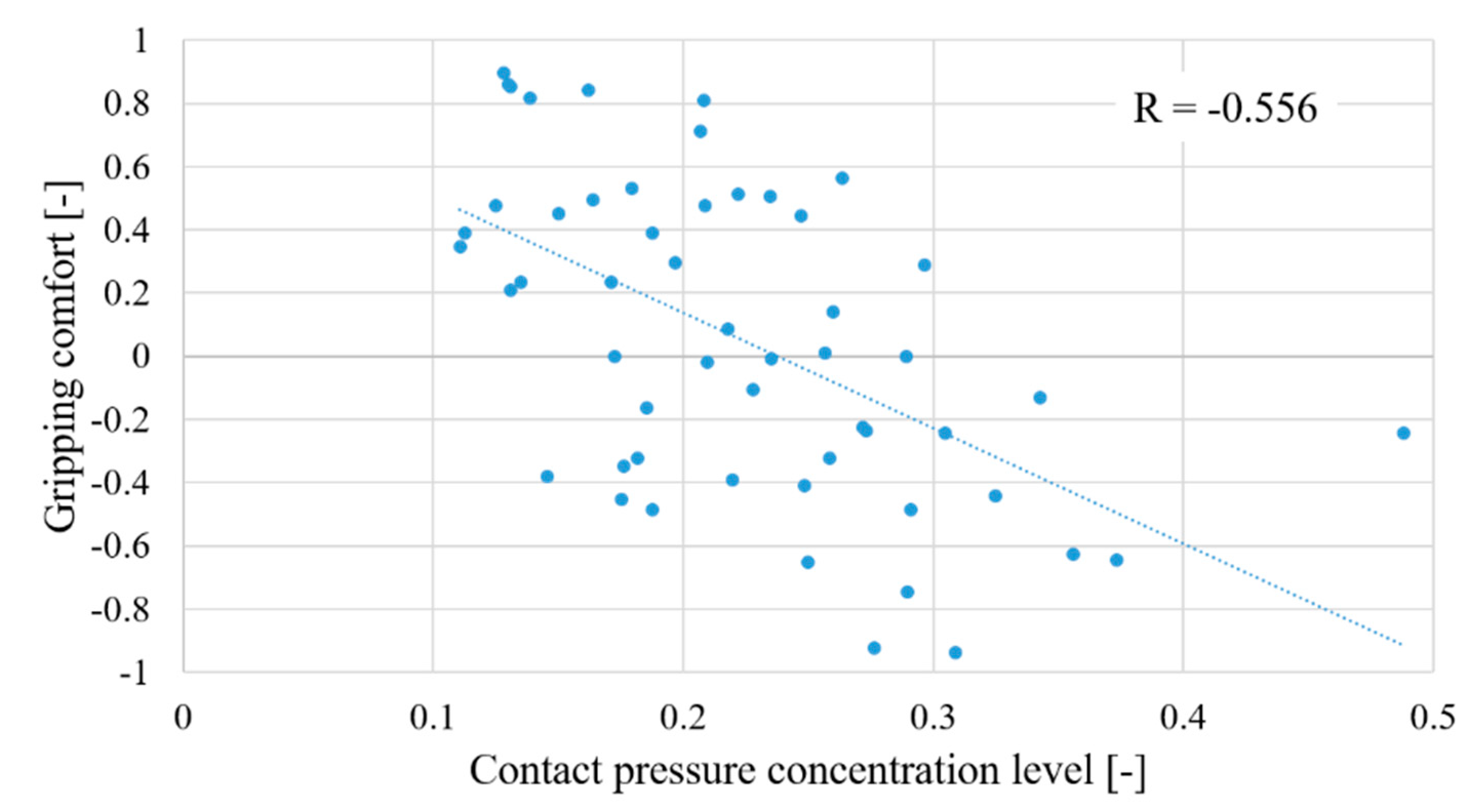
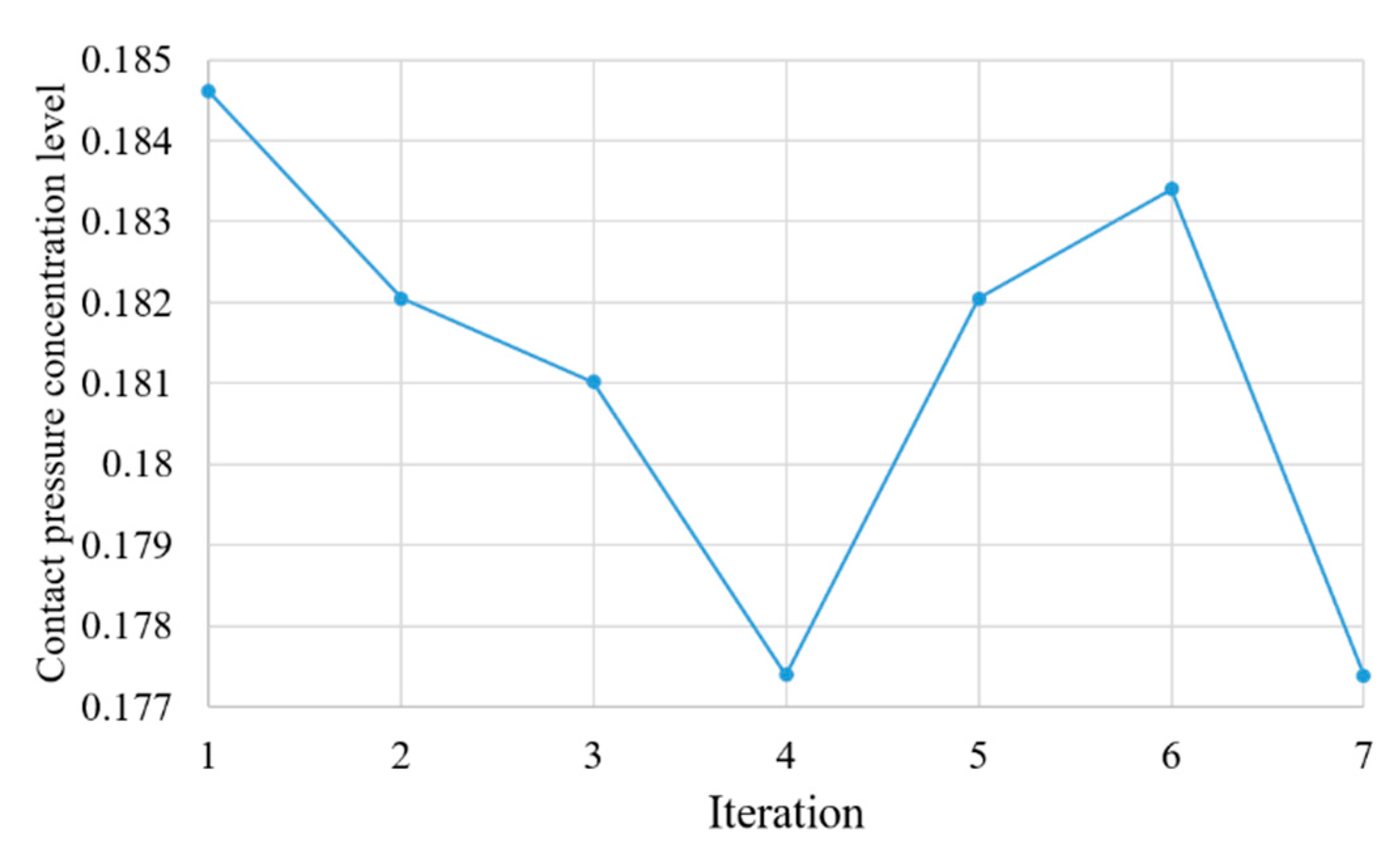
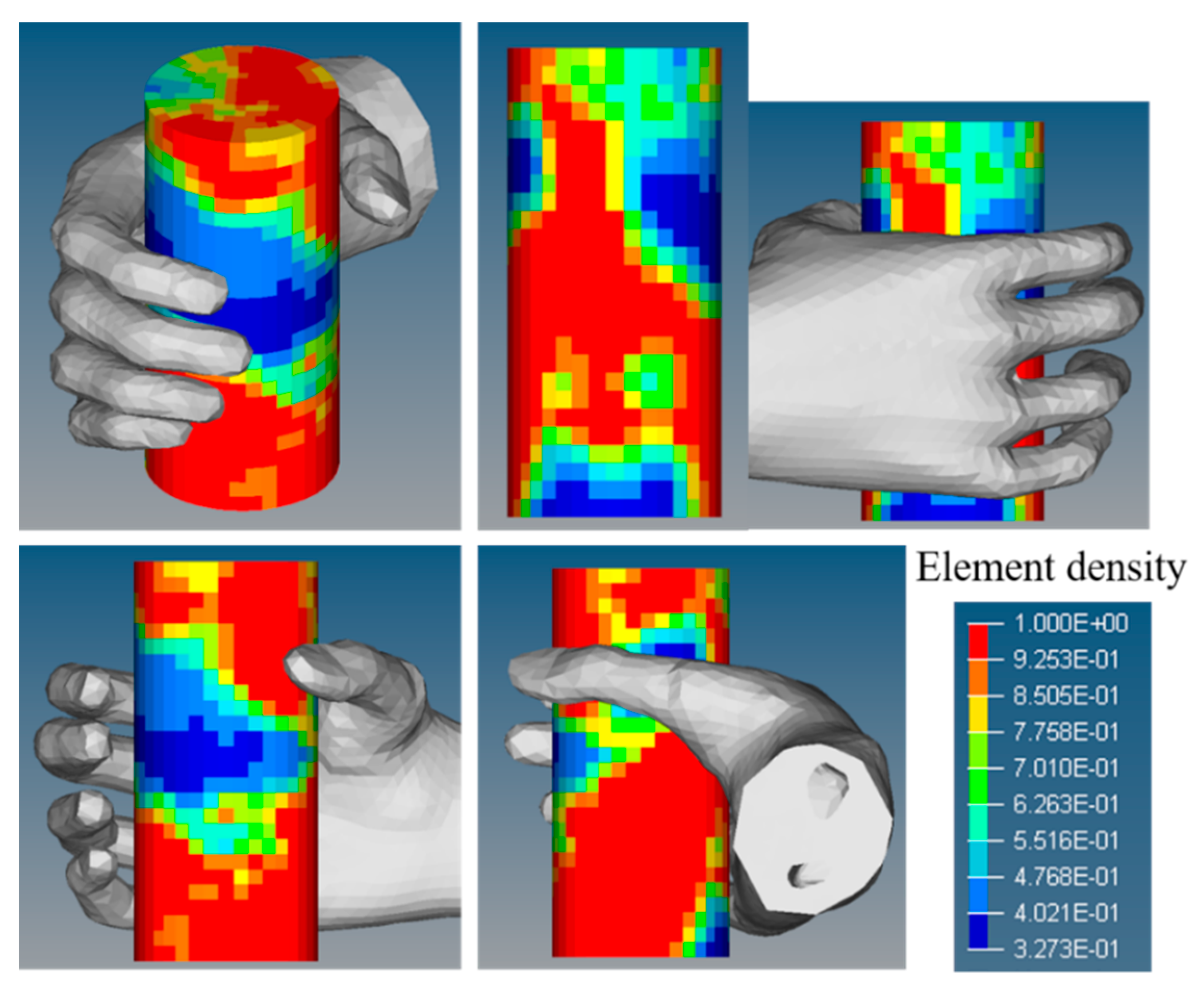
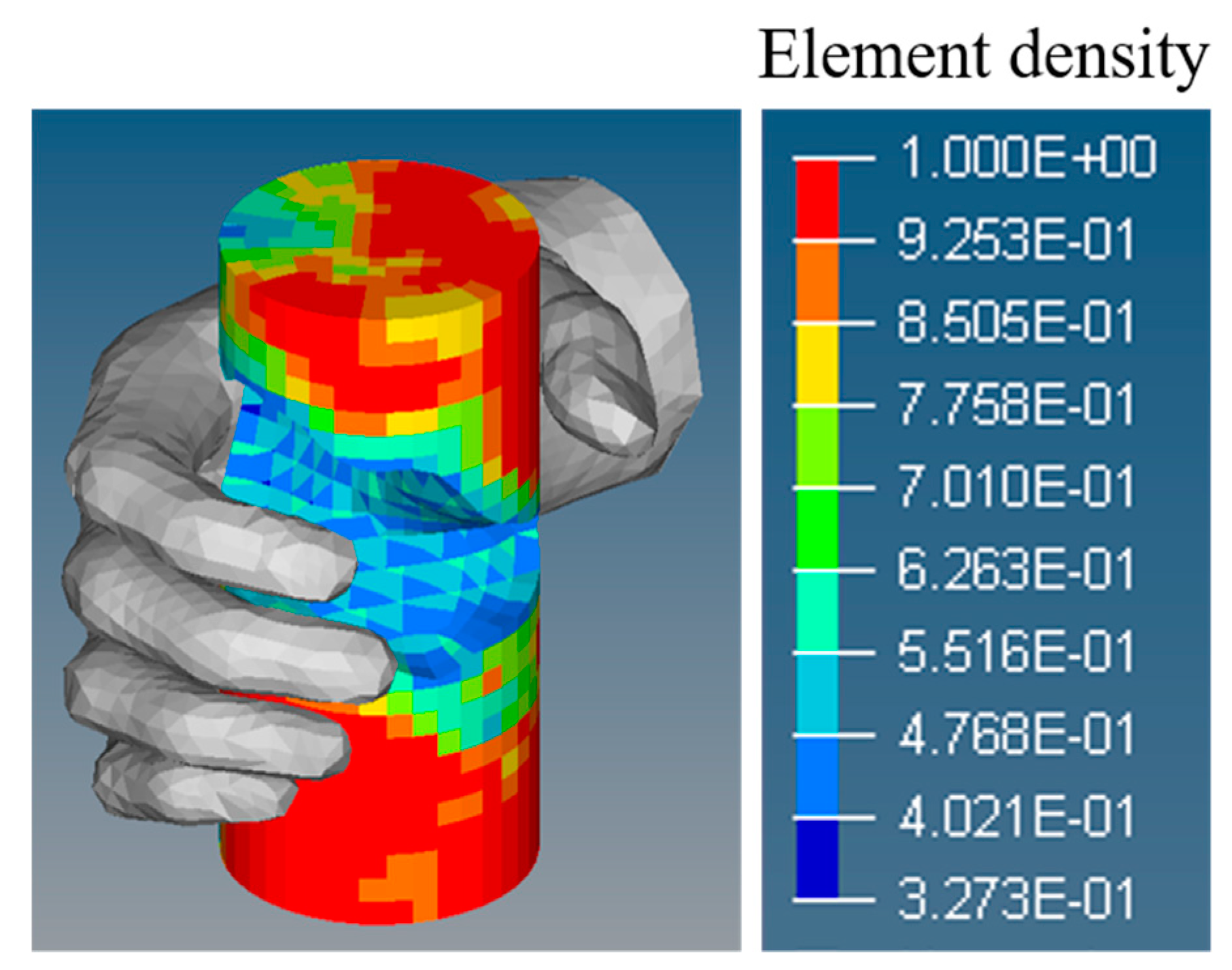
3. Results and Discussion
4. Conclusions
Author Contributions
Funding
Conflicts of Interest
References
- Nagamachi, M. Kansei Engineering: A new ergonomic consumer-oriented technology for product development. Int. J. Ind. Ergon. 1995, 15, 3–11. [Google Scholar] [CrossRef]
- Kuijt-Evers, L.F.M.; Groenesteijn, L.; de Looze, M.P.; Vink, P. Identifying factors of comfort in using hand tools. Appl. Ergon. 2004, 35, 453–458. [Google Scholar] [CrossRef] [PubMed]
- Kuijt-Evers, L.F.M.; Bosch, T.; Huysmans, M.A.; de Looze, M.P.; Vink, P. Association between objective and subjective measurements of comfort and discomfort in hand tools. Appl. Ergon. 2007, 38, 643–654. [Google Scholar] [CrossRef] [PubMed]
- Lin, J.H.; McGorry, R.W. Predicting subjective perceptions of powered tool torque reactions. Appl. Ergon. 2009, 40, 47–55. [Google Scholar] [CrossRef]
- Kong, Y.K.; Kim, D.M.; Lee, K.S.; Jung, M.C. Comparison of comfort, discomfort, and continuum ratings of force levels and hand regions during gripping exertions. Appl. Ergon. 2012, 43, 283–289. [Google Scholar] [CrossRef]
- Hokari, K.; Pramudita, J.A.; Ito, M.; Noda, S.; Tanabe, Y. The relationships of gripping comfort to contact pressure and hand posture during gripping. Int. J. Ind. Ergon. 2019, 70, 84–91. [Google Scholar] [CrossRef]
- Coelho, R.M.; Alves de Sousa, R.J.; Fernandes, F.A.O.; Teixeira-Dias, F. New composite liners for energy absorption purposes. Mater. Des. 2013, 43, 384–392. [Google Scholar] [CrossRef]
- Harih, G.; Dolšak, B. Recommendations for tool-handle material choice based on finite element analysis. Appl. Ergon. 2014, 45, 577–585. [Google Scholar] [CrossRef]
- Hokari, K.; Arimoto, R.; Pramudita, J.A.; Ito, M.; Noda, S.; Tanabe, Y. Palmar Contact Pressure Distribution During Grasping A Cylindrical Object: Parameter Study Using Hand Finite Element Model. Adv. Exp. Mech. 2019, 4, 135–140. [Google Scholar] [CrossRef]
- Miller, A.; Allen, P.; Santos, V.; Valero-Cuevas, F. From robotic hands to human hands: A visualization and simulation engine for grasping research. Ind. Robot 2005, 32, 55–63. [Google Scholar] [CrossRef]
- Miyata, N.; Shimizu, Y.; Motoki, Y.; Maeda, Y.; Mochimaru, M. Hand MoCap using an individual model with a skeleton and a surface skin. Int. J. Hum. Factors Model. Simul. 2012, 3, 147–168. [Google Scholar] [CrossRef]
- Sancho-Bru, J.L.; Mora, M.C.; León, B.E.; Pérez-González, A.; Iserte, J.L.; Morales, A. Grasp modelling with a biomechanical model of the hand. Comput. Methods Biomech. Biomed. Eng. 2014, 17, 297–310. [Google Scholar] [CrossRef]
- Chamoret, D.; Bodo, M.; Roth, S. A first step in finite element simulation of a grasping task. Comput. Assist. Surg. 2016, 21 (Suppl. 1), 22–29. [Google Scholar] [CrossRef]
- Harih, G.; Tada, M. (Eds) Grasping Simulations Using Finite Element Digital Human Hand Model. In Proceedings of the 20th Congress of the International Ergonomics Association (IEA 2018), Florence, Italy, 26–30 August 2018; pp. 117–127. [Google Scholar] [CrossRef]
- Sancho-Bru, J.L.; Giurintano, D.J.; Pérez-González, A.; Vergara, M. Optimum Tool Handle Diameter for a Cylinder Grip. J. Hand Ther. 2003, 16, 337–342. [Google Scholar] [CrossRef]
- Eksioglu, M. Relative optimum grip span as a function of hand anthropometry. Int. J. Ind. Ergon. 2004, 34, 1–12. [Google Scholar] [CrossRef]
- Garneau, C.J.; Parkinson, M.B. Optimization of Tool Handle Shape for a Target User Population. In Proceedings of the ASME 2009 International Design Engineering Technical Conferences & Computers and Information in Engineering Conference, San Diego, CA, USA, 30 August–2 September 2009; pp. 1029–1036. [Google Scholar] [CrossRef]
- Harih, G.; Dolšak, B. Tool-handle design based on a digital human hand model. Int. J. Ind. Ergon. 2013, 43, 288–295. [Google Scholar] [CrossRef]
- Maeno, T.; Kobayashi, K.; Yamazaki, N. Relationship between the Structure of Human Finger Tissue and the Location of Tactile Receptors. JSME Int. J. Ser. C 1998, 41, 94–100. [Google Scholar] [CrossRef]
- Kouchi, M. AIST Japanese Hand Dimensions Data. Available online: www.airc.aist.go.jp/dhrt/hand/index.html (accessed on 17 November 2016).
- Napier, J.R. The prehensile movements of the human hand. J. Bone Joint Surg. 1956, 38, 902–913. [Google Scholar] [CrossRef]
- Mason, C.R.; Gomez, J.E.; Ebner, T.J. Hand synergies during reach-to-grasp. J. Neurophysiol. 2001, 86, 2896–2910. [Google Scholar] [CrossRef]
- Aldien, Y.; Welcome, D.; Rakheja, S.; Dong, R.; Boileau, P.E. Contact pressure distribution at hand–handle interface: Role of hand forces and handle size. Int. J. Ind. Ergon. 2005, 35, 267–286. [Google Scholar] [CrossRef]
- Saga, N.; Tesen, S.; Dobashi, H.; Nagase, J. Design of a peristaltic crawling robot using 3-D link mechanisms. Int. J. Biomechatronics Biomed. Robot. 2013, 2, 111–117. [Google Scholar] [CrossRef]
- Pramudita, J.A.; Kamiya, S.; Ujihashi, S.; Choi, H.Y.; Ito, M.; Watanabe, R.; Crandall, J.R.; Kent, R.W. Estimation of conditions evoking fracture in finger bones under pinch loading based on finite element analysis. Comput. Methods Biomech. Biomed. Eng. 2017, 20, 35–44. [Google Scholar] [CrossRef] [PubMed]
- Ito, M. Construction of integrated head laceration prediction method in infant fall using the evaluation methods of protective reaction and skin laceration. Ph.D. Thesis, Tokyo Institute of Technology, Tokyo, Japan, 2017. [Google Scholar]
- Zhang, M.; Mak, A.F. In vivo friction properties of human skin. Prosthet. Orthot. Int. 1999, 23, 135–141. [Google Scholar] [CrossRef] [PubMed]
- Ramírez, J.F.; Pavón, J.J.; Toro, A. Experimental assessment of friction coefficient between polypropylene and human skin using instrumented sclerometer. Proc. Inst. Mech. Eng. Part J J. Eng. Tribol. 2014, 229, 259–265. [Google Scholar] [CrossRef]
- Wu, J.Z.; Welcome, D.E.; McDowell, T.W.; Xu, X.S.; Dong, R.G. Modeling of the interaction between grip force and vibration transmissibility of a finger. Med. Eng. Phys. 2017, 45, 61–70. [Google Scholar] [CrossRef]
- Dühring, M.B.; Jensen, J.S.; Sigmund, O. Acoustic design by topology optimization. J. Sound Vib. 2008, 317, 557–575. [Google Scholar] [CrossRef]
- Liu, J.; Ma, Y. A survey of manufacturing oriented topology optimization methods. Adv. Eng. Softw. 2016, 100, 161–175. [Google Scholar] [CrossRef]
- Mirzendehdel, A.M.; Suresh, K. Support structure constrained topology optimization for additive manufacturing. Comput Aided Des. 2016, 81, 1–13. [Google Scholar] [CrossRef]
- Radhakrishnan, S.; Nagaravindra, M. Analysis of hand forces in health and disease during maximum isometric grasping of cylinders. Med. Biol. Eng. Comput. 1993, 31, 372–376. [Google Scholar] [CrossRef]
- Goislard De Monsabert, B.; Rossi, J.; Berton, E.; Vigouroux, L. Quantification of hand and forearm muscle forces during a maximal power grip task. Med. Sci. Sports Exerc. 2012, 44, 1906–1916. [Google Scholar] [CrossRef]
- Rossi, J.; Berton, E.; Grélot, L.; Barla, C.; Vigouroux, L. Characterisation of forces exerted by the entire hand during the power grip: Effect of the handle diameter. Ergonomics 2012, 55, 682–692. [Google Scholar] [CrossRef] [PubMed]
- Kong, Y.K.; Lowe, B.D. Optimal cylindrical handle diameter for grip force tasks. Int. J. Ind. Ergon. 2005, 35, 495–507. [Google Scholar] [CrossRef]
- Vigouroux, L.; Rossi, J.; Foissac, M.; Grélot, L.; Berton, E. Finger force sharing during an adapted power grip task. Neurosci. Lett. 2011, 504, 290–294. [Google Scholar] [CrossRef] [PubMed]
- Nicholas, J.W.; Corvese, R.J.; Woolley, C.; Armstrong, T.J. Quantification of hand grasp force using a pressure mapping system. Work 2012, 41 (Suppl. 1), 605–612. [Google Scholar] [CrossRef]
- Kamakura, N.; Matsuo, M.; Ishii, H.; Mitsuboshi, F.; Miura, Y. Patterns of static prehension in normal hands. Am. J. Occup. Ther. 1890, 34, 437–445. [Google Scholar] [CrossRef]
- Vergara, M.; Sancho-Bru, J.L.; Gracia-Ibáñez, V.; Pérez-González, A. An introductory study of common grasps used by adults during performance of activities of daily living. J. Hand Ther. 2014, 27, 225–233. [Google Scholar] [CrossRef]
- Lee, K.S.; Jung, M.C. Three-dimensional finger joint angles by hand posture and object properties. Ergonomics 2016, 59, 890–900. [Google Scholar] [CrossRef]
- Harih, G.; Dolšak, B. Comparison of subjective comfort ratings between anatomically shaped and cylindrical handles. Appl. Ergon. 2014, 45, 943–954. [Google Scholar] [CrossRef]
- Yakou, T.; Yamamoto, K.; Koyama, M.; Hyodo, K. Sensory Evaluation of Grip Using Cylindrical Objects. JSME Int. J. Ser. C 1997, 40, 730–735. [Google Scholar] [CrossRef][Green Version]
- Lee, K.S.; Jung, M.C. Common patterns of voluntary grasp types according to object shape, size, and direction. Int. J. Ind. Ergon. 2014, 44, 761–768. [Google Scholar] [CrossRef]



| Component | Number | Element Type | |
|---|---|---|---|
| Elements | Nodes | ||
| Skin | 5362 | 2693 | Triangular shell |
| Subcutaneous soft tissue | 30,480 | 7979 | Tetrahedron solid |
| Bones | 13,633 | 4677 | Tetrahedron solid |
| Cylinder | 3198 | 4428 | Hexahedron solid |
| Density [kg/mm3] | Young’s Modulus [GPa] | Poisson’s Ratio | Literature | |
|---|---|---|---|---|
| Skin | 0.90 × 10−6 | 8.00 × 10−5 | 0.48 | [19,25] |
| Subcutaneous soft tissue | 0.90 × 10−6 | - | 0.48 | [25] |
| Bones | 1.80 × 10−6 | - | - | [25] |
| Cylinder | 1.04 × 10−6 | 2.00 | 0.39 | [24] |
| p | 1 | 2 | 3 | 4 |
|---|---|---|---|---|
| μp [GPa] | 15.7 | 9.32 | −15.7 | −9.32 |
| αp | −1.21 | 9.34 | −1.21 | 9.34 |
| Element Size | Number of | Average Differences of Contact Pressures | Calculation Time [h] | |||
|---|---|---|---|---|---|---|
| Skin | Subcutaneous Soft Tissue | |||||
| Elements | Nodes | Elements | Nodes | |||
| 4 mm | 7918 | 3971 | 38,854 | 10,070 | 7.8% against 5 mm | 7.9 |
| 5 mm | 5362 | 2693 | 30,480 | 7979 | 26% against 6 mm | 4.2 |
| 6 mm | 4112 | 2081 | 25,327 | 6820 | - | 3.3 |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Hokari, K.; Pramudita, J.A.; Ito, M.; Okada, K.; Tanabe, Y. Computational Method to Optimize Design of Gripping Part of Products via Grasping Motion Simulation to Maximize Gripping Comfort. Appl. Sci. 2020, 10, 3265. https://doi.org/10.3390/app10093265
Hokari K, Pramudita JA, Ito M, Okada K, Tanabe Y. Computational Method to Optimize Design of Gripping Part of Products via Grasping Motion Simulation to Maximize Gripping Comfort. Applied Sciences. 2020; 10(9):3265. https://doi.org/10.3390/app10093265
Chicago/Turabian StyleHokari, Kazuki, Jonas A. Pramudita, Masato Ito, Kazuya Okada, and Yuji Tanabe. 2020. "Computational Method to Optimize Design of Gripping Part of Products via Grasping Motion Simulation to Maximize Gripping Comfort" Applied Sciences 10, no. 9: 3265. https://doi.org/10.3390/app10093265
APA StyleHokari, K., Pramudita, J. A., Ito, M., Okada, K., & Tanabe, Y. (2020). Computational Method to Optimize Design of Gripping Part of Products via Grasping Motion Simulation to Maximize Gripping Comfort. Applied Sciences, 10(9), 3265. https://doi.org/10.3390/app10093265





