A “Double Accuracy Theory” and Experimental Research on Precision Grinding
Abstract
1. Introduction
2. Theoretical Analysis of Grinding Machine Accuracy
3. Accuracy Theory of Precision Three-Coordinate Measuring Instrument
4. Double Accuracy Theoretical Analysis
5. Experimental Analysis
5.1. Experiment of Cylindrical Grinding
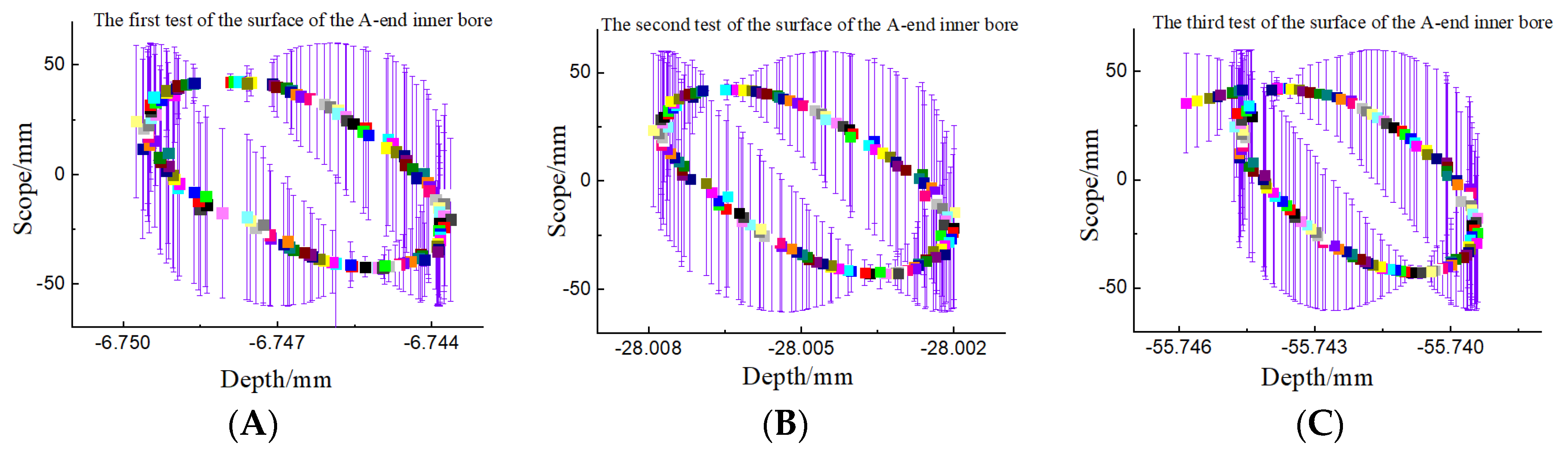
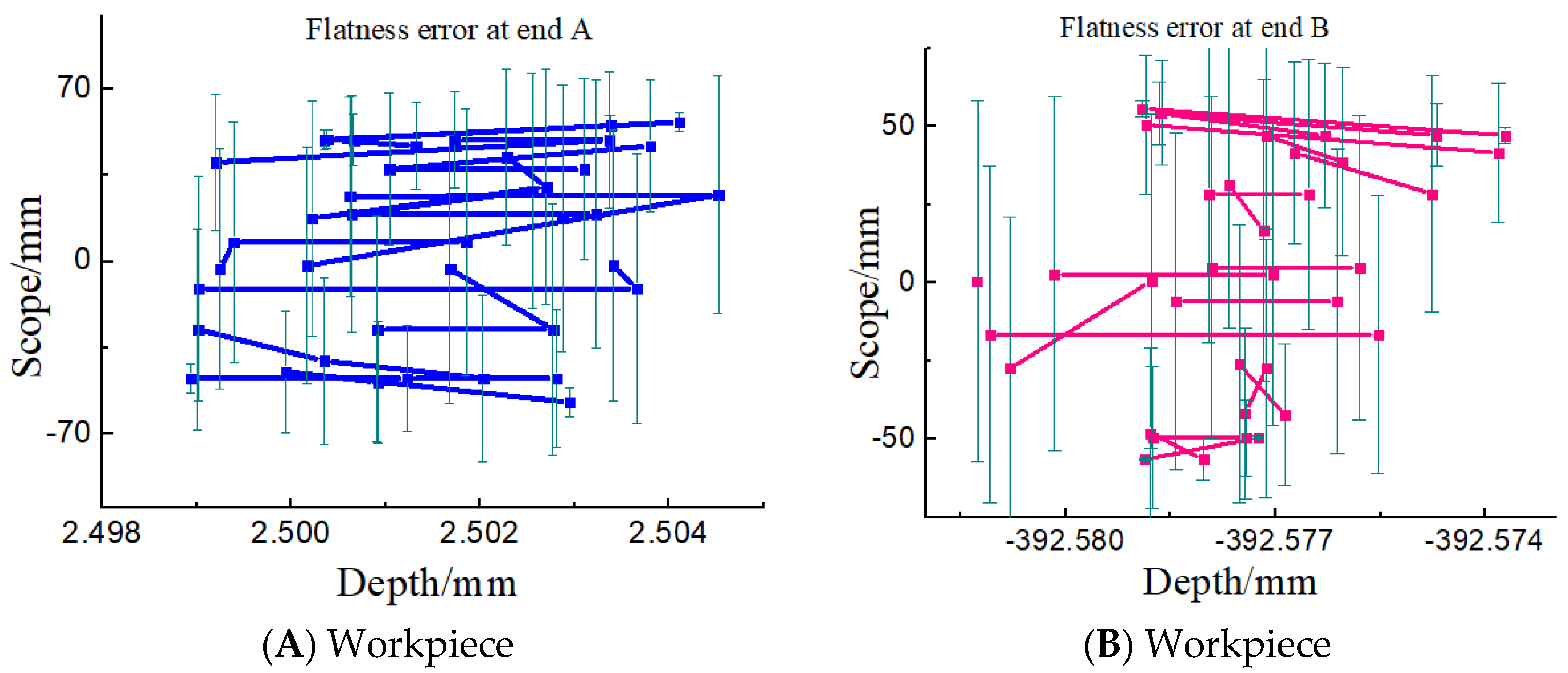
5.2. Precision Three Coordinate Measurement Analysis
6. Conclusions
- (1)
- For the multi-body system theory, the “double accuracy” theory is proposed in this paper. Firstly, the spatial coordinate system of grinding machine and three-coordinate measuring instrument is established, and the precision theory of grinding machine and three-coordinate measuring instrument is analyzed. Secondly, the two theories are combined for analysis and theoretical verification of the model.
- (2)
- Aiming to better explain the “double accuracy” theory, experiments are carried out in the article to prove that through the machining of the motorized spindle, the Swiss high precision grinding machine is used for fine grinding, and the machined motorized spindle is detected. At the same time, the shape and position tolerances, such as roundness, verticality, end face flatness and coaxiality, are obtained. Showing from the results, the main reason for the change of two-stage linear error lies in the fact that the workpiece is clamped by a double-top clamping method. The expansion sleeve has a certain outward expansion tension, which maximizes the error between the two sections of the workpiece. The roundness of A and B is 5 μm and 0.5 μm, respectively; the verticality is 3 μm and 4.5 μm, respectively; the flatness is 7.2 μm and 8.4 μm, respectively; and the coaxiality is 1.2 μm, respectively. At the same time, the coaxiality error difference between the two ends of the motorized spindle is analyzed to be 0.0294 mm. The main reason for this is the accuracy of tooling and fixture, the size of grinding wheel abrasive particles and the processing technology.
- (3)
- The research of this method not only theoretically expounds the precision relation between grinding and subsequent measurement, but also actually analyzes the causes of the errors and the areas that need improvement in the future. At the same time, the differences with the existing research results are analyzed, which lays a theoretical and experimental foundation for similar processing analysis.
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
Appendix A
References
- Smirnov, V.A.; Repko, A.V. Workpiece temperature variations during flat peripheral grinding. Manag. Syst. Prod. Eng. 2018, 26, 93–98. [Google Scholar] [CrossRef]
- Fan, J.; Wang, H.; Tang, Y. Error modeling and identification technology for a CNC camshaft grinder. J. Vib. Shock 2017, 36, 257–264. [Google Scholar]
- Qu, D.; Ding, F.; Zhou, Z.Q.; Xue, J.D.; Wang, B. Experimental study on grinding the micro wall of flexible joints with controllable force. Int. J. Adv. Manuf. Technol. 2017, 89, 1485–1494. [Google Scholar] [CrossRef]
- Liu, J.; Hong, Y. Analysis and robust design of geometric accuracy of a three-axis CNC surface grinding machine. Hong Kong J. Soc. Sci. 2016, 4, 43. [Google Scholar]
- Sato, A.; Shen, K.; Minami, M.; Matsuno, T. Improvement of force-sensorless grinding accuracy with resistance compensation. Artif. Life Robot. 2017, 22, 509–514. [Google Scholar] [CrossRef]
- Chakule, R.R.; Chaudhari, S.S.; Talmale, P.S. Evaluation of the effects of machining parameters on MQL based surface grinding process using response surface methodology. J. Mech. Sci. Technol. 2017, 31, 3907–3916. [Google Scholar] [CrossRef]
- Liu, S.; Yang, P.; Kang, H. Secondary separation technology of spindle rotation error based on three-point method. Manuf. Technol. Mach. Tool 2008, 112–115. [Google Scholar]
- Cappa, S.; Reynaerts, D.; Al-Bender, F. A sub-nanometre spindle error motion separation technique. Precis. Eng. 2014, 38, 458–471. [Google Scholar] [CrossRef]
- Ferreira, F.I.; de Aguiar, P.R.; Lopes, W.N.; Rossinoli Martins, C.H.; de Souza Ruzzi, R.; Bianchi, E.C.; D’Addona, D.M. Inferential measurement of the dresser width for the grinding process automation. Int. J. Adv. Manuf. Technol. 2019, 100, 3055–3066. [Google Scholar] [CrossRef]
- Zhang, Y.-N.; Lin, B.; Liu, J.-J.; Key, J.Y. An experimental study on mechanical modeling of ceramics based on microstructure. Appl. Sci. 2015, 5, 1337–1349. [Google Scholar] [CrossRef]
- Han, X.; Wu, T. Analysis of acoustic emission in precision and high-efficiency grinding technology. Int. J. Adv. Manuf. Technol. 2013, 67, 1997–2006. [Google Scholar] [CrossRef]
- Jiang, C.; Li, H.; Mai, Y.; Guo, D. Material removal monitoring in precision cylindrical plunge grinding using acoustic emission signal. Proc. Inst. Mech. Eng. Part C. J. Mech. Eng. Sci. 2013, 228, 715–722. [Google Scholar] [CrossRef]
- Kim, H.Y.; Kim, S.R.; Ahn, J.H.; Kim, S.H. Process monitoring of centerless grinding using acoustic emission. J. Mater. Process. Tech. 2001, 111, 273–278. [Google Scholar] [CrossRef]
- Zhong, G.; Liu, P.; Mei, X.; Wang, Y.; Xu, F.; Yang, S. Design optimization approach of a large-scale moving framework for a large 5-axis machining center. Appl. Sci. 2018, 8, 1598. [Google Scholar] [CrossRef]
- Qin, H.J.; Li, Y.; Xiong, X. Workpiece pose optimization for milling with flexible-joint robots to improve quasi-static performance. Appl. Sci. 2019, 9, 1044. [Google Scholar] [CrossRef]
- Xing, Y.; Zhang, L.; He, B.; Wang, S. Precision reverse design of numerical controlled (NC) machine on the basis of multibody theory. Nongye Jixie Xuebao/Trans. Chin. Soc. Agric. Mach. 2014, 45, 282–287. [Google Scholar]
- Su, S.; Li, S. A study on prediction modeling of machining accuracy for CNC machine tools. ICPN 2002, 2, 123–127. [Google Scholar]
- Liu, Y.; Zhang, Y. Thermal error compensation technology for machining centers based on multi-body theory model. J. Mech. Eng. 2002, 38, 127–130. [Google Scholar] [CrossRef]
- Wang, W.; Yang, J.; Yao, X.; Fan, F.; Li, Z. Comprehensive modeling and real-time compensation of geometric error and thermal error of numerical control machine tools. J. Mech. Eng. 2012, 48, 165–170. [Google Scholar] [CrossRef]
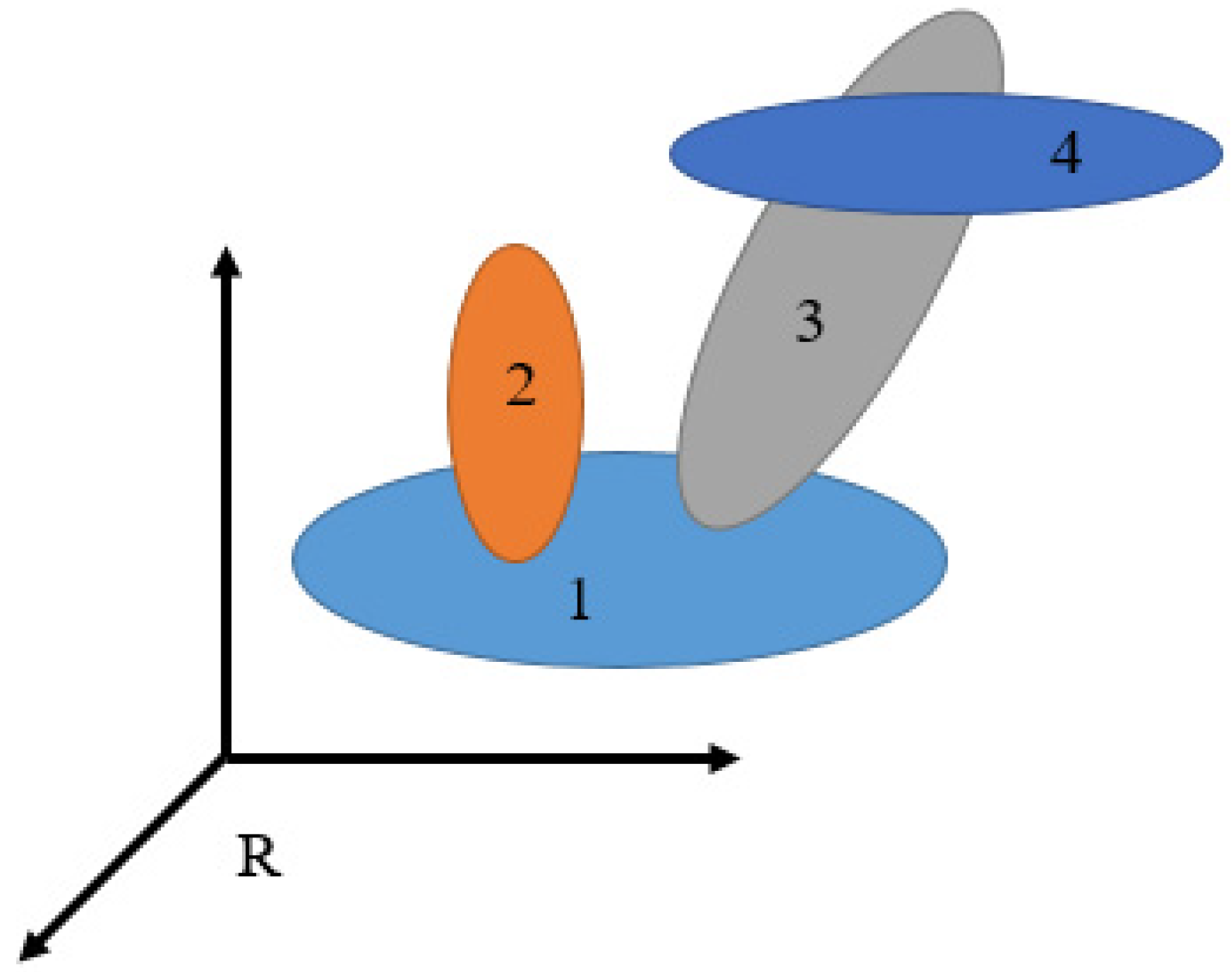
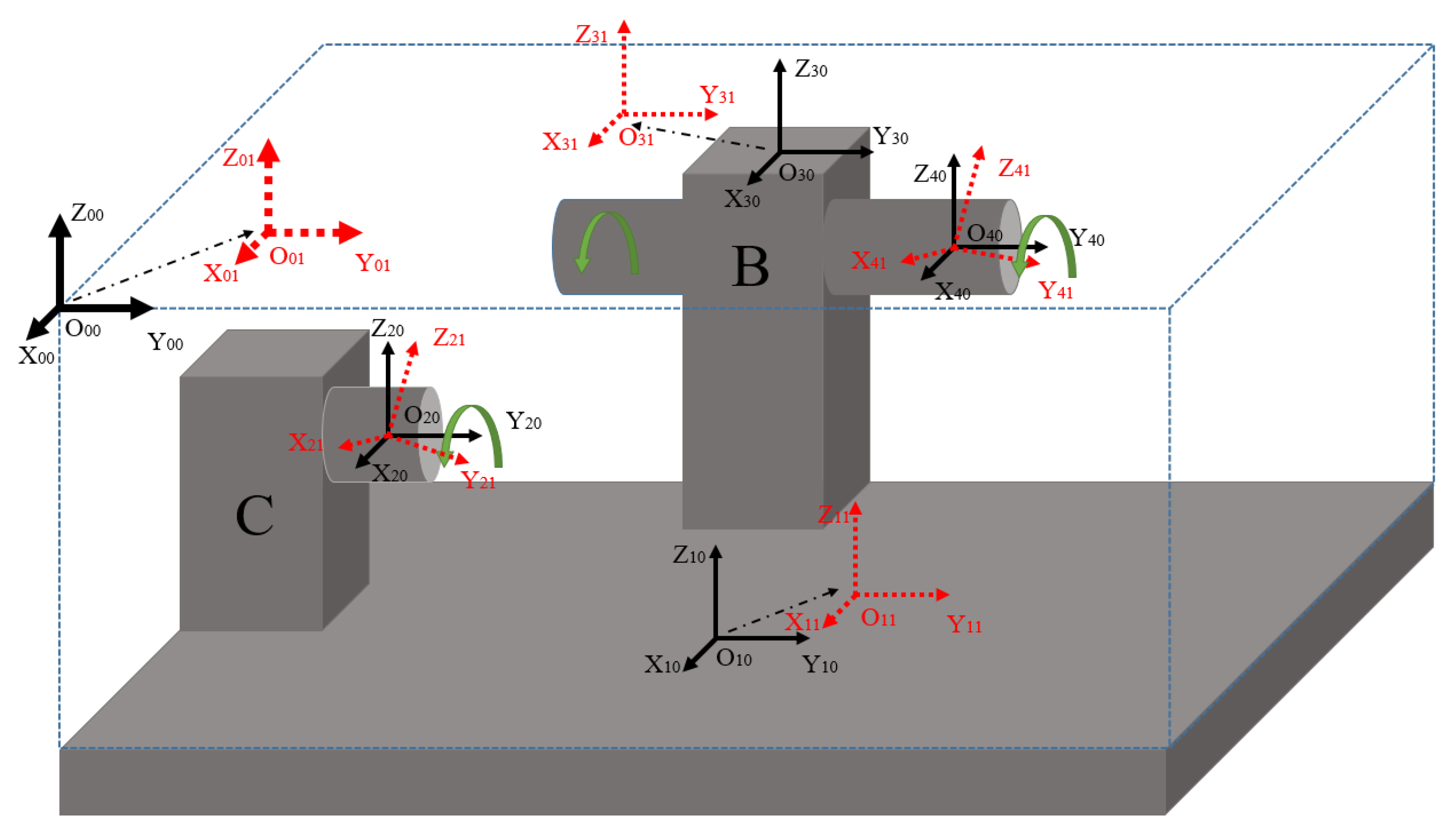
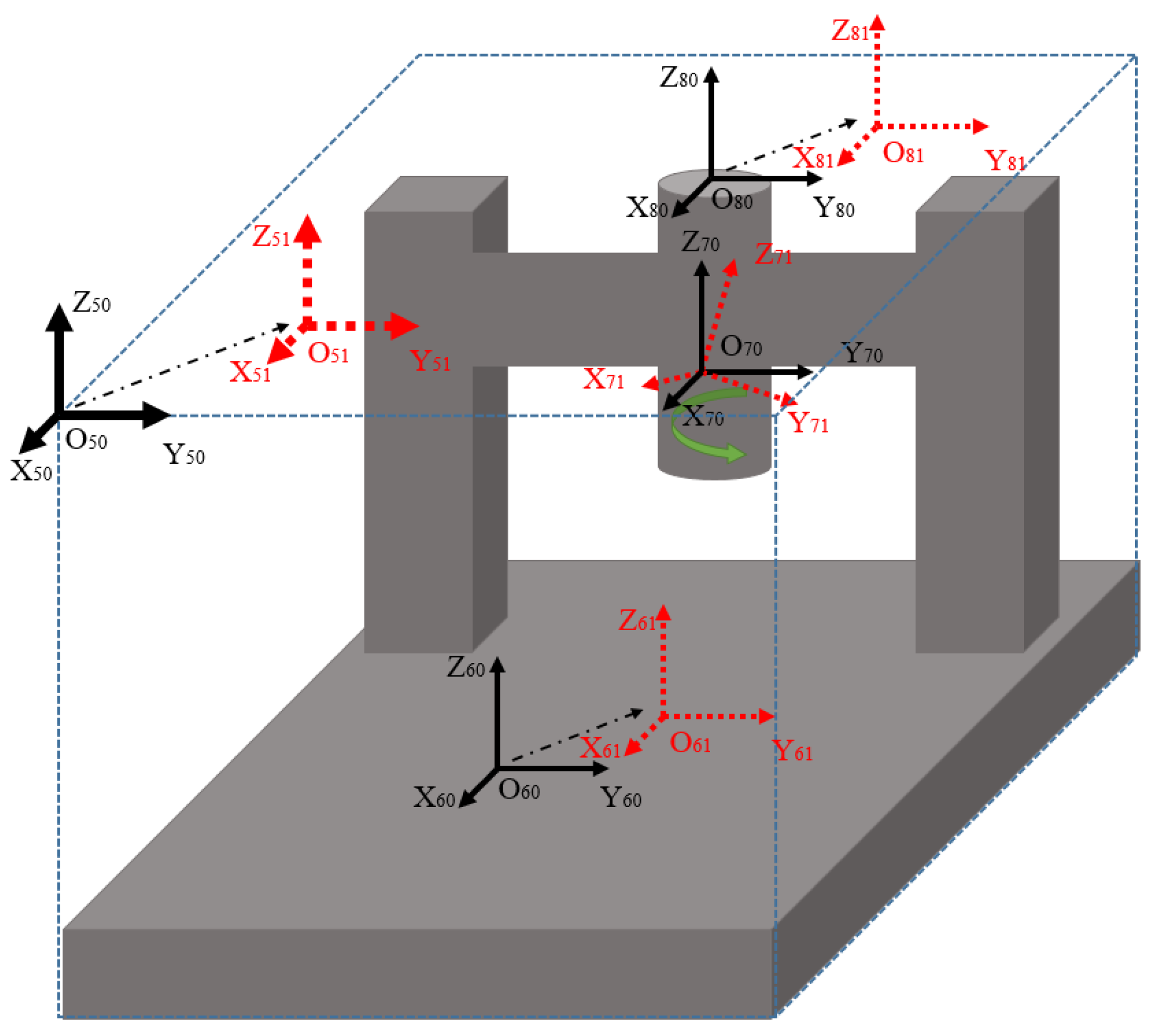



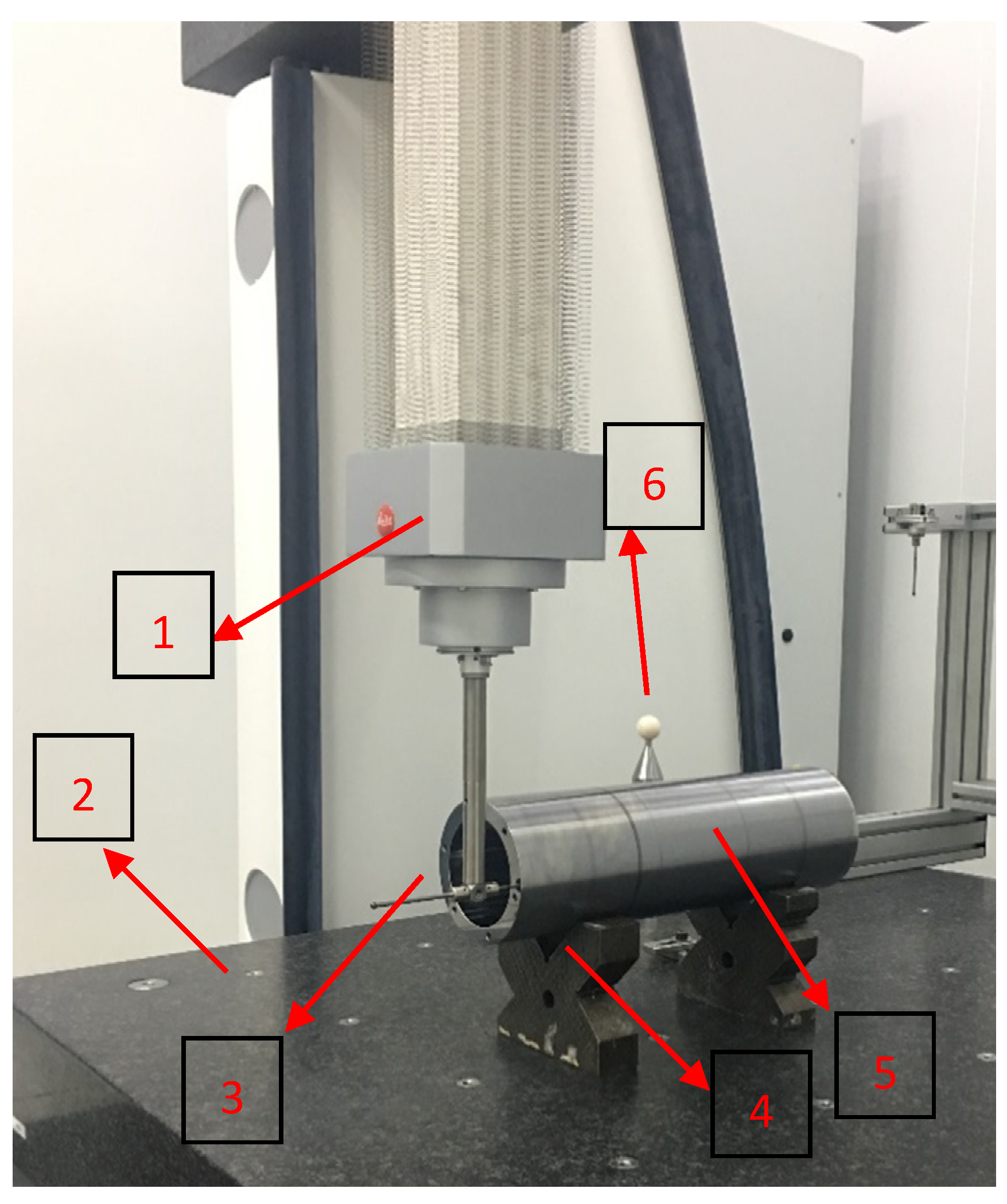

| j | 1 | 2 | 3 | 5 |
|---|---|---|---|---|
| L0(j) | 1 | 2 | 3 | 4 |
| L1(j) | 0 | 1 | 1 | 3 |
| L2(j) | 0 | 0 | 0 | 1 |
| L3(j) | 0 | 0 | 0 | 0 |
| L4(j) | 0 | 0 | 0 | 0 |
| L5(j) | 0 | 0 | 0 | 0 |
| Test Items | Error/mm | Test Items | Error/mm |
|---|---|---|---|
| End face 3 jumping | 0.0072 | Internal bore surface 1 for the first time | 0.0201 |
| End face 4 runout | 0.0084 | Inner bore surface 1 s time | 0.0195 |
| A verticality of end face | 00030 | Inner bore surface 1 third time | 0.0056 |
| B verticality of end face | 0.0045 | Internal bore surface 2 for the first time | 0.0158 |
| A end coaxiality | 0.0306 | Inner bore surface 2 s time | 0.0116 |
| B end coaxiality | 0.0012 | Inner bore surface 2 third time | 0.0005 |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Hu, L.; Li, Y.; Zha, J.; Chen, Y. A “Double Accuracy Theory” and Experimental Research on Precision Grinding. Appl. Sci. 2020, 10, 2030. https://doi.org/10.3390/app10062030
Hu L, Li Y, Zha J, Chen Y. A “Double Accuracy Theory” and Experimental Research on Precision Grinding. Applied Sciences. 2020; 10(6):2030. https://doi.org/10.3390/app10062030
Chicago/Turabian StyleHu, Lai, Yipeng Li, Jun Zha, and Yaolong Chen. 2020. "A “Double Accuracy Theory” and Experimental Research on Precision Grinding" Applied Sciences 10, no. 6: 2030. https://doi.org/10.3390/app10062030
APA StyleHu, L., Li, Y., Zha, J., & Chen, Y. (2020). A “Double Accuracy Theory” and Experimental Research on Precision Grinding. Applied Sciences, 10(6), 2030. https://doi.org/10.3390/app10062030





