Impact of Cyclic Loading on Shakedown in Cohesive Soils—Simple Hysteresis Loop Model
Abstract
Featured Application
Abstract
1. Introduction
- Equations in which the accumulated plastic strain is calculated as a function of the first cycle of loading, the initial density, or the value of repeating stress level.
- The relationship between the permanent strain in the referenced cycle, which is dependable on the state of stress, void ratio, the stress amplitude, which are constant.
- The equations which are able to predict the permanent strain development as a function of the number of cycles in the stated of stress, and other factors.
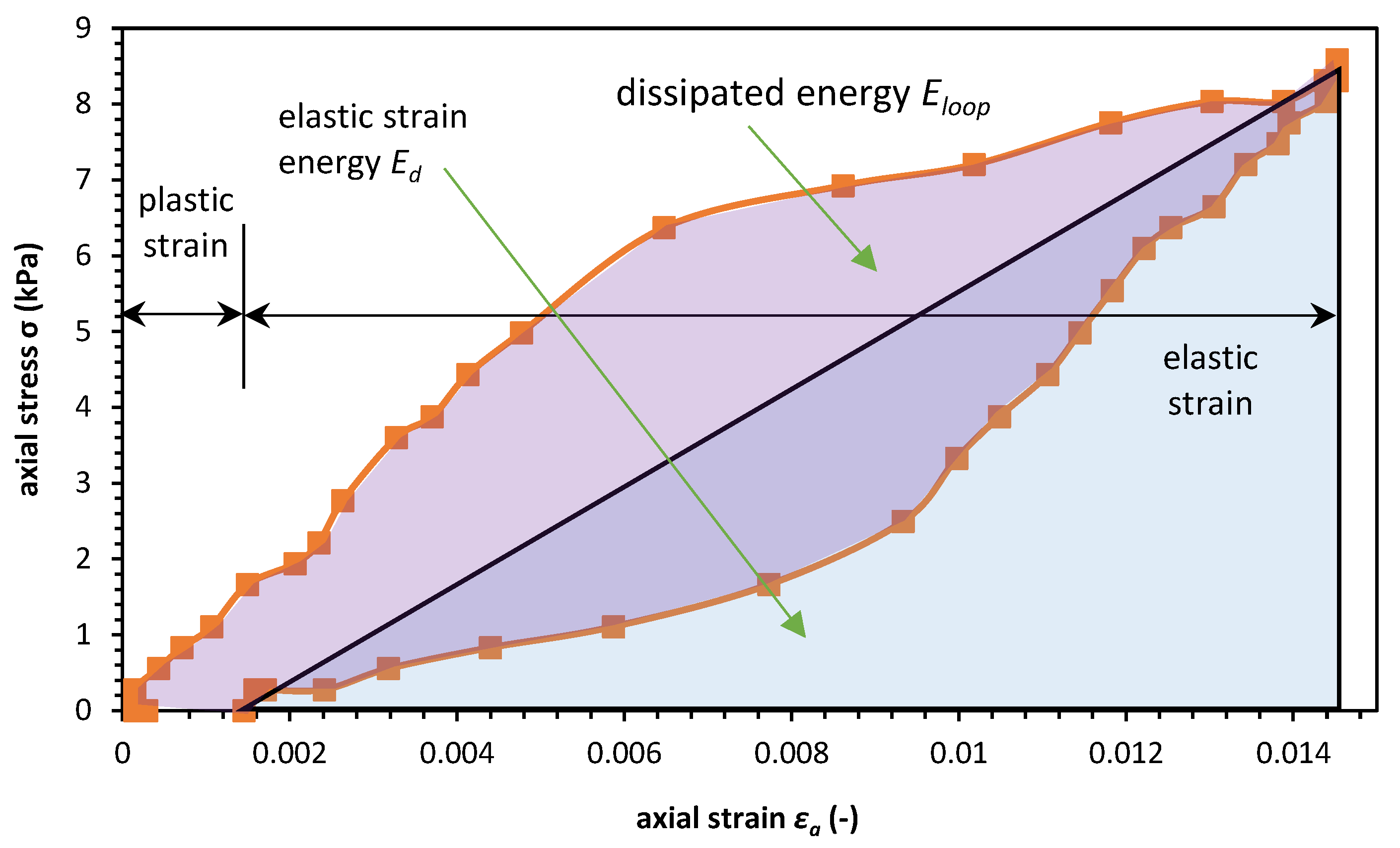
2. Simple Hysteresis Loop Model
3. Materials and Methods
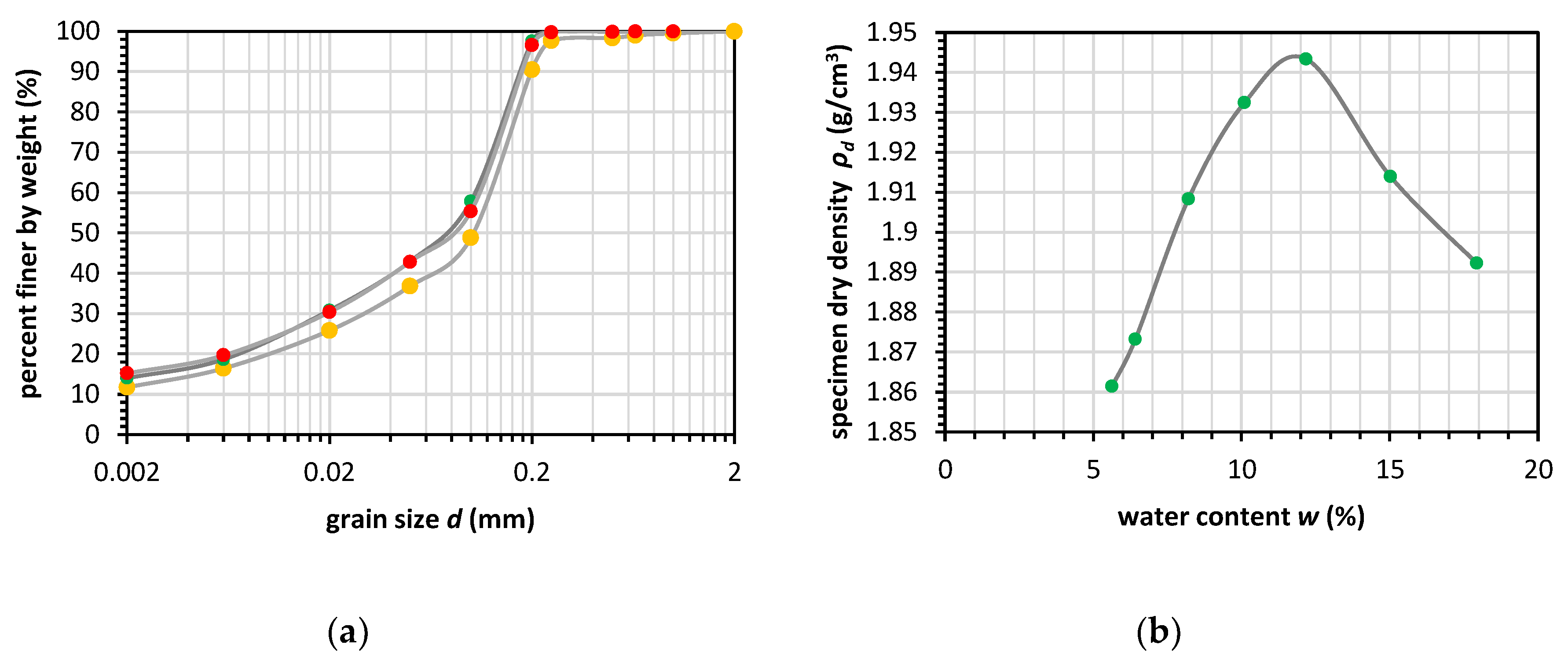
3.1. The Cohesive Soil Properties
3.2. Sample Properties, and Preparation to the Test
3.3. Test Procedure and Test Program
4. Results
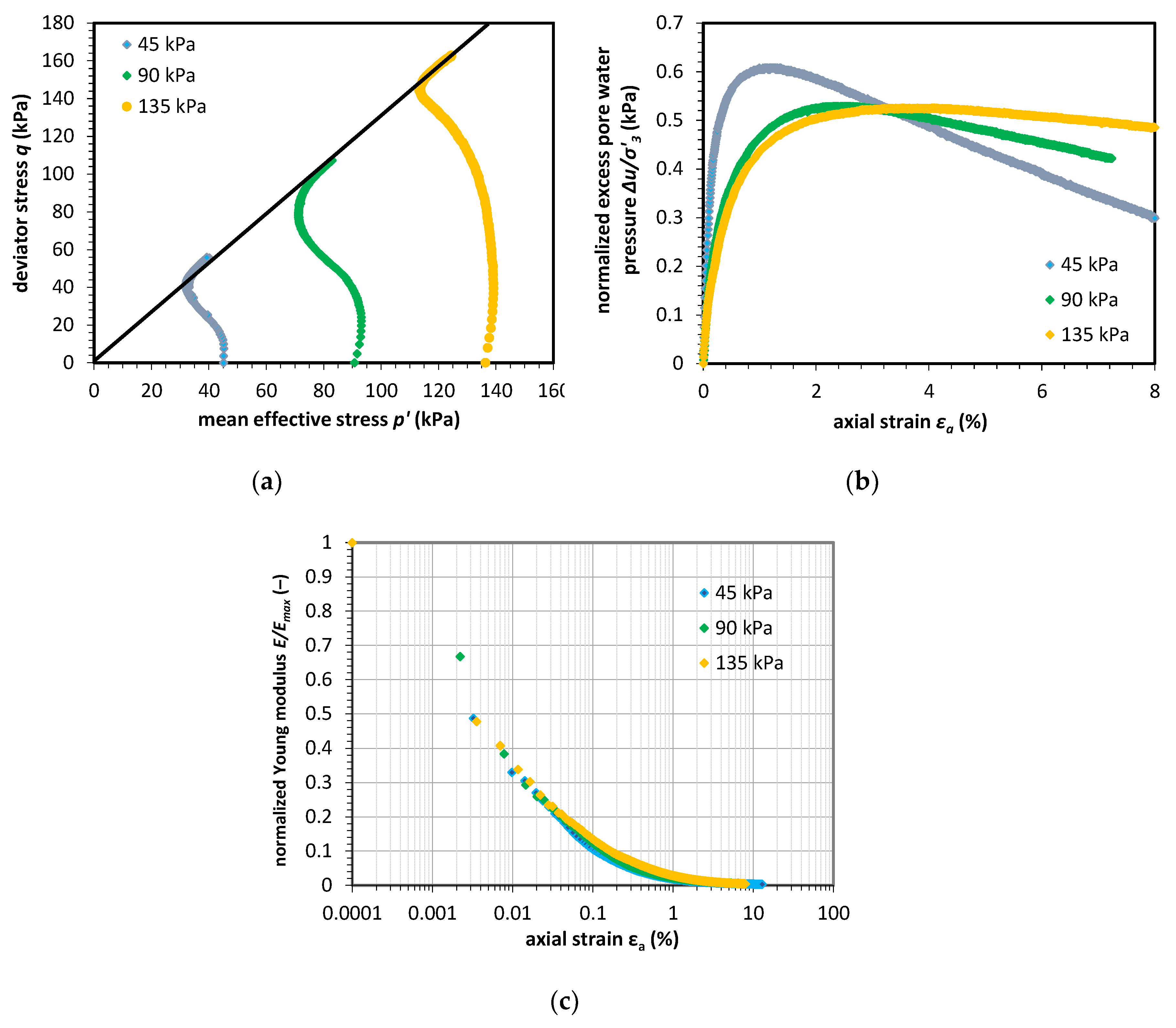
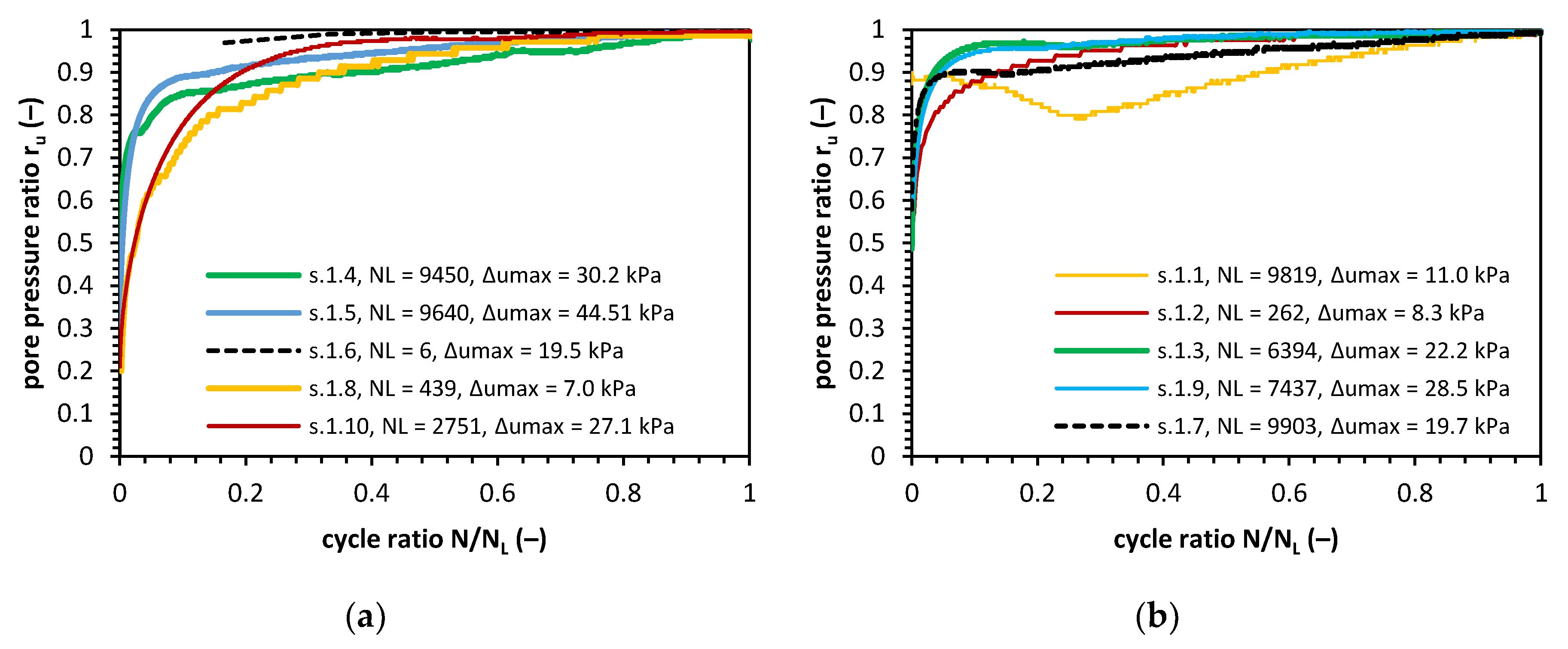
4.1. Pore Pressure Characteristics
4.2. Deformability Characteristics
4.3. Model Validation
5. Conclusions
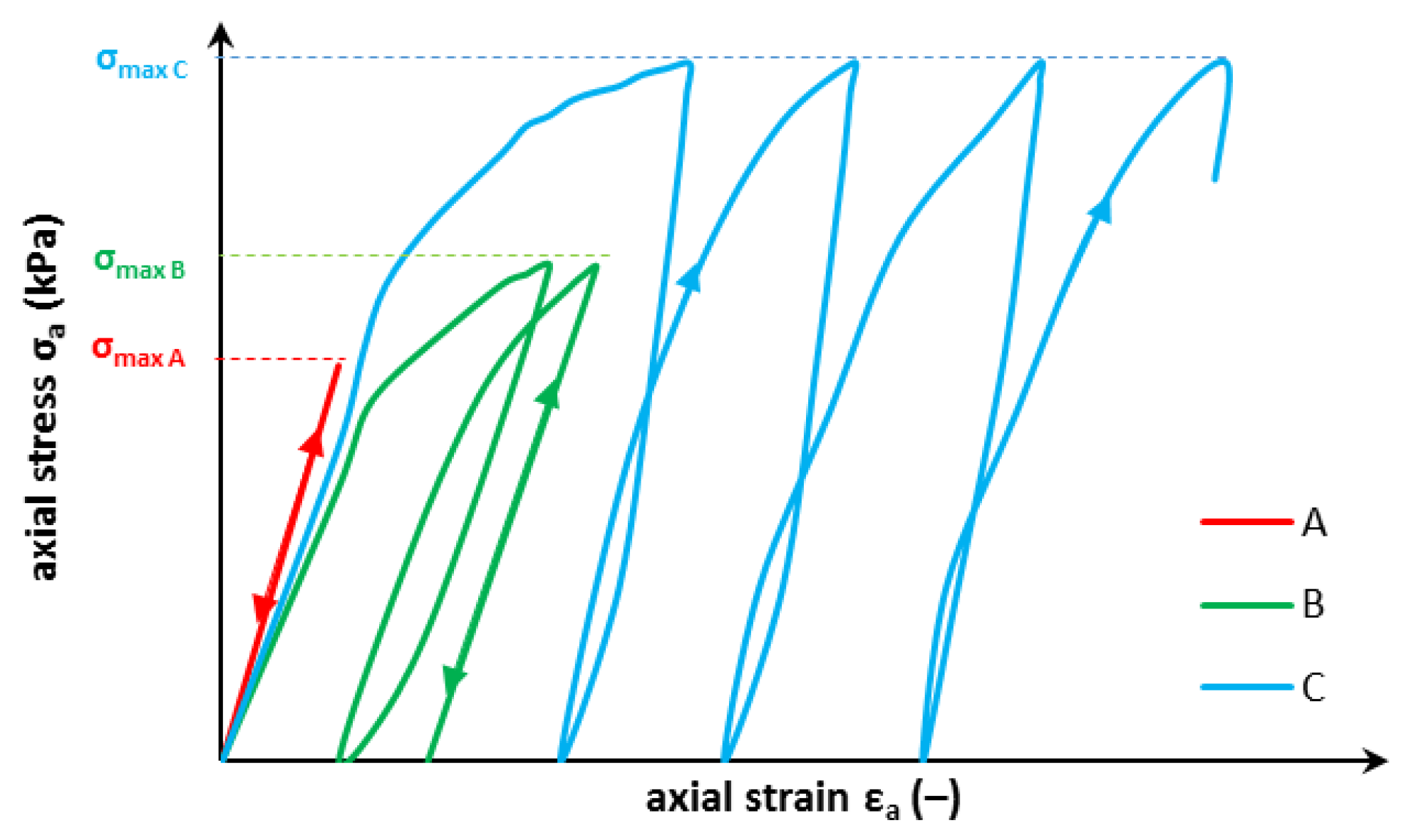
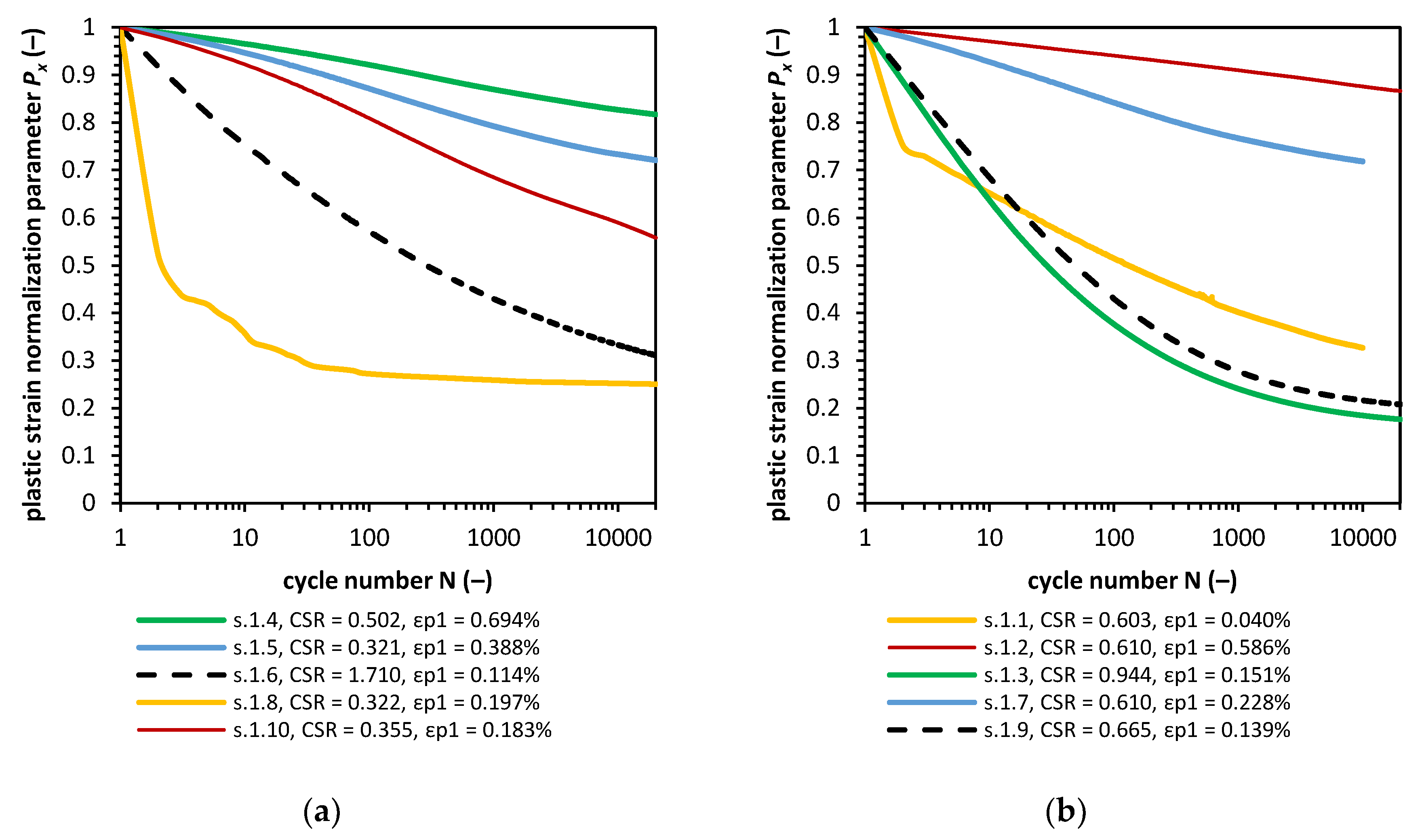
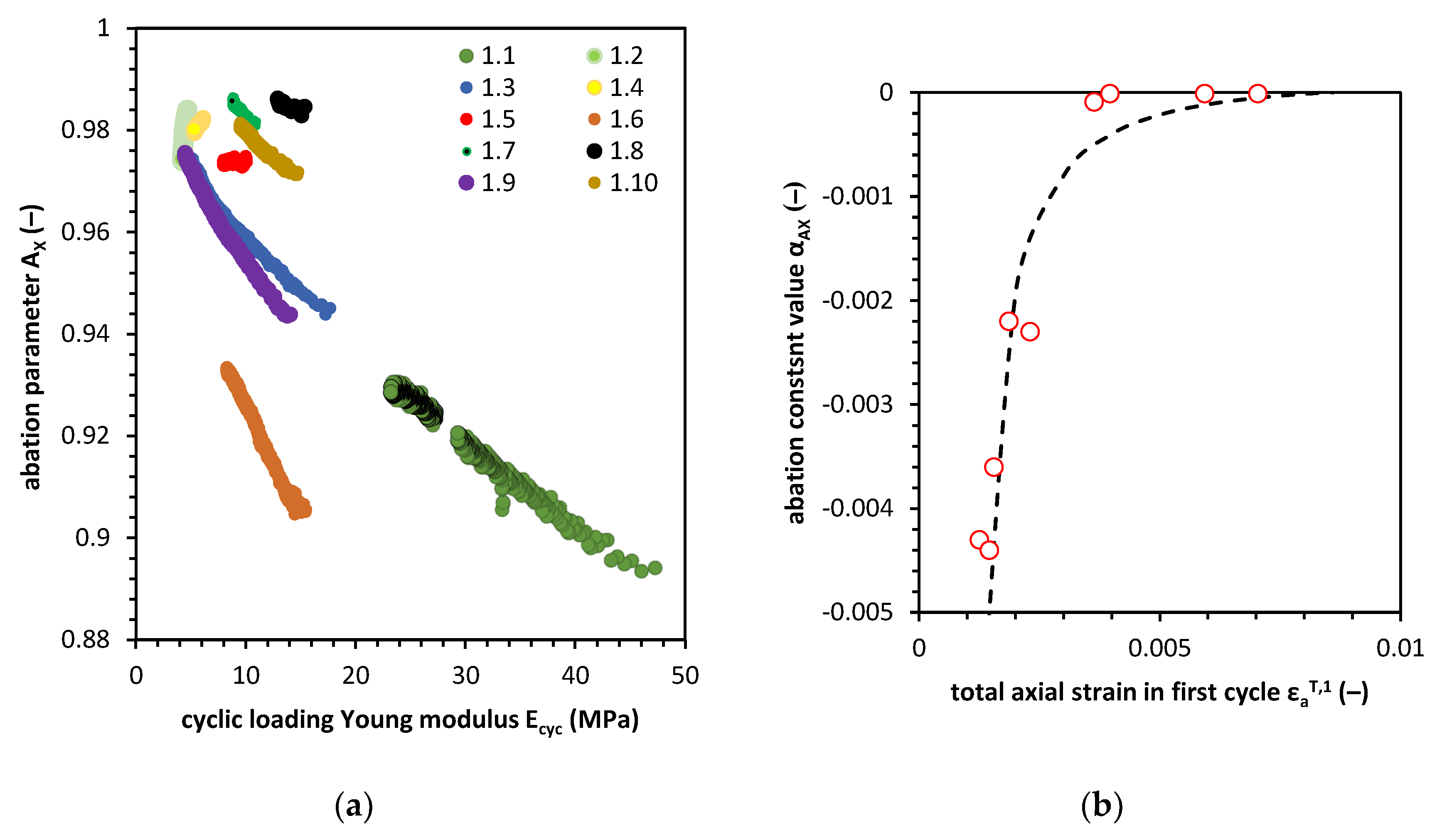
- During all tests, the axial strain follows the same pattern. The first few cycles constitute a major part of the registered deformations. After this event, the increase in axial strain is lower and the amount of accumulated plastic strain is lower with each cycle. This behavior was defined as abation, which means the accumulation potential during cyclic loading abates with the running test. Specimens under higher critical state ratios show greater accumulation of deformations.
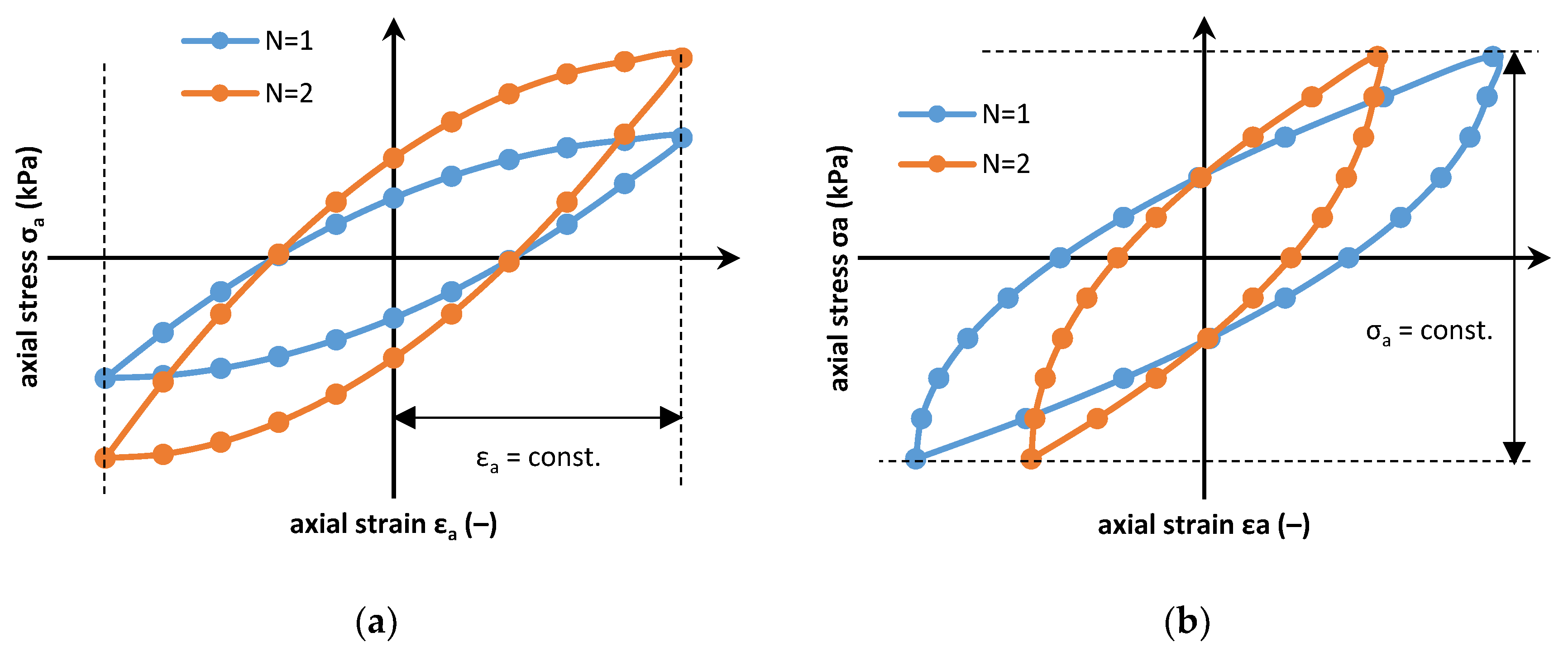
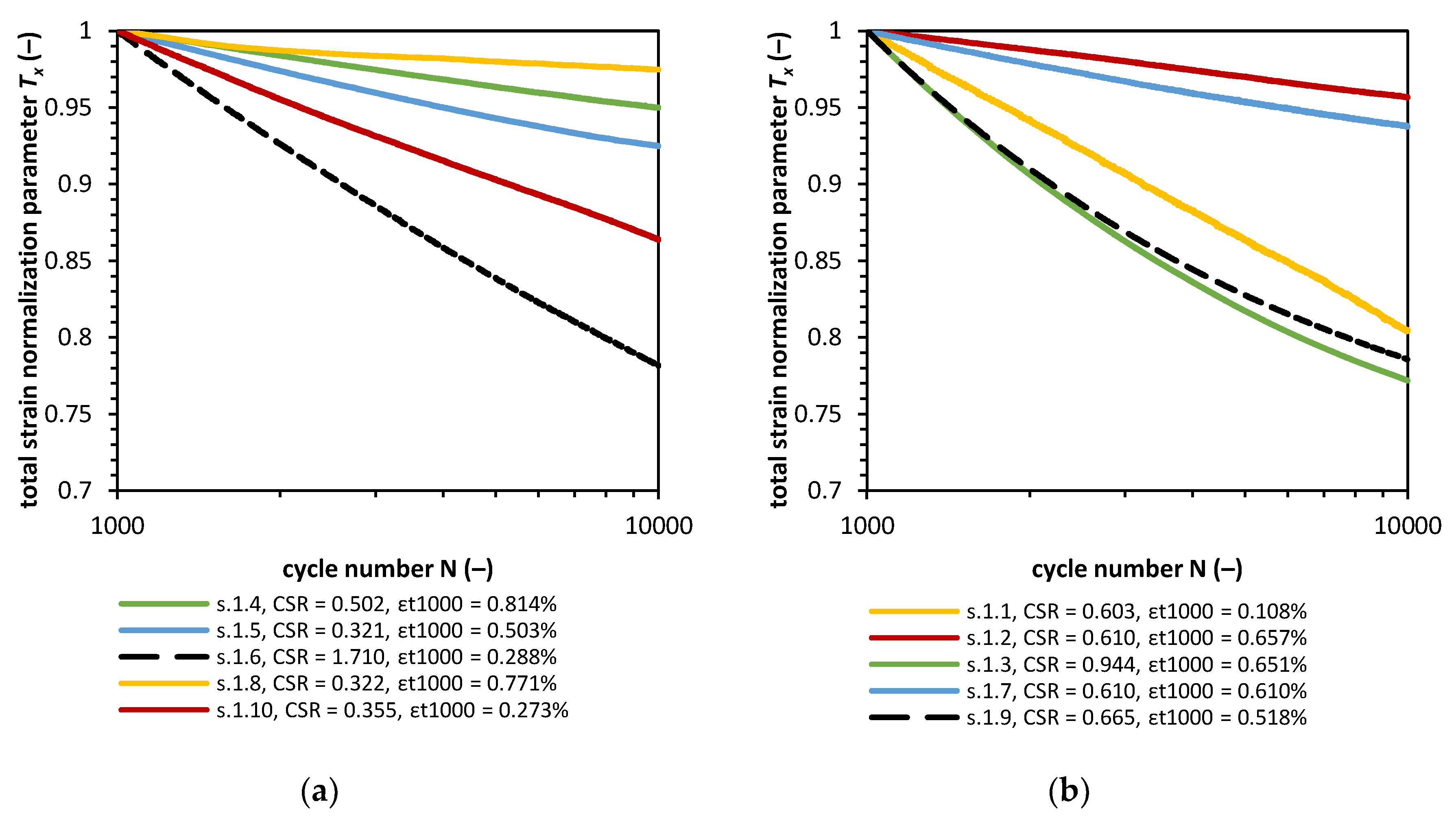
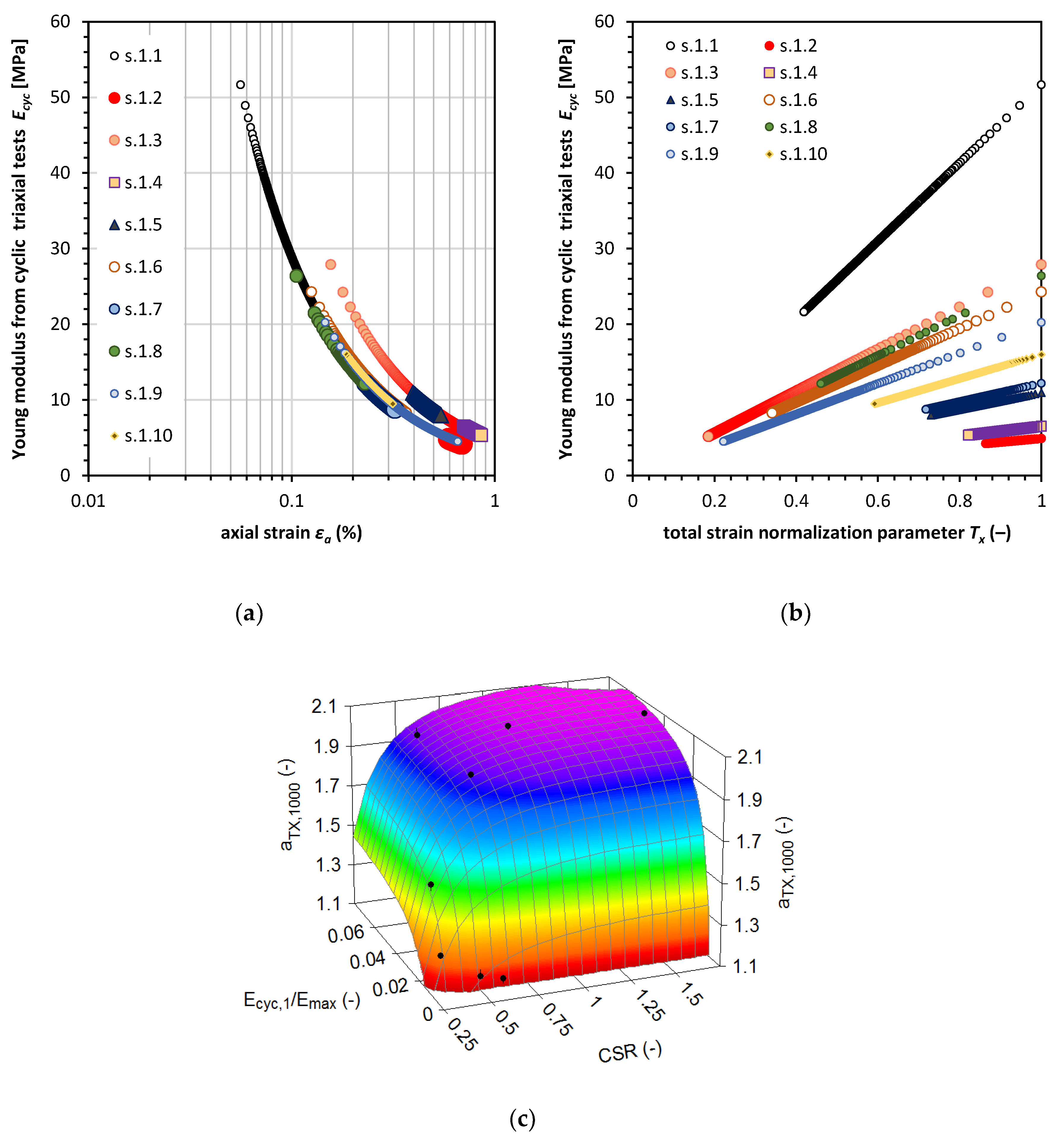
- Registered strains were normalized with the use of first strain value as a reference. We introduced the total and plastic strain normalization parameters, and power function was employed to describe the strain–number of cycles relationship. This approach resulted in the sample stiffness analysis which showed a linear relationship between cyclic Young modulus Ecyc degradation and the total strain normalization parameter Tx.
- The abation parameter Ax, which describes the plastic strain related to the total strain in one cycle, has shown satisfying linear relationship cyclic Young modulus degradation characteristics. This relationship also shows that the soil response to cyclic loading can be described in terms of abation rather than in terms of shakedown. Nevertheless, after numerous cycles, when the rate of plastic strain decreases to zero, the shakedown will occur and the soil respond to cyclic loading might be called as a shakedown
- Based on the abovementioned stiffness–strain–stress considerations, we derived the SHLM. This model can simulate the strain development during cyclic loading and, therefore, enables engineers to model the soil deformation when subjected to cyclic loading. The model needs four constants, namely: maximum shear modulus Emax, total strain in the first cycle caused by cyclic loading , the maximal deviator stress qmax and the initial effective confining pressure σ’3. The SHLM is based on the six more hidden parameters which remain constant in this study and which might later be changed when more cyclic triaxial data would be employed for analysis. Nevertheless, the SHLM delivers a close forecast of plastic and elastic strain accumulation in terms of prognosis precision and quantity of strain accumulation. We estimated the mean percentage error of SHLM to be equal 12.18% based on ex-post analysis.
Author Contributions
Funding
Conflicts of Interest
Appendix A
| Soil Sample No | Parameter Value, aPX (–) | Parameter Value, bPX (–) | Coeff. of Determination, R2 |
|---|---|---|---|
| 1.1 | 0.7475 | −0.0904 | 0.997 |
| 1.2 | 1.0238 | −0.0169 | 0.998 |
| 1.3 | 0.5030 | −0.1130 | 0.989 |
| 1.4 | 1.0100 | −0.0218 | 0.999 |
| 1.5 | 0.9943 | −0.0333 | 0.996 |
| 1.6 | 0.9189 | −0.1106 | 0.999 |
| 1.7 | 0.9276 | −0.0279 | 0.997 |
| 1.8 | 0.2750 | −0.0097 | 0.968 |
| 1.9 | 0.5423 | −0.1010 | 0.986 |
| 1.10 | 1.0613 | −0.0637 | 0.999 |
| e (–) | CSR (–) | σ’3 (kPa) | qm (kPa) | qa (kPa) | Δε1 (–) | Δε10 (–) | bPX (–) | |
|---|---|---|---|---|---|---|---|---|
| R (–) | −0.316 0.373 | −0.819 0,003 | 0.355 0.344 | 0.047 0.897 | −0.484 0.157 | 0.068 0.982 | 0.008 0.982 | 0.381 0.277 |
| e (–) | 0.373 0.228 | −0.295 0.408 | −0.211 0.559 | −0.016 0.965 | 0.499 0.142 | 0.452 0.190 | −0.171 0.636 | |
| CSR (–) | −0.752 0.012 | −0.096 0.793 | 0.346 0.328 | 0.188 0.603 | 0.335 0.345 | −0.658 0.039 | ||
| σ’3 (kPa) | 0.472 0.168 | 0.245 0.495 | −0.194 0.591 | −0.348 0.324 | 0.576 0.081 | |||
| qm (kPa) | 0.851 0.002 | −0.168 0.643 | −0.129 0.723 | 0.025 0.946 | ||||
| qa (kPa) | −0.161 0.656 | −0.098 0.789 | −0.179 0.621 | |||||
| Δε1 (–) | 0.952 0.000 | −0.280 0.434 | ||||||
| Δε10 (–) | −0.538 0.108 |
| Soil Sample No | Tx,1000 | Tx,1 | ||||
|---|---|---|---|---|---|---|
| Parameter Value, aTX,1000 (–) | Parameter Value, bTX,1000 (–) | Coeff. of Determination, R2 (–) | Parameter Value, aTX,1 (–) | Parameter Value, bTX,1 (–) | Coeff. of Determination, R2 (–) | |
| 1.1 | 1.9389 | −0.0951 | 0.999 | 1.01955 | −0.09632 | 0.999 |
| 1.2 | 1.1479 | −0.0198 | 0.999 | 1.021349 | −0.01803 | 0.994 |
| 1.3 | 2.0554 | −0.10757 | 0.989 | 0.57083 | −0.15921 | 0.945 |
| 1.4 | 1.1653 | −0.02228 | 0.999 | 1.017794 | −0.02336 | 0.998 |
| 1.5 | 1.2559 | −0.0335 | 0.996 | 1.018177 | −0.03671 | 0.995 |
| 1.6 | 2.0787 | −0.10642 | 0.999 | 0.949901 | −0.11188 | 0.999 |
| 1.7 | 1.2040 | −0.02729 | 0.997 | 0.959363 | −0.03209 | 0.984 |
| 1.8 | 1.0582 | −0.00899 | 0.968 | 0.546481 | −0.01959 | 0.526 |
| 1.9 | 1.9281 | −0.09863 | 0.986 | 0.827068 | −0.14835 | 0.949 |
| 1.10 | 1.5347 | −0.06229 | 0.999 | 1.076369 | −0.06499 | 0.998 |
| e (–) | CSR (–) | σ’3 (kPa) | qm (kPa) | qa (kPa) | aTX,1000 (–) | bTX,1000 (–) | |
|---|---|---|---|---|---|---|---|
| R (–) | −0.316 0.373 | −0.819 0,003 | 0.355 0.344 | 0.047 0.897 | −0.484 0.157 | −0.395 0.259 | 0.369 0.294 |
| e (–) | 0.373 0.228 | −0.295 0.408 | −0.211 0.559 | −0.016 0.965 | 0.223 0.535 | −0.176 0.626 | |
| CSR (–) | −0.752 0.012 | −0.096 0.793 | 0.346 0.328 | 0.673 0.033 | −0.642 0.045 | ||
| σ’3 (kPa) | 0.472 0.168 | 0.245 0.495 | −0.604 0.064 | 0.579 0.079 | |||
| qm (kPa) | 0.851 0.002 | −0.053 0.886 | 0.041 0.909 | ||||
| qa (kPa) | −0.162 0.656 | −0.157 0.665 | |||||
| aTX (–) | -0.998 0.000 |
Appendix B
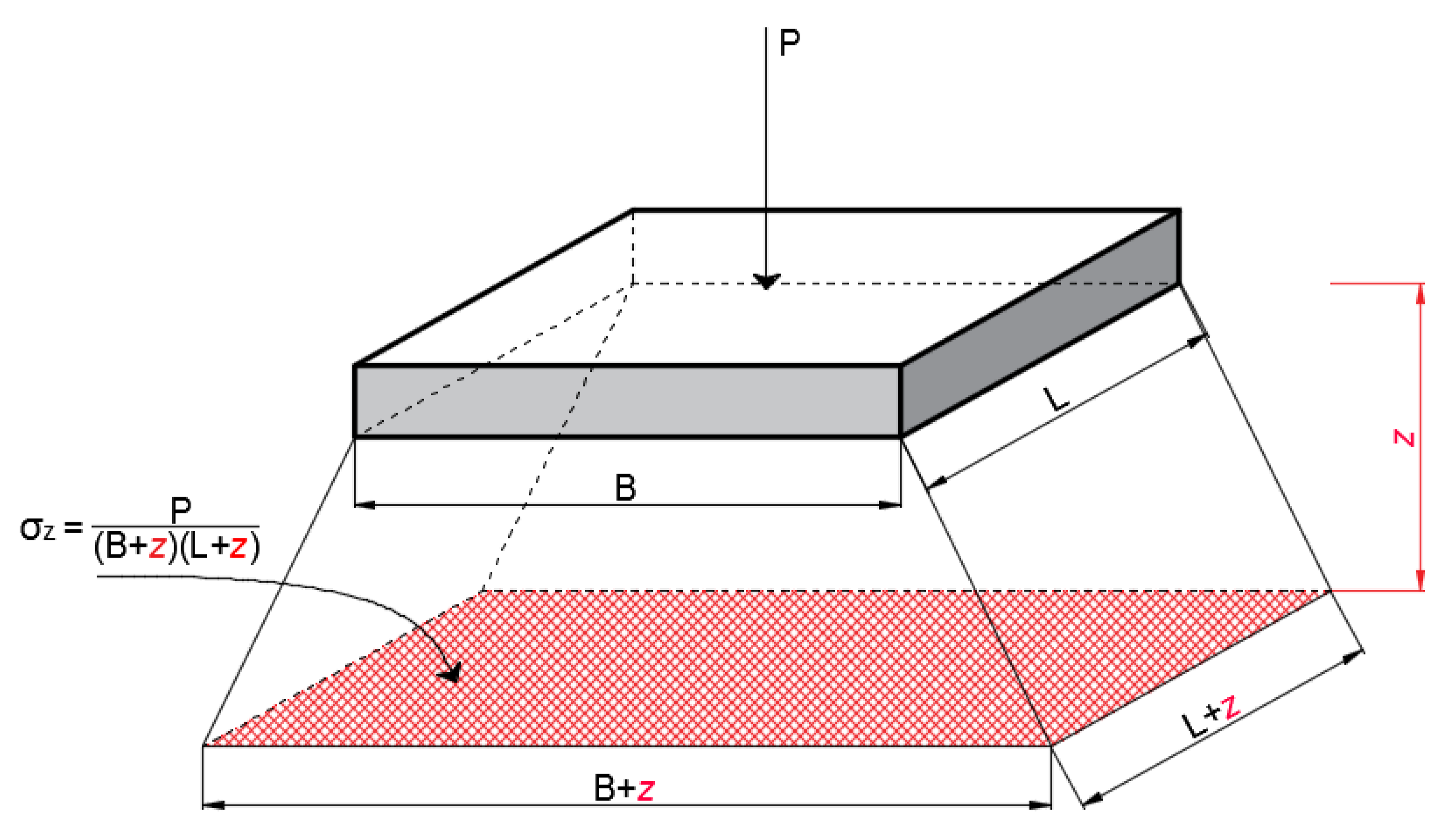
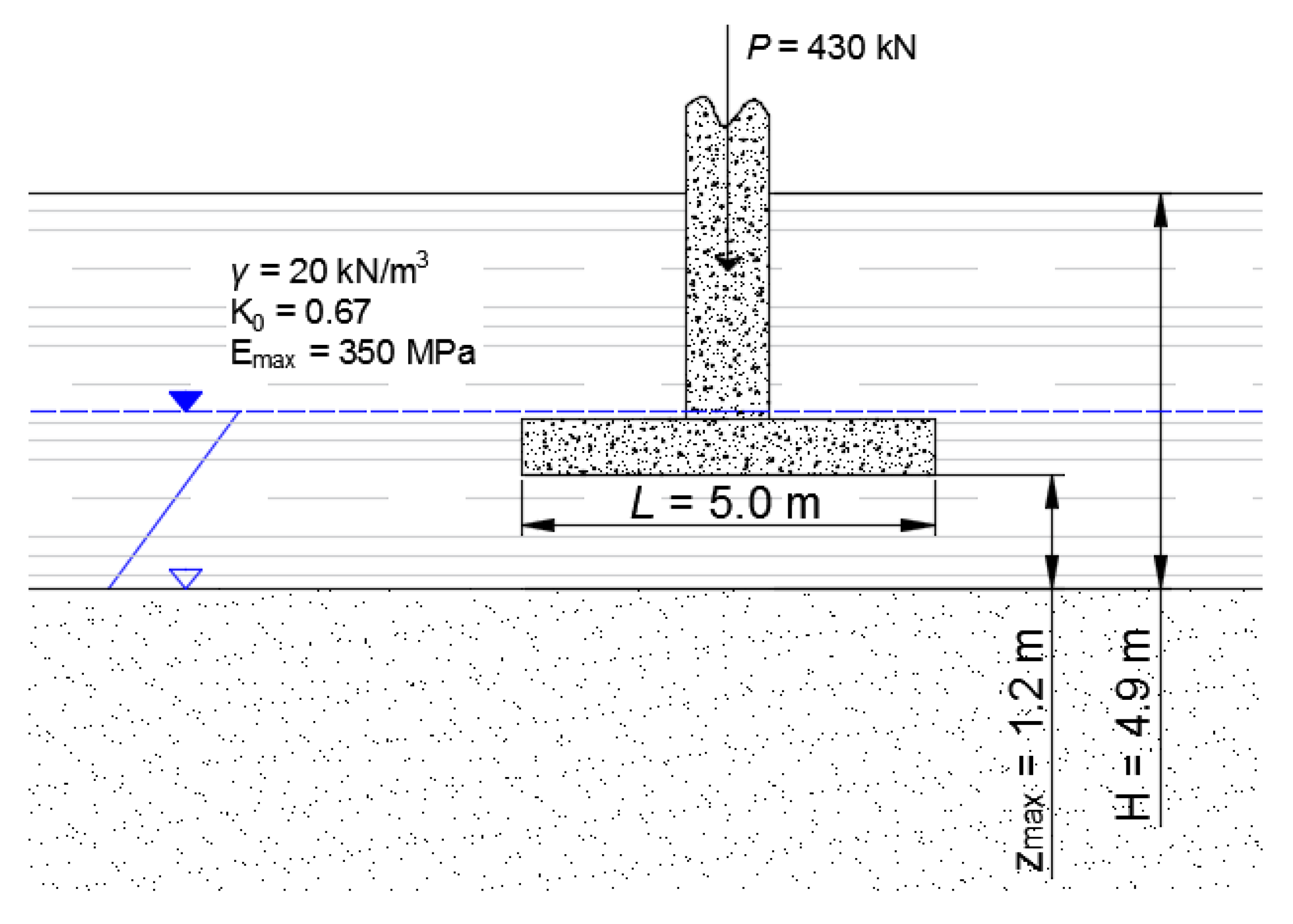
| z (m) | σmax = qmax (kPa) | Emax (MPa) | σ’3 (kPa) | εaT,1 (–) |
|---|---|---|---|---|
| 0.0–0.2 | 43.0 | 300.0 | 45.0 | 0.005 |
| 0.2–0.4 | 37.6 | 305.0 | 47.7 | 0.0045 |
| 0.4–0.6 | 33.2 | 310.0 | 50.3 | 0.004 |
| 0.6–0.8 | 29.5 | 315.0 | 53.0 | 0.0035 |
| 0.8–1.0 | 26.5 | 320.0 | 55.7 | 0.003 |
| 1.0–1.2 | 23.9 | 325.0 | 58.3 | 0.0025 |
| Parameter | α | β | γ | βTX,1000 | Δa | Δb |
| Value (–) | 2.0796 | 0.100419 | 0.003034 | −0.147 | −175.883 | −23211.4 |
| z (m) | aTX,1000 (–) | bTX,1 (–) | αAX (–) |
|---|---|---|---|
| 0.2 | 1.402216 | −0.04969 | −0.00032 |
| 0.4 | 1.453458 | −0.05497 | −0.00044 |
| 0.6 | 1.505418 | −0.06013 | −0.0006 |
| 0.8 | 1.557821 | −0.06516 | −0.00085 |
| 1 | 1.61031 | −0.07003 | −0.00125 |
| 1.2 | 1.6624 | −0.07471 | −0.00186 |
| z (m) | Cycle Number N (–) | |
|---|---|---|
| 1 | 1000000 | |
| 20 | 0.198532 | 0.197084 |
| 40 | 0.198845 | 0.197533 |
| 60 | 0.199094 | 0.197921 |
| 80 | 0.199294 | 0.198264 |
| 100 | 0.199458 | 0.198573 |
| 120 | 0.199592 | 0.198856 |
| ∑ (m) | 1.1948 | 1.1882 |
| Settlement (mm) | 5.2 | 11.8 |
References
- Bogusz, W.; Godlewski, T. Philosophy of geotechnical design in civil engineering—Possibilities and risks. Bull. Pol. Acad. Sci. Tech. Sci. 2019, 67, 289–306. [Google Scholar]
- Soból, E.; Sas, W.; Szymański, A. Scale Effect in Direct Shear Tests on Recycled Concrete Aggregate. Stud. Geotech. Mech. 2015, 37, 45–49. [Google Scholar] [CrossRef]
- Gabrys, K.; Sas, W.; Soból, E.; Głuchowski, A. Torsional Shear Device for Testing the Dynamic Properties of Recycled Material. Stud. Geotech. Mech. 2016, 38, 15–24. [Google Scholar] [CrossRef]
- Abdelkrim, M.; Bonnet, G.; de Buhan, P. A computational procedure for predicting the long term residual settlement of a plat-form induced by repeated traffic loading. Comput. Geotech. 2003, 30, 463–476. [Google Scholar] [CrossRef]
- Araya, A.A.; Huurman, M.; Molenaar, A.A.A. Integrating Traditional Characterization Techniques in Mechanistic Pavement Design Approaches; First Congress of Transportation and Development Institute (TDI): Chicago, IL, USA, 2011; pp. 596–606. [Google Scholar]
- O’Reilly, M.P.; Brown, S.F. Cyclic Loading of Soils; Blackie and Son: London, UK, 1991. [Google Scholar]
- Peralta, P.; Achmus, M. An experimental investigation of piles in sand subjected to lateral cyclic loads. In Proceedings of the Physical Modelling in Geotechnics, Two Volume Set; Informa UK Limited: Zurich, Switzerland, 2010; pp. 985–990. [Google Scholar]
- Sas, W.; Głuchowski, A.; Szynański, A. Behavior of recycled concrete aggregate improved with lime addition during cyclic loading. Int. J. GEOMATE 2016, 10, 1662–1669. [Google Scholar] [CrossRef]
- Cai, Y.; Gu, C.; Wang, J.; Juang, C.H.; Xu, C.; Hu, X. One-Way Cyclic Triaxial Behavior of Saturated Clay: Comparison between Constant and Variable Confining Pressure. J. Geotech. Geoenviron. Eng. 2013, 139, 797–809. [Google Scholar] [CrossRef]
- Cai, Y.; Sun, Q.; Guo, L.; Juang, C.H.; Wang, J. Permanent deformation characteristics of saturated sand under cyclic loading. Can. Geotech. J. 2015, 52, 795–807. [Google Scholar] [CrossRef]
- Gräbe, P.; Clayton, C.R.I. Effects of Principal Stress Rotation on Permanent Deformation in Rail Track Foundations. J. Geotech. Geoenviron. Eng. 2009, 135, 555–565. [Google Scholar] [CrossRef]
- Huang, M.S.; Li, J.J.; Li, X.Z. Cumulative deformation behaviour of soft clay in cyclic undrained tests. Chin. J. Geotech. Eng. 2006, 28, 891–895. [Google Scholar]
- Chai, J.; Miura, N. Traffic-Load-Induced Permanent Deformation of Road on Soft Subsoil. J. Geotech. Geoenviron. Eng. 2002, 128, 907–916. [Google Scholar] [CrossRef]
- Pasten, C.; Santamarina, J.C. Thermally Induced Long-Term Displacement of Thermoactive Piles. J. Geotech. Geoenviron. Eng. 2014, 140, 06014003. [Google Scholar] [CrossRef]
- Barksdale, R.D. Laboratory evaluation of rutting in base course materials. In Proceedings of the Third International Conference on the Structural Design of Asphalt Pavements, Grosvenor House, Park Lane, London, UK, 11–15 September 1972. [Google Scholar]
- Lekarp, F.; Isacsson, U.; Dawson, A.R. State of the Art. I: Resilient Response of Unbound Aggregates. J. Transp. Eng. 2000, 126, 66–75. [Google Scholar] [CrossRef]
- Osouli, A.; Adhikari, P.; Tutumluer, E.; Shoup, H. Properties of aggregate fines influencing modulus and deformation behaviour of unbound aggregates. Int. J. Pavement Eng. 2019, 1–16. [Google Scholar] [CrossRef]
- Li, L.; Liu, J.; Zhang, X.; Li, P.; Saboundjian, S. Characterizing Permanent Deformation of Alaskan Granular Base–Course Materials. J. Mater. Civ. Eng. 2019, 31, 04019267. [Google Scholar] [CrossRef]
- Lal, M.H.; Noolu, V.; Pillai, R.J.; Kurre, P.; Praveen, G.V. A review on permanent deformation of granular material. Indian J. Public Heal. Res. Dev. 2018, 9, 1158. [Google Scholar] [CrossRef]
- Lekarp, F.; Dawson, A.R. Modelling permanent deformation behaviour of unbound granular materials. Constr. Build. Mater. 1998, 12, 9–18. [Google Scholar] [CrossRef]
- Sun, Q.; Dong, Q.; Cai, Y.; Wang, J. Modeling permanent strains of granular soil under cyclic loading with variable confining pressure. Acta Geotech. 2019, 1–13. [Google Scholar] [CrossRef]
- Gidel, G.; Hornych, P.; Chauvin, J.J.; Breysse, D.; Denis, A. A new approach for investigating the permanent deformation behaviour of unbound granular material using the repeated load triaxial apparatus. Bull. Liaison Lab. Ponts Chaussées 2001, 233, 5–21. [Google Scholar]
- Wang, H.-L.; Chen, R.; Qi, S.; Cheng, W.; Cui, Y.-J. Long-Term Performance of Pile-Supported Ballastless Track-Bed at Various Water Levels. J. Geotech. Geoenviron. Eng. 2018, 144, 04018035. [Google Scholar] [CrossRef]
- Wang, H.-L.; Chen, R.; Cheng, W.; Qi, S.; Cui, Y.-J. Full-scale model study on variations of soil stress in geosynthetic-reinforced pile-supported track bed with water level change and cyclic loading. Can. Geotech. J. 2019, 56, 60–68. [Google Scholar] [CrossRef]
- Trinh, V.N.; Tang, A.M.; Cui, Y.J.; Dupla, J.C.; Canou, J.; Calon, N.; Lambert, L.; Robinet, A.; Schoen, O. Mechanical characterisation of the fouled ballast in ancient railway track sub-structure by large-scale triaxial tests. Soils Found. 2012, 52, 511–523. [Google Scholar] [CrossRef]
- Wang, H.-L.; Cui, Y.-J.; Lamas-Lopez, F.; Dupla, J.-C.; Canou, J.; Calon, N.; Saussine, G.; Aimedieu, P.; Chen, R. Permanent Deformation of Track-Bed Materials at Various Inclusion Contents under Large Number of Loading Cycles. J. Geotech. Geoenviron. Eng. 2018, 144, 04018044. [Google Scholar] [CrossRef]
- Yu, H.S.; Khong, C.D.; Wang, J.; Zhang, G. Experimental evaluation and extension of a simple critical state model for sand. Granul. Matter 2005, 7, 213–225. [Google Scholar] [CrossRef]
- Yu, H.S. Plasticity and Geotechnics; Springer: New York, NY, USA, 2006. [Google Scholar]
- Konig, J.A. Shakedown of Elastic-Plastic Structures; Elsevier: Berlin, Germany, 1987. [Google Scholar]
- Werkmeister, S. Shakedown analysis of unbound granular materials using accelerated pavement test results from New Zeland’s CAPTIF facility. In Geotechnical Special Publication 154; Huang, B., Meier, R., Prozzi, J., Tutumluer, E., Eds.; ASCE: Shanghai, China, 2006; pp. 220–228. [Google Scholar]
- Werkmeister, S.; Dawson, A.R.; Wellner, F. Permanent Deformation Behavior of Granular Materials and the Shakedown Concept. Transp. Res. Rec. J. Transp. Res. Board 2001, 1757, 75–81. [Google Scholar] [CrossRef]
- Pande, G.N. Shakedown of Foundations Subjected to Cyclic Loads, Soil Mechanics-Transient and Cyclic Loads; Pande, G.N., Zienkiewicz, O.C., Eds.; John Wiley & Sons: New York, NY, USA, 1982; pp. 469–489. [Google Scholar]
- Boulbibane, M.; Ponter, A.R.S. The linear matching method for the shakedown analysis of geotechnical problems. Int. J. Numer. Anal. Methods Géoméch. 2006, 30, 157–179. [Google Scholar] [CrossRef]
- Werkmeister, S. Shakedown Analysis of Unbound Granular Materials using Accelerated Pavement Test Results from New Zealand’s CAPTIF Facility. Adv. Unsaturated Soil Seepage Environ. Geotech. 2006, 154, 220–228. [Google Scholar]
- Tao, M.; Mohammad, L.; Nazzal, M.D.; Zhang, Z.; Wu, Z. Application of Shakedown Theory in Characterizing Traditional and Recycled Pavement Base Materials. J. Transp. Eng. 2010, 136, 214–222. [Google Scholar] [CrossRef]
- Nega, A.; Nikraz, H.; Al-Qadi, I. Simulation of Shakedown Behavior for Flexible Pavement’s Unbound Granular Layer. Airfield Highw. Pavements 2015, 2015, 801–812. [Google Scholar]
- Soliman, H.; Shalaby, A. Permanent deformation behavior of unbound granular base materials with varying moisture and fines content. Transp. Geotech. 2015, 4, 1–12. [Google Scholar] [CrossRef]
- Melan, E. Zur plastizitat desraumlichen Kontinuums. Ing. Arch. 1938, 9, 116–125. [Google Scholar] [CrossRef]
- Koiter, W.T. General theorems for elastic-plastic solids. In Progressin Solid Mechanics; Sneddon, I.N., Hill, R., Eds.; North Holland Press: London, UK, 1960; pp. 167–221. [Google Scholar]
- Pham, C. Shakedown theory for elastic plastic kinematic hardening bodies. Int. J. Plast. 2007, 23, 1240–1259. [Google Scholar]
- Głuchowski, A.; Soból, E.; Szymański, A.; Sas, W. Undrained Pore Pressure Development on Cohesive Soil in Triaxial Cyclic Loading. Appl. Sci. 2019, 9, 3821. [Google Scholar] [CrossRef]
- Goldscheider, M.; Gudehus, G. Einige Bodenmechanische Probleme bei Küsten-und Offshore-Bauwerken. In Vorträge der Baugrundtagung; Deutsche Gesellschaft für Erd- und Grundbau: Nurnberg, Germany, 1976; pp. 507–522. [Google Scholar]
- Kenig, K. Litologia glin morenowych na Nizu Polskim-podstawowe metody badawcze. Biul. Panstwowego. Inst. Geol. 2009, 437, 1–57. [Google Scholar]
- Wang, H.-L.; Cui, Y.-J.; Lamas-Lopez, F.; Dupla, J.-C.; Canou, J.; Calon, N.; Saussine, G.; Aimedieu, P.; Chen, R. Effects of inclusion contents on resilient modulus and damping ratio of unsaturated track-bed materials. Can. Geotech. J. 2017, 54, 1672–1681. [Google Scholar] [CrossRef]
- Bajda, M.; Falkowski, T. Badania geotechniczne w ocenie budowy geologicznej fragmentu Skarpy Warszawskiej w rejonie ulicy Tamka. Landf. Anal. 2014, 26, 77–84. [Google Scholar] [CrossRef]
- Rudolph, C.; Bienen, B.; Grabe, J. Effect of variation of the loading direction on the displacement accumulation of large-diameter piles under cyclic lateral loading in sand. Can. Geotech. J. 2014, 51, 1196–1206. [Google Scholar] [CrossRef]
- Seed, H.B.; Martin, P.P.; Lysmer, J. The Generation and Dissipation of Pore Water Pressures during Soil Liquefaction; Report No. UCB/EERC-75/26; Earthquake Engineering Center, University of California: Berkeley, CA, USA, 1975. [Google Scholar]
- Idriss, I.M.; Dobry, R.; Sing, R.D. Nonlinear behavior of soft clays during cyclic loading. J. Geotech. Geoenviron. Eng. 1978, 104, 14265. [Google Scholar]
- Zhou, J.; Gong, X. Strain degradation of saturated clay under cyclic loading. Can. Geotech. J. 2001, 38, 208–212. [Google Scholar] [CrossRef]
- Fang, H.Y. Foundation Engineering Handbook; Springer Science & Business Media: New York, NY, USA, 2013. [Google Scholar]


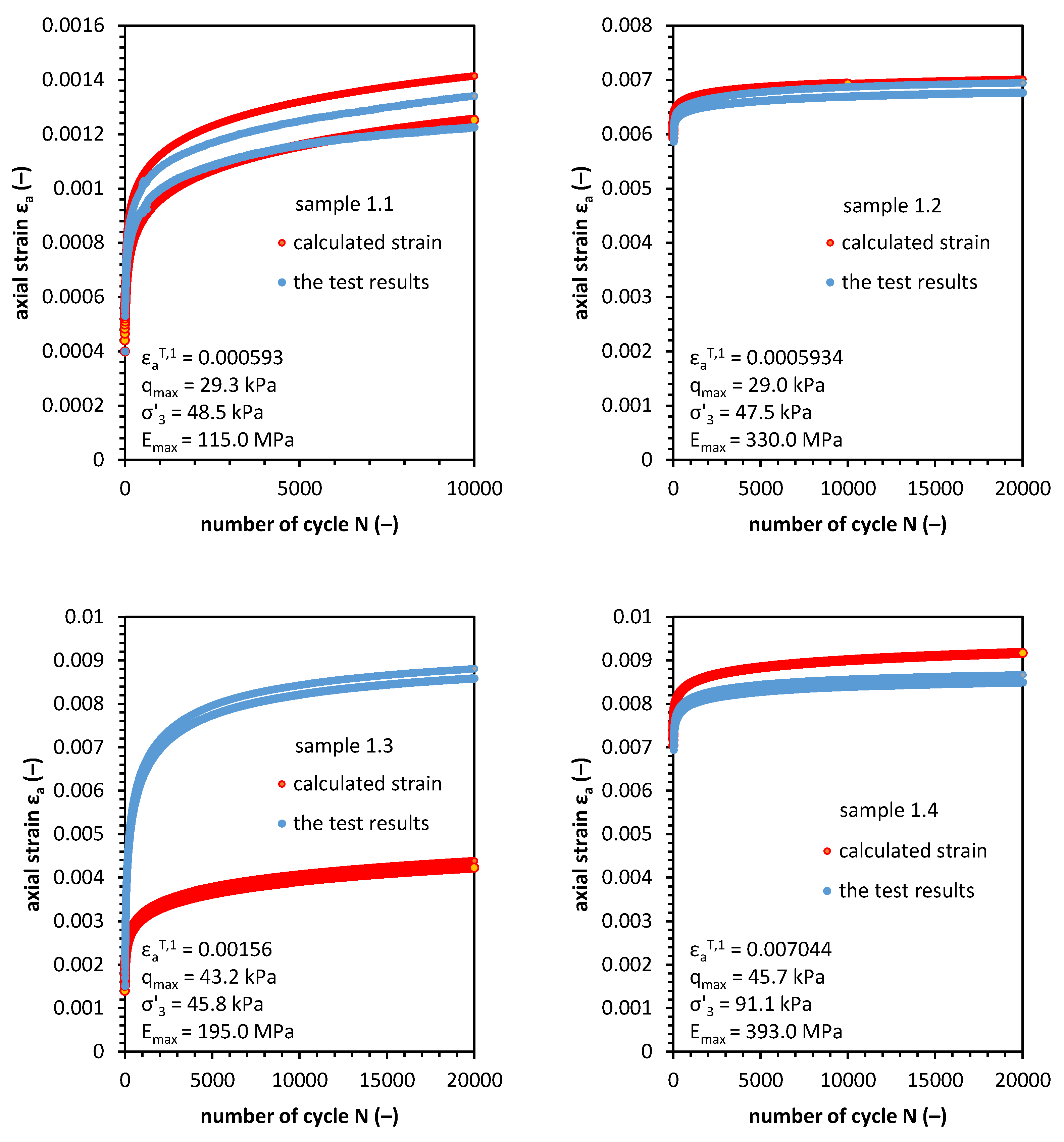
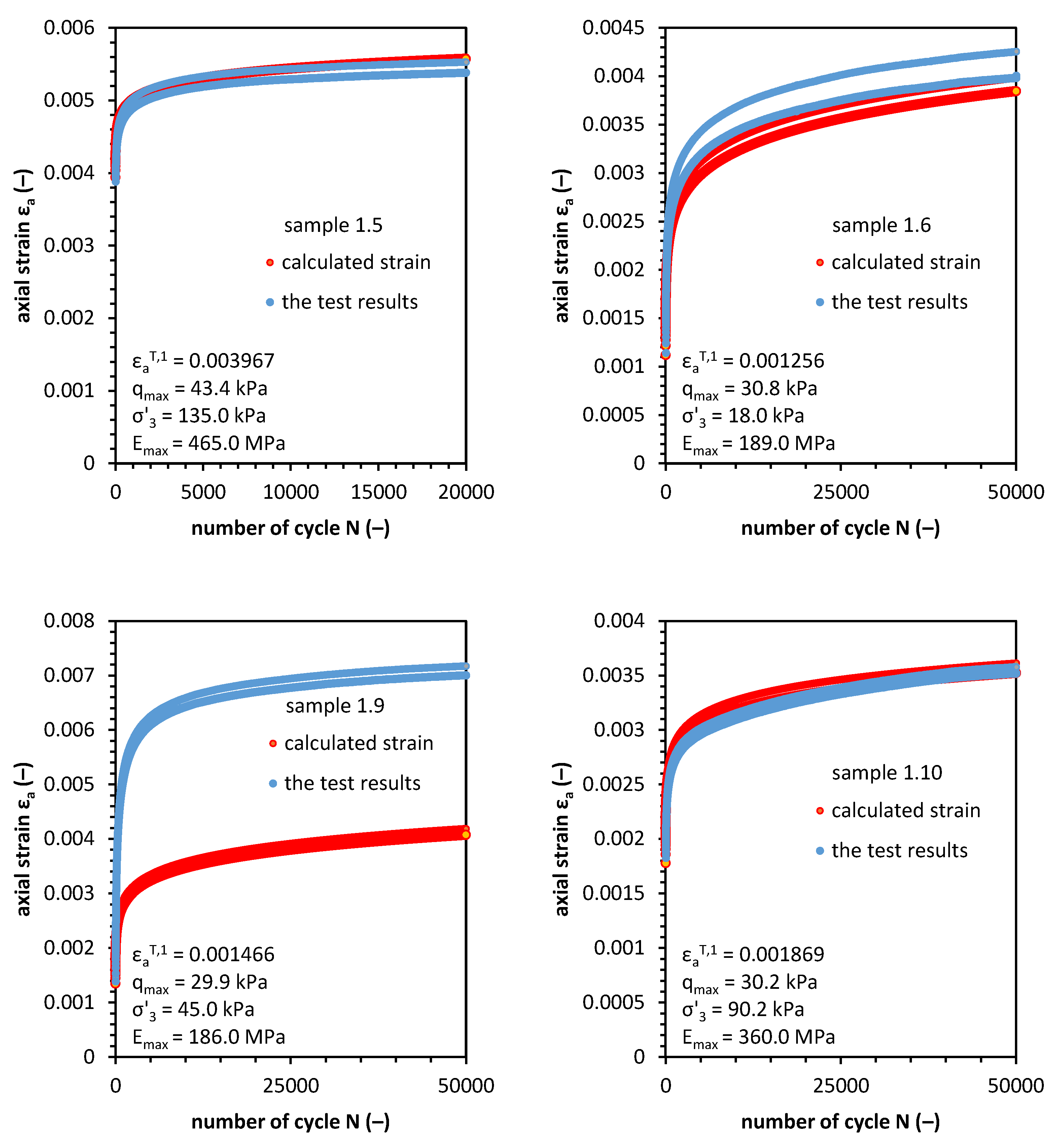
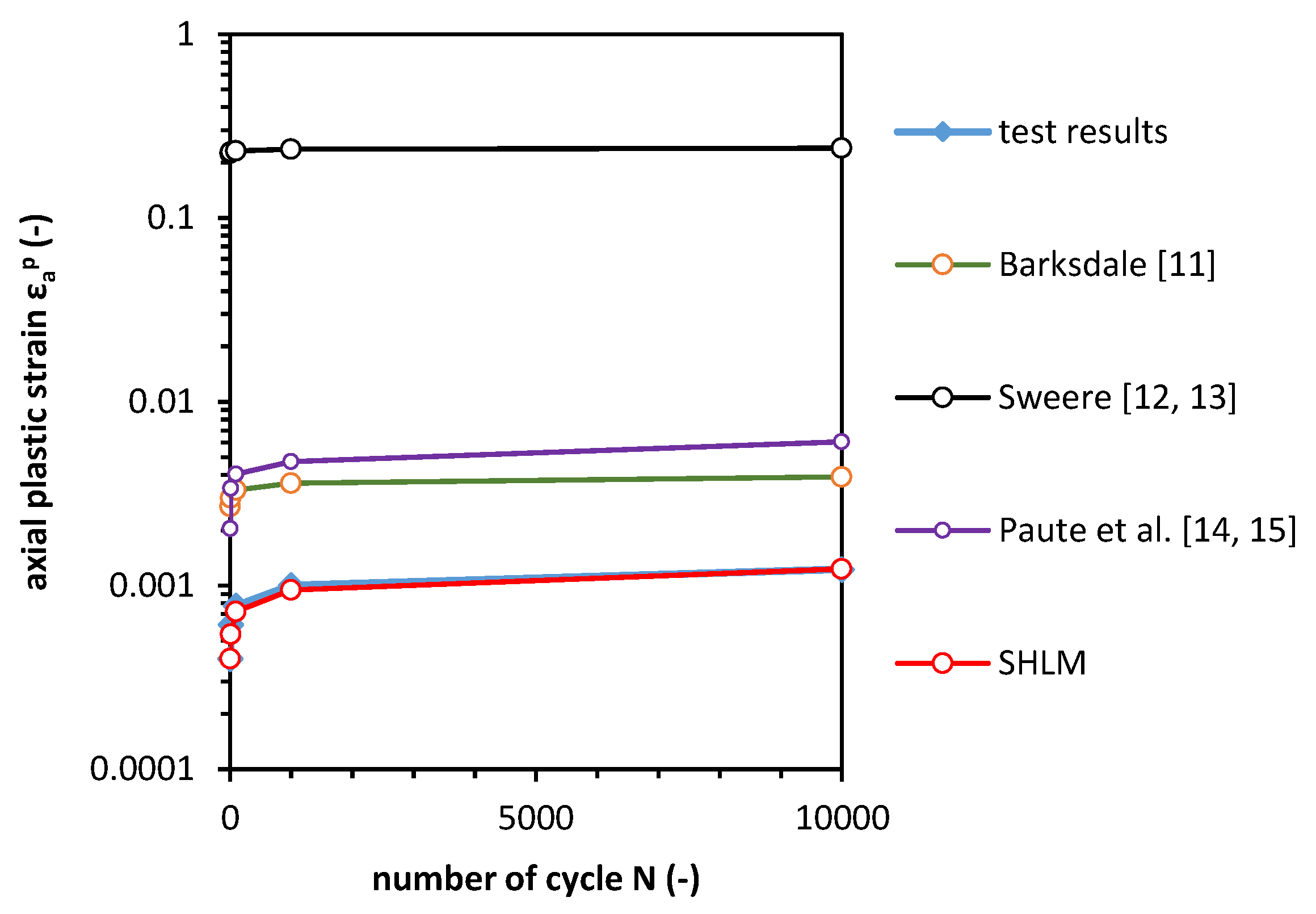
| Soil Sample No | Moisture Content, w (%) | Dry Density, ρd (g/cm3) | Void Ratio, e0 (–) | Shear Modulus, Gmax (MPa) |
|---|---|---|---|---|
| 1.1 | 13.76 | 1.932 | 0.391 | 115.0 |
| 1.2 | 12.81 | 1.972 | 0.358 | 110.0 |
| 1.3 | 13.58 | 1.904 | 0.383 | 65.0 |
| 1.4 | 12.77 | 1.984 | 0.324 | 131.0 |
| 1.5 | 13.58 | 1.948 | 0.366 | 155.0 |
| 1.6 | 13.49 | 1.897 | 0.387 | 63.0 |
| 1.7 | 13.27 | 1.892 | 0.370 | – |
| 1.8 | 13.81 | 1.797 | 0.390 | – |
| 1.9 | 15.53 | 1.841 | 0.332 | 62.0 |
| 1.10 | 16.37 | 1.935 | 0.323 | 120.0 |
| Soil Sample No | Maximal Deviator Stress, qmax (kPa) | Minimal Deviator Stress, qmin (kPa) | Mean Deviator Stress, qm (kPa) | Deviator Stress Amplitude, qa (kPa) | Effective Confining Pressure, σ’3,0 (kPa) | Cyclic Stress Ratio, CSR (–) |
|---|---|---|---|---|---|---|
| 1.1 | 29.3 | 23.7 | 26.5 | 2.8 | 48.5 | 0.603 |
| 1.2 | 29.0 | 23.7 | 26.4 | 2.6 | 47.5 | 0.610 |
| 1.3 | 43.2 | 35.3 | 39.3 | 4.0 | 45.8 | 0.944 |
| 1.4 | 45.7 | 37.4 | 41.5 | 4.2 | 91.1 | 0.502 |
| 1.5 | 43.4 | 35.0 | 39.2 | 4.2 | 135 | 0.321 |
| 1.6 | 30.8 | 22.8 | 26.8 | 4.0 | 18 | 1.710 |
| 1.7 | 28.2 | 23.1 | 25.6 | 2.6 | 46.2 | 0.610 |
| 1.8 | 29.4 | 23.1 | 26.2 | 3.2 | 91.5 | 0.322 |
| 1.9 | 29.9 | 24.5 | 27.2 | 2.7 | 45 | 0.665 |
| 1.10 | 30.2 | 24.5 | 27.4 | 2.9 | 90.2 | 0.335 |
| εaT,10000 Test (–) | εaT,10000 Prognosis (–) | Sample No. | Error (–) | Procentage Error (%) |
|---|---|---|---|---|
| 0.00134 | 0.00139 | 1.1 | −0.00005 | −4.06% |
| 0.00687 | 0.00705 | 1.2 | −0.00018 | −2.62% |
| 0.00844 | 0.00407 | 1.3 | 0.00436 | 51.72% |
| 0.00857 | 0.00889 | 1.4 | −0.00032 | −3.71% |
| 0.00544 | 0.00532 | 1.5 | 0.00012 | 2.21% |
| 0.00368 | 0.00335 | 1.6 | 0.00033 | 9.05% |
| 0.00659 | 0.00364 | 1.9 | 0.00296 | 44.83% |
| 0.00316 | 0.00336 | 1.10 | −0.00020 | 0.00% |
| Index | Value |
|---|---|
| ME | 0.00087 |
| MPE | 12.18% |
| MAE | 0.001066 |
| MAPE | 15.57% |
| I2 | 0.0976 |
| I12 | 21.41% |
| I22 | 1.23% |
| I32 | 76.83% |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Głuchowski, A.; Sas, W. Impact of Cyclic Loading on Shakedown in Cohesive Soils—Simple Hysteresis Loop Model. Appl. Sci. 2020, 10, 2029. https://doi.org/10.3390/app10062029
Głuchowski A, Sas W. Impact of Cyclic Loading on Shakedown in Cohesive Soils—Simple Hysteresis Loop Model. Applied Sciences. 2020; 10(6):2029. https://doi.org/10.3390/app10062029
Chicago/Turabian StyleGłuchowski, Andrzej, and Wojciech Sas. 2020. "Impact of Cyclic Loading on Shakedown in Cohesive Soils—Simple Hysteresis Loop Model" Applied Sciences 10, no. 6: 2029. https://doi.org/10.3390/app10062029
APA StyleGłuchowski, A., & Sas, W. (2020). Impact of Cyclic Loading on Shakedown in Cohesive Soils—Simple Hysteresis Loop Model. Applied Sciences, 10(6), 2029. https://doi.org/10.3390/app10062029







