Assessment of Load Losses Caused by Harmonic Currents in Distribution Transformers Using the Transformer Loss Calculator Software
Abstract
:1. Introduction
2. Materials and Methods
2.1. Transformer Load Losses Adapted from IEEE C57.110 and ANSI/UL 1561-1562 Standards
- are the nominal cooper (Joule) losses, as a function of the ohmic short-circuit resistance of the transformer () and the RMS value of the secondary rated current ();
- are the Rated Winding Eddy Current Losses;
- are the Rated Other Stray Losses;
- are the Rated Total Stray Losses.
2.1.1. Load Losses Adapted from the IEEE Standard C57.110
- is the short-circuit ohmic resistance of the transformer, calculated when direct currents are flowing through the windings of the transformer;
- is the RMS value of the harmonic current of order and phase .
2.1.2. Load Losses Adapted from the ANSI/UL Std. 1561 and 1562
2.2. Transformer Loss Calculator Software
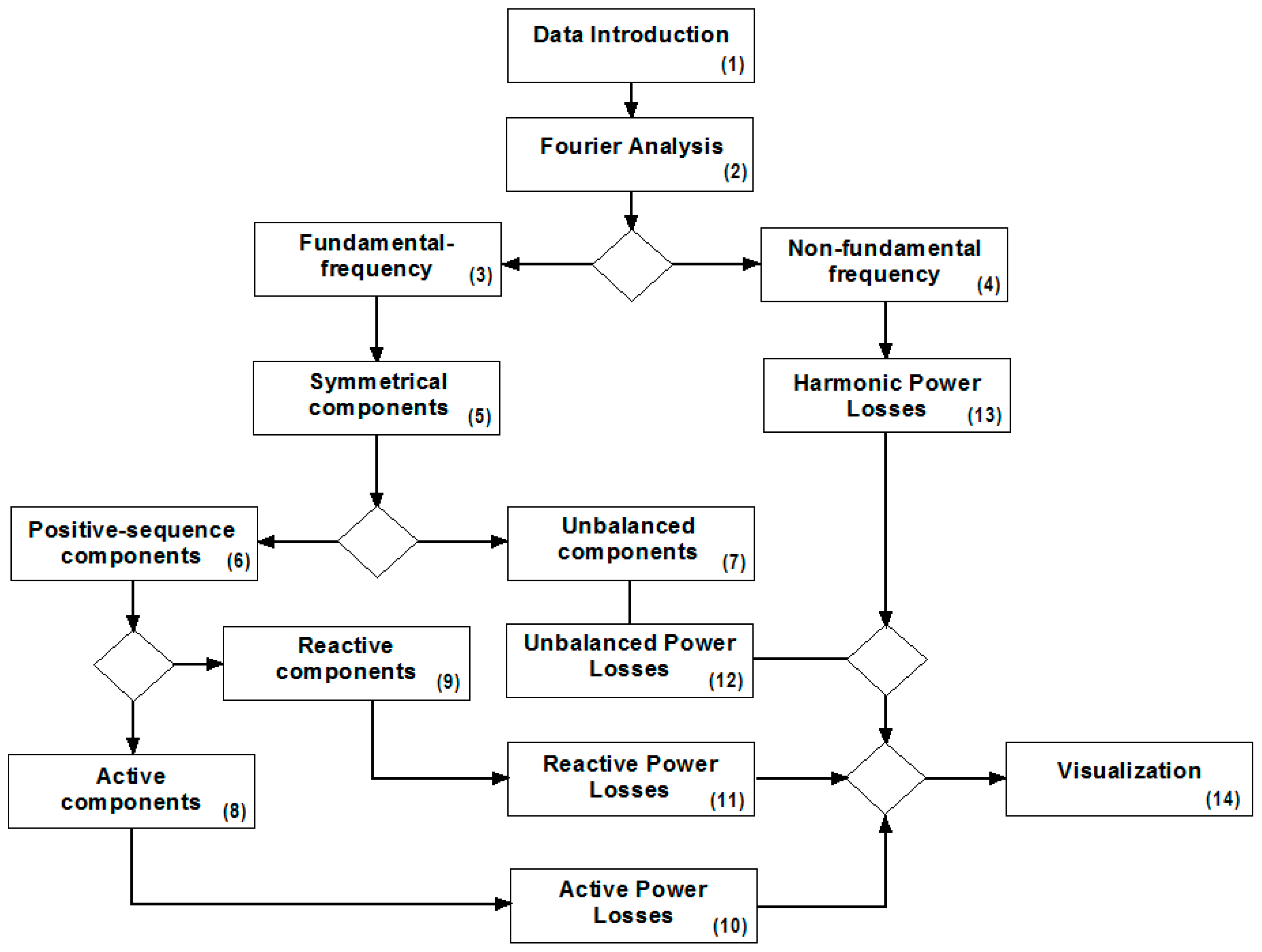
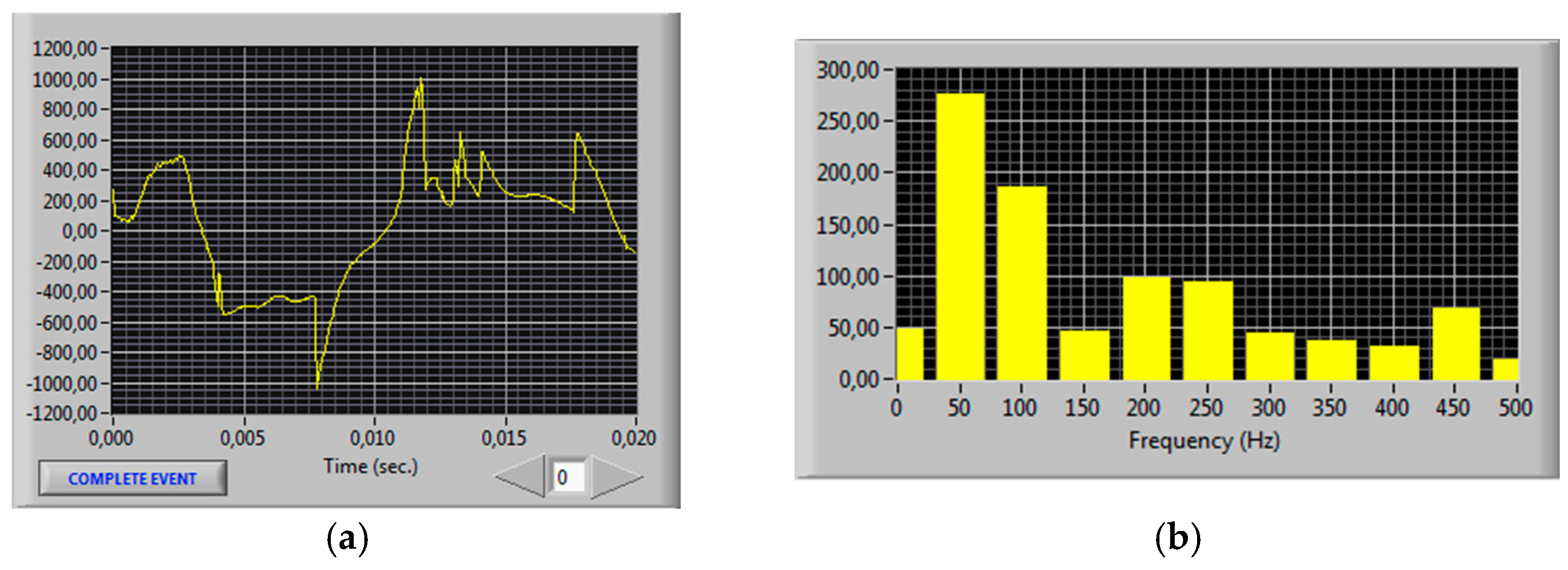
2.2.1. Operational Sequence of the TLC Software
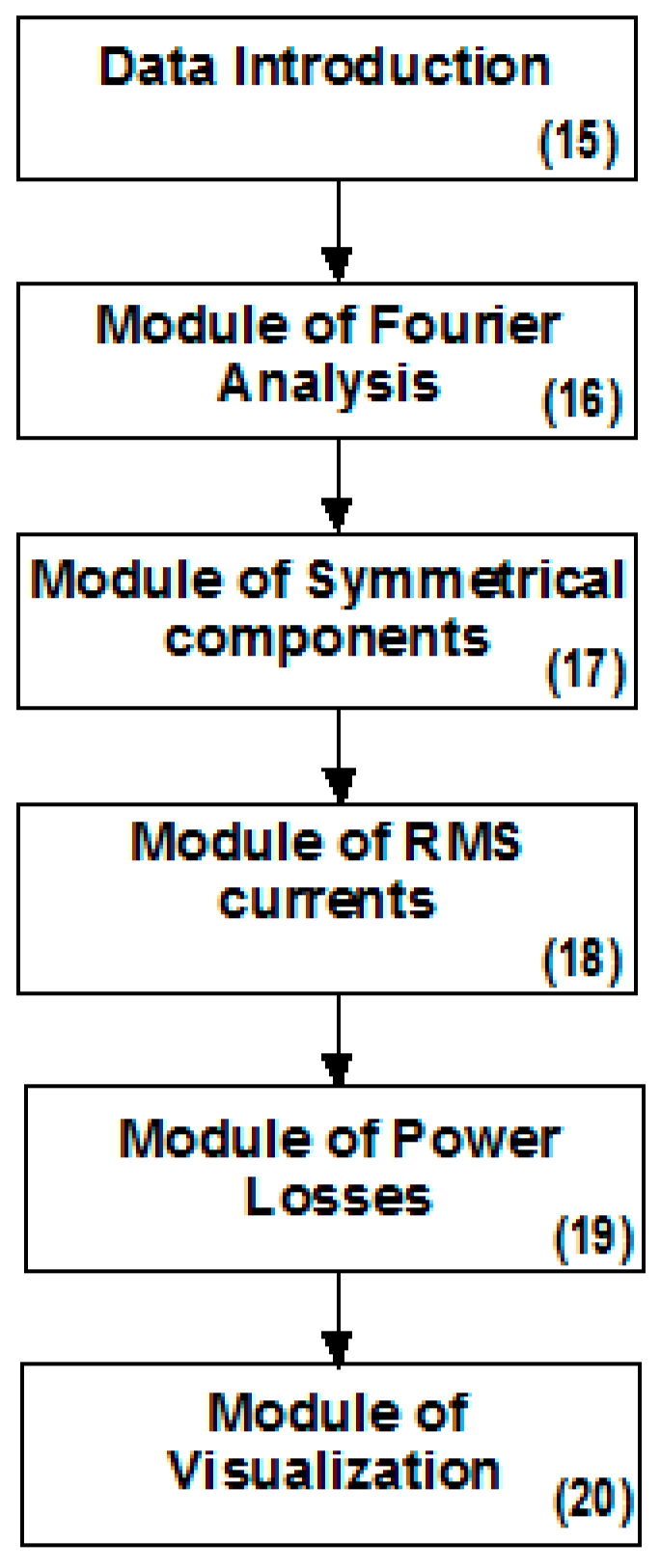
2.2.2. Programing Modules of TLC Software
- Secondary current ():
- Fundamental frequency current () and its components active (), reactive (), and unbalanced ():
- Non-fundamental frequency current ():
- Total load losses:
- Losses due to active currents (),
- Losses due to reactive currents (),
- Losses due to unbalanced currents (),
2.2.3. Load Losses Adapted from Standard ANSI/UL Standard 1561/1562
3. Results
- The first zone, supplied by transformer 1, is eminently residential, made up of dwellings, and has values of , which are not too high in each phase ();
- The second zone, supplied by transformer 2, is also residential but has shops, with cold stores, LED, and discharge lighting, as well as small industrial companies, with high values of in each phase.
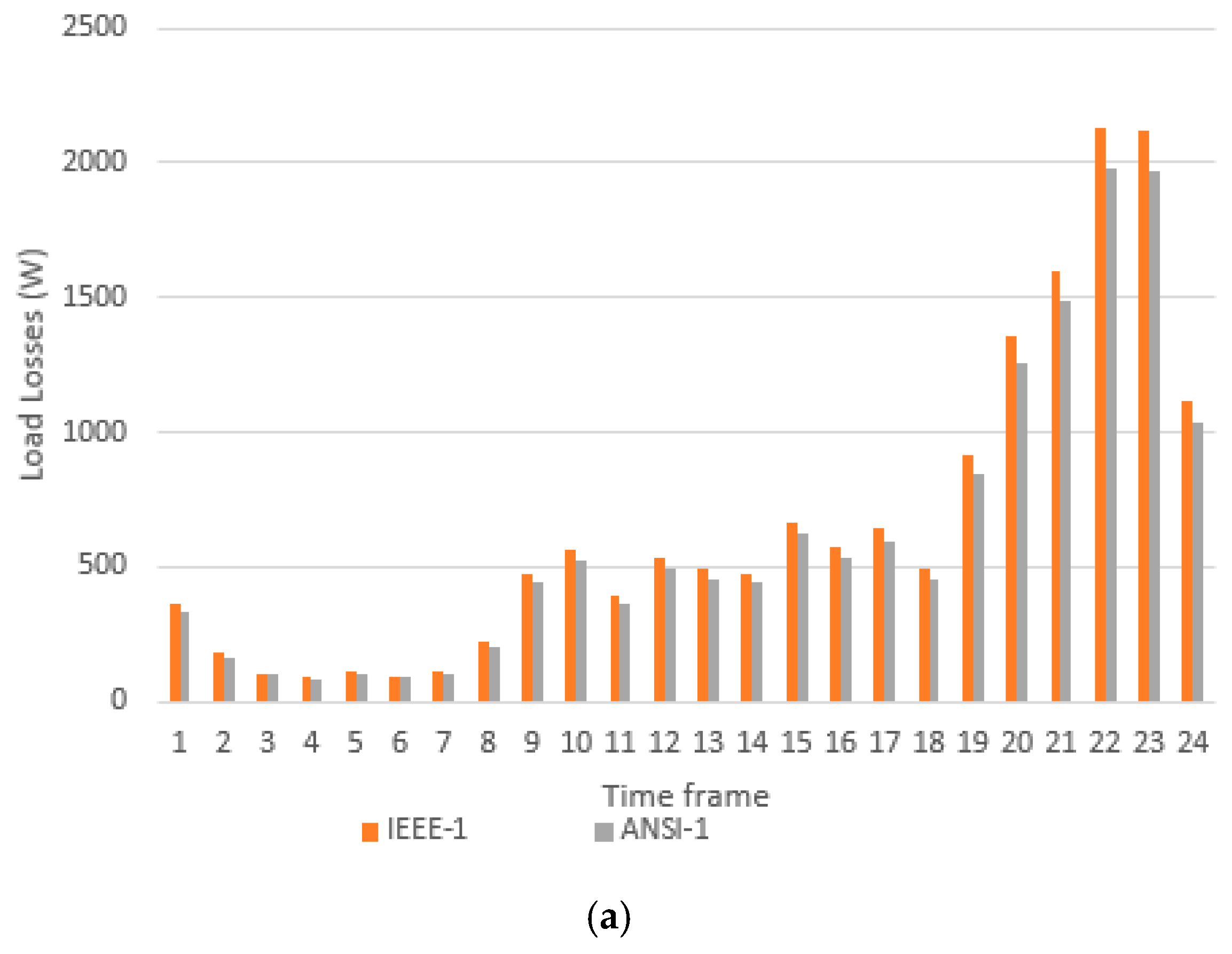
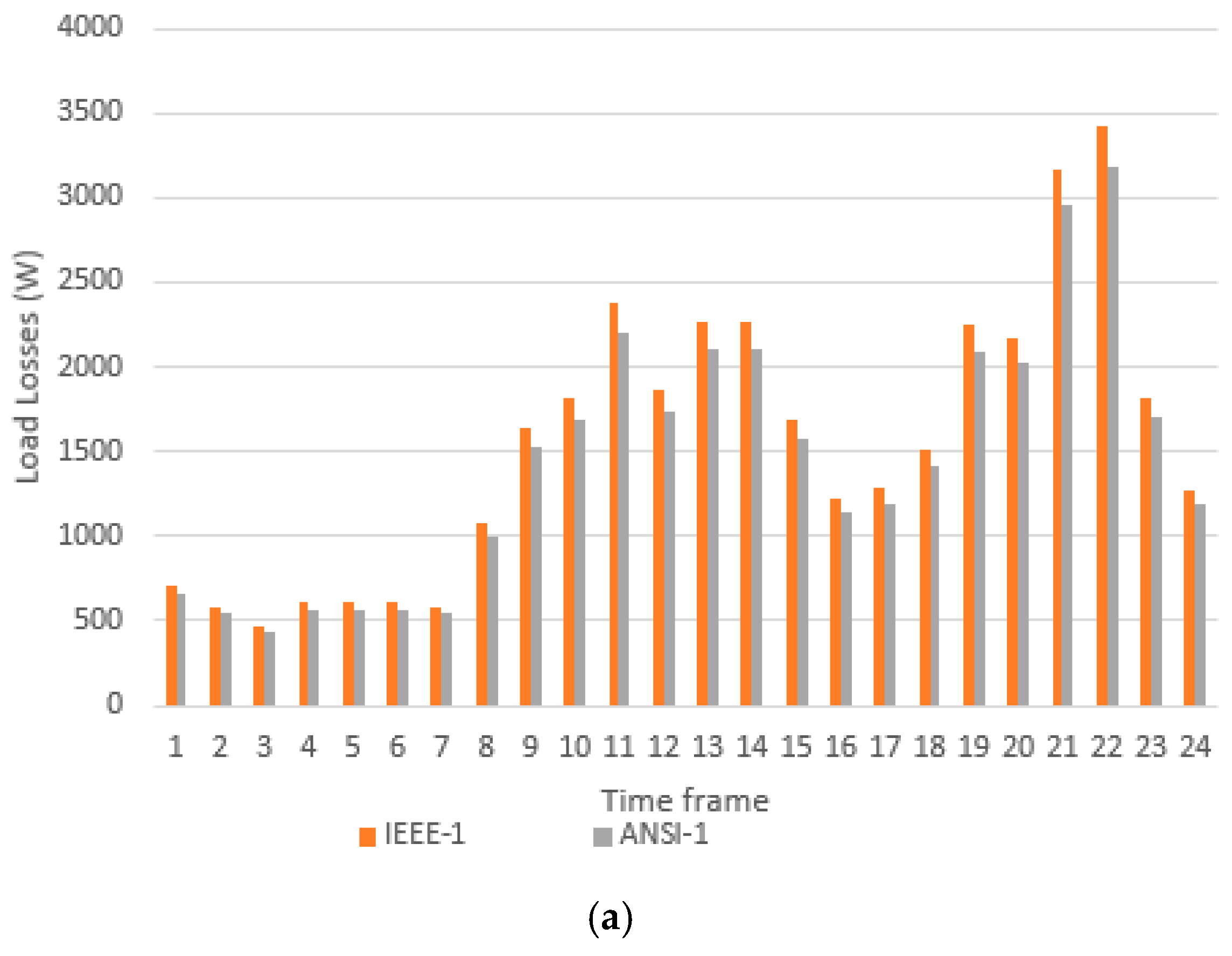
3.1. Losses and Daily CO2 Emissions in Transformer 1
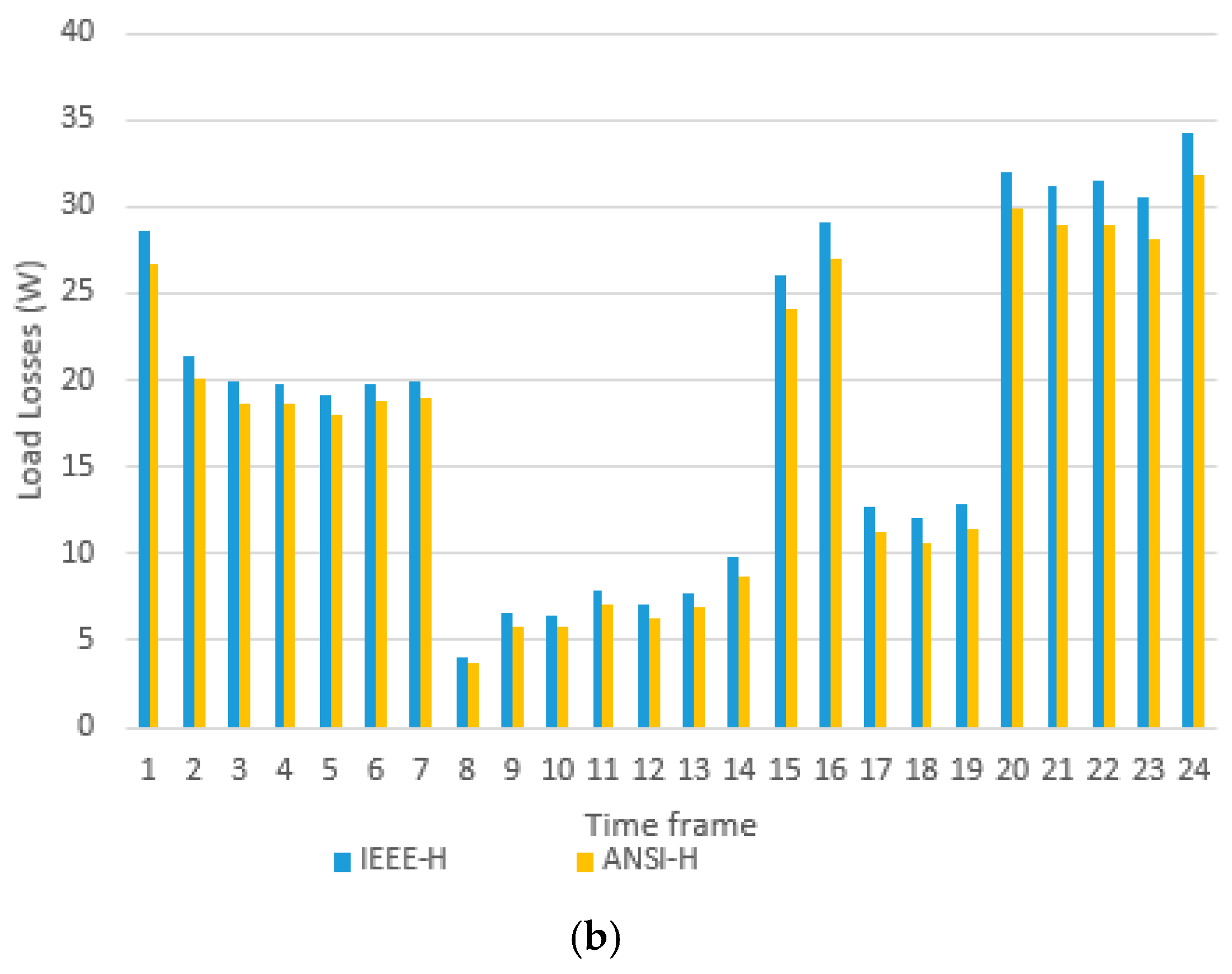
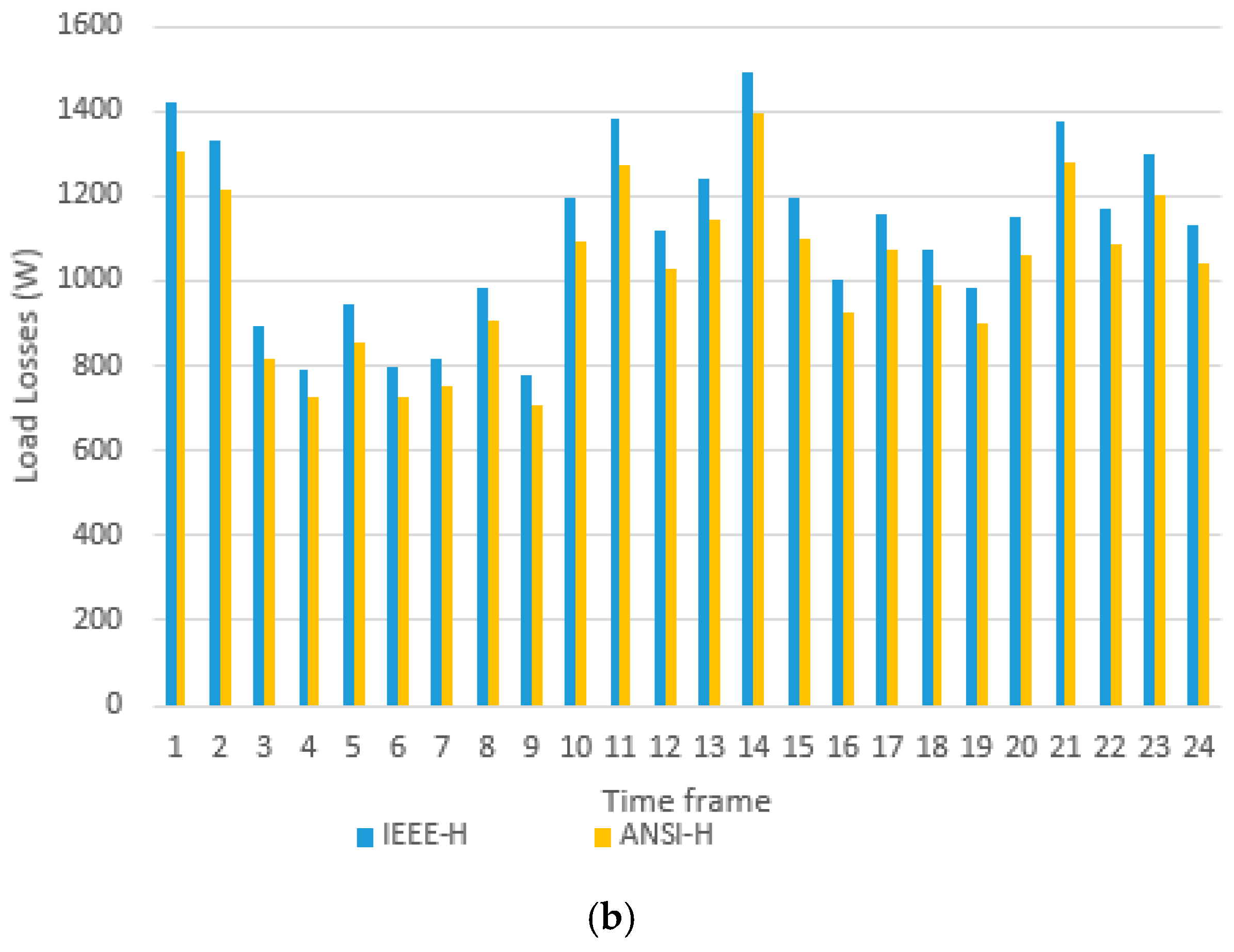
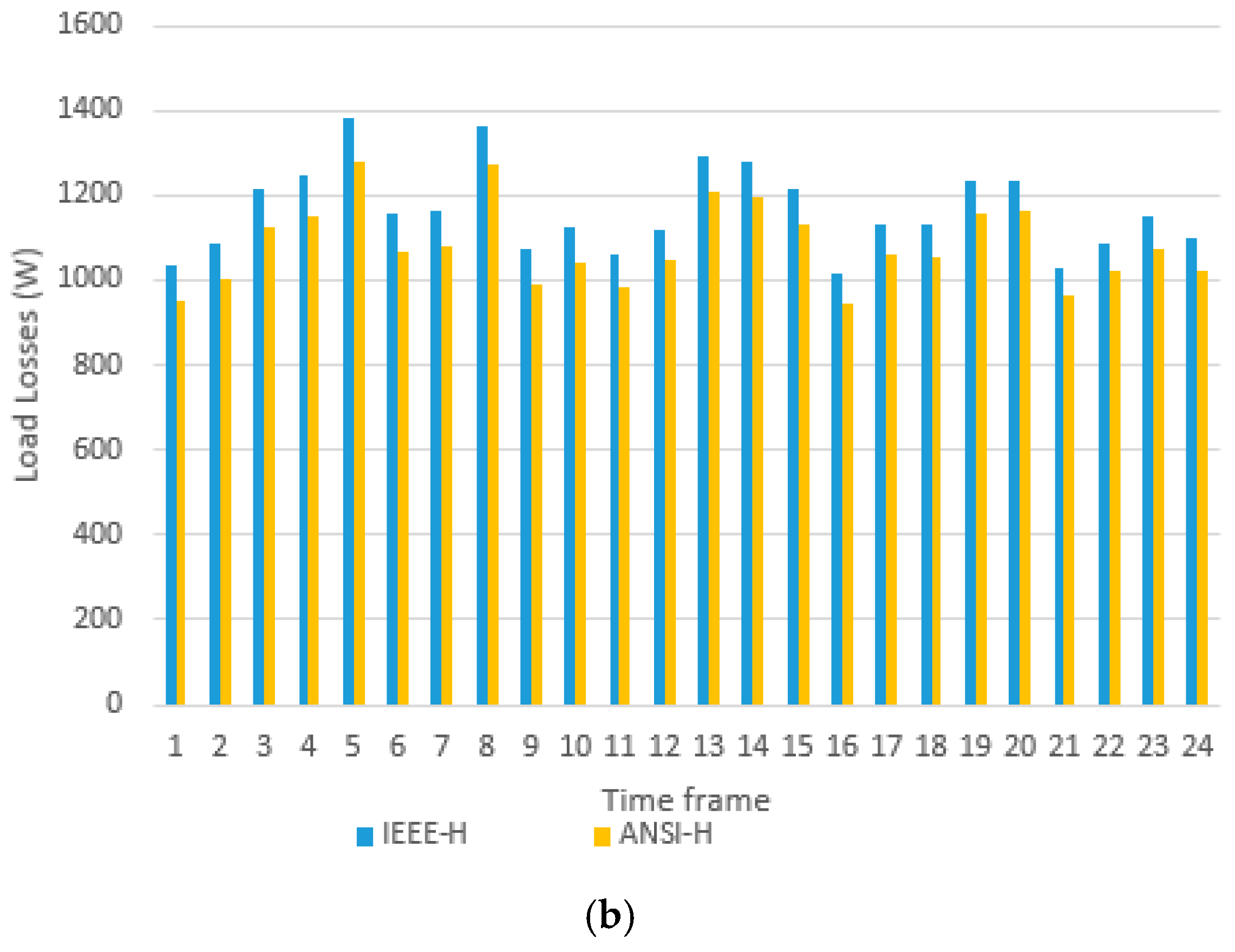
3.2. Losses and Daily CO2 Emissions from Transformer 2
4. Discussion
5. Conclusions
- The values of losses and CO2 emissions obtained according to the IEEE C57.110 standard are always higher than those calculated using the ANSI/UL Standards 1651 and 1562 because the latter standards do not include the effects of Other Stray Losses. However, the differences between the results of those standards are not too large; they are less than 7%, even with a high .
- The current distortion rates (), between 10 and 20%, cause only very slight load losses and CO2 emissions, which are less than 5% of the total.
- The current distortion rates () of 40–60% produce high losses and CO2 emissions, which can be higher than 40% of the total.
Author Contributions
Funding
Conflicts of Interest
References
- U4E. Accelerating the Global Adoption of Energy-Efficient Transformers; UN Environment, Economy Division, Energy & Climate; Branch: Paris, France, 2017. [Google Scholar]
- Biryulin, V.I.; Gorlov, A.N.; Larin, O.M.; Kudelina, D.V. Calculation of power losses in the transformer substation. In Proceedings of the 13th International Scientific-Technical Conference on Actual Problems of Electronics Instrument Engineering (APEIE), Novosibirsk, Russia, 3–6 October 2016. [Google Scholar] [CrossRef]
- Mahrous, A.T.; Salah, K.; Ziad, M.A. K-Factor and transformer losses calculations under harmonics. In Proceedings of the 2016 Eighteenth International Middle East Power Systems Conference (MEPCON), Cairo, Egypt, 27–29 December 2016. [Google Scholar] [CrossRef]
- Contreras-Ramirez, D.; Lata-García, J. K-Factor Analysis to Increase the Actual Capacity of Electrical Distribution Transformers. In Springer Communication, Smart Technologies and Innovation for Society; Springer: Singapore, 2021; pp. 367–379. [Google Scholar] [CrossRef]
- Elmoudi, A.; Lehtonen, M.; Nordman, H. Effect of harmonics on transformers loss of life. In Proceedings of the 2006 IEEE International Symposium on Electrical Insulation, Toronto, ON, Canada, 11–14 June 2006. [Google Scholar] [CrossRef]
- Gouda, O.E.; Amer, G.M.; Salem, W.A.A. Predicting transformer temperature rise and loss of life in the presence of harmonic load currents. Ain Shams Eng. J. 2012, 3, 113–121. [Google Scholar] [CrossRef]
- Godina, R.; Rodrigues, E.M.G.; Matias, J.C.O.; Catalão, J.P.S. Effect of Loads and Other Key Factors on Oil-Transformer Aging: Sustainability Benefits and Challenges. Energies 2015, 8, 12147–12186. [Google Scholar] [CrossRef]
- Mushtaq, I.H. Improving the cooling performance of electrical distribution transformer using transformer oil—Based MEPCM suspension. Eng. Sci. Technol. 2017, 20, 502–510. [Google Scholar] [CrossRef]
- Mikhak-Beyranvand, M.; Faiz, J.; Rezaeealam, B. Thermal analysis and derating of a power transformer with harmonic loads. IET Gener. Transm. Distrib. 2020, 14, 1233–1241. [Google Scholar] [CrossRef]
- European Cooper Institute. Coppers Contribution to a Low Carbon Future. A Plan to Decarbonize Europe by 25 Percent. 2018. Available online: https://copperalliance.sk/uploads/2018/05/coppers-contribution-to-a-low-carbon-future-a-plan-to-decarbonise-europe-by-25-percent.pdf (accessed on 1 March 2022).
- León-Martínez, V.; Andrada-Monrós, C.; Molina-Cañamero, L.; Cano-Martínez, J.; Peñalvo-López, E. Decarbonization of Distribution Transformers Based on Current Reduction: Economic and Environmental Impacts. Energies 2021, 21, 7207. [Google Scholar] [CrossRef]
- León-Martínez, V.; Peñalvo-López, E.; Andrada-Monrós, C.; Cano-Martínez, J.; León-Vinet, A.; Molina-Cañamero, L. Procedure for Improving the Energy, Environmental and Economic Sustainability of Transformation Houses. Appl. Sci. 2022, 9, 4204. [Google Scholar] [CrossRef]
- Energy Data from the Valencian Community; Valencian Energy Institute (IVACE), Sustainable Economy Valencian Community Ministry: Valencia, Spain, 2020. (In Spanish)
- IEA. Emission Factors 2022. International Energy Agency (IEA). Available online: https://www.iea.org/data-and-statistics/data-product/emissions-factors-2022 (accessed on 1 March 2022).
- EPA. Greenhouse Gas Equivalences Calculator; US Environmental Protection Agency: Washington, DC, USA, 2023. Available online: https//www.energy-and-the-environment/greenhouse-gas-equivalency-calculator (accessed on 29 August 2023).
- Thango, B.A.; Jordan, J.A.; Nnachi, A.F. A Novel Approach for Evaluating Eddy Current Loss in Wind Turbine Generator Step-Up Transformers. Adv. Sci. Technol. Eng. Syst. 2021, 6, 488–498. [Google Scholar] [CrossRef]
- Thango, B.A.; Akuru, U.B.; Nnachi, A.F. Corrected Estimation of the Transformer Winding Eddy Losses for Utility-Scale Solar Photovoltaic Plant Application. In Proceedings of the 2021 International Conference on Electrical, Computer and Energy Technologies (ICECET), Cape Town, South Africa, 9–10 December 2021. [Google Scholar] [CrossRef]
- Jakus, D.; Vasilj, J.; Čađenović, R.; Sarajčev, P. Optimising the transformer substation topology in order to minimise annual energy losses. IET Gener. Transm. Distrib. 2018, 12, 1323–1330. [Google Scholar] [CrossRef]
- Alame, D.; Azzouz, M.; Kar, N.C. Impact Assessment of Electric Vehicle Charging on Distribution Transformers Including State-of-Charge. In Proceedings of the 2018 IEEE 61st International Midwest Symposium on Circuits and Systems (MWSCAS), Windsor, ON, Canada, 5–8 August 2018. [Google Scholar] [CrossRef]
- Yaghoobi, J.; Alduraibi, A.; Martin, D.; Zare, F.; Eghbal, D.; Memisevic, R. Impact of high-frequency harmonics (0–9 kHz) generated by grid-connected inverters on distribution transformers. Int. J. Electr. Power Energy Syst. 2020, 122, 106177. [Google Scholar] [CrossRef]
- Megahed, T.F.; Kotb, M.F. Improved design of LED lamp circuit to enhance distribution transformer capability based on a comparative study of various standards. Energy Rep. 2022, 8, 445–465. [Google Scholar] [CrossRef]
- Chen, H.; Huang, Y.; Hu, H.; Wang, J.; Wang, W. Analysis of the influence of voltage harmonics on the maximum load capacity of the power supply transformer for the LHCD system. In Proceedings of the 2023 IEEE 6th International Electrical and Energy Conference (CIEEC), Hefei, China, 12–14 May 2023. [Google Scholar] [CrossRef]
- Sima, L.; Miteva, N.; Dagan, K.J. A novel approach to power loss calculation for power transformers supplying nonlinear loads. Electr. Power Syst. Res. 2023, 223, 109582. [Google Scholar] [CrossRef]
- IEEE Std C57.110™-2018; (Revision of IEEE Std C57.110-2008); IEEE Recommended Practice for Establishing Liquid Immersed and Dry-Type Power and Distribution. Transformer Capability When Supplying Nonsinusoidal Load Currents. Transformers Committee of the IEEE Power and Energy Society: New York, NY, USA, 2018; pp. 1–68. [CrossRef]
- Laso-Perez, A.; Martinez-Torre, R.; Morning-Canteli, M.; Cervero-García, D.; Sáez-Castro, J.A. A comparative between IEEE and EN in the transformer derating when supplying nonsinusoidal load current. A practical case. Renew. Energy Power Qual. J. (REPQJ) 2020, 18, 747–752. [Google Scholar] [CrossRef]
- ANSI/UL Standard 1561; Standard for Dry-Type General Purpose and Power Transformers. Ed 4, March 2, 2011, ANSI Approved May 13. Underwriters Laboratories Inc. (UL): Northbrook IL, USA, 2019.
- ANSI/UL Standard 1562; Transformers, Distribution, Dry-Type, Over 600 V. Ed 4, January 25, 2013, Revised August 11. Underwriters Laboratories Inc. (UL): Northbrook IL, USA, 2020.
- Deshpande, K.; Holmukhe, R.; Angal, Y.; Patil, Y. K-Factor Transformers and Non-linear Loads. Available online: https://powerquality.blog/2021/11/24/k-factor-transformers-and-non-linear-loads/ (accessed on 18 July 2023).
- ABB. Loading Transformers with Non-Sinusoidal Currents. K-Factor. ABB, 1LES100070-ZB–Rev. 1. pp. 1–7. Available online: https://library.e.abb.com/public/be0cadaf6a6708fcc1257792005162cb/Loading_transformers_with_non_sinusoidal_currents_KFactor.pdf (accessed on 14 June 2023).
- Fortescue, C.L. Method of symmetrical coordinates applied to solution of poly-phase networks. IEEE Trans. Am. Inst. Electr. Eng. 1918, XXXVII, 1027–1140. [Google Scholar] [CrossRef]
- C57.12.90™-2006 IEEE; Standard Test Code for Liquid-Immersed Distribution, Power, and Regulating Transformers. The Institute of Electrical and Electronics Engineers: New York, NY, USA, 2009.
- González, I.; Calderón, A.J. Integration of open source hardware Arduino platform in automation systems applied to Smart Grids/Micro-Grids. Sustain. Energy Technol. Assess. 2019, 36, 100557. [Google Scholar] [CrossRef]
- Leena, N.; Durai Raj, B.; Gunabalan, R. Computer-based laboratory teaching tools: An overview of LabVIEW and MATLAB. In Proceedings of the IEEE International Conference on Engineering Education: Innovative Practices and Future Trends (AICERA), Kottayam, India, 19–21 July 2012. [Google Scholar] [CrossRef]
- Nandagopal, V.; Maheswari, V.; Kannan, C. Newly Constructed Real Time ECG Monitoring System Using LabView. Sci. Res. Circuits Syst. 2016, 7, 4227–4235. [Google Scholar] [CrossRef]
- Schmidt, E.; Akopian, D.; Pack, D.J. Development of a Real-Time Software-Defined GPS Receiver in a LabVIEW-Based Instrumentation Environment. IEEE Trans. Instrum. Meas. 2018, 67, 2082–2096. [Google Scholar] [CrossRef]
- Pavithra, G.; Rao, V.V. Remote Monitoring and Control of VFD fed Three Phase Induction Motor with PLC and LabVIEW software. In Proceedings of the 2nd International Conference on I-SMAC (IoT in Social, Mobile, Analytics and Cloud), Palladam, India, 30–31 August 2018. [Google Scholar] [CrossRef]
- Rincón-Quintero, A.D.; Del Portillo-Valdés, L.A.; Meneses-Jácome, A.; Ascanio-Villabona, J.G.; Tarazona-Romero, B.E.; Durán-Sarmiento, M.A. Performance Evaluation and Effectiveness of a Solar-Biomass Hybrid Dryer for Drying Homogeneous of Cocoa Beans Using LabView Software and Arduino Hardware. In Proceedings of the International Conference on Intelligent Information Technology, Ho Chi Minh, Vietnam, 25–28 February 2021; pp. 238–252. [Google Scholar] [CrossRef]
- Villacorta, J.J.; del-Val, L.; Martínez, R.D.; Balmori, J.-A.; Magdaleno, A.; López, G.; Izquierdo, A.; Lorenzana, A.; Basterra, L.-A. Design and Validation of a Scalable, Reconfigurable and Low-Cost Structural Health Monitoring System. Sensors 2021, 21, 648. [Google Scholar] [CrossRef]
- Sivaranjani, S.; Velmurugan, S.; Kathiresan, K.; Karthik, M.; Gunapriya, B.; Gokul, C.; Suresh, M. Visualization of virtual environment through labVIEW platform. Materals Today Proc. 2021, 45, 2306–2312. [Google Scholar] [CrossRef]

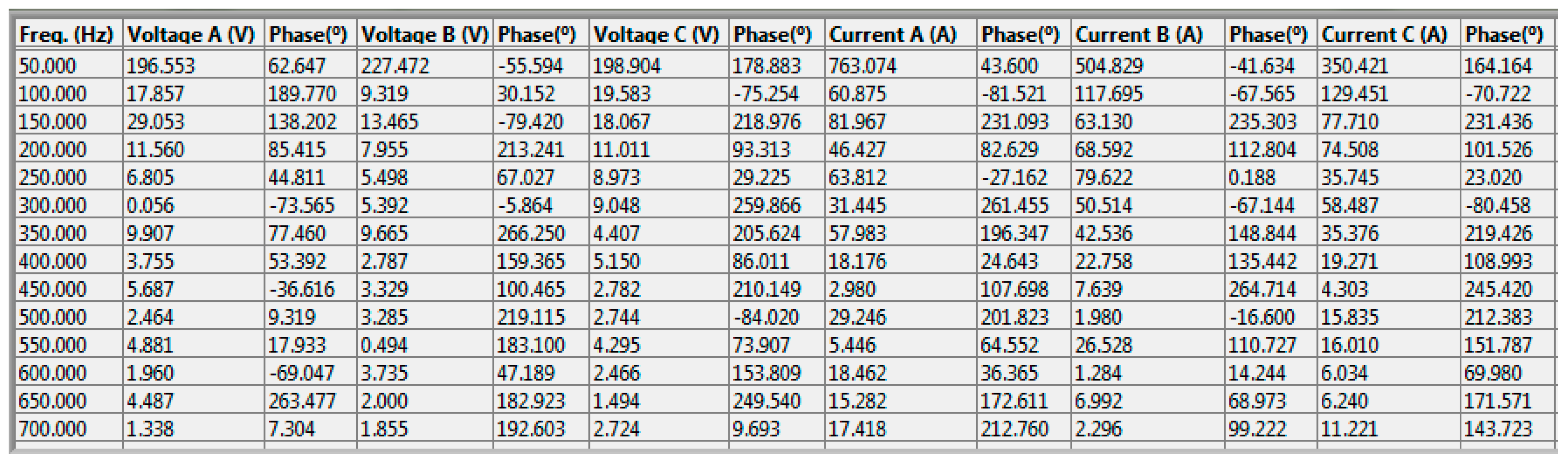
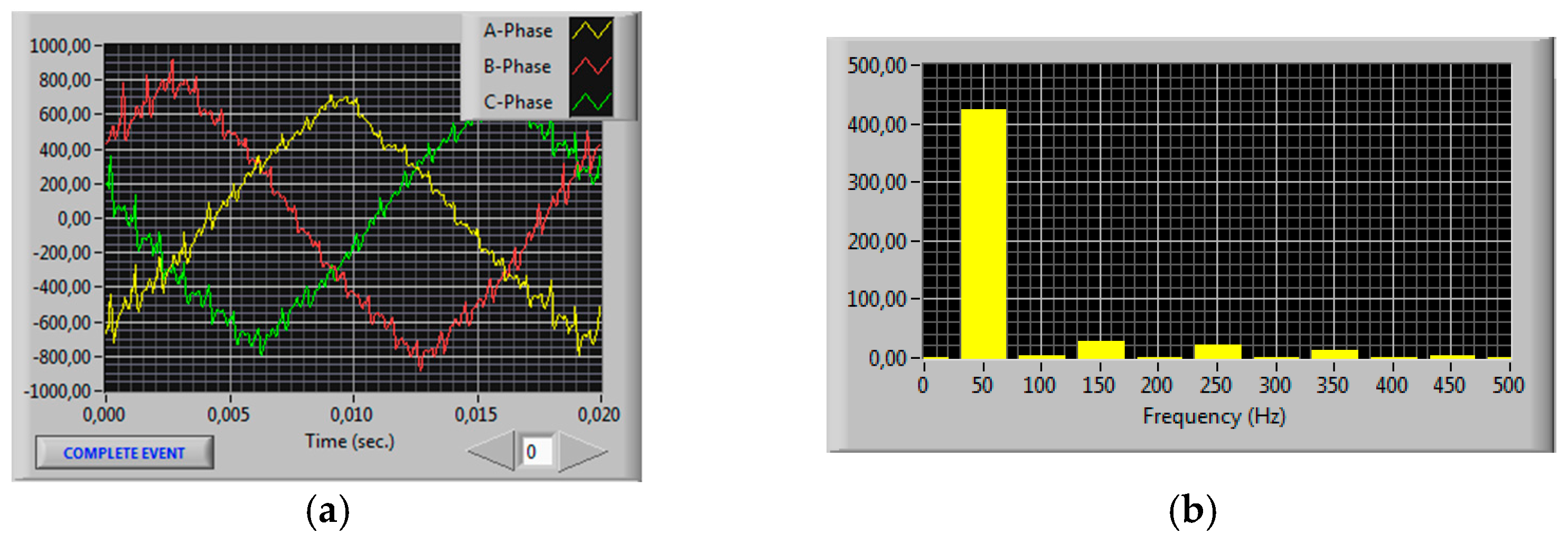



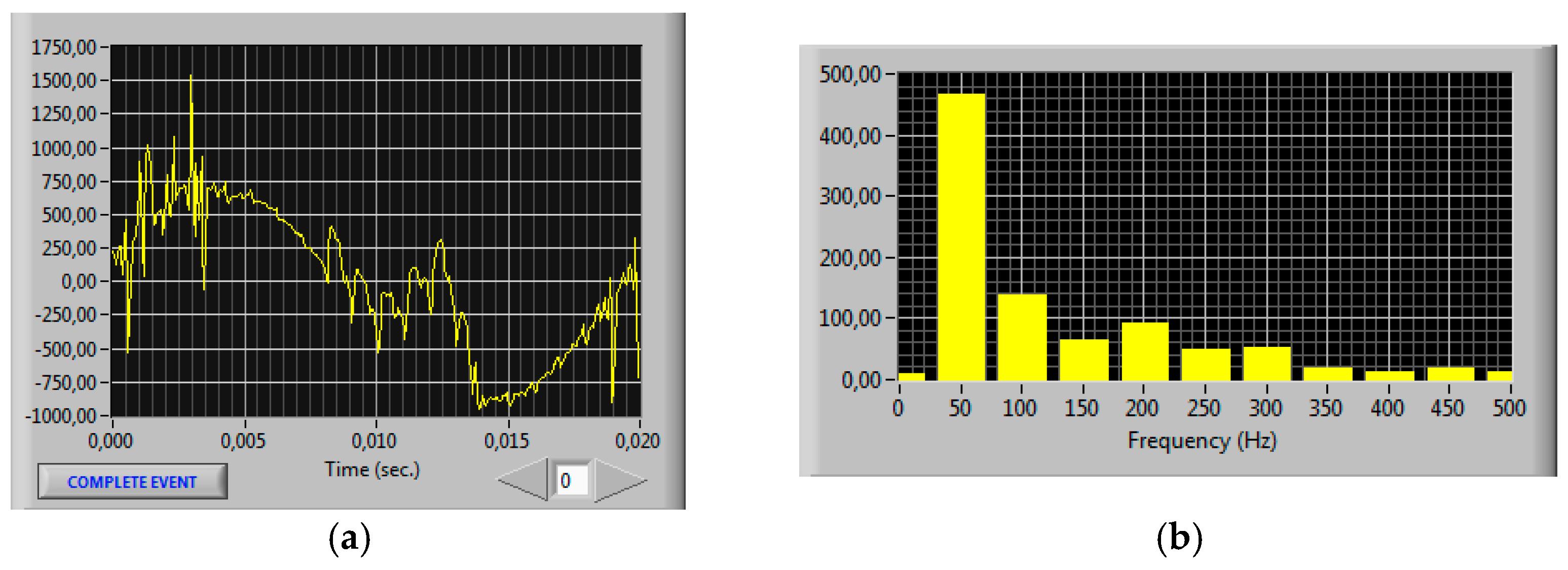
| POWER (kVA) | (W) | (W) | (W) | Line-to-Line Vacuum Voltage (V) |
|---|---|---|---|---|
| 1000 | 10,500 | 420 | 818 | 420 |
| Time Frame | Time | Fundamental Currents (A) | THD% | ||||
|---|---|---|---|---|---|---|---|
| Phase A | Phase B | Phase C | Phase A | Phase B | Phase C | ||
| 1 | 0:02 | 380.036 | 280.334 | 349.042 | 10.58 | 14.53 | 12.29 |
| 2 | 1:02 | 260.92 | 229.703 | 230.069 | 13.68 | 16.18 | 16.64 |
| 3 | 2:02 | 191.006 | 190.796 | 177.276 | 16.32 | 18.19 | 18.77 |
| 4 | 3:02 | 167.664 | 144.969 | 137.565 | 17.01 | 21.93 | 23.17 |
| 5 | 4:02 | 130.938 | 111.515 | 123.03 | 21.14 | 27.47 | 25.04 |
| 6 | 5:02 | 150.915 | 130.791 | 121.391 | 16.67 | 21.54 | 23.43 |
| 7 | 6:02 | 133.478 | 112.819 | 122.518 | 18.72 | 25.78 | 23.47 |
| 8 | 7:02 | 142.586 | 102.358 | 129.976 | 17.06 | 26.33 | 21.24 |
| 9 | 8:02 | 140.924 | 172.741 | 125.513 | 17.94 | 18.14 | 23.96 |
| 10 | 9:02 | 217.398 | 189.012 | 217.198 | 14.59 | 15.43 | 13.99 |
| 11 | 10:02 | 343.127 | 223.374 | 282.705 | 9.47 | 14.83 | 11.70 |
| 12 | 11:02 | 349.679 | 276.687 | 320.302 | 9.70 | 12.69 | 11.78 |
| 13 | 12:02 | 354.129 | 335.705 | 269.704 | 9.55 | 11.14 | 13.14 |
| 14 | 13:02 | 364.809 | 312.967 | 258.381 | 11.02 | 13.95 | 14.90 |
| 15 | 14:02 | 374.324 | 337.248 | 272.889 | 11.00 | 11.71 | 14.24 |
| 16 | 15:02 | 272.626 | 276.445 | 245.403 | 15.66 | 14.48 | 18.24 |
| 17 | 16:02 | 322.359 | 256.481 | 189.705 | 13.86 | 17.37 | 24.20 |
| 18 | 17:02 | 287.144 | 261.985 | 241.365 | 15.07 | 19.05 | 19.02 |
| 19 | 18:02 | 339.659 | 315.582 | 346.154 | 14.17 | 16.39 | 14.98 |
| 20 | 19:02 | 383.66 | 329.891 | 369.324 | 13.08 | 15.36 | 14.11 |
| 21 | 20:02 | 422.833 | 502.692 | 447.894 | 12.73 | 10.76 | 11.90 |
| 22 | 21:02 | 580.505 | 541.733 | 464.959 | 9.56 | 10.73 | 12.07 |
| 23 | 22:02 | 564.522 | 487.523 | 429.691 | 9.52 | 11.45 | 13.36 |
| 24 | 23:02 | 380.785 | 371.051 | 320.873 | 12.37 | 13.17 | 15.75 |
| Time Frame | Time | Fundamental Currents (A) | THD% | ||||
|---|---|---|---|---|---|---|---|
| Phase A | Phase B | Phase C | Phase A | Phase B | Phase C | ||
| 1 | 0:02 | 256.527 | 223.284 | 244.489 | 15.86 | 18.59 | 16.49 |
| 2 | 1:02 | 163.891 | 181.419 | 162.51 | 19.41 | 20.05 | 22.58 |
| 3 | 2:02 | 140.102 | 124.302 | 126.969 | 21.48 | 27.44 | 27.15 |
| 4 | 3:02 | 140.065 | 112.912 | 107.147 | 10.19 | 28.01 | 30.12 |
| 5 | 4:02 | 150.183 | 132.856 | 117.545 | 18.23 | 22.73 | 26.44 |
| 6 | 5:02 | 147.487 | 97.322 | 121.537 | 17.77 | 29.97 | 24.79 |
| 7 | 6:02 | 143.68 | 117.637 | 134.975 | 18.38 | 24.90 | 22.51 |
| 8 | 7:02 | 198.247 | 147.379 | 212.268 | 12.96 | 19.87 | 13.86 |
| 9 | 8:02 | 297.828 | 279.307 | 250.466 | 10.04 | 10.82 | 13.06 |
| 10 | 9:02 | 318.37 | 290.044 | 292.534 | 8.09 | 10.37 | 11.28 |
| 11 | 10:02 | 256.716 | 265.426 | 229.31 | 10.34 | 13.32 | 14.81 |
| 12 | 11:02 | 321.838 | 256.139 | 300.275 | 9.33 | 13.47 | 10.86 |
| 13 | 12:02 | 259.129 | 243.364 | 332.616 | 12.24 | 14.12 | 10.28 |
| 14 | 13:02 | 298.438 | 239.186 | 288.279 | 11.35 | 15.29 | 12.24 |
| 15 | 14:02 | 375.407 | 262.883 | 334.748 | 11.44 | 15.18 | 12.06 |
| 16 | 15:02 | 245.77 | 318.558 | 338.143 | 16.15 | 13.08 | 12.65 |
| 17 | 16:02 | 298.109 | 321.629 | 343.467 | 12.56 | 12.78 | 12.62 |
| 18 | 17:02 | 256.703 | 263.249 | 318.419 | 14.38 | 14.56 | 13.10 |
| 19 | 18:02 | 356.648 | 391.825 | 400.244 | 11.09 | 10.71 | 10.29 |
| 20 | 19:02 | 501.284 | 388.833 | 503.274 | 8.42 | 11.42 | 8.38 |
| 21 | 20:02 | 551.973 | 433.372 | 531.722 | 8.15 | 10.12 | 8.24 |
| 22 | 21:02 | 615.821 | 555.172 | 584.791 | 7.48 | 8.35 | 8.24 |
| 23 | 22:02 | 662.701 | 560.962 | 521.991 | 7.05 | 8.13 | 9.59 |
| 24 | 23:02 | 451.841 | 431.891 | 383.634 | 11.03 | 11.96 | 12.84 |
| Time Frame | Time | Fundamental Currents (A) | THD% | ||||
|---|---|---|---|---|---|---|---|
| Phase A | Phase B | Phase C | Phase A | Phase B | Phase C | ||
| 1 | 0:00 | 260.048 | 250.148 | 549.749 | 70.60 | 71.57 | 39.82 |
| 2 | 1:00 | 278.239 | 260.066 | 544.526 | 72.09 | 66.64 | 42.72 |
| 3 | 2:00 | 276.985 | 240.753 | 425.387 | 68.93 | 57.36 | 54.22 |
| 4 | 3:00 | 248.599 | 239.669 | 410.847 | 59.21 | 71.01 | 36.97 |
| 5 | 4:00 | 313.33 | 221.065 | 578.245 | 65.63 | 61.44 | 42.28 |
| 6 | 5:00 | 232.953 | 253.647 | 407.075 | 65.07 | 68.20 | 43.31 |
| 7 | 6:00 | 231.01 | 215.224 | 478.693 | 69.26 | 62.47 | 42.09 |
| 8 | 7:00 | 204.259 | 200.562 | 395.236 | 64.59 | 78.71 | 40.49 |
| 9 | 8:00 | 272.849 | 287.224 | 468.403 | 57.98 | 66.79 | 36.46 |
| 10 | 9:00 o’clock | 281.268 | 265.11 | 512.295 | 68.65 | 63.69 | 43.65 |
| 11 | 10:00 | 312.636 | 373.305 | 544.483 | 66.48 | 52.55 | 41.23 |
| 12 | 11:00 | 411.159 | 406.561 | 617.135 | 49.73 | 51.79 | 33.94 |
| 13 | 12:00 | 374.348 | 380.28 | 614.553 | 52.49 | 56.92 | 33.98 |
| 14 | 13:00 | 372.745 | 407.866 | 712.213 | 54.10 | 51.11 | 28.04 |
| 15 | 14:00 p.m. | 392.063 | 389.628 | 658.772 | 54.69 | 51.42 | 32.28 |
| 16 | 15:00 | 322.007 | 288.37 | 573.041 | 55.60 | 65.17 | 33.49 |
| 17 | 16:00 | 348.959 | 319.456 | 485.147 | 54.48 | 60.30 | 41.46 |
| 18 | 17:00 | 299.725 | 257.722 | 533.28 | 62.02 | 66.24 | 36.32 |
| 19 | 18:00 | 316.776 | 337.183 | 582.824 | 54.70 | 59.73 | 32.47 |
| 20 | 19:00 | 311.264 | 316.519 | 603.691 | 62.14 | 60.17 | 34.21 |
| 21 | 20:00 | 438.887 | 428 | 648.861 | 48.36 | 50.66 | 32.04 |
| 22 | 21:00 | 413.944 | 425.264 | 694.68 | 48.00 | 51.49 | 27.60 |
| 23 | 22:00 | 344.851 | 375.56 | 565.459 | 57.90 | 53.75 | 37.82 |
| 24 | 23:00 | 307.032 | 307.242 | 586.034 | 62.77 | 62.19 | 34.44 |
| Time Frame | Time | Fundamental Currents (A) | CURRENT THD% | ||||
|---|---|---|---|---|---|---|---|
| Phase A | Phase B | Phase C | Phase A | Phase B | Phase C | ||
| 1 | 0:00 | 247.502 | 282.254 | 452.289 | 71.54 | 64.40 | 46.26 |
| 2 | 1:00 | 222.941 | 217.087 | 431.941 | 71.85 | 73.80 | 46.04 |
| 3 | 2:00 | 200.871 | 203.342 | 378.634 | 75.80 | 74.95 | 50.37 |
| 4 | 3:00 | 232.058 | 229.298 | 431.867 | 73.81 | 71.66 | 47.75 |
| 5 | 4:00 | 226.531 | 213.053 | 443.779 | 72.86 | 74.50 | 47.65 |
| 6 | 5:00 | 251.78 | 239.705 | 420.374 | 70.59 | 69.57 | 48.09 |
| 7 | 6:00 | 214.722 | 213.536 | 435.969 | 74.29 | 73.82 | 46.62 |
| 8 | 7:00 | 275.391 | 312.453 | 588.434 | 64.18 | 62.66 | 34.93 |
| 9 | 8:00 | 396.45 | 428.569 | 675.11 | 54.19 | 50.33 | 30.43 |
| 10 | 9:00 | 392.997 | 450.682 | 721.696 | 52.24 | 47.53 | 28.03 |
| 11 | 10:00 | 493.038 | 554.132 | 774.502 | 44.07 | 40.79 | 24.51 |
| 12 | 11:00 | 465.675 | 452.764 | 696.076 | 47.32 | 47.69 | 27.23 |
| 13 | 12:00 | 498.188 | 533.941 | 752.85 | 44.33 | 41.62 | 25.52 |
| 14 | 13:00 | 486.077 | 535.109 | 756.094 | 44.62 | 41.56 | 25.80 |
| 15 | 14:00 | 375.441 | 450.244 | 688.358 | 54.51 | 46.91 | 29.25 |
| 16 | 15:00 | 348.78 | 371.5 | 576.752 | 55.70 | 54.21 | 35.27 |
| 17 | 16:00 | 358.338 | 358.739 | 602.023 | 54.32 | 56.39 | 33.08 |
| 18 | 17:00 | 409.214 | 403.265 | 634.637 | 49.88 | 51.31 | 30.98 |
| 19 | 18:00 | 495.427 | 515.191 | 761.428 | 44.24 | 41.80 | 25.53 |
| 20 | 19:00 | 462.99 | 497.983 | 769.576 | 44.91 | 43.95 | 24.24 |
| 21 | 20:00 | 624.84 | 611.888 | 878.478 | 35.76 | 36.61 | 20.31 |
| 22 | 21:00 | 679.033 | 609.481 | 909.602 | 32.61 | 34.79 | 19.54 |
| 23 | 22:00 | 425.532 | 467.868 | 695.509 | 47.55 | 46.33 | 26.75 |
| 24 | 23:00 | 317.204 | 388.835 | 603.883 | 57.80 | 51.69 | 33.61 |
| Transformer | IEEE Std C57.110 | ANSI/UL 1561-1562 | Other Stray Losses | ||||
|---|---|---|---|---|---|---|---|
| Fundamental | Harmonics | Total | Fundamental | Harmonics | Total | ||
| No. 1 (Nov 13) | 13.468 (13.327) | 0.615 (0.609) | 14.084 (13.936) | 12.530 (12.405) | 0.572 (0.567) | 13.102 (12.972) | 0.982 (0.964) |
| No. 1 (Nov 14) | 15.776 (15.643) | 0.45 (0.445) | 16.226 (16.089) | 14.676 (14.553) | 0.416 (0.411) | 15.092 (14.965) | 1.133 (1.124) |
| No. 2 (Oct 9) | 25.467 (23.513) | 26.711 (24.925) | 52.179 (48.438) | 23.693 (21.875) | 24.597 (22.762) | 48.290 (44.637) | 3.889 (3.801) |
| No. 2 (Oct 10) | 37.306 (35.330) | 27.935 (26.358) | 65.241 (61.689) | 34.706 (32.868) | 26.002 (24.928) | 60.708 (57.797) | 4.533 (3.892) |
| Transformer | IEEE Std C57.110 | ANSI/UL Std. 1561-1562 | Other Stray Losses | ||||
|---|---|---|---|---|---|---|---|
| Fundamental | Harmonics | Total | Fundamental | Harmonics | Total | ||
| No. 1 | 5488.34 (5438.87) | 183.68 (181.73) | 5672 (5620.60) | 5105.87 (5060.61) | 170.12 (168.42) | 5276 (5529.03) | 396.03 (391.56) |
| No. 2 | 12,231.57 (11,513.16) | 10,053.18 (9453.17) | 22,284.76 (20,966.33) | 11,379.173 (10,710.83) | 9326.27 (8845.41) | 20,705.44 (19,556.24) | 1579.3 (1410.10) |
| Transformer | IEEE Std C57.110 | ANSI/UL Std. 1561-1562 | Other Stray Losses | ||||
|---|---|---|---|---|---|---|---|
| Fundamental | Harmonics | Total | Fundamental | Harmonics | Total | ||
| No. 1 | 845.2 (837.58) | 28.29 (28.00) | 873.49 (865.58) | 786.3 (779.33) | 26.2 (25.94) | 812.5 (805.27) | 61.0 (60.3) |
| No. 2 | 1883.66 (1773.02) | 1548.19 (1455.79) | 3431.85 (3228.81) | 1752.39 (1649.47) | 1436.24 (1362.19) | 3188.64 (3011.66) | 243.21 (217.15) |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
León-Martínez, V.; Peñalvo-López, E.; Montañana-Romeu, J.; Andrada-Monrós, C.; Molina-Cañamero, L. Assessment of Load Losses Caused by Harmonic Currents in Distribution Transformers Using the Transformer Loss Calculator Software. Environments 2023, 10, 177. https://doi.org/10.3390/environments10100177
León-Martínez V, Peñalvo-López E, Montañana-Romeu J, Andrada-Monrós C, Molina-Cañamero L. Assessment of Load Losses Caused by Harmonic Currents in Distribution Transformers Using the Transformer Loss Calculator Software. Environments. 2023; 10(10):177. https://doi.org/10.3390/environments10100177
Chicago/Turabian StyleLeón-Martínez, Vicente, Elisa Peñalvo-López, Joaquín Montañana-Romeu, Clara Andrada-Monrós, and Laura Molina-Cañamero. 2023. "Assessment of Load Losses Caused by Harmonic Currents in Distribution Transformers Using the Transformer Loss Calculator Software" Environments 10, no. 10: 177. https://doi.org/10.3390/environments10100177
APA StyleLeón-Martínez, V., Peñalvo-López, E., Montañana-Romeu, J., Andrada-Monrós, C., & Molina-Cañamero, L. (2023). Assessment of Load Losses Caused by Harmonic Currents in Distribution Transformers Using the Transformer Loss Calculator Software. Environments, 10(10), 177. https://doi.org/10.3390/environments10100177










