A Multidisciplinary Geophysical Approach to Characterize a Fracture Zone: The Southern Limit of the Mining District of Linares-La Carolina, Spain
Abstract
1. Introduction
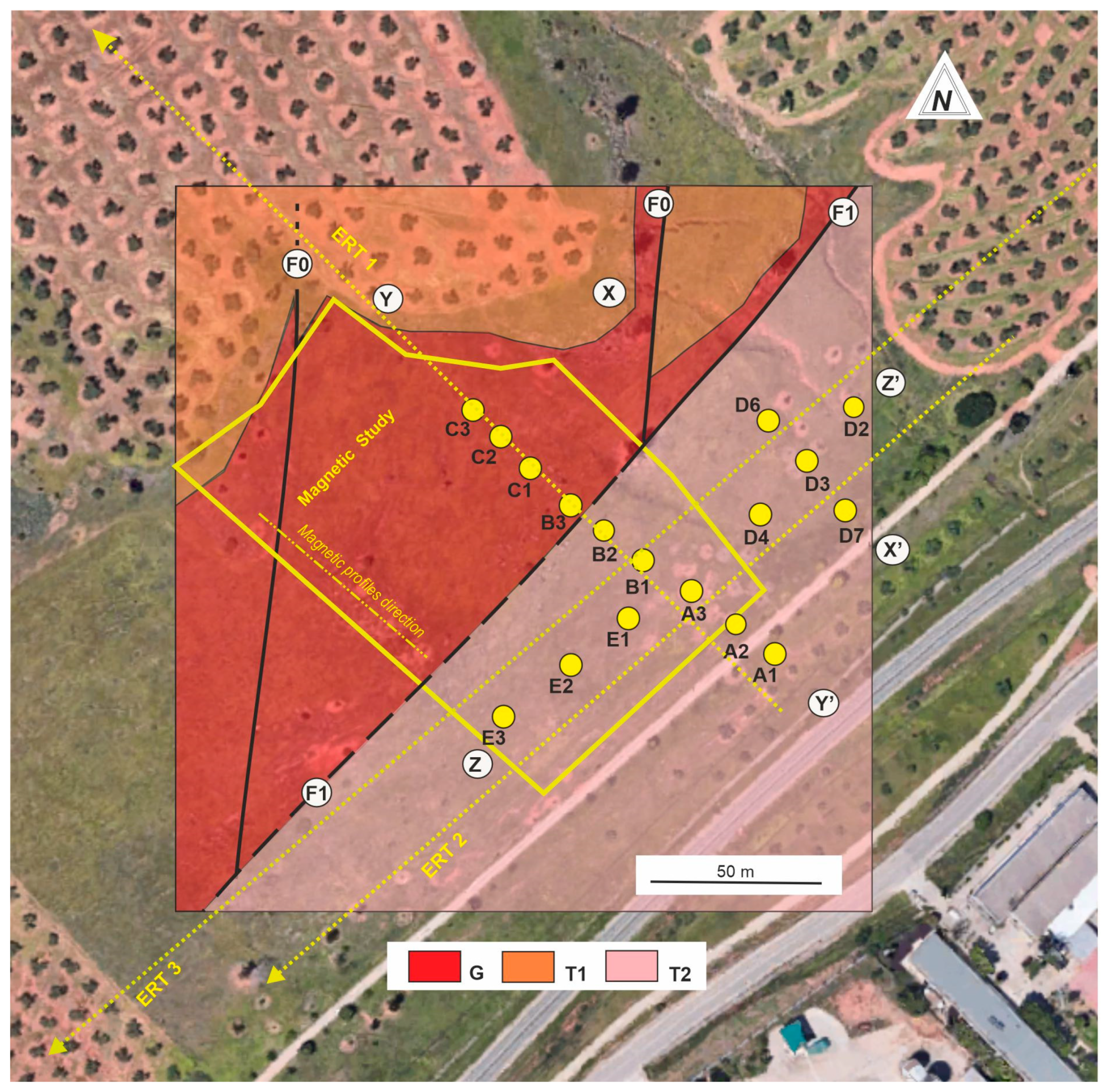
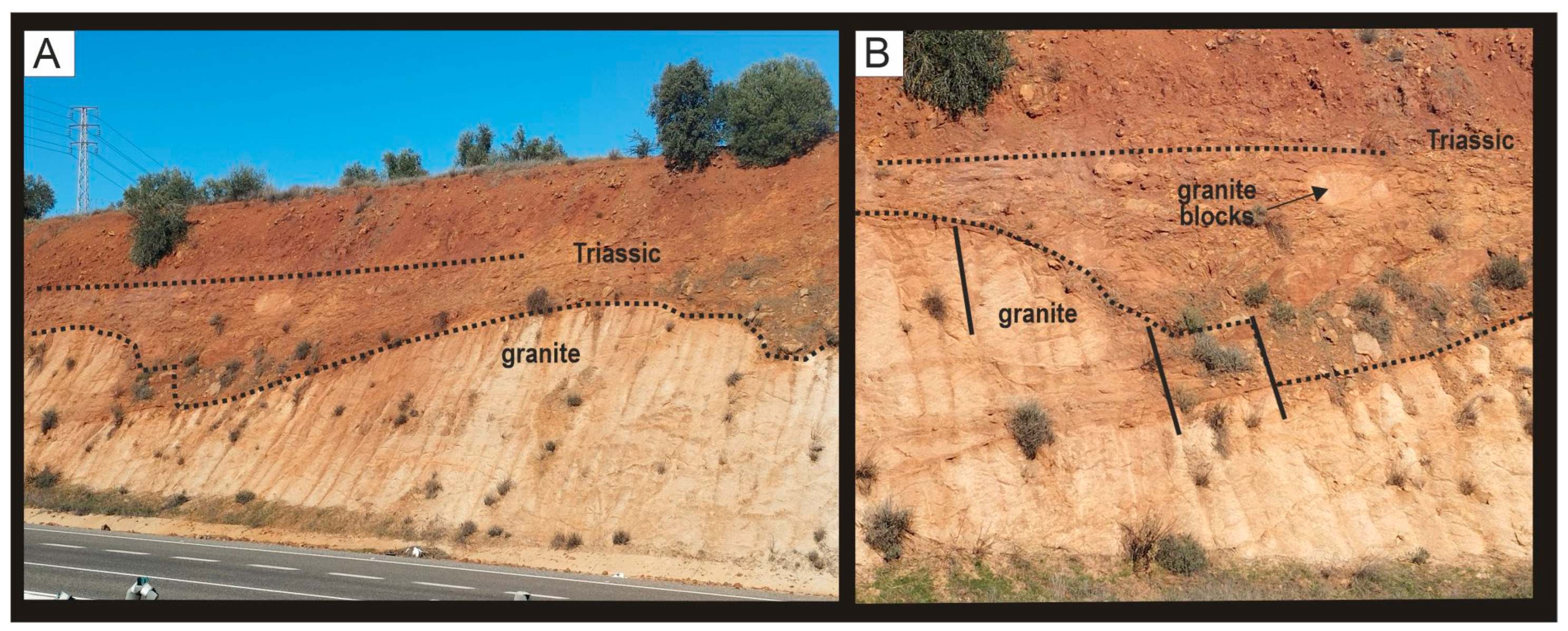
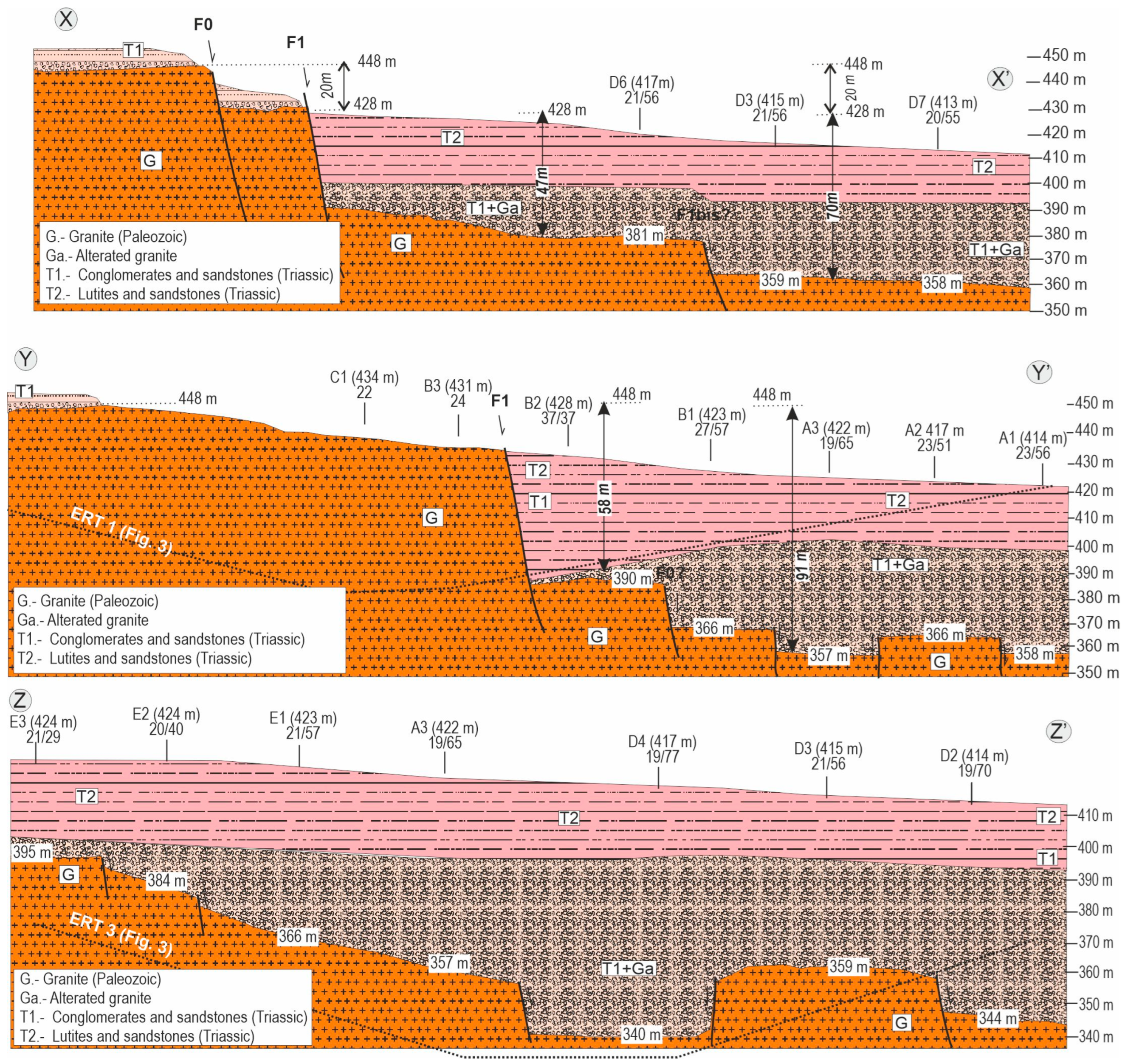
2. Local Geological Survey
3. Materials and Geophysical Methods
3.1. Electrical Resistivity Tomography
3.2. Time Domain Electromagnetic Method
3.3. Magnetic Method
4. Results and Discussion
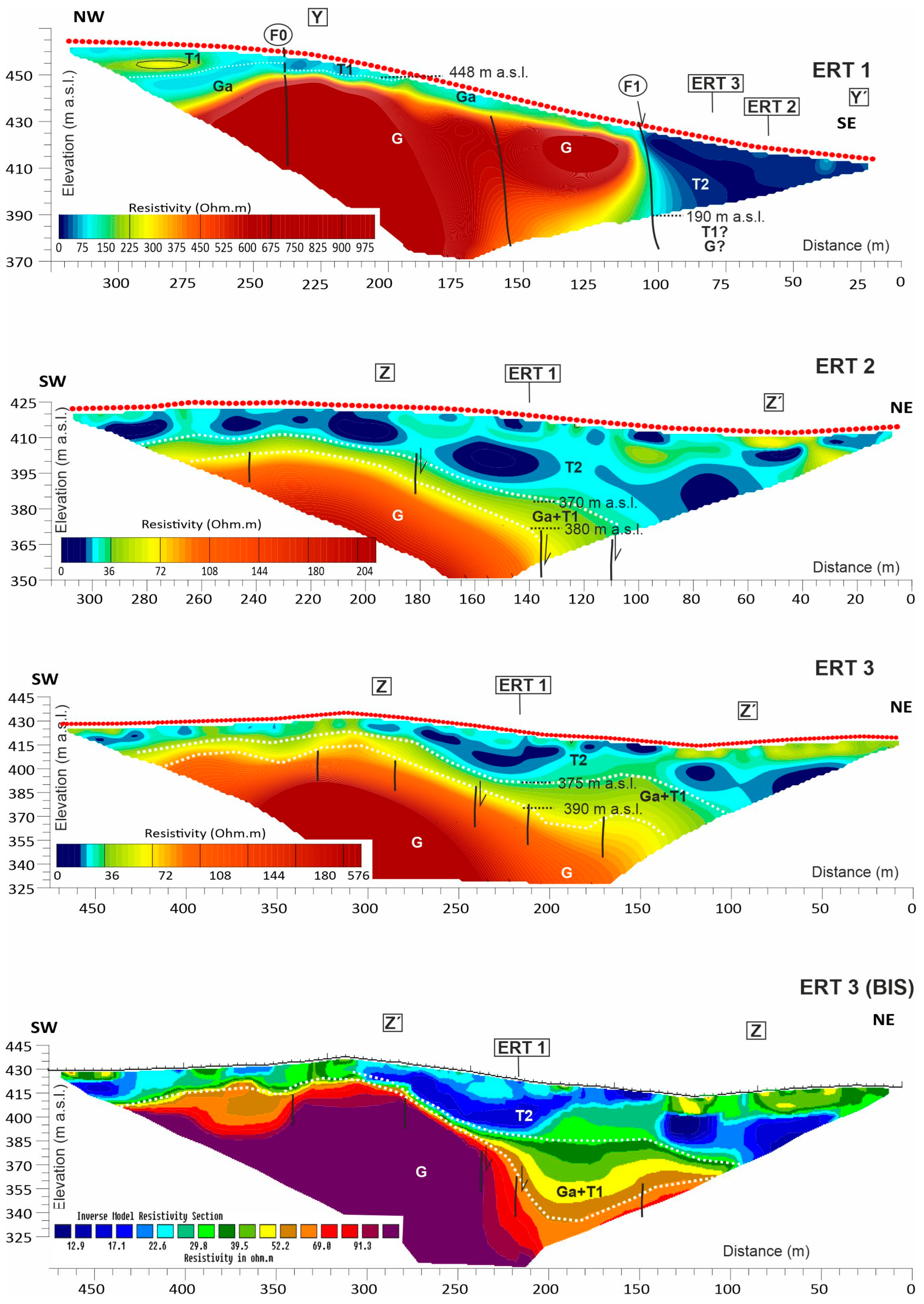
4.1. Electrical Resistivity Tomography
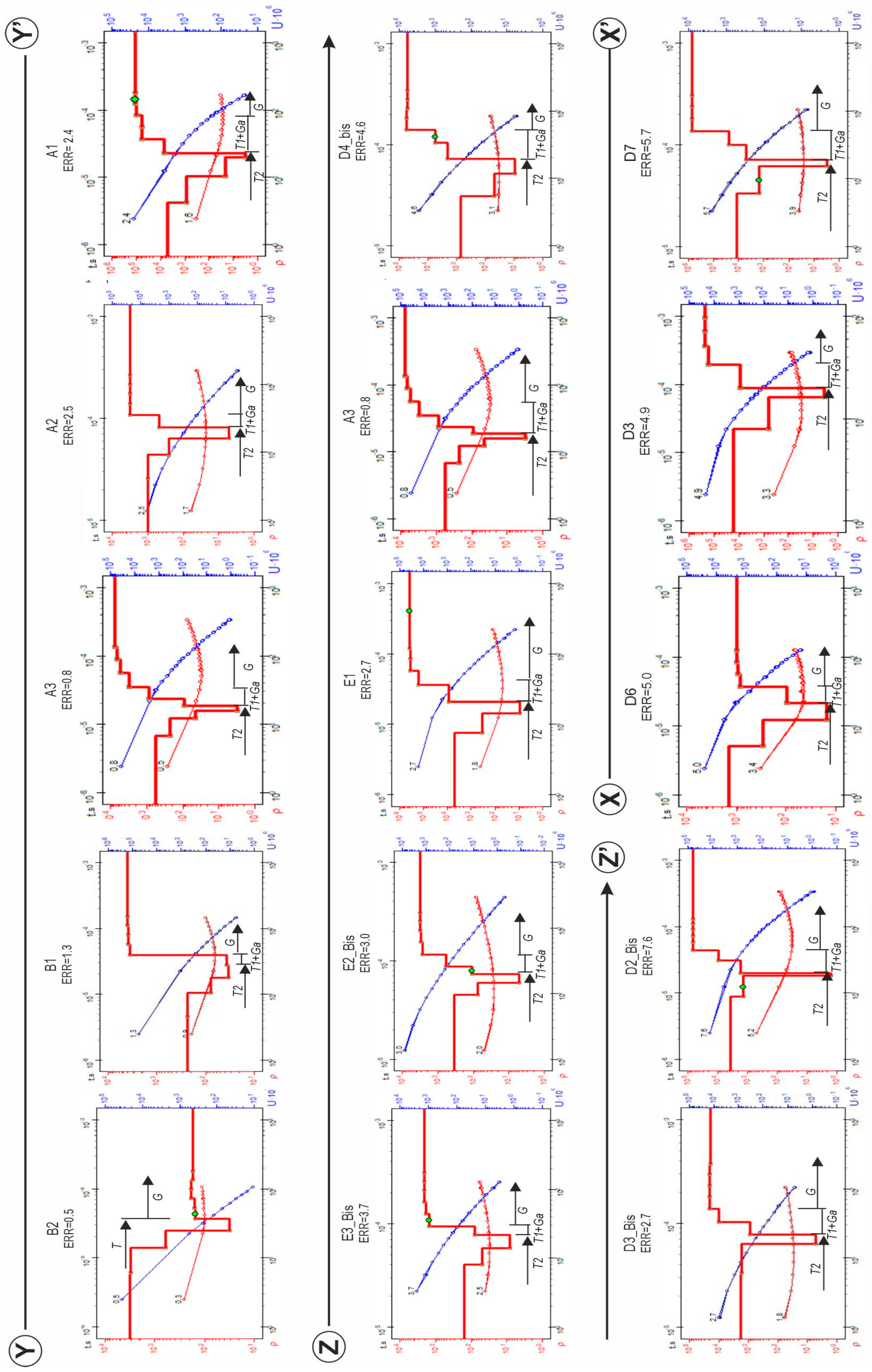
4.2. Time Domain Electromagnetic Method
4.3. Magnetic Methods
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Telford, W.M.; Geldart, L.P.; Sheriff, R.E. Applied Geophysics; Cambridge University Press: Cambridge, UK, 1990; p. 770. [Google Scholar]
- Everett, M.E. Near-Surface Applied Geophysics; Cambridge University Press: Cambridge, UK, 2013; p. 403. [Google Scholar] [CrossRef]
- Reynolds, J.M. An Introduction to Applied and Environmental Geophysics; John Wiley and Sons Ltd.: Chichester, UK, 2011; p. 796. [Google Scholar]
- Loke, M.H.; Barker, R.D. Rapid least-squares inversion of apparent resistivity pseudosections by a quasi-Newton method. Geophys. Prospect. 1996, 44, 131–152. [Google Scholar] [CrossRef]
- Loke, M.H.; Dahlin, T. A comparison of the Gauss-Newton and quasi-Newton methods in resistivity imaging inversion. J. Appl. Geophys. 2002, 49, 149–162. [Google Scholar] [CrossRef]
- Blanchy, G.; Saneiyan, S.; Boyd, J.; McLachlan, P.; Binley, A. ResIPy, an intuitive open source software for complex geoelectrical inversion/modeling. Comput. Geosci. 2020, 137, 104423. [Google Scholar] [CrossRef]
- André, F.; Van Leeuwen, C.; Saussez, S.; Van Durmen, R.; Bogaer, P.; Moghadas, D.; de Resseguier, L.; Delvaux, B.; Vreecken, H.; Lambot, S. High-resolution imaging of a vineyard in south of France using ground-penetrating radar, electromagnetic induction and electrical resistivity tomography. J. Appl. Geophys. 2012, 78, 113–122. [Google Scholar] [CrossRef]
- Peña, P.A.; Manteca, J.I.; Martínez-Pagán, P.; Teixidó, T. Magnetic gradient map of the mine tailings in Portman Bay (Murcia, Spain) and its contribution to the understanding of the bay infilling process. J. Appl. Geophys. 2013, 95, 115–120. [Google Scholar] [CrossRef]
- Rey, J.; Martínez, J.; Mendoza, R.; Sandoval, S.; Tarasov, V.; Kaminsky, K.; Hidalgo, M.C.; Morales, K. Geophysical characterization of aquifers in southeast Spain using ERT, TDEM, and vertical seismic reflection). Appl. Sci. 2020, 10, 7365. [Google Scholar] [CrossRef]
- Mendoza, M.; Rey, J.; Martínez, J.; Hidalgo, M.C.; Sandoval, S. Geophysical characterisation of geologic features with mining implications from ERT, TDEM and seismic reflection (Mining District of Linares-La Carolina, Spain). Ore Geol. Rev. 2021, 139, 104581. [Google Scholar] [CrossRef]
- Carrasco, J.; Carrasco, P.; Porras, D.; Martín, I. Drone Magnetic and Time Domain Electromagnetic Exploration in Metamorphic Formations: Tool for the Identification of Strategic Sites for Aquifer Exploitation. Appl. Sci. 2023, 13, 10949. [Google Scholar] [CrossRef]
- Penna, N.d.S.; Porsani, J.L.; Rangel, R.C.; Costa, V.H.H.; Oliveira, N.C.d.; Stangari, M.C.; Sousa, C.d.C.B.d.F. Near-Surface Geophysical Characterization of a Marble Deposit to Promote a Sustainable Small-Scale Mining. Remote Sens. 2024, 16, 1147. [Google Scholar] [CrossRef]
- Rapetsoa, M.K.; Gomo, S.; Manzi, M.S.D.; James, I.; Dildar, J.; Sihoyiya, M.; Mutshafa, N.; Durrheim, R. Multi-geophysical methods for characterizing fractures in an open pit mine, western Bushveld Complex, South Africa. Geophys. Prospect. 2024, 72, 1950–1970. [Google Scholar] [CrossRef]
- Rey, J.; Mendoza, R.; Hidalgo, M.C.; Marinho, B. Testing the Efficacy of Indirect Methods on Characterization of Sedimentary Basins by Correlation of Direct Data and Geophysical Techniques. Appl. Sci. 2024, 14, 7308. [Google Scholar] [CrossRef]
- Azcárate, J.E. Mapa Geológico y Memoria Explicativa de la hoja 905 (Linares), Escala 1:50.000; Instituto Geológico y Minero de España: Madrid, Spain, 1977; p. 35. [Google Scholar]
- Lillo, J. Geology and Geochemistry of Linares-La Carolina Pb-Ore Field (Southeastern Border of the Hesperian Massif). Ph.D. Thesis, University Leeds, Leeds, UK, 1992. [Google Scholar]
- Larrea, F.J.; Carracedo, M.; Ortega Cuesta, L.; Gil Ibarguchi, J.I. El Plutón de Linares (Jaén): Cartografía, petrología y geoquímica. Cuad. Lab. Xeológico Laxe Coruña 1994, 19, 335–346. [Google Scholar]
- ENADIMSA. Investigación de la Demarcación San Juan al Oeste de la Falla de Guarromán-Filón I; Technical Report; Empresa Nacional ADARO de Investigaciones Mineras S.A.: Linares, España, 1985; p. 42. [Google Scholar]
- Store, H.; Storz, W.; Jacob, F. Electrical resistivity tomography to investigate geological structures of earth’s upper crust. Geophys. Prospect. 2000, 48, 455–471. [Google Scholar] [CrossRef]
- Sasaki, Y. Resolution of resistivity tomography inferred from numerical simulation. Geophys. Prospect. 1992, 40, 453–464. [Google Scholar] [CrossRef]
- Maillet, G.M.; Rizzo, E.; Revil, A.; Vella, C. High resolution electrical resistivity tomography (ERT) in a transition zone environment: Application for detailed internal architecture and infilling processes study of a Rhône River paleo-channel. Mar. Geophys. Res. 2005, 26, 317–328. [Google Scholar] [CrossRef]
- Pucci, S.; Finizola, S.; Civico, R.; Sapia, V.; Barde-Cabusson, S.; Orefice, S.; Peltier, A.; Villani, F.; Ricci, T.; De Martini, P.M.; et al. Deep electrical resistivity tomography along the tectonically active Middle Aterno Valley (2009 L’Aquila earthquake area, Central Italy). Geophys. J. Int. 2016, 207, 967–982. [Google Scholar] [CrossRef]
- Porras, D.; Carrasco, J.; Carrasco, P.; González, P.J. Imaging extensional fault systems using deep electrical resistivity tomography: A case study of the Baza fault, Betic Cordillera, Spain. J. Appl. Geophys. 2022, 202, 104673. [Google Scholar] [CrossRef]
- Martínez, J.; Benavente, J.; García-Aróstegui, J.L.; Hidalgo, M.C.; Rey, J. Contribution of electrical resistivity tomography to the study of detrital aquifers affected by seawater intrusión-extrusion effects: The river Vélez delta (Vélez-Málaga, southern Spain). Eng. Geol. 2009, 108, 161–168. [Google Scholar] [CrossRef]
- Rey, J.; Martínez, J.; Hidalgo, M.C.; Rojas, D. Heavy metal pollution in the Quaternary Garza basin: A multidisciplinary study of the environmental risks posed by mining (Linares, southern Spain). Catena 2013, 110, 234–242. [Google Scholar] [CrossRef]
- Martínez-Pagán, P.; Faz Cano, A.; Aracil, E.; Arocena, J.M. Electrical resistivity imaging revealed the spatial properties of mine tailing ponds in the Sierra Minera of Southeast Spain. J. Environ. Eng. Geophys. 2009, 14, 63–76. [Google Scholar] [CrossRef]
- Martínez, J.; Hidalgo, M.C.; Rey, J.; Garrido, J.; Kohfahl, C.; Benavente, J.; Rojas, D. A Multidisciplinary Characterization of a Tailings Pond in the Linares-La Carolina Mining District, Spain. J. Geochem. Explor. 2016, 162, 62–71. [Google Scholar] [CrossRef]
- Gabarrón, M.; Martínez-Pagán, P.; Martínez-Segura, M.A.; Bueso, M.C.; Martínez-Martínez, S.; Faz, A.; Acosta, J.A. Electrical Resistivity Tomography as a Support Tool for Physicochemical Properties Assessment of Near-Surface Waste Materials in a Mining Tailing Pond (El Gorguel, SE Spain). Minerals 2020, 10, 559. [Google Scholar] [CrossRef]
- Martínez, J.; Rey, J.; Dueñas, J.; Hidalgo, M.C.; Benavente, J. Electrical tomography applied to the detection of subsurface cavities. J. Cave Karst 2012, 75, 28–37. [Google Scholar] [CrossRef]
- Konaté, A.A.; Kaba, O.B.; Conté, M.S.M.; Zaheer, M.; Thiam, B.M.; Oularé, F.; Cissé, M. Detection of subsurface cavities in the structurally bounded Labe prefecture area, Republic of Guinea using two-dimensional electrical resistivity tomography (ERT). Geomech. Geoengin. 2024, 19, 569–585. [Google Scholar] [CrossRef]
- Dahlin, T.; Zhou, B.A. numerical comparison of 2D resistivity imaging with 10 electrode arrays. Geophys. Prospect. 2004, 52, 379–398. [Google Scholar] [CrossRef]
- Loke, M.H. Tutorial: 2-D and 3-D Electrical Imaging Surveys. Available online: www.geotomosoft.com (accessed on 5 November 2014).
- DMT. High-Resolution Resistivity Meter for Underground Measurements & Mineral Exploration. Available online: https://www.dmt-group.com/products/geo-measuring-systems/resistivity-meter.html (accessed on 8 August 2024).
- Nabighian, N.M. Electromagnetic Method in Applied Geophysics; Society of Exploration Geophysics: Tulsa, Okla, 1988; p. 971. [Google Scholar]
- McNeill, J.D. Principles and Application of Time Domain Electromagnetic Techniques for Resistivity Sounding; Technical Note TN-27; Geonics Ltd.: Mississauga, ON, Canada, 1994; Available online: http://www.geonics.com/pdfs/technicalnotes/tn27.pdf (accessed on 15 January 2024).
- Goldman, M.; Rabinovich, B.; Rabinovich, M.; Gilad, D.; Gev, I.; Shirov, M. Application of the integrated NMR-TDEM method in groundwater exploration in Israel. J. Appl. Geophys. 1994, 31, 27–52. [Google Scholar] [CrossRef]
- Auken, E.; Jørgensen, F.; Sørensen, K.I. Large-scale TEM investigation for groundwater. Explor. Geophys. 2003, 34, 188–194. [Google Scholar] [CrossRef]
- Zhu, Z.; Shan, Z.; Pang, Y.; Wang, W.; Chen, M.; Li, G.; Sun, H.; Revil, A. The transient electromagnetic (TEM) method reveals the role of tectonic faults in seawater intrusion at Zhoushan islands (Hangzhou Bay, China). Eng. Geol. 2024, 330, 107425. [Google Scholar] [CrossRef]
- Hallbauer-Zadorozhnaya, V.Y.; Stettler, E. Time Domain Electromagnetic Sounding to delineate hydrocarbon Contamination of ground water. In Symposium on the Application of Geophysics to Engineering and Environmental Problems; European Association of Geoscientists & Engineers: Forth, TX, USA, 2009; pp. 241–251. [Google Scholar] [CrossRef]
- Sridhar, M.; Markandeyulu, A.; Chaturvedi, A.K. Mapping subtrappean sediments and delineating structure with the aid of heliborne time domain electromagnetics: Case study from Kaladgi Basin, Karnataka. J. Appl. Geophys. 2017, 136, 9–18. [Google Scholar] [CrossRef]
- AIE-2 Instruments. Available online: http://zond-geo.com/english/services/equipment/aie-2-instruments/ (accessed on 8 August 2024).
- Blakely, R. Potencial Theory in Gravity and Magnetic Applications; Cambridge University Press: Cambridge, UK, 1995; 441p. [Google Scholar]
- Abedi, M.; Fournier, D.; Devriese, S.; Oldenburg, D.W. Integrated inversion of airborne geophysics over a structural geological unit: A case study for delineation of a porphyry copper zone in Iran. J. Appl. Geophys. 2018, 152, 188–202. [Google Scholar] [CrossRef]
- Fries, M.; Machado Zago, M.; Garcia da Silva, F. A geophysical study contributing to analysis and characterization of a localized copper occurrence. J. Appl. Geophys. 2020, 179, 104129. [Google Scholar] [CrossRef]
- Louzada da Costa Carvalhêdo, L.; Chatack Carmelo, A.; Francisquini Botelho, N. Geophysical-geological model of the Pedra Branca massif in the Goiás Tin Province, Brazil. J. S. Am. Earth Sci. 2020, 101, 102593. [Google Scholar] [CrossRef]
- Nabighian, M.N.; Grauch, V.J.S.; Hansen, R.O.; LaFehr, T.R.; Li, Y.; Peirce, J.W.; Phillips, J.D.; Ruder, M.E. The historical development of the magnetic method inexploration. Geophysics 2005, 70, 33ND–61ND. [Google Scholar] [CrossRef]
- Abdelazeem, M.; Gobashy, M.M. A solution to unexploded ordnance detection problem from its magnetic anomaly using Kaczmarz regularization. Interpretation 2016, 4, 61–69. [Google Scholar] [CrossRef]
- Alva-Valdivia, L.M.; Guerrero-Díaz, P.; Urrutia-Fucugauchia, J.; Agarwal, A.; Caballero-Miranda, C.I. Rock-magnetism and magnetic anomaly modelling of Las Truchas, case study. J. S. Am. Earth Sci. 2020, 97, 102409. [Google Scholar] [CrossRef]
- Benítez, M.E.; Prezzi, C.; Benallivián Justiniano, C.A.; Verdecchia, S.O.; De Martino, F.J.; Carlini, M.; Lanfranchini, M.E. Ground magnetic survey and 3D geophysical model of ultrabasic rocks from the Martín García Complex (Buenos Aires, Argentina). J. S. Am. Earth Sci. 2023, 121, 104117. [Google Scholar] [CrossRef]
- Bouzekraoui, M.; Es-Sabbar, B.; Karaoui, B.; Essalhi, M.; Saadi, M. Structural analysis, tectonic fracturing modeling, and kinematic evolution along the South Atlas Fault at the northern border of the Tinghir-Errachidia-Boudenib basin (Pre-African Trough, Morocco). J. Afr. Earth Sci. 2024, 212, 105193. [Google Scholar] [CrossRef]
- Okiwelu, A.A.; Obianwu, V.I.; Eze Ohara, E.; Ude, I.A. Magnetic anomaly patterns, fault-block tectonism and hydrocarbon related structural features in the Niger Delta basin. IOSR J. Appl. Geol. Geophys. 2014, 2, 31–46. [Google Scholar]
- Mochales, T.; Pueyo, E.L.; Casas, A.M.; Soriano, M.A. Magnetic prospection as an efficient tool for doline detection. A case study in the central Ebro Basin (Northern Spain). Geological Society of London. Spec. Publ. 2005, 279, 73–84. [Google Scholar] [CrossRef]
- Mendoza, R.; Marinho, B.; Rey, J. GPR and magnetic techniques to locate ancient mining galleries (Linares, South-East Spain). Int. J. Geophys. 2023, 2023, 6633599. [Google Scholar] [CrossRef]
- GEM Systems. Available online: https://www.gemsys.ca/ultra-high-sensitivity-potassium/ (accessed on 8 August 2024).
- Sánchez, M.A.; Weidmann, M.C.; Ariza, J.P.; Martínez, M.P.; Ruiz, F. Análisis de campo magnético mediante métodos de filtrado de anomalías en la sierra pampeana de Velasco. Latinmag Lett. 2011, 1, 1–7. [Google Scholar]


Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Rey, J.; Mendoza, R.; Vilchez, J.; Hidalgo, M.C.; Fernández, I.; Berman, S. A Multidisciplinary Geophysical Approach to Characterize a Fracture Zone: The Southern Limit of the Mining District of Linares-La Carolina, Spain. Geosciences 2024, 14, 228. https://doi.org/10.3390/geosciences14090228
Rey J, Mendoza R, Vilchez J, Hidalgo MC, Fernández I, Berman S. A Multidisciplinary Geophysical Approach to Characterize a Fracture Zone: The Southern Limit of the Mining District of Linares-La Carolina, Spain. Geosciences. 2024; 14(9):228. https://doi.org/10.3390/geosciences14090228
Chicago/Turabian StyleRey, Javier, Rosendo Mendoza, José Vilchez, M. Carmen Hidalgo, Isla Fernández, and Sara Berman. 2024. "A Multidisciplinary Geophysical Approach to Characterize a Fracture Zone: The Southern Limit of the Mining District of Linares-La Carolina, Spain" Geosciences 14, no. 9: 228. https://doi.org/10.3390/geosciences14090228
APA StyleRey, J., Mendoza, R., Vilchez, J., Hidalgo, M. C., Fernández, I., & Berman, S. (2024). A Multidisciplinary Geophysical Approach to Characterize a Fracture Zone: The Southern Limit of the Mining District of Linares-La Carolina, Spain. Geosciences, 14(9), 228. https://doi.org/10.3390/geosciences14090228






