Investigating the Behavior of an Onshore Wall and Trench Combination Ahead of a Tsunami-Like Wave
Abstract
:1. Introduction
2. Focus and Objectives
3. Materials and Methods
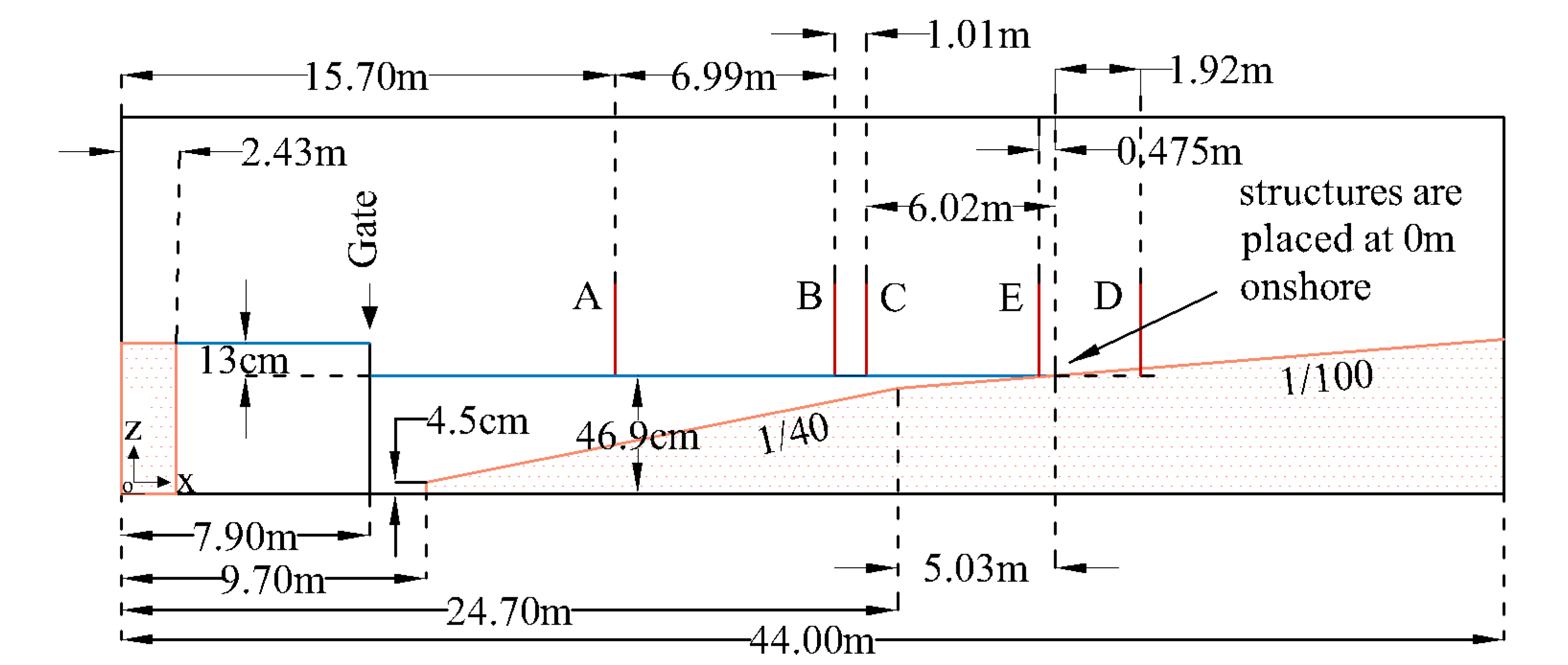
3.1. Experimental Setup
3.2. Numerical Setup
3.3. Simulations with Onshore Structures
3.4. Comparing the Combined Wall and Trench System with Single Seawall System
3.5. Evaluating the Behavior of the Structure Ahead of Different Tsunami Conditions
4. Results and Discussion
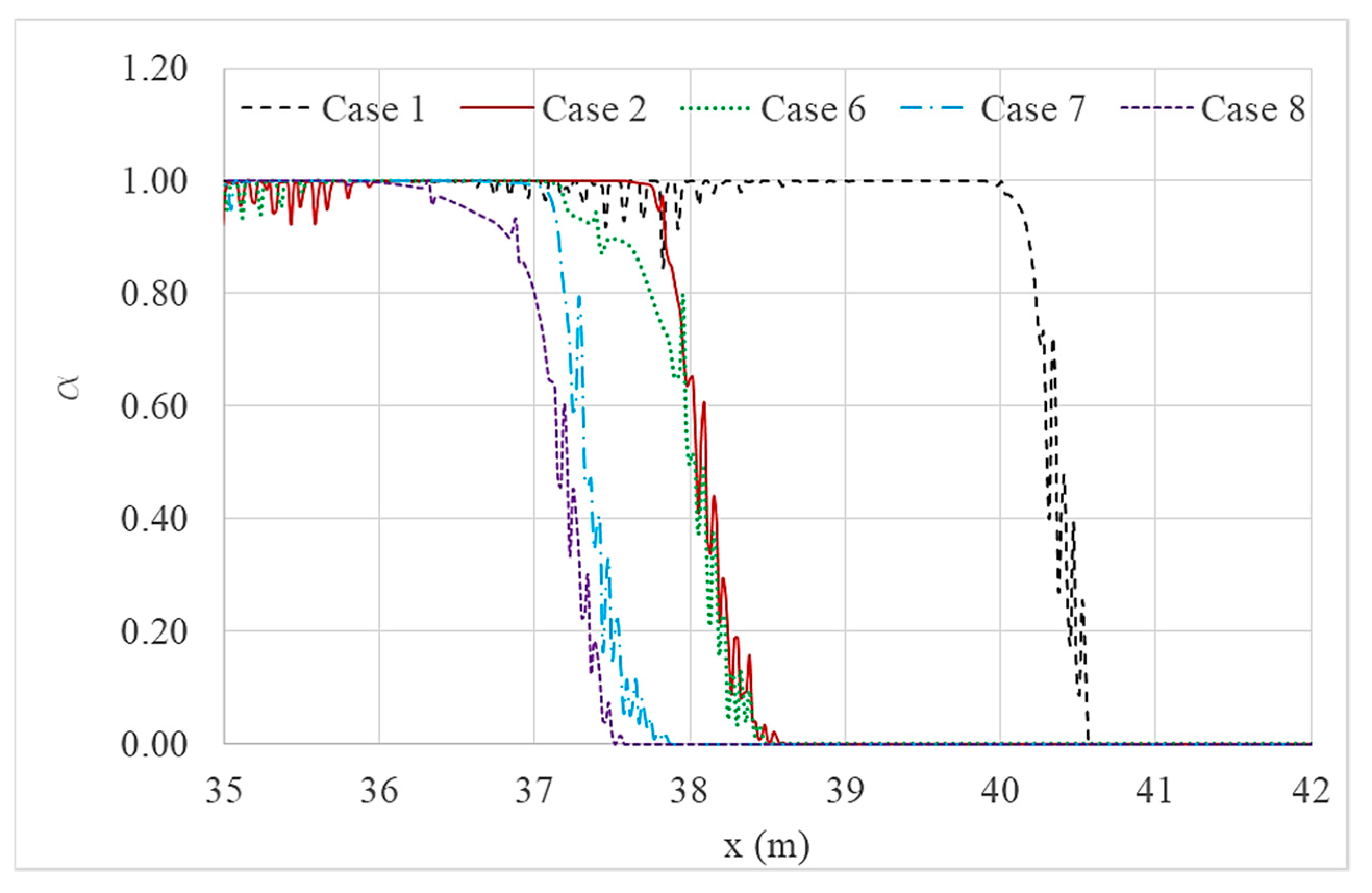
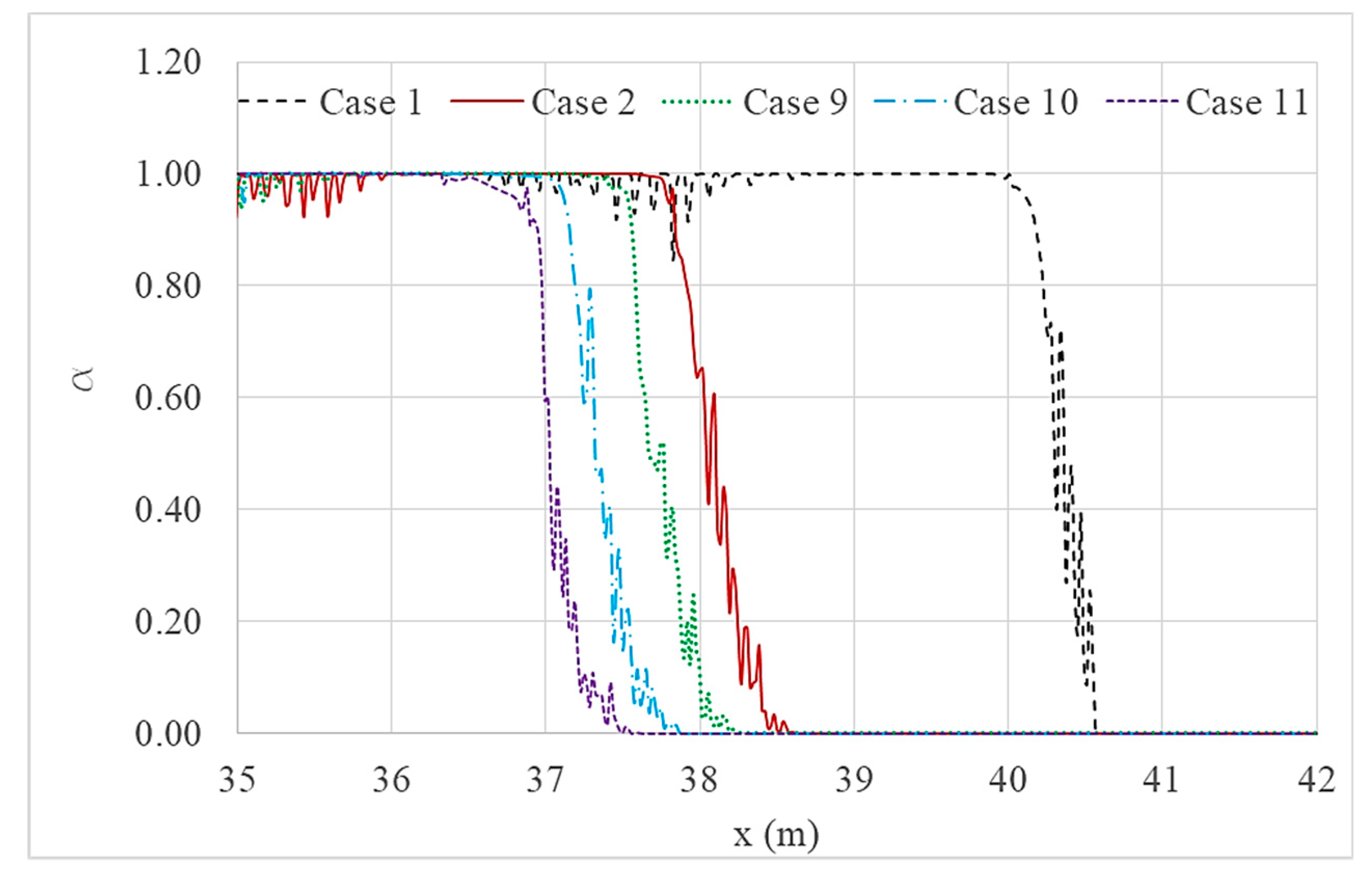
4.1. Comparison of Wave Transformation
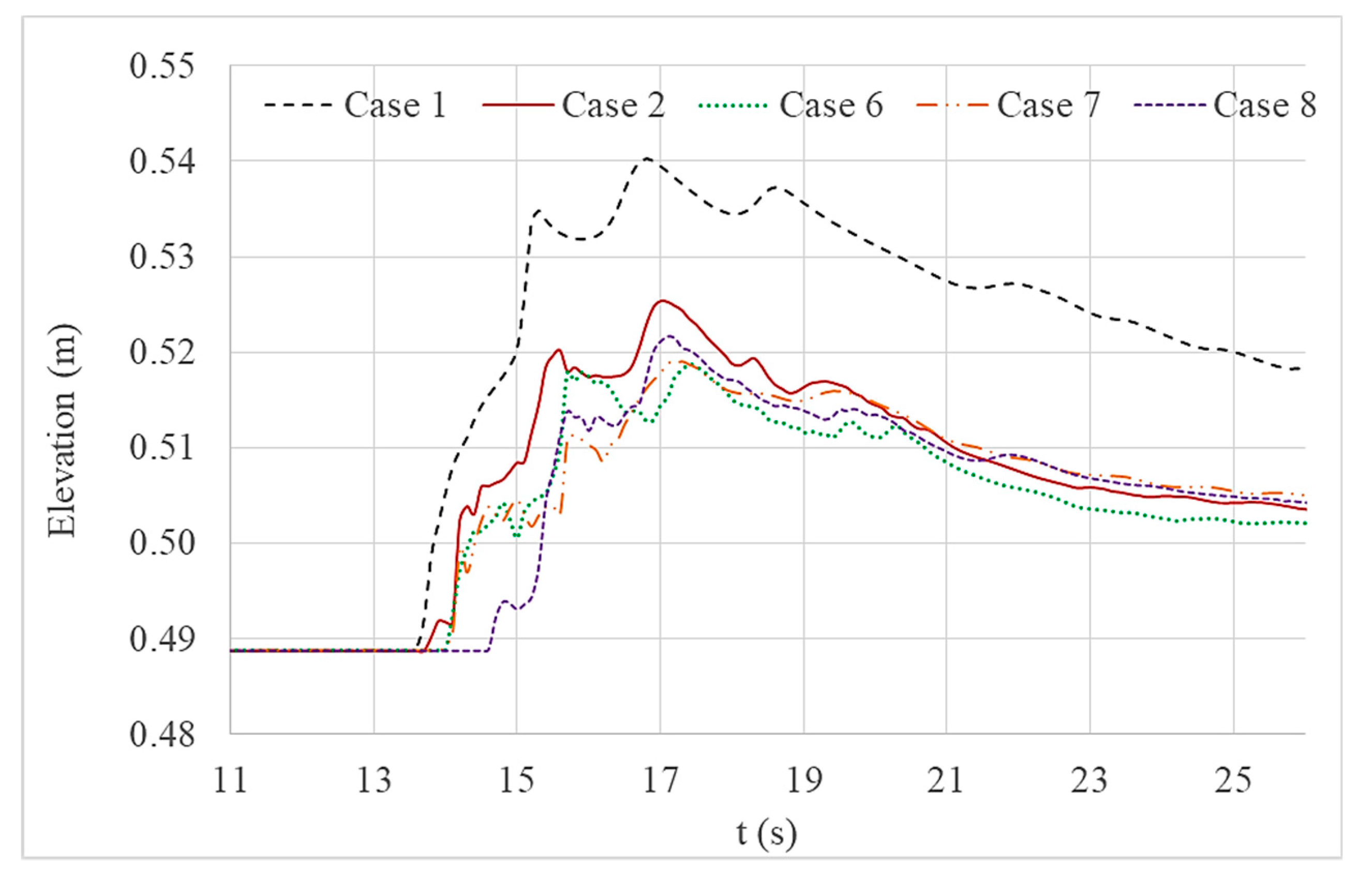
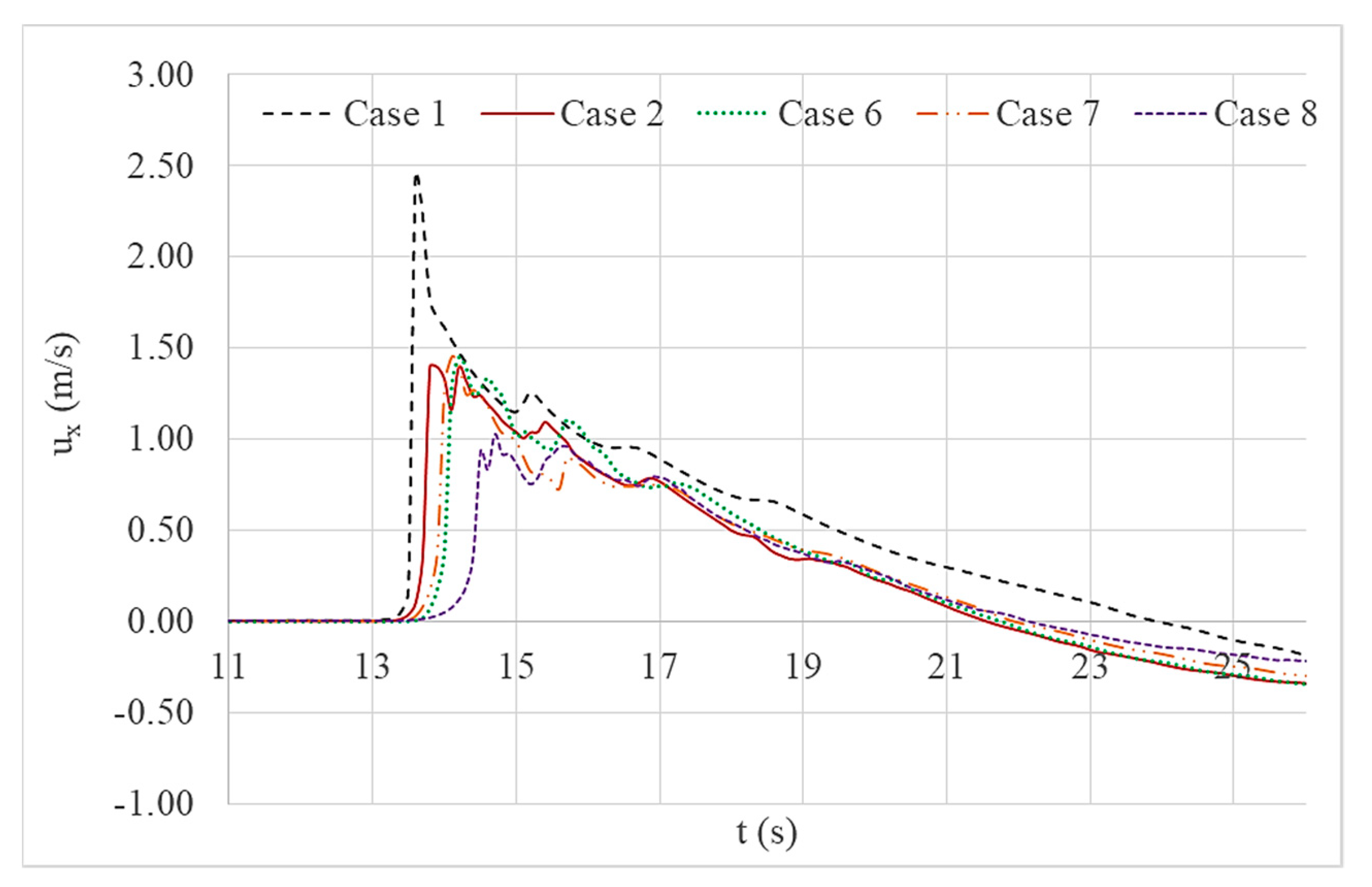
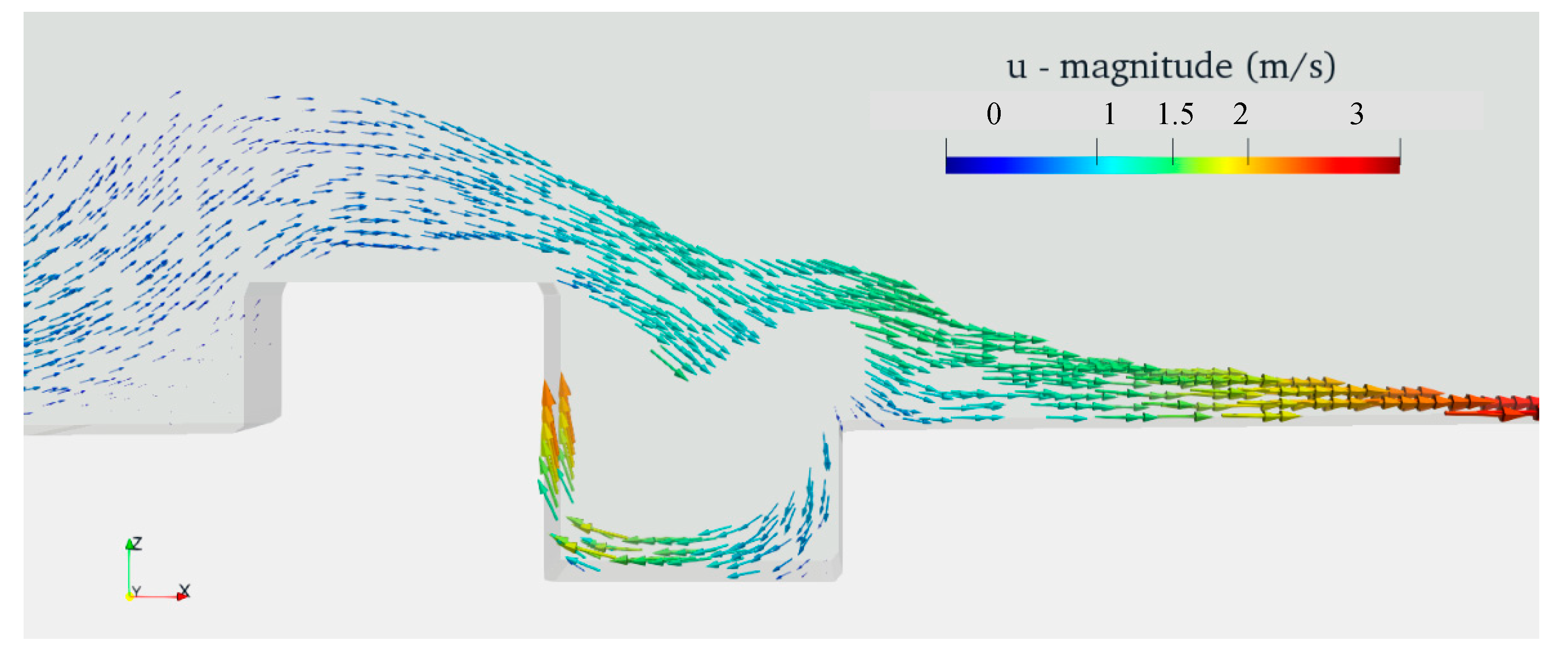
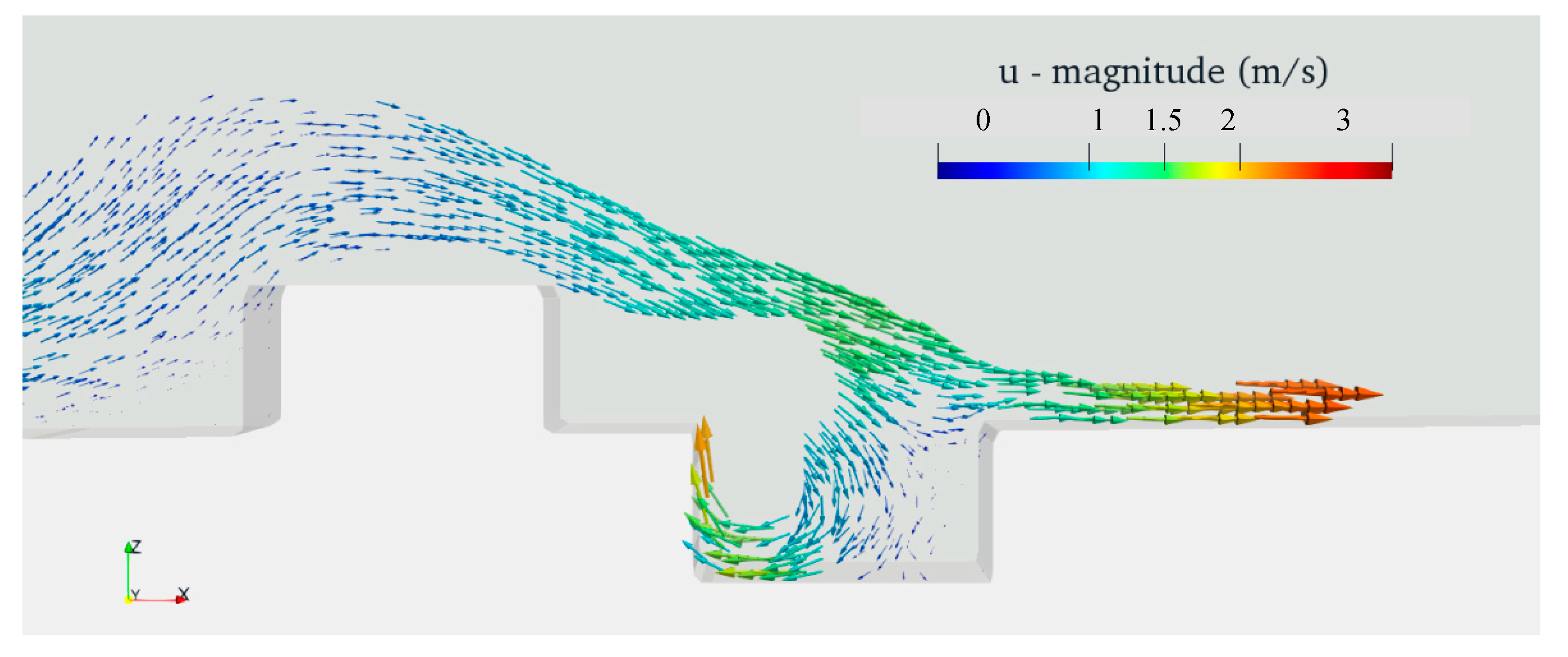
4.2. Assessment of Onshore Structures
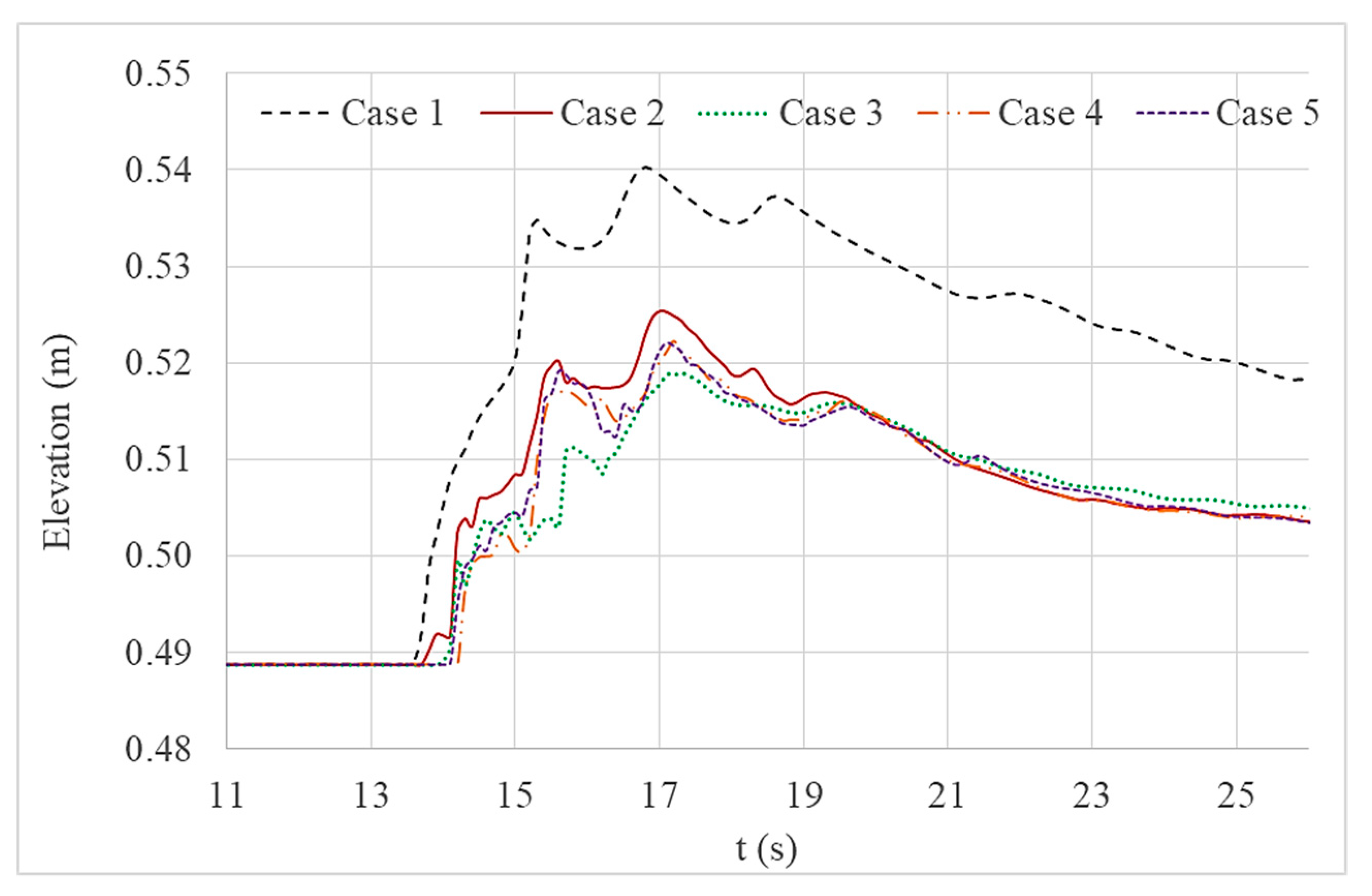
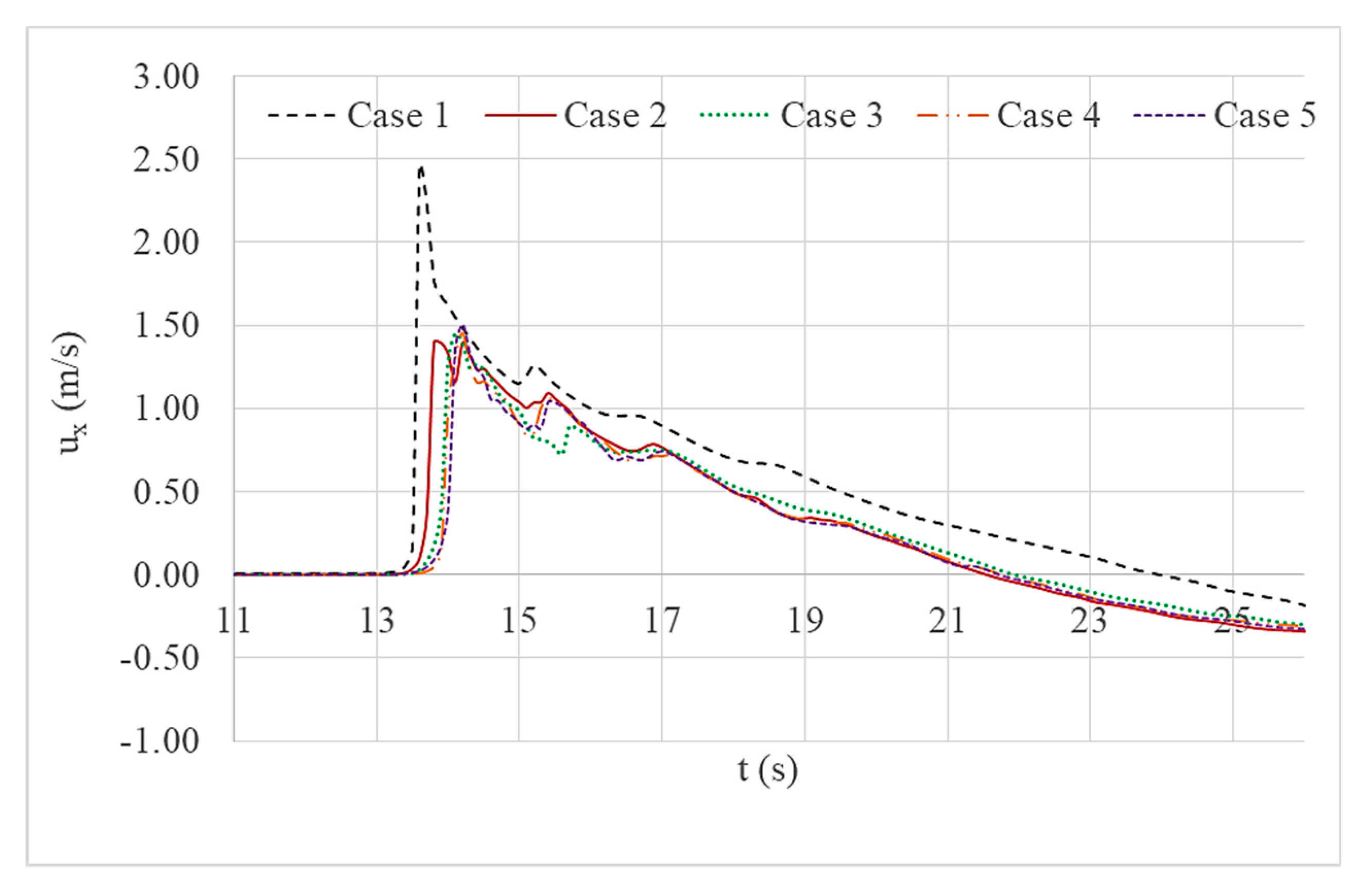
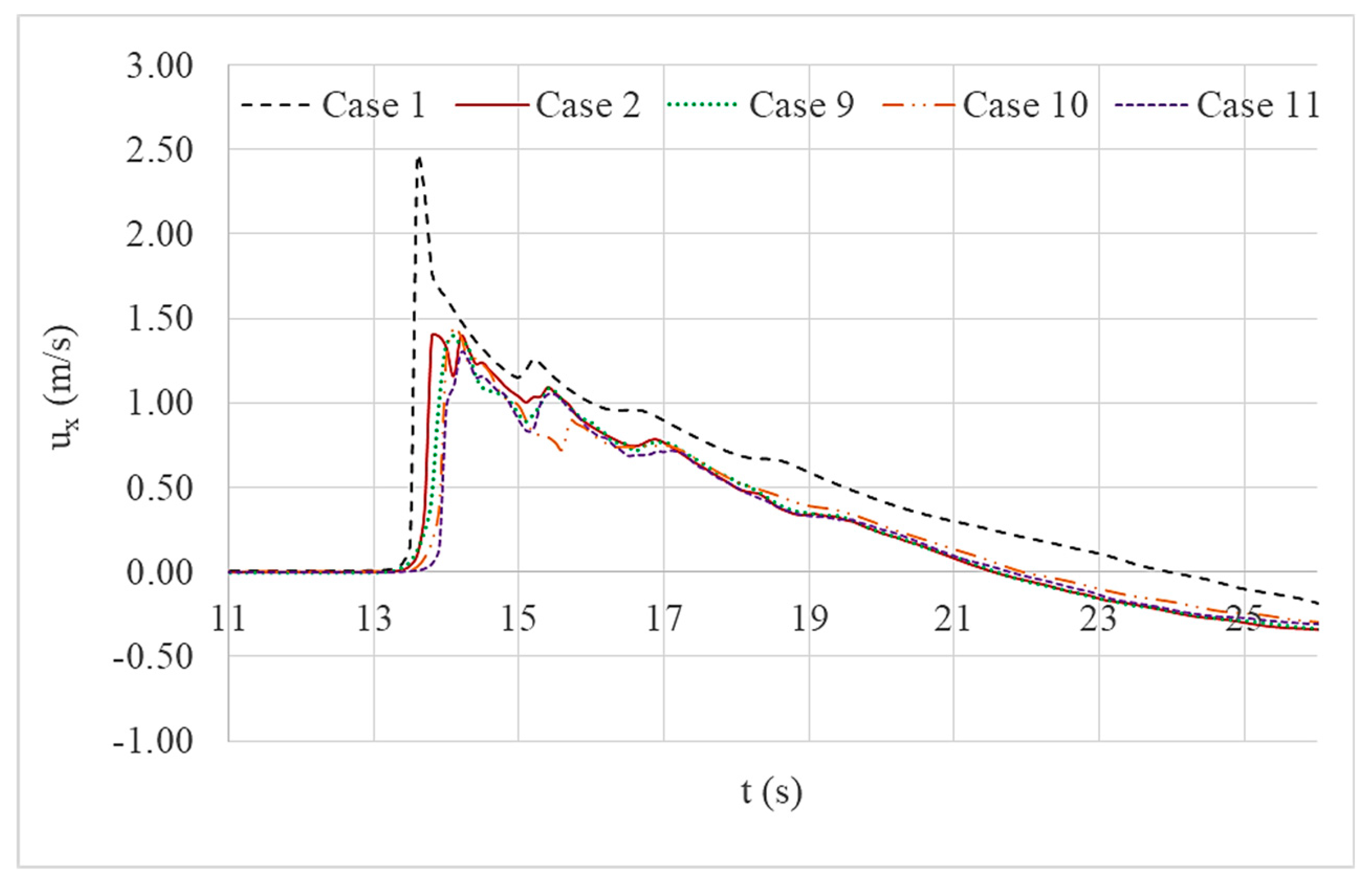
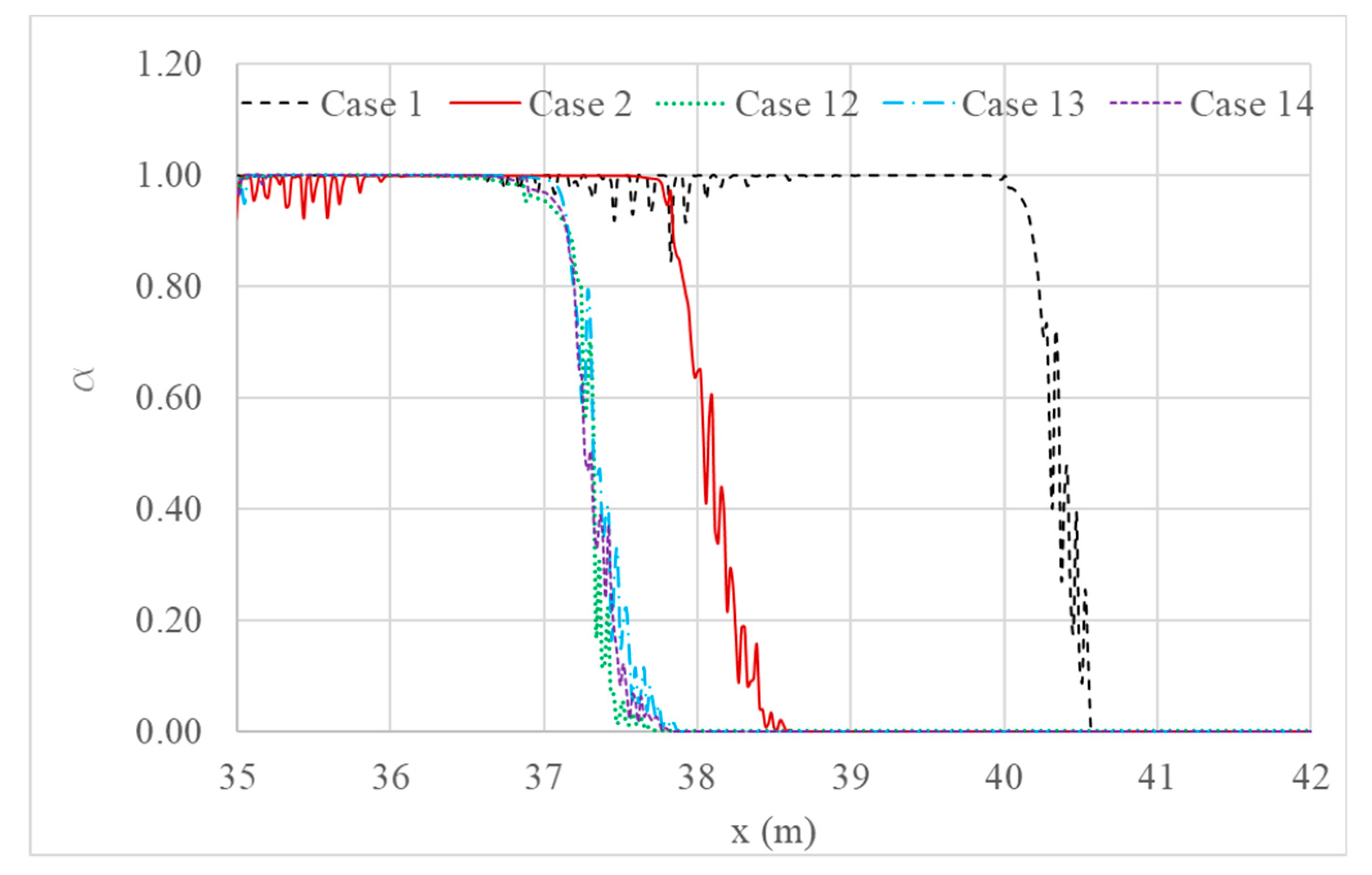
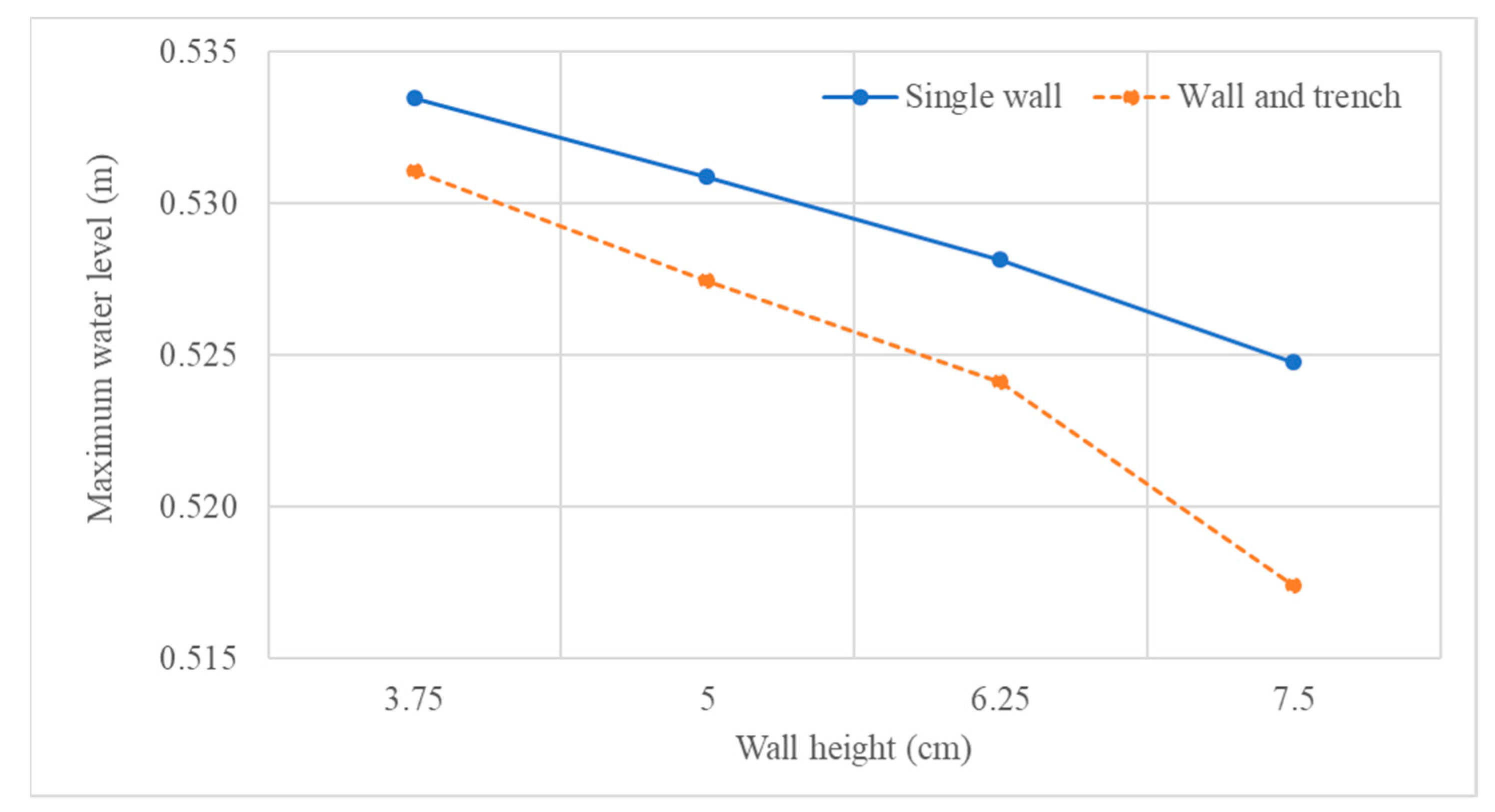
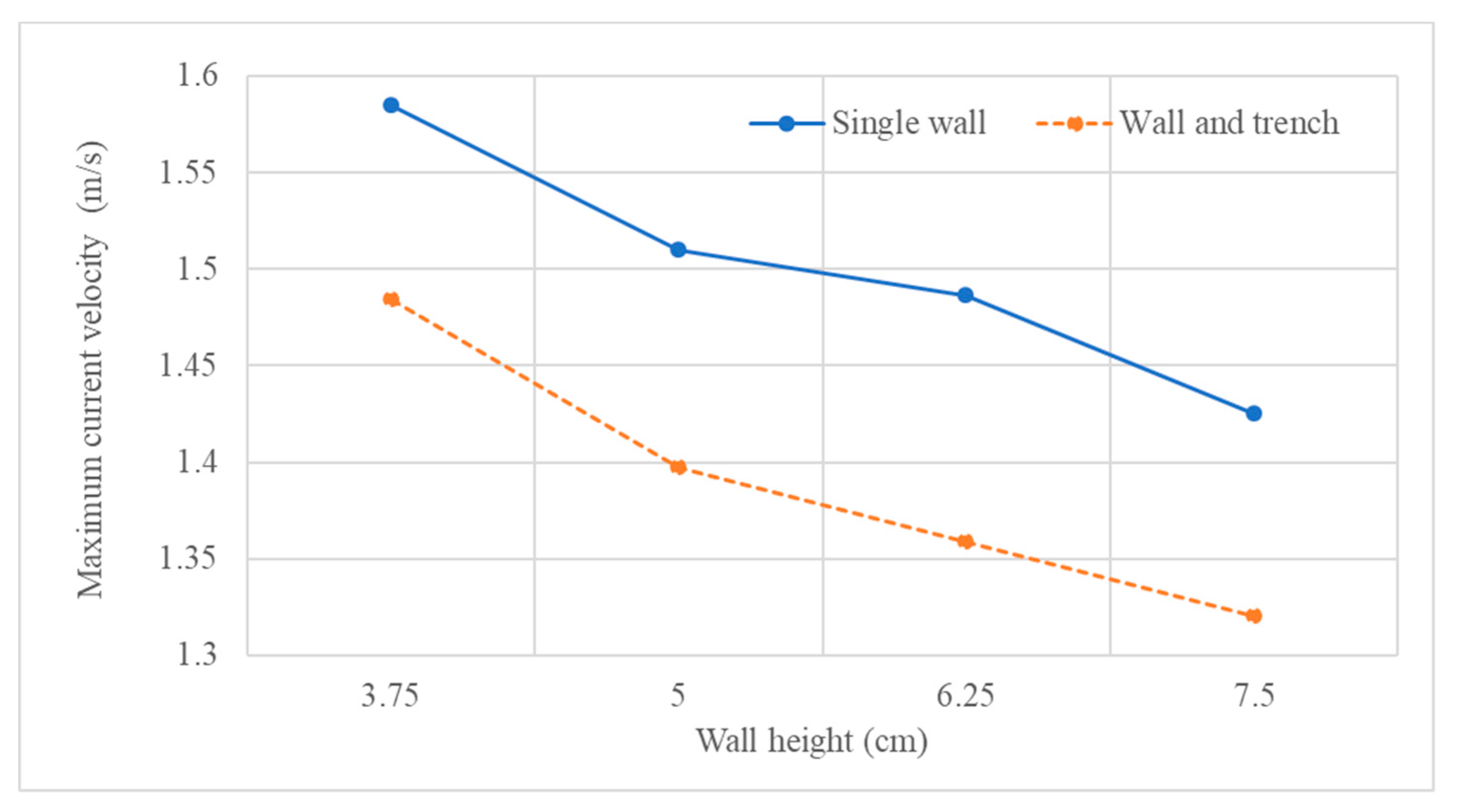
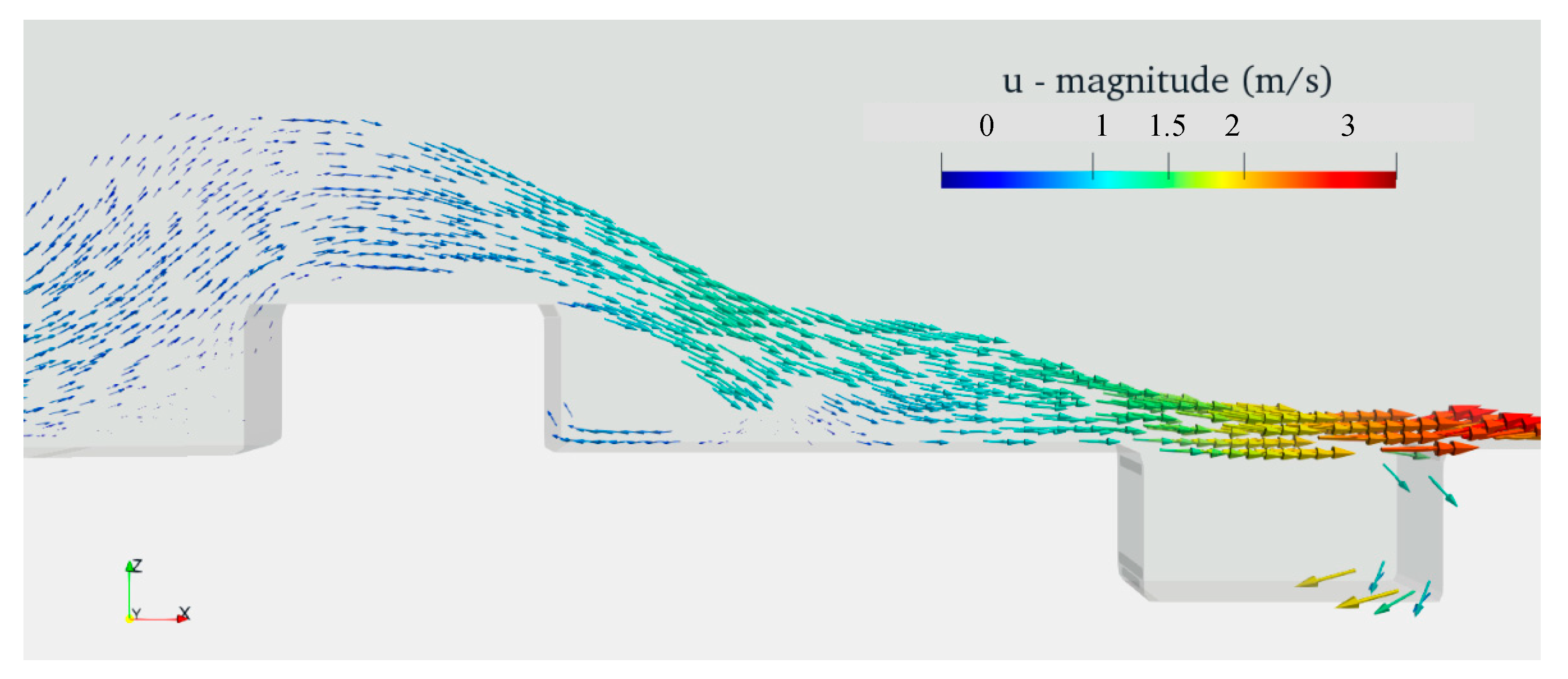
4.3. Comparing the Combined Wall and Trench System with Single Seawall System
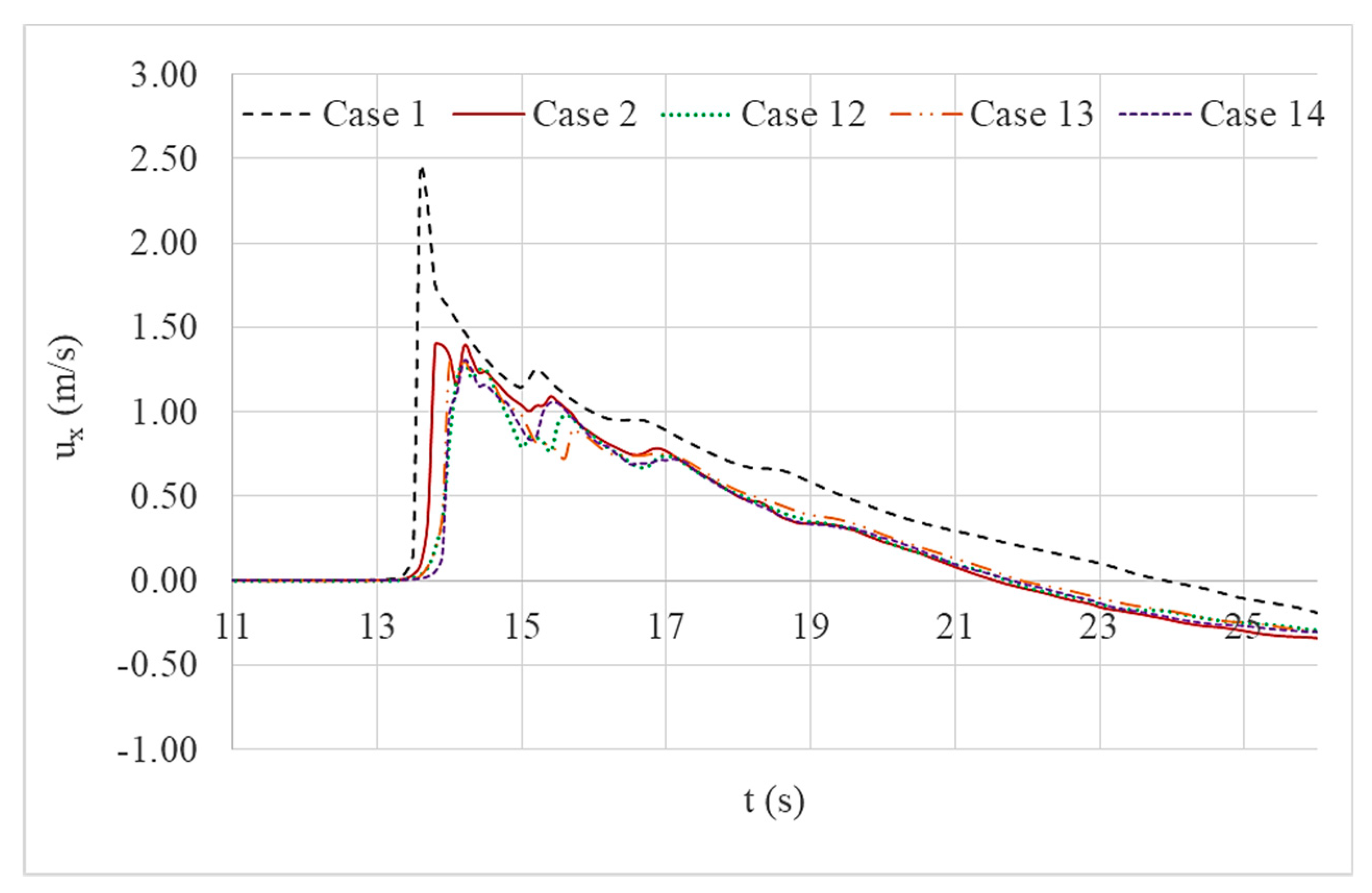
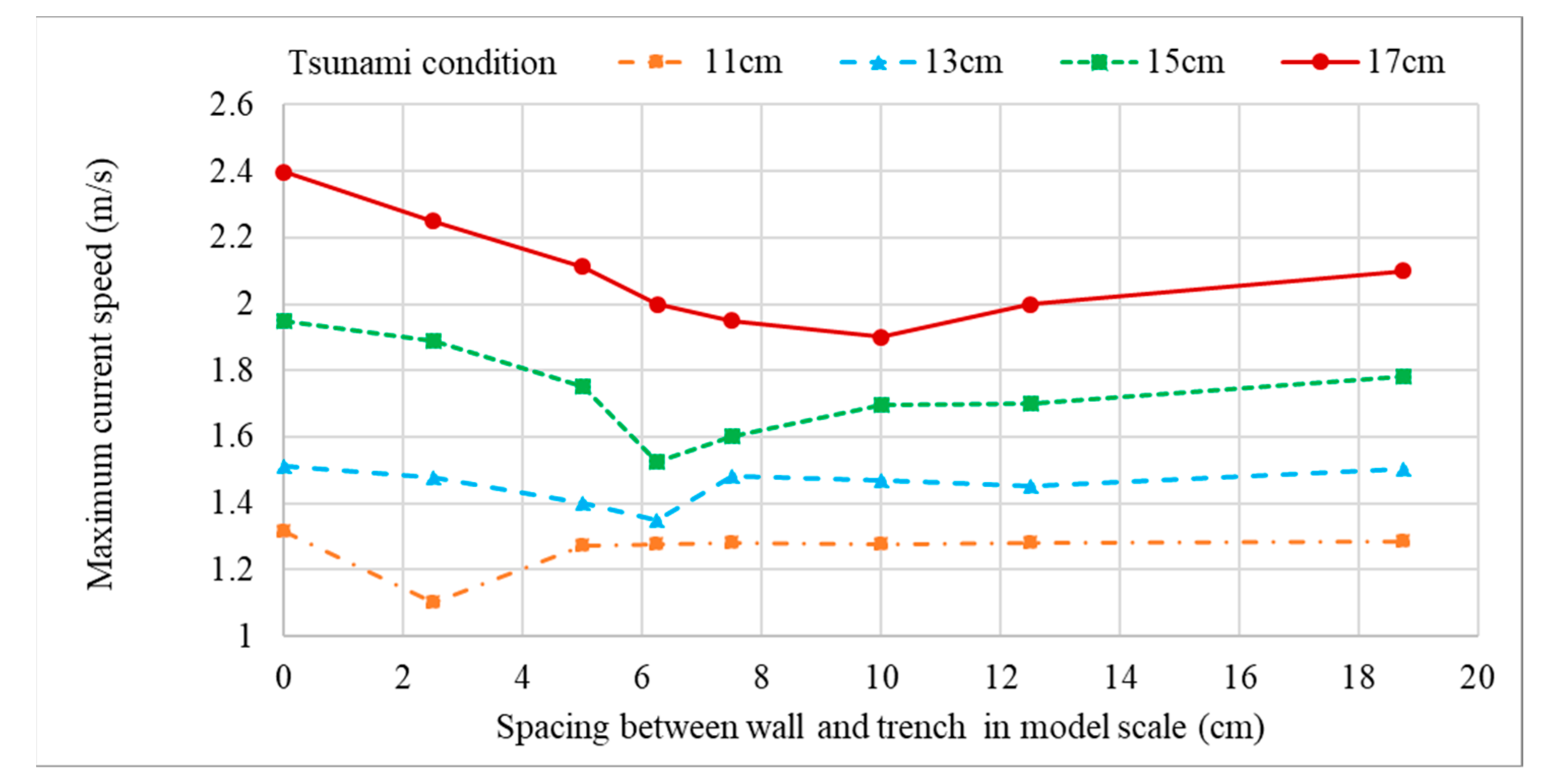
4.4. Evaluating the Behavior of the Structure Ahead of Different Tsunami Conditions
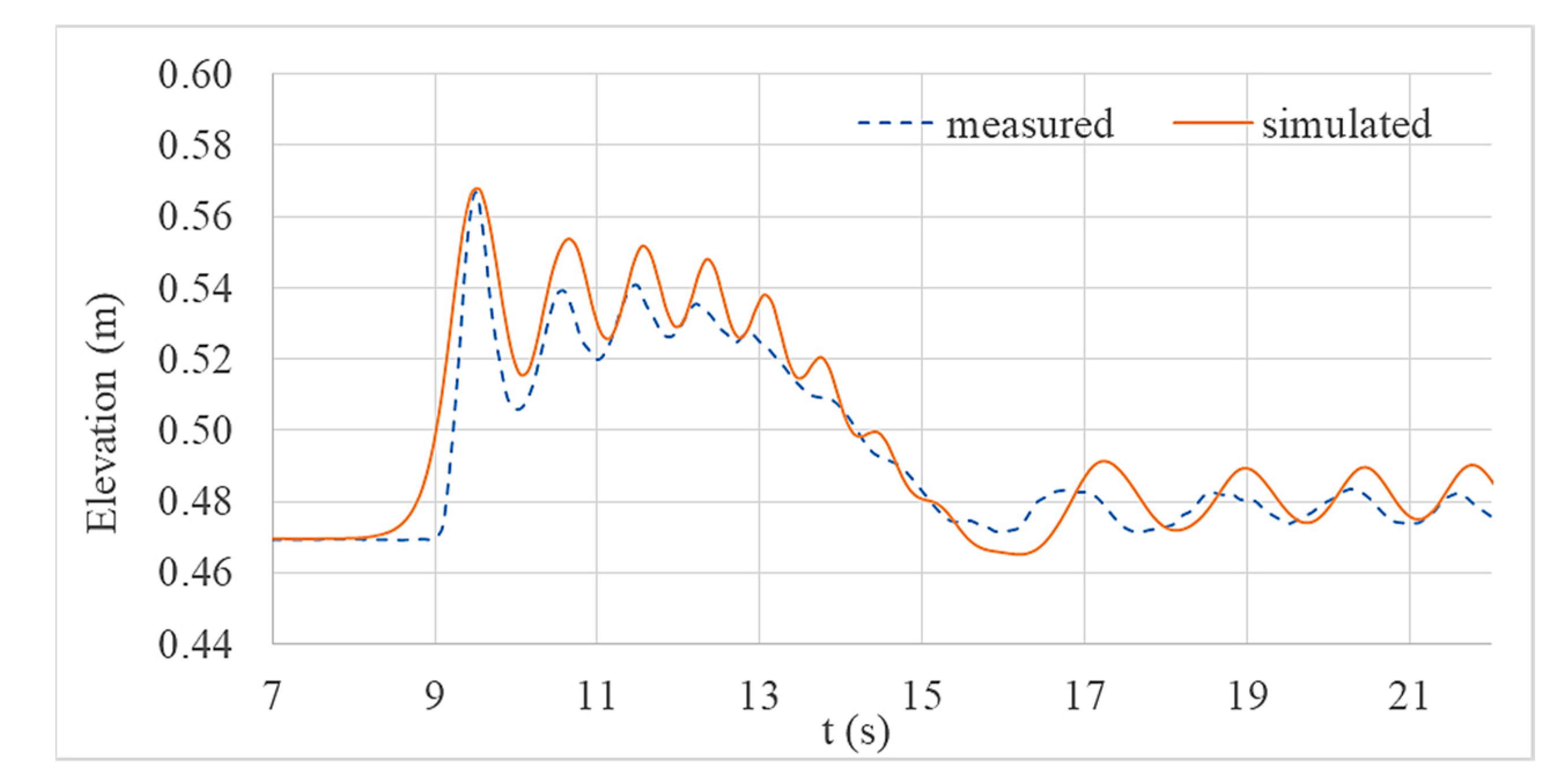
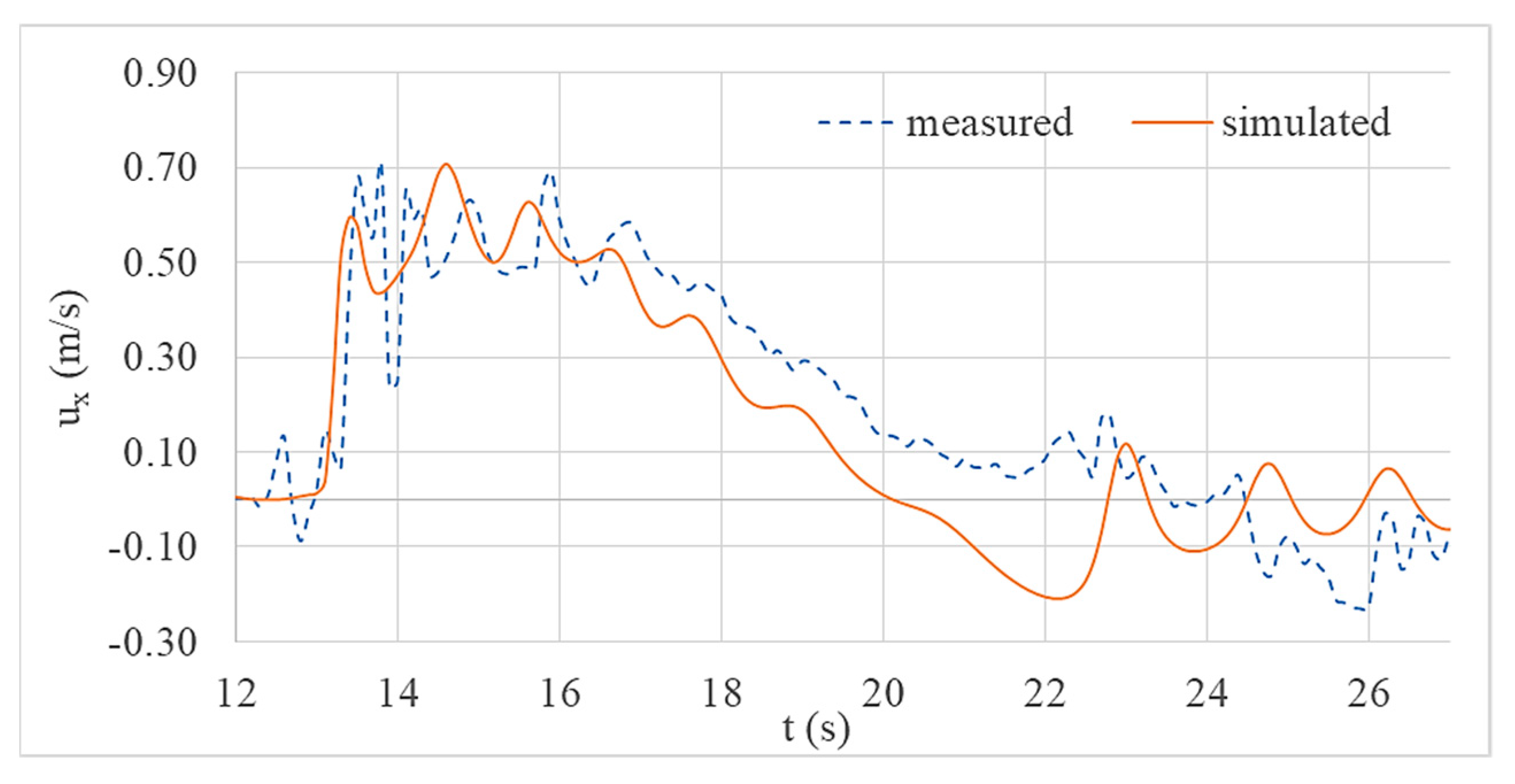
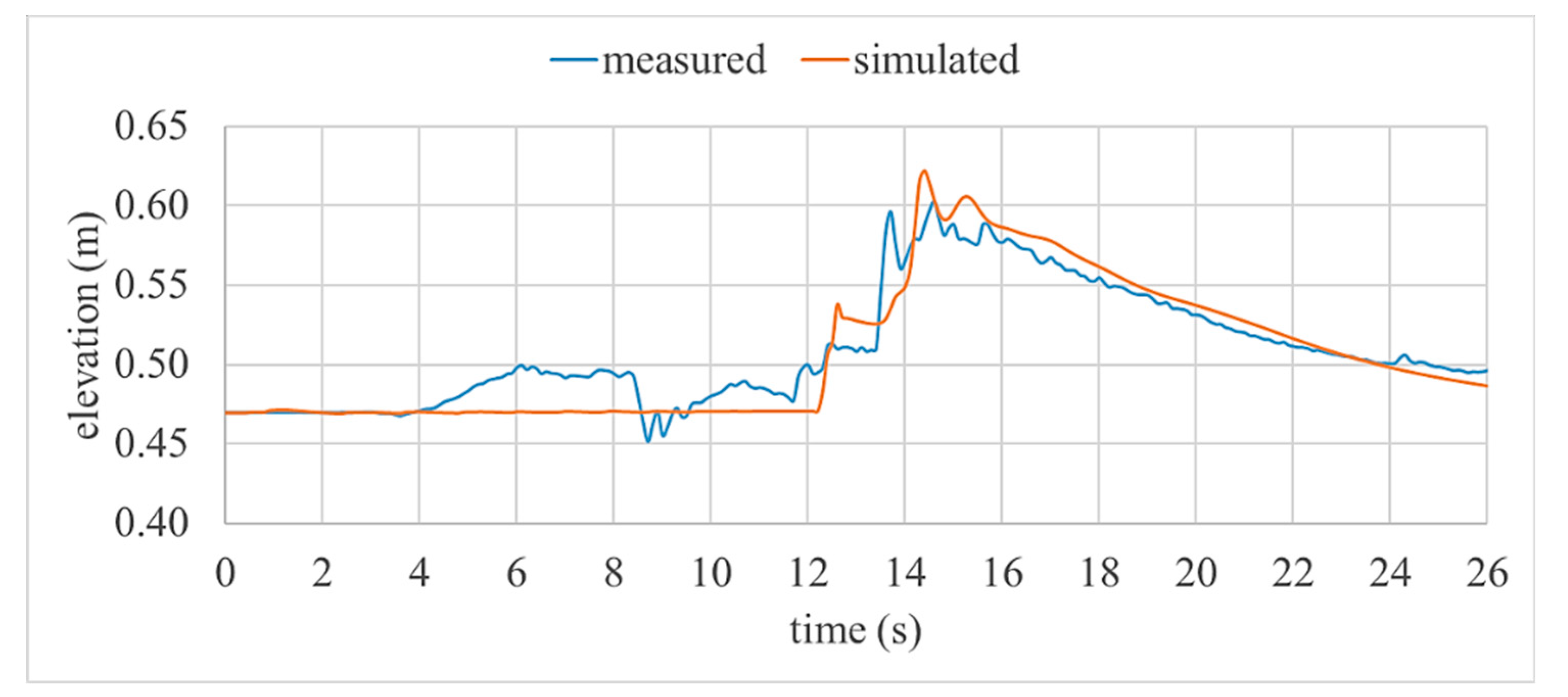
5. Validation of the Numerical Model
6. Conclusions
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Goring, B.D.G.; Raichlen, F. Propagation of longwaves onto shelf. J. Waterw. Port Coast. Ocean Eng. 1992, 118, 43–61. [Google Scholar] [CrossRef]
- Raby, A.; Macabuag, J.; Pomonis, A.; Wilkinson, S.; Rossetto, T. Implications of the 2011 Great East Japan Tsunami on sea defence design. Int. J. Disaster Risk Reduct. 2015, 14, 332–346. [Google Scholar] [CrossRef] [Green Version]
- Synolakis, C.E.; Yalciner, A.C.; Imamura, F.; Fritz, H.M.; Suppasri, A.; Mas, E.; Kalligeris, N.; Necmioglu, O.; Ozer, C.; Zaytsev, A.; et al. Field survey of the coastal impact of the 11 March 2011 great East Japan tsunami. In Proceedings of the European Geosciences Union (EGU) General Assembly, Antalya, Turkey, 31 October–1 November 2011; p. 1. [Google Scholar]
- Rahman, M.M.; Schaab, C.; Nakaza, E. Experimental and numerical modeling of tsunami mitigation by canals. J. Waterw. Port Coast. Ocean Eng. 2017, 143, 1–11. [Google Scholar] [CrossRef]
- Kimura, S. When a seawall is visible: Infrastructure and obstruction in post-tsunami reconstruction in Japan. Sci. Cult. 2016, 25, 23–43. [Google Scholar] [CrossRef] [Green Version]
- Hsiao, S.C.; Lin, T.C. Tsunami-like solitary waves impinging and overtopping an impermeable seawall: Experiment and RANS modeling. Coast. Eng. 2010, 57, 1–18. [Google Scholar] [CrossRef]
- Esteban, M.; Glasbergen, T.; Takabatake, T.; Hofland, B.; Nishizaki, S.; Nishida, Y.; Stolle, J.; Nistor, I.; Bricker, J.; Takagi, H.; et al. Overtopping of coastal structures by tsunami waves. Geosciences 2017, 7, 121. [Google Scholar] [CrossRef] [Green Version]
- Zaha, T.; Tanaka, N.; Kimiwada, Y. Flume experiments on optimal arrangement of hybrid defense system comprising an embankment, moat, and emergent vegetation to mitigate inundating tsunami current. Ocean Eng. 2019, 173, 45–57. [Google Scholar] [CrossRef]
- Muhammad, R.A.H.; Tanaka, N. Energy reduction of a tsunami current through a hybrid defense system comprising a sea embankment followed by a coastal forest. Geosciences 2019, 9, 247. [Google Scholar] [CrossRef] [Green Version]
- Rao, R.; Vijayaraghavan, B.; Sarma, S.; Satyanarayanan, M. Buckingham Canal saved people in Andhra Pradesh (India) from the tsunami of 26 December 2004. Curr. Sci. 2005, 89, 12–13. [Google Scholar]
- Tokida, K.; Tanimoto, R. Lessons and views on hardware countermeasures with earth banks against tsunami estimated in 2011 Great East Japan Earthquake. In Proceedings of the International Symposium on Engineering Lessons Learned from the 2011 Great East Japan Earthquake, Tokyo, Japan, 1–4 March 2012; pp. 1–4. [Google Scholar]
- Dao, N.X.; Adithyawan, M.B.; Tanaka, H. Sensitivity analysis of shore-parallel canal for tsunami wave energy reduction. J. Japan Soc. Civ. Eng. Ser. B3 Ocean Eng. 2013, 69, I_401–I_406. [Google Scholar] [CrossRef] [Green Version]
- Silva, A.; Araki, S. Submerged wall-trench systems to suppress tsunami impact on coast. Proc. Int. Offshore Polar Eng. Conf. 2019, 3, 3253–3260. [Google Scholar]
- NOWPHAS. Obseved Water Level Data of the Great East Japan Tsunami. Available online: https://nowphas.mlit.go.jp/pastdata/ (accessed on 5 January 2020).
- Grilli, S.T.; Ioualalen, M.; Asavanant, J.; Shi, F.; Kirby, J.T.; Watts, P. Source constraints and model simulation of the December 26, 2004, Indian Ocean tsunami. J. Waterw. Port Coast Ocean. Eng. 2007, 133, 414–428. [Google Scholar] [CrossRef] [Green Version]
- Damián, S.M.; Nigro, M.N. An extended mixture model for the simultaneous treatment of small-scale and large-scale interfaces. Inter. J. Numer. Methods Fluids 2014, 75, 547–574. [Google Scholar] [CrossRef]
- Lopes, P.M.B. Free-Surface Flow Interface and Air-Entrainment Modelling Using OpenFOAM; Thesis Project in Hydraulic, Water Resources and Environment Doctoral Program in Civil Engineering; University of Coimbra: Coimbra, Portugal, 2013. [Google Scholar]
- Jasak, H. Error Analysis and Estimation for Finite Volume Method with Applications to Fluid Flow; Imperial College of Science, Technology and Medicine: London, UK, 1996. [Google Scholar]
- Ubbink, O. Numerical Prediction of Two Fluid Systems with Sharp Interfaces; University of London: Lodon, UK, 1997. [Google Scholar]
- Rusche, H. Computational Dispersed Two-Phase Dynamics Flows of At Phase Fractions. Ph.D. Thesis, University of London, London, UK, 2003. [Google Scholar]
- Deshpande, S.S.; Anumolu, L.; Trujillo, M.F. Evaluating the performance of the two-phase flow solver interFoam. Comput. Sci. Discov. 2012, 5, 014016. [Google Scholar] [CrossRef]
- Cox, D.T.; Kobayashi, N.; Okayasu, A. Vertical variations of fluid velocities and shear stress in surf zones. Proc. Coast. Eng. Conf. 1995, 1, 98–112. [Google Scholar] [CrossRef]
- Park, I.R.; Kim, K.S.; Kim, J.; Van, S.H. Numerical investigation of the effects of turbulence intensity on dam-break flows. Ocean. Eng. 2012, 42, 176–187. [Google Scholar] [CrossRef]
- Zhainakov, A.Z.; Kurbanaliev, A.Y. Verification of the open package OpenFOAM on dam break problems. Thermophys. Aeromechanics 2013, 20, 451–461. [Google Scholar] [CrossRef]







| ControlDict | Scheme/Value |
| adjustTimeStep | true |
| maxCo | 0.5 |
| maxAlphaCo | 0.5 |
| fvSchemes | Scheme/Value |
| ddt | Euler |
| grad | Gauss linear |
| div(rhoPhi,U) | Gauss linearUpwind grad(U) |
| div(phirb, alpha) | Gauss linear |
| div(phirb, alpha) | Gauss linear |
| div(phi,k) | Gauss upwind |
| div(phi,epsilon) | Gauss upwind |
| laplacian | Gauss linear corrected |
| interplolation | linear |
| snGrad | corrected |
| fvSolution | Scheme/Value |
| alpha.water. (solver, tol, relTol) | smoothSolver, 1 × 10−8, 0 |
| pcorr(solver, prec, tol, relTol) | PCG, DIC, 1 × 10−8, 0 |
| p_rgh(solver, prec, tol, relTol) | PCG, DIC, 1 × 10−8, 0 |
| U|K|epsilon | smoothSolver, 1 × 10−8, 0 |
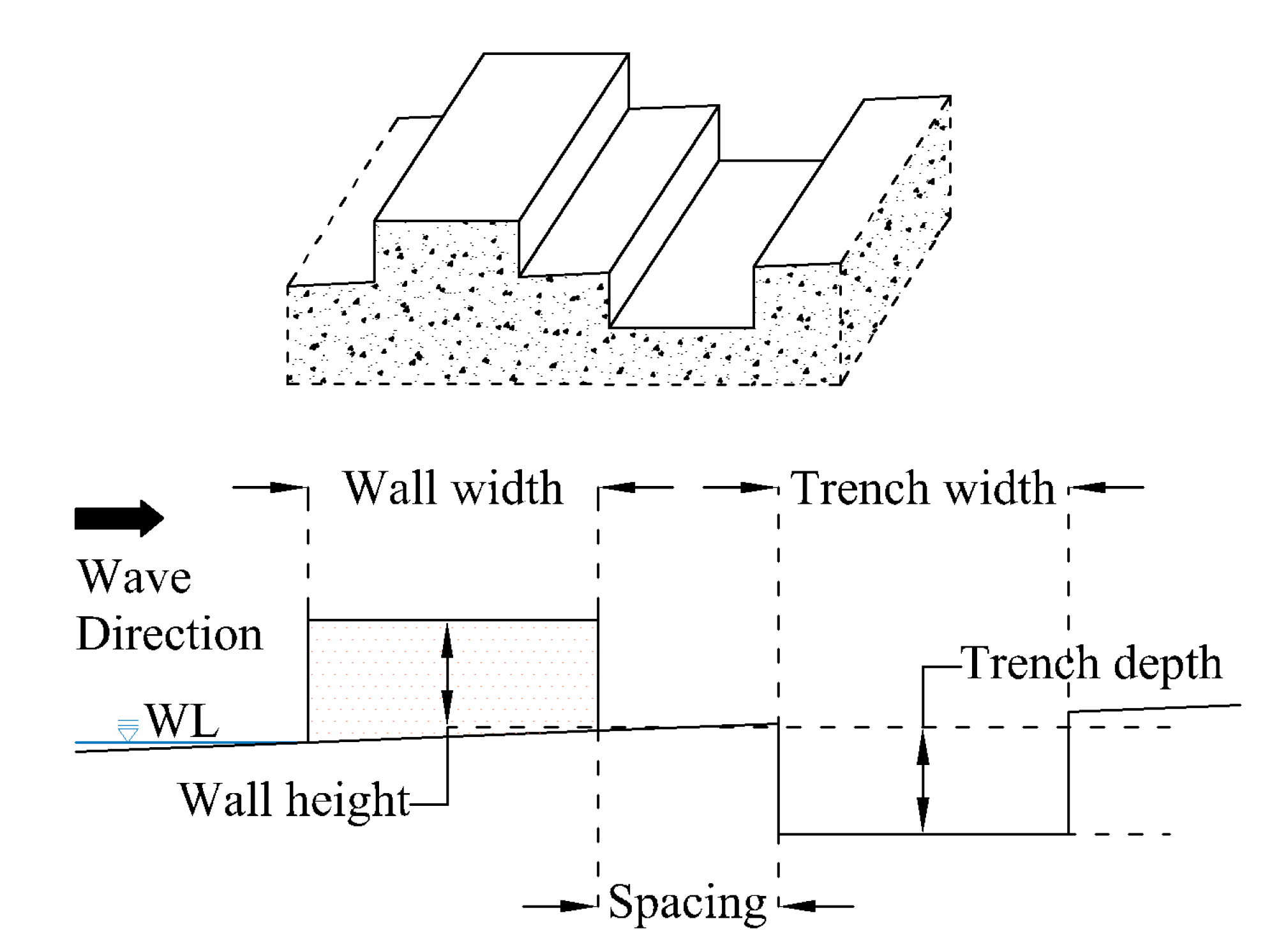
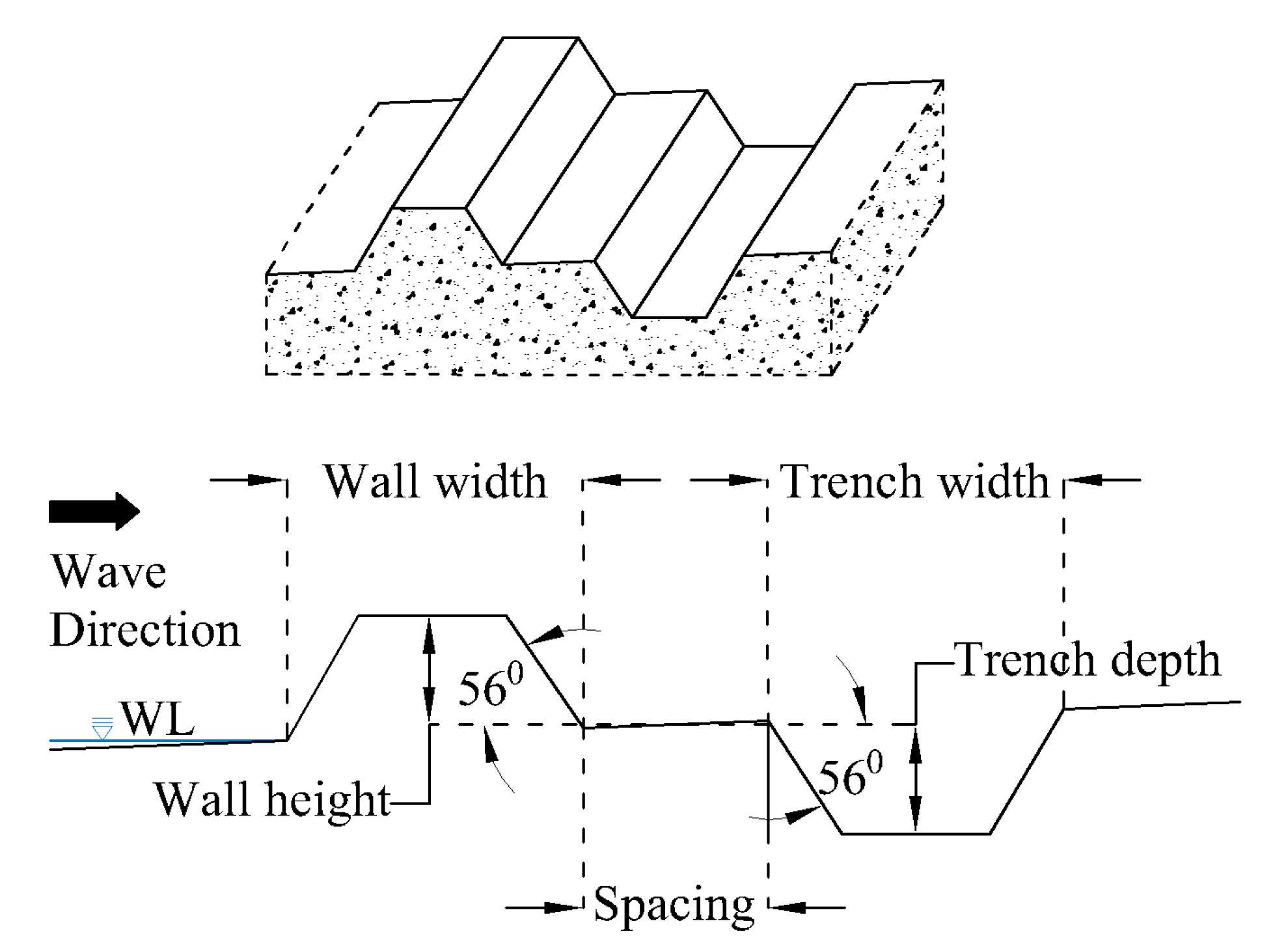
| Case No. | Description | Wall Height (cm) | Wall Width (cm) | Trench Depth (cm) | Trench Width (cm) | Spacing (cm) |
|---|---|---|---|---|---|---|
| 1 | Without Structures | - | - | - | - | - |
| 2 | Single wall | 6.25 | 12.5 | - | - | - |
| 3 | Wall-trench | 6.25 | 12.5 | 6.25 | 12.5 | 6.25 |
| 4 | Wall-trench | 6.25 | 12.5 | 6.25 | 12.5 | 12.5 |
| 5 | Wall-trench | 6.25 | 12.5 | 6.25 | 12.5 | 18.75 |
| 6 | Wall-trench | 6.25 | 12.5 | 6.25 | 6.25 | 12.5 |
| 7 | Wall-trench | 6.25 | 12.5 | 6.25 | 12.5 | 12.5 |
| 8 | Wall-trench | 6.25 | 12.5 | 6.25 | 18.75 | 12.5 |
| 9 | Wall-trench | 6.25 | 6.25 | 6.25 | 12.5 | 12.5 |
| 10 | Wall-trench | 6.25 | 12.5 | 6.25 | 12.5 | 12.5 |
| 11 | Wall-trench | 6.25 | 18.75 | 6.25 | 12.5 | 12.5 |
| 12 | Wall-trench | 6.25 | 12.5 | 3.125 | 12.5 | 12.5 |
| 13 | Wall-trench | 6.25 | 12.5 | 6.25 | 12.5 | 12.5 |
| 14 | Wall-trench | 6.25 | 12.5 | 9.375 | 12.5 | 12.5 |
| Case No. | Description | Wall Height (cm) | Wall Width (cm) | Trench Depth (cm) | Trench Width (cm) | Spacing (cm) |
|---|---|---|---|---|---|---|
| 15 | Single wall | 5 | 12.5 | - | - | - |
| 16 | Single wall | 6.25 | 12.5 | - | - | - |
| 17 | Single wall | 7.5 | 12.5 | - | - | - |
| 18 | Single wall | 3.75 | 12.5 | 6.25 | 12.5 | 6.25 |
| 19 | Wall-trench | 5 | 12.5 | 6.25 | 12.5 | 6.25 |
| 20 | Wall-trench | 6.25 | 12.5 | 6.25 | 12.5 | 6.25 |
| 21 | Wall-trench | 7.5 | 12.5 | 6.25 | 12.5 | 6.25 |
| 22 | Wall-trench | 5 | 12.5 | - | - | - |
| Tsunami Condition (cm) | Description | Wall Height (cm) | Wall Width (cm) | Trench Depth (cm) | Trench Width (cm) | Spacing (cm) | |||
|---|---|---|---|---|---|---|---|---|---|
| 11 | 13 | 15 | 17 | ||||||
| Case no. | |||||||||
| 23 | 24 | 25 | 26 | Wall-trench | 6.25 | 12.5 | 6.25 | 12.5 | 0 |
| 27 | 28 | 29 | 30 | Wall-trench | 6.25 | 12.5 | 6.25 | 12.5 | 2.5 |
| 31 | 32 | 33 | 34 | Wall-trench | 6.25 | 12.5 | 6.25 | 12.5 | 5 |
| 35 | 36 | 37 | 38 | Wall-trench | 6.25 | 12.5 | 6.25 | 12.5 | 6.25 |
| 39 | 40 | 41 | 42 | Wall-trench | 6.25 | 12.5 | 6.25 | 12.5 | 7.5 |
| 43 | 44 | 45 | 46 | Wall-trench | 6.25 | 12.5 | 6.25 | 12.5 | 10 |
| 47 | 48 | 49 | 50 | Wall-trench | 6.25 | 12.5 | 6.25 | 12.5 | 12.5 |
| 51 | 52 | 53 | 54 | Wall-trench | 6.25 | 12.5 | 6.25 | 12.5 | 18.75 |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Silva, A.; Araki, S. Investigating the Behavior of an Onshore Wall and Trench Combination Ahead of a Tsunami-Like Wave. Geosciences 2020, 10, 310. https://doi.org/10.3390/geosciences10080310
Silva A, Araki S. Investigating the Behavior of an Onshore Wall and Trench Combination Ahead of a Tsunami-Like Wave. Geosciences. 2020; 10(8):310. https://doi.org/10.3390/geosciences10080310
Chicago/Turabian StyleSilva, Akalanka, and Susumu Araki. 2020. "Investigating the Behavior of an Onshore Wall and Trench Combination Ahead of a Tsunami-Like Wave" Geosciences 10, no. 8: 310. https://doi.org/10.3390/geosciences10080310
APA StyleSilva, A., & Araki, S. (2020). Investigating the Behavior of an Onshore Wall and Trench Combination Ahead of a Tsunami-Like Wave. Geosciences, 10(8), 310. https://doi.org/10.3390/geosciences10080310




