Optimization of Axial Magnetic Bearing Actuators for Dynamic Performance
Abstract
:1. Introduction
2. Thrust Magnetic Bearing Model and Performance
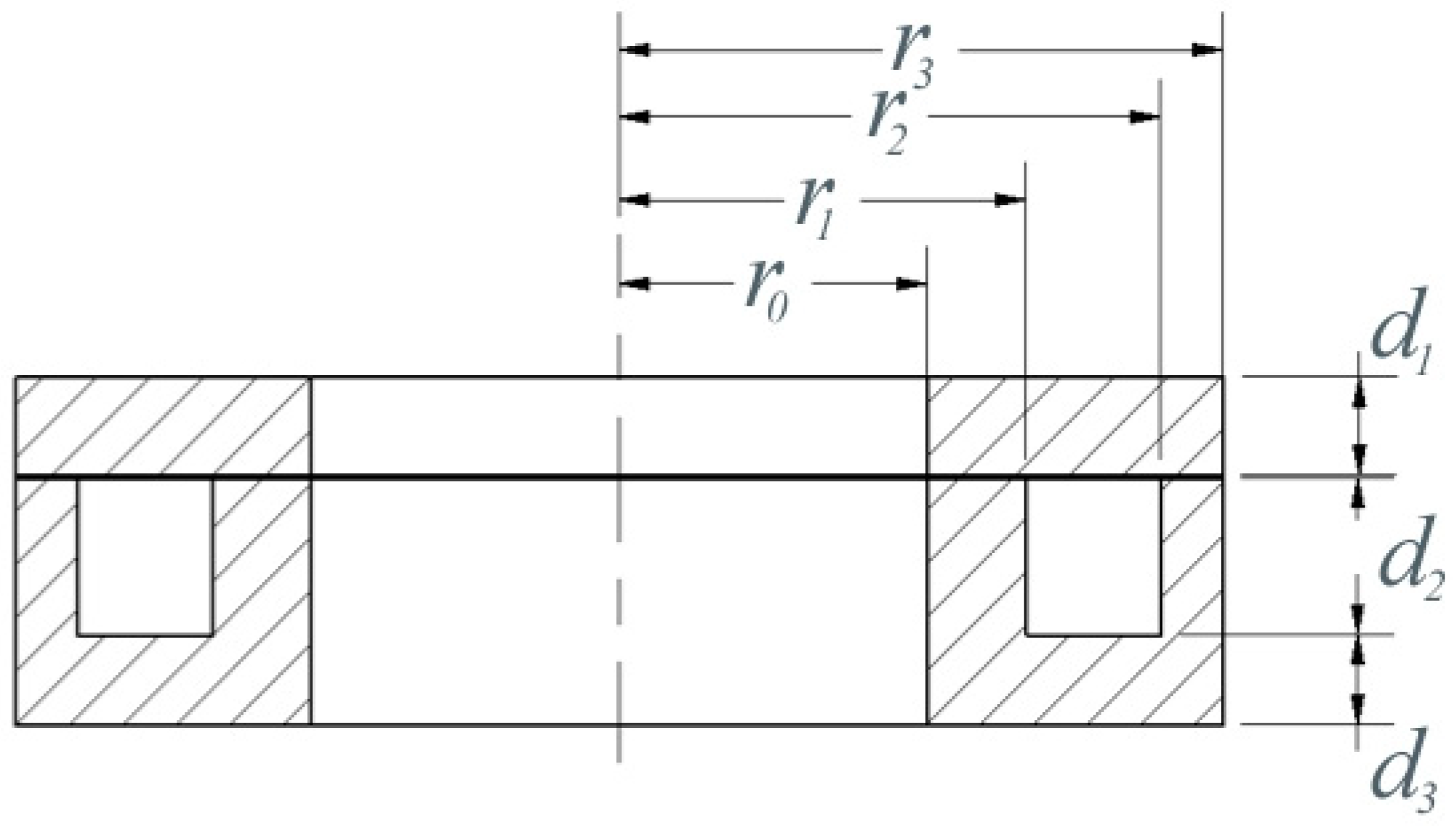
2.1. Axial Actuator Model
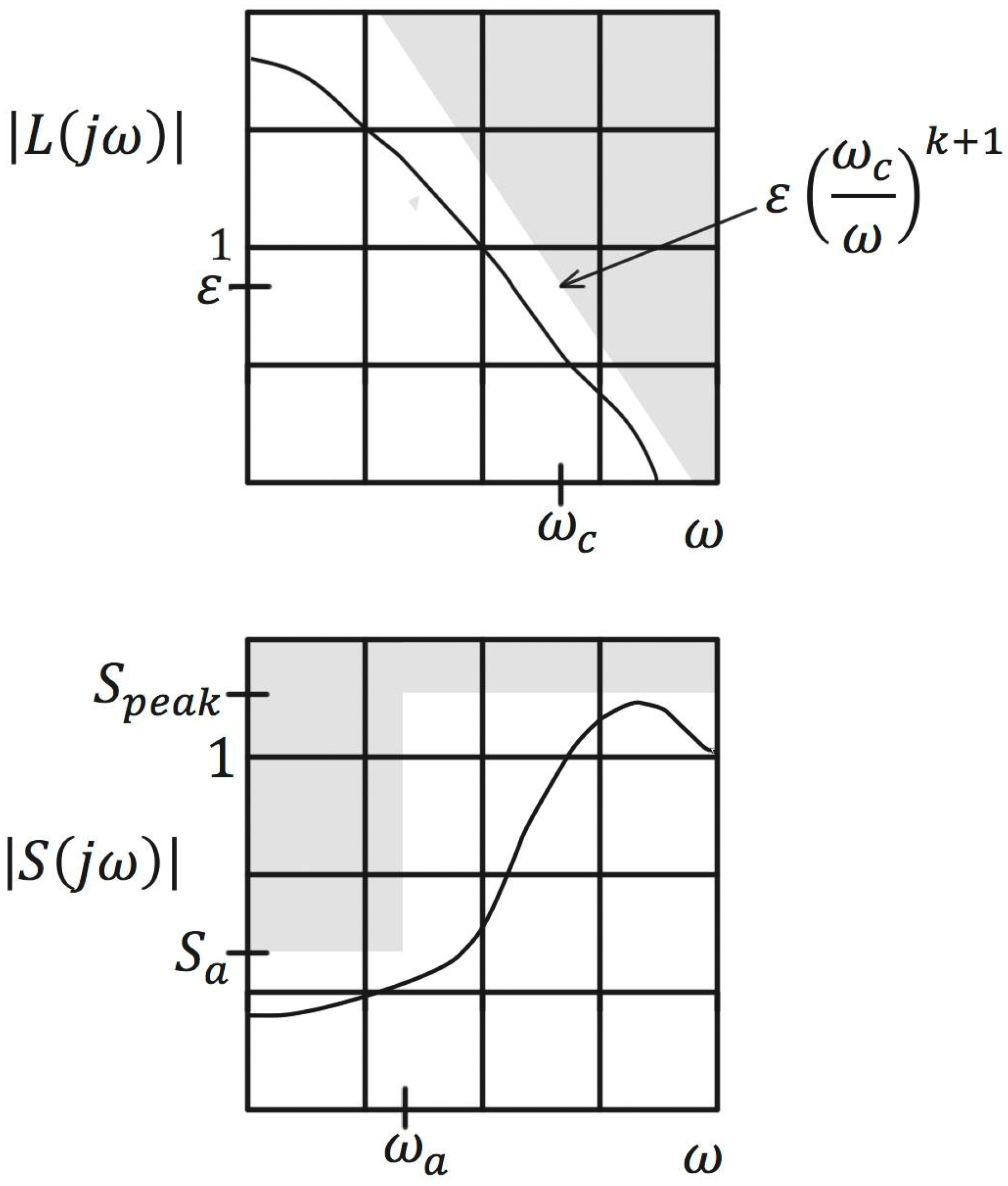
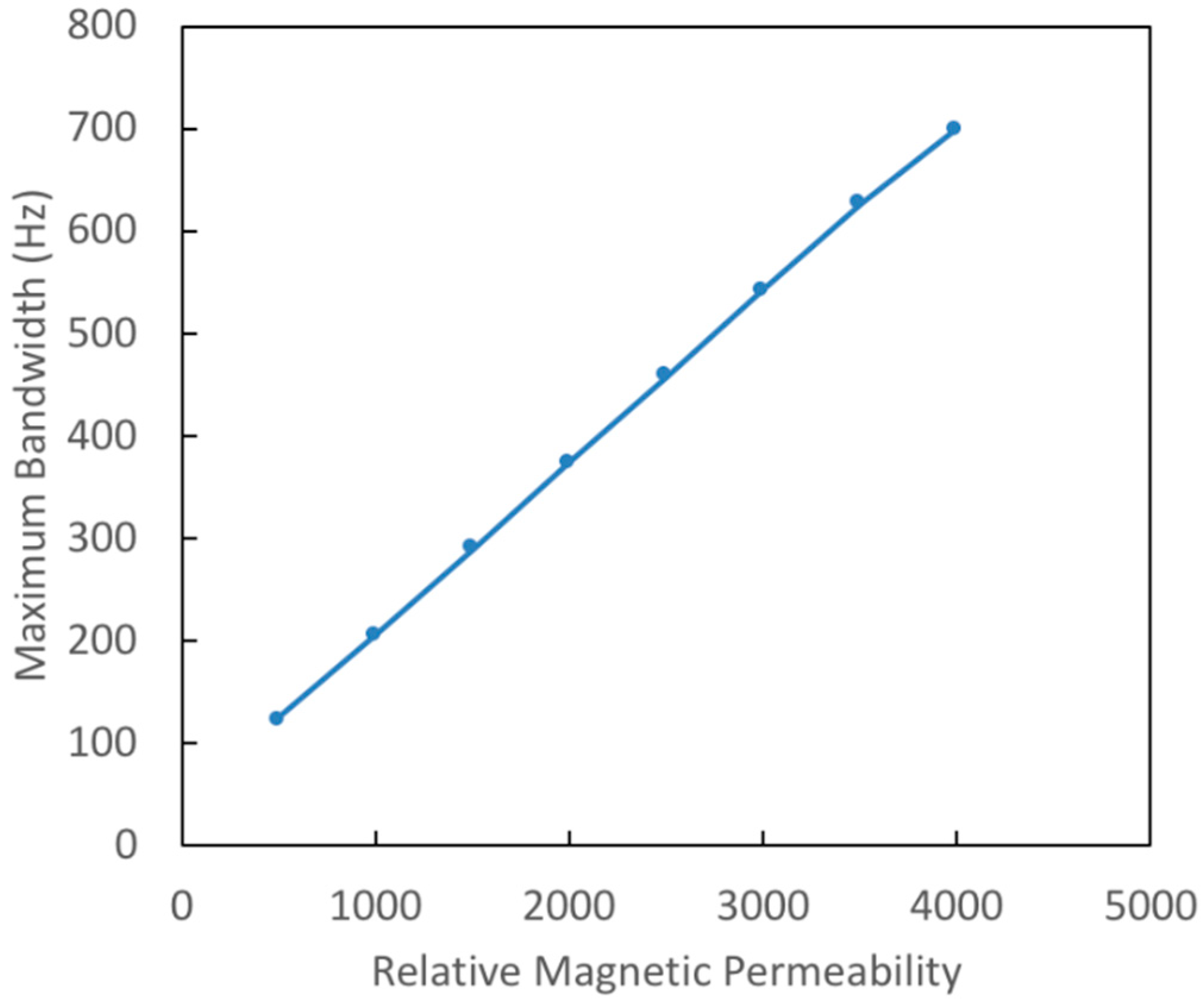
2.2. The Importance of Magnetic Actuator Bandwidth
3. Optimization
3.1. Design Constraints
- Outer radius constraint—the outer radius () must not exceed a bound determined either by the available space within the housing or the allowable hoop stress in the thrust disk at maximum speed;
- Inner radius constraint—the inner radius () must be sufficient to accommodate the shaft and allow for assembly;
- Axial length constraint—the total length of the actuator must not exceed a bound determined from the machine design and its rotordynamics;
- Peak force constraint—adequate pole face area must be provided so as to generate the specified maximum force. Furthermore, the cross-sectional area of all segments of the flux path must be greater than or equal to that of the pole face, thus, ensuring that the material’s saturation flux density can be achieved at the pole face;
- Continuous force constraint—the cross-sectional area of the coil must be sufficiently large so as to provide the number of ampere-turns required to generate the specified continuous force without causing coil overheating.
3.2. Optimization Method
3.3. Pareto-Optimal Curves
4. Results
4.1. Design Example
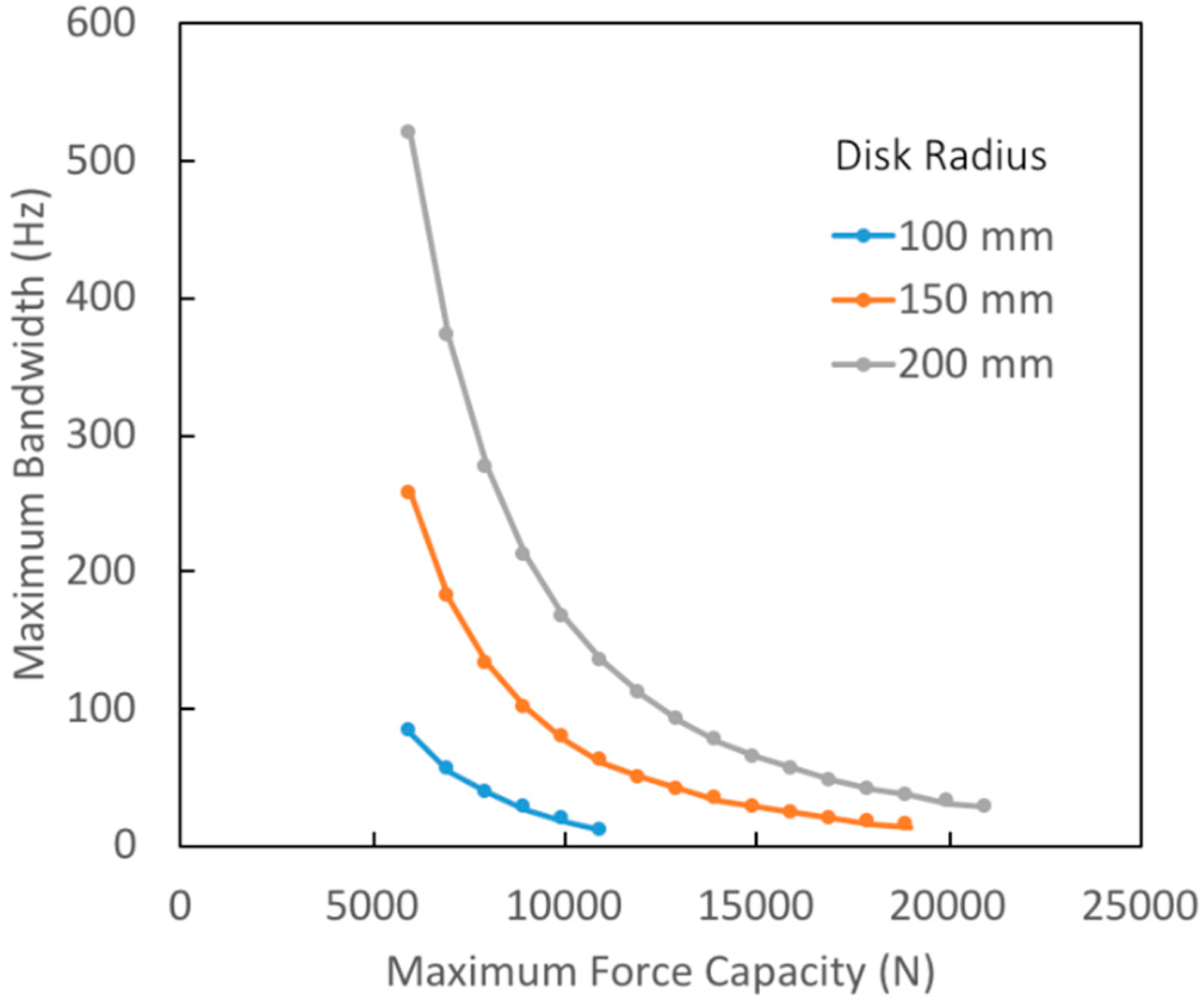
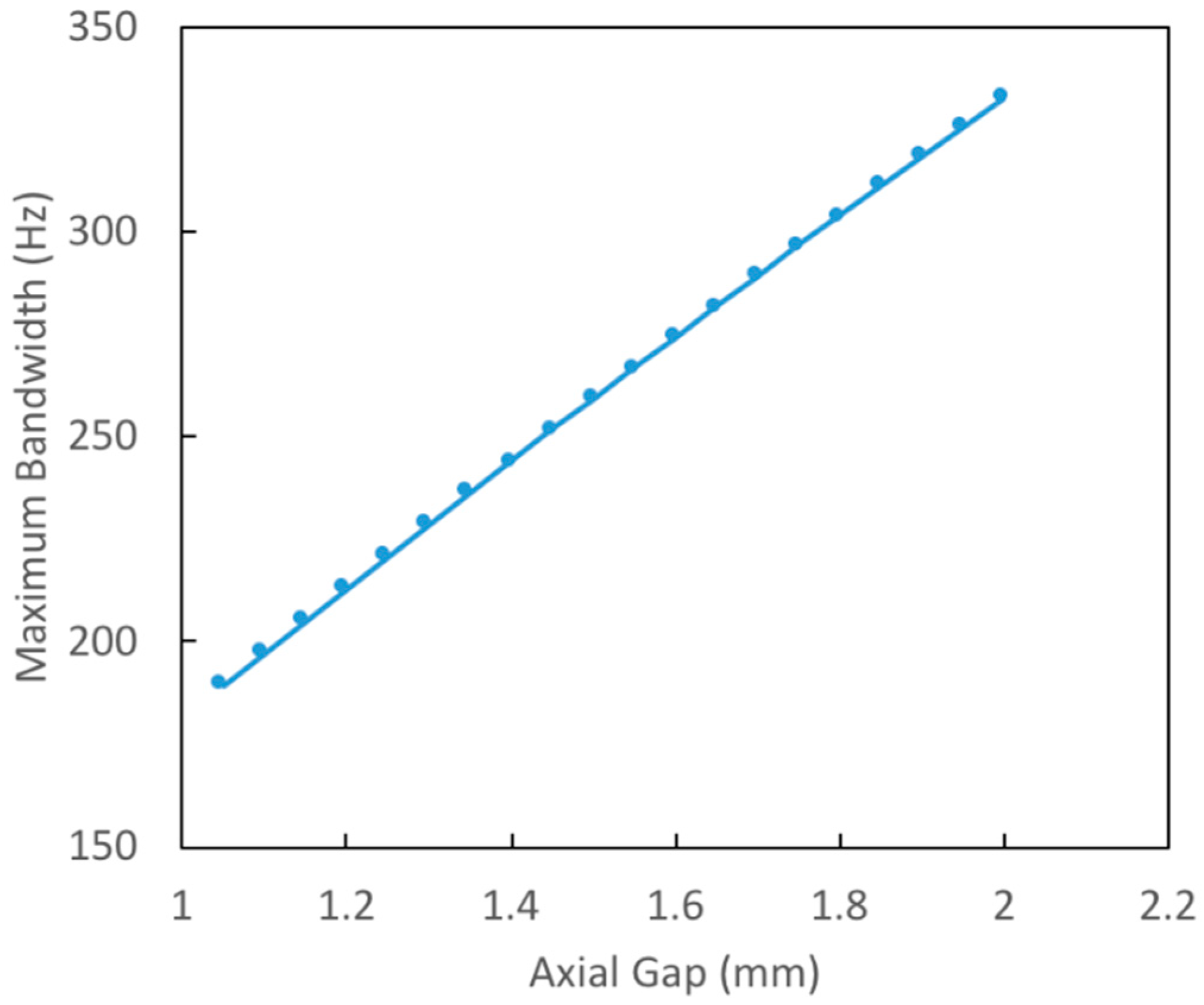
4.2. Pareto-Optimal Results
5. Discussion
6. Conclusions
Author Contributions
Funding
Conflicts of Interest
Appendix A
Appendix B
References
- An, D.K.; Zmood, R.B.; Kirk, J.A. The influence of eddy currents on magnetic actuator performance. Proc. IEEE 1987, 75, 259–260. [Google Scholar]
- Feeley, J. A simple dynamic model for eddy currents in a magnetic actuator. IEEE Trans. Magn. 1996, 32, 453–458. [Google Scholar] [CrossRef]
- Kucera, L.; Ahrens, M. A model for axial magnetic bearings including eddy currents. In Proceedings of the Third International Symposium on Magnetic Suspension Technology, Tallahassee, FL, USA, 13–15 December 1995; pp. 421–438. [Google Scholar]
- Zhu, L.; Knospe, C. Modeling of nonlaminated electromagnetic suspension systems. IEEE/ASME Trans. Mechatron. 2010, 15, 59. [Google Scholar]
- Zhu, L.; Knospe, C.; Maslen, E. Analytic model for a nonlaminated cylindrical magnetic actuator including eddy currents. IEEE Trans. Magn. 2005, 41, 1248–1258. [Google Scholar]
- Knospe, C.; Zhu, L. Performance Limitations of Non-laminated Magnetic Suspension Systems. IEEE Trans. Control Syst. Technol. 2010, 19, 327–336. [Google Scholar] [CrossRef]
- Sun, Y.; Ho, Y.-S.; Yu, L. Dynamic stiffnesses of active magnetic thrust bearing including eddy-current effects. IEEE Trans. Magn. 2009, 45, 139–149. [Google Scholar] [CrossRef]
- Whitlow, Z.W. Modeling and Control of Non-Laminated Active Magnetic Thrust Bearings. Master’s Thesis, University of Virginia, Charlottesville, VA, USA, 2014. [Google Scholar]
- Whitlow, Z.; Fittro, R.; Knospe, C. Dynamic Performance of Segmented Active Magnetic Thrust Bearings. IEEE Trans. Magn. 2016, 52, 1–11. [Google Scholar] [CrossRef]
- Freudenberg, J.; Looze, D. A sensitivity tradeoff for plants with time delay. IEEE Trans. Autom. Control 1987, 32, 99–104. [Google Scholar] [CrossRef]
- Yoon, S.; Lin, Z.; Allaire, P. Control of Surge in Centrifugal Compressors by Active Magnetic Bearings; Springer: London, UK, 2013. [Google Scholar]


| Constraint | Mathematical Expression |
|---|---|
| Outer radius | |
| Inner radius | |
| Axial length | |
| Peak force | where |
| Continuous force | where |
| Parameters | |
| Gap (mm) | 1.158 |
| Saturation flux density (Tesla) | 1.2 |
| Relative permeability | 1000 |
| Iron conductivity (MS/m) | 2 |
| Maximum coil current density (A/cm2) | 300 |
| Constraints | |
| Outer radius (mm) | 150 |
| Inner radius (mm) | 50 |
| Axial length (mm) | not active |
| Peak force (N) | 6600 |
| Continuous force (N) |
| Dimension | Nominal Value | Optimized Value |
| (mm) | 51.7 | 114.7 |
| (mm) | 68.3 | 122.5 |
| (mm) | 90.9 | 143.8 |
| (mm) | 101.3 | 150.0 |
| (mm) | 16.5 | 7.5 |
| (mm) | 26.3 | 28.0 |
| (mm) | 15.2 | 7.5 |
| Performance | Nominal Value | Optimized Value |
| (Hz) | 51.8 | 206.5 |
© 2018 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Spece, H.; Fittro, R.; Knospe, C. Optimization of Axial Magnetic Bearing Actuators for Dynamic Performance. Actuators 2018, 7, 66. https://doi.org/10.3390/act7040066
Spece H, Fittro R, Knospe C. Optimization of Axial Magnetic Bearing Actuators for Dynamic Performance. Actuators. 2018; 7(4):66. https://doi.org/10.3390/act7040066
Chicago/Turabian StyleSpece, Henry, Roger Fittro, and Carl Knospe. 2018. "Optimization of Axial Magnetic Bearing Actuators for Dynamic Performance" Actuators 7, no. 4: 66. https://doi.org/10.3390/act7040066
APA StyleSpece, H., Fittro, R., & Knospe, C. (2018). Optimization of Axial Magnetic Bearing Actuators for Dynamic Performance. Actuators, 7(4), 66. https://doi.org/10.3390/act7040066






