Offset-Free Model Predictive Control for Active Magnetic Bearing Systems
Abstract
1. Introduction
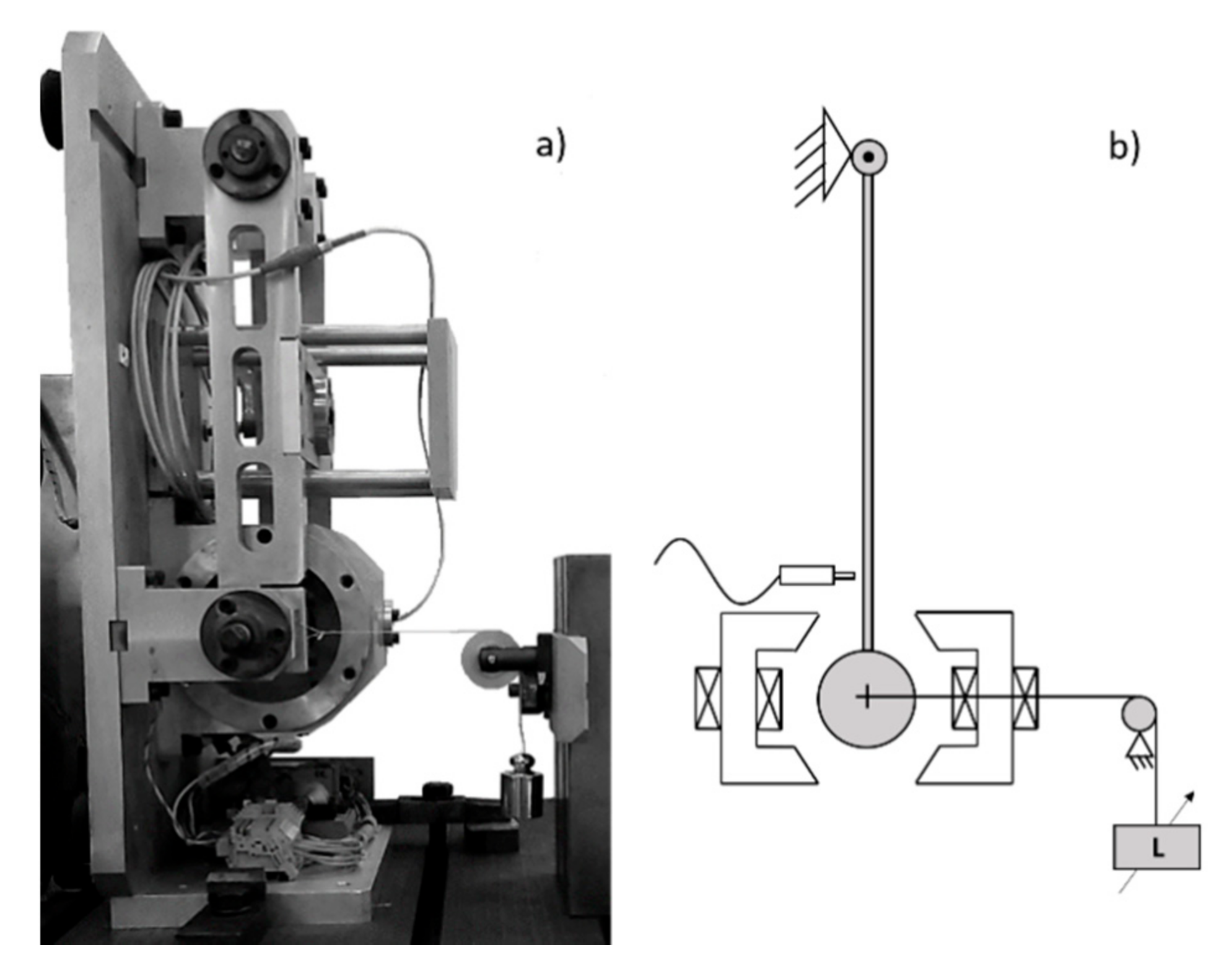
2. Single Degree of Freedom Active Magnetic Bearing System
3. Modeling
4. Offset-Free Model Predictive Control Design
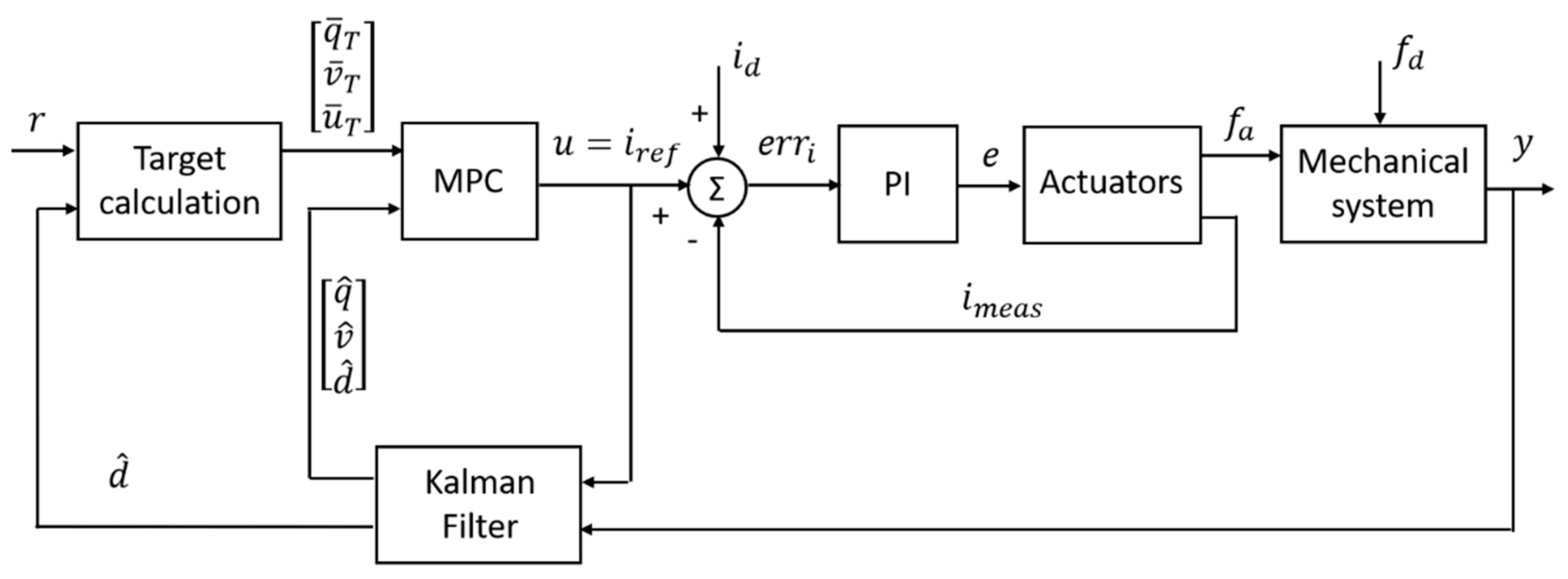
4.1. Control System Architecture
4.2. Target Calculation and MPC Problem Formulation
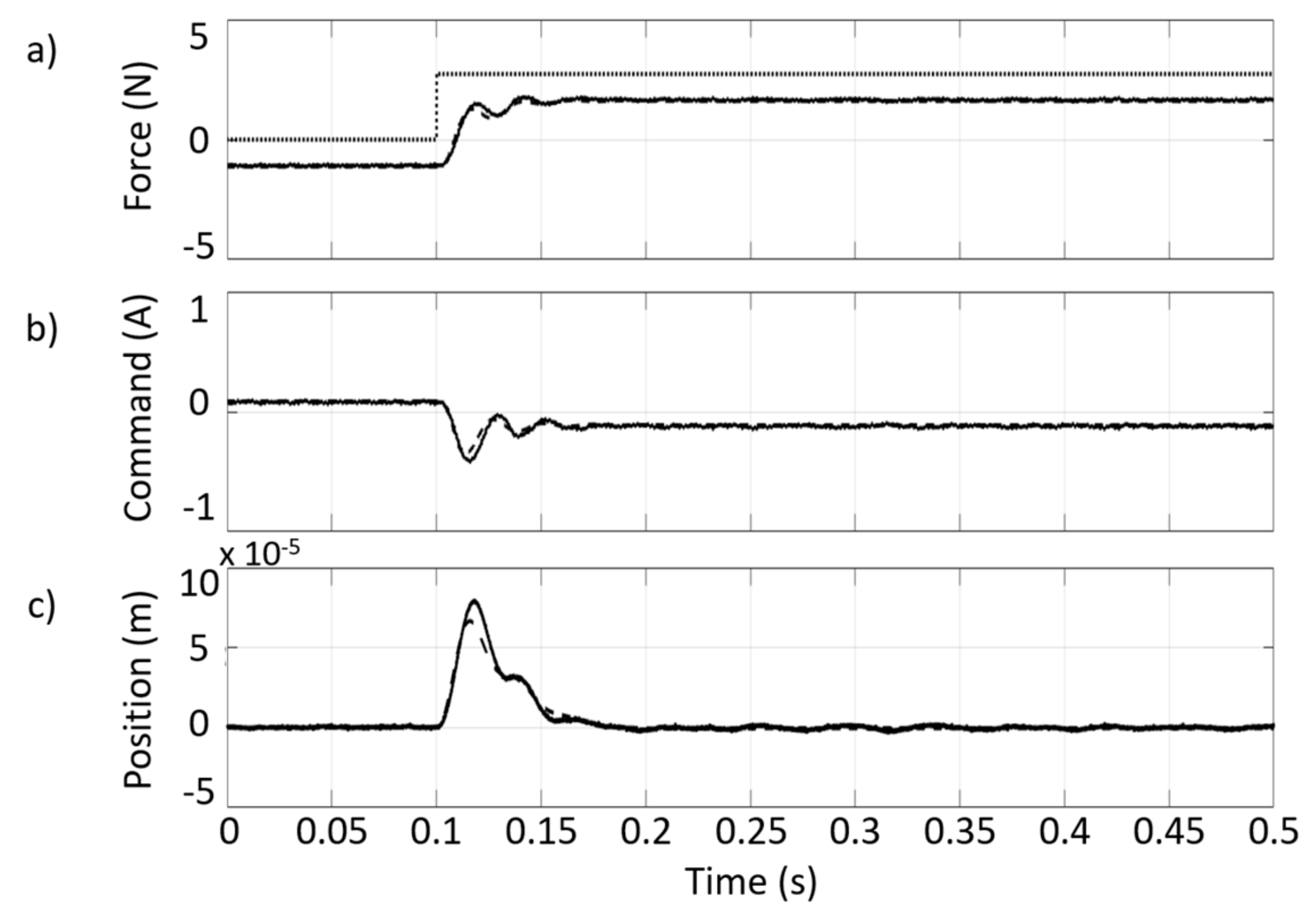
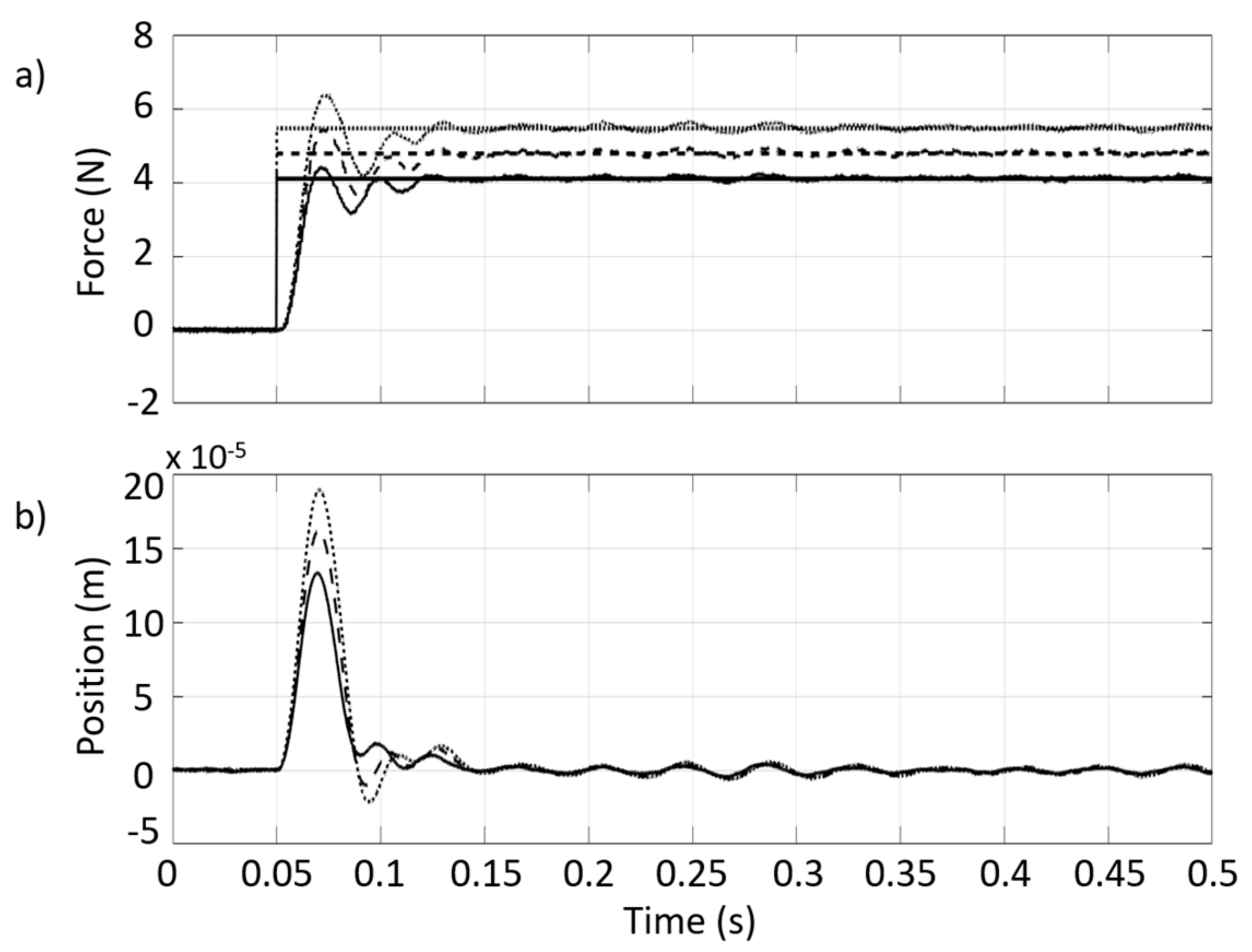
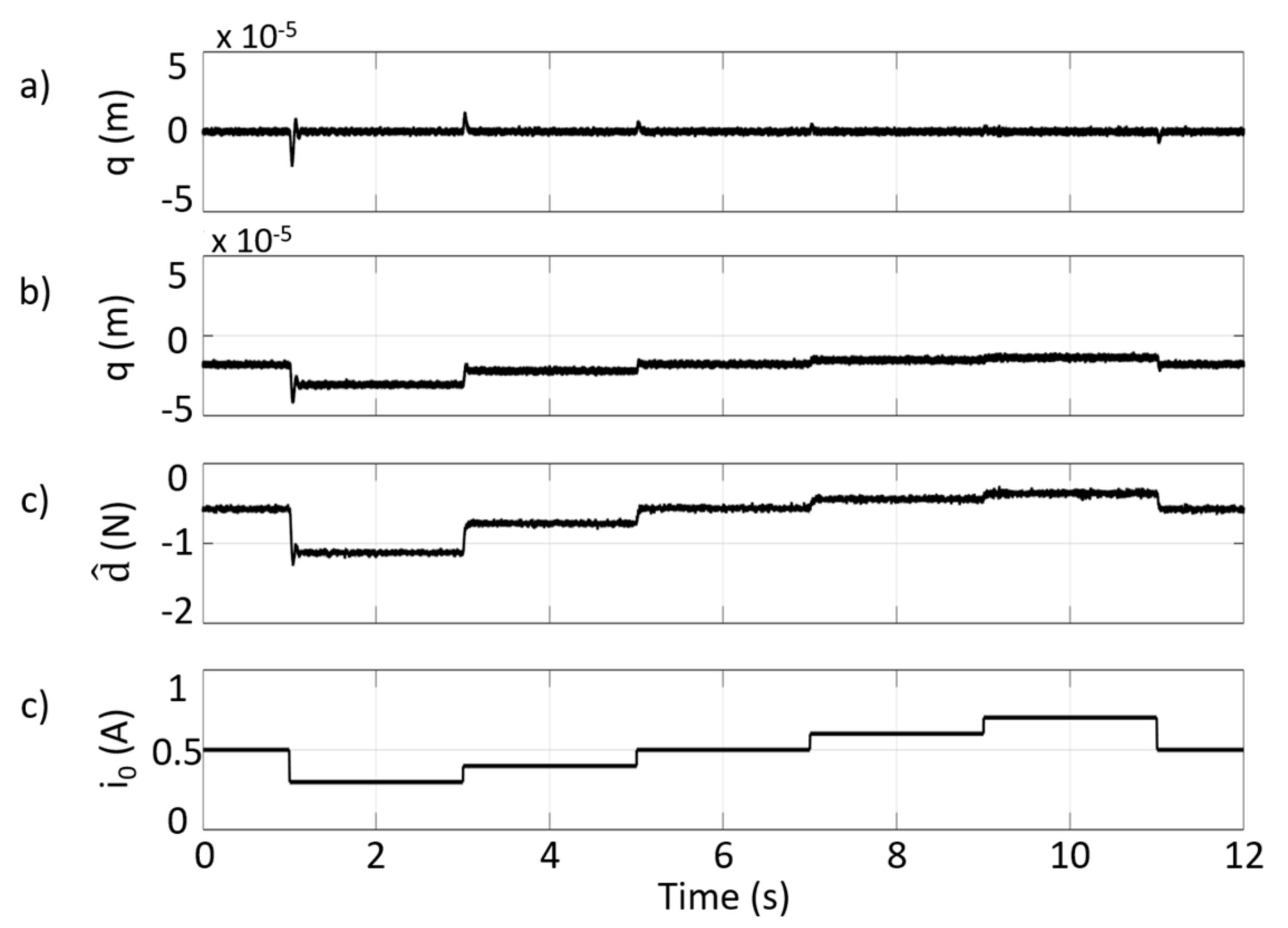
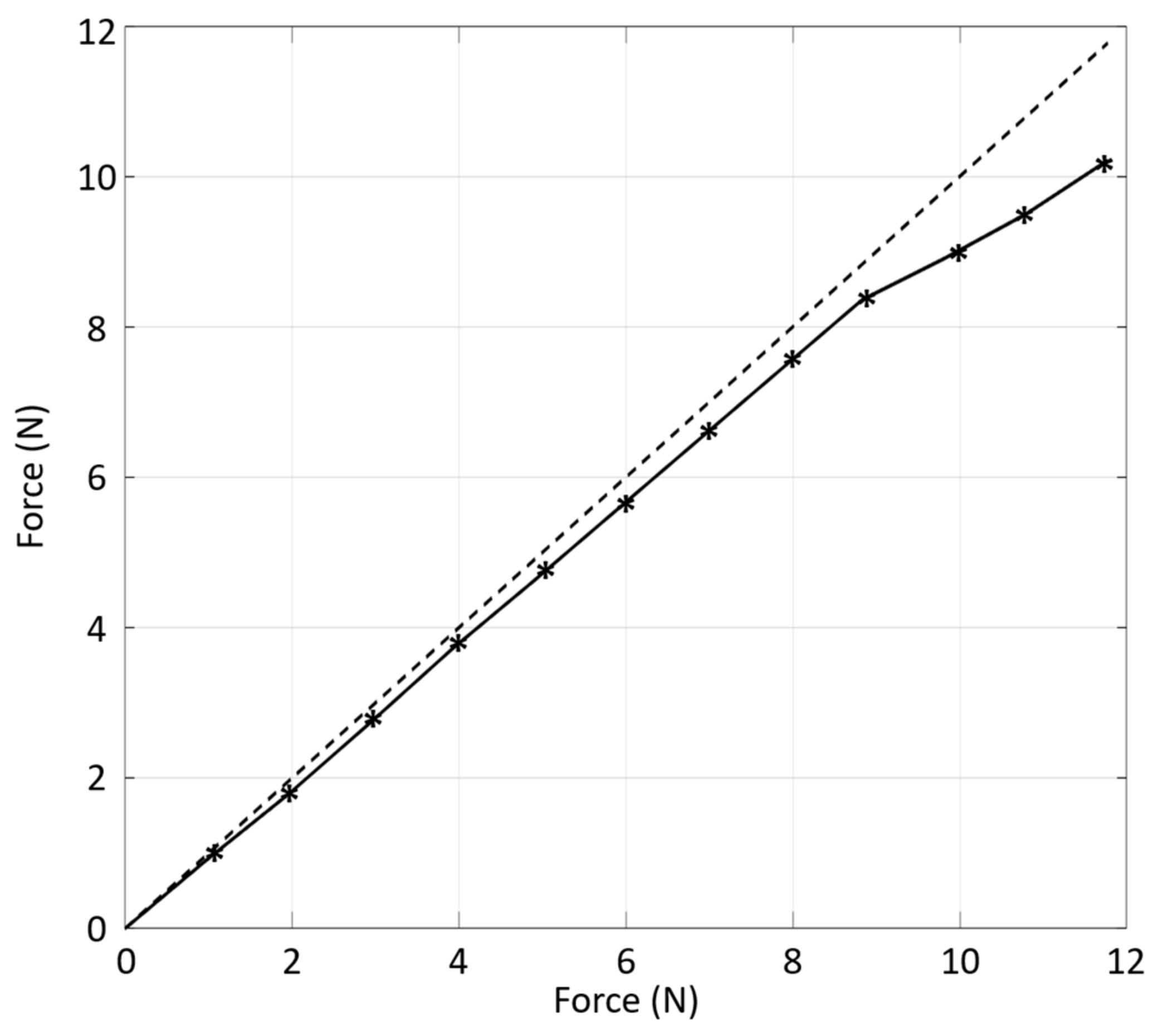
5. Experimental Results and Discussion
6. Conclusions
Author Contributions
Funding
Conflicts of Interest
References
- Bleuler, H.; Cole, M.; Keogh, P.; Larsonneur, R.; Maslen, E.; Nordmann, R.; Okada, Y.; Schweitz, G.; Traxler, A. Magnetic Bearings: Theory, Design, and Application to Rotating Machinery; Springer Science & Business Media: Berlin/Heidelberg, Germany, 2009. [Google Scholar]
- Chiba, A.; Fukao, T.; Ichikawa, O.; Oshima, M.; Takemoto, M.; Dorrell, D.G. Magnetic Bearings and Bearingless Drives; Elsevier: Amsterdam, The Netherlands, 2005. [Google Scholar]
- Song, X.; Throckmorton, A.L.; Untaroiu, A.; Patel, S.; Allaire, P.E.; Wood, H.G.; Olsen, D.B. Axial flow blood pumps. ASAIO J. 2003, 49, 355–364. [Google Scholar] [PubMed]
- Filatov, A.; Hawkins, L.; McMullen, P. Homopolar Permanent-Magnet-Biased Actuators and Their Application in Rotational Active Magnetic Bearing Systems. Actuators 2016, 5, 26. [Google Scholar] [CrossRef]
- Chen, S.-L.; Weng, C.-C. Robust control of a voltage-controlled three-pole active magnetic bearing system. IEEE/ASME Trans. Mechatron. 2010, 15, 381–388. [Google Scholar] [CrossRef]
- Darbandi, S.M.; Behzad, M.; Salarieh, H.; Mehdigholi, H. Linear output feedback control of a three-pole magnetic bearing. IEEE/ASME Trans. Mechatron. 2014, 19, 1323–1330. [Google Scholar]
- Noh, M.D.; Maslen, E.H. Self-sensing magnetic bearings using parameter estimation. IEEE Trans. Instrum. Meas. 1997, 46, 45–50. [Google Scholar] [CrossRef]
- Mizuno, T.; Bleuler, H. Self-sensing magnetic bearing control system design using the geometric approach. Control Eng. Pract. 1995, 3, 925–932. [Google Scholar] [CrossRef]
- Bonfitto, A.; Tonoli, A.; Silvagni, M. Sensorless active magnetic dampers for the control of rotors. Mechatronics 2017, 47, 195–207. [Google Scholar] [CrossRef]
- Lei, S.; Palazzolo, A. Control of flexible rotor systems with active magnetic bearings. J. Sound Vib. 2008, 314, 19–38. [Google Scholar] [CrossRef]
- Ren, Z.; Stephens, L.S. Closed-loop performance of a six degree-of-freedom precision magnetic actuator. IEEE/ASME Trans. Mechatron. 2005, 10, 666–674. [Google Scholar] [CrossRef]
- Grabner, H.; Silber, S.; Amrhein, W. Bearingless torque motor-modeling and control. In Proceedings of the 13th International Symposium on Magnetic Bearings (ISMB), Arlington, VA, USA, 6–9 August 2012. [Google Scholar]
- Silber, S.; Grabner, H.; Lohninger, R.; Amrhein, W. Design aspects of bearingless torque motors. In Proceedings of the 13th International Symposium on Magnetic Bearings (ISMB), Arlington, VA, USA, 6–9 August 2012. [Google Scholar]
- Maslen, E.; Montie, D. Sliding mode control of magnetic bearings: A hardware perspective. J. Eng. Gas Turbines Power 2001, 123, 878–885. [Google Scholar] [CrossRef]
- Sivrioglu, S.; Nonami, K. Sliding mode control with time-varying hyperplane for AMB systems. IEEE/ASME Trans. Mechatron. 1998, 3, 51–59. [Google Scholar] [CrossRef]
- Nonami, K.; He, W.; Nishimura, H. Robust control of magnetic levitation systems by means of H∞ control/μ-synthesis. JSME Int. J. Ser. C Dyn. Control Robot. Des. Manuf. 1994, 37, 513–520. [Google Scholar] [CrossRef]
- Hac, A.; Tomizuka, M. Application of learning control to active damping of forced vibration for periodically time variant systems. J. Vib. Acoust. 1990, 112, 489–496. [Google Scholar] [CrossRef]
- Betschon, F.; Knospe, C.R. Reducing magnetic bearing currents via gain scheduled adaptive control. IEEE/ASME Trans. Mechatron. 2001, 6, 437–443. [Google Scholar] [CrossRef]
- Ulbig, A.; Olaru, S.; Dumur, D.; Boucher, P. Explicit nonlinear predictive control for a magnetic levitation system. Asian J. Control 2010, 12, 434–442. [Google Scholar] [CrossRef]
- Fama, R.C.; Lopes, R.V.; Milhan, A.; Galvão, R.; Lastra, B. Predictive control of a magnetic levitation system with explicit treatment of operational constraints. In Proceedings of the 18th International Congress of Mechanical Engineering, Ouro Preto, Brazil, 6–11 November 2005. [Google Scholar]
- Cavalca, M.S.M.; Galvão, R.K.H.; Yoneyama, T. Robust model predictive control for a magnetic levitation system employing linear matrix inequalities. ABCM Symp. Ser. Mechatron. 2010, 4, 147–155. [Google Scholar]
- Bächle, T.; Hentzelt, S.; Graichen, K. Nonlinear model predictive control of a magnetic levitation system. Control Eng. Pract. 2013, 21, 1250–1258. [Google Scholar] [CrossRef]
- Klaučo, M.; Kalúz, M.; Kvasnica, M. Real-time implementation of an explicit MPC-based reference governor for control of a magnetic levitation system. Control Eng. Pract. 2017, 60, 99–105. [Google Scholar] [CrossRef]
- Tsao, J.-G.; Sheu, L.-T.; Yang, L.-F. Adaptive synchronization control of the magnetically suspended rotor system. Dyn. Control 2000, 10, 239–253. [Google Scholar] [CrossRef]
- Zhang, C.; Tseng, K.J.; Xiao, Y.; Zhu, K.Y. Model-based predictive control for a compact and efficient flywheel energy storage system with magnetically assisted bearings. In Proceedings of the 2004 IEEE 35th Annual Power Electronics Specialists Conference (IEEE Cat. No.04CH37551), Aachen, Germany, 20–25 June 2004; Volume 5, pp. 3573–3579. [Google Scholar]
- Chowdhury, A.; Sarjaš, A. Finite Element Modelling of a Field-Sensed Magnetic Suspended System for Accurate Proximity Measurement Based on a Sensor Fusion Algorithm with Unscented Kalman Filter. Sensors 2016, 16, 1504. [Google Scholar] [CrossRef] [PubMed]
- Castellanos, L.M.; Bonfitto, A.; Tonoli, A.; Amati, N. Identification of Force-Displacement and Force-Current Factors in an Active Magnetic Bearing System. In Proceedings of the 18th Annual IEEE International Conference on Electro Information Technology, Rochester, MI, USA, 3–5 May 2018. [Google Scholar]
- Afonso, R.J.M.; Galvão, R.K.H. Predictive control of a magnetic levitation system with infeasibility handling by relaxation of output constraints. ABCM Symp. Ser. Mechatron. 2007, 3, 11–18. [Google Scholar]
- Pannocchia, G.; Rawlings, J.B. Disturbance models for offset-free model-predictive control. AIChE J. 2003, 49, 426–437. [Google Scholar] [CrossRef]
- Rawlings, J.B. Tutorial overview of model predictive control. IEEE Control Syst. 2000, 20, 38–52. [Google Scholar]
- Wang, X.; Ding, B.; Yang, X.; Ye, Z. Design and Application of Offset-Free Model Predictive Control Disturbance Observation Method. J. Control Sci. Eng. 2016, 2016, 7279430. [Google Scholar] [CrossRef]
- Maeder, U.; Borrelli, F.; Morari, M. Linear offset-free model predictive control. Automatica 2009, 45, 2214–2222. [Google Scholar] [CrossRef]
- Pannocchia, G. Offset-free tracking MPC: A tutorial review and comparison of different formulations. In Proceedings of the 2015 European Control Conference (ECC), Linz, Austria, 15–17 July 2015; pp. 527–532. [Google Scholar]
- Borrelli, F.; Morari, M. Offset free model predictive control. In Proceedings of the 2007 46th IEEE Conference on Decision and Control, New Orleans, LA, USA, 12–14 December 2007; pp. 1245–1250. [Google Scholar]
- Muske, K.R.; Badgwell, T.A. Disturbance modeling for offset-free linear model predictive control. J. Process Control 2002, 12, 617–632. [Google Scholar] [CrossRef]
- Morari, M.; Maeder, U. Nonlinear offset-free model predictive control. Automatica 2012, 48, 2059–2067. [Google Scholar] [CrossRef]
- Kim, S.-K.; Choi, D.-K.; Lee, K.-B.; Lee, Y.I. Offset-free model predictive control for the power control of three-phase AC/DC converters. IEEE Trans. Ind. Electron. 2015, 62, 7114–7126. [Google Scholar] [CrossRef]
- Bender, F.A.; Göltz, S.; Bräunl, T.; Sawodny, O. Modeling and Offset-Free Model Predictive Control of a Hydraulic Mini Excavator. IEEE Trans. Autom. Sci. Eng. 2017, 14, 1682–1694. [Google Scholar] [CrossRef]
- Askaria, M.; Moghavvemi, M.; Almurib, H.A.F.; Muttaqi, K.M. An offset-free multivariable model predictive control for quadruple tanks system. In Proceedings of the 2014 IEEE Industry Applications Society Annual Meeting, Vancouver, BC, Canada, 5–9 October 2014; pp. 1–8. [Google Scholar]
- Pannocchia, G.; Laachi, N.; Rawlings, J.B. A candidate to replace PID control: SISO-constrained LQ control. AIChE J. 2005, 51, 1178–1189. [Google Scholar] [CrossRef]
- Skogestad, S.; Postlewhite, I. Multivariable Feedback Control: Analysis and Design; Wiley: New York, NY, USA, 2001. [Google Scholar]
- Zometa, P.; Kögel, M.; Findeisen, R. μAO-MPC: A free code generation tool for embedded real-time linear model predictive control. In Proceedings of the 2013 American Control Conference (ACC), Washington, DC, USA, 17–19 June 2013; pp. 5320–5325. [Google Scholar]
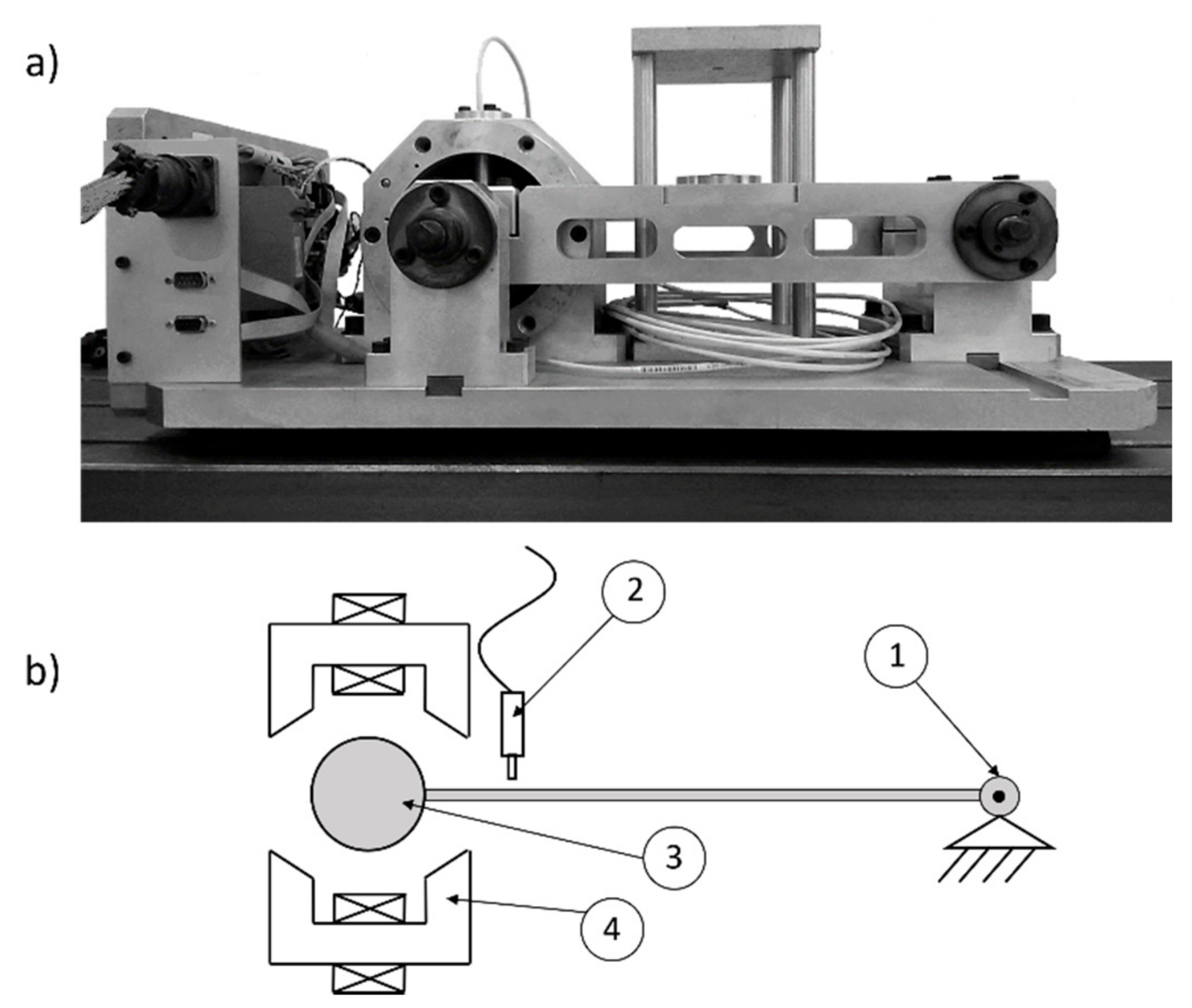
| Symbol | Name | Value | Unit |
|---|---|---|---|
| Mass | |||
| Cross-section area at the air gap | |||
| Nominal airgap | |||
| Number of turns | - | ||
| Coil resistance | |||
| Coil nominal inductance | |||
| Current-force factor | |||
| Electromagnet negative stiffness | |||
| Back-electromotive-force factor |
| Parameter | Value |
|---|---|
| Parameter | Value |
|---|---|
| 12 | |
| 1 | |
© 2018 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Bonfitto, A.; Castellanos Molina, L.M.; Tonoli, A.; Amati, N. Offset-Free Model Predictive Control for Active Magnetic Bearing Systems. Actuators 2018, 7, 46. https://doi.org/10.3390/act7030046
Bonfitto A, Castellanos Molina LM, Tonoli A, Amati N. Offset-Free Model Predictive Control for Active Magnetic Bearing Systems. Actuators. 2018; 7(3):46. https://doi.org/10.3390/act7030046
Chicago/Turabian StyleBonfitto, Angelo, Luis Miguel Castellanos Molina, Andrea Tonoli, and Nicola Amati. 2018. "Offset-Free Model Predictive Control for Active Magnetic Bearing Systems" Actuators 7, no. 3: 46. https://doi.org/10.3390/act7030046
APA StyleBonfitto, A., Castellanos Molina, L. M., Tonoli, A., & Amati, N. (2018). Offset-Free Model Predictive Control for Active Magnetic Bearing Systems. Actuators, 7(3), 46. https://doi.org/10.3390/act7030046








