Design Optimization of a Miniaturized Pneumatic Artificial Muscle and Experimental Validation
Abstract
1. Introduction
2. Mathematical Modeling
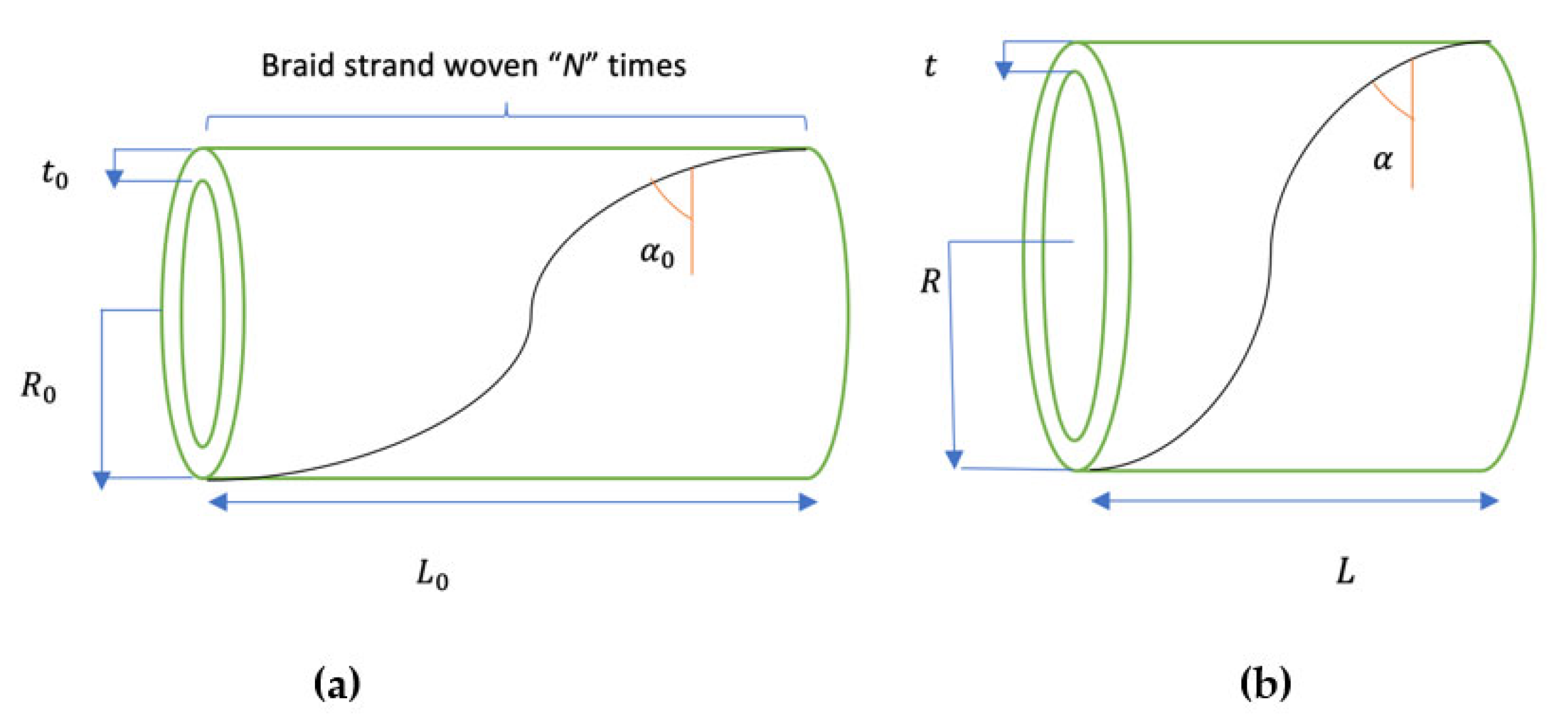
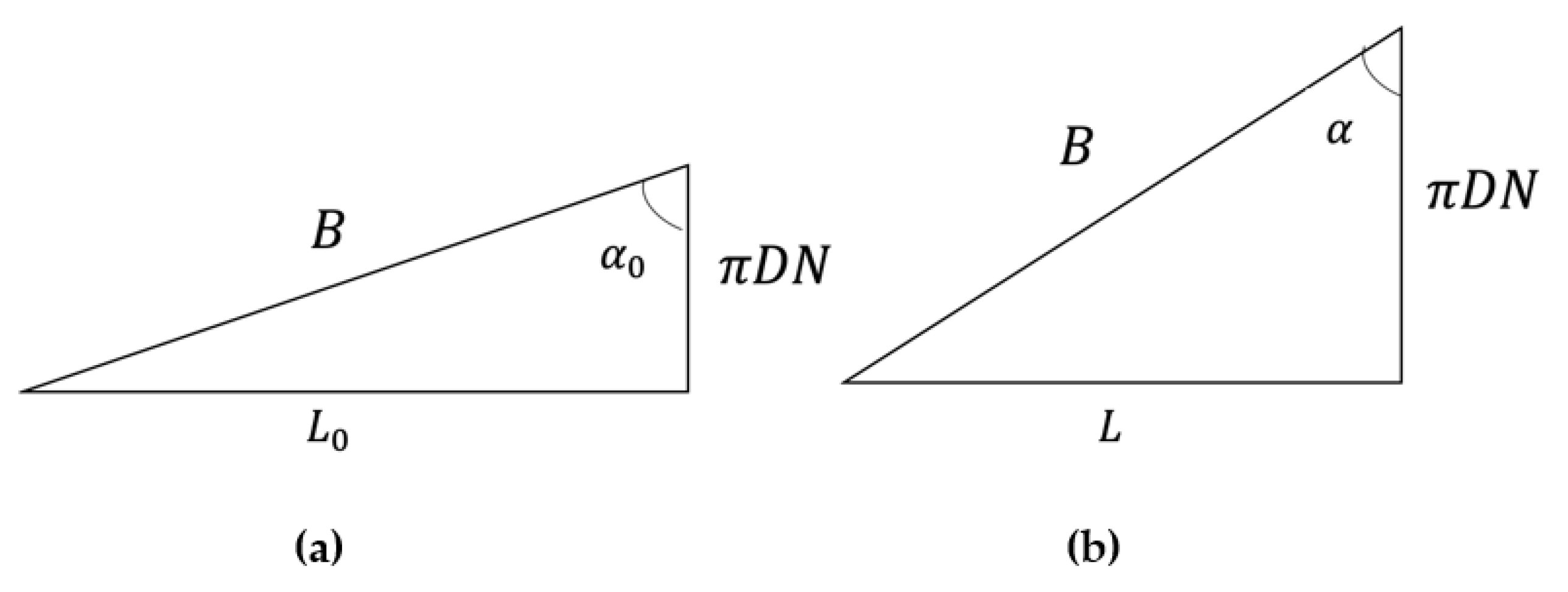
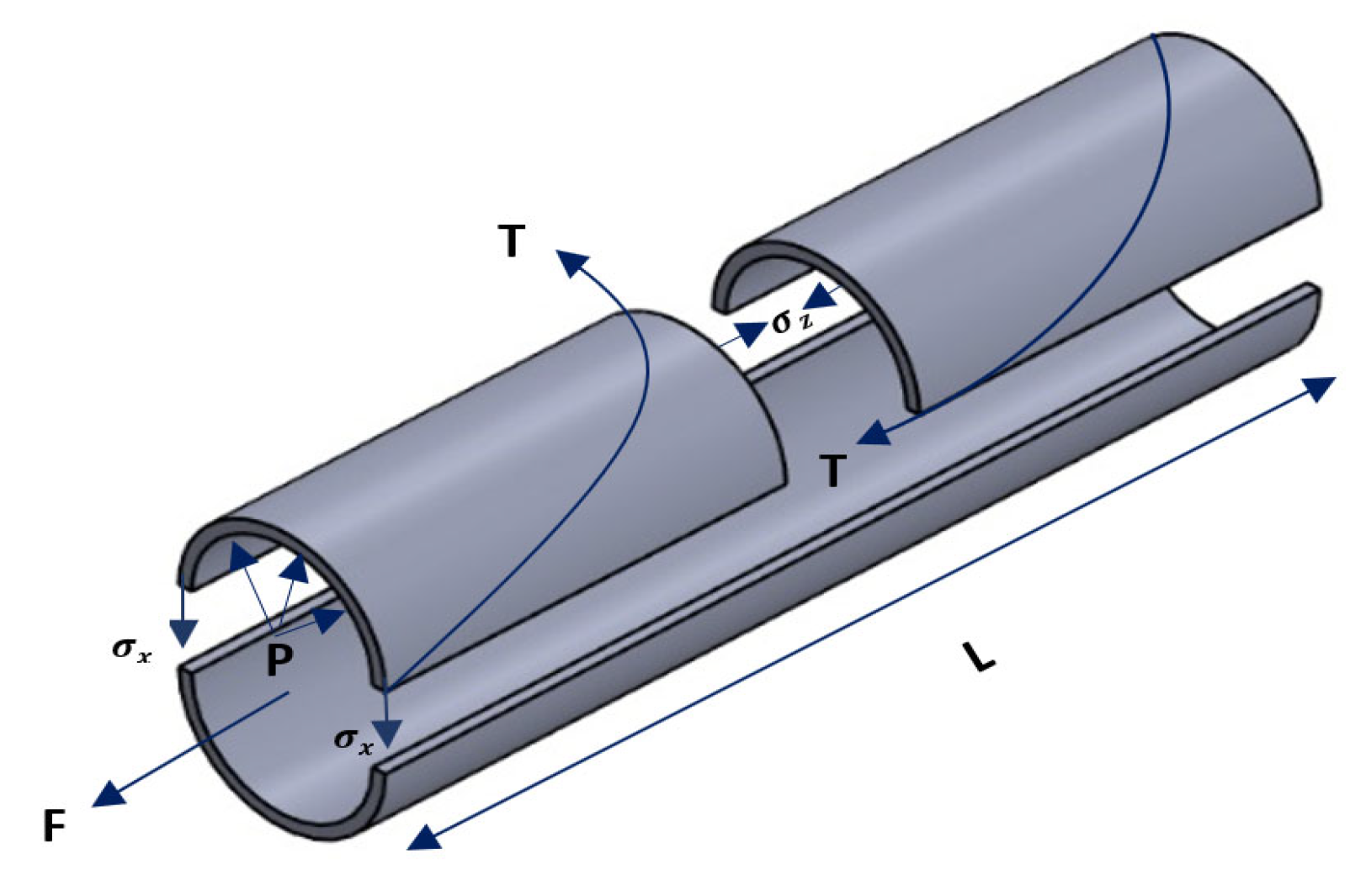
2.1. Mathematical Formulation for the Output Force
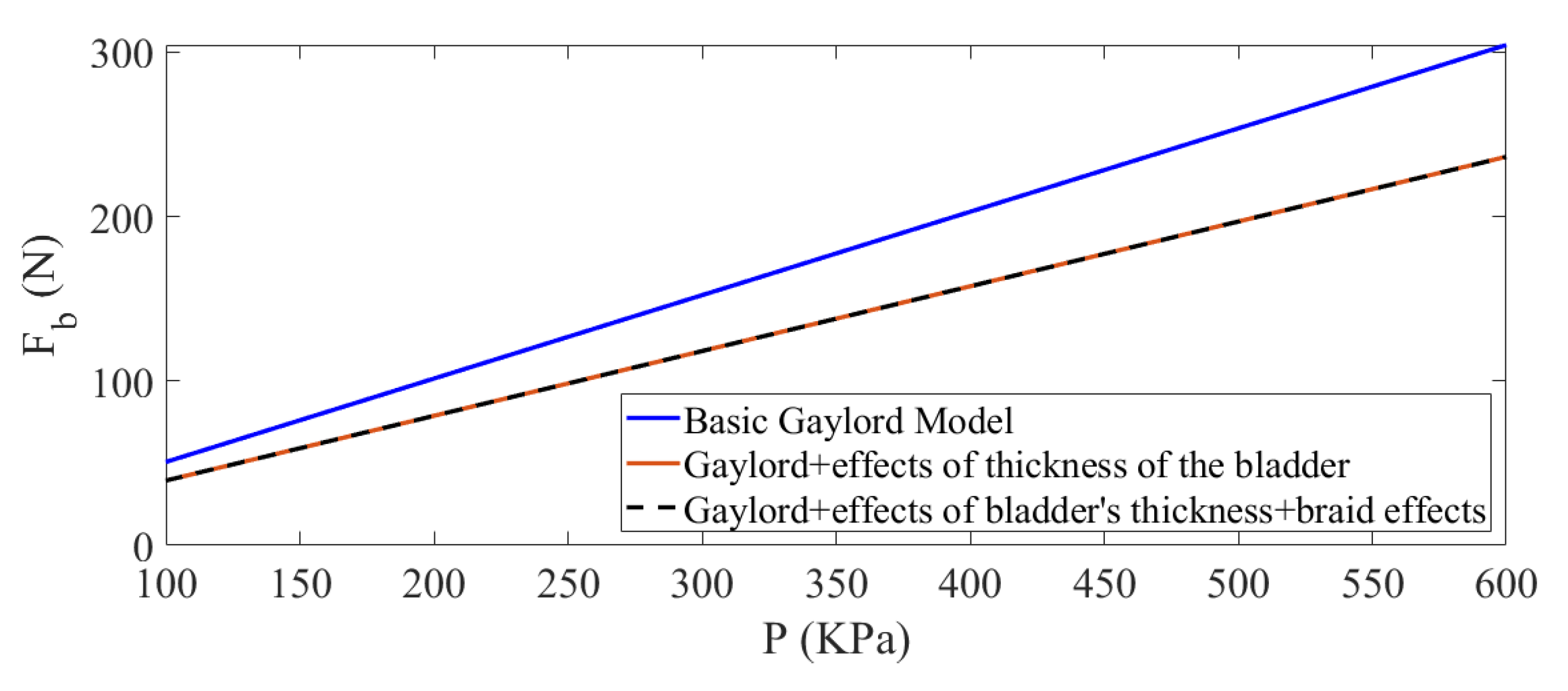
2.2. Refined Blocked Force Equation
3. Optimization Formulation
3.1. Optimization Formulation: Case I
3.2. Optimization Formulation: Case II
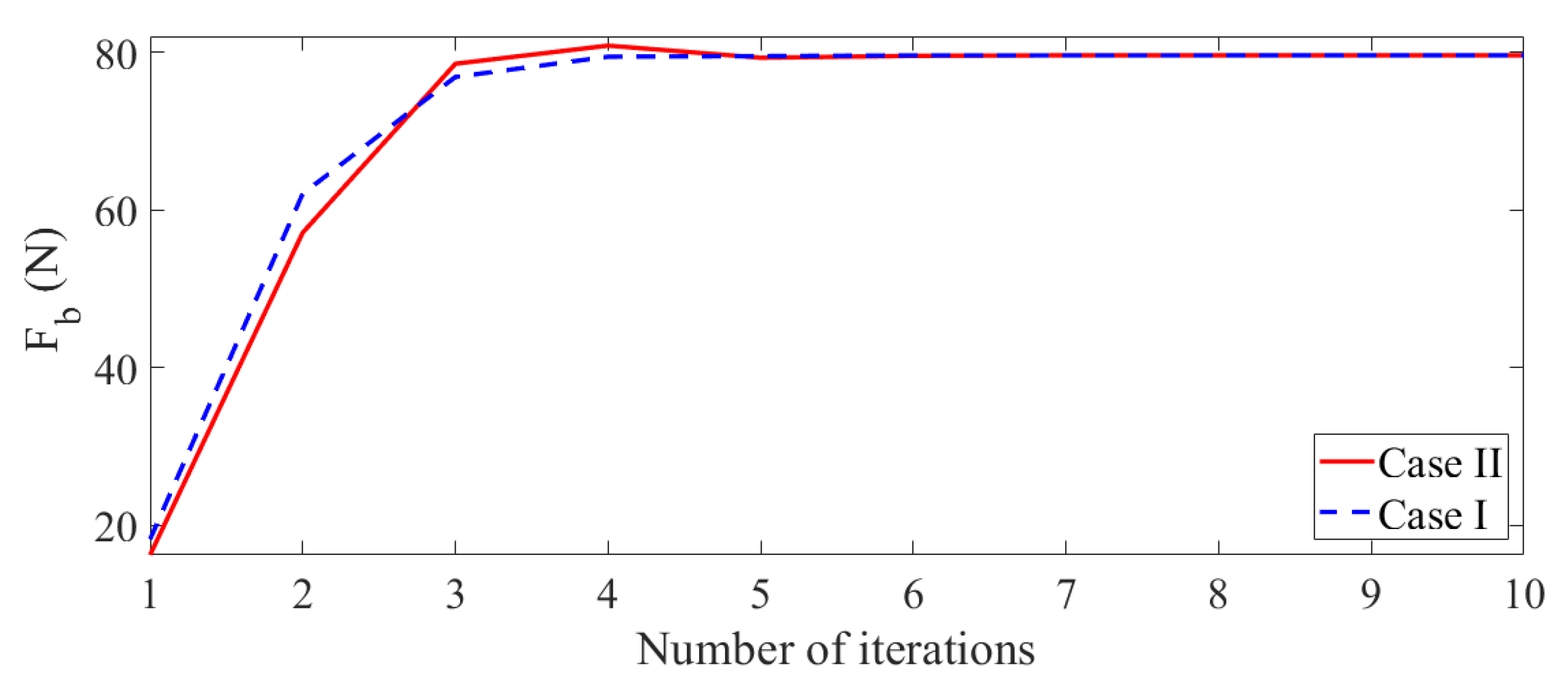
4. Optimization Results
5. Experimental Verification
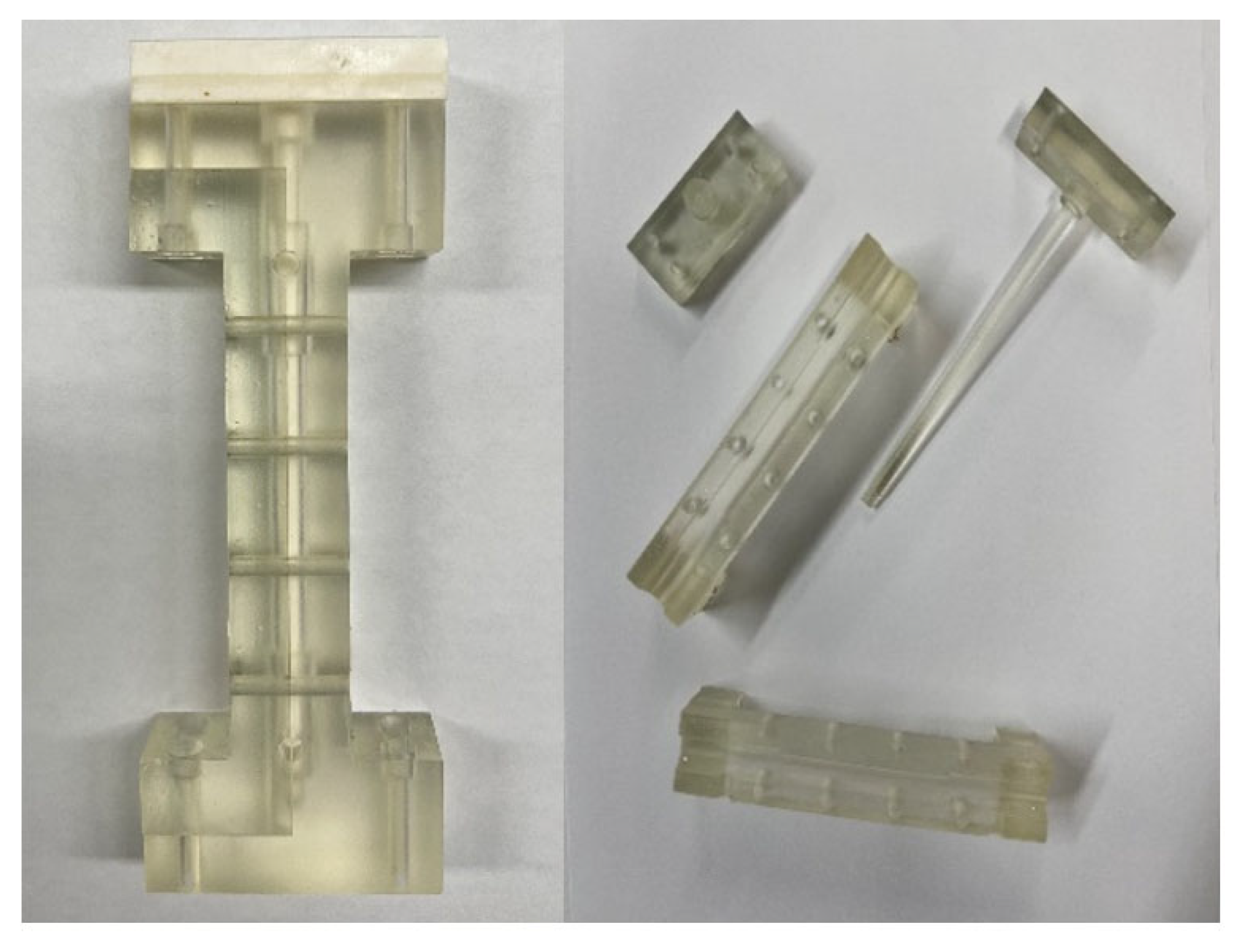
5.1. Fabrication of the Optimized MPAM
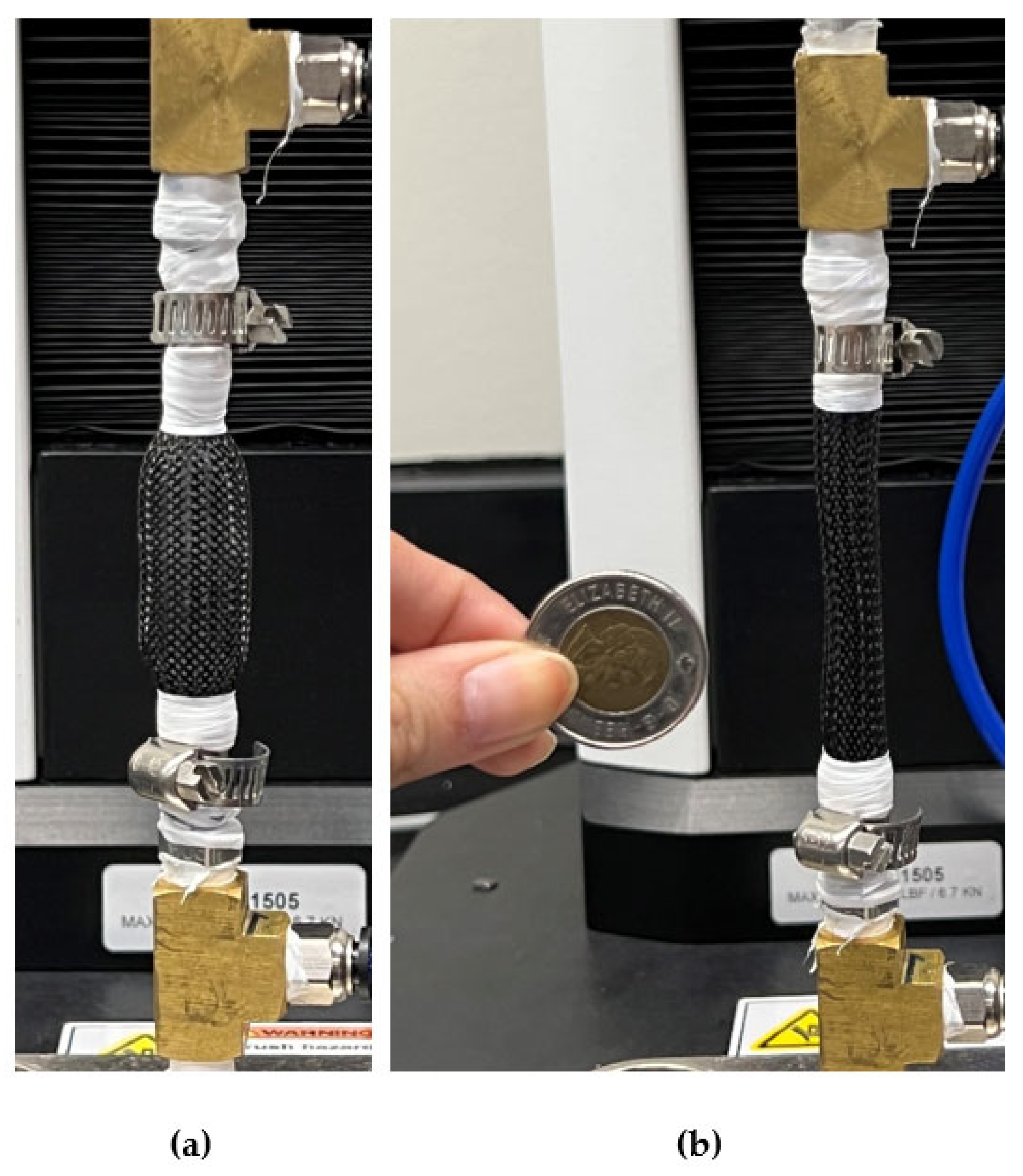
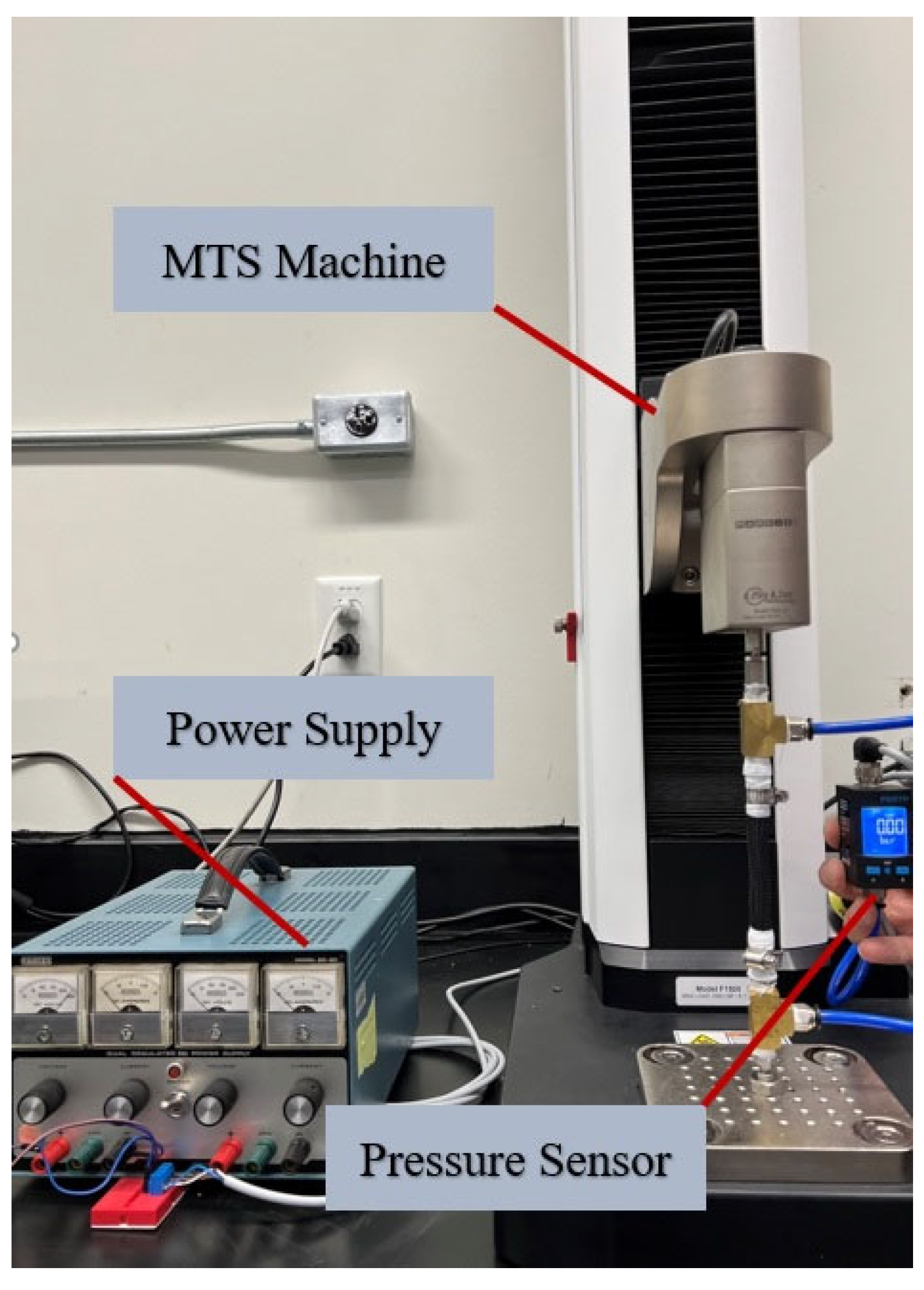
5.2. Test Setup and Procedure
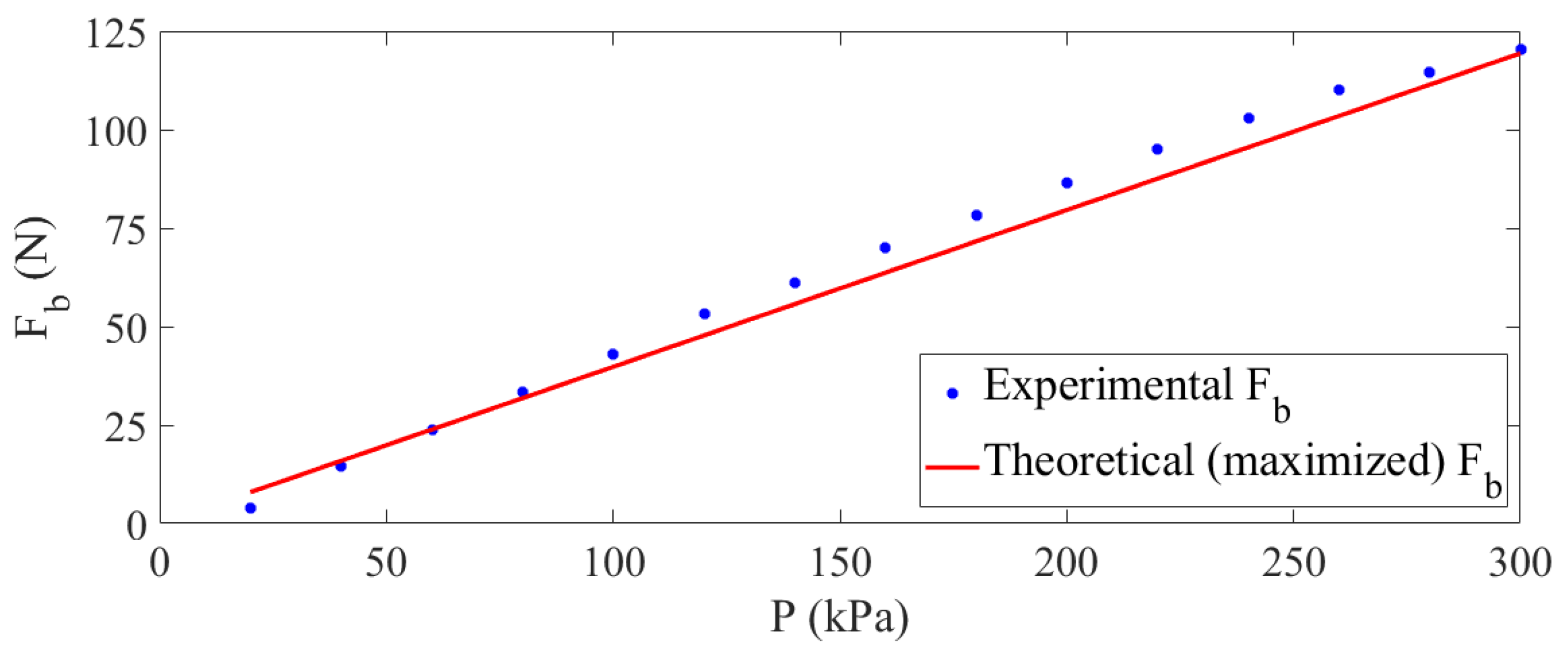
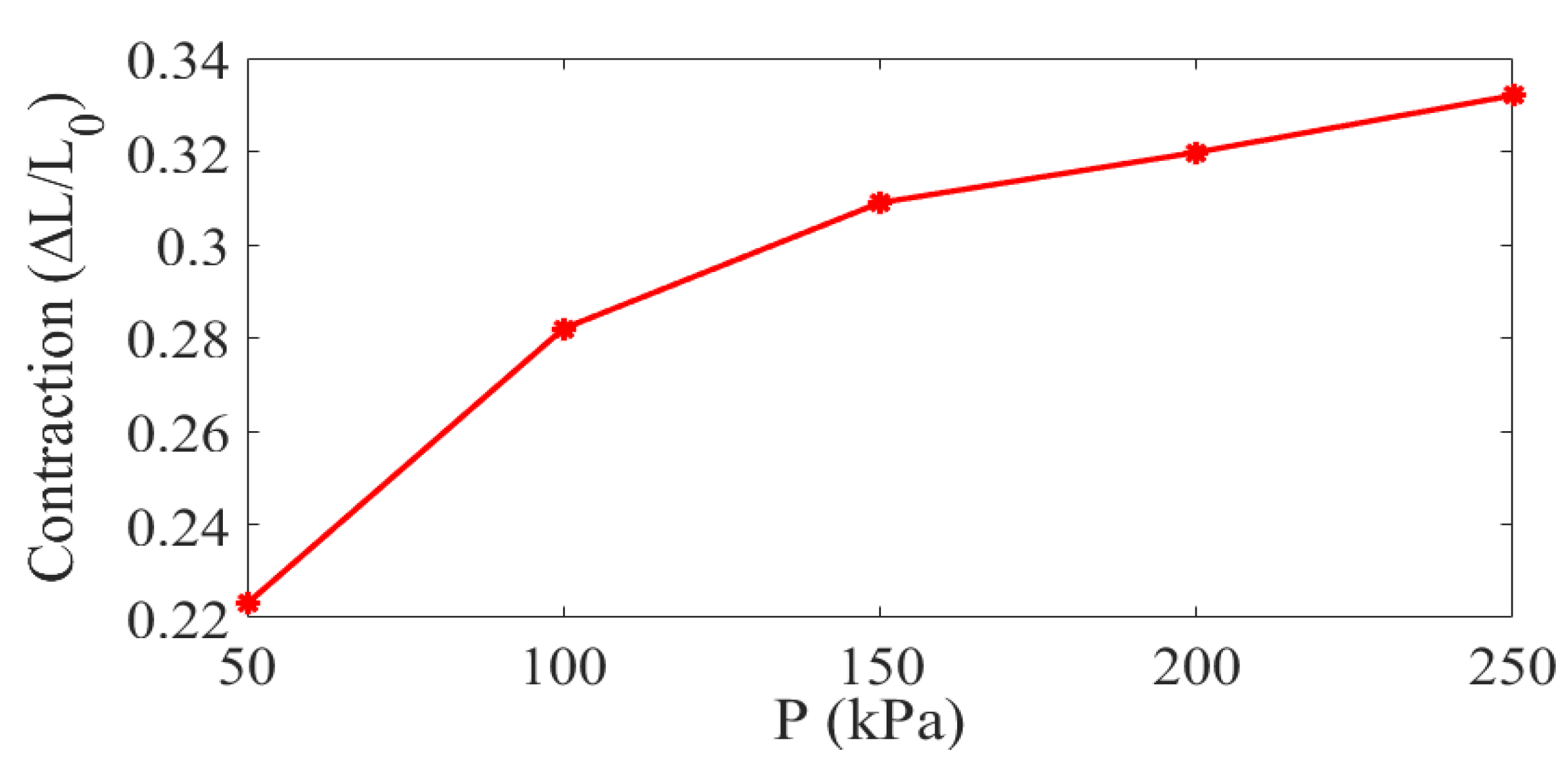
5.3. Experimental Results
6. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Schröder, J.; Erol, D.; Kawamura, K.; Dillman, R. Dynamic pneumatic actuator model for a model-based torque controller. In Proceedings of the IEEE International Symposium on Computational Intelligence in Robotics and Automation, CIRA, Kobe, Japan, 16–20 July 2003; Volume 1, pp. 342–347. [Google Scholar] [CrossRef]
- Schröder, J.; Kawamura, K.; Gockel, T.; Dillmann, R. Improved Control of a Humanoid Arm Driven by Pneumatic Actuators. In Proceedings of Humanoids. 2003. Available online: https://www.techfak.uni-bielefeld.de/~rhaschke/lehre/WS04/humanoids/papers/McKibben.pdf (accessed on 21 May 2023).
- Wang, Y.; Xu, Q. Design and testing of a soft parallel robot based on pneumatic artificial muscles for wrist rehabilitation. Sci. Rep. 2021, 11, 1273. [Google Scholar] [CrossRef] [PubMed]
- Zhong, J.U.N.; Zhao, D.; He, C.; Zhu, Y.U.E.; Zhang, Q. A Rehabilitation Robot Driven by Pneumatic Artificial Muscles. J. Mech. Med. Biol. 2020, 20, 20400084. [Google Scholar] [CrossRef]
- Magnetti Gisolo, S.; Muscolo, G.G.; Paterna, M.; De Benedictis, C.; Ferraresi, C. Feasibility study of a passive pneumatic exoskeleton for upper limbs based on a mckibben artificial muscle. In Mechanisms and Machine Science; Springer Science and Business Media B.V.: Berlin/Heidelberg, Germany, 2021; pp. 208–217. [Google Scholar] [CrossRef]
- Kalita, B.; Dwivedy, S.K. Nonlinear dynamic response of pneumatic artificial muscle: A theoretical and experimental study. Int. J. Non-Linear Mech. 2020, 125, 103544. [Google Scholar] [CrossRef]
- Choi, H.S.; Lee, C.H.; Baek, Y.S. Design and Validation of a Two-Degree-of-Freedom Powered Ankle-Foot Orthosis with Two Pneumatic Artificial Muscles. Mechatronics 2020, 72, 102469. [Google Scholar] [CrossRef]
- Lee, Y.K.; Shimoyama, I. A skeletal framework artificial hand actuated by pneumatic artificial muscles. In Proceedings of the 1999 IEEE International Conference on Robotics and Automation (Cat. No.99CH36288C), Detroit, MI, USA, 10–15 May 1999; Volume 2, pp. 926–931. [Google Scholar] [CrossRef]
- Robinson, R.M.; Kothera, C.S.; Woods, B.K.S.; Vocke, R.D.; Wereley, N.M. High specific power actuators for robotic manipulators. J. Intell. Mater. Syst. Struct. 2011, 22, 1501–1511. [Google Scholar] [CrossRef]
- Tondu, B.; Ippolito, S.; Guiochet, J.; Daidie, A. A Seven-degrees-of-freedom robot-arm driven by pneumatic artificial muscles for humanoid robots. Int. J. Robot. Res. 2005, 24, 257–274. [Google Scholar] [CrossRef]
- Kalita, B.; Leonessa, A.; Dwivedy, S.K. A Review on the Development of Pneumatic Artificial Muscle Actuators: Force Model and Application. Actuators 2022, 11, 288. [Google Scholar] [CrossRef]
- McKibben, J.L. Artificial Muscle. 1957. Available online: https://cyberneticzoo.com/ (accessed on 22 December 2022).
- Takagi, T.; Sakaguchi, Y. Rubbertuator, Bridgestone Company. 1983. Available online: https://cyberneticzoo.com/ (accessed on 7 February 2022).
- Mižáková, J.; Pitel, J.; Tóthová, M. Pneumatic artificial muscle as actuator in mechatronic system. Appl. Mech. Mater. 2013, 460, 81–90. [Google Scholar] [CrossRef]
- Park, Y.L.; Wood, R.J. Smart pneumatic artificial muscle actuator with embedded microfluidic sensing. In Proceedings of the IEEE Sensors, Baltimore, MD, USA, 3–6 November 2013. [Google Scholar] [CrossRef]
- Wakimoto, S.; Misumi, J.; Suzumori, K. New concept and fundamental experiments of a smart pneumatic artificial muscle with a conductive fiber. Sens. Actuators A Phys. 2016, 250, 48–54. [Google Scholar] [CrossRef]
- Kanno, R.; Watanabe, S.; Shimizu, K.; Shintake, J. Self-Sensing McKibben Artificial Muscles Embedded with Dielectric Elastomer Sensor. IEEE Robot. Autom. Lett. 2021, 6, 6274–6280. [Google Scholar] [CrossRef]
- Fu, C.; Wang, K.; Tang, W.; Nilghaz, A.; Hurren, C.; Wang, X.; Xu, W.; Su, B.; Xia, Z. Multi-sensorized pneumatic artificial muscle yarns. Chem. Eng. J. 2022, 446, 137241. [Google Scholar] [CrossRef]
- do Rosario Carvalho, A.D.; Karanth, P.N.; Desai, V. Design and characterization of a pneumatic muscle actuator with novel end-fittings for medical assistive applications. Sens. Actuators A Phys. 2021, 331, 112877. [Google Scholar] [CrossRef]
- Tjahyono, A.P.; Aw, K.C.; Devaraj, H.; Surendra, W.; Haemmerle, E.; Travas-Sejdic, J. A five-fingered hand exoskeleton driven by pneumatic artificial muscles with novel polypyrrole sensors. Ind. Robot. Int. J. Robot. Res. Appl. 2013, 40, 251–260. [Google Scholar] [CrossRef]
- Chakravarthy, S.; Aditya, K.; Ghosal, A. Experimental characterization and control of miniaturized pneumatic artificial muscle. J. Med. Devices 2014, 8, 041011. [Google Scholar] [CrossRef]
- Lathrop, B.; Ourak, M.; Poorten, E.V. Miniature Pneumatic Artificial Muscles for Use in Surgical Devices, ACTUATOR. In Proceedings of the International Conference and Exhibition on New Actuator Systems and Applications, Mannheim, Germany, 29–30 June 2022. [Google Scholar]
- Markus, A.T.; Sobczyk, M.R.; Perondi, E.A. Modeling, Control, and Simulation of a 3-Degree of Freedom Mechanism Actuated by Pneumatic Artificial Muscles for Upper Limb Prosthesis Application. J. Mech. Robot. 2023, 15, 011002. [Google Scholar] [CrossRef]
- Ashwin, K.P.; Ghosal, A. Static Modeling of Miniaturized Pneumatic Artificial Muscles, Kinematic Analysis, and Experiments on an Endoscopic End-Effector. IEEE/ASME Trans. Mechatron. 2019, 24, 1429–1439. [Google Scholar] [CrossRef]
- Tadano, K.; Akai, M.; Kadota, K.; Kawashima, K. Development of grip amplified glove using bi-articular mechanism with pneumatic artificial rubber muscle. In Proceedings of the IEEE International Conference on Robotics and Automation, Anchorage, AK, USA, 3–7 May 2010; pp. 2363–2368. [Google Scholar] [CrossRef]
- Vashisth, A.; Zhu, B.; Wimmer, B.M.; Bakis, C.E.; Rahn, C.D. Evaluation of Millimeter-Size Fluidic Flexible Matrix Composite Tubes. 2013. Available online: http://asmedigitalcollection.asme.org/SMASIS/proceedings-pdf/SMASIS2013/56048/V002T06A029/4459446/v002t06a029-smasis2013-3344.pdf (accessed on 15 March 2022).
- Doumit, M.; Leclair, J. Development and testing of stiffness model for pneumatic artificial muscle. Int. J. Mech. Sci. 2017, 120, 30–41. [Google Scholar] [CrossRef]
- Salahuddin, B.; Warren, H.; Spinks, G.M. A comprehensive test method for measuring actuation performance of McKibben artificial muscles. Smart Mater. Struct. 2021, 30, 045016. [Google Scholar] [CrossRef]
- Pillsbury, T.E.; Kothera, C.S.; Wereley, N.M. Effect of bladder wall thickness on miniature pneumatic artificial muscle performance. Bioinspir. Biomim. 2015, 10, 055006. [Google Scholar] [CrossRef]
- Kothera, C.S.; Jangid, M.; Sirohi, J.; Wereley, N.M. Experimental characterization and static modeling of McKibben actuators. J. Mech. Des. 2009, 131, 091010. [Google Scholar] [CrossRef]
- Gentry, M.F.; Wereley, N.M. Effects of Braid Angle on Pneumatic Artificial Muscle Actuator Performance. 2008. Available online: https://proceedings.asmedigitalcollection.asme.org (accessed on 10 October 2021).
- Joe, S.; Totaro, M.; Wang, H.; Beccai, L. Development of the ultralight hybrid pneumatic artificial muscle: Modelling and optimization. PLoS ONE 2021, 16, e0250325. [Google Scholar] [CrossRef] [PubMed]
- Sangian, D.; Naficy, S.; Spinks, G.M.; Tondu, B. The effect of geometry and material properties on the performance of a small hydraulic McKibben muscle system. Sens. Actuators A Phys. 2015, 234, 150–157. [Google Scholar] [CrossRef]
- Solano, B.; Rotinat-Libersa, C. Compact and lightweight hydraulic actuation system for high performance millimeter scale robotic applications: Modeling and experiments. J. Intell. Mater. Syst. Struct. 2011, 22, 1479–1487. [Google Scholar] [CrossRef]
- Yang, H.D.; Greczek, B.T.; Asbeck, A.T. Modeling and analysis of a high-displacement pneumatic artificial muscle with integrated sensing. Front. Robot. AI 2019, 5, 136. [Google Scholar] [CrossRef] [PubMed]
- Tomori, H.; Sato, Y.; Ando, S. Cyclic Failure Testing of Straight-Fiber Pneumatic Artificial Muscles for Optimizing Durability. In Proceedings of the IECON 2019—45th Annual Conference of the IEEE Industrial Electronics Society, Convention Center, Lisbon, Portugal, 14–17 October; 2019. [Google Scholar]
- Xiao, W.; Hu, D.; Chen, W.; Yang, G.; Han, X. Design, Characterization and Optimization of Multi-directional Bending Pneumatic Artificial Muscles. J. Bionic Eng. 2021, 18, 1358–1368. [Google Scholar] [CrossRef]
- Diteesawat, R.S.; Helps, T.; Taghavi, M.; Rossiter, J. Characteristic Analysis and Design Optimization of Bubble Artificial Muscles. Soft Robot. 2021, 8, 186–199. [Google Scholar] [CrossRef]
- Lathrop, R.; Ourak, M.; Deprest, J.; Poorten, E.V. Concentric Dual-Chamber Pneumatic Artificial Muscles: Miniature Actuators Designed for Use in Minimally Invasive Surgical Instruments. J. Med. Robot. Res. 2022, 7, 22410070. [Google Scholar] [CrossRef]
- Kwon, J.; Yoon, S.J.; Park, Y.L. Flat Inflatable Artificial Muscles with Large Stroke and Adjustable Force–Length Relations. IEEE Trans. Robot. 2020, 36, 743–756. [Google Scholar] [CrossRef]
- Kim, W.; Park, H.; Kim, J. Compact Flat Fabric Pneumatic Artificial Muscle (ffPAM) for Soft Wearable Robotic Devices. IEEE Robot. Autom. Lett. 2021, 6, 2603–2610. [Google Scholar] [CrossRef]
- Faudzi, A.A.M.; Endo, G.; Kurumaya, S.; Suzumori, K. Long-Legged Hexapod Giacometti Robot Using Thin Soft McKibben Actuator. IEEE Robot. Autom. Lett. 2018, 3, 100–107. [Google Scholar] [CrossRef]
- Kurumaya, S.; Suzumori, K.; Nabae, H.; Wakimoto, S. Musculoskeletal lower-limb robot driven by multifilament muscles. ROBOMECH J. 2016, 3, 18. [Google Scholar] [CrossRef]
- Tondu, B. Modelling of the McKibben artificial muscle: A review. J. Intell. Mater. Syst. Struct. 2012, 23, 225–253. [Google Scholar] [CrossRef]
- Ferraresi, C.; Franco, W.; Bertetto, A.M. Flexible Pneumatic Actuators: A Comparison between The McKibben and the Straight Fibres Muscles. J. Robot. Mechatron. 2001, 13, 56–63. [Google Scholar] [CrossRef]
- Chou, C.-P.; Hannaford, B. Measurement and Modeling of McKibben Pneumatic Artificial Muscles; University of Washington, Department of Electrical Engineering: Seattle, WA, USA, 1996. [Google Scholar]
- Schulte, H.F., Jr.; Adamski, D.F.; Pearson, J.R. Characteristics of the Braided Fluid Actuator; Technical Report No. 5; The University of Michigan, Department of Physical Medicine and Rehabilitation Orthetics Research Project: Ann Arbor, MI, USA, 1961. [Google Scholar]
- Ping Chou, C.; Hannaford, B. Static and dynamic characteristics of McKibben pneumatic artificial muscles. In Proceedings of the 1994 IEEE International Conference on Robotics and Automation, San Diego, CA, USA, 8–13 May 1994; Volume 1, pp. 281–286. [Google Scholar] [CrossRef]
- Davis, S.; Caldwell, D.G. Braid effects on contractile range and friction modeling in pneumatic muscle actuators. Int. J. Robot. Res. 2006, 25, 359–369. [Google Scholar] [CrossRef]
- Tondu, B. Modeling and Control of McKibben Artificial Muscle Robot Actuators. IEEE Control Syst. 2000, 20, 15–38. [Google Scholar] [CrossRef]
- Woods, B.K.S.; Kothera, C.S.; Wereley, N.M. Wind tunnel testing of a helicopter rotor trailing edge flap actuated via Pneumatic Artificial Muscles. J. Intell. Mater. Syst. Struct. 2011, 22, 1513–1528. [Google Scholar] [CrossRef]
- Gaylord, R.H. Fluid Actuated Motor System and Stroking Devices. U.S. Patent 2,844,126, 22 July 1958. [Google Scholar]
- Ball, E.; Garcia, E. Effects of bladder geometry in pneumatic artificial muscles. J. Med. Devices 2016, 10, 041001. [Google Scholar] [CrossRef]
- Tu, Q.; Wang, Y.; Yue, D.; Dwomoh, F.A. Analysis on the Impact Factors for the Pulling Force of the McKibben Pneumatic Artificial Muscle by a FEM Model. J. Robot. 2020, 2020, 4681796. [Google Scholar] [CrossRef]
- Hocking, E.G.; Wereley, N.M. Analysis of nonlinear elastic behavior in miniature pneumatic artificial muscles. Smart Mater. Struct. 2012, 22, 014016. [Google Scholar] [CrossRef]
- Wang, G.N.; Wereley, M.; Pillsbury, T. Non-linear quasi-static model of pneumatic artificial muscle actuators. J. Intell. Mater. Syst. Struct. 2015, 26, 541–553. [Google Scholar] [CrossRef]




| Design Variable | ||
|---|---|---|
| 0.45 | 3 | |
| 0.16 | 1.5 | |
| α (°) | 36 | 72 |
| 0.005 | 2.5 | |
| 30 | 100 |
| Optimum Values | Cost Function (N) | |||||
|---|---|---|---|---|---|---|
( | n | |||||
| Case I | 3 | 0.6762 | 72 | 0.0025 | 50 | 79.5811 |
| Case II | 3 | 0.6762 | 72 | --- | --- | 79.5876 |
| P (kPa) | (°) | Cost Function (Case I) (N) | Cost Function (Case II) (N) | ||
|---|---|---|---|---|---|
| 3 | 0.6762 | 72 | 19.8965 | 19.8969 | |
| 3 | 0.6762 | 72 | 39.7038 | 39.7922 | |
| 3 | 0.6762 | 72 | 79.5811 | 79.5876 | |
| 3 | 0.6762 | 72 | 119.3668 | 119.3813 | |
| 3 | 0.6762 | 72 | 159.1492 | 159.1751 | |
| 3 | 0.6762 | 72 | 198.9487 | 198.9689 | |
| 3 | 0.6762 | 72 | 238.7335 | 238.7627 |
| Optimum Values | Optimized Cost Function | No. of Iterations | |||
|---|---|---|---|---|---|
(°) | |||||
| GA | 3 | 0.7509 | 72 | 77.1344 | 3 |
| GA+SQP | 3 | 0.6762 | 72 | 79.5875 | 5 |
(°) | Cost Function (N) | |||
|---|---|---|---|---|
| 3 | 0.65693 | 72 | 80.2197 | |
| 3 | 0.69566 | 72 | 79.58755 | |
| 3 | 0.67621 | 72 | 78.9497 |
| Reference | D (mm) | t (mm) | L (mm) | Materials | Blocked Force (N) | Free Contraction (%) |
|---|---|---|---|---|---|---|
| [30] | 9.525 | 1.587 | 152 | Silicone rubber PET | 40 | 4.6 |
| [56] | 4.6 | 0.4 | 43.9 | V330 elastomer bladder | 71 | 26.8 |
| [18] | 3 | 0.5 | 39.16 | Silicone tubing and PET braid | 60 | 44 |
| [33] | 10 | 1.6 | 270 | Latex and PET | 100 | 15 |
| Festo | 6 | --- | 30 | --- | 105 | 10 |
| Present work | 6 | 0.7 | 50 | Ecoflex-50 PET braids | 120 | 33.2 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zabihollah, S.; Moezi, S.A.; Sedaghati, R. Design Optimization of a Miniaturized Pneumatic Artificial Muscle and Experimental Validation. Actuators 2023, 12, 221. https://doi.org/10.3390/act12060221
Zabihollah S, Moezi SA, Sedaghati R. Design Optimization of a Miniaturized Pneumatic Artificial Muscle and Experimental Validation. Actuators. 2023; 12(6):221. https://doi.org/10.3390/act12060221
Chicago/Turabian StyleZabihollah, Shakila, Seyed Alireza Moezi, and Ramin Sedaghati. 2023. "Design Optimization of a Miniaturized Pneumatic Artificial Muscle and Experimental Validation" Actuators 12, no. 6: 221. https://doi.org/10.3390/act12060221
APA StyleZabihollah, S., Moezi, S. A., & Sedaghati, R. (2023). Design Optimization of a Miniaturized Pneumatic Artificial Muscle and Experimental Validation. Actuators, 12(6), 221. https://doi.org/10.3390/act12060221








