Increasing the Force Exertion of a Soft Actuator Using Externally Attachable Inter-Chamber Plates
Abstract
1. Introduction
2. Materials and Methods
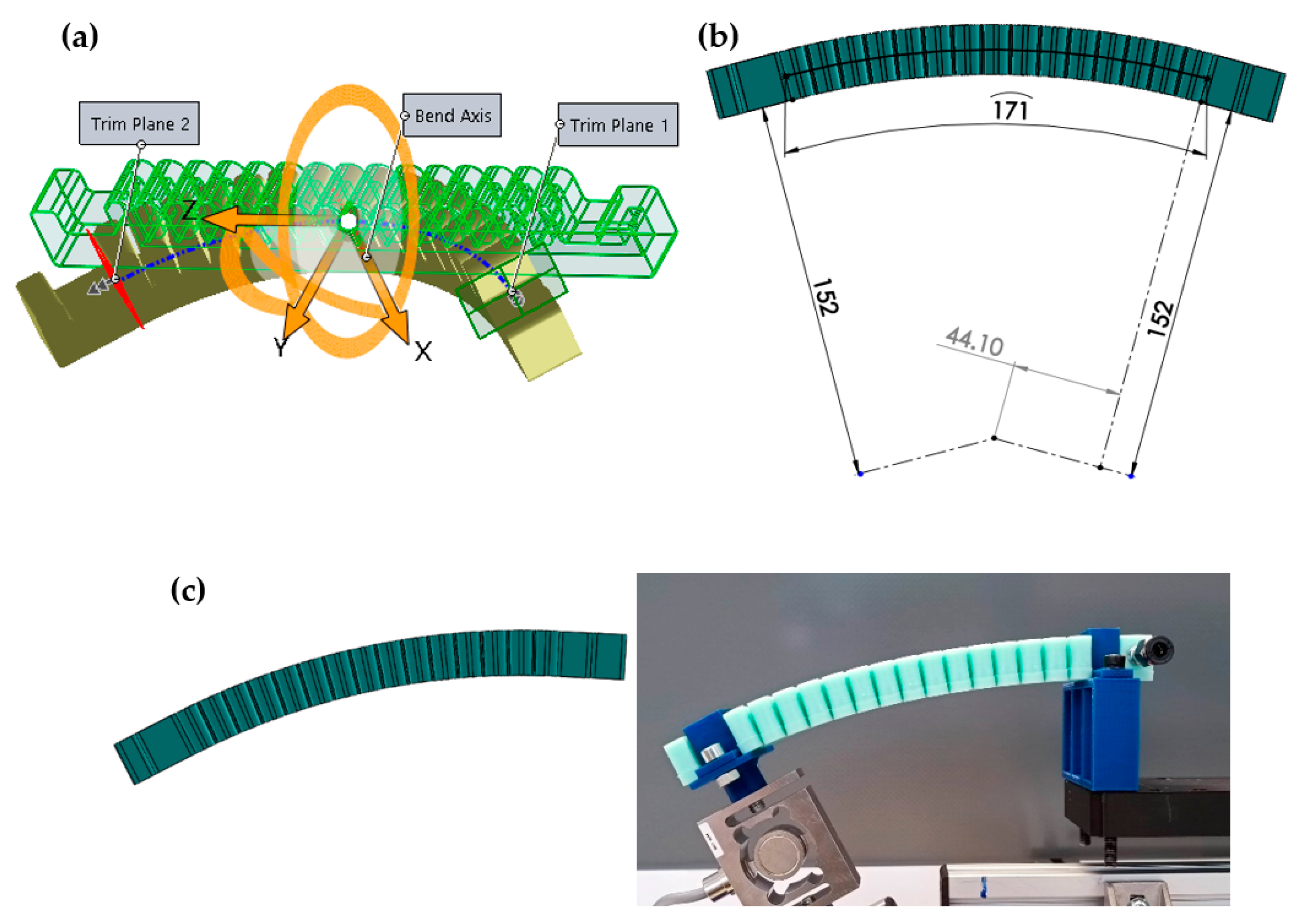
2.1. Structural Design and Fabrication of Soft Actuator
2.2. Structural Design and Fabrication of External Inter-Chamber Plates
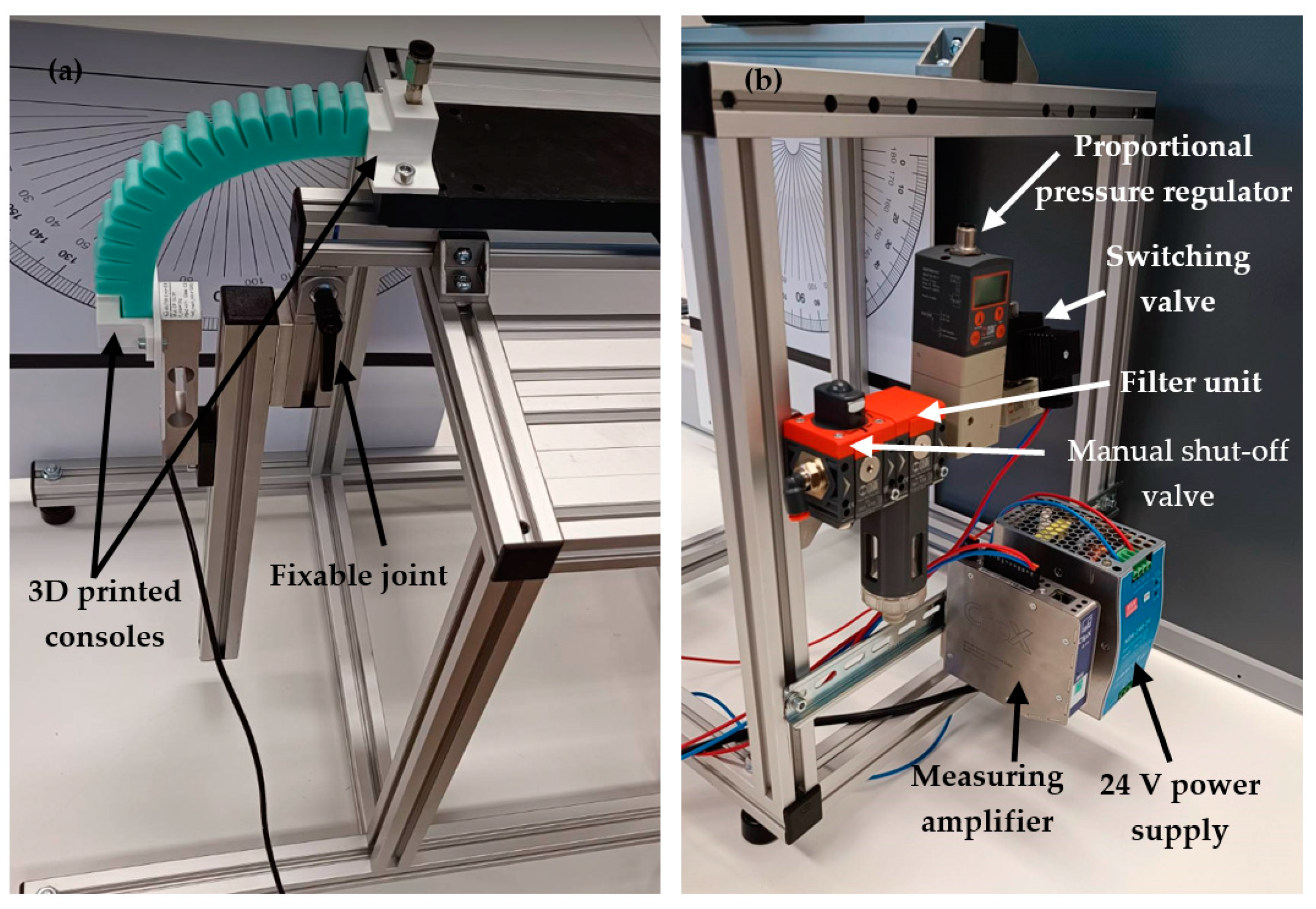
2.3. Experimental Setup
3. Results
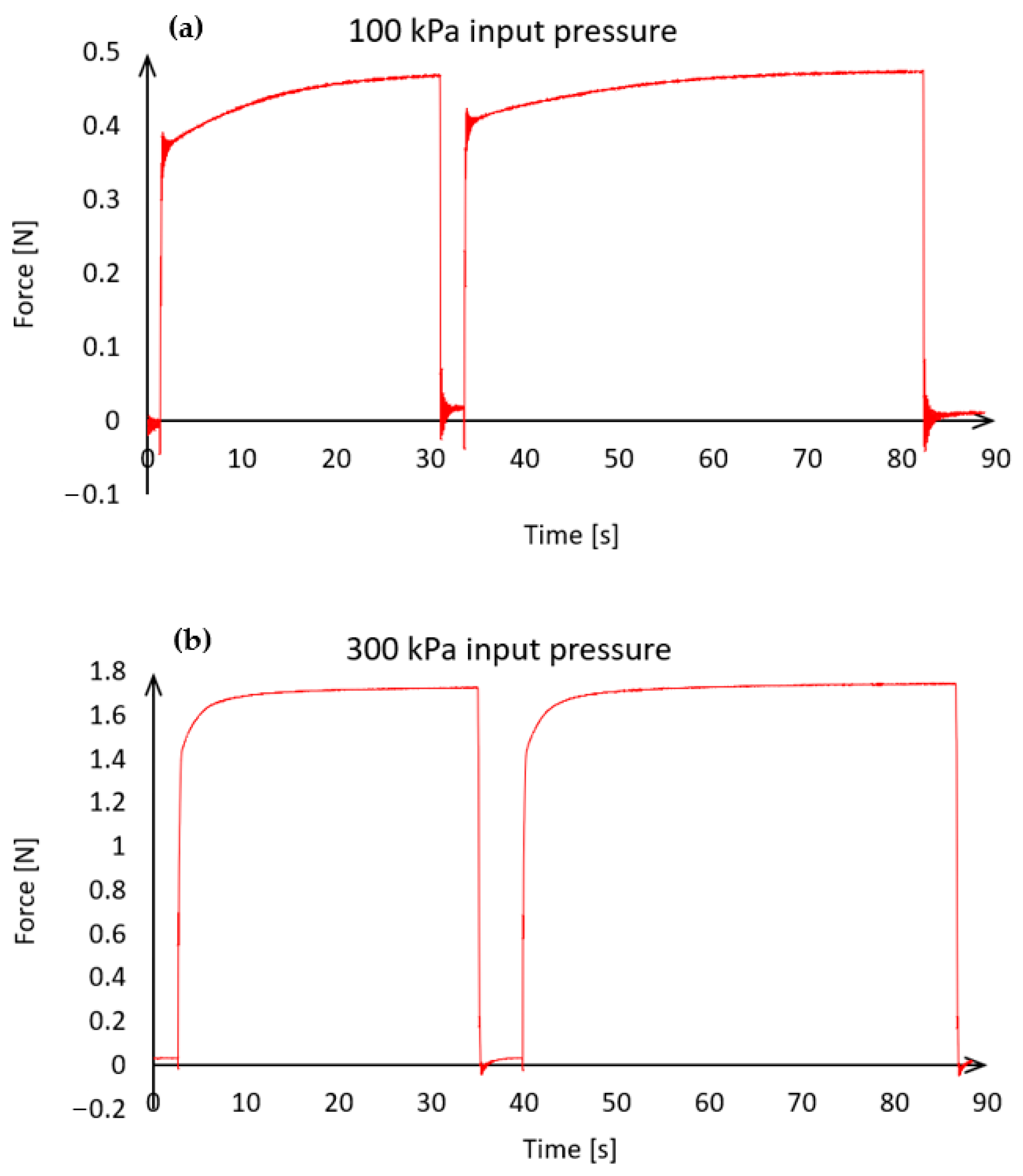
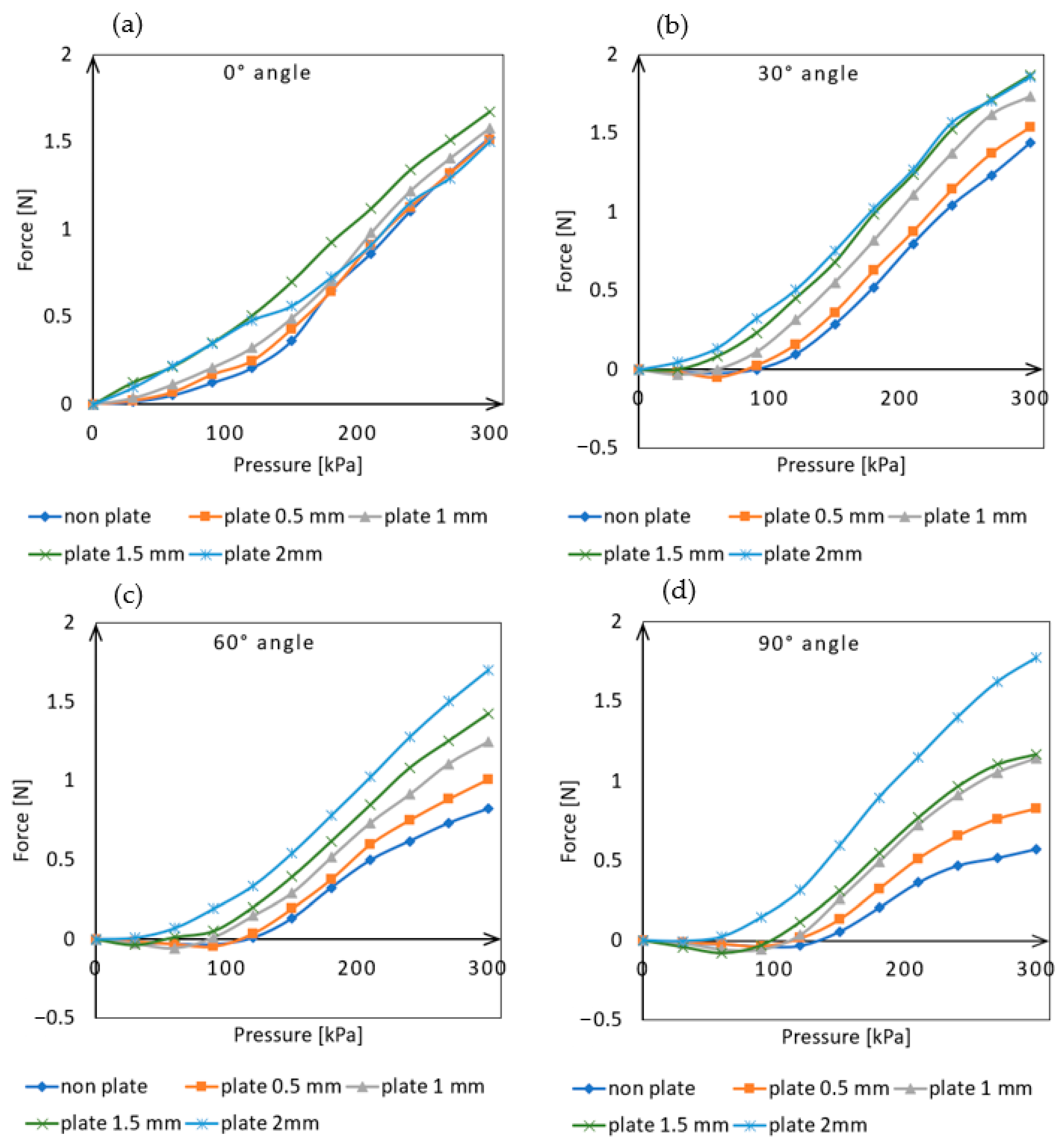
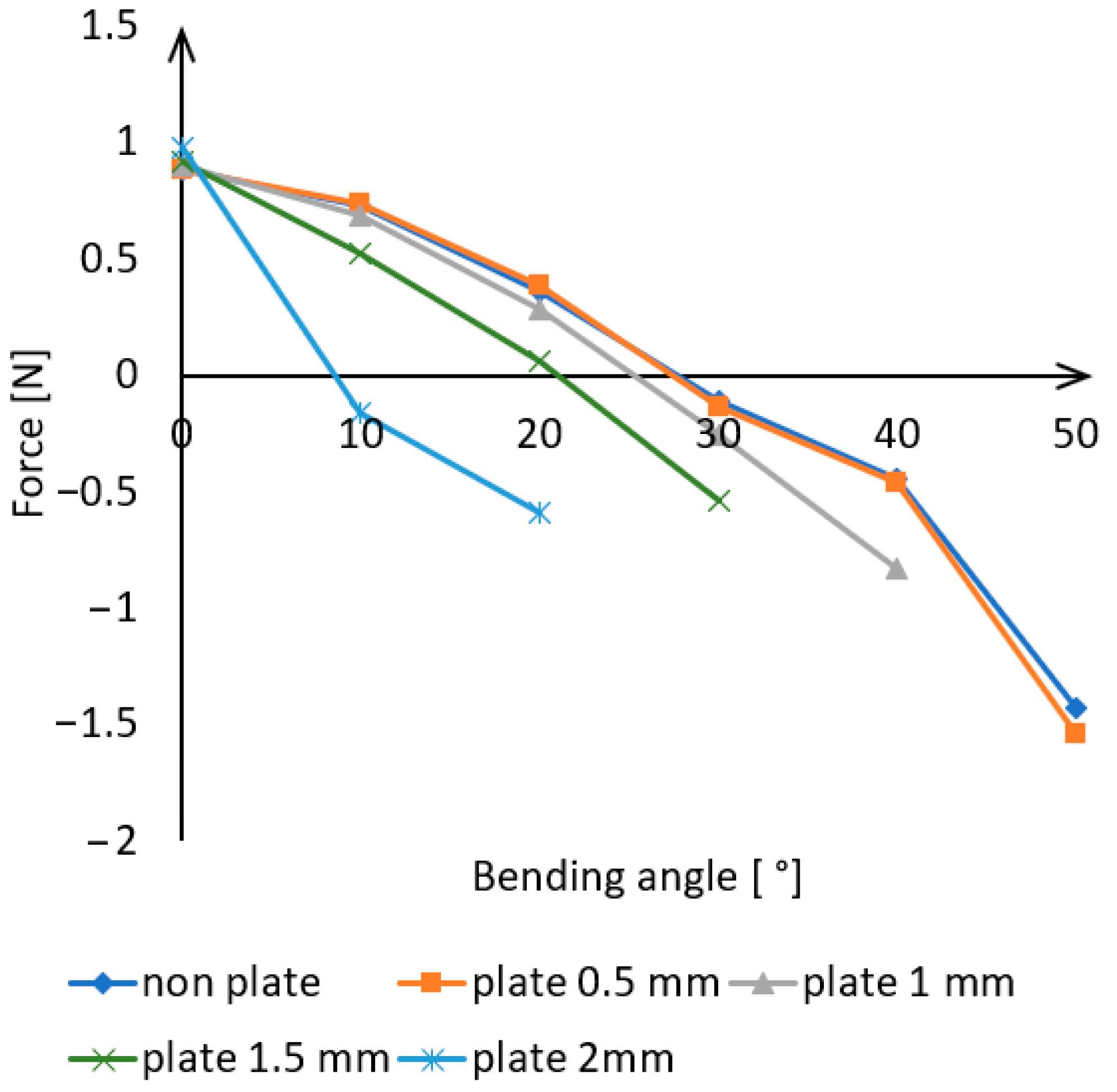
3.1. Force Measurement with Externally Attachable Inter-Chamber Plates
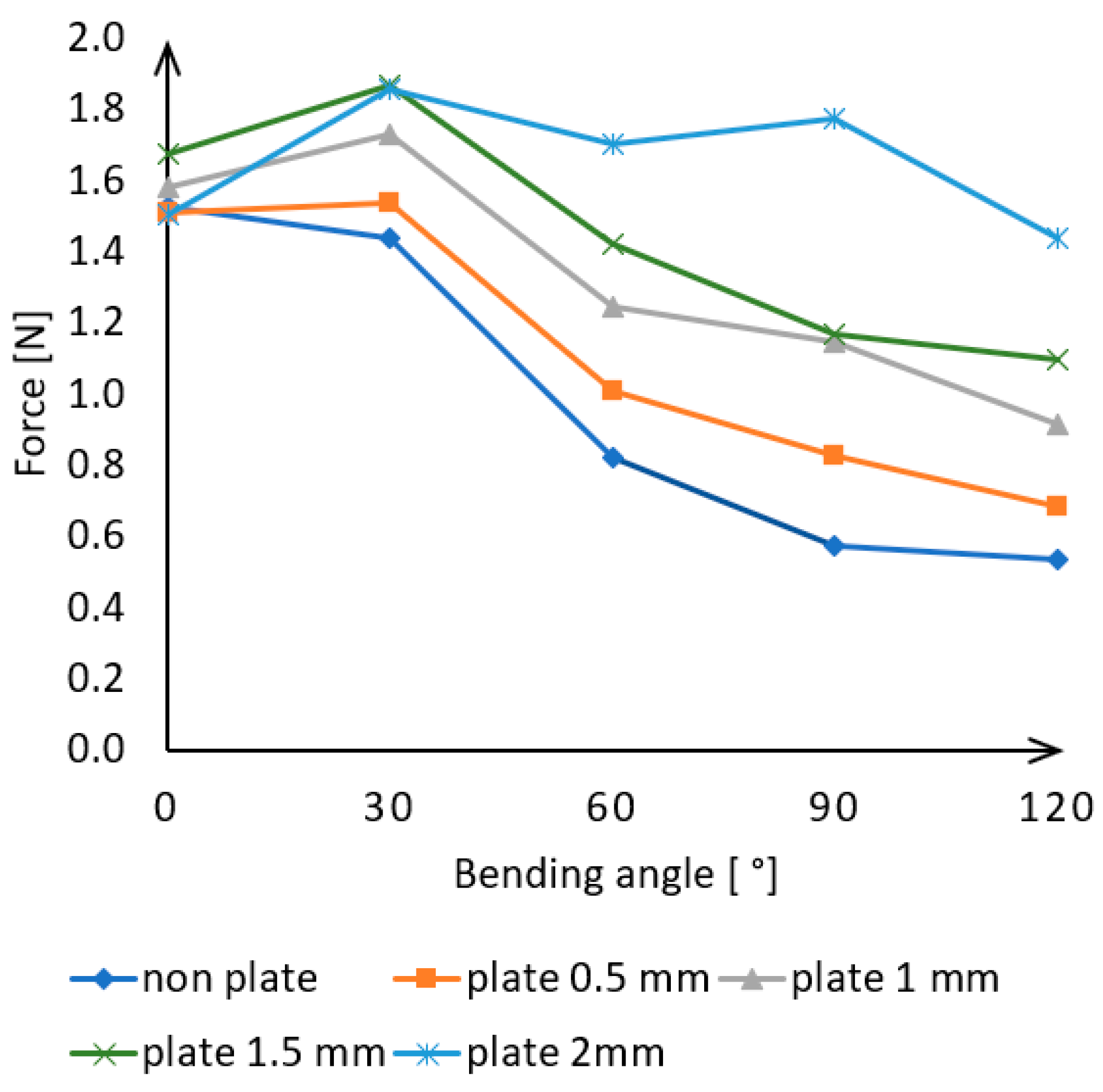
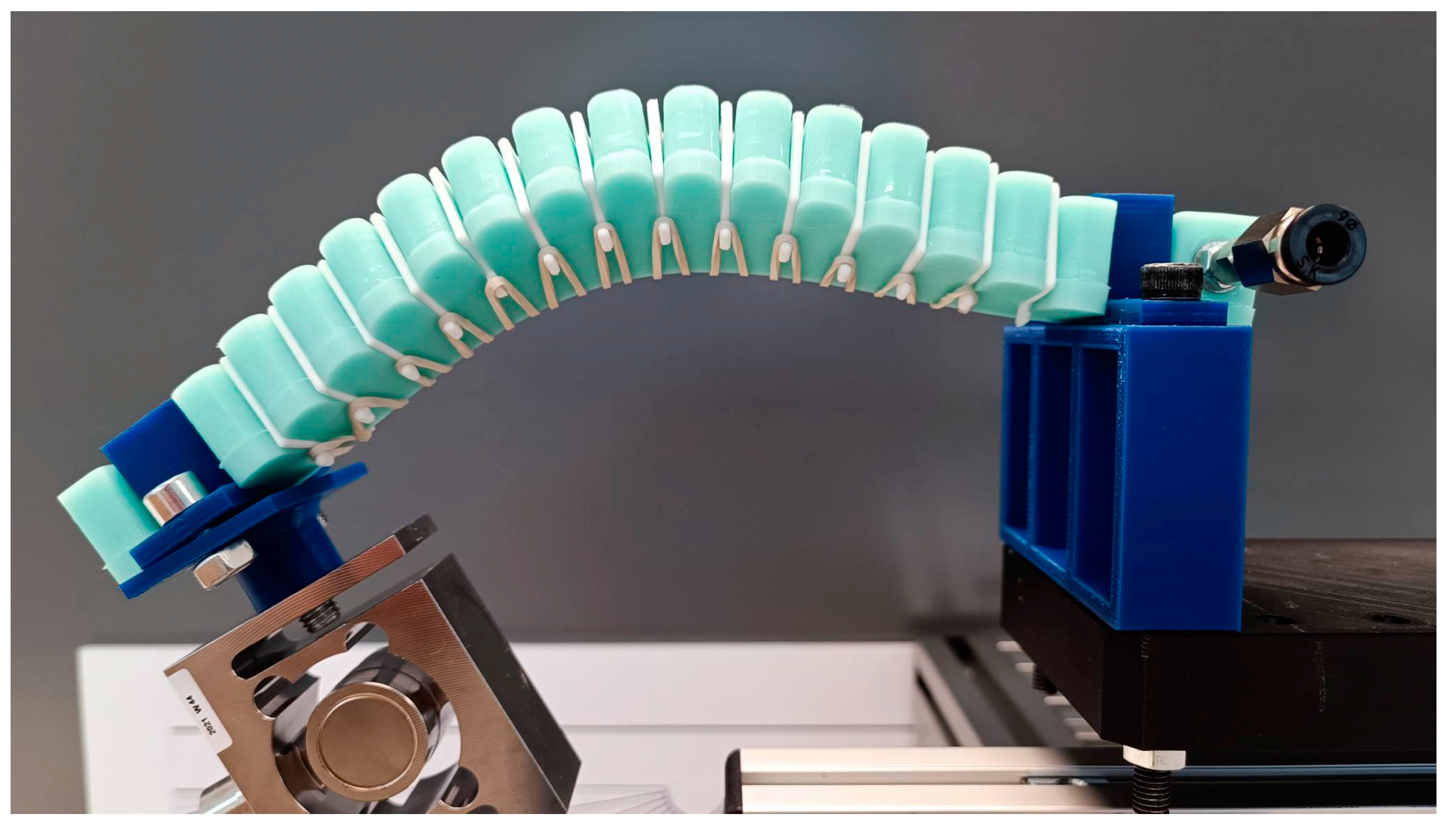
3.2. External Inter-Chamber Plates Effect on Passive Lateral Bending
4. Discussion
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Wei, Y.; Jia, D. Research on Robotic Arm Movement Grasping System Based on MYO. J. Phys. Conf. Ser. 2021, 1754, 012173. [Google Scholar] [CrossRef]
- Giberti, H.; Abbattista, T.; Carnevale, M.; Giagu, L.; Cristini, F. A Methodology for Flexible Implementation of Collaborative Robots in Smart Manufacturing Systems. Robotics 2022, 11, 9. [Google Scholar] [CrossRef]
- Matheson, E.; Minto, R.; Zampieri, E.G.G.; Faccio, M.; Rosati, G. Human–Robot Collaboration in Manufacturing Applications: A Review. Robotics 2019, 8, 100. [Google Scholar] [CrossRef]
- Trivedi, D.; Rahn, C.D.; Kier, W.M.; Walker, I.D. Soft robotics: Biological inspiration, state of the art, and future research. Appl. Bionics Biomech. 2008, 5, 99–117. [Google Scholar] [CrossRef]
- Rus, D.; Tolley, M.T. Design, fabrication and control of soft robots. Nature 2015, 521, 467–475. [Google Scholar] [CrossRef]
- Chou, C.-P.; Hannaford, B.; Hannaford, B. Measurement and modeling of McKibben pneumatic artificial muscles. IEEE Trans Robot. Autom 1996, 12, 90–102. [Google Scholar] [CrossRef]
- Sárosi, J.; Bíró, I.; Nemeth, J.; Cveticanin, L. Dynamic modeling of a pneumatic muscle actuator with two-direction motion. Mech. Mach. Theory 2015, 85, 25–34. [Google Scholar] [CrossRef]
- Marchese, A.D.; Katzschmann, R.K.; Rus, D. A Recipe for Soft Fluidic Elastomer Robots. Soft Robot. 2015, 2, 7–25. [Google Scholar] [CrossRef]
- Youn, J.-H.; Jeong, S.M.; Hwang, G.; Kim, H.; Hyeon, K.; Kyung, K.-U. Dielectric Elastomer Actuator for Soft Robotics Applications and Challenges. Appl. Sci. 2020, 10, 640. [Google Scholar] [CrossRef]
- Tse, Z.T.H.; Chen, Y.; Hovet, S.; Ren, H.; Cleary, K.; Xu, S.; Wood, B.; Monfaredi, R. Soft Robotics in Medical Applications. J Med. Robot. Res 2018, 3, 1841006. [Google Scholar] [CrossRef]
- Ashuri, T.; Armani, A.; Hamidi, R.J.; Hamidi, R.J.; Reasnor, T.; Ahmadi, S.; Iqbal, K. Biomedical soft robots: Current status and perspective. Biomed. Eng. Lett. 2020, 10, 369–385. [Google Scholar] [CrossRef]
- Deimel, R.; Brock, O. A novel type of compliant and underactuated robotic hand for dexterous grasping. Int. J. Robot. Res. 2016, 35, 161–185. [Google Scholar] [CrossRef]
- Suzumori, K.; Iikura, S.; Tanaka, H. Applying a flexible microactuator to robotic mechanisms. IEEE Control Syst. Mag. 1992, 12, 21–27. [Google Scholar] [CrossRef]
- Onal, C.D.; Marchese, A.D.; Rus, D. autonomous soft robotic fish capable of escape maneuvers using fluidic elastomer actuators. Soft Robot. 2014, 1, 75–87. [Google Scholar] [CrossRef]
- Tolley, M.T.; Shepherd, R.F.; Mosadegh, B.; Galloway, K.C.; Wehner, M.; Karpelson, M.; Wood, R.J.; Whitesides, G.M. Resilient, untethered soft robot. Soft Robot. 2015, 1, 213–223. [Google Scholar] [CrossRef]
- Verl, A.; Albu-Schffer, A.; Brock, O.; Raatz, A. Soft Robotics: Transferring Theory to Application; Springer: Berlin/Heidelberg, Germany, 2015. [Google Scholar]
- Chiaradia, D.; Xiloyannis, M.; Antuvan, C.W.; Frisoli, A.; Masia, L. Design and embedded control of a soft elbow exosuit. Int. Conf. Soft Robot. 2018, 565–571. [Google Scholar] [CrossRef]
- Park, S.-J.; Park, C.H. Suit-type Wearable Robot Powered by Shape-memory-alloy-based Fabric Muscle. Sci. Rep. 2019, 9, 9157. [Google Scholar] [CrossRef]
- Liu, Z.; Yin, X.; Peng, K.; Wang, X.; Chen, Q. Soft pneumatic actuators adapted in multiple environments: A novel fuzzy cascade strategy for the dynamics control with hysteresis compensation. Mechatronics 2022, 84, 102797. [Google Scholar] [CrossRef]
- Xavier, M.S.; Tawk, C.; Zolfagharian, A.; Pinskier, J.; Howard, D.; Young, T.; Lai, J.; Harrison, S.M.; Yong, Y.K.; Bodaghi, M.; et al. Soft Pneumatic Actuators: A Review of Design, Fabrication, Modeling, Sensing, Control and Applications. IEEE Access 2022, 10, 59442–59485. [Google Scholar] [CrossRef]
- Miriyev, A.; Stack, K.W.; Lipson, H. Soft material for soft actuators. Nat. Commun. 2017, 8, 596. [Google Scholar] [CrossRef] [PubMed]
- Wang, J.; Jue, W. Alex Chortos Control Strategies for Soft Robot Systems. Adv. Intell. Syst. 2022, 4, 2100165. [Google Scholar] [CrossRef]
- Chen, W.; Xiong, C.; Liu, C.; Peimin, L.; Chen, Y. Fabrication and dynamic modeling of bidirectional bending soft actuator integrated with optical waveguide curvature sensor. Soft Robot. 2019, 6, 495–506. [Google Scholar] [CrossRef] [PubMed]
- Hu, W.; Mutlu, R.; Li, W.; Alici, G. A Structural Optimisation Method for a Soft Pneumatic Actuator. Robotics 2018, 7, 24. [Google Scholar] [CrossRef]
- Paez, L.; Agarwal, G.; Paik, J. Design and Analysis of a Soft Pneumatic Actuator with Origami Shell Reinforcement. Soft Robot. 2016, 3, 109–119. [Google Scholar] [CrossRef]
- Polygerinos, P.; Wang, Z.; Overvelde, J.T.B.; Galloway, K.C.; Wood, R.J.; Bertoldi, K.; Walsh, C.J. Modeling of Soft Fiber-Reinforced Bending Actuators. IEEE Trans. Robot. 2015, 31, 778–789. [Google Scholar] [CrossRef]
- Yap, H.K.; Ang, B.W.K.; Lim, J.H.; Goh, J.C.H.; Yeow, C.-H. A fabric-regulated soft robotic glove with user intent detection using EMG and RFID for hand assistive application. In Proceedings of the 2016 IEEE International Conference on Robotics and Automation (ICRA), Stockholm, Sweden, 16–21 May 2016; pp. 3537–3542. [Google Scholar] [CrossRef]
- Mosadegh, B.; Polygerinos, P.; Keplinger, C.; Wennstedt, S.W.; Shepherd, R.; Gupta, U.; Shim, J.; Bertoldi, K.; Walsh, C.J.; Whitesides, G.M. Pneumatic Networks for Soft Robotics that Actuate Rapidly. Adv. Funct. Mater. 2014, 24, 2163–2170. [Google Scholar] [CrossRef]
- Jing, X.; Chen, S.; Zhang, C.; Xie, F. Increasing Bending Performance of Soft Actuator by Silicon Rubbers of Multiple Hardness. Machines 2022, 10, 272. [Google Scholar] [CrossRef]
- Chen, Y.; Le, S.; Tan, Q.C.; Lau, O.; Wan, F.; Song, C. A reconfigurable hybrid actuator with rigid and soft components. In Proceedings of the 2017 IEEE international conference on robotics and automation (ICRA), Singapore, 29 May–3 June 2017; pp. 58–63. [Google Scholar] [CrossRef]
- Galloway, K.C.; Polygerinos, P.; Walsh, C.J.; Wood, R.J. Mechanically programmable bend radius for fiber-reinforced soft actuators. In Proceedings of the IEEE International Conference on Advanced Robotics, Tainan, Taiwan, 31 May–2 June 2013; pp. 1–6. [Google Scholar] [CrossRef]
- Sun, Z.; Guo, Z.; Wei, T. Design of wearable hand rehabilitation glove with soft hoop-reinforced pneumatic actuator. J. Cent. South Univ. 2019, 26, 106–119. [Google Scholar] [CrossRef]
- Zhu, L.; Wang, F.; Liu, S.; Tian, Y.; Zhang, D. Modeling and Analysis of Soft Pneumatic Network Bending Actuators. IEEE-ASME Trans. Mechatron. 2020, 26, 2195–2203. [Google Scholar] [CrossRef]






| 3D Printer Manufacturing Features | Values |
|---|---|
| Nozzle diameter | 0.4 mm |
| Nozzle temperature | 210 °C |
| Object table temperature | 60 °C |
| Printing speed | 50 mm/s |
| Layer height | 0.2 mm |
| Outer layer number | 3 |
| Infill density | 30% |
| 3D Printer Manufacturing Features | Values |
|---|---|
| Nozzle diameter | 0.4 mm |
| Nozzle temperature | 210 °C |
| Object table temperature | 60 °C |
| Printing speed | 0 mm/s |
| Layer height | 0.1 mm |
| Outer layer number | 2 |
| Infill density | 100% |
| Measuring Angle [°] | Trimming Pane Distance from Hinge Point [mm] |
|---|---|
| 30 | 59.81 |
| 60 | 34.6 |
| 90 | 5.52 |
| 120 | −37.61 |
| Measuring Angle [°] | Trimming Pane Distance from Hinge Point [mm] |
|---|---|
| 10 | 71.54 |
| 20 | 57.81 |
| 30 | 44.1 |
| 40 | 30.19 |
| 50 | 15.83 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Mészáros, A.; Sárosi, J. Increasing the Force Exertion of a Soft Actuator Using Externally Attachable Inter-Chamber Plates. Actuators 2023, 12, 222. https://doi.org/10.3390/act12060222
Mészáros A, Sárosi J. Increasing the Force Exertion of a Soft Actuator Using Externally Attachable Inter-Chamber Plates. Actuators. 2023; 12(6):222. https://doi.org/10.3390/act12060222
Chicago/Turabian StyleMészáros, Attila, and József Sárosi. 2023. "Increasing the Force Exertion of a Soft Actuator Using Externally Attachable Inter-Chamber Plates" Actuators 12, no. 6: 222. https://doi.org/10.3390/act12060222
APA StyleMészáros, A., & Sárosi, J. (2023). Increasing the Force Exertion of a Soft Actuator Using Externally Attachable Inter-Chamber Plates. Actuators, 12(6), 222. https://doi.org/10.3390/act12060222







