Power Saving in Magnetorquers by Operating in Cryogenic Environments
Abstract
1. Introduction
2. Theory
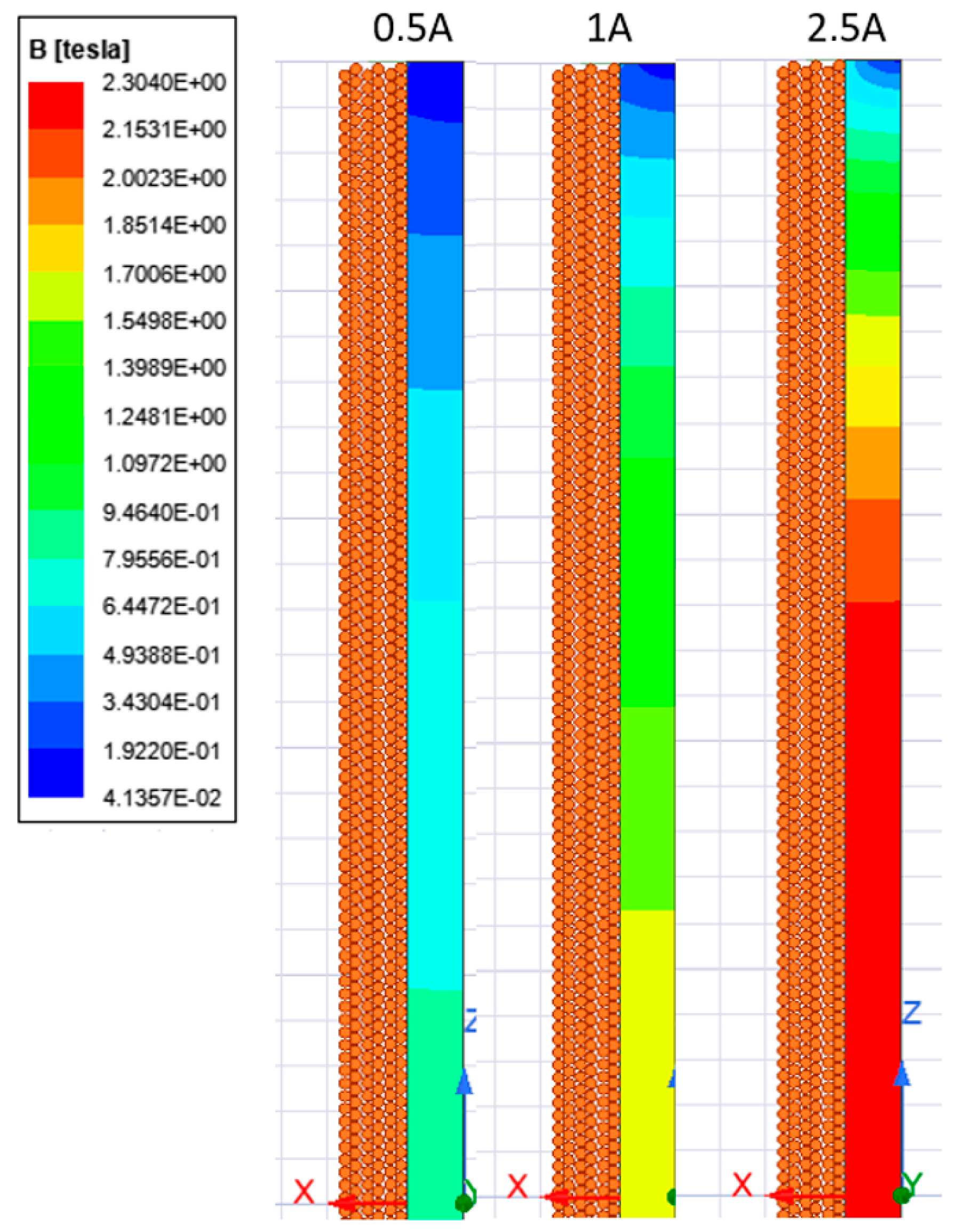
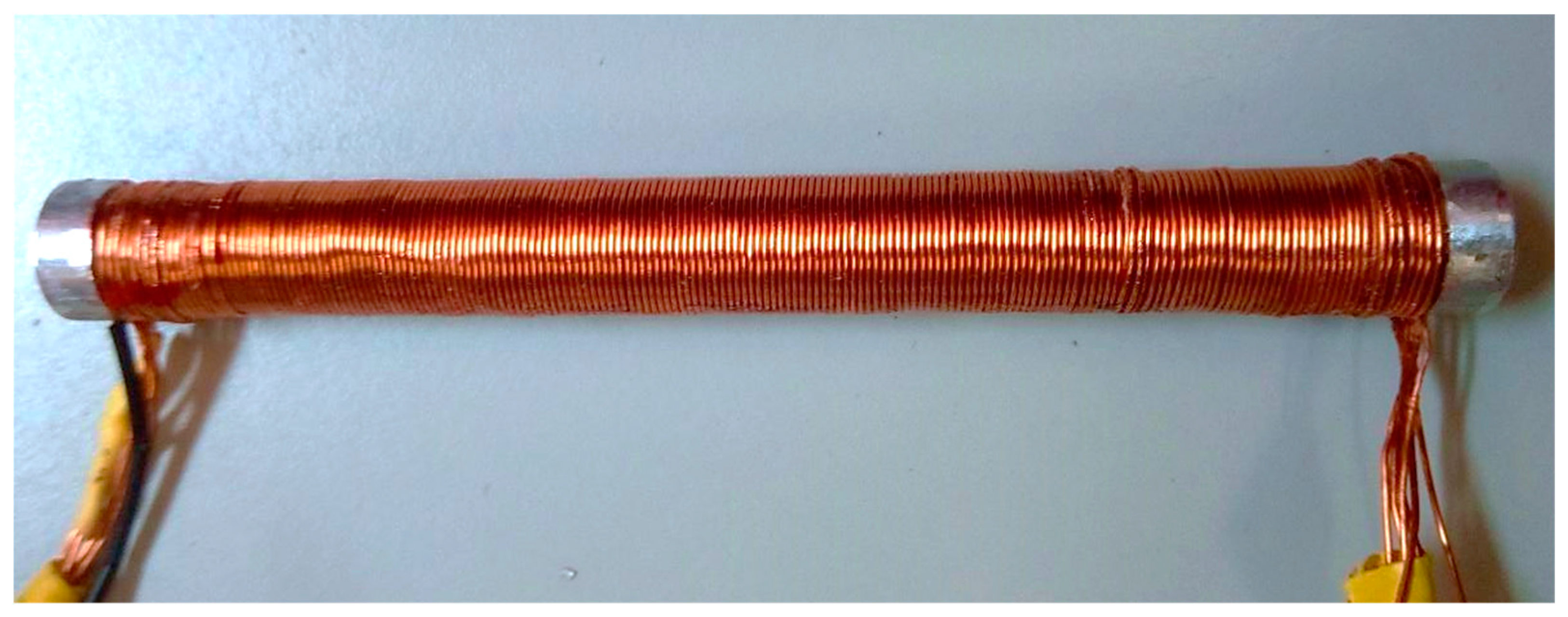
2.1. Magnetorquer Design and Electromagnetic FEA Model
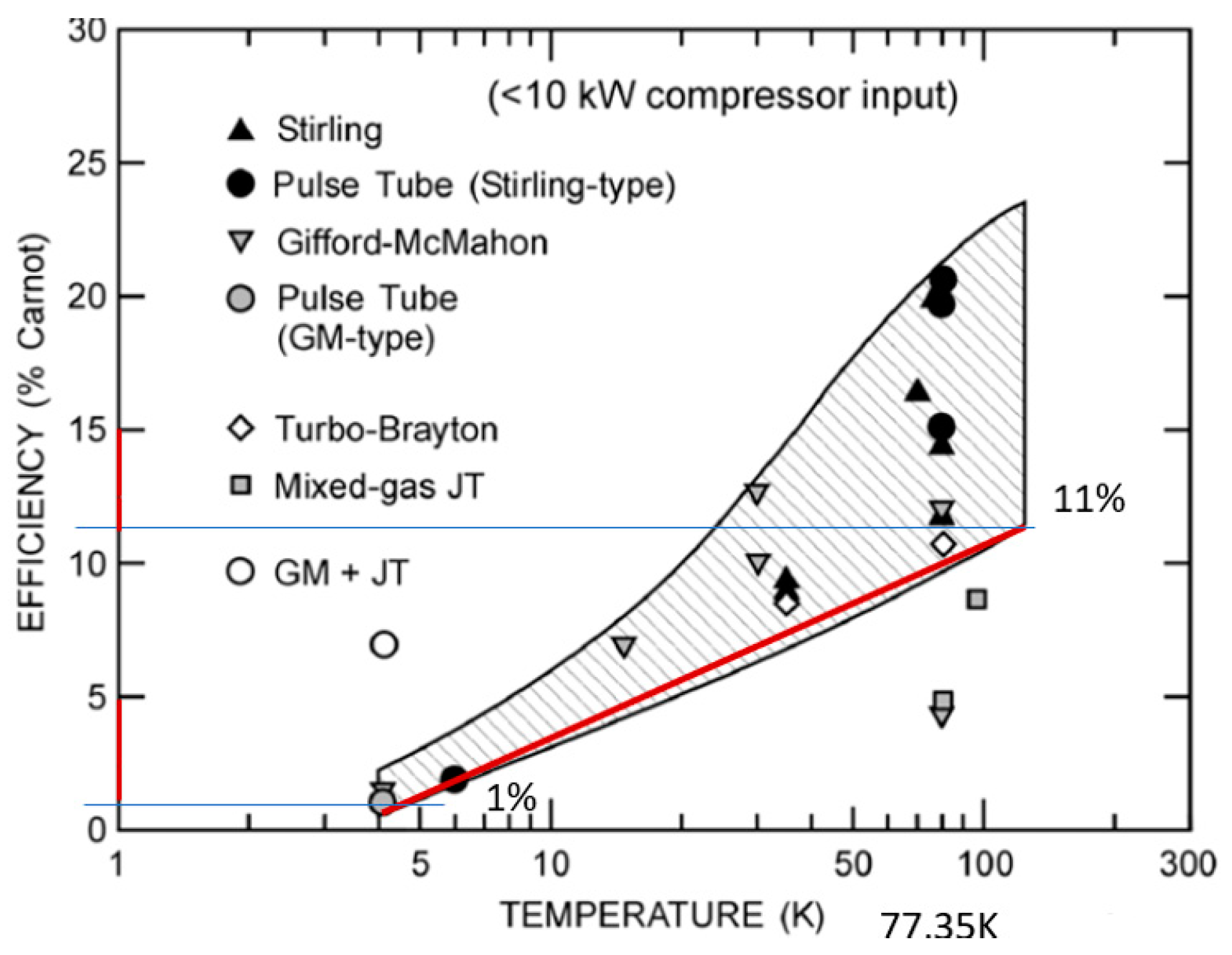
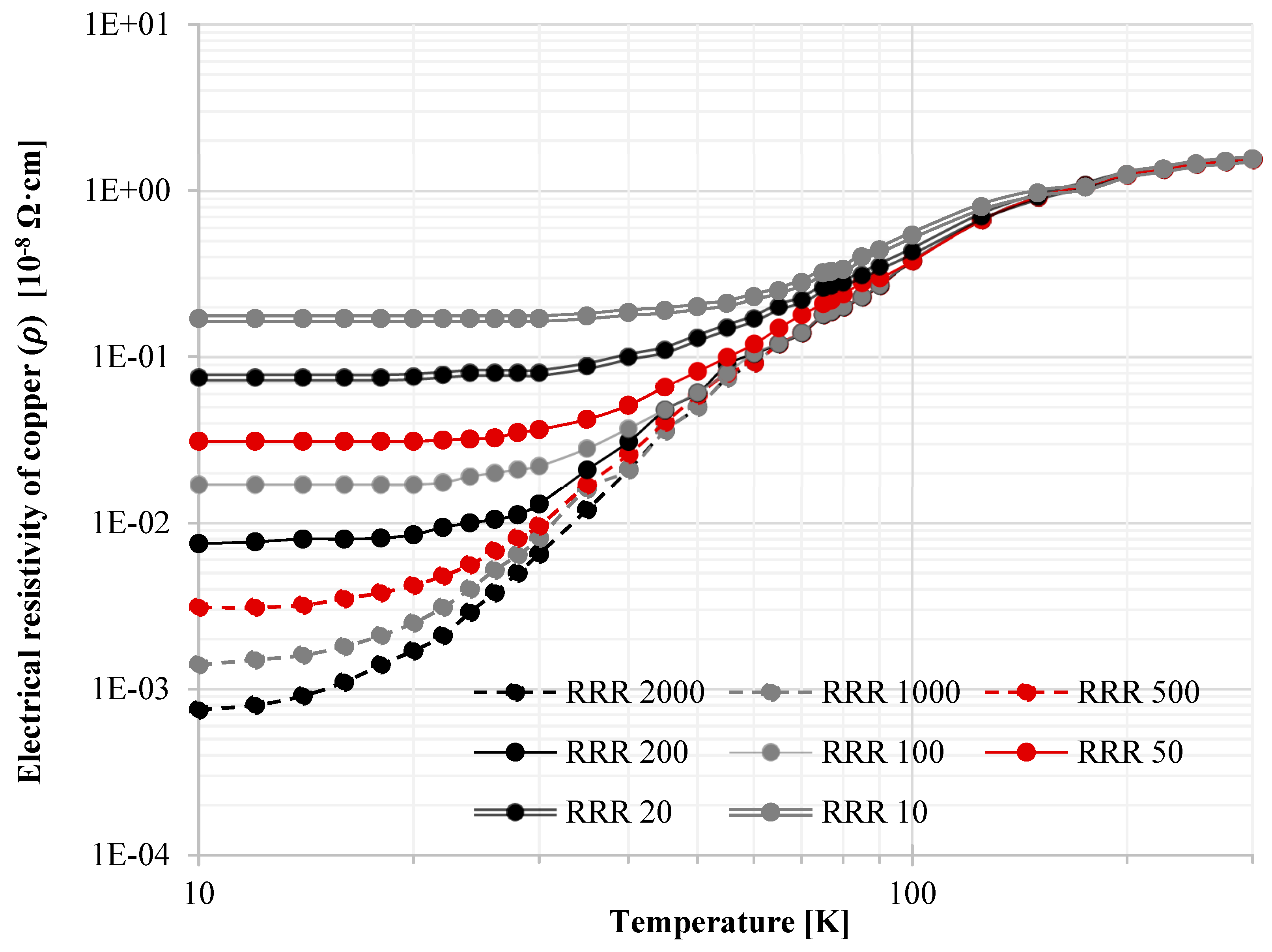
2.2. Thermal Behavior of a Magnetorquer in Cryogenics and Cryocooling Systems
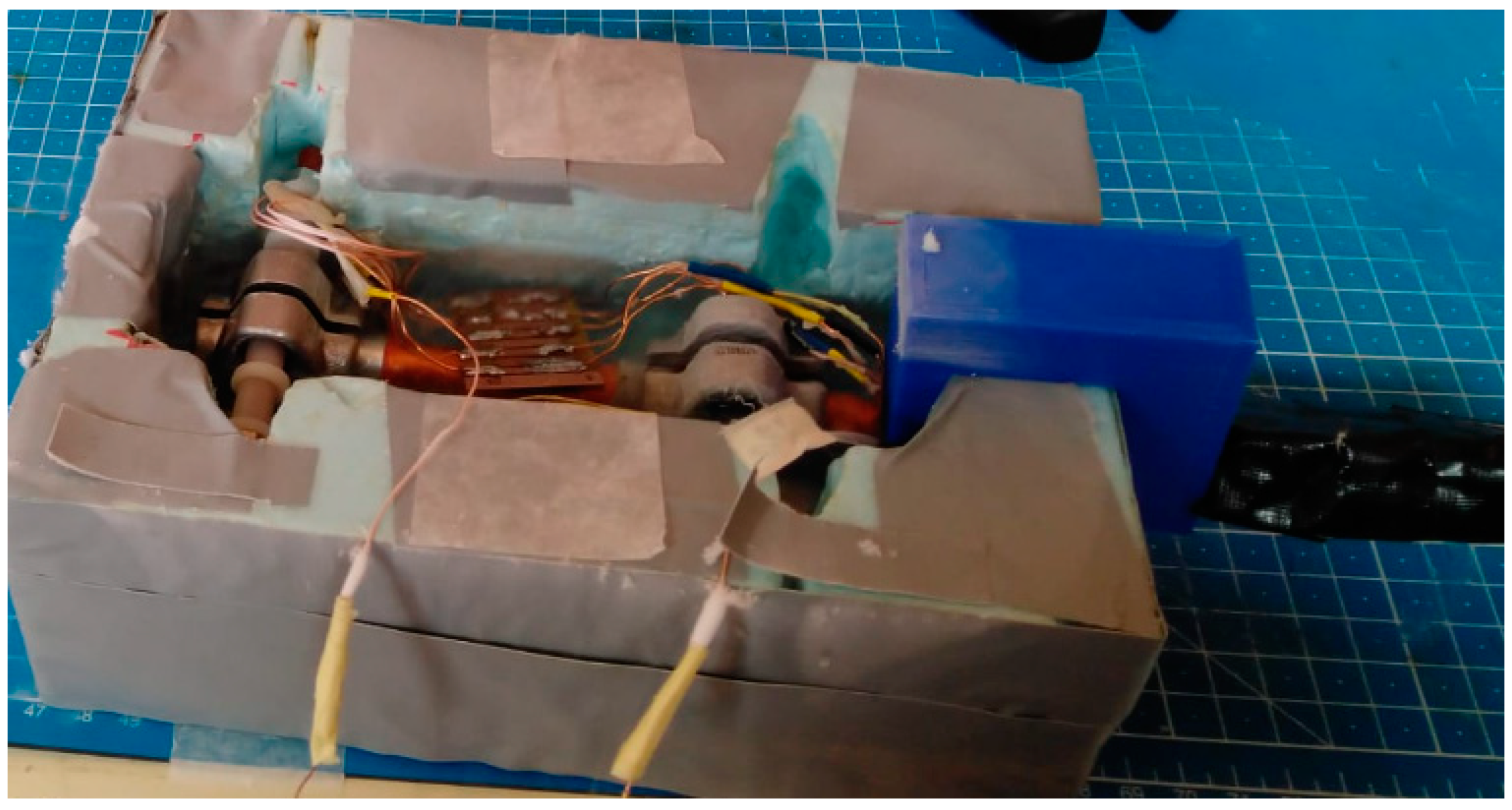
3. Experimental Procedure: Room and Cryogenic Temperature Testing
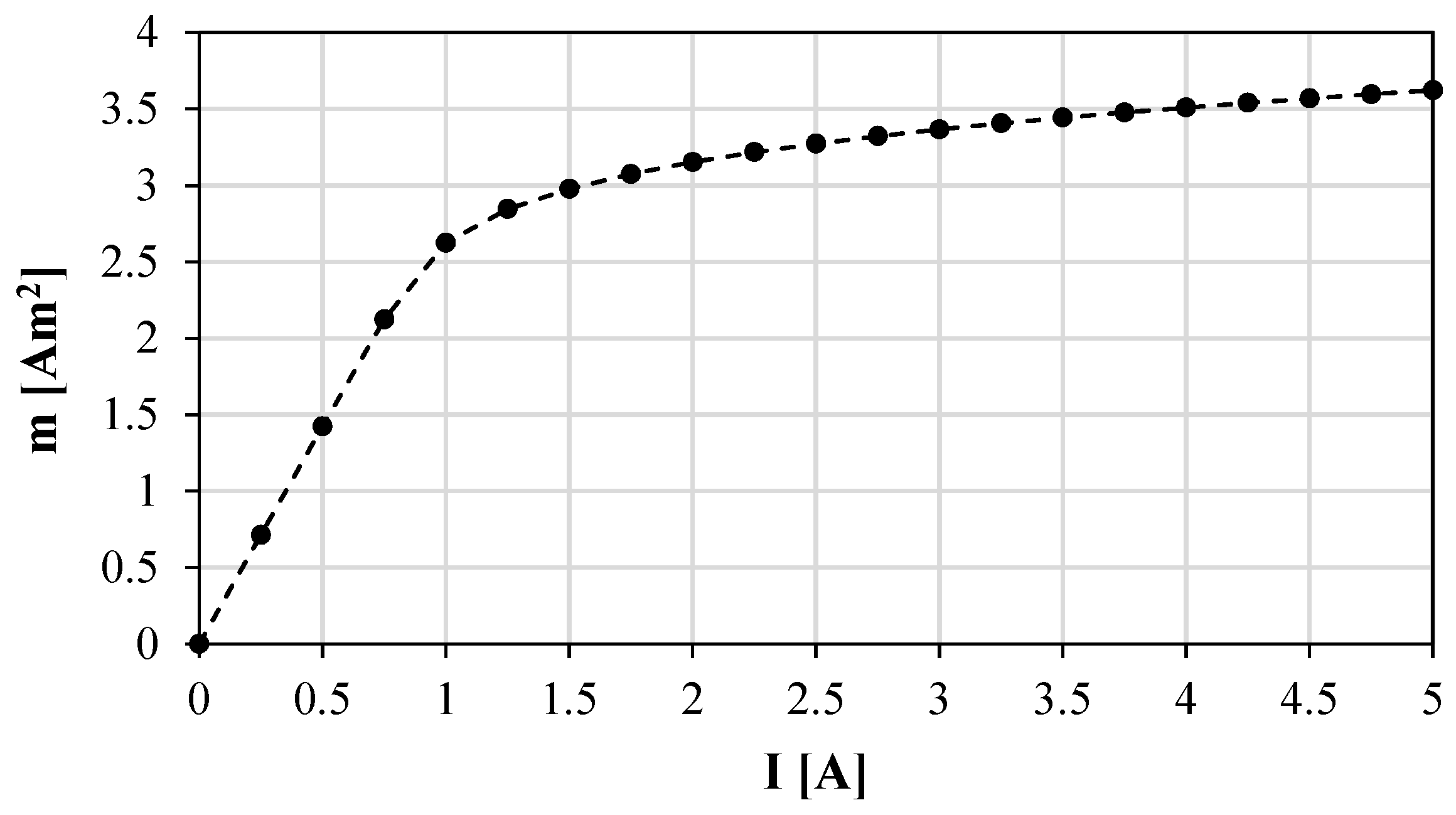
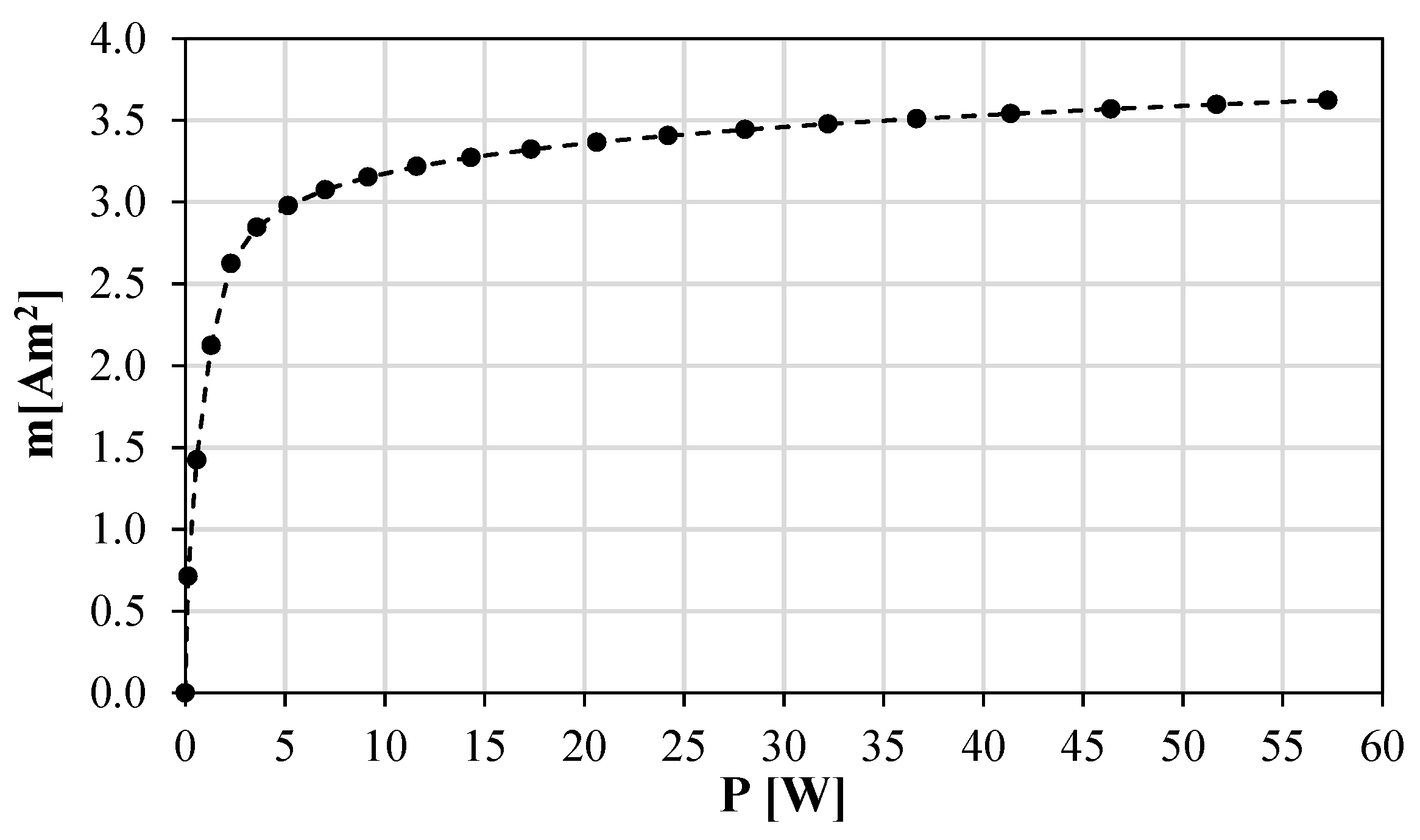
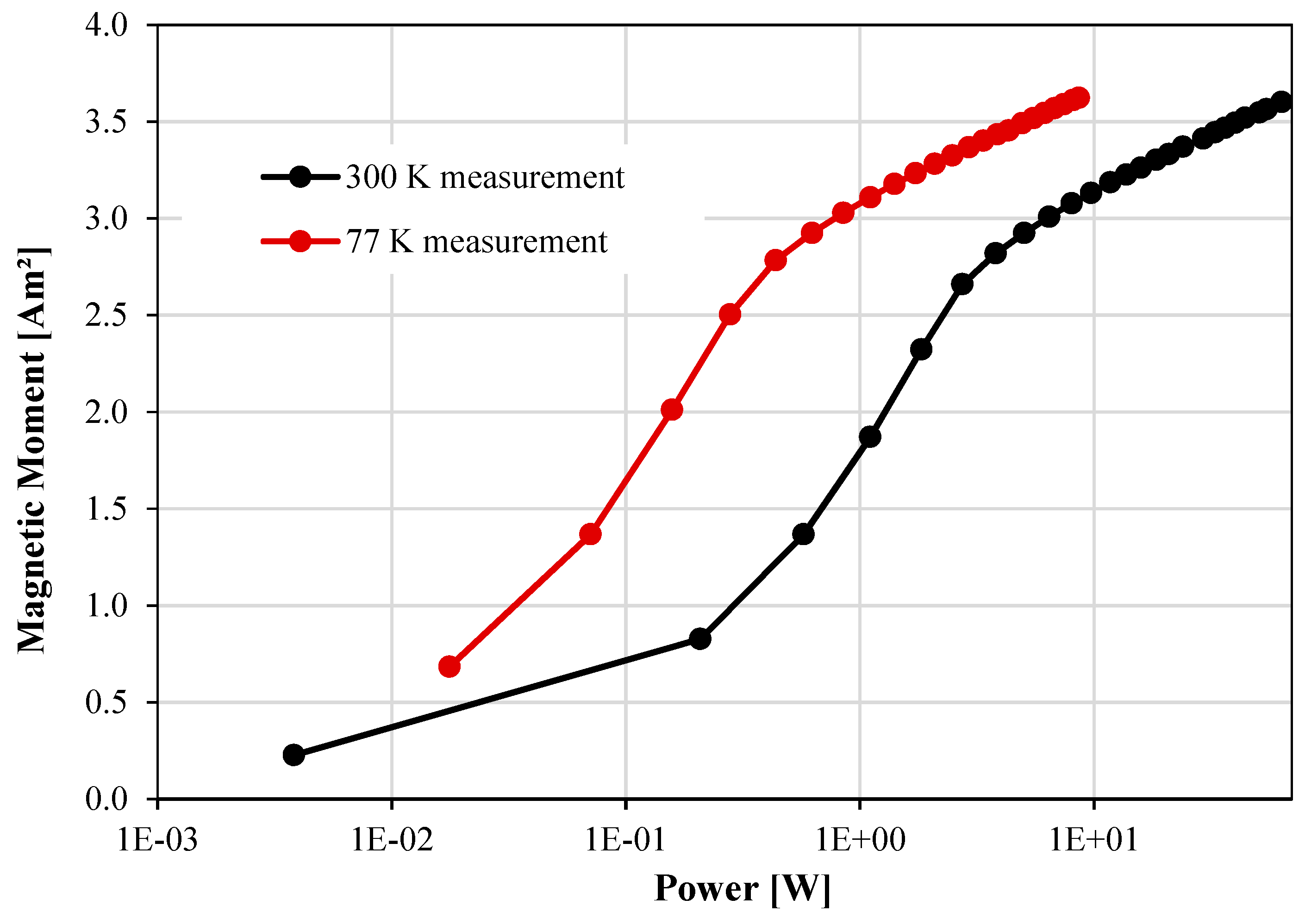
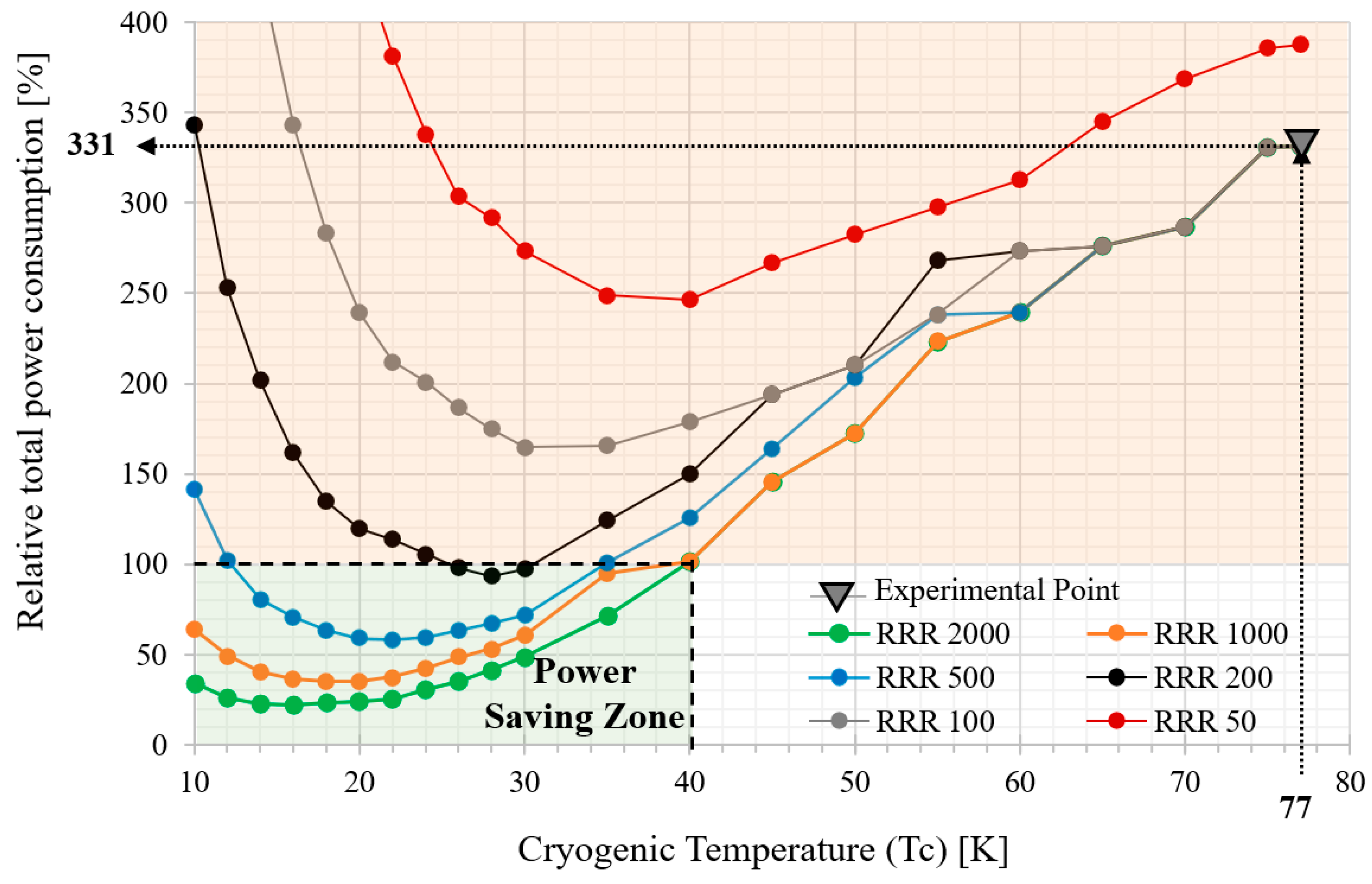
4. Results
5. Discussion
6. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
Appendix A
| Mission | Application | Type/Class | Launch Year | Cryogenic System | In-Flight Temp. (k) | Lifetime |
|---|---|---|---|---|---|---|
| ERS (ESA)-1/2 | Earth observation | P/L (ATSR) | 1991/1995 | Stirling cooler | 80 | 2 y |
| CRISTA (DARA, D) | Earth observation | P/L (STS-66/85) | 1994/197 | 4He cryostat | 2.5–12 | 10 d |
| SFU (ISAS/NASDA/MITI) | Science (IR) | Instrument (IRST) | 1995 | 4He cryostat + 3He SC | 0.3 | 30 d |
| MSX (BMDO, US) | MP/UV to FIR | Satellite (observat.) | 1996 | sH2 cryostat | <8 | 600 d |
| BETSCE (NASA) | Technology | P/L (STS-77) | 1996 | H2 Stirling + JT + Sorpt. | 10 | <1 d |
| CheX (NASA) | Tech./MS | P/L (STS-87) | 1997 | 4He cryostat | 1.6 | >6 d |
| HST (NASA) | Science (NIR) | Nicmos, instrument | 1997 | sN2 cryostat | 60 | 700 d |
| WIRE (NASA) | Science (IR) | Satellite (surveyor) | 1999 | Dual, sH2 cryostat | <7.5 | 120 d |
| RHESSI (NASA) | Science (solar phys.) | Satellite (observat.) | 2002 | Stirling cooler | 85 | >5 yr |
| FACET (NASA/JPL) | Technology | P/L (STS) | 2003 | sCO2 + sNe cryostat | 19 | >6 d |
| Suzaku (ISAS, NASA) | Science (X-ray) | Satellite (observat.) | 2005 | sNe + 4He cryost. + ADR | 0.065 | 730 d |
| XEUS (ESA) | Science (X-ray) | Instrument (observat.) | 2005 | Stirling cool. + ADR | 0.05–0.3 | >10 yr |
| ISS/Bosch (ESA) | Tech./TLC | P/L(ISS) | 2005 | Mechanical cooler | 77 | >1 yr |
| Akari (ISAS) | Science (IR) | Satellite (observat.) | 2006 | 4He (λ) cryost. + cooler | 1.8 | 550 d |
References
- Wekerle, T.; Filho, J.B.P.; da Costa, L.E.V.L.; Trabasso, L.G. Status and trends of smallsats and their launch vehicles—An up-to-date review. J. Aerosp. Technol. Manag. 2017, 9, 269–286. [Google Scholar] [CrossRef]
- Lombardo, M.; Zannoni, M.; Gai, I.; Casajus, L.G.; Gramigna, E.; Manghi, R.L.; Tortora, P.; Di Tana, V.; Cotugno, B.; Simonetti, S.; et al. Design and Analysis of the Cis-Lunar Navigation for the ArgoMoon CubeSat Mission. Aerospace 2022, 9, 659. [Google Scholar] [CrossRef]
- Inamori, T.; Kawashima, R.; Saisutjarit, P.; Sako, N.; Ohsaki, H. Magnetic plasma deorbit system for nano- and micro-satellites using magnetic torquer interference with space plasma in low Earth orbit. Acta Astronaut. 2015, 112, 192–199. [Google Scholar] [CrossRef]
- Park, J.H.; Matsuzawa, S.; Inamori, T.; Jeung, I.S. Nanosatellite constellation deployment using on-board magnetic torquer interaction with space plasma. Adv. Space Res. 2018, 61, 2010–2021. [Google Scholar] [CrossRef]
- Mesch, F. Magnetic components for the attitude control of space vehicles. IEEE Trans. Magn. 1969, 5, 586–592. [Google Scholar] [CrossRef]
- Rajagopal, K.R. Design and development of a permanent magnet torquer for a gyroscope. IEEE Trans. Magn. 2001, 37, 2630–2633. [Google Scholar] [CrossRef]
- Rajagopal, K.R.; Singh, B.; Singh, B.P.; Vedachalam, N. Novel methods of temperature compensation for permanent magnet sensors and actuators. IEEE Trans. Magn. 2001, 37, 1995–1997. [Google Scholar] [CrossRef]
- Zhu, L.; Guo, J.; Gill, E. Analytical Field and Torque Analysis of a Reaction Sphere. IEEE Trans. Magn. 2018, 54, 1–11. [Google Scholar] [CrossRef]
- McChesney, C. Attitude control actuators for a simulated spacecraft. In Proceedings of the AIAA Guidance, Navigation, and Control Conference, Portland, OR, USA, 8–11 August 2011; pp. 1–25. [Google Scholar] [CrossRef]
- Bellar, A.; Mohammed, M.A.S.; Adnane, A. Minimum power consumption of the microsatellite attitude control using pyramidal reaction wheel configuration. In Proceedings of the 8th International Conference on Modelling, Identification and Control (ICMIC), Algiers, Algeria, 15–17 November 2016; Volume 2016, pp. 253–257. [Google Scholar] [CrossRef]
- NASA Smallsats: In-Space Propulsion Systems. Available online: https://www.nasa.gov/smallsat-institute/sst-soa/in-space-propulsion#_Toc114987899 (accessed on 24 March 2023).
- van Schyndel, J.; Goos, E.; Naumann, C.; Hardi, J.S.; Oschwald, M. Effects of Compounds in Liquefied Methane on Rocket Engine Operation. Aerospace 2022, 9, 698. [Google Scholar] [CrossRef]
- Silik, Y.; Yaman, U. Control of rotary inverted pendulum by using on–off type of cold gas thrusters. Actuators 2020, 9, 95. [Google Scholar] [CrossRef]
- Nyberg, E.; Cervelló, D.L.I.; Minami, I. Tribology in space robotic actuators: Experimental method for evaluation and analysis of gearboxes. Aerospace 2021, 8, 75. [Google Scholar] [CrossRef]
- Alcover-Sanchez, R.; Soria, J.M.; Pérez-Aracil, J.; Pereira, E.; Diez-Jimenez, E. Design and experimental characterization of a novel passive magnetic levitating platform. Smart Struct. Syst. 2022, 29, 499–512. [Google Scholar] [CrossRef]
- Diez-Jimenez, E.; Alén-Cordero, C.; Alcover-Sánchez, R.; Corral-Abad, E. Modelling and test of an integrated magnetic spring-eddy current damper for space applications. Actuators 2021, 10, 8. [Google Scholar] [CrossRef]
- Muñoz-Martínez, M.; Diez-Jimenez, E.; Gómez-García, M.J.; Rizzo, R.; Musolino, A. Torque and bearing reaction forces simulation of micro-magnetic gears. Appl. Comput. Electromagn. Soc. J. 2019, 34, 541–546. [Google Scholar]
- Fiorillo, F.; Santoni, F.; Ferrara, E.; Battagliere, M.L.; Bottauscio, O.; Graziani, F. Soft magnets for passive attitude stabilization of small satellites. IEEE Trans. Magn. 2010, 46, 670–673. [Google Scholar] [CrossRef]
- Serrano-Tellez, J.; Romera-Juarez, F.; González-De-María, D.; Lamensans, M.; Argelaguet-Vilaseca, H.; Pérez-Díaz, J.-L.; Sánchez-Casarrubios, J.; Díez-Jiménez, E.; Valiente-Blanco, I. Experience on a cryogenic linear mechanism based on superconducting levitation. In Proceedings of the SPIE—The International Society for Optical Engineering, Amsterdam, The Netherlands, 2 June 2012; Volume 8450. [Google Scholar] [CrossRef]
- Esnoz-Larraya, J.; Valiente-Blanco, I.; Cristache, C.; Sanchez-Garcia-Casarrubios, J.; Rodriguez-Celis, F.; Diez-Jimenez, E.; Perez-Diaz, J.L. OPTIMAGDRIVE: High performance magntic gears development for space applications. In Proceedings of the 17th European Space Mechanisms and Tribology Symposium, Hatfield, UK, 20–22 September 2017; pp. 1–5. [Google Scholar]
- Cristache, C.; Diez-Jimenez, E.; Valiente-Blanco, I.; Sanchez-Garcia-Casarrubios, J.; Perez-Diaz, J.-L. Aeronautical magnetic torque limiter for passive protection against overloads. Machines 2016, 4, 17. [Google Scholar] [CrossRef]
- Perez-Diaz, J.L.; Diez-Jimenez, E.; Valiente-Blanco, I.; Cristache, C.; Alvarez-Valenzuela, M.-A.; Sanchez-Garcia-Casarrubios, J. Contactless mechanical components: Gears, torque limiters and bearings. Machines 2014, 2, 312–324. [Google Scholar] [CrossRef]
- Perez-Diaz, J.L.; Diez-Jimenez, E.; Valiente-Blanco, I.; Cristache, C.; Alvarez-Valenzuela, M.-A.; Sanchez-Garcia-Casarrubios, J.; Ferdeghini, C.; Canepa, F.; Hornig, W.; Carbone, G.; et al. Performance of Magnetic-Superconductor Non-contact Harmonic Drive for Cryogenic space applications. Machines 2015, 3, 138–156. [Google Scholar] [CrossRef]
- Diez-Jimenez, E.; Sanchez-Montero, R.; Martinez-Muñoz, M. Towards miniaturization of magnetic gears: Torque performance assessment. Micromachines 2017, 9, 16. [Google Scholar] [CrossRef]
- Martinez-Muñoz, M.; Diez-Jimenez, E.; Villalba-Alumbreros, G.V.; Michalowski, M.; Lastra-Sedano, A. Geometrical dependence in fixtures for 2D multipole micromagnets magnetization pattering. Appl. Comput. Electromagn. Soc. J. 2019, 34, 1092–1101. [Google Scholar]
- Martinez-Muñoz, M.; DIez-Jimenez, E.; Sanchez-Montero, R.; Lopez-Espi, P.L.; Martinez-Rojas, J.A. Analysis of the geometric parameters influence in PCB fixtures for 2D multipole magnetization patterning of thin layer micro-magnets. Int. J. Appl. Electromagn. Mech. 2019, 61, 59–71. [Google Scholar] [CrossRef]
- Diez-Jimenez, E.; Valiente-Blanco, I.; Villalba-Alumbreros, G.; Fernandez-Munoz, M.; Lopez-Pascual, D.; Lastra-Sedano, A.; Moron-Alguacil, C.; Martinez-Perez, A. Multilayered Microcoils for Microactuators and Characterization of Their Operational Limits in Body-Like Environments. Trans. Mechatron. 2022, 1–6. [Google Scholar] [CrossRef]
- Misra, R.; Wisniewski, R.; Zuyev, A. Attitude Stabilization of a Satellite Having Only Electromagnetic Actuation Using Oscillating Controls. Aerospace 2022, 9, 444. [Google Scholar] [CrossRef]
- Celani, F. Quaternion versus Rotation Matrix Feedback for Spacecraft Attitude Stabilization Using Magnetorquers. Aerospace 2022, 9, 24. [Google Scholar] [CrossRef]
- Feng, G.; Zhang, C.; Zhang, H.; Li, W. Theoretical and Experimental Investigation of Geomagnetic Energy Effect for LEO Debris Deorbiting. Aerospace 2022, 9, 511. [Google Scholar] [CrossRef]
- Ivanov, D.S.; Ovchinnikov, M.Y.; Penkov, V.I.; Roldugin, D.S.; Doronin, D.M.; Ovchinnikov, A.V. Advanced numerical study of the three-axis magnetic attitude control and determination with uncertainties. Acta Astronaut. 2017, 132, 103–110. [Google Scholar] [CrossRef]
- Curatolo, A.; Bahu, A.; Modenini, D. Automatic Balancing for Satellite Simulators with Mixed Mechanical and Magnetic Actuation. Aerospace 2022, 9, 223. [Google Scholar] [CrossRef]
- Mehrjardi, M.F.; Mirshams, M. Design and manufacturing of a research magnetic torquer rod. In Proceedings of the Fourth International Conference on Experimental Mechanics, Singapore, 18–20 November 2009; Volume 7522, p. 75221W. [Google Scholar] [CrossRef]
- Cervettini, G.; Pastorelli, S.; Park, H.; Lee, D.Y.; Romano, M. Development and Experimentation of a CubeSat Magnetic Attitude Control System Testbed. IEEE Trans. Aerosp. Electron. Syst. 2021, 57, 1345–1350. [Google Scholar] [CrossRef]
- Mughal, M.R.; Ali, H.; Ali, A.; Praks, J.; Reyneri, L.M. Optimized Design and Thermal Analysis of Printed Magnetorquer for Attitude Control of Reconfigurable Nanosatellites. IEEE Trans. Aerosp. Electron. Syst. 2020, 56, 736–747. [Google Scholar] [CrossRef]
- Sorensen, N.J. Efficiency-Optimized Design of PCB-Integrated Magnetorquers for CubeSats. IEEE Trans. Aerosp. Electron. Syst. 2021, 57, 3623–3632. [Google Scholar] [CrossRef]
- Lee, J.; Ng, A.; Jobanputra, R. On determining dipole moments of a magnetic torquer rod—Experiments and discussions. Can. Aeronaut. Space J. 2002, 48, 61–67. [Google Scholar] [CrossRef]
- Allen, C.S.; Giraudo, M.; Moratto, C.; Yamaguchi, N. Spaceflight environment. In Space Safety and Human Performance; Elsevier: Amsterdam, The Netherlands, 2017; pp. 87–138. [Google Scholar]
- Ilcev, S.D. LOW Earth Orbits (LEO). In Proceedings of the 20th International Crimean Conference on Microwave and Telecommunication Technology, Sevastopol, Ukraine, 13–17 September 2010; pp. 406–408. [Google Scholar] [CrossRef]
- Collaudin, B.; Rando, N. Cryogenics in space: A review of the missions and of the technologies. Cryogenics 2000, 40, 797–819. [Google Scholar] [CrossRef]
- Diez-jimenez, E.; Perez-diaz, J.L.; Garcia-Prada, J.C. Mechanical Method for Experimental Determination of the First-Penetration Field in. IEEE Trans. Appl. Supercond. 2012, 22, 9003106. [Google Scholar] [CrossRef]
- Perez-Diaz, J.L.; Valiente-Blanco, I.; Diez-Jimenez, E.; Sanchez-Garcia-Casarrubios, J. Superconducting Non-Contact Device for Precision Positioning in Cryogenic Environments. IEEE/ASME Trans. Mechatron. 2014, 19, 598–605. [Google Scholar] [CrossRef]
- Valiente-Blanco, I.; Diez-Jimenez, E.; Sanchez-Garcia-Casarrubios, J.; Perez-Diaz, J.L. Improving Resolution and Run Outs of a Superconducting Noncontact Device for Precision Positioning. IEEE/ASME Trans. Mechatron. 2014, 20, 1992–1996. [Google Scholar] [CrossRef]
- Collaudin, B.; Gavila, E. Cryogenic Integration in Satellites. 2019. Available online: https://indico.cern.ch/event/792215/contributions/3558254/attachments/1918508/3172791/Cryogenic_integration_in_satellites_-_ThalesAleniaSpace.pdf (accessed on 25 March 2023).
- Ansoft Ansys Maxwell v15—Help Assistant. 2018. Available online: http://ansoft-maxwell.narod.ru/en/CompleteMaxwell3D_V15.pdf (accessed on 21 January 2023).
- Candini, G.P.; Piergentili, F.; Santoni, F. Designing, Manufacturing, and Testing a Self-Contained and Autonomous Nanospacecraft Attitude Control System. J. Aerosp. Eng. 2014, 27, 04014033. [Google Scholar] [CrossRef]
- Candinia, G.P.; Piergentilib, F.; Santoni, F. Miniaturized attitude control system for nanosatellites. Acta Astronaut. 2012, 81, 325–334. [Google Scholar] [CrossRef]
- CubeSatShop Cubesat NCTR-M002 Magnetic Rod. Available online: https://www.cubesatshop.com/product/nctr-m002-magnetorquer-rod/ (accessed on 23 January 2023).
- CubeSatShop Cubesat NCTR-M012 Magnetic Rod. Available online: https://www.cubesatshop.com/product/nctr-m012-magnetorquer-rod/ (accessed on 23 January 2023).
- Sputnix SX-MT Magnetic Torquers. Available online: https://satsearch.co/products/sputnix-magnetic-torquer-sx-mt (accessed on 20 January 2023).
- Radebaugh, R. Cryocoolers: The state of the art and recent developments. J. Phys. Condens. Matter 2009, 21, 164219. [Google Scholar] [CrossRef]
- Calatroni, S. Materials & Properties: Thermal & Electrical Characteristics. arXiv 2020, arXiv:2006.02842, 1–20. Available online: http://arxiv.org/abs/2006.02842 (accessed on 19 January 2023).


| Quantity | Symbol | Value |
|---|---|---|
| Core radius (mm) | Rint | 2.5 |
| External winding radius (mm) | Rext | 5.45 |
| Length of the magnetorquer (mm) | L | 100 |
| Wire diameter (mm) | Dcable | 0.5 |
| Turns per layer | n | 185 |
| Device mass (g) | mass | 63.26 |
| Current (A) | I | 0.5 |
| Electrical resistance at 300 K (ohm) | R | 1.13 |
| Power consumption at 300 K (W) | Pw | 0.565 |
| Magnetic moment (Am2) | m | 1.42 |
| Length × Ø (mm × mm) | m (Am2) | m/Power (Am2/W) | m/I (Am2/A) | m/Mass (Am2/kg) | Ref. |
|---|---|---|---|---|---|
| 40 × 10.6 | 0.018 | 0.267 | 0.360 | 1.200 | [46,47] |
| 70 × 9 | 0.200 | 1.000 | 5.000 | 6.667 | [48] |
| 94 × 13 | 1.190 | 1.488 | 7.438 | 22.453 | [49] |
| 140 × 16 | 1.000 | 2.5 | - | 5 | [50] |
| 100 × 10.9 | 1.42 | 2.52 | 2.85 | 22.5 | This work |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Villalba-Alumbreros, G.; Lopez-Pascual, D.; Valiente-Blanco, I.; Diez-Jimenez, E. Power Saving in Magnetorquers by Operating in Cryogenic Environments. Actuators 2023, 12, 181. https://doi.org/10.3390/act12050181
Villalba-Alumbreros G, Lopez-Pascual D, Valiente-Blanco I, Diez-Jimenez E. Power Saving in Magnetorquers by Operating in Cryogenic Environments. Actuators. 2023; 12(5):181. https://doi.org/10.3390/act12050181
Chicago/Turabian StyleVillalba-Alumbreros, Gabriel, Diego Lopez-Pascual, Ignacio Valiente-Blanco, and Efren Diez-Jimenez. 2023. "Power Saving in Magnetorquers by Operating in Cryogenic Environments" Actuators 12, no. 5: 181. https://doi.org/10.3390/act12050181
APA StyleVillalba-Alumbreros, G., Lopez-Pascual, D., Valiente-Blanco, I., & Diez-Jimenez, E. (2023). Power Saving in Magnetorquers by Operating in Cryogenic Environments. Actuators, 12(5), 181. https://doi.org/10.3390/act12050181







