Analytical Analysis of a Novel Flux Adjustable Permanent Magnet Eddy Current Coupling with Double-Sided Conductor
Abstract
1. Introduction
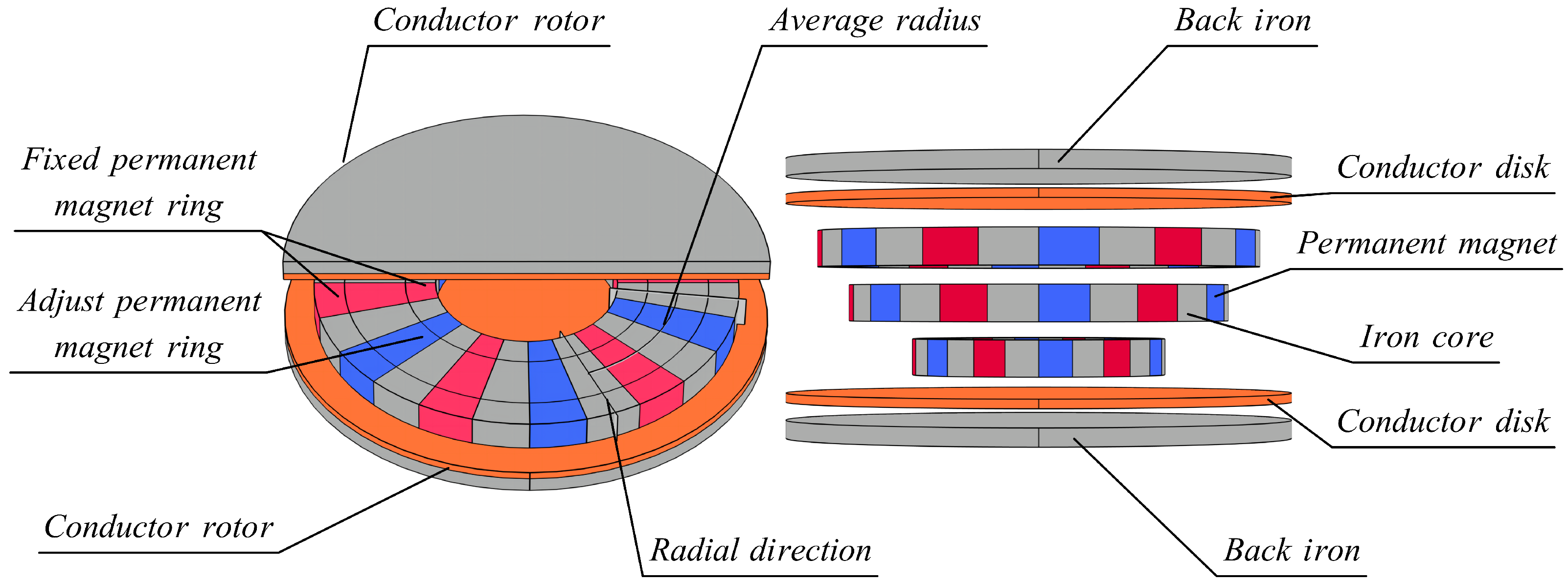
2. Topology
3. Analytical Model
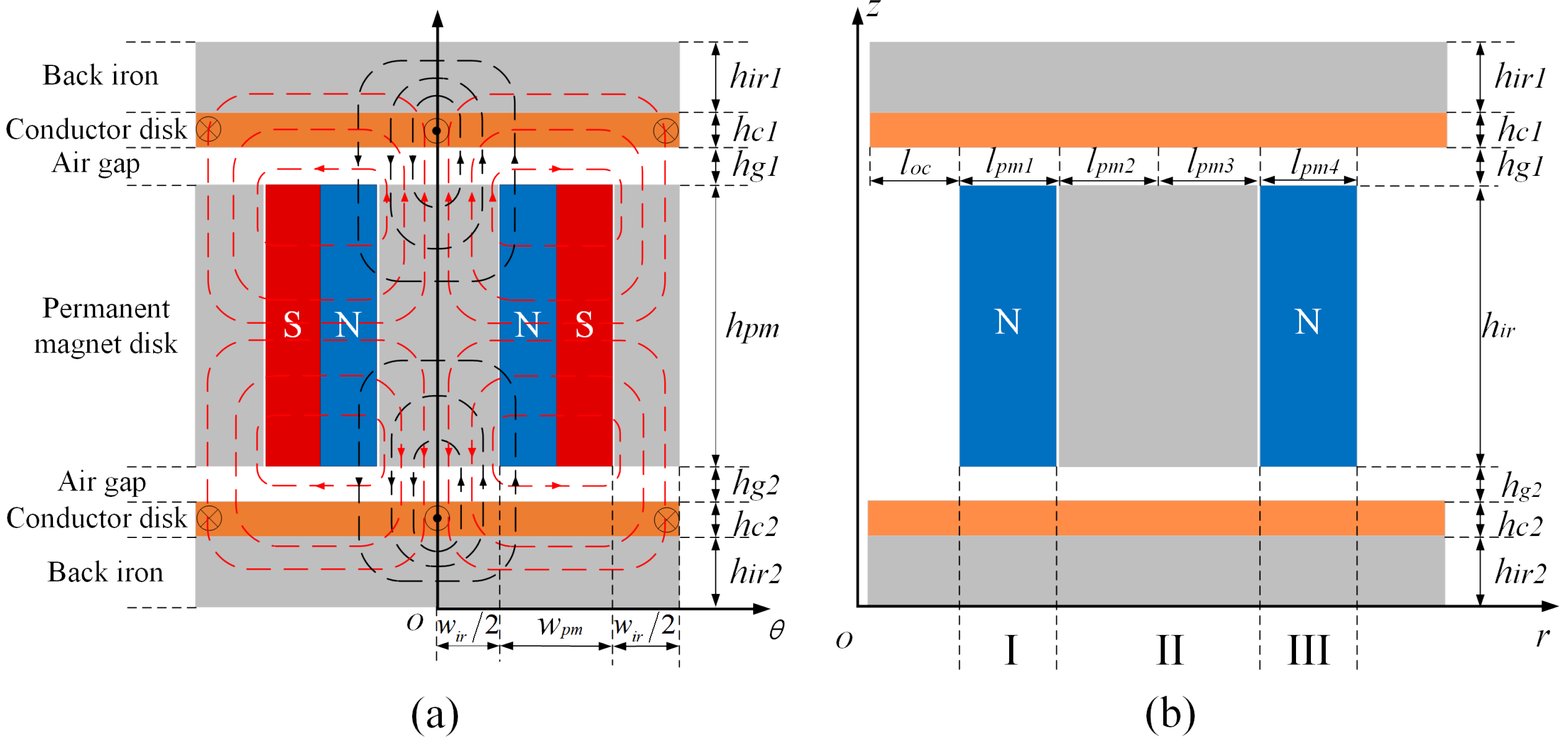
3.1. Field Analysis
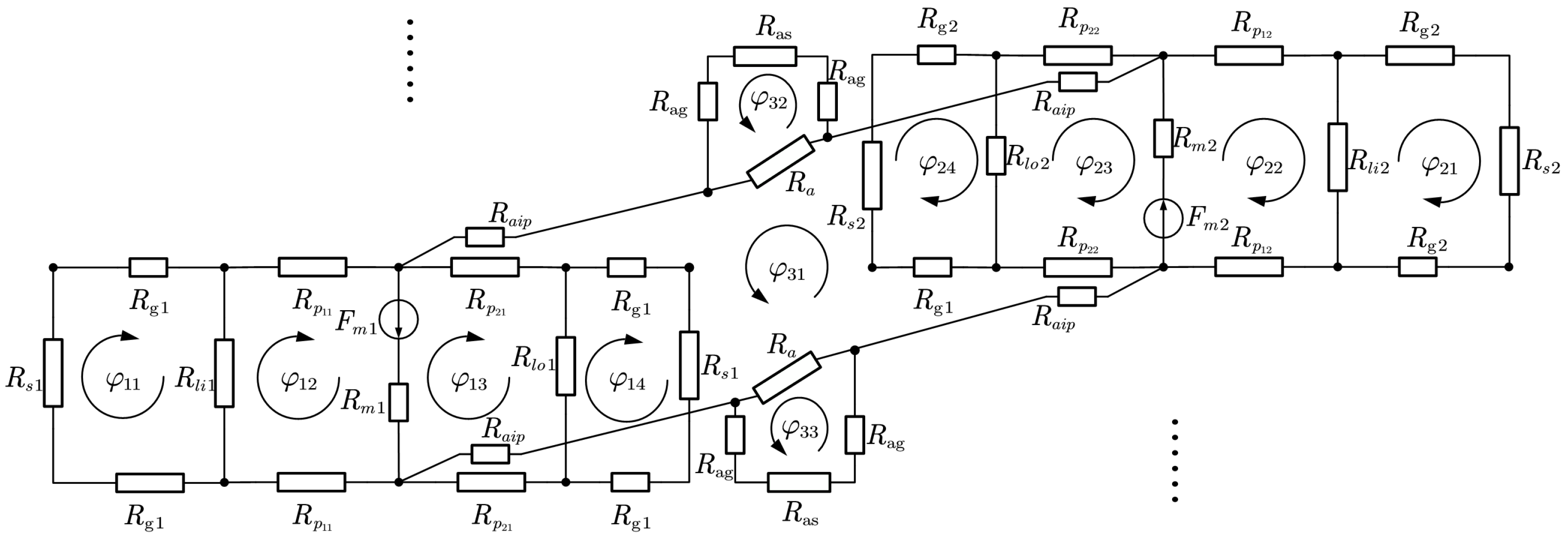
3.2. Induction Field Analysis
3.3. Torque Calculation
4. Figures and Tables
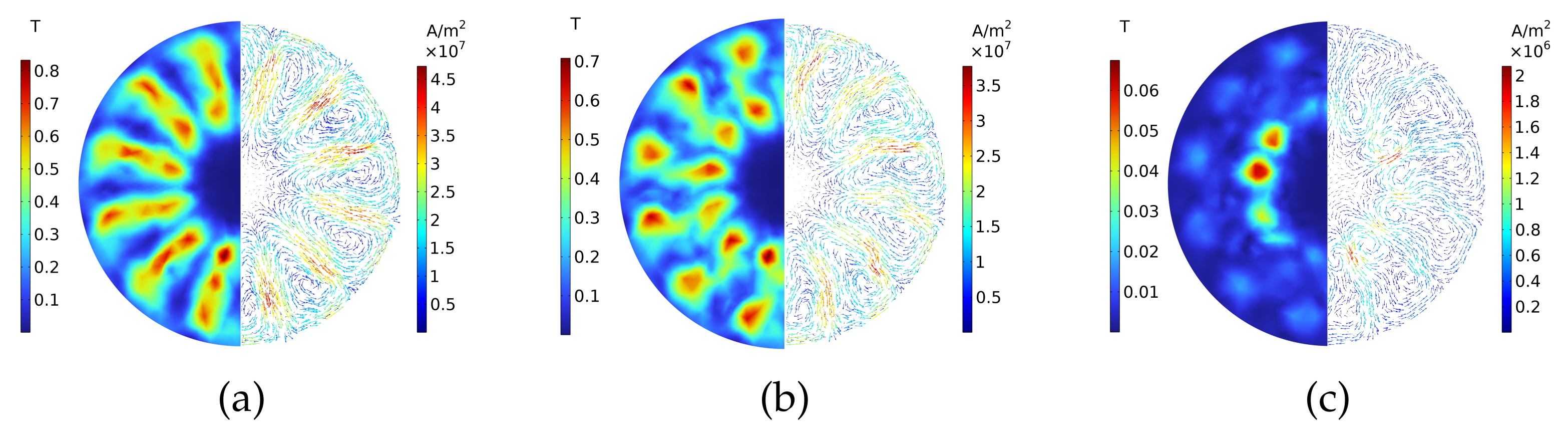
4.1. Magnetic Field Distribution
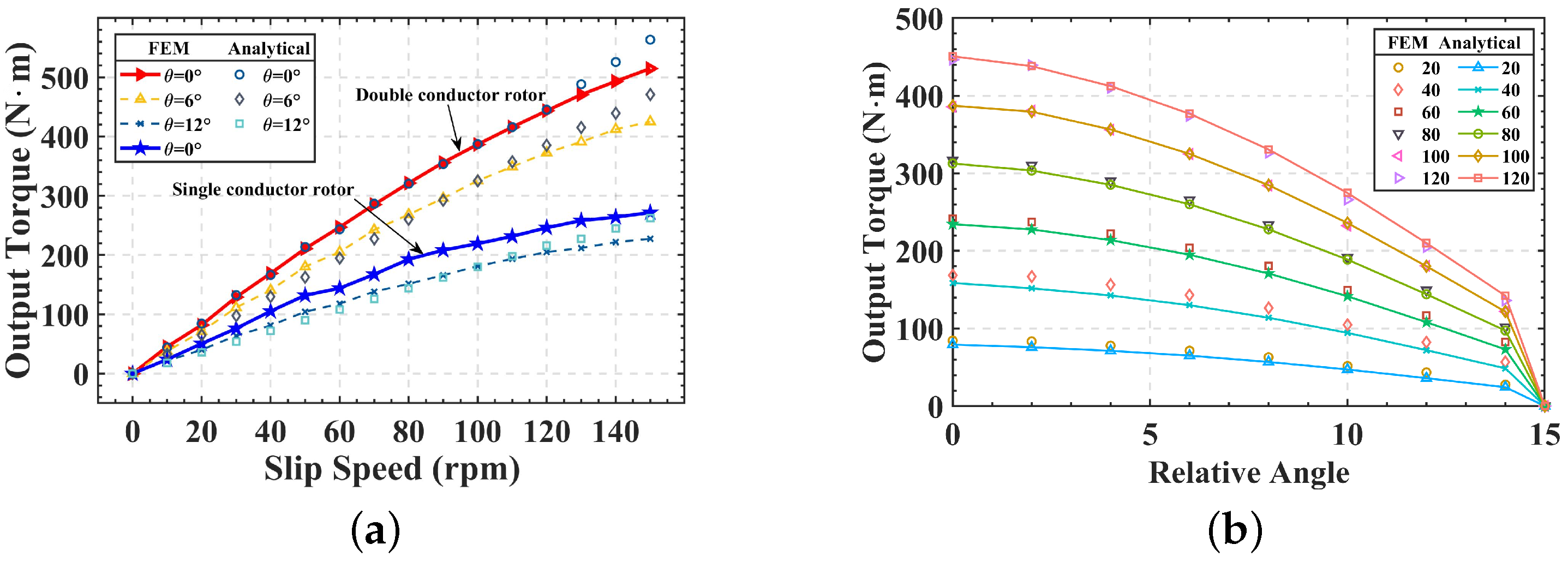
4.2. Output Torque Regulation Characteristic
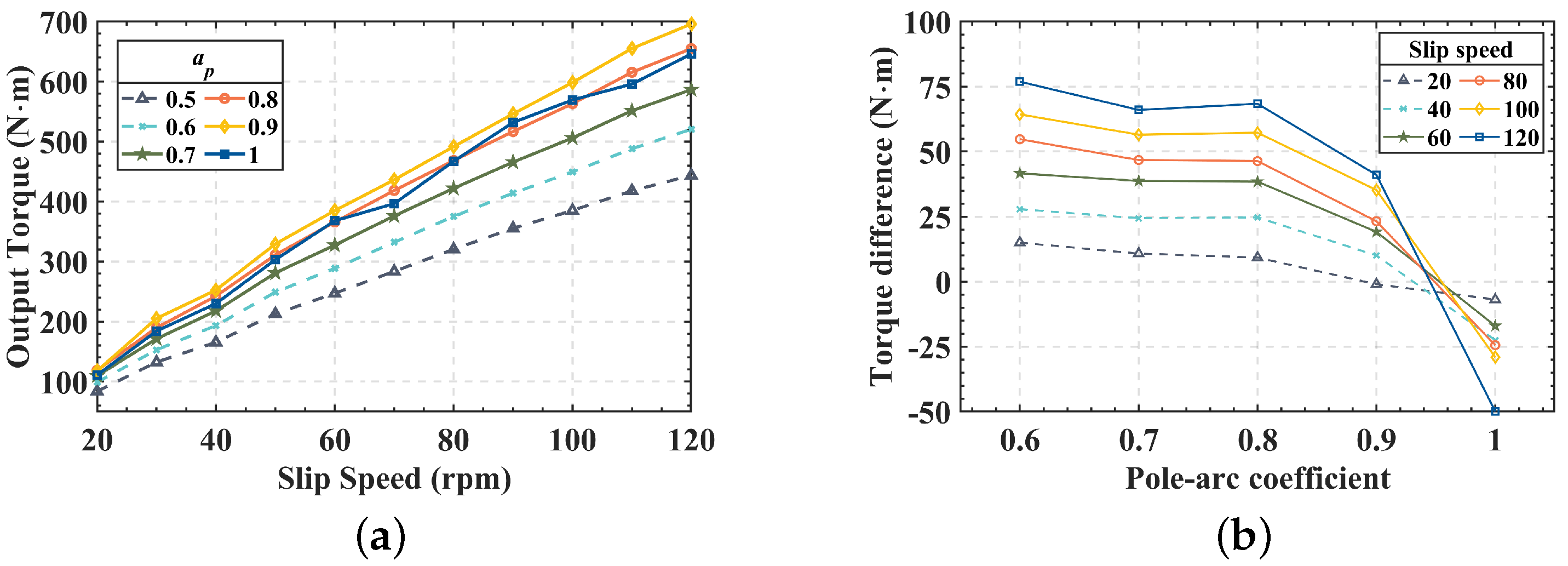
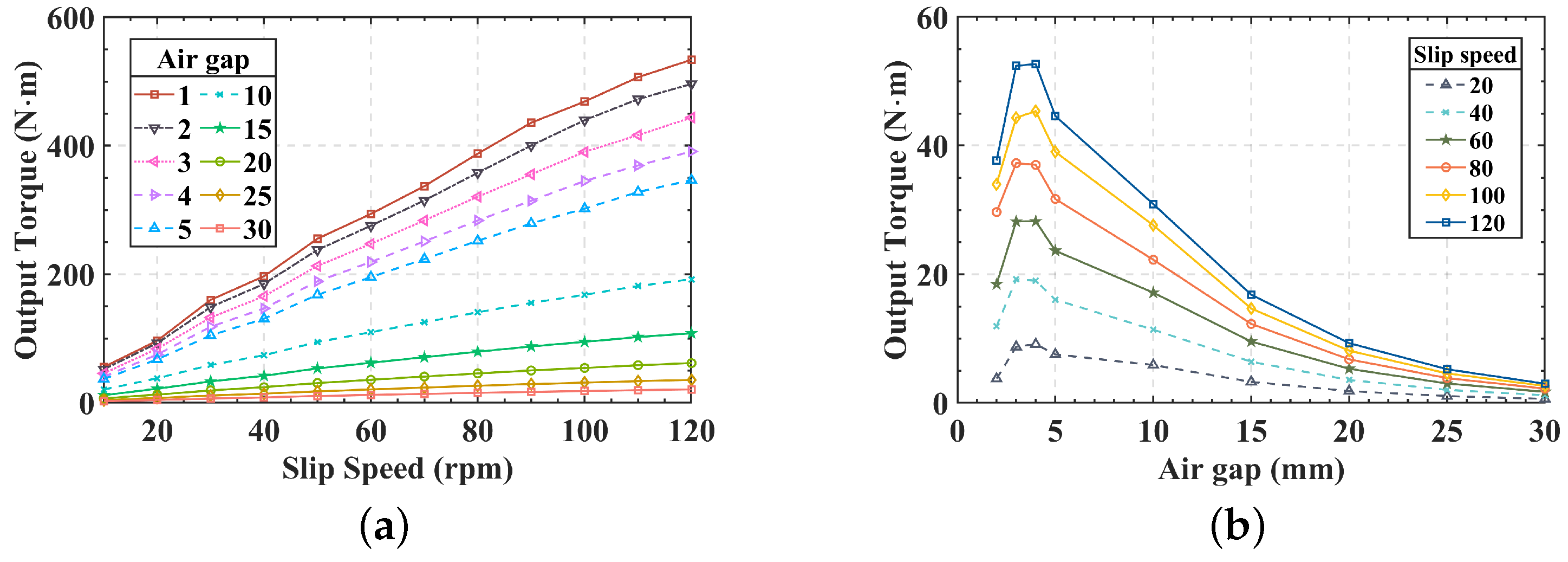
4.3. Sensitivity Analysis of Structural Parameters
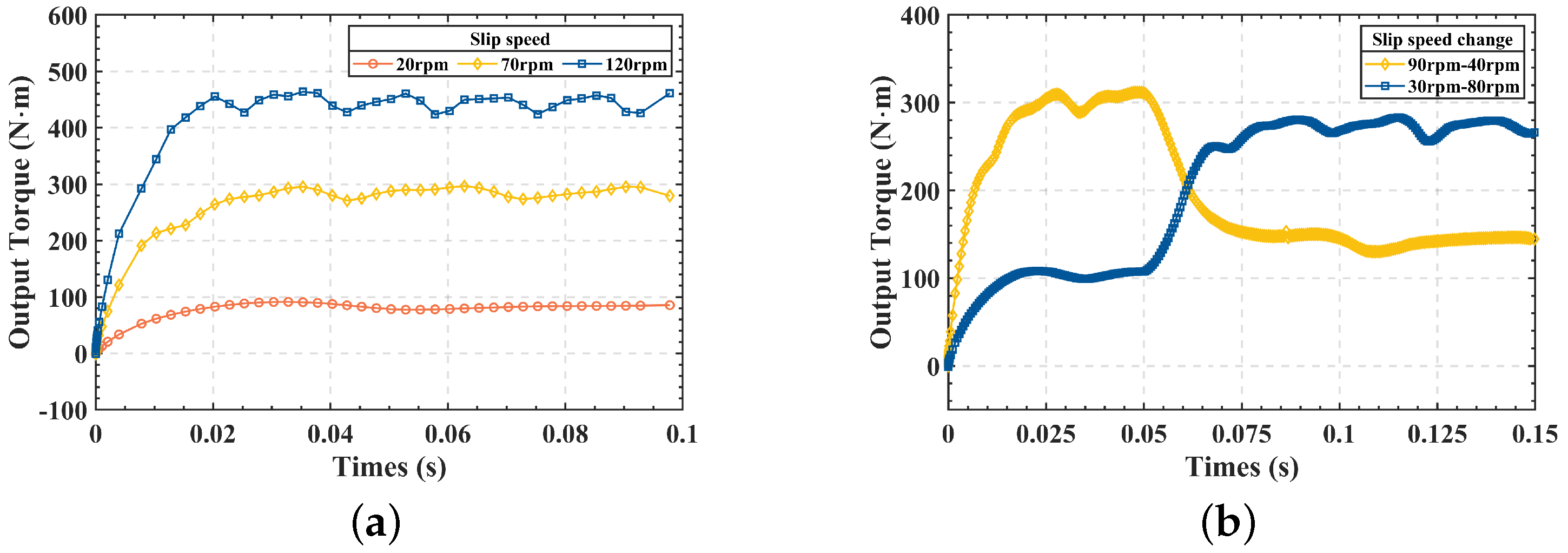
4.4. Dynamic Characteristics
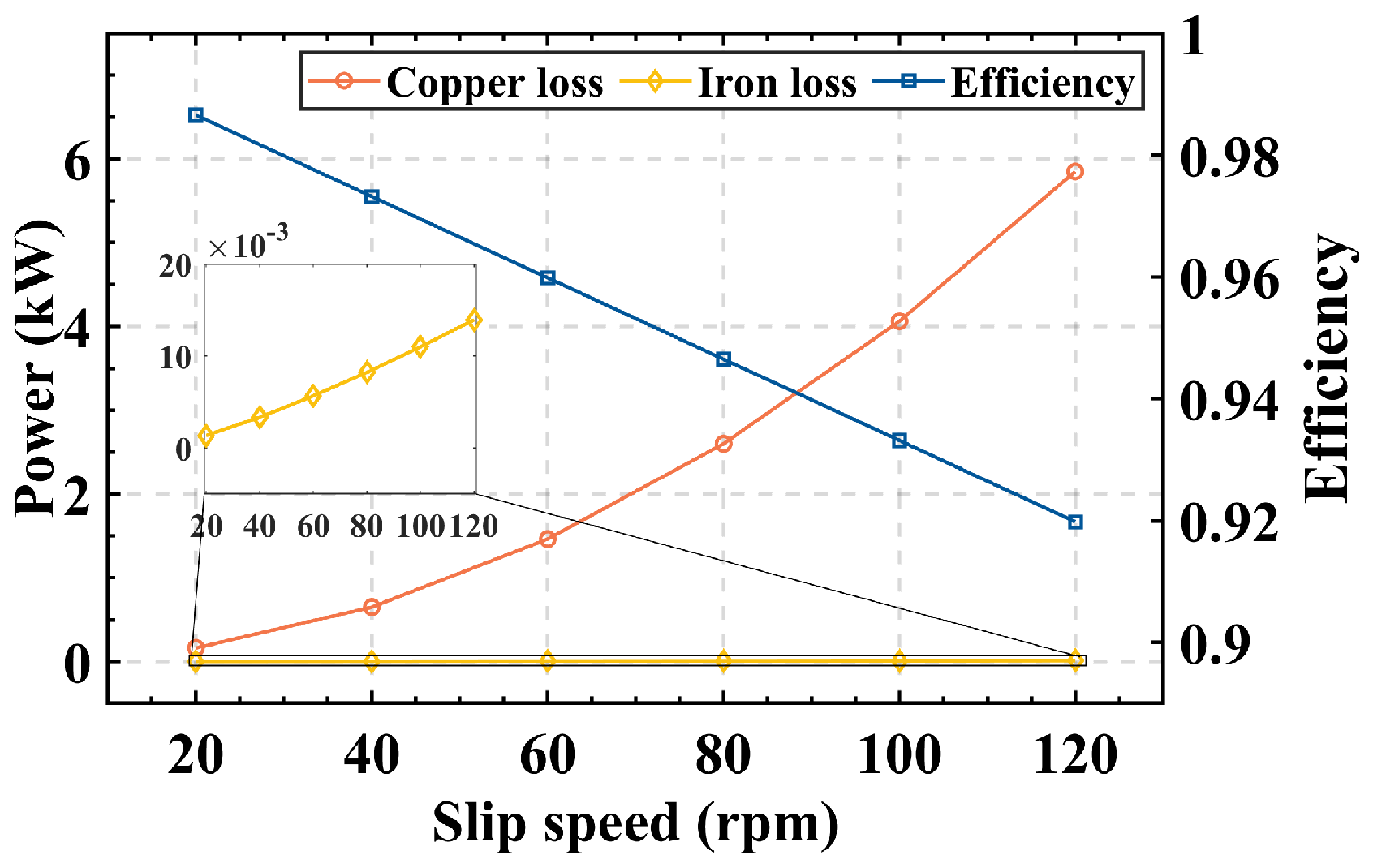
4.5. Loss and Efficiency
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
Nomenclatures
| The magnetomotive force of the permanent magnets in FPR | |
| The magnetomotive force of the permanent magnets in APR | |
| The coercivity of the permanent magnet | |
| The conductivity of the conductor | |
| The slip angular velocity between the conductor rotor and the permanent magnet rotor | |
| B | The iron core magnetic density |
| The remanent magnetic flux density of the PM | |
| The vacuum permeability | |
| The relative permeability of the permanent magnet | |
| The relative permeability of the iron core | |
| p | The number of pole pairs |
| The radial length of the permanent magnet | |
| The radial length of the iron core | |
| The air-gap length bettwen UCR and PMR | |
| The thickness of copper disk in UCR | |
| The iron core thickness | |
| The air-gap length bettwen LCR and PMR | |
| The thickness of copper disk in LCR | |
| The magnet thickness | |
| The magnet height of UFPR | |
| The half magnet height of APR | |
| The half magnet height of APR | |
| The magnet height of LFPR | |
| The air-gap reluctance between FPR and the upper conductor disk | |
| The air-gap reluctance between the APR and the upper conductor disk | |
| The air-gap reluctance between FPR and the lower conductor disk | |
| The air-gap reluctance between the APR and the lower conductor disk | |
| The equivalent reluctance of the back iron in the FPR | |
| The equivalent reluctance of the back iron in the APR | |
| The leakage reluctance of the upper conductor disk and FPR | |
| The leakage reluctance of the lower conductor disk and FPR | |
| The reluctance of the iron core of FPR | |
| The equivalent reluctance of the FPR permanent magnet | |
| The leakage reluctance between the upper conductor disk and the APR | |
| The leakage reluctance between the lower conductor disk and the APR | |
| The equivalent reluctance of the permanent magnets in the APR | |
| The equivalent reluctance of iron core in APR | |
| The regulation reluctance | |
| The regulation air gap reluctance | |
| The iron core reluctance through the regulation loop | |
| The back iron reluctance through the conductor rotor in the regulation loop | |
| The average radius of the permanent magnet rotor | |
| J | The density of eddy currents in the copper disk |
| The Russell–Norsworthy factor of upper part one-half polar pair | |
| The Russell–Norsworthy factor of lower part one-half polar pair | |
| The iron consumption coefficient | |
| f | The slip frequency |
| The relative angle | |
| The length of the upper part regulation reluctance | |
| The length of the lower part regulation reluctance |
References
- Dai, X.; Liang, Q.; Cao, J.; Long, Y.; Mo, J.; Wang, S. Analytical Modeling of Axial-Flux Permanent Magnet Eddy Current Couplings With a Slotted Conductor Topology. IEEE Trans. Magn. 2016, 52, 8000315. [Google Scholar] [CrossRef]
- Mohammadi, S.; Kirtley, J.; Niaz Azari, M. Modelling of Axial-flux Eddy-current Couplers. IET Electr. Power Appl. 2020, 14, 1238–1246. [Google Scholar] [CrossRef]
- Belguerras, L.; Mezani, S.; Lubin, T. Analytical Modeling of an Axial Field Magnetic Coupler with Cylindrical Magnets. IEEE Trans. Magn. 2021, 57, 8000305. [Google Scholar] [CrossRef]
- Kenne Telezing, B.J.; Yang, C.; Ombolo, P.D.; Peng, Z.; Tai, J.; Zhu, L. Torque Characteristics Analysis of a Novel Hybrid Superconducting Magnetic Coupling With Axial-Flux Using a Magnetic Equivalent Circuit Model. IEEE Access 2022, 10, 45594–45604. [Google Scholar] [CrossRef]
- Canova, A.; Vusini, B. Analytical Modeling of Rotating Eddy-Current Couplers. IEEE Trans. Magn. 2005, 41, 24–35. [Google Scholar] [CrossRef]
- Lubin, T.; Rezzoug, A. 3-D Analytical Model for Axial-Flux Eddy-Current Couplings and Brakes Under Steady-State Conditions. IEEE Trans. Magn. 2015, 51, 8203712. [Google Scholar] [CrossRef]
- Lubin, T.; Rezzoug, A. Steady-State and Transient Performance of Axial-Field Eddy-Current Coupling. IEEE Trans. Ind. Electron. 2015, 62, 2287–2296. [Google Scholar] [CrossRef]
- Lubin, T.; Mezani, S.; Rezzoug, A. Simple Analytical Expressions for the Force and Torque of Axial Magnetic Couplings. IEEE Trans. Energy Convers. 2012, 27, 536–546. [Google Scholar] [CrossRef]
- Jin, P.; Tian, Y.; Lu, Y.; Guo, Y.; Lei, G.; Zhu, J. 3-D Analytical Magnetic Field Analysis of the Eddy Current Coupling With Halbach Magnets. IEEE Trans. Magn. 2020, 56, 7501904. [Google Scholar] [CrossRef]
- Wallace, A.; von Jouanne, A. Industrial Speed Control: Are PM Couplings an Alternative to VFDs? IEEE Ind. Appl. Mag. 2001, 7, 57–63. [Google Scholar] [CrossRef]
- Wallace, A.; von Jouanne, A.; Williamson, S.; Smith, A. Performance Prediction and Test of Adjustable, Permanent-Magnet, Load Transmission Systems. In Proceedings of the Conference Record of the 2001 IEEE Industry Applications Conference, Chicago, IL, USA, 30 September–4 October 2001; 36th IAS Annual Meeting (Cat. No.01CH37248). IEEE: Chicago, IL, USA, 2001; Volume 3, pp. 1648–1655. [Google Scholar] [CrossRef]
- Tonoli, A.; Amati, N. Dynamic Modeling and Experimental Validation of Eddy Current Dampers and Couplers. J. Vib. Acoust. 2008, 130, 021011. [Google Scholar] [CrossRef]
- Nehl, T.; Lequesne, B.; Gangla, V.; Gutkowski, S.; Robinson, M.; Sebastian, T. Nonlinear Two-Dimensional Finite Element Modeling of Permanent Magnet Eddy Current Couplings and Brakes. IEEE Trans. Magn. 1994, 30, 3000–3003. [Google Scholar] [CrossRef]
- Wallace, A.; Lamb, K. A High Efficiency, Alignment and Vibration Tolerant, Coupler Using High Energy-Product Permanent Magnets. IEE Conf. Publ. 1995, 5, 232–236. [Google Scholar] [CrossRef]
- Choi, J.Y.; Jang, S.M. Analytical Magnetic Torque Calculations and Experimental Testing of Radial Flux Permanent Magnet-Type Eddy Current Brakes. J. Appl. Phys. 2012, 111, 07E712. [Google Scholar] [CrossRef]
- Mohammadi, S.; Mirsalim, M.; Vaez-Zadeh, S.; Talebi, H.A. Analytical Modeling and Analysis of Axial-Flux Interior Permanent-Magnet Couplers. IEEE Trans. Ind. Electron. 2014, 61, 5940–5947. [Google Scholar] [CrossRef]
- Wang, S.; Guo, Y.; Cheng, G.; Li, D. Performance Study of Hybrid Magnetic Coupler Based on Magneto Thermal Coupled Analysis. Energies 2017, 10, 1148. [Google Scholar] [CrossRef]
- Tian, M.; Zhao, W.; Wang, X.; Wang, D.; Yang, Y.; Diao, J.; Ma, X. Analysis on a Novel Flux Adjustable Permanent Magnet Coupler With a Double-Layer Permanent Magnet Rotor. IEEE Trans. Magn. 2018, 54, 8109205. [Google Scholar] [CrossRef]
- Li, Y.; Lin, H.; Yang, H.; Fang, S.; Wang, H. Analytical Analysis of a Novel Flux Adjustable Permanent Magnet Eddy-Current Coupling with a Movable Stator Ring. IEEE Trans. Magn. 2018, 54, 8000404. [Google Scholar] [CrossRef]
- Li, Y.; Lin, H.; Huang, H.; Yang, H.; Tao, Q.; Fang, S. Analytical Analysis of a Novel Brushless Hybrid Excited Adjustable Speed Eddy Current Coupling. Energies 2019, 12, 308. [Google Scholar] [CrossRef]
- Li, Y.; Lin, H.; Tao, Q.; Lu, X.; Yang, H.; Fang, S.; Wang, H. Analytical Analysis of an Adjustable-Speed Permanent Magnet Eddy-Current Coupling With a Non-Rotary Mechanical Flux Adjuster. IEEE Trans. Magn. 2019, 55, 5. [Google Scholar] [CrossRef]
- Li, Y.; Lin, H.; Yang, H. A Novel Squirrel-Cage Rotor Permanent Magnet Adjustable Speed Drive With a Non-Rotary Mechanical Flux Adjuster. IEEE Trans. Energy Convers. 2021, 36, 1036–1044. [Google Scholar] [CrossRef]
- Li, Z.; Zhang, L.; Qu, B.; Yang, H.; Wang, D. Evaluation and Analysis of Novel Flux-adjustable Permanent Magnet Eddy Current Couplings with Multiple Rotors. IET Electr. Power Appl. 2021, 15, 754–768. [Google Scholar] [CrossRef]
- Yang, F.; Zhu, J.; Yang, C.; Ding, Y.; Hang, T. A Simple Method to Calculate the Torque of Magnet-Rotating-Type Axial Magnetic Coupler Using a New Magnetic Equivalent Circuit Model. IEEE Trans. Magn. 2022, 58, 8003212. [Google Scholar] [CrossRef]
- Deshan, K.; Dazhi, W.; Wenhui, L.; Sihan, W.; Zhong, H. Analysis of a Novel Flux Adjustable Axial Flux Permanent Magnet Eddy Current Coupler. IET Electr. Power Appl. 2023, 17, 181–194. [Google Scholar] [CrossRef]


| Symbol | Quantity | Value |
|---|---|---|
| Magnet inner radius | 6 cm | |
| Magnet outer radius | 14 cm | |
| Magnet thickness | 2 cm | |
| Magnet height of FPR | 2 cm | |
| Half-height of APR magnet | 2 cm | |
| Thickness of copper disk | 0.5 cm | |
| Air-gap length | 0.3 cm | |
| Thickness of back iron | 0.5 cm | |
| Copper disk radius | 0.95 cm | |
| p | Number of pole pairs | 6 |
| Conductivity of conductor | 58 MS/m | |
| Remanence of the PM | 1.21 T | |
| Permanent magnet coercivity | 980 kA/m | |
| Pole-arc coefficient | 0.5 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Kong, D.; Wang, D.; Ni, Y.; Song, K.; Qi, Y.; Li, Y. Analytical Analysis of a Novel Flux Adjustable Permanent Magnet Eddy Current Coupling with Double-Sided Conductor. Actuators 2023, 12, 105. https://doi.org/10.3390/act12030105
Kong D, Wang D, Ni Y, Song K, Qi Y, Li Y. Analytical Analysis of a Novel Flux Adjustable Permanent Magnet Eddy Current Coupling with Double-Sided Conductor. Actuators. 2023; 12(3):105. https://doi.org/10.3390/act12030105
Chicago/Turabian StyleKong, Deshan, Dazhi Wang, Yongliang Ni, Keling Song, Yufei Qi, and Yanming Li. 2023. "Analytical Analysis of a Novel Flux Adjustable Permanent Magnet Eddy Current Coupling with Double-Sided Conductor" Actuators 12, no. 3: 105. https://doi.org/10.3390/act12030105
APA StyleKong, D., Wang, D., Ni, Y., Song, K., Qi, Y., & Li, Y. (2023). Analytical Analysis of a Novel Flux Adjustable Permanent Magnet Eddy Current Coupling with Double-Sided Conductor. Actuators, 12(3), 105. https://doi.org/10.3390/act12030105




