Design and Testing of Disconnection Actuators for Enhancing Safety and Preventing Failure Escalation
Abstract
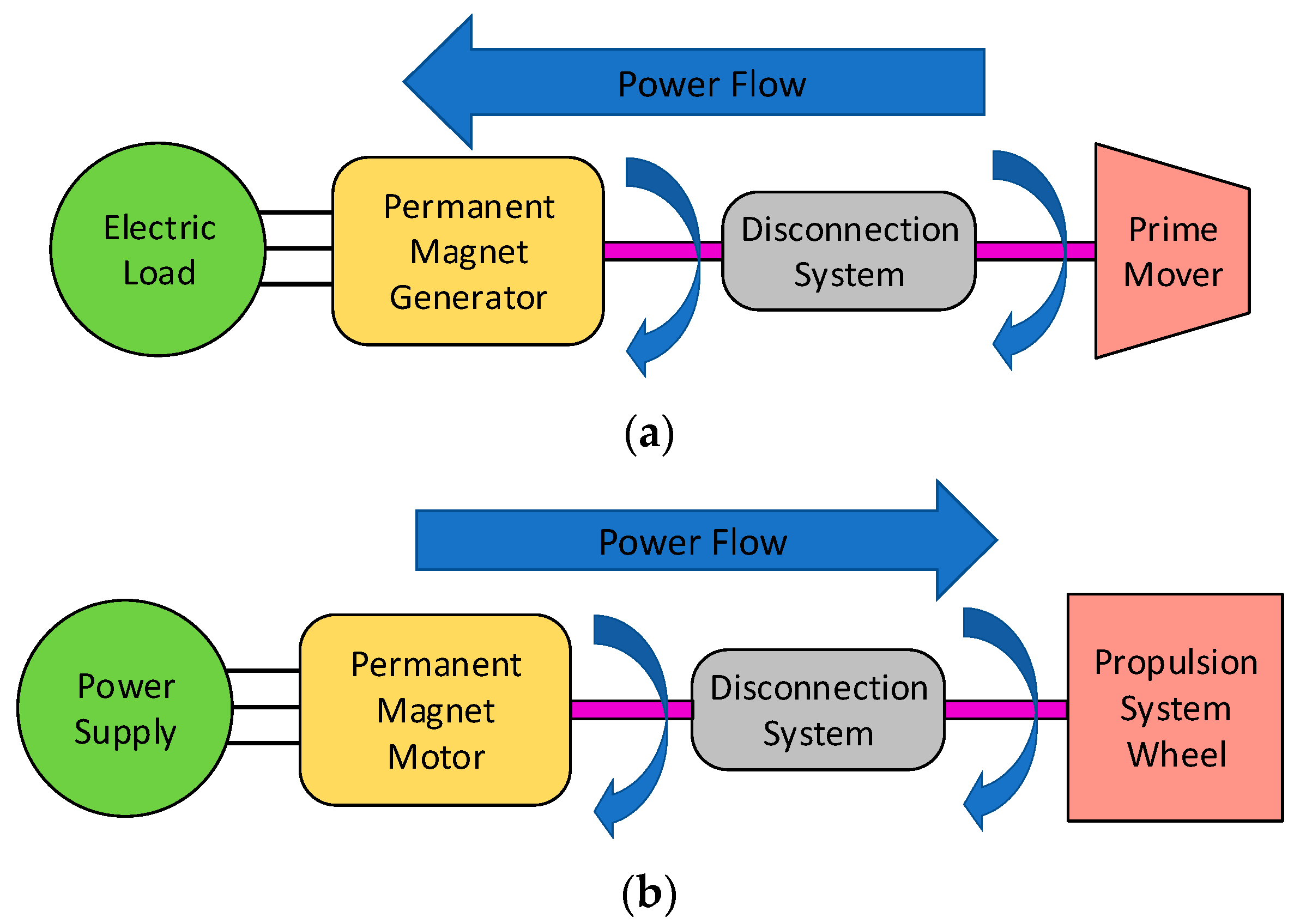
:1. Introduction
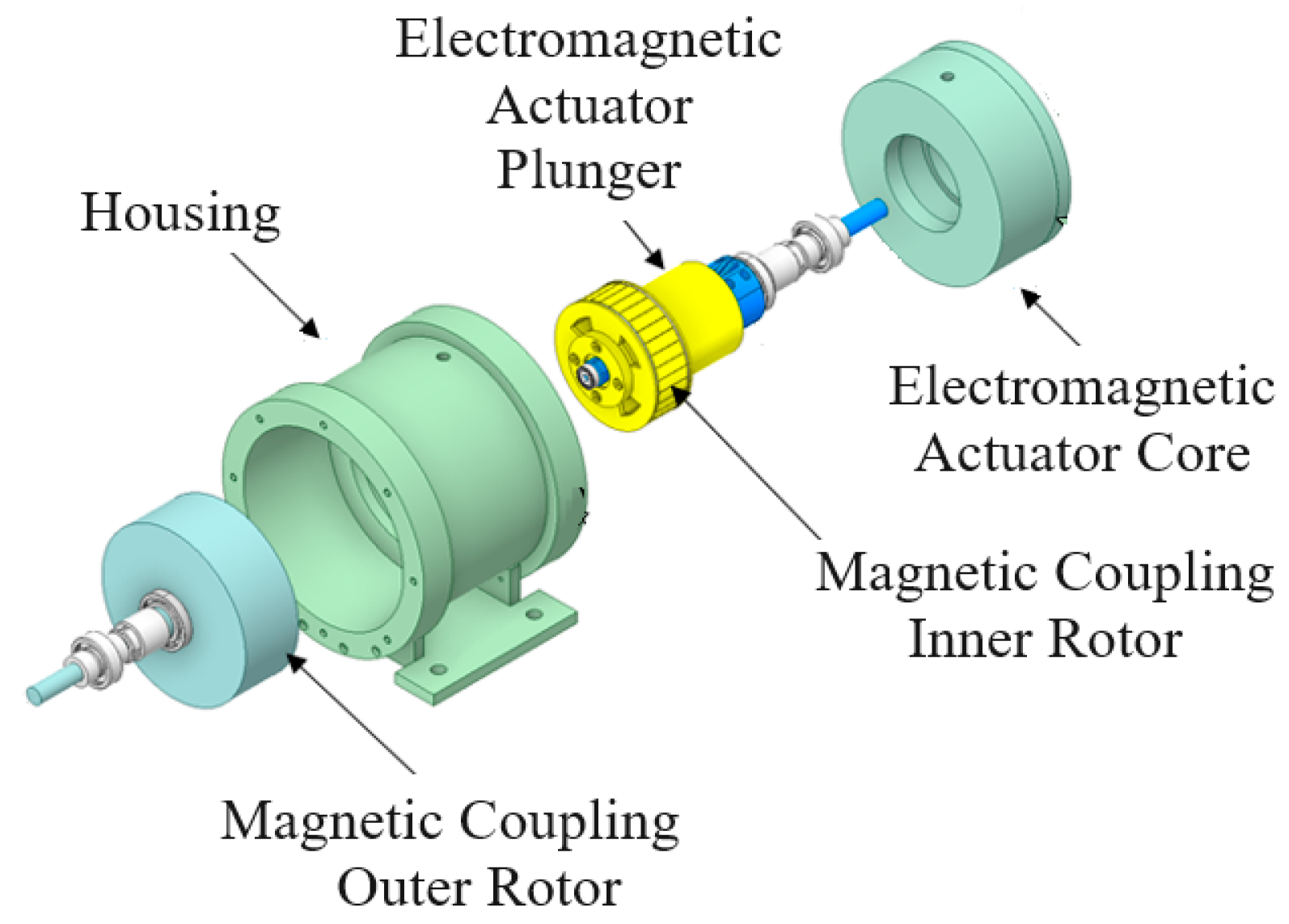
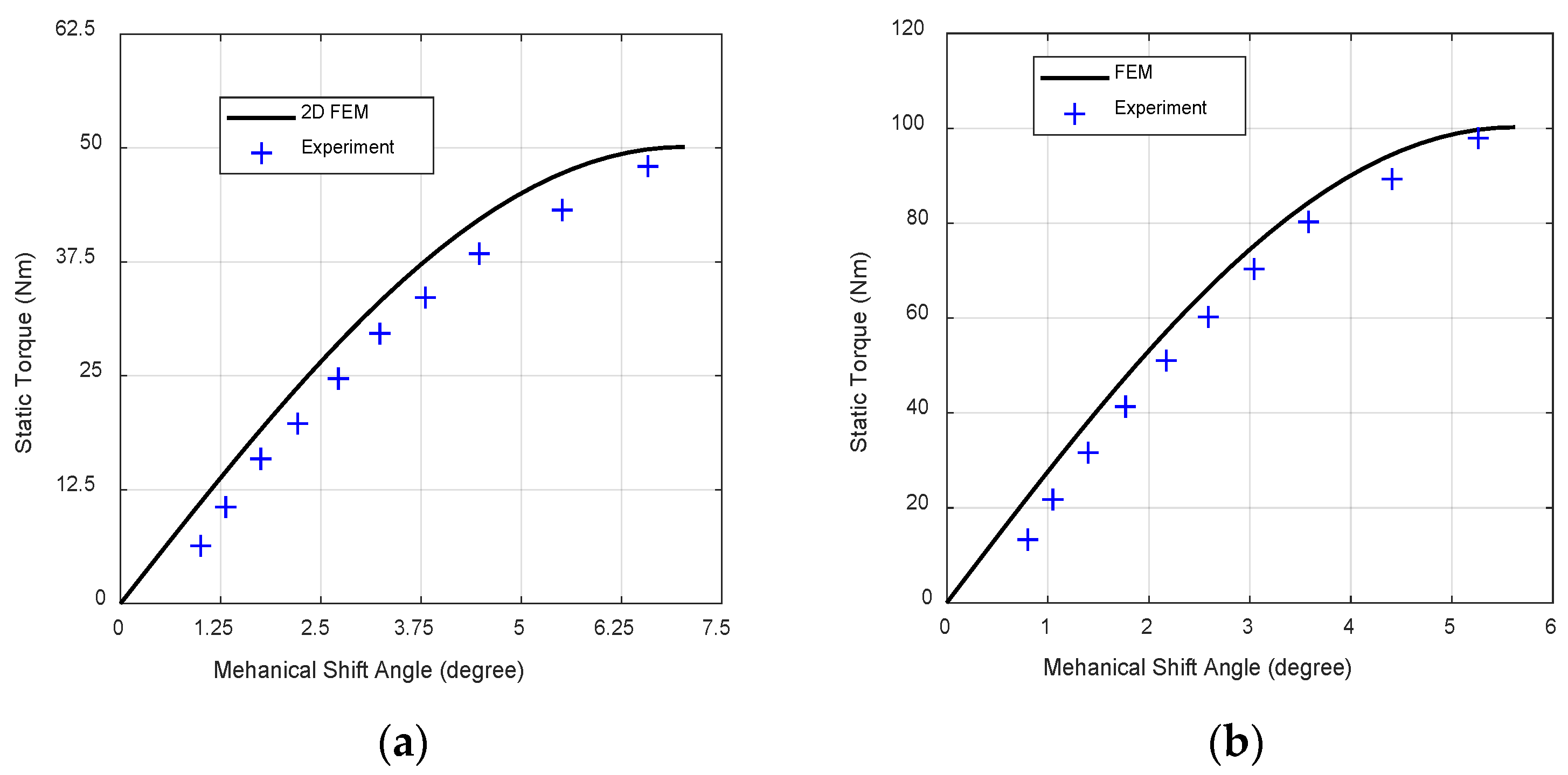
2. Coaxial Magnetic Coupling Design
- Zero transmitted torque, which occurs when the two sets of permanent magnets are radially aligned (i.e., a shift angle equal to zero).
- Maximum transmitted static torque, which takes place when the two sets of permanent magnets are radially shifted by the maximum allowable angle.
3. Axial Pulling Force
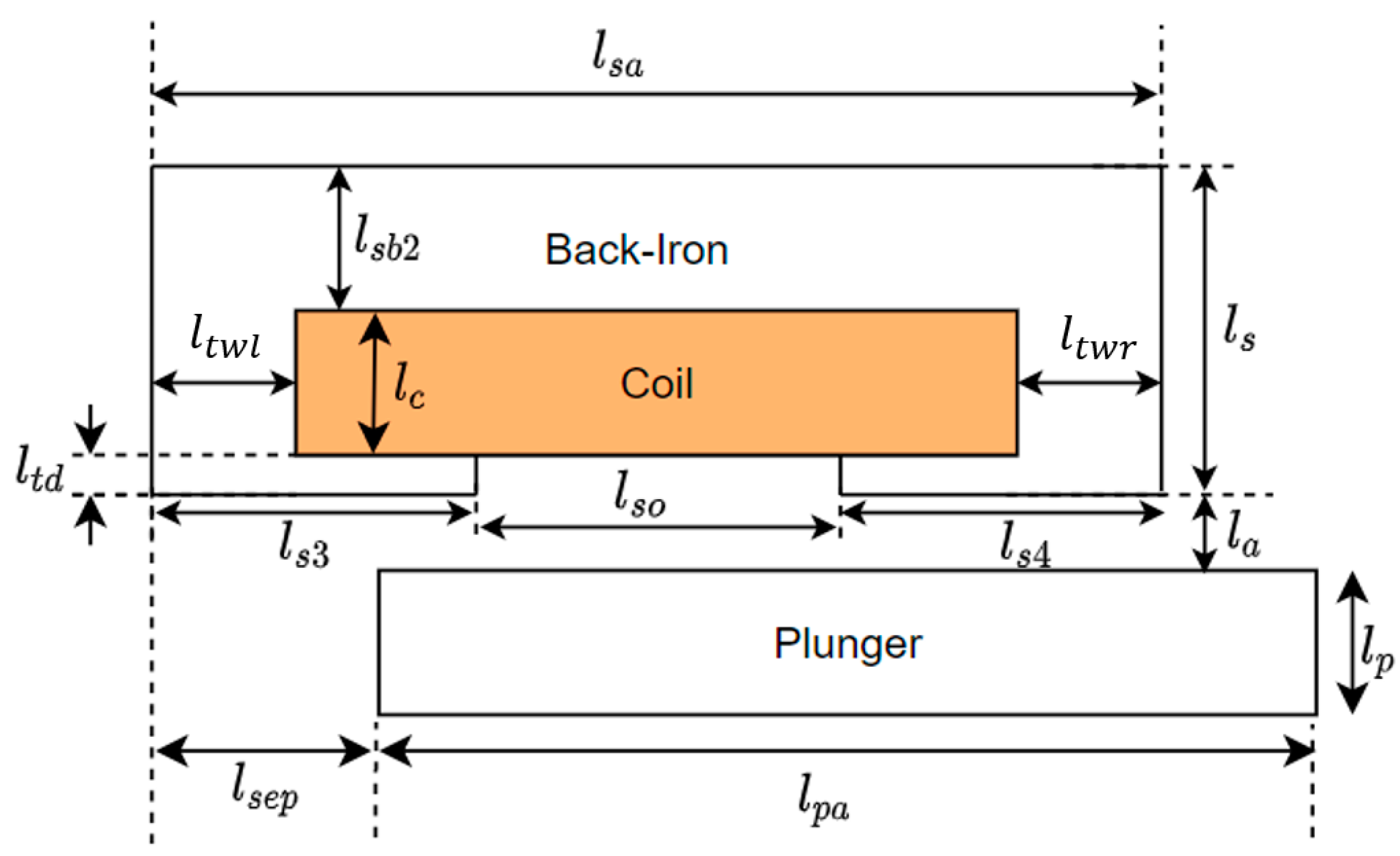
4. Electromagnetic Device Design and Optimization
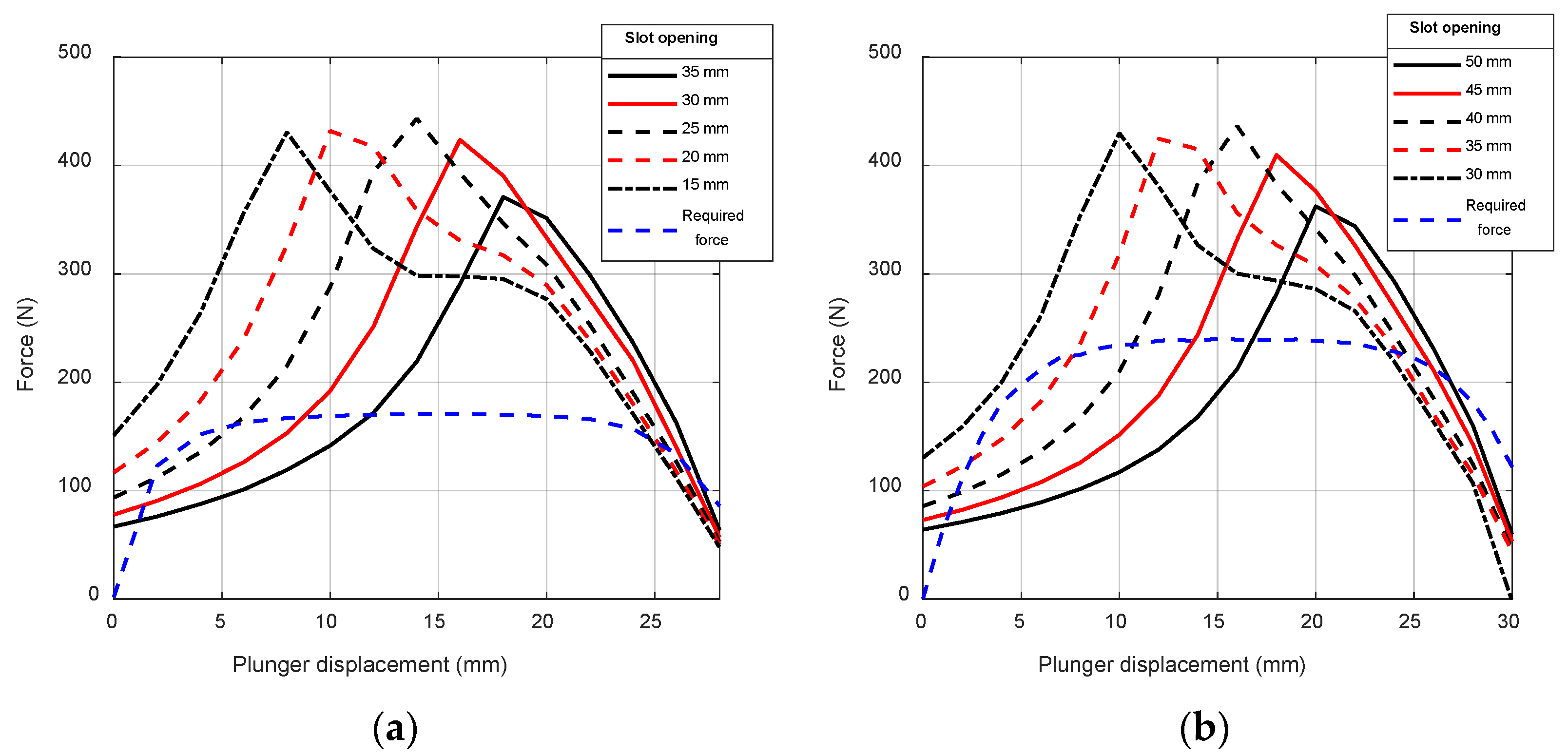
4.1. Slot Opening Length () Trade-Off Study
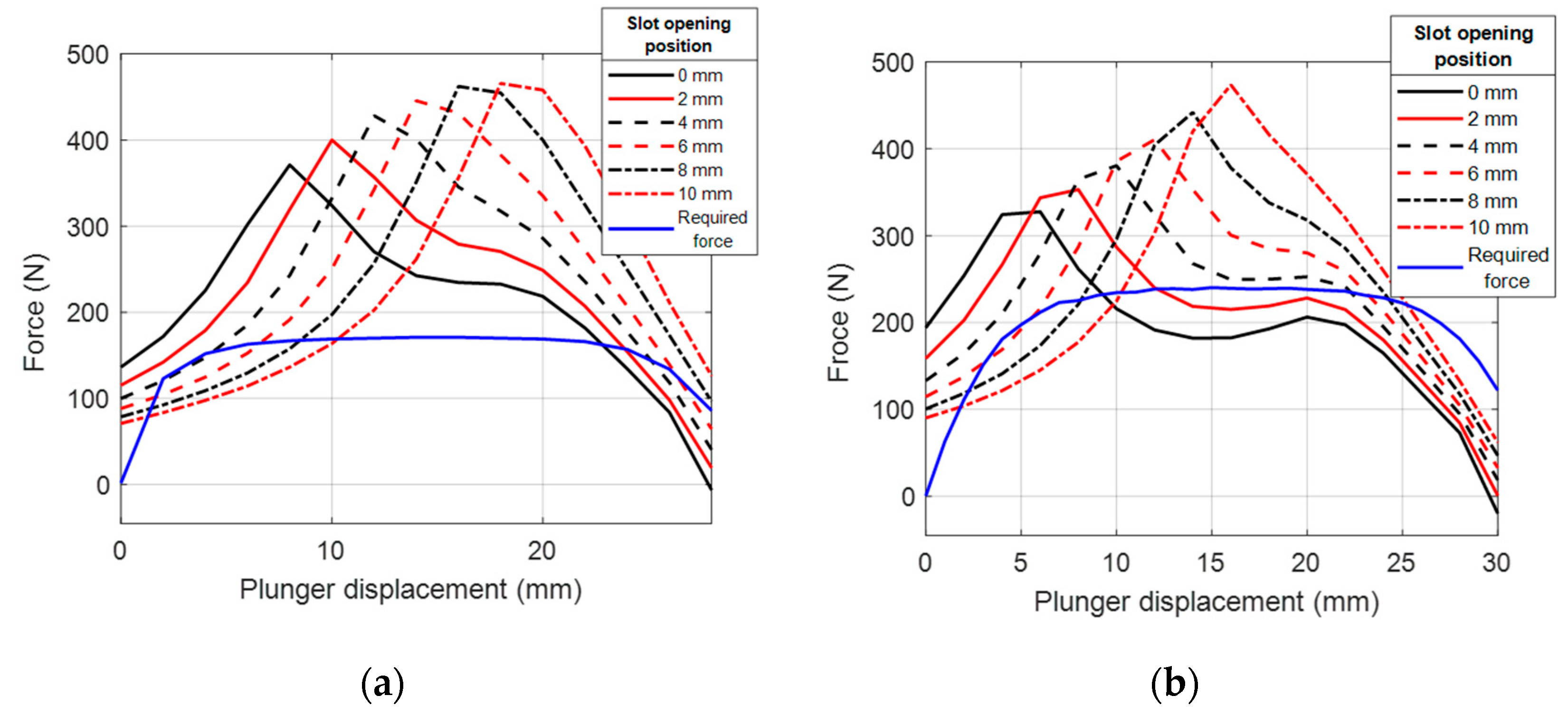
4.2. Slot Opening Location ( and ) Trade-Off Study
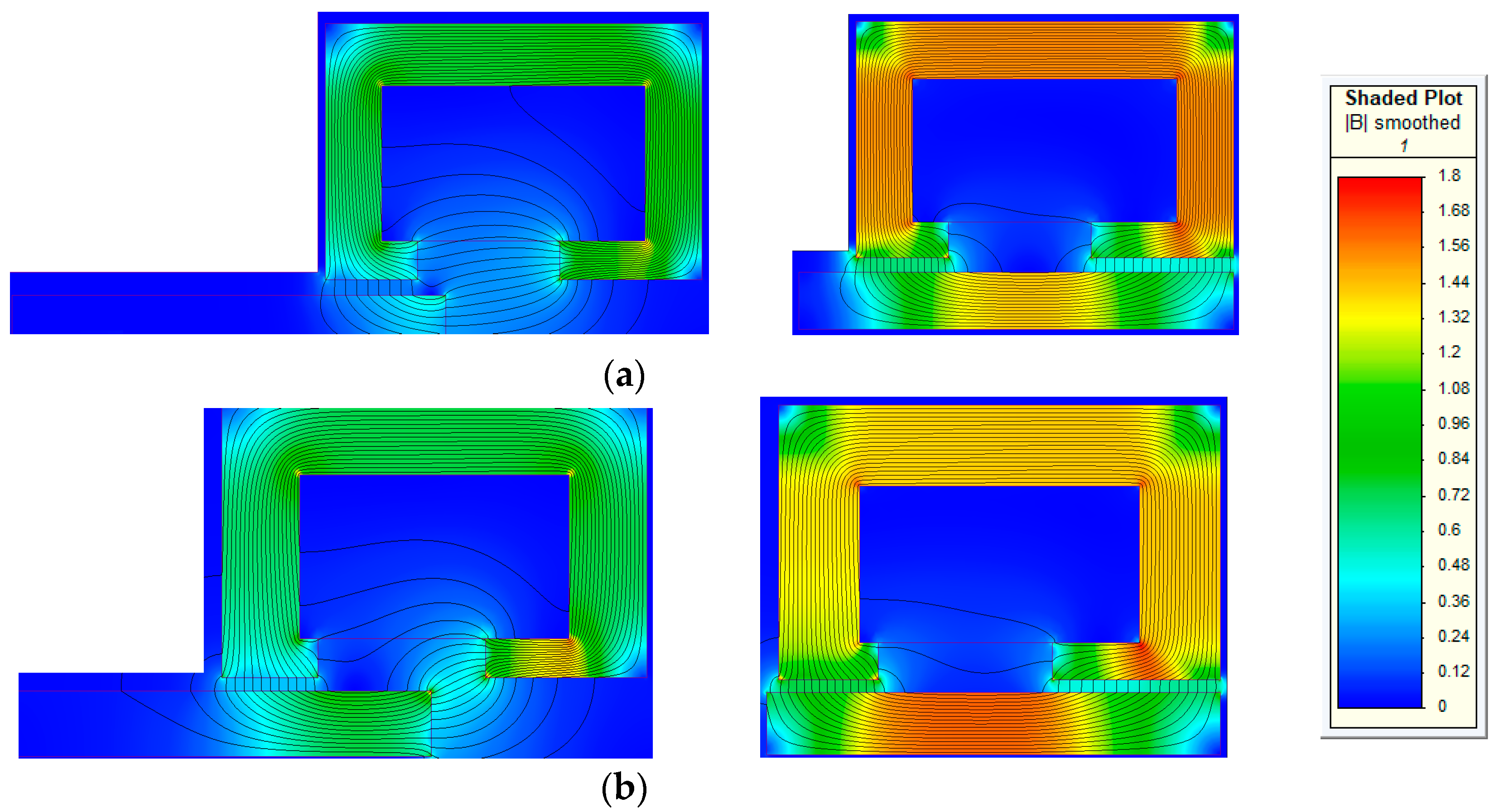
4.3. Electromagnetic Device Final Design and Manufacturing Considerations
- Disconnection actuator in engaged mode (i.e., pre-fault detection situation), where the electromagnetic device is in the initial position and the torque is transferred through the magnetic coupling;
- Disconnection actuator in disengaged mode (i.e., post-fault detection situation), where the electromagnetic device is in the final position and the torque transmission is inhibited.
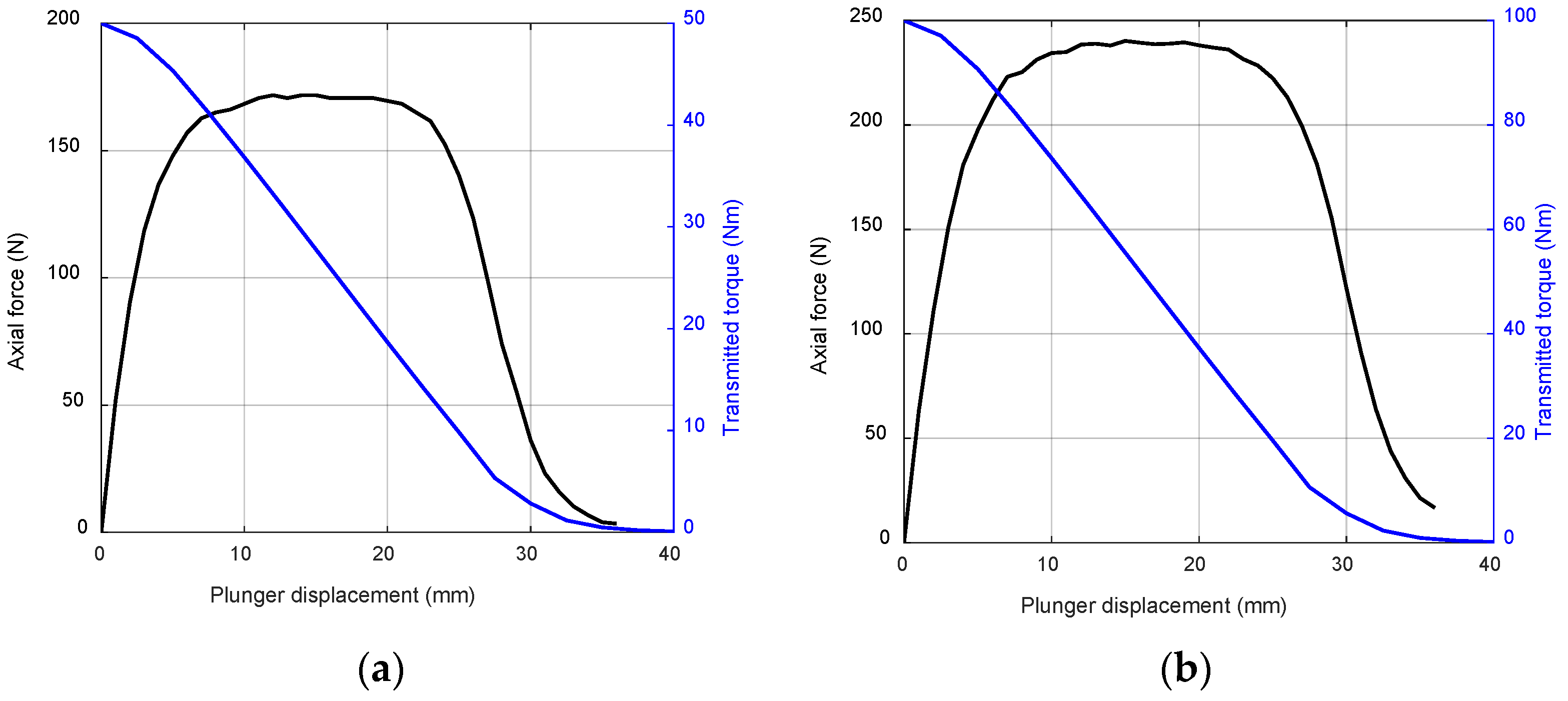
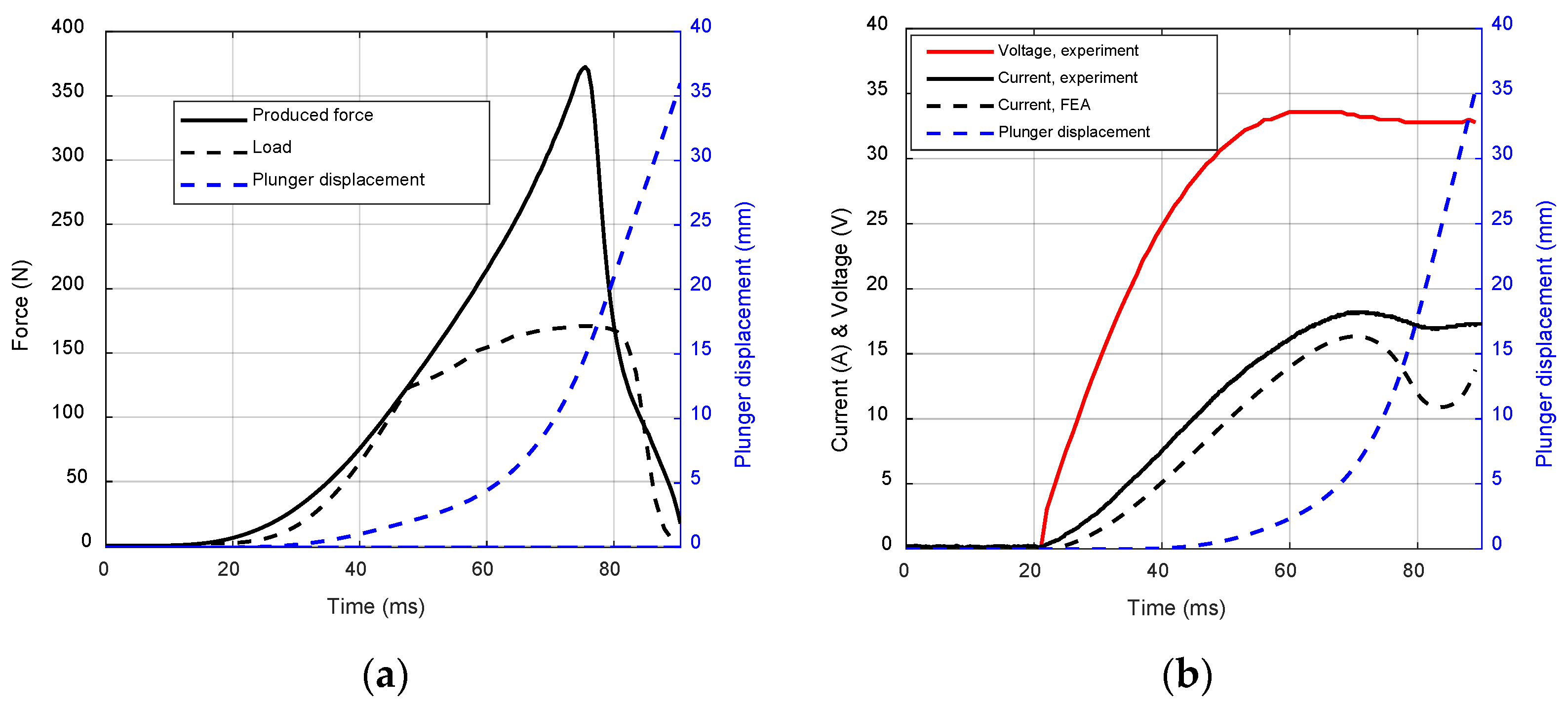
5. Experimental Validation of the Disconnection Capability
6. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Rohith, G.; Devika, K.B.; Menon, P.P.; Subramanian, S.C. Sustainable Heavy Goods Vehicle Electrification Strategies for Long-Haul Road Freight Transportation. IEEE Access 2023, 11, 26459–26470. [Google Scholar] [CrossRef]
- Schmid, F.; Taube, L.; Rieck, J.; Behrendt, F. Electrification of Waste Collection Vehicles: Technoeconomic Analysis Based on an Energy Demand Simulation Using Real-Life Operational Data. IEEE Trans. Transp. Electrif. 2020, 7, 604–615. [Google Scholar] [CrossRef]
- Fang, S.; Wang, Y.; Gou, B.; Xu, Y. Toward Future Green Maritime Transportation: An Overview of Seaport Microgrids and All-Electric Ships. IEEE Trans. Veh. Technol. 2020, 69, 207–219. [Google Scholar] [CrossRef]
- Rosero, J.A.; Ortega, J.A.; Aldabas, E.; Romeral, L. Moving towards a more electric aircraft. IEEE Aerosp. Electron. Syst. Mag 2007, 22, 3–9. [Google Scholar] [CrossRef]
- Marciello, V.; Di Stasio, M.; Ruocco, M.; Trifari, V.; Nicolosi, F.; Meindl, M.; Lemoine, B.; Caliandro, P. Design Exploration for Sustainable Regional Hybrid-Electric Aircraft: A Study Based on Technology Forecasts. Aerospace 2023, 10, 165. [Google Scholar] [CrossRef]
- Mazzoleni, M.; Rito, G.D.; Previdi, F. Electro-Mechanical Actuators for the More Electric Aircraft; Springer International Publishing: New York, NY, USA, 2021; p. 264. [Google Scholar]
- Al-Timimy, A.; Giangrande, P.; Degano, M.; Galea, M.; Gerada, C. Comparative study of permanent magnet-synchronous and permanent magnet-flux switching machines for high torque to inertia applications. In Proceedings of the 2017 IEEE Workshop on Electrical Machines Design, Control and Diagnosis (WEMDCD), Nottingham, UK, 20–21 April 2017; pp. 45–51. [Google Scholar]
- Wheeler, P.; Sirimanna, T.S.; Bozhko, S.; Haran, K.S. Electric/Hybrid-Electric Aircraft Propulsion Systems. Proc. IEEE 2021, 109, 1115–1127. [Google Scholar] [CrossRef]
- Tomšić, Ž.; Raos, S.; Rajšl, I.; Ilak, P. Role of Electric Vehicles in Transition to Low Carbon Power System—Case Study Croatia. Energies 2020, 13, 6516. [Google Scholar] [CrossRef]
- Gutfleisch, O.; Willard, M.A.; Brück, E.; Chen, C.H.; Sankar, S.G.; Liu, J.P. Magnetic Materials and Devices for the 21st Century: Stronger, Lighter, and More Energy Efficient. Adv. Mater. 2010, 23, 821–842. [Google Scholar] [CrossRef]
- Vizentin, G.; Vukelic, G.; Murawski, L.; Recho, N.; Orovic, J. Marine Propulsion System Failures—A Review. J. Mar. Sci. Eng. 2020, 8, 662. [Google Scholar] [CrossRef]
- Tom, L.; Khowja, M.; Vakil, G.; Gerada, C. Commercial Aircraft Electrification—Current State and Future Scope. Energies 2021, 14, 8381. [Google Scholar] [CrossRef]
- Sarlioglu, B.; Morris, C.T. More electric aircraft: Review, challenges, and opportunities for commercial transport aircraft. IEEE Trans. Transp. Electrif. 2015, 1, 54–64. [Google Scholar] [CrossRef]
- Shi, Z.; Sun, X.; Cai, Y.; Yang, Z. Robust Design Optimization of a Five-Phase PM Hub Motor for Fault-Tolerant Operation Based on Taguchi Method. IEEE Trans. Energy Convers. 2020, 35, 2036–2044. [Google Scholar] [CrossRef]
- Al-Timimy, A.; Degano, M.; Giangrande, P.; Calzo, G.L.; Xu, Z.; Galea, M.; Gerada, C.; Zhang, H.; Xia, L. Design and optimization of a high power density machine for flooded industrial pump. In Proceedings of the 2016 XXII International Conference on Electrical Machines (ICEM), Lausanne, Switzerland, 4–7 September 2016; pp. 1480–1486. [Google Scholar]
- Ji, Y.; Giangrande, P.; Zhao, W.; Wang, H.; Madonna, V.; Zhang, H.; Galea, M. Moving Towards Partial Discharge-Free Design of Electrical Machines for More Electric Aircraft Applications. IEEE Trans. Transp. Electrif. 2023, 9, 4668–4679. [Google Scholar] [CrossRef]
- Żyluk, A.; Zieja, M.; Tomaszewska, J.; Michalski, M.; Kordys, K. Service Life Prediction for Rotating Electrical Machines on Aircraft in Terms of Temperature Loads. Energies 2022, 16, 218. [Google Scholar] [CrossRef]
- Imoru, O.; Jimoh, A.A.; Hamam, Y. Origin and Manifestation of Electrical Machine Faults-A Review. In Proceedings of the 2nd IEEE Conference on Power Engineering and Renewable Energy (ICPERE), Bali, Indonesia, 9–11 December 2014. [Google Scholar]
- Li, S.; Zhang, X.; Jiang, J.; Wang, Y. Modeling and Simulation for the Synchro-Self-Shifting Clutch with the Couple Sliding Components. In Proceedings of the 2010 Second International Conference on Computer Modeling and Simulation, Sanya, China, 22–24 January 2010; IEEE: Piscataway, NJ, USA, 2010. [Google Scholar]
- Montazeri, M.; Fashandi, S.A.M. Modeling and Simulation of a Two-Shaft Gas Turbine Propulsion System Containing a Frictional Plate–Type Clutch. Proc. Inst. Mech. Eng. Part M J. Eng. Marit. Environ. 2019, 233, 502–514. [Google Scholar] [CrossRef]
- Łosiewicz, Z.; Mironiuk, W.; Cioch, W.; Sendek-Matysiak, E.; Homik, W. Application of Generator-Electric Motor System for Emergency Propulsion of a Vessel in the Event of Loss of the Full Serviceability of the Diesel Main Engine. Energies 2022, 15, 2833. [Google Scholar] [CrossRef]
- Hendry, M.L.; Bellamy, N. Advantages and Experience of Using SSS (Synchro-Self-Shifting) Clutches in Hybrid Propulsion Such as CODELOG or CODELAG Naval Marine Systems. In Proceedings of the Aircraft Engine; Fans and Blowers; Marine; Honors and Awards, Phoenix, AZ, USA, 17–21 June 2019; American Society of Mechanical Engineers: New York, NY, USA, 2019. [Google Scholar]
- Pourgol-Mohammad, M.; Hejazi, A.; Soleimani, M.; Ghasemi, P.; Ahmadi, A.; Jalali-Vahid, D. Design for reliability of automotive systems; case study of dry friction clutch. Int. J. Syst. Assur. Eng. Manag. 2017, 8, 572–583. [Google Scholar] [CrossRef]
- Tweedy, O.; Akcay, Y.; Giangrande, P.; Galea, M. Concept and Demonstration of a Coaxial Magnetic Coupling With Electromagnetic Disconnection for Aircraft Permanent Magnet Generators. IEEE Trans. Transp. Electrif. 2023, 9, 4094–4103. [Google Scholar] [CrossRef]
- Park, J.T.; Lee, T.W.; Hong, D.K.; Chang, J.H. Magnetic–mechanical performance analysis and experimental validation of noncontact coaxial magnetic gear for a contra-rotating propeller in an electric outboard. IEEE Trans. Magn. 2020, 57, 8202605. [Google Scholar] [CrossRef]
- Liu, C.T.; Chung, H.Y.; Hwang, C.C. Design assessments of a magnetic-geared double-rotor permanent magnet generator. IEEE Trans. Magn. 2013, 50, 1–4. [Google Scholar] [CrossRef]
- Wu, W.; Lovatt, H.C.; Dunlop, J.B. Analysis and design optimization of magnetic couplings using 3D finite element modelling. IEEE Trans. Magn. 1997, 33, 4083–4094. [Google Scholar] [CrossRef]
- Arslan, S.; Iskender, I.; Navruz, T.S. Finite Element Method-Based Optimisation of Magnetic Coupler Design for Safe Operation of Hybrid UAVs. Aerospace 2023, 10, 140. [Google Scholar] [CrossRef]
- Akcay, Y.; Giangrande, P.; Tweedy, O.; Galea, M. Fast and Accurate 2D Analytical Subdomain Method for Coaxial Magnetic Coupling Analysis. Energies 2021, 14, 4656. [Google Scholar] [CrossRef]
- Ahmad, S.S.; Vakil, G.; Gerada, C.; Benarous, M. Damping of Oscillation in Permanent Magnet Torque Limiter. In Proceedings of the 2023 IEEE International Electric Machines & Drives Conference (IEMDC), San Francisco, CA, USA, 15–18 May 2023; IEEE: Piscataway, NJ, USA, 2023. [Google Scholar]
- Lubin, T.; Mezani, S.; Rezzoug, A. Simple Analytical Expressions for the Force and Torque of Axial Magnetic Couplings. IEEE Trans. Energy Convers. 2012, 27, 536–546. [Google Scholar] [CrossRef]
- Furlani, E. A two-dimensional analysis for the coupling of magnetic gears. IEEE Trans. Magn. 1997, 33, 2317–2321. [Google Scholar] [CrossRef]
- Pugi, L.; Allotta, B.; Pagliai, M. Redundant and reconfigurable propulsion systems to improve motion capability of underwater vehicles. Ocean. Eng. 2018, 148, 376–385. [Google Scholar] [CrossRef]
- Bartalucci, L.; Cavuoti, C.; Secciani, N.; Gelli, J.; Della Valle, A.; Allotta, B.; Ridolfi, A. 3D-Printing-Oriented Mechanical Redesign of a Hand Exoskeleton System for Rehabilitative Tasks. In Proceedings of the 6th International Conference on Biomedical Imaging, Signal Processing, Xiamen, China, 29–31 October 2021. [Google Scholar]
- Allotta, B.; Pugi, L.; Gelli, J.; Lupia, M. Design and Fast Prototyping of a Wearable Safety Device for Divers. IFAC-PapersOnLine 2016, 49, 547–552. [Google Scholar] [CrossRef]
- Naseer, M.U.; Kallaste, A.; Asad, B.; Vaimann, T.; Rassõlkin, A. A review on additive manufacturing possibilities for electrical machines. Energies 2021, 14, 1940. [Google Scholar] [CrossRef]

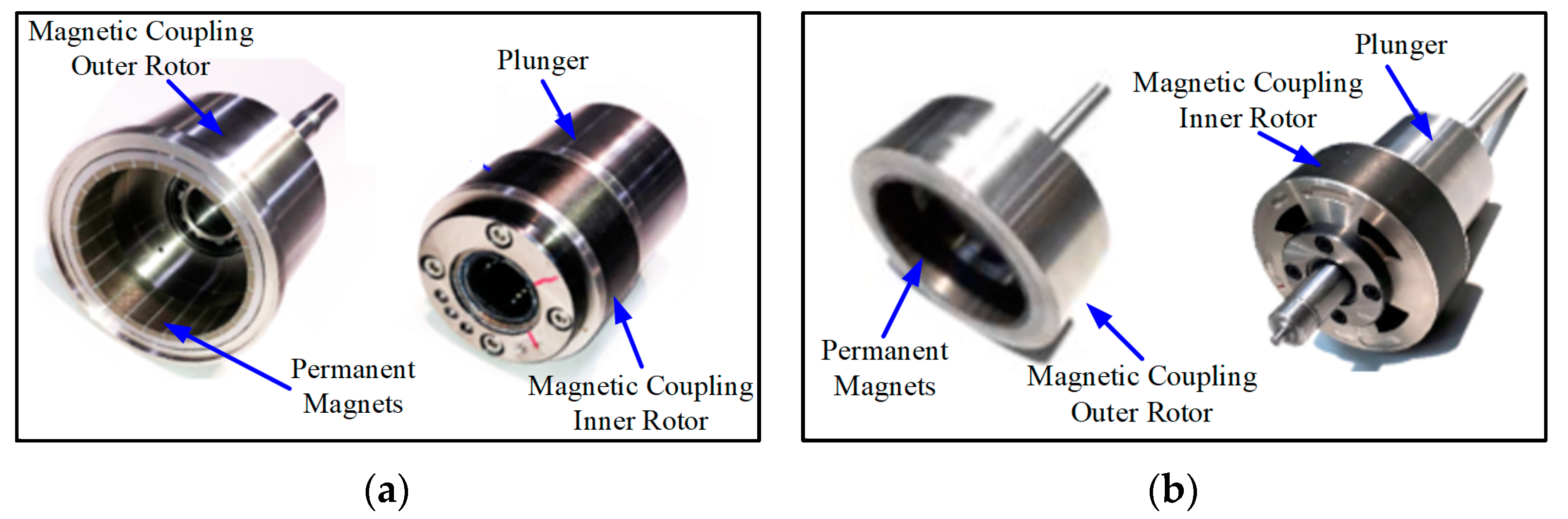


| Parameter | Actuator 1 | Actuator 2 |
|---|---|---|
| Speed (rpm) | 20,000 | 5000 |
| Torque (Nm) | 50 | 100 |
| Parameter | Actuator 1 | Actuator 2 |
|---|---|---|
| Rated torque (Nm) | 50 | 100 |
| Outer radius (mm) | 46.5 | 66.5 |
| Inner radius (mm) | 29 | 40 |
| Active length (mm) | 27.5 | 30 |
| PM thickness (mm) | 3.375 | 4 |
| Airgap thickness (mm) | 2 | 2.5 |
| Pole pair number | 13 | 16 |
| Peak static torque (Nm) | 50 | 100 |
| Rated speed (rpm) | 20,000 | 5000 |
| Parameter | Actuator 1 | Actuator 2 |
|---|---|---|
| Minimum stroke length | 27.5 mm | 30 mm |
| Minimum force | 170 N | 240 N |
| Symbol | Parameter |
|---|---|
| Core active length | |
| Plunger active length | |
| Core thickness | |
| Slot thickness | |
| Plunger thickness | |
| Airgap thickness | |
| Slot opening length | |
| Left tooth width | |
| Right tooth width | |
| Tooth tip depth |
| Parameter | Actuator 1 | Actuator 2 |
|---|---|---|
| Core active length () | 55 mm | 70 mm |
| Plunger active length ) | 57 mm | 72 mm |
| Core thickness ) | 10 mm | 10 mm |
| Slot thickness ( | 20 mm | 20 mm |
| Plunger thickness ) | 10 mm | 10 mm |
| Airgap thickness () | 2 mm | 2 mm |
| Left tooth width ) | 10 mm | 10 mm |
| Right tooth width | 10 mm | 10 mm |
| Tooth tip depth () | 4 mm | 4 mm |
| Stroke length ) | 28 mm | 30 mm |
| DC | 15 A | 15 A |
| Parameter | Actuator 1 | Actuator 2 |
|---|---|---|
| Core active length () | 53 mm | 71 mm |
| Plunger active length ) | 61 mm | 73 mm |
| Core thickness ) | 8 mm | 13 mm |
| Slot thickness ( | 8 mm | 13 mm |
| Plunger thickness ) | 8 mm | 10 mm |
| Airgap thickness () | 2 mm | |
| Slot opening ) | 20 mm | 28 mm |
| Left tooth width ) | 8 mm | 13 mm |
| Right tooth width | 8 mm | 13 mm |
| Tooth tip depth () | 5 mm | 6 mm |
| Stroke length ) | 36 mm | 36 mm |
| Left shoe width ) | 13 mm | 16 mm |
| Right shoe width ) | 20 mm | 27 mm |
| DC | 15 A | |
| Number of turns | 290 | 300 |
| Plunger material | Stainless steel grade 416 | |
| Core material | Stainless steel grade 416 | |
| Permanent magnets | Neodymium-iron-boron 40/23 | |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Akcay, Y.; Tweedy, O.; Giangrande, P.; Galea, M. Design and Testing of Disconnection Actuators for Enhancing Safety and Preventing Failure Escalation. Actuators 2023, 12, 429. https://doi.org/10.3390/act12110429
Akcay Y, Tweedy O, Giangrande P, Galea M. Design and Testing of Disconnection Actuators for Enhancing Safety and Preventing Failure Escalation. Actuators. 2023; 12(11):429. https://doi.org/10.3390/act12110429
Chicago/Turabian StyleAkcay, Yusuf, Oliver Tweedy, Paolo Giangrande, and Michael Galea. 2023. "Design and Testing of Disconnection Actuators for Enhancing Safety and Preventing Failure Escalation" Actuators 12, no. 11: 429. https://doi.org/10.3390/act12110429
APA StyleAkcay, Y., Tweedy, O., Giangrande, P., & Galea, M. (2023). Design and Testing of Disconnection Actuators for Enhancing Safety and Preventing Failure Escalation. Actuators, 12(11), 429. https://doi.org/10.3390/act12110429








